Modeling Liquid Droplet Sizes in Gas–Liquid Annular Flow
Abstract
1. Introduction
2. Review of Previous Experimental Studies
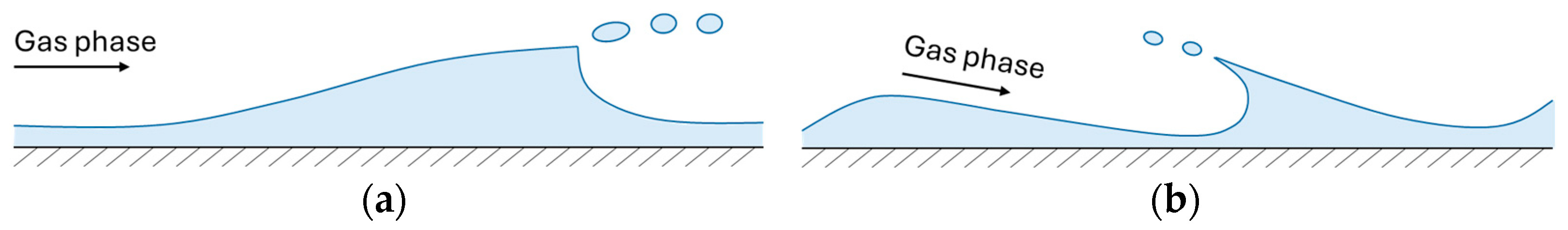
2.1. Droplet Entrainment Mechanism
2.2. Effect of Fluid Properties on Droplet Size
2.2.1. Effect of Fluid Viscosity on Droplet Size
2.2.2. Effect of Fluid Density on Droplet Size
2.2.3. Effect of Surface Tension on Droplet Size
2.3. Effect of Flowing Conditions on Droplet Size
2.3.1. Effect of Superficial Gas Velocity on Droplet Size
2.3.2. Effect of Superficial Liquid Velocity on Droplet Size
2.4. Effects of Pipe Internal Diameter and Inclination on Droplet Size
3. Droplet-Size Modeling
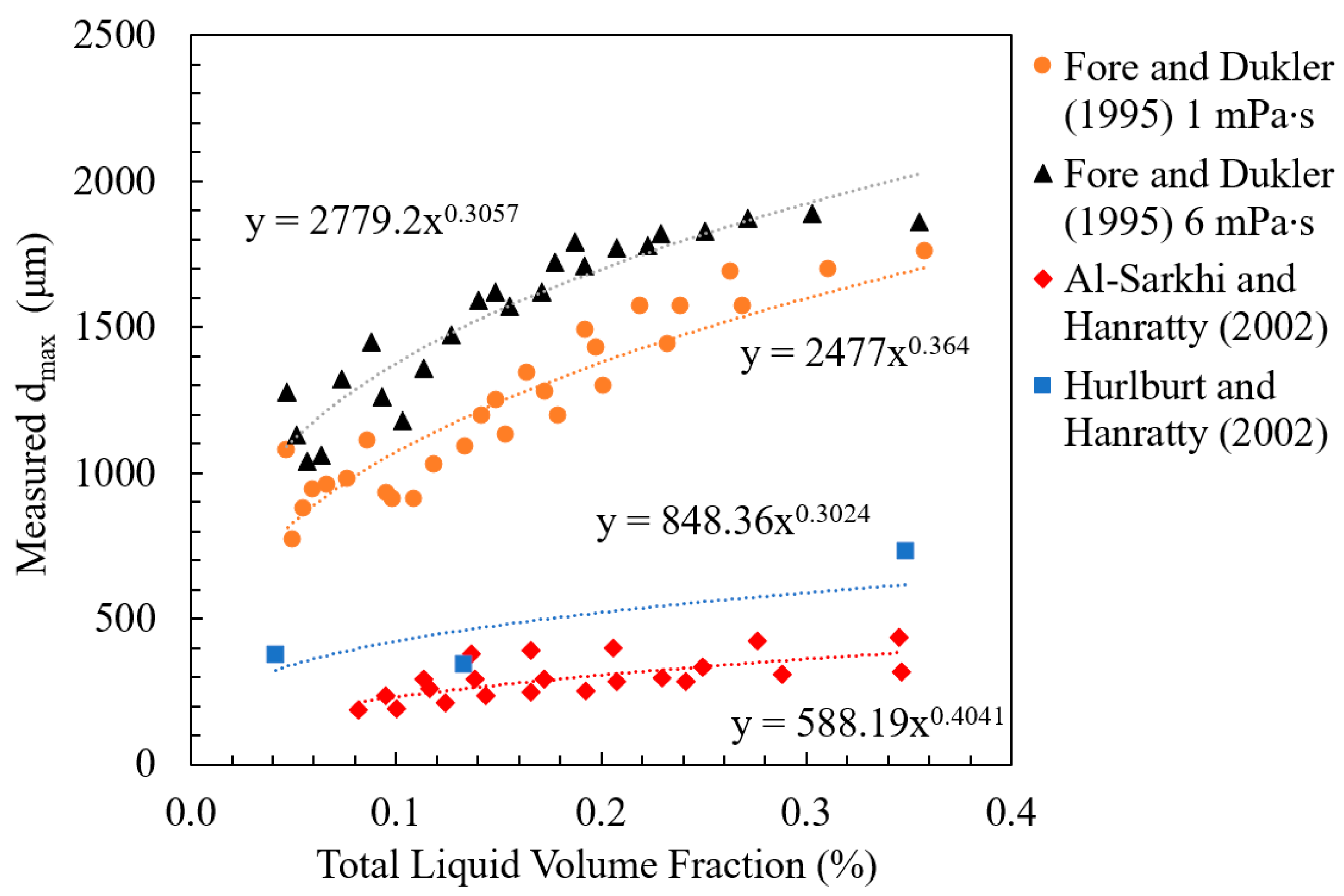
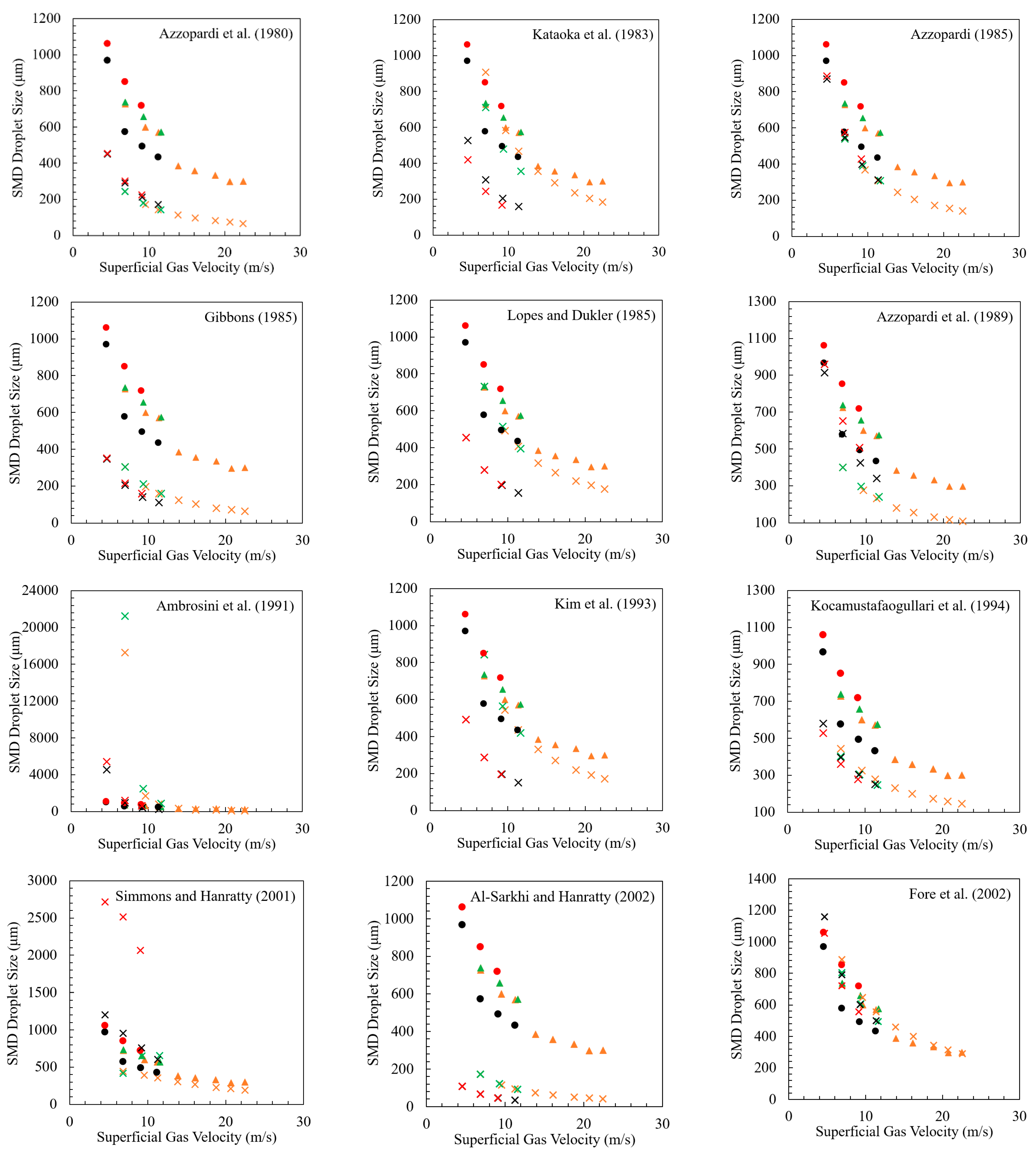
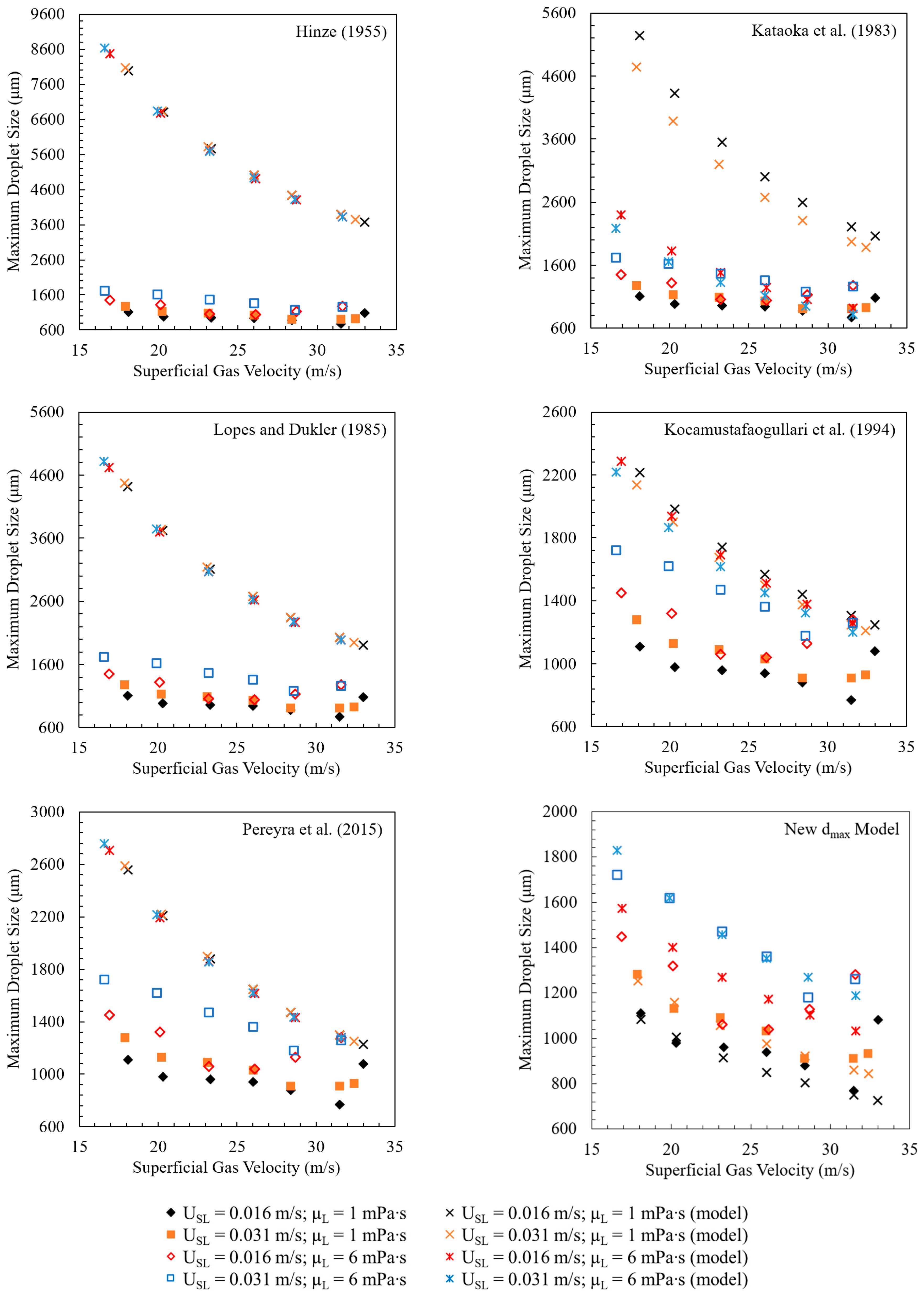
3.1. Modeling the Maximum Droplet Size
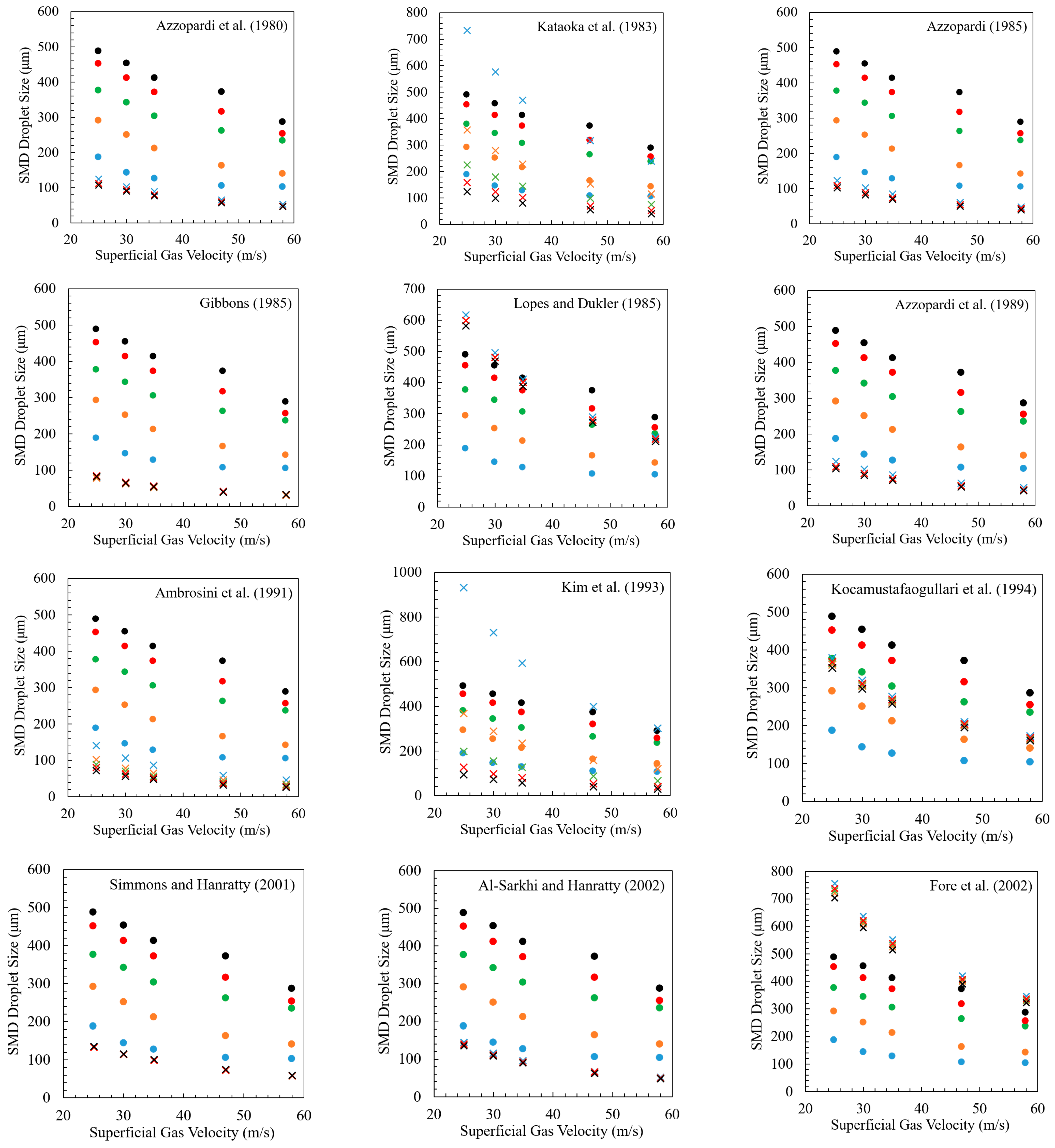
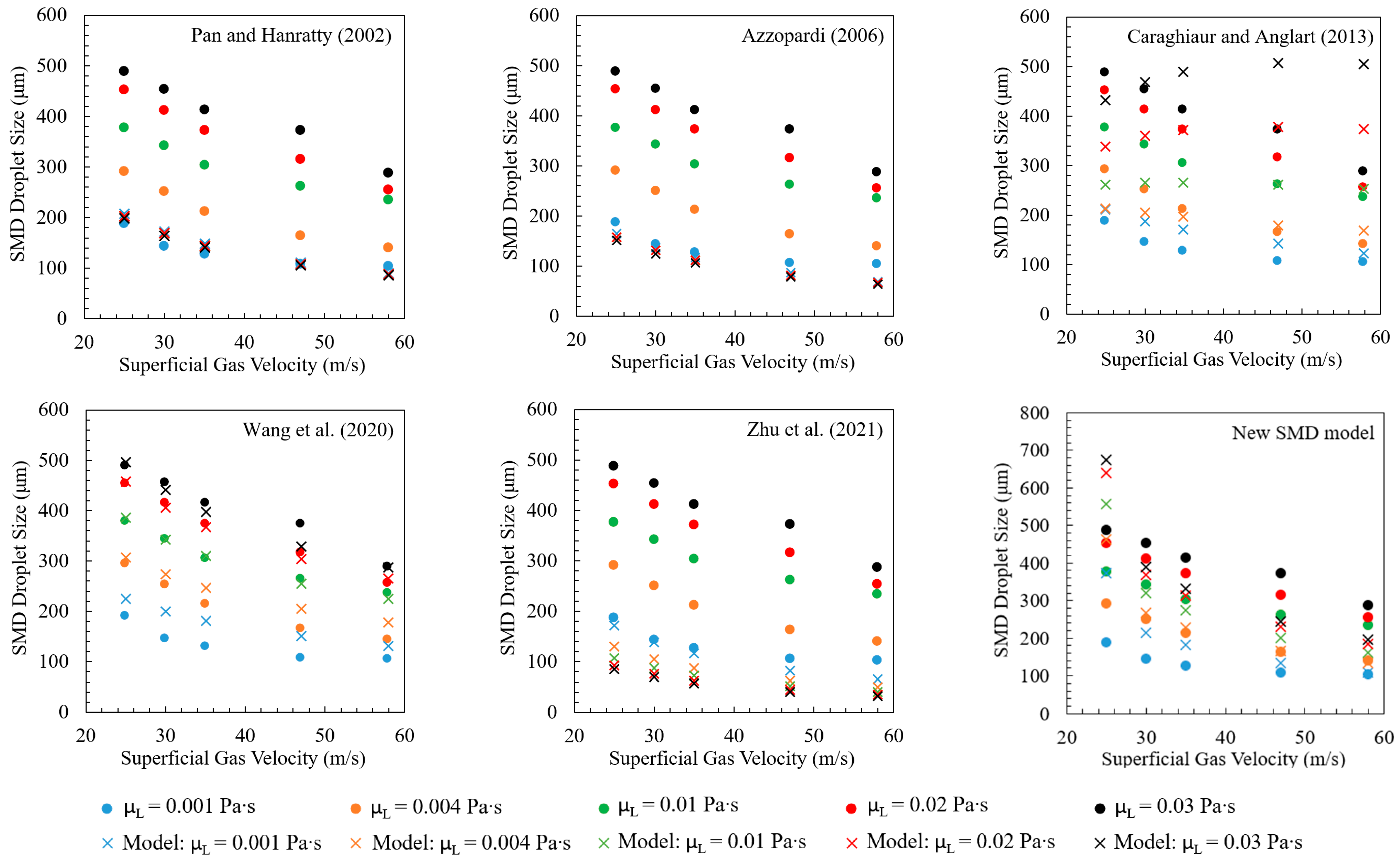
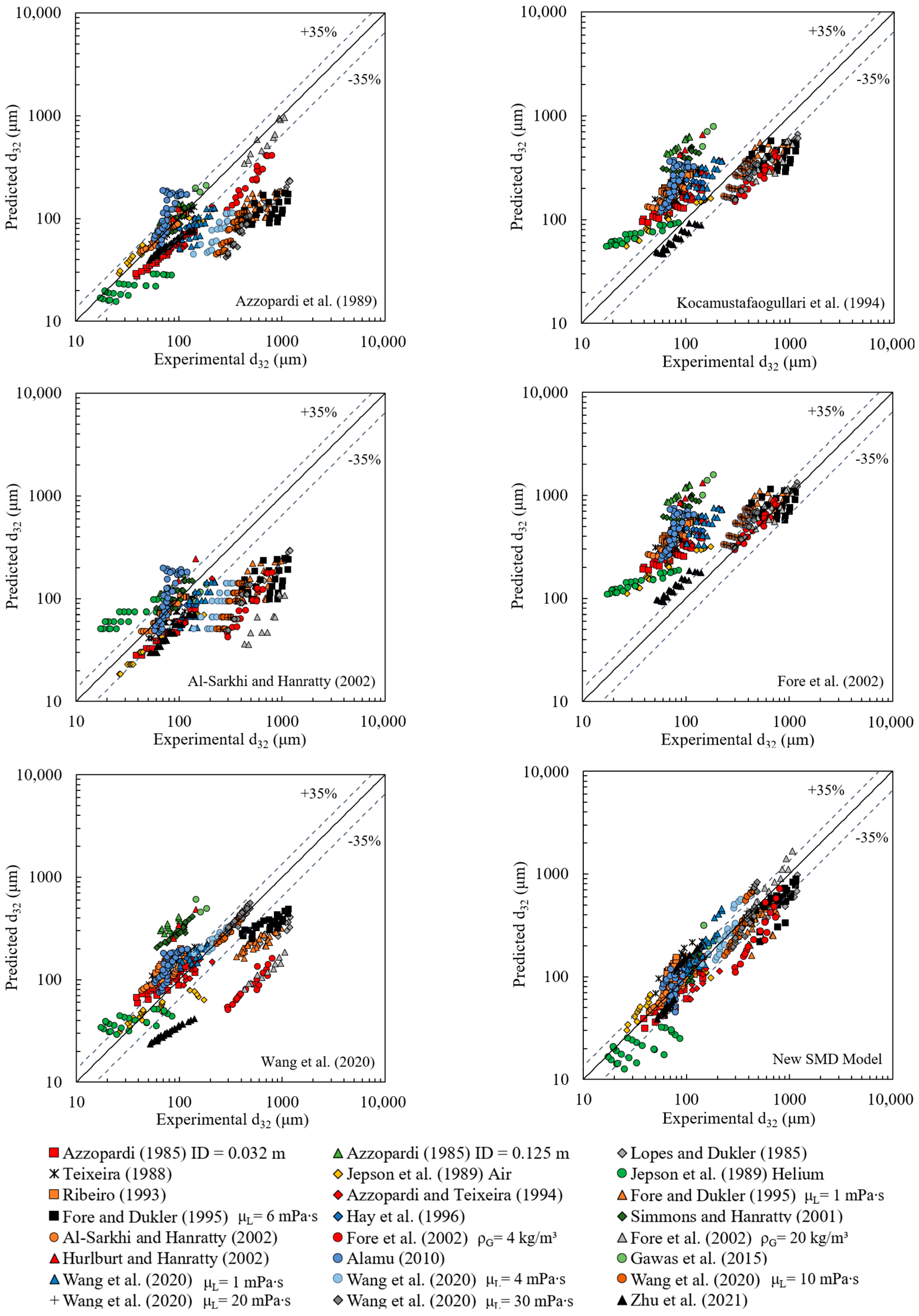
3.2. Modeling the Sauter Mean Diameter
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A

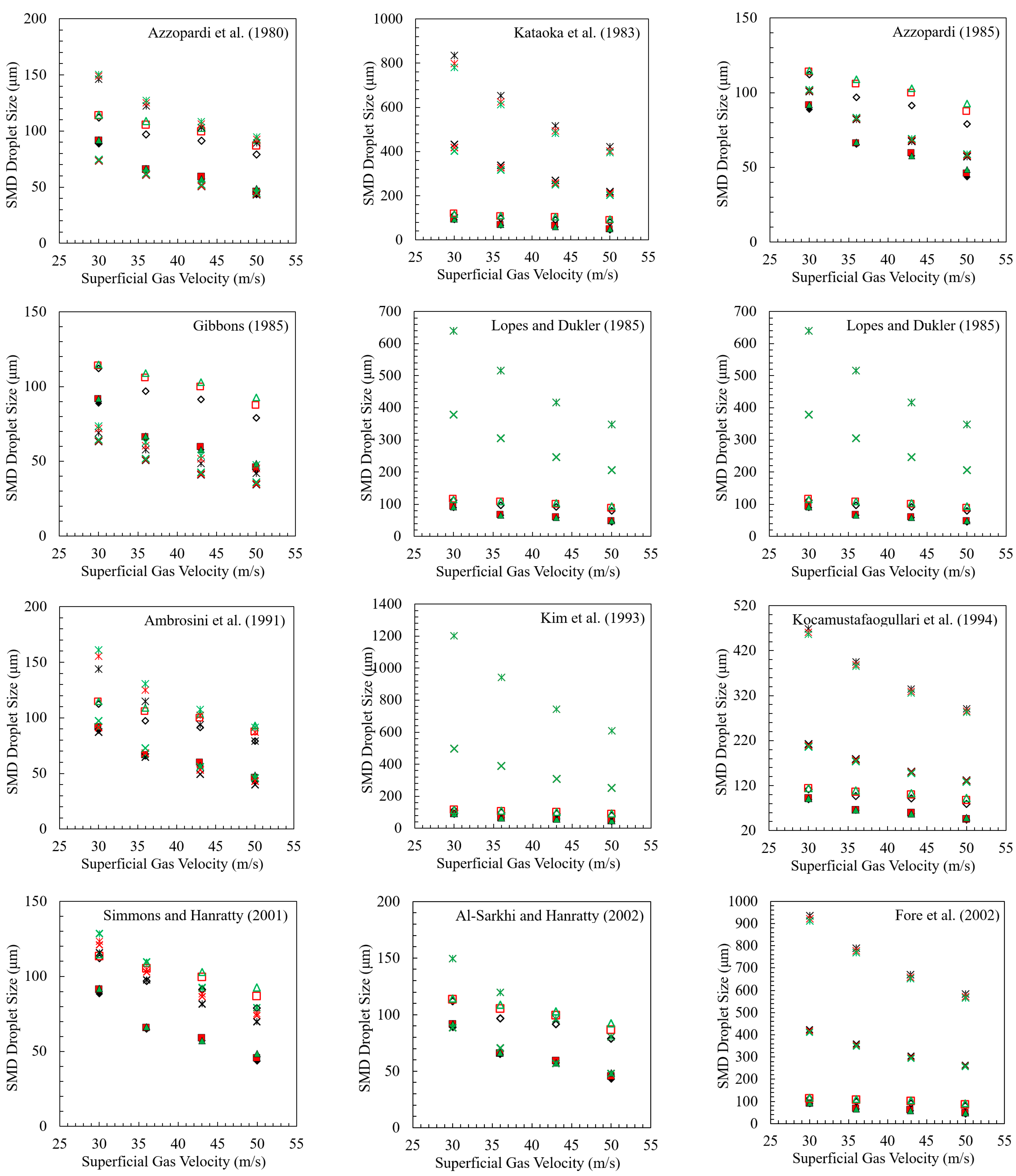
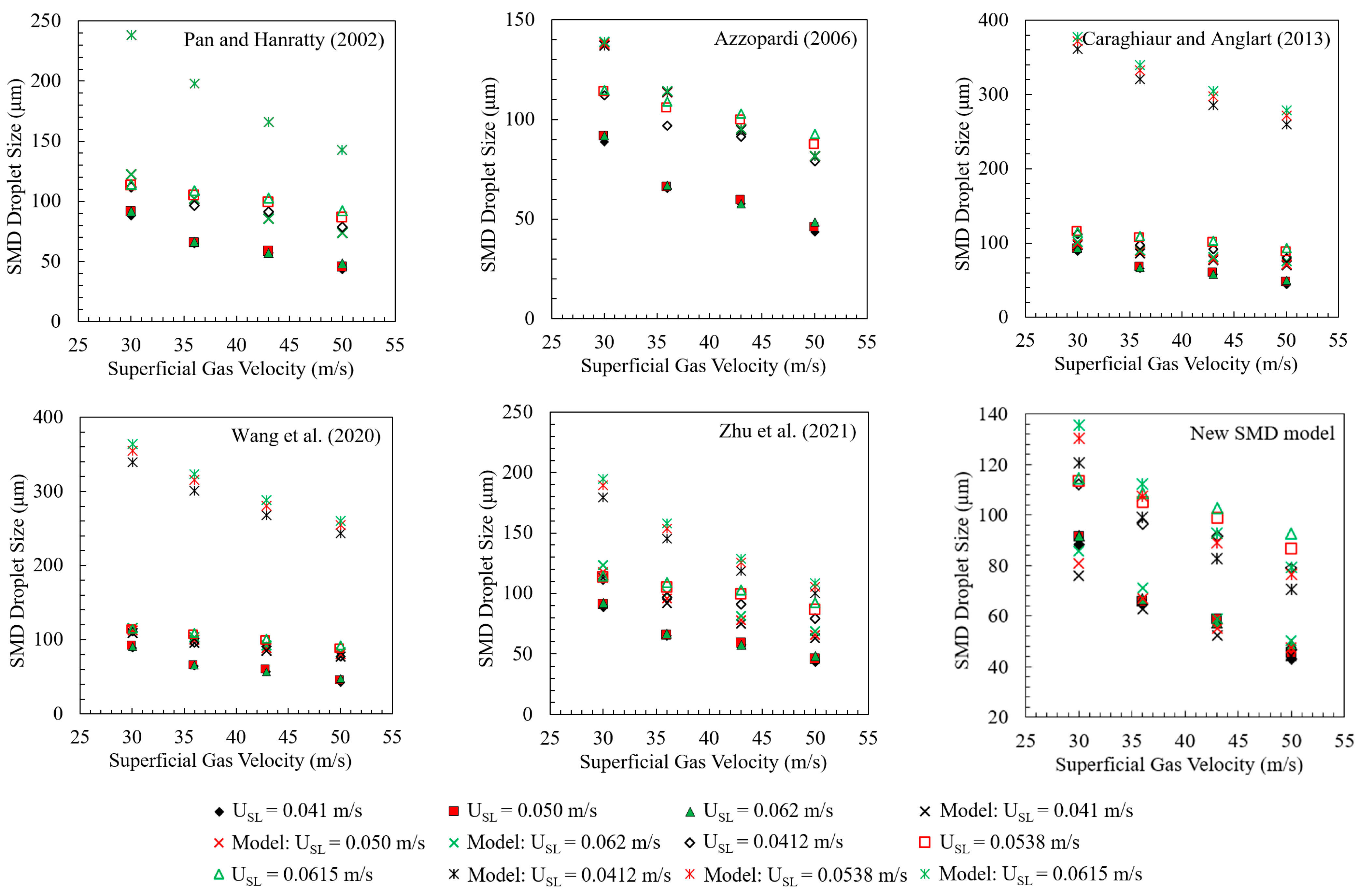
Appendix B
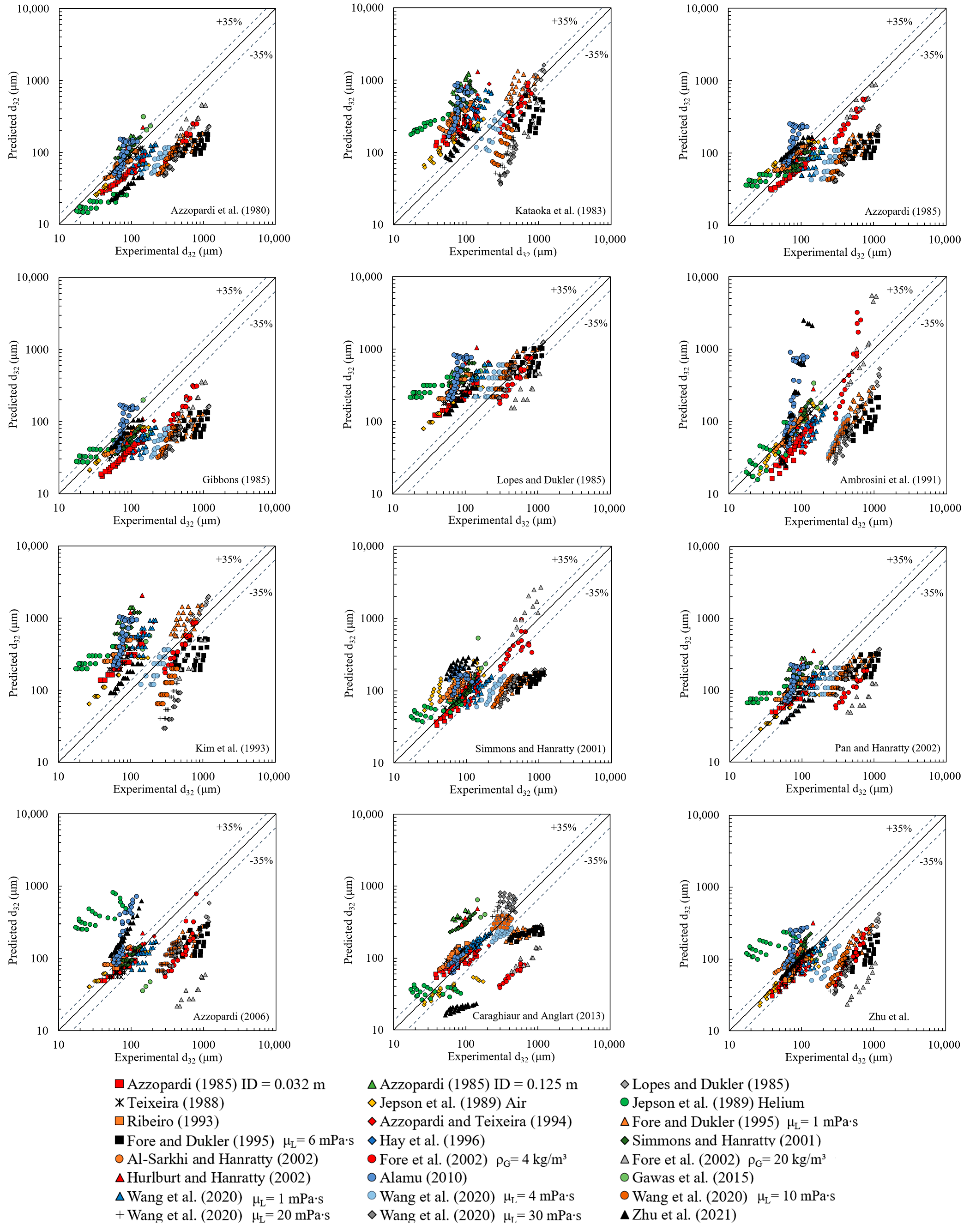
| Authors | dmax/d32 Based on Experimental Data | dmax/d32 Based on New Model Predictions |
|---|---|---|
| Lopes and Dukler (1985) [40] | 2.30 | 2.37 |
| Hurlburt and Hanratty (2002) [61] | 3.10 | 4.66 |
| Al-Sarkhi and Hanratty (2002) [58]; data analysis | 4.37 | 5.43 |
| Fore et al. (2002) [48]; data analysis | 2.65 | 3.41 |
| Gawas et al. (2015) [55]; data analysis | 2.74 | 1.89 |
References
- Azzopardi, B.J. Drops in annular two-phase flow. Int. J. Multiph. Flow 1997, 23, 1–53. [Google Scholar] [CrossRef]
- Berna, C.; Escrivá, A.; Muñoz-Cobo, J.L.; Herranz, L.E. Review of droplet entrainment in annular flow: Interfacial waves and onset of entrainment. Prog. Nuclear Energy 2014, 74, 14–43. [Google Scholar] [CrossRef]
- Andreussi, P.; Azzopardi, B.J. Droplet deposition and interchange in annular two-phase flow. Int. J. Multiph. Flow 1983, 9, 681–695. [Google Scholar] [CrossRef]
- Stoner, H.M.; Koh, C.A. Perspective on the role of particle size measurements in gas hydrate agglomeration predictions. Fuel 2021, 304, 121385. [Google Scholar] [CrossRef]
- Al Munif, E.; Alrashed, A.; Karatayev, K.; Miskimins, J.; Fan, Y. Modeling the Effects of Various Liquid Droplet Sizes in Acoustic Deliquification Techniques. Available online: https://onepetro.org/speeesc/proceedings-abstract/21EESC/2-21EESC/472408 (accessed on 1 November 2021).
- Azzopardi, B.J. Drop sizes in annular two-phase flow. Exp. Fluids 1985, 3, 53–59. [Google Scholar] [CrossRef]
- Ribeiro, A.M.; Bott, T.R.; Jepson, D.M. The influence of a bend on drop sizes in horizontal annular two-phase flow. Int. J. Multiph. Flow 2001, 27, 721–728. [Google Scholar] [CrossRef]
- Koh, C.A.; Sum, A. Natural Gas Hydrates in Flow Assurance; Gulf Professional Publishing: Houston, TX, USA, 2010. [Google Scholar]
- Ishii, M.; Grolmes, M.A. Inception criteria for droplet entrainment in two-phase concurrent film flow. AIChE J. 1975, 21, 308–318. [Google Scholar] [CrossRef]
- Teixeira, J.C.F. Turbulence in Annular Two Phase Flow; University of Birmingham: Birmingham, UK, 1988. [Google Scholar]
- Fore, L.B.; Dukler, A.E. The distribution of drop size and velocity in gas-liquid annular flow. Int. J. Multiph. Flow 1995, 21, 137–149. [Google Scholar] [CrossRef]
- Brauner, N. The prediction of dispersed flows boundaries in liquid–liquid and gas–liquid systems. Int. J. Multiph. Flow 2001, 27, 885–910. [Google Scholar] [CrossRef]
- Caraghiaur, D.; Anglart, H. Drop deposition in annular two-phase flow calculated with Lagrangian Particle Tracking. Nucl. Eng. Des. 2013, 265, 856–866. [Google Scholar] [CrossRef]
- Hinze, J.O. Fundamentals of the hydrodynamic mechanism of splitting in dispersion processes. AIChE J. 1955, 1, 289–295. [Google Scholar] [CrossRef]
- Wang, L.-S.; Krull, B.; Lucas, D.; Meller, R.; Schlegel, F.; Tekavčič, M.; Xu, J. Simulation of droplet entrainment in annular flow with a morphology adaptive multifield two-fluid model. Phys. Fluids 2023, 35, 103312. [Google Scholar] [CrossRef]
- Han, H.; Gabriel, K. A Numerical Study of Entrainment Mechanism in Axisymmetric Annular Gas-Liquid Flow. J. Fluids Eng. 2006, 129, 293–301. [Google Scholar] [CrossRef]
- Kumar, P.; Das, A.K.; Mitra, S.K. Physical understanding of gas-liquid annular flow and its transition to dispersed droplets. Phys. Fluids 2016, 28, 072101. [Google Scholar] [CrossRef]
- Liu, X.-L.; Hu, S.-Q.; Wang, Y.; Liu, L.-L.; Zhang, Y. Numerical investigation on the droplet entrainment from gas sheared liquid film of hydroxyl-terminated polybutadiene/paraffin fuels. Phys Fluids 2023, 35, 012108. [Google Scholar] [CrossRef]
- Xie, Z.; Hewitt, G.F.; Pavlidis, D.; Salinas, P.; Pain, C.C.; Matar, O.K. Numerical study of three-dimensional droplet impact on a flowing liquid film in annular two-phase flow. Chem. Eng. Sci. 2017, 166, 303–312. [Google Scholar] [CrossRef]
- Fan, W.; Li, H.; Anglart, H. Numerical investigation of spatial and temporal structure of annular flow with disturbance waves. Int. J. Multiph. Flow 2019, 110, 256–272. [Google Scholar] [CrossRef]
- Rodriguez, J.M. Numerical Simulation of Two-Phase Annular Flow. Ph.D. Dissertation, Rensselaer Polytechnic Institute, Troy, NY, USA, 2009. [Google Scholar]
- Liu, Y.; Cui, J.; Li, W.Z. A two-phase, two-component model for vertical upward gas–liquid annular flow. Int. J. Heat. Fluid. Flow 2011, 32, 796–804. [Google Scholar] [CrossRef]
- Nie, B.C.; Guan, X.; Vanden-Broeck, J.-M.; Dias, F. Air/water interfacial waves with a droplet at the tip of their crest. Phys Fluids 2023, 35, 012101. [Google Scholar] [CrossRef]
- Abobaker, E.; Elsanoose, A.; Shirokoff, J.; Rahman, M.A. CFD Simulation of Two-Phase Vertical Annular Flow in Both Upward and Downward Direction in a Small Pipe. In Proceedings of the ASME 2019 38th International Conference on Ocean, Offshore and Arctic Engineering, Glasgow, Scotland, UK, 9–14 June 2019. V008T11A051. [Google Scholar]
- Saxena, A.; Prasser, H.-M. A study of two-phase annular flow using unsteady numerical computations. Int. J. Multiph. Flow 2020, 126, 103037. [Google Scholar] [CrossRef]
- Huo, J.; Wang, L.; Tang, J.; Shi, X. Droplets impact against the random rough surface with a liquid film. Phys Fluids 2023, 35, 063314. [Google Scholar]
- Yun, G.; Ishiwatari, Y.; Ikejiri, S.; Oka, Y. Numerical analysis of the onset of droplet entrainment in annular two-phase flow by hybrid method. Ann. Nucl. Energy 2010, 37, 230–240. [Google Scholar] [CrossRef]
- Xie, H.; Koshizuka, S.; Oka, Y. Numerical Simulation of Liquid Drop Deposition in Annular-Mist Flow Regime of Boiling Water Reactor. J. Nucl. Sci. Technol. 2004, 41, 569–578. [Google Scholar] [CrossRef][Green Version]
- Wang, S.; Wang, H.; Wu, Y.; Cheng, Y. Numerical simulation of the droplet formation involving fluids with high viscosity ratio by lattice Boltzmann method. Phys. Fluids 2023, 35, 063107. [Google Scholar]
- Liu, Y.; Li, W.Z. Numerical Simulation of Droplet Size Distribution in Vertical Upward Annular Flow. J. Fluids Eng. 2010, 132, 121402. [Google Scholar] [CrossRef]
- Azzopardi, B.J. Mechanisms of Entrainment in Annular Two Phase Flow; UKAEA Atomic Energy Research Establishment: Abingdon, UK, 1983. [Google Scholar]
- Zhang, Z.; Wang, Z.; Liu, H.; Gao, Y.; Li, H.; Sun, B. Experimental study on entrained droplets in vertical two-phase churn and annular flows. Int. J. Heat. Mass. Transf. 2019, 138, 1346–1358. [Google Scholar] [CrossRef]
- Badie, S.; Lawrence, C.J.; Hewitt, G.F. Axial viewing studies of horizontal gas–liquid flows with low liquid loading. Int. J. Multiph. Flow 2001, 27, 1259–1269. [Google Scholar] [CrossRef]
- Lecoeur, N. Interfacial Behaviour in Stratified and Stratifying Annular Flows. Ph.D. Dissertation, Imperial College London, London, UK, 2013. [Google Scholar]
- Pham, S.H.; Kawara, Z.; Yokomine, T.; Kunugi, T. Detailed observations of wavy interface behaviors of annular two-phase flow on rod bundle geometry. Int. J. Multiph. Flow 2014, 59, 135–144. [Google Scholar] [CrossRef]
- Cherdantsev, A.V.; Hann, D.B.; Azzopardi, B.J. Study of gas-sheared liquid film in horizontal rectangular duct using high-speed LIF technique: Three-dimensional wavy structure and its relation to liquid entrainment. Int. J. Multiph. Flow 2014, 67, 52–64. [Google Scholar] [CrossRef]
- Troitskaya, Y.; Kandaurov, A.; Ermakova, O.; Kozlov, D.; Sergeev, D.; Zilitinkevich, S. Bag-breakup fragmentation as the dominant mechanism of sea-spray production in high winds. Sci. Rep. 2017, 7, 1614. [Google Scholar] [CrossRef]
- Cherdantsev, A.V. Experimental Investigation of Mechanisms of Droplet Entrainment in Annular Gas-Liquid Flows: A Review. Water 2022, 14, 3892. [Google Scholar] [CrossRef]
- Berna, C.; Escrivá, A.; Muñoz-Cobo, J.L.; Herranz, L.E. Review of droplet entrainment in annular flow: Characterization of the entrained droplets. Prog. Nuclear Energy 2015, 79, 64–86. [Google Scholar] [CrossRef]
- Lopes, J.C.B.; Dukler, A.E. Droplet Sizes, Dynamics and Deposition in Vertical Annular Flow; Report No.: NUREG/CR-4424; Department of Chemical Engineering, Houston University: Houston, TX, USA, October 1985. [Google Scholar] [CrossRef]
- Kocamustafaogullari, G.; Smits, S.R.; Razi, J. Maximum and mean droplet sizes in annular two-phase flow. Int. J. Heat. Mass. Transf. 1994, 37, 955–965. [Google Scholar] [CrossRef]
- Whalley, P.B.; Hewitt, G.F. Photographic Studies of Two-Phase Flow Using a Parallel Light Technique; UKAEA Atomic Energy Research Establishment: Abingdon, UK, 1979. [Google Scholar]
- Tayali, N.E.; Bates, C.J.; Yeoman, M.L. Drop Size and Velocity Measurements in Vertical Developing Annular Two-Phase Flow. In Proceedings of the 3rd International Conference on Laser Anemometry Advances and Application, Swansea, UK, 26–29 September 1990. [Google Scholar]
- Azzopardi, B.J.; Teixeira, J.C.F. Detailed Measurements of Vertical Annular Two-Phase Flow—Part I: Drop Velocities and Sizes. J. Fluids Eng. 1994, 116, 792–795. [Google Scholar] [CrossRef]
- Ueda, T. Entrainment Rate and Size of Entrained Droplets in Annular Two-Phase Flow. Bull. JSME 1979, 22, 1258–1265. [Google Scholar] [CrossRef]
- Kataoka, I.; Ishii, M.; Mishima, K. Generation and Size Distribution of Droplet in Annular Two-Phase Flow. J. Fluids Eng. 1983, 105, 230–238. [Google Scholar] [CrossRef]
- Gibbons, D.B. Drop Formation in Annular Two-Phase Flow. Ph.D. Thesis, University of Birmingham, Birmingham, UK, 1985. [Google Scholar]
- Fore, L.B.; Ibrahim, B.B.; Beus, S.G. Visual measurements of droplet size in gas–liquid annular flow. Int. J. Multiph. Flow 2002, 28, 1895–1910. [Google Scholar] [CrossRef][Green Version]
- Wang, Z.; Liu, H.; Zhang, Z.; Sun, B.; Zhang, J.; Lou, W. Research on the effects of liquid viscosity on droplet size in vertical gas–liquid annular flows. Chem. Eng. Sci. 2020, 220, 115621. [Google Scholar] [CrossRef]
- Jepson, D.M.; Azzopardi, B.J.; Whalley, P.B. The effect of gas properties on drops in annular flow. Int. J. Multiph. Flow 1989, 15, 327–339. [Google Scholar] [CrossRef]
- Pan, L.; Hanratty, T.J. Correlation of entrainment for annular flow in vertical pipes. Int. J. Multiph. Flow 2002, 28, 363–384. [Google Scholar] [CrossRef]
- Patruno, L.E.; Marchioro Ystad, P.A.; Jenssen, C.B.; Marchetti, J.M.; Dorao, C.A.; Svendsen, H.F.; Jakobsen, H.A. Liquid entrainment—Droplet size distribution for a low surface tension mixture. Chem. Eng. Sci. 2010, 65, 5272–5284. [Google Scholar] [CrossRef]
- van Nimwegen, A.T.; Portela, L.M.; Henkes, R.A.W.M. The effect of surfactants on air–water annular and churn flow in vertical pipes. Part 1: Morphology of the air–water interface. Int. J. Multiph. Flow 2015, 71, 133–145. [Google Scholar] [CrossRef]
- Magrini, K.L. Liquid Entrainment in Annular Gas-liquid Flow in Inclined Pipes. Master’s Thesis, The University of Tulsa, Tulsa, OK, USA, 2012. [Google Scholar]
- Gawas, K.; Pereyra, E.; Sarica, C. Experimental study of drop size distribution for air-oil two-phase flow in large diameter pipe. In Proceedings of the SPE Annual Technical Conference and Exhibition, Houston, TX, USA, 28–30 September 2015. [Google Scholar] [CrossRef]
- Ribeiro, A.M. Studies of Gas–Liquid Flow in Bends. Ph.D. Thesis, University of Birmingham, Birmingham, UK, 1993. [Google Scholar]
- Bates, C.J.; Sheriff, J.M. High data rate measurements of droplet dynamics in a vertical gas-liquid annular flow. Flow. Meas. Instrum. 1992, 3, 247–256. [Google Scholar] [CrossRef]
- Al-Sarkhi, A.; Hanratty, T.J. Effect of pipe diameter on the drop size in a horizontal annular gas–liquid flow. Int. J. Multiph. Flow 2002, 28, 1617–1629. [Google Scholar] [CrossRef]
- Simmons, M.J.H.; Hanratty, T.J. Droplet size measurements in horizontal annular gas–liquid flow. Int. J. Multiph. Flow 2001, 27, 861–883. [Google Scholar] [CrossRef]
- Schadel, S.A.; Hanratty, T.J. Interpretation of atomization rates of the liquid film in gas-liquid annular flow. Int. J. Multiph. Flow 1989, 15, 893–900. [Google Scholar] [CrossRef]
- Hurlburt, E.; Hanratty, T. Measurement of drop size in horizontal annular flow with the immersion method. Exp. Fluids 2002, 32, 692–699. [Google Scholar] [CrossRef]
- Azzopardi, B.J.; Freeman, G.; King, D.J. Drop Sizes and Deposition in Annular Two-Phase Flow; UKAEA Report AERE-R9634; United Kingdom Atomic Energy Authority: Abingdon, UK, 1980.
- Zaidi, S.H.; Altunbas, A.; Azzopardi, B.J. A comparative study of phase Doppler and laser diffraction techniques to investigate drop sizes in annular two-phase flow. Chem. Eng. J. 1998, 71, 135–143. [Google Scholar] [CrossRef]
- Hay, K.J.; Liu, Z.-C.; Hanratty, T.J. Relation of deposition to drop size when the rate law is nonlinear. Int. J. Multiph. Flow 1996, 22, 829–848. [Google Scholar] [CrossRef]
- Alamu, M.B. Investigation of Periodic Structures in Gas-Liquid Flow; University of Nottingham: Nottingham, UK, 2010. [Google Scholar]
- Zhu, F.; Yan, C.; Liu, A.; Gu, H.; Gong, S. Droplet size of vertically upward annular flow in a narrow rectangular channel. Chem. Eng. Res. Des. 2021, 174, 107–115. [Google Scholar] [CrossRef]
- Wicks, M.I.I.I.; Dukler, A.E. In situ measurements of drop size distribution in two-phase flow—A new method for electrically conducting liquids. In Proceeding of International Heat Transfer Conference; Begellhouse: Danbury, CT, USA, 2019. [Google Scholar] [CrossRef]
- Pogson, J.T.; Roberts, J.H.; Waibler, P.J. An Investigation of the Liquid Distribution in Annular-Mist Flow. J. Heat Transf. 1970, 92, 651–658. [Google Scholar] [CrossRef]
- Tatterson, D.F. Rates of Atomization and Drop Size in Annular Two-Phase Flow. Ph.D. Thesis, University of Illinois at Urbana-Champaign, Urbana, IL, USA, 1975. [Google Scholar]
- Andreussi, P.; Romano, G.; Zanelli, S. Drop size distribution in annular mist flow. In Proceedings of the First Inter-national Conference on Liquid Atomization and Spray Systems, Tokyo, Japan, 27–31 August 1978. [Google Scholar]
- Lopes, J.C.B.; Dukler, A.E. Droplet entrainment in vertical annular flow and its contribution to momentum transfer. AIChE J. 1986, 32, 1500–1515. [Google Scholar] [CrossRef]
- Rastogi, A.; Fan, Y. Experimental and modeling study of onset of liquid accumulation. J. Nat. Gas Sci. Eng. 2020, 73, 103064. [Google Scholar] [CrossRef]
- Mugele, R.A.; Evans, H.D. Droplet Size Distribution in Sprays. Ind. Eng. Chem. 1951, 43, 1317–1324. [Google Scholar] [CrossRef]
- Kolmogorov, A.N. On the Disintegration of Drops in Turbulent Flow. Dokl. Akad. Nauk. SSSR 1949, 66, 825–828. [Google Scholar]
- Batchelor, G.K. The Theory of Homogeneous Turbulence; Cambridge University Press: Cambridge, UK, 1953. [Google Scholar]
- Shoham, O. Mechanistic Modeling of Gas-Liquid Two-Phase Flow in Pipes; Society of Petroleum Engineers: Houston, TX, USA, 2006. [Google Scholar]
- Tatterson, D.F.; Dallman, J.C.; Hanratty, T.J. Drop sizes in annular gas-liquid flows. AIChE J. 1977, 23, 68–76. [Google Scholar] [CrossRef]
- Pereyra, E.J.; Shoham, O.; Gomez, L.E.; Mohan, R.S. Integration Methodology of Separation Units Using Steady-State Particle Size Approach. In Proceedings of the Twenty-fifth International Ocean and Polar Engineering Conference, Kona, HI, USA, 21–26 June 2015. [Google Scholar]
- Beggs, D.H.; Brill, J.P. A study of two-phase flow in inclined pipes. J. Pet. Technol. 1973, 25, 607–617. [Google Scholar] [CrossRef]
- Azzopardi, B.J.; Teixeira, J.C.F.; Jepson, D.M. Drop sizes and velocities in vertical annular two-phase flow. In Proceedings of the International Conference on Mechanics of Two-Phase Flows, Taipei, Taiwan, 12–15 June 1989; pp. 12–15. [Google Scholar]
- Ambrosini, W.; Andreussi, P.; Azzopardi, B.J. A physically based correlation for drop size in annular flow. Int. J. Multiph. Flow 1991, 17, 497–507. [Google Scholar] [CrossRef]
- Kim, B.H.; Peterson, G.P.; Kihm, K.D. Analytical and Experimental Investigation of Entrainment in Capillary Pumped Wicking Structures. J. Energy Res. Technol. 1993, 115, 278–286. [Google Scholar] [CrossRef]
- Azzopardi, B.J. Gas-Liquid Flows; Begell House: New York, NY, USA, 2006. [Google Scholar]
- Wallis, G.B. One-Dimensional Two-Phase Flow; McGraw-Hill: New York, NY, USA, 1969. [Google Scholar]
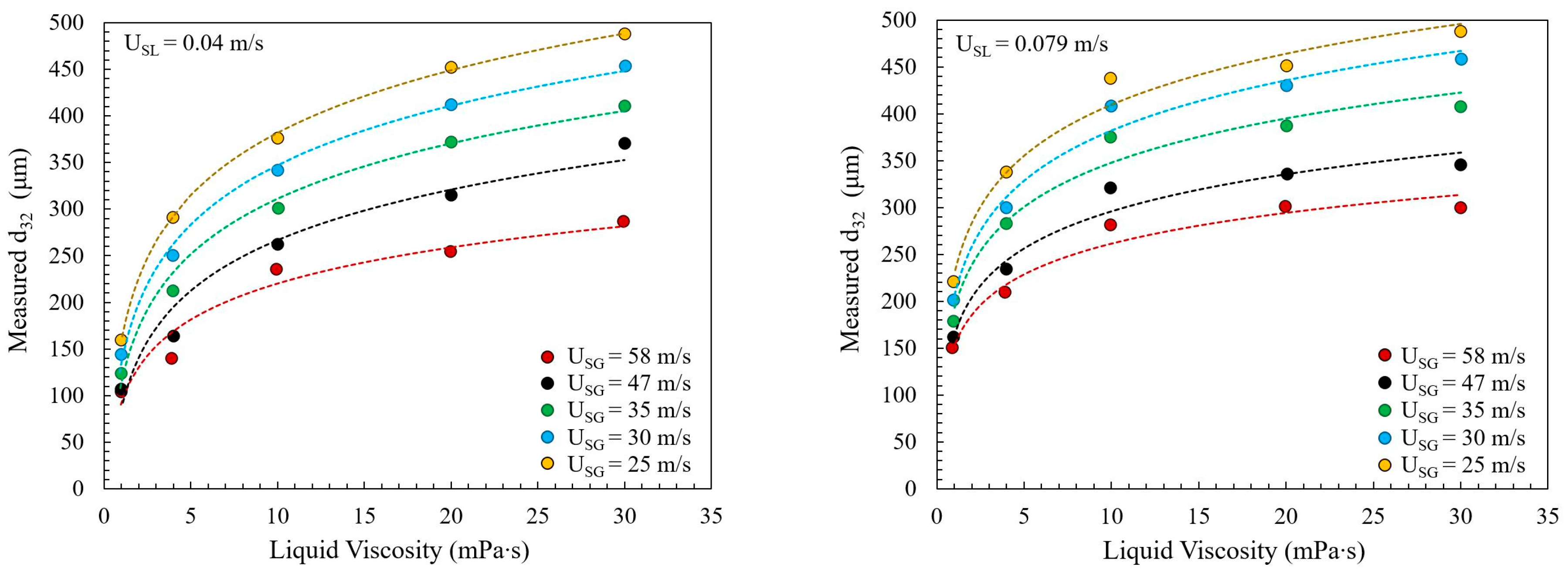
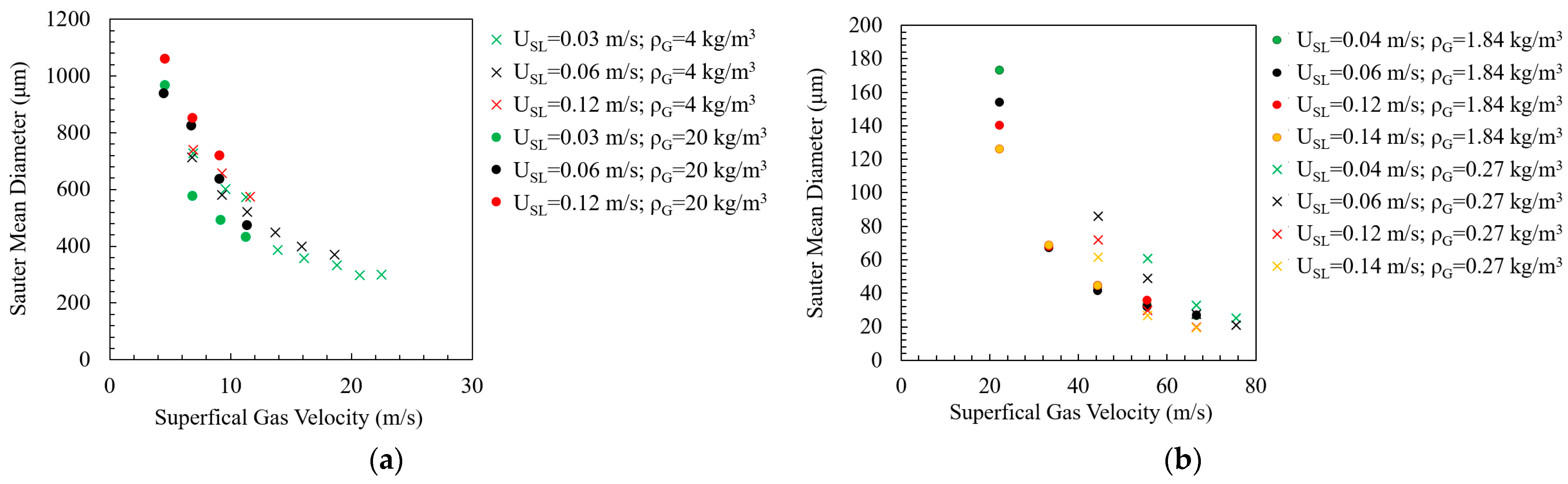

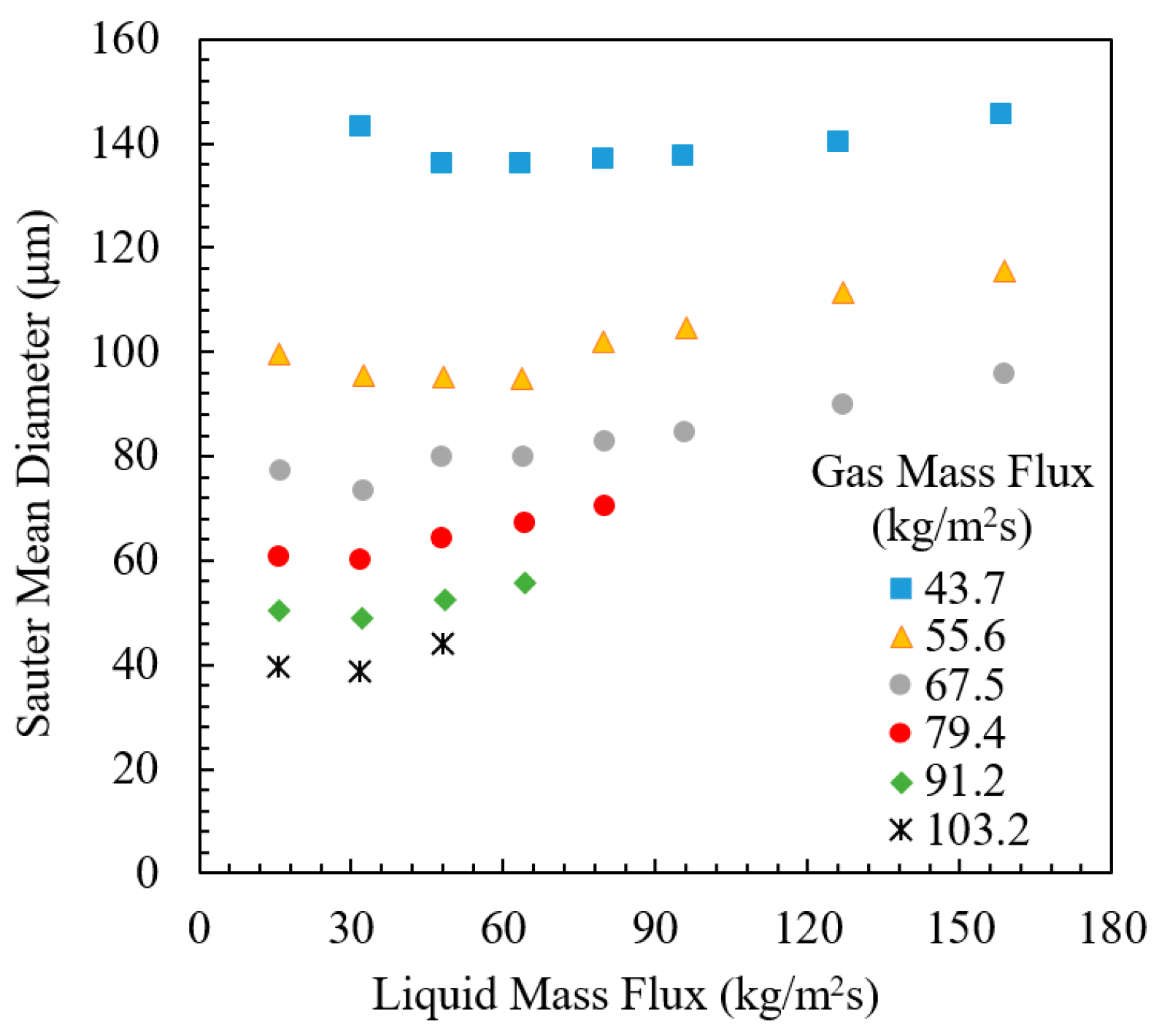
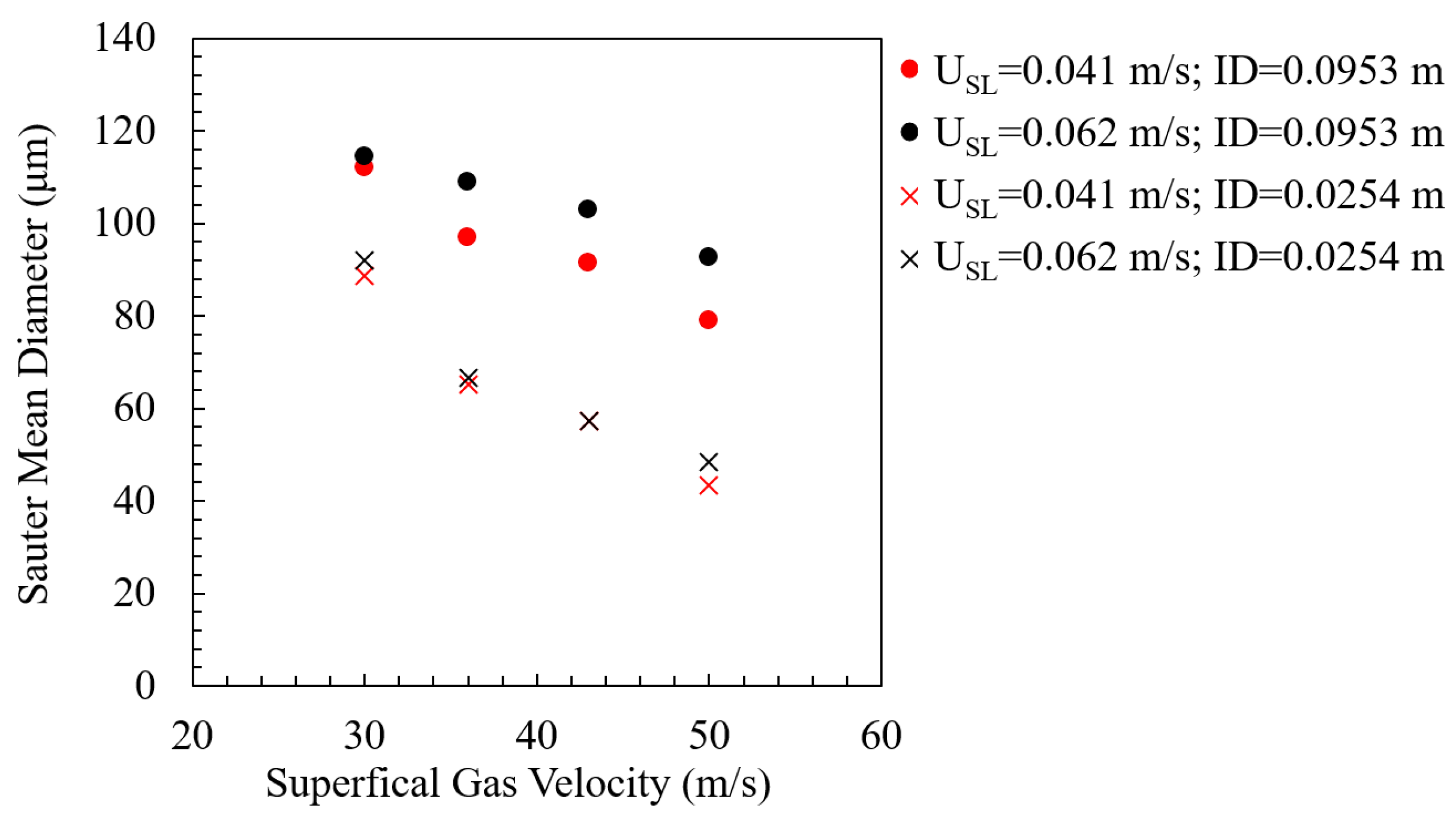

| Authors | Fluids and Flow Geometry | Operational Conditions | Measurement Technique | ||
|---|---|---|---|---|---|
| Lopes and Dukler (1985) [40] | Air–water; vertical upflow pipe ID = 0.051 m | 833 | 53 | 1 atm, 20 °C; | Phase Doppler Anemometry |
| Fore and Dukler (1995a) [11] | Air–water; vertical upflow pipe ID = 0.051 m | 760 | 53 | 1 atm, 20 °C; | Laser Grating |
| Fore and Dukler (1995a) [11] | Air–water/glycerin Vertical upflow pipe ID = 0.051 m | 870 | 303 | 1 atm, 20 °C; | Laser Grating |
| Hay et al. (1996) [64] | Air–water; vertical upflow pipe ID = 0.042 m | 816 | 45 | 1 atm, 20 °C; | Direct In Situ Photography |
| Al-Sarkhi and Hanratty (2002) [58] | Air–water; horizontal flow pipe ID = 0.025 m | 816 | 45 | 1 atm, 20 °C; | Laser Diffraction |
| Fore et al. (2002) [48] | Nitrogen–water; vertical upflow 101.6 × 5.08 mm rectangular duct | 250 | 37 | 3.4 atm, 38 °C; | Video Images |
| Fore et al. (2002) [48] | Nitrogen–water; vertical upflow 101.6 × 5.08 mm rectangular duct | 50 | 37 | 17 atm, 38 °C; | Video Images |
| Hurlburt and Hanratty (2002) [61] | Air–water; horizontal flow pipe ID = 0.095 m | 816 | 45 | 1 atm, 20 °C; | Immersion Method |
| Gawas et al. (2015) [55] | Air–mineral oil; horizontal flow pipe ID = 0.152 m | 360 | 78 | 1 atm, 20 °C; | Backlit High-Speed Visualization |
| Authors | Fluids and Flow Geometry | Operational Conditions | Measurement Technique | ||
|---|---|---|---|---|---|
| Azzopardi (1985) [6] | Air–water, vertical upward ID = 0.032 m | 816 | 50 | 1 atm; 20 °C; ReSL = 500–5000 ReSG = 69,500–164,000 | Laser Diffraction |
| Azzopardi (1985) [6] | Air–water, vertical upward ID = 0.125 m | 816 | 50 | 1 atm; 20 °C; ReSL = 1000–3200 ReSG = 224,000–320,600 | Laser Diffraction |
| Lopes and Dukler (1985) [40] | Air–water, vertical upward ID = 0.051 m | 833 | 53 | 1 atm, 20 °C ReSL = 1600–5800 ReSG = 42,600–75,200 | Phase Doppler Anemometry |
| Teixeira (1988) [10] | Air–water, vertical upward ID = 0.032 m | 555 | 64 | 1.5 atm, 15 °C ReSL = 450–3500 ReSG = 78,250–163,300 | Laser Diffraction and Phase Doppler Anemometry |
| Jepson et al. (1989) [50] | Air–water, vertical upward ID = 0.010 m | 544 | 53 | 1.5 atm, 20 °C ReSL = 400–1400 ReSG = 20,900–62,800 | Laser Diffraction |
| Jepson et al. (1989) [50] | Helium–water, vertical upward ID = 0.010 m | 3733 | 56 | 1.5 atm, 20 °C ReSL = 400–1400 ReSG = 6500–11,100 | Laser Diffraction |
| Ribeiro (1993) [56] | Air–water, horizontal ID = 0.032 m | 591–640 | 64 | 1 atm, 20 °C ReSL = 500–3000 ReSG = 71,500–125,100 | Laser Diffraction |
| Azzopardi and Teixeira (1994) [44] | Air–water, vertical upward ID = 0.032 m | 833 | 53 | 1 atm, 20 °C ReSL = 500–1400 ReSG = 39,200–89,000 | Laser Diffraction |
| Fore and Dukler (1995a) [11] | Air–water, vertical upward ID = 0.051 m | 694–847 | 53 | 1 atm, 20 °C ReSL = 750–3000 ReSG = 51,800–120,700 | Laser Grating |
| Fore and Dukler (1995a) [11] | Air–water/glycerin vertical upward; ID = 0.051 m | 794–956 | 303 | 1 atm, 20 °C ReSL = 140–560 ReSG = 48,800–114,000 | Laser Grating |
| Hay et al. (1996) [64] | Air–water, vertical upward ID = 0.042 m | 816 | 45 | 1 atm, 20 °C ReSL = 1000–5700 ReSG = 92,600 | Direct In Situ Photography |
| Simmons and Hanratty (2001) [59] | Air–water, horizontal ID = 0.095 m | 816 | 45 | 1 atm, 20 °C ReSL = 1700–13,000 ReSG = 175,000–292,000 | Laser Diffraction |
| Al-Sarkhi and Hanratty (2002) [58] | Air–water, horizontal ID = 0.025 m | 816 | 45 | 1 atm, 20 °C ReSL = 1100–3500 ReSG = 46,700–77,800 | Laser Diffraction |
| Fore et al. (2002) [48] | Nitrogen–water; vertical upward 101.6 × 5.08 mm rectangular duct | 250 | 37 | 3.4 atm, 38 °C ReSL = 300–2600 ReSG = 10,800–35,700 | Photographic |
| Fore et al. (2002) [48] | Nitrogen–water, vertical upflow 101.6 × 5.08 mm rectangular duct | 50 | 37 | 17 atm, 38 °C ReSL = 300–1300 ReSG = 35,700–90,500 | Photographic |
| Hurlburt and Hanratty (2002) [61] | Air–water, horizontal ID = 0.095 m | 816 | 45 | 1 atm, 20 °C ReSL = 1600–7400 ReSG = 117,000–210,000 | Oil Immersion |
| Alamu (2010) [65] | Air–water, vertical upward ID = 0.019 m | 714 | 56 | 1.4 atm, 20 °C ReSL = 950–3700 ReSG = 19,400–63,400 | Light Scattering |
| Gawas et al. (2015) [55] | Air–mineral oil, horizontal ID = 0.152 m | 360 | 78 | 1 atm, 20 °C ReSL = 800–8200 ReSG = 296,000–408,000 | Backlit High-Speed Visualization |
| Wang et al. (2020) [49] | Air–water, vertical upward ID = 0.050 m | 816–955 | 50–1500 | 1 atm, 20 °C ReSL = 100–4000 ReSG = 76,500–177,600 | Particle Dynamic Analyzer |
| Zhu et al. (2021) [66] | Air–water, vertical upward 70 × 2 mm narrow rectangular channel | 816 | 50 | 1 atm, 20 °C ReSL = 580–1170 ReSG = 4760–9530 | Laser Diffraction |
| Models | Hinze (1955) [14] | Kataoka et al. (1983) [46] | Lopes and Dukler (1985) [40] | Kocamustafaogullari et al. (1994) [41] | Pereyra et al. (2015) [78] | New Model |
|---|---|---|---|---|---|---|
| AARE (%) | 417 | 154 | 185 | 71 | 84 | 14.8 |
| Models | Correlations |
|---|---|
| Azzopardi et al. (1980) [62] | |
| Kataoka et al. (1983) [46] | |
| Azzopardi (1985) [6] | |
| Gibbons (1985) [47] | |
| Lopes and Dukler (1985) [40] | |
| Azzopardi et al. (1989) [80] | |
| Ambrosini et al. (1991) [81] | |
| Kim et al. (1993) [82] | |
| Kocamustafaogullari et al. (1994) [41] | |
| Simmons and Hanratty (2001) [59] | |
| Al-Sarkhi and Hanratty (2002) [58] | |
| Fore et al. (2002) [48] | |
| Pan and Hanratty (2002b) [51] | |
| Azzopardi (2006) [83] | |
| Caraghiaur and Anglart (2013) [13] | |
| Wang et al. (2020) [49] | |
| Zhu et al. (2021) [66] |
| Models | AARE (%) | Models | AARE (%) |
|---|---|---|---|
| Azzopardi et al. (1980) [62] | 49 | Simmons and Hanratty (2001) [59] | 63 |
| Kataoka et al. (1983) [46] | 228 | Al-Sarkhi and Hanratty (2002) [58] | 53 |
| Azzopardi (1985) [6] | 49 | Fore et al. (2002) [48] | 259 |
| Gibbons (1985) [47] | 55 | Pan and Hanratty (2002b) [51] | 59 |
| Lopes and Dukler (1985) [40] | 214 | Azzopardi (2006) [83] | 116 |
| Azzopardi et al. (1989) [80] | 44 | Caraghiaur and Anglart (2013) [13] | 67 |
| Ambrosini et al. (1991) [81] | 120 | Wang et al. (2020) [49] | 62 |
| Kim et al. (1993) [82] | 293 | Zhu et al. (2021) [66] | 67 |
| Kocamustafaogullari et al. (1994) [41] | 107 | New Model | 29 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Karatayev, K.; Fan, Y. Modeling Liquid Droplet Sizes in Gas–Liquid Annular Flow. Energies 2024, 17, 3094. https://doi.org/10.3390/en17133094
Karatayev K, Fan Y. Modeling Liquid Droplet Sizes in Gas–Liquid Annular Flow. Energies. 2024; 17(13):3094. https://doi.org/10.3390/en17133094
Chicago/Turabian StyleKaratayev, Kanat, and Yilin Fan. 2024. "Modeling Liquid Droplet Sizes in Gas–Liquid Annular Flow" Energies 17, no. 13: 3094. https://doi.org/10.3390/en17133094
APA StyleKaratayev, K., & Fan, Y. (2024). Modeling Liquid Droplet Sizes in Gas–Liquid Annular Flow. Energies, 17(13), 3094. https://doi.org/10.3390/en17133094