A Light-Load Efficiency Improvement Technique for an Inductive Power Transfer System through a Reconfigurable Circuit
Abstract
1. Introduction
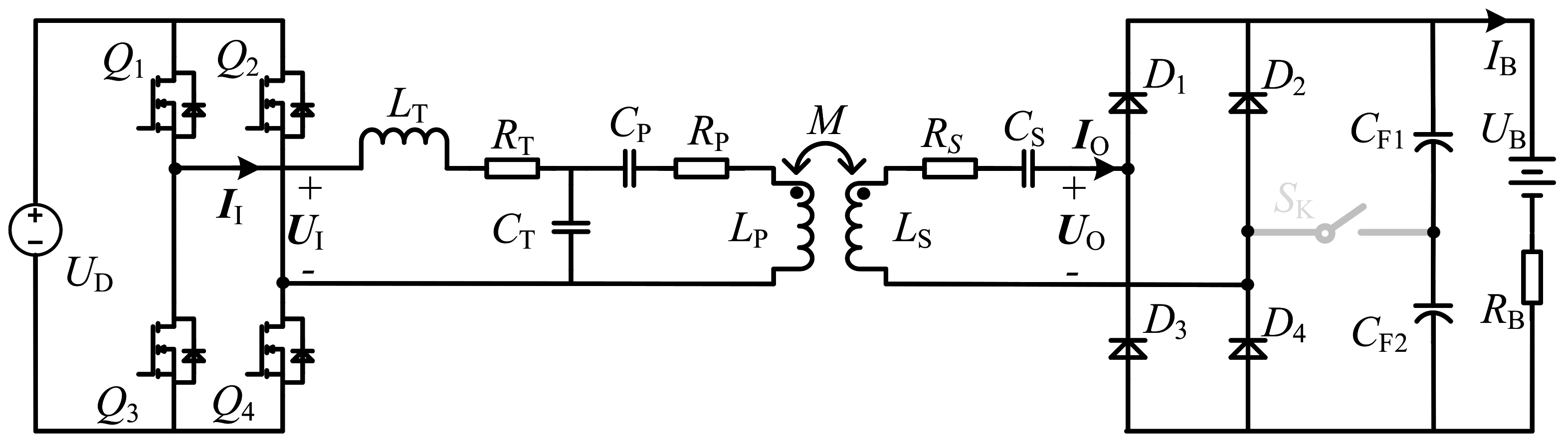
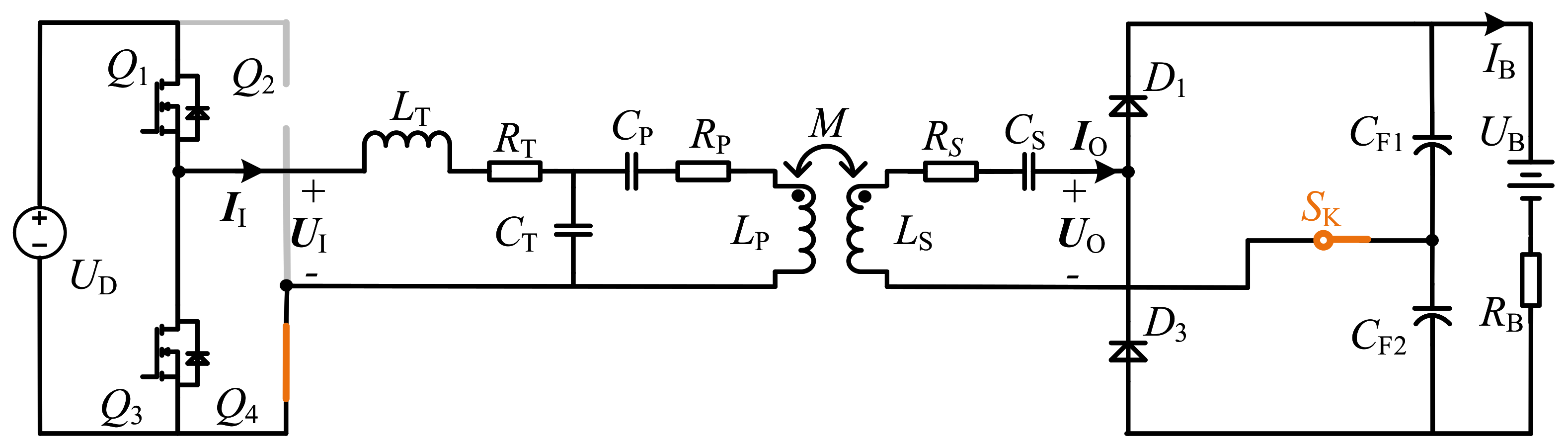
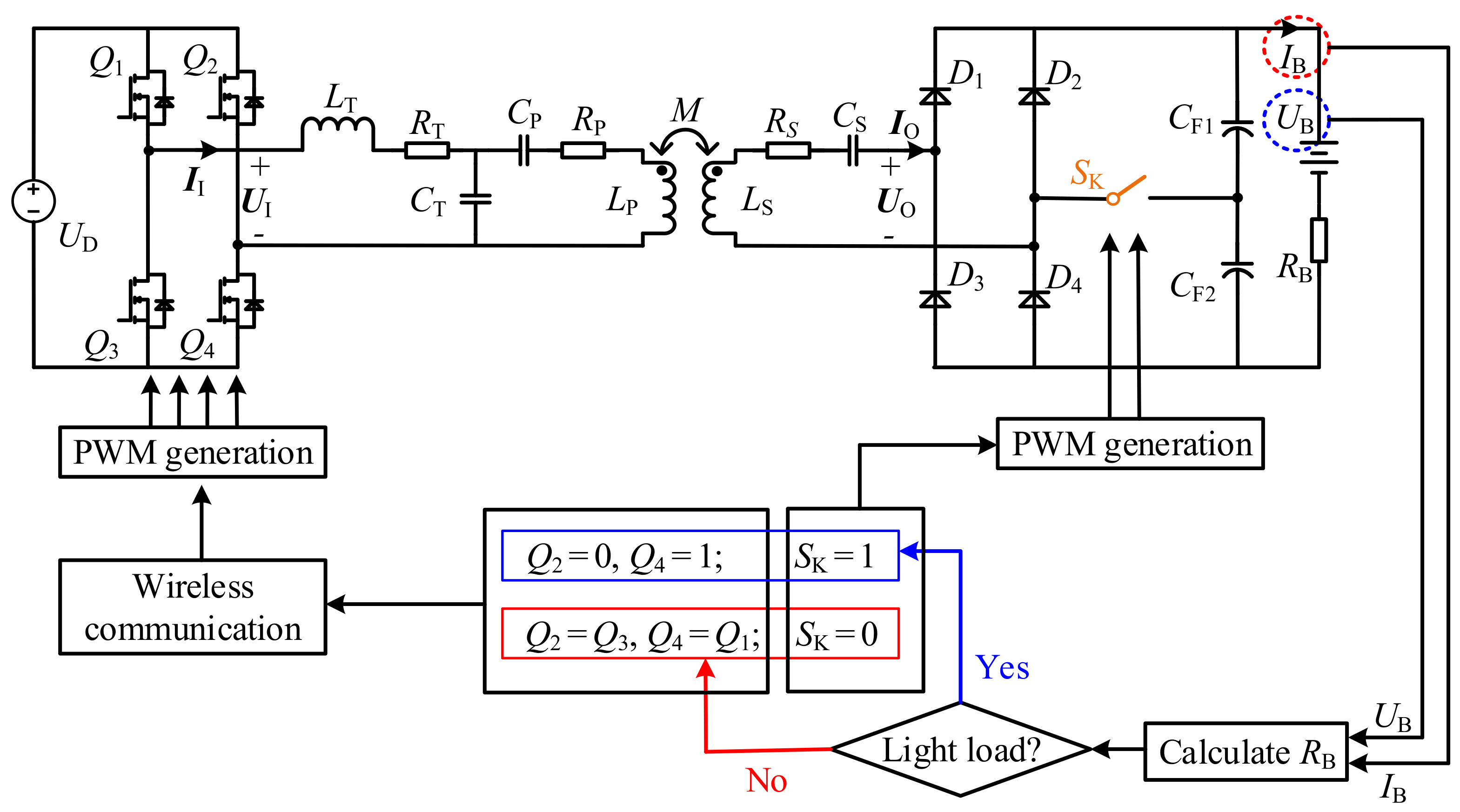
2. Principle of the Light-Load Efficiency Improvement Technique
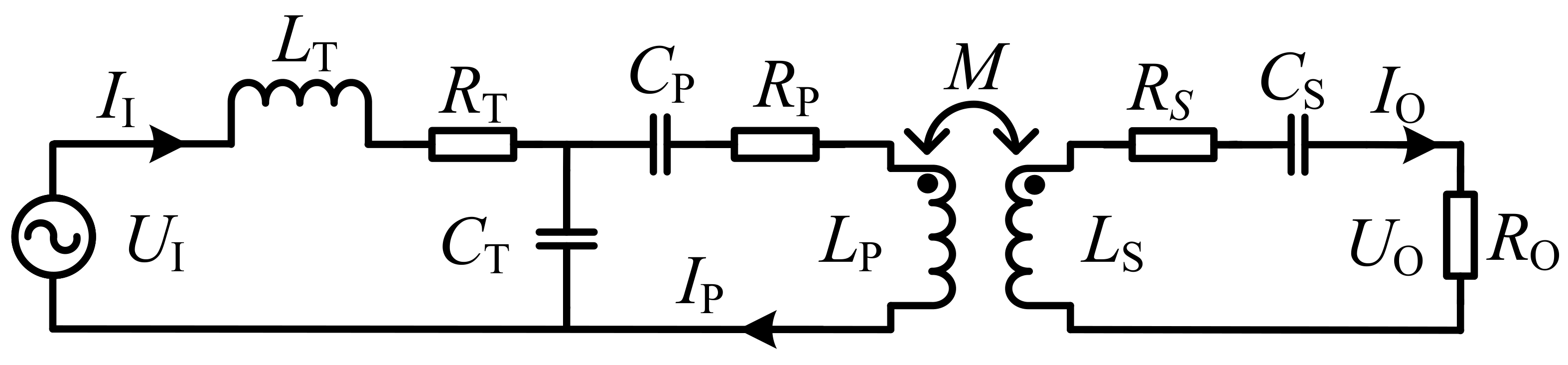
3. Theoretical Analysis
3.1. Analysis of LLEI Technique
3.2. The Consistency of CV Charging in Light-Load and Heavy-Load Modes
3.3. Implementation of the Presented LLEI Technique
- (1)
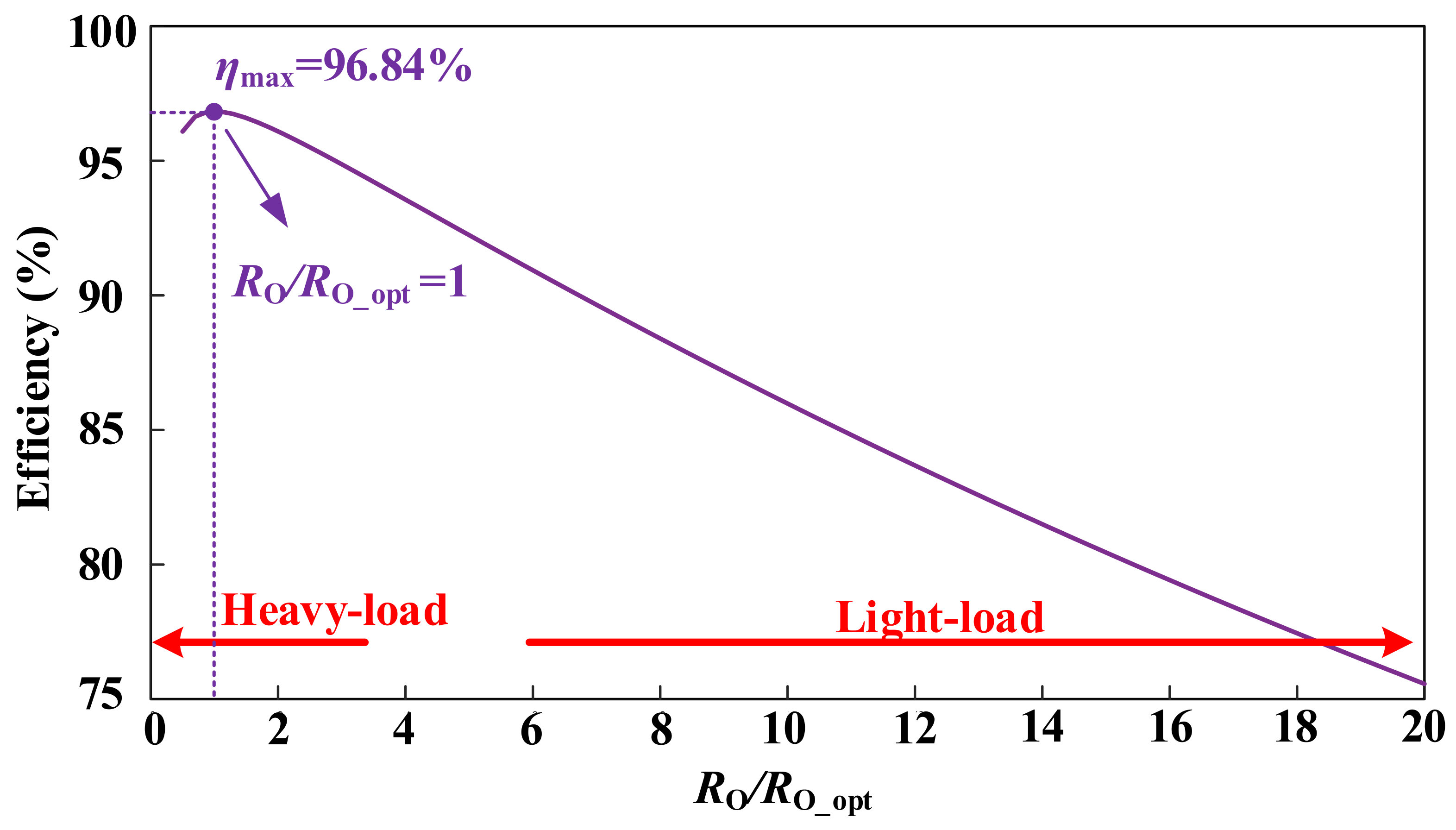
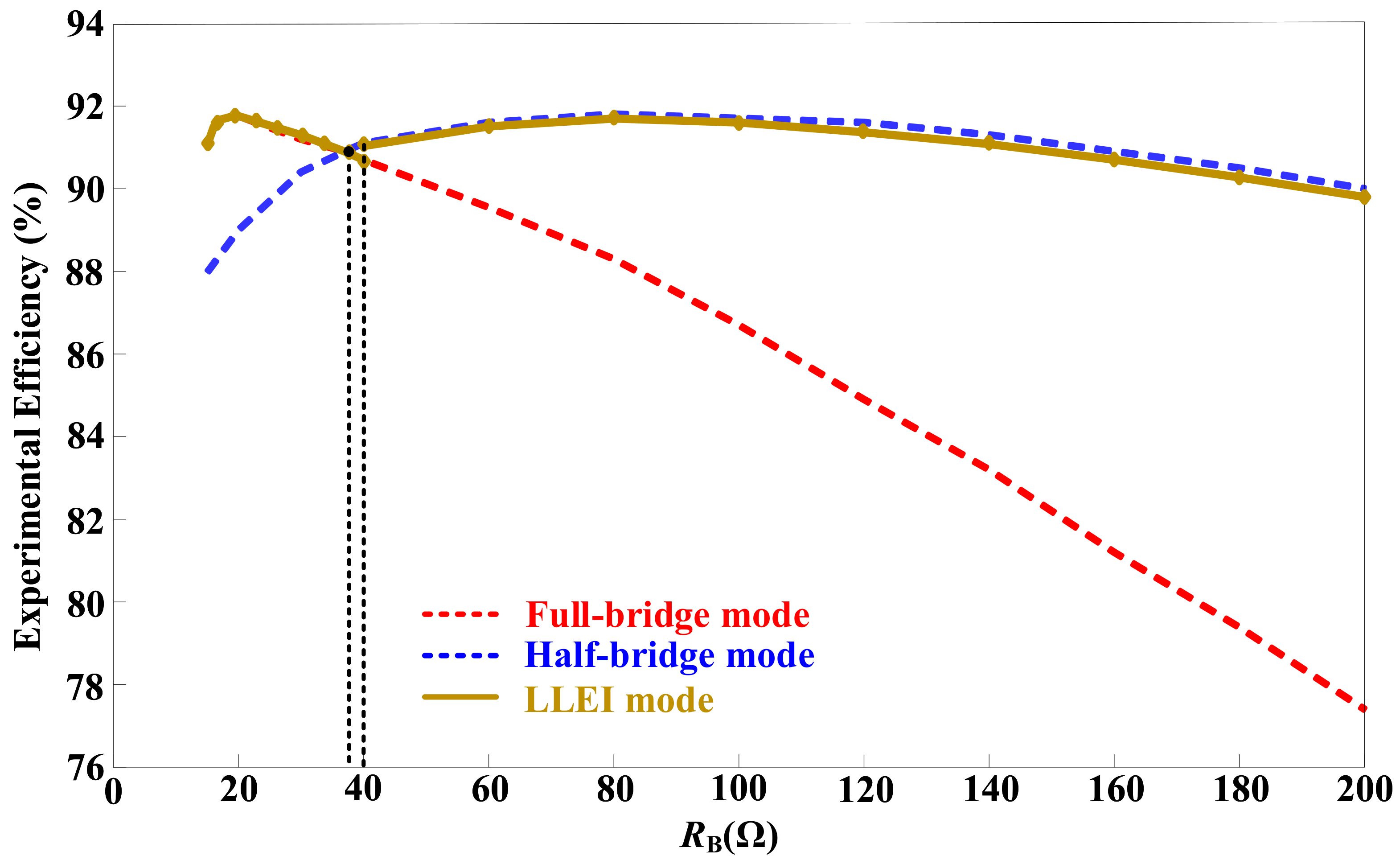
- The IPT system in either half-bridge or full-bridge mode has its respective optimal load resistance to improve efficiency. In addition, the optimal load resistance of the full-bridge mode is a quarter of that of the half-bridge, which validates the previous analysis well.
- (2)
- The system efficiency in either half-bridge or full-bridge mode is significantly affected by the time-varying load resistance. However, under heavy-load condition, efficiency in full-bridge mode is relatively higher, while efficiency in half-bridge mode is relatively higher under light-load conditions.
- (3)
- This LLEI technique selects the optimal parts of the efficiency curves in half-bridge and full-bridge modes, thereby improving overall efficiency.
4. Experimental Validation
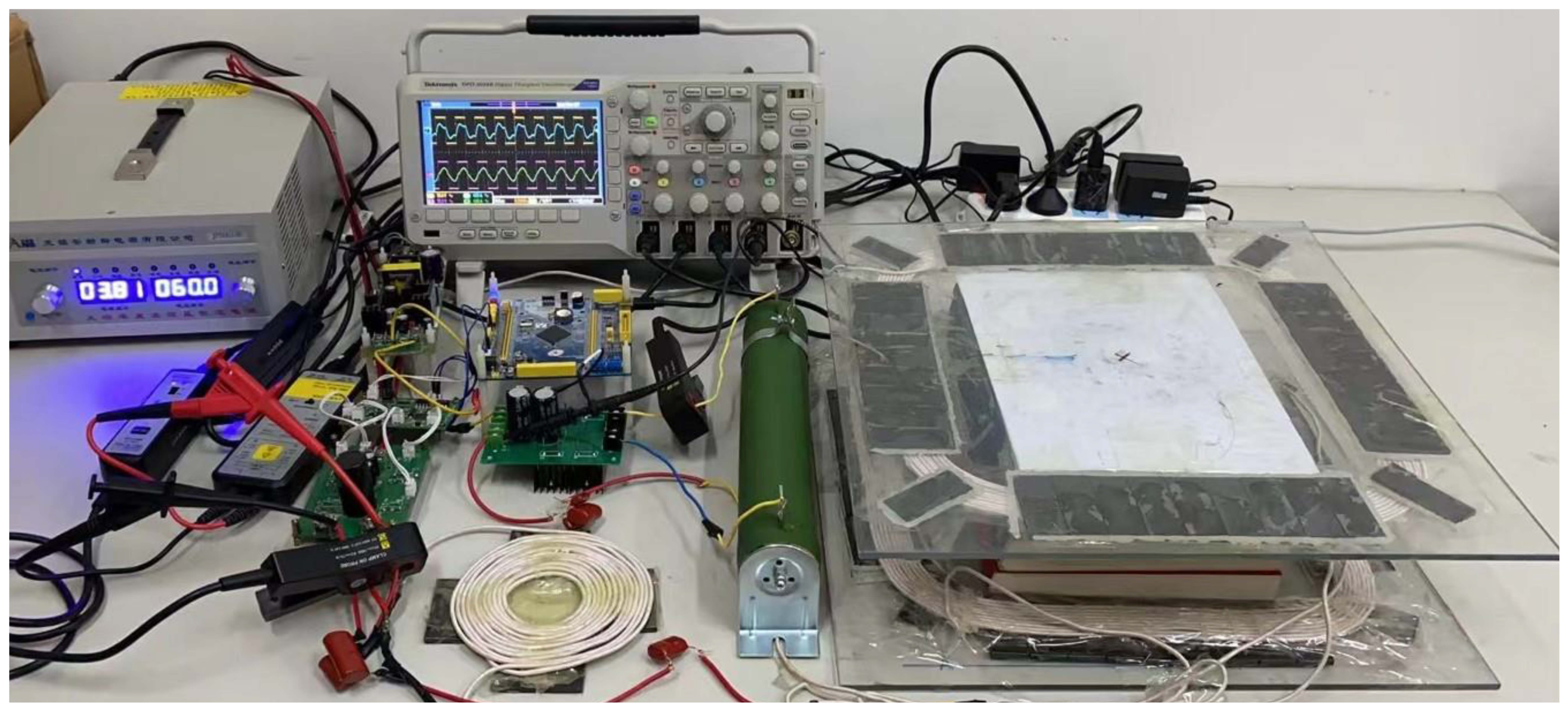
4.1. Experimental Prototype
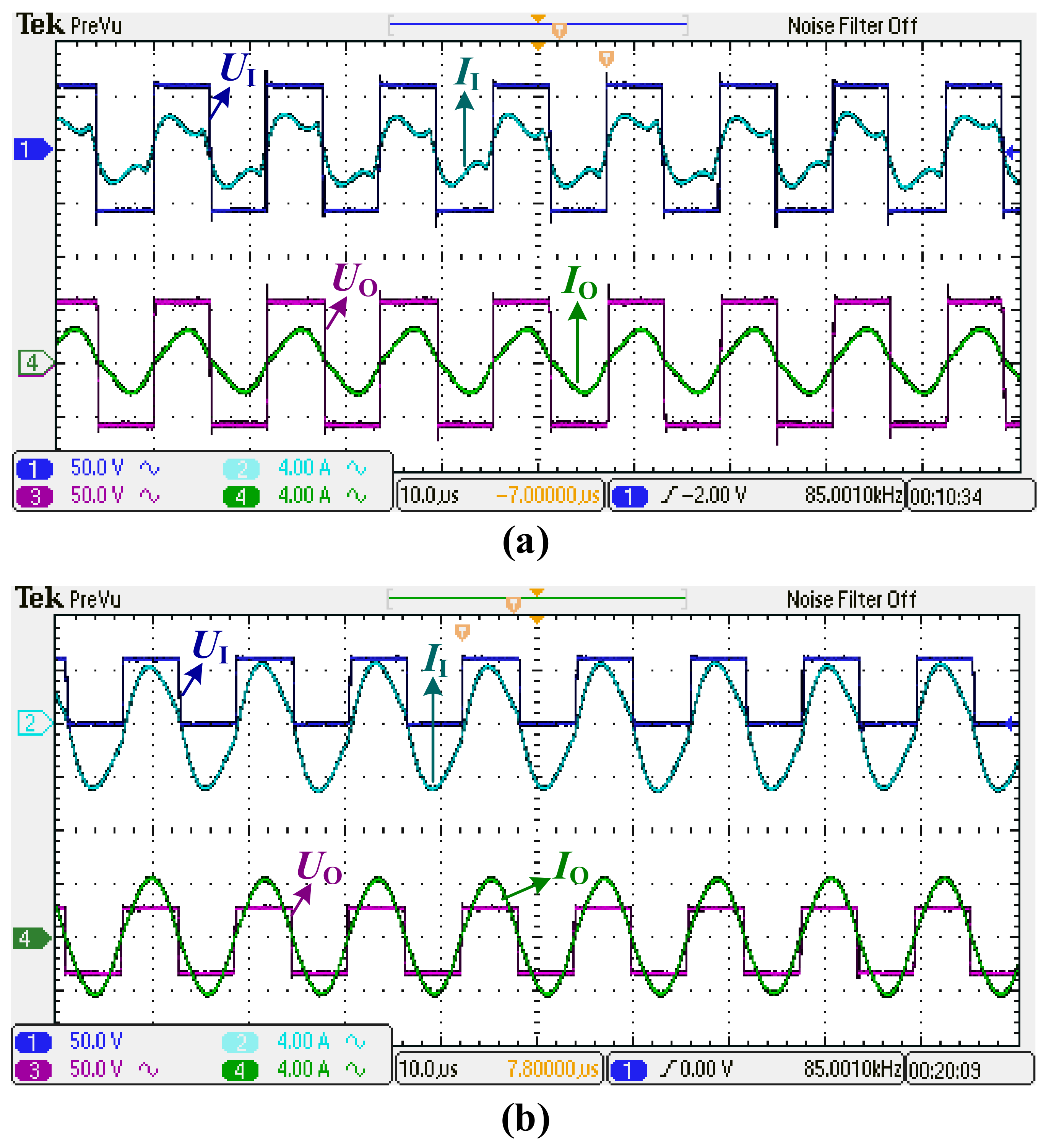
4.2. Experimental Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhang, Y.; Wu, Y.; Jiang, S.; Zhou, M.; Zhou, H.; Mao, X.; Jin, T.; Chen, X. An Integrated Electric Vehicle Charging System of Wireless Power Transfer and Auxiliary Power Module with Shared Converter and Magnetic Coupler. IEEE Trans. Ind. Electron. 2024, 71, 10414–10421. [Google Scholar] [CrossRef]
- Yang, L.; Jiang, S.; Zane, R.A.; Carlson, R. A Compact Dual-Channel WPT System with Power Boosting Function Based on Dual-Sided Decoupling Coils. IEEE J. Emerg. Sel. Top. Power Electron. 2023, 11, 2525–2534. [Google Scholar] [CrossRef]
- Bagchi, A.C.; Kamineni, A.; Wang, C.; Cai, C. Review and Comparative Analysis of Topologies and Control Methods in Dynamic Wireless Charging of Electric Vehicles. IEEE J. Emerg. Sel. Top. Power Electron. 2023, 9, 4947–4962. [Google Scholar] [CrossRef]
- Wu, S.; Cai, C.; Zhang, H.; Liu, X.; Chai, W. A Free-Positioning IPT System via Reconfigurable Coil Array Transmitter for Unmanned Aerial Vehicle Applications. IEEE Trans. Transp. Electrif. 2024. online ahead of pub. [Google Scholar]
- Chen, Z.; Zhang, X.; Xu, F.; Li, M.; Yuan, Z.; Yang, Q. Wide Rotation-Misalignment-Tolerance Design of Magnetic Coupled Structure for AUVs Wireless Charging System. IEEE Trans. Ind. Electron. 2024. online ahead of pub. [Google Scholar]
- Gu, Y.; Wang, J.; Liang, Z.; Zhang, Z. Communication-Free Power Control Algorithm for Drone Wireless In-Flight Charging Under Dual-Disturbance of Mutual Inductance and Load. IEEE Trans. Ind. Inform. 2024, 30, 3703–3714. [Google Scholar] [CrossRef]
- Xiao, C.; Cheng, M.; Wei, K. An LCC-C compensated wireless charging system for implantable cardiac pacemakers: Theory, experiment, and safety evaluation. IEEE Trans. Power Electron. 2018, 33, 4894–4905. [Google Scholar] [CrossRef]
- Liu, C.; Jiang, C.; Song, J.; Chau, K.T. An effective sandwiched wireless power transfer system for charging implantable cardiac pacemaker. IEEE Trans. Ind. Electron. 2019, 66, 4108–4117. [Google Scholar] [CrossRef]
- Feng, J.; Li, Q.; Lee, F.C.; Fu, M. Transmitter Coils Design for Free-Positioning Omnidirectional Wireless Power Transfer System. IEEE Trans. Ind. Inform. 2019, 15, 4656–4664. [Google Scholar] [CrossRef]
- Du, S.; Chan, E.K.; Wen, B.; Hong, J.; Widmer, H.; Wheatley, C.E. Wireless Power Transfer Using Oscillating Magnets. IEEE Trans. Ind. Electron. 2018, 65, 6259–6269. [Google Scholar] [CrossRef]
- Yan, Z.; Song, B.; Zhang, Y.; Zhang, K.; Mao, Z.; Hu, Y. A Rotation-Free Wireless Power Transfer System with Stable Output Power and Efficiency for Autonomous Underwater Vehicles. IEEE Trans. Power Electron. 2019, 34, 4005–4008. [Google Scholar] [CrossRef]
- Rong, E.; Sun, P.; Qiao, K.; Zhang, K.; Yang, G.; Wu, Z. Six-Plate and Hybrid-Dielectric Capacitive Coupler for Underwater Wireless Power Transfer. IEEE Trans. Power Electron. 2024, 39, 2867–2881. [Google Scholar] [CrossRef]
- Cai, C.; Wang, J.; Saeedifard, M.; Zhang, P.; Chen, R.; Zhang, J. Gyrator-Gain Variable WPT Topology for MC-Unconstrained CC Output Customization Using Simplified Capacitance Tuning. IEEE Trans. Ind. Electron. 2024, 71, 3594–3605. [Google Scholar] [CrossRef]
- Cai, C.; Wang, J.; Zhao, Y.; Luo, Y.; Yuan, Z.; Mei, X.; Rao, Y.; Yang, L.; Hong, Y.; Wang, C. Hybrid Interference Field Mitigation of Dual-Rectangular Transmitter Pad for Universal Wireless Charging Area Expansion. IEEE Trans. Transp. Electrif. 2023. online ahead of pub. [Google Scholar]
- Li, H.; Li, J.; Wang, K.; Chen, W.; Yang, X. A Maximum Efficiency Point Tracking Control Scheme for Wireless Power Transfer Systems Using Magnetic Resonant Coupling. IEEE Trans. Power Electron. 2015, 30, 3998–4008. [Google Scholar] [CrossRef]
- Zhong, W.X.; Hui, S.Y.R. Maximum Energy Efficiency Tracking for Wireless Power Transfer Systems. IEEE Trans. Power Electron. 2015, 30, 4025–4034. [Google Scholar] [CrossRef]
- Huang, Z.; Wong, S.; Tse, C.K. Control Design for Optimizing Efficiency in Inductive Power Transfer Systems. IEEE Trans. Power Electron. 2018, 33, 4523–4534. [Google Scholar] [CrossRef]
- Yang, Y.; Zhong, W.; Kiratipongvoot, S.; Tan, S.; Hui, S.Y.R. Dynamic Improvement of Series–Series Compensated Wireless Power Transfer Systems Using Discrete Sliding Mode Control. IEEE Trans. Power Electron. 2018, 33, 6351–6360. [Google Scholar] [CrossRef]
- Diekhans, T.; De Doncker, R.W. A Dual-Side Controlled Inductive Power Transfer System Optimized for Large Coupling Factor Variations and Partial Load. IEEE Trans. Power Electron. 2015, 30, 6320–6328. [Google Scholar] [CrossRef]
- Nguyen, B.X.; Vilathgamuwa, D.M.; Foo, G.H.B.; Wang, P.; Ong, A.; Madawala, U.K.; Nguyen, T.D. An Efficiency Optimization Scheme for Bidirectional Inductive Power Transfer Systems. IEEE Trans. Power Electron. 2015, 30, 6310–6319. [Google Scholar] [CrossRef]
- Karakitsios, I.; Palaiogiannis, F.; Markou, A.; Hatziargyriou, N.D. Optimizing the Energy Transfer, with a High System Efficiency in Dynamic Inductive Charging of EVs. IEEE Trans. Veh. Technol. 2018, 67, 4728–4742. [Google Scholar] [CrossRef]
- Li, H.; Fang, J.; Chen, S.; Wang, K.; Tang, Y. Pulse Density Modulation for Maximum Efficiency Point Tracking of Wireless Power Transfer Systems. IEEE Trans. Power Electron. 2018, 33, 5492–5501. [Google Scholar] [CrossRef]
- Li, Z.; Song, K.; Jiang, J.; Zhu, C. Constant Current Charging and Maximum Efficiency Tracking Control Scheme for Supercapacitor Wireless Charging. IEEE Trans. Power Electron. 2018, 33, 9088–9100. [Google Scholar] [CrossRef]
- Yang, L.; Shi, Y.; Wang, M.; Ren, L. Constant Voltage Charging and Maximum Efficiency Tracking for WPT Systems Employing Dual-Side Control Scheme. IEEE J. Emerg. Sel. Top. Power Electron. 2022, 10, 945–955. [Google Scholar] [CrossRef]
- Zhong, W.; Hui, S.Y.R. Maximum Energy Efficiency Operation of Series-Series Resonant Wireless Power Transfer Systems Using On-Off Keying Modulation. IEEE Trans. Power Electron. 2018, 33, 3595–3603. [Google Scholar] [CrossRef]
- Huang, Z.; Wong, S.; Tse, C.K. An Inductive-Power-Transfer Converter with High Efficiency Throughout Battery-Charging Process. IEEE Trans. Power Electron. 2019, 34, 10245–10255. [Google Scholar] [CrossRef]
- Geng, Y.; Li, B.; Yang, Z. A High Efficiency Charging Strategy for a Supercapacitor Using a Wireless Power Transfer System Based on Inductor/Capacitor/Capacitor (LCC) Compensation Topology. Energies 2017, 10, 135. [Google Scholar] [CrossRef]
- Liu, F.; Chen, K.; Zhao, Z.; Li, K.; Yuan, L. Transmitter-Side Control of Both the CC and CV Modes for the Wireless EV Charging System with the Weak Communication. IEEE J. Emerg. Sel. Top. Power Electron. 2018, 6, 955–965. [Google Scholar] [CrossRef]



| Parameter | Experimental Value | Parameter | Experimental Value |
|---|---|---|---|
| f | 85 kHz | M | 26.5 |
| 100 | 0.20 | ||
| 100 | 0.20 | ||
| 26.5 | 0.05 | ||
| 132.3 | 35.06 | ||
| 47.7 |
| Parameter | Experimental Value | Parameter | Experimental Value |
|---|---|---|---|
| 60 V | 60 V | ||
| f | 85 kHz | M | 26.82 |
| 101.1 | 0.21 | ||
| 100.7 | 0.21 | ||
| 26.61 | 0.06 | ||
| 47.5 | 35.1 | ||
| 133.1 |
| Component | Type |
|---|---|
| MOSFET for HFI | IRFP250N |
| MOSFET for AC switch | IRFP250N |
| Diode for rectifier | MBR16100CT |
| Hall voltage sensor | HVS-AS3.3 |
| Hall current sensor | HCS-ES3.3 |
| Parameter | Specific Information |
|---|---|
| Live wire | 400 stands with diameter of 2.8 mm |
| Transmitter-side coil | Size of 32 cm × 32 cm, 10 turns |
| Receiver-side coil | Size of 32 cm × 32 cm, 10 turns |
| Air-gap | 10 cm |
| Ferrite | PC 40 |
| Proposed in | [15] | [16] | [17] | [18] | [19] | [20] | [21] | [22] | [23] | [24] | [25] | This Work |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Compensation topology | SS | SS | SS | SS | SS | SS | SS | SS | SS | SS | SS | LCC-S |
| Operating frequency (kHz) | 515 | 100 | 200 | 100 | 20 | 48 | 27.9 | 917 | 85.5 | 97.56 | 85.5 | 85 |
| Without DC-DC converters | No | Yes | Yes | Yes | No | Yes | No | Yes | Yes | Yes | No | Yes |
| Complex and tedious calculations | Yes | No | Yes | No | Yes | Yes | Yes | Yes | Yes | No | No | Yes |
| Transmitter-side current/power sensors | Yes | Yes | Yes | Yes | Yes | Yes | Yes | Yes | No | No | Yes | Yes |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, X.; Wang, J.; Yang, L. A Light-Load Efficiency Improvement Technique for an Inductive Power Transfer System through a Reconfigurable Circuit. Energies 2024, 17, 3024. https://doi.org/10.3390/en17123024
Zhou X, Wang J, Yang L. A Light-Load Efficiency Improvement Technique for an Inductive Power Transfer System through a Reconfigurable Circuit. Energies. 2024; 17(12):3024. https://doi.org/10.3390/en17123024
Chicago/Turabian StyleZhou, Xuebin, Jiabin Wang, and Lin Yang. 2024. "A Light-Load Efficiency Improvement Technique for an Inductive Power Transfer System through a Reconfigurable Circuit" Energies 17, no. 12: 3024. https://doi.org/10.3390/en17123024
APA StyleZhou, X., Wang, J., & Yang, L. (2024). A Light-Load Efficiency Improvement Technique for an Inductive Power Transfer System through a Reconfigurable Circuit. Energies, 17(12), 3024. https://doi.org/10.3390/en17123024







