Quantitative Analysis of Fracture Roughness and Multi-Field Effects for CO2-ECBM Projects
Abstract
1. Introduction
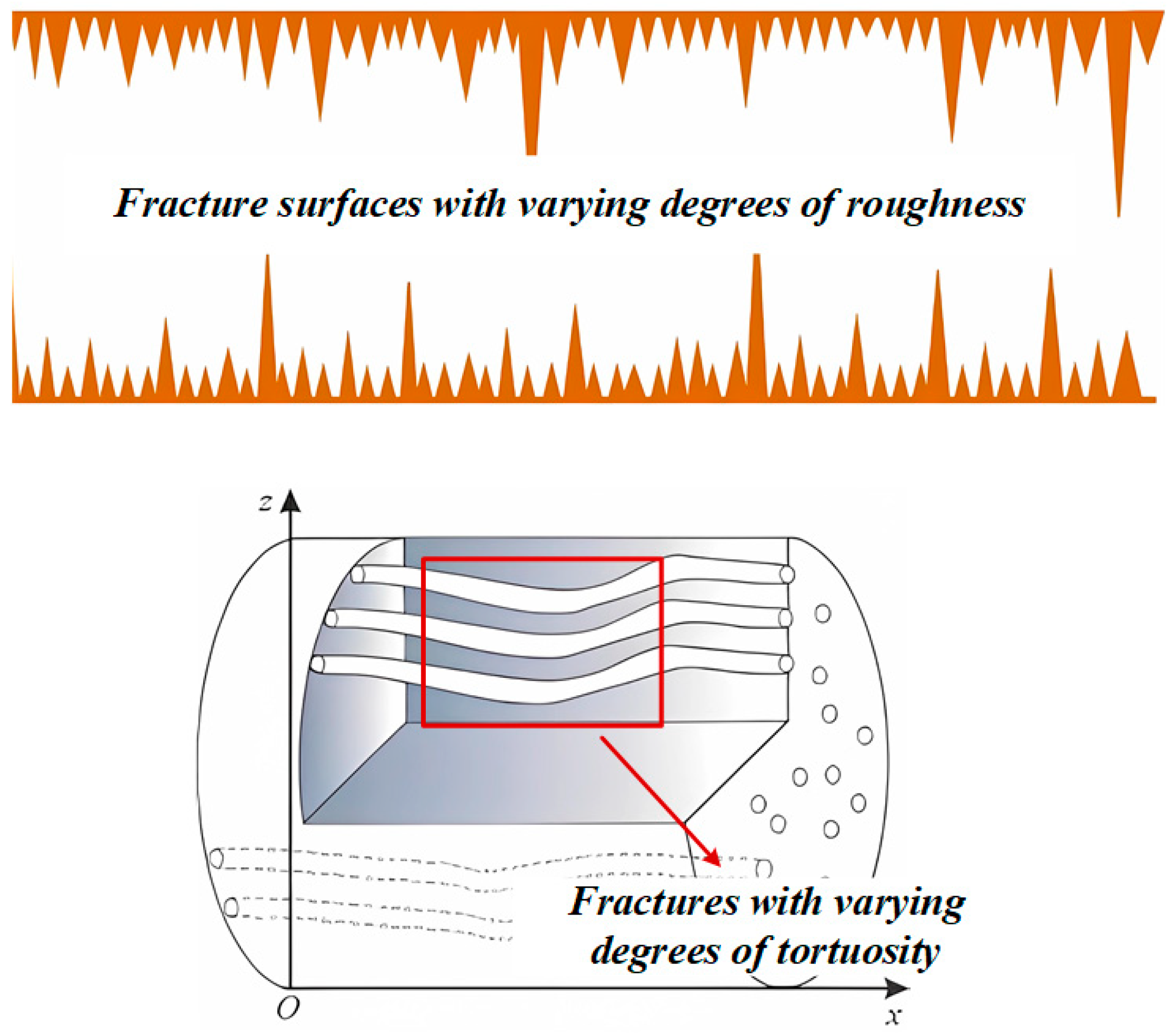
2. Conceptual Model
3. Governing Equations
3.1. Permeability and Porosity Models Considering Fracture Roughness Evolution in CO2-ECBM Projects
3.2. The Gas Flow Model in CO2-ECBM Projects
3.3. The Thermal Conduction Model in CO2-ECBM Projects
3.4. The Coalbed Deformation Model
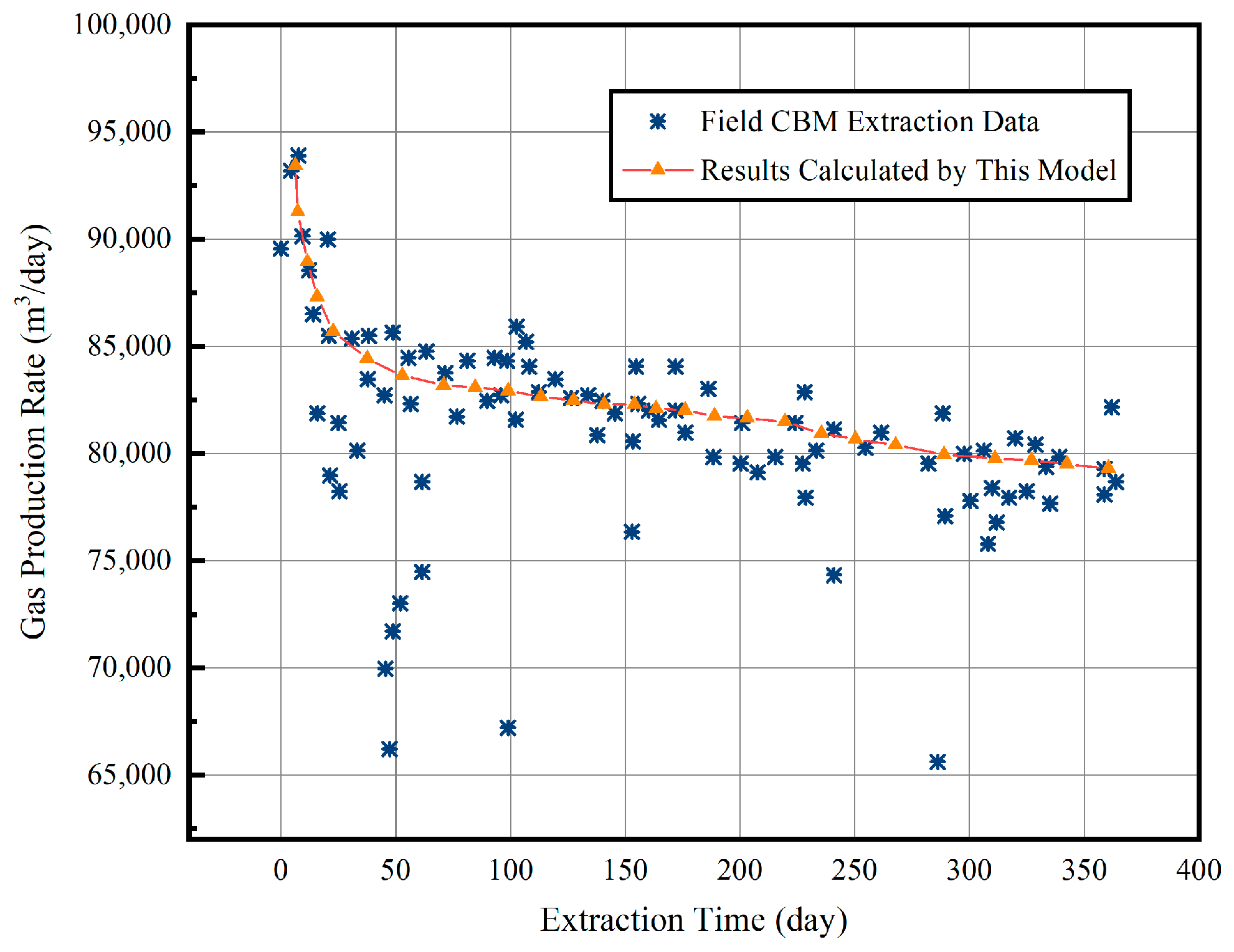
4. The Verification of Model Correctness and Advantages
5. Results and Discussion
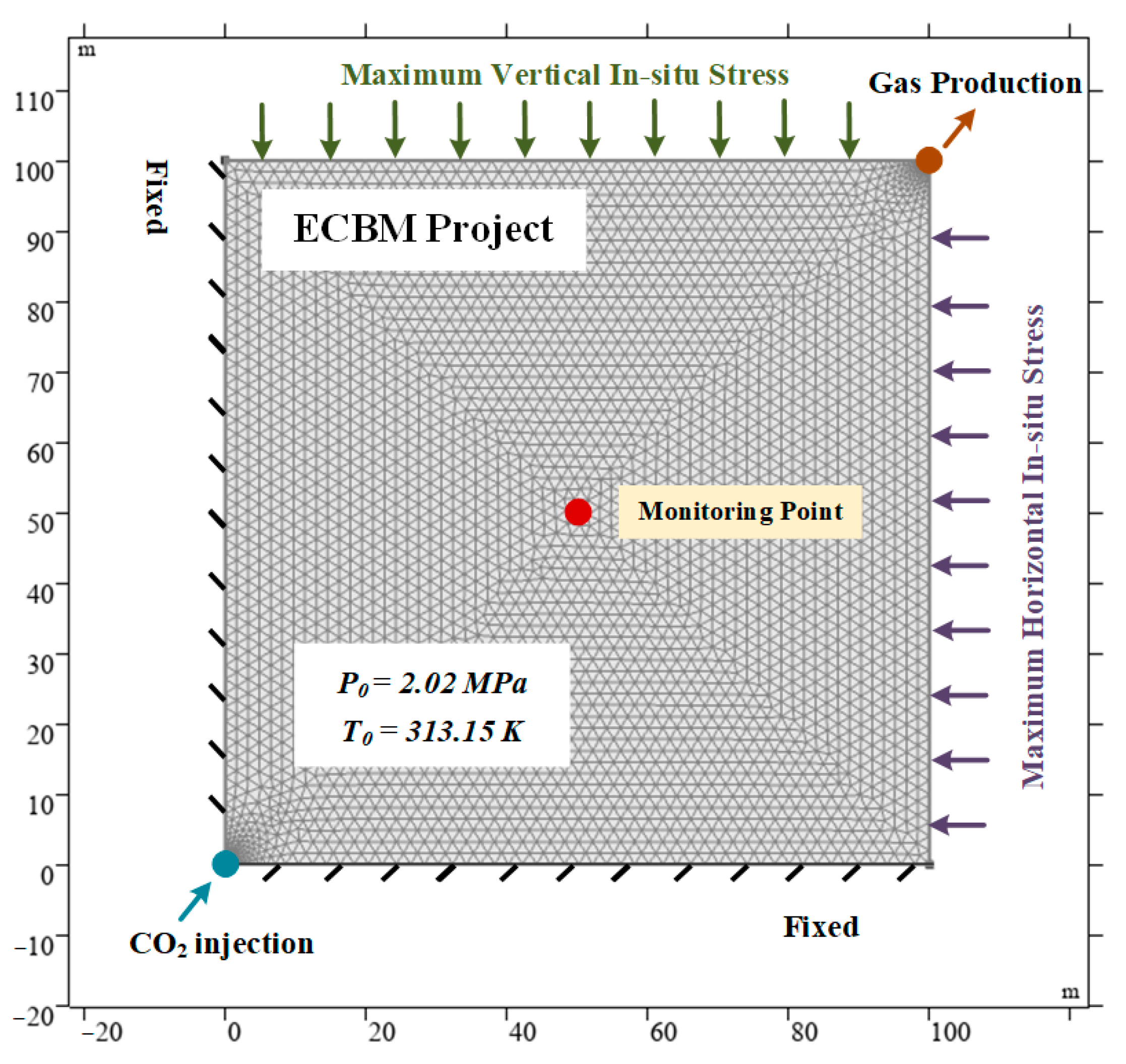
5.1. The Numerical Model for the Field CO2-ECBM Project
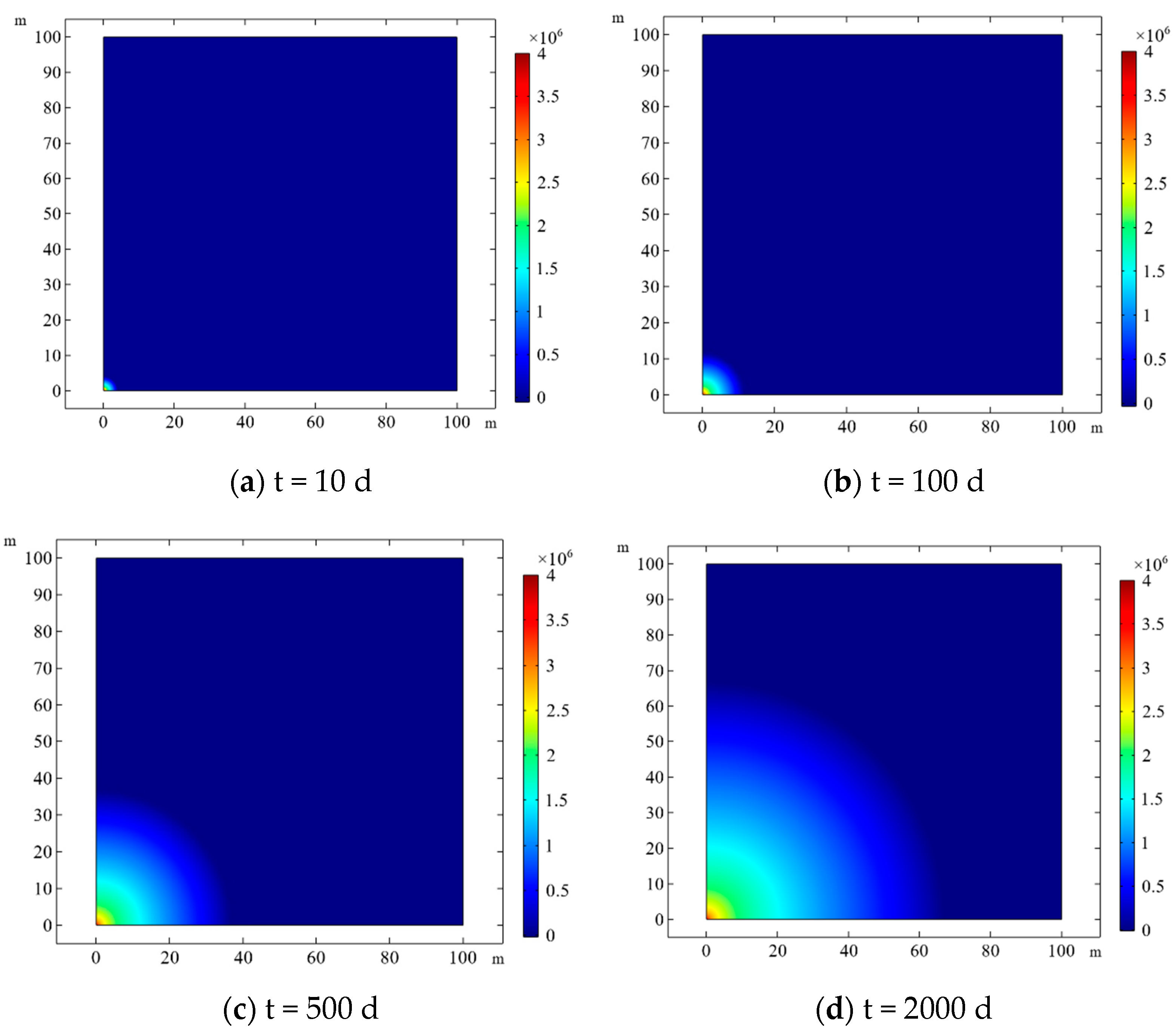
5.2. The Simulation Results of the Field CO2-ECBM Project
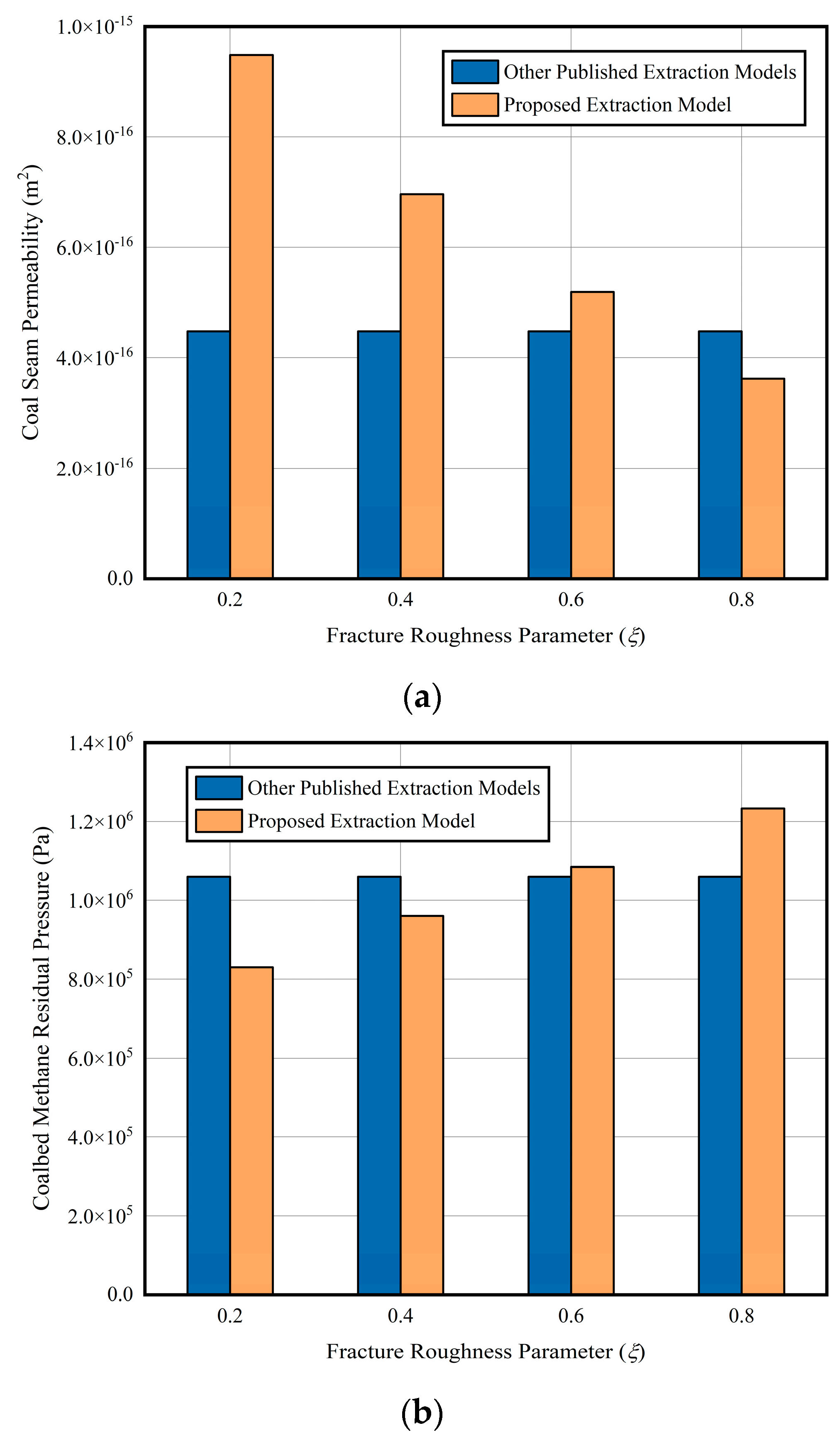
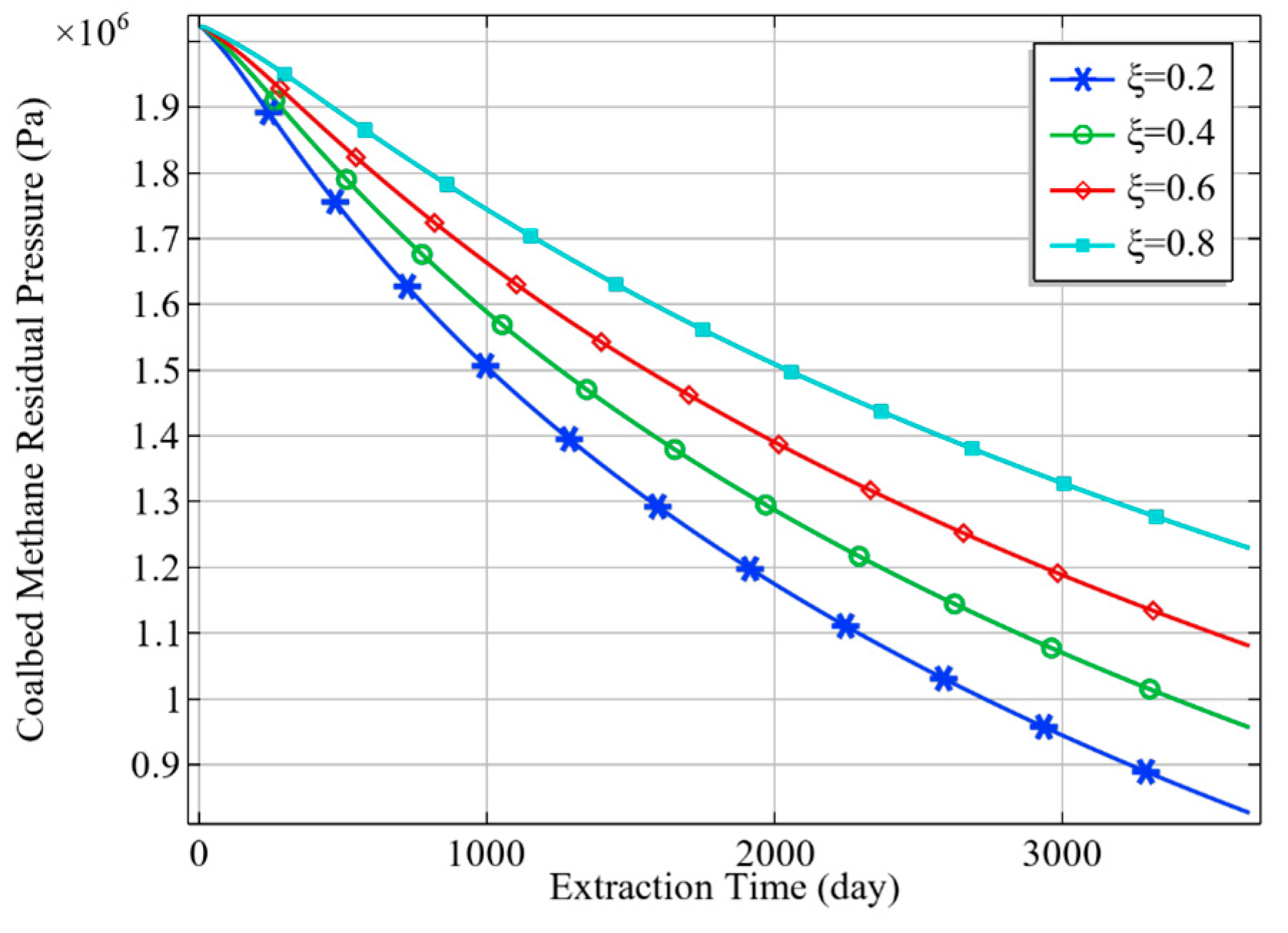
5.3. Quantitative Investigation for the Impact of Fracture Roughness on CO2-ECBM Projects
6. Conclusions
- The model accurately and quantitatively characterizes the contribution of fracture roughness in CO2-ECBM engineering, showing significant interactions between the two gas pressures, their adsorption strengths, and the coal layer’s permeability, all correlated with ξ.
- The quantitative parameter ξ for fracture roughness substantially influences coal layer porosity and permeability. At identical extraction times, a smaller ξ results in higher coal layer permeability and porosity.
- The adsorption strength of CO2 and CH4 is highly sensitive to ξ. Changes in fracture roughness alter the pressures of both gases in the coal layer, as well as altering their adsorption quantities significantly. When ξ decreases from 0.8 to 0.2, CO2 adsorption increases by 20.8%, and the adsorbed CH4 content increases by 24.6% after 3000 days of extraction.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Xue, H.T.; Gui, X.H.; Wang, G.D.; Yang, X.; Gong, H.R.; Du, F. Prediction of gas drainage changes from nitrogen replacement: A study of a TCN deep learning model with integrated attention mechanism. Fuel 2024, 357, 129797. [Google Scholar] [CrossRef]
- Ye, D.Y.; Liu, G.N.; Zou, X.; Yang, Y.G.; Wang, F.T.; Gao, F. A Dual Fractal Approach to Thermal-Hydrological-Mechanical Interactions of Unconventional Reservoir. Rock Mech. Rock Eng. 2022, 55, 7081–7101. [Google Scholar] [CrossRef]
- Liang, S.; Liang, Y.; Elsworth, D.; Yao, Q.; Fu, X.; Kang, J.; Hao, Y.; Wang, M. Permeability evolution and production characteristics of inclined coalbed methane reservoirs on the southern margin of the Junggar Basin, Xinjiang, China. Int. J. Rock Mech. Min. Sci. 2023, 171, 105581. [Google Scholar] [CrossRef]
- Shi, Y.; Lin, B.; Liu, T.; Zhao, Y.; Hao, Z. Synergistic ECBM extraction technology and engineering application based on hydraulic flushing combing gas injection displacement in low permeability coal seams. Fuel 2022, 318, 123688. [Google Scholar] [CrossRef]
- Mukherjee, M.; Misra, S. A review of experimental research on Enhanced Coal Bed Methane (ECBM) recovery via CO2 sequestration. Earth-Sci. Rev. 2018, 179, 392–410. [Google Scholar] [CrossRef]
- Faltinson, J.; Gunter, B. Integrated Economic Model CO2 capture, transport, ECBM and saline aquifer storage. Energy Procedia 2009, 1, 4001–4005. [Google Scholar] [CrossRef][Green Version]
- Fang, H.; Sang, S.; Wang, Z.; Guo, J.; Liu, H.; Xu, H.; Kang, J.; Hao, Y.; Wang, M. Numerical analysis of temperature effect on CO2 storage capacity and CH4 production capacity during the CO2-ECBM process. Energy 2023, 171, 130022. [Google Scholar] [CrossRef]
- Lin, J.; Ren, T.; Wang, G.; Booth, P.; Nemcik, J. Experimental study of the adsorption-induced coal matrix swelling and its impact on ECBM. J. Earth Sci. 2017, 28, 917–925. [Google Scholar] [CrossRef]
- Ye, D.Y.; Liu, G.N.; Wang, F.T.; Gao, F.; Yang, T.T.; Zhu, J.Y. Fractal hydrological-thermal-mechanical analysis of unconventional reservoir: A fracture-matrix structure model for gas extraction. Int. J. Heat Mass Transf. 2023, 202, 123670. [Google Scholar] [CrossRef]
- Asif, M.; Wang, L.; Naveen, P.; Longinos, S.N.; Hazlett, R.; Ojha, K.; Panigrahi, D.C. Influence of competitive adsorption, diffusion, and dispersion of CH4 and CO2 gases during the CO2-ECBM process. Fuel 2024, 358, 130065. [Google Scholar] [CrossRef]
- Fang, H.; Yu, S.; Zhang, S.; Sang, S.; Guo, J.; Wang, Z.; Wang, X. Coupling Mechanism of THMC Fields in Crushed Soft Coal with Low Permeability after CO2 Injection and Its Application in CO2-ECBM Technology. Energy Fuels 2024, 38, 6891–6911. [Google Scholar] [CrossRef]
- Zheng, S.; Yao, Y.; Sang, S.; Liu, D.; Wang, M.; Liu, S. Dynamic characterization of multiphase methane during CO2-ECBM: An NMR relaxation method. Fuel 2022, 324, 124526. [Google Scholar] [CrossRef]
- Niu, Q.; Cao, L.; Sang, S.; Wang, W.; Zhou, X.; Yuan, W.; Ji, Z.; Chang, J.; Li, M. Experimental study on the softening effect and mechanism of anthracite with CO2 injection. Int. J. Rock Mech. Min. Sci. 2021, 138, 104614. [Google Scholar] [CrossRef]
- Fang, H.; Xu, H.; Sang, S.; Liu, S.; Song, S.; Liu, H. 3D reconstruction of coal pore network and its application in CO2-ECBM process simulation at laboratory scale. Front. Earth Sci. 2022, 16, 523–539. [Google Scholar] [CrossRef]
- Liu, X.; Sang, S.; Zhou, X.; Wang, Z.; Niu, Q.; Mondal, D. Modelling of geomechanical response for coal and ground induced by CO2-ECBM recovery. Gas Sci. Eng. 2023, 113, 204953. [Google Scholar] [CrossRef]
- Talapatra, A. A study on the carbon dioxide injection into coal seam aiming at enhancing coal bed methane (ECBM) recovery. J. Pet. Explor. Prod. Technol. 2020, 10, 1965–1981. [Google Scholar] [CrossRef]
- Liu, Z.D.; Chen, X.H.; Tian, F.C.; Zhu, W.C.; Hu, Z.; Su, W.W.; Wang, G. Dynamic effects of rough elements in fractures on coal permeability during different stages of methane extraction. Gas Sci. Eng. 2023, 119, 205118. [Google Scholar] [CrossRef]
- Najafi, M.; Abousadi, Z.A.; Honarvar, B.; Sajadian, S.A. Publisher Correction: Separation of hydrocarbons from activated carbon as a porous substance in a glycol regeneration process using supercritical carbon dioxide. Sci. Rep. 2022, 12, 21607. [Google Scholar] [CrossRef] [PubMed]
- Pan, J.; Du, X.; Wang, X.; Hou, Q.; Wang, Z.; Yi, J.; Li, M. Pore and permeability changes in coal induced by true triaxial supercritical carbon dioxide fracturing based on low-field nuclear magnetic resonance. Energy 2024, 286, 129492. [Google Scholar] [CrossRef]
- Hol, S.; Gensterblum, Y.; Massarotto, P. Sorption and changes in bulk modulus of coal—experimental evidence and governing mechanisms for CBM and ECBM applications. Int. J. Coal Geol. 2014, 128, 119–133. [Google Scholar] [CrossRef]
- Tang, S.L.; Liu, Q.; Tang, H.; Yang, F. Study on the Movement of Pulverized Coal Particles in Fractal Fracture Network. ACS Omega 2023, 8, 26369–26378. [Google Scholar] [CrossRef] [PubMed]
- Guo, H.J.; Tang, H.L.; Wu, Y.C.; Wang, K.; Xu, C. Gas seepage in underground coal seams: Application of the equivalent scale of coal matrix-fracture structures in coal permeability measurements. Fuel 2021, 288, 119641. [Google Scholar] [CrossRef]
- Jing, Y.; Armstrong, R.T.; Mostaghimi, P. Digital coal: Generation of fractured cores with microscale features. Fuel 2017, 207, 93–101. [Google Scholar] [CrossRef]
- Li, Y.T.; Zhao, Y.X.; Jiang, Y.D.; Zhang, B.; Song, H.H.; Liu, B. Characteristics of Pore and Fracture of Coal with Bursting Proneness Based on DIC and Fractal Theory. Energies 2020, 13, 5404. [Google Scholar] [CrossRef]
- Ye, D.Y.; Liu, G.N.; Zhou, J.H.; Yang, T.T.; Cheng, G.J.; Fan, S.D. A fractal power-law approach for investigating the interactions between reservoir microstructure and gas migration. Phys. Fluids 2023, 35, 016604. [Google Scholar] [CrossRef]
- Ye, D.; Liu, G.; Ma, T.; Cheng, G.; Fan, S.; Yang, T. The mechanics of frost heave with stratigraphic microstructure evolution. Eng. Geol. 2023, 319, 107119. [Google Scholar] [CrossRef]
- Li, L.; Zhang, S.F.; Li, Z.Q.; Chen, X.J.; Wang, L.; Feng, S.L. An Experimental and Numerical Study of Abrupt Changes in Coal Permeability with Gas Flowing through Fracture-Pore Structure. Energies 2022, 15, 7842. [Google Scholar] [CrossRef]
- Liu, X.X.; Sheng, J.C.; Liu, J.S.; Hu, Y.J. Evolution of Coal Permeability during Gas InjectionFrom Initial to Ultimate Equilibrium. Energies 2018, 11, 2800. [Google Scholar] [CrossRef]
- Morga, R. Changes of semifusinite and fusinite surface roughness during heat treatment determined by atomic force microscopy. Int. J. Coal Geol. 2011, 88, 218–226. [Google Scholar] [CrossRef]
- Xia, W.C.; Li, Y.F. Role of Roughness Change on Wettability of Taixi Anthracite Coal Surface before and after the Heating Process. Energy Fuels 2016, 30, 281–284. [Google Scholar] [CrossRef]
- Chen, Y.R.; Xia, W.C.; Xie, G.Y. Contact angle and induction time of air bubble on flat coal surface of different roughness. Fuel 2018, 222, 35–41. [Google Scholar] [CrossRef]
- Xia, T.Q.; Zhou, F.B.; Gao, F.; Kang, J.H.; Liu, J.S.; Wang, J.G. Simulation of coal self-heating processes in underground methane-rich coal seams. Int. J. Coal Geol. 2015, 141–142, 1–12. [Google Scholar] [CrossRef]
- Fang, H.H.; Sang, S.X.; Liu, S.Q. Establishment of dynamic permeability model of coal reservoir and its numerical simulation during the CO2-ECBM process. J. Pet. Sci. Eng. 2019, 179, 885–898. [Google Scholar] [CrossRef]
- Kong, X.G.; Wang, E.Y.; Liu, Q.L.; Li, Z.H.; Li, D.X.; Cao, Z.Y.; Niu, Y. Dynamic permeability and porosity evolution of coal seam rich in CBM based on the flow-solid coupling theory. J. Nat. Gas Sci. Eng. 2017, 40, 61–71. [Google Scholar] [CrossRef]
- Zhang, X.; Shi, W.; Hu, Q.; Zhai, G.; Wang, R.; Xu, X.; Xu, Z.; Meng, F.; Liu, F. Pressure–dependent fracture permeability of marine shales in the Northeast Yunnan area, Southern China. Int. J. Coal Geol. 2019, 214, 103237. [Google Scholar] [CrossRef]
- Ye, D.; Lin, X.; Yang, X.; Li, X.; Zhao, R.; Ma, H. How to quantitatively investigate the effect of fracture roughness on gas flow: A new fully coupled interdisciplinary model. Phys. Fluids 2023, 35, 126610. [Google Scholar] [CrossRef]
- Yang, H.; Wang, L.; Yang, C.; Guo, I.; Guo, W.; Bi, Z.; Zhao, G. Visualization and quantitative statistics of experimental hydraulic fracture network based on optical scanning. J. Nat. Gas Sci. Eng. 2022, 105, 104718. [Google Scholar] [CrossRef]
- Li, W.; Frash, L.P.; Welch, N.J.; Carey, W.I.; Meng, M.; Wigand, M. Stress-dependent fracture permeability measurements and implications for shale gas production. Fuel 2021, 290, 119984. [Google Scholar] [CrossRef]
- Guo, P.; Li, X.; Li, S.; Yang, W. Quantitative analysis of anisotropy effect on hydrofracturing efficiency and process in shale using X-ray computed tomography and acoustic emission. Rock Mech. Rock Eng. 2021, 54, 5715–5730. [Google Scholar] [CrossRef]
- Fan, J.; Feng, R.; Wang, J.; Wang, Y. Laboratory investigation of coal deformation behavior and its influence on permeability evolution during methane displacement by CO2. Rock Mech. Rock Eng. 2017, 50, 1725–1737. [Google Scholar] [CrossRef]
- Mora Carlos, A.; Wattenbarger, R.A.; McKetta, S. Comparison of computation methods for CBM performance. In Proceedings of the Canadian International Petroleum Conference, Calgary, AB, Canada, 12–14 June 2007. [Google Scholar]
- Fang, H.; Sang, S.; Liu, S. The coupling mechanism of the thermal-hydraulic-mechanical fields in CH4-bearing coal and its application in the CO2-enhanced coalbed methane recovery. J. Pet. Sci. Eng. 2019, 181, 106177. [Google Scholar] [CrossRef]










| Parameter, Unit | Value |
|---|---|
| Initial Coal Temperature, K | 294.26 |
| CBM Langmuir Volume Constant, m3/kg | 0.0256 |
| CO2 Langmuir Volume Constant, m3/kg | 0.0477 |
| CBM Langmuir Volumetric Strain Constant | 0.0128 |
| CO2 Langmuir Volumetric Strain Constant | 0.0237 |
| Young’s Modulus for Coal, MPa | 2713 |
| Young’s Modulus for Coal Solid, MPa | 4070 |
| Poisson Ratio | 0.34 |
| Coal Thermal Expansion Coefficient, K−1 | 2.4 × 10−5 |
| Coal Density, kg/m3 | 1.52 × 103 |
| CBM Density at Standard Condition, kg/m3 | 0.717 × 103 |
| CO2 Density at Standard Condition, kg/m3 | 1.977 × 103 |
| Coal Specific Heat Capacity, J/(K·kg) | 1255 |
| CO2 Specific Heat Capacity, J/(K·kg) | 1250 |
| CBM Specific Heat Capacity, J/(K·kg) | 2227 |
| CO2 Dynamic Viscosity, Pa·s | 1.38 × 10−5 |
| CBM Dynamic Viscosity, Pa·s | 1.84 × 10−5 |
| Parameter, Unit | Value |
|---|---|
| Initial Coal Temperature, K | 313.15 |
| Initial Coal Pressure, MPa | 2.02 |
| Fracture Roughness | 0.17–0.97 |
| Initial Coal Porosity | 0.0423 |
| Initial Coal Permeability, m2 | 5.14 × 10−16 |
| CBM Langmuir Volume Constant, m3/kg | 0.0256 |
| CO2 Langmuir Volume Constant, m3/kg | 0.0477 |
| CBM Langmuir Volumetric Strain Constant | 0.0128 |
| CO2 Langmuir Volumetric Strain Constant | 0.0237 |
| Young’s Modulus for Coal, MPa | 2710 |
| Young’s Modulus for Coal Solid, MPa | 8134 |
| Poisson Ratio | 0.345 |
| Coal Thermal Expansion Coefficient, K−1 | 2.4 × 10−5 |
| Coal Density, kg/m3 | 1.25 × 103 |
| Coal Solid Density, kg/m3 | 1.47 × 103 |
| Universal Gas Constant, J/(K·mol) | 8.314 |
| CBM Density at Standard Condition, kg/m3 | 0.717 × 103 |
| CO2 Density at Standard Condition, kg/m3 | 1.977 × 103 |
| Coal Specific Heat Capacity, J/(K·kg) | 1255 |
| CO2 Specific Heat Capacity, J/(K·kg) | 1250 |
| CBM Specific Heat Capacity, J/(K·kg) | 2227 |
| CO2 Dynamic Viscosity, Pa·s | 1.38 × 10−5 |
| CBM Dynamic Viscosity, Pa·s | 1.03 × 10−5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, L.; Shan, Y. Quantitative Analysis of Fracture Roughness and Multi-Field Effects for CO2-ECBM Projects. Energies 2024, 17, 2851. https://doi.org/10.3390/en17122851
Zhang L, Shan Y. Quantitative Analysis of Fracture Roughness and Multi-Field Effects for CO2-ECBM Projects. Energies. 2024; 17(12):2851. https://doi.org/10.3390/en17122851
Chicago/Turabian StyleZhang, Lingshuo, and Yafei Shan. 2024. "Quantitative Analysis of Fracture Roughness and Multi-Field Effects for CO2-ECBM Projects" Energies 17, no. 12: 2851. https://doi.org/10.3390/en17122851
APA StyleZhang, L., & Shan, Y. (2024). Quantitative Analysis of Fracture Roughness and Multi-Field Effects for CO2-ECBM Projects. Energies, 17(12), 2851. https://doi.org/10.3390/en17122851





