Maximizing Solar Share in Robust System Spinning Reserve-Constrained Economic Operation of Hybrid Power Systems
Abstract
1. Introduction
- (i)
- What are the limits of the robust SSR as well as of the corresponding RE share?
- (ii)
- To what level can the RE share be increased such that the allocated SSR lies within the robust range and is dedicated for thermal contingencies only?
- (iii)
- How much can the RE power share be supported by a set of committed thermal units under the robust SSR if is supposed to be dispatched for RE as well as thermal outages?
- (iv)
- What is the value of the maximum SSR that can be achieved with a set of committed thermal units?
- (i)
- The solar share is maximized within the boundaries of a robust SSR such that the power deficit does not exist.
- (ii)
- The range of a robust SSR is determined that can accommodate an additional RE share provided that compromise on the power deficit is possible.
- (iii)
- This study minimizes the operational cost and maximizes the number of solar plants with an ON status in order to enhance the solar power availability.
- (iv)
- The proposed model was implemented and simulated on an IEEE-RTS 26-unit system.
- (v)
- The ORCUC was carried out using LR, and ED was executed through a Lambda iteration and using the binary search method [2]. Furthermore, a set of Pareto-optimal solutions was found for the selection of solar plants.
2. System Model and Problem Formulation
2.1. System Model
2.2. Problem Formulation
- Answer (i)
- The answer to question (i) defines the overall structure of the model in terms of a robust SSR and RE share.
- Answer (ii)
- This answer evaluates the RE share and range of a robust SSR for the scenario if the is dedicated for thermal contingencies. The evaluated RE share should be limited to make the system resilient to any RE outages.
- Answer (iii)
- This answer evaluates the RE share and the limits of a robust SSR for an undedicated scenario.
- Answer (iv)
- This answer evaluates the overall SSR, whether robust or non-robust, and the corresponding RE share.
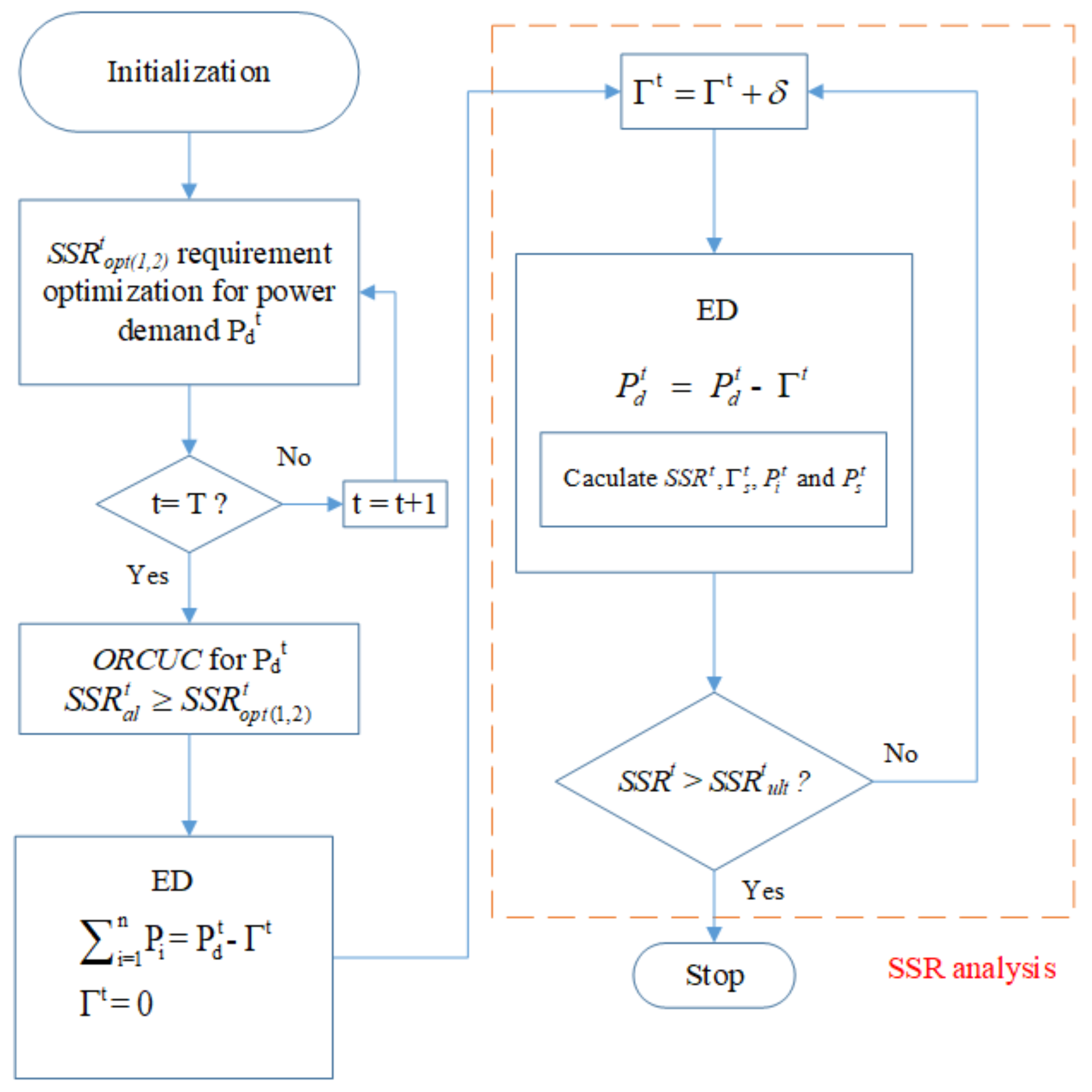
3. Proposed Solution
3.1. Sub-Problem I
- (i)
- Set = 0 and carry out ORCUC via LR to minimize the scheduling cost and to allocate the SSR, i.e., . The Lagrangian function is formulated aswhere and are assigned as non-negative Lagrangian multipliers to coupling constraints (8) and (16). Power loss is ignored for simplicity in the power balance constraint. The LR method temporarily relaxes the coupling constraints, and then via dual optimization, the Lagrangian function is maximized as a function of Lagrangian multiplier and while minimizing as a function of control variables and ; that is,whereThe Lagrangian in Equation (36) can be rewritten asThe first term of Equation (39), i.e., , can be minimized separately for each thermal generation unit, whereas the second term of the equation is constant and can be dropped. Thus, the simplified problem is given by
- (ii)
- Once the units are committed, ED optimization is carried out to calculate optimal powers of the committed generation units using the binary search lambda iteration algorithm [2]. In this algorithm, the optimal power output of each unit is found on the basis of an incremental cost rate ().If , then , andif , then .Binary search proceeds as follows:The following conditions are verified, and is updated as follows:If , thenand .If , thenand , andIf tolerance, the algorithm is terminated.
- (iii)
- For a set of committed thermal units, problem (34) is solved to maximize the solar share within the range of a robust SSR. To solve this problem, the SSR ranges must be defined, and as well as the solar share limit must be evaluated for each range. Thus, to answer the questions posed in the Introduction section, the data sets , , and are generated to define the SSR ranges for the answers (ii), (iii), and (iv), respectively. The solar share is initialized with a value equal to zero and increased iteratively with step size . For each iteration, ED is carried out to evaluate , , and . The resulting evaluations of are allocated to either of the previously defined ranges based on the following criteriaThe process is repeated until convergence. For any total number of iterations, say Y, the data sets are given bywhere D, S, and C are the maximum number of iterations for each range, respectively. The maximum limits of the robust SSR range and solar share are found when is dedicated to thermal contingencies only, i.e., the outcomes of answer (ii) are as follows:where is the index of the maximum value in , i.e.,Furthermore, the SSR for solar outage, i.e., , can be evaluated simply by subtracting the from . Similarly, the evaluations corresponding to answers (iii) and (iv) are given bywhere and are the indices of the maximum values in and , respectively, i.e.,In this way, the problem is solved to evaluate the robust SSR, and the procedure is called SSR analysis. The procedural flow of the solution of problem (34) is shown in Figure 2, in which the highlighted portion shows the SSR analysis. For further clarifications, a graphical illustration of the SSR analysis will be discussed in detail in Section 4.
3.2. Sub-Problem II
4. Test System and Simulation Results
5. Conclusions
- (i)
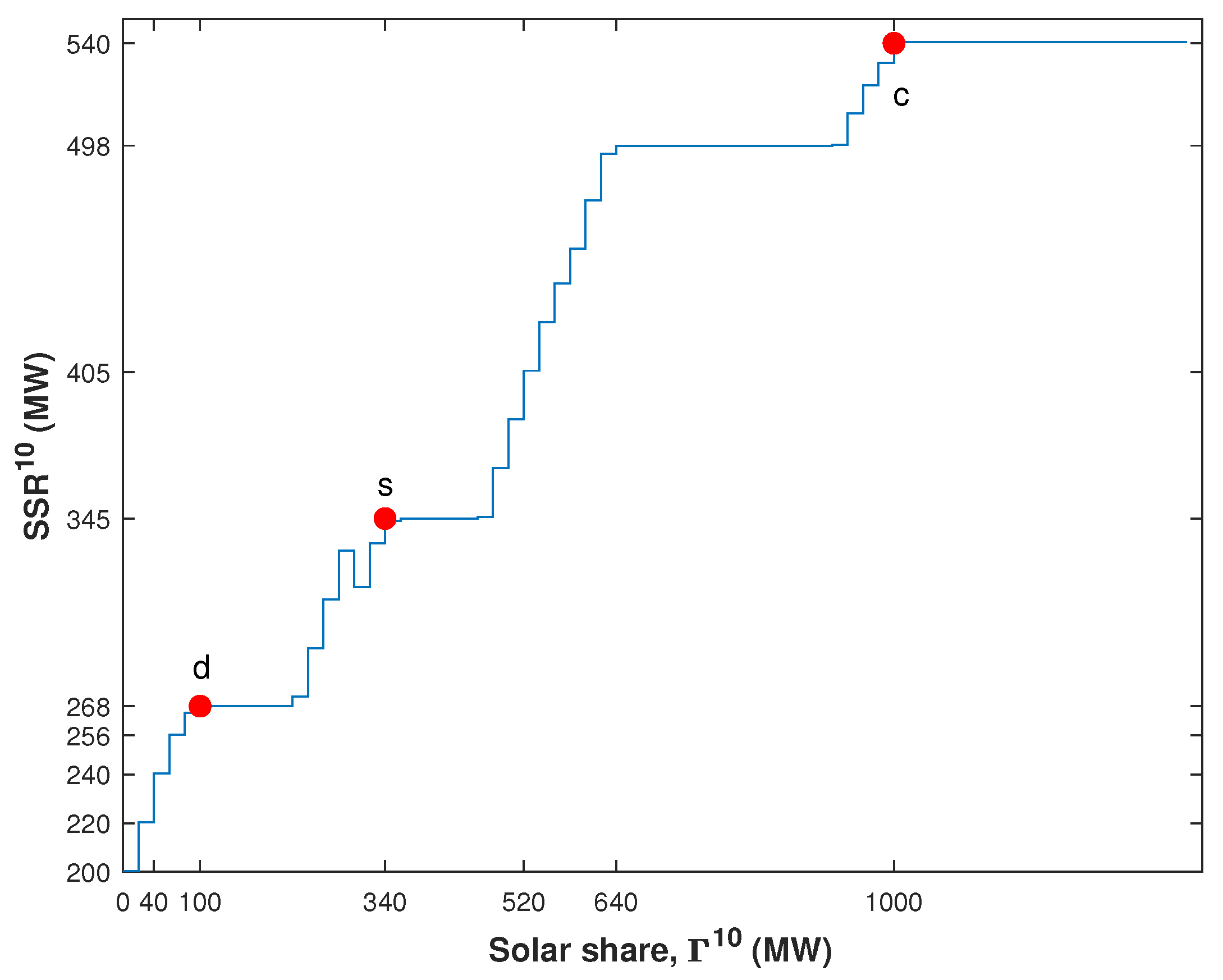
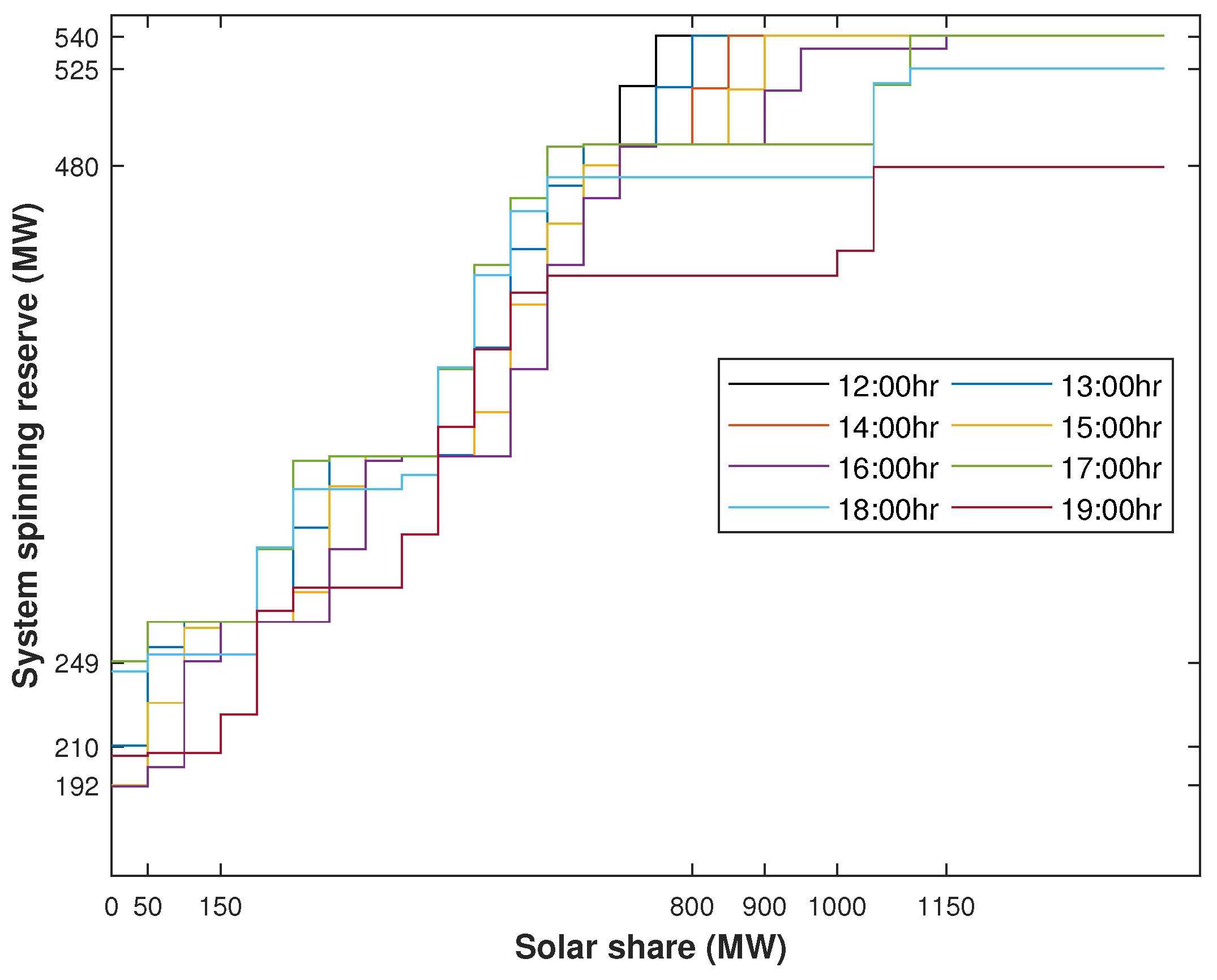
- Committed thermal units could provide a limited robust SSR to facilitate a given solar share. Thus, the amount of penetrated solar share at any time was limited by the available robust reserve at that time. For instance, came out to be 345 MW and 108 MW for solar shares of 340 MW and 100 MW, respectively, depending on the condition whether was allowed to be dispatched or restricted for a solar power outage event. Beyond these allocations, the robust SSR starts to become smaller than the solar share; therefore, loss of load would be experienced by the power system for a complete outage of the solar share.
- (ii)
- A set of committed units in a time slot could provide a certain amount of the ultimate SSR, which is 540 MW in the case of the 10-th hour.
- (iii)
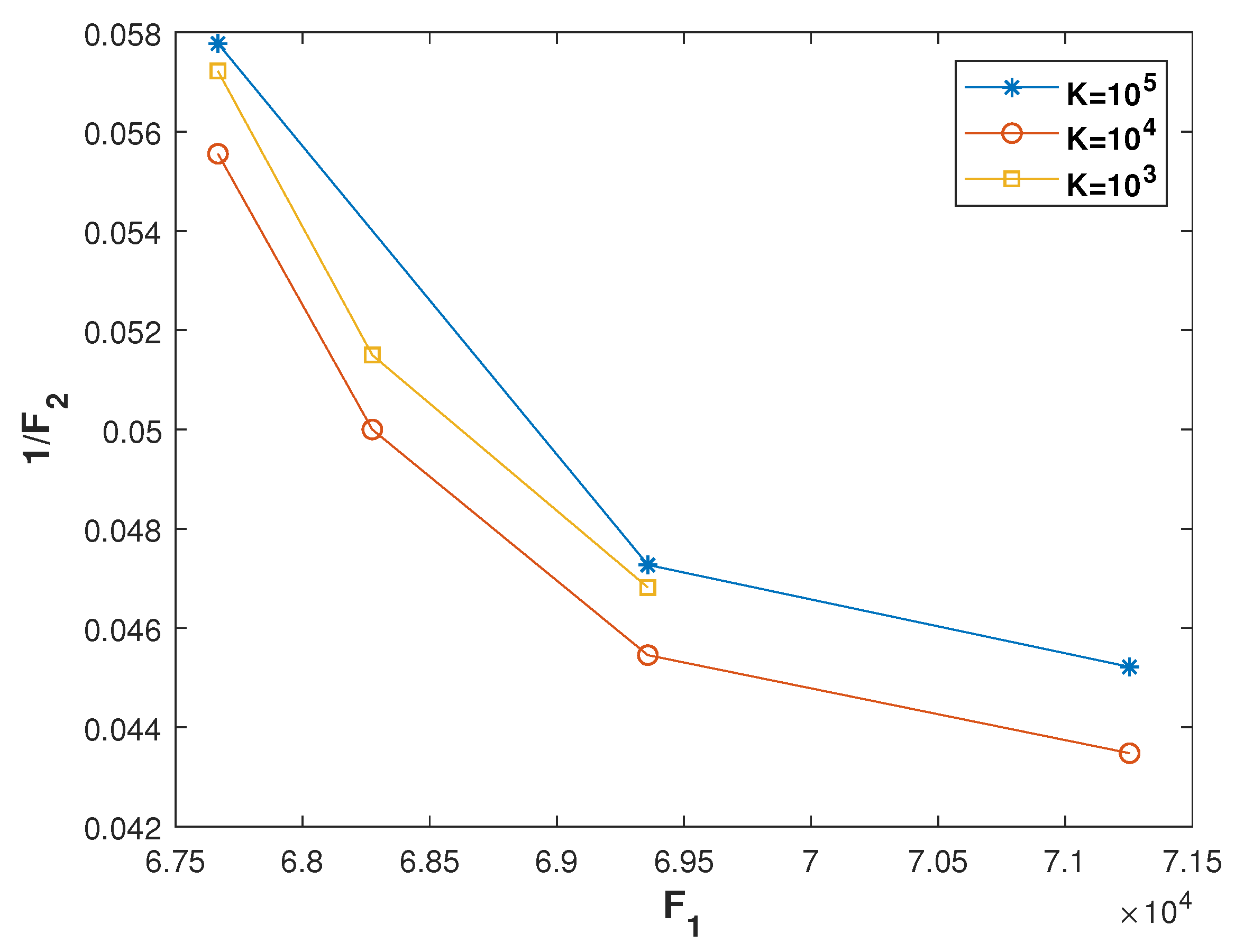
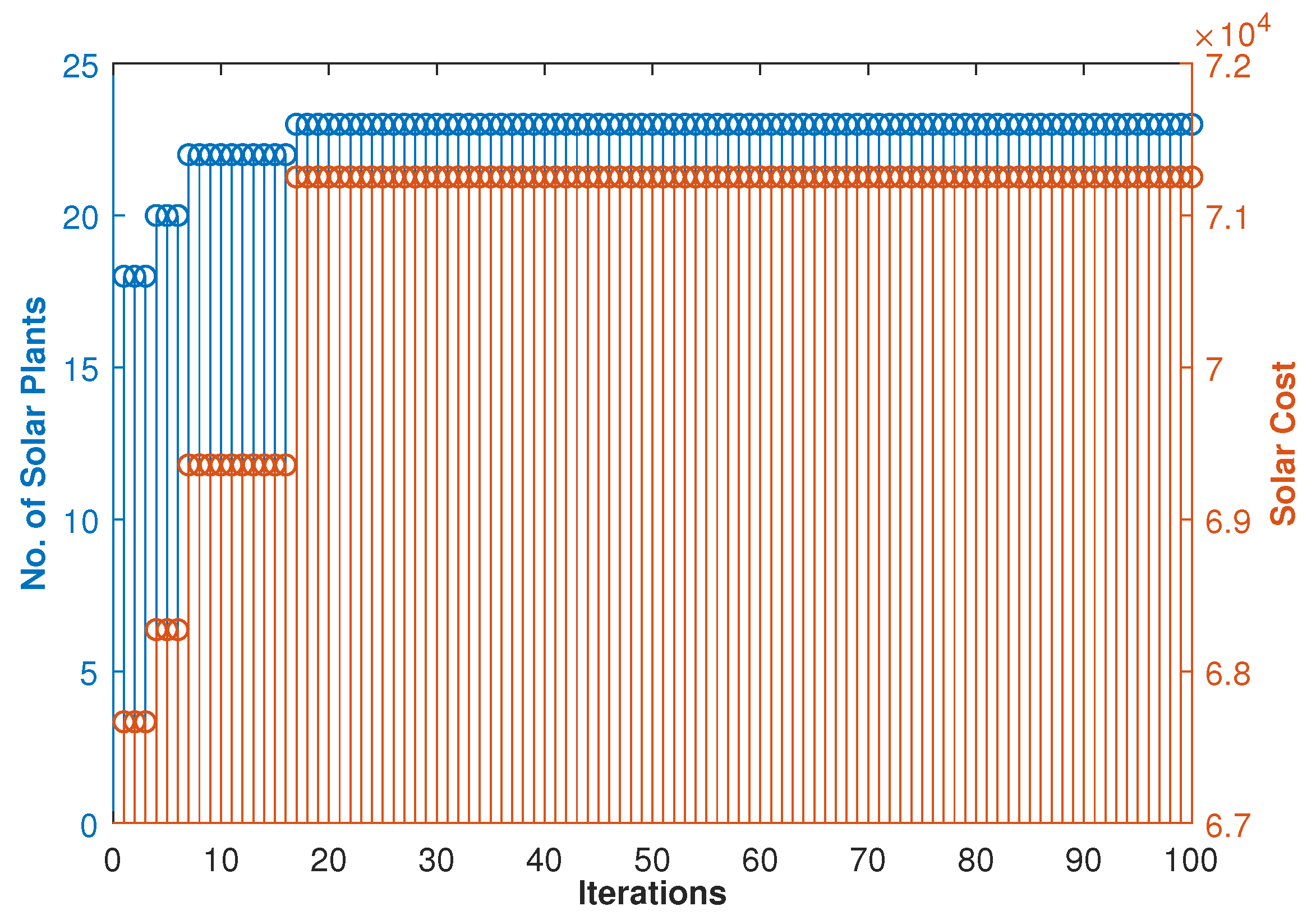
- Only a few such solutions were obtainable within the feasible binary search space when Pareto-optimal solutions were obtained for the contradictory objectives of solar cost minimization and the maximization of the number of solar plants. The highest number of such solutions were obtained with the value of parameter K empirically set to . Although this work investigated many critical issues of HPS, some aspects, such as network constraints, storage systems, and RE sources other than solar power, have not been covered.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Binary variable used to represent whether loss of load occurs when an
outage of the i-th unit occurs in period t; | |
| Binary variable used to represent whether loss of load occurs when a simultaneous outage of units i and j occurs during period t; | |
| Expected energy not served during time t; | |
| Power generated by the i-th thermal unit in time t; | |
| The minimum power of the i-th unit; | |
| The maximum power of the i-th unit; | |
| Penetrated solar power in time t; | |
| Power generated by the j-thsolar plant; | |
| Binary variable used to represent the ON and OFF status of a solar plant; | |
| Power demand in interval t; | |
| Outage probability of the i-th unit at time t; | |
| Probability of the simultaneous outage of the i-th and j-th unit at time t; | |
| Up-reserve power of the i-th unit in time t; | |
| Down-reserve power of the i-thunit in time t; | |
| Ramp-up rate of the i-th unit; | |
| Ramp-down rate of the i-th unit; | |
| Solar radiation at any given time t; | |
| System spinning reserve available at time t; | |
| Allocated SSR in time interval t; | |
| Optimal SSR for first- and second-order thermal contingency event; | |
| The ultimate in time interval t; | |
| T | Time duration of each optimization interval; |
| Ambient temperature; | |
| Consecutive cumulative ON time of the i-th unit until time t; | |
| Consecutive cumulative OFF time of the i-th unit until time t; | |
| The minimum uptime of the thermal generator; | |
| The minimum downtime of the thermal generator; | |
| Binary variable for the ON and OFF status of the i-th thermal unit at time t; | |
| Reference cell temperature; | |
| Value of lost load; | |
| Outage replacement rate of unit i; | |
| Temperature coefficient; | |
| Rated power of the j-th solar plant; | |
| The maximum allowable time for a thermal unit to ramp up or down; | |
| Per unit cost of the j-th solar plant; | |
| Step size for solar share increment; | |
| The maximum limit of the solar share within a robust range of the SSR; | |
| The maximum solar share for the range in which a power deficit is experienced by the power system; | |
| Solar share based on the SSR during time t; | |
| The maximum limit of the solar share during time t. |
References
- Adefarati, T.; Bansal, R.; Shongwe, T.; Naidoo, R.; Bettayeb, M.; Onaolapo, A. Optimal energy management, technical, economic, social, political and environmental benefit analysis of a grid-connected PV/WT/FC hybrid energy system. Energy Convers. Manag. 2023, 292, 117390. [Google Scholar] [CrossRef]
- Wood, A.J.; Wollenberg, B.F.; Sheblé, G.B. Power Generation, Operation, and Control; John Wiley & Sons: Hoboken, NJ, USA, 2013. [Google Scholar]
- Chiang, C.L. Genetic-based algorithm for power economic load dispatch. IET Gener. Transm. Distrib. 2007, 1, 261–269. [Google Scholar] [CrossRef]
- Jeyakumar, D.; Jayabarathi, T.; Raghunathan, T. Particle swarm optimization for various types of economic dispatch problems. Int. J. Electr. Power Energy Syst. 2006, 28, 36–42. [Google Scholar] [CrossRef]
- Lee, K.Y.; Sode-Yome, A.; Park, J.H. Adaptive Hopfield neural networks for economic load dispatch. IEEE Trans. Power Syst. 1998, 13, 519–526. [Google Scholar] [CrossRef]
- Mantawy, A.; Soliman, S.; El-Hawary, M. A new tabu search algorithm for the long-term hydro scheduling problem. In Proceedings of the LESCOPE’02—2002 Large Engineering Systems Conference on Power Engineering, Halifax, NS, Canada, 26–29 June 2002; pp. 29–34. [Google Scholar]
- Ardakani, F.F.; Mozafari, S.B.; Soleymani, S. Scheduling energy and spinning reserve based on linear chance constrained optimization for a wind integrated power system. Ain Shams Eng. J. 2022, 13, 101582. [Google Scholar] [CrossRef]
- Ahmadi-Khatir, A.; Bozorg, M.; Cherkaoui, R. Probabilistic spinning reserve provision model in multi-control zone power system. IEEE Trans. Power Syst. 2013, 28, 2819–2829. [Google Scholar] [CrossRef]
- Lee, C.; Liu, C.; Mehrotra, S.; Shahidehpour, M. Modeling transmission line constraints in two-stage robust unit commitment problem. IEEE Trans. Power Syst. 2013, 29, 1221–1231. [Google Scholar] [CrossRef]
- An, Y.; Zeng, B. Exploring the modeling capacity of two-stage robust optimization: Variants of robust unit commitment model. IEEE Trans. Power Syst. 2014, 30, 109–122. [Google Scholar] [CrossRef]
- Brini, S.; Abdallah, H.H.; Ouali, A. Economic dispatch for power system included wind and solar thermal energy. Leonardo J. Sci. 2009, 14, 204–220. [Google Scholar]
- Khan, N.A.; Sidhu, G.A.S.; Gao, F. Optimizing combined emission economic dispatch for solar integrated power systems. IEEE Access 2016, 4, 3340–3348. [Google Scholar] [CrossRef]
- Khan, N.A.; Sidhu, G.A.S.; Awan, A.B.; Ali, Z.; Mahmood, A. Modeling and operation optimization of RE integrated microgrids considering economic, energy, and environmental aspects. Int. J. Energy Res. 2019, 43, 6721–6739. [Google Scholar] [CrossRef]
- Khan, N.A.; Awan, A.B.; Mahmood, A.; Member, I.; Razzaq, S.; Zafar, A.; Sidhu, G.A.S. Combined emission economic dispatch of power system including solar photo voltaic generation. Energy Convers. Manag. 2015, 92, 82–91. [Google Scholar] [CrossRef]
- Nikolaidis, P.; Chatzis, S.; Poullikkas, A. Renewable energy integration through optimal unit commitment and electricity storage in weak power networks. Int. J. Sustain. Energy 2019, 38, 398–414. [Google Scholar] [CrossRef]
- Lorca, A.; Sun, X.A. Multistage robust unit commitment with dynamic uncertainty sets and energy storage. IEEE Trans. Power Syst. 2016, 32, 1678–1688. [Google Scholar] [CrossRef]
- Psarros, G.N.; Papathanassiou, S.A. Comparative assessment of priority listing and mixed integer linear programming unit commitment methods for non-interconnected island systems. Energies 2019, 12, 657. [Google Scholar] [CrossRef]
- Ortega-Vazquez, M.A.; Kirschen, D.S. Estimating the spinning reserve requirements in systems with significant wind power generation penetration. IEEE Trans. Power Syst. 2008, 24, 114–124. [Google Scholar] [CrossRef]
- Wang, M.; Gooi, H.; Chen, S. Optimising probabilistic spinning reserve using an analytical expected-energy-not-supplied formulation. IET Gener. Transm. Distrib. 2011, 5, 772–780. [Google Scholar] [CrossRef]
- Wang, M.Q.; Gooi, H. Spinning reserve estimation in microgrids. IEEE Trans. Power Syst. 2011, 26, 1164–1174. [Google Scholar] [CrossRef]
- Ortega-Vazquez, M.A.; Kirschen, D.S. Optimizing the spinning reserve requirements using a cost/benefit analysis. IEEE Trans. Power Syst. 2007, 22, 24–33. [Google Scholar] [CrossRef]
- Wang, M.; Yang, M.; Liu, Y.; Han, X.; Wu, Q. Optimizing probabilistic spinning reserve by an umbrella contingencies constrained unit commitment. Int. J. Electr. Power Energy Syst. 2019, 109, 187–197. [Google Scholar] [CrossRef]
- Wen, X.; Abbes, D.; Francois, B. Stochastic optimization for security-constrained day-ahead operational planning under pv production uncertainties: Reduction analysis of operating economic costs and carbon emissions. IEEE Access 2021, 9, 97039–97052. [Google Scholar] [CrossRef]
- Nikolaidis, P.; Poullikkas, A. Co-optimization of active power curtailment, load shedding and spinning reserve deficits through hybrid approach: Comparison of electrochemical storage technologies. IET Renew. Power Gener. 2022, 16, 92–104. [Google Scholar] [CrossRef]
- Håberg, M. Fundamentals and recent developments in stochastic unit commitment. Int. J. Electr. Power Energy Syst. 2019, 109, 38–48. [Google Scholar] [CrossRef]
- Shukla, A.; Singh, S.N. Multi-objective unit commitment with renewable energy using hybrid approach. IET Renew. Power Gener. 2016, 10, 327–338. [Google Scholar] [CrossRef]
- Boqtob, O.; El Moussaoui, H.; El Markhi, H.; Lamhamdi, T. Optimal robust unit commitment of microgrid using hybrid particle swarm optimization with sine cosine acceleration coefficients. Int. J. Renew. Energy Res. (IJRER) 2019, 9, 1125–1134. [Google Scholar]
- Nikolaidis, P.; Chatzis, S. Gaussian process-based Bayesian optimization for data-driven unit commitment. Int. J. Electr. Power Energy Syst. 2021, 130, 106930. [Google Scholar] [CrossRef]
- Nikolaidis, P.; Poullikkas, A. A novel cluster-based spinning reserve dynamic model for wind and PV power reinforcement. Energy 2021, 234, 121270. [Google Scholar] [CrossRef]
- Alves, E.F.; Polleux, L.; Guerassimoff, G.; Korpås, M.; Tedeschi, E. Allocation of Spinning Reserves in Autonomous Grids Considering Frequency Stability Constraints and Short-Term Solar Power Variations. IEEE Access 2023, 11, 29896–29908. [Google Scholar] [CrossRef]
- George, D.T.; Raj, R.E.; Rajkumar, A.; Mabel, M.C. Optimal sizing of solar-wind based hybrid energy system using modified dragonfly algorithm for an institution. Energy Convers. Manag. 2023, 283, 116938. [Google Scholar] [CrossRef]
- Idoko, L.; Anaya-Lara, O.; McDonald, A. Enhancing PV modules efficiency and power output using multi-concept cooling technique. Energy Rep. 2018, 4, 357–369. [Google Scholar] [CrossRef]
- Bouffard, F.; Galiana, F.D. An electricity market with a probabilistic spinning reserve criterion. IEEE Trans. Power Syst. 2004, 19, 300–307. [Google Scholar] [CrossRef]
- Wang, C.; Shahidehpour, S. Effects of ramp-rate limits on unit commitment and economic dispatch. IEEE Trans. Power Syst. 1993, 8, 1341–1350. [Google Scholar] [CrossRef]

| t (h) | Unit (1–26) | |||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 0 | 1 | 1 | 1 |
| 2 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 0 | 1 | 1 | 1 |
| 3 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 0 | 1 | 1 | 1 |
| 4 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 0 | 1 | 1 | 1 |
| 5 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 0 | 1 | 1 | 1 |
| 6 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 1 | 1 | 1 | 1 | 1 | 0 | 1 | 1 | 1 | 1 | 0 | 0 | 0 | 1 | 1 | 1 |
| 7 | 1 | 1 | 1 | 1 | 1 | 0 | 0 | 0 | 0 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 0 | 0 | 0 | 1 | 1 | 1 |
| 8 | 1 | 1 | 1 | 1 | 1 | 0 | 0 | 0 | 0 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 9 | 1 | 1 | 1 | 1 | 1 | 1 | 0 | 0 | 0 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 10 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 11 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 12 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 13 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 14 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 15 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 16 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 17 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 18 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 0 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 19 | 1 | 1 | 1 | 1 | 1 | 0 | 0 | 0 | 0 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 20 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 21 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 22 | 1 | 1 | 1 | 1 | 1 | 0 | 0 | 0 | 0 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 23 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 0 | 1 | 1 | 1 |
| 24 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 0 | 0 | 0 | 1 | 1 | 1 |
| Time of the Day | 10:00 | 11:00 | 12:00 | 13:00 | 14:00 | 15:00 | 16:00 | 17:00 | 18:00 |
|---|---|---|---|---|---|---|---|---|---|
| Robust for solar share at point ‘d’ (MW) | 108 | 21 | 118 | 118 | 110 | 106 | 31 | 110 | 92 |
| Solar share at point ‘d’ = (MW) | 100 | 20 | 100 | 100 | 100 | 100 | 20 | 100 | 80 |
| Robust at point ‘s’ = (MW) | 344 | 268 | 345 | 345 | 345 | 345 | 301 | 345 | 330 |
| Solar share at point ‘s’ = (MW) | 340 | 260 | 340 | 340 | 340 | 340 | 300 | 340 | 320 |
| at point ‘c’ = (MW) | 540 | 540 | 540 | 540 | 540 | 540 | 540 | 540 | 525 |
| (MW) | 2600 | 2670 | 2590 | 2590 | 2550 | 2620 | 2650 | 2550 | 2530 |
| Thermal generation (MW) for | 2260 | 2410 | 2250 | 2250 | 2210 | 2280 | 2350 | 2210 | 2210 |
| Thermal generation (MW) for | 2500 | 2660 | 2490 | 2490 | 2450 | 2520 | 2630 | 2450 | 2450 |
| Thermal fuel cost ($/MWh) with | 31,210 | 32,974 | 30,883 | 30,883 | 30,321 | 31,309 | 32,319 | 30,321 | 30,107 |
| Thermal fuel cost ($/MWh) with | 36,889 | 38,496 | 36,696 | 36,696 | 35,930 | 37,324 | 38,025 | 35,930 | 35,354 |
| 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| Thermal fuel cost without ORCUC ($/MWh) | 36,150 | 37,796 | 35,763 | 35,928 | 34,955 | 36,619 | 37,324 | 34,955 | 34,572 |
| Reserve cost ($) | 739 | 700 | 933 | 768 | 975 | 705 | 701 | 975 | 782 |
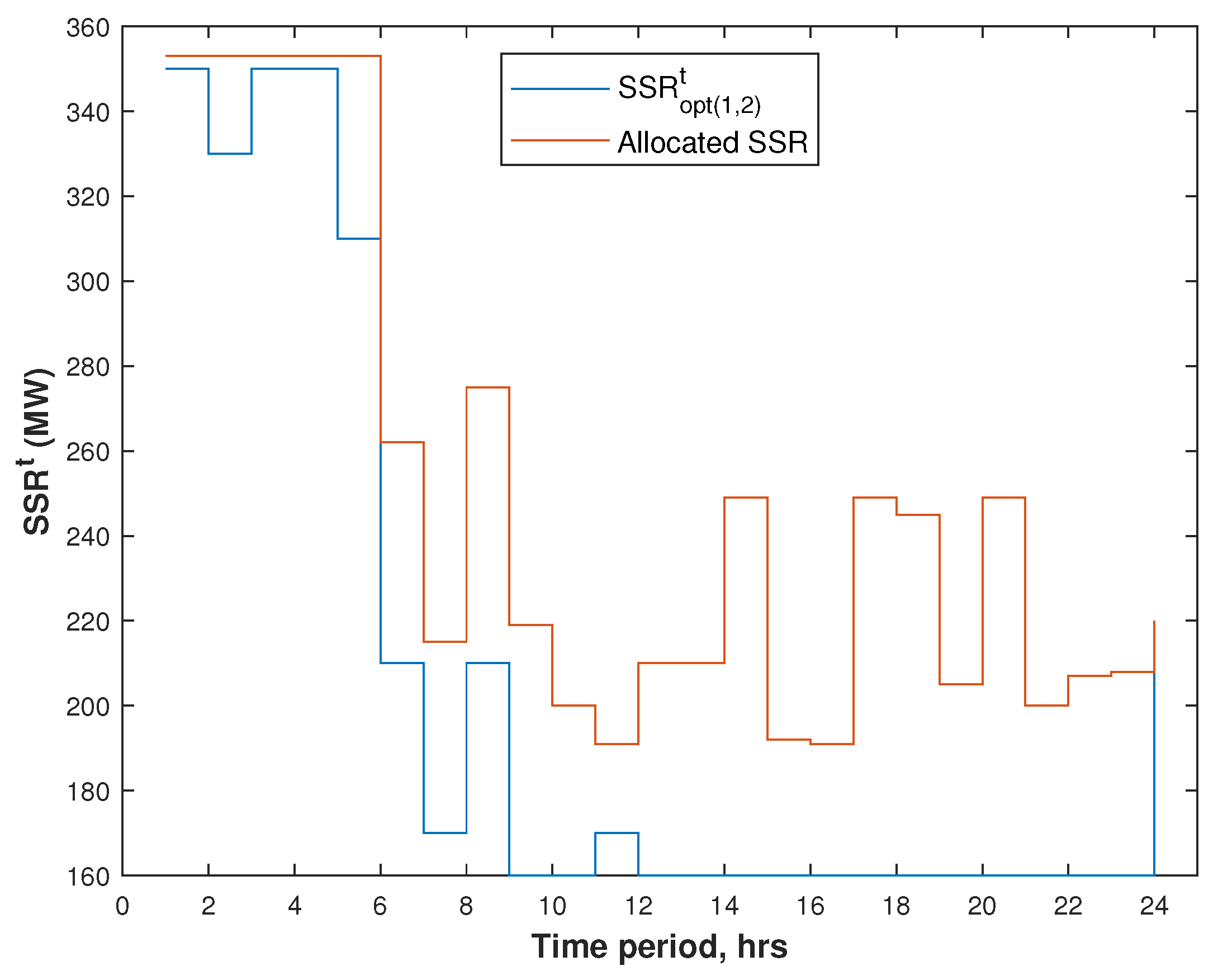
| Time of the Day | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| before inclusion of solar share | 353 | 353 | 353 | 353 | 353 | 262 | 215 | 275 | 219 | 200 | 191 | 210 | 210 | 249 | 192 | 191 | 249 | 245 | 205 | 249 | 200 | 207 | 208 | 220 |
| 350 | 330 | 350 | 350 | 310 | 210 | 170 | 210 | 160 | 160 | 170 | 160 | 160 | 160 | 160 | 160 | 160 | 160 | 160 | 160 | 160 | 160 | 160 | 210 |
| (MW) | 0 | 20 | 40 | 60 | 80 | 120 | - | - | |
| 200 | 220 | 240 | 256 | 265 | 268 | - | - | ||
| 40 | 60 | 80 | 96 | 105 | 108 | - | - |
| t (h) | ED Results of Thermal Units with | |||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| U1 | U2 | U3 | U4 | U5 | U6 | U7 | U8 | U9 | U10 | U11 | U12 | U13 | U14 | U15 | U16 | U17 | U18 | U19 | U20 | U21 | U22 | U23 | U24 | U25 | U26 | |
| 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 15.2 | 15.2 | 15.2 | 15.2 | 25 | 25 | 25 | 100.37 | 95.98 | 92.17 | 88.81 | 68.95 | 68.95 | 0 | 248.96 | 400 | 400 |
| 2 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 15.2 | 15.2 | 15.2 | 15.2 | 25 | 25 | 25 | 104.7 | 100.22 | 96.34 | 92.94 | 68.95 | 68.95 | 0 | 262.07 | 400 | 400 |
| 3 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 15.2 | 15.2 | 15.2 | 15.2 | 25 | 25 | 25 | 98.92 | 94.57 | 90.78 | 87.45 | 68.95 | 68.95 | 0 | 244.58 | 400 | 400 |
| 4 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 15.2 | 15.2 | 15.2 | 15.2 | 25 | 25 | 25 | 100.37 | 95.98 | 92.17 | 88.81 | 68.95 | 68.95 | 0 | 248.95 | 400 | 400 |
| 5 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 15.2 | 15.2 | 15.2 | 15.2 | 25 | 25 | 25 | 107.59 | 103.06 | 99.13 | 95.69 | 68.95 | 68.95 | 0 | 270.82 | 400 | 400 |
| 6 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 34.45 | 34.45 | 34.45 | 34.45 | 49.09 | 41.9 | 0 | 135.09 | 130.55 | 126.62 | 123.19 | 0 | 0 | 0 | 305.82 | 400 | 400 |
| 7 | 2.4 | 2.4 | 2.4 | 2.4 | 2.4 | 0 | 0 | 0 | 0 | 42.78 | 40.39 | 38.26 | 35.92 | 25 | 25 | 25 | 155 | 155 | 154.13 | 150.69 | 0 | 0 | 0 | 340.83 | 400 | 400 |
| 8 | 2.4 | 2.4 | 2.4 | 2.4 | 2.4 | 0 | 0 | 0 | 0 | 62.03 | 59.64 | 57.51 | 55.17 | 50.5 | 50.5 | 50.5 | 155 | 155 | 155 | 155 | 106.42 | 86.78 | 68.95 | 350 | 400 | 400 |
| 9 | 2.4 | 2.4 | 2.4 | 2.4 | 2.4 | 4 | 0 | 0 | 0 | 76 | 76 | 76 | 74.42 | 76 | 76 | 76 | 155 | 155 | 155 | 155 | 85.68 | 68.95 | 68.95 | 350 | 400 | 400 |
| 10 | 2.4 | 2.4 | 2.4 | 2.4 | 2.4 | 4 | 4 | 4 | 4 | 37.84 | 35.55 | 33.5 | 31.27 | 39 | 39 | 39 | 155 | 155 | 155 | 155 | 68.95 | 68.95 | 68.95 | 350 | 400 | 400 |
| 11 | 2.4 | 2.4 | 2.4 | 2.4 | 2.4 | 4 | 4 | 4 | 4 | 95.25 | 76 | 73.28 | 70.11 | 25 | 25 | 25 | 155 | 155 | 155 | 155 | 68.95 | 68.95 | 68.95 | 350 | 400 | 400 |
| 12 | 2.4 | 2.4 | 2.4 | 2.4 | 2.4 | 4 | 4 | 4 | 4 | 46.08 | 43.62 | 41.43 | 39.016 | 25 | 25 | 25 | 155 | 155 | 155 | 155 | 68.95 | 68.95 | 68.95 | 350 | 400 | 400 |
| 13 | 2.4 | 2.4 | 2.4 | 2.4 | 2.4 | 4 | 4 | 4 | 4 | 46.08 | 43.61 | 41.43 | 39.01 | 25 | 25 | 25 | 155 | 155 | 155 | 155 | 68.95 | 68.95 | 68.95 | 350 | 400 | 400 |
| 14 | 2.4 | 2.4 | 2.4 | 2.4 | 2.4 | 4 | 4 | 4 | 4 | 35.78 | 33.53 | 31.51 | 29.33 | 25 | 25 | 25 | 155 | 155 | 155 | 155 | 68.95 | 68.95 | 68.95 | 350 | 400 | 400 |
| 15 | 2.4 | 2.4 | 2.4 | 2.4 | 2.4 | 4 | 4 | 4 | 4 | 53.81 | 51.18 | 48.88 | 46.28 | 25 | 25 | 25 | 155 | 155 | 155 | 155 | 68.95 | 68.95 | 68.95 | 350 | 400 | 400 |
| 16 | 2.4 | 2.4 | 2.4 | 2.4 | 2.4 | 4 | 4 | 4 | 4 | 71.85 | 68.84 | 66.23 | 63.23 | 25 | 25 | 25 | 155 | 155 | 155 | 155 | 68.95 | 68.95 | 68.95 | 350 | 400 | 400 |
| 17 | 2.4 | 2.4 | 2.4 | 2.4 | 2.4 | 4 | 4 | 4 | 4 | 35.77 | 33.53 | 31.51 | 29.33 | 25 | 25 | 25 | 155 | 155 | 155 | 155 | 68.95 | 68.95 | 68.95 | 350 | 400 | 400 |
| 18 | 2.4 | 2.4 | 2.4 | 2.4 | 2.4 | 4 | 4 | 4 | 0 | 36.8 | 34.54 | 32.5 | 30.3 | 25 | 25 | 25 | 155 | 155 | 155 | 155 | 68.95 | 68.95 | 68.95 | 350 | 400 | 400 |
| 19 | 2.4 | 2.4 | 2.4 | 2.4 | 2.4 | 0 | 0 | 0 | 0 | 42.48 | 40.08 | 37.96 | 35.63 | 25 | 25 | 25 | 155 | 155 | 155 | 155 | 68.95 | 68.95 | 68.95 | 350 | 400 | 400 |
| 20 | 2.4 | 2.4 | 2.4 | 2.4 | 2.4 | 4 | 4 | 4 | 4 | 35.78 | 33.53 | 31.51 | 29.33 | 25 | 25 | 25 | 155 | 155 | 155 | 155 | 68.95 | 68.95 | 68.95 | 350 | 400 | 400 |
| 21 | 2.4 | 2.4 | 2.4 | 2.4 | 2.4 | 4 | 4 | 4 | 4 | 95.25 | 95.25 | 95.25 | 95.25 | 78.12 | 71.36 | 64.67 | 155 | 155 | 155 | 155 | 68.95 | 68.95 | 68.95 | 350 | 400 | 400 |
| 22 | 2.4 | 2.4 | 2.4 | 2.4 | 2.4 | 0 | 0 | 0 | 0 | 76 | 76 | 76 | 76 | 69.3 | 62.38 | 55.47 | 155 | 155 | 155 | 155 | 68.95 | 68.95 | 68.95 | 350 | 400 | 400 |
| 23 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 56.20 | 53.52 | 51.173 | 48.53 | 32.3 | 25.38 | 25 | 155 | 155 | 155 | 155 | 68.95 | 68.95 | 0 | 350 | 400 | 400 |
| 24 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 16.2 | 15.2 | 15.2 | 15.2 | 25 | 25 | 25 | 145.55 | 140.21 | 135.67 | 131.77 | 0 | 0 | 0 | 350 | 400 | 400 |
| Number of Plants | (MW) | Unit Rate ($/KWh) |
|---|---|---|
| 3 | 10 | 0.19, 2 × 0.18 |
| 5 | 12 | 5 × 0.19 |
| 1 | 15 | 0.2 |
| 3 | 18 | 3 × 0.2 |
| 2 | 20 | 2 × 0.23 |
| 4 | 24 | 4 × 0.23 |
| 4 | 25 | 4 × 0.23 |
| 2 | 30 | 2 × 0.24 |
| 5 | 35 | 0.25, 0.26, 0.23, 2 × 0.24 |
| 7 | 40 | 2 × 0.27, 2 × 0.275, 3 × 0.28 |
| 2 | 50 | 2 × 0.18 |
| 1 | 60 | 0.21 |
| 1 | 80 | 0.22 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Saeed, R.M.M.; Khan, N.A.; Shakir, M.; Sidhu, G.A.S.; Awan, A.B.; Baseer, M.A. Maximizing Solar Share in Robust System Spinning Reserve-Constrained Economic Operation of Hybrid Power Systems. Energies 2024, 17, 2794. https://doi.org/10.3390/en17112794
Saeed RMM, Khan NA, Shakir M, Sidhu GAS, Awan AB, Baseer MA. Maximizing Solar Share in Robust System Spinning Reserve-Constrained Economic Operation of Hybrid Power Systems. Energies. 2024; 17(11):2794. https://doi.org/10.3390/en17112794
Chicago/Turabian StyleSaeed, Rana Muhammad Musharraf, Naveed Ahmed Khan, Mustafa Shakir, Guftaar Ahmad Sardar Sidhu, Ahmed Bilal Awan, and Mohammad Abdul Baseer. 2024. "Maximizing Solar Share in Robust System Spinning Reserve-Constrained Economic Operation of Hybrid Power Systems" Energies 17, no. 11: 2794. https://doi.org/10.3390/en17112794
APA StyleSaeed, R. M. M., Khan, N. A., Shakir, M., Sidhu, G. A. S., Awan, A. B., & Baseer, M. A. (2024). Maximizing Solar Share in Robust System Spinning Reserve-Constrained Economic Operation of Hybrid Power Systems. Energies, 17(11), 2794. https://doi.org/10.3390/en17112794







