Voltage and Current Unbalance Reduction in Power Networks with Distributed Generation and Electric Vehicles

Abstract

The current development of prosumer microsources and the expected spread of electric vehicles may cause the appearance of significant current and voltage unbalance in low-voltage (LV) networks. This unbalance, which is an unfavorable phenomenon, may occur when using single-phase photovoltaic (PV) microsources and single-phase home chargers for electric vehicles. This paper presents a proposal for the symmetrization of the LV network using devices for the reconfiguration of phases in the power supply. Both the different locations of these devices and the different objective functions for device implementation are analyzed. The research was carried out on an example LV network, taking into account several variants of the development of PV microsources and home chargers for electric vehicles. The analysis indicates that the appropriate location of phase reconfiguration devices and the use of an appropriate objective function leads to a significant reduction in unfavorable unbalancing in the LV network.

1. Introduction

By 2050, the European Union (EU) wants to achieve climate neutrality [1]. Various programs are used to achieve this aim, including programs for increasing the share of renewable energy sources, resigning from the registration of combustion cars, or resigning from individual combustion-based heating furnaces. This is related to the large-scale emergence of new facilities in the power system, such as photovoltaic (PV) sources, electric vehicle (EV) chargers, or heat pumps. Single-phase facilities, in some cases, can significantly impact the operation of the low-voltage (LV) network, which is used for distributed generation.

Wind and PV sources, as well as energy storage facilities [2] and EVs that can operate in V2G or G2V modes [3,4,5], are connected to a local network. Thanks to local production and close consumption, the use of smart metering and smart charging causes self-consumption to increase [6,7,8]. In such a system, there is no need to transmit power over long distances, which avoids increased power losses. The intelligent management of electric vehicles connected to the grid allows for technical and economic benefits [9]. Such intelligent management is also considered on the basis of DC microgrids [10]. However, it should be indicated that in networks with distributed generation, there may be problems with power quality [11]. One of the problems is power outages, e.g., in systems with wind turbines [12]. The behavior of such systems is recommended to be analyzed using real-time simulators [13]. The greatest problems occur in networks with PV sources because they cause a significant increase in network voltage, often exceeding the permissible value [14,15,16], and are also the reason for a significant voltage unbalance [17,18,19,20]. The problem of increased voltage/current unbalance, as well as increased power loss, may be aggravated by connecting EVs to the LV power network [21,22].

In order to mitigate the unfavorable voltage parameters in the network with distributed generation, various solutions are used. In paper [23], an insulated gate bipolar transistor-based parallel active filter was used for current/voltage unbalance compensation. It has been proven that such a filter can compensate for the unbalance in load current or in the voltage source. Paper [24] proposes a Three Phase Optimal Power Flow method to minimize the voltage unbalance in LV distribution networks with a high penetration of PV sources. Consequently, the reactive power management from these sources significantly reduces voltage unbalance. Paper [25] describes voltage unbalance compensation and minimizing loss in the distribution system with the use of a three-phase individual-step voltage regulator. The application of this system enables the individual tap control of each phase. Paper [26] proposes a method to mitigate voltage unbalance caused by single-phase PV inverters in LV systems. The method is based on the uneven absorption and injection of reactive power by three-phase PV inverters. Each phase is controlled here to achieve this uneven power injection. In turn, paper [27] presents strategies for the control of single-phase and three-phase generating units cooperating with the inverters to improve the voltage magnitude and compensate for the current unbalance. A dynamic voltage restorer—a series-connected advanced power electronic custom-based tool—is discussed in paper [28]. This restorer is installed between the voltage source and the loads to alleviate voltage disturbances. The aforementioned solution is recommended, among others, as a cost-effective method for improving the voltage profile and reducing the voltage unbalance. The authors of paper [29] consider the effect of an unbalanced load with its step change on the voltage unbalance factor. They implemented a positive sequence controller strategy for the control of voltage for varying loads. In paper [30], the authors draw attention to the problem of connecting single-phase distributed energy resources and modern single-phase loads to the network. They analyze the effectiveness of using on-load tap changers, line-voltage regulators, and a Zic-Zac-Load balancer. When reviewing the most recent literature, it should be noted that the following solutions for improving the voltage parameters in the network are currently being discussed. Paper [31] focuses on optimal voltage regulator placement in relation to the optimal placement of EV chargers and PV sources. An optimal allocation of distributed generations is considered in paper [32]. In this case, PV sources, wind turbines, and conventional sources were used so as to minimize voltage drops and the level of power losses. In the analyzed case, more than 2% of power loss reduction, as well as an improvement in voltage levels, was achieved. The problem of optimization in networks with distributed generation is also discussed in publication [33]. Here, optimal EV charging station integration based on the voltage profile and power losses is presented. A genetic algorithm was used for proper network planning. In the case of paper [34], a comprehensive management plan for distributed PV grid-connected voltage based on shunt reactors and energy storage is proposed. In paper [35], a test microgrid consisting of a synchronous generator, PV system, battery storage system, and controllable balanced and unbalanced loads is discussed. It has been proven that it is possible to simultaneously decrease voltage unbalances and frequency deviations with the use of the advanced control structures of the distributed generation sources based on power electronics devices. Paper [36] proposes a neighborhood-collaborative mechanism-based voltage control and voltage unbalance alleviation method for individual PV inverters. This method is integrated with the proposed current unbalance mitigation. There are also methods related to network reconfiguration [37,38,39,40], but relatively few methods are focused on phase rearrangement, such as the Particle Swarm Optimization method, which can find the optimum phase rearrangement as the minimized imbalance on the feeder [41], and is a method of phase rearrangement and dynamic phase and load balancing along a feeder with a radial structure [42] or Dynamic Switching Devices, which are fast power electronic devices for changing the phase connection of a solar PV [43] or measurable data phase-reconfiguration devices [44]. In [45], a droop-based control approach is developed to compensate for the unbalanced output voltages of generators in islanded systems. Paper [46] proposes a control strategy for the converter to suppress the unbalance and distortion of the network-side current and achieve reference voltage output under the unbalanced network voltage.

Based on an analysis of the literature, it can be concluded that a reduction in voltage/current unbalance, as well as the reduction in power losses in networks with distributed generation, are performed primarily with the use of power electronic devices and with the help of the optimal location of sources, e.g., PV sources. So far, the automatic switching/reconfiguration of phases in the network, depending on the voltage/current unbalance caused by the connected single-phase sources and single-phase loads, has not been deeply considered. The implementation of such a proposal is being considered in one of the Polish local power networks. This paper describes the possible benefits of this, calculated on the basis of a real network study. The proposed idea and obtained results may be applicable to many other networks.

In this paper, phase reconfiguration is understood as the change in the sequence of the supply phases. At the same time, it is only possible to shift the phases consecutively. For example, if the sequence of the phases supplying the consumer is L1-L2-L3, it is only possible to change it to L2-L3-L1 or L3-L1-L2.

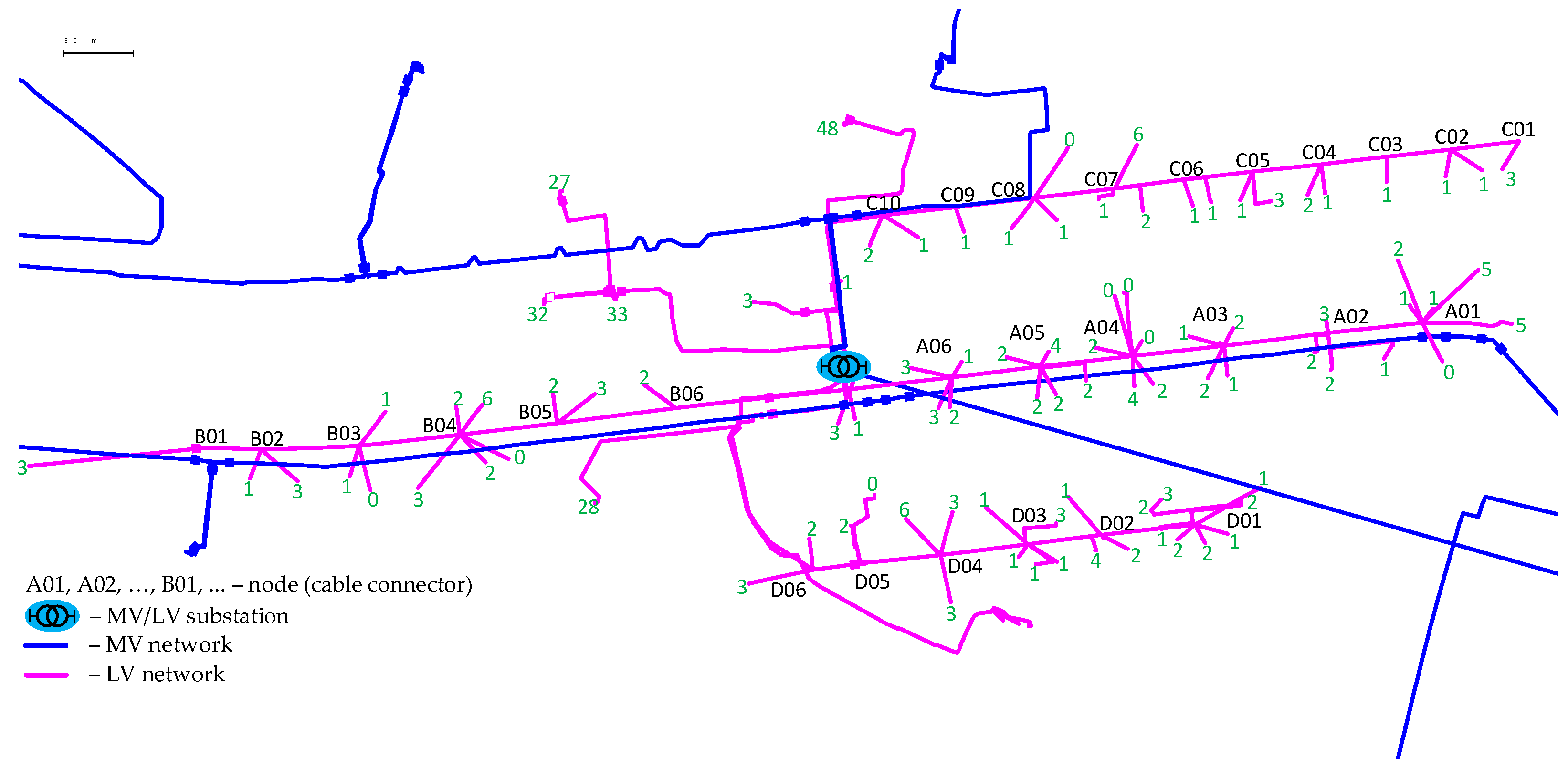
2. Description of the Analyzed Power Network

The real LV network with a nominal voltage equal to 400/230 V was used in the analyses (Figure 1). It is an urban cable network where most of the customers are those living in single-family houses and multi-family houses. The total length of power lines is 4.24 km, and cable cross sections are between 16 mm² and 240 mm². The network is supplied by a 400 kVA transformer—15 kV (MV side) and 0.4 kV (LV side). In Figure 1, this can be recognized by the number of consumers/prosumers written at the ends of the line connections. If this number is at least a dozen or so, it means that it is a multi-family building. In total, 337 private consumers are supplied in the entire network. Each of these consumers has a bidirectional energy meter.

In general, the LV network is characterized by current and voltage unbalancing. The reason is single-phase loads, which constitute the vast majority of electrical installations for private/domestic consumers. It can, therefore, be concluded that unbalancing is a “natural” state in LV networks, and its level may change often. Figure 2 shows two examples (two randomly selected cases) from the considered LV network, which illustrate the difference in currents in individual phases during the day. These values were measured by Advanced Metering Infrastructure (AMI) system meters and represent the average current value for 15 min.

For further analyses related to the development of prosumer installations, it was assumed that some prosumers in the network have single-phase PV microsources with a power of 3.7 kW. Moreover, the same prosumers have electric vehicles. It was assumed that there are 28 prosumers of this nature in the considered network, which constitutes 8.3% of the total number of participants connected to it. The generation of PV sources was obtained from the Photovoltaic Geographical Information System (PVGIS) [47] (Figure 3a). In the case of electric vehicles, it was assumed that they were charged in the evening and at night from single-phase chargers with a power of 3.8 kW (Figure 3b). Single-phase PV microsources and electric vehicle chargers were used to highlight the problems that can (and do) occur in the LV network. The powers of these objects were assumed for national conditions, in which the current flow of a standard single-phase load should not exceed 16 A.

In fact, prosumers with PV microsources and electric vehicles may be connected to different areas of the LV network. As this may have a significant impact on the network’s operation, the following two different ways of allocating prosumers were tested:

(1)

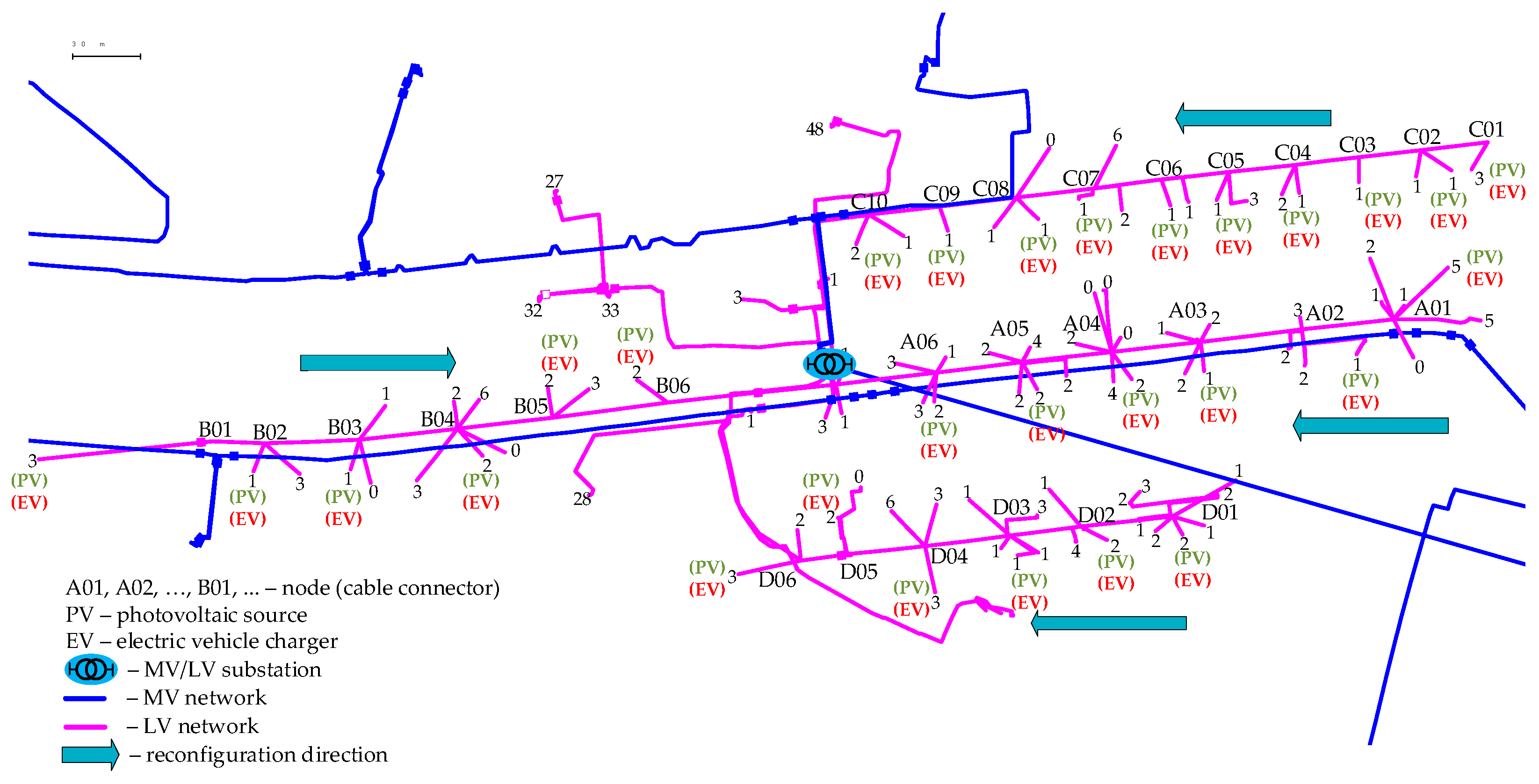
In the first variant (W1), prosumers are distributed evenly along the entire length of the main supply lines. Their location is marked in Figure 4 by adding the marks “PV” and “EV”.

(2)

In the second variant (W2), prosumers with PV microsources and electric vehicles are clustered closer to each other (Figure 5). In this case, more than one such prosumer is connected to one network point (node/cable connector).

In both variants (W1 and W2), the number of prosumers with PV and EV remains the same.

This paper considers phase reconfiguration in the LV network in order to reduce current and voltage unbalance. In practice, the phase reconfiguration device can be placed only at one point of the network (e.g., at the beginning of the main power line), or it can be located at many points of the network (e.g., at the point of connection for selected customers/prosumers). In general, three locations for phase reconfiguration devices can be defined:

(1)

In main power lines, there may be several such devices located along the line. Figure 4 and Figure 5 show the proposed locations, marked as green squares with the descriptions “A1”, “A2”, etc.;

(2)

At the point of connection of the prosumer, i.e., in the “node/cable connector”;

(3)

At the MV/LV distribution substation, at the beginning of the main power lines.

A separate analysis has shown that the least effective solution is the third option. Therefore, this solution was omitted from further analyses.

3. Objective Functions

Symmetrization of the LV network is understood in this paper as a reduction in voltage unbalance and/or as a reduction in current unbalance. The following two approaches, utilizing the reconfiguration of the supply phases (line conductor reconfiguration), are considered as follows:

(1)

Distributed Main Circuit Reconfiguration (hereinafter referred to as DMCR)—reconfiguration performed in the main power lines, which affects a specific group of prosumers’ supply “behind” (downstream) the device;

(2)

Final Individual Circuit Reconfiguration (hereinafter referred to as FICR)—phase reconfiguration of prosumers with a PV microsource and an electric vehicle at the point of their connection to the network.

In both of the above cases (DMCR and FICR), it is necessary to measure phase voltages and currents as well as currents flowing in neutral conductors. The place of current/voltage measurement may be at the place where the phase reconfiguration device is installed or at a place remote from it. This is also related to the operation of devices, which, depending on the adopted strategy, can operate autonomously or be controlled by a master controller installed in the MV/LV substation. It should be noted that the analyzed Distribution System Operator (DSO) has remote communication capabilities using Power Line Communication (PLC) technology. However, at the moment, it does not yet have controllers designed for the tasks described in the paper.

Depending on the location of the phase reconfiguration devices and the location of the measurements, four example objective functions were proposed, which are called “scenarios” (Figure 6).

• Devices installed at prosumers (for FICR implementation):
  • The minimization of the voltage unbalance factor measured at the point of installation of the phase reconfiguration device (mark: FICR-LVc)—objective function (scenario) no. 1—is as follows:
  K_V(FICR-LVc) = min{k_V,_n} for I_j ≤ I_z,j and V_w ≤ V_max and V_w ≥ V_min,

  (1)

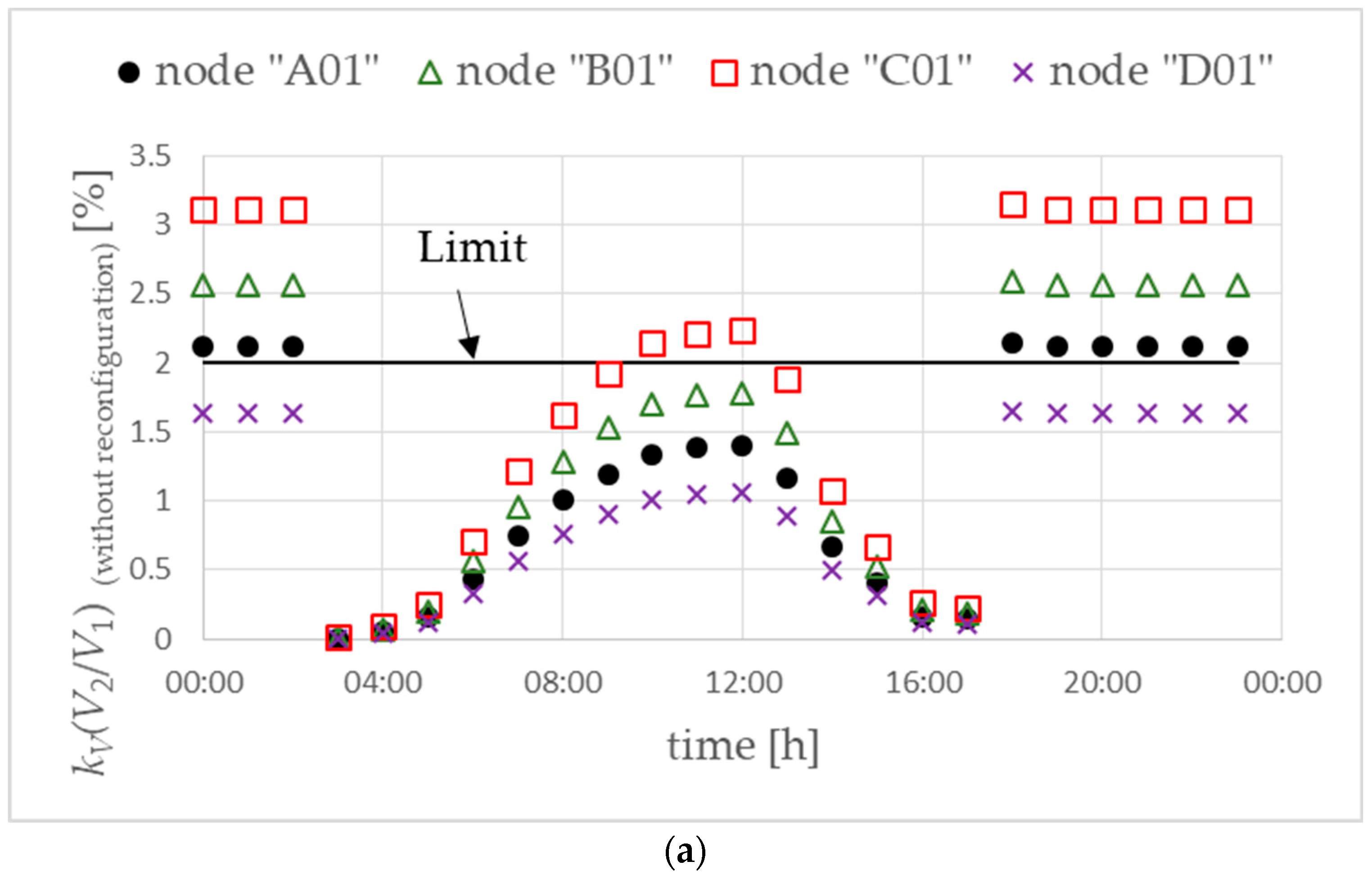
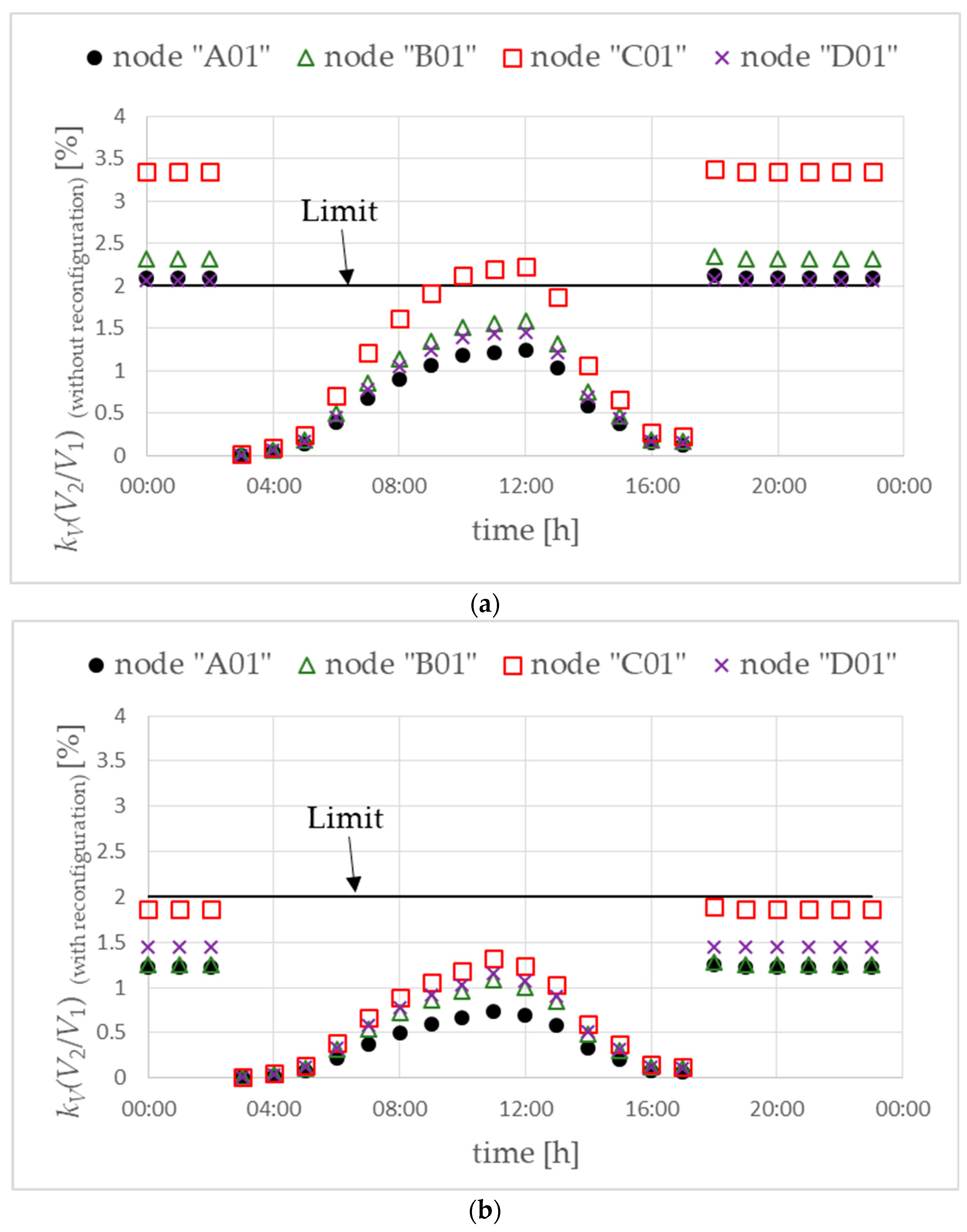
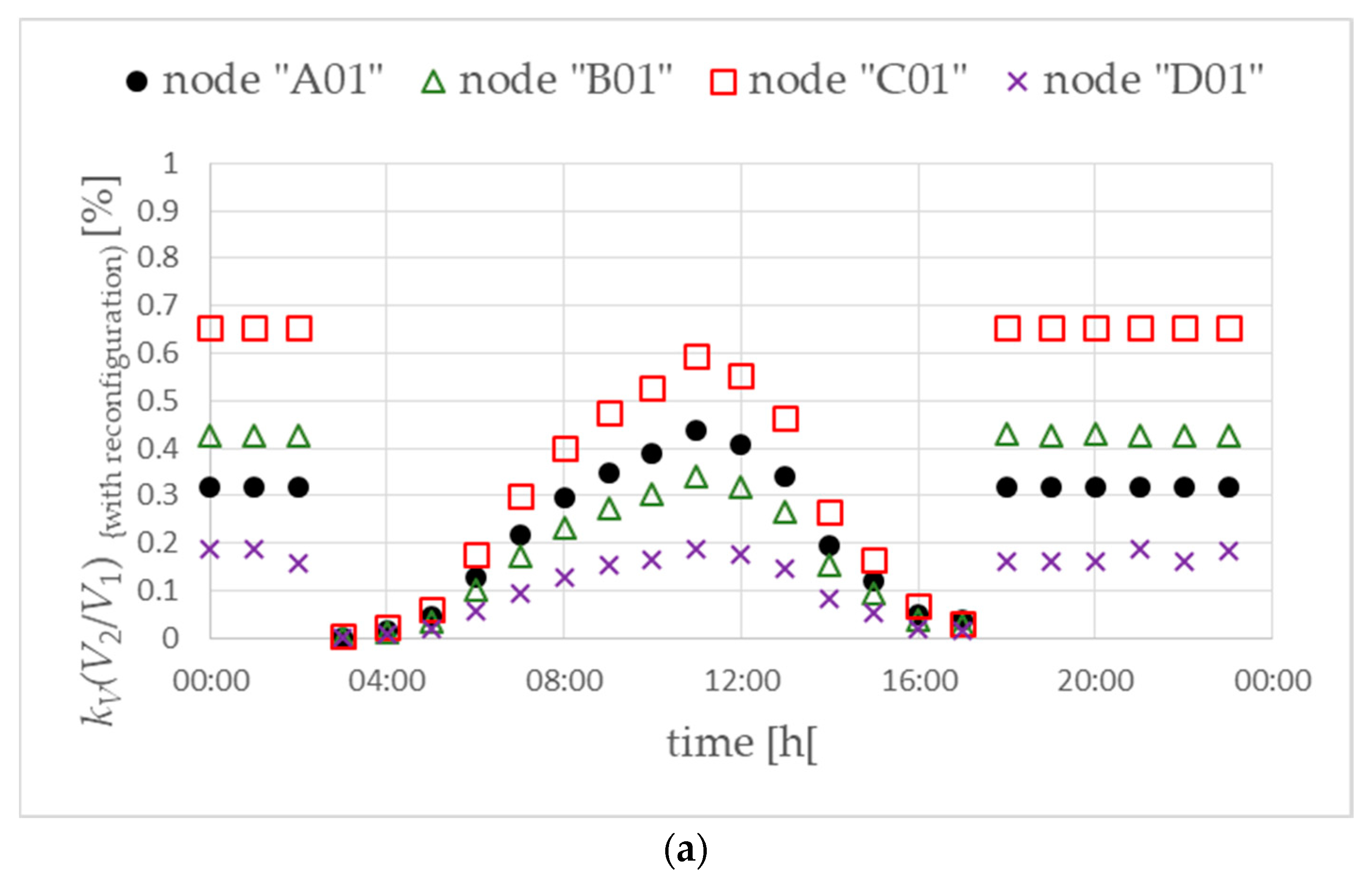
  where k_V,n—voltage unbalance factor (defined in (5)) at the point of application of the phase reconfiguration device, I_j—current in the j-th power line, I_z,j—current-carrying capacity of the j-th power line, V_w—voltage of the w-th network node, V_max—maximum permissible line-to-neutral voltage of the analyzed network (253 V), and V_min—minimum permissible line-to-neutral voltage of the analyzed network (207 V).

  b.

  The minimization of currents flowing in neutral conductors at the point of installation of the phase reconfiguration device (mark: FICR-Lic)—objective function (scenario) no. 2—is as follows:

  K_I(FICR-Lic) = min{I_c,_i} for I_j ≤ I_z and V_w ≤ V_max and V_w ≥ V_min,

  (2)

  where I_c,j—I_N current in the j-th power line, at the point of application of the phase reconfiguration device (other symbols as in (1)).

  c.

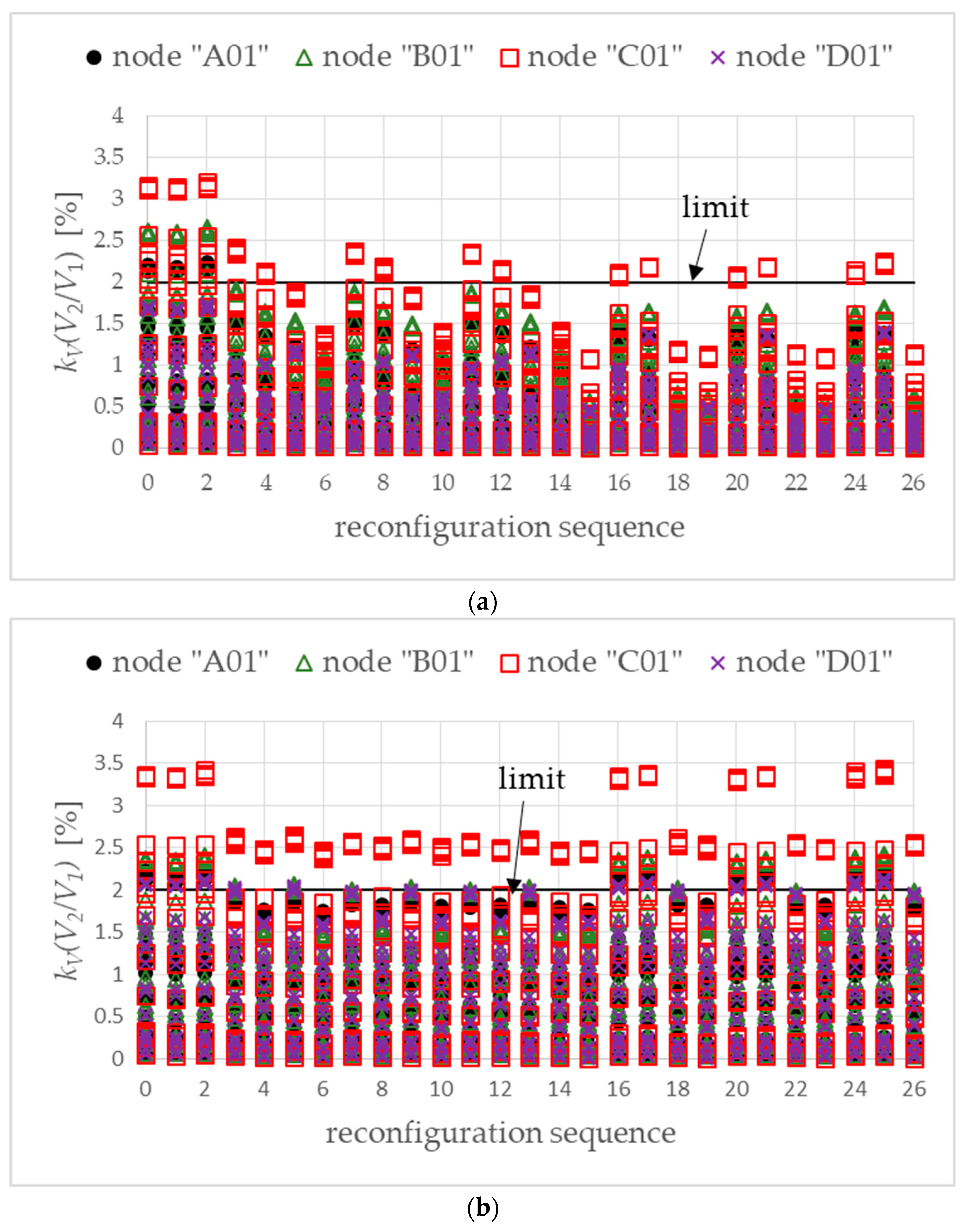
  The minimization of currents flowing in neutral conductors in the MV/LV distribution substation (mark: FICR-Mis)—objective function (scenario) no. 3—is as follows:

  K_I(FICR-Mic) = min{I_s,_i} for I_j ≤ I_z and V_w ≤ V_max and V_w ≥ V_min,

  (3)

  where I_s,i—I_N current in the i-th main power line in the MV/LV substation (other symbols as in (1)).

• The devices installed in main power lines (for DMCR implementation).

  a.

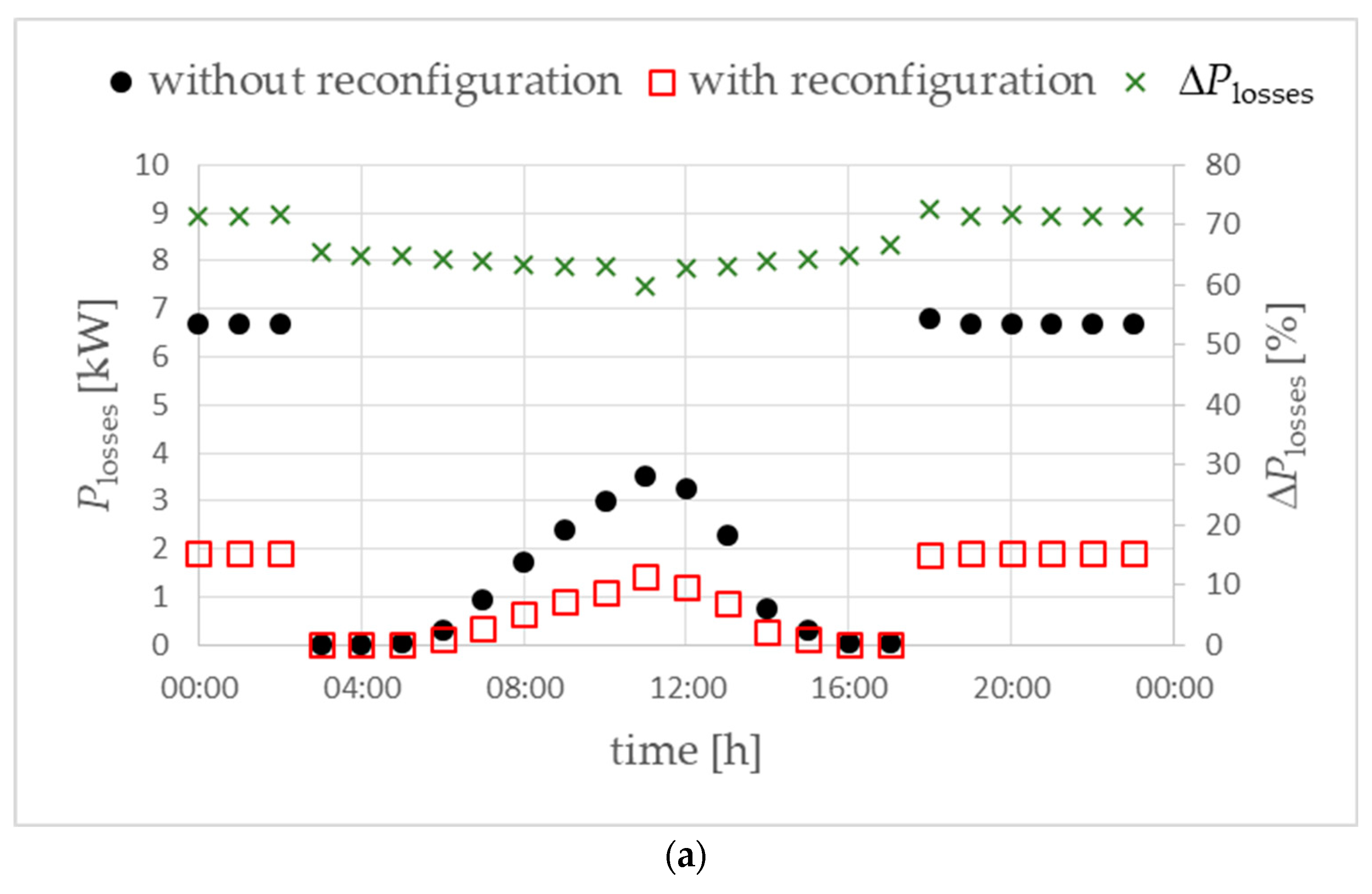
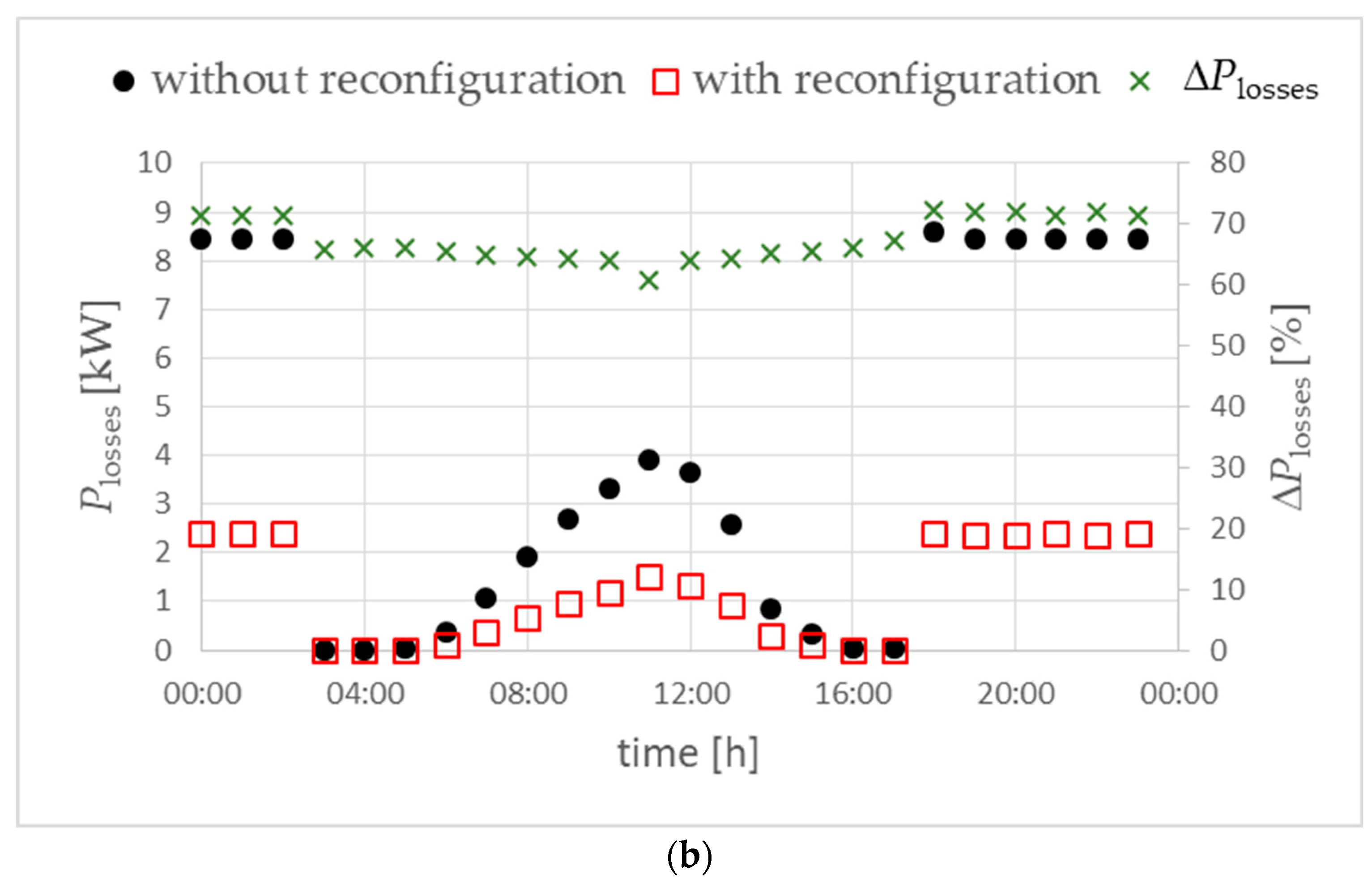
  The minimization of currents flowing in neutral conductors in the MV/LV distribution substation (mark: DMCR-Mis)—objective function (scenario) no. 4—is as follows.

K_I(DMCR-Mis) = min{I_s,_i} for I_j ≤ I_z and V_w ≤ V_max and V_w ≥ V_min,

(4)

where symbols are the same as those explained in (1), (2), and (3).

Calculations for a given objective function were made iteratively with the use of DigSILENT PowerFactory 2022 software using an author’s algorithm automating this process.

The analyses also use the base scenario, which means no phase reconfiguration in the network. It is used to verify the effectiveness of the proposed objective functions.

4. Results

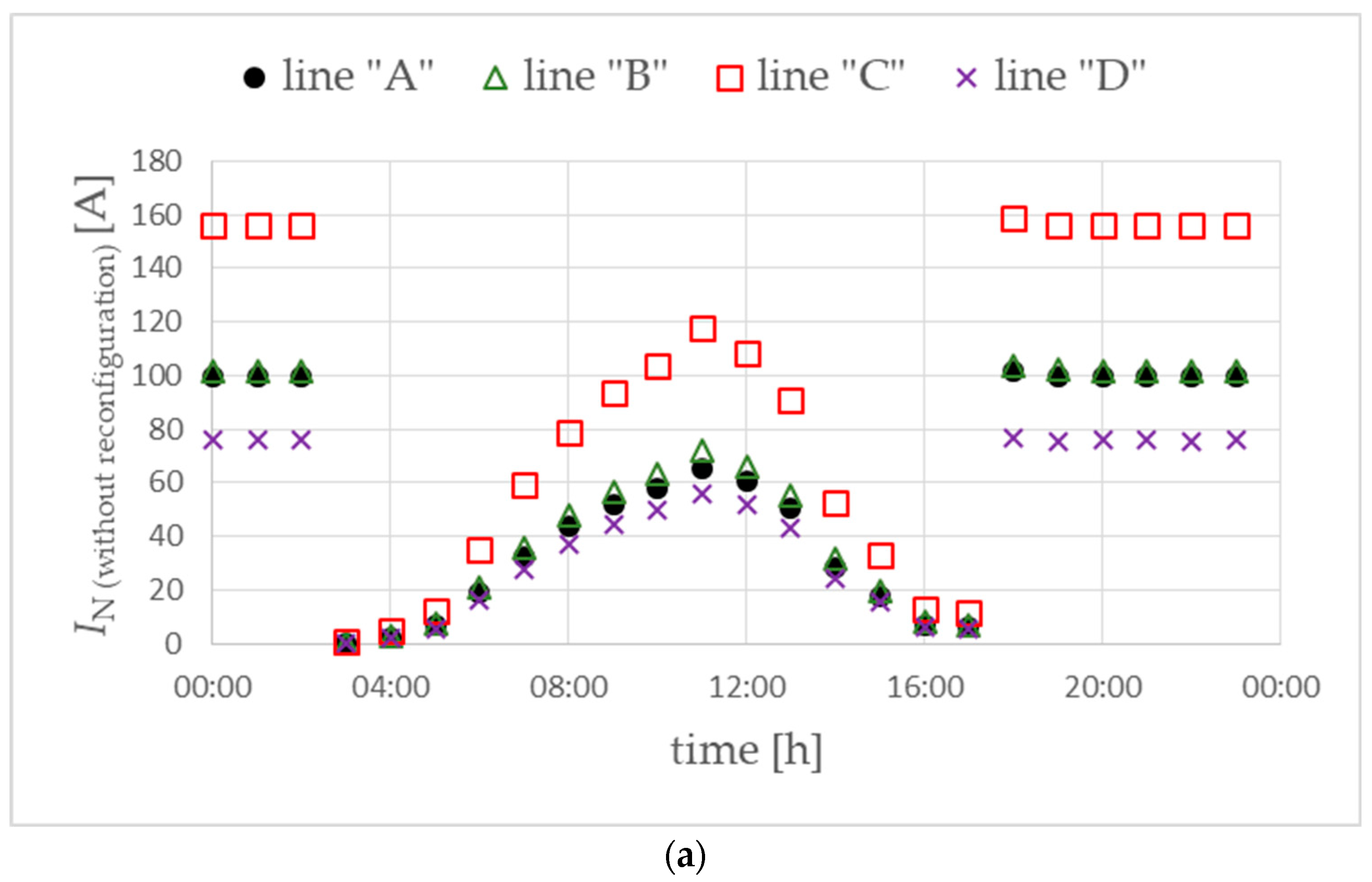
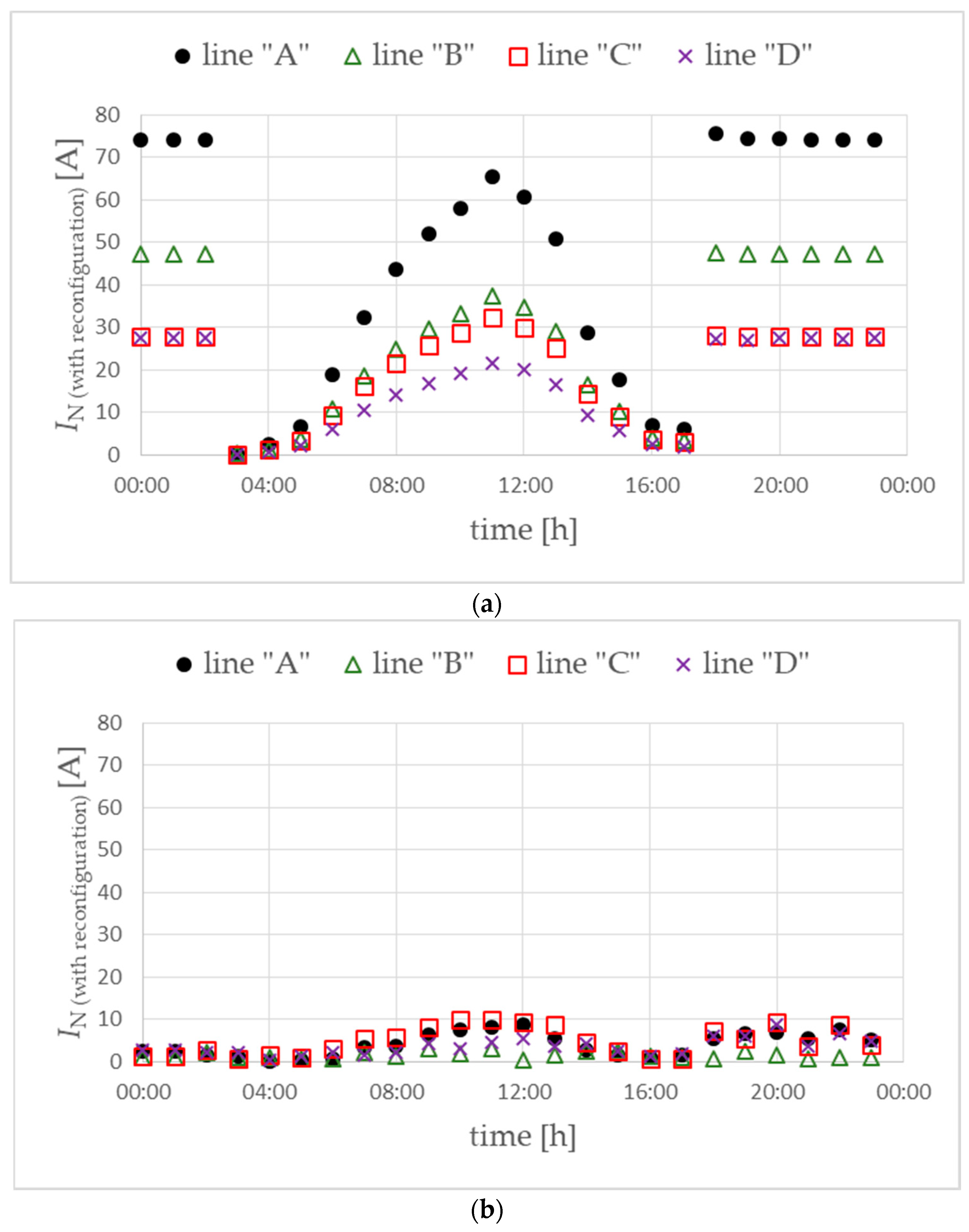
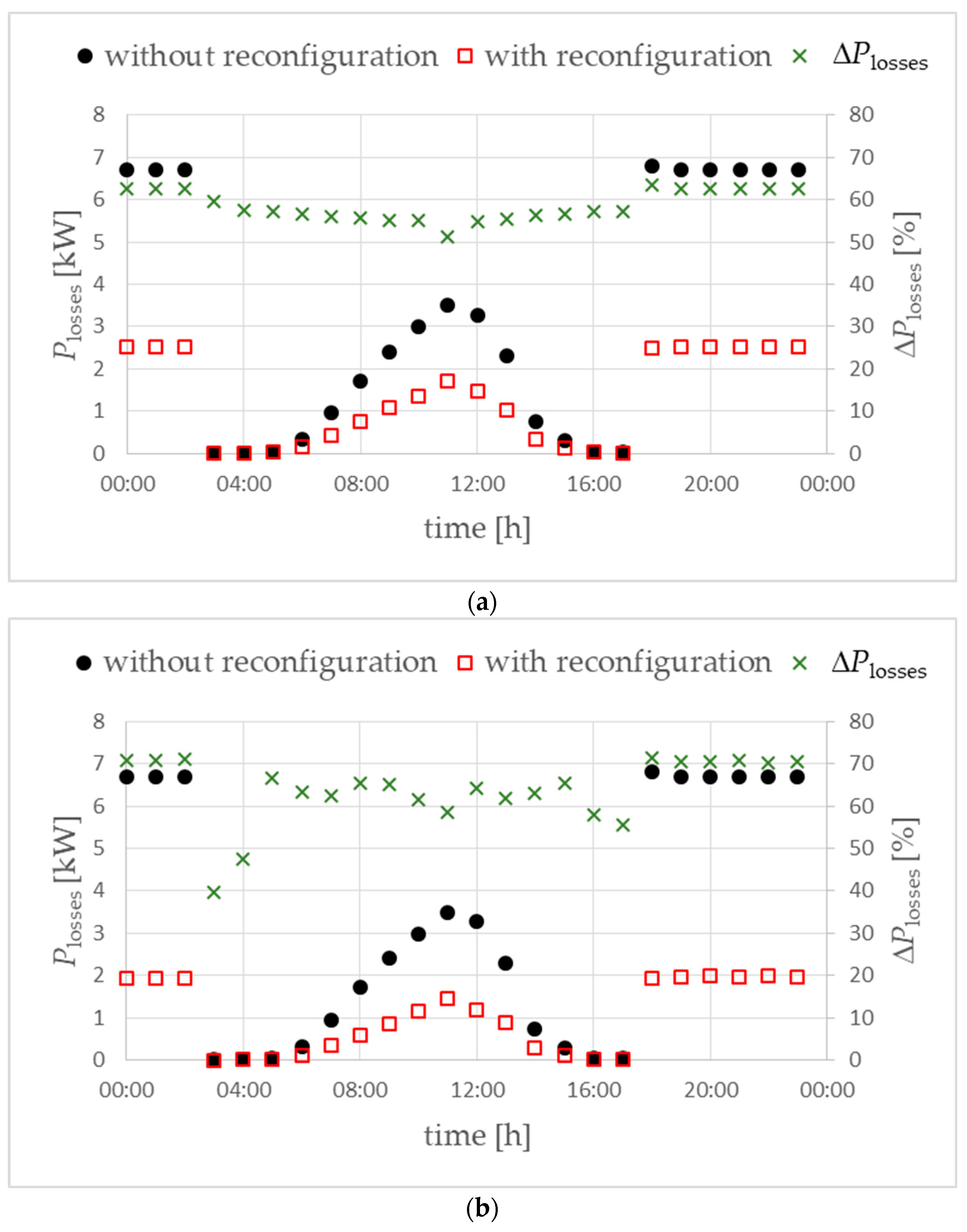
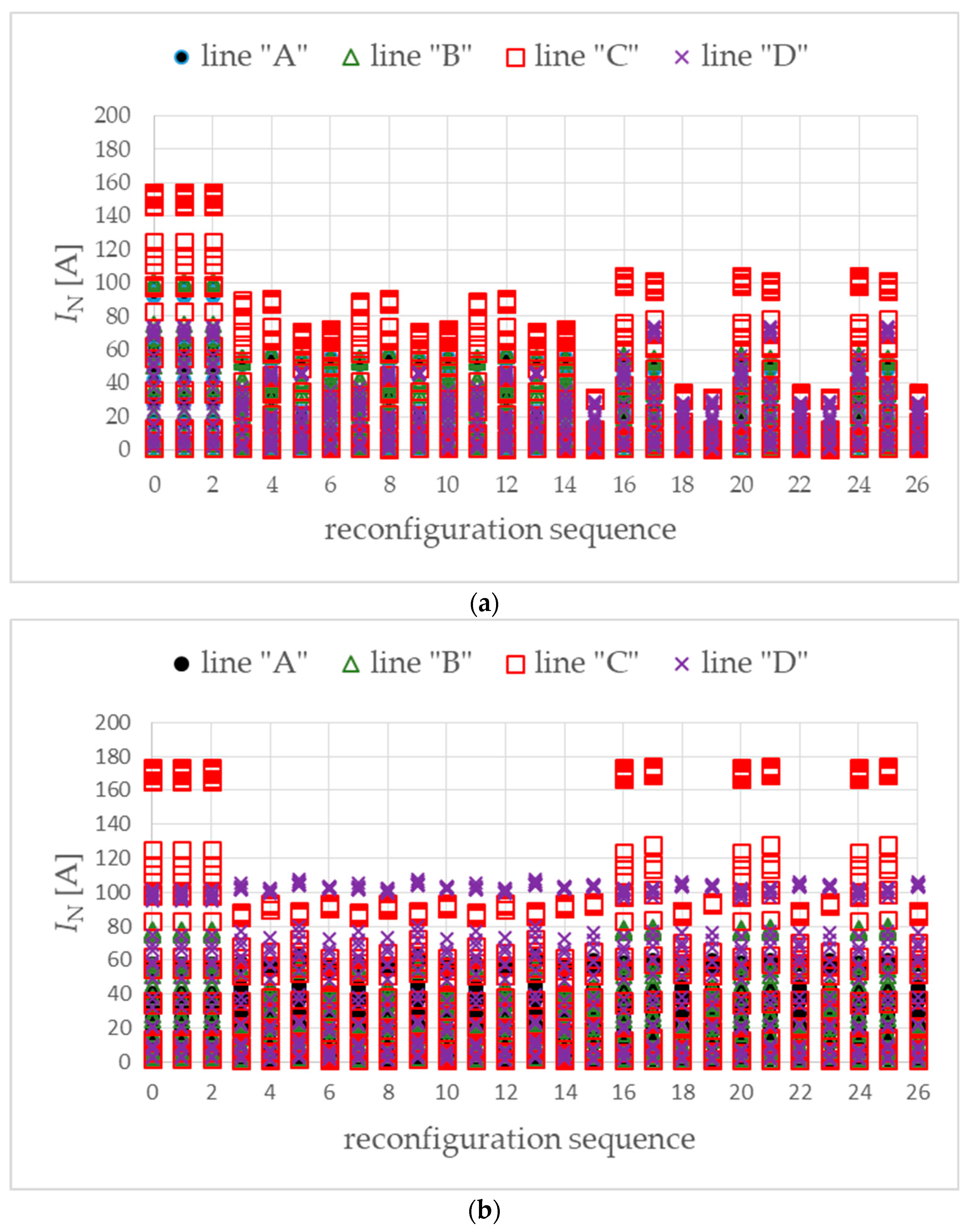
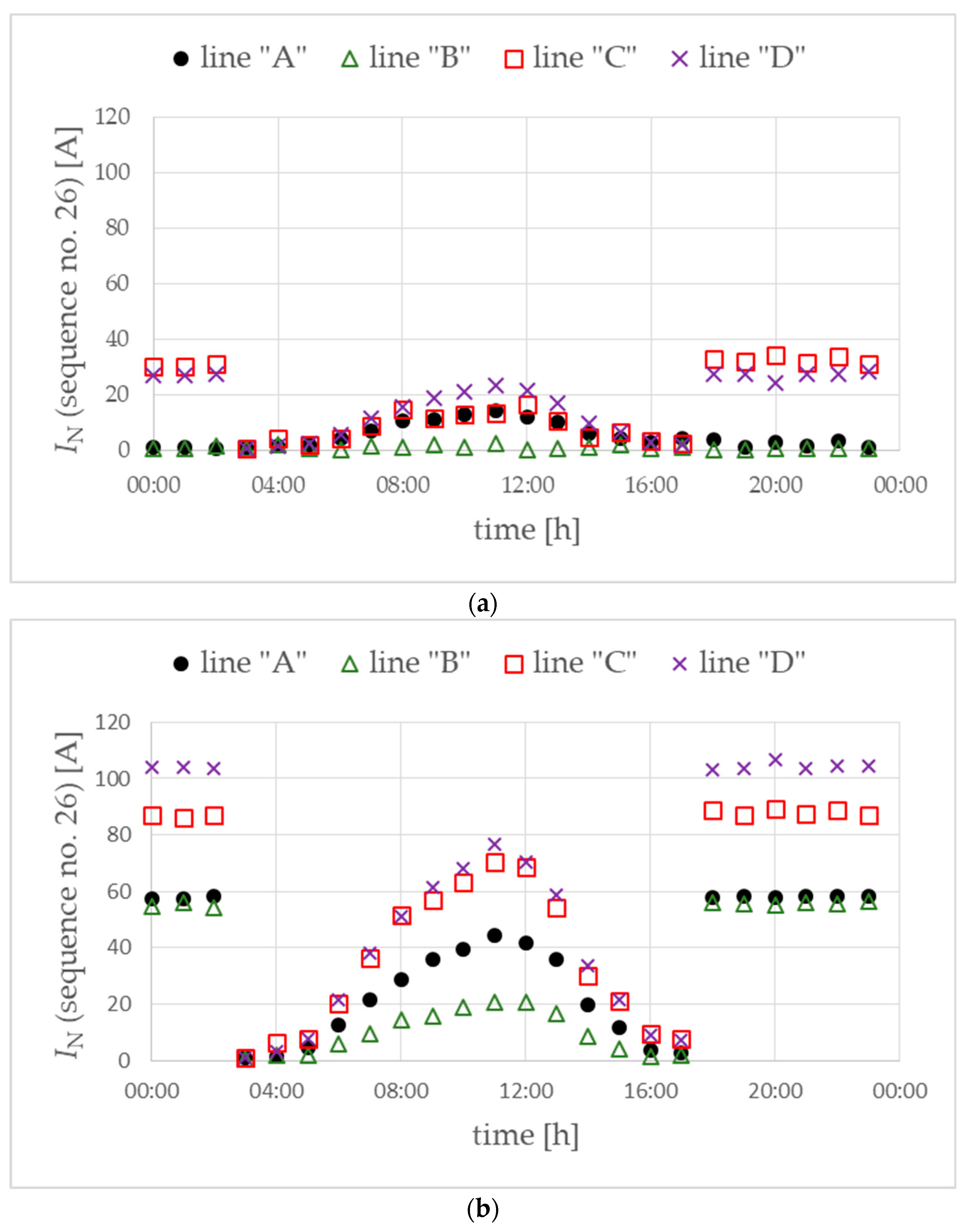
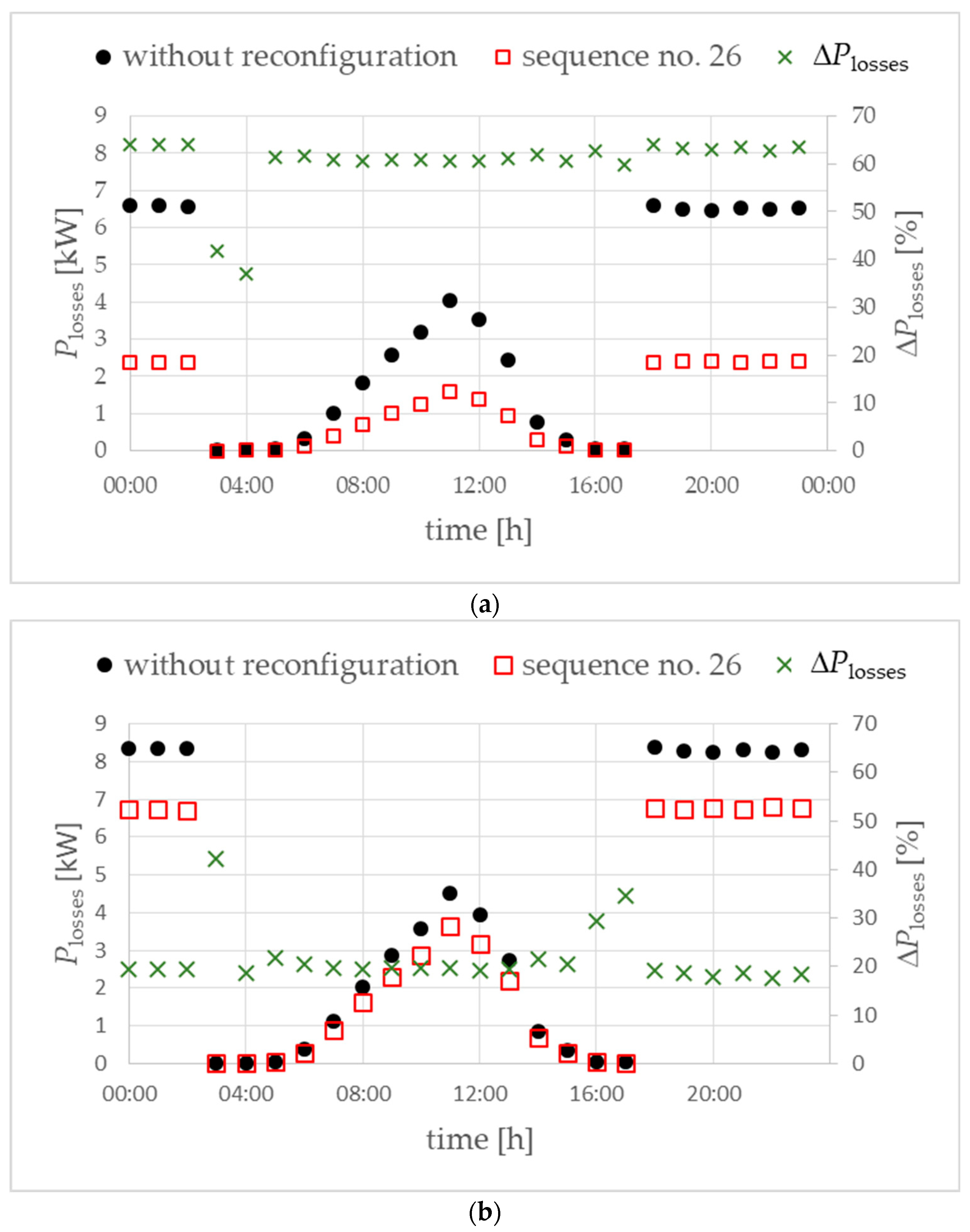
As mentioned above, the analyses assumed two different variants of network operation (W1 and W2), which are also related to the location of prosumers with a PV microsource and an electric vehicle (Figure 4 and Figure 5). In both of these variants, the following connections of PV microsources and Evs are planned as follows:

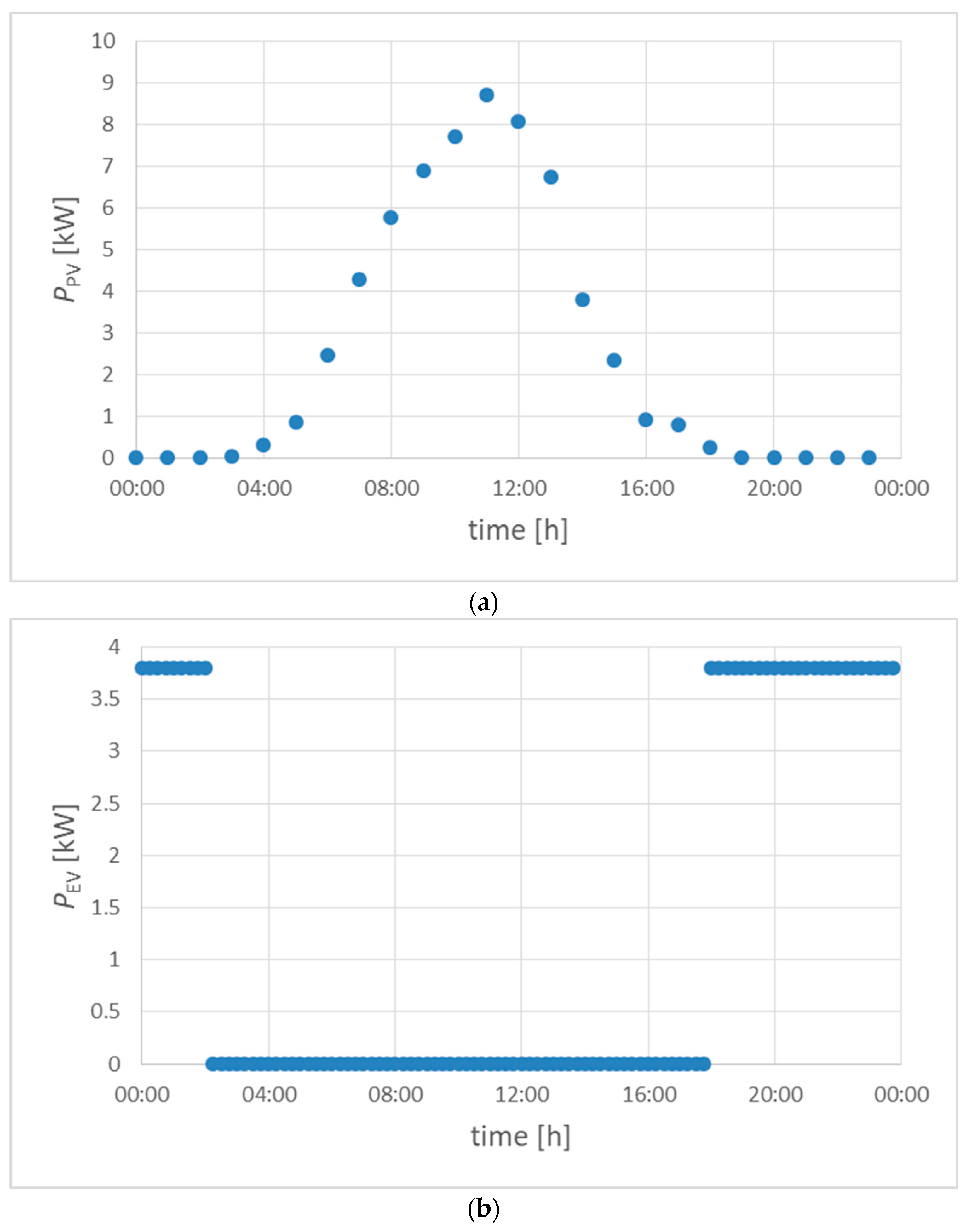
• PV microsources (3.7 kW) are connected to phase L2,
• Evs are charged (3.8 kW) from phase L3.

Each time, phase reconfiguration is carried out as their shift. Therefore, if the phase sequence is L1-L2-L3 at the point of reconfiguration, only sequence L2-L3-L1 or L3-L1-L2 is possible. Thanks to this, problems related to the correct power supply of three-phase drives are avoided in the network.

The following values were adopted as criteria for assessing the phase reconfiguration efficiency:

• Current flowing in the neutral conductor;
• Voltage unbalance factor defined by [48,49]:

K_V = (V₂/V₁) × 100%,

(5)

where V₁ and V₂ are the positive-sequence and negative-sequence voltages, respectively.

The lower the above-mentioned values, the better the effectiveness of a given solution. In addition, power losses P_losses in the network are presented to show the effectiveness of the proposed objective functions.

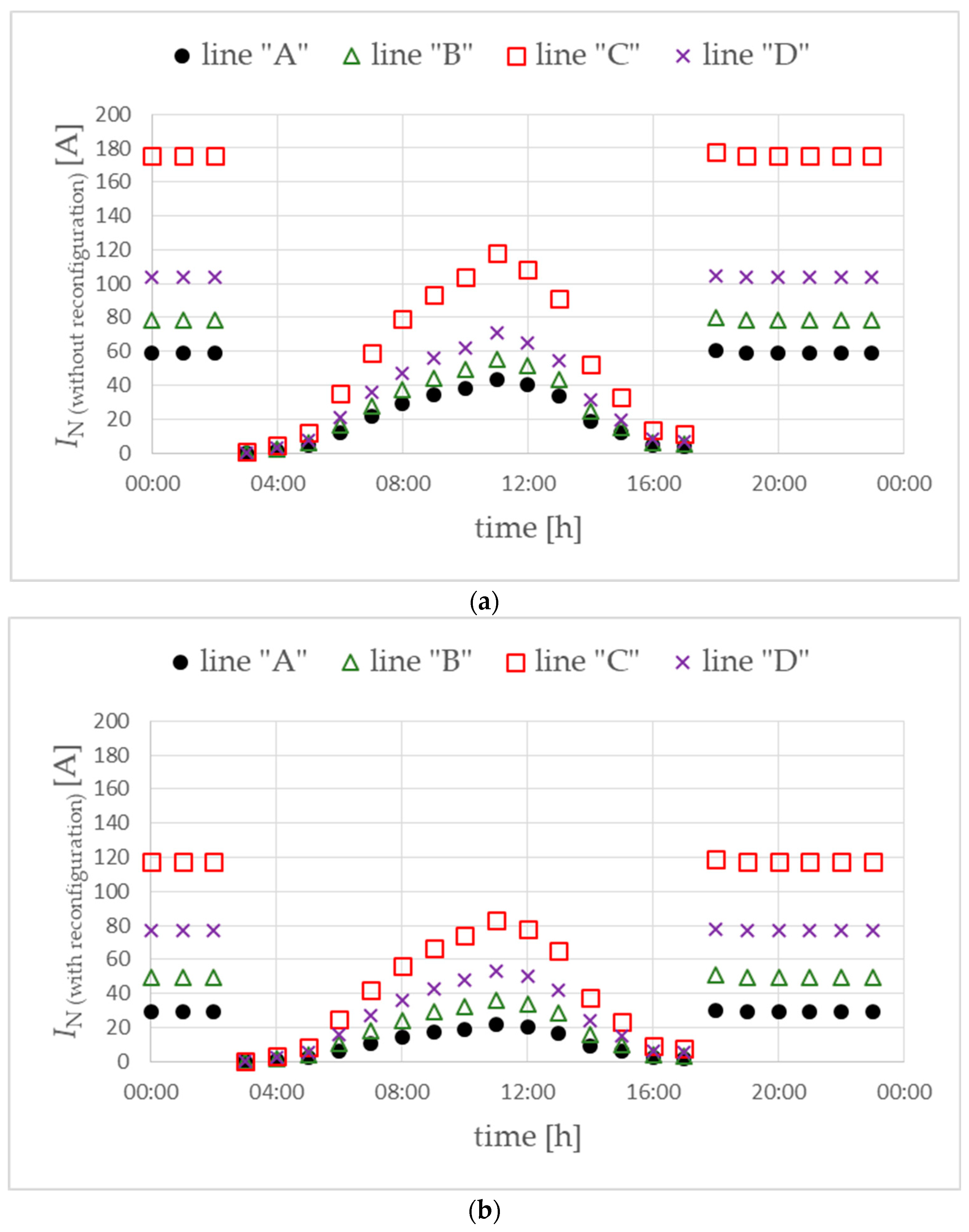
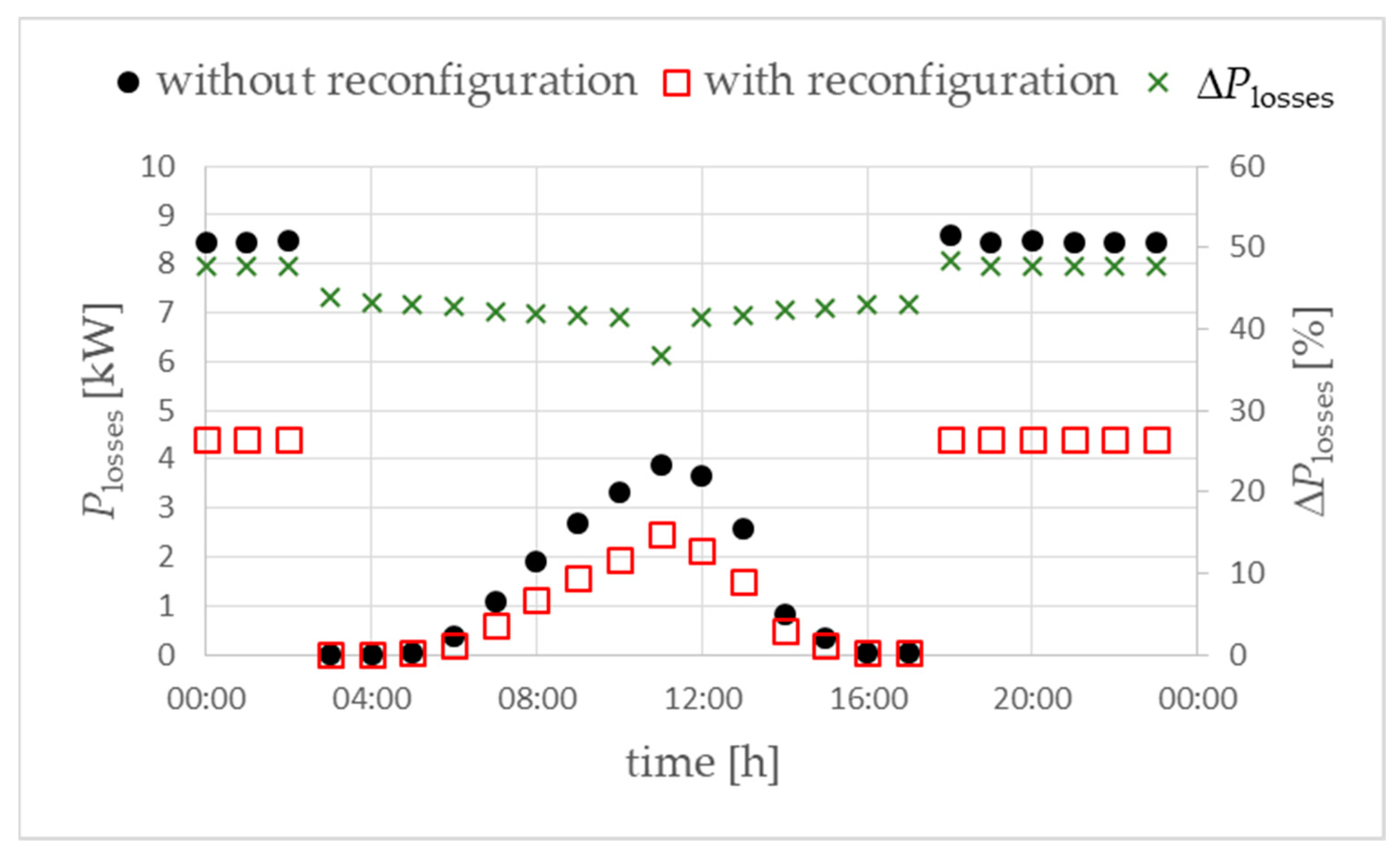
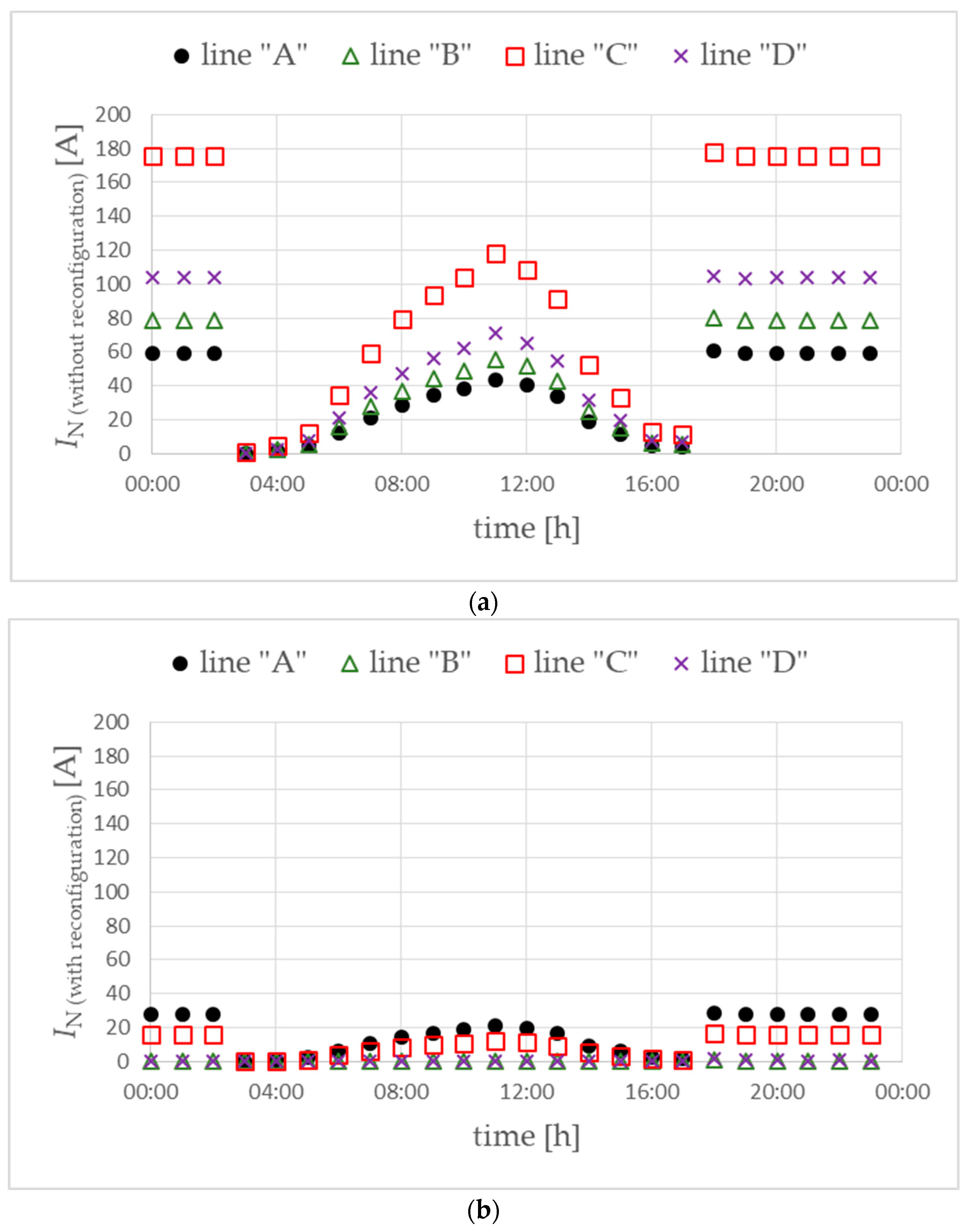
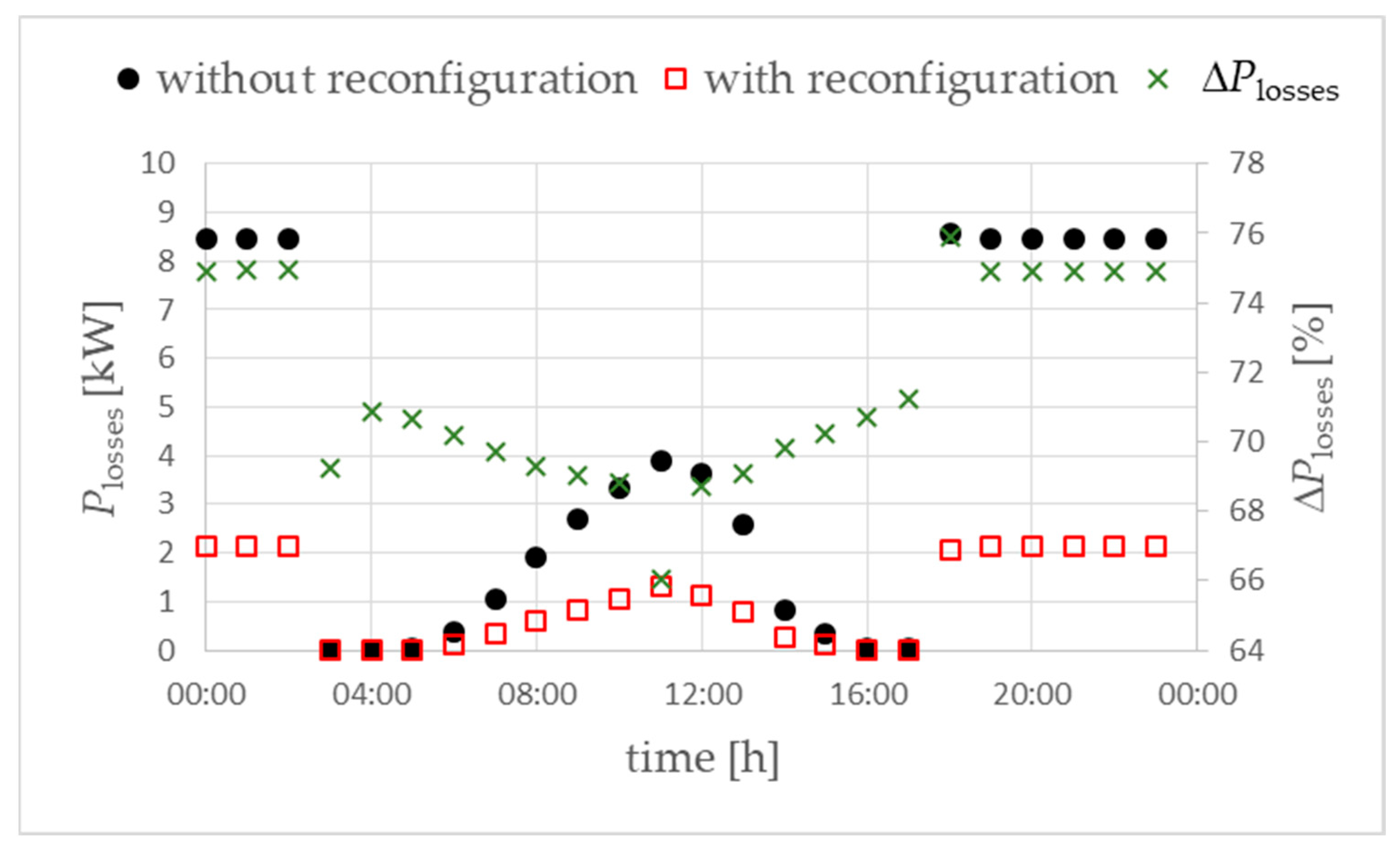
4.1. Objective Function FICR-LVc (No. 1)

The first considered objective function is the minimization of the voltage unbalance factor k_V at the point of installation of phase reconfiguration devices—in this case, at prosumers (FICR LVs). At the same time, it is assumed that these devices operate autonomously. This seems to be the simplest solution among those proposed in this paper. In such a case, phase reconfiguration devices should be sized for power no higher than the maximum contracted load of the prosumer. It is also relatively easy to measure voltages at the place where the device is installed.

In general, phase reconfiguration cannot take place simultaneously in all devices in the network because such action would not have the intended effect. It was, therefore, assumed that reconfiguration took place “sequentially”, starting with the devices closest to the ends of the main power lines (Figure 7).

After phase reconfiguration for prosumers with PV microsources and electric vehicles, a reduction in the value of the voltage unbalance factor k_V was obtained to a level below 1.2% (Figure 8b). This was a significant improvement in relation to the values observed without taking into account phase reconfiguration (Figure 8a—base scenario), in which the permissible value, defined in [48] at the level of 2%, was exceeded. The high values obtained in the base scenario resulted from the assumption of connecting all PV sources to phase L2 and electric vehicle chargers to phase L3.

The use of phase reconfiguration for selected prosumers also reduces the value of currents flowing in the neutral conductors in the main power lines (Figure 9). The lowest effect is observed for the main power line “A” when power is generated by PV sources.

Reducing currents in neutral conductors transfers into lower active power losses in the analyzed network, which, depending on the hour, are reduced by approximately 50–60% (Figure 10).

The obtained results show that the use of an objective function minimizing the voltage unbalance factor at prosumers has a limited impact on reducing current unbalance and, consequently, on reducing power losses in the network.

The above considerations assumed the order of operation of phase reconfiguration devices from the end of the main power lines towards the power supply (MV/LV substation). The reverse situation was also considered, in which reconfiguration started from the MV/LV substation towards the end of the main power lines. In this case, much better results were obtained, i.e., the voltage unbalance factor reached very low values (Figure 11b). This solution also translates into relatively low current values in the neutral conductors (Figure 12b) in the main power lines, measured in the MV/LV substation. At the same time, network power losses are reduced by approximately 65–70% as presented in Figure 13 (in the case of the opposite direction of reconfiguration, it was 50–60%).

Further analyses were carried out for the uneven location of prosumers with a PV microsource and an electric vehicle (variant W2). In this case, phase reconfiguration is assumed at prosumers, starting from the end of the main power lines towards the distribution MV/LV substation. The value of the voltage unbalance factor without phase reconfiguration, i.e., in the base scenario, is slightly higher than for the W1 variant (Figure 14a vs. Figure 8a). This is due to the different locations of prosumers with a PV microsource and an electric vehicle.

The use of phase reconfiguration in selected prosumers reduces the voltage unbalance factor below the permissible value of 2%. However, the values are clearly higher than even the location of prosumers with PVs and EVs (Figure 14b vs. Figure 8b).

Lower efficiency of phase reconfiguration for the LV network configuration in the W2 variant means a lower reduction in the values of neutral conductor currents (Figure 15b vs. Figure 9b) despite higher currents in the base scenario (Figure 15a vs. Figure 9a). As a consequence, a lower reduction in power losses is observed (Figure 16 vs. Figure 10), only by about 40–50%.

The above analyses show that in the case of minimizing the voltage unbalance factor at the point of phase reconfiguration, the direction of reconfiguration is important. A much better effect is achieved if the reconfiguration starts from the distribution MV/LV substation towards the end of the main power lines. In real implementation, a specific order/direction of operation for phase reconfiguration devices can be achieved using different device startup delays.

Moreover, the location of prosumers in the network also affects the achieved efficiency. In general, it can be said that the uneven location of this type of prosumers in the network reduces the efficiency of phase reconfiguration.

4.2. Objective Function FICR-LIc (No. 2)

Another analyzed objective function (FICR-LIc) is the minimization of currents flowing in neutral conductors. For this purpose, phase reconfiguration devices are installed for prosumers that have a photovoltaic microsource and an electric vehicle. This approach requires measuring currents in the neutral conductors at the point where the prosumer is connected to the main power line. However, if more phase reconfiguration devices are connected at one point in the network (points marked in Figure 4 and Figure 5 as “node, cable connector”), this measurement can be common for all devices. This analysis uses the W2 network variant (Figure 5), in which prosumers with a PV microsource and an electric vehicle are located unevenly in the network.

The use of the FICR-LIc objective function allows for a significant reduction in the voltage unbalance factor (Figure 17). At the same time, a clearly better result is obtained here than for the FICR-LVc objective function, the results of which are presented in the previous section (Figure 17b vs. Figure 14b).

In the case under consideration, a significant reduction in the neutral conductor current measured in the distribution MV/LV substation was also achieved (Figure 18). Much lower values were obtained than for the FICR-LVc objective function, in which the voltage unbalance factor was minimized (Figure 18b vs. Figure 15b).

Reducing the neutral conductor currents reduces the active power losses in the analyzed network by approximately 65–75% (Figure 19).

The results presented above for the FICR-LVc and FICR-LIc objective functions show that it matters what parameter is minimized. When using phase reconfiguration devices for selected prosumers, much better efficiency is achieved by minimizing the currents flowing in the neutral conductors than by minimizing the voltage unbalance factor.

4.3. Objective Function FICR-MIs (No. 3)

The FICR-MI objective function minimizes the neutral conductor currents measured in the MV/LV substation using phase reconfiguration devices at prosumers with a PV microsource and an electric vehicle. In such a case, it is necessary to use a main controller in the MV/LV substation and ensure communication between the controller and the phase reconfiguration devices. Considerations were carried out for both variants (W1 and W2) for the location of prosumers with a PV microsource and an electric vehicle. For both variants, a significant reduction in the voltage unbalance factor is obtained (Figure 20). At the same time, slightly lower values are obtained for the W1 variant, in which prosumers with PV and EV are located evenly (Figure 20a).

The effectiveness of minimizing currents in the neutral conductor measured in the MV/LV substation is also observed in the values of these currents (Figure 21). They obtain relatively low values compared to the values obtained without reconfiguration (Figure 21a vs. Figure 9a and Figure 21b vs. Figure 15a).

An effective reduction in the currents flowing in the neutral conductors also results in a significant reduction in active power losses in the analyzed network (Figure 22) by approximately 60–70%.

The above results indicate that the use of phase reconfiguration devices at selected prosumers, combined with the minimization of currents in neutral conductors measured in the MV/LV substation, effectively reduces the unbalance that occurs in the analyzed LV network.

4.4. Objective Function DMCR-MIs (No. 4)

The last scenario considered is the use of the DMCR-MIs objective function to minimize the currents flowing in the neutral conductors in the MV/LV substation (the central point of the network). The objective function is implemented using phase reconfiguration devices installed at selected points of the main power lines. In this case, two variants of the location of prosumers with a PV microsource and an electric vehicle were also considered: variants W1 and W2. The location of the phase reconfiguration devices is shown in Figure 4 or Figure 5. In each main power line, three devices were used in the same places for the W1 and W2 variants. Because of this, it was possible to draw conclusions about the effectiveness of the considered scenario for various network structures.

When using devices installed in main power lines, every possible reconfiguration combination is checked for three devices in each main power line. At the same time, it is assumed that the phase sequence can only occur as follows: L1-L2-L3, L2-L3-L1, or L3-L1-L2. From the above, there are 27 phase combinations in these three devices used in each main power line, assuming that the reconfiguration “0” means no reconfiguration.

Phase reconfiguration devices effectively reduce the voltage unbalance factor for the variant in which prosumers with PVs and EVs are evenly located (Figure 23a). For the uneven location of these prosumers, there is no case that would allow for the voltage unbalance factor to be below the permissible value (Figure 23b).

In the considered approach, for the W1 variant, there are reconfiguration sequences that ensure an effective reduction in both the value of the voltage unbalance factor and the value of the neutral conductor currents (Figure 24a). Such reconfiguration sequences are, for example, no. “15”, “18”, “19”, “22”, “23” and “26”. For the selected reconfiguration sequence (no. “26”), the values of neutral conductor currents during the day are shown in Figure 25.

The use of phase reconfiguration devices in the main power lines while evenly locating the prosumers results in a relatively good reduction in active power losses (Figure 26a). In turn, in the case of the uneven location of prosumers (variant W2), the effect obtained is much lower (Figure 26b).

The above analysis demonstrates a close relationship between the location of prosumers with a high-load unbalance in the network and the effectiveness of the solution with phase reconfiguration devices in the main power lines.

5. Conclusions

This paper considers the possibility of the symmetrization of the LV network using phase reconfiguration devices located either in the prosumer connection points or in the main power lines. These considerations omitted the aspect of the technical solution of the device itself and focused on the effectiveness of using the proposed objective functions and the reconfiguration process itself. The presented analyses allowed us to draw the following conclusions:

• The application of phase reconfiguration in selected prosumers, compared to the reconfiguration in the main power lines, gives a better effect in a network with single-phase PV microsources and electric vehicles, charged using single-phase chargers;
• It is necessary to apply boundary conditions, i.e., the minimum voltage unbalance factor or the minimum I_N current below which the devices do not perform reconfiguration. This can avoid unnecessary (inefficient) reconfiguration;
• Based on the measured values, inferences about the reconfiguration efficiency should be introduced in the decision-making algorithms, which can verify the necessity of reconfiguration. This reduces the number of ineffective reconfigurations;
• Phase reconfiguration devices installed at individual prosumers should be sized in relation to the contracted power. Therefore, these are relatively low-power devices;
• Phase reconfiguration devices installed in main power lines should be sized to supply power to the prosumers “behind” (downstream) the device. If there is more than one device in the main power line, they will have different power ratings. It remains an open issue to determine the expected load, which can be defined, for example, on the basis of the historical loads of prosumers;
• The effectiveness of the operation of phase reconfiguration devices located in the main power lines strongly depends on the location of prosumers with high load unbalance;
• Some of the proposed scenarios require the use of a main controller at the distribution MV/LV substation, as well as communication between the controller and phase reconfiguration devices. This is an additional element increasing the cost of symmetrization in the LV network;
• If the database of historical measurements of prosumers contains measurements of energy, power, or currents independently for each phase, it may facilitate the decision on the appropriateness of using phase reconfiguration devices. Using measurements in a mathematical model would make the decision easier.

Based on the above considerations, it is possible to determine the effectiveness of the objective function and the ease of implementing a given solution. A synthetic comparison is presented in

Table 1. Recommendation of the considered objective functions.

Function	Efficiency	Ease of Implementation	Speed of Calculation
DMCR-MIs	~ 1	+	+
FICR-MIs	+	+	++
FICR-LIc	++	++	++
FICR-LVc (phase reconfiguration from the MV/LV substation to the end of main power lines)	+++	+++	++
FICR-LVc (phase reconfiguration from the end of main power lines to the MV/LV substation)	+	+++	++

¹ “~“ means a non-recommended solution; “+” number means the level of the recommended solution.

.

The comparison from Table 1 shows that for the analyzed real network presented in Figure 4 and Figure 5, the best solution is to use the FICR-LVc objective function with phase reconfiguration from the MV/LV substation to the end of the main power lines.