Integrating Floating Photovoltaics with Hydroelectricity
Abstract
1. Introduction
- -
- development of a modular concept facilitating the involvement of multiple investors in the construction of FPV systems and promotion of the integration of new energy sources into the business opportunities of local communities, fostering sustainable development and renewable energy initiatives;
- -
- integration of FPV into the Orlovac HPP, establishing essential infrastructure for effectively harnessing the energy generated by FPV, with the introduction of flexibility in production scheduling for the Orlovac HPP-FPV Buško Lake system, thus enhancing operational adaptability.
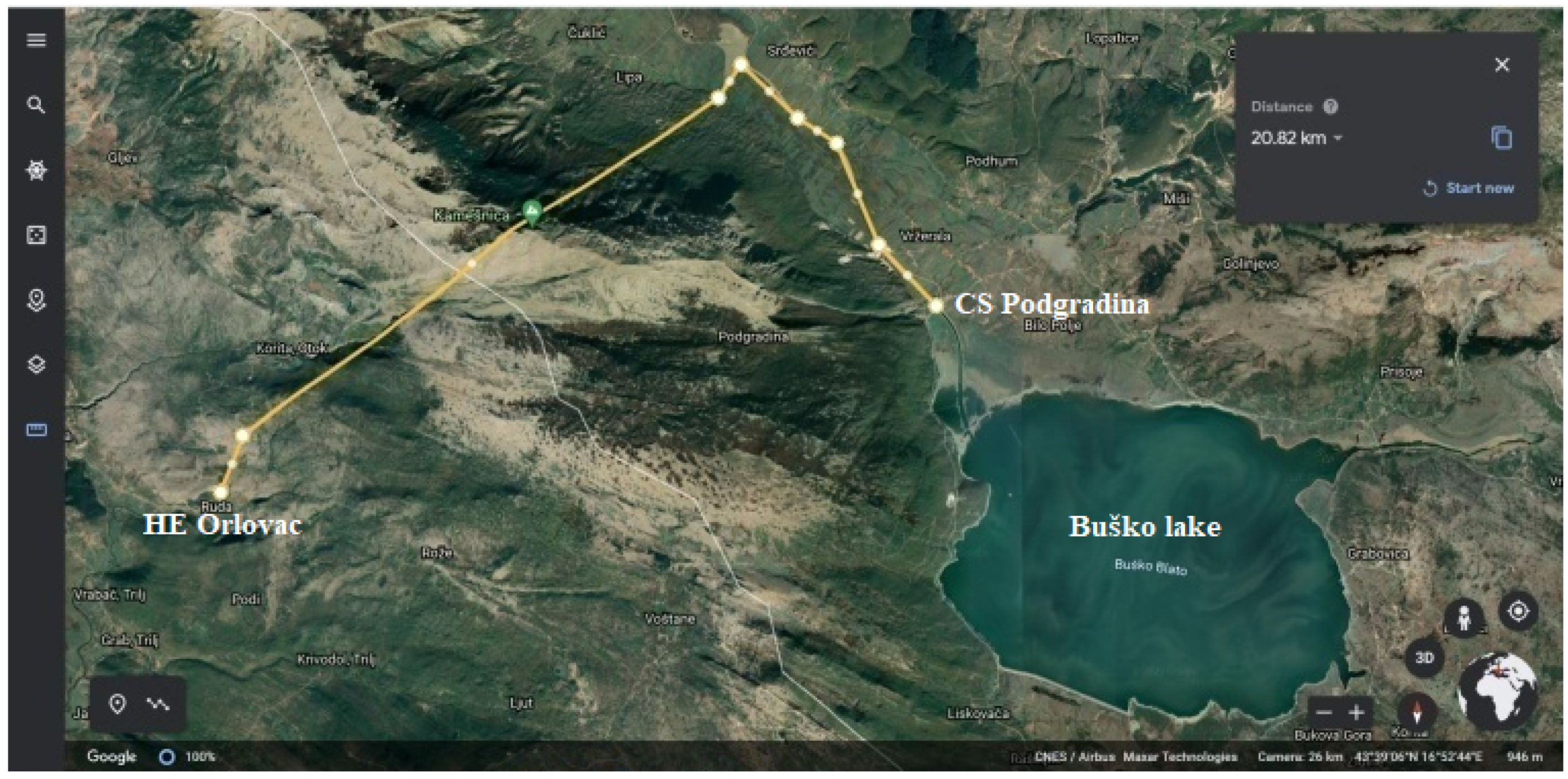
2. System of HPP Orlovac-Buško Lake
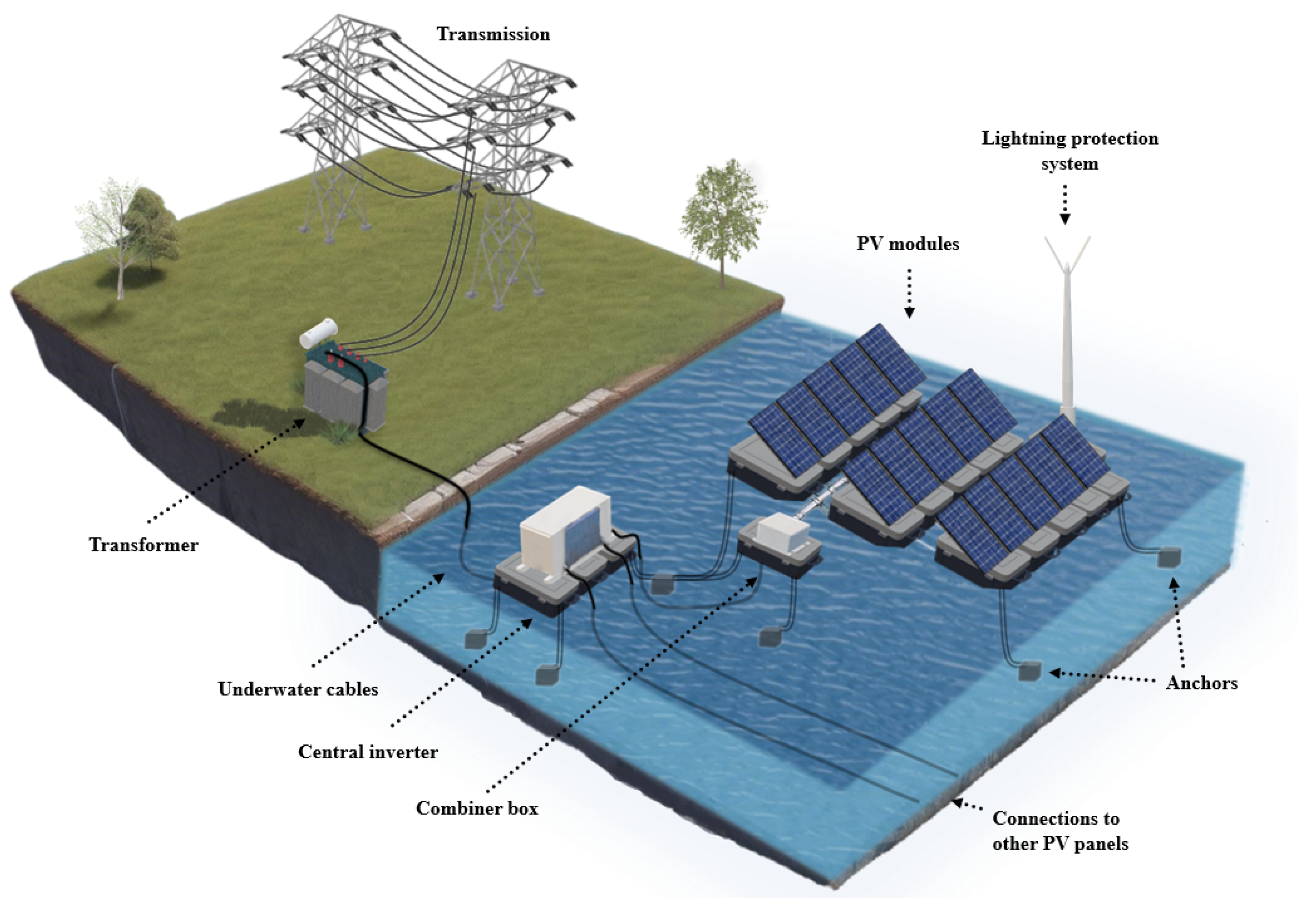
3. Floating Photovoltaic System Design
3.1. Structure of the Basic 3 MW Module
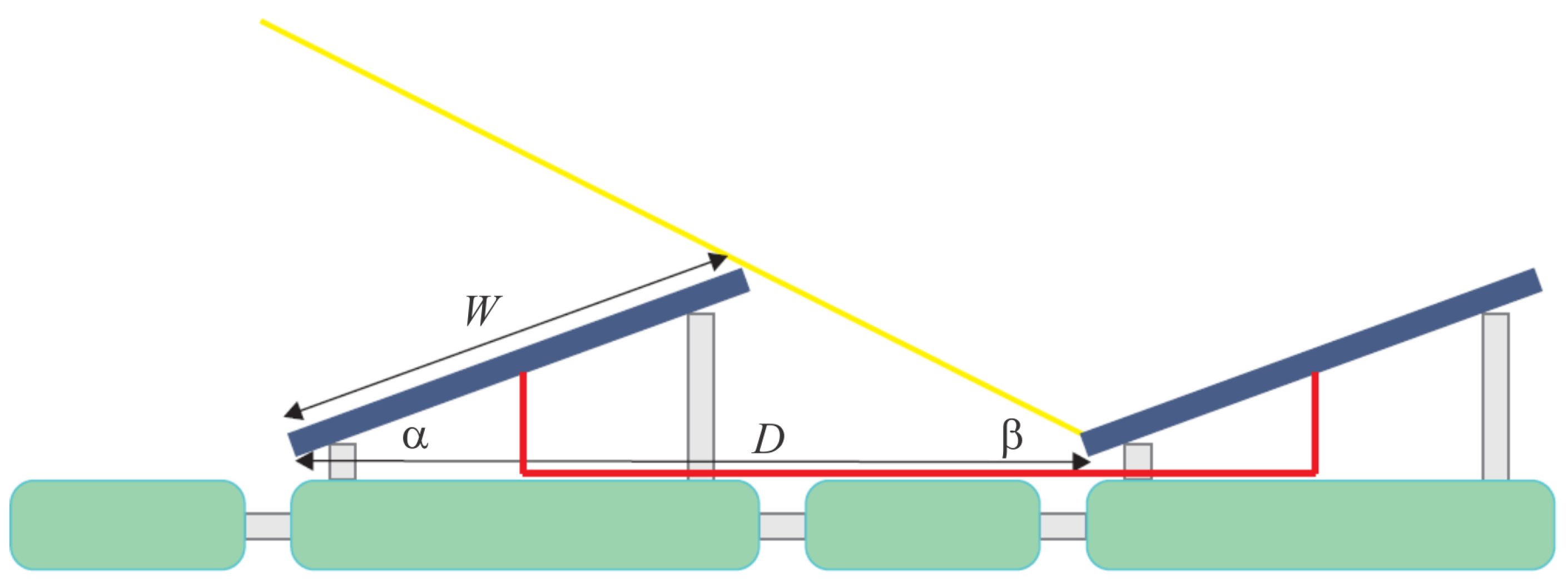
3.2. System Geometry
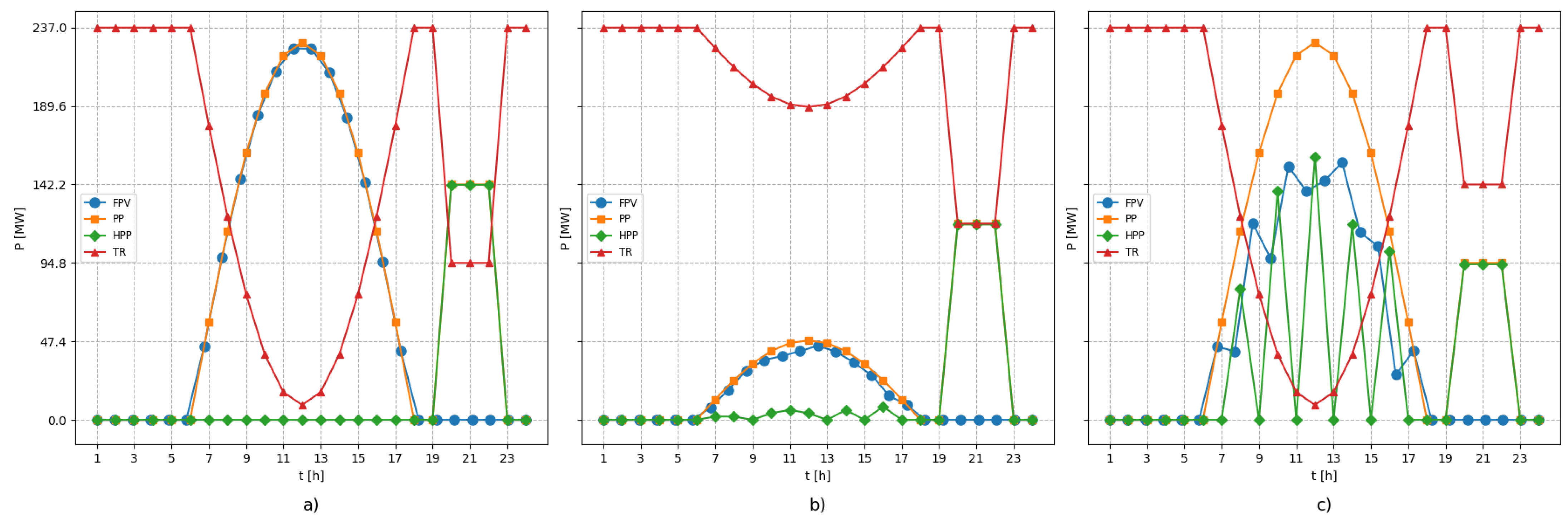
4. Techno-Economic Analysis of the Designed System
- -
- increases production by 91%,
- -
- keeps the maximum production the same, conditioned by the available capacity of the transmission line,
- -
- increases the number of full load working hours (237 MW) to 2852, or by 91.5%,
- -
- achieves excellent predictability compared to standalone FPV, which has poor predictability,
- -
- sees the daily period of available tertiary reserve decrease by 12 h, corresponding to the period when FPV production is expected.
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| FPV | floating photovoltaic |
| PV | photovoltaic |
| HPP | hydroelectric power plant |
| PS | pumping station |
| IRENA | International Renewable Energy Agency |
| PHESS | Pumped Hydroelectric Energy Storage Systems |
| UV | ultra-violet |
| MPPT | maximum power point tracking |
| DC | direct current |
| AC | alternating current |
| BoS | balance of system |
| LCoE | levelized cost of electricity |
| LLC | life cycle cost |
| LCEP | life cycle energy production |
References
- Rahman, S.; de Castro, A. Environmental impacts of electricity generation: A global perspective. IEEE Trans. Energy Convers. 1995, 10, 307–314. [Google Scholar] [CrossRef]
- Wilson, G.M.; Al-Jassim, M.; Metzger, W.K.; Glunz, S.W.; Verlinden, P.; Xiong, G.; Mansfield, L.M.; Stanbery, B.J.; Zhu, K.; Yan, Y.; et al. The 2020 photovoltaic technologies roadmap. J. Phys. Appl. Phys. 2020, 53, 493001. [Google Scholar] [CrossRef]
- Hemeida, M.G.; Hemeida, A.M.; Senjyu, T.; Osheba, D. Renewable Energy Resources Technologies and Life Cycle Assessment: Review. Energies 2022, 15, 9417. [Google Scholar] [CrossRef]
- Hannah, R.; Max, R.; Rosado, P. Renewable Energy, Our World in Data. 2020. Available online: https://ourworldindata.org/renewable-energy (accessed on 15 January 2024).
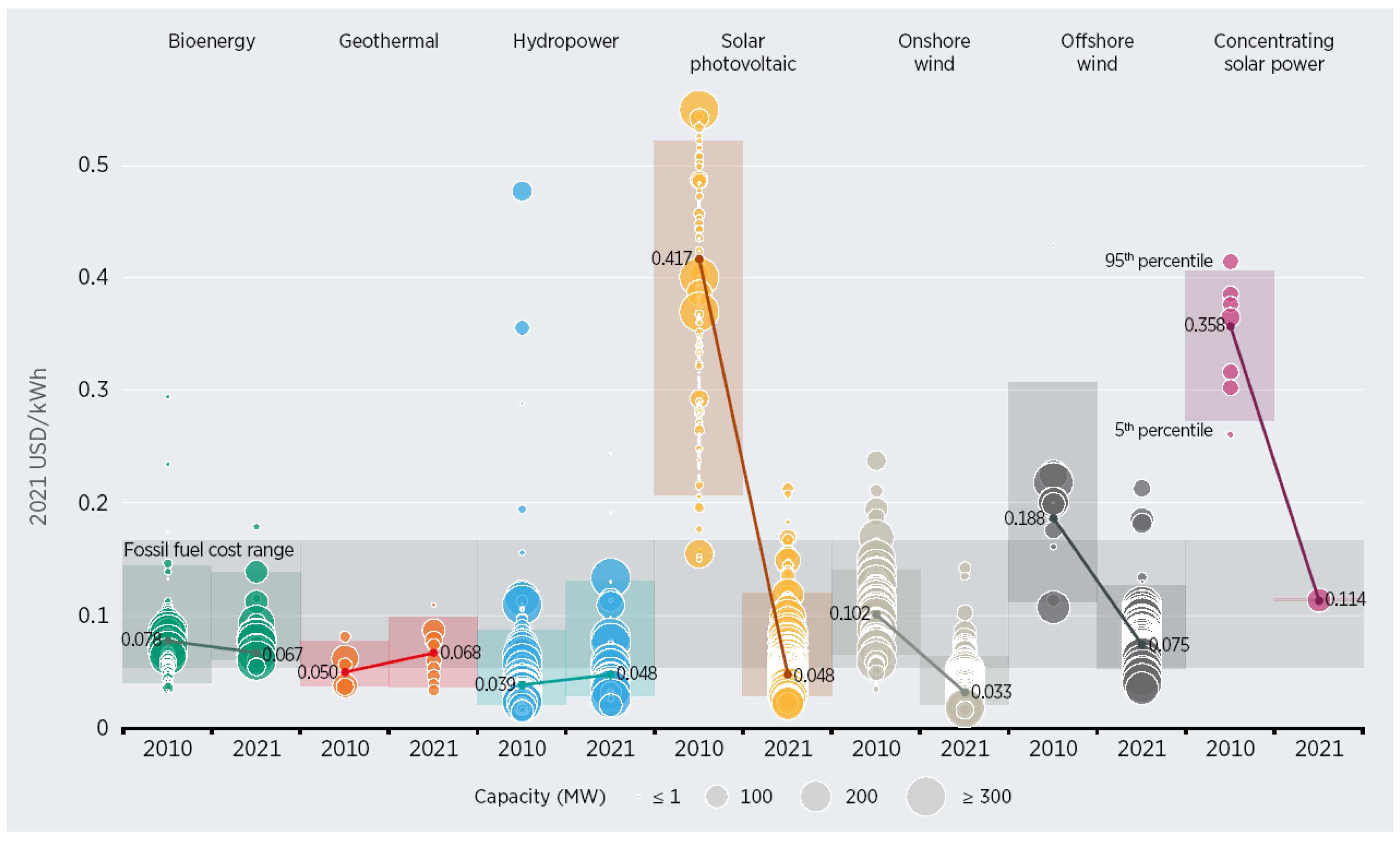
- IRENA. Renewable Power Generation Costs in 2021; International Renewable Energy Agency: Abu Dhabi, United Arab Emirates, 2022. [Google Scholar]
- Pašalić, S.; Akšamović, A.; Avdaković, S. Floating Photovoltaic Plants on Artificial Accumulations — Example of Jablanica Lake. In Proceedings of the 2018 IEEE International Energy Conference (ENERGYCON), Limassol, Cyprus, 3–7 June 2018; pp. 1–6. [Google Scholar] [CrossRef]
- Durković, V.; Đurišić, Ž. Analysis of the Potential for Use of Floating PV Power Plant on the Skadar Lake for Electricity Supply of Aluminium Plant in Montenegro. Energies 2017, 10, 1505. [Google Scholar] [CrossRef]
- Nguyen, N.H.; Le, B.C.; Nguyen, L.N.; Bui, T.T. Technical Analysis of the Large Capacity Grid-Connected Floating Photovoltaic System on the Hydropower Reservoir. Energies 2023, 16, 3780. [Google Scholar] [CrossRef]
- Gorjian, S.; Sharon, H.; Ebadi, H.; Kant, K.; Scavo, F.B.; Tina, G.M. Recent technical advancements, economics and environmental impacts of floating photovoltaic solar energy conversion systems. J. Clean. Prod. 2021, 278, 124285. [Google Scholar] [CrossRef]
- Ramasamy, V.; Margolis, R. Floating Photovoltaic System Cost Benchmark: Q1 2021 Installations on Artificial Water Bodies; National Renewable Energy Lab. (NREL): Golden, CO, USA, 2021. [CrossRef]
- Gadzanku, S.; Mirletz, H.; Lee, N.; Daw, J.; Warren, A. Benefits and Critical Knowledge Gaps in Determining the Role of Floating Photovoltaics in the Energy-Water-Food Nexus. Sustainability 2021, 13, 4317. [Google Scholar] [CrossRef]
- Bošnjaković, M.; Santa, R.; Crnac, Z.; Bošnjaković, T. Environmental Impact of PV Power Systems. Sustainability 2023, 15, 11888. [Google Scholar] [CrossRef]
- Šantić, A.; Aksamovic, A. Photovoltaic plants in Bosnia and Herzegovina — State and perspectives. In Proceedings of the 2018 41st International Convention on Information and Communication Technology, Electronics and Microelectronics (MIPRO), Opatija, Croatia, 21–25 May 2018; pp. 1501–1506. [Google Scholar] [CrossRef]
- Tawalbeh, M.; Al-Othman, A.; Kafiah, F.; Abdelsalam, E.; Almomani, F.; Alkasrawi, M. Environmental impacts of solar photovoltaic systems: A critical review of recent progress and future outlook. Sci. Total Environ. 2021, 759, 143528. [Google Scholar] [CrossRef]
- Abid, M.K.; Kumar, M.V.; Raj, V.A.; Dhas, M.D.K. Environmental Impacts of the Solar Photovoltaic Systems in the Context of Globalization. Ecol. Eng. Environ. Technol. 2023, 24, 231–240. [Google Scholar] [CrossRef]
- Turney, D.; Fthenakis, V. Environmental impacts from the installation and operation of large-scale solar power plants. Renew. Sustain. Energy Rev. 2011, 15, 3261–3270. [Google Scholar] [CrossRef]
- Goldberg, Z.A. Solar energy development on farmland: Three prevalent perspectives of conflict, synergy and compromise in the United States. Energy Res. Soc. Sci. 2023, 101, 103145. [Google Scholar] [CrossRef]
- van de Ven, D.-J.; Capellan-Peréz, I.; Arto, I.; Cazcarro, I.; De Castro, C.; Patel, P.; Gonzalez-Eguino, M. The potential land requirements and related land use change emissions of solar energy. Sci. Rep. 2021, 11, 2907. [Google Scholar] [CrossRef] [PubMed]
- Bevk, T.; Golobič, M. Contentious eye-catchers: Perceptions of landscapes changed by solar power plants in Slovenia. Renew. Energy 2020, 152, 999–1010. [Google Scholar] [CrossRef]
- Vuichard, P.; Stauch, A.; Wüstenhagen, R. Keep it local and low-key: Social acceptance of alpine solar power projects. Renew. Sustain. Energy Rev. 2021, 138, 110516. [Google Scholar] [CrossRef]
- Zhang, P.; Yue, C.; Li, Y.; Tang, X.; Liu, B.; Xu, M.; Wang, M.; Wang, L. Revisiting the land use conflicts between forests and solar farms through energy efficiency. J. Clean. Prod. 2024, 434, 139958. [Google Scholar] [CrossRef]
- Fiorentini, L.; Marmo, L.; Danzi, E.; Puccia, V. Fire risk assessment of photovoltaic plants. A case study moving from two large fires: From accident investigation and forensic engineering to fire risk assessment for reconstruction and permitting purposes. Chem. Eng. Trans. 2016, 48, 427–432. [Google Scholar]
- Ong, N.A.F.M.N.; Sadiq, M.A.; Said, M.S.M.; Jomaas, G.; Tohir, M.Z.M.; Kristensen, J.S. Fault tree analysis of fires on rooftops with photovoltaic systems. J. Build. Eng. 2022, 46, 103752. [Google Scholar]
- Liang, Y.; Kleijn, R.; van der Voet, E. Increase in demand for critical materials under IEA Net-Zero emission by 2050 scenario. Appl. Energy 2023, 346, 121400. [Google Scholar] [CrossRef]
- Carrara, S.; Alves Dias, P.; Plazzotta, B.; Pavel, C. Raw Materials Demand for Wind and Solar PV Technologies in the Transition Towards a Decarbonised Energy System; Publications Office of the European Union: Luxembourg, 2020. [Google Scholar]
- Faircloth, C.C.; Wagner, K.H.; Woodward, K.E.; Rakkwamsuk, P.; Gheewala, S.H. The environmental and economic impacts of photovoltaic waste management in Thailand. Resour. Conserv. Recycl. 2019, 143, 260–272. [Google Scholar] [CrossRef]
- Lunardi, M.M.; Alvarez-Gaitan, J.P.; Bilbao, J.I.; Corkish, R. A review of recycling processes for photovoltaic modules. In Solar Panels and Photovoltaic Materials; InTech: London, UK, 2018; Volume 30. [Google Scholar]
- Isherwood, P.J. Reshaping the module: The path to comprehensive photovoltaic panel recycling. Sustainability 2022, 14, 1676. [Google Scholar] [CrossRef]
- Nkuissi, H.J.T.; Konan, F.K.; Hartiti, B.; Ndjaka, J.M. Toxic materials used in thin film photovoltaics and their impacts on environment. In Reliability and Ecological Aspects of Photovoltaic Modules; IntechOpen: Rijeka, Croatia, 2020. [Google Scholar]
- Keeratimahat, K.; Bruce, A.; MacGill, I. Analysis of short-term operational forecast deviations and controllability of utility-scale photovoltaic plants. Renew. Energy 2021, 167, 343–358. [Google Scholar] [CrossRef]
- Gao, M.; Li, J.; Hong, F.; Long, D. Short-term forecasting of power production in a large-scale photovoltaic plant based on LSTM. Appl. Sci. 2019, 9, 3192. [Google Scholar] [CrossRef]
- Alcañiz, A.; Grzebyk, D.; Ziar, H.; Isabella, O. Trends and gaps in photovoltaic power forecasting with machine learning. Energy Rep. 2023, 9, 447–471. [Google Scholar] [CrossRef]
- Polleux, L.; Guerassimoff, G.; Marmorat, J.P.; Sandoval-Moreno, J.; Schuhler, T. An overview of the challenges of solar power integration in isolated industrial microgrids with reliability constraints. Renew. Sustain. Energy Rev. 2022, 155, 111955. [Google Scholar] [CrossRef]
- Tajjour, S.; Chandel, S.S. Experimental investigation of a novel smart energy management system for performance enhancement of conventional solar photovoltaic microgrids. Discov. Energy 2023, 3, 8. [Google Scholar] [CrossRef]
- Mansouri, N.; Lashab, A.; Guerrero, J.M.; Cherif, A. Photovoltaic power plants in electrical distribution networks: A review on their impact and solutions. IET Renew. Power Gener. 2020, 14, 2114–2125. [Google Scholar] [CrossRef]
- Cerchio, M.; Gullí, F.; Repetto, M.; Sanfilippo, A. Hybrid energy network management: Simulation and optimisation of large scale PV coupled with hydrogen generation. Electronics 2020, 9, 1734. [Google Scholar] [CrossRef]
- Li, W.; Ren, H.; Chen, P.; Wang, Y.; Qi, H. Key operational issues on the integration of large-scale solar power generation—A literature review. Energies 2020, 13, 5951. [Google Scholar] [CrossRef]
- Cazzaniga, R.; Rosa-Clot, M.; Rosa-Clot, P.; Tina, G.M. Integration of PV floating with hydroelectric power plants. Heliyon 2019, 5. [Google Scholar] [CrossRef]
- Vourdoubas, J. Integration of Floating Solar Photovoltaic Systems with Hydropower Plants in Greece. Eur. J. Eng. Technol. Res. 2023, 8, 6–12. [Google Scholar] [CrossRef]
- Farfan, J.; Breyer, C. Combining floating solar photovoltaic power plants and hydropower reservoirs: A virtual battery of great global potential. Energy Procedia 2018, 155, 403–411. [Google Scholar] [CrossRef]
- Silvério, N.M.; Barros, R.M.; Tiago Filho, G.L.; Redón-Santafé, M.; dos Santos, I.F.S.; de Mello Valerio, V.E. Use of floating PV plants for coordinated operation with hydropower plants: Case study of the hydroelectric plants of the São Francisco River basin. Energy Convers. Manag. 2018, 171, 339–349. [Google Scholar] [CrossRef]
- Rauf, H.; Gull, M.S.; Arshad, N. Complementing hydroelectric power with floating solar PV for daytime peak electricity demand. Renew. Energy 2020, 162, 1227–1242. [Google Scholar] [CrossRef]
- Rauf, H.; Gull, M.S.; Arshad, N. Integrating floating solar PV with hydroelectric power plant: Analysis of Ghazi barotha reservoir in Pakistan. Energy Procedia 2019, 158, 816–821. [Google Scholar] [CrossRef]
- Zeidan, M.; Al-soud, M.; Dmour, M.; Alakayleh, Z.; Al-Qawabah, S. Integrating a Solar PV System with Pumped Hydroelectric Storage at the Mutah University of Jordan. Energies 2023, 16, 5769. [Google Scholar] [CrossRef]
- Coban, H.H. Hydropower Planning in Combination with Batteries and Solar Energy. Sustainability 2023, 15, 10002. [Google Scholar] [CrossRef]
- Kakoulaki, G.; Sanchez, R.G.; Amillo, A.G.; Szabó, S.; De Felice, M.; Farinosi, F.; De Felice, L.; Bisselink, B.; Seliger, R.; Kougias, I.; et al. Benefits of pairing floating solar photovoltaics with hydropower reservoirs in Europe. Renew. Sustain. Energy Rev. 2023, 171, 112989. [Google Scholar] [CrossRef]
- Quaranta, E.; Aggidis, G.; Boes, R.M.; Comoglio, C.; De Michele, C.; Patro, E.R.; Georgievskaia, E.; Harby, A.; Kougias, I.; Muntean, S.; et al. Assessing the energy potential of modernizing the European hydropower fleet. Energy Convers. Manag. 2021, 246, 114655. [Google Scholar] [CrossRef]
- Sanchez, R.G.; Kougias, I.; Moner-Girona, M.; Fahl, F.; Jäger-Waldau, A. Assessment of floating solar photovoltaics potential in existing hydropower reservoirs in Africa. Renew. Energy 2021, 169, 687–699. [Google Scholar] [CrossRef]
- Barbón, A.; Rodríguez-Fernández, C.; Bayón, L.; Aparicio-Bermejo, J. Evaluating the Potential of Floating Photovoltaic Plants in Pumped Hydropower Reservoirs in Spain. Electronics 2024, 13, 832. [Google Scholar] [CrossRef]
- Barbón, A.; Gutiérrez, Á.; Bayón, L.; Bayón-Cueli, C.; Aparicio-Bermejo, J. Economic analysis of a pumped hydroelectric storage-integrated floating PV system in the day-ahead Iberian electricity market. Energies 2023, 16, 1705. [Google Scholar] [CrossRef]
- Venturini, P.; Gagliardi, G.G.; Agati, G.; Cedola, L.; Migliarese Caputi, M.V.; Borello, D. Integration of Floating Photovoltaic Panels with an Italian Hydroelectric Power Plant. Energies 2024, 17, 851. [Google Scholar] [CrossRef]
- Eltamaly, A.M. A novel energy storage and demand side management for entire green smart grid system for NEOM city in Saudi Arabia. Energy Storage 2024, 6, e515. [Google Scholar] [CrossRef]
- Goswami, A.; Sadhu, P.; Goswami, U.; Sadhu, P.K. Floating solar power plant for sustainable development: A techno-economic analysis. Environ. Prog. Sustain. Energy 2019, 38, e13268. [Google Scholar] [CrossRef]
- Orlovac Hydroelectric Power Plant. Available online: https://bit.ly/OrlovacProduction (accessed on 22 November 2023).
- Thin Film Solar Panel Mid Clamp/CE. Available online: https://bit.ly/SICSOLAR (accessed on 16 November 2023).
- Floating Solar a Bold and Innovative Solution. Available online: https://bit.ly/CieletTerre (accessed on 22 November 2023).
- Sungrow Floating PV. Available online: https://bit.ly/SungrowFPV (accessed on 22 November 2023).
- Sungrow Floating Solar Installation. Available online: https://bit.ly/SungrowFPVinstallation (accessed on 22 November 2023).
- Jinko Solar Panel Tiger 475 W Mono-Facial. Available online: https://bit.ly/3ujuAfN (accessed on 22 November 2023).
- SMA Sunny Tripower 20000TL-3 Phases PV. Available online: https://bit.ly/SMASolar (accessed on 22 November 2023).
- Song, J.; Choi, Y. Analysis of the Potential for Use of Floating Photovoltaic Systems on Mine Pit Lakes: Case Study at the Ssangyong Open-Pit Limestone Mine in Korea. Energies 2016, 9, 102. [Google Scholar] [CrossRef]
- Akšamović, A.; Konjicija, S.; Odžak, S.; Pašalić, S.; Grebović, S. DC cabling of large-scale photovoltaic power plants. Appl. Sci. 2022, 12, 4500. [Google Scholar] [CrossRef]
- Akšamović, A.; Odžak, S.; Tihak, A.; Grebović, S.; Konjicija, S. DC cable cross-section selection for PV plants. In Journal of Physics: Conference Series; IOP Publishing: Bristol, UK, 2022; Volume 2339, p. 012001. [Google Scholar]
- 4 mm Single Core TUV H1Z2Z2-K Solar Cable For Solar Panel and Inverter. Available online: https://bit.ly/TUVH1Z2Z2-K (accessed on 16 November 2023).
- H07BN4-F (6381TQ) EN 50525-2-21 Flexible Rubber Cable. Available online: https://www.elandcables.com/media/38328/h07bn4-f-6381tq-en-50525-2-21-flexible-rubber-cable.pdf (accessed on 16 November 2023).
- Acharya, M.; Devraj, S. Floating Solar Photovoltaic (FSPV): A Third Pillar to Solar PV Sector; The Energy and Resources Institute: New Delhi, India, 2019. [Google Scholar]
- Aksamovic, A.; Odžak, S.; Fejzić, A. Measurement of the Diffuse Component of Solar Radiation. In Proceedings of the International Conference on Physical Aspects of Environment, Zrenjanin, Serbia, 24–26 August 2023; p. 11. [Google Scholar]
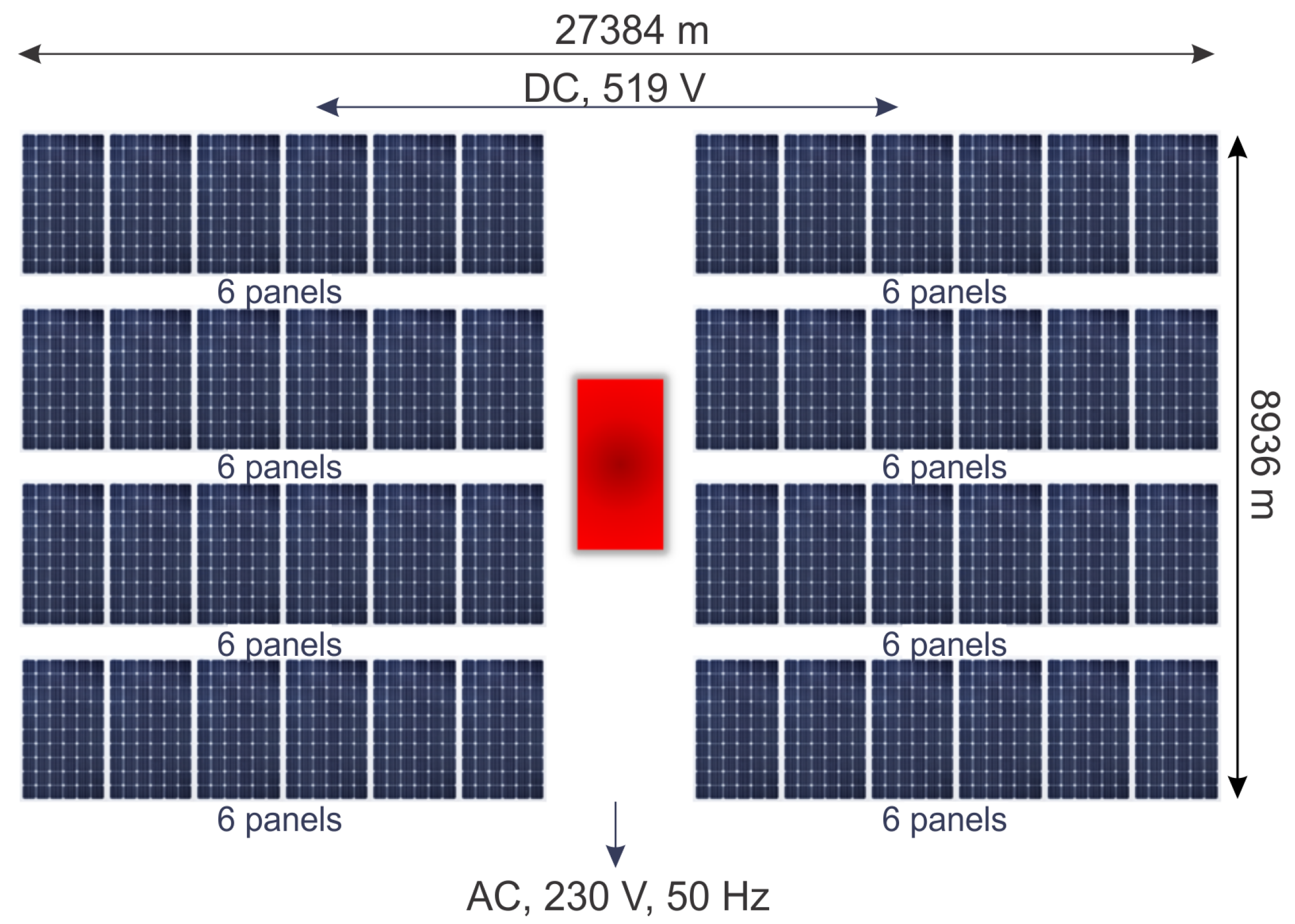
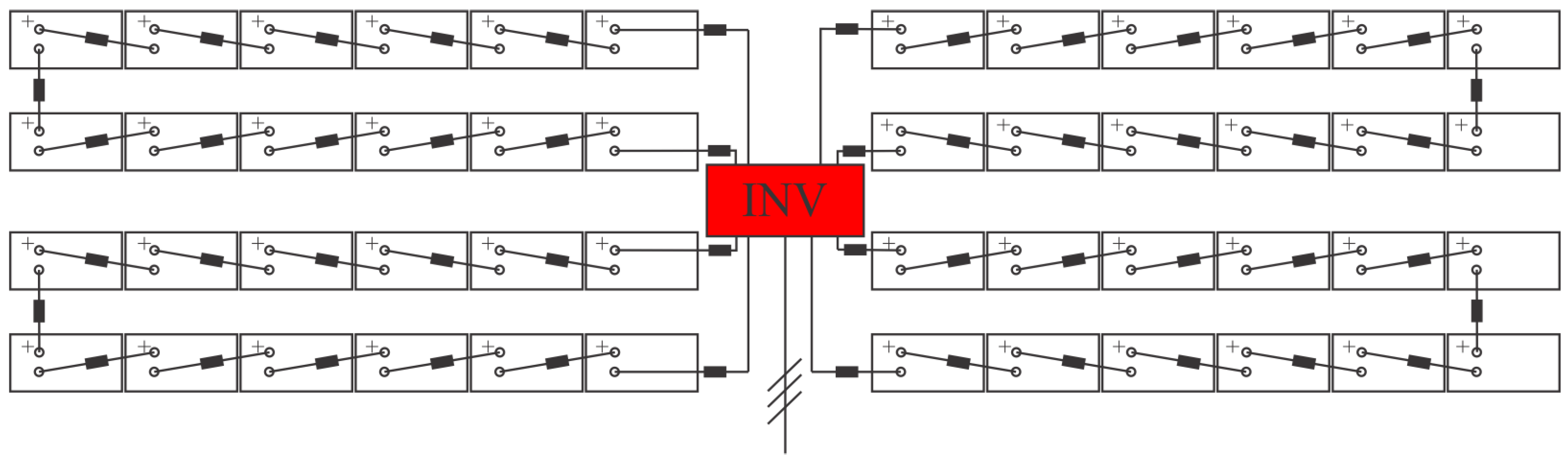
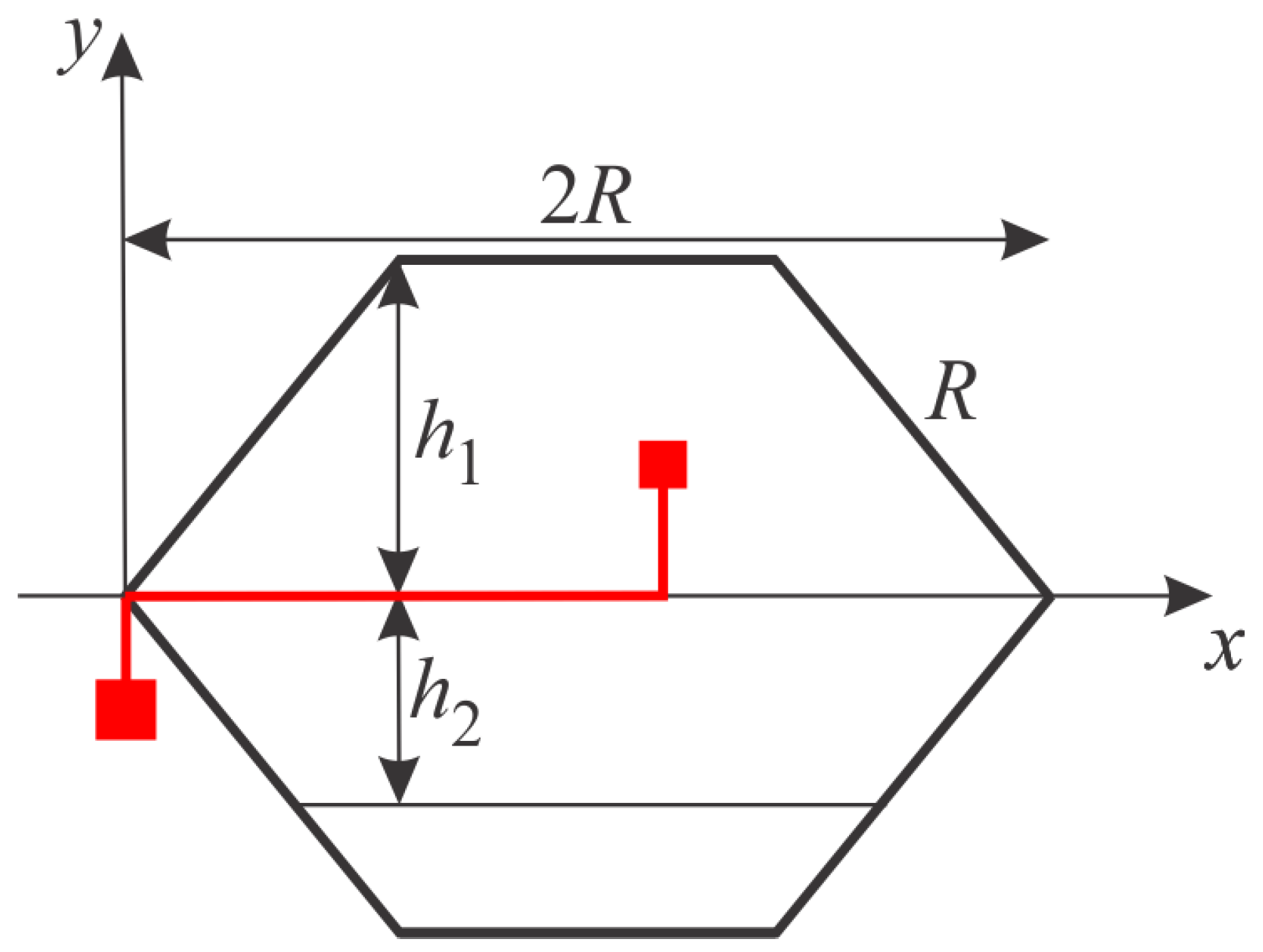
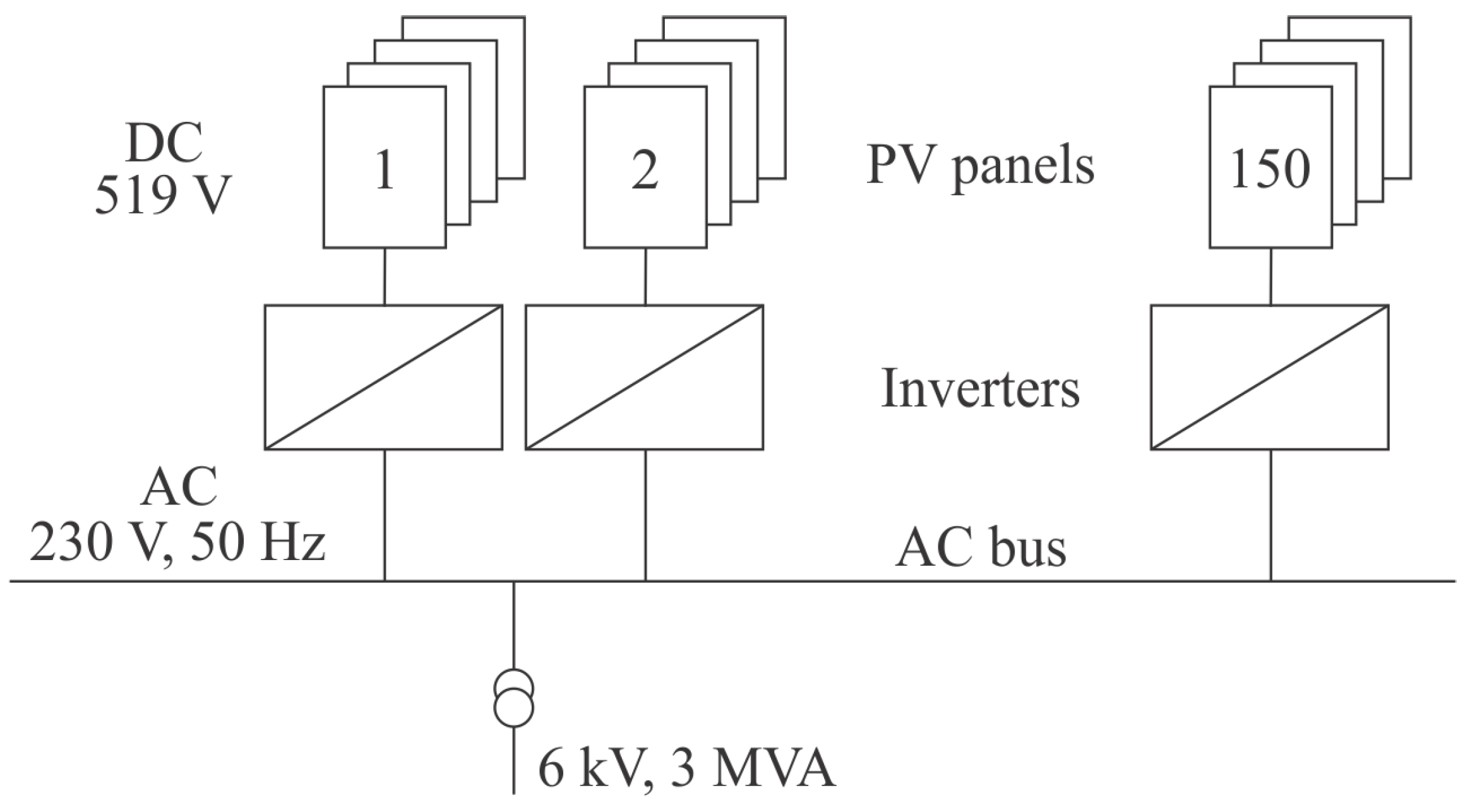
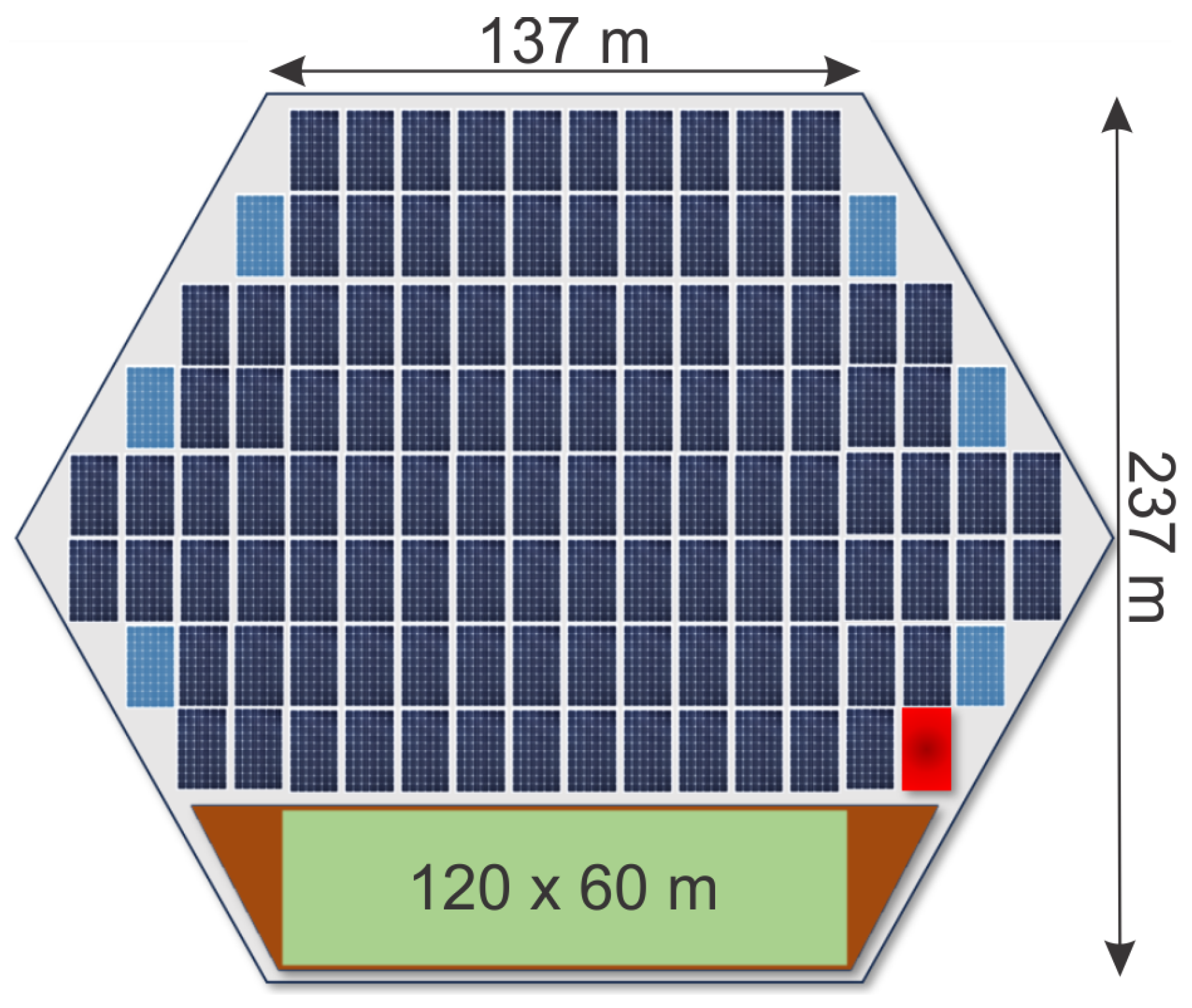
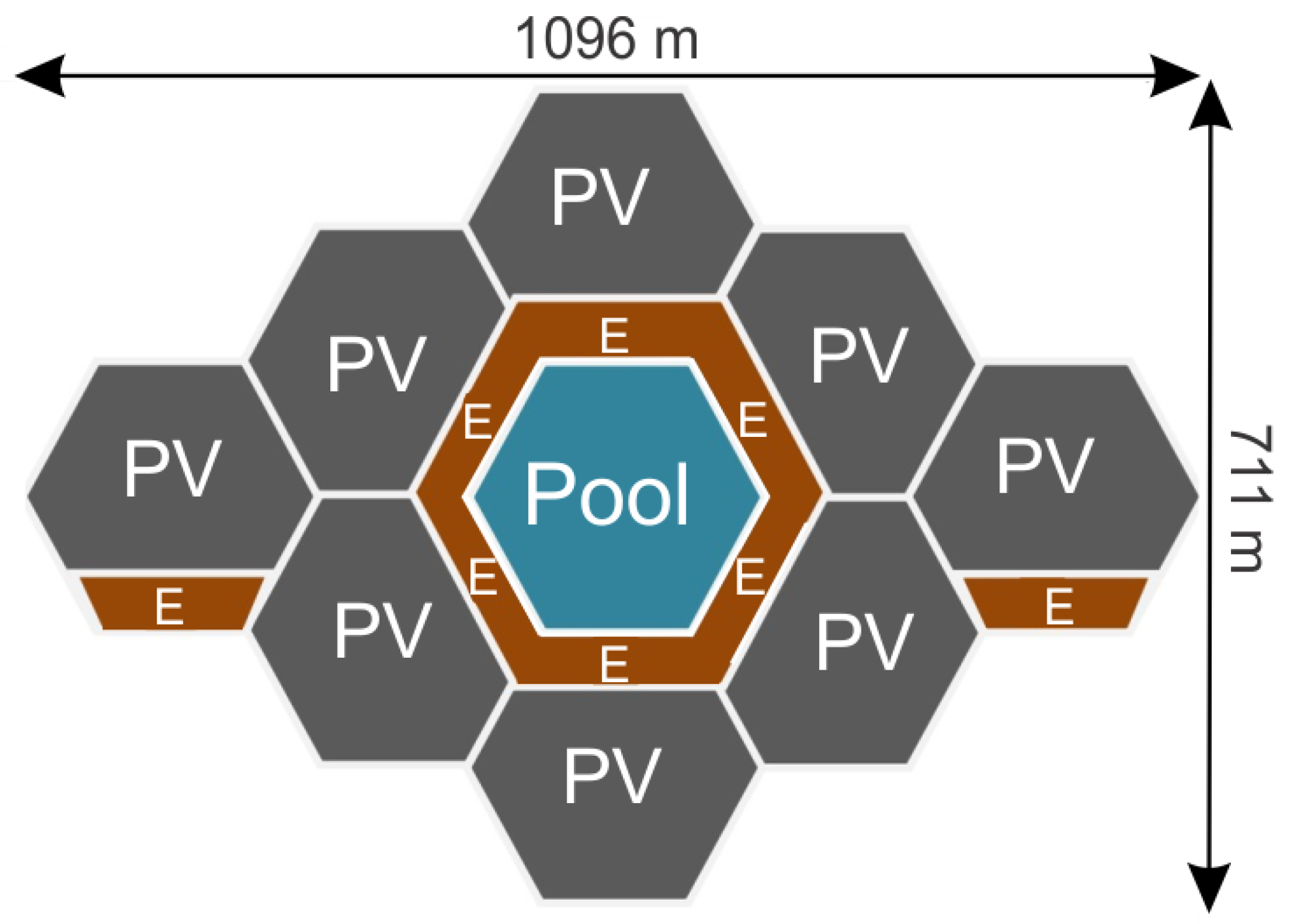


| Parameter | Value |
|---|---|
| Maximum Power () | 470 W |
| Maximum Power Voltage () | V |
| Maximum Power Current () | A |
| Open-circuit Voltage () | V |
| Short-circuit Current () | A |
| Module Efficiency STC (%) | 20.93% |
| Operating Temperature | °C to °C |
| Maximum System Voltage | 1000 V DC |
| Nominal Operating Cell Temperature (NOCT) | 45 °C ± 2 °C |
| Dimensions | mm |
| Mass | 26.1 kg |
| Output Cables | TUV (+): 290 mm (−): 145 mm |
| Price | 155 € |
| Parameter | Value |
|---|---|
| Maximum efficiency | 98.4% |
| DC input voltage up to | 1000 V |
| Power | 20 kW |
| voltage range/rated input voltage | 320 V to 800 V/600 V |
| Max. input current input A/input B | 33 A/33 A |
| Module Efficiency STC (%) | 20.93% |
| Operating Temperature | [ °C, °C] |
| Maximum System Voltage | 1000 V DC |
| Nominal Operating Cell Temperature (NOCT) | 45 °C ± 2 °C |
| Dimensions (W/H/D) | 661/682/264 mm |
| Mass | 61 |
| Operating temperature range | °C to °C |
| Degree of protection | IP65 |
| Price | 2845 € |
| Parameter | Value |
|---|---|
| Cross section | 4 mm2 |
| Rate voltage | 1000 V |
| Rated current | 30 A (4 mm2) |
| Protect degree | IP67 |
| Operating Temperature | °C to °C |
| Contact resistance of plug connectors | ≤5 m |
| Conductor resistance km −1 | 5.09 |
| Price | 0.22 €/m |
| Parameter | Value |
|---|---|
| Cross section | 4 mm2 |
| Rate voltage | 750 V |
| Rated current | 50 A (4 mm2) |
| Protect degree | IP67 |
| Operating Temperature | °C to °C |
| Conductor resistance km −1 | 4.95 |
| Price | 5.02 €/m |
| Parameter | Quantity | Price (€) | Total (€) |
|---|---|---|---|
| Number of inverters | 150 | 2845 | 426,750 |
| Number of panels | 7200 | 155 | 1,116,000 |
| 3 MW | |||
| Area of PV | 36,705 m2 | ||
| Area of E | 7200 m2 | ||
| Total area | 48,162 m2 | ||
| Length | 137 m | ||
| Mass (inverters & panels) | 197,070 kg | ||
| DC cable (1 × 4 mm2) | 21,600 m | 0.22 | 4752 |
| AC cable (4 × 4 mm2) | 28,395.5 m | 5.02 | 142,545.41 |
| Parameter | Value |
|---|---|
| Modules | 35–40% |
| Inverters | 8–12% |
| Wiring | 10–12% |
| Anchoring and mooring | 2% |
| Floating platform | 28–35% |
| BoS (Balance of System) | 4% |
| Assembly | 2% |
| Parameter | Value (€) | Percentage (%) |
|---|---|---|
| Modules | 1,116,000 | 36.0 |
| Inverters | 426,750 | 13.8 |
| Wiring | 350,000 | 11.3 |
| Anchoring and mooring | 65,000 | 2.1 |
| Floating platform | 950,000 | 30.7 |
| BoS (Balance of System) | 125,000 | 4.0 |
| Assembly | 65,000 | 2.1 |
| Total | 3,097,750 | 100.0 |
| Parameter | Standalone FPV | Standalone HPP | Hybrid HPP & FPV |
|---|---|---|---|
| Average annual generation () | 323 | 353 | 676 (+91.5%) |
| Maximal Power () | 240 | 237 | 237 (0%) |
| Annual working hour at maximal power | 1345 | 1489 | 2852 (+91.53%) |
| Possibility of production planning | low | great | great |
| Daily period of available tertiary reserve | 0−0 | 0−24 | 0−6 and 18−24 (−50%) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Madeško, M.; Helać, V.; Fejzić, A.; Konjicija, S.; Akšamović, A.; Grebović, S. Integrating Floating Photovoltaics with Hydroelectricity. Energies 2024, 17, 2760. https://doi.org/10.3390/en17112760
Madeško M, Helać V, Fejzić A, Konjicija S, Akšamović A, Grebović S. Integrating Floating Photovoltaics with Hydroelectricity. Energies. 2024; 17(11):2760. https://doi.org/10.3390/en17112760
Chicago/Turabian StyleMadeško, Mirsad, Vahid Helać, Ajdin Fejzić, Samim Konjicija, Abdulah Akšamović, and Selma Grebović. 2024. "Integrating Floating Photovoltaics with Hydroelectricity" Energies 17, no. 11: 2760. https://doi.org/10.3390/en17112760
APA StyleMadeško, M., Helać, V., Fejzić, A., Konjicija, S., Akšamović, A., & Grebović, S. (2024). Integrating Floating Photovoltaics with Hydroelectricity. Energies, 17(11), 2760. https://doi.org/10.3390/en17112760











