Dynamics and Wake Interference Mechanism of Long Flexible Circular Cylinders in Side-by-Side Arrangements
Abstract
1. Introduction
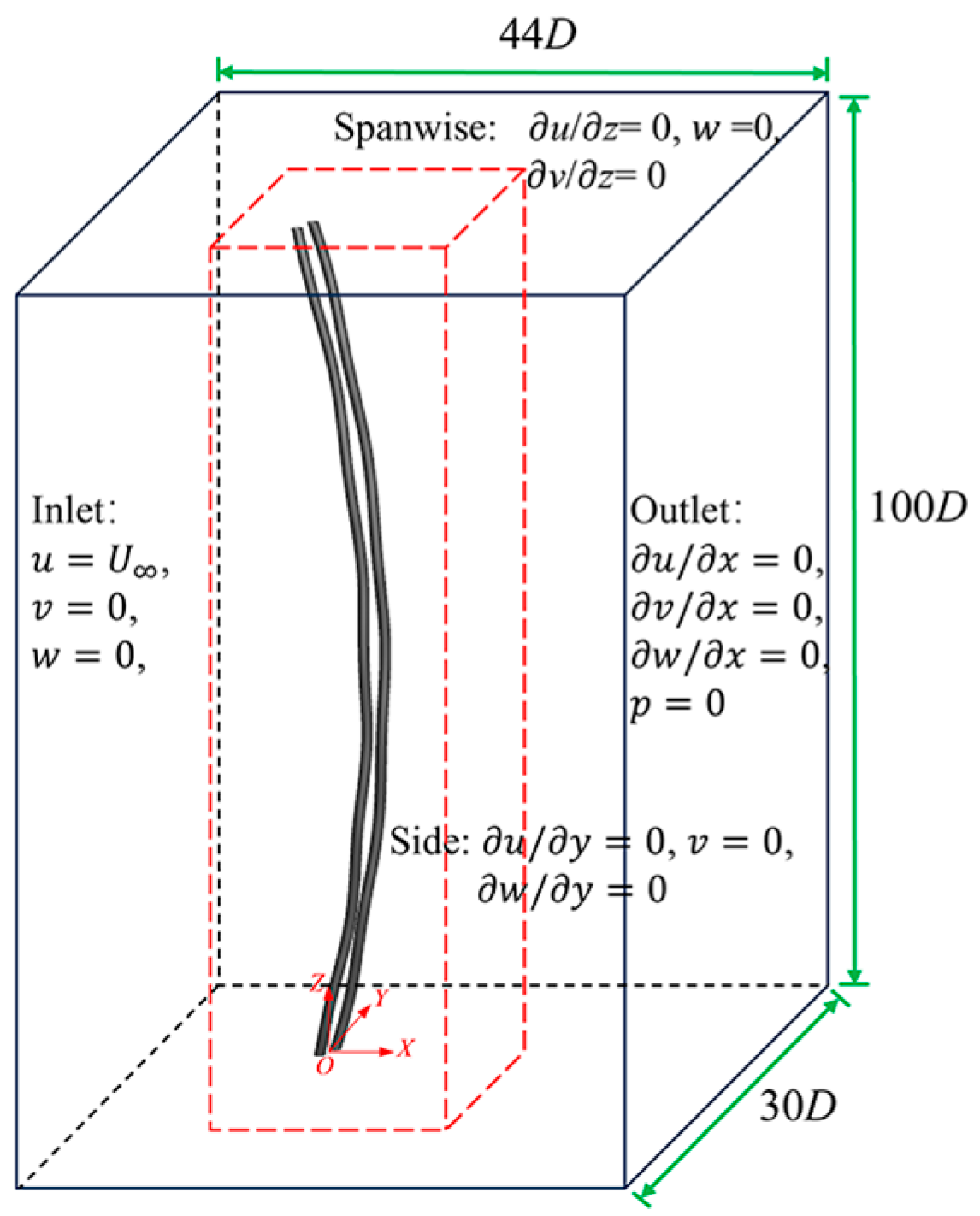
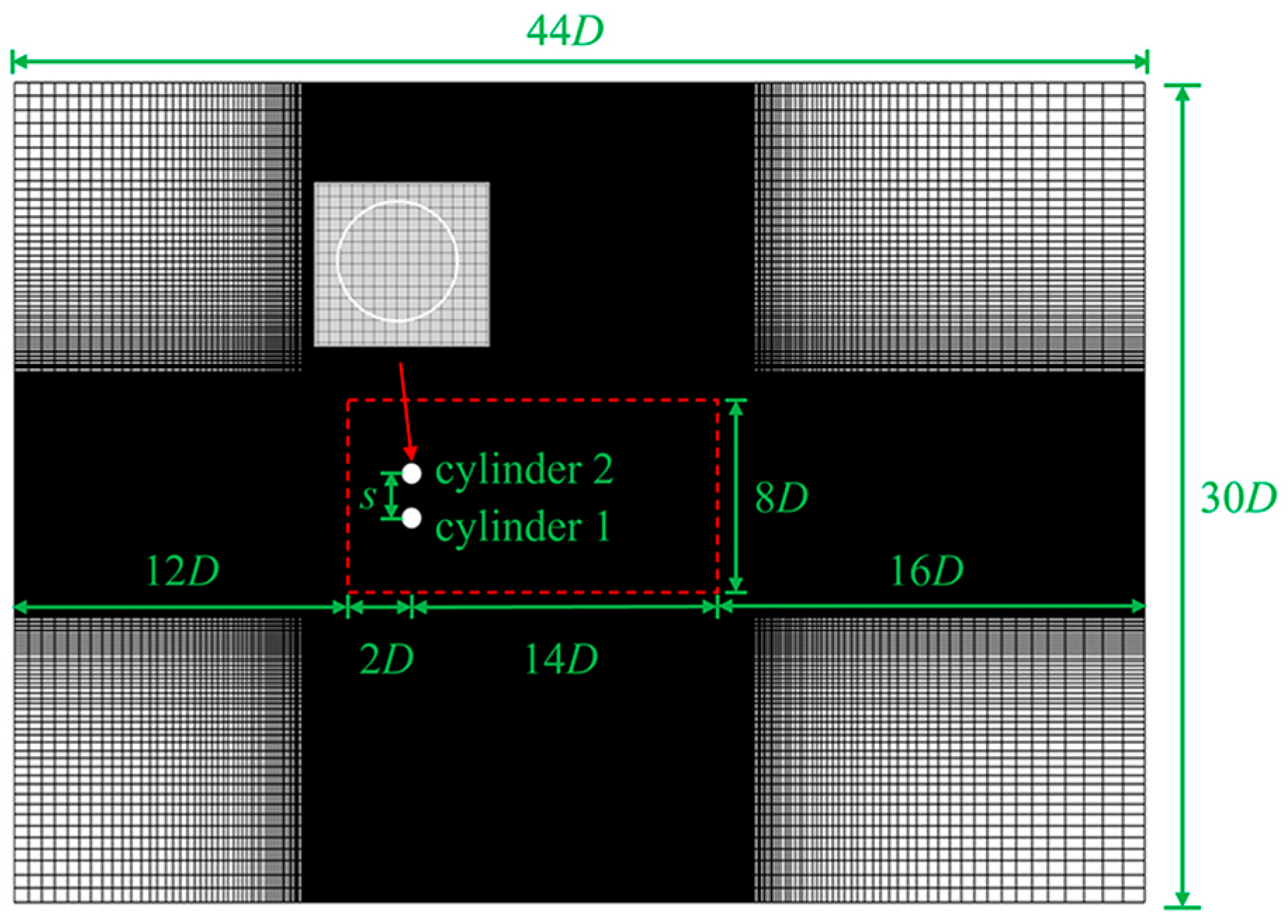
2. Numerical Model and Validation
2.1. Numerical Methods
2.2. Fluid-Structure Interaction Model
3. Flow around Two Side-by-Side Stationary Cylinders
4. Vortex-Induced Vibration of Two Side-by-Side Flexible Cylinders
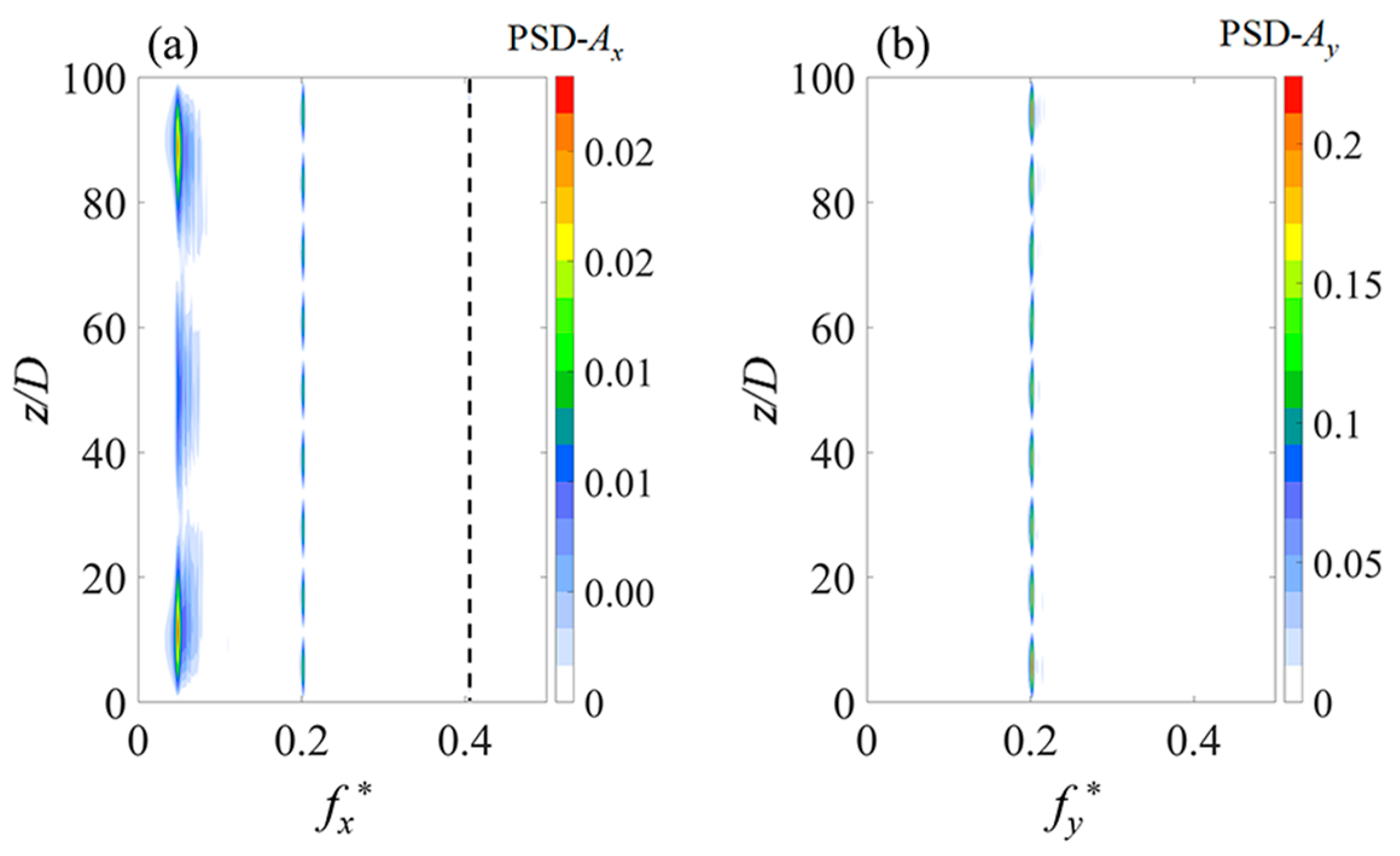
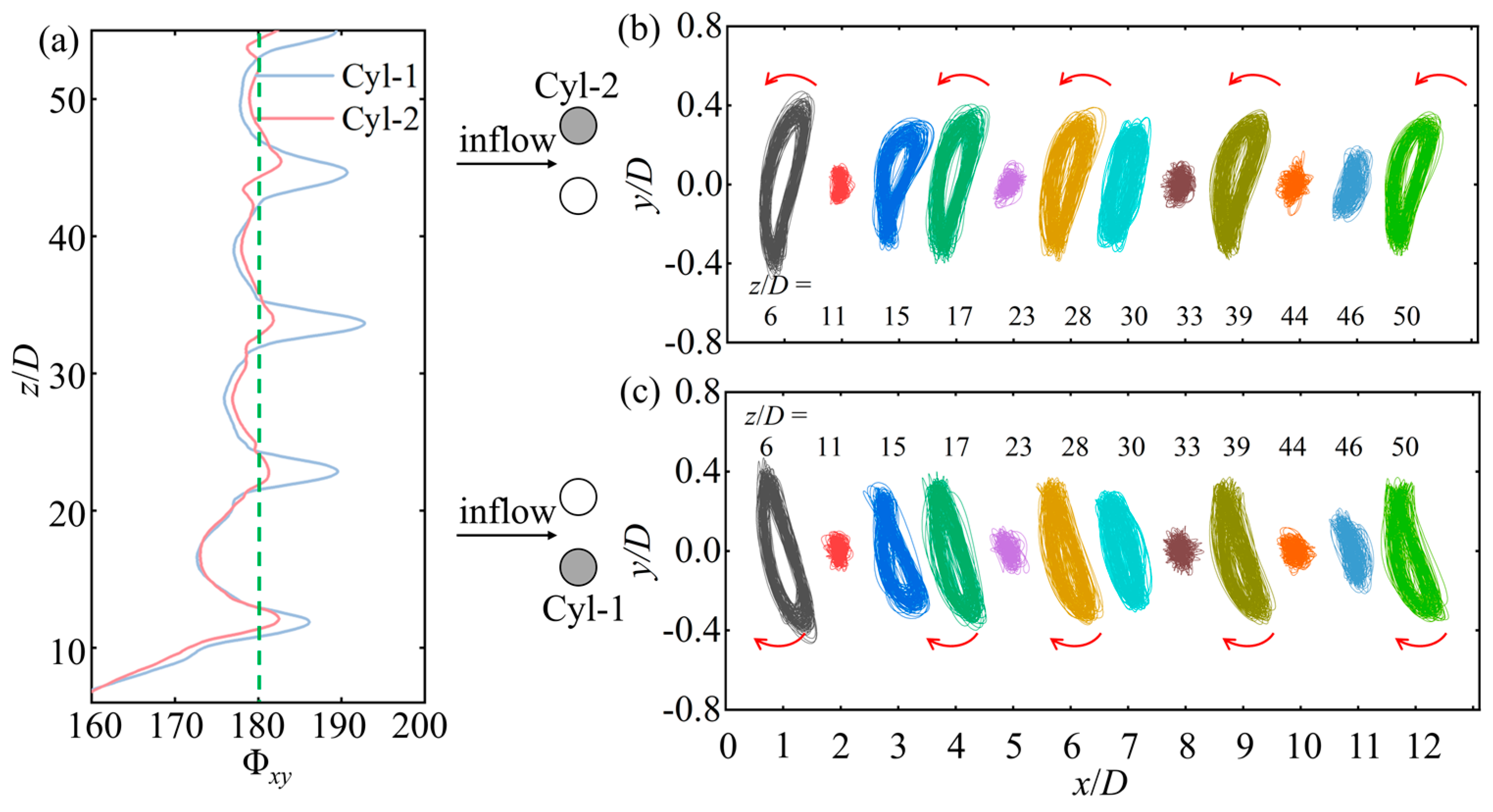
4.1. Vibration Response
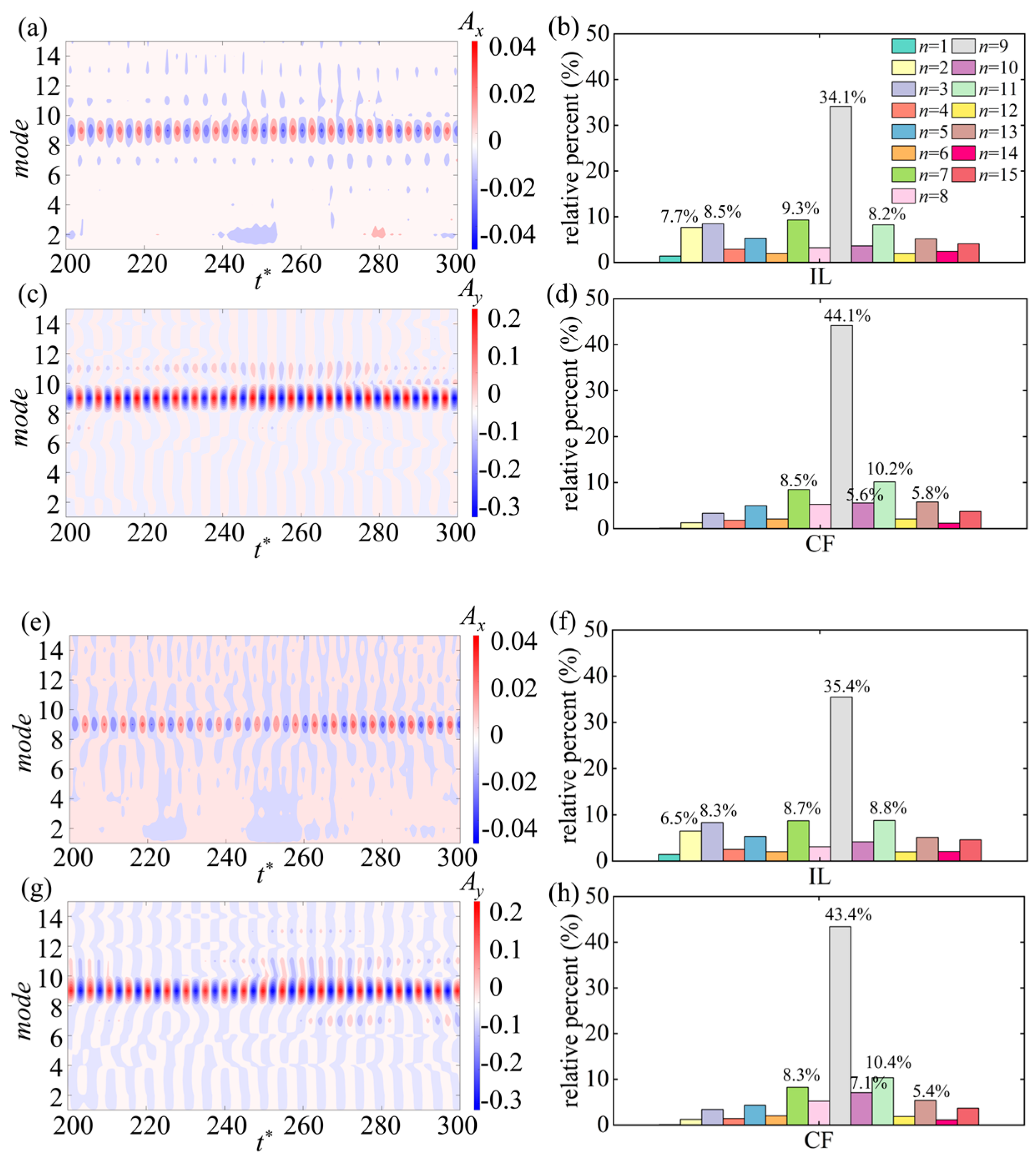
4.2. Mode Competition and Transition
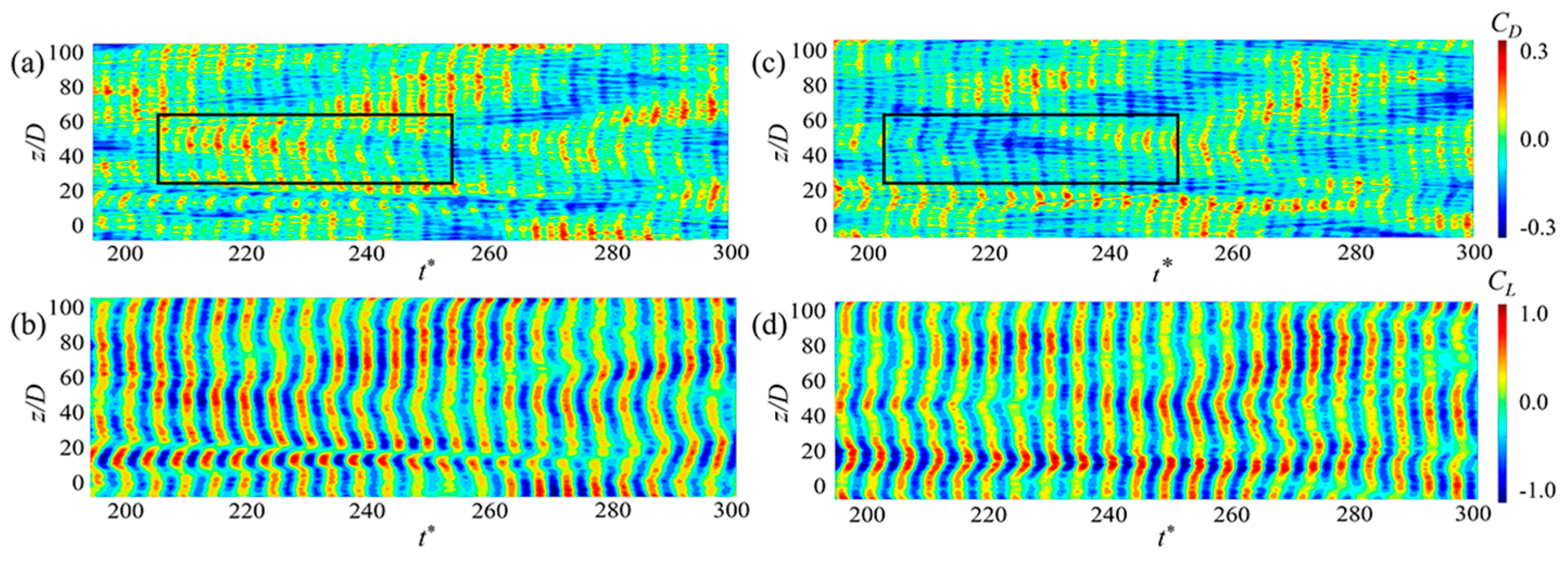
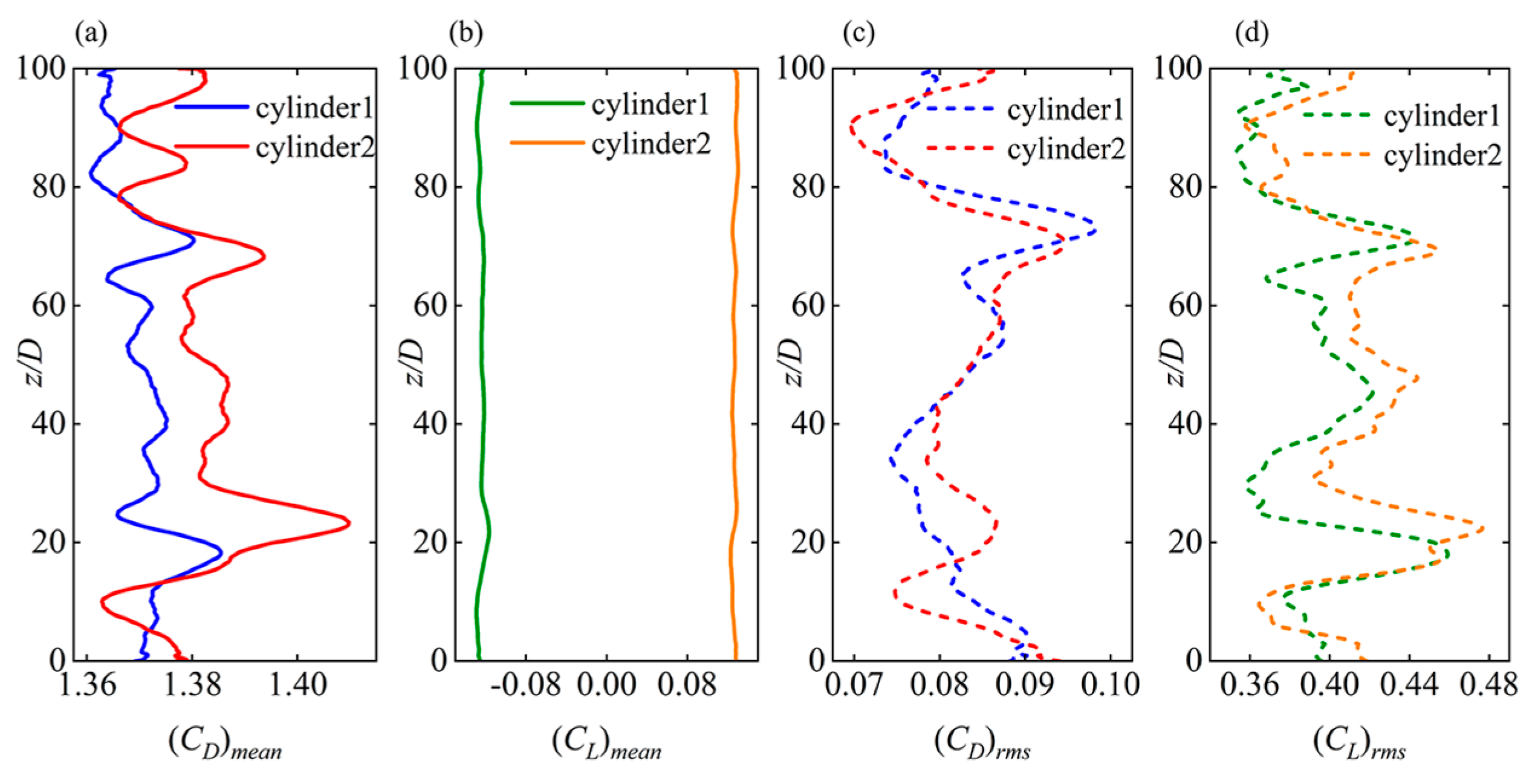
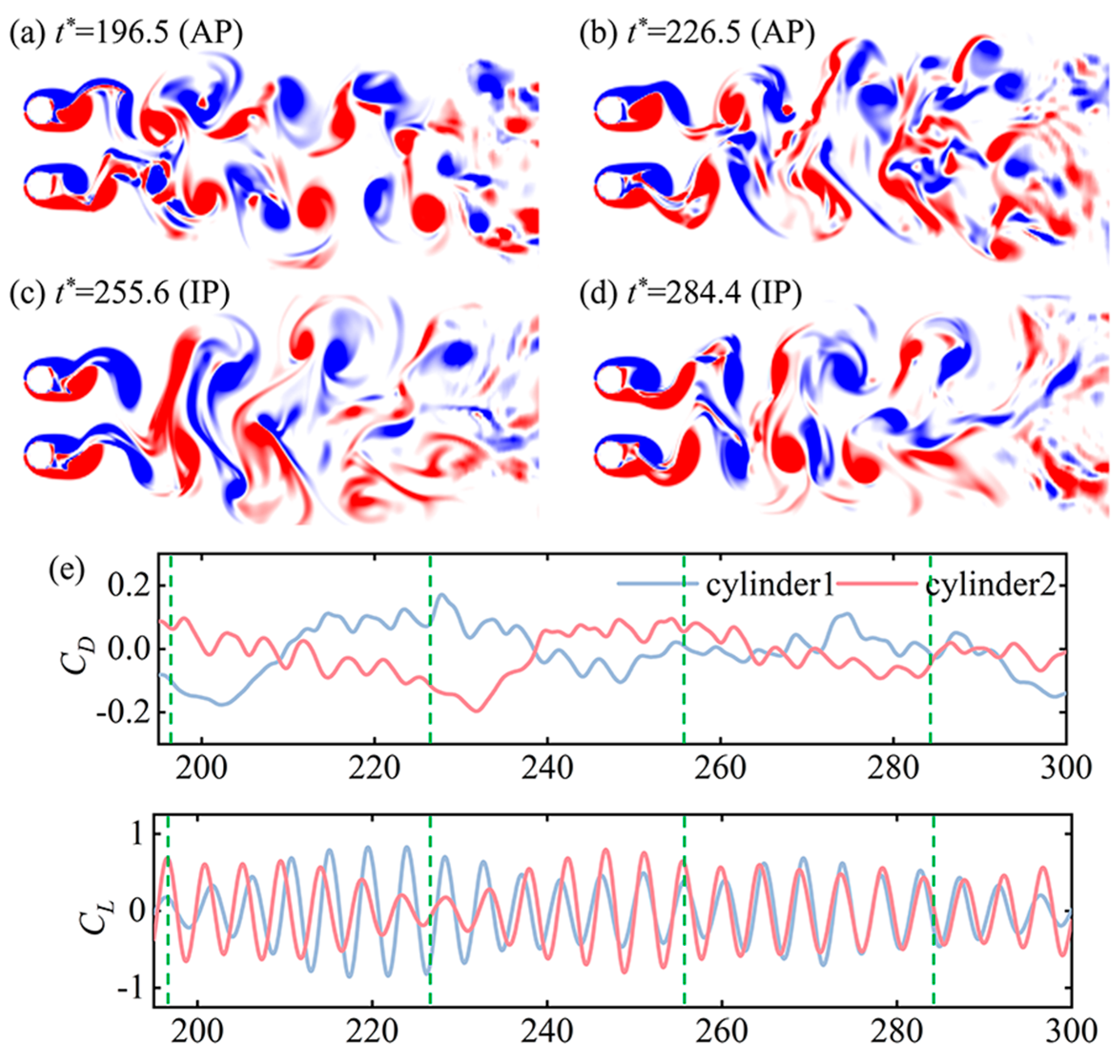
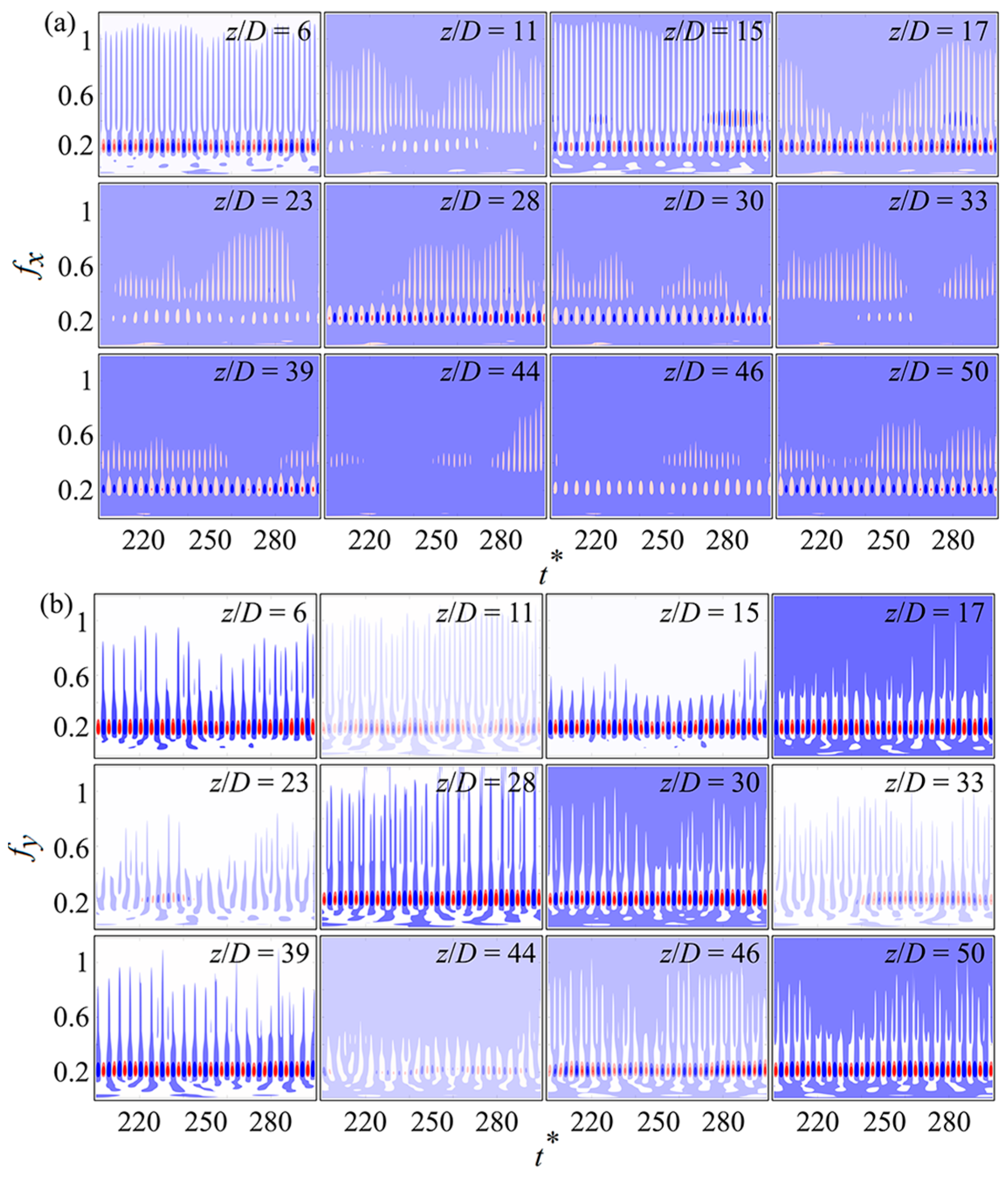
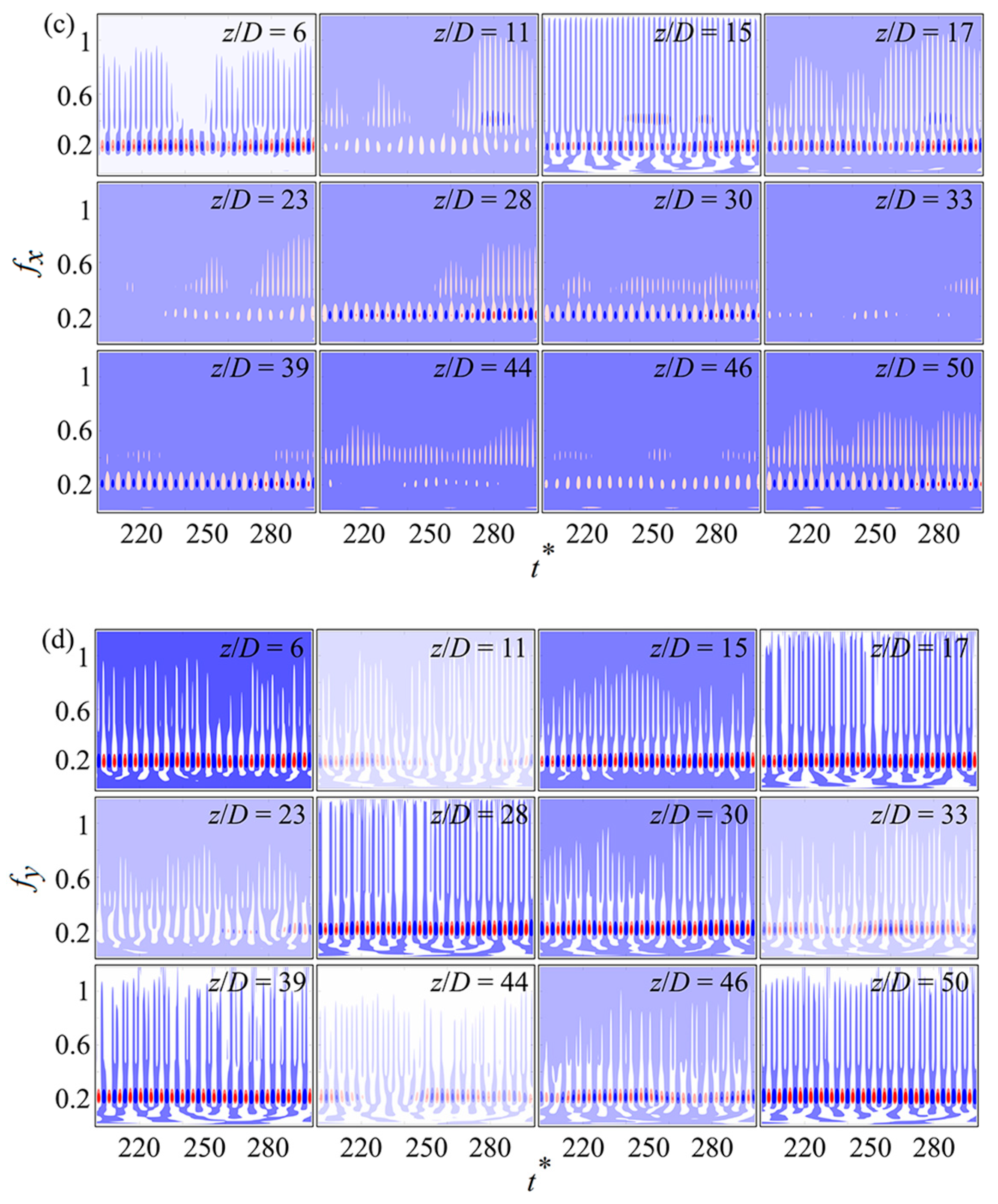
4.3. Hydrodynamic Forces and Energy Transfer
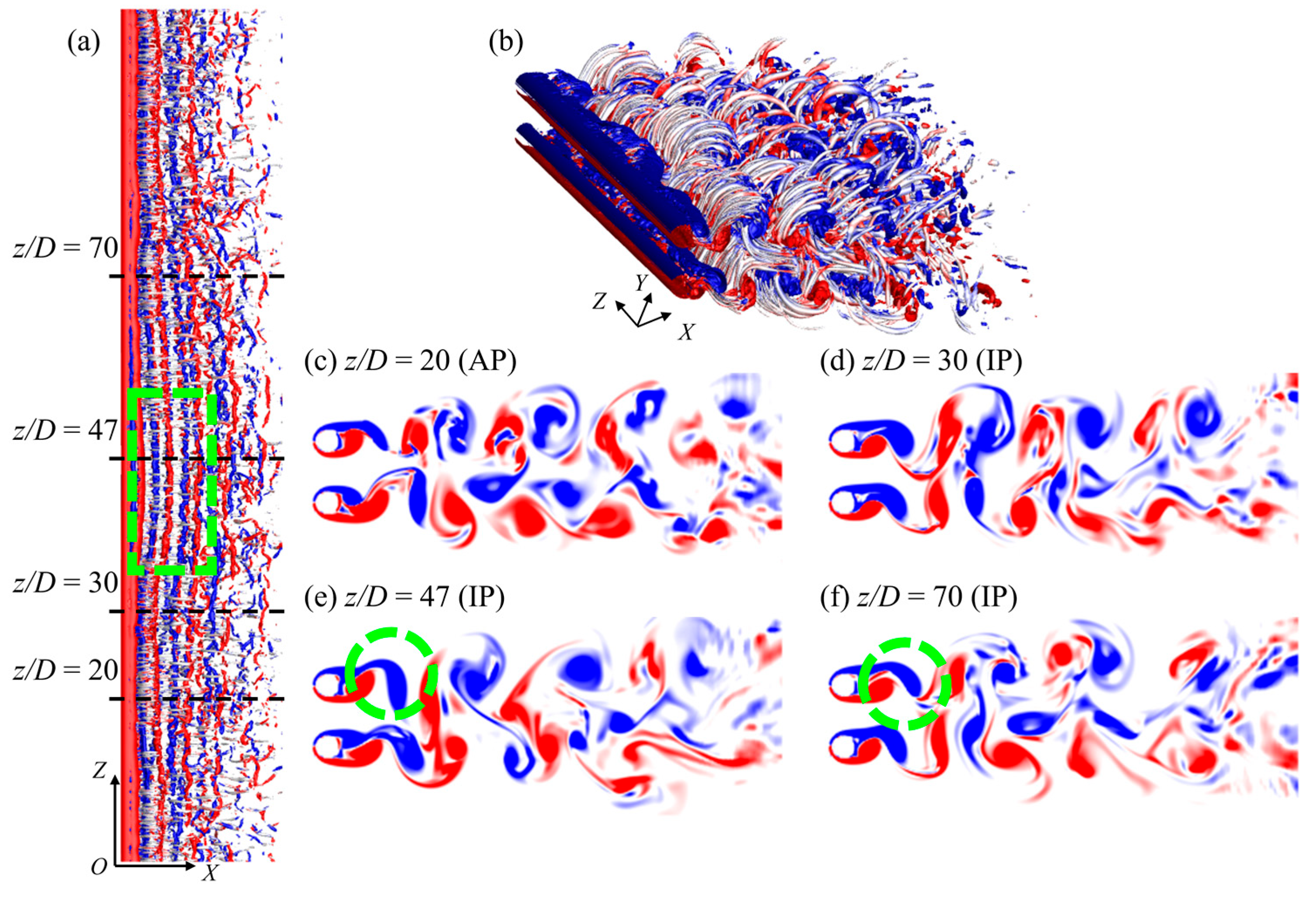
4.4. Wake Patterns
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| (Ax)rms | (Ay)rms | fx | fy | (CD)mean | (CD)rms | (CL)rms | |
|---|---|---|---|---|---|---|---|
| Present | 0.145 | 0.544 | 0.340 | 0.170 | 2.014 | 0.657 | 0.952 |
| Bourguet et al. (2015) [33] | 0.149 | 0.532 | 0.342 | 0.171 | 2.100 | 0.677 | 0.915 |
| Difference (%) | 2.68 | 2.26 | 0.58 | 0.58 | 4.10 | 2.92 | 4.04 |
| (CD)mean | (CL)mean | St | |
|---|---|---|---|
| Present | 1.424 | 0.176 | 0.163 |
| Kang (2003) [42] | 1.43 (0.42) | 0.178 (1.14) | 0.164 (0.61) |
| Lee et al. (2009) [43] | 1.423 (0.07) | 0.178 (1.14) | - |
| Bao et al. (2013) [44] | 1.431 (0.49) | 0.177 (0.57) | - |
| Carini et al. (2014) [45] | 1.408 (1.12) | - | 0.163 (0.00) |
References
- Zdravkovich, M.M.; Bearman, P.W. Flow Around Circular Cylinders—Volume 1: Fundamentals. J. Fluids Eng. 1998, 120, 216. [Google Scholar] [CrossRef]
- Ren, C.; Liu, Z.; Cheng, L.; Tong, F.; Xiong, C. Three-dimensional wake transitions of steady flow past two side-by-side cylinders. J. Fluid Mech. 2023, 972, A17. [Google Scholar] [CrossRef]
- Huera-Huarte, F.J.; Gharib, M. Flow-induced vibrations of a side-by-side arrangement of two flexible circular cylinders. J. Fluids Struct. 2011, 27, 354–366. [Google Scholar] [CrossRef]
- Liu, Y.; Li, P.; Wang, Y.; Liu, L.-H.; Wang, F.; Guo, H.-Y.; Fu, Q. An Experimental Study on Dynamics Features of Three Side-by-Side Flexible Risers Undergoing Vortex-Induced Vibrations in A Uniform Flow. China Ocean Eng. 2020, 34, 500–512. [Google Scholar] [CrossRef]
- Zdravkovich, M.M. The effects of interference between circular cylinders in cross flow. J. Fluids Struct. 1987, 1, 239–261. [Google Scholar] [CrossRef]
- Wang, Z.J.; Zhou, Y. Vortex interactions in a two side-by-side cylinder near-wake. Int. J. Heat Fluid Flow 2005, 26, 362–377. [Google Scholar] [CrossRef]
- Afgan, I.; Kahil, Y.; Benhamadouche, S.; Sagaut, P. Large eddy simulation of the flow around single and two side-by-side cylinders at subcritical Reynolds numbers. Phys. Fluids 2011, 23, 075101. [Google Scholar] [CrossRef]
- Zhou, Y.; Feng, S.X.; Alam, M.M.; Bai, H.L. Reynolds number effect on the wake of two staggered cylinders. Phys. Fluids 2009, 21, 125105. [Google Scholar] [CrossRef]
- Supradeepan, K.; Roy, A. Characterisation and analysis of flow over two side by side cylinders for different gaps at low Reynolds number: A numerical approach. Phys. Fluids 2014, 26, 063602. [Google Scholar] [CrossRef]
- Kim, S.; Alam, M.M. Characteristics and suppression of flow-induced vibrations of two side-by-side circular cylinders. J. Fluids Struct. 2015, 54, 629–642. [Google Scholar] [CrossRef]
- Chen, W.; Ji, C.; Xu, W.; Liu, S.; Campbell, J. Response and wake patterns of two side-by-side elastically supported circular cylinders in uniform laminar cross-flow. J. Fluids Struct. 2015, 55, 218–236. [Google Scholar] [CrossRef]
- Munir, A.; Zhao, M.; Wu, H.; Lu, L. Effects of gap ratio on flow-induced vibration of two rigidly coupled side-by-side cylinders. J. Fluids Struct. 2019, 91, 102726. [Google Scholar] [CrossRef]
- Xu, W.; Wu, H.; Sha, M.; Wang, E. Numerical study on the flow-induced vibrations of two elastically mounted side-by-side cylinders at subcritical Reynolds numbers. Appl. Ocean Res. 2022, 124, 103191. [Google Scholar] [CrossRef]
- Ramberg, S.E. The effects of yaw and finite length upon the vortex wakes of stationary and vibrating circular cylinders. J. Fluid Mech. 1983, 128, 81–107. [Google Scholar] [CrossRef]
- Silva-Leon, J.; Cioncolini, A. Effect of Inclination on Vortex Shedding Frequency Behind a Bent Cylinder: An Experimental Study. Fluids 2019, 4, 100. [Google Scholar] [CrossRef]
- Zhou, Y.; Wang, Z.J.; So, R.M.C.; Xu, S.J.; Jin, W. Free vibrations of two side-by-side cylinders in a cross-flow. J. Fluid Mech. 2001, 443, 197–229. [Google Scholar] [CrossRef]
- So, R.M.C.; Wang, X.Q. Vortex-Induced Vibrations of Two Side-by-Side Euler-Bernoulli Beams. J. Sound Vib. 2003, 259, 677–700. [Google Scholar] [CrossRef]
- Han, Q.; Ma, Y.; Xu, W.; Zhang, S. An experimental study on the hydrodynamic features of two side-by-side flexible cylinders undergoing flow-induced vibrations in a uniform flow. Mar. Struct. 2018, 61, 326–342. [Google Scholar] [CrossRef]
- Xu, W.; Cheng, A.; Ma, Y.; Gao, X. Multi-mode flow-induced vibrations of two side-by-side slender flexible cylinders in a uniform flow. Mar. Struct. 2018, 57, 219–236. [Google Scholar] [CrossRef]
- Ji, C.; Munjiza, A.; Williams, J.J.R. A novel iterative direct-forcing immersed boundary method and its finite volume applications. J. Comput. Phys. 2012, 231, 1797–1821. [Google Scholar] [CrossRef]
- Peskin, C.S. The immersed boundary method. Acta Numer. 2003, 11, 479–517. [Google Scholar] [CrossRef]
- Van der Vorst, H.A. Bi-CGSTAB: A Fast and Smoothly Converging Variant of Bi-CG for the Solution of Nonsymmetric Linear Systems. SIAM J. Sci. Stat. Comput. 1992, 13, 631–644. [Google Scholar] [CrossRef]
- Vandiver, J.K.; Jaiswal, V.; Jhingran, V. Insights on vortex-induced, traveling waves on long risers. J. Fluids Struct. 2009, 25, 641–653. [Google Scholar] [CrossRef]
- Dahl, J.M.; Hover, F.S.; Triantafyllou, M.S.; Oakley, O.H. Dual resonance in vortex-induced vibrations at subcritical and supercritical Reynolds numbers. J. Fluid Mech. 2010, 643, 395–424. [Google Scholar] [CrossRef]
- Gao, Y.; Zhang, Z.; Zou, L.; Zong, Z.; Yang, B. Effect of boundary condition and aspect ratio on vortex-induced vibration response of a circular cylinder. Ocean Eng. 2019, 188, 106244. [Google Scholar] [CrossRef]
- Bourguet, R.; Triantafyllou, M.S. The onset of vortex-induced vibrations of a flexible cylinder at large inclination angle. J. Fluid Mech. 2016, 809, 111–134. [Google Scholar] [CrossRef]
- Jiang, H.; Cheng, L.; Draper, S.; An, H. Two- and three-dimensional instabilities in the wake of a circular cylinder near a moving wall. J. Fluid Mech. 2017, 812, 435–462. [Google Scholar] [CrossRef]
- Zhang, Z.; Ji, C.; Alam, M.M.; Xu, D. Dynamics and wake structure of a near-wall flexible cylinder. Int. J. Mech. Sci. 2022, 222, 107229. [Google Scholar] [CrossRef]
- Chen, W.L.; Ji, C.N.; Xu, D. Vortex-induced vibrations of two side-by-side circular cylinders with two degrees of freedom in laminar cross-flow. Comput. Fluids 2019, 193, 104288. [Google Scholar] [CrossRef]
- Yan, Y.; Chen, W.; Zhang, Z.; Ji, C.; Srinil, N. Features and mechanisms of asymmetric wake evolution downstream of two parallel circular cylinders. Phys. Fluids 2023, 35, 107132. [Google Scholar] [CrossRef]
- Williamson, C.H.K.; Govardhan, R. Vortex-Induced Vibrations. Annu. Rev. Fluid Mech. 2004, 36, 413–455. [Google Scholar] [CrossRef]
- Zhou, Y.; Zhang, H.J.; Yiu, M.W. The turbulent wake of two side-by-side circular cylinders. J. Fluid Mech. 2002, 458, 303–332. [Google Scholar] [CrossRef]
- Bourguet, R.; Em Karniadakis, G.; Triantafyllou, M.S. On the validity of the independence principle applied to the vortex-induced vibrations of a flexible cylinder inclined at 60°. J. Fluids Struct. 2015, 53, 58–69. [Google Scholar] [CrossRef]
- Fan, D.X.; Wang, Z.C.; Triantafyllou, M.S.; Karniadakis, G.E. Mapping the properties of the vortex-induced vibrations of flexible cylinders in uniform oncoming flow. J. Fluid Mech. 2019, 881, 815–858. [Google Scholar] [CrossRef]
- Dahl, J.M.; Hover, F.S.; Triantafyllou, M.S.; Dong, S.; Karniadakis, G.E. Resonant vibrations of bluff bodies cause multivortex shedding and high frequency forces. Phys. Rev. Lett. 2007, 99, 144503. [Google Scholar] [CrossRef] [PubMed]
- Bourguet, R.; Karniadakis, G.E.; Triantafyllou, M.S. Vortex-induced vibrations of a long flexible cylinder in shear flow. J. Fluid Mech. 2011, 677, 342–382. [Google Scholar] [CrossRef]
- Bourguet, R.; Lucor, D.; Triantafyllou, M.S. Mono- and multi-frequency vortex-induced vibrations of a long tensioned beam in shear flow. J. Fluids Struct. 2012, 32, 52–64. [Google Scholar] [CrossRef]
- Jauvtis, N.; Williamson, C.H.K. The effect of two degrees of freedom on vortex-induced vibration at low mass and damping. J. Fluid Mech. 2004, 509, 23–62. [Google Scholar] [CrossRef]
- Franzini, G.R.; Gonçalves, R.T.; Meneghini, J.R.; Fujarra, A.L.C. One and two degrees-of-freedom Vortex-Induced Vibration experiments with yawed cylinders. J. Fluids Struct. 2013, 42, 401–420. [Google Scholar] [CrossRef]
- Zhang, Z.; Ji, C.; Xu, D.; Zhu, H.; Derakhshandeh, J.F.; Chen, W. Effect of yaw angle on vibration mode transition and wake structure of a near-wall flexible cylinder. Phys. Fluids 2022, 34, 077106. [Google Scholar] [CrossRef]
- Bourguet, R.; Triantafyllou, M.S. Vortex-induced vibrations of a flexible cylinder at large inclination angle. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2015, 373, 20140108. [Google Scholar] [CrossRef] [PubMed]
- Kang, S. Characteristics of flow over two circular cylinders in a side-by-side arrangement at low Reynolds numbers. Phys. Fluids 2003, 15, 2486–2498. [Google Scholar] [CrossRef]
- Lee, D.S.; Ha, M.Y.; Yoon, H.S.; Balachandar, S. A numerical study on the flow patterns of two oscillating cylinders. J. Fluids Struct. 2009, 25, 263–283. [Google Scholar] [CrossRef]
- Bao, Y.; Zhou, D.; Tu, J. Flow characteristics of two in-phase oscillating cylinders in side-by-side arrangement. Comput. Fluids 2013, 71, 124–145. [Google Scholar] [CrossRef]
- Carini, M.; Giannetti, F.; Auteri, F. On the origin of the flip–flop instability of two side-by-side cylinder wakes. J. Fluid Mech. 2014, 742, 552–576. [Google Scholar] [CrossRef]







Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chang, S.; Zhang, L.; Zhang, Z.; Ji, C. Dynamics and Wake Interference Mechanism of Long Flexible Circular Cylinders in Side-by-Side Arrangements. Energies 2024, 17, 2741. https://doi.org/10.3390/en17112741
Chang S, Zhang L, Zhang Z, Ji C. Dynamics and Wake Interference Mechanism of Long Flexible Circular Cylinders in Side-by-Side Arrangements. Energies. 2024; 17(11):2741. https://doi.org/10.3390/en17112741
Chicago/Turabian StyleChang, Shuqi, Luoning Zhang, Zhimeng Zhang, and Chunning Ji. 2024. "Dynamics and Wake Interference Mechanism of Long Flexible Circular Cylinders in Side-by-Side Arrangements" Energies 17, no. 11: 2741. https://doi.org/10.3390/en17112741
APA StyleChang, S., Zhang, L., Zhang, Z., & Ji, C. (2024). Dynamics and Wake Interference Mechanism of Long Flexible Circular Cylinders in Side-by-Side Arrangements. Energies, 17(11), 2741. https://doi.org/10.3390/en17112741








