Real-Time Co-Simulation and Grid Integration of PMSG-Based Hydrokinetic Energy Conversion Systems via Power-Hardware-in-the-Loop Technics
Abstract
1. Introduction
1.1. State of the Art
1.2. Paper Contribution
2. Mathematical Model
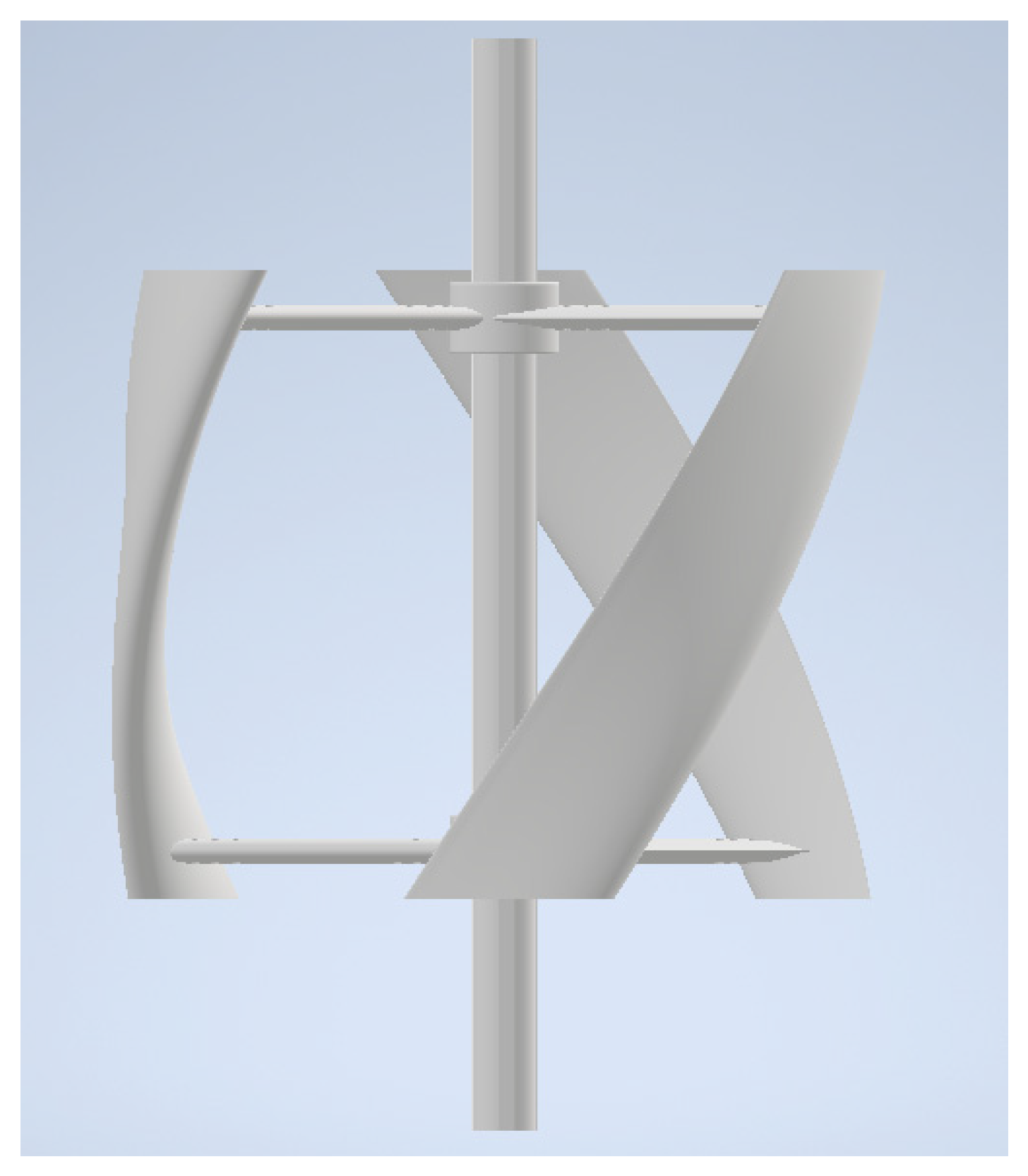
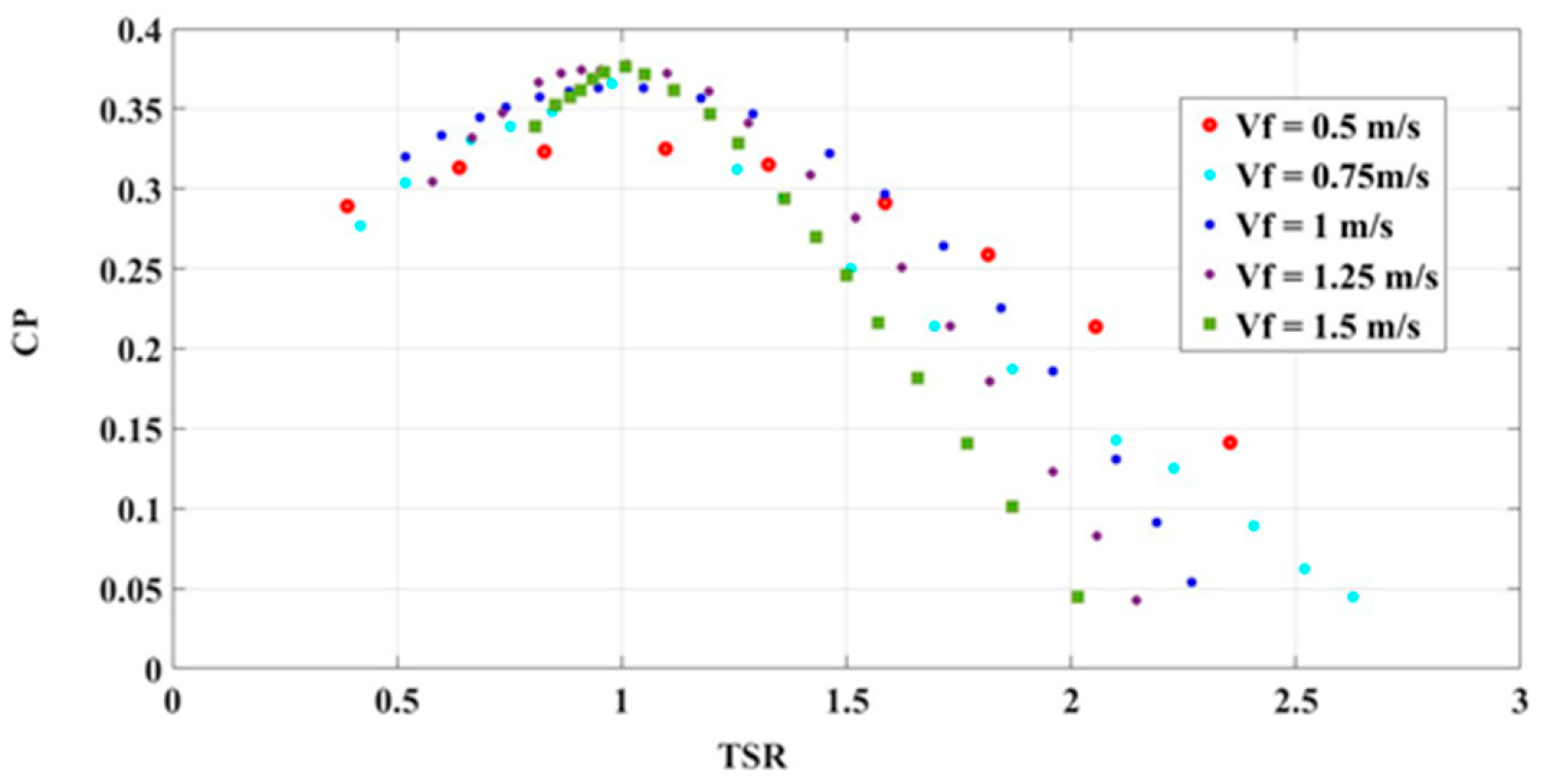
2.1. Gorlov Turbine
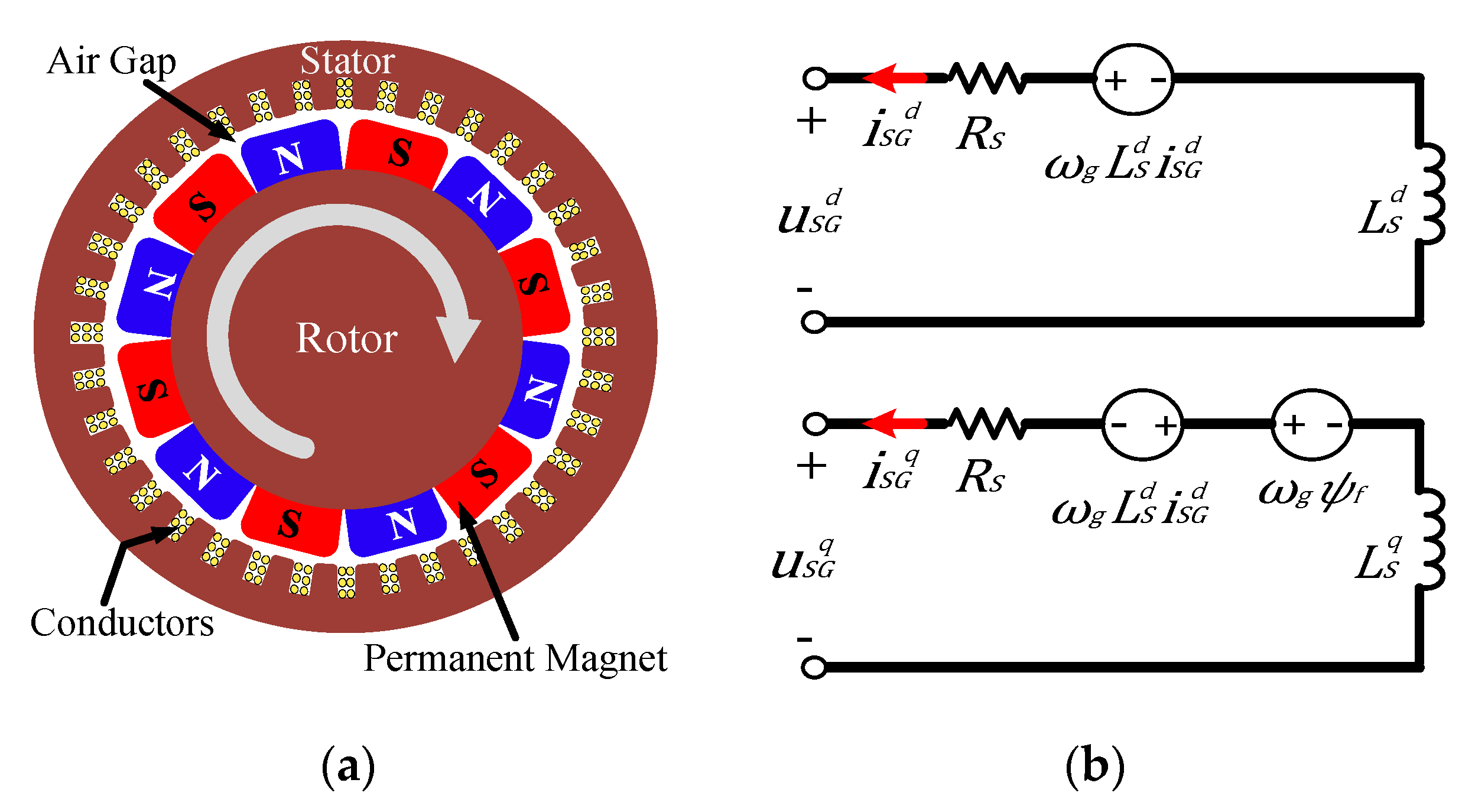
2.2. PMSG Generator
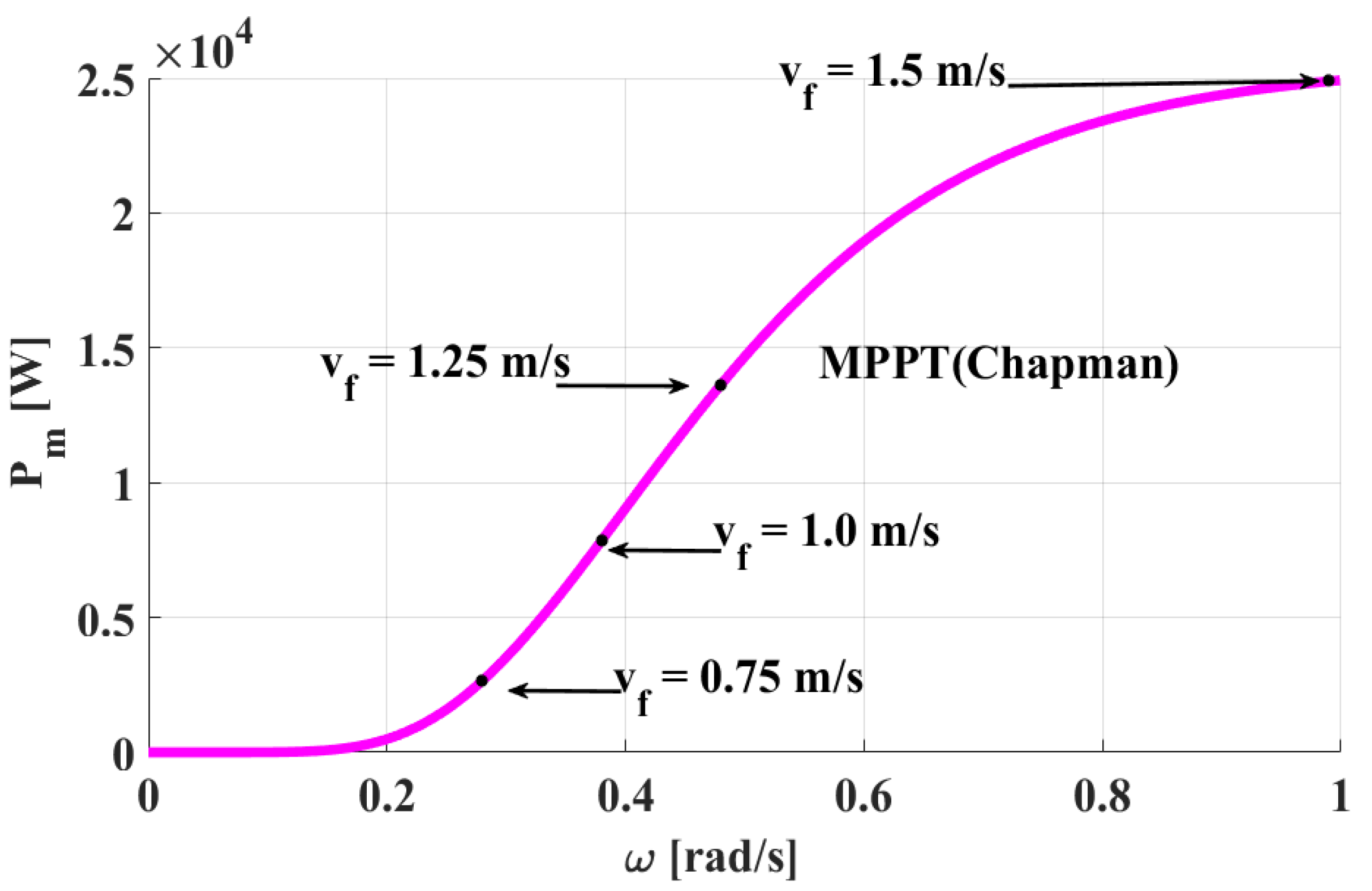
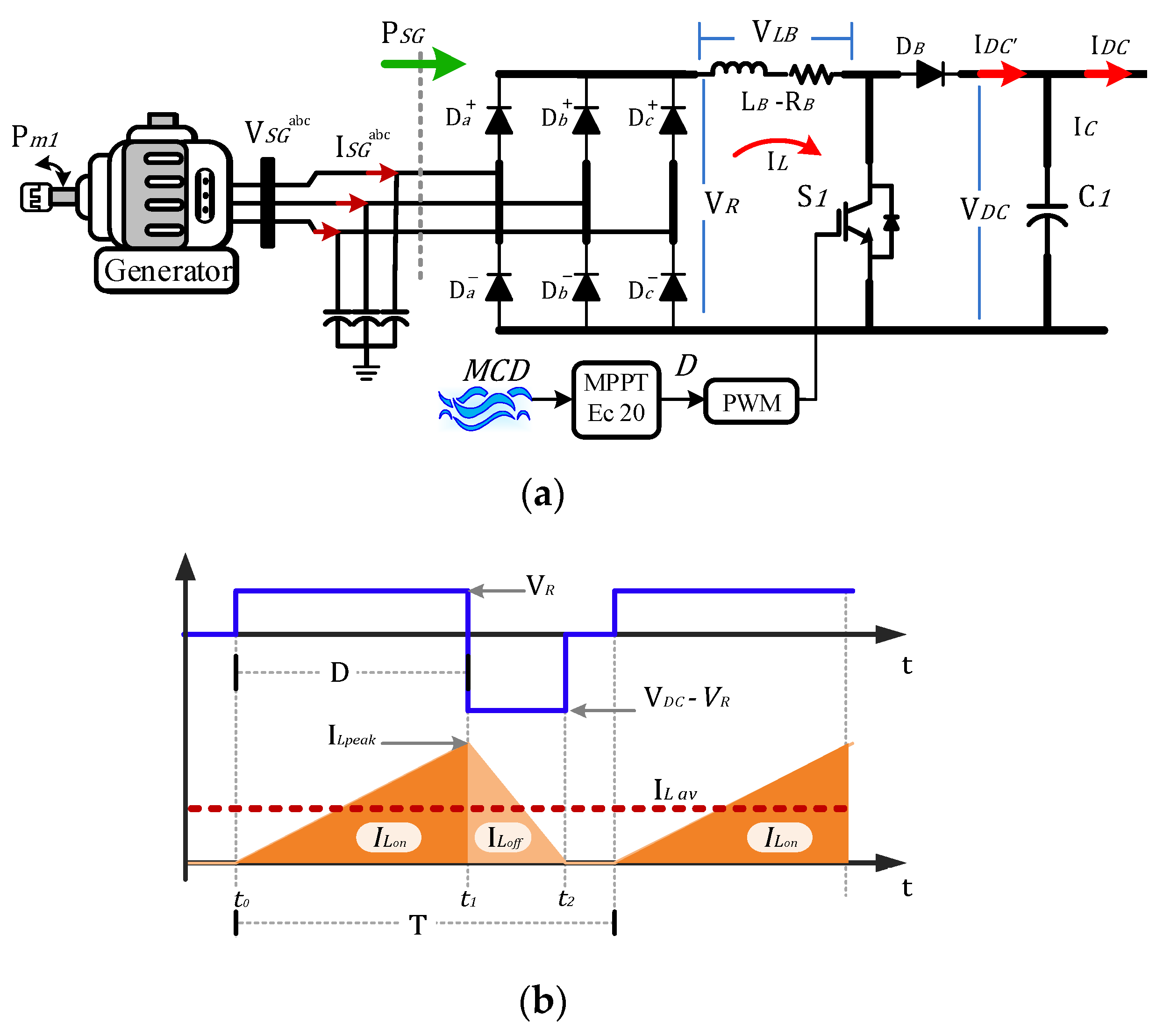
2.3. PFC DC/DC Boost Converter
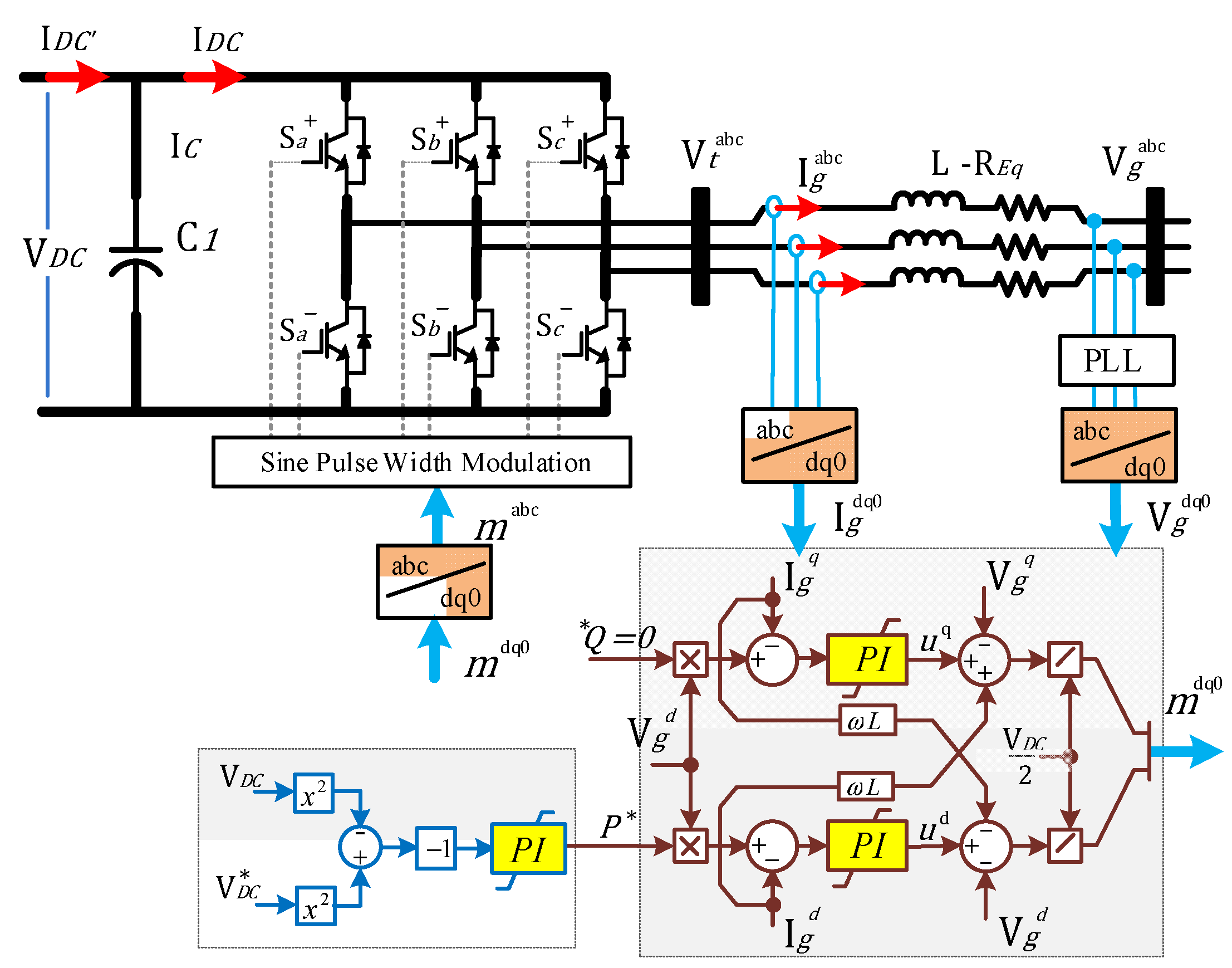
2.4. VSC Model
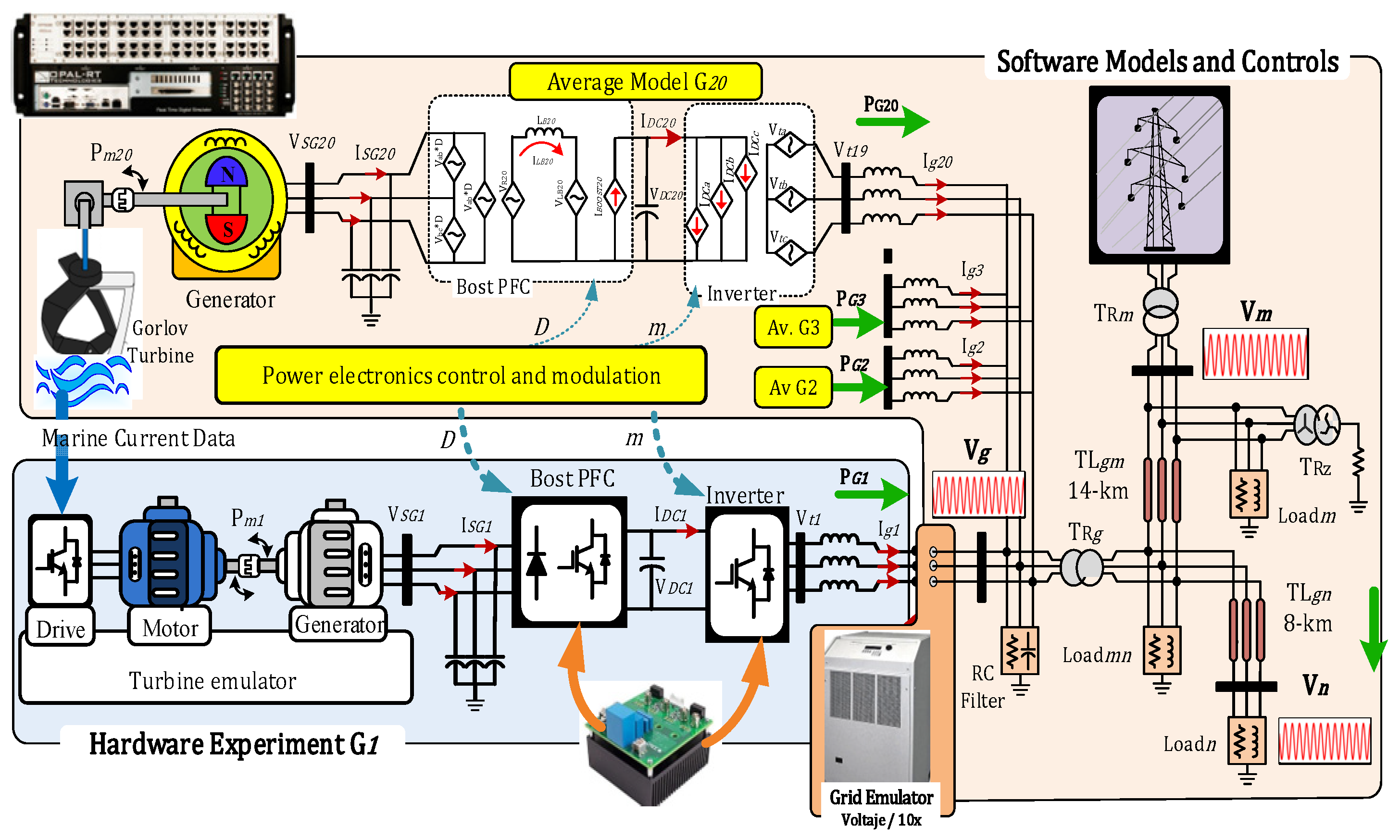
3. Numerical Simulation and Experimental Results
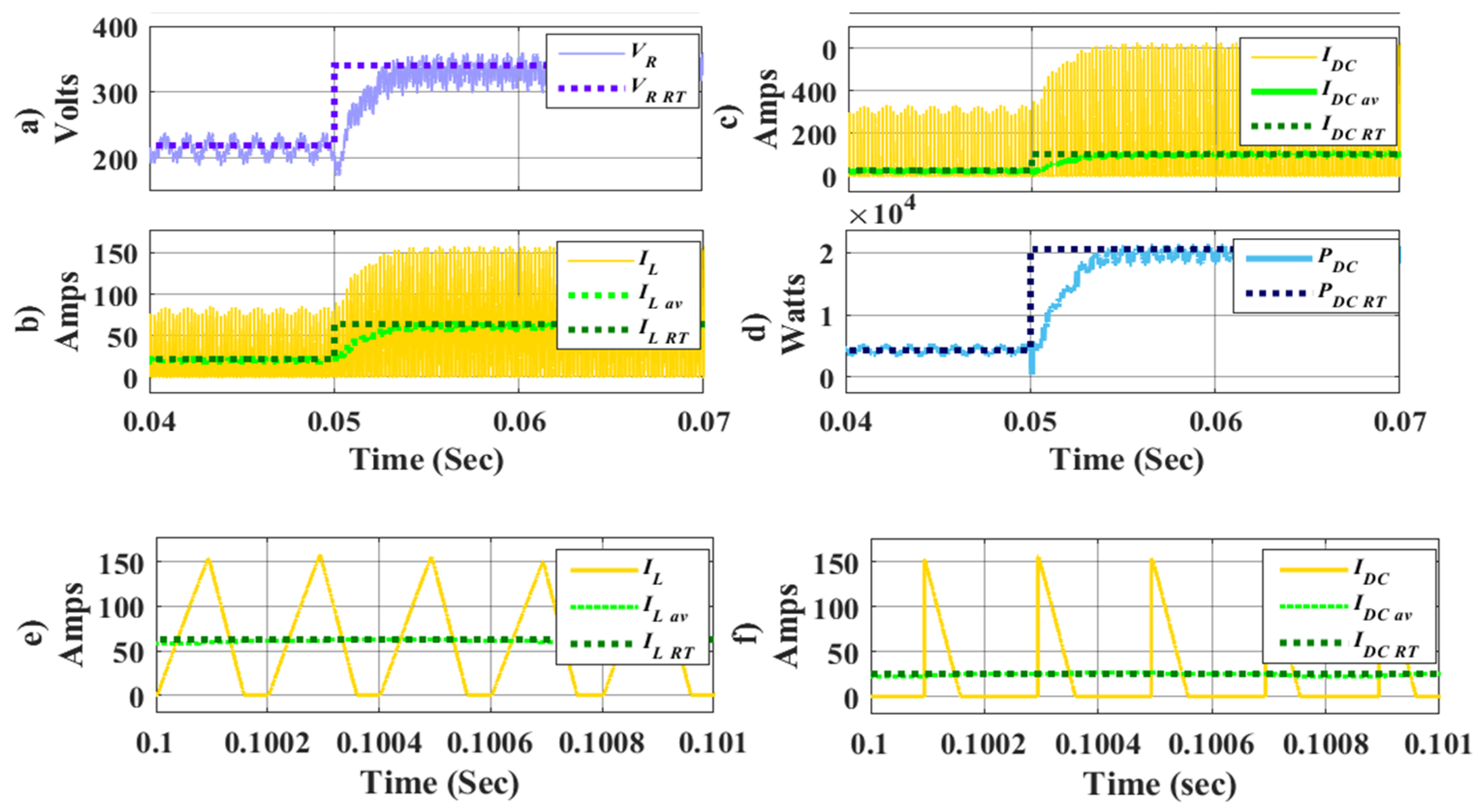
3.1. Real-Time Simulation Models
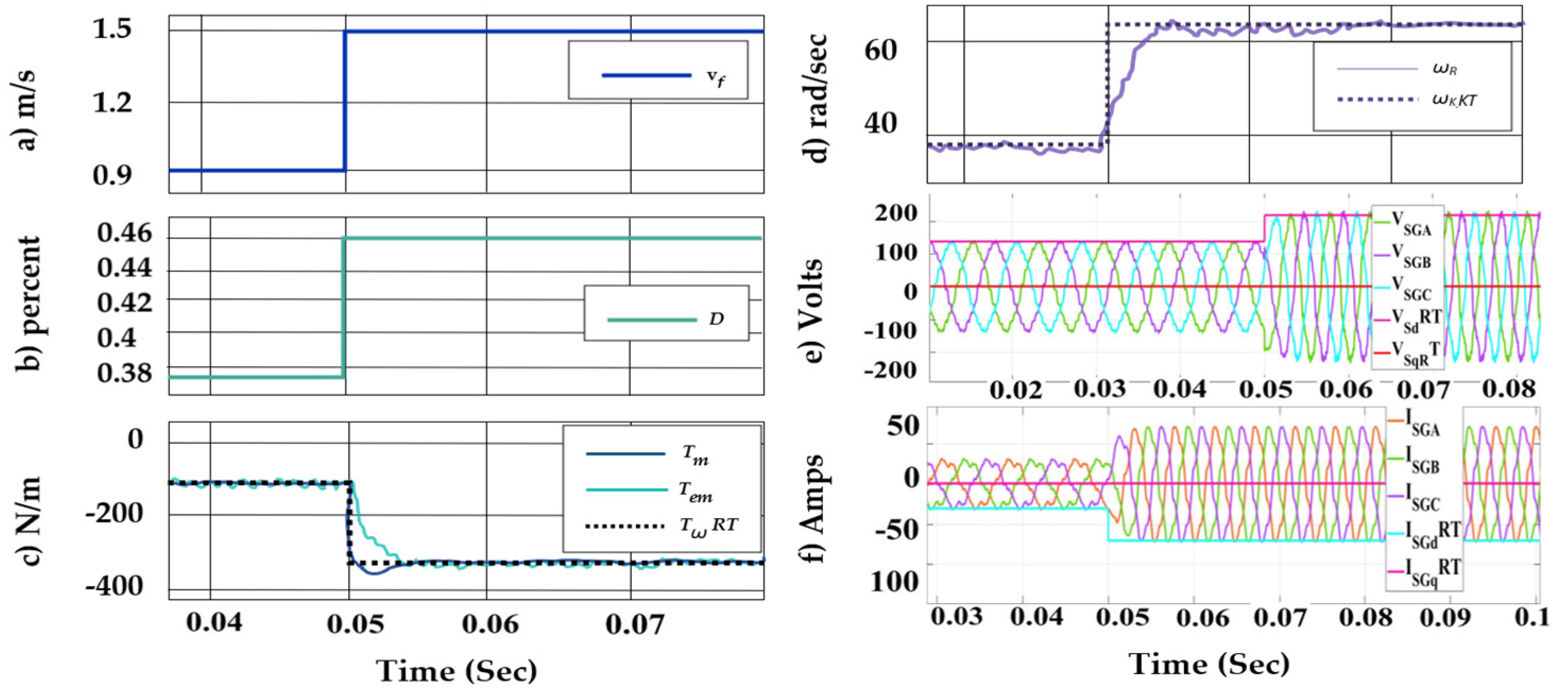
3.2. Experimental Results
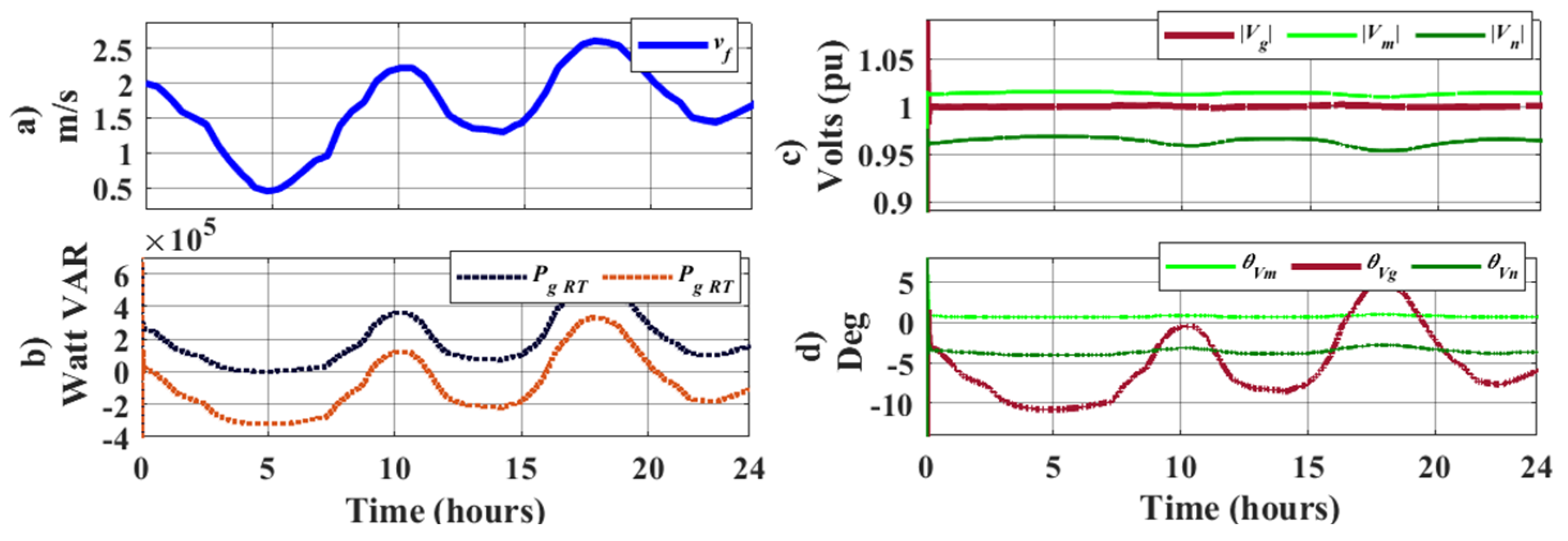
3.3. Power-Hardware-in-the-Loop Integrations
4. Conclusions
5. Future Works
5.1. Volt/Var Regulation
5.2. BESS Integration
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Nomenclature | Abbreviation |
| At | Transversal Area |
| DC | Direct Current |
| Cpmax | Maximum Power Coefficient |
| DFIG | Double Fed Induction Generator |
| Fc | Commutation Frequency |
| HIL | Hardware in the Loop |
| MIL | Model in the Loop |
| MPPT | Maximum Power Point Tracking |
| Np | PMSG Poles par Number |
| OWC | Oscillating Water Column |
| OWEC | Ocean Wave Energy Converters |
| PHIL | Power Hardware in The Loop |
| Pm | Mechanical Power |
| PMSG | Permanent Magnet Synchronous Generator |
| SIL | Software in the Loop |
| Electromagnetic torque | |
| Tmax | Maximum Torque |
| Tm | Mechanic Torque |
| TSR | Tip Speed Ratio |
| Vf | Flow Velocity |
| Vr | Boost Input Voltage |
| VSC | Voltage Source Converters |
| WEC | Wave Hardware in the Energy Converter |
| Symbols | |
| Φ | Inclination angle |
| b | Friction coefficient |
| D | Service cycle |
| fcom | Commutation VSC |
| fline | Line frequency |
| Esg | PMSG induced voltage |
| The phase current of VSC | |
| ILavmod | Average current |
| ILpeak | Peak current |
| Isg | PMSG generator current |
| J | Inertial momentum |
| LB | Boost inductance |
| y | The components of induction in the axis d and axis q. |
| L-Req | Impedance of VSC |
| Modulation index | |
| Rs | The winding resistance of the generator stator |
| T | Period |
| The permanent magnet flux | |
| The component in the voltage of the axle generator stator axe d | |
| The component in the voltage of the generator stator in axe q | |
| VDC | Boost output voltage |
| Mains voltage of VSC | |
| Vsg | PMSG terminal voltage |
| The final AC voltage | |
| ωg | Mechanical speed |
| ωmax | Maximum rotational speed |
References
- Gorr Pozzi, E. Wave energy Resource Harnessing assessment in a subtropical costal Region of the pacific. J. Mar. Sci. Eng. 2021, 9, 1264. [Google Scholar] [CrossRef]
- Khan, J.; Leon, D.; Moshref, A.; Arabi, S.; Bhuyan, G. Network Security Assessments for Integrating Large-Scale Tidal Current and Ocean Wave Resources into Future Electrical Grids. Proc. IEEE 2013, 101, 956–977. [Google Scholar] [CrossRef]
- Couch, S.J.; Wallace, A.R.; Bryden, I.G. Overview of the SUPERGEN Marine Energy Research Program. In Proceedings of the 2007 International Conference on Clean Electrical Power, Capri, Italy, 21–23 May 2007; pp. 312–314. [Google Scholar]
- Rahman, S.; Saha, S.; Islam, S.N.; Arif, M.T.; Mosadeghy, M.; Haque, M.E.; Oo, A.M. Analysis of Power Grid Voltage Stability with High Penetration of Solar PV Systems. In Proceedings of the 2020 IEEE Industry Applications Society Annual Meeting, Detroit, MI, USA, 10–16 October 2020; pp. 1–8. [Google Scholar]
- Cao, S.; Lin, N.; Dinavahi, V. Faster-than-real-time hardware emulation of transients and dynamics of a grid of microgrids. IEEE Open Access J. Power Energy 2023, 10, 36–47. [Google Scholar] [CrossRef]
- Opal-RT Corporate Headquarters. Power hil (p-hil): A Revolution in the Industry. Available online: https://blob.opal-rt.com/medias/L00161_0439.pdf (accessed on 1 May 2024).
- Chen, H.; Tang, T.; Aït-Ahmed, N.; Benbouzid, M.E.H.; Machmoum, M.; Zaïm, M.E.-H. Attraction, Challenge and Current Status of Marine Current Energy. IEEE Access 2018, 6, 12665–12685. [Google Scholar] [CrossRef]
- VLacerda, A.; Araujo, E.P.; Cheah-Mañe, M.; Gomis-Bellmunt, O. Phasor Modeling Approaches and Simulation Guidelines of Voltage-Source Converters in Grid-Integration Studies. IEEE Access 2022, 10, 51826–51838. [Google Scholar] [CrossRef]
- Baracho, F.R.A.C.; Coelho, A.M.L.; Pereira, F.C.S.; Silveira, P.M. A theoretical and practical approach for under excitation protection and control studies of large hydrogenerators in a real-time environment. In Proceedings of the 2017 IEEE Industry Applications Society Annual Meeting, Cincinnati, OH, USA, 1–5 October 2017; pp. 1–11. [Google Scholar]
- Prabakar, K.; Pratt, A.; Krishnamurthy, D.; Maitra, A. Hardware-in-the-Loop Test Bed and Test Methodology for Microgrid Controller Evaluation. In Proceedings of the 2018 IEEE/PES Transmission and Distribution Conference and Exposition (T&D), Denver, CO, USA, 16–19 April 2018; pp. 1–9. [Google Scholar]
- Chen, C.-A.; Li, X.; Zuo, L.; Ngo, K.D.T. An innovative generic platform to simulate real-time PTO for ocean energy converters based on SIL method. IEEE Trans. Ind. Electron. 2018, 68, 3262–3272. [Google Scholar] [CrossRef]
- Borner, T. Real Time Hybrid Modeling for Ocean Wave Energy Converters; University of California: Berkeley, CA, USA, 2015. [Google Scholar]
- Li, L.; Yuan, Z.; Gao, Y.; Zhang, X. Wave Force Prediction Effect on the Energy Absorption of a Wave Energy Converter with Real-Time Control. IEEE Trans. Suwithn. Energy 2019, 10, 615–624. [Google Scholar] [CrossRef]
- Amon, E.A.; Brekken, T.K.A.; Schacher, A.A. Maximum Power Point Tracking for Ocean Wave Energy Conversion. IEEE Trans. Ind. Appl. 2012, 48, 1071086. [Google Scholar] [CrossRef]
- M’zoughi, F.; Bouallègue, S.; Ayadi, M. Modeling and SIL simulation of an oscillating water column for ocean energy conversion. In Proceedings of the IREC2015 The Sixth International Renewable Energy Congress, Sousse, Tunisia, 24–26 March 2015; pp. 1–6. [Google Scholar]
- Kelly, J.F.; Christie, R. Applying Hardware-in-the-Loop capabilities to an ocean renewable energy device emulator. In Proceedings of the 2017 Twelfth International Conference on Ecological Vehicles and Renewable Energies (EVER), Monte-Carlo, Monaco, 11–13 April 2017; pp. 1–7. [Google Scholar]
- Erdogan, N.; Murray, D.B.; Giebhardt, J.; Wecker, M.; Donegan, J. Real-Time Hardware Emulation of a Power Take-Off Model for Grid-Connected Tidal Energy Systems. In Proceedings of the 2019 IEEE International Electric Machines & Drives Conference (IEMDC), San Diego, CA, USA, 11–15 May 2019; pp. 1368–1372. [Google Scholar]
- Caraiman, G.; Nichita, C.; Mînzu, V.; Dakyo, B.; Joen, C.H. Study of a real time emulator for marine current energy conversion. In Proceedings of the XIX International Conference on Electrical Machines IC-EM 2010, Rome, Italy, 6–8 September 2010; pp. 1–6. [Google Scholar]
- Caraiman, G.; Nichita, C.; Mînzu, V.; Dakyo, B.; Jo, C.H. Real time marine current turbine emulator: Design, development and control strategies. In Proceedings of the 2012 International Conference on Electrical Machines, Marseille, France, 2–5 September 2012; pp. 2145–2150. [Google Scholar]
- Caraiman, G.; Nichita, C.; Mînzu, V.; Dakyo, B. Marine current turbine emulator design based on hardware in the loop simulator structure. In Proceedings of the 14th International Power Electronics and Motion Control Conference EPE-PEMC 2010, Ohrid, Macedonia, 6–8 September 2010; pp. T12-101–T12-107. [Google Scholar]
- Ramirez, D.; Bartolome, J.P.; Martinez, S.; Herrero, L.C.; Blanco, M. Emulation of an OWC Ocean Energy Plant with PMSG and Irregular Wave Model. IEEE Trans. Sustain. Energy 2015, 6, 1515–1523. [Google Scholar] [CrossRef]
- Athié, G.; Jiménez, T.R.; De la Miyar-Loza, M.; Enríquez, C.; Sheinbaum, J.; Marín, M.; Mariño, I. Variability of the Yucatan current in the Mexican Caribbean and its effect, on the upwelling of Yucatán. In Proceedings of the 2021 First International Congress of Cemie-Ocean, Online, 2–4 June 2021. [Google Scholar]
- Fontes, J.H.; Félix, A.; Mendoza, E. On the Marine Energy Resource of México. J. Mar. Sci. Eng. 2019, 7, 191. [Google Scholar] [CrossRef]
- Gorlov, A.M. Unidirectional Helical Reaction Turbine Operable under Reversible Fluid Flow for Power Systems. U.S. Patent 5,451,137, 19 September 1995. [Google Scholar]
- Jayaram, V.; Bavanish, B. A brief study of the influence of the index revolution on the performance of Gorlov Helical Turbine. Int. J. Renew. Energy Res. 2022, 12, 827–845. [Google Scholar]
- Yang, B.; Shu, X.W. Hydrofoil optimization and experimental validation in helical vertical axis turbine for power generation from marine current. Ocean Eng. 2012, 42, 35–46. [Google Scholar] [CrossRef]
- Mejia-Ruiz, G.E.; Guerrero, J.M.R.; Paternina, M.R.A.; de la Cruz, J.; Mendez, A.Z.; Sandoval, A.P.P. Enhancing Grid Integration and Design of Low Speed PMSGs by Exploiting SRF-PLL -Based Sensorless Control and Holistic Modeling. IEEE Trans. Energy Convers. 2022, 37, 2962–2973. [Google Scholar] [CrossRef]
- Chowdhury, M.M.; Haque, M.E.; Saha, S.; Mahmud, M.A.; Gargoom, A.; Oo, A.M.T. An Enhanced Control Scheme for an IPM Synchronous Generator Based wind Turbine with MTPA Trajectory and Maximum Power Extraction. IEEE Trans. Energy Convers. 2018, 33, 556–566. [Google Scholar] [CrossRef]
- Luo, F.L.; Ye, H. Ordinary DC/DC Converters, Fundamental Converters, Boost Converter. In Power Electronics, Advanced Conversion Technologies; CRC Press: Boca Raton, FL, USA, 2010; pp. 146–149. [Google Scholar]
- Zhao, Y.; Kong, S.; Liu, D.; Guo, D. Modeling and simulation of maximum wind energy capture by segmented slope duty-cycle perturbation method. In Proceedings of the 2021 33rd, Chinese Control and Decision Conference (CCDC), Kunming, China, 22–24 May 2021; pp. 564–568. [Google Scholar]
- Yazdani, A.; Iravani, R. Grid-Imposed Frequency VSC System: Control in αβ-Frame. In Voltage-Sourced Converters in Power Systems, Modeling, Control and Applications; Wiley: Toronto, ON, Canada, 2010; pp. 160–203. [Google Scholar]





| Publication | Year | Type of Turbine | Type Generator | Power Converters Analysis | Electrical Grid Voltage Analysis | Multiple Generators |
|---|---|---|---|---|---|---|
| [11] | 2018 | Buoy | Linear | |||
| [12] | 2014 | |||||
| [13] | 2018 | Buoy | Linear | |||
| [14] | 2012 | Buoy | Linear | √ | ||
| [15] | 2017 | Well | PMSG | |||
| [16] | 2019 | Piles | PMSG | |||
| [17] | 2010 | Piles | DFIG | |||
| [18] | 2012 | Piles | DFIG | |||
| [19] | 2010 | Piles | DFIG | |||
| [20] | 2021 | Piles | DFIG | |||
| [21] | 2015 | Well | PMSG | √ | √ | |
| Proposed | 2023 | Gorlov | PMSG | √ | √ | √ |
| Scaling with an Experimental Result | Real-Time Hydrokinetic Unit | ||
|---|---|---|---|
| GRID | kW | kW | |
| A | A | ||
| PMSG | RPM | RPM | |
| A | A | ||
| Converters | |||
| kHz | kHz | ||
| kHz | kHz | ||
| F | F | ||
| V | V |
| Motor driver | Three-phase 440 V VFD007EL43A |
| Three-phase induction motor | GE Cage Rotor, P 1HP, nominal voltage 230 v |
| AC/DC converter | Three-phase rectifier 1600 V MDS100A. |
| Inverter and boost converters | KIT8020CRD8FF1217P-1 CREE SiC MOSFET Kit |
| Voltage and current sensors | USM 3IV Taraz Technologies® |
| Real-time simulator | OP5600 HIL module |
| Power amplifier | California Instruments network emulator MX30-3Pi |
| Voltage | Short Circuit Impedance | ||
|---|---|---|---|
| Distribution Feeder | 115 kV | 10.992 + j 28.78 Ω | |
| Distribution Lines | Distance | Impedance/Km | |
| L1 | 8 km | 0.115 Ω/Km 1.05 × 10−3 H/km | |
| L2 | 3 km | 0.115 Ω/Km 1.05 × 10−3 H/km | |
| Loads | Power | Power Factor | |
| Load 1 | 5.6626 MW | 0.874 (-) | |
| Load 2 | 3.0 MW | 0.93 (-) | |
| Load 3 | 1.158 MW | 0.95 (-) | |
| Transformers | Power | Impedance Winding 1 and 2 | |
| TR 1 | 20 MW 115/18.8 kV | 0.0027 + j 30.1600 Ω 0.0027 + j 30.1600 Ω | |
| TR 2 | 20 MW 115/18.8 kV | 0.0040 + j 18.850 Ω 0.0040 + j 18.850 Ω | |
| MC m/s | Mechanic PMSG | Power Converters PFC-Boost and VSC | Electrical Grid Nodal Voltages (pu) | |||||
|---|---|---|---|---|---|---|---|---|
rad/s | Τ N/m | D Mag | MW | |||||
| 0.3 | 23 | 2.67 | 0.83 | 0.0830 | ||||
| 0.9 | 40 | 120 | 0.744 | 0.172 | ||||
| 1.5 | 48 | 350 | 0.666 | 0.231 | ||||
| 1.7 | 55 | 500 | 0.595 | 0.501 | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jasso-Ruiz, U.; Rodríguez-Rodríguez, J.R.; Mendoza, E.; Echeverría, C.; Salgado-Herrera, N.M. Real-Time Co-Simulation and Grid Integration of PMSG-Based Hydrokinetic Energy Conversion Systems via Power-Hardware-in-the-Loop Technics. Energies 2024, 17, 2662. https://doi.org/10.3390/en17112662
Jasso-Ruiz U, Rodríguez-Rodríguez JR, Mendoza E, Echeverría C, Salgado-Herrera NM. Real-Time Co-Simulation and Grid Integration of PMSG-Based Hydrokinetic Energy Conversion Systems via Power-Hardware-in-the-Loop Technics. Energies. 2024; 17(11):2662. https://doi.org/10.3390/en17112662
Chicago/Turabian StyleJasso-Ruiz, Ubaldo, Juan Ramón Rodríguez-Rodríguez, Edgar Mendoza, Carlos Echeverría, and Nadia Maria Salgado-Herrera. 2024. "Real-Time Co-Simulation and Grid Integration of PMSG-Based Hydrokinetic Energy Conversion Systems via Power-Hardware-in-the-Loop Technics" Energies 17, no. 11: 2662. https://doi.org/10.3390/en17112662
APA StyleJasso-Ruiz, U., Rodríguez-Rodríguez, J. R., Mendoza, E., Echeverría, C., & Salgado-Herrera, N. M. (2024). Real-Time Co-Simulation and Grid Integration of PMSG-Based Hydrokinetic Energy Conversion Systems via Power-Hardware-in-the-Loop Technics. Energies, 17(11), 2662. https://doi.org/10.3390/en17112662







