Continuum Modeling of Slightly Wet Fluidization with Electrical Capacitance Tomograph Validation
Abstract
1. Introduction
2. Motivation and Objectives of the Study
3. Theory
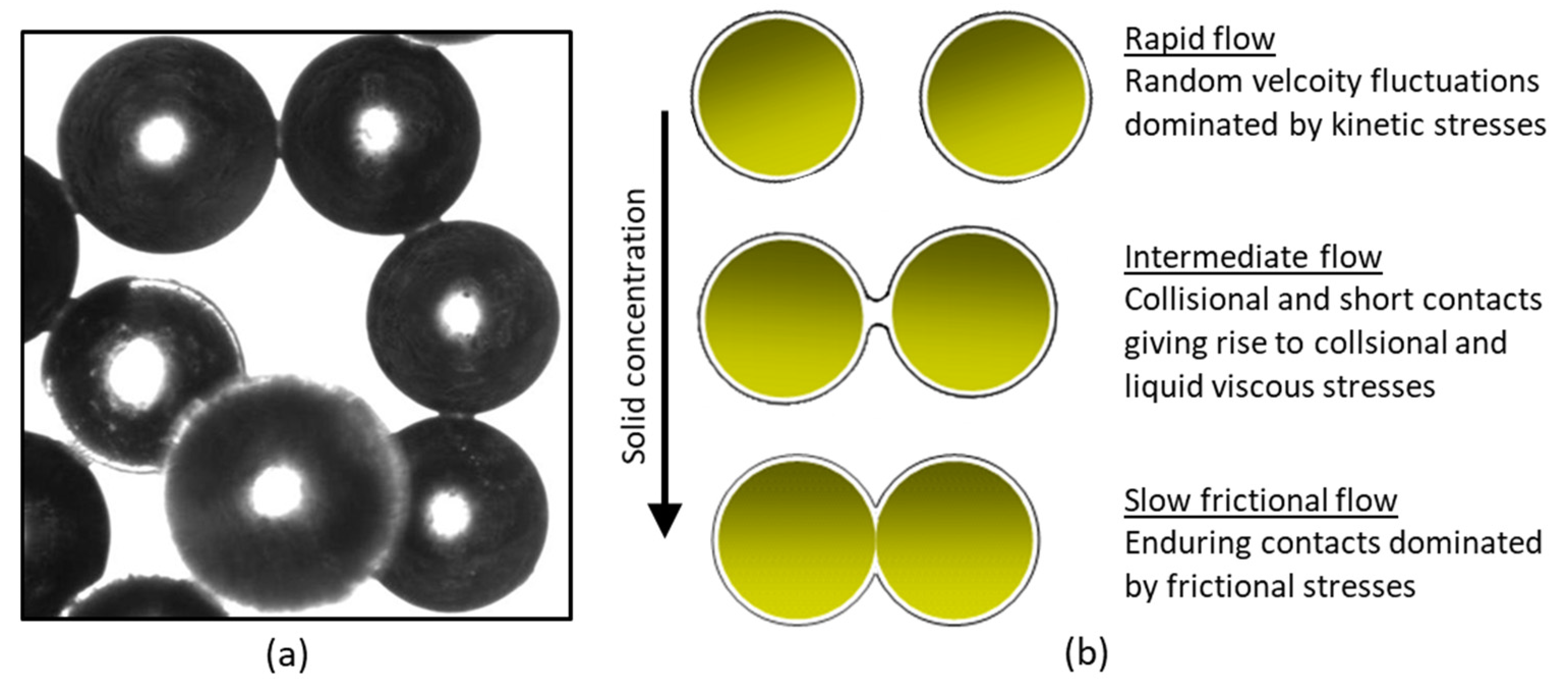
3.1. Solid Stress Tensor
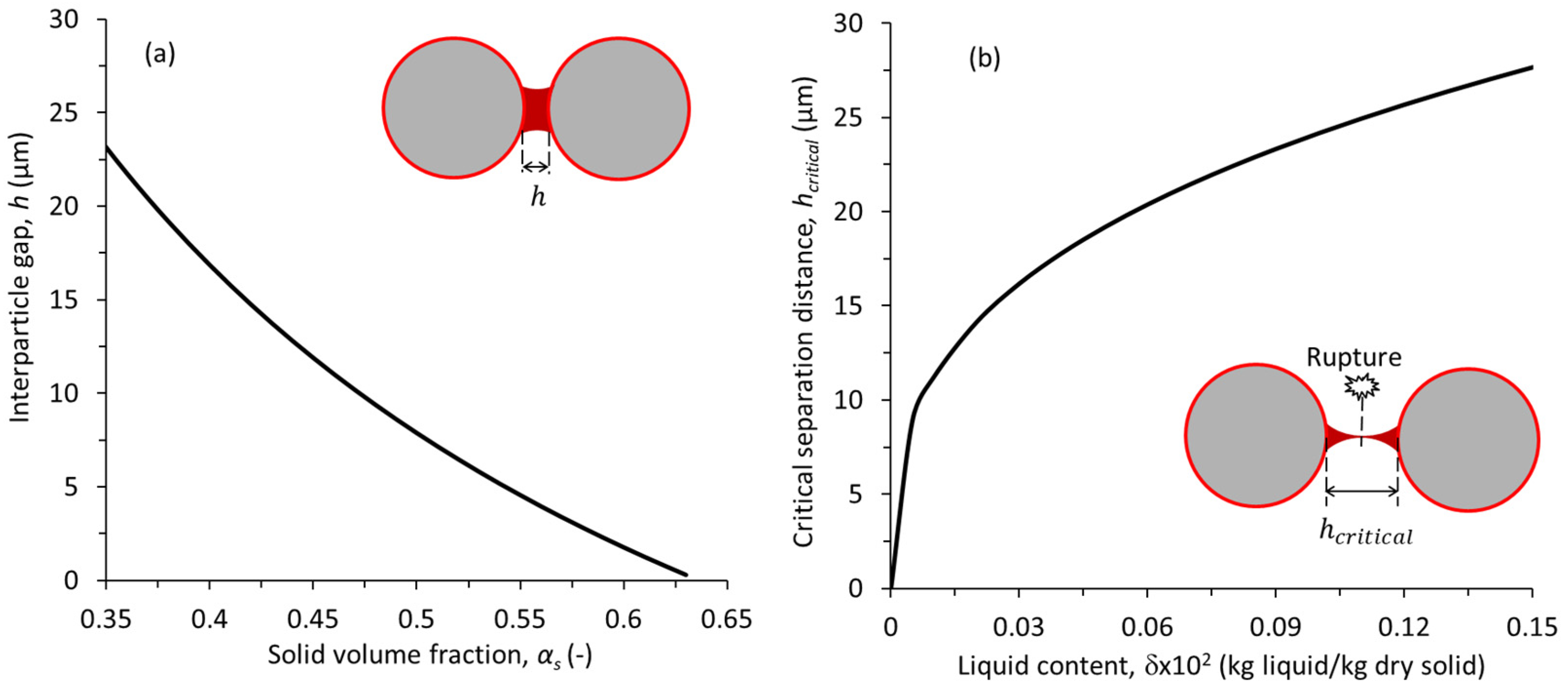
3.2. Inter-Particle Gap and Liquid Bridge Rupture Distance
3.3. Energy Dissipation
4. Experiments
5. Computational Model
5.1. Meshing of the Simulation Domain and Solution Procedure
5.2. Boundary Conditions and Simulation Parameter
6. Results and Discussion
6.1. Boundary Conditions and Simulation Parameter
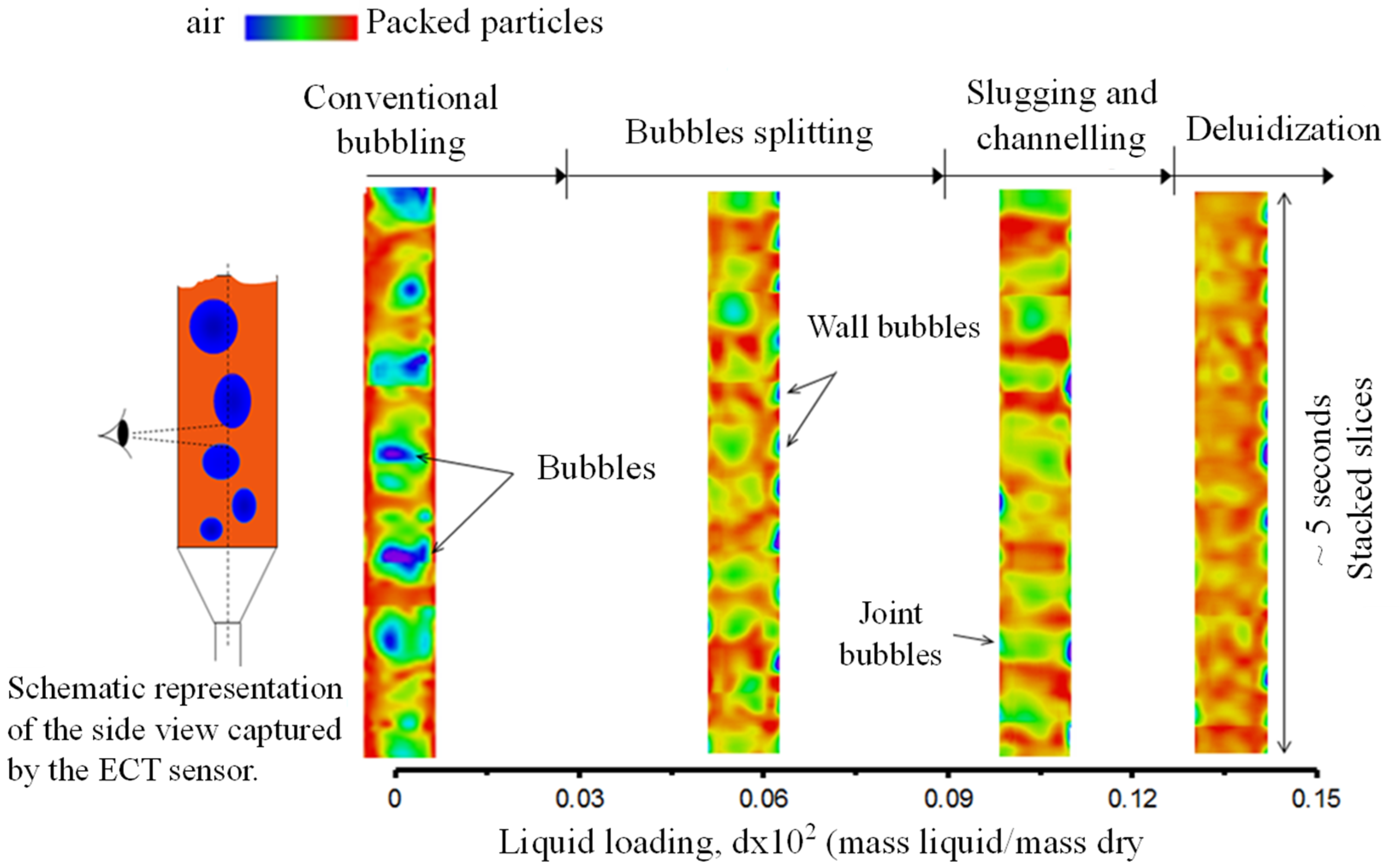
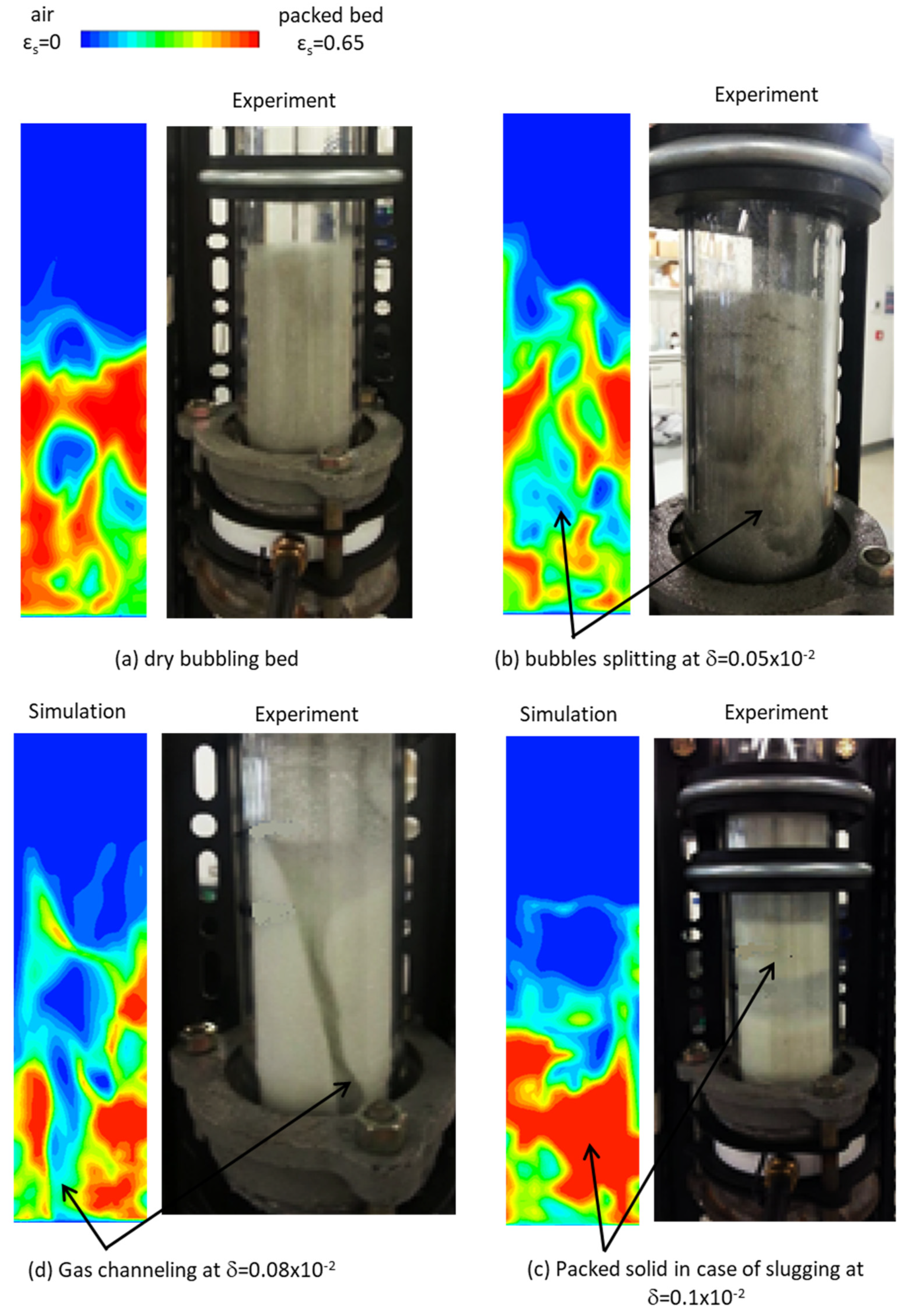
- At a small liquid presence (δ < 0.08 × 10−2) there is a decrease in the bubble size, as a result of bubbles splitting, and intensified wall-bubble movements. This may also change to gas channeling with most of the gas rising through a channel adjacent to the wall. In some way, this resembles the fluidization behavior of Geldart A dry particles [2].
- At an intermediate liquid presence (0.08 × 10−2 < δ < 0.12 × 10−2) slugging is observed (jointly rising bubbles occupying more than half of the column cross-section), typically causing the bed to rise and collapse as a piston. This is of great similarity to the fluidization behavior of highly cohesive powder such as Geldart C dry particles [2].
- At a considerably large liquid presence (δ > 0.12 × 10−2) the bed becomes de-fluidized with the gas mainly escaping through one or more channels.
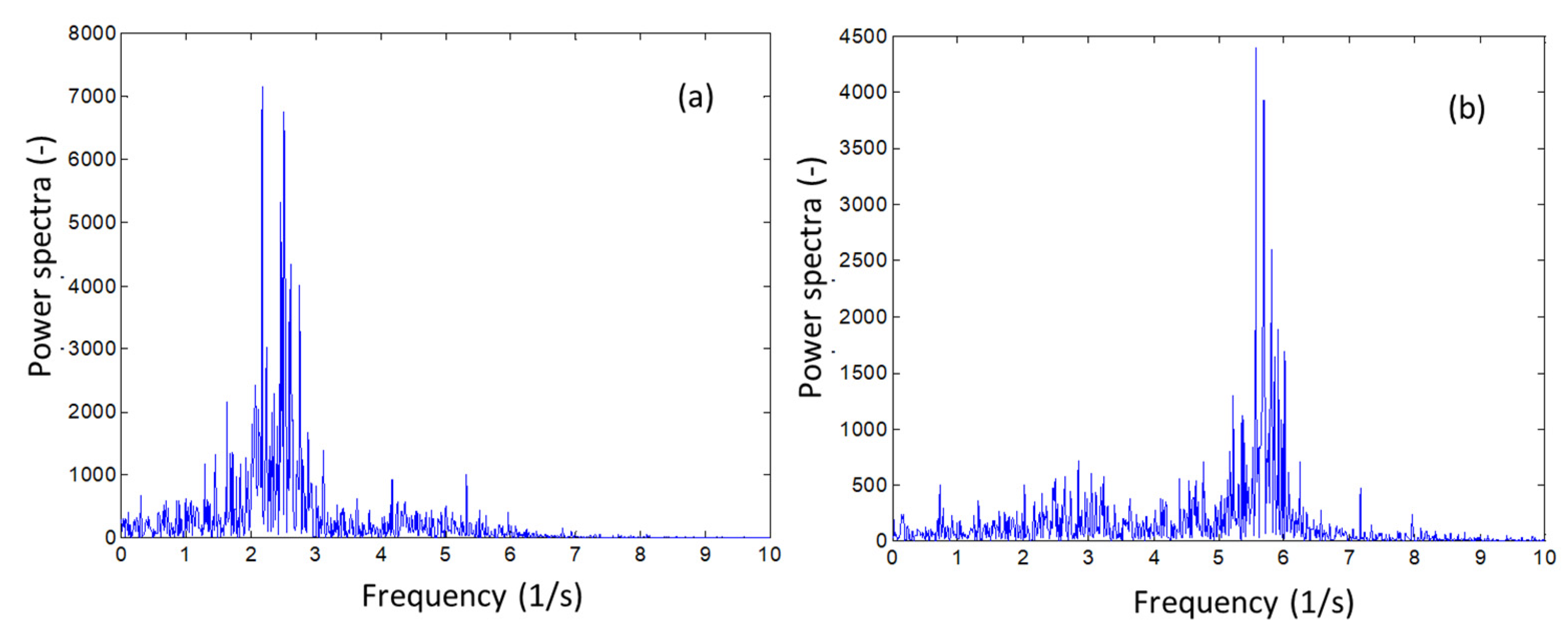
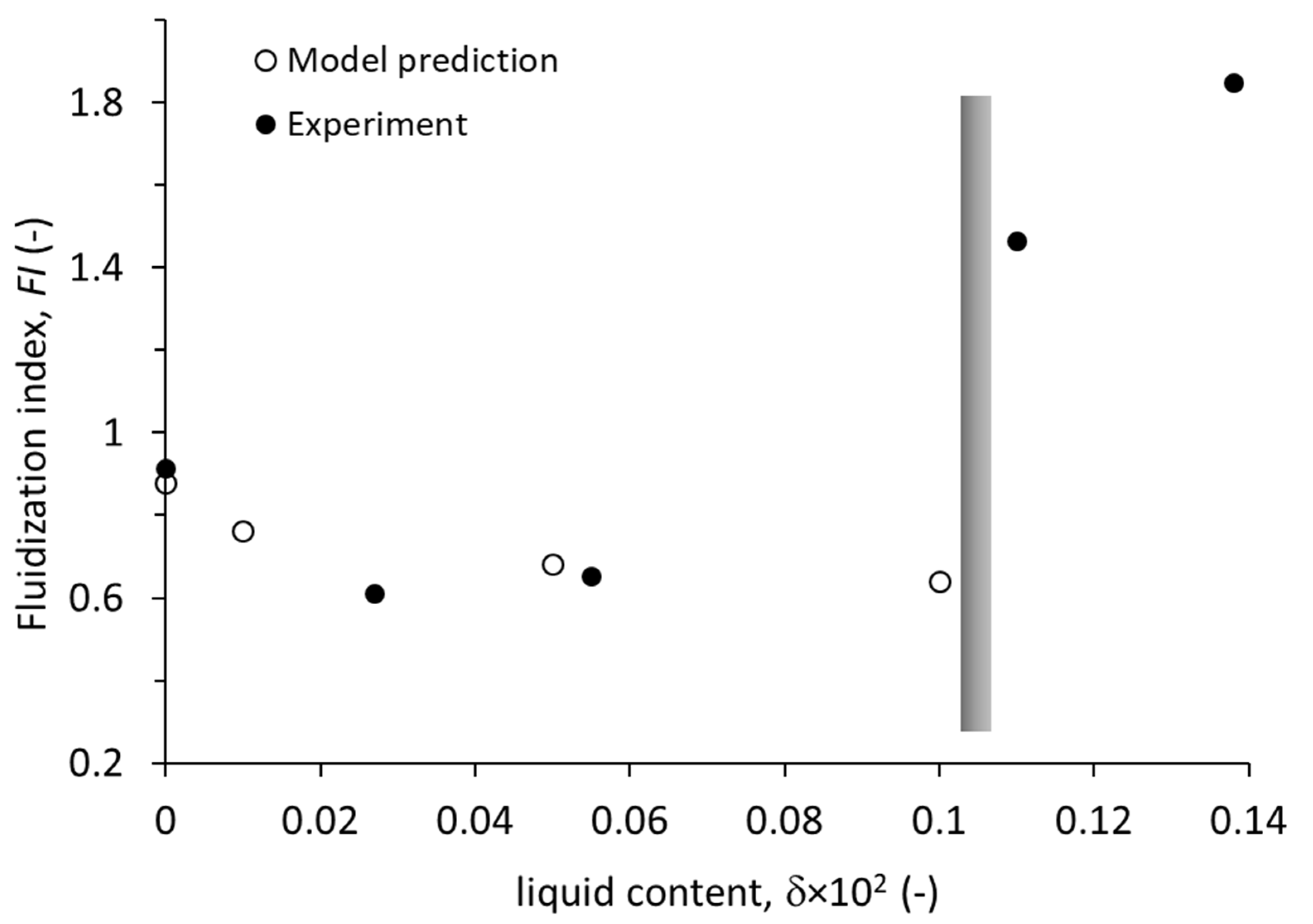
6.2. Comparison of the Experiment Results with the Model Predictions
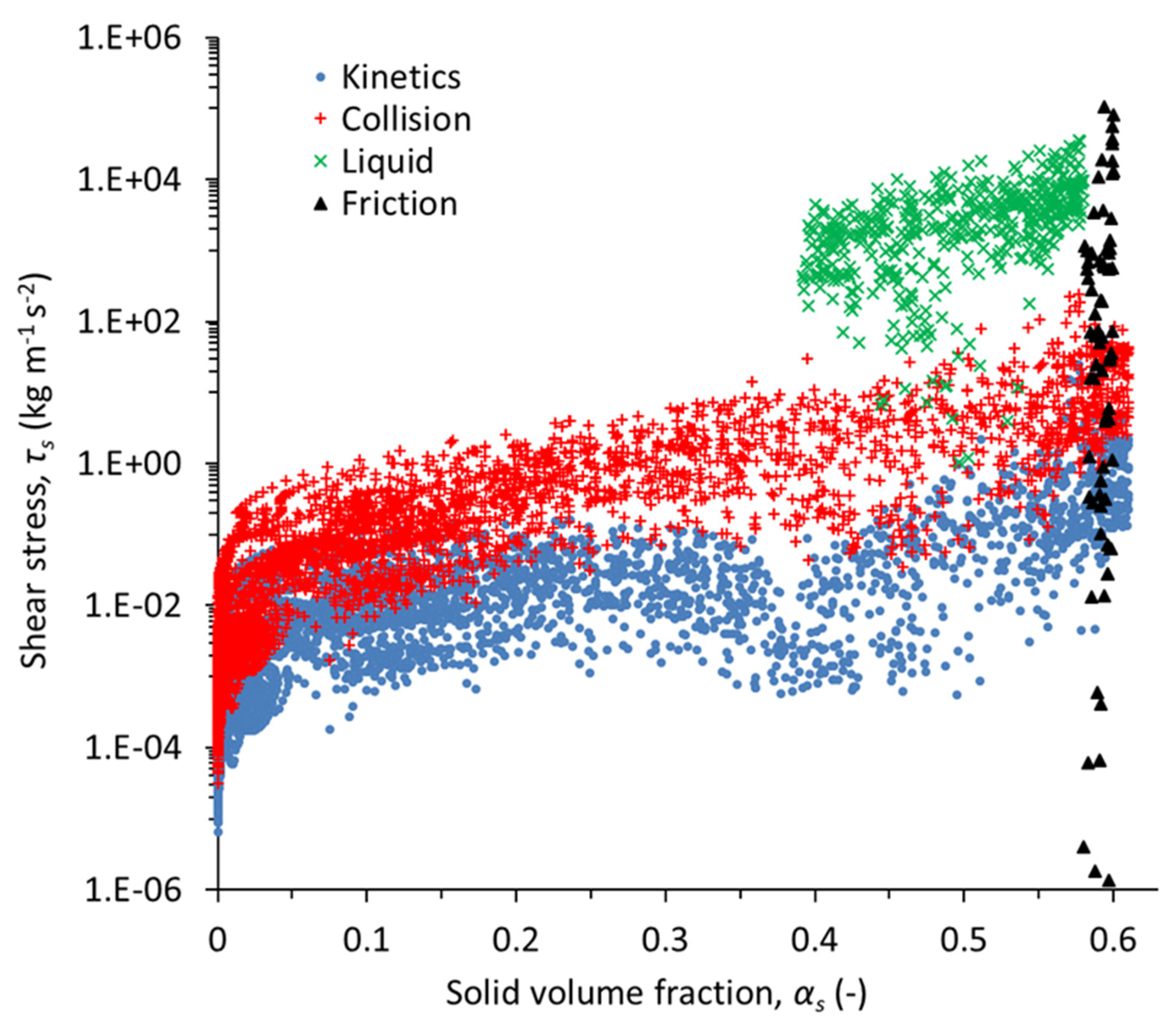
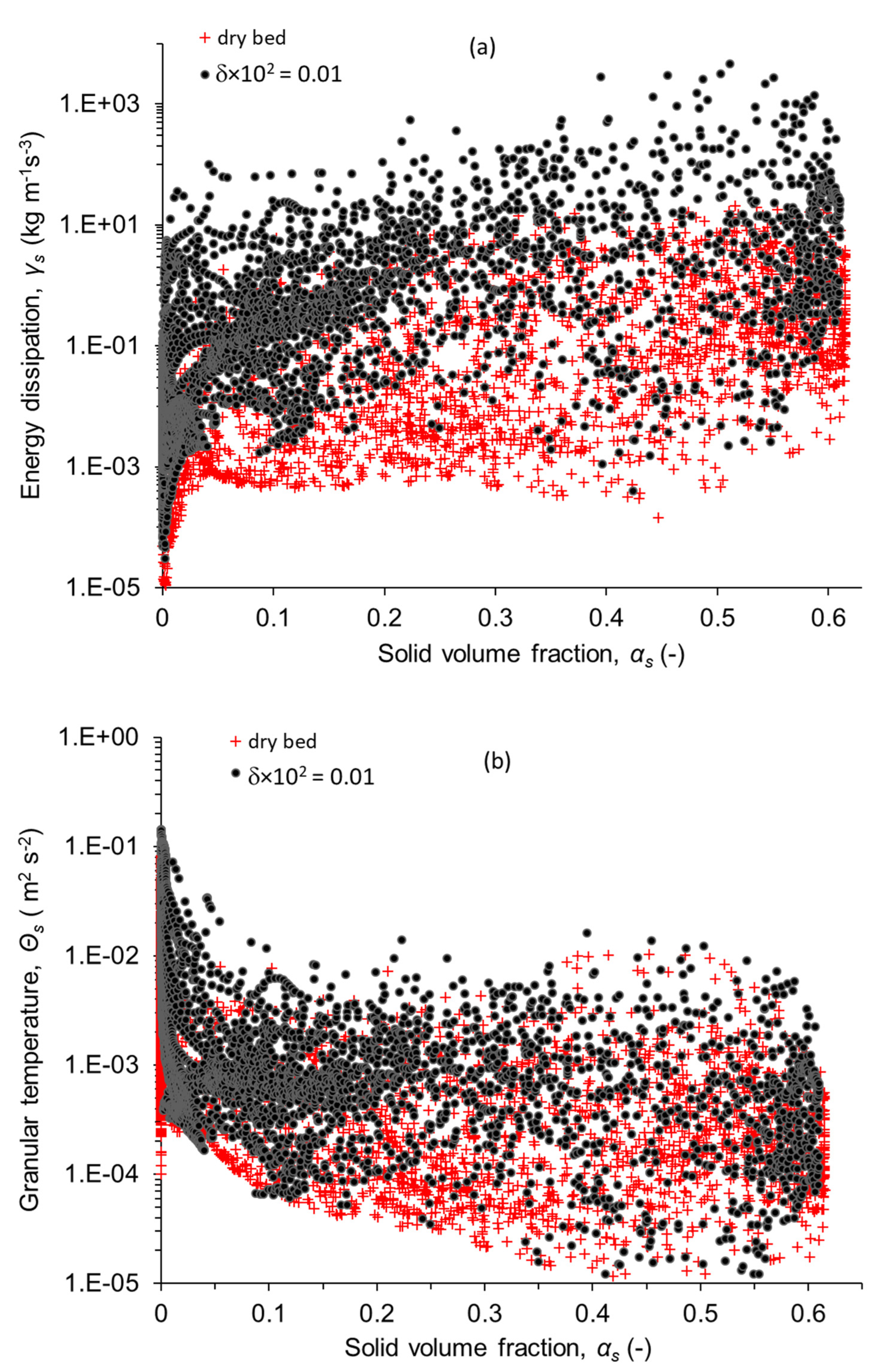
6.3. Solid Shear Stress, Energy Dissipation, and Granular Temperature
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| Notations | |
| Courant number (-) | |
| drag coefficient (-) | |
| particle diameter of solid phase (m) | |
| dry particle-particle restitution coefficient (-) | |
| particle-particle restitution coefficient (-) | |
| particle-wall restitution coefficient (-) | |
| wet particle-particle restitution coefficient (-) | |
| dynamic liquid bridge force (kg m s−2) | |
| gravity (m s−2) | |
| Radial distribution function (-) | |
| inter-particle gap (m) | |
| particle surface asperity (m) | |
| critical separation distance (m) | |
| Unit vector (-) | |
| second invariant of the deviatoric stress tensor (s−2) | |
| liquid and solid mass (kg) | |
| number of particles per unit area (m−2) | |
| pressure (pa) | |
| strain rate (s−1) | |
| dimensionless Stokes number (-) | |
| critical dimensionless Stokes number (-) | |
| Reynolds number of solid phase (-) | |
| Time (s) | |
| particle approach/collission velocity (m s−1) | |
| gas and solid velocity vector (m s−1) | |
| particle velocity at wall (m s−1) | |
| terminal velocity correlation (-) | |
| Greek symbols | |
| volume fraction of gas and solid phase , respectively (-) | |
| minimum solid volume fraction to trigger friction (=0.52) (-) | |
| maximum solid volume fraction at packing (=0.63) (-) | |
| momentum exchange coefficient (kg m−3 s−1) | |
| collisional energy dissipation (kg m−1 s−3) | |
| liquid to dry solid mass ratio (-) | |
| granular temperature of solid phase (m2 s−2) | |
| diffusion coefficient of granular energy (kg m−1 s−1) | |
| liquid dynamic viscosity (kg m−1 s−1) | |
| viscosity of solid phase due to collision (kg m−1 s−1) | |
| viscosity of solid phase due to kinetics (kg m−1 s−1) | |
| viscosity of solid phase due to friction (kg m−1 s−1) | |
| viscosity of solid phase due to liquid bridge (kg m−1 s−1) | |
| Solid, gas, and liquid densities, respectively (kg m−3) | |
| shear stress tensor (kg m−1 s−2) | |
| angle of Internal friction (Degree) | |
| internal angle of lubrication (Degree) | |
| energy exchange between gas-solid phase (kg m−1 s−1) | |
| liquid bridge angle (rad) | |
| specularity coefficient (-) |
References
- Liu, G. Application of the Two-Fluid Model with Kinetic Theory of Granular Flow in Liquid–Solid Fluidized Beds. In Granularity in Materials Science; George, K., Athanasios, C.M., Eds.; IntechOpen: Rijeka, Croatia, 2018. [Google Scholar]
- Geldart, D. Types of gas fluidization. Powder Technol. 1973, 7, 285–292. [Google Scholar] [CrossRef]
- Geldart, D. Gas Fluidization Technology; John Wiley & Sons: Chichester, NY, USA, 1986. [Google Scholar]
- Seville, J.; Clift, R. The effect of thin liquid layers on fluidization characteristics. Powder Technol. 1984, 37, 117–129. [Google Scholar] [CrossRef]
- McLaughlin, L.J.; Rhodes, M.J. Prediction of fluidized bed behavior in the presence of liquid bridges. Powder Technol. 2001, 114, 213–223. [Google Scholar] [CrossRef]
- Makkawi, Y.T. Investigation of Dry and Semi-Wet Fluidized Bed Hydrodynamics Utilizing Twin-Plane Electrical Capacitance Tomography. Ph.D. Thesis, Heriot-Watt University, Edinburgh, Scotland, 2004. [Google Scholar]
- Makkawi, Y.T.; Wright, P.C. Tomographic analysis of dry and semi-wet bed fluidization: The effect of small liquid loading and particle size on the bubbling behavior. Chem. Eng. Sci. 2004, 59, 201–213. [Google Scholar] [CrossRef]
- Tardos, G.; Pfeffer, R. Chemical reaction induced agglomeration and defluidization of fluidized beds. Powder Technol. 1995, 85, 29–35. [Google Scholar] [CrossRef]
- Mikami, T.; Kamiya, H.; Horio, M. Numerical simulation of cohesive powder behavior in a fluidized bed. Chem. Eng. Sci. 1998, 53, 1927–1940. [Google Scholar] [CrossRef]
- Xu, B.; Yu, A.; Chew, S.; Zulli, P. A study of the effect of liquid-induced forces on gas–solid flow by a combined continuum and discrete model. In Proceedings of the Second International Conference on CFD in Minerals & Process Industries CSIRO, Melborne, Australia, 6−8 December 1999. [Google Scholar]
- Jain, K.; Shi, D.; McCarthy, J. Discrete characterization of cohesion in gas–solid flows. Powder Technol. 2004, 146, 160–167. [Google Scholar] [CrossRef]
- Lim, C.; Wee, E.; Tan, H.; Beng, R.; Xiao, Z. Mixing behaviors of wet granular materials in gas fluidized bed systems. AIChE J. 2013, 59, 4058–4067. [Google Scholar]
- He, Y.; Peng, W.; Wang, T.; Yan, S. DEM Study of Wet Cohesive Particles in the Presence of Liquid Bridges in a Gas Fluidized Bed. Math. Probl. Eng. 2014, 2014, 316568. [Google Scholar] [CrossRef]
- Wang, M.; Zhu, W.; Sun, Q.; Zhang, X. A DEM simulation of dry and wet particle flow behaviors in riser. Powder Technol. 2014, 267, 221–233. [Google Scholar] [CrossRef]
- Motlagh, A.H.; Pougatch, K.; Maturi, A.; Salcudean, M.; Grace, J.R.; Grecov, D.; McMillan, J. CFD Study of Wet Agglomerate Growth and Breakage in a Fluidized Bed Containing Hot Silica Sand Particles with Evaporative Liquid Injection. Ind. Eng. Chem. Res. 2019, 58, 4396–4411. [Google Scholar] [CrossRef]
- Berzi1, D.; Fraccarollo, L. Turbulence Locality and Granularlike Fluid Shear Viscosity in Collisional Suspensions. Phys. Rev. Lett. 2015, 115, 194501–194505. [Google Scholar] [CrossRef] [PubMed]
- Tamrakar, A.; Ramachandran, R. CFD–DEM–PBM coupled model development and validation of a 3D top-spray fluidized bed wet granulation process. Comput. Chem. Eng. 2019, 9, 249–270. [Google Scholar] [CrossRef]
- Fries, L.; Antonyuk, S.; Heinrich, S.; Palzer, S. DEM–CFD modeling of a fluidized bed spray granulator. Chem. Eng. Sci. 2011, 66, 2340–2355. [Google Scholar] [CrossRef]
- Atkinson, J.H.; Brandsby, P. Mechanics of Soils; McGraw-Hill: London, UK, 1978. [Google Scholar]
- Samadani, A.; Kudrolli, A. Angle of repose and segregation in cohesive granular matter. Phys. Rev. 2001, 64, 051301. [Google Scholar] [CrossRef] [PubMed]
- Adams, M.; Perchard, V. The cohesive forces between particles with interstitial liquid. Inst. Chem. Eng. Symp. Ser. 1985, 91, 147–160. [Google Scholar]
- Chua, K.W.; Makkawi, Y.T.; Hounslow, M.J. A priori prediction of aggregation efficiency and rate constant for fluidized bed melt granulation. Chem. Eng. Sci. 2013, 98, 291–297. [Google Scholar] [CrossRef]
- Nowak, S.; Samadani, A.; Kudrolli, A. Maximum angle of stability of a wet granular pile. Nat. Phys. 2005, 1, 50–52. [Google Scholar] [CrossRef]
- Woodcock, L.V. Developments in the non-newtonian rheology of glass forming systems. In Molecular Dynamics and Relaxation Phenomena in Glasses: Proceedings of a Workshop Held at the Zentrum für interdisziplinäre Forschung Universität Bielefeld, Bielefeld, FRG 11–13 November 1985; Springer: Berlin/Heidelberg, Germany, 2005; pp. 113–124. [Google Scholar]
- Lian, G.; Thornton, C.; Adams, M.J. A theoretical study of the liquid bridge forces between two rigid spherical bodies. J. Colloid Interface Sci. 1993, 161, 138–147. [Google Scholar] [CrossRef]
- Goldschmidt, M.; Kuipers, J.; Van Swaaij, W. Hydrodynamic modeling of dense gas-fluidised beds using the kinetic theory of granular flow: Effect of coefficient of restitution on bed dynamics. Chem. Eng. Sci. 2001, 56, 571–578. [Google Scholar] [CrossRef]
- Lun, C.; Savage, S.B.; Jeffrey, D.; Chepurniy, N. Kinetic theories for granular flow: Inelastic particles in Couette flow and slightly inelastic particles in a general flowfield. J. Fluid Mech. 1983, 140, 223–256. [Google Scholar] [CrossRef]
- Davis, R.H.; Rager, D.A.; Good, B.T. Elastohydrodynamic rebound of spheres from coated surfaces. J. Fluid Mech. 2002, 468, 107–119. [Google Scholar] [CrossRef]
- Ennis, B.J.; Tardos, G.; Pfeffer, A. Microlevel-Based Characterization of Granulation Phenomena. Powder Technol. 1991, 65, 257–272. [Google Scholar] [CrossRef]
- Thielmann, F.; Naderi, M.; Ansari, M.A.; Stepanek, F. The effect of primary particle surface energy on agglomeration rate in fluidised bed wet granulation. Powder Technol. 2008, 181, 160–168. [Google Scholar] [CrossRef]
- Makkawi, Y.; Wright, P. Optimization of experiment span and data acquisition rate for reliable electrical capacitance tomography measurement in fluidization studies—A case study. Meas. Sci. Technol. 2002, 13, 1831. [Google Scholar] [CrossRef]
- Makkawi, Y.; Ocone, R. Integration of ECT measurements with hydrodynamic modeling of conventional gas–solid bubbling bed. Chem. Eng. Sci. 2007, 62, 4304–4315. [Google Scholar] [CrossRef]
- Kunii, D.; Levenspiel, O. Fluidization Engineering; Howard, B., Ed.; Elsevier: Amsterdam, The Netherlands; Butterworth-Heinemann: Butterworth, Malaysia, 2003. [Google Scholar]
- Ding, J.; Gidaspow, D. A bubbling fluidization model using kinetic theory of granular flow. AIChE J. 1990, 36, 523–538. [Google Scholar] [CrossRef]
- Courant, R.; Friedrichs, K.; Lewy, H. On the partial difference equations of mathematical physics. IBM J. 1967, 11, 215–234. [Google Scholar] [CrossRef]
- Vasquez, S.; Ivanov, V. A phase coupled method for solving multiphase problems on unstructured meshes. In Proceedings of the ASME FEDSM’00: ASME 2000 Fluids Engineering Division Summer Meeting, Boston, MA, USA, 11–15 June 2000. [Google Scholar]
- Johnson, P.C.; Jackson, R. Frictional–collisional constitutive relations for granular materials, with application to plane shearing. J. Fluid Mech. 1987, 176, 67–93. [Google Scholar] [CrossRef]
- De Jong, J.A.; Hoffmann, A.C.; Finkers, J. Properly determine powder flowability to maximize plant output. Chem. Eng. Prog. 1999, 95, 25–34. [Google Scholar]
- Makkawi, Y.; Ocone, R. Modeling the particle stress at the dilute-intermediate-dense flow regimes: A review. KONA Powder Part. J. 2005, 23, 49–63. [Google Scholar] [CrossRef]
- Makkawi, Y.; Wright, P.; Ocone, R. The effect of friction and inter-particle cohesive forces on the hydrodynamics of gas–solid flow: A comparative analysis of theoretical predictions and experiments. Powder Technol. 2006, 163, 69–79. [Google Scholar] [CrossRef]
- Gidaspow, D.; Bezburuah, R.; Ding, J. Hydrodynamics of Circulating Fluidized Beds, Kinetic Theory Approach, in Fluidization VII. In Proceedings of the 7th Engineering Foundation Conference on Fluidization, ECI Symposium Series, Gold Coast, Australia, 3–8 May 1992; pp. 75–82. [Google Scholar]


| Process | Problems |
|---|---|
| Coal/biomass gasification | Oil/tar leading to agglomeration and severe degradation in the low-temperature regions of fluidized bed reactors |
| Catalytic cracking | Surface catalyst melting at high temperatures leads to dead zones and de-fluidization. |
| Pneumatic conveying | Moisture leading to solid slugging, high-pressure drop, wear, and line blockage |
| Fluidized bed coating/drying | Liquid presence leads to undesired agglomeration and particle segregation |
| Particle | Glass beads, Density = 2500 [kg/m3] Diameter = 350 [µm] |
| Fluidization velocity | Up to 0.8 [m/s] |
| Fluidization medium | Air at ambient condition |
| liquid used in wet condition | Silicon oil (Fluid 500, Dow Corning Ltd., UK) dynamic viscosity = 0.4945 [kg/m.s] surface tension = 0.0165 [N/m] density = 969 [kg/m3] |
| Statics bed height | 13 [cm] (large column) 5 [cm] (small column) |
| Liquid content in wet condition | = 0.027 − 0.138 × 10−2 [-] |
| Solids pressure: where |
| Radial distribution function: |
| Gas-solid drag coefficient: |
| where |
| Diffusion coefficient of granular energy: |
| Kinetic viscosity: |
| Collisional viscosity: |
| Frictional viscosity: |
| Strain rate ( = gas or solid): |
| Fluidization velocity, U | 0.8 [m/s] |
| Gas outlet pressure, | 0 [Pag] |
| Wall-particle restitution coefficient, | 0.8 [-] |
| Dry particle-particle restitution coefficient, | 0.9 [-] |
| Maximum allowable solid concentration, | 0.61 [-] |
| Critical frictional solid concentration | 0.58 [-] |
| Internal angle of friction, | 30 [deg] |
| liquid contact angle, | 0.175 [rad] |
| Specularity coefficient, | 0.5 [-] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Makkawi, Y.; Yu, X.; Ocone, R.; Generalis, S. Continuum Modeling of Slightly Wet Fluidization with Electrical Capacitance Tomograph Validation. Energies 2024, 17, 2656. https://doi.org/10.3390/en17112656
Makkawi Y, Yu X, Ocone R, Generalis S. Continuum Modeling of Slightly Wet Fluidization with Electrical Capacitance Tomograph Validation. Energies. 2024; 17(11):2656. https://doi.org/10.3390/en17112656
Chicago/Turabian StyleMakkawi, Yassir, Xi Yu, Raffaella Ocone, and Sotos Generalis. 2024. "Continuum Modeling of Slightly Wet Fluidization with Electrical Capacitance Tomograph Validation" Energies 17, no. 11: 2656. https://doi.org/10.3390/en17112656
APA StyleMakkawi, Y., Yu, X., Ocone, R., & Generalis, S. (2024). Continuum Modeling of Slightly Wet Fluidization with Electrical Capacitance Tomograph Validation. Energies, 17(11), 2656. https://doi.org/10.3390/en17112656






_Constantinou_Generalis.png)


