Harvesting Electric Energy Using Thermoelectric Generators in a Residential Heating Application
Abstract
1. Introduction
- Assess the relative importance of hot-side temperature, cold-side airflow, and cold-side geometry on the performance of an array of TEGs.
- Compare the performance of an array of small TEGs and a single, large TEG of the same surface area.
2. Materials and Methods
2.1. Assessing TEG Performance
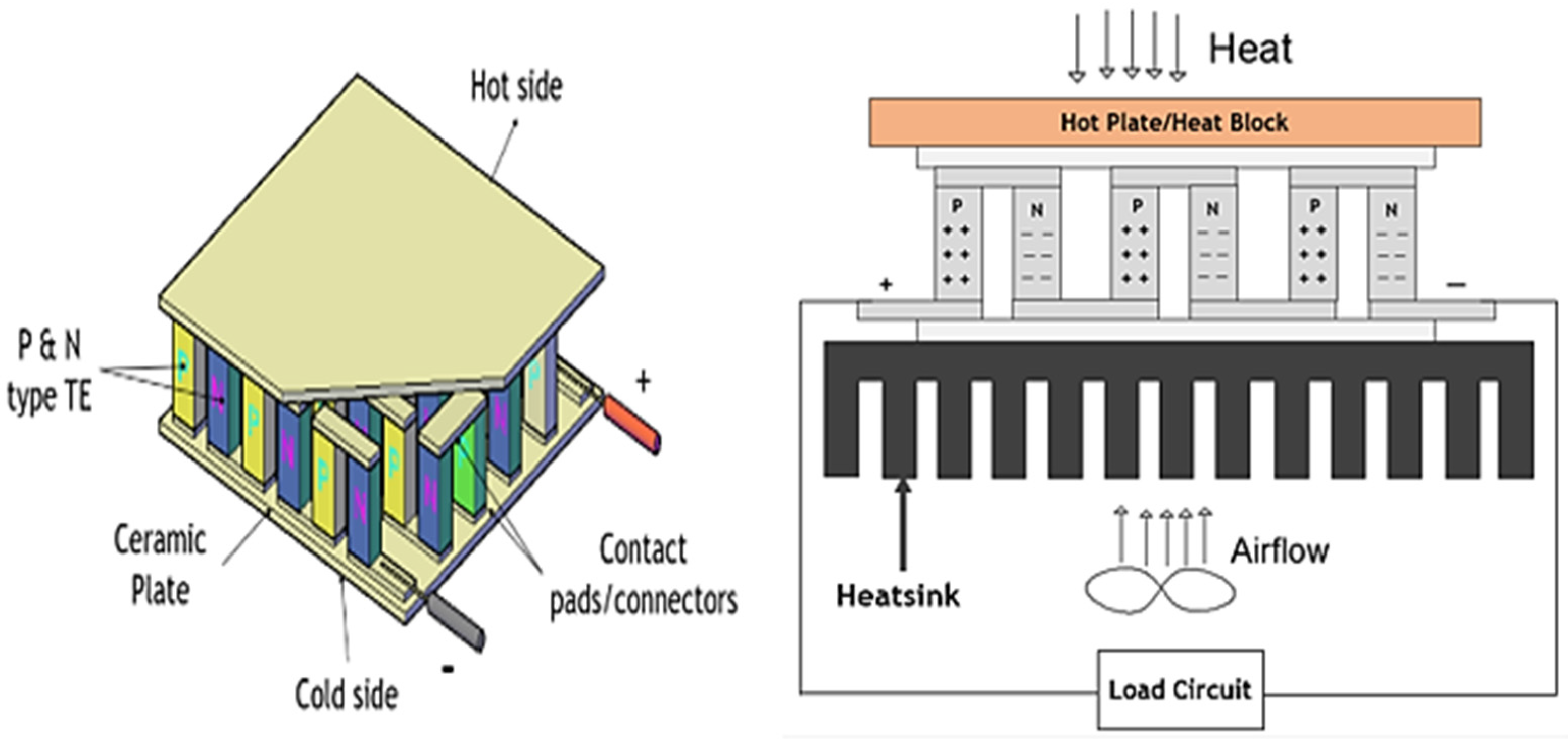
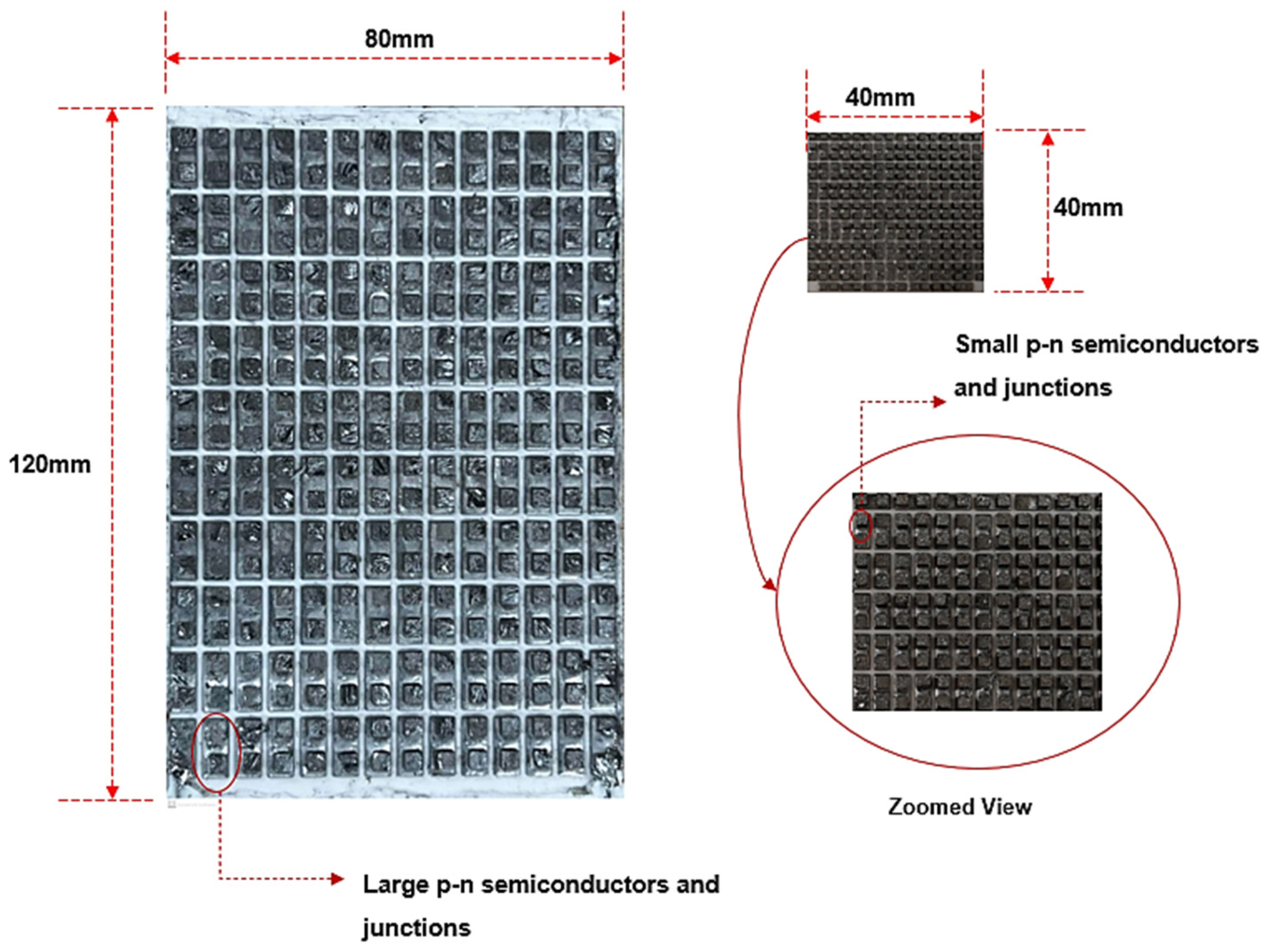
Sample Thermoelectric Generators
2.2. ANOVA and Taguchi Methodologies
2.3. Experimental Set-Up and Test Procedures
2.3.1. Experimental Procedure
2.3.2. Set-Up for the Taguchi Architecture
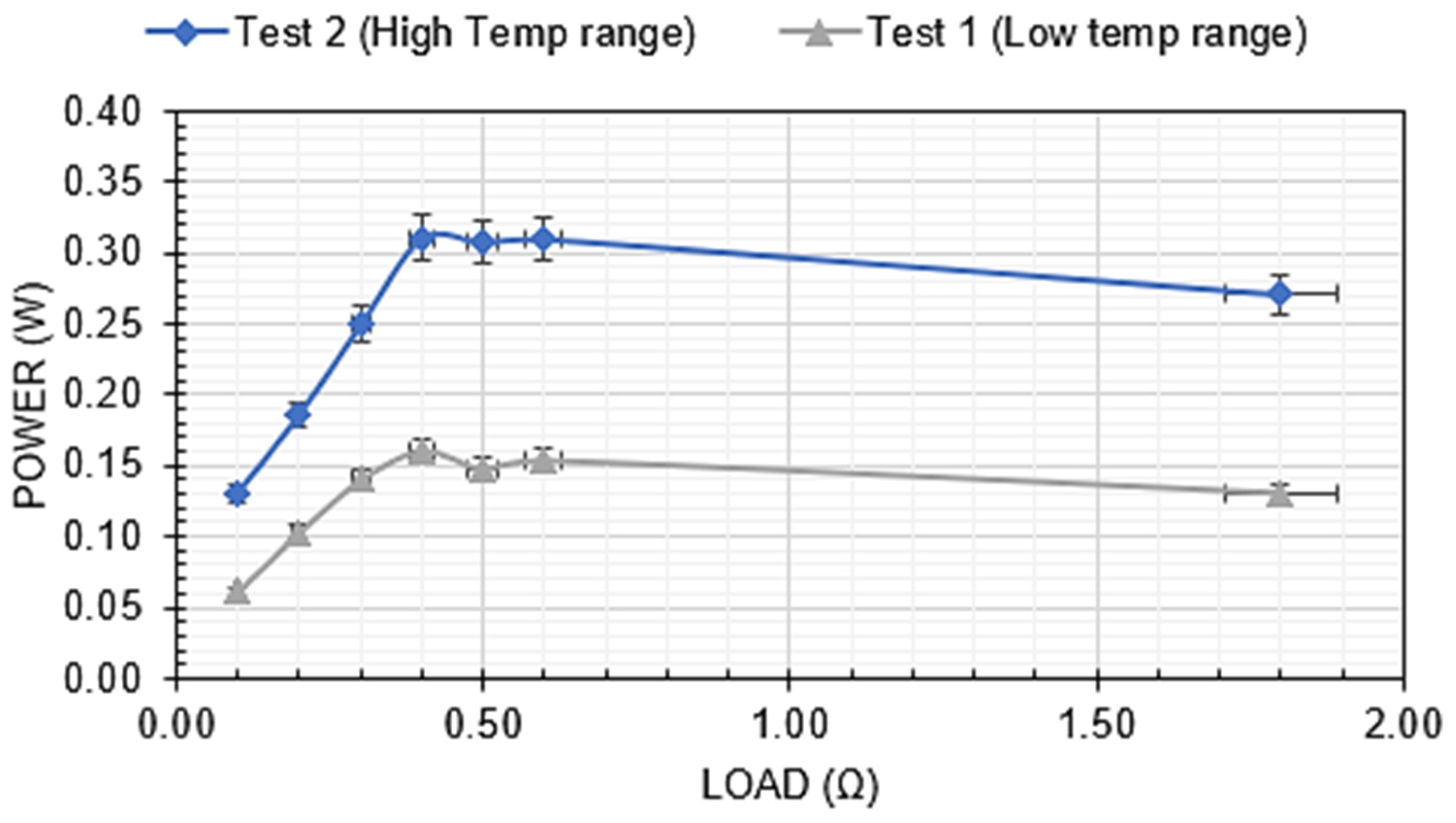
2.4. Establishing the Optimum Load
3. Results and Discussions
3.1. L27 Orthogonal Table
3.2. Main Effects Results
- Null Hypothesis (HO) = The factors and levels of hot-side temperature, air speed, and the air duct configuration do not influence the power output.
- Alternate Hypothesis (HA) = The factors and levels of hot-side temperature, air speed, and the air duct configuration influence the power output.
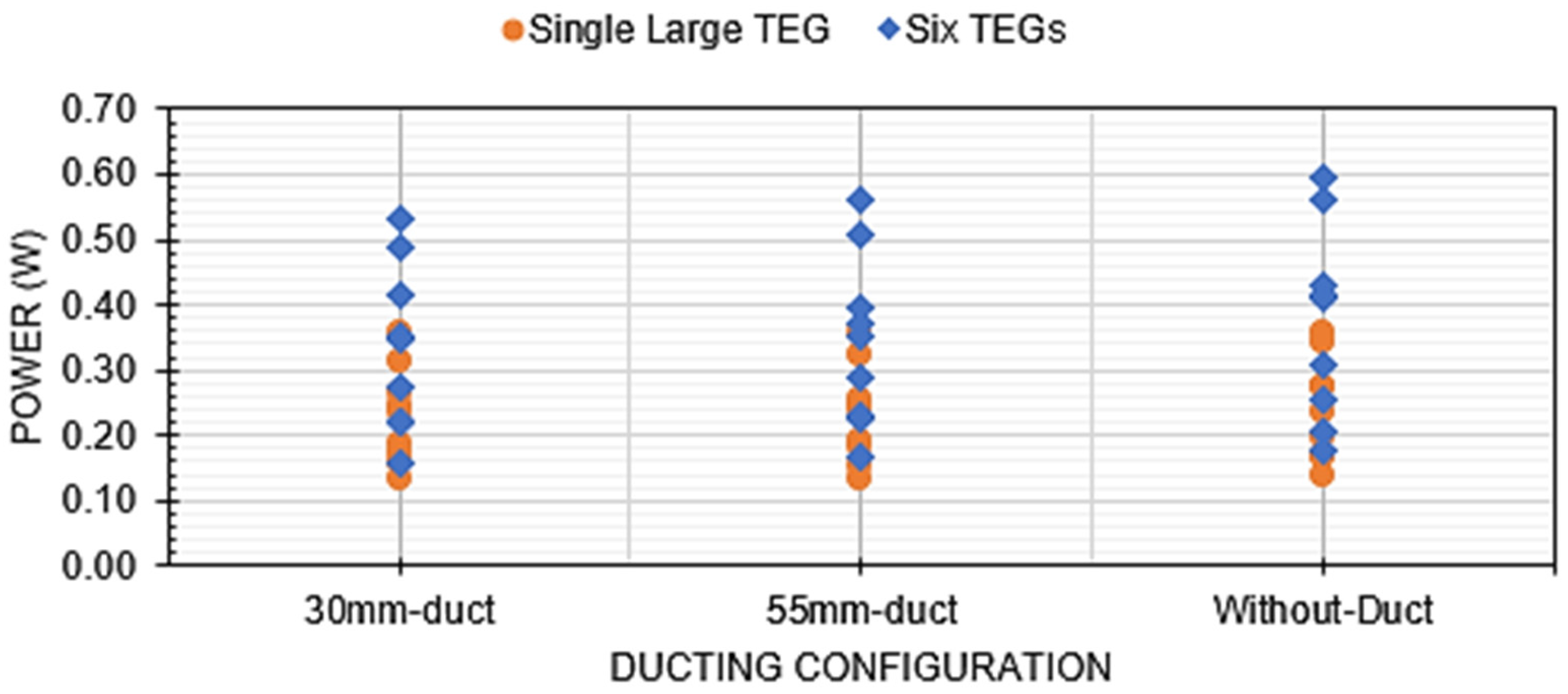
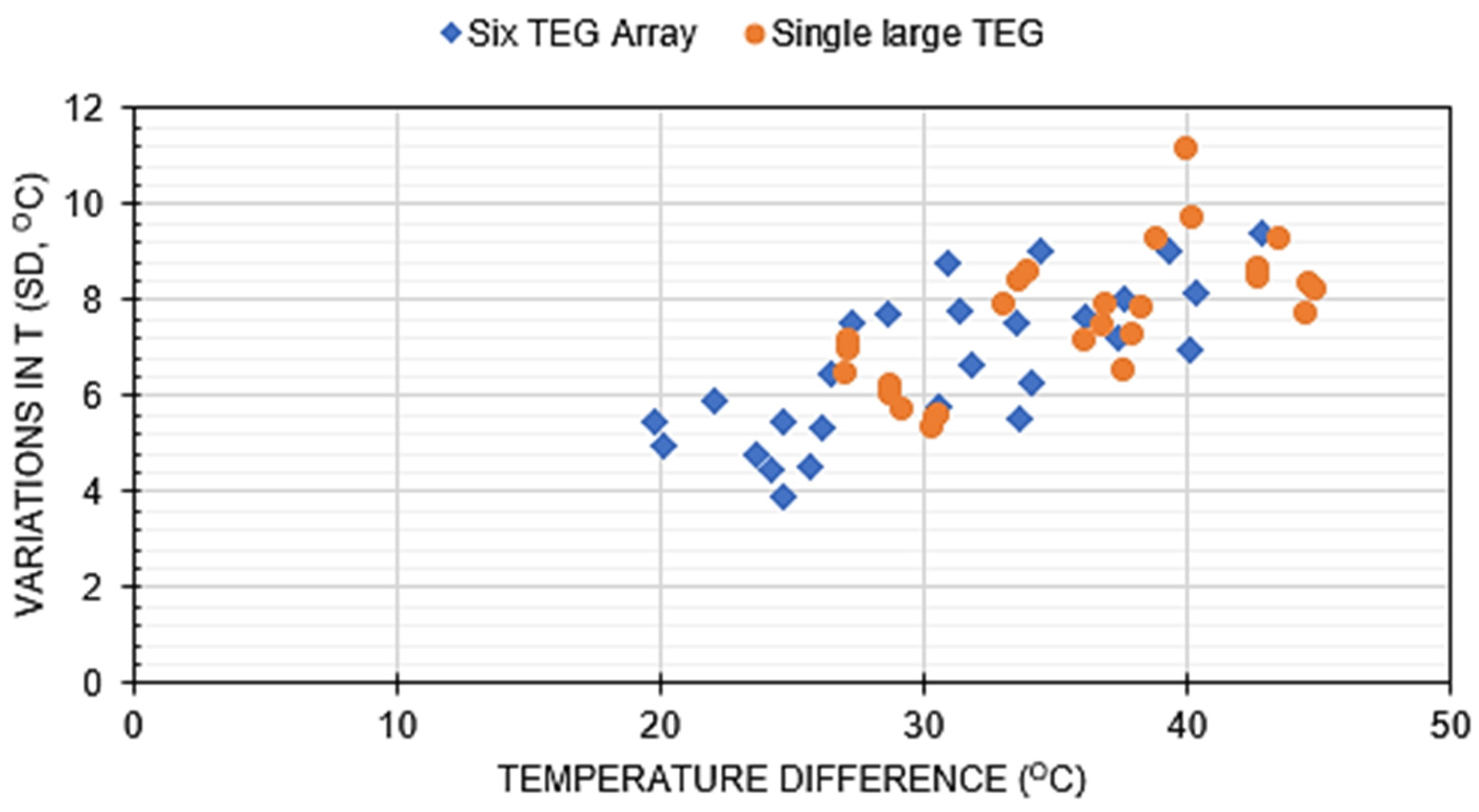
3.2.1. Results Comparison for Single versus Multiple TEG Arrays
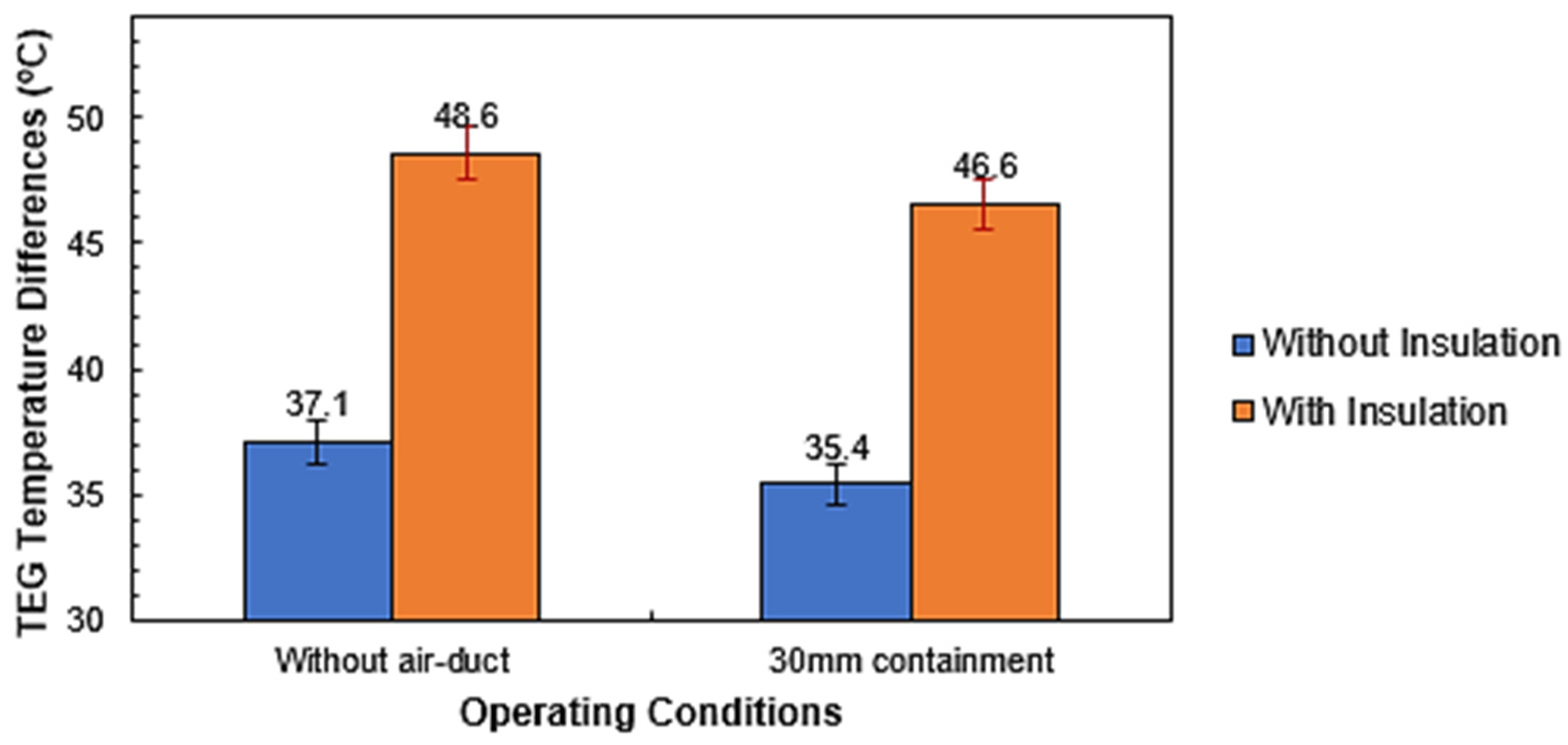
3.2.2. Further Comparison
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| TEG | Thermoelectric Generator |
| ANOVA | Analysis of Variance |
| DOEs | Design of Experiments |
| PCMs | Phase Change Materials |
| ZT | Thermoelectric Figure of Merit |
| DT | Temperature Difference |
| S | Seebeck Coefficient |
| S/S | Samples per Second |
| DAQ | Data Acquisition |
References
- Kane, A.; Verma, V.; Singh, B. Temperature dependent analysis of thermoelectric module using Matlab/SIMULINK. In Proceedings of the 2012 IEEE International Conference on Power and Energy (PECon), Kota Kinabalu, Malaysia, 2–5 December 2012; pp. 632–637. [Google Scholar] [CrossRef]
- Beretta, D.; Neophytou, N.; Hodges, J.M.; Kanatzidis, M.G.; Narducci, D.; Gonzalez, M.M.; Beekman, M.; Balke, B.; Cerretti, G.; Tremel, W.; et al. Thermoelectrics: From history, a window to the future. Mater. Sci. Eng. R Rep. 2019, 138, 100501. [Google Scholar] [CrossRef]
- Jouhara, H.; Żabnieńska-Gńra, A.; Khordehgah, N.; Doraghi, Q.; Ahmad, L.; Norman, L.; Axcell, B.; Wrobel, L.; Dai, S. Thermoelectric generator (TEG) technologies and applications. Int. J. Thermofluids 2021, 9, 100063. [Google Scholar] [CrossRef]
- Mal, R.; Prasad, R.; Vijay, V.K. Multi-functionality clean biomass cookstove for off-grid areas. Process. Saf. Environ. Prot. 2016, 104, 85–94. [Google Scholar] [CrossRef]
- Champier, D.; Bédécarrats, J.P.; Kousksou, T.; Rivaletto, M.; Strub, F.; Pignolet, P. Study of a TE (thermoelectric) generator incorporated in a multifunction wood stove. Energy 2011, 36, 1518–1526. [Google Scholar] [CrossRef]
- Khan, Z.; Khan, Z.; Ghafoor, A. A review of performance enhancement of PCM based latent heat storage system within the context of materials, thermal stability and compatibility. Energy Convers. Manag. 2016, 115, 132–158. [Google Scholar] [CrossRef]
- Mižáková, J.; Piteľ, J.; Hošovský, A.; Pavlenko, I.; Ochowiak, M.; Khovanskyi, S. Biomass Combustion Control in Small and Medium-Scale Boilers Based on Low Cost Sensing the Trend of Carbon Monoxide Emissions. Processes 2021, 9, 2030. [Google Scholar] [CrossRef]
- Lyubomirsky, I.; Lyakhovitskaya, V.; Guillemoles, J.F.; Riess, I.; Triboulet, R.; Cahen, D. Evidence for thermodynamically stable p/n junc-tion, formed by Ag doping of (Hg,Cd)Te. J. Cryst. Growth 1996, 161, 90–93. [Google Scholar] [CrossRef]
- Kondaguli, R.S.; Malaji, P.V. Geometry design and performance evaluation of thermoelectric generator. Eur. Phys. J. Spec. Top. 2022, 231, 1587–1597. [Google Scholar] [CrossRef]
- Terasaki, I. 1.09—Thermal Conductivity and Thermoelectric Power of Semiconductors. In Comprehensive Semiconductor Science and Technology; Bhattacharya, P., Fornari, R., Kamimura, H., Eds.; Elsevier: Amsterdam, The Netherlands, 2011; pp. 326–358. [Google Scholar] [CrossRef]
- Schwurack, R.; Bärschneider, J.; Unz, S.; Beckmann, M. Performance enhancement of a thermoelectric system with improved natural convection cooling by utilizing the chimney effect. Energy Convers. Manag. 2021, 237, 114118. [Google Scholar] [CrossRef]
- Susanto, F.; Salim, A.T.A.; Romandoni, N.; Wahyudi, N.; Indarto, B.; Junaedi, Z.M.A.; Basyar, K.A.; Furqan, J.A.; Putra, G.A.B. Application of Thermoelectric Generator TEG Type Parallel Series Electric Circuit Produces Electricity from Heat Rocket Stove. J. Phys. Conf. Ser. 2021, 1845, 012036. [Google Scholar] [CrossRef]
- Karami, N.; Moubayed, N. New modeling approach and validation of a thermoelectric generator. In Proceedings of the 2014 IEEE 23rd International Symposium on Industrial Electronics (ISIE), Istanbul, Turkey, 1–4 June 2014; pp. 586–591. [Google Scholar] [CrossRef]
- Liang, G.; Zhou, J.; Huang, X. Analytical model of parallel thermoelectric generator. Appl. Energy 2011, 88, 5193–5199. [Google Scholar] [CrossRef]
- Bayendang, N.P.; Kahn, M.T.; Balyan, V. Thermoelectric Generators (TEGs) and Thermoelectric Coolers (TECs) Modeling and Optimal Operation Points Investigation. Adv. Sci. Technol. Eng. Syst. J. 2022, 7, 60–78. [Google Scholar] [CrossRef]
- Barry, M.M. Analytical and Experimental Studies of Thermoelectric Devices and Materials. Ph.D. Thesis, University of Pittsburgh, Pittsburgh, PA, USA, 2016. Available online: https://www.proquest.com/docview/1844067912/abstract/91d65fac3a12441apq/1 (accessed on 27 November 2023).
- Siddiqui, F. Study of TEG When Connected in Series and Parallel Combinations Along with a DC-DC Converter. 2017. Available online: https://www.semanticscholar.org/paper/Study-of-TEG-When-Connected-in-Series-and-Parallel-Siddiqui/1943778b09f0e88269a469e29fe5167e4f6ded18 (accessed on 16 May 2024).
- Terzioğlu, H. Analysis of effect factors on thermoelectric generator using Taguchi method. Measurement 2020, 149, 106992. [Google Scholar] [CrossRef]
- Chen, W.-H.; Uribe, M.C.; Luo, D.; Jin, L.; Saw, L.H.; Lamba, R. Taguchi optimization and analysis of variance for thermoelectric generators with forced convection air cooling. Appl. Therm. Eng. 2023, 231, 120878. [Google Scholar] [CrossRef]
- Shende, D.M.D.; Sarje, D.S.H.; Borse, D.S.L.; Joshi, D.S.P. Thermal Performance of Radial Heat Sinks under Forced Convection. Eur. Chem. Bull. 2023, 12, 3674–3686. [Google Scholar]
- Chukwurah, U. Experimental Evaluation of the Performance of a Thermoelectric Generator Energy Recovery System in an Adapted Vertical Configuration. Master’s Thesis, SFU Summit Research Repository, Simon Fraser University, Burnaby, BC, Canada, 2024. [Google Scholar]








| Six TEGs AVG Hot Plate Temperature | Large-Size TEG AVG Hot Plate Temperature | ||
|---|---|---|---|
| High (TEG 1, 2, 3) | 102 °C, 114 °C, 110 °C | High (POS 1, 4, 5) | 107 °C, 104 °C, 109 °C |
| Medium (TEG 1, 2, 3) | 89 °C, 100 °C, 96 °C | Medium (POS 1, 4, 5) | 95 °C, 93 °C, 97 °C, |
| Low (TEG 1, 2, 3) | 73 °C, 81 °C, 79 °C | Low (POS 1, 4, 5) | 80 °C, 78 °C, 82 °C |
| Run | Hot-Side Temp | Air Speed | Ducting Type | Power at 30 Ω (Six TEGS) | Power at 0.4 Ω (Large TEG) |
|---|---|---|---|---|---|
| 1 | H | L | 30 mm | 0.35 | 0.24 |
| 2 | L | L | 30 mm | 0.16 | 0.13 |
| 3 | M | H | 55 mm | 0.40 | 0.26 |
| 4 | H | L | W/O duct | 0.42 | 0.27 |
| 5 | H | L | 55 mm | 0.37 | 0.24 |
| 6 | L | H | W/O duct | 0.26 | 0.17 |
| 7 | L | M | 55 mm | 0.23 | 0.15 |
| 8 | M | M | W/O duct | 0.41 | 0.24 |
| 9 | M | H | 30 mm | 0.41 | 0.26 |
| 10 | L | L | 55 mm | 0.17 | 0.13 |
| 11 | M | L | W/O duct | 0.31 | 0.20 |
| 12 | H | H | 30 mm | 0.53 | 0.36 |
| 13 | M | L | 55 mm | 0.29 | 0.19 |
| 14 | H | M | 55 mm | 0.51 | 0.32 |
| 15 | H | H | W/O duct | 0.60 | 0.36 |
| 16 | L | M | W/O duct | 0.21 | 0.16 |
| 17 | M | L | 30 mm | 0.27 | 0.18 |
| 18 | L | H | 55 mm | 0.23 | 0.18 |
| 19 | M | M | 30 mm | 0.35 | 0.24 |
| 20 | L | L | W/O duct | 0.18 | 0.14 |
| 21 | M | H | W/O duct | 0.43 | 0.27 |
| 22 | L | M | 30 mm | 0.22 | 0.16 |
| 23 | H | H | 55 mm | 0.56 | 0.36 |
| 24 | H | M | 30 mm | 0.49 | 0.31 |
| 25 | M | M | 55 mm | 0.35 | 0.24 |
| 26 | L | H | 30 mm | 0.22 | 0.17 |
| 27 | H | M | W/O duct | 0.56 | 0.34 |
| Source | Dependent Variable | Type-III Sum of Squares | Df | Mean Square | F | Sig. |
|---|---|---|---|---|---|---|
| Corrected Model | Six TEG Avg. Power | 0.434 | 6 | 0.072 | 95.647 | <0.001 |
| Large TEG Avg. Power | 0.137 | 6 | 0.023 | 102.896 | <0.001 | |
| Intercept | Six TEG Avg. Power | 3.322 | 1 | 3.322 | 4391.626 | <0.001 |
| Large TEG Avg. Power | 1.467 | 1 | 1.467 | 6620.891 | <0.001 | |
| Hot-Side Temp | Six TEG Avg. Power | 0.353 | 2 | 0.176 | 233.038 | <0.001 |
| Large TEG Avg. Power | 0.112 | 2 | 0.056 | 253.127 | <0.001 | |
| Air Speed | Six TEG Avg. Power | 0.074 | 2 | 0.037 | 48.905 | <0.001 |
| Large TEG Avg. Power | 0.024 | 2 | 0.012 | 54.415 | <0.001 | |
| Air Duct Configuration | Six TEG Avg. Power | 0.008 | 2 | 0.004 | 4.999 | 0.017 |
| Large TEG Avg. Power | 0.001 | 2 | 0.000 | 1.147 | 0.338 | |
| Error | Six TEG Avg. Power | 0.015 | 20 | 0.001 | ||
| Large TEG Avg. Power | 0.004 | 20 | 0.000 | |||
| Total | Six TEG Avg. Power | 3.771 | 27 | |||
| Large TEG Avg. Power | 1.609 | 27 | ||||
| Corrected Total | Six TEG Avg. Power | 0.449 | 26 | |||
| Large TEG Avg. Power | 0.141 | 26 | ||||
| R Squared = 0.969 (Adjusted R Squared = 0.959) | ||||||
| R Squared = 0.966 (Adjusted R Squared = 0.956) | ||||||
| Six TEGs | Single, Large TEG | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Run | Hot-Side Temp. | Air Speed | Air Duct | TEG 1 (°C) | TEG 4 (°C) | TEG 5 (°C) | Power at 30 Ω (W) | POS 1 (°C) | POS 2 (°C) | POS 5 (°C) | Power at 0.4 Ω (W) |
| 1 | H | L | 30 mm | 23 | 33 | 38 | 0.35 | 32 | 35 | 50 | 0.25 |
| 2 | L | L | 30 mm | 15 | 21 | 24 | 0.16 | 22 | 25 | 34 | 0.13 |
| 3 | M | H | 55 mm | 27 | 38 | 37 | 0.40 | 32 | 35 | 47 | 0.26 |
| 4 | H | L | W/O duct | 24 | 38 | 41 | 0.42 | 33 | 37 | 51 | 0.27 |
| 5 | H | L | 55 mm | 22 | 32 | 39 | 0.37 | 33 | 35 | 53 | 0.25 |
| 6 | L | H | W/O duct | 20 | 31 | 27 | 0.26 | 26 | 29 | 36 | 0.17 |
| 7 | L | M | 55 mm | 18 | 26 | 27 | 0.23 | 24 | 26 | 36 | 0.15 |
| 8 | M | M | W/O duct | 25 | 39 | 37 | 0.41 | 30 | 35 | 46 | 0.24 |
| 9 | M | H | 30 mm | 27 | 37 | 37 | 0.41 | 33 | 35 | 45 | 0.26 |
| 10 | L | L | 55 mm | 14 | 21 | 25 | 0.17 | 22 | 24 | 35 | 0.13 |
| 11 | M | L | W/O duct | 20 | 32 | 34 | 0.31 | 27 | 31 | 44 | 0.20 |
| 12 | H | H | 30 mm | 32 | 45 | 43 | 0.53 | 38 | 42 | 53 | 0.36 |
| 13 | M | L | 55 mm | 19 | 29 | 34 | 0.29 | 28 | 30 | 43 | 0.19 |
| 14 | H | M | 55 mm | 28 | 42 | 42 | 0.51 | 36 | 40 | 53 | 0.32 |
| 15 | H | H | W/O duct | 33 | 51 | 45 | 0.60 | 37 | 43 | 54 | 0.36 |
| 16 | L | M | W/O duct | 18 | 29 | 27 | 0.21 | 24 | 27 | 36 | 0.17 |
| 17 | M | L | 30 mm | 19 | 28 | 32 | 0.27 | 27 | 30 | 42 | 0.19 |
| 18 | L | H | 55 mm | 21 | 29 | 28 | 0.23 | 26 | 29 | 37 | 0.18 |
| 19 | M | M | 30 mm | 24 | 33 | 35 | 0.35 | 31 | 34 | 44 | 0.24 |
| 20 | L | L | W/O duct | 15 | 25 | 26 | 0.18 | 22 | 25 | 35 | 0.14 |
| 21 | M | H | W/O duct | 28 | 43 | 38 | 0.43 | 32 | 36 | 46 | 0.27 |
| 22 | L | M | 30 mm | 19 | 26 | 27 | 0.22 | 25 | 27 | 36 | 0.16 |
| 23 | H | H | 55 mm | 31 | 47 | 43 | 0.56 | 38 | 43 | 54 | 0.36 |
| 24 | H | M | 30 mm | 29 | 42 | 41 | 0.49 | 36 | 40 | 52 | 0.31 |
| 25 | M | M | 55 mm | 24 | 35 | 36 | 0.35 | 31 | 35 | 45 | 0.24 |
| 26 | L | H | 30 mm | 20 | 27 | 27 | 0.22 | 26 | 29 | 36 | 0.17 |
| 27 | H | M | W/O duct | 29 | 46 | 43 | 0.56 | 36 | 41 | 54 | 0.35 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chukwurah, U.; McTaggart-Cowan, G. Harvesting Electric Energy Using Thermoelectric Generators in a Residential Heating Application. Energies 2024, 17, 2562. https://doi.org/10.3390/en17112562
Chukwurah U, McTaggart-Cowan G. Harvesting Electric Energy Using Thermoelectric Generators in a Residential Heating Application. Energies. 2024; 17(11):2562. https://doi.org/10.3390/en17112562
Chicago/Turabian StyleChukwurah, Ugochukwu, and Gordon McTaggart-Cowan. 2024. "Harvesting Electric Energy Using Thermoelectric Generators in a Residential Heating Application" Energies 17, no. 11: 2562. https://doi.org/10.3390/en17112562
APA StyleChukwurah, U., & McTaggart-Cowan, G. (2024). Harvesting Electric Energy Using Thermoelectric Generators in a Residential Heating Application. Energies, 17(11), 2562. https://doi.org/10.3390/en17112562






