Time-Dependent Multi-Particle Model Describing the Hydrogen Absorption of Nanocrystalline Magnesium Powders: A Case Study
Abstract
1. Introduction
- (I)
- Physisorption of the H2 molecules by weak van der Waals interaction onto the free surface of magnesium powder particles;
- (II)
- Dissociation of hydrogen molecules into two H atoms;
- (III)
- Chemisorption of hydrogen atoms;
- (IV)
- Diffusion into bulk lattice sites, preferably along grain boundaries and dislocations and as a final step;
- (V)
2. Classical Kinetic (Single Particle) Models of Hydrogenation
2.1. Surface Controlled Model
2.2. Contracting Volume Model
2.3. Johnson–Mehl–Avrami Model
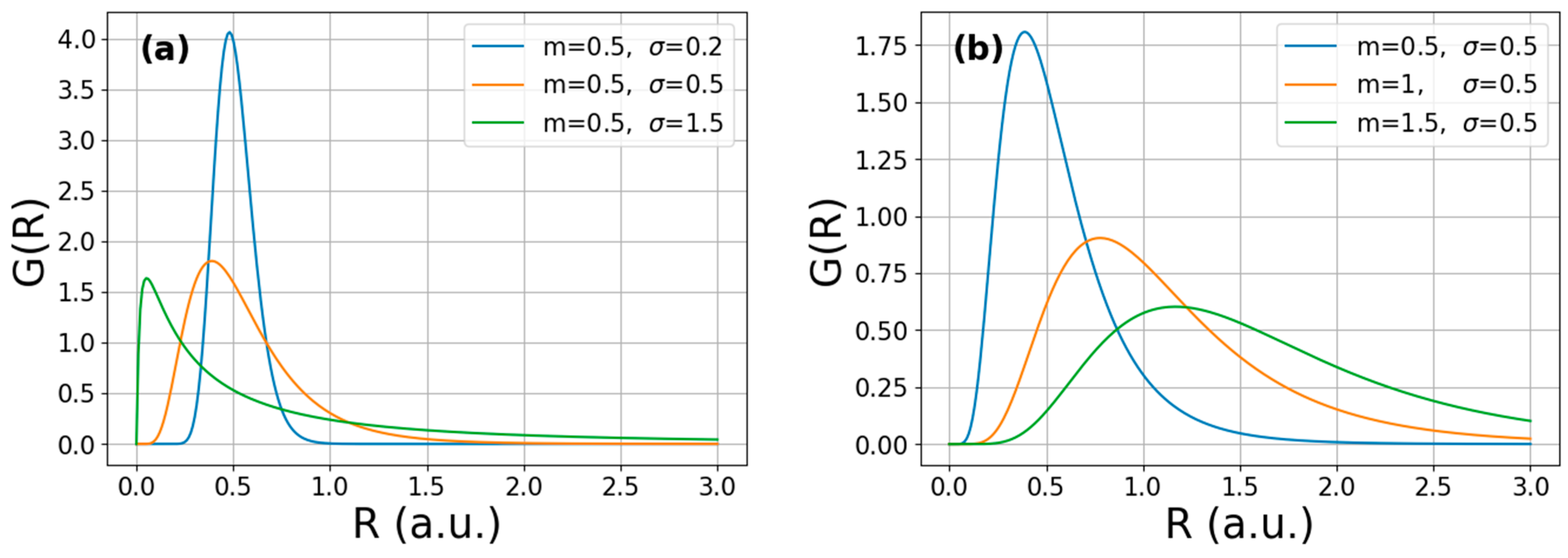
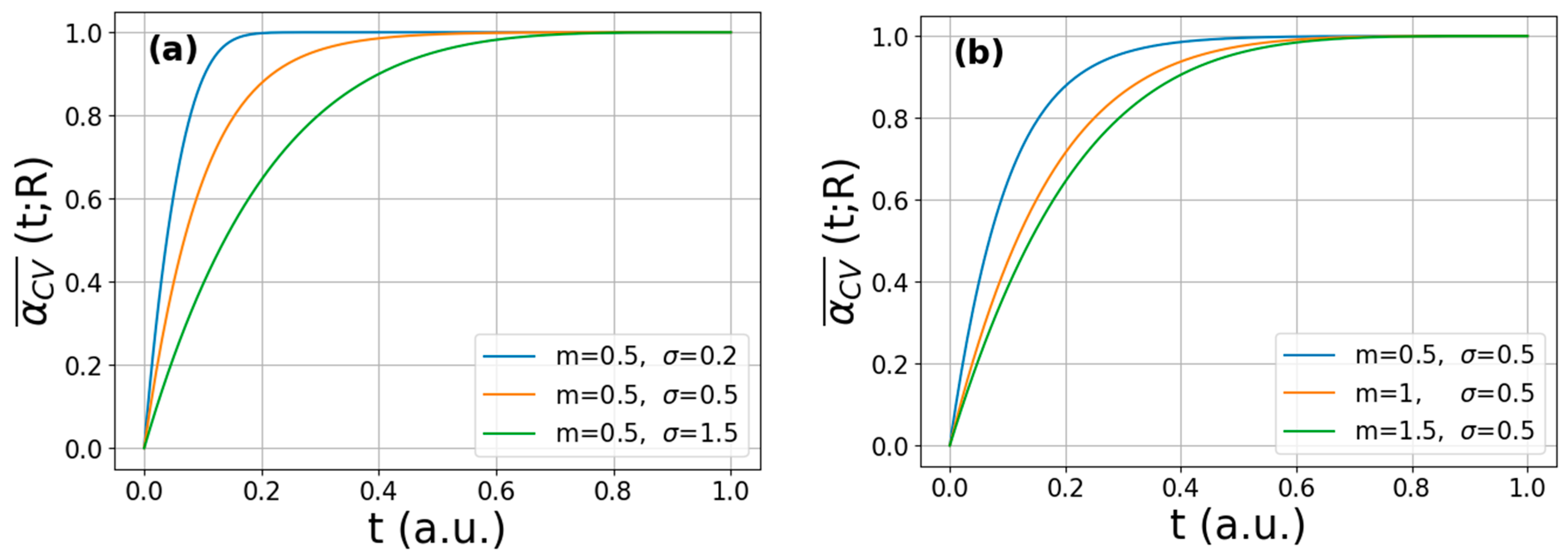
3. Multi-Particle Model of Hydrogenation
4. Experimental Section
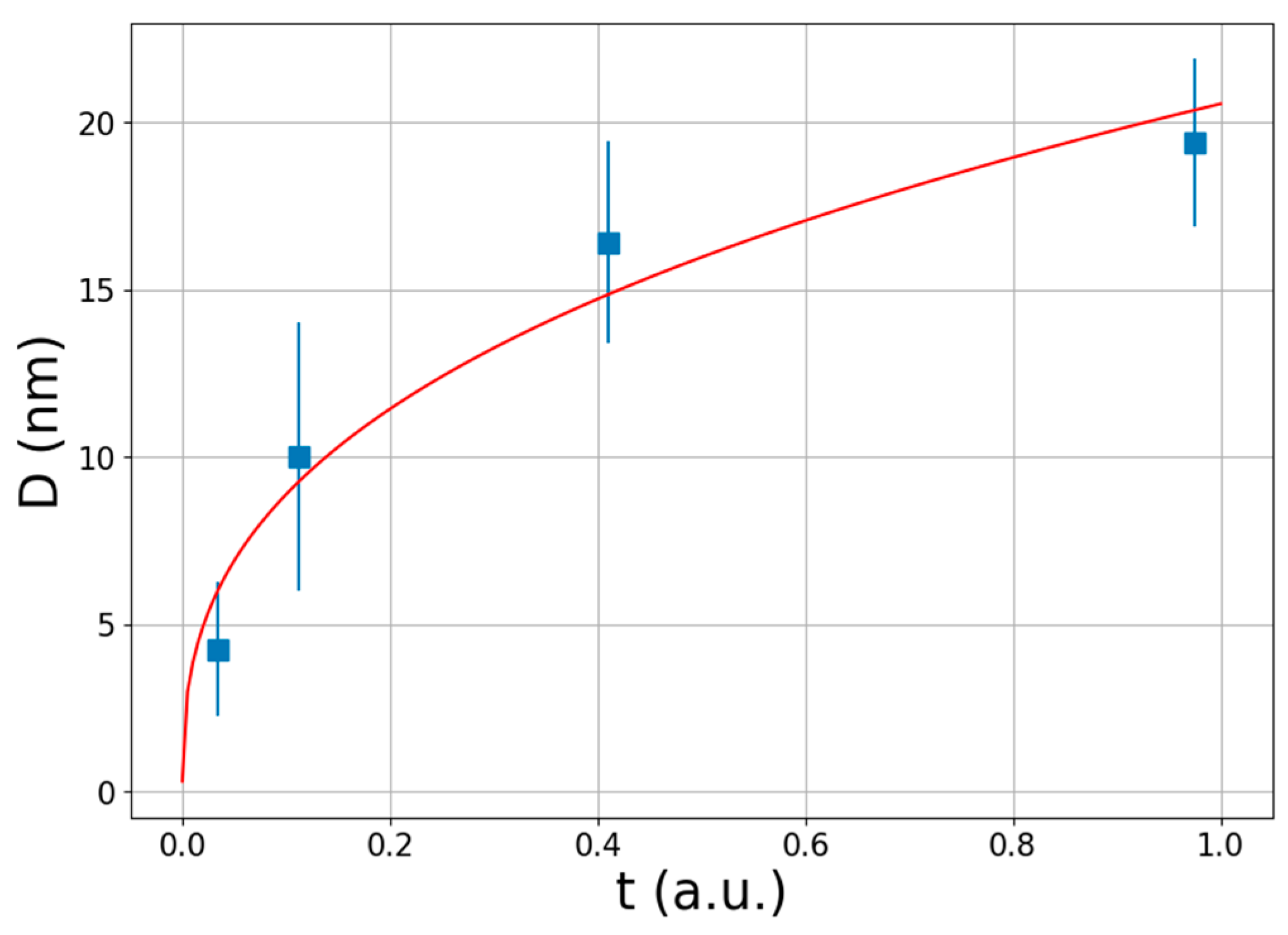
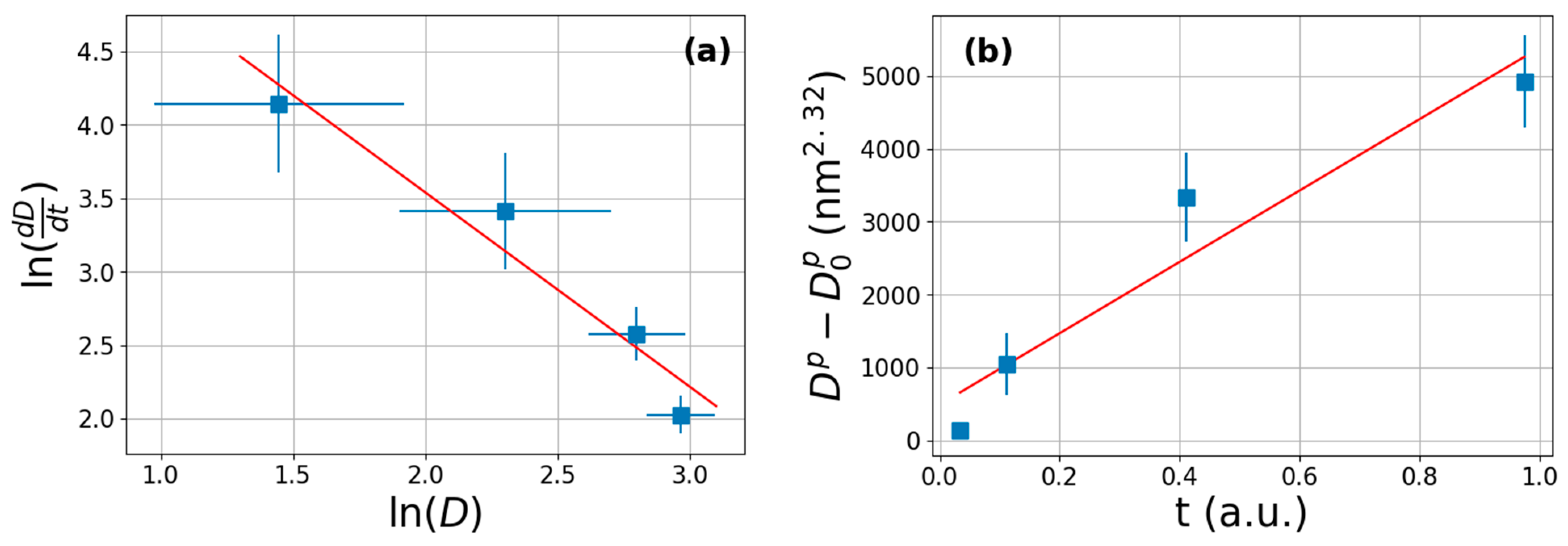
5. Results and Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Borgschulte, A. The Hydrogen Grand Challenge. Front. Energy Res. 2016, 4, 11. [Google Scholar] [CrossRef]
- Jackson, R.B.; Friedlingstein, P. Persistent fossil fuel growth threatens the Paris Agreement and planetary health. Environ. Res. Lett. 2019, 14, 12001. [Google Scholar] [CrossRef]
- Rönnebro, E.C.E.; Majzoub, E.H. Recent advances in metal hydrides for clean energy applications. MRS Bull. 2013, 38, 452–458. [Google Scholar] [CrossRef]
- Abdalla, A.M.; Hossain, S.; Nisfindy, O.B.; Azad, A.T.; Dawood, M.; Azad, A.K. Hydrogen Production, Storage, Transportation and Key Challenges with Applications: A Review. Energy Convers. Manag. 2018, 165, 602–627. [Google Scholar] [CrossRef]
- Hirscher, M.; Yartys, V.A.; Baricco, M.; Bellosta von Colbe, J.; Blanchard, D.; Bowman, R.C.; Broom, D.P.; Buckley, C.E.; Chang, F.; Chen, P.; et al. Materials for hydrogen-based energy storage—Past, recent progress and future outlook. J. Alloys Compd. 2020, 827, 153548. [Google Scholar] [CrossRef]
- Yang, J.; Sudik, A.; Wolverton, C.; Siegel, D.J. High capacity hydrogenstorage materials: Attributes for automotive applications and techniques for materials discovery. Chem. Soc. Rev. 2010, 39, 656–675. [Google Scholar] [CrossRef] [PubMed]
- Schlabach, L.; Zuttel, A. Hydrogen-Storage Materials for Mobile Applications. Nature 2001, 414, 353–358. [Google Scholar] [CrossRef] [PubMed]
- Aguey-Zinsou, K.-F.; Ares-Fernández, J.-R. Hydrogen in Magnesium: New Perspectives toward Functional Stores. Energy Environ. Sci. 2010, 3, 526–543. [Google Scholar] [CrossRef]
- von Colbe, J.B.; Ares, J.-R.; Barale, J.; Baricco, M.; Buckley, C.; Capurso, G.; Gallandat, N.; Grant, D.M.; Guzik, M.N.; Jacob, I.; et al. Application of hydrides in hydrogen storage and compression: Achievements, outlook and perspectives. Int. J. Hydrogen Energy 2019, 44, 7780–7808. [Google Scholar] [CrossRef]
- Lin, H.-J.; Lu, Y.-S.; Zhang, L.-T.; Liu, H.-Z.; Edalati, K.; Révész, Á. Recent Advances in Metastable Alloys for Hydrogen Storage: A Review. Rare Met. 2022, 41, 1797–1817. [Google Scholar] [CrossRef]
- Drawer, C.; Lange, J.; Kaltschmitt, M. Metal Hydrides for Hydrogen Storage—Identification and Evaluation of Stationary and Transportation Applications. J. Energy Storage 2024, 77, 109988. [Google Scholar] [CrossRef]
- Klopčič, N.; Grimmer, I.; Winkler, F.; Sartory, M.; Trattner, A. A review on metal hydride materials for hydrogen storage. J. Energy Storage 2023, 72, 108456. [Google Scholar] [CrossRef]
- Huot, J.; Cuevas, F.; Deledda, S.; Edalati, K.; Filinchuk, Y.; Grosdider, T.; Hauback, B.C.; Heere, M.; Jensen, T.R.; Latroche, M.; et al. Mechanochemistry of Metal Hydrides: Recent Advances. Materials 2019, 12, 2778. [Google Scholar] [CrossRef]
- Liu, Y.; Chabane, D.; Elkedim, O. Intermetallic Compounds Synthesized by Mechanical Alloying for Solid-State Hydrogen Storage: A Review. Energies 2021, 14, 5758. [Google Scholar] [CrossRef]
- Yartys, V.A.; Lototskyy, M.V.; Akiba, E.; Albert, R.; Antonov, V.E.; Ares, J.R.; Baricco, M.; Bourgeois, N.; Buckley, C.E.; Bellosta von Colbe, J.M.; et al. Magnesium based materials for hydrogen based energy storage: Past, present and future. Int. J. Hydrogen Energy 2019, 44, 7809–7859. [Google Scholar] [CrossRef]
- Li, Q.; Peng, X.; Pan, F. Magnesium-Based Materials for Energy Conversion and Storage. J. Magnes. Alloys 2021, 9, 2223–2224. [Google Scholar] [CrossRef]
- Gupta, A.; Faisal, M. Magnesium Based Multi-Metallic Hybrids with Soot for Hydrogen Storage. Int. J. Hydrogen Energy 2024, 53, 93–104. [Google Scholar] [CrossRef]
- Song, J.; She, J.; Chen, D.; Pan, F. Latest Research Advances on Magnesium and Magnesium Alloys Worldwide. J. Magnes. Alloys 2020, 8, 1–41. [Google Scholar] [CrossRef]
- Ouyang, L.; Liu, F.; Wang, H.; Liu, J.; Yang, X.S.; Sun, L.; Zhu, M. Magnesium-based hydrogen storage compounds: A review. J. Alloys Compd. 2020, 832, 154865. [Google Scholar] [CrossRef]
- Li, Z.-Y.; Sun, Y.-J.; Zhang, C.-C.; Wei, S.; Zhao, L.; Zeng, J.-L.; Cao, Z.; Zou, Y.-J.; Chu, H.-L.; Xu, F.; et al. Optimizing hydrogen ad/desorption of Mg-based hydrides for energy-storage applications. Mater Sci. Technol. 2023, 141, 221–235. [Google Scholar] [CrossRef]
- Zhu, M.; Lu, Y.; Ouyang, L.; Wang, H. Thermodynamic Tuning of Mg-Based Hydrogen Storage Alloys: A Review. Materials 2013, 6, 4654–4674. [Google Scholar] [CrossRef] [PubMed]
- Pasquini, L. The Effects of Nanostructure on the Hydrogen Sorption Properties of Magnesium-Based Metallic Compounds: A Review. Crystals 2018, 8, 106. [Google Scholar] [CrossRef]
- Yang, Y.; Zhang, X.; Zhang, L.; Zhang, W.; Liu, H.; Huang, Z.; Yang, L.; Gu, C.; Sun, W.; Gao, M.; et al. Recent Advances in Catalyst-Modified Mg-Based Hydrogen Storage Materials. J. Mater. Sci. Technol. 2023, 163, 182–211. [Google Scholar] [CrossRef]
- Révész, Á.; Gajdics, M. Improved H-Storage Performance of Novel Mg-Based Nanocomposites Prepared by High-Energy Ball Milling: A Review. Energies 2021, 14, 6400. [Google Scholar] [CrossRef]
- Edalati, K.; Akiba, E.; Botta, W.J.; Estrin, Y.; Floriano, R.; Fruchart, D.; Grosdidier, T.; Horita, Z.; Huot, J.; Li, H.W.; et al. Impact of Severe Plastic Deformation on Kinetics and Thermodynamics of Hydrogen Storage in Magnesium and Its Alloys. J. Mater. Sci. Technol. 2023, 146, 221–239. [Google Scholar] [CrossRef]
- Lyu, J.; Lider, A.; Kudiiarov, V. Using Ball Milling for Modification of the Hydrogenation/Dehydrogenation Process in Magnesium-Based Hydrogen Storage Materials: An Overview. Metals 2019, 9, 768. [Google Scholar] [CrossRef]
- Suryanarayana, C. Mechanical alloying and milling. Prog. Mater. Sci. 2001, 46, 1–184. [Google Scholar] [CrossRef]
- Edalati, K.; Bachmaier, A.; Beloshenko, V.A.; Beygelzimer, Y.; Blank, V.D.; Botta, W.J.; Bryła, K.; Čížek, J.; Divinski, S.; Enikeev, N.A.; et al. Nanomaterials by Severe Plastic Deformation: Review of Historical Developments and Recent Advances. Mater. Res. Lett. 2022, 10, 163–256. [Google Scholar] [CrossRef]
- Sadhasivam, T.; Kim, H.-T.; Jung, S.; Roh, S.-H.; Park, J.-H.; Jung, H.-Y. Dimensional effects of nanostructured Mg/MgH2 for hydrogen storage applications. Renew. Sustain. Energy Rev. 2017, 72, 523–534. [Google Scholar] [CrossRef]
- Bennett, C.M.J.; Patman, A.J.; Milanese, C.; Eastwick, C.N.; Walker, G.S. Effect of powder characteristics for a magnesium based metal hydride store. Int. J. Hydrogen Energy 2014, 39, 19646–19655. [Google Scholar] [CrossRef]
- Varin, R.A.; Czujko, T.; Wronski, Z. Particle size, grain size and γ-MgH2 effects on the desorption properties of nanocrystalline commercial magnesium hydride processed by controlled mechanical milling. Nanotechnology 2006, 17, 3856–3865. [Google Scholar] [CrossRef]
- Fátay, D.; Révész, Á.; Spassov, T. Particle size and catalytic effect on the dehydriding of MgH2. J. Alloys Compd. 2005, 399, 237–241. [Google Scholar] [CrossRef]
- Fátay, D.; Spassov, T.; Delchev, P.; Ribárik, G.; Révész, Á. Microstructural development in nanocrystalline MgH2 during H-absorption/desorption cycling. Int. J. Hydrogen Energy 2007, 32, 2914–2919. [Google Scholar] [CrossRef]
- Novakovic, J.G.; Novakovic, N.; Kurko, S.; Govedarovic, S.M.; Pantic, T.; Mamula, B.P.; Batalovic, K.; Radakovic, J.; Rmus, J.; Shelyapina, M.; et al. Influence of defects on the stability and hydrogen-sorption behavior of Mg-based hydrides. Chem. Phys. Chem. 2019, 20, 1216–1247. [Google Scholar] [CrossRef] [PubMed]
- Révész, Á.; Kánya, Z.; Verebélyi, T.; Szabó, P.J.; Zhilyaev, A.P.; Sapssov, T. The effect of high-pressure torsion on the microstructure and hydrogen absorption kinetics of ball-milled Mg70Ni30. J. Alloys Compd. 2010, 504, 83–88. [Google Scholar] [CrossRef]
- Khan, D.; Zou, J.; Zeng, X.; Ding, W. Hydrogen storage properties of nanocrystalline Mg2Ni prepared from compressed 2MgH2–Ni powder. Int. J. Hydrogen Energy 2018, 43, 22391–22400. [Google Scholar] [CrossRef]
- Révész, Á.; Gajdics, M.; Spassov, T. Microstructural evolution of ball-milled Mg–Ni powder during hydrogen sorption. Int. J. Hydrogen Energy 2013, 38, 8342–8349. [Google Scholar] [CrossRef]
- Varin, R.A.; Czujko, T.; Wasmund, E.B.; Wronski, Z.S. Catalytic effects of various forms of nickel on the synthesis rate and hydrogen desorption properties of nanocrystalline magnesium hydride (MgH2) synthesized by controlled reactive mechanical milling (CRMM). J. Alloys Compd. 2007, 432, 217–231. [Google Scholar] [CrossRef]
- Antiqueira, F.J.; Leiva, D.R.; Zepon, G.; de Cunha, B.F.R.F.; Figueroa, S.J.A.; Botta, W.J. Fast Hydrogen Absorption/Desorption Kinetics in Reactive Milled Mg-8 Mol% Fe Nanocomposites. Int. J. Hydrogen Energy 2020, 45, 12408–12418. [Google Scholar] [CrossRef]
- Song, M.; Zhang, L.; Yao, Z.; Zheng, J.; Shang, D.; Chen, L.; Li, H. Unraveling the Degradation Mechanism in Hydrogen Storage Property of Fe Nanocatalysts Modified MgH2. Inorg. Chem. Front. 2022, 9, 3874–3884. [Google Scholar] [CrossRef]
- Paramonov, R.; Spassov, T.; Nagy, P.; Révész, Á. Synergetic Effect of FeTi in Enhancing the Hydrogen-Storage Kinetics of Nanocrystalline MgH2. Energies 2024, 17, 794. [Google Scholar] [CrossRef]
- Barkhordarian, G.; Klassen, T.; Bormann, R. Fast hydrogen sorption kinetics of nanocrystalline Mg using Nb2O5 as catalyst. Scr. Mater. 2003, 49, 213–217. [Google Scholar] [CrossRef]
- Croston, D.L.; Grant, D.M.; Walker, G.S. The catalytic effect of titanium oxide based additives on the dehydrogenation and hydrogenation of milled MgH2. J. Alloys Compd. 2010, 492, 251–258. [Google Scholar] [CrossRef]
- Song, M.Y.; Kwon, I.H.; Kwon, S.N.; Park, C.G.; Park, H.R.; Bae, J.-S. Preparation of hydrogen-storage alloy Mg–10wt% Fe2O3 under various milling conditions. Int. J. Hydrogen Energy 2006, 31, 43–47. [Google Scholar] [CrossRef]
- Yun, H.; Wang, H.; Bai, J.; Wang, X.; Dai, X.; Hou, X.; Xu, Y. Activation and Hydrogen Storage Properties of Mg-Based Composites Synthesized by Catalytic Mechanochemical Hydrogenation Strategy. Int. J. Hydrogen Energy 2024, 50, 1025–1039. [Google Scholar] [CrossRef]
- Campos, R.B.V.; Camargo, S.A.S., Jr.; Brum, M.C.; Dos Santos, D.S. Hydrogen uptake enhancement by the use of a magnesium hydride and carbon nanotubes mixture. Mater. Res. 2017, 20, 85–88. [Google Scholar] [CrossRef]
- Chen, J.; Kuo, C.-H.; Ku, J.-R.; Yan, P.-S.; Huang, C.-J.; Jeng, M.-S.; Tsau, F.-H. Highly improved with hydrogen storage capacity and fast kinetics in Mg-based nanocomposites by CNTs. J. Alloys Compd. 2013, 568, 78–83. [Google Scholar] [CrossRef]
- Gajdics, M.; Spassov, T.; Kis, V.K.; Schafler, E.; Révész, Á. Microstructural and Morphological Investigations on Mg-Nb2O5-CNT Nanocomposites Processed by High-Pressure Torsion for Hydrogen Storage Applications. Int. J. Hydrogen Energy 2020, 45, 7917–7928. [Google Scholar] [CrossRef]
- Crivello, J.-C.; Dam, B.; Denys, R.V.; Dornheim, M.; Grant, D.M.; Huot, J.; Jensen, T.R.; de Jongh, P.; Latroche, M.; Milanese, C.; et al. Review of Magnesium Hydride-Based Materials: Development and Optimisation. Appl. Phys. A 2016, 122, 97. [Google Scholar] [CrossRef]
- Pang, Y.; Li, Q. A review on kinetic models and corresponding analysis methods for hydrogen storage materials. Int. J. Hydrogen Energy 2016, 41, 18072–18087. [Google Scholar] [CrossRef]
- Shang, Y.; Pistidda, C.; Gizer, G.; Klassen, T.; Dornheim, M. Mg-based materials for hydrogen storage. J. Magnes. Alloys 2021, 9, 1837–1860. [Google Scholar] [CrossRef]
- Mintz, M.H.; Zeiri, Y. Hydriding kinetics of powders. J. Alloys Compd. 1995, 216, 159–175. [Google Scholar] [CrossRef]
- Asakuma, Y.; Miyauchi, S.; Yamamoto, T.; Aoki, H.; Miura, T. Numerical analysis of absorbing and desorbing mechanism for the metal hydride by homogenization method. Int. J. Hydrogen Energy 2003, 28, 529. [Google Scholar] [CrossRef]
- Chou, K.C.; Li, Q.; Lin, Q.; Jiang, L.J.; Xu, K.D. Kinetics of absorption and desorption of hydrogen in alloy powder. Int. J. Hydrogen Energy 2005, 30, 301. [Google Scholar] [CrossRef]
- Luo, Q.; Li, J.; Li, B.; Liu, B.; Shao, H.; Li, Q. Kinetics in Mg-based hydrogen storage materials: Enhancement and mechanism. J. Magnes. Alloys 2019, 7, 58–71. [Google Scholar] [CrossRef]
- Evard, E.; Gabis, I.; Yartys, V.A. Kinetics of hydrogen evolution from MgH2: Experimental studies, mechanism and modelling. Int. J. Hydrogen Energy 2010, 35, 9060–9069. [Google Scholar] [CrossRef]
- Barkhordarian, G.; Klassen, T.; Borman, R. Kinetic investigation of the effect of milling time on the hydrogen sorption reaction of magnesium catalysed with different Nb2O5 contents. J. Alloys Compd. 2006, 407, 249–255. [Google Scholar] [CrossRef]
- Liu, J.; Zhang, X.; Li, Q.; Chou, K.C.; Xu, K.D. Investigation on kinetics mechanism of hydrogen absorption in the La2Mg17-based composites. Int. J. Hydrogen Energy 2009, 34, 1951–1957. [Google Scholar] [CrossRef]
- Schweppe, F.; Martin, M.; Fromm, E. Model on hydride formation describing surface control, diffusion control and transition regions. J. Alloys Compd. 1997, 261, 254–258. [Google Scholar] [CrossRef]
- Gabis, I.E.; Voit, A.P.; Evard, E.A.; Zaika, Y.V.; Chernov, I.A.; Yartys, V.A. Kinetics of hydrogen desorption from the powders of metal hydrides. J. Alloys Compd. 2005, 404–406, 312–316. [Google Scholar] [CrossRef]
- Jacobs, P.W.M.; Tompkins, F.C. Classification and Theory of Solid Reactions. In Chemistry of the Solid State; Garner, W.E., Ed.; Butterworth: London, UK, 1955; pp. 184–212. [Google Scholar]
- Révész, Á.; Fátay, D. Microstructural evolution of ball-milled MgH2 during a complete dehydrogenation–hydrogenation cycle. J. Power Sources 2010, 195, 6997–7002. [Google Scholar] [CrossRef]
- Avrami, M. Kinetics of phase change II. J. Chem. Phys. 1940, 8, 212–224. [Google Scholar]
- Révész, Á.; Paramonov, R.; Spassov, T.; Gajdics, M. Microstructure and Hydrogen Storage Performance of Ball-Milled MgH2 Catalyzed by FeTi. Energies 2023, 16, 1061. [Google Scholar] [CrossRef]
- Krill, C.E.; Birringer, R. Estimating grain-size distributions in nanocrystalline materials from X-ray diffraction profile analysis. Phil. Mag. 1998, 77, 621–640. [Google Scholar] [CrossRef]
- Gubicza, J.; Szépvölgyi, J.; Mohai, I.; Zsoldos, L.; Ungár, T. Particle size distribution and dislocation density determined by high resolution X-ray diffraction in nanocrystalline silicon nitride powders. Mater. Sci. Eng. 2000, 280, 263–269. [Google Scholar] [CrossRef]
- Révész, Á.; Gajdics, M.; Varga, L.K.; Krállics, G.; Péter, L.; Spassov, T. Hydrogen Storage of Nanocrystalline Mg–Ni Alloy Processed by Equal-Channel Angular Pressing and Cold Rolling. Int. J. Hydrogen Energy 2014, 39, 9911–9917. [Google Scholar] [CrossRef]
- Révész, Á. Multiparticle Reaction Function of Ball-Milled MgH2 Powders. Jpn. J. Appl. Phys. 2009, 48, 076511. [Google Scholar] [CrossRef]
- Révész, Á.; Fátay, D.; Spassov, T. Hydriding kinetics of ball-milled nanocrystalline MgH2 powders. J. Mater. Res. 2007, 22, 3144–3151. [Google Scholar] [CrossRef]
- Ribárik, G.; Jóni, B.; Ungár, T. Global optimum of microstructure parameters in the CMWP line-profile-analysis method by combining Marquardt-Levenberg and Monte-Carlo procedures. J. Mater. Sci. Technol. 2019, 35, 1508–1514. [Google Scholar] [CrossRef]
- Ribárik, G.; Gubicza, J.; Ungár, T. Correlation between strength and microstructure of ball-milled Al–Mg alloys determined by X-ray diffraction. Mater. Sci. Eng. A 2004, 387, 343–347. [Google Scholar] [CrossRef]
- Atkinson, H.V. Overview no. 65: Theories of normal grain growth in pure single phase systems. Acta Metall. 1988, 3, 469–491. [Google Scholar] [CrossRef]
- Zhou, C.; Peng, Y.; Zhang, Q. Growth kinetics of MgH2 nanocrystallites prepared by ball milling. J. Mater. Sci. Technol. 2020, 50, 178–183. [Google Scholar] [CrossRef]
- Zhou, C.Q.; Zhang, Q.A. Effect of Pr3Al11 nanoparticles on crystallite growth kinetics of nanocrystalline Mg. J. Alloys Compd. 2019, 804, 299–304. [Google Scholar] [CrossRef]
- Denys, R.V.; Riabov, A.B.; Maehlen, J.P.; Lotosky, M.V.; Solberg, J.K.; Yartis, V.A. In Situ synchrotron X-ray diffraction studies of hydrogen desorption and absorption properties of Mg and Mg–Mm–Ni after reactive ball milling in hydrogen. Acta Mater. 2009, 13, 3989–4000. [Google Scholar] [CrossRef]
- Denys, R.V.; Poletaev, A.A.; Maehlen, J.P.; Solberg, J.K.; Tarasov, B.P.; Yartis, V.A. Nanostructured rapidly solidified LaMg11Ni alloy. II. In Situ synchrotron X-ray diffraction studies of hydrogen absorption–desorption behaviours. Int. J. Hydrogen Energy 2012, 7, 5710–5722. [Google Scholar] [CrossRef]
- Gajdics, M.; Calizzi, M.; Pasquini, L.; Schafler, E.; Révész, Á. Characterization of a nanocrystalline Mg–Ni alloy processed by high-pressure torsion during hydrogenation and dehydrogenation. Int. J. Hydrogen Energy 2016, 23, 9803–9809. [Google Scholar] [CrossRef]
- Ahmad, M.A.N.; Sazelee, N.; Ali, N.A.; Ismail, M. An Overview of the Recent Advances of Additive-Improved Mg(BH4)2 for Solid-State Hydrogen Storage Material. Energies 2022, 15, 862. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Révész, Á.; Pintér, Á. Time-Dependent Multi-Particle Model Describing the Hydrogen Absorption of Nanocrystalline Magnesium Powders: A Case Study. Energies 2024, 17, 2322. https://doi.org/10.3390/en17102322
Révész Á, Pintér Á. Time-Dependent Multi-Particle Model Describing the Hydrogen Absorption of Nanocrystalline Magnesium Powders: A Case Study. Energies. 2024; 17(10):2322. https://doi.org/10.3390/en17102322
Chicago/Turabian StyleRévész, Ádám, and Áron Pintér. 2024. "Time-Dependent Multi-Particle Model Describing the Hydrogen Absorption of Nanocrystalline Magnesium Powders: A Case Study" Energies 17, no. 10: 2322. https://doi.org/10.3390/en17102322
APA StyleRévész, Á., & Pintér, Á. (2024). Time-Dependent Multi-Particle Model Describing the Hydrogen Absorption of Nanocrystalline Magnesium Powders: A Case Study. Energies, 17(10), 2322. https://doi.org/10.3390/en17102322





