Simulation and Experimental Verification of Dispersion and Explosion of Hydrogen–Methane Mixture in a Domestic Kitchen †
Abstract
1. Introduction
2. Materials and Methods
2.1. Numerical Simulation
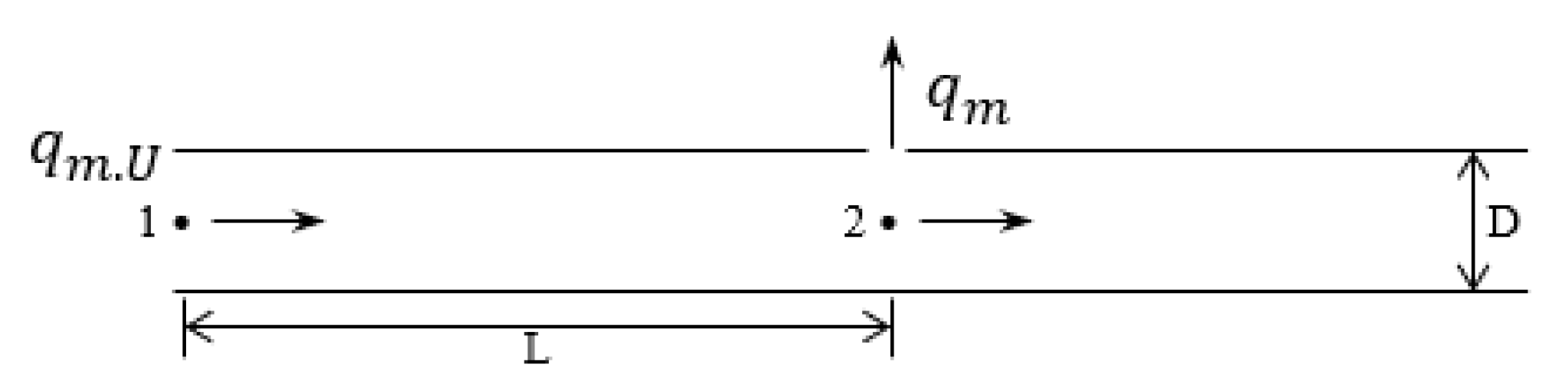
2.1.1. Leakage Mathematical Model
- The small-bore leak model:
- The pipeline leakage model:
- The integrated pipe–small bore model:When a small hole develops a leak, the resistance to gas flow along the pipeline causes the pressure at the leak to be lower than the initial pressure of the pipeline. If we assume that all the gas in the pipeline flows out through the leak and there is no flow downstream of the leak, then the pressure at the leak will be at its highest and the flow rate of the leak will also be at its highest. Therefore,By combining the above four formulas and inputting the data for calculation, we can obtain the relevant parameters required for all sizes of leakage.
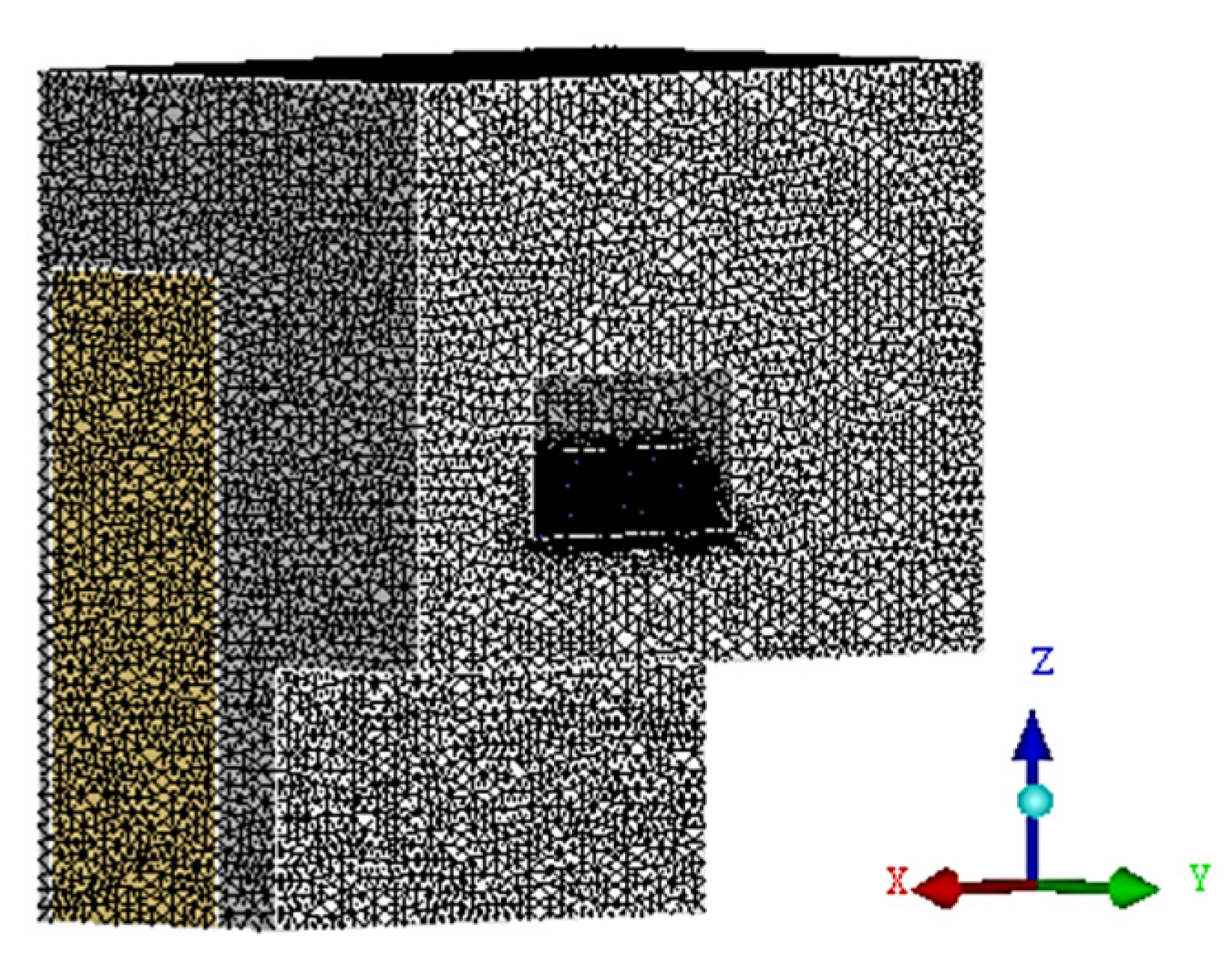
2.1.2. Numerical Simulation Models
2.1.3. Numerical simulation parameter setting
- 1.
- Leakage dispersion numerical simulation.
- Continuity equation:
- Momentum equation:
- Energy equation:
- Density equation:
- Composition equation:
- 2.
- Explosion numerical simulation.
- Dalton’s theorem for ideal gases:
- Isentropic ratio:
- Pressure–density–temperature relationship:
- By employing the finite volume method and applying the appropriate boundary conditions, the N-S equation can be solved accurately. This enables us to determine the variations in key parameters within the relevant region, including temperature, overpressure value, flame velocity, combustion products, and combustion material consumption:
- The turbulent pulsation kinetic energy k is
- Turbulent pulsation kinetic energy dissipation rate ε for
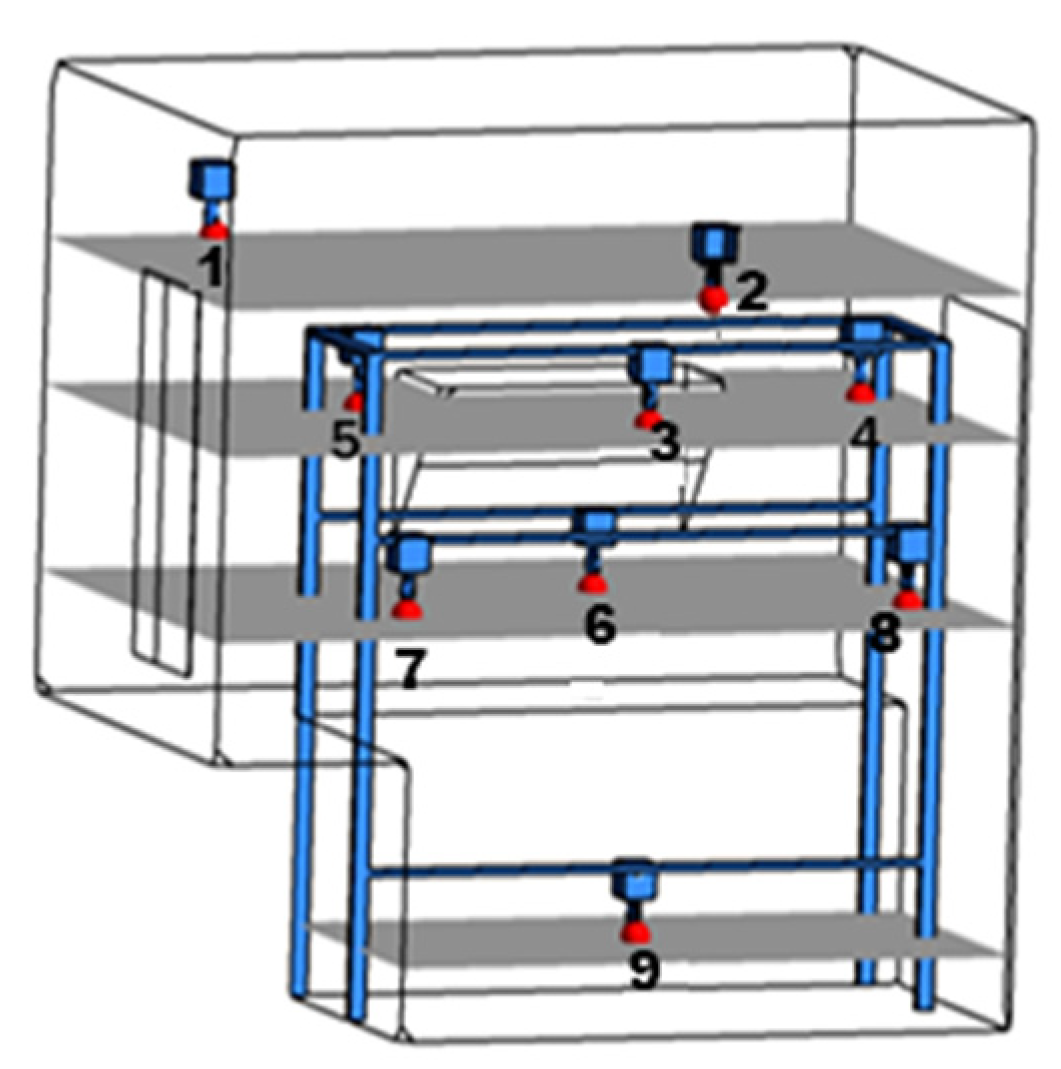
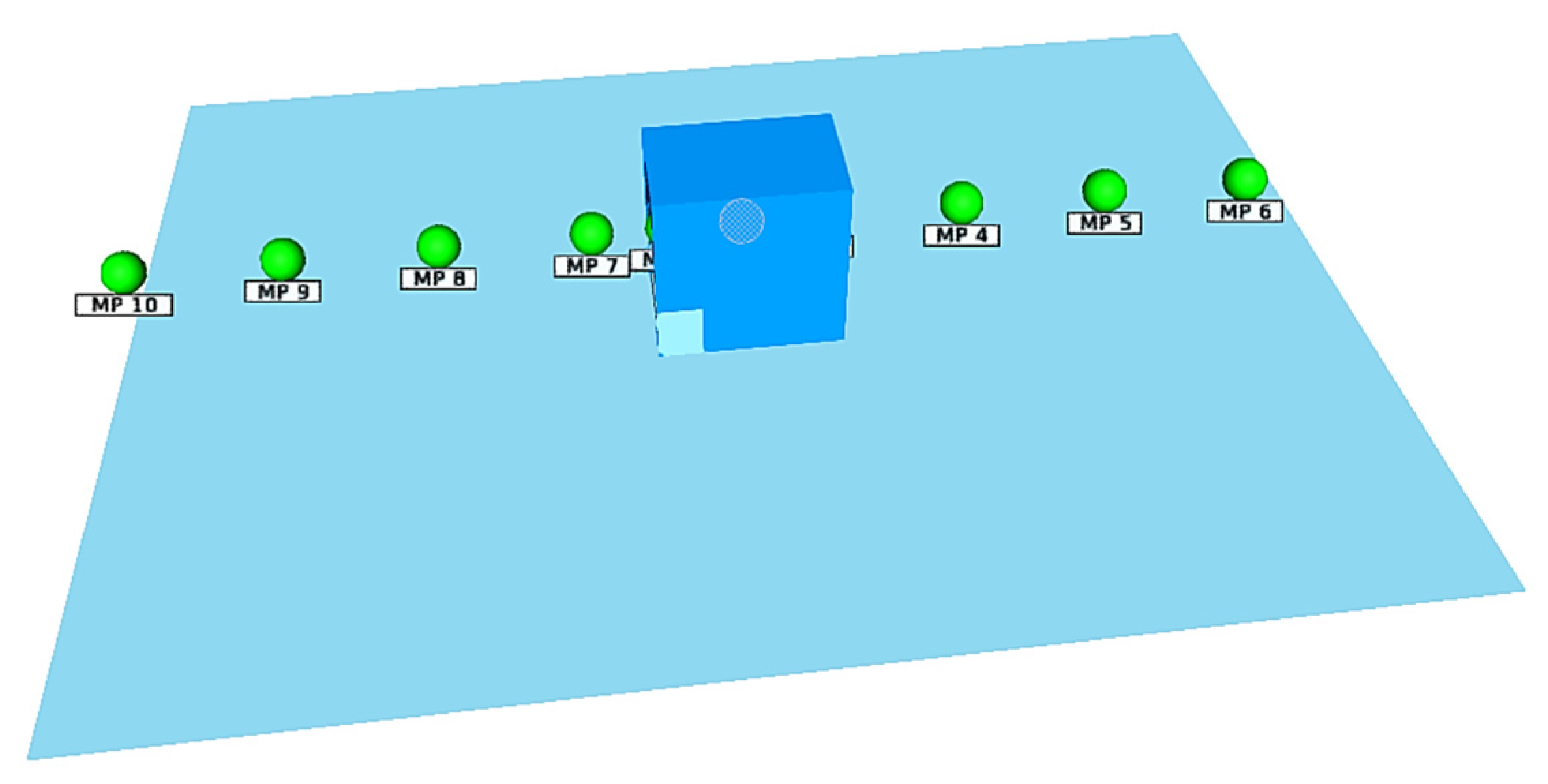
- Detector layout coordinates: MP1 (0.5, 1.25, 1.3), MP2 (0.5, 0.25, 1.3), MP3 (0.5, 2.25, 1.3), MP4 (0.5, 4.25, 1.3), MP5 (0.5, 6.25, 1.3), MP6 (0.5, 8.25, 1.3), MP7 (0.5, −0.75, 1.3), MP8 (0.5, −2.75, 1.3), MP9 (0.5, −4.75, 1.3), MP10 (0.5, −6.75, 1.3);
- Ignition source coordinates: the central position of the operating table (1.25, 0.8, 1.3);
- Three-dimensional parameter settings: P (explosion static overpressure), PROD (combustion product concentration), VVEC (gas velocity), DRAG (explosion dynamic overpressure);
- Gas composition setting: CH4 and H2 are selected from the material library, and the volume fraction of mixed gas in the simulation is adjusted according to different working conditions;
- Pressure relief plate: During the explosion simulation, the doors and windows have two states: all open and all closed. When the working condition is set to all closed doors and windows, the doors and windows are considered “POPOUT”-type pressure relief plates, with the door opening pressure set at 0.1 bar and the window opening pressure set at 0.05 bar.
2.2. Experimental Setup
3. Results and Discussion
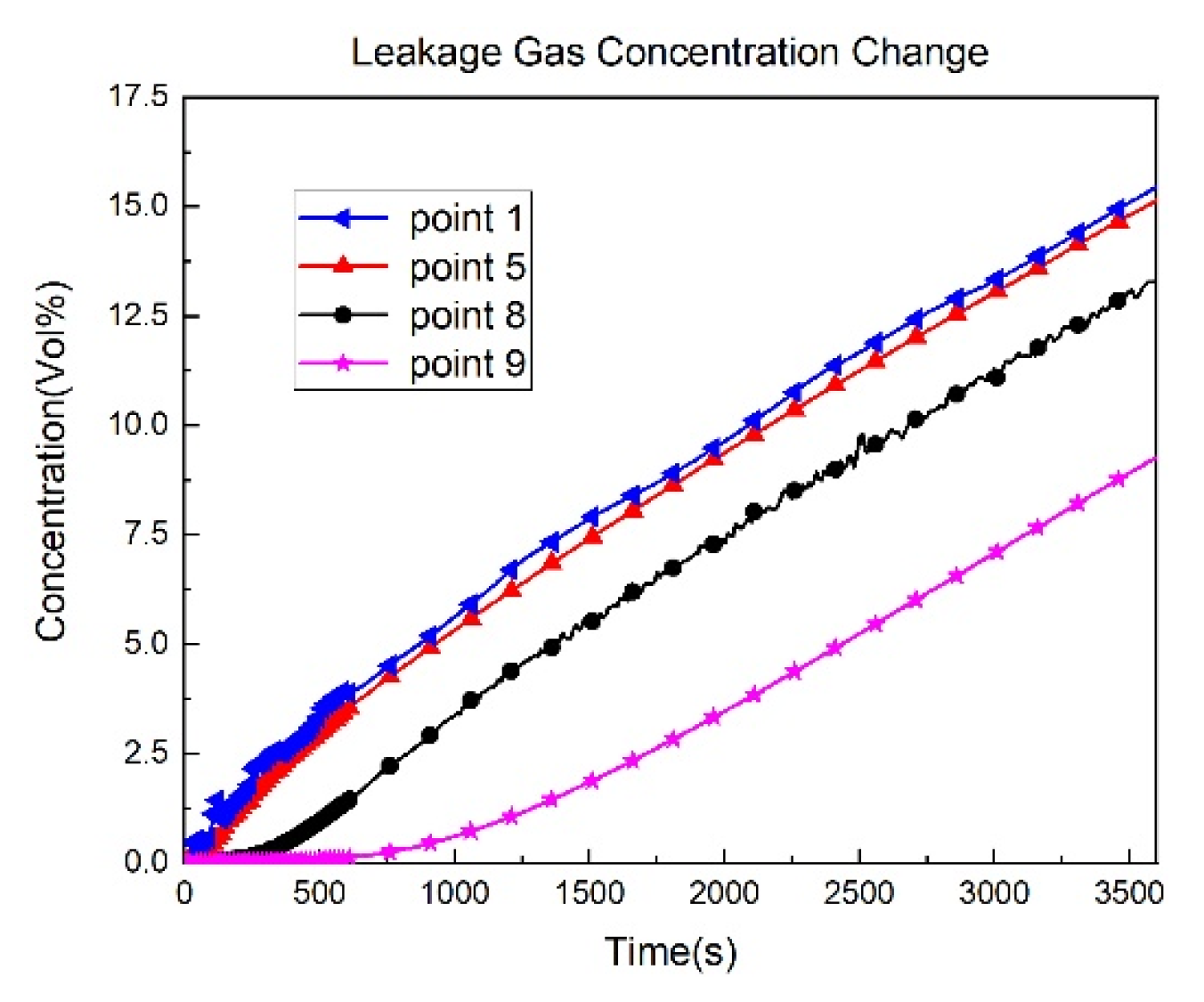
3.1. Leakage Dispersion Numerical Simulation Results
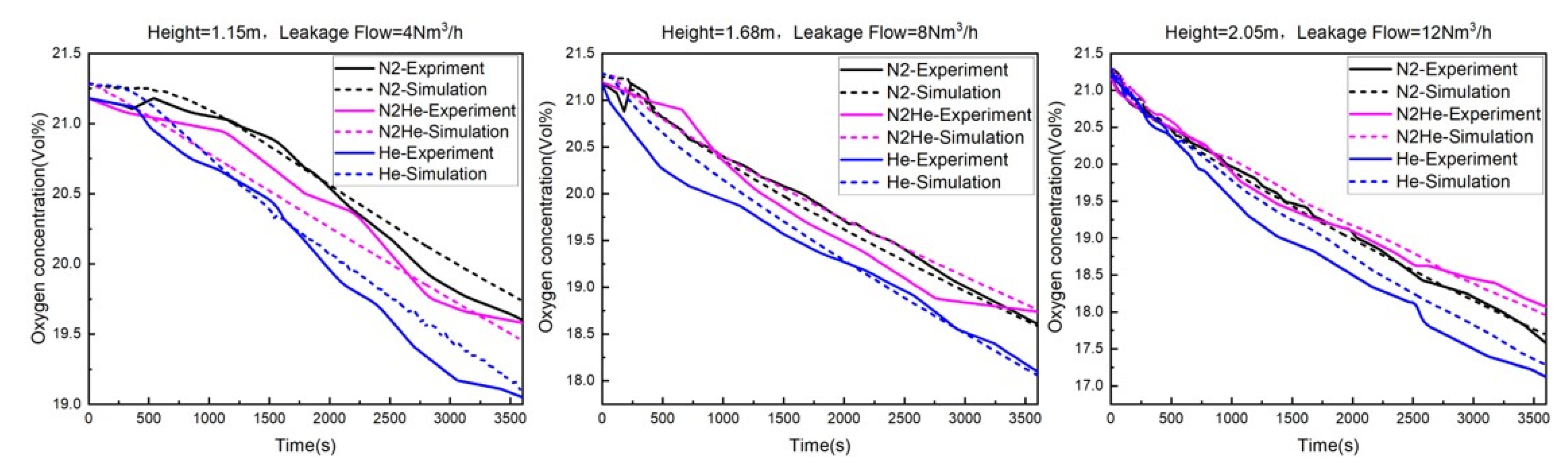
3.2. Experimental Verification Results
3.3. Study of the Consequences of Spill Dispersion
3.4. Results of Explosion Simulation
3.4.1. The Condition with All Doors and Windows Closed
3.4.2. The Condition with All Doors and Windows Opened
4. Conclusions
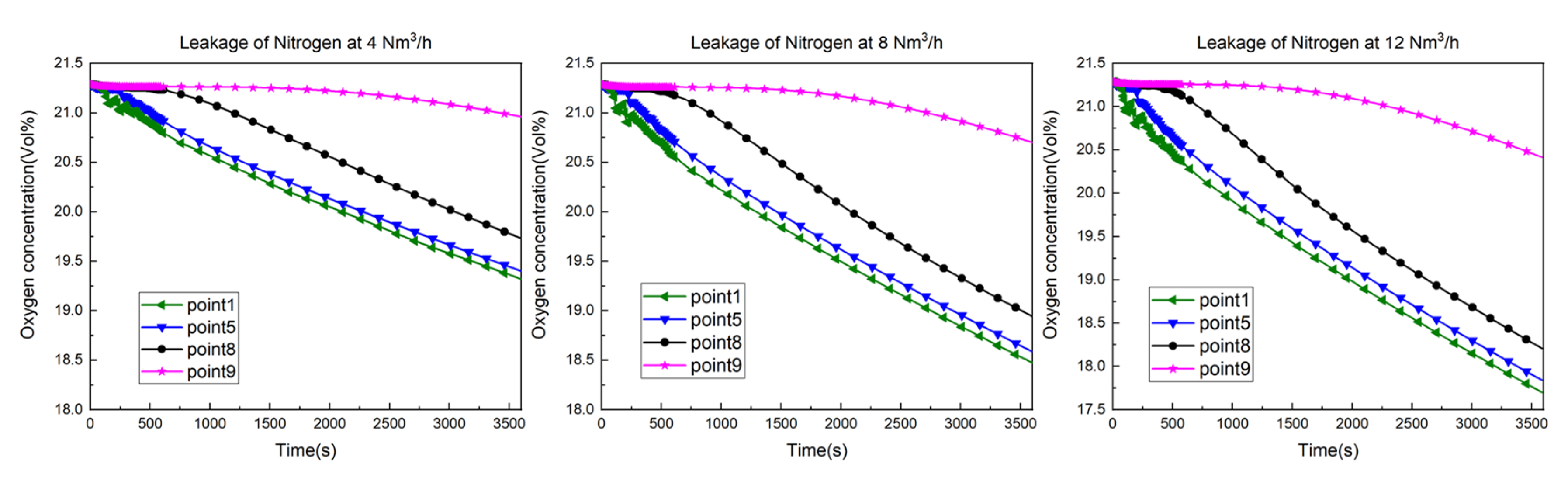
- As the leakage flow rate increases, the concentration of oxygen at each detection point decreases more rapidly, and the average concentration of the leaking gas in the kitchen increases.
- The blending of helium accelerates the dispersion of nitrogen. As the proportion of helium increases, the average concentration of the leaking gas in the confined space also increases. On the other hand, if the leaking gas is a blend of hydrogen and natural gas, an increase in the proportion of hydrogen blending leads to an expansion of the combustible range in the kitchen and an increase in risk.
- The leaking gas exhibits a stratification phenomenon in the kitchen, with the concentration gradually decreasing from top to bottom. This stratification is more apparent for small leaks and becomes less pronounced as the leak volume increases.
- The trend of the oxygen concentration change at the experimental detection point aligns with the trend observed at the same monitoring point through numerical simulation. Both show a gradual decrease as the leakage time increases. Multiple sets of data were compared and analyzed, revealing that the error range between the experimental data and simulation results of the oxygen concentration at nine monitoring points did not exceed 5% under different leakage flow rates and gas leaks. This falls within an acceptable range. Consequently, it can be concluded that the experimental results are essentially consistent with the simulation results. This fully confirms the feasibility of using the numerical simulation method to study gas diffusion in confined spaces and verifies the accuracy of the simulation.
- The comparison diagram illustrates the streamline of the Y = 1.15m plane for pure nitrogen leakage at various flow rates in the numerical simulation. It shows that as the leakage rate increases, the airflow velocity in the upper part of the kitchen also increases. Consequently, there is an increase in the entrainment of air by the leaking gas and the intensity of the indoor airflow. This provides evidence for the weakening of the gas stratification in the kitchen as the leakage rate increases.
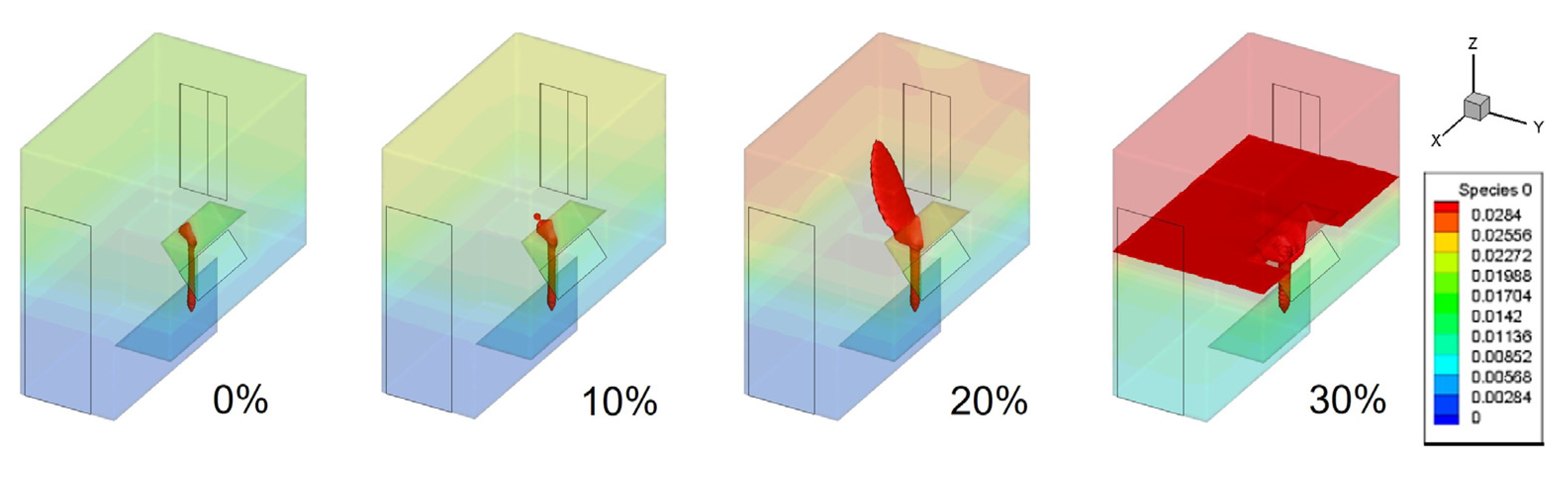
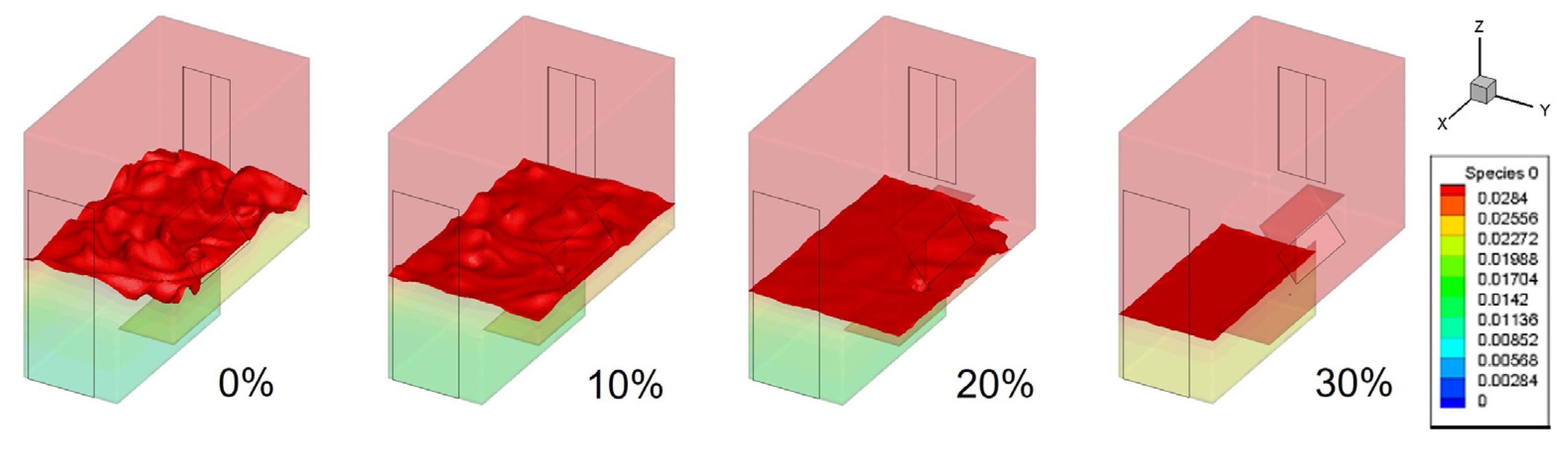
- A leak of hydrogen-blended natural gas initially spreads upwards, creating an explosive area larger than the lower explosive limit range at the leak outlet and the top of the kitchen. As the leak continues, the explosive area gradually moves downwards until the kitchen is filled with the hydrogen-blended gas.
- In the case of different proportions of hydrogen blending, a higher proportion of hydrogen leads to a larger volume of the explosive area in the kitchen and a shorter time to fill the kitchen with an explosive volume. Blending hydrogen into the gas promotes the spread of gas after the leak, increasing the risk of leakage.
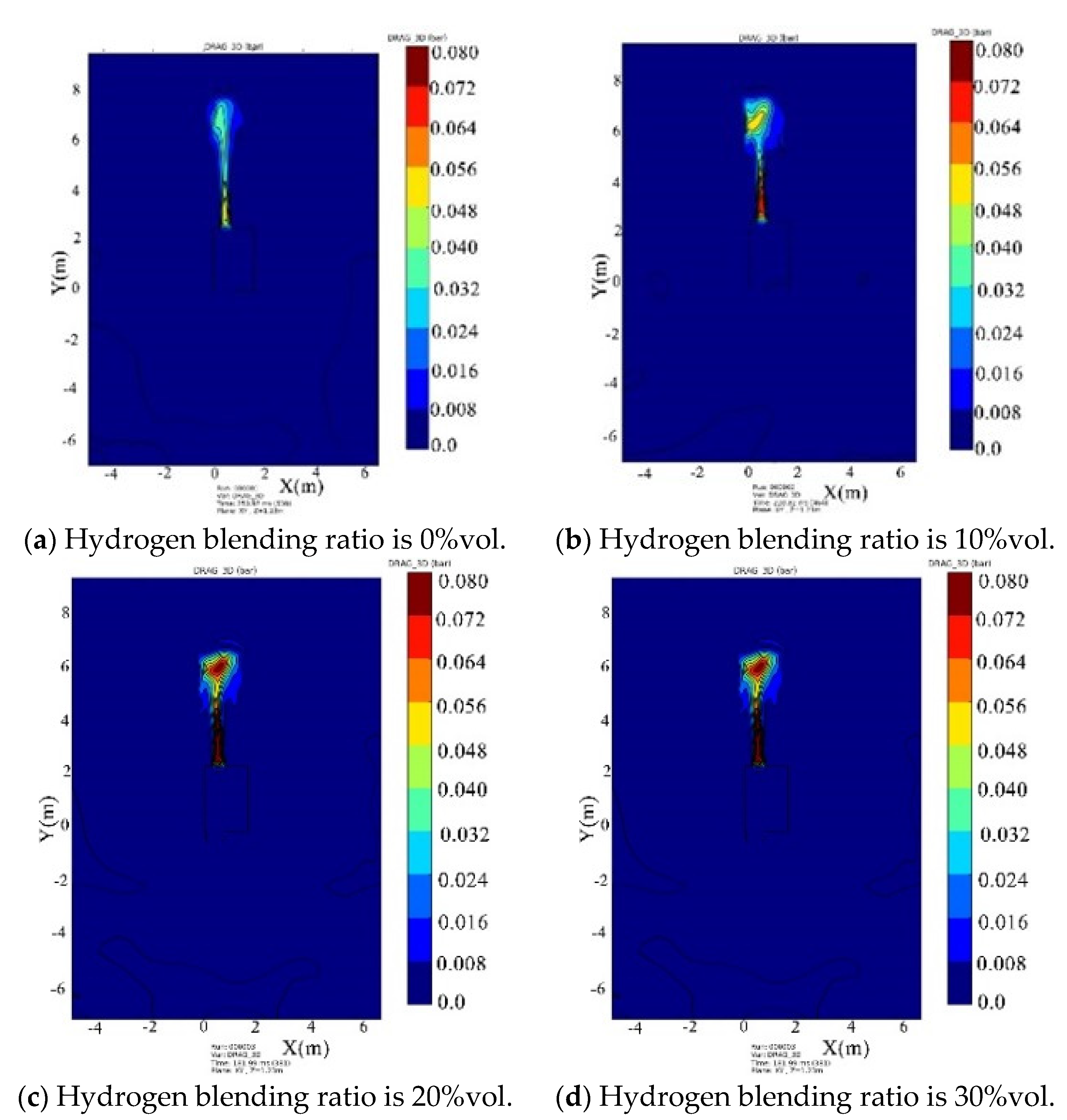
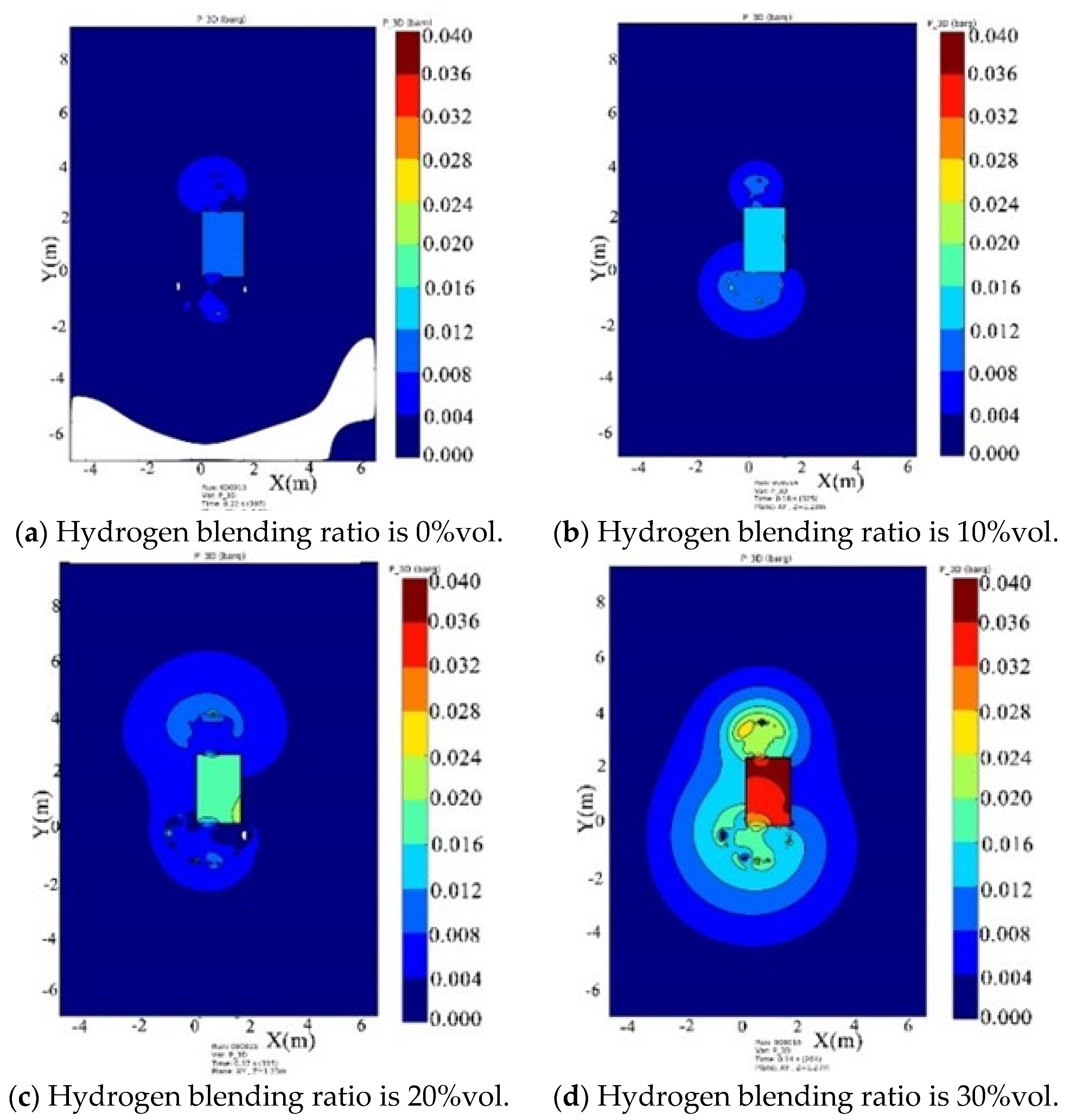
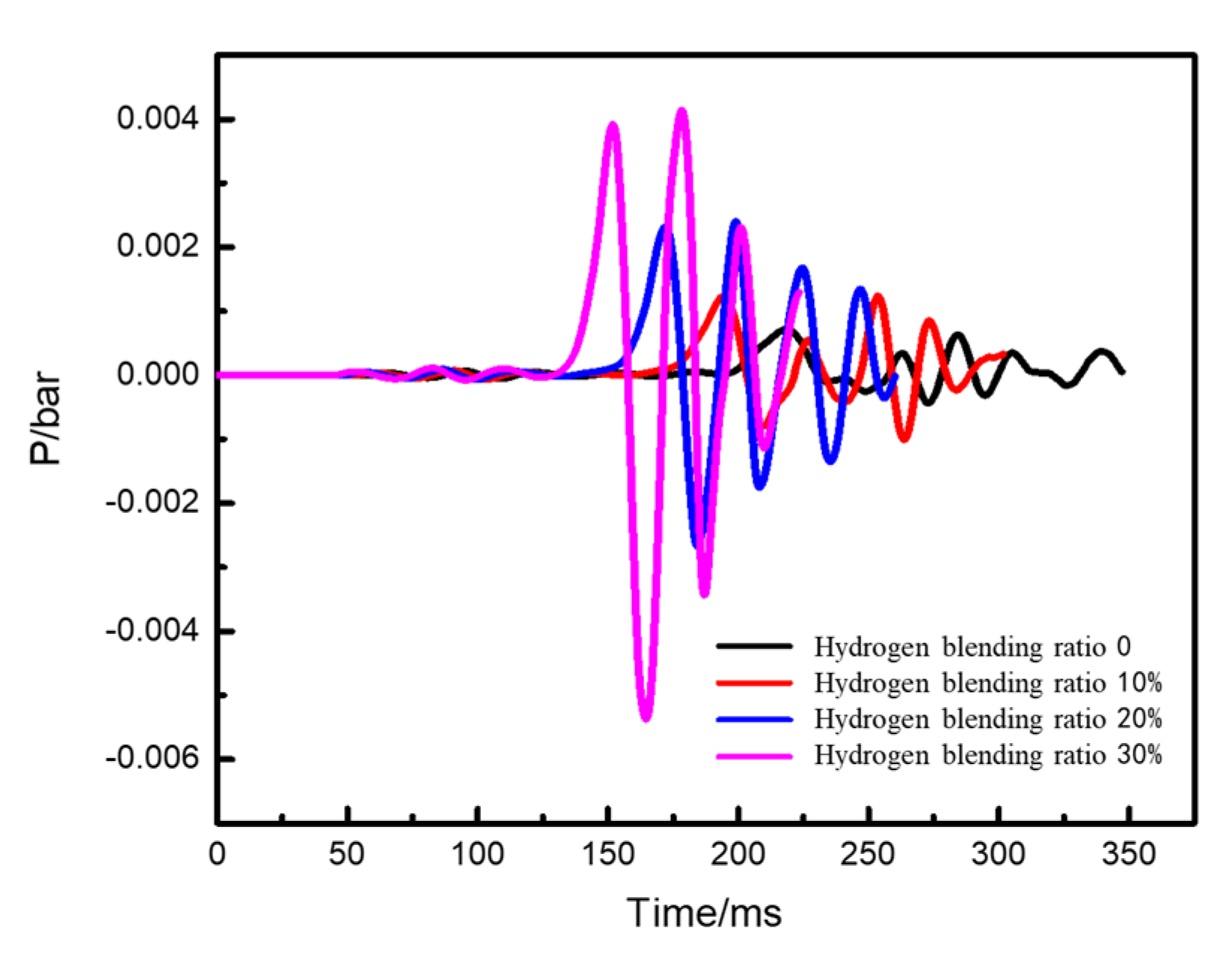
- Under the condition of kitchen doors and windows being closed, the windows start to relieve pressure at 0.1 bar, while the doors relieve pressure at 0.05 bar. The blast wave’s impact range on the window side remains relatively constant and is not affected by changes in the hydrogen blending ratio. However, the shock wave strength increases with an increase in the ratio. When the hydrogen blending ratio reaches 30% by volume, the pressure of the door will be relieved.
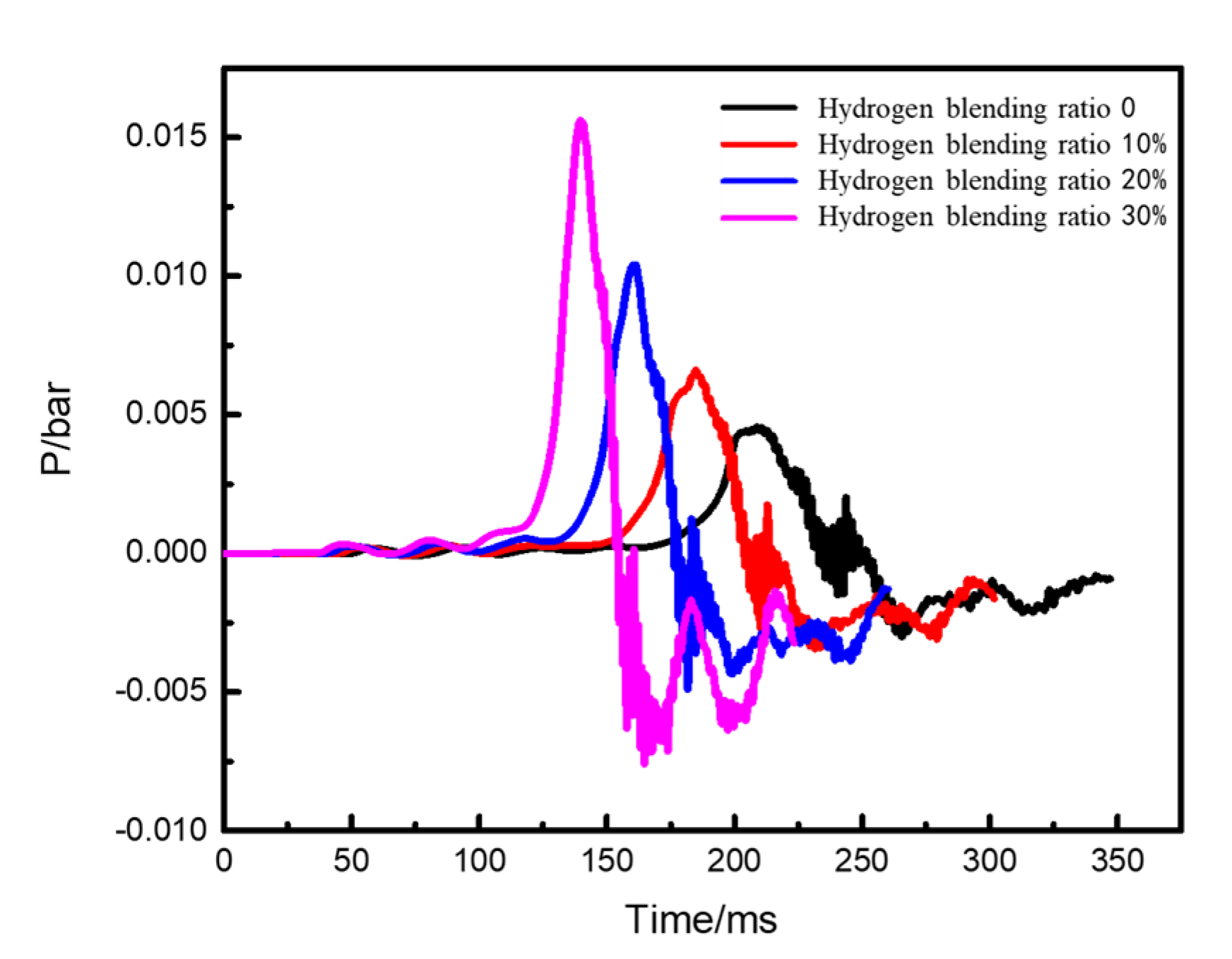
- Under the condition of the kitchen doors and windows being open, the overpressure in the space caused by the explosion gradually increases with an increase in the hydrogen blending ratio. The influence range surrounding the kitchen is circular in the horizontal plane, and both the impact range and strength of the shock wave increase with the ratio.
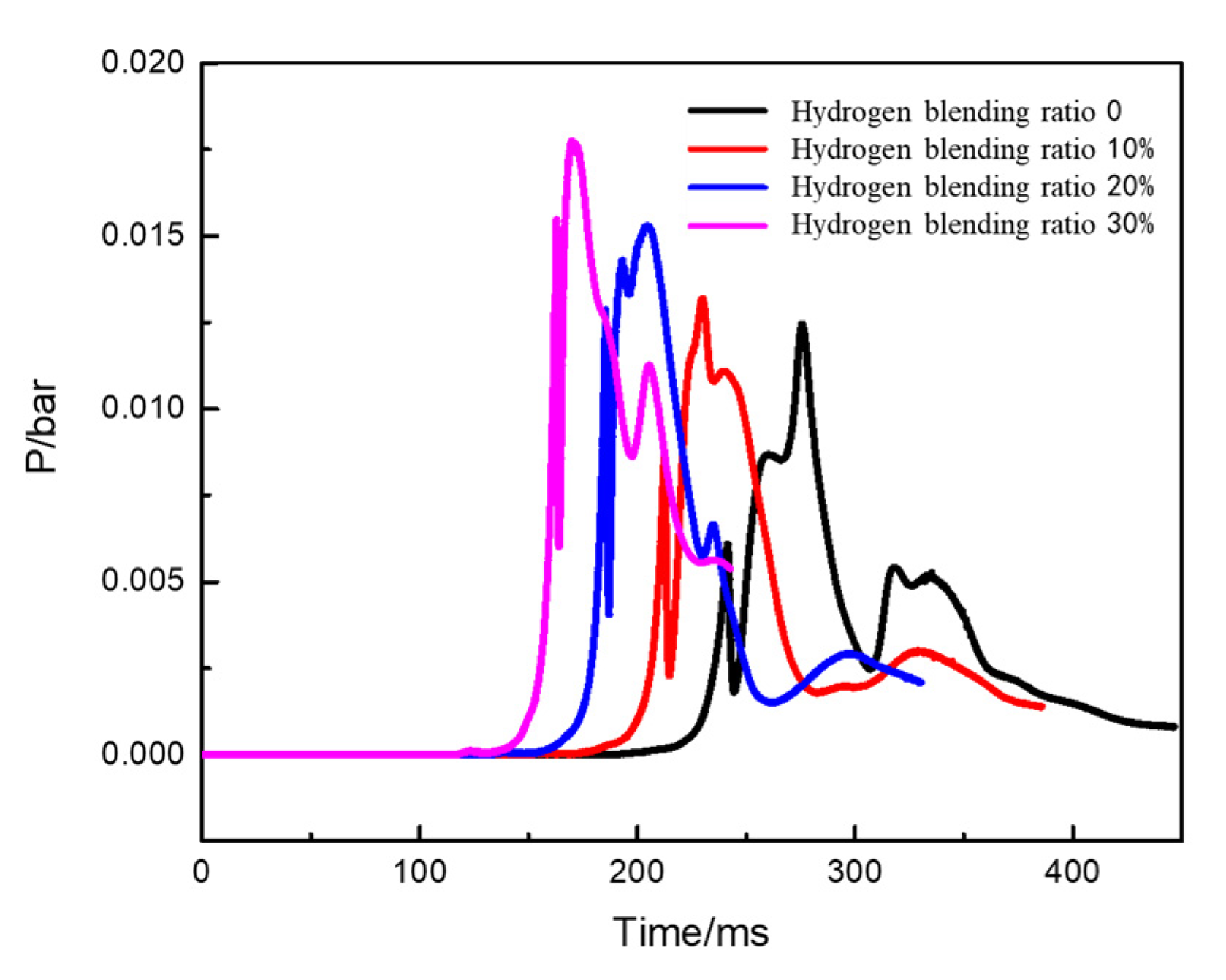
- With an increase in the hydrogen blending ratio, the peak pressure of the explosion significantly increases, the explosion occurs earlier, and the duration of the explosion peak shortens. This indicates that a higher volume concentration of hydrogen blended in natural gas leads to an earlier ignition time and a stronger explosion intensity. To some extent, blending hydrogen increases the hazard of natural gas explosion in confined spaces.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Prasad, K.; Pitts, W.M.; Yang, J.C. A Numerical Study of the Release and Dispersion of a Buoyant Gas in Partially Confined Spaces. Int. J. Hydrogen Energy 2011, 36, 5200–5210. [Google Scholar] [CrossRef]
- Bernard-Michel, G.; Houssin-Agbomson, D. Comparison of Helium and Hydrogen Releases in 1 M3 and 2 M3 Two Vents Enclosures: Concentration Measurements at Different Flow Rates and for Two Diameters of Injection Nozzle. Int. J. Hydrogen Energy 2017, 42, 7542–7550. [Google Scholar] [CrossRef]
- Afghan Haji Abbas, M.; Kheradmand, S.; Sadoughipour, H. Numerical Study of the Effect of Hydrogen Leakage Position and Direction on Hydrogen Distribution in a Closed Enclosure. Int. J. Hydrogen Energy 2020, 45, 23872–23881. [Google Scholar] [CrossRef]
- Bauwens, C.R.; Dorofeev, S.B. CFD Modeling and Consequence Analysis of an Accidental Hydrogen Release in a Large Scale Facility. Int. J. Hydrogen Energy 2014, 39, 20447–20454. [Google Scholar] [CrossRef]
- Hajji, Y.; Bouteraa, M.; Cafsi, A.E.; Belghith, A.; Bournot, P.; Kallel, F. Dispersion and Behavior of Hydrogen during a Leak in a Prismatic Cavity. Int. J. Hydrogen Energy 2014, 39, 6111–6119. [Google Scholar] [CrossRef]
- Choi, J.; Hur, N.; Kang, S.; Lee, E.D.; Lee, K.-B. A CFD Simulation of Hydrogen Dispersion for the Hydrogen Leakage from a Fuel Cell Vehicle in an Underground Parking Garage. Int. J. Hydrogen Energy 2013, 38, 8084–8091. [Google Scholar] [CrossRef]
- Lowesmith, B.J.; Hankinson, G.; Spataru, C.; Stobbart, M. Gas Build-up in a Domestic Property Following Releases of Methane/Hydrogen Mixtures. Int. J. Hydrogen Energy 2009, 34, 5932–5939. [Google Scholar] [CrossRef]
- Marangon, A.; Carcassi, M.N. Hydrogen–Methane Mixtures: Dispersion and Stratification Studies. Int. J. Hydrogen Energy 2014, 39, 6160–6168. [Google Scholar] [CrossRef]
- Liu, A.; Huang, J.; Li, Z.; Chen, J.; Huang, X.; Chen, K.; Xu, W.B. Numerical Simulation and Experiment on the Law of Urban Natural Gas Leakage and Diffusion for Different Building Layouts. J. Nat. Gas Sci. Eng. 2018, 54, 1–10. [Google Scholar] [CrossRef]
- Middha, P.; Engel, D.; Hansen, O.R. Can the Addition of Hydrogen to Natural Gas Reduce the Explosion Risk? Int. J. Hydrogen Energy 2011, 36, 2628–2636. [Google Scholar] [CrossRef]
- Song, B.; Jiao, W.; Cen, K.; Tian, X.; Zhang, H.; Lu, W. Quantitative Risk Assessment of Gas Leakage and Explosion Accident Consequences inside Residential Buildings. Eng. Fail. Anal. 2021, 122, 105257. [Google Scholar] [CrossRef]
- Zhang, S.; Ma, H.; Huang, X.; Peng, S. Numerical Simulation on Methane-Hydrogen Explosion in Gas Compartment in Utility Tunnel. Process Saf. Environ. Prot. 2020, 140, 100–110. [Google Scholar] [CrossRef]
- Wang, D.; Qian, X.; Yuan, M.; Ji, T.; Xu, W.; Liu, S. Numerical Simulation Analysis of Explosion Process and Destructive Effect by Gas Explosion Accident in Buildings. J. Loss Prev. Process Ind. 2017, 49, 215–227. [Google Scholar] [CrossRef]
- Lowesmith, B.J.; Hankinson, G.; Johnson, D.M. Vapour Cloud Explosions in a Long Congested Region Involving Methane/Hydrogen Mixtures. Process Saf. Environ. Prot. 2011, 89, 234–247. [Google Scholar] [CrossRef]







| Part | Boundary Condition |
|---|---|
| Operating table | Wall |
| Wall | Wall |
| Doors and windows | Wall |
| Cooker hood | Wall |
| Leak point | Velocity—inlet |
| A gap in the window | Pressure—outlet |
| Hydrogen Blending Ratios | Leakage Flow Rate (m3/h) | Leakage Rate (m/s) |
|---|---|---|
| 0%vol | 1.85 | 3.067 |
| 10%vol | 1.94 | 3.211 |
| 20%vol | 2.04 | 3.377 |
| 30%vol | 2.30 | 3.570 |
| Gas Composition | Molar Mass (g/mol) | Density 1 (kg/m3) | Higher Heating Value (kJ/m3) | Lower Explosive Limit (%vol) |
|---|---|---|---|---|
| 100%volHe | 4 | 0.0032 | Null | Null |
| 100%volN2 | 28 | 0.0226 | Null | Null |
| 80%volHe + 20%volN2 | 8.8 | 0.0071 | Null | Null |
| 100%volCH4 | 16 | 0.0129 | 37,768 | 4.60 |
| 90%volCH4 + 10%volH2 | 14.6 | 0.0118 | 35,200 | 4.53 |
| 80%volCH4 + 20%volH2 | 13.2 | 0.0107 | 32,632 | 4.47 |
| 70%volCH4 + 30%volH2 | 11.8 | 0.0095 | 30,064 | 4.40 |
| Composition of Gas Cylinders | Pressure/MPa | Capacity/L |
|---|---|---|
| N2 | 14.0 ± 0.5 | 40 |
| He | 14.0 ± 0.5 | 40 |
| 80%N2 + 20%He | 9.5 ± 0.5 | 40 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, H.; Deng, Q.; Huang, X.; Li, D.; Pan, F. Simulation and Experimental Verification of Dispersion and Explosion of Hydrogen–Methane Mixture in a Domestic Kitchen. Energies 2024, 17, 2320. https://doi.org/10.3390/en17102320
Xu H, Deng Q, Huang X, Li D, Pan F. Simulation and Experimental Verification of Dispersion and Explosion of Hydrogen–Methane Mixture in a Domestic Kitchen. Energies. 2024; 17(10):2320. https://doi.org/10.3390/en17102320
Chicago/Turabian StyleXu, Haidong, Qiang Deng, Xiaomei Huang, Du Li, and Fengwen Pan. 2024. "Simulation and Experimental Verification of Dispersion and Explosion of Hydrogen–Methane Mixture in a Domestic Kitchen" Energies 17, no. 10: 2320. https://doi.org/10.3390/en17102320
APA StyleXu, H., Deng, Q., Huang, X., Li, D., & Pan, F. (2024). Simulation and Experimental Verification of Dispersion and Explosion of Hydrogen–Methane Mixture in a Domestic Kitchen. Energies, 17(10), 2320. https://doi.org/10.3390/en17102320








