1. Introduction
The concept of “Distortion Power” has been known in electrical engineering since 1927 [
1,
2,
3,
4]. The author of this formulation was C.I. Budeanu [
1]. According to Budeanu’s basic theory, there can be three powers in a system with nonsinusoidal waveforms, i.e., active power, reactive power, and the aforementioned distortion power. Over the years, countless scientists from all over the world have tried to explain why there is an increase in distortion power after connecting the optimal capacity to compensate for reactive power [
5,
6,
7,
8,
9,
10]. For nearly 100 years, since the Budeanu theory was developed, no one has been able to relate the parameters of the load to the distortion power [
11], and, for the same number of years, no one has been able to explain why compensating for reactive power with a capacitor leads to an amplification in the value of distortion power [
2,
7,
8,
9,
10]. It was only through the work of the authors of [
11] and using the Currents’ Physical Components (CPC) theory by Czarnecki [
2,
4,
5,
6] that it was possible to determine the component powers involved in Budeanu’s distortion power. As there was no correlation between the distortion power and the equivalent parameters of the load in earlier work, it was also impossible to build an effective passive compensator. It was also impossible to find the optimal capacity [
7,
8,
9,
10], or, once the optimal capacity was chosen, the problem of increasing waveform distortion could not be interpreted, despite the fragmentary reactive power compensation described by Budeanu.
The approach to passive compensator design has declined in recent years. However, in the 1980s and 1990s, it was completely different. It was during these two decades that most works referring to compensators/filters/passive systems were produced [
12,
13,
14,
15,
16,
17,
18]. Depending on the approach and energy description, it was attempted to find the optimal capacity or inductance [
12,
19,
20] and build an LC compensator system with series and parallel connected elements [
2,
6,
21,
22] so that specific current harmonics caused by the nonsinusoidal voltage waveform could be compensated. These decades also saw the development of a method based on minimizing reactive current rather than fully compensating for it [
2,
6,
21,
22,
23,
24,
25]. To date, passive compensators are used in conjunction with power electronic filters (known as active power filters), forming hybrid systems [
13,
15,
22,
26,
27]. In principle, the approach to hybrid systems is relatively basic, as it involves fitting capacitors or chokes for a chosen amount of reactive power and then adding a power electronics system to compensate for the remaining reactive power, which is a significantly lower value [
26,
27,
28,
29,
30].
This might seem to be abandoning the use of passive compensators. However, due to economic aspects, they are still extensively used in various areas. The first such sector in which passive compensators have been used for many years almost without interruption is street lighting [
31,
32,
33,
34,
35]. Although there has been a rapid expansion in the use of LED lighting worldwide [
36,
37], passive systems are still being implemented. Only the design of the compensator has changed, as, previously, capacitors were used for compensation, while now, due to the capacitive nature of reactive power generated by electronic systems, chokes are installed [
35,
36]. The second area of the use of reactance compensators is in the broadly considered industry [
38,
39,
40,
41]. The industrial community uses passive systems because of their potential for power factor buffering [
38,
39,
41]. In this area, passive systems very often differ from each other, mainly in terms of how chokes or capacitors are connected. In systems where reactive power does not frequently change over a short period, typical control via contactors is most often used. Where higher responsiveness of the compensator system is needed, power electronic components are used for control, e.g., transistors or thyristors. Another area where passive compensators are applied is Renewable Energy Sources [
14,
42,
43,
44,
45]. Compensators, or LC filters, are used when correcting the supply conditions of heat pumps. Recent studies realized by the authors of this publication show that reactance compensators together with a voltage regulator should also be adopted for photovoltaic installations. The reason is the increase in voltage at the point of connection of such a PV installation [
44,
45]. The last area where cost-effective passive systems should be used to compensate for reactive power or improve power conditions is the sector of wireless power transmission (WPT) [
46,
47,
48,
49,
50,
51]. Such systems are characterized by the use of coils for energy transfer and are primarily based on the power supply system that is locally available. A specific type of passive compensators are interference filters, which can be found in a great many devices [
52,
53,
54].
The article is divided into eight Sections.
Section 1 presents a description of Budeanu’s power theory and a literature review related to the design of passive compensators and reactance elements selected for hybrid systems. An area where those systems can be used has also been added to the literature review. The
Section 2 is a short description of the extended Budeanu theory with the current components of a single-phase linear load powered from a nonsinusoidal voltage source.
Section 3 describes the process of designing passive compensators in the form of the optimal capacity or optimal inductance. This section presents the relationships and assumptions that should be satisfied to compensate for the Budeanu reactive current. The
Section 4 is theoretical illustration 1, which shows the calculation of the original system. Thus, the equivalent parameters of the load and the values of the component currents before compensation were obtained. The rest of
Section 4 presents the value of the capacitor with the optimal capacity and also the necessary calculations that resulted in a change in the values of the various equivalent parameters and the component currents of the load.
Section 5 describes further reactance compensator structures designed based on the Currents’ Physical Components (CPC) theory. The section includes information on LC- or L-compensator structures and the relationships through which the optimal values for capacitor capacity and choke inductance are accomplished. The
Section 6 is theoretical illustration 2, in which an LC compensator is added to the original system. This section presents the calculated equivalent parameters and component currents of a single-phase load after compensation with an LC compensator. The
Section 7 presents an analysis of the results obtained from different compensator structures. The phenomenon of an increase in the distortion of the current waveform of the load due to the use of a capacitor with an optimally chosen capacity was also analyzed, which has been described quite widely in the literature. The final
Section 8 presents conclusions based on the literature review, the obtained calculated values, and the performed analysis.
2. Currents’ Components in Extended Budeanu Theory
The extended Budeanu theory is described in [
11]. The basis that made it possible to develop the Budeanu approach is the Currents’ Physical Components (CPC) theory made by Czarnecki [
2,
4,
5,
6]. Both approaches are based on the identification of equivalent single-phase load parameters. It is, therefore, possible to identify the component currents of the load and relate them to physical phenomena by means of specific equivalent parameters. The individual component currents, together with the voltage, form the powers of a single-phase linear load, following the extended Budeanu theory.
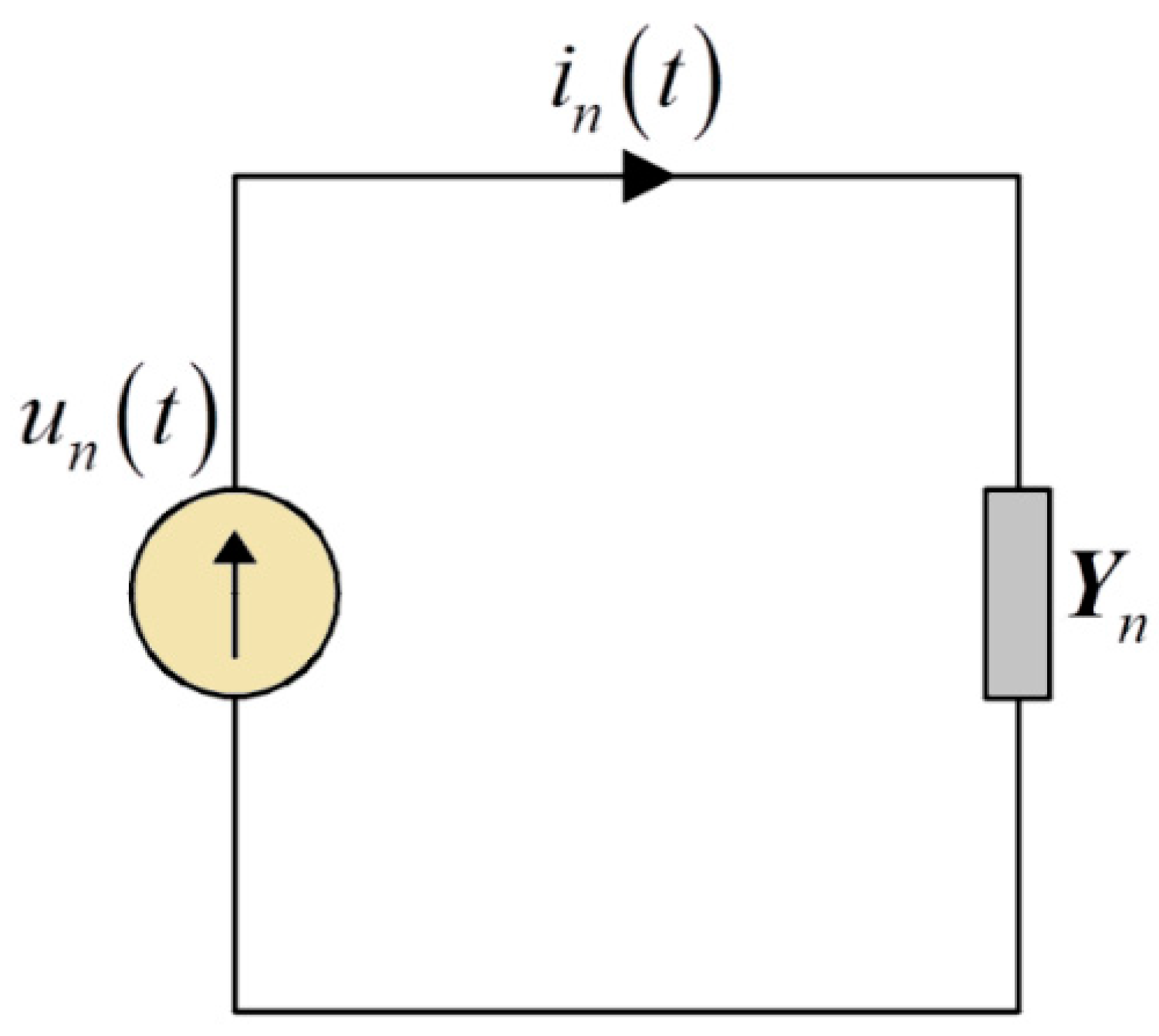
A load, connected in a single-phase system (
Figure 1), can be powered by the nonsinusoidal voltage source, whose waveform can be described as
where
is the complex RMS (CRMS) value of the voltage harmonic, and
is the harmonic order.
The result of applying voltage (1) to the terminals of the time-invariant linear load (LTI) of
Figure 1 is a line current with a waveform represented by the equation:
where
is the load admittance for specific harmonics, and
is the CRMS value of the current harmonic.
If the LTI load shown in
Figure 1 is configured with an impedance, its admittance at different harmonic frequencies is
where
and
denote the conductance and susceptance for individual harmonics.
From relationships (1) and (3), the active current
, for which the load is equivalent to the primary load given the active power
P obtained from the sum of the active powers of the respective harmonics, is as follows:
where
denotes the equivalent conductance of the entire system and is represented by the relation:
Budeanu’s reactive current
waveform, which is the equivalent susceptance of the entire system, takes the following form:
where the equivalent susceptance
takes the relation:
The RMS values of the active current
and also of Budeanu’s reactive current
are
In consequence of the variation in the conductance values for the specific harmonics in the system, there can also be the scattered current
(developed and defined by Czarnecki), the waveform of which is described as
The RMS value of the scattered current
is
The last current’s component of the LTI load is Budeanu’s complemented reactive current, which waveform takes the following form:
The RMS value of Budeanu’s complemented reactive current
is
Following relations (4), (6), (10), and (12), the load current in a single-phase system has four components:
for the reason of the orthogonality [
2,
11,
55] of the specific currents, the notation is true:
If the current components are multiplied by the square of the RMS value of the nonsinusoidal voltage
, the power equation describing the single-phase load is achieved:
In publication [
11], it was noticed that the deformation power
, defined by Budeanu, consists of (maximum) two powers:
Based on the deformation power equation (17), the equation for the deformation current can be derived:
This means that the deformation power as well as the deformation current can be associated with the equivalent parameters of the LTI load associated with two currents, i.e., the scattered current and the Budeanu complemented reactive current. The scattered current is associated with the resistance, so this means that its compensation can only be accomplished through power electronics systems (series compensation is not considered). Thus, there remains the question of the Budeanu complemented reactive current, which can be compensated through passive systems due to its association with reactance elements.
Both before and after compensation, it is possible to determine the
power factor, which is defined as follows:
It should be noted that this is the power factor, which is determined along with the existing harmonics. Commonly, the power factor (especially in publications related to power electronic converters) is close to unity. This is somewhat true, but only if the power factor is described by the trigonometric function of the cosine and only applies to the fundamental harmonic of the current.
3. Selected Structures of Passive Compensators—Part 1
The major way to correct the power factor value is passive compensation (usually parallel). Such a compensator makes it possible to change the supply current in such a way as to reduce its RMS value. The use of this type of compensation, as a rule, does not significantly change the conditions of the power supply of the load, although it does slightly modify the supply voltage.
In the simplest situation, a passive compensator is a reactance element in the form of a capacitor with an optimally selected capacity. Nowadays, more and more often, mainly due to power electronics structures, chokes with an optimally selected inductance are used. The discussed structures are shown in
Figure 2.
When designing a passive compensator in the form of a capacitor or choke, it should be assumed that it does not affect the equivalent conductance or the conductance for harmonic frequencies . This means that the compensator does not change the active current or the scattered current. The reactance compensator, however, modifies the susceptance seen from the power source. Therefore, it changes the current associated with the reactance elements (the Budeanu reactive current and Budeanu complemented reactive current).
If the susceptance of the passive compensator for harmonic frequencies is
, then the Budeanu reactive current has a waveform:
at the same time, the susceptance of the reactance compensator also affects the value of the Budeanu complemented reactive current:
The RMS value of the Budeanu reactive current is defined by the relation:
and the RMS value of the Budeanu complemented reactive current equals
In addition, ideally, the susceptance of the compensator would be such that for each harmonic present in the supply voltage, the condition would occur [
2,
6]:
The choice of the optimal capacitor capacity results from the following relationship:
In contrast, the optimal inductance of the choke is selected based on the equation:
Selecting the optimal capacity or inductance based on relationships (25) and (26) can, in the worst situation, lead to resonant current amplification for the fundamental harmonic.
If the condition in relationship (24) is accomplished, then the total compensation of the Budeanu complemented reactive current and the Budeanu reactive current is possible. In addition, it is possible to achieve the maximum power factor
, namely,
The above means that it is not possible to completely compensate for the distortion current and, at the same time, the distortion power proposed by Budeanu in a single-phase system, as long as the conductance changes along with the frequency value.
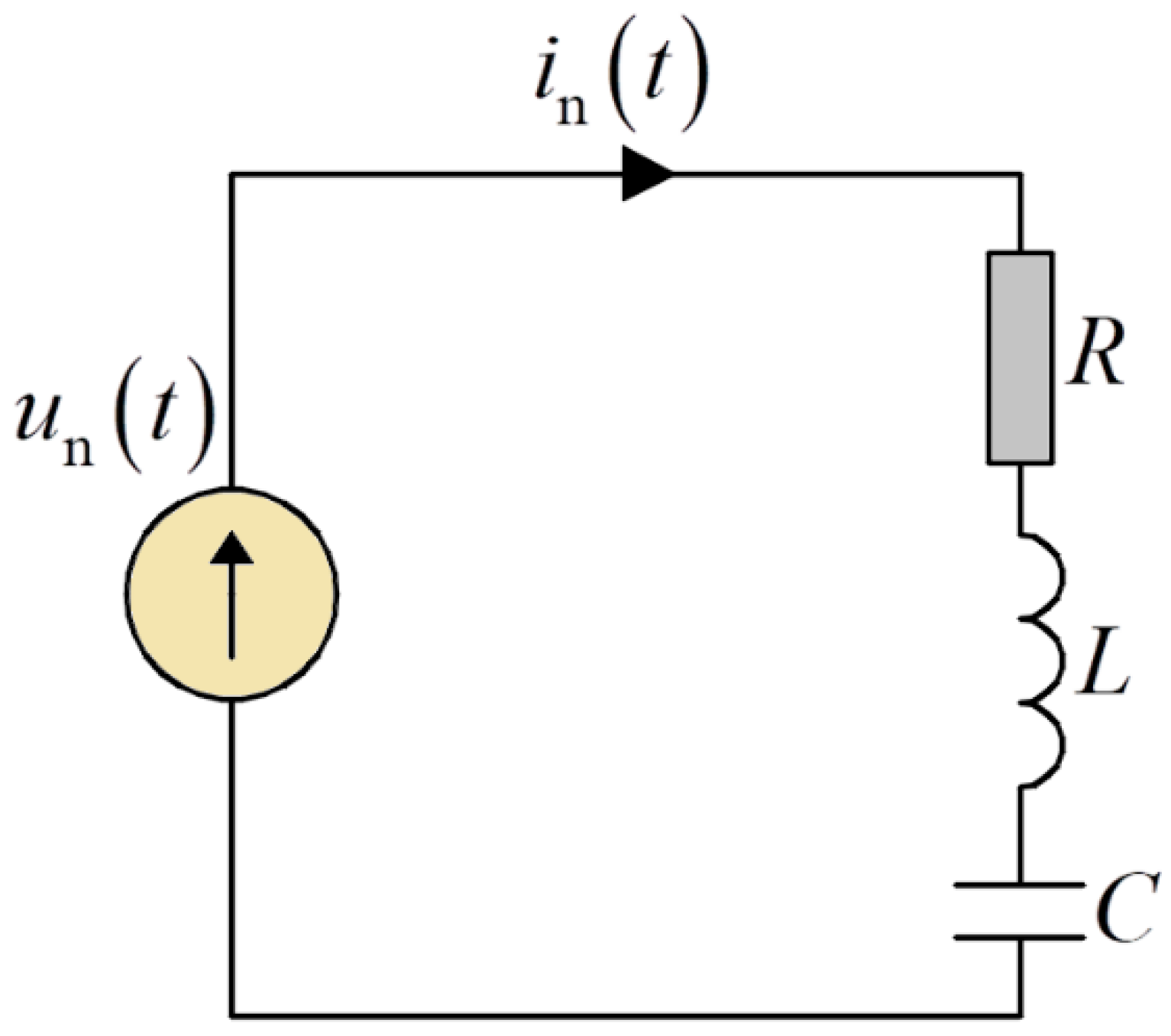
4. Theoretical Illustration 1
The single-phase circuit powered by the nonsinusoidal voltage in
Figure 3 was used to provide a theoretical illustration. All analyses were calculated under the assumptions of the linearity and invariability of the load’s parameters over time.
The values of the individual elements, presented in
Figure 3, are included in
Table 1.
As a result of the supply voltage and load parameters that have been described, the equivalent load parameters’ values described by expressions (3), (5), and (7) are given in
Table 2.
By applying a voltage to the terminals of the load, the currents of the individual harmonics and the active and reactive powers [
11] for the harmonics are received. All the achieved results, plus the RMS or sum values, are summarized in
Table 3.
At the same time, the system shown in
Figure 3 has an apparent power
of 16,659.2 VA and a distortion power
of 1793.6 VA.
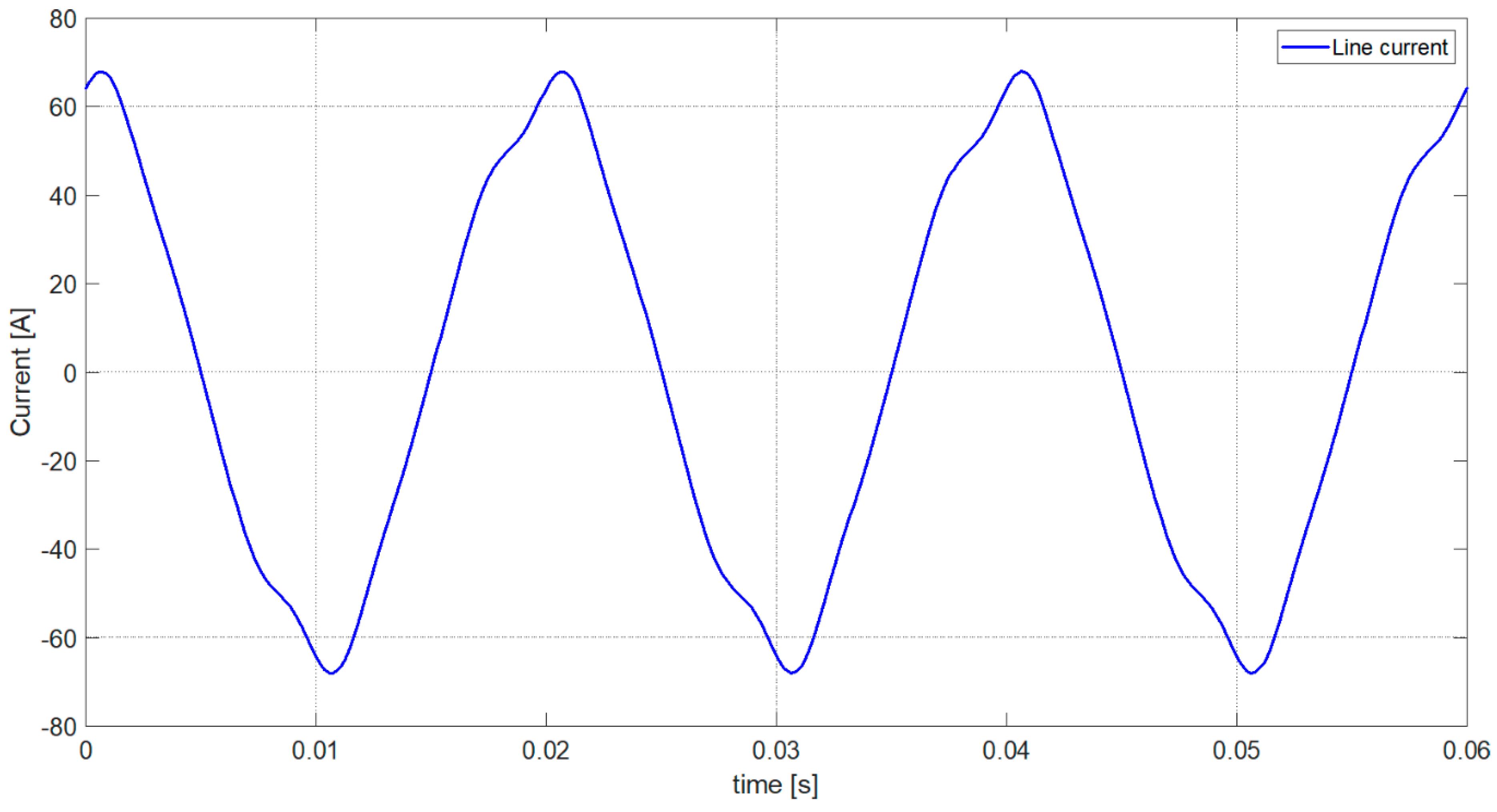
The line current waveform of a single-phase load, before compensation, is presented in
Figure 4.
Based on the relationships for the active current (4), Budeanu reactive current (6), scattered current (10), and Budeanu complemented reactive current (12), the CRMS values of the individual component currents are listed in
Table 4.
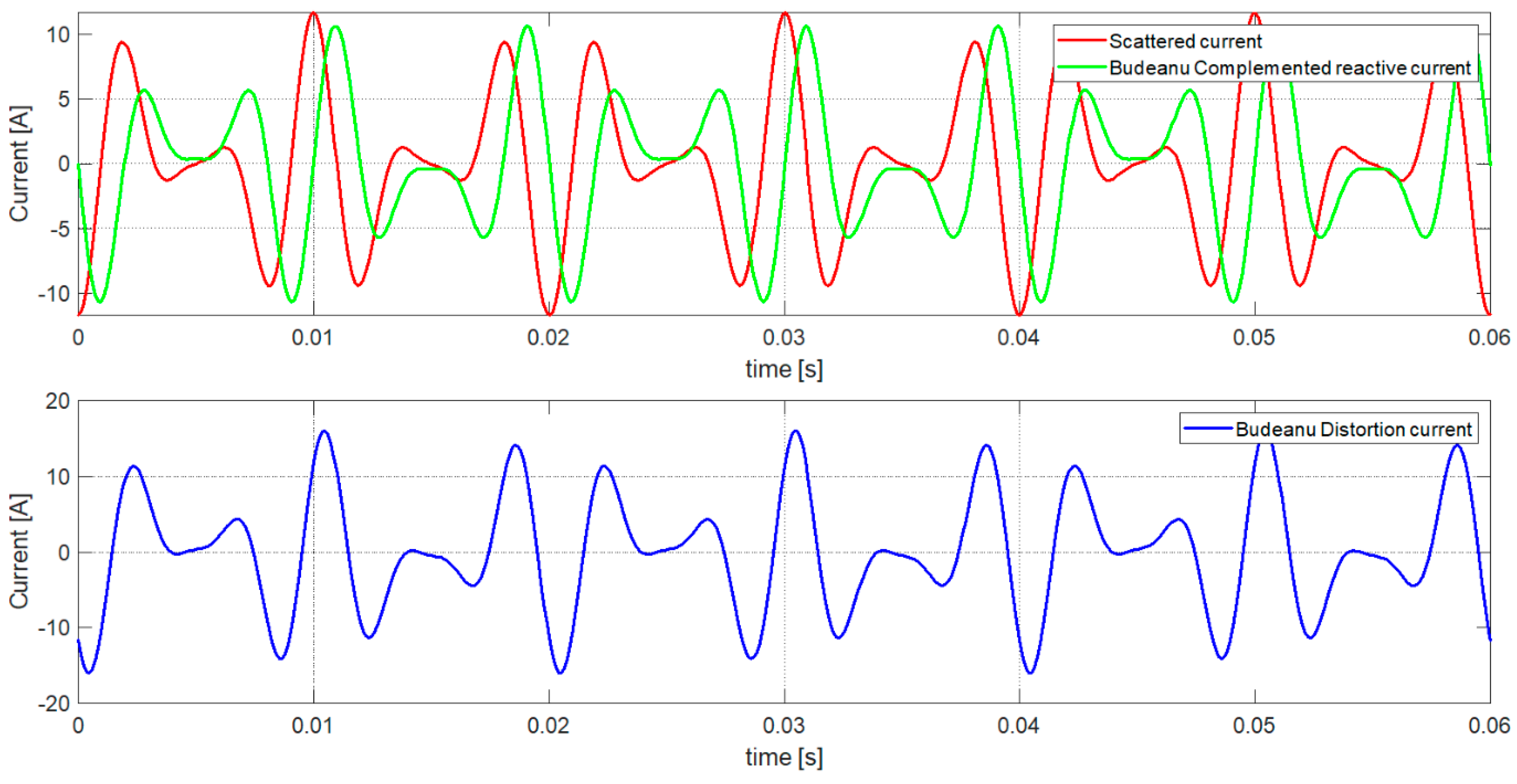
Thereby, the RMS value of the scattered current is 5.611 A, and the Budeanu complemented reactive current is 5.316 A. The existence of both currents in the system causes the Budeanu distortion current to have two components. The RMS value of the Budeanu distortion current is equal to 7.729 A.
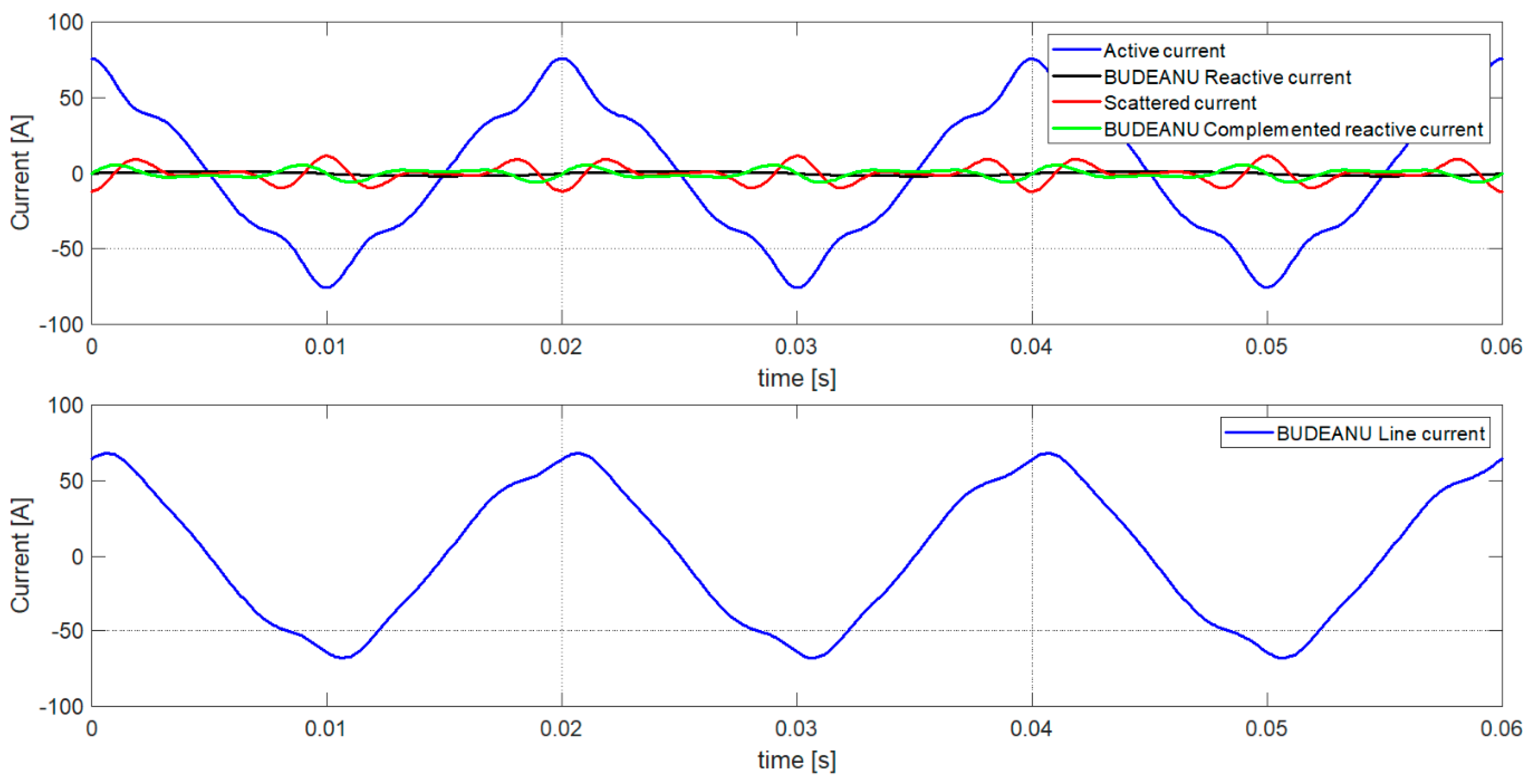
The component currents’ waveforms according to the developed Budeanu theory are presented in
Figure 5.
Figure 6, however, shows the distortion current waveform along with its components, namely, the scattered current and the Budeanu complemented reactive current.
Based on the relation (19), the
power factor of the system shown in
Figure 4 is
In the above theoretical illustration 1, it can be seen that a capacitor should be used to compensate for Budeanu’s reactive power. The selection of the capacitor capacity is based on Equation (25). After calculating the optimal value of the capacitor capacity for the system shown in
Figure 3, the equivalent parameters of the load seen from the side of the power source changed. As a result of the change in the equivalent parameters, the component currents associated with the reactance elements change, and, consequently, so do the component powers. Below,
Figure 7 shows the system with the connected capacitor with the optimal capacity.
Based on calculations ensuant from the ability to compensate for the equivalent susceptance of the entire system, the optimal capacitance is . The optimal capacity was determined from Equation (25), with the susceptance value for the fundamental harmonic replaced by the equivalent susceptance value of the entire system.
After applying a voltage to the terminals of the load, the following results for the equivalent parameters of the load were obtained.
Table 5 summarizes the equivalent parameters of the load with the included optimal capacity.
Table 6 includes the harmonic currents and the active and reactive powers’ values after connecting a compensator to the equivalent susceptance for the whole system.
After connecting the optimal capacity, as shown in
Figure 7 and summarized in
Table 6, it follows that the RMS value of current
decreased from 71.79 A to 56.33 A. Therefore, Budeanu’s reactive power also decreased from 12964.1 var to 854.6 var and changed its character from inductive to capacitive. The compensated system has an apparent power
of 13,071.7 VA and a distortion power
of 7993.4 VA.
The line current waveform in a single-phase load after compensation with the optimal capacity is presented in
Figure 8.
Based on the relationships for the active current (4), Budeanu reactive current (6), scatter current (10), and Budeanu complemented reactive current (12), the CRMS values of the individual component currents after compensation with the optimal capacity are summarized in
Table 7.
It can be observed from
Table 7 that both the active current and the scattered current did not change their CRMS values because they are related to the resistive element. The component currents associated with the reactance elements of the system changee. While the Budeanu reactive current takes on a satisfactory CRMS, the CRMS of the Budeanu complemented reactive current significantly increases. This means that the RMS value of the scattered current
is still 5.611 A, and the Budeanu complemented reactive current
increases from a value of 5.316 A to a value of 33.986 A. The significant boost of the RMS value of the Budeanu complement reactive current impacts an increase in the RMS value of the Budeanu distortion current
, which is now 34.446 A.
Figure 9 presents the component currents’ waveforms according to the extended Budeanu theory after the connection of the optimal capacity.
Figure 10, in contrast, shows the waveform of the distortion current with its components, namely, the scattered current and the Budeanu complemented reactive current, after connecting the optimal capacity.
Based on the relation (19), the power factor
of the system shown in
Figure 8 is
5. Selected Structures of Passive Compensators—Part 2
Another type of reactance compensator is a combination of a series capacitor and choke (LC compensator) or a choke alone (L).
Figure 11 shows the possible structures of the passive compensators discussed in this section.
The basic principle of selecting a compensator structure is simple. If, for the fundamental harmonic frequency, the susceptance has an inductive character, then the structure of the compensator of the LC-type should be selected [
2,
6,
21]. This is related to the fact that above the fundamental harmonic frequency, the reactance of the LC-type compensator is inductive and does not cause resonant harmonic amplification. The second case involves an L-type compensator [
2,
6,
21]. This is used when the capacitive nature of the susceptance is responsible for the Budeanu reactive current.
The first step in selecting the optimal parameters of an LC compensator is to choose the initiative capacitance
, which is the capacitance needed for the total reactive power compensation of the fundamental harmonic. Most conveniently, the initiative capacitance is determined from the following relationship:
The second step is the choice of capacitance
, which, as the relationship below shows, depends only on the choice of the resonance frequency
, namely,
After calculating the capacitance of
and choosing the resonant frequency
, it is possible to choose the optimal inductance, depending on the aforementioned two parameters:
A slightly more difficult step is to determine, through an iterative relationship, the optimal capacity, i.e., the capacity through which the RMS value of the current of the power source (the component(s) associated with the reactance elements) can be minimized. This relationship takes the following form:
The final step is to check the resonant frequency limitation, that is, to satisfy the condition:
If everything is fine, then stay with the selected optimal inductance. If condition (32) is not satisfied, then another value of inductance is selected, and the calculation must be repeated.
If the susceptance of the load has a capacitive character, then a compensator built from the choke itself should be used. Accordingly, the relation (28) takes the following form:
The next step is to check whether the initiative inductance coincides with the optimal inductance, which is determined from the following relationship:
The design procedure outlined above is a simplified proceeding of the minimum needed to select the appropriate capacitance and inductance parameters. A more detailed description of the procedure for selecting LC and L compensator parameters can be found in [
2,
6].
6. Theoretical Illustration 2
For theoretical illustration 2, the same system as the one shown in
Figure 3 was chosen. The difference is in the use of a different type of passive compensator built from an LC element. The design methodology and the relationships based on which appropriate capacitance and inductance parameters are selected are described in
Section 5.
After calculating the optimal value of the capacitor capacity (31) and choosing the optimal value of the choke inductance (30) for the system presented in
Figure 3, the equivalent load parameters observed from the power source change. As a result of the change in equivalent parameters, the component currents associated with the reactance elements change, and, consequently, so do the component powers. Below,
Figure 12 shows a system with a connected capacitor with the optimal capacity and a choke with the optimal inductance.
Based on calculations ensuant from the ability to minimize the Budeanu reactive current, the optimal capacitance is . In addition, given that the power system may be operated with no danger and that the currents do not resonate at the fundamental harmonic or the characteristic harmonics of the higher orders, an optimal inductance value for the of was selected. The optimal capacity was determined from Equation (31), with the susceptance values for individual harmonics replaced by the value of the equivalent susceptance of the whole system.
When voltage is applied to the system terminals, the following results for the equivalent load parameters were obtained.
Table 8 lists the equivalent load parameters with the LC compensator connected.
Table 9 lists the harmonic currents and the active reactive powers’ values after connecting the minimizing compensator of the Budeanu reactive current.
After connecting the LC compensator, as shown in
Figure 12 and from the information in
Table 9, the current’s RMS value
decreased from 71.79 A to 44.885 A. At the same time, the reactive power also decreased from 12,964.1 var to 257.7 var. The compensated system has an apparent power
of 10,415.9 VA and a distortion power
of 1476.7 VA.
The line current waveform of a load after compensation with an LC compensator is presented in
Figure 13.
Based on the relationships for the active current (4), Budeanu reactive current (6), scattered current (10), and Budeanu complemented reactive current (12), the CRMS values of the individual component currents after minimizing the Budeanu reactive current are summarized in
Table 10.
As can be seen from
Table 10, both the active current and the scattered current have not changed their CRMS because they are related to the resistive element. What did change was the component currents associated with the reactance elements of the system. The obtained CRMS values of the Budeanu reactive current and the Budeanu complemented reactive current are at a very good level. This means that the RMS value of the scattered current
is still 5.611 A, and the value of the Budeanu complemented reactive current
drops from 5.316 A to 3.002 A. The reduction in the RMS value of the Budeanu complemented reactive current impacts a reduction in the RMS value of the Budeanu distortion current
, which equals 6.363 A.
Figure 14 presents the component currents’ waveforms according to the extended Budeanu theory after connecting the LC compensator.
Figure 15, in contrast, shows the waveform of the distortion current with its components, that is, the scattered current and the Budeanu complemented reactive current after the LC compensator is connected.
Based on relation (19), the power factor
of the system shown in
Figure 12 is
7. Analysis of the Obtained Results
Table 11 lists the RMS values of the component currents and the percentage change relative to the value of the LTI load’s primary system for each solution, namely,
the RMS values of the component currents of the load shown in
Figure 3;
the RMS values of the component currents of the load shown in
Figure 7;
the RMS values of the component currents of the load shown in
Figure 12.
As can be seen from the data listed in
Table 11, both the RMS values of the active current
and the scattered current
do not change. This is following theoretical principles, which state that reactance elements do not have internal resistances. The omission of the resistance parameter in the design of passive compensators results in no change in the component currents associated with this quantity.
Further analyzing the values presented in
Table 11, one can see a reduction in the RMS value of the Budeanu reactive current. This reaction of the reactive component of the Budeanu is observable both for the system with the selected optimal value of the capacitor capacity
, which is 6.59% of the original value of the Budeanu reactive current (
Figure 3). A lower value for the Budeanu reactive current
was obtained when the LC compensator was placed in the system in
Figure 12. This value equals 1.99% of the value of the Budeanu reactive component. It can, therefore, be considered that both approaches to reactive power reduction fulfill their expectations.
The situation is slightly different if looking at the RMS values of the Budeanu complement reactive current. In the system with the optimal capacitor capacity value, the RMS value of the Budeanu complemented reactive current
increased by 639.3% compared to the original value shown in
Figure 3, with a simultaneous increase in the distortion of the load’s current waveform, as shown in
Figure 8. In the second type of compensator, i.e., the LC structure, the RMS value of the Budeanu complemented reactive current
decreased by 43.53% compared to the original value, with an inconsiderable increase in the distortion of the load’s current waveform, as shown in
Figure 13.
To closely follow the increase in the distortion of the load’s current waveform, it is necessary to look at
Table 12.
It can be seen from
Table 12 that as the Budeanu complemented reactive current for higher harmonics increases, the distortion of the load current also increases. The increase in the CRMS value of the Budeanu complemented reactive current is a straight consequence of the increase in the susceptance
for individual harmonics. The original system (
Table 2) has inductive susceptances
for each harmonic. The system, with the compensator in the form of the optimally selected capacitor capacitance (
Table 5), has an inductive susceptance of a small value for the fundamental harmonic, whereupon for the third, fifth, and seventh harmonics it has capacitive susceptances, whose values increase when the harmonic order increases. Relating the susceptance parameter to the voltage of the individual harmonic, we obtain the value of the Budeanu complemented reactive current, which, as can be seen from
Table 12, has a significant value around the value of the current of the individual harmonics and, thus, deforms the current waveform of the load to a lower (
Figure 3 and
Figure 12) or higher level (
Figure 7).
The information in
Table 11 and
Table 12 is summarized very well by the power factor
. The smaller the RMS value of the individual component currents, i.e., the scattered current, the Budeanu reactive current, and the Budeanu complemented reactive current in the load current relative to the active current, is, then the higher the value of the power factor
is.
8. Conclusions
The studies presented in this publication indicate that it is possible to select the parameters of a compensator with the optimal capacity or optimal inductance. In the extended Budeanu theory, the equivalent susceptance of the whole system represents the Budeanu reactive current. Based on this equivalent parameter, the selection of the optimal capacity is not affected by the occurrence of resonant gain since, in systems with nonsinusoidal waveforms, the equivalent susceptance is different from the susceptance for the fundamental harmonic.
The second type of compensator discussed in this paper is designed using the Currents’ Physical Components (CPC) theory. In theoretical illustration 2 shown above, the LC compensator not only significantly compensates for the Budeanu reactive current but also reduces the RMS value of the Budeanu complemented reactive current. In addition, it slightly affects the deformation of the load current waveform and contributes to a decrease in the RMS value of the Budeanu distortion current.
Both of the discussed passive compensator structures have the effect of limiting the RMS value of the LTI load current. Picking the optimal capacity reduces the RMS value of the load current and the RMS value of the Budeanu reactive current while increasing the Budeanu complemented reactive current, the Budeanu distortion current, and, thus, the Budeanu distortion power. The LC structure reduces the RMS value of the load current and the RMS value of the Budeanu reactive current while minimizing the Budeanu complemented reactive current, the Budeanu distortion current, and the Budeanu distortion power.