Numerical Investigation on the Transition Flow around NLF Airfoil
Abstract
1. Introduction
2. Numerical Method
2.1. Transition Model
2.2. Physical Model and Computational Domain
2.3. Numerical Settings
2.4. Independence Test of Mesh
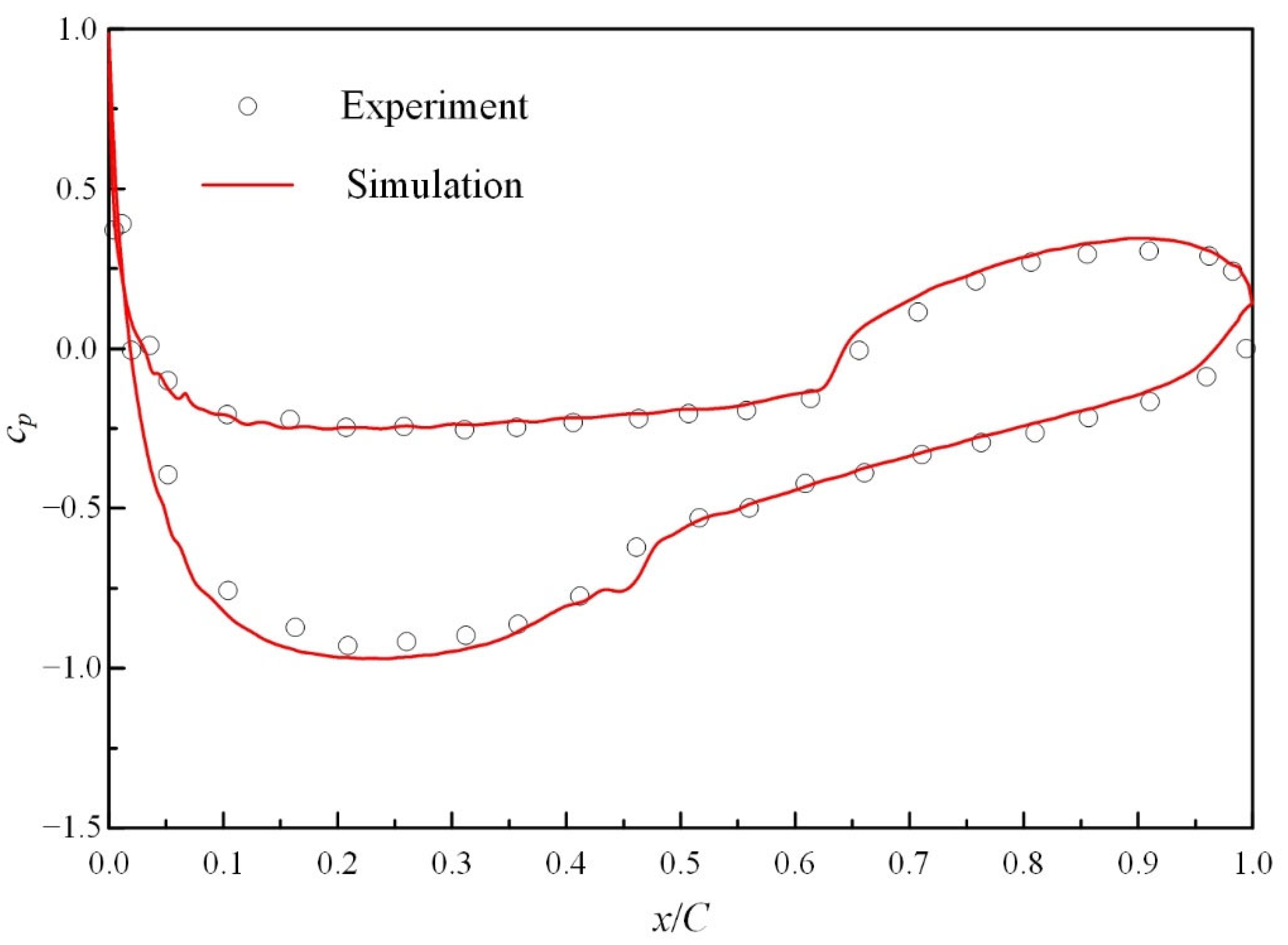
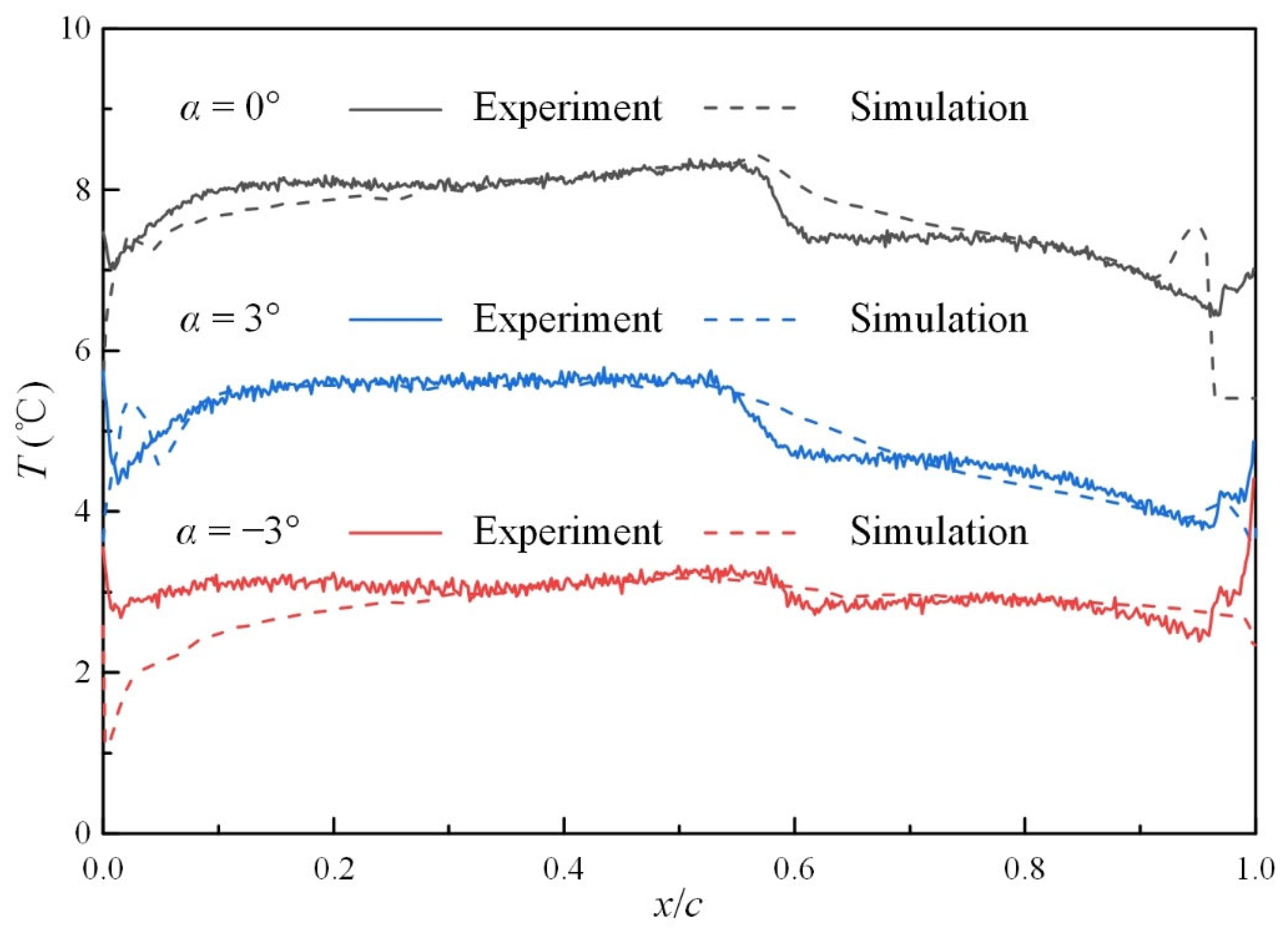
2.5. Verification of Numerical Methods
3. Result and Discussion
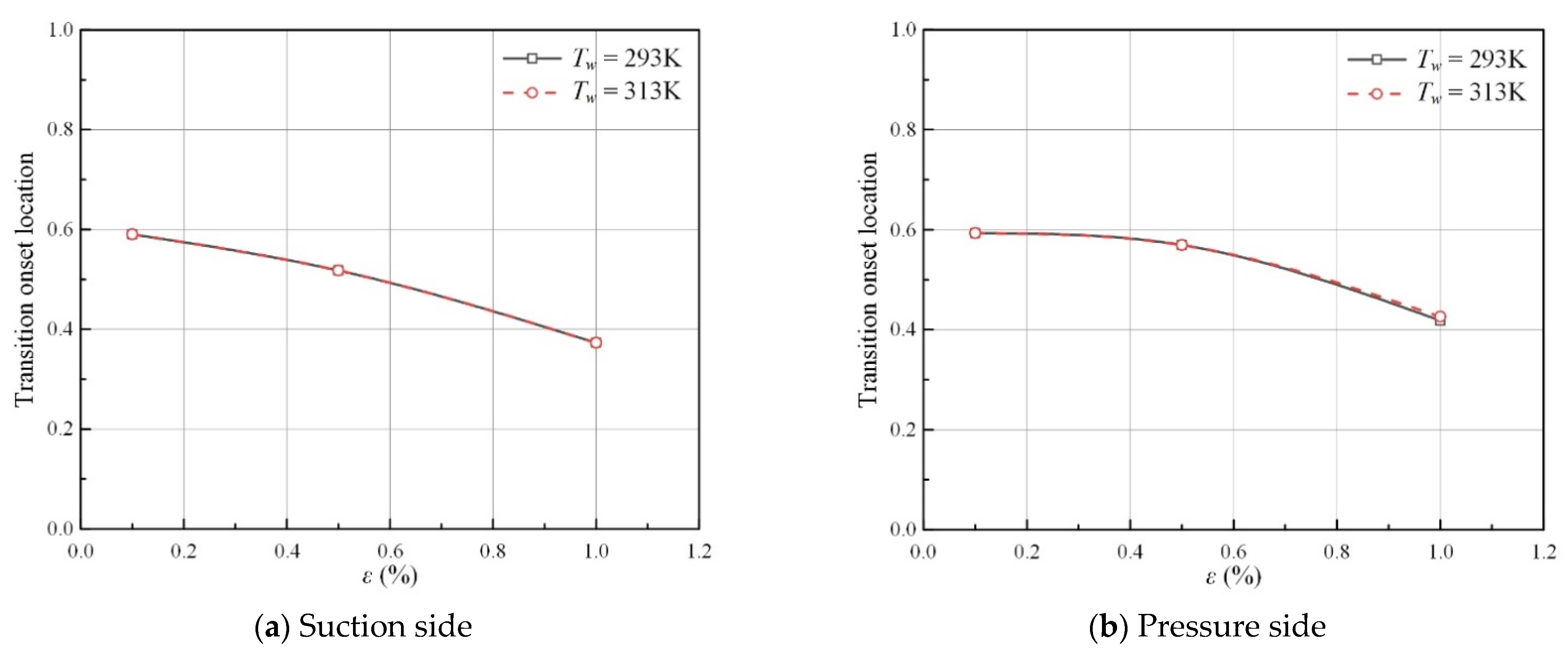
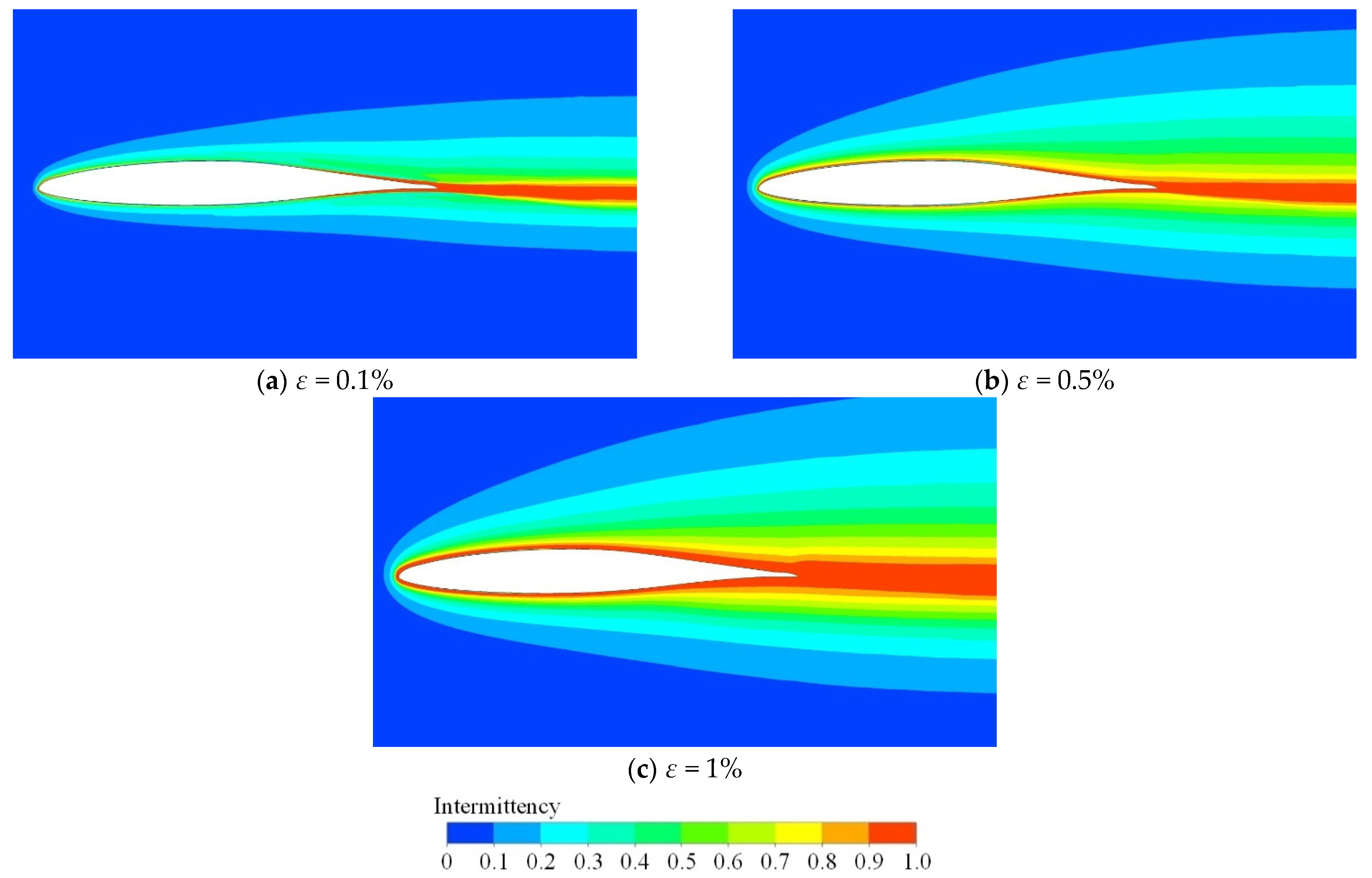
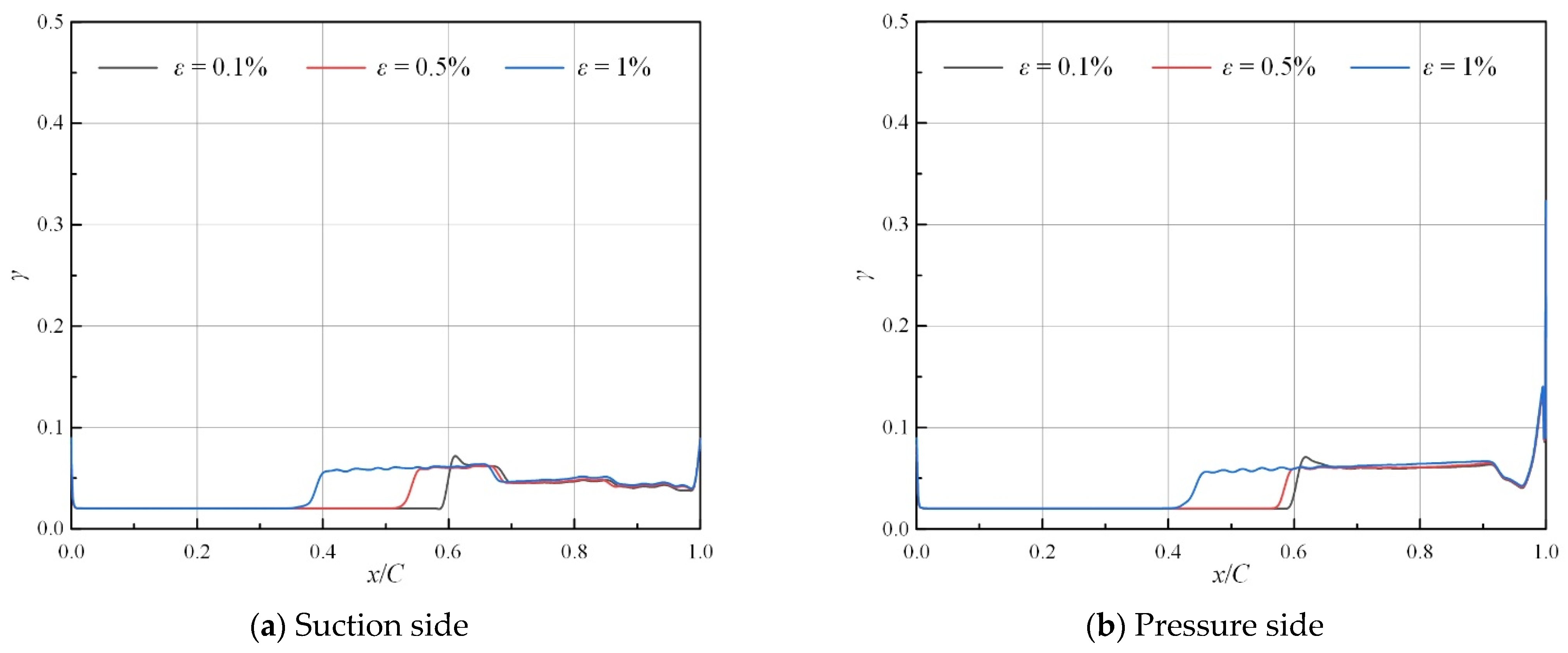
3.1. Influence of Turbulent Intensity
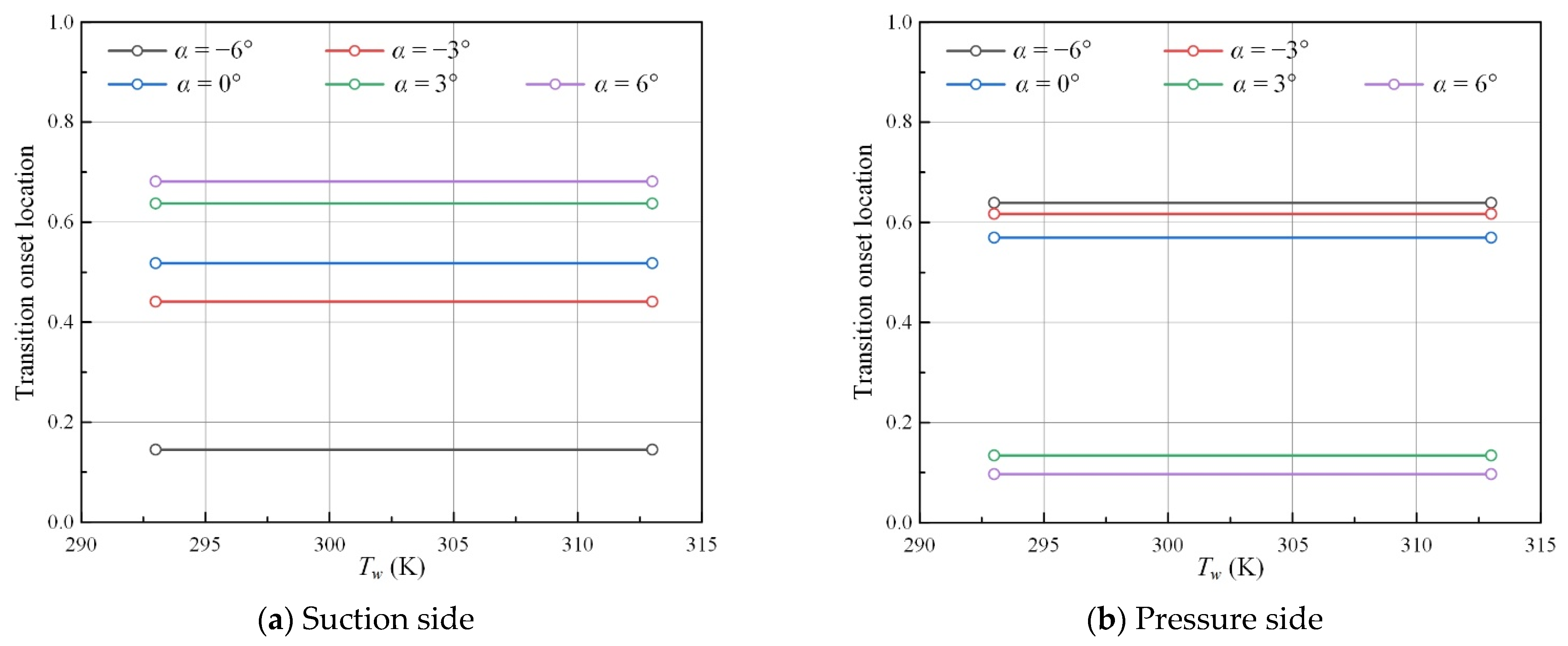
3.2. Influence of Surface Temperature
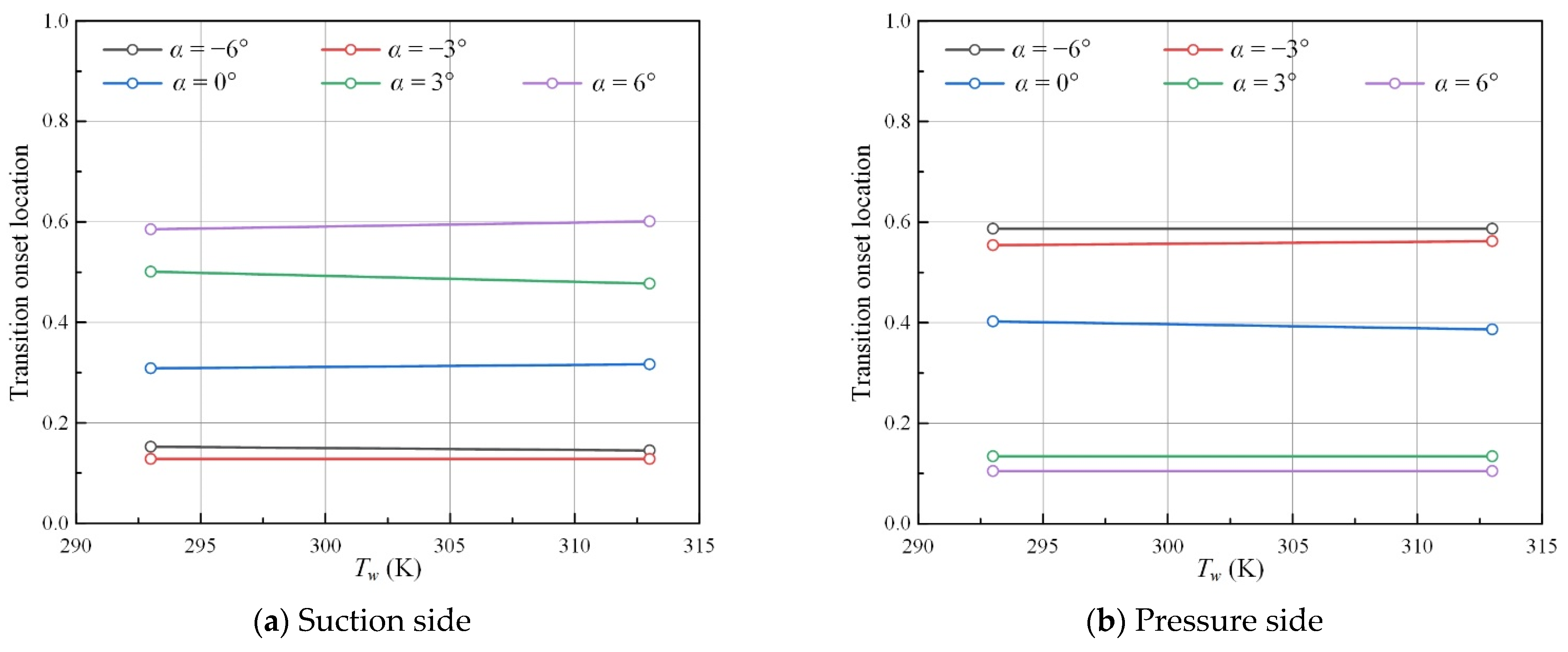
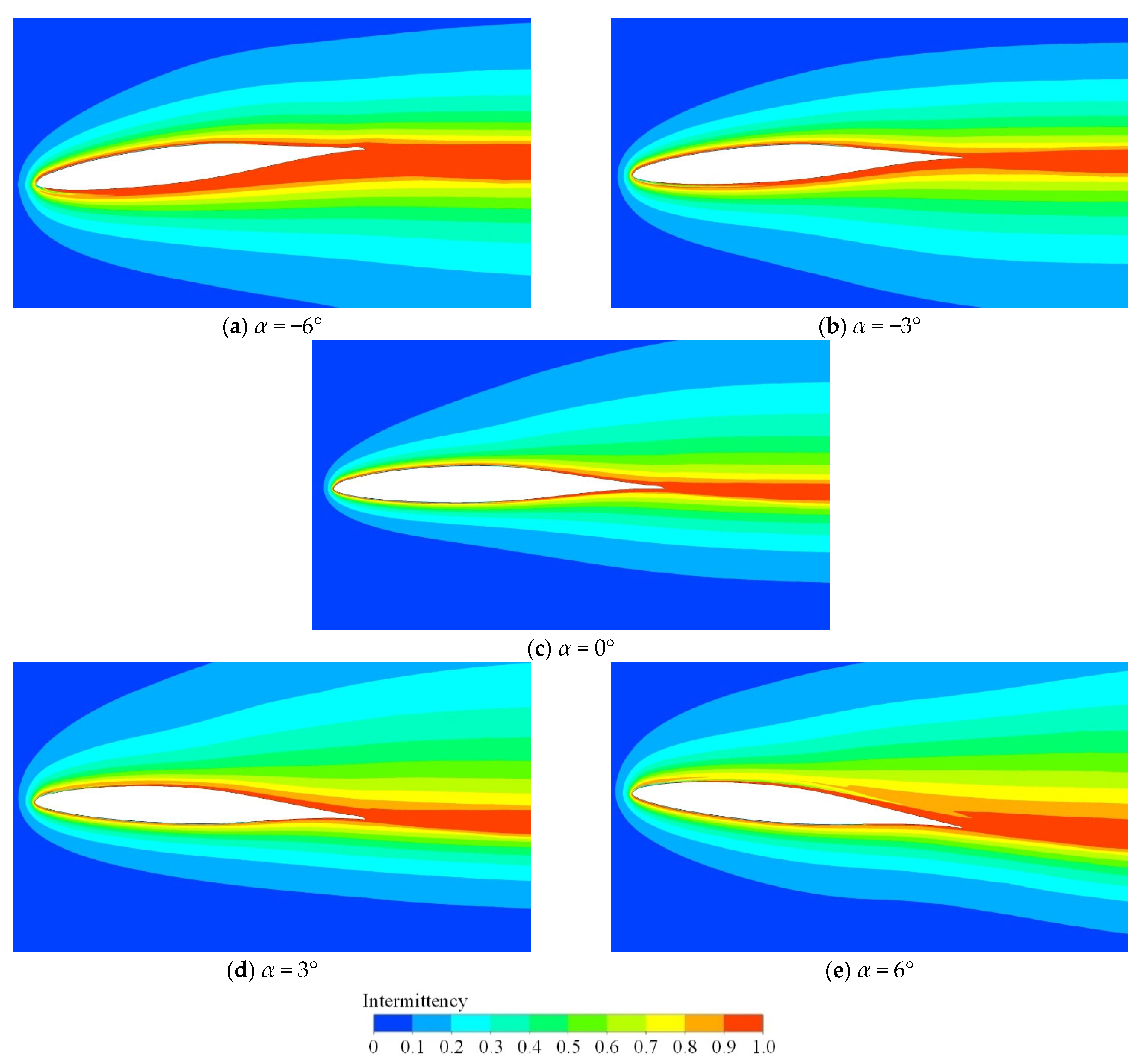
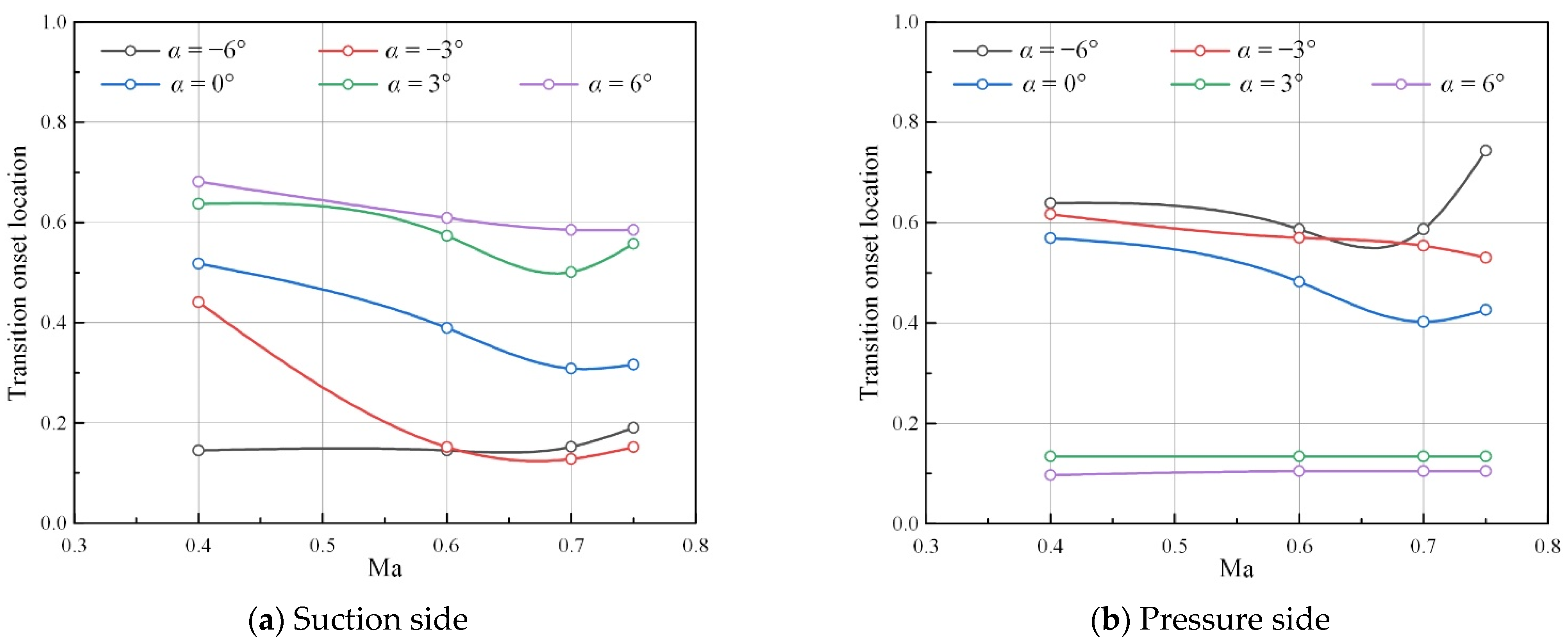
3.3. Influence of Angle of Attack
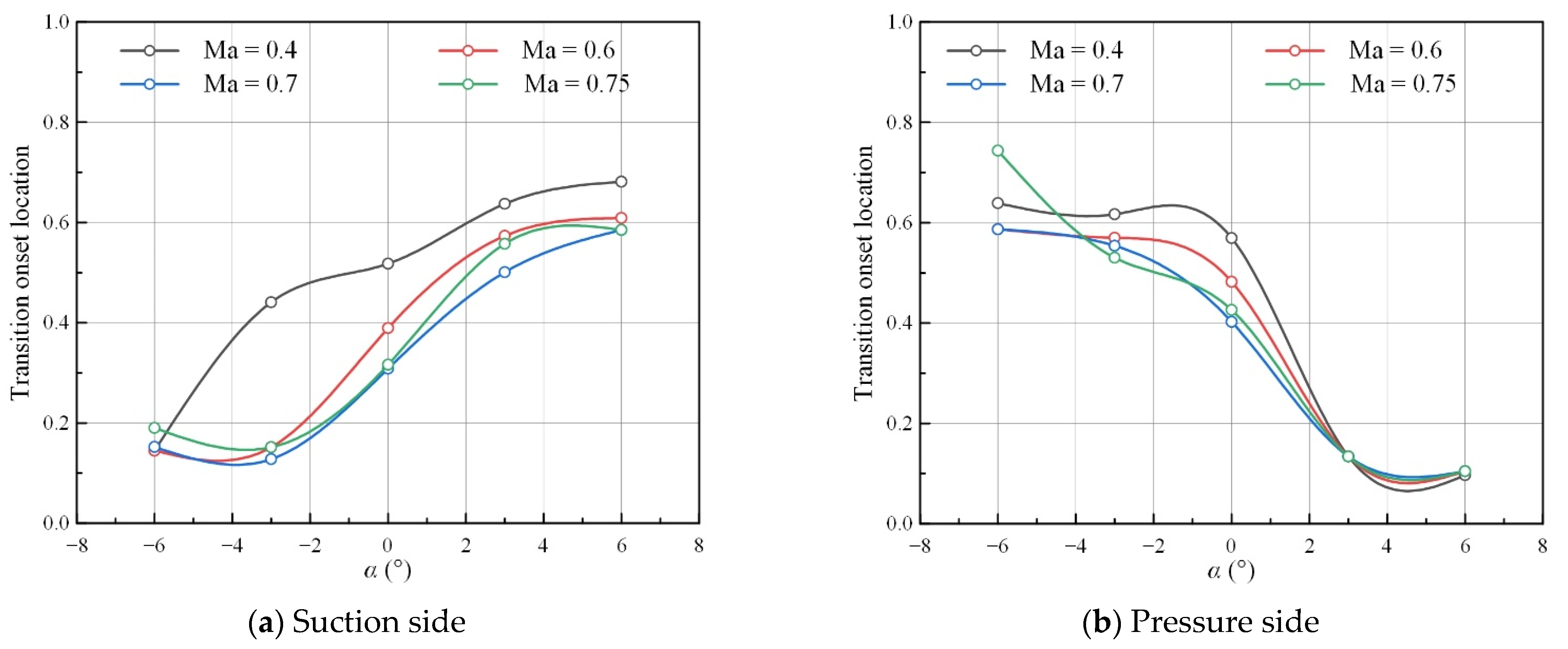
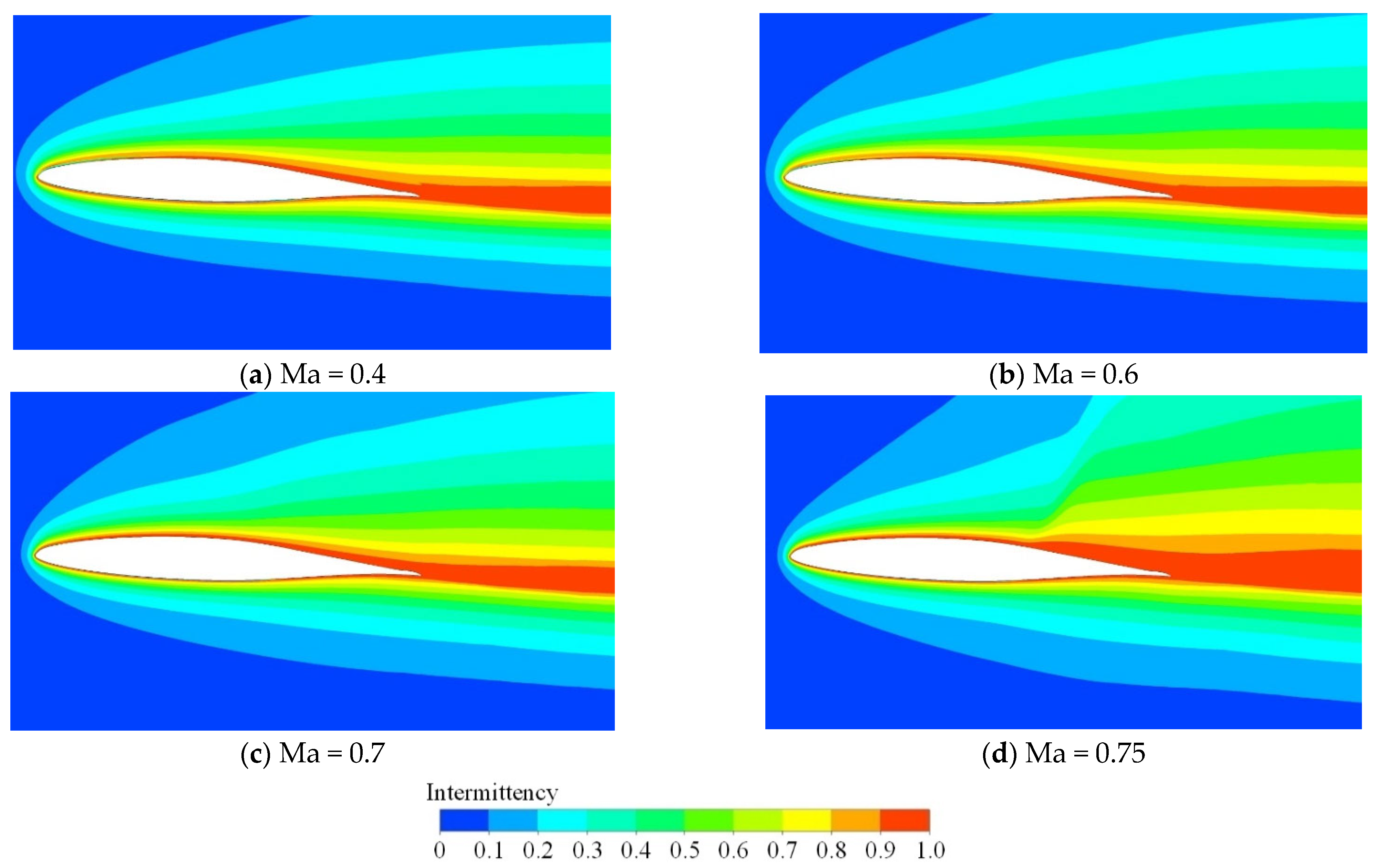
3.4. Influence of Mach Number
3.5. Correlation
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| γ | intermittency |
| k | turbulent kinetic energy |
| ω | turbulent frequency |
| kL | laminar kinetic energy |
| Reθ | momentum thickness Reynolds number |
| ρ | density |
| ui | velocity |
| μ | dynamic viscosity |
| μt | turbulent eddy viscosity |
| σk | constant in governing equation of k |
| γeff | effective intermittency |
| S | strain rate |
| β | model coefficient |
| σω | constant in governing equation of ω |
| αω | model coefficient in governing equation of ω |
| F1 | function in k-ω turbulence model |
| σω,2 | constant in governing equation of ω |
| Ce1 | constant in governing equation of γ |
| Ca1 | constant in governing equation of γ |
| Flength | empirical correlation that controls the length of the transition region |
| Fonset | empirical correlation that controls the transition onset location |
| Ce2 | constant in governing equation of γ |
| Ca2 | constant in governing equation of γ |
| Ω | vorticity magnitude |
| Fturb | model coefficient in governing equation of γ |
| cθ | constant in governing equation of Reθ |
| Reθt | transition Reynolds number |
| Fθ | blending function to turn off the source term in the boundary layer |
| σθ | constant in governing equation of Reθ |
| C | chord length |
| ε | turbulent intensity |
| α | angle of attack |
| Tw | surface temperature |
| xtol,s | transition onset location on suction side |
| xtol,p | transition onset location on pressure side |
| θ | normalized angle of attack |
| τ | normalized surface temperature |
Appendix A
| ε/% | Tw/K | Transition Onset Location | |
|---|---|---|---|
| Suction Side | Pressure Side | ||
| 0.1 | 293 | 0.5903 | 0.5931 |
| 0.5 | 293 | 0.5180 | 0.5694 |
| 1 | 293 | 0.3731 | 0.4182 |
| 0.1 | 313 | 0.5903 | 0.5931 |
| 0.5 | 313 | 0.5180 | 0.5694 |
| 1 | 313 | 0.3731 | 0.4262 |
| Tw/K | α/° | Transition Onset Location | |
|---|---|---|---|
| Suction Side | Pressure Side | ||
| 293 | −6 | 0.1447 | 0.6391 |
| 313 | −6 | 0.1447 | 0.6391 |
| 293 | −3 | 0.4409 | 0.6169 |
| 313 | −3 | 0.4409 | 0.6169 |
| 293 | 0 | 0.5180 | 0.5694 |
| 313 | 0 | 0.5180 | 0.5694 |
| 293 | 3 | 0.6374 | 0.1344 |
| 313 | 3 | 0.6374 | 0.1344 |
| 293 | 6 | 0.6815 | 0.0969 |
| 313 | 6 | 0.6815 | 0.0969 |
| Tw/K | α/° | Transition Onset Location | |
|---|---|---|---|
| Suction Side | Pressure Side | ||
| 293 | −6 | 0.1447 | 0.5869 |
| 313 | −6 | 0.1447 | 0.5869 |
| 293 | −3 | 0.1519 | 0.5697 |
| 313 | −3 | 0.1519 | 0.5776 |
| 293 | 0 | 0.3892 | 0.4819 |
| 313 | 0 | 0.3892 | 0.4819 |
| 293 | 3 | 0.5732 | 0.1344 |
| 313 | 3 | 0.5732 | 0.1344 |
| 293 | 6 | 0.6092 | 0.1048 |
| 313 | 6 | 0.5931 | 0.0969 |
| Tw/K | α/° | Transition Onset Location | |
|---|---|---|---|
| Suction Side | Pressure Side | ||
| 293 | −6 | 0.1523 | 0.5869 |
| 313 | −6 | 0.1447 | 0.5869 |
| 293 | −3 | 0.1278 | 0.5539 |
| 313 | −3 | 0.1278 | 0.5618 |
| 293 | 0 | 0.3086 | 0.4023 |
| 313 | 0 | 0.3166 | 0.3863 |
| 293 | 3 | 0.5009 | 0.1344 |
| 313 | 3 | 0.4768 | 0.1344 |
| 293 | 6 | 0.5851 | 0.1048 |
| 313 | 6 | 0.6012 | 0.1048 |
| Tw/K | α/° | Transition Onset Location | |
|---|---|---|---|
| Suction Side | Pressure Side | ||
| 293 | −6 | 0.1904 | 0.7436 |
| 313 | −6 | 0.1827 | 0.7511 |
| 293 | −3 | 0.1519 | 0.5303 |
| 313 | −3 | 0.1439 | 0.5933 |
| 293 | 0 | 0.3166 | 0.4262 |
| 313 | 0 | 0.3489 | 0.5614 |
| 293 | 3 | 0.5572 | 0.1344 |
| 313 | 3 | 0.5331 | 0.1344 |
| 293 | 6 | 0.5851 | 0.1048 |
| 313 | 6 | 0.6012 | 0.1048 |
| α/° | Ma | Transition Onset Location | |
|---|---|---|---|
| Suction Side | Pressure Side | ||
| −6 | 0.4 | 0.1447 | 0.6391 |
| −3 | 0.4 | 0.4409 | 0.6169 |
| 0 | 0.4 | 0.5180 | 0.5694 |
| 3 | 0.4 | 0.6374 | 0.1344 |
| 6 | 0.4 | 0.6815 | 0.0969 |
| −6 | 0.6 | 0.1447 | 0.5869 |
| −3 | 0.6 | 0.1519 | 0.5697 |
| 0 | 0.6 | 0.3892 | 0.4819 |
| 3 | 0.6 | 0.5732 | 0.1344 |
| 6 | 0.6 | 0.6092 | 0.1048 |
| −6 | 0.7 | 0.1523 | 0.5869 |
| −3 | 0.7 | 0.1278 | 0.5539 |
| 0 | 0.7 | 0.3086 | 0.4023 |
| 3 | 0.7 | 0.5009 | 0.1344 |
| 6 | 0.7 | 0.5851 | 0.1048 |
| −6 | 0.75 | 0.1904 | 0.7436 |
| −3 | 0.75 | 0.1519 | 0.5303 |
| 0 | 0.75 | 0.3166 | 0.4262 |
| 3 | 0.75 | 0.5572 | 0.1344 |
| 6 | 0.75 | 0.5851 | 0.1048 |
| α/° | Ma | Transition Onset Location | |
|---|---|---|---|
| Suction Side | Pressure Side | ||
| −6 | 0.4 | 0.1447 | 0.6391 |
| −6 | 0.6 | 0.1447 | 0.5869 |
| −6 | 0.7 | 0.1523 | 0.5869 |
| −6 | 0.75 | 0.1904 | 0.7436 |
| −3 | 0.4 | 0.4409 | 0.6169 |
| −3 | 0.6 | 0.1519 | 0.5697 |
| −3 | 0.7 | 0.1278 | 0.5539 |
| −3 | 0.75 | 0.1519 | 0.5303 |
| 0 | 0.4 | 0.5180 | 0.5694 |
| 0 | 0.6 | 0.3892 | 0.4819 |
| 0 | 0.7 | 0.3086 | 0.4023 |
| 0 | 0.75 | 0.3166 | 0.4262 |
| 3 | 0.4 | 0.6374 | 0.1344 |
| 3 | 0.6 | 0.5732 | 0.1344 |
| 3 | 0.7 | 0.5009 | 0.1344 |
| 3 | 0.75 | 0.5572 | 0.1344 |
| 6 | 0.4 | 0.6815 | 0.0969 |
| 6 | 0.6 | 0.6092 | 0.1048 |
| 6 | 0.7 | 0.5851 | 0.1048 |
| 6 | 0.75 | 0.5851 | 0.1048 |
References
- Fedorov, A. Transition and Stability of High-Speed Boundary Layers. Annu. Rev. Fluid Mech. 2011, 43, 79–95. [Google Scholar] [CrossRef]
- Jovanovic, M.R. From Bypass Transition to Flow Control and Data-Driven Turbulence Modeling: An Input–Output Viewpoint. Annu. Rev. Fluid Mech. 2021, 53, 311–345. [Google Scholar] [CrossRef]
- Chen, X.; Dong, S.; Tu, G.; Yuan, X.; Chen, J. Boundary layer transition and linear modal instabilities of hypersonic flow over a lifting body. J. Fluid Mech. 2022, 938, A8. [Google Scholar] [CrossRef]
- Halila, G.L.O.; Martins, J.R.A.; Fidkowski, K.J. Adjoint-based aerodynamic shape optimization including transition to turbulence effects. Aerosp. Sci. Technol. 2020, 107, 106243. [Google Scholar] [CrossRef]
- Halila, G.L.O.; Antunes, A.P.; da Silva, R.G.; Azevedo, J.L.F. Effects of boundary layer transition on the aerodynamic analysis of high-lift systems. Aerosp. Sci. Technol. 2019, 90, 233–245. [Google Scholar] [CrossRef]
- Menter, F.R. Two-Equation Eddy-Viscosity Turbulence Models for Engineering Applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Langtry, R.B.; Menter, F.R. Correlation-Based Transition Modeling for Unstructured Parallelized Computational Fluid Dynamics Codes. AIAA J. 2009, 47, 2894–2906. [Google Scholar] [CrossRef]
- Xiao, H.; Cinnella, P. Quantification of model uncertainty in RANS simulations: A review. Prog. Aerosp. Sci. 2019, 108, 1–31. [Google Scholar] [CrossRef]
- Argyropoulos, C.D.; Markatos, N.C. Recent advances on the numerical modelling of turbulent flows. Appl. Math. Model. 2015, 39, 693–732. [Google Scholar] [CrossRef]
- Dhawan, S.; Narasimha, R. Some properties of boundary layer during the transition from laminar to turbulent motion. J. Fluid Mech. 1958, 3, 418–435. [Google Scholar] [CrossRef]
- Cho, J.R.; Chung, M.K. A k-ε-γ equation turbulence model. J. Fluid Mech. 1992, 237, 301–322. [Google Scholar] [CrossRef]
- Steelant, J.; Dick, E. Modeling of laminar-turbulent transition for high freestream turbulence. ASME J. Fluids Eng. 2001, 123, 22–30. [Google Scholar] [CrossRef]
- Bradshaw, P. Turbulence: The chief outstanding difficulty of our subjects. Exp. Fluids 1994, 16, 203–216. [Google Scholar] [CrossRef]
- Mayle, R.E.; Schula, A. The path to predicting bypass transition. ASME J. Turbomach. 1997, 119, 405–411. [Google Scholar] [CrossRef]
- Walters, D.K.; Cokljat, D. A three equation eddy viscosity model for Reynolds Averaged Navier Stokes simulations of transitional flow. ASME J. Fluids Eng. 2008, 130, 121401. [Google Scholar] [CrossRef]
- Mayle, R.E. The role of laminar-turbulent transition in gas turbine engines. ASME J. Turbomach. 1991, 113, 509–537. [Google Scholar] [CrossRef]
- Abu-Ghannam, B.J.; Shaw, R. Natural transition pf boundary layers—The effects of turbulence, pressure gradient, and flow history. J. Mech. Eng. Sci. 1980, 22, 213–228. [Google Scholar] [CrossRef]
- Menter, F.R.; Langtry, R.B.; Volker, S. Transition modelling for general purpose CFD codes. Flow Turbul. Combust. 2006, 77, 277–303. [Google Scholar] [CrossRef]
- Wang, L.; Fu, S. Modeling flow transition in a hypersonic boundary layer with Reynolds-averaged Navier-Stokes approach. Sci. China Ser. G Phys. Mech. Astron. 2009, 52, 768–774. [Google Scholar] [CrossRef]
- Fu, S.; Wang, L. RANS modeling of high-speed aerodynamic flow transition with consideration of stability theory. Prog. Aerosp. Sci. 2013, 58, 36–59. [Google Scholar] [CrossRef]
- Papp, J.L.; Dash, S.M. Rapid engineering approach to modeling hypersonic laminar to turbulent transitional flows. J. Spacecr. Rocket. 2005, 42, 467–475. [Google Scholar] [CrossRef]
- Robitaille, M.; Mosahebi, A.; Laurendeau, E. Design of adaptive transonic laminar airfoils using the γ-Reθt transition model. Aerosp. Sci. Technol. 2015, 46, 60–71. [Google Scholar] [CrossRef]
- Reeh, A.D.; Tropea, C. Behaviour of a natural laminar flow aerofoil in fight through atmospheric turbulence. J. Fluid Mech. 2015, 767, 394–429. [Google Scholar] [CrossRef]
- Malik, M.; Liao, W.; Li, F.; Choudhari, M. Discrete-Roughness-Element-Enhanced Swept-Wing Natural Laminar Flow at High Reynolds Numbers. AIAA J. 2015, 53, 2321–2334. [Google Scholar] [CrossRef]
- Rashad, R.; Zingg, D.W. Aerodynamic Shape Optimization for Natural Laminar Flow Using a Discrete-Adjoint Approach. AIAA J. 2016, 54, 3321–3337. [Google Scholar] [CrossRef]
- Shi, Y.; Mader, C.A.; He, S.; Haila, G.L.O.; Martins, J.R.R.A. Natural Laminar-Flow Airfoil Optimization Design Using a Discrete Adjoint Approach. AIAA J. 2020, 58, 4702–4722. [Google Scholar] [CrossRef]
- Xu, J.; Bai, J.; Qiao, L.; Zhang, Y.; Fu, Z. Fully Local Formulation of a Transition Closure Model for Transitional Flow Simulations. AIAA J. 2016, 54, 3015–3023. [Google Scholar] [CrossRef]
- Xu, J.; Fu, Z.; Bai, J.; Zhang, Y.; Duan, Z.; Zhang, Y. Study of boundary layer transition on supercritical natural laminar flow wing at high Reynolds number through wind tunnel experiment. Aerosp. Sci. Technol. 2018, 80, 221–231. [Google Scholar] [CrossRef]
- Li, J.; Gao, Z.; Huang, J.; Zhao, K. Robust design of NLF airfoils. Chin. J. Aeronaut. 2013, 26, 309–318. [Google Scholar] [CrossRef]
- Zhao, K.; Gao, Z.H.; Huang, J. Robust design of natural laminar flow supercritical airfoil by multi-objective evolution method. Appl. Math. Mech. 2014, 35, 191–202. [Google Scholar] [CrossRef]
- Zhang, Y.; Fang, X.; Chen, H.; Fu, S.; Duan, Z.; Zhang, Y. Supercritical natural laminar flow airfoil optimization for regional aircraft wing design. Aerosp. Sci. Technol. 2015, 43, 152–164. [Google Scholar] [CrossRef]
- Qin, N.; Wong, W.S.; Moigne, A.L. Three-dimensional contour bumps for transonic wing drag reduction. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2008, 222, 619–629. [Google Scholar] [CrossRef]
- Huang, H.; Xiong, J.; Liu, X.; Zhu, M.; Li, Y. Study of the boundary layer transition detection technique based on TSP. J. Exp. Fluid Mech. 2019, 33, 79–84. [Google Scholar]
- Somers, D.M. Design and Experimental Results for a Natural-Laminar-Flow Airfoil for General Aviation Applications; NASA Technical Paper 1861; NASA: Washington, DC, USA, 1981. [Google Scholar]
- Sammak, S.; Mojgani, R.; Boroomand, M. RANS Investigation of the Transitional Flow Over Stepped NLF(1)-0416. In Proceedings of the ASME 2012 International Mechanical Engineering Congress & Exposition, Houston, TX, USA, 9–15 November 2012. [Google Scholar]











| No. | Mesh Quantity | Streamwise Grids | Lift Coefficient | Drag Coefficient |
|---|---|---|---|---|
| 1 | 2.39 × 106 | 282 | 0.2672 | 0.0053 |
| 2 | 3.07 × 106 | 376 | 0.2781 | 0.0051 |
| 3 | 3.75 × 106 | 470 | 0.2797 | 0.0051 |
| 4 | 4.43 × 106 | 564 | 0.2800 | 0.0050 |
| Turbulent Intensity ε/% | Surface Temperature Tw/K | Angle of Attack α/° | Mach Number Ma |
|---|---|---|---|
| 0.1, 0.5, 1 | 293, 313 | −6, −3, 0, 3, 6 | 0.4, 0.6, 0.7, 0.75 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, H.; Tan, L.; Liu, M.; Liu, X.; Zhu, B. Numerical Investigation on the Transition Flow around NLF Airfoil. Energies 2023, 16, 1826. https://doi.org/10.3390/en16041826
Wang H, Tan L, Liu M, Liu X, Zhu B. Numerical Investigation on the Transition Flow around NLF Airfoil. Energies. 2023; 16(4):1826. https://doi.org/10.3390/en16041826
Chicago/Turabian StyleWang, Hongbiao, Lei Tan, Ming Liu, Xiang Liu, and Baoshan Zhu. 2023. "Numerical Investigation on the Transition Flow around NLF Airfoil" Energies 16, no. 4: 1826. https://doi.org/10.3390/en16041826
APA StyleWang, H., Tan, L., Liu, M., Liu, X., & Zhu, B. (2023). Numerical Investigation on the Transition Flow around NLF Airfoil. Energies, 16(4), 1826. https://doi.org/10.3390/en16041826







