Analytical Approach to Current Rating of Three-Phase Power Cable with Round Conductors
Abstract
:1. Introduction
2. Current Rating of the Power Cable
2.1. General Methodology
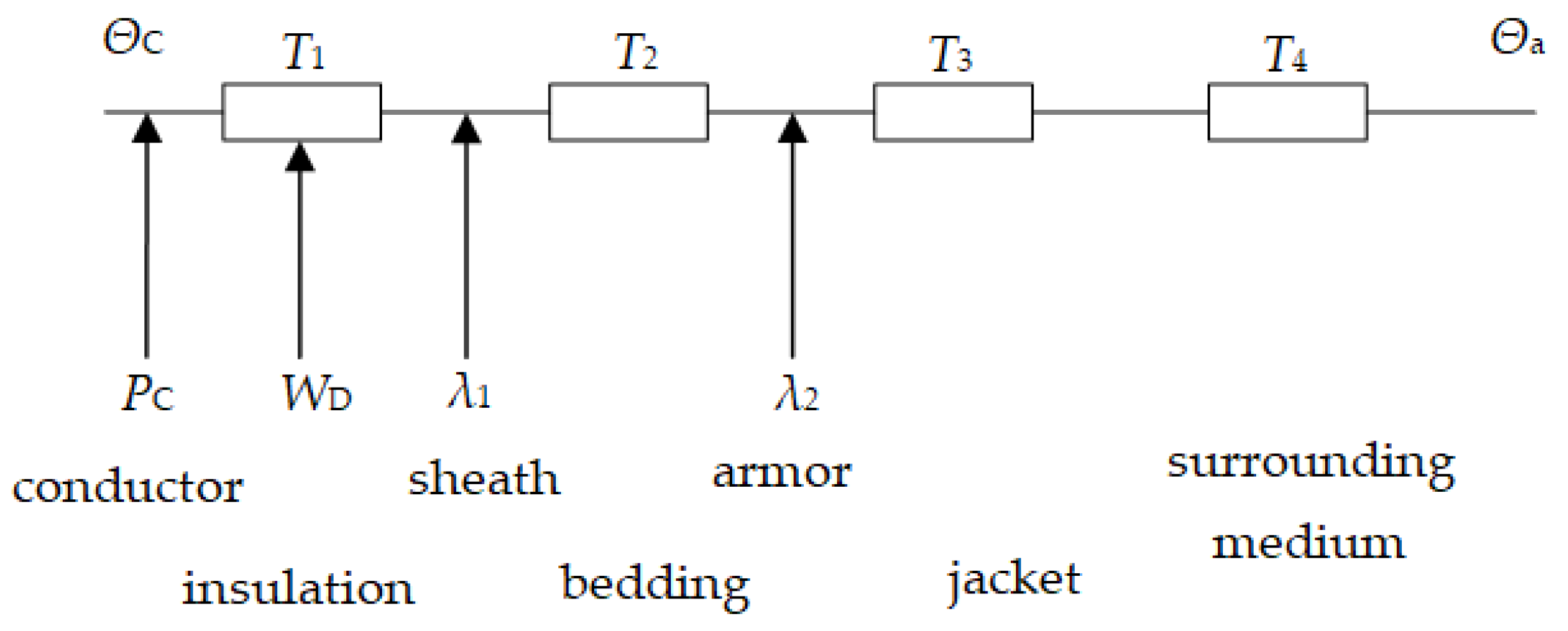
- ΔΘ is the temperature rise of the conductor above the ambient temperature [K],
- R is the conductor resistance determined for the maximum operating temperature [Ω/m],
- WD refers to the dielectric losses per length unit [W/m],
- T1 is the thermal resistance between the conductor and the screen [Km/W],
- T2 is the thermal resistance of the bedding between the screen and the armor [Km/W],
- T3 is the thermal resistance of the external jacket [Km/W],
- T4 is the thermal resistance of the surrounding medium [Km/W],
- λ1 is the screen losses factor (ratio of the total losses in the screens to the total conductor losses or ratio of losses in one sheath to the losses in one conductor),
- λ2 is the armor losses factor (ratio of the total losses in the armor to the total conductor losses, or ratio of losses in one armor to the losses in one conductor),
- n is the number of conductors in a cable.
- Establishing of the cable type and its geometrical and physical parameters.
- Determining the physical conditions in which the cable is laid.
- Assuming the acceptable increase in temperature (ΔΘ).
- Evaluating the thermal resistances of the individual insulation layers of the cable.
- Computing the resistance of the phase conductors.
- Determining the power ratios λ1 and λ2 using tables for specific placement of conductors, their material and excitation parameters.
- Evaluating the dielectric losses in a similar manner.
- Calculating the rated current of the cable.
2.2. Three-Phase Cable with Round Conductors
3. Exemplary Computations
4. Validation
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| C | capacitance of the insulation layer |
| Da | external diameter of the cable armor |
| De | external diameter of the cable |
| Ds | internal diameter of the armor bedding |
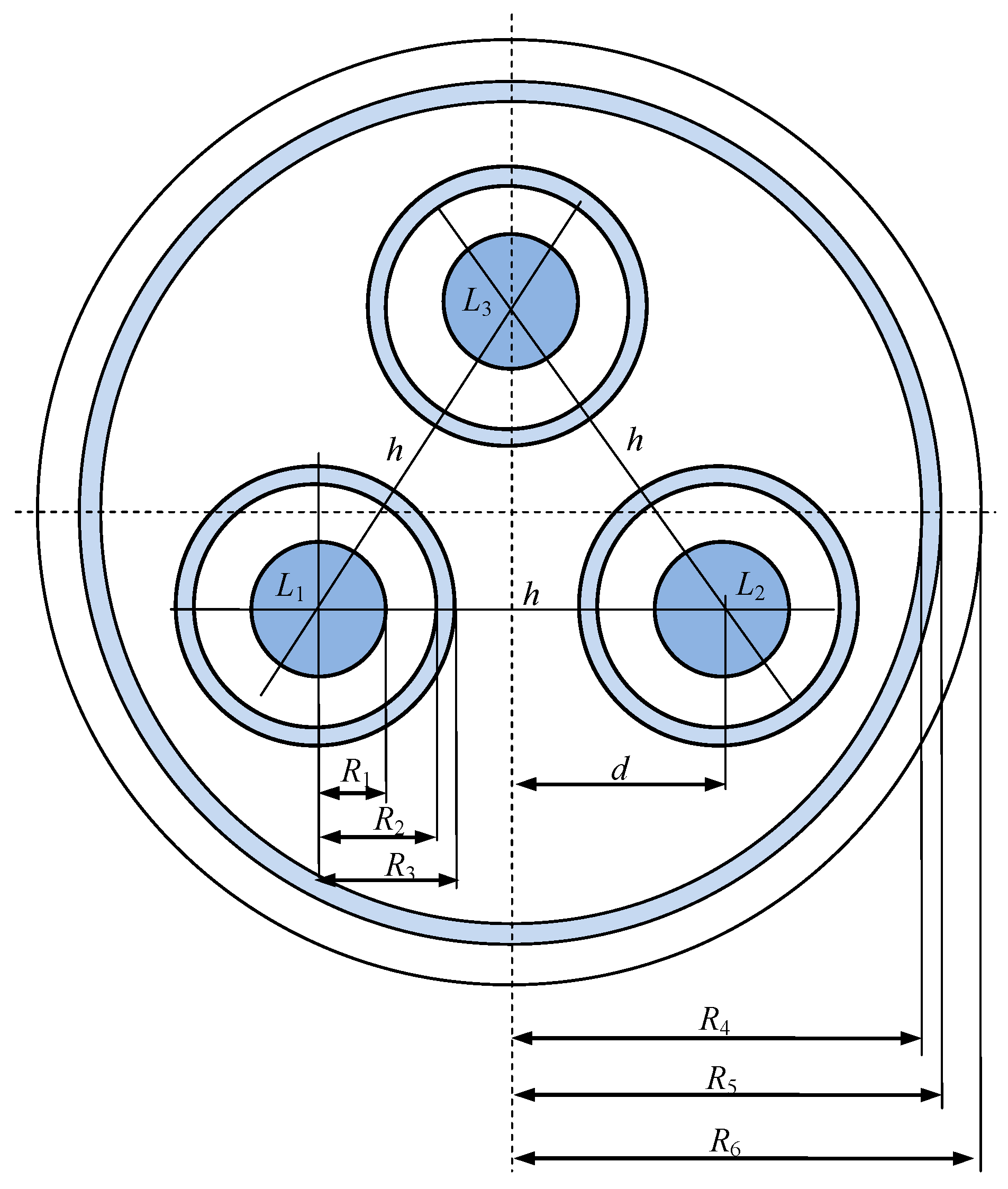
| d | distance between the conductor axes |
| dc | diameter of the conductor |
| f | frequency |
| G | geometrical coefficient |
| complex rms current | |
| modified Bessel function of the first kind and of n order | |
| complex rms current density vector | |
| j | imaginary unit |
| modified Bessel function of the second kind and of n order | |
| L | distance from the surface of the ground to the cable axis |
| l | length of the cable |
| n | number of conductors in the cable |
| PC | power losses in the phase conductors |
| r, θ, z | cylindrical coordinates |
| Wd | dielectric losses |
| Θa | ambient temperature |
| ΘC | conductor temperature |
| ΔΘ | temperature rise of the conductor above the ambient temperature |
| R | conductor resistance |
| R1 | radius of the phase conductor |
| R2 | internal radius of the screen |
| R3 | external radius of the screen |
| R4 | internal radius of the armor |
| R5 | external radius of the armor |
| R6 | radius of the cable |
| T1 | thermal resistance between conductor and screen |
| T2 | thermal resistance of bedding between screen and armor |
| T3 | thermal resistance of external jacket |
| T4 | thermal resistance of surrounding medium |
| t1 | material thickness between the conductor and the screen |
| t2 | thickness of the armor bedding |
| t3 | thickness of outer jacket |
| U | operating voltage |
| α | temperature coefficient for resistivity |
| ε | electrical permittivity |
| electrical conductivity of the conductor | |
| electrical conductivity of the armor | |
| electrical conductivity of the screen | |
| complex propagation constant | |
| λ1 | screen losses factor |
| λ2 | armor losses factor |
| ρins | thermal resistance of the insulation |
| ρf | thermal resistance of the filling material |
| ρsoil | thermal resistivity of the soil |
| μo | magnetic permeability of the vacuum |
| ω | angular frequency of current and voltage |
References
- Benato, R.; Colla, L.; Dambone Sessa, S.; Marelli, M. Review of high current rating insulated cable solutions. Electr. Power Syst. Res. 2016, 133, 36–41. [Google Scholar] [CrossRef]
- Cigre 640. A Guide for Rating Calculations of Insulated Cables. In Proceedings of the 9th International Conference on Insulated Power Cables, Versailles, France, 21–25 June 2015. [Google Scholar]
- Chengke, Z.; Huajie, Y.; Xiang, D. Review of recent research towards power cable life cycle management. High Volt. 2017, 2, 179–187. [Google Scholar]
- Holyk, C.; Anders, G.J. Power cable rating calculations—A historical perspective. IEEE Ind. Appl. Mag. 2015, 21, 6–64. [Google Scholar] [CrossRef]
- Worzyk, T. Submarine Power Cables; Springer: Berlin, Germany, 2009. [Google Scholar]
- Park, K.; Kwon, I.; Lee, B. Calculation Method of Allowable Continuous Current for Direct Burial Laying HVDC Underground Cable. Energies 2021, 14, 6431. [Google Scholar] [CrossRef]
- Rachek, M.; Larbi, S.N. Magnetic eddy-current and thermal coupled models for the finite-element behavior analysis of underground power cables. IEEE Trans. Magn. 2008, 44, 4739–4746. [Google Scholar] [CrossRef]
- Del Pino, J.C.; Cruz, P. Magnetic field shielding of underground cable duct banks. Prog. Electromagn. Res. 2013, 138, 1–19. [Google Scholar] [CrossRef]
- Mazzanti, G. Life estimation of HVDC cables under the time-varying electrothermal stress associated with load cycles. IEEE Trans. Power Deliv. 2015, 30, 931–939. [Google Scholar] [CrossRef]
- Sedaghat, A.; De Leon, F. Thermal Analysis of Power Cables in Free Air: Evaluation and Improvement of the IEC Standard Ampacity Calculations. IEEE Trans. Power Deliv. 2014, 29, 2306–2314. [Google Scholar] [CrossRef]
- Bicen, Y. Trend adjusted lifetime monitoring of underground power cable. Electr. Power Syst. Res. 2017, 143, 189–196. [Google Scholar] [CrossRef]
- Shabani, H.; Vahidi, B. A probabilistic approach for optimal power cable ampacity computation by considering uncertainty of parameters and economic constraints. Electr. Power Energy Syst. 2019, 106, 432–443. [Google Scholar] [CrossRef]
- Zarchi, D.A.; Vahidi, B. Multi objective self adaptive optimization method to maximize ampacity and minimize cost of underground cables. J. Comput. Des. Eng. 2018, 5, 401–408. [Google Scholar] [CrossRef]
- Gouda, O.E.; Dein, A.Z.E.; Amer, G.M. Effect of the Formation of the Dry Zone around Underground Power Cables on Their Ratings. IEEE Trans. Power Deliv. 2011, 26, 972–978. [Google Scholar] [CrossRef]
- Zarchi, D.A.; Vahidi, B. Optimal placement of underground cables to maximise total ampacity considering cable lifetime. IET Gener. Transm. Distrib. 2016, 10, 263–269. [Google Scholar] [CrossRef]
- Jiang, X.; Corr, E.; Stephen, B.; Stewart, B.G. Impact of increased penetration of low carbon technologies on cable lifetime estimations. Electricity 2022, 3, 13. [Google Scholar] [CrossRef]
- De Leon, F. Major Factors Affecting Cable Ampacity. In Proceedings of the 2006 IEEE Power Engineering Society General Meeting, Montreal, QC, Canada, 18–22 June 2006. [Google Scholar]
- Brakelmann, H.; Anders, G.J. Current rating considerations in designing HVDC cable installations. IEEE Trans. Power Deliv. 2018, 33, 2315–2323. [Google Scholar] [CrossRef]
- International Electrotechnical Commission. Calculation of the Current Rating, Parts 1 and 2, IEC Standard 60287-1 and IEC Standard 60287-2; International Electrotechnical Commission: Geneva, Switzerland, 2006. [Google Scholar]
- De Leon, F.; Anders, G.J. Effects of Backfilling on Cable Ampacity Analyzed With the Finite Element Method. IEEE Trans. Power Deliv. 2008, 23, 537–543. [Google Scholar] [CrossRef]
- Kocar, I.; Ertas, A. Thermal analysis for determination of current carrying capacity of PE and XLPE insulated power cables using finite element method. In Proceedings of the 12th IEEE Mediterranean Electrotechnical Conference, Dubrovnik, Croatia, 12–15 May 2004. [Google Scholar]
- Benato, R.; Dambone Sessa, S. A New Multiconductor Cell Three-Dimension Matrix based Analysis Applied to a Three-Core Armored Cable. IEEE Trans. Power Deliv. 2017, 33, 1636–1646. [Google Scholar] [CrossRef]
- Chien, C.H.; Bucknall, R.W.G. Harmonic Calculations of Proximity Effect on Impedance Characteristics in Subsea Power Transmission Cables. IEEE Trans. Power Deliv. 2009, 24, 2150–2158. [Google Scholar] [CrossRef]
- Chatzipetros, D.; Pilgrim, J.A. Impact of Proximity Effects on Sheath Losses in Trefoil Cable Arrangements. IEEE Trans. Power Deliv. 2019, 35, 455–463. [Google Scholar] [CrossRef]
- Gudmundsdottir, U.S. Proximity effect in fast transient simulations of an underground transmission cable. Electr. Power Syst. Res. 2014, 111, 50–56. [Google Scholar] [CrossRef]
- Lin, Y.; Xu, Z. Cable Sheath Loss Reduction Strategy Research Based on Coupled Line Model. Trans. Power Deliv. 2015, 30, 2303–2311. [Google Scholar] [CrossRef]
- Del-Pino-Lopez, J.C.; Cruz-Romero, P. Use of 3D-FEM Tools to Improve Loss Allocation in Three-Core Armored Cables. Energies 2021, 14, 2434. [Google Scholar] [CrossRef]
- Del-Pino-López, J.C.; Cruz-Romero, P.; Sanchez-Díaz, L.C. Loss Allocation in Submarine Armored Three-core HVAC Power Cables. In Proceedings of the 2020 IEEE International Conference on Environment and Electrical Engineering and 2020 IEEE Industrial and Commercial Power Systems Europe (EEEIC/I and CPS Europe), Madrid, Spain, 9–12 June 2020. [Google Scholar]
- Kossyvakis, D.N.; Chrysochos, A.I.; Pavlou, K. Calculation of Losses in Three-Core Submarine Cables for Fractional Frequency Transmission Operation. In Proceedings of the 2018 IEEE International Conference on High Voltage Engineering and Application (ICHVE), Athens, Greece, 10–13 September 2018. [Google Scholar]
- Moutassem, W.; Anders, G.J. Calculation of the Eddy Current and Hysteresis Losses in Sheathed Cables Inside a Steel Pipe. Trans. Power Deliv. 2010, 35, 2054–2063. [Google Scholar] [CrossRef]
- Wang, Z.; Wang, W.; Li, Z.; Li, S. Thermoelectric Coupling Study of Three-core XLPE Submarine Cable by Finite Element Simulation. In Proceedings of the 2019 IEEE Conference on Electrical Insulation and Dielectric Phenomena (CEIDP), Richland, WA, USA, 20–23 October 2019. [Google Scholar]
- Garrido, C.; Otero, A.F.; Cidras, J. Theoretical model to calculate steady-state and transient ampacity and temperature in buried cables. IEEE Trans. Power Deliv. 2003, 18, 667–678. [Google Scholar] [CrossRef]
- Xu, X.B.; Liu, G. Investigation of the magnetic field produced by unbalanced phase current in an underground three-phase pipe type cable. Electr. Power Syst. Res. 2002, 62, 153–160. [Google Scholar] [CrossRef]
- Millar, R.J.; Lehtonen, M. A robust framework for cable rating and temperature monitoring. IEEE Trans. Power Deliv. 2006, 21, 313–321. [Google Scholar] [CrossRef]
- Demoulias, C.; Labridis, D.P.; Dokopoulos, P.S.; Gouramanis, K. Ampacity of Low Voltage Power Cables Under Non-sinusoidal Currents. IEEE Trans. Power Deliv. 2007, 22, 584–594. [Google Scholar] [CrossRef]
- Hughes, T.; Henstock, T.J.; Pilgrim, J.A.; Dix, J.K.; Gernon, T.M.; Thompson, C.E.L. Effect of Sediment Properties on the Thermal Performance of Submarine HV Cables. IEEE Trans. Power Deliv. 2015, 30, 2443–2450. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, X.; Zhang, H.; Liu, J.; Zhang, C.; Jiao, J. Analysis on the Temperature Field and the Ampacity of XLPE Submarine HV Cable Based on Electro-Thermal-Flow Multiphysics Coupling Simulation. Polymers 2020, 12, 952. [Google Scholar] [CrossRef]
- Yang, L.; Qiu, W.; Huang, J.; Hao, Y.; Fu, M.; Hou, S.; Li, L. Comparison of Conductor-Temperature Calculations Based on Different Radial-Position-Temperature 531 Detection for High-Voltage Power Cable. Energies 2018, 11, 117. [Google Scholar] [CrossRef]
- Terracciano, M.; Purushothaman, S.; Leon, F.D.; Farahani, A.V. Thermal analysis of cables in unfilled troughs: Investigation of the IEC standard and a methodical approach for cable rating. IEEE Trans. Power Deliv. 2012, 27, 1423–1431. [Google Scholar] [CrossRef]
- Benato, R.; Paolucci, A. Multiconductor cell analysis of skin effect in Milliken type cables. Electr. Power Syst. Res. 2012, 90, 99–106. [Google Scholar] [CrossRef]
- Pagnetti, A.; Xemard, A.; Paladian, F.; Nucci, C.A. An improved method for the calculation of the internal impedances of solid and hollow conductors with the inclusion of proximity effect. IEEE Trans. Power Deliv. 2012, 27, 2063–2072. [Google Scholar] [CrossRef]
- McLachlan, N.W. Bessel Functions for Engineering; PWN: Warsaw, Poland, 1964. [Google Scholar]
- Piątek, Z. Modeling of Lines, Cables and High Current Busducts; Wydawnictwo Politechniki Częstochowskiej: Czestochowa, Poland, 2007. (In Polish) [Google Scholar]
- Fernandez, E.; Patrick, J. Emergency and Cyclic Ratings of HV Cables. 2019. Available online: https://elek.com.au/wp-content/uploads/2019/02/Emergency-and-cyclic-ratings-of-HV-cables.pdf (accessed on 20 September 2022).


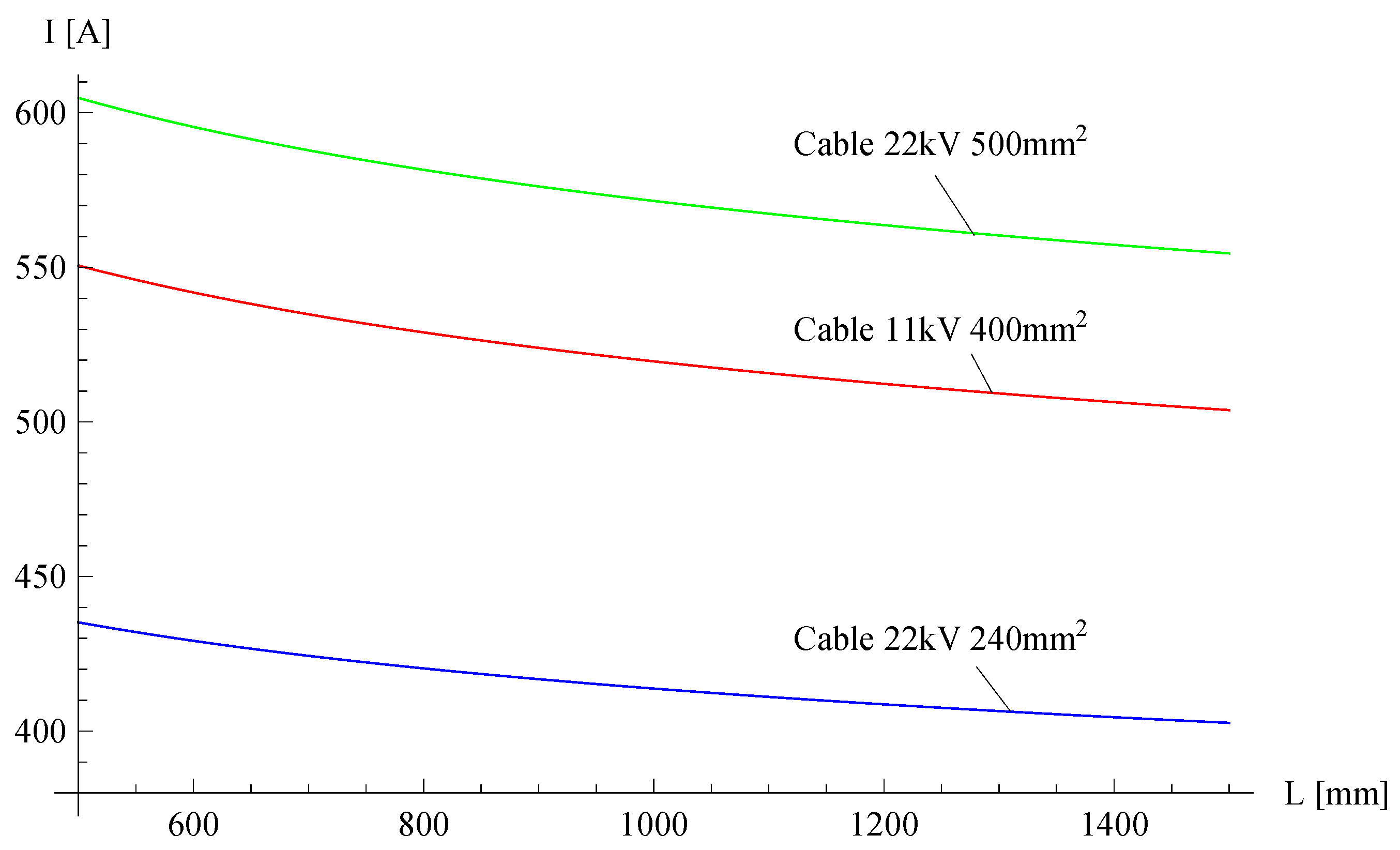
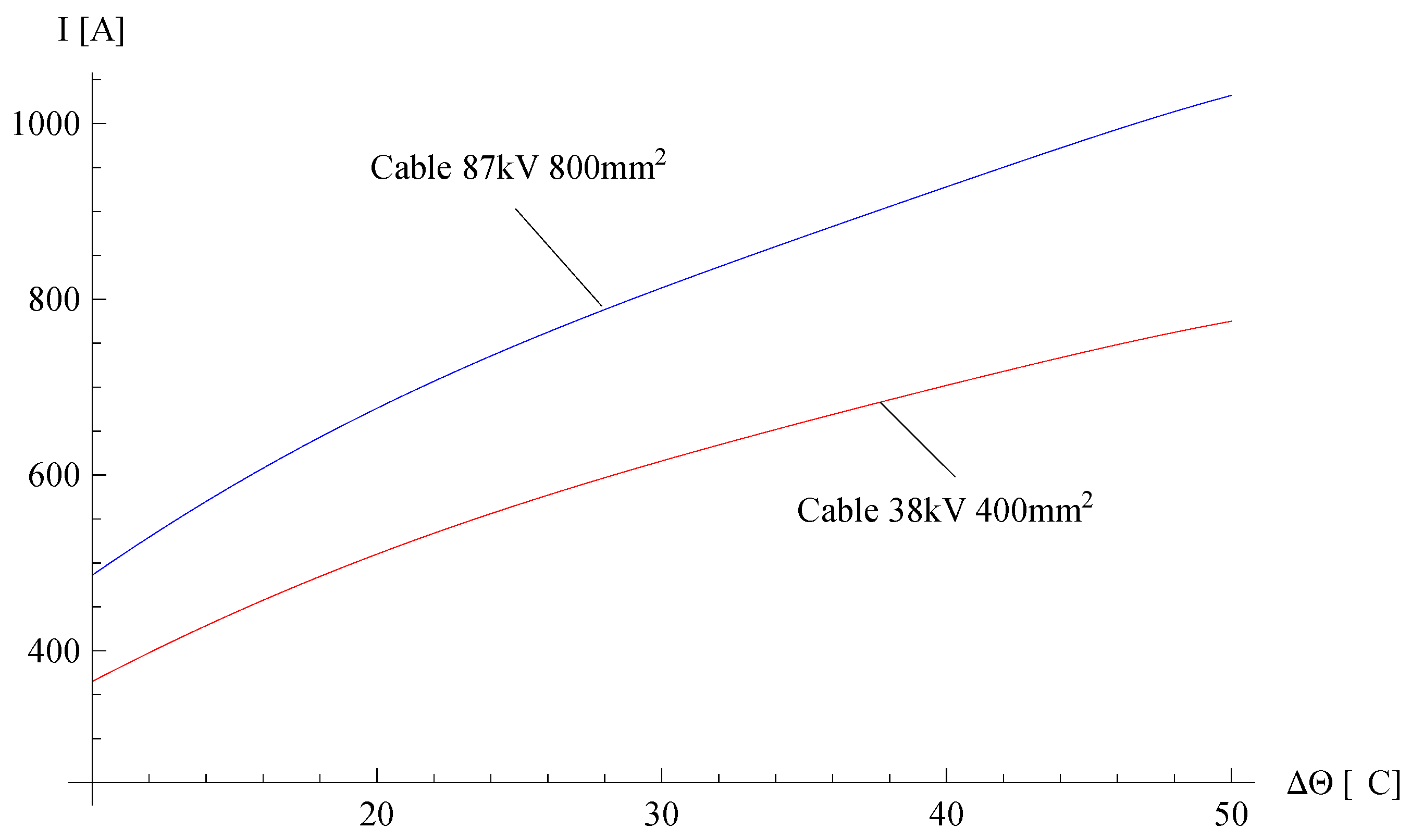
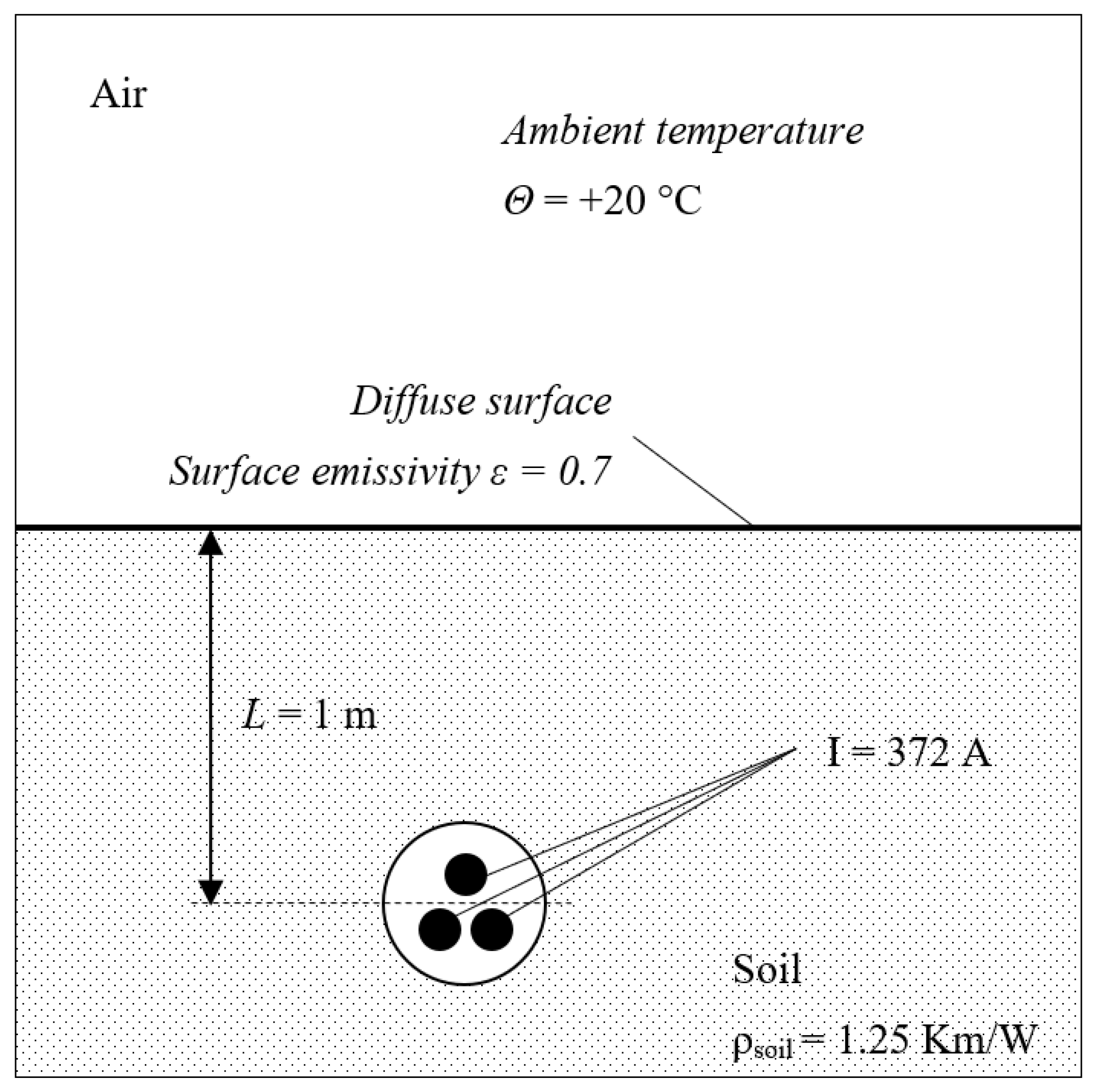

| Cable Parameters | Unit | Cable 11 kV 400 mm2 | Cable 22 kV 240 mm2 | Cable 22 kV 500 mm2 |
|---|---|---|---|---|
| Operating voltage | kV | 11 | 22 | 22 |
| Number of cores | - | 3 | 3 | 3 |
| Conductor cross-section area | mm2 | 400 | 240 | 500 |
| Conductor diameter | mm | 23.6 | 18.5 | 26.5 |
| Insulation diameter | mm | 35.3 | 34.1 | 42.5 |
| Insulation thickness | mm | 5.85 | 7.8 | 8 |
| Screen diameter | mm | - | - | - |
| Screen thickness | mm | - | - | - |
| Armor bedding diameter | mm | 81.2 | 78.5 | 97.1 |
| Armor bedding thickness | mm | 2.56 | 2.51 | 2.76 |
| Armor diameter | mm | 87.5 | 84.8 | 103.4 |
| Armor thickness | mm | 3.15 | 3.15 | 3.15 |
| Jacket diameter | mm | 95.5 | 92.6 | 112.5 |
| Jacket thickness | mm | 4 | 3.9 | 4.55 |
| Structure | Material | Thermal Resistivity (Km/W) |
|---|---|---|
| Conductor | Copper | - |
| Insulation | XLPE | 3.5 |
| Filler | PP | 5 |
| Armor bedding | PVC | 5 |
| Armor | Aluminum | - |
| Jacket | PVC | 5 |
| Cable Parameters | Unit | Cable 38 kV 400 mm2 | Cable 87 kV 800 mm2 |
|---|---|---|---|
| Operating voltage | kV | 38 | 87 |
| Number of cores | - | 3 | 3 |
| Conductor cross-section area | mm2 | 400 | 800 |
| Conductor diameter | mm | 23.6 | 34.8 |
| Insulation diameter | mm | 47.6 | 77.2 |
| Insulation thickness | mm | 12 | 21.2 |
| Screen diameter | mm | 48 | 82.2 |
| Screen thickness | mm | 0.2 | 2.5 |
| Armor bedding diameter | mm | 122.5 | 194 |
| Armor bedding thickness | mm | 2.5 | 2.5 |
| Armor diameter | mm | 130.5 | 206 |
| Armor thickness | mm | 4 | 6 |
| Jacket diameter | mm | 136.5 | 212 |
| Jacket thickness | mm | 3 | 3 |
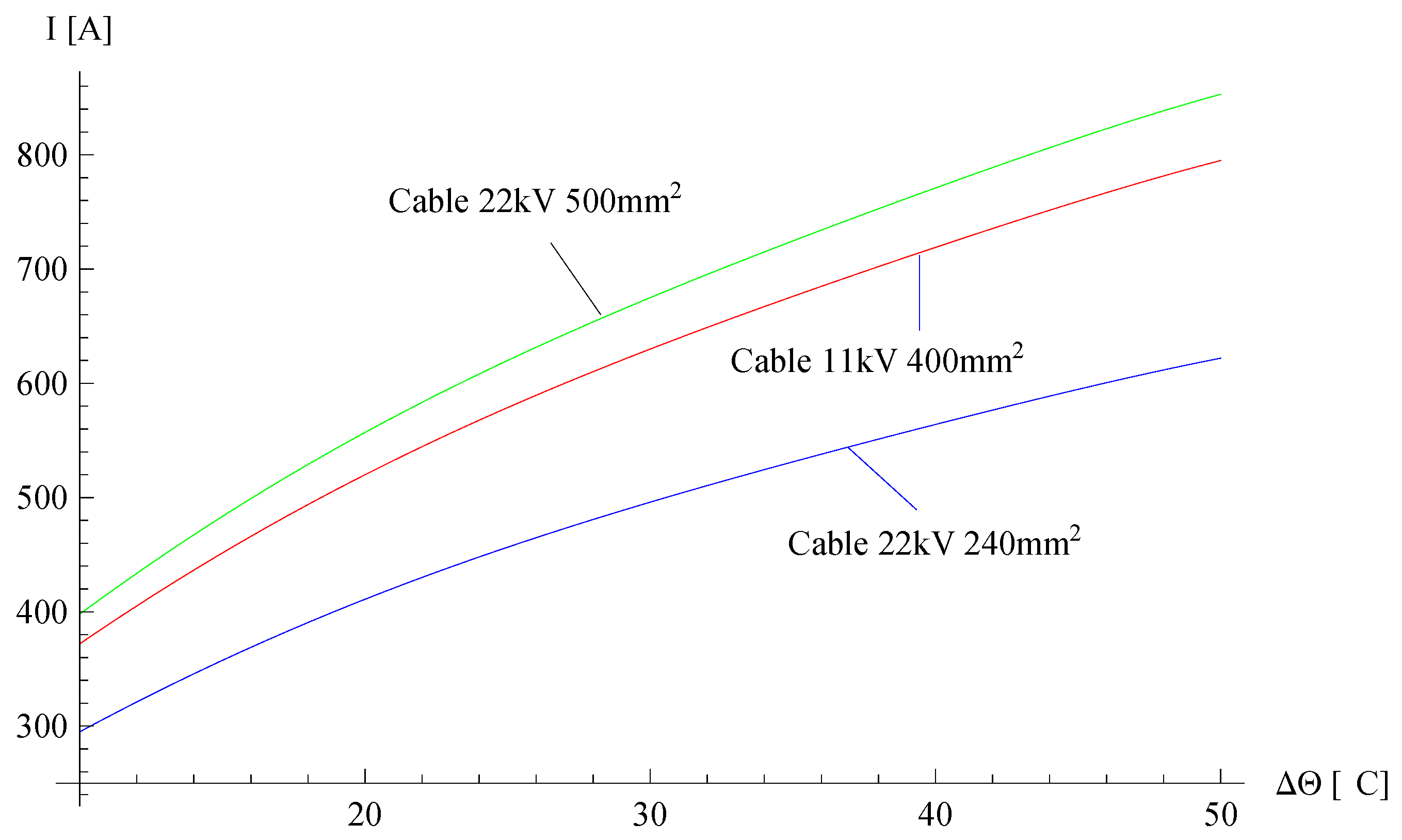
| Cable 11 kV 400 mm2 | ||||||
| I [A] | 372 | 520 | 630 | 719 | 795 | |
| ΔΘ [°C] | AM | 10 | 20 | 30 | 40 | 50 |
| FEM | 12 | 23 | 34 | 44 | 54 | |
| Cable 22 kV 240 mm2 | ||||||
| I [A] | 295 | 411 | 496 | 564 | 622 | |
| ΔΘ [°C] | AM | 10 | 20 | 30 | 40 | 50 |
| FEM | 11 | 22 | 32 | 42 | 51 | |
| Cable 22 kV 500 mm2 | ||||||
| I [A] | 398 | 557 | 675 | 771 | 853 | |
| ΔΘ [°C] | AM | 10 | 20 | 30 | 40 | 50 |
| FEM | 11 | 22 | 32 | 42 | 52 | |
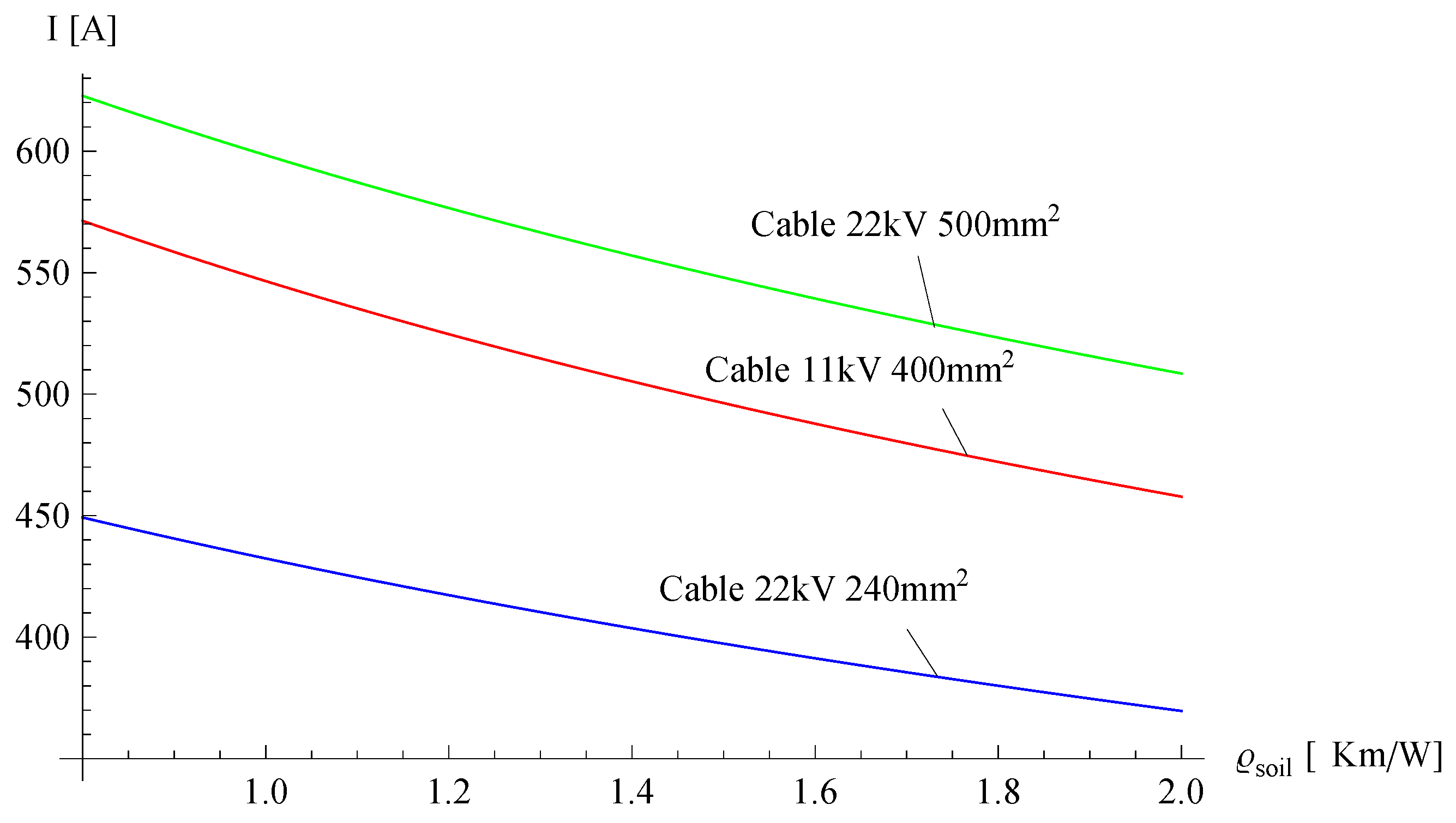
| Cable 11 kV 400 mm2 | ||||||
| ΔΘ [°C] | 10 | 20 | 30 | 40 | 50 | |
| I [A] | AM | 372 | 520 | 630 | 719 | 795 |
| IEC | 370 | 516 | 624 | 712 | 787 | |
| Cable 22 kV 240 mm2 | ||||||
| ΔΘ [°C] | 10 | 20 | 30 | 40 | 50 | |
| I [A] | AM | 295 | 411 | 496 | 564 | 622 |
| IEC | 294 | 409 | 493 | 560 | 617 | |
| Cable 22 kV 500 mm2 | ||||||
| ΔΘ [°C] | 10 | 20 | 30 | 40 | 50 | |
| I [A] | AM | 398 | 557 | 675 | 771 | 853 |
| IEC | 397 | 555 | 672 | 767 | 848 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Szczegielniak, T.; Jabłoński, P.; Kusiak, D. Analytical Approach to Current Rating of Three-Phase Power Cable with Round Conductors. Energies 2023, 16, 1821. https://doi.org/10.3390/en16041821
Szczegielniak T, Jabłoński P, Kusiak D. Analytical Approach to Current Rating of Three-Phase Power Cable with Round Conductors. Energies. 2023; 16(4):1821. https://doi.org/10.3390/en16041821
Chicago/Turabian StyleSzczegielniak, Tomasz, Paweł Jabłoński, and Dariusz Kusiak. 2023. "Analytical Approach to Current Rating of Three-Phase Power Cable with Round Conductors" Energies 16, no. 4: 1821. https://doi.org/10.3390/en16041821
APA StyleSzczegielniak, T., Jabłoński, P., & Kusiak, D. (2023). Analytical Approach to Current Rating of Three-Phase Power Cable with Round Conductors. Energies, 16(4), 1821. https://doi.org/10.3390/en16041821







