Enhancing Stability of Grid-Supporting Inverters from an Analytical Point of View with Lessons from Microgrids
Abstract
:1. Introduction
- Fundamental view based on small grid setup with accessible influence parameters:
- Further understanding of grid-supporting inverters:
- Simple derivation of control algorithms for grid operators:
2. Modeling in a Time Domain
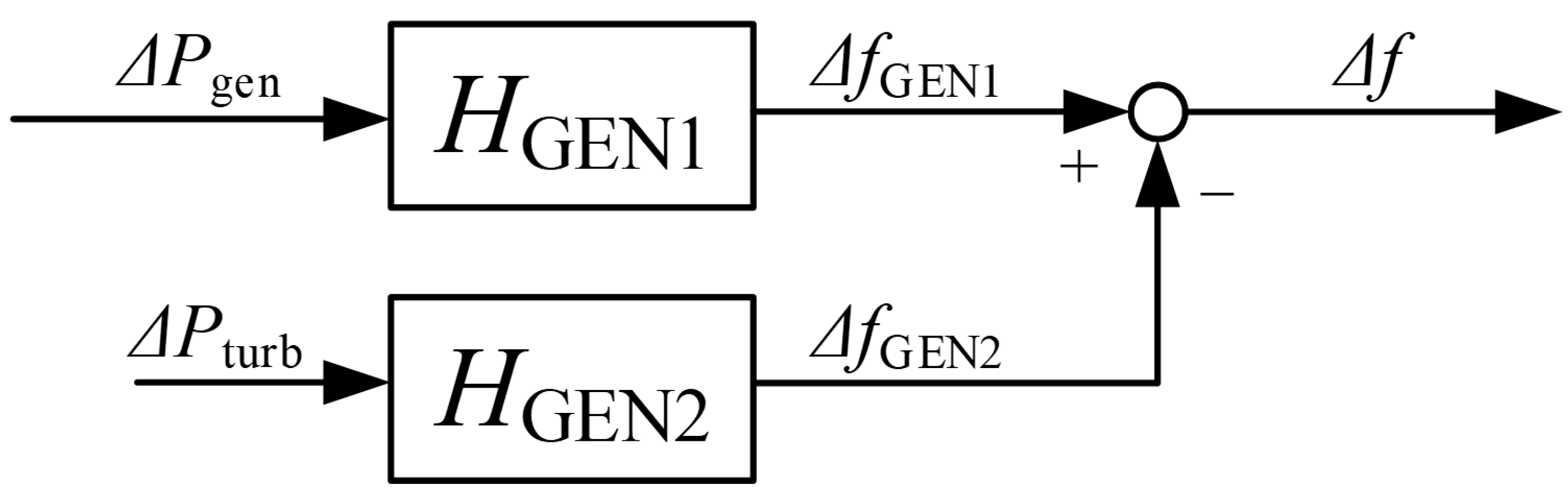
2.1. Modeling of Generator
2.2. Modeling of Grid-Supporting Inverter
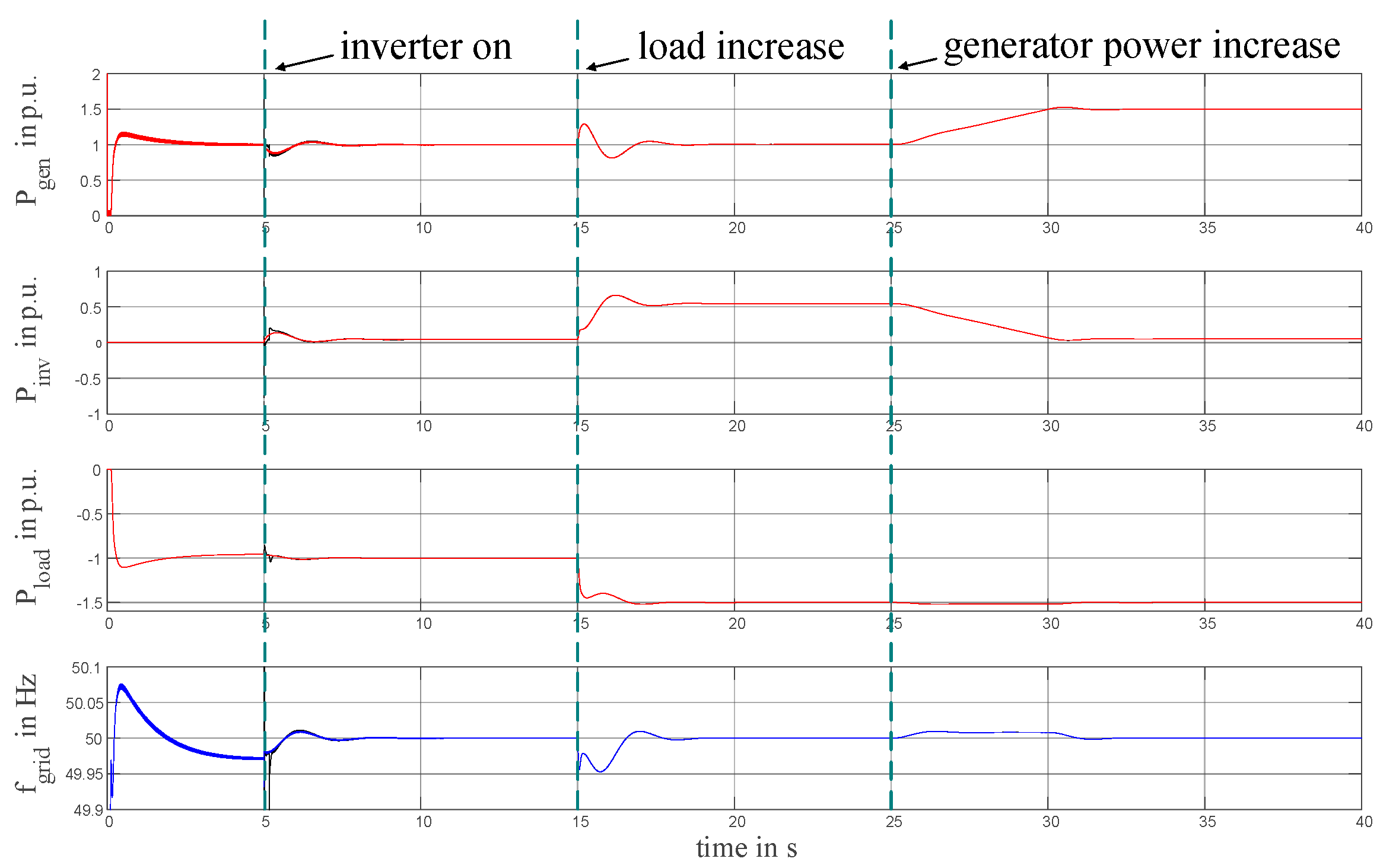
2.3. Dynamic Investigation of Time Domain Model
- 0 s: Initialization with balanced power values of the grid system, stable after 5 s
- 5 s: Inverter starts
- 15 s: Power increase at load by 0.5 MVA
- 25 s: Power increase at the generator by 0.5 MVA
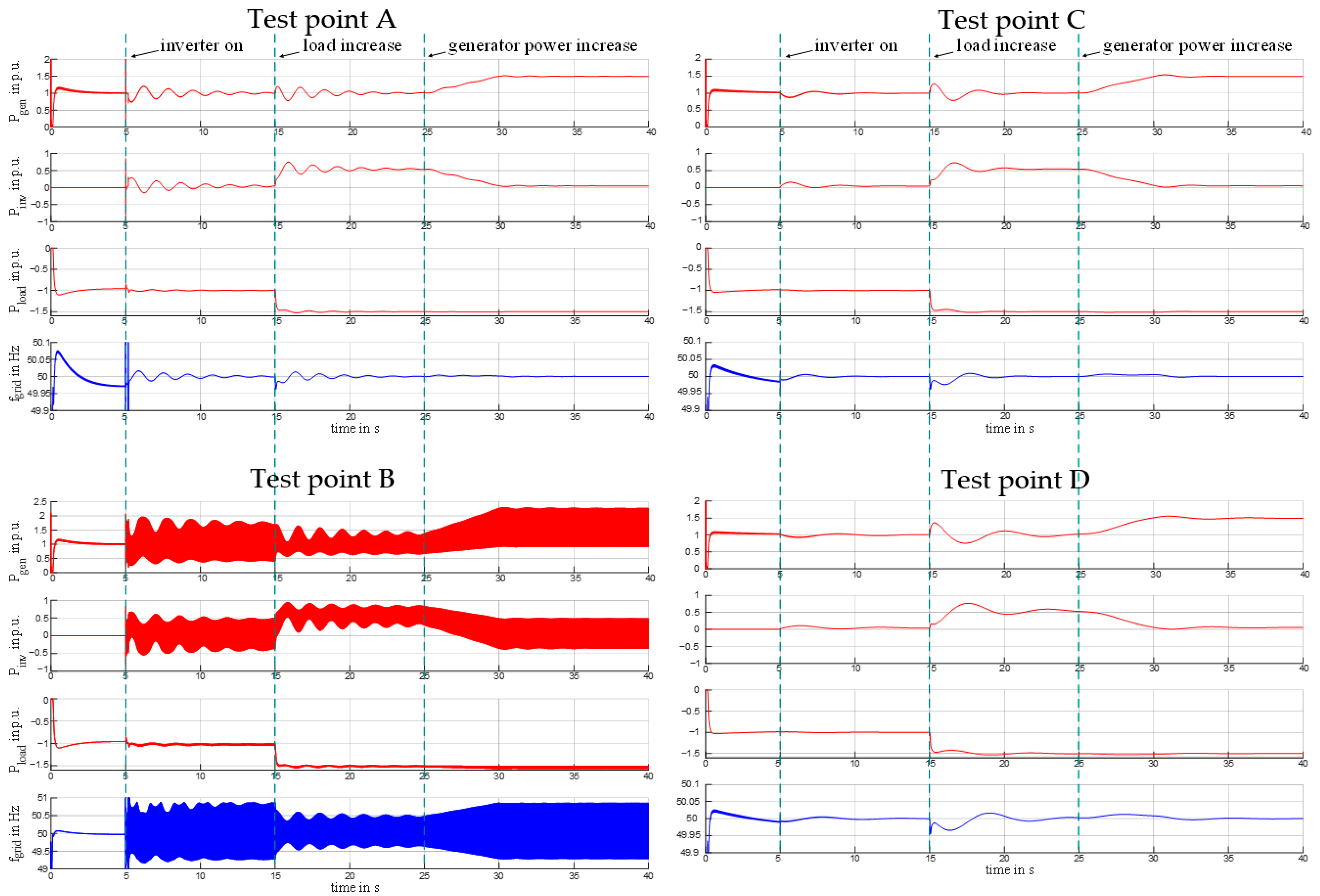
- 0 s: At the start of the simulation, the inverter is off, and the load and generator are initialized at 1 MVA. A small oscillation is observed at both the generator and the load. A positive sign indicates power input (here from the generator), and a negative sign indicates power consumption (here from the load).
- 5 s: The inverter is powered up, and there is a 2 s transient in the power and grid frequency curves. It can be noted that the frequency changes in the 100 mHz range.
- 15 s: At this point, the load increases its consumed power to 1.5 MW. Both the generator and the inverter respond to the power change, although the generator responds faster with a short power peak. This peak is derived from generator inertia, which actually reduces the rotational speed of the generator. Meanwhile, the inverter increases its active power, thus reducing the deficit in active power, and stabilizes the frequency. Since the frequency gradient becomes zero, the power peak of the generator originating from the inertia becomes zero as well. Due to the overshoot in inverter output power, the frequency rises up, and the generator active power counteracts with a reduction and thus drops below 1 MW. Finally, a steady state is reached with the inverter covering the extra load and the generator running with its original set point.Looking directly at the frequency, a maximum reduction of 50 mHz is visible. Due to the inverter’s PI control in the f/P loop, the frequency is not only stabilized but also restored to 50 Hz.
- 25 s: After the system has fully stabilized, the operating point of the generator is now increased to 1.5 MW. The inverter reacts immediately by reducing its output to almost zero. In terms of frequency, this power redistribution has caused a temporary grid frequency increase of 10 mHz.
3. Modeling in Small-Signal Domain
3.1. Modeling of Generator
3.2. Modeling of Grid-Supporting Inverter
3.3. Modeling of ZIP Load
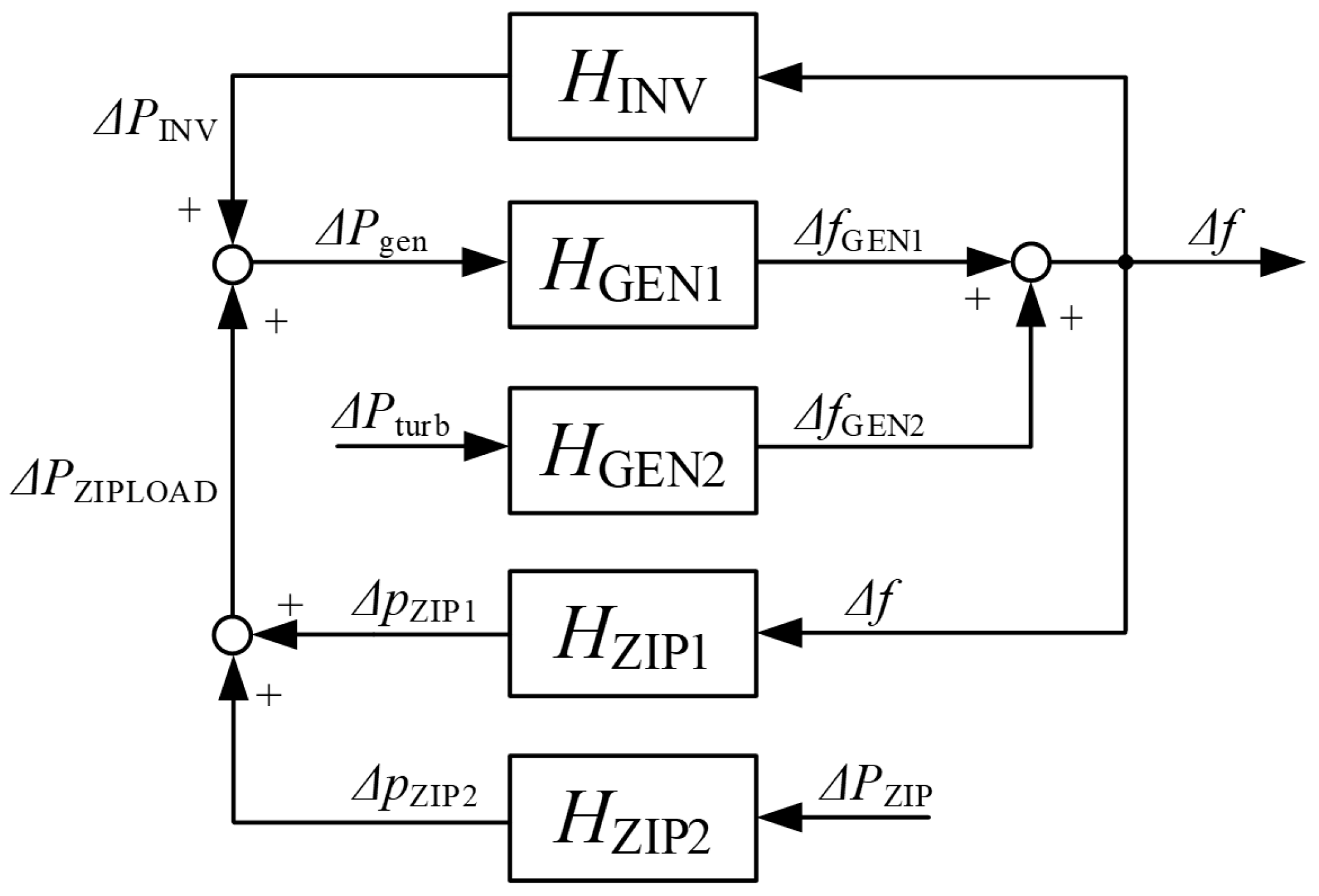
3.4. Overall Model in Small-Signal Domain
4. Investigation of the Analytical Model
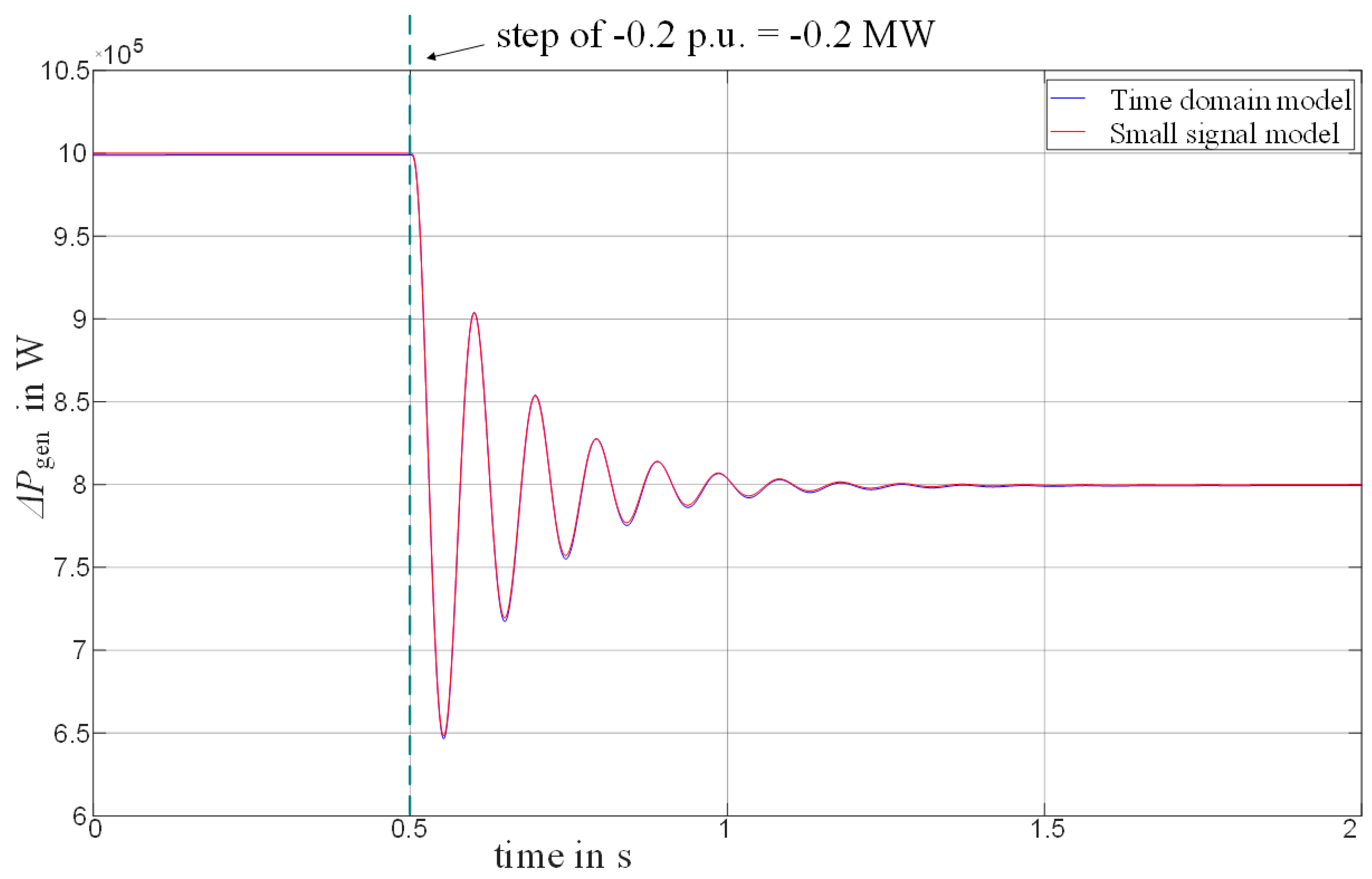
4.1. Validation of the Analytical Model
4.2. Stability Investigation
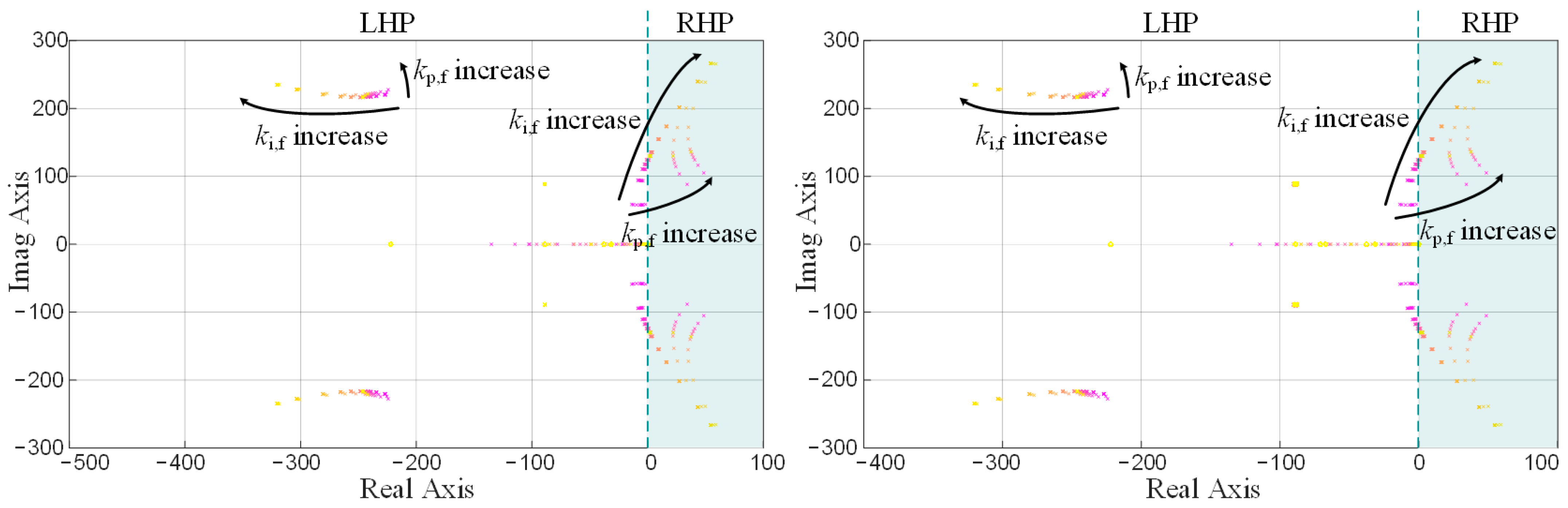
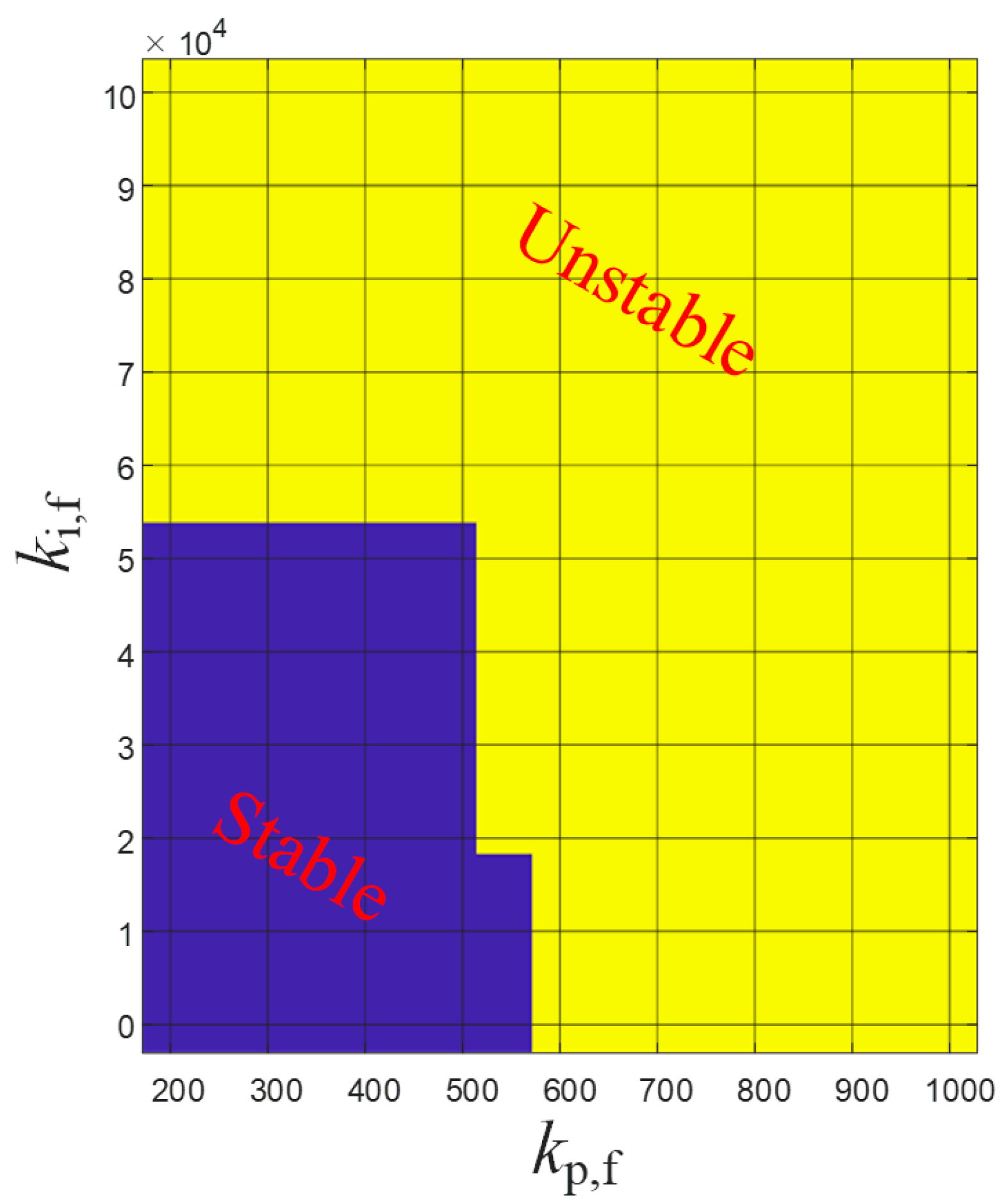
4.2.1. Stability Depending on the Variation of the PI Controller
- Poles with only real components (σ + j0):When located on the left half plane (LHP), these poles depict an exponentially decaying component in the time domain. Here, the location of the pole determines the rate of decay, with poles located further away from the origin, leading to faster decay rates. Therefore, poles far from the origin are components offering the transfer function quick stabilization to a settled value. If a real pole was to appear on the right half of the plane (RHP), it would correspond to an exponentially increasing component and render the system unstable.
- Poles with an imaginary component, which appear as complex conjugated poles (σ + j ω):Complex conjugated poles located in the LHP lead to a decaying sinusoidal component in the time domain. Again, the rate of decay is determined by the location of the real part of the pole σ, while the frequency of the sinusoidal oscillation is determined by ω. Vice versa, complex conjugate poles in the RHP result in an increasing sinusoidal component in the time domain, leading to an oscillation that continually increases, making the system unstable.
- Poles at the origin (0 + j0):These poles result in a constant that is determined by the initial values. Here, the set values are P0, Q0, Vref and fref of the inverter.
4.2.2. Stability Depending on the Variation of the Generator Inertia
- Poles with only real components (σ + j0)
- Poles with an imaginary component, which appear as complex conjugated poles (σ + j ω)
- Poles at the origin (0 + j0)
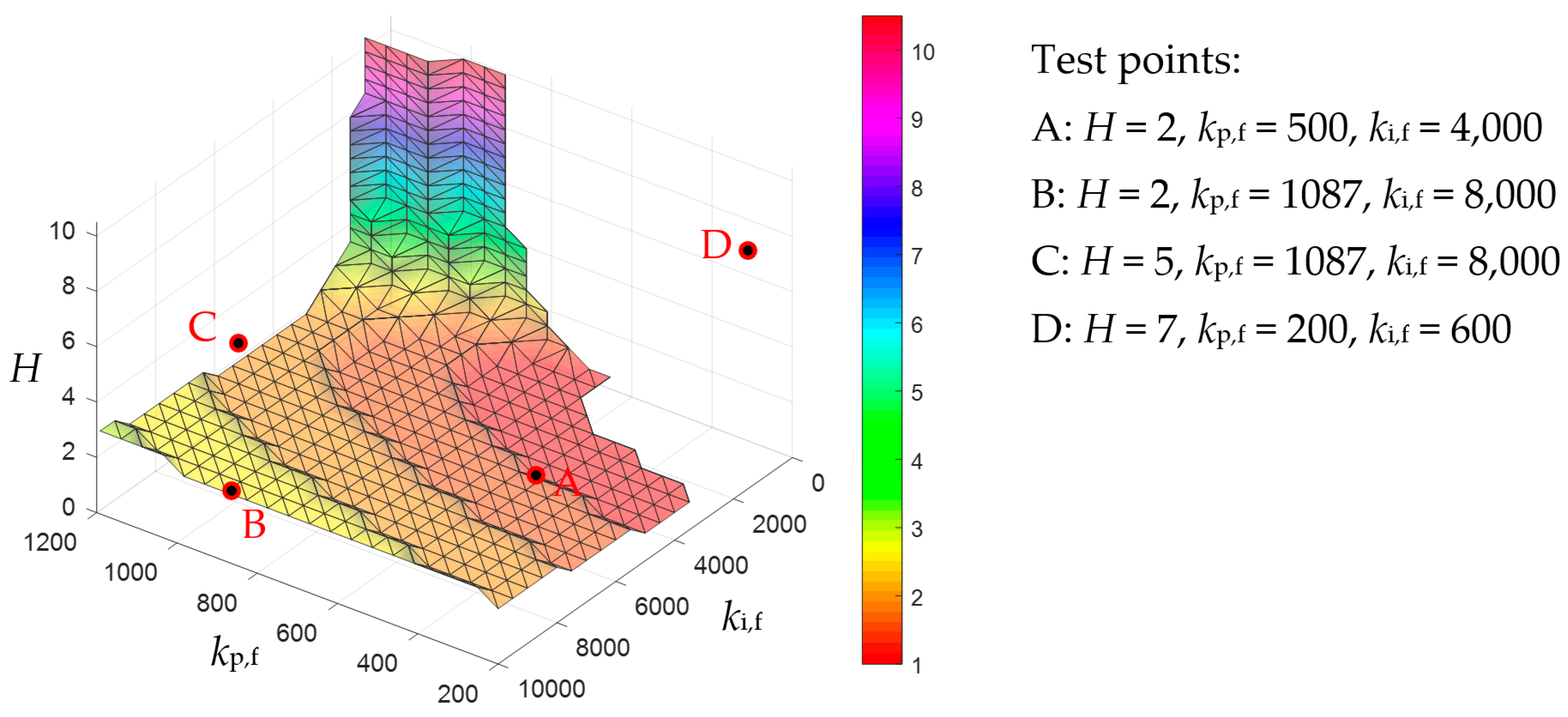
4.2.3. Visualization in a 3D Plot
4.2.4. Time Domain Validation
5. Discussion
- The assumption that both the grid and inverter could impact microgrid stability was validated. Changes in grid parameters and inverter control parameters were identified as potential destabilizing factors.
- Initially, a grid-supporting inverter was mathematically derived using small-signal modeling and the corresponding transfer function. The importance of applying the generalized Nyquist criterion to multi-input and multi-output systems was emphasized, revealing the significant role of pole type in subsequent behavior.
- Stability is notably influenced by the PLL, expressing poles with imaginary parts, typically as complex conjugated pole pairs. This study explores additional types of poles that positively affect the converter’s behavior based on their location on the LHP, following the generalized Nyquist criterion.
- The system’s stability is notably influenced by the value of kp,f, as indicated by the analytical study. Exceeding 518 in this grid system renders the system unstable, even with increased generator inertia. High ki,f values affect the oscillation behavior of the output signal, with simulations indicating 5000 as a limit, beyond which slow, decreasing oscillations occur. A significant discrepancy is observed when comparing these values with control values of rotating generators, being hundreds of times larger. This underscores the need for grid operators to expand their knowledge to effectively control a grid heavily influenced by inverters.
- The evaluation of rotating inertia showed that increasing it can convert unstable microgrid behavior into stability by shifting poles. A noteworthy finding is that an increase in inertia (0.5 to 1 p.u.) is often sufficient for stability, enabling active participation of the inverter in grid operation. However, when scaled to the entire European grid, this proportion becomes impractical, requiring a significant contribution from rotating generators at some point.
- Evaluation of grid impedance and visualization in a pole zero map
- Evaluation of inverter impedance and visualization in a pole zero map
- Comparison of inverter impedance poles with grid impedance poles. In case poles of the inverter system alone are already on the RHP, compensation mechanisms must be applied, or poles must be shifted with the control parameters. Should the combination of poles result in poles on the RHP, the inverter control parameters should be changed.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sahoo, A.K.; Mahmud, K.; Crittenden, M.; Ravishankar, J.; Padmanaban, S.; Blaabjerg, F. Communication-Less Primary and Secondary Control in Inverter-Interfaced AC Microgrid: An Overview. IEEE J. Emerg. Sel. Top. Power Electron. 2021, 9, 5164–5182. [Google Scholar] [CrossRef]
- Gui, Y.; Nainar, K.; Bendtsen, J.D.; Diewald, N.; Iov, F.; Yang, Y.; Blaabjerg, F.; Xue, Y.; Liu, J.; Hong, T.; et al. Voltage Support with PV Inverters in Low-Voltage Distribution Networks: An Overview. IEEE J. Emerg. Sel. Topics Power Electron. 2023, 1. [Google Scholar] [CrossRef]
- Rocabert, J.; Luna, A.; Blaabjerg, F.; Rodríguez, P. Control of Power Converters in AC Microgrids. IEEE Trans. Power Electron. 2012, 27, 4734–4749. [Google Scholar] [CrossRef]
- Zhang, Z.; Lehmal, C.; Hackl, P.; Schuerhuber, R. Transient Stability Analysis and Post-Fault Restart Strategy for Current-Limited Grid-Forming Converter. Energies 2022, 15, 3552. [Google Scholar] [CrossRef]
- Lin, Q.; Uno, H.; Ogawa, K.; Kanekiyo, Y.; Shijo, T.; Arai, J.; Matsuda, T.; Yamashita, D.; Otani, K. Field Demonstration of Parallel Operation of Virtual Synchronous Controlled Grid-Forming Inverters and a Diesel Synchronous Generator in a Microgrid. IEEE Access 2022, 10, 39095–39107. [Google Scholar] [CrossRef]
- Zhong, Q.-C.; Weiss, G. Synchronverters: Inverters that mimic synchronous generators. IEEE Trans. Ind. Electron. 2011, 58, 1259–1267. [Google Scholar] [CrossRef]
- Sandelic, M.; Peyghami, S.; Sangwongwanich, A.; Blaabjerg, F. Reliability aspects in microgrid design and planning: Status and power electronics-induced challenges. Renew. Sustain. Energy Rev. 2022, 159, 112127. [Google Scholar] [CrossRef]
- Zhang, Z.; Schuerhuber, R.; Fickert, L.; Friedl, K. Study of stability after low voltage ride-through caused by phase-locked loop of grid-side converter. Int. J. Electr. Power Energy Syst. 2021, 129, 106765. [Google Scholar] [CrossRef]
- Zanjani, M.G.M.; Mazlumi, K.; Kamwa, I. Combined analysis of distribution-level PMU data with transmission-level PMU for early detection of long-term voltage instability. IET Gener. Transm. Distrib. 2019, 13, 3634–3641. [Google Scholar] [CrossRef]
- Kundur, P.; Paserba, J.; Ajjarapu, V.; Andersson, G.; Bose, A.; Canizares, C.; Hatziargyriou, N.; Hill, D.; Stankovic, A.; Taylor, C.; et al. Definition and Classification of Power System Stability IEEE/CIGRE Joint Task Force on Stability Terms and Definitions. IEEE Trans. Power Syst. 2004, 19, 1387–1401. [Google Scholar] [CrossRef]
- da Cunha, G.L.; Fernandes, R.A.; Fernandes, T.C.C. Small-signal stability analysis in smart grids: An approach based on distributed decision trees. Electr. Power Syst. Res. 2022, 203, 107651. [Google Scholar] [CrossRef]
- Zhang, J.; Chung, C.Y.; Wang, Z.; Zheng, X. Instantaneous Electromechanical Dynamics Monitoring in Smart Transmission Grid. IEEE Trans. Ind. Inform. 2016, 12, 844–852. [Google Scholar] [CrossRef]
- Liu, C.; Sun, K.; Rather, Z.H.; Chen, Z.; Bak, C.L.; Thogersen, P.; Lund, P. A Systematic Approach for Dynamic Security Assessment and the Corresponding Preventive Control Scheme Based on Decision Trees. IEEE Trans. Power Syst. 2014, 29, 717–730. [Google Scholar] [CrossRef]
- Qiao, L.; Xue, Y.; Kong, L.; Wang, F. Nodal Admittance Matrix Based Area Partition Method for Small-Signal Stability Analysis of Large-Scale Power Electronics Based Power Systems. In Proceedings of the 2021 IEEE Applied Power Electronics Conference and Exposition (APEC), Phoenix, AZ, USA, 14–17 June 2021. [Google Scholar]
- Qiao, L.; Xue, Y.; Kong, L.; Wang, F. A Potential Issue of Using the MIMO Nyquist Criterion in Impedance-Based Stability Analysis. IEEE Open J. Power Electron. 2022, 3, 899–904. [Google Scholar] [CrossRef]
- Lehmal, C.; Zhang, Z.; Renner, H.; Schürhuber, R. Requirements for grid supporting inverter in relation with frequency and voltage support. In Proceedings of the CIRED 2023: 27th International Conference & Exhibition on Electricity Distribution, Rome, Italy, 12–15 June 2023; Available online: https://www.cired2023.org/ (accessed on 7 December 2023).
- Simpson-Porco, J.W.; Dörfler, F.; Bullo, F. Synchronization and power sharing for droop-controlled inverters in islanded microgrids. Automatica 2013, 49, 2603–2611. [Google Scholar] [CrossRef]
- Zhong, Q.-C. Robust Droop Controller for Accurate Proportional Load Sharing Among Inverters Operated in Parallel. IEEE Trans. Ind. Electron. 2013, 60, 1281–1290. [Google Scholar] [CrossRef]
- Yang, N.; Paire, D.; Gao, F.; Miraoui, A.; Liu, W. Compensation of droop control using common load condition in DC microgrids to improve voltage regulation and load sharing. Int. J. Electr. Power Energy Syst. 2015, 64, 752–760. [Google Scholar] [CrossRef]
- Guerrero, J.; GarciadeVicuna, L.; Matas, J.; Castilla, M.; Miret, J. A Wireless Controller to Enhance Dynamic Performance of Parallel Inverters in Distributed Generation Systems. IEEE Trans. Power Electron. 2004, 19, 1205–1213. [Google Scholar] [CrossRef]
- Alsafran, A. Literature Review of Power Sharing Control Strategies in Islanded AC Microgrids with Nonlinear Loads. In Proceedings of the 2018 IEEE PES Innovative Smart Grid Technologies Conference Europe (ISGT-Europe), Sarajevo, Bosnia and Herzegovina, 21–25 October 2018. [Google Scholar]
- Arbab-Zavar, B.; Palacios-Garcia, E.J.; Vasquez, J.C.; Guerrero, J.M. Smart Inverters for Microgrid Applications: A Review. Energies 2019, 12, 840. [Google Scholar] [CrossRef]
- Nutkani, I.U.; Loh, P.C.; Wang, P.; Blaabjerg, F. Autonomous Droop Scheme With Reduced Generation Cost. IEEE Trans. Ind. Electron. 2014, 61, 6803–6811. [Google Scholar] [CrossRef]
- Kundur, P. Power System Stability and Control; McGraw-Hill: New York, NY, USA, 1998. [Google Scholar]
- Ahmad, S.; Mekhilef, S.; Mokhlis, H. An improved power control strategy for grid-connected hybrid microgrid without park transformation and phase-locked loop system. Int. Trans. Electr. Energy Syst. 2021, 31, e12922. [Google Scholar] [CrossRef]
- Joseph, S.B.; Dada, E.G.; Abidemi, A.; Oyewola, D.O.; Khammas, B.M. Metaheuristic algorithms for PID controller parameters tuning: Review, approaches and open problems. Heliyon 2022, 8, e09399. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Harnefors, L.; Blaabjerg, F. Unified Impedance Model of Grid-Connected Voltage-Source Converters. IEEE Trans. Power Electron. 2018, 33, 1775–1787. [Google Scholar] [CrossRef]
- Gu, Y.; Zhu, R.; Qi, X.; Wu, W.; Aini, H.; Chang, X. Small-Signal Impedance-based Stability Analysis of Self-Synchronizing Grid-Following Converters. In Proceedings of the 2022 IEEE International Power Electronics and Application Conference and Exposition (PEAC), Guangzhou, China, 4–7 November 2022. [Google Scholar]
- Bequette, B.W. Process Control: Modeling Design and Simulation; Prentice-Hall of India Private Limited: New Delhi, India, 2006. [Google Scholar]
- Wen, B.; Boroyevich, D.; Mattavelli, P.; Shen, Z.; Burgos, R. Influence of phase-locked loop on dq frame impedance of three-phase voltage source converters and the impact on system stability. In Proceedings of the 2013 Twenty-Eighth Annual IEEE Applied Power Electronics Conference and Exposition (APEC), Long Beach, CA, USA, 17–21 March 2013; pp. 897–904. [Google Scholar] [CrossRef]
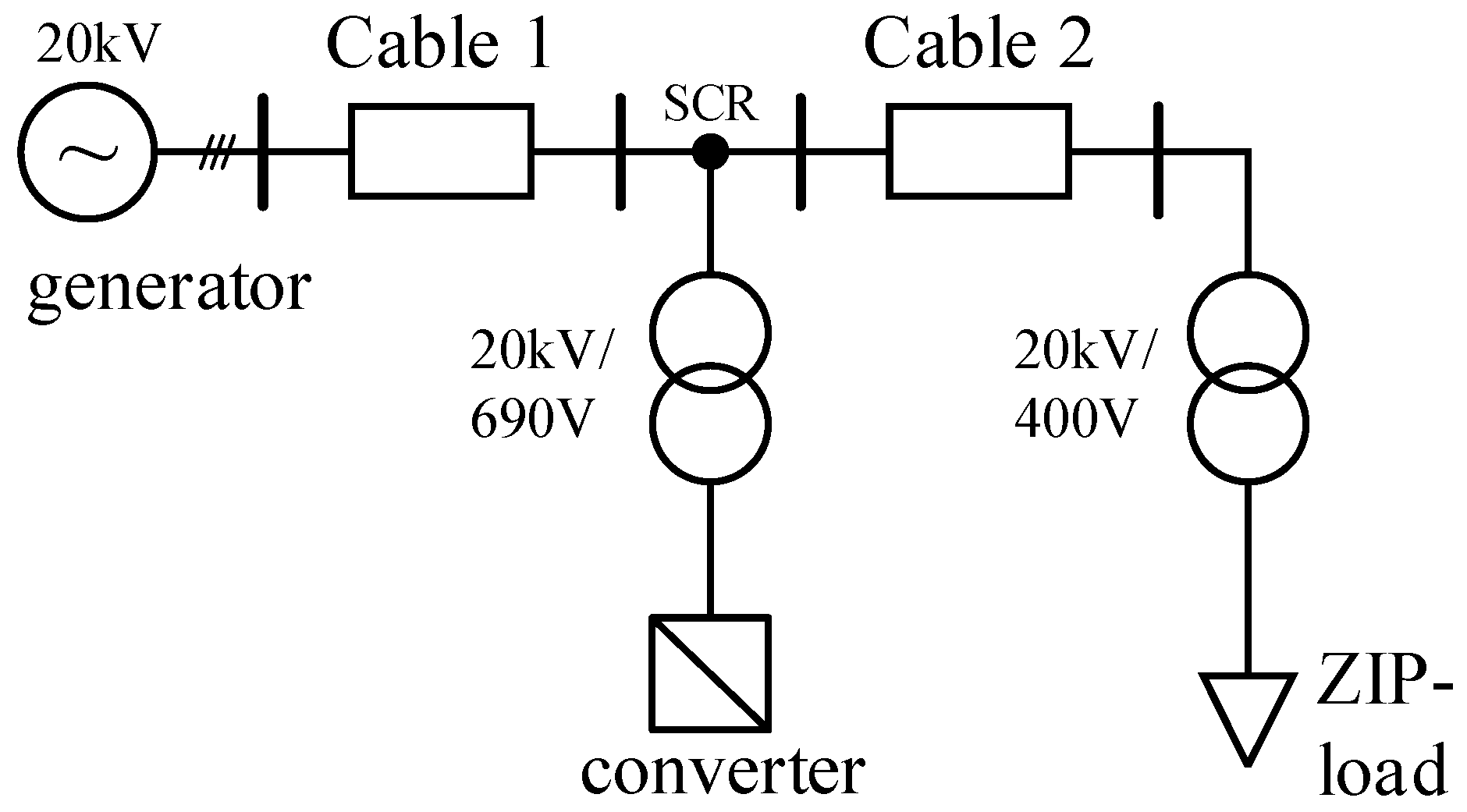

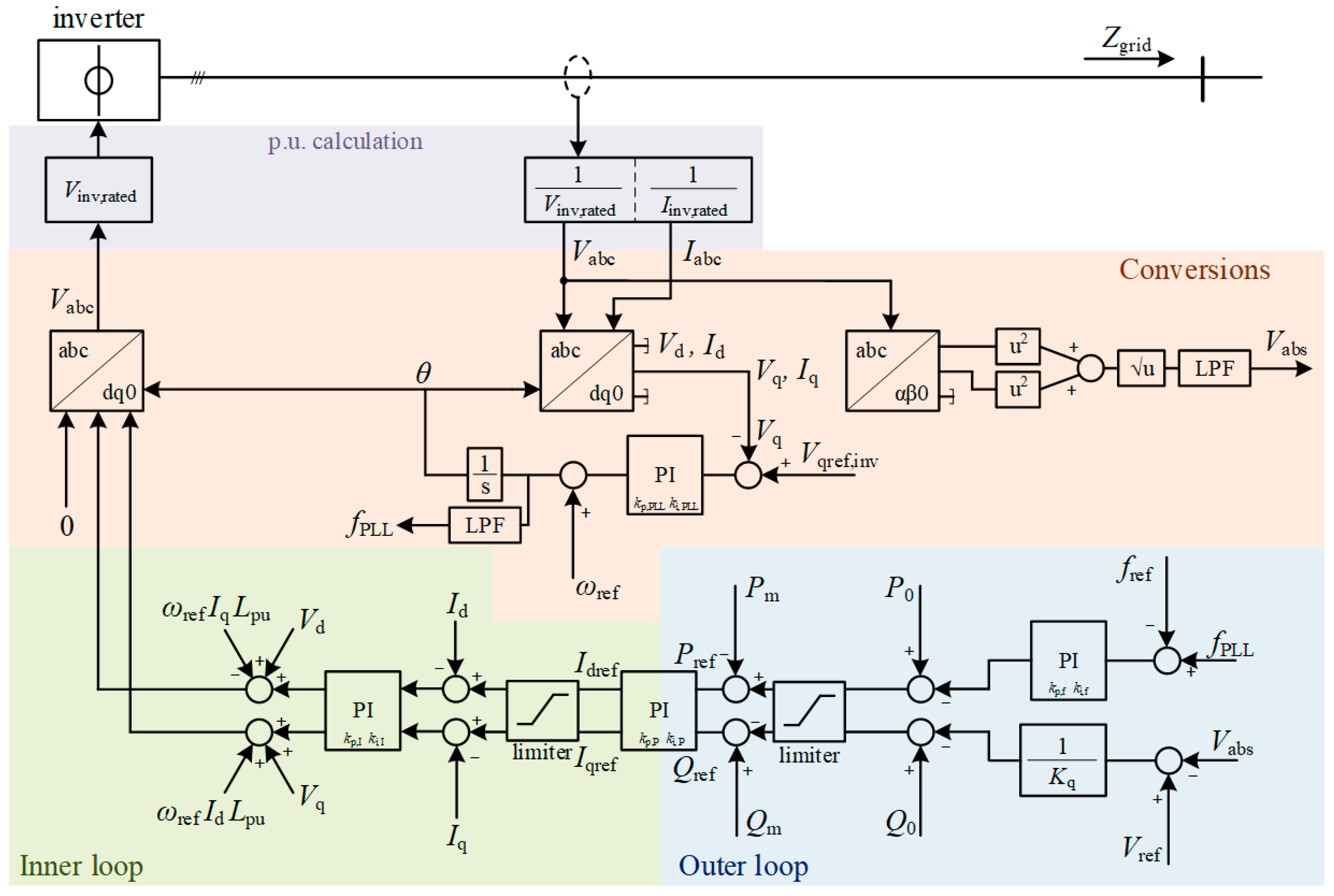



| Grid parameters: | Vgrid | 20 kV |
| fgrid | 50 Hz | |
| Cables: | Rpos | 0.15 Ω/km |
| Lpos | 0.42 mH/km | |
| Cpos | 0.25 μF/km | |
| l1, l2 | 6 km, 1 km | |
| Transformers: | ST,inv | 1 MVA |
| RHV,inv | 0.003 p.u. | |
| LHV,inv | 0.03 p.u. | |
| ST,load | 2 MVA | |
| RHV,load | 0.0029 p.u. | |
| LHV,load | 0.029 p.u. |
| Pbase | 1 MW |
| Pop | 1 MW and 1.5 MW |
| fn | 50 Hz |
| fref | 50 Hz |
| H | variable |
| Rg | 8 Ω |
| Xg | 0.8 Ω |
| Vinv,rated | 690 V |
| Iinv,rated | 1.183 kA |
| Vqref,inv | 0 p.u. |
| kp,PLL | 37.7 |
| ki,PLL | 5.36 |
| fref,inv | 50 Hz |
| ωref | |
| kp,f, ki,f | variable |
| Kq | variable |
| P0, Q0 | 0 p.u., 0 p.u. |
| kp,P, ki,P | 0.44, 56.43 |
| kp,I, ki,I | 2.00, 31.48 |
| Lpu | 0.318 mH |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lehmal, C.; Zhang, Z.; Renner, H.; Schürhuber, R. Enhancing Stability of Grid-Supporting Inverters from an Analytical Point of View with Lessons from Microgrids. Energies 2023, 16, 8054. https://doi.org/10.3390/en16248054
Lehmal C, Zhang Z, Renner H, Schürhuber R. Enhancing Stability of Grid-Supporting Inverters from an Analytical Point of View with Lessons from Microgrids. Energies. 2023; 16(24):8054. https://doi.org/10.3390/en16248054
Chicago/Turabian StyleLehmal, Carina, Ziqian Zhang, Herwig Renner, and Robert Schürhuber. 2023. "Enhancing Stability of Grid-Supporting Inverters from an Analytical Point of View with Lessons from Microgrids" Energies 16, no. 24: 8054. https://doi.org/10.3390/en16248054
APA StyleLehmal, C., Zhang, Z., Renner, H., & Schürhuber, R. (2023). Enhancing Stability of Grid-Supporting Inverters from an Analytical Point of View with Lessons from Microgrids. Energies, 16(24), 8054. https://doi.org/10.3390/en16248054





