1. Introduction
The severe consequences of the energy crisis and global climate change, which have arisen due to the overuse of fossil energy supplies since the commencement of the Industrial Revolution, have considerably affected both the economic circumstances and livelihoods of individuals [
1]. During the 75th session of the United Nations General Assembly, China made a public declaration to enhance its national autonomous contribution towards mitigating CO
2 emissions and fostering the development of a low-carbon society. China set forth its commitment to reach the “carbon peaking” milestone by 2030, followed by the ultimate goal of achieving “carbon neutrality” by 2060 [
2,
3].
Coal-fired power plants provide about 50% of China’s power system’s total installed capacity, contributing significantly to carbon emissions [
4]. Coal-fired power facilities fitted with carbon capture and storage (CCS) technologies have demonstrated their efficiency in mitigating CO
2 emissions [
5]. CCS can capture CO
2 from cogeneration emissions [
6]. Ref. [
7] provided an overview of the present level of development and fundamental concepts of CCS, with a specific focus on post-combustion technology. The primary method of sequestering CO
2 collected by CCS involved injecting deep, porous rock formations. However, this approach was subject to many economic and safety constraints [
8]. Utilizing power to gas (P2G) technology presented a viable approach to address this issue. The P2G process involves the electrolysis of water to generate hydrogen gas. This hydrogen gas is then utilized in the Sabatier reaction, which reacts with CO
2 to yield methane (CH
4) [
9]. Ref. [
10] presented a low-carbon economic dispatch model that incorporates the utilization of P2G and post-combustion CCS technologies in the context of electricity and natural gas systems. Ref. [
11] proposed a two-tier optimum scheduling model for an integrated energy system incorporating CCS and P2G technologies. A linked CCS-P2G model was developed to incorporate carbon trading and the green certification market [
12]. The study’s findings revealed a significant reduction of 50% in CO
2 emissions and a noteworthy decrease of 22.24% in operational expenses. Ref. [
13] examined integrating CCS and P2G technologies to investigate the correlation between reducing carbon emissions and implementing renewable energy utilization techniques.
Combined heat and power (CHP) is one of the major components of IES, which is distinguished by energy conservation and high efficiency [
14]. The inflexibility inherent in CHP systems resulted in significant wind abandonment. A proposed model for scheduling electric thermal systems incorporating CHP, thermal energy storage, and demand response aimed to mitigate wind abandonment and reduce pollutant emissions [
15]. The P2G process can harness unused wind energy and convert it into CH
4. Ref. [
16] presented a microgrid system that integrated combined cooling, heating, and power (CCHP) with P2G technology to enhance the efficiency of electrical connections. Ref. [
17] presented an academic model known as the integrated natural gas, heat, and power dispatch (INGHPD) model. This model aimed to effectively manage the demand for natural gas, heat, and electricity by incorporating wind and P2G units. Ref. [
18] presented a multi-objective optimal operation strategy for an integrated electricity–natural gas–thermal energy system. This strategy considered heat demand, P2G technology, and gas storage. The proposed method aimed to minimize both carbon emissions and operating costs. Most research efforts had concentrated on investigating the integrated operation of P2G and CHP systems. At the same time, there was a limited body of literature exploring the combined processes of P2G, CHP, and CCS technologies. Ref. [
19] presented the concept of CCS to capture CO
2 emissions from a CHP plant. The captured CO
2 was then utilized as a carbon feedstock for P2G applications. Ref. [
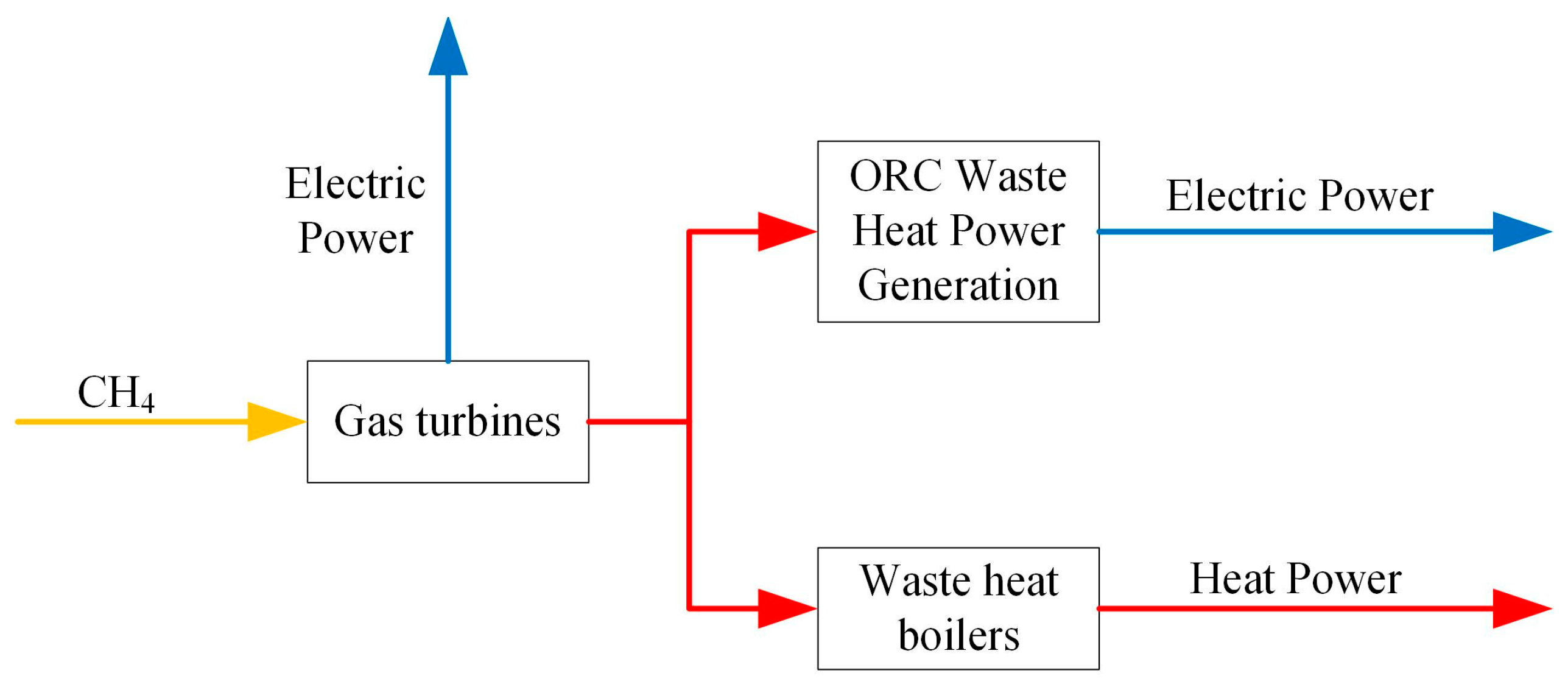
20] utilized the Organic Rankine Cycle (ORC) within a CHP system. The assessment of technical and economic costs was conducted through a life cycle approach. Ref. [
21] explored the addition of solar thermal (ST) and ORC technologies in traditional combined cooling, heating, and power (CCHP) systems. This study focused on the thermodynamic evaluation of the CCHP-ST-ORC configuration. Ref. [
22] conducted an experiment on IES that integrates ORC and P2G technologies. The research focused on analyzing the optimal thermoelectric ratios for various configurations of IES. Ref. [
23] analyzed a low-carbon economic dispatch model for a multi-energy virtual power plant. Additionally, it introduced an ORC waste heat power generation unit to address the issue of insufficient carbon capture during peak periods in the low-load process.
Ref. [
24] used the life cycle assessment approach to examine the greenhouse gas emissions associated with various energy chains within IES. Additionally, the authors evaluated the costs of carbon trading by utilizing carbon emission coefficients and incorporating stepped carbon trading incentive and penalty mechanisms. Ref. [
25] employed a phased carbon pricing mechanism to enhance the limitations on carbon emissions and the low-carbon advantages of IES. Ref. [
26] acknowledged the significance of integrating electricity, gas, and heat systems through waste heat recovery in P2G processes. Additionally, it proposed implementing a carbon trading mechanism to facilitate low-carbon operations in IES. Ref. [
27] examined the effects of the simultaneous implementation of heat grids, P2G technology, and carbon trading mechanisms on the integrated regional energy system. This integrated approach enhanced the economic and environmental advantages of the overall energy system. Ref. [
28] presented a multi-objective optimal scheduling model for IES. This model considered a multi-timescale stepped carbon trading mechanism to effectively handle the uncertainty related to renewable energy supply and load demand.
Ref. [
29] proposed a low-carbon economic dispatch with source-load coordination, which utilizes the energy time-shift advantages of CCS and P2G and combines the participation of electric vehicles in demand-side response to improve the system’s low-carbon and economic performance. Ref. [
30] proposed a two-layer optimization model of an integrated electricity–gas system considering carbon capture, utilization, and storage. It introduced a stepped carbon trading mechanism, effectively reducing the system’s operating cost and carbon emissions. Ref. [
31] proposed an integrated electricity–heat–gas energy system incorporating coupled operation of oxygen-rich combustion capture and power to gas (OCC-P2G) and hydrogen-doped gas equipment, and the proposed model has low economic costs and carbon emissions. Ref. [
32] constructed a carbon emission unit cost model with a dynamic reward and punishment pricing mechanism, which is an optimal scheduling model considering carbon emission, energy procurement, and equipment operation costs. It constructed a model with good economic efficiency. Ref. [
33] proposed a synergistic optimization model for the integrated energy system of cooling–heat–electricity–gas, considering the ladder carbon trading mechanism and multi-energy demand response, which enhances the economics of the integrated energy system.
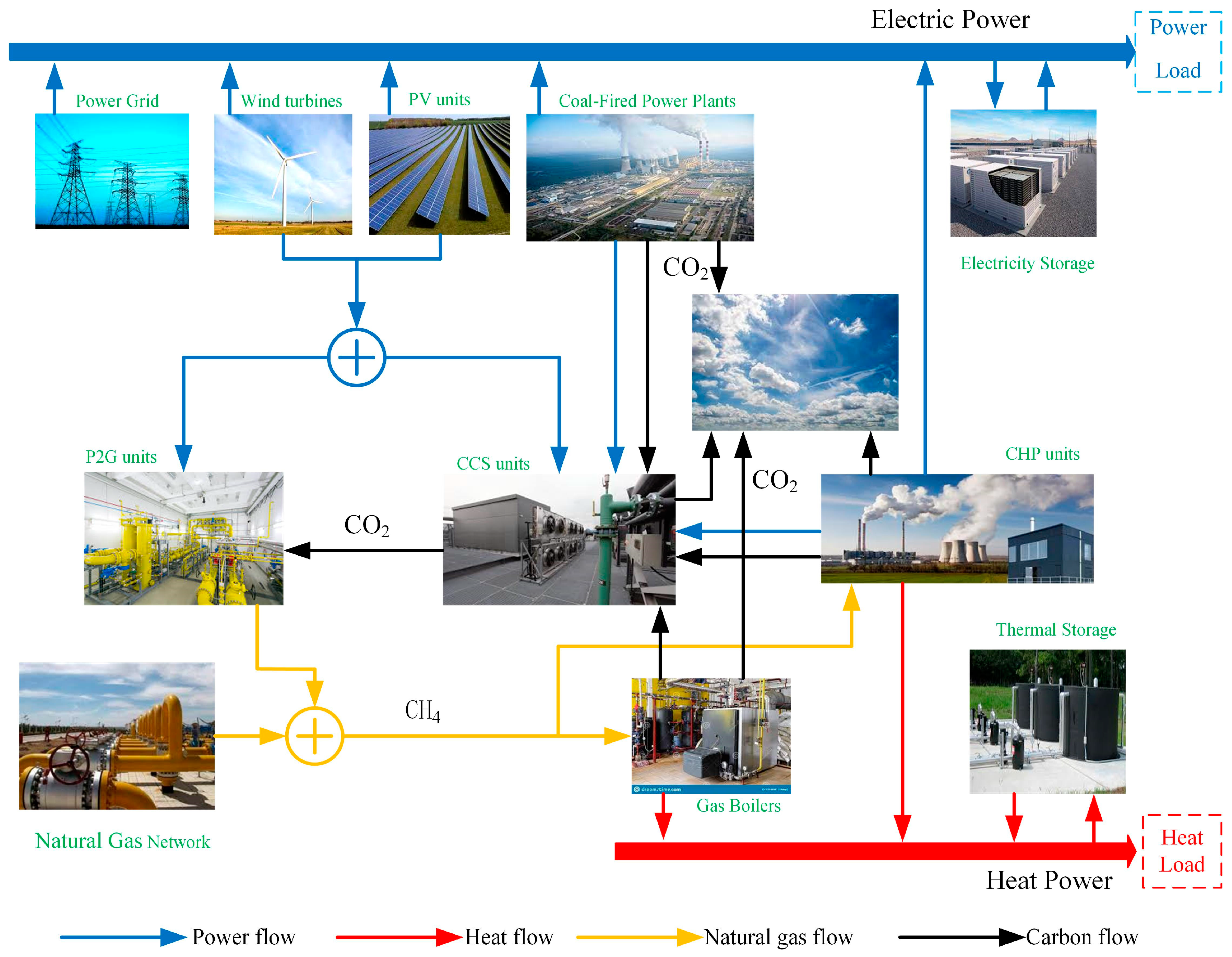
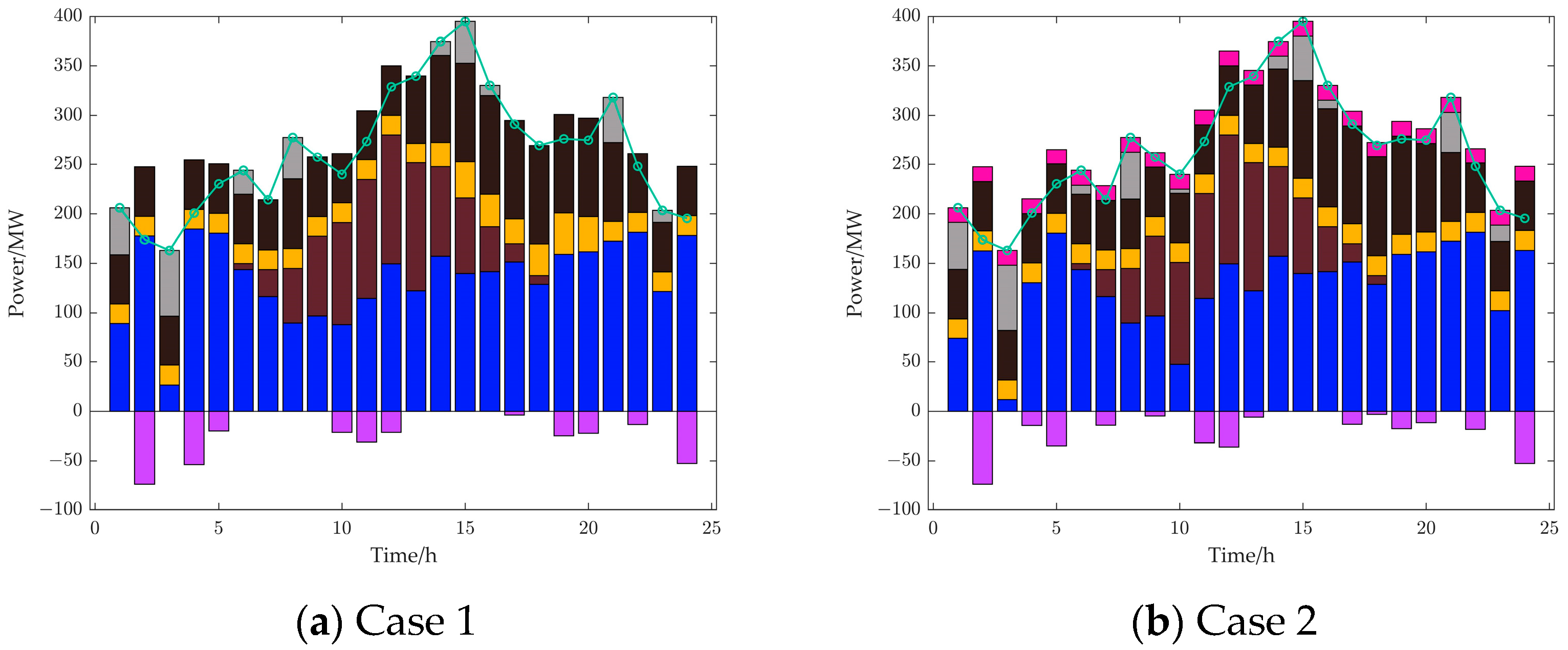
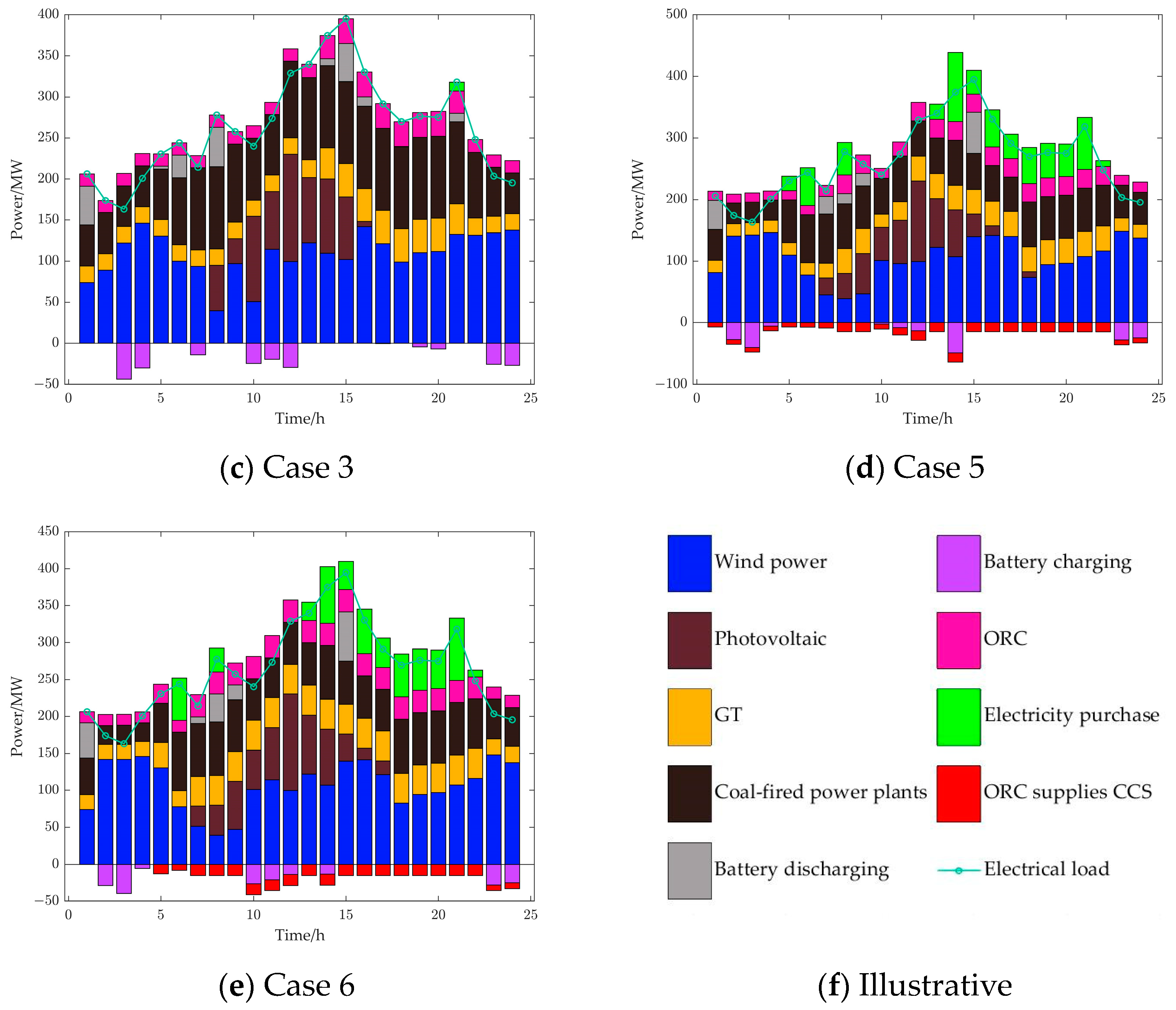
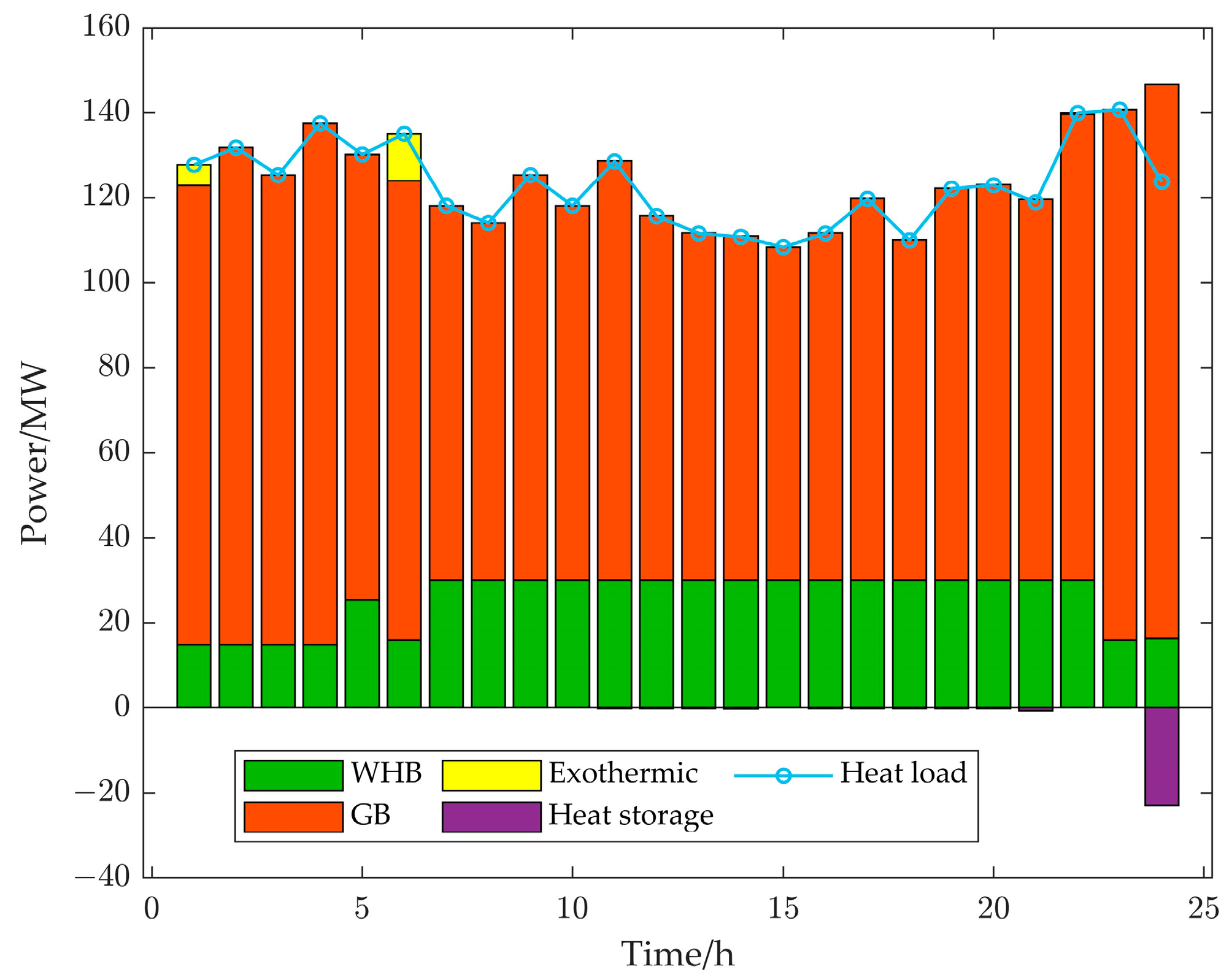
In summary, few current studies have considered the coupled operation of integrated energy systems containing an ORC CHP, CCS, and P2G. CCS can capture CHP and carbon dioxide emitted from coal-fired power plants to supply P2G to synthesize natural gas, which can be supplied to CHP for power generation and heat production. CCS and P2G can increase the consumption of new energy sources and reduce the curtailment of wind and solar energy. CCS and P2G can increase new energy consumption and reduce the curtailment of wind and light. An ORC can enable the operation of CHP thermoelectric decoupling and reduce the mutual constraints between power generation and heat production. The main contributions of this paper are summarized as follows.
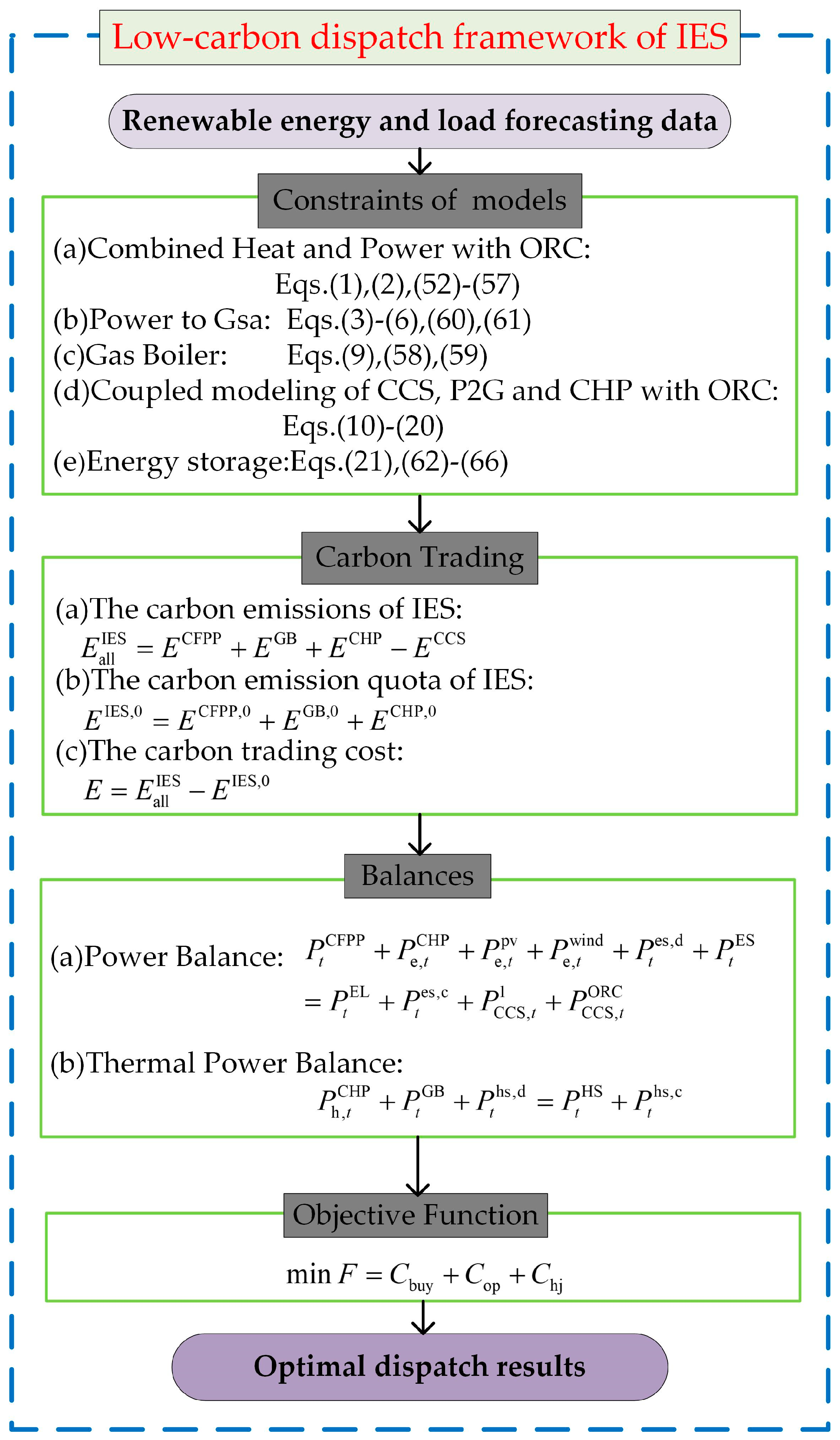
1. Establishment of an optimal scheduling model containing an ORC, CHP, CCS, and P2G, which further improves the coupling of electricity, gas, and heat.
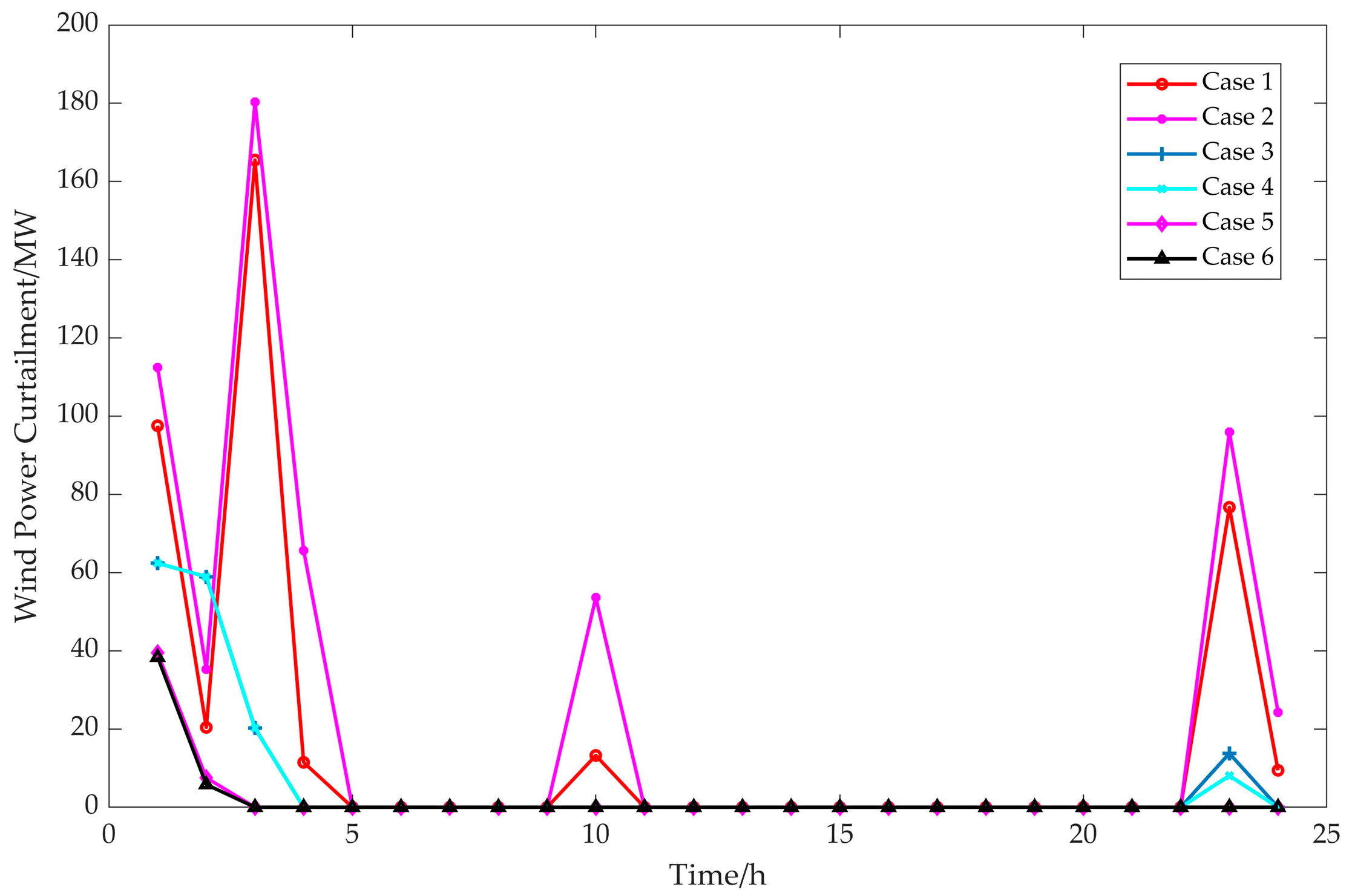
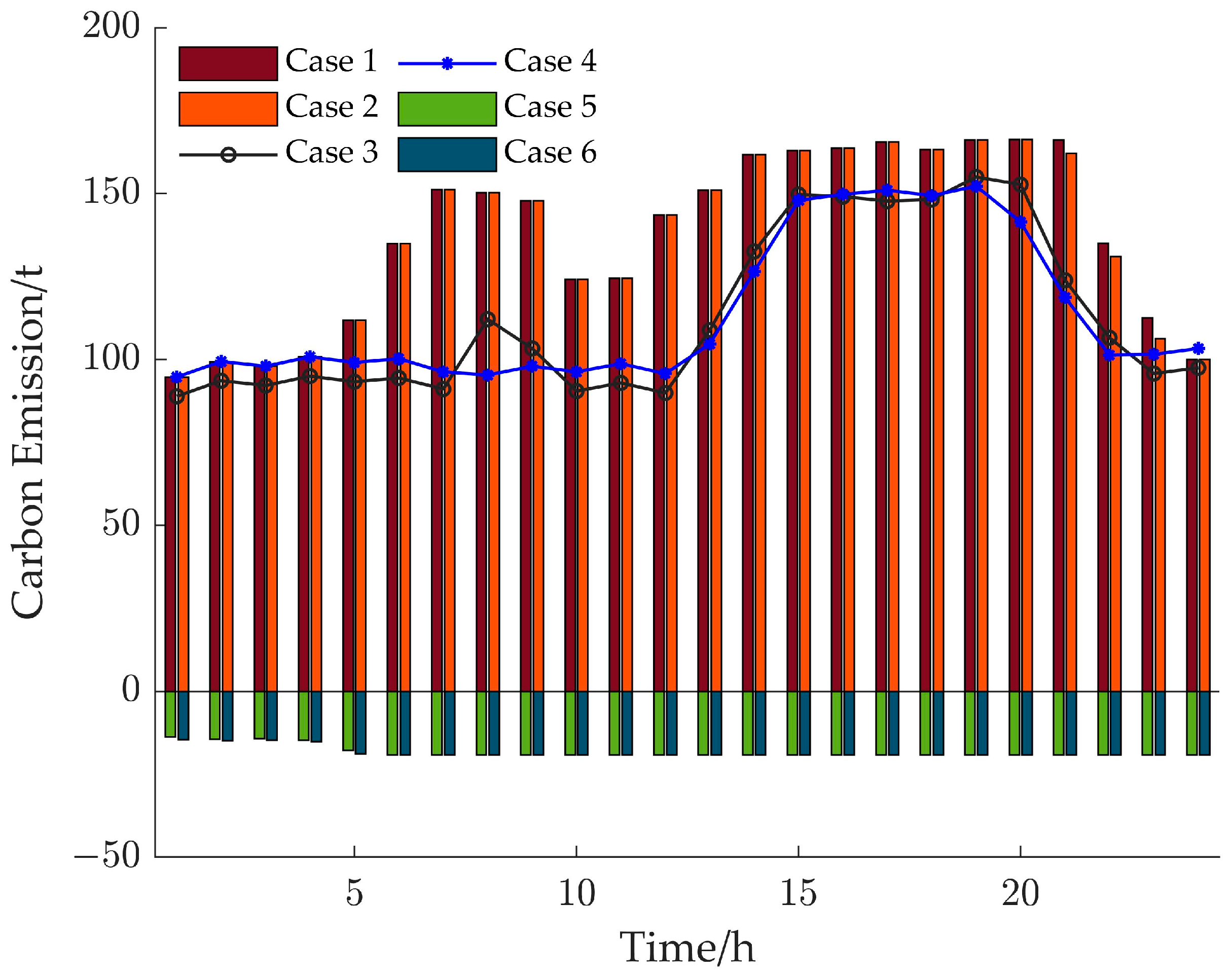
2. Comparing the operating costs and carbon emissions of different subsystem couplings, the proposed coupling model considering stepped carbon trading and ORC, CHP, CCS, and P2G reduces the operating costs, decreases carbon emissions, and increases new energy consumption.
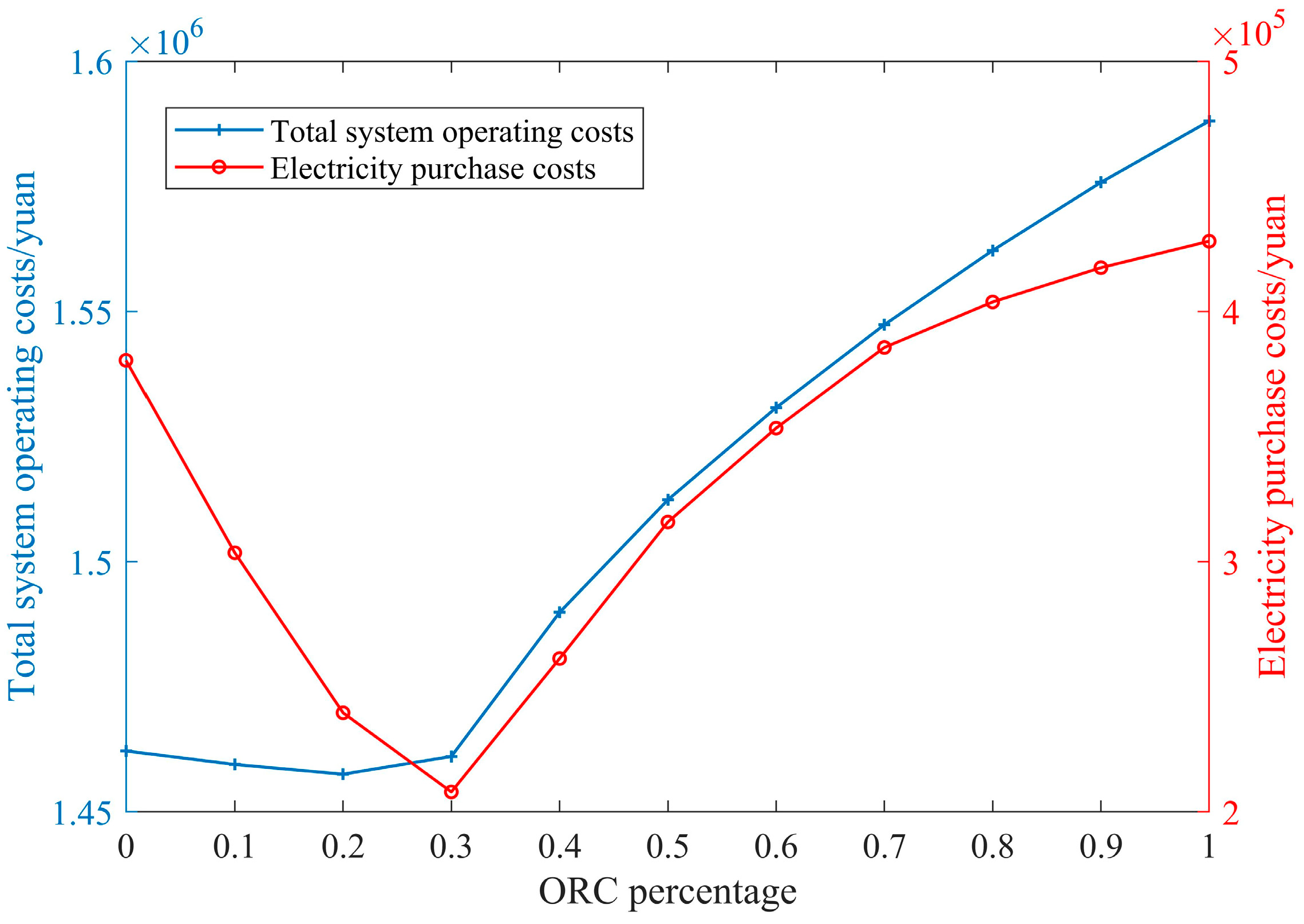
3. The effect of an ORC on the optimal scheduling model is investigated to analyze the optimal occupancy ratio.
Following this is the remainder of the paper. Consisting of ORC, CHP, CCS, and P2G coupling, the integrated energy system is described in
Section 2. The principle of ladder-type carbon trading is examined in
Section 3. The constraints and objective functions of the integrated energy system are delineated in
Section 4.
Section 5 verifies the validity of the proposed model by analyzing the different operation modes.
Section 6 concludes the paper.