Unsteady Magnetohydrodynamic Radiative Casson Nanofluid within Chemically Reactive Flow over a Stretchable Surface with Variable Thickness through a Porous Medium
Abstract
:1. Introduction
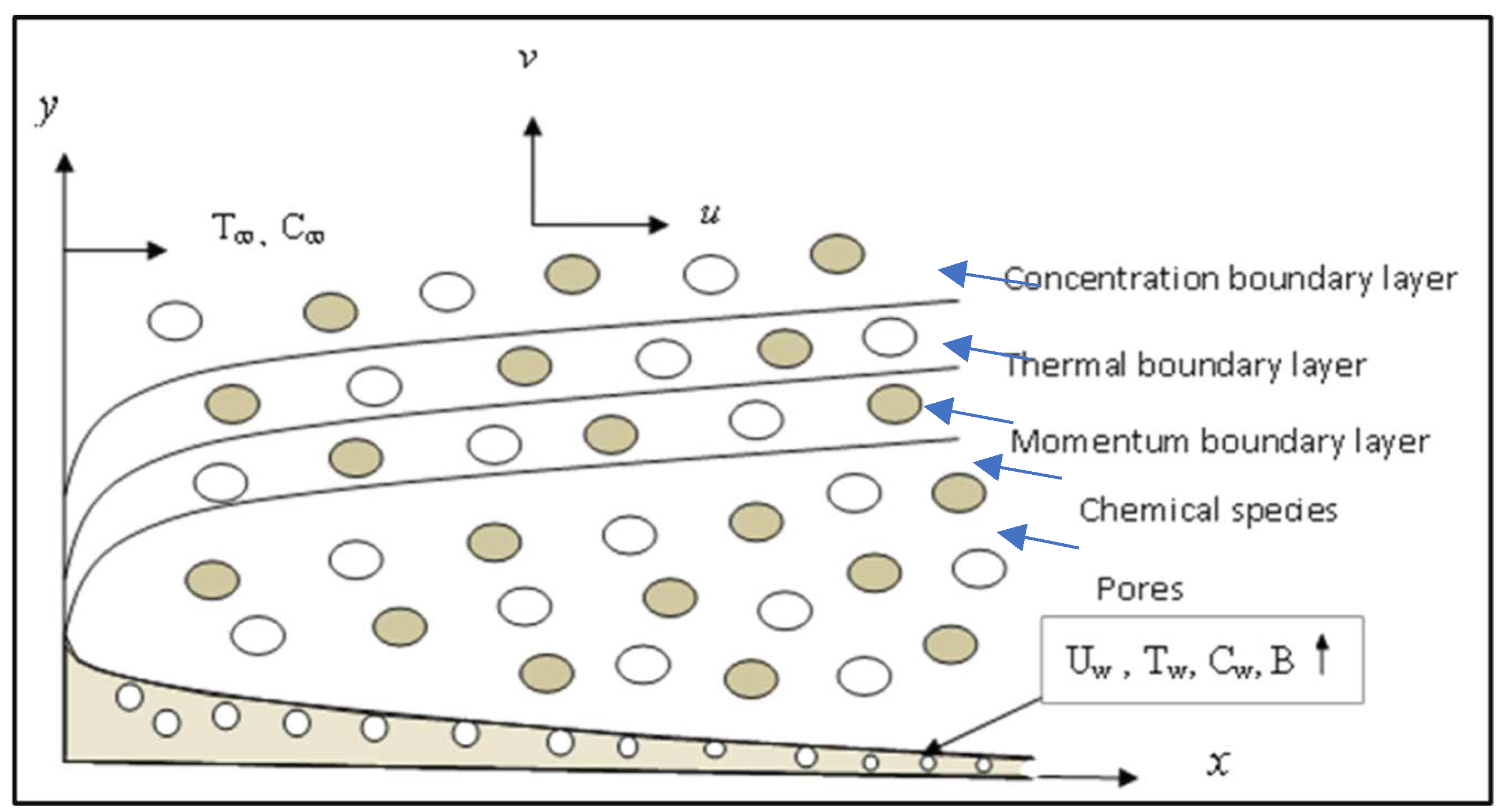
2. Analysis and Formulation of the Problem
3. Numerical Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Cf | local skin-friction coefficient (N·m−2) |
| Cp | specific heat at constant pressure (J/kg·K) |
| Gr | Grashof number (-) |
| g | acceleration due to gravity (m s−2) |
| B | strength of the magnetic field (Tesla) |
| kf | thermal conductivity of the fluid (W/m K) |
| ks | thermal conductivity of the solid (W/m K) |
| Ec | Eckert number (-) |
| K | porosity parameter (-) |
| Nt | thermophoretic parameter |
| Nb | Brownian motion parameter |
| Sc | Schmidt number (-) |
| fw | surface flow flux parameter (-) |
| Rc | Chemical reaction parameter |
| M | magnetic parameter (-) |
| NT | temperature ratio parameter (-) |
| Nu | local Nusselt number (-) |
| Pr | Prandtl number (-) |
| qr | radiative heat flux (W/m2) |
| Rd | radiative parameter (-) |
| Re | Reynolds number (-) |
| Shx | Sherwood number (-) |
| Q | heat generation coefficient (J/m2·K·s) |
| Tf | temperature of the fluid (K) |
| qw | surface heat flux (w/m2) |
| qc | mass flux (kg/m2·s) |
| T∞ | temperature of the ambient fluid (K) |
| Tw | temperature at the surface (K) |
| (u, v) | fluid velocities in the x, y-directions (ms−1) |
| (x, y) | axis direction (m) |
| Greek symbols | |
| ψ | stream function (kg/m·s) |
| Θ | nanofluid dimensionless temperature (-) |
| Φ | nanofluid dimensionless concentration (-) |
| τw | shearing stress (N/m2) |
| ρ | density of the fluid (kg m−3) |
| µ | dynamic viscosity of the fluid (N s/m2) |
| υ | kinematic viscosity (N s/m2) |
| γ | thermal variation parameter (W/m3·K) |
| α | conjugate conduction heat parameter |
| θ | dimensionless temperature (-) |
| heat generation factor (-) | |
| volume fraction (-) | |
| β | coefficient of thermal expansion (1/K) |
| η | space variable (-) |
| Subscripts | |
| w | wall conditions |
| ∞ | ambient temperature |
| Superscript | |
| \ | differentiation with respect to y |
References
- Keller, H.B. Numerical Methods in Boundary Layer Theory. Annu. Rev. Fluid Mech. 1978, 10, 417–433. [Google Scholar] [CrossRef]
- Cebeci, T.; Bradshaw, P. Physical and Computational Aspects of Convective Heat Transfer; Springer: New York, NY, USA, 1984. [Google Scholar]
- Elbashbeshy, E.M.A.; Bazid, M.A. Heat transfer over an unsteady stretching surface. Heat Mass Transf. 2004, 41, 1–4. [Google Scholar] [CrossRef]
- Ishak, A. Unsteady MHD flow and heat transfer over a stretching sheet. J. Appl. Sci. 2010, 10, 2127–2131. [Google Scholar] [CrossRef]
- Ibrahim, W.; Shanker, B. Unsteady MHD boundary layer flow and heat transfer due to stretching sheet in the presence of heat source or sink. Comput. Fluids J. 2012, 70, 21–28. [Google Scholar] [CrossRef]
- Makinde, O.D.; Khan, W.A.; Gulham, J.R. MHD variable viscosity reacting flow over a convectively heated plate in a porous medium with thermophoresis and radiative heat transfer. Int. J. Heat Mass Transf. 2016, 93, 595–604. [Google Scholar] [CrossRef]
- Sheikholeslami, M. Numerical simulation of magnetic nanofluid natural convection in porous media. Phy. Lett. A. 2017, 381, 494–503. [Google Scholar] [CrossRef]
- Elbashbeshy, E.M.A.; Eldabe, N.T.M.; Sedki, A.M. Effects of pressure stress work and thermal radiation on free convection flow around a sphere embedded in a porous medium with Newtonian heating. Therm. Sci. 2018, 22, 401–412. [Google Scholar] [CrossRef]
- Eldabe, N.T.M.; Ghaly, A.Y.; Mohamoud, M.S.H. MHD boundary layer chemical reacting flow with heat transfer of Eyring-Powell nanofluid past a stretching sheet. Microsyst. Technol. 2018, 24, 4945–4953. [Google Scholar] [CrossRef]
- Trivedi, M.; Ansari, M.S.; Motsa, S.S. Flow and heat transfer of a nanofluid by mixed convection with non-uniform heat source/sink and magnetic field effect: A numerical approach. Comput. Therm. Sci. 2019, 11, 189–203. [Google Scholar]
- Mahabaleshwar, U.S.; Nagaraju, K.R.; Lorenzini, G. Effect of mass transfer and MHD induced Navier’s slip flow due to non-linear stretching sheet. J. Eng. Thermophyscis 2019, 28, 578–600. [Google Scholar] [CrossRef]
- Khan, A.; Naem, S.; Vafai, K. Dufour, and Soret effects on Darcy-Forchheimer flow of second-grade fluid with the variable magnetic field and thermal conductivity. In International Journal of Numerical Methods for Heat and Fluid Flow; Emerald Publishing Limited: Bingley, UK, 2019; ISSN 0961-5539. [Google Scholar] [CrossRef]
- Maleki, H.; Alsarraf, J.; Safaei, M.R. Heat transfer and nanofluid flow over a porous plate with radiation and slip boundary conditions. J. Cent. South Univ. 2019, 26, 1099–1115. [Google Scholar] [CrossRef]
- Pordanjani, A.H.; Aghakhani, S.; Afrand, M. Investigation of free convection heat transfer entropy generation of nanofluid flow inside a cavity affected by a magnetic field and thermal radiation. J. Therm. Anal. Calorim. 2019, 137, 997–1019. [Google Scholar] [CrossRef]
- Chaudhary, S.; Kanika, K.M. Heat generation/absorption and radiation effects on hydromagnetic stagnation point flow of nanofluids toward a heated porous stretching/shrinking sheet with suction/injection. J. Porous Medium 2020, 23, 27–49. [Google Scholar] [CrossRef]
- Reddy, P.S.; Sreedevi, P. MHD boundary layer heat and mass transfer flow of nanofluid through porous media over an inclined plate with chemical reaction. Multidiscip. Model Mater Struct 2021, 17, 317–336. [Google Scholar] [CrossRef]
- Eldabe, N.T.M.; Abou-Zeid, M.Y.; Mohamed, M.A.A. MHD peristaltic non-Newtonianewtonian power-law nanofluid through a non-Darcy porous medium inside a non-uniform inclined channel. Arch. Appl. Mech. 2021, 91, 1067–1077. [Google Scholar] [CrossRef]
- Sedki, A.M.; Abo-Dahab, S.M.; Bouslimi, J.; Mahmoud, K.H. Thermal radiation effect on unsteady mixed convection boundary layer flow and heat transfer of nanofluid over permeable stretching surface through a porous medium in the presence of heat generation. Sci. Prog. 2021, 104, 1–19. [Google Scholar] [CrossRef]
- Sedki, A.M. Effect of thermal radiation and chemical radiation on MHD mixed convective heat and mass transfer in nanofluid flow due to a nonlinear stretching surface through a porous medium. Results Mater. 2022, 16, 100334. [Google Scholar] [CrossRef]
- Mukhopadhyay, S. Effects of thermal radiation on Casson fluid flow and heat transfer over an unsteady stretching surface subjected to suction/blowing. Chin. Phys. B 2013, 22, 114702. [Google Scholar] [CrossRef]
- Makanda, G.; Shaw, S.; Sibanda, P. Diffusion of chemically reactive species in Casson fluid flow over an nsteady stretching surface in porous medium in the presence of a magnetic field. Math. Probl. Eng. 2015, 2015, 724596. [Google Scholar] [CrossRef]
- Raju, C.S.K.; Sandeep, N.; Reddy, J.V.R. Heat and mass transfer in magnetohydrodynamic Casson fluid over an exponentially permeable stretching surface. Eng. Sci. Technol. 2016, 19, 45–52. [Google Scholar] [CrossRef]
- Oyelakin, I.S.; Mondal, S.; Sibanda, P. Unsteady Casson nanofluid flow over a stretching sheet with thermal radiation, convective and slip boundary conditions. Alex. Eng. J. 2016, 55, 1025–1035. [Google Scholar] [CrossRef]
- Krishnamurthy, M.R.; Gireesha, B.J.; Gorla, R.S.R. Thermal radiation and chemical reaction effects on boundary layer slip flow and melting heat transfer of nanofluid induced by nonlinearly stretching sheet. Nonlinear Eng. 2016, 5, 147–159. [Google Scholar] [CrossRef]
- Ullah, I.; Bhattacharyya, K.; Shafie, S.; Ilyas Khan, I. Unsteady MHD mixed convection slip flow of Casson fluid over nonlinearly stretching sheet embedded in a porous medium with chemical reaction, thermal radiation, heat generation/absorption, and convective boundary conditions. PLoS ONE 2016, 11, 0165348. [Google Scholar] [CrossRef] [PubMed]
- Prasad, K.V.; Vajravelu, K.; Vaidya, H. MHD Casson nanofluid flow and heat transfer at a stretching sheet with variable thickness. J. Nanofluids 2016, 5, 423–435. [Google Scholar] [CrossRef]
- Tamoor, M.; Waqas, M.; Khan, M.I.; Alsaedi, A.; Hayat, T. Magnetohydrodynamic flow of Casson fluid over a stretching cylinder. Results Phys. 2017, 7, 498–502. [Google Scholar] [CrossRef]
- Naqvi, S.M.R.S.; Muhammad, T.; Asma, M. Hydromagnetic flow of Casson nanofluid over a porous stretching cylinder with Newtonian heat and mass conditions. Phys. A 2020, 550, 123988. [Google Scholar] [CrossRef]
- Shit, G.C.; Mandal, S. Entropy analysis on unsteady MHD flow of Casson nanofluid over a stretching vertical plate with thermal radiation effect. Int. J. Appl. Comput. Math. 2020, 6, 2. [Google Scholar] [CrossRef]
- Krishna, M.V.; Ahammad, N.A.; Chamkha, A.J. Radiative MHD flow of Casson hybrid nanofluid over an infinite exponentially accelerated vertical porous surface. Cas Stud. Therm. Eng. 2021, 27, 101229. [Google Scholar] [CrossRef]
- Bejawada, S.G.; Reddy, Y.D.; Jamshed, W.; Nisar, K.S.; Alharbi, A.N.; Chouikh, R. Radiation effect on MHD Casson fluid flow over an inclined non-linear surface with chemical reaction in a Forchheimer porous medium. Alex. Eng. J. 2022, 61, 8207–8220. [Google Scholar] [CrossRef]
- Sedki, A.M. Computational analysis of MHD Cross nanofluid containing gyrotactic microorganisms over permeable horizontal cylinder through a porous medthe ium in presence of thermal radiation and chemical reaction. Partial Differ. Equ. Appl. Math. 2023, 7, 100508. [Google Scholar] [CrossRef]
- Khan, M.N.; Ahmad, S.; Wang, Z.; Fadhl, B.M.; Irshad, K. Enhancement in the efficiency of heat recovery in a Williamson hybrid nanofluid over a vertically thin needle with entropy generation. Heliyon 2023, 9, 17665. [Google Scholar] [CrossRef] [PubMed]
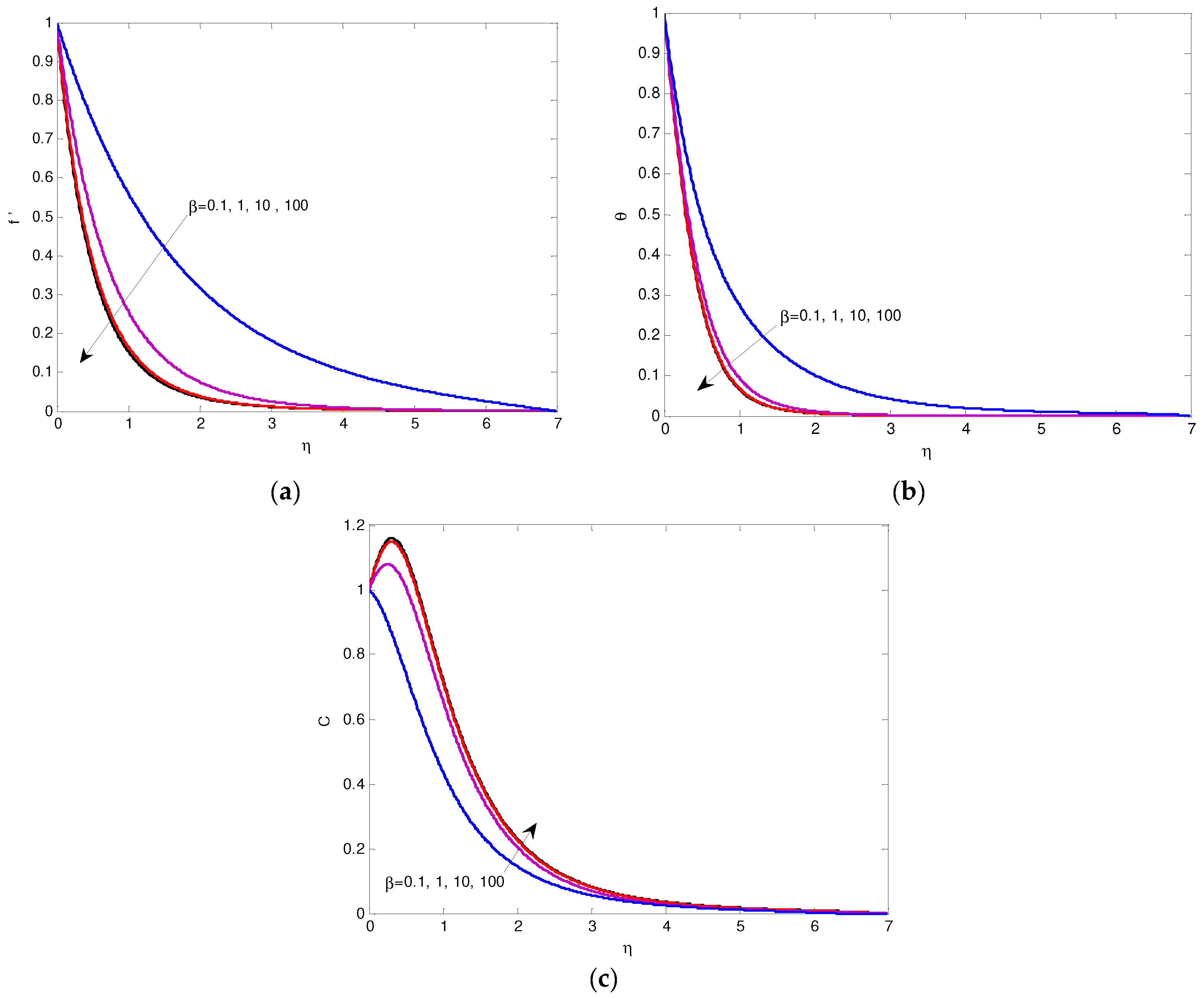
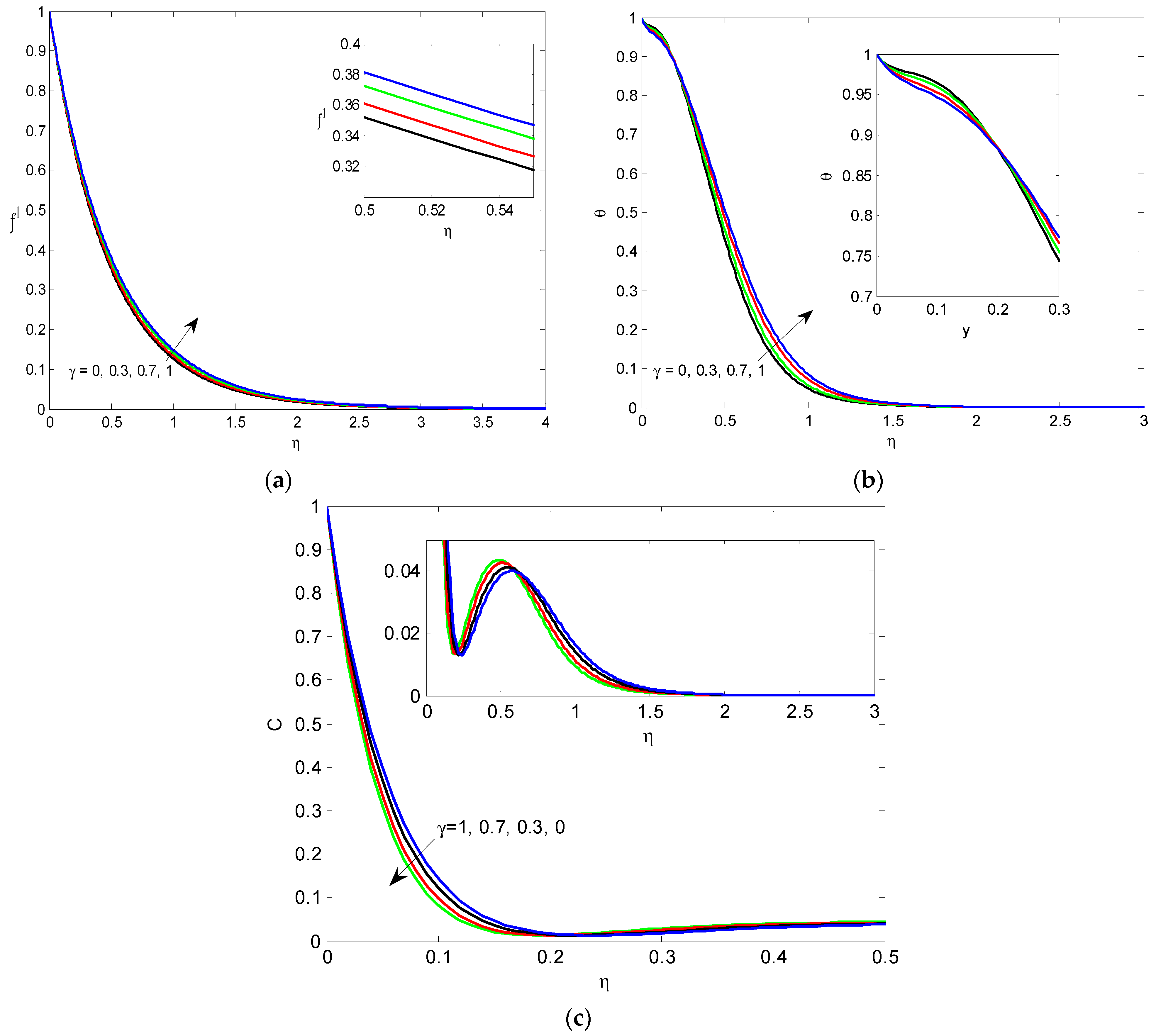
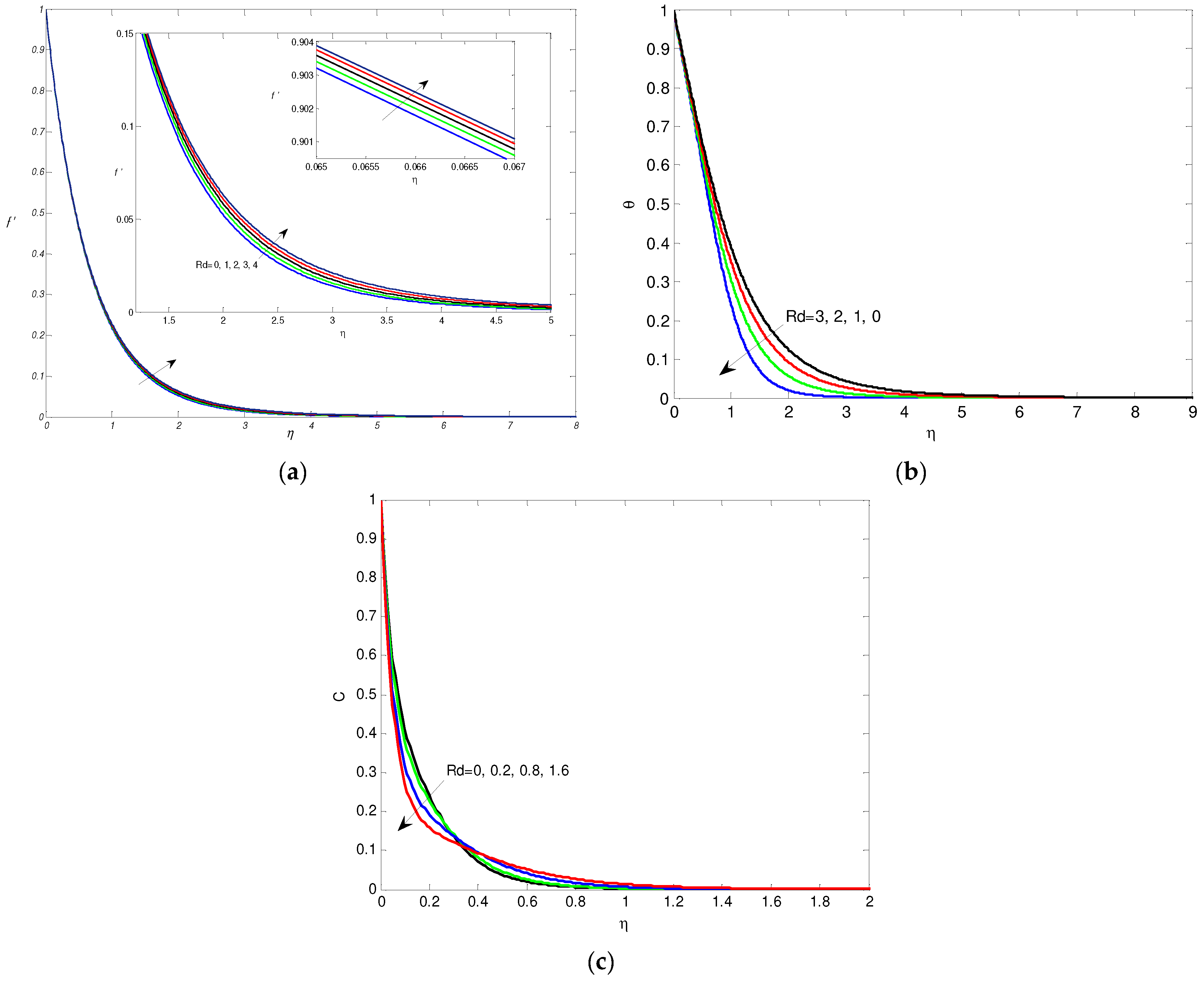
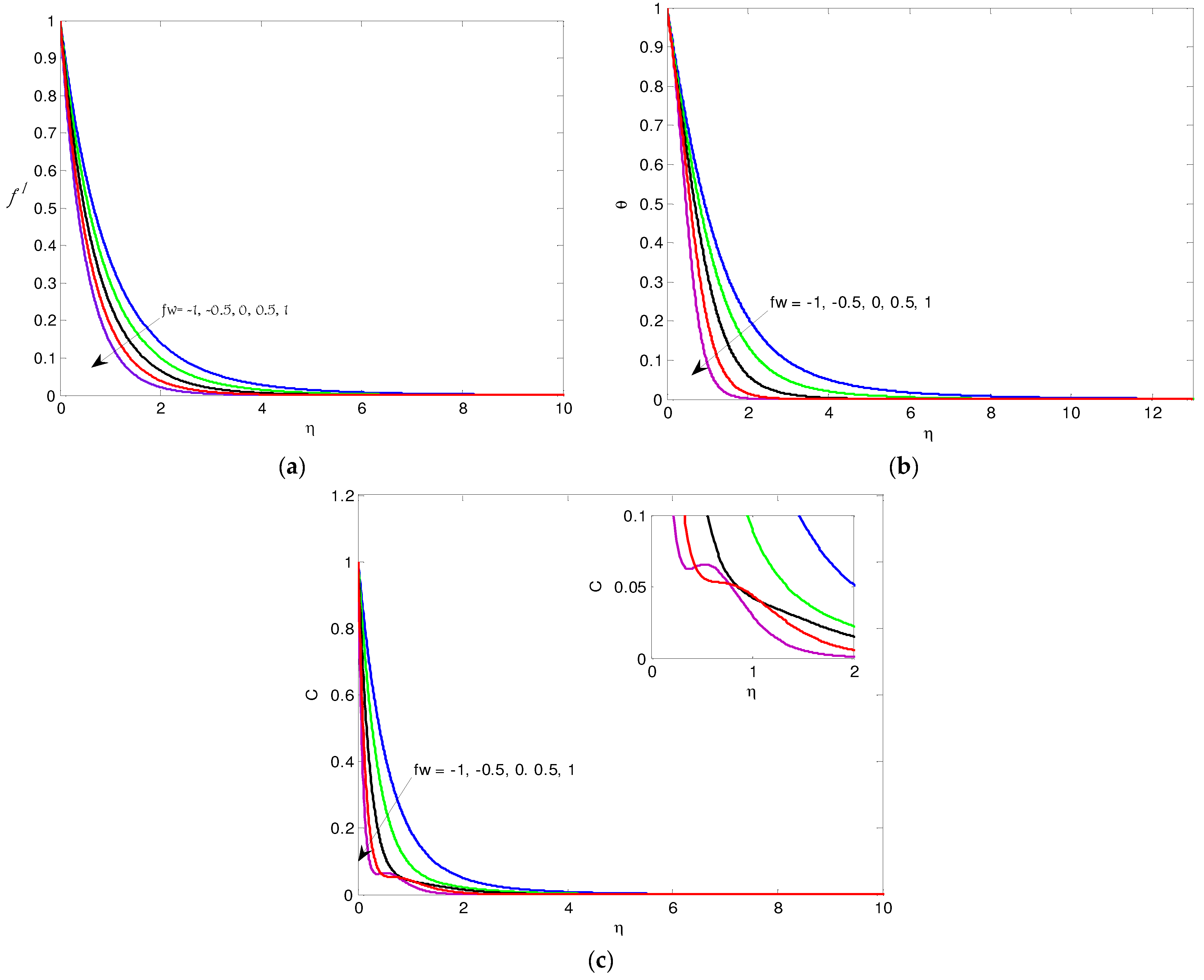
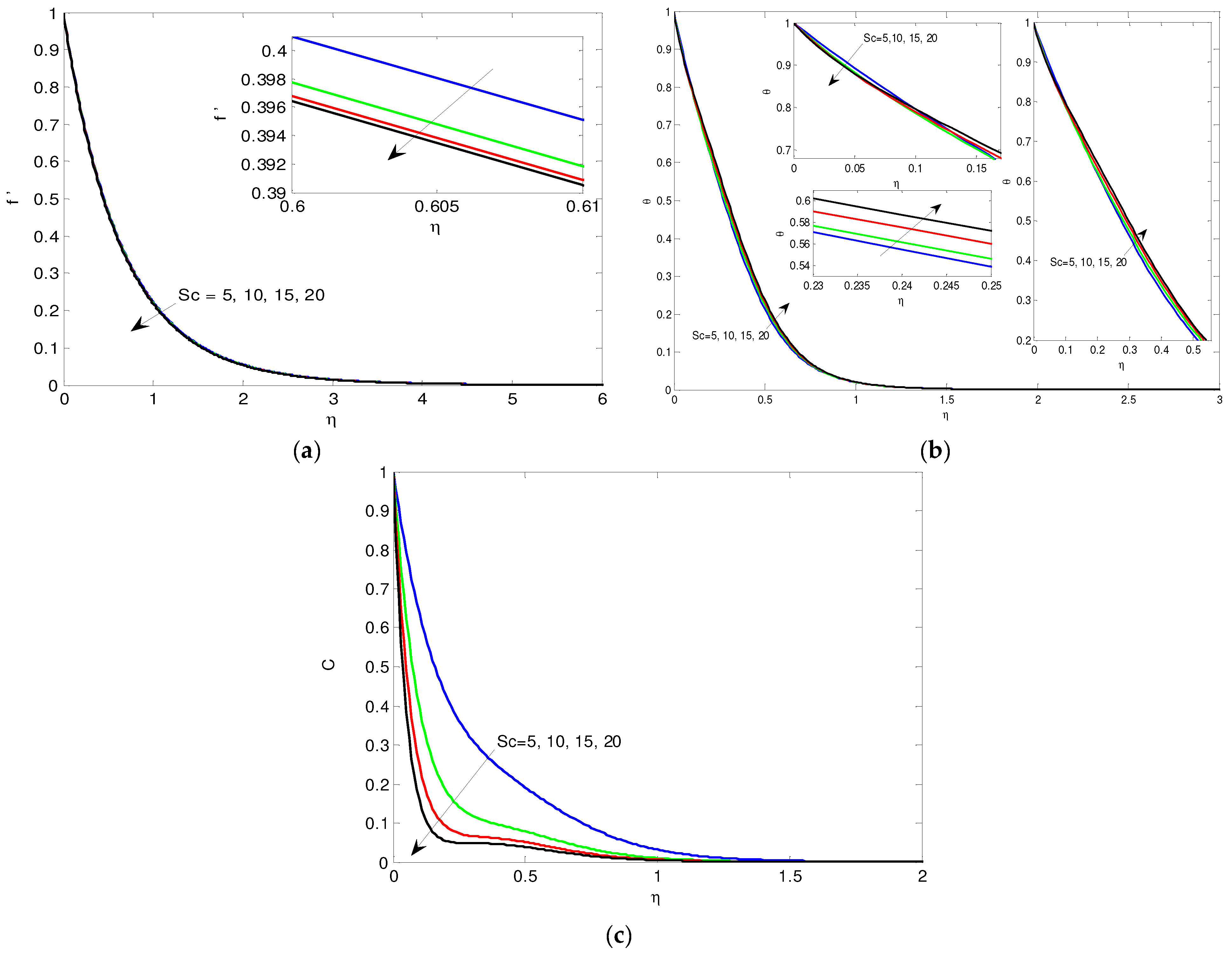
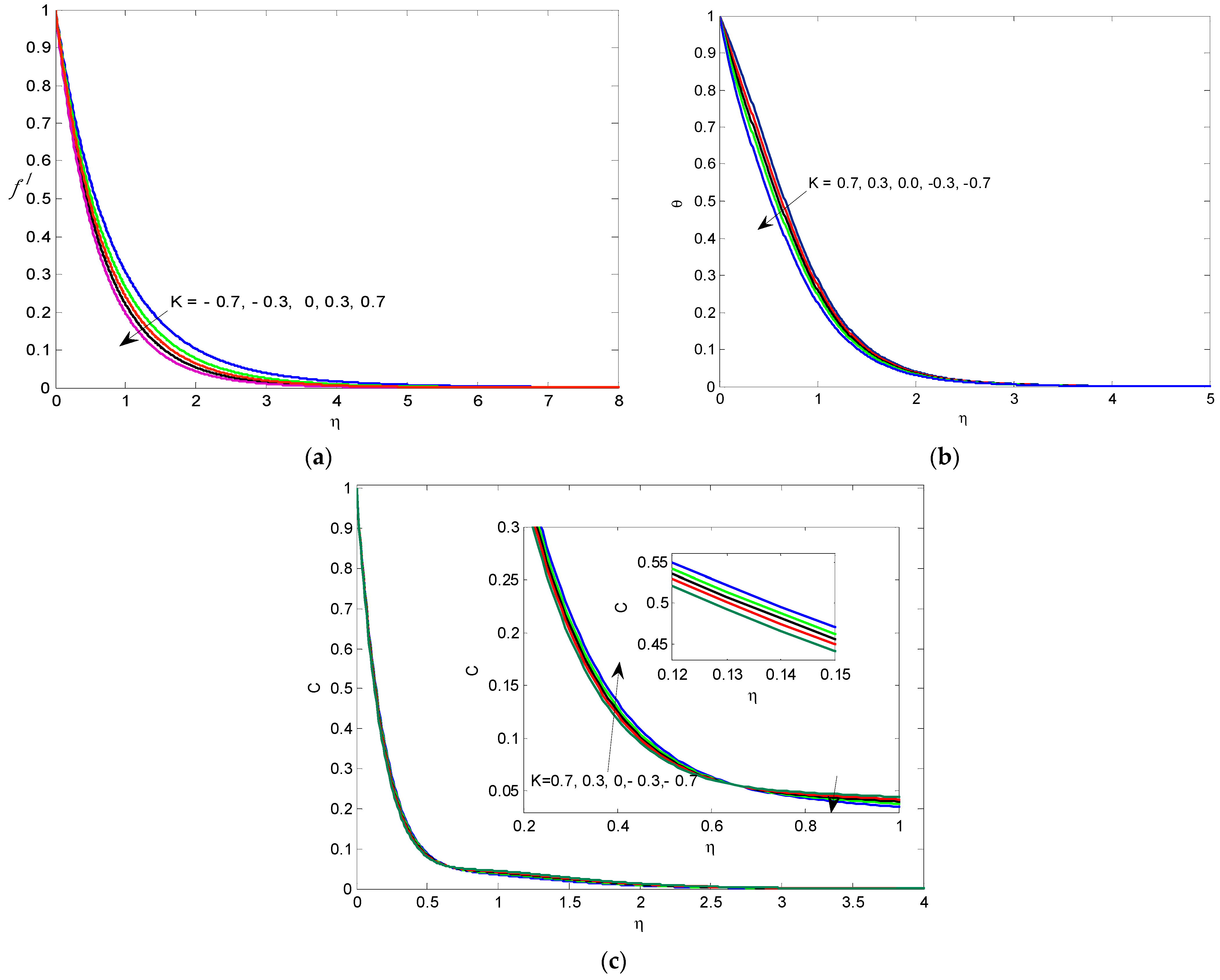
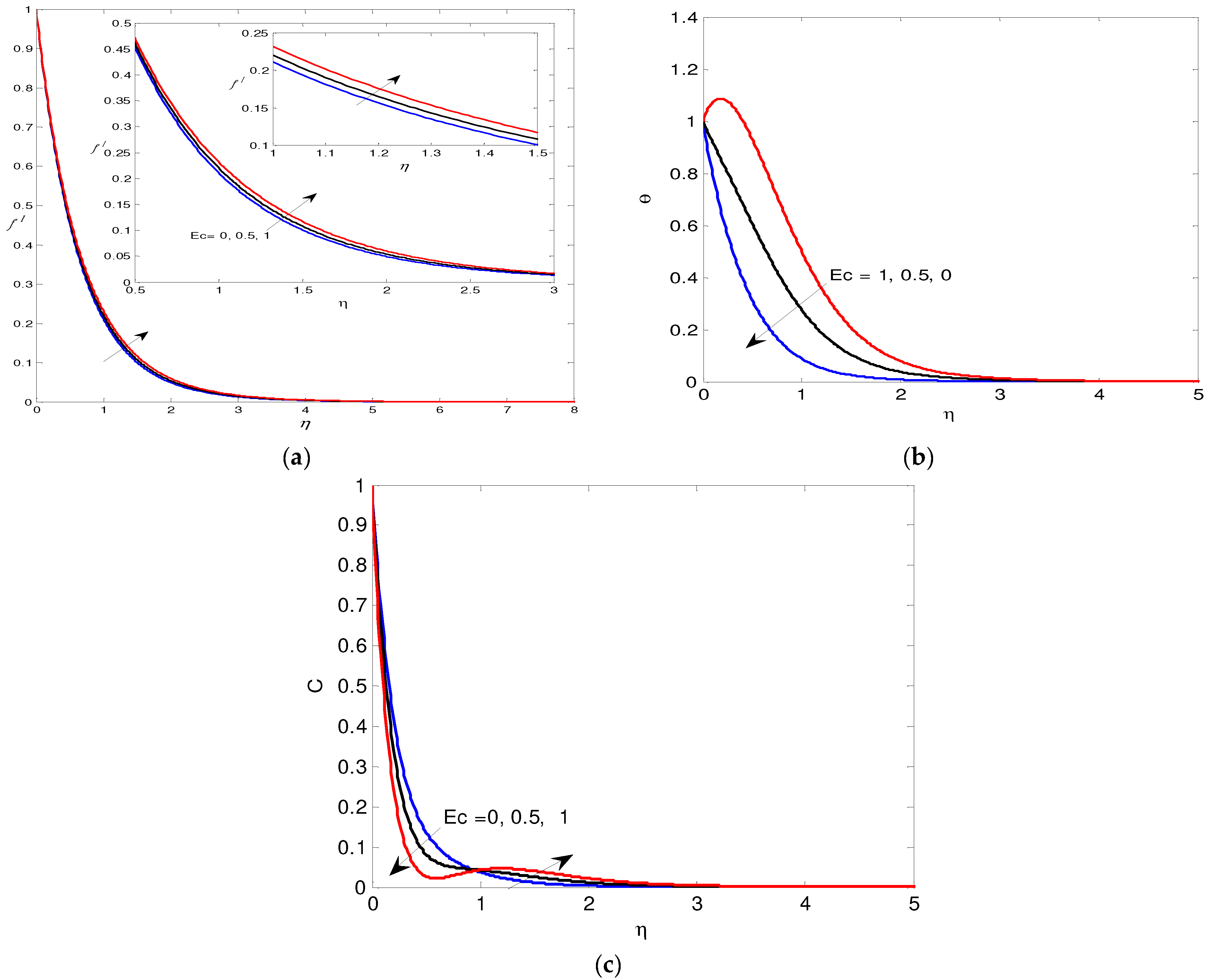
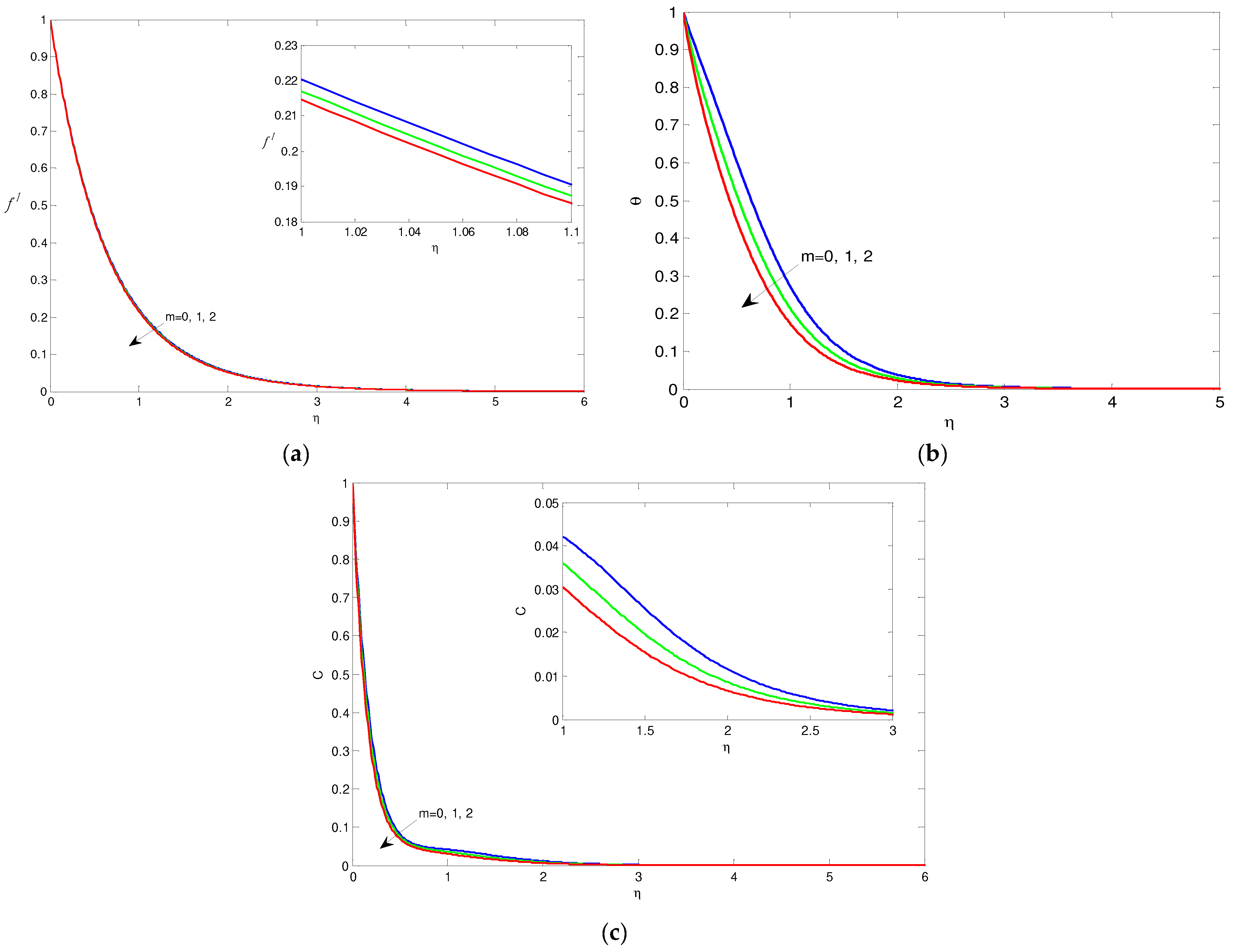
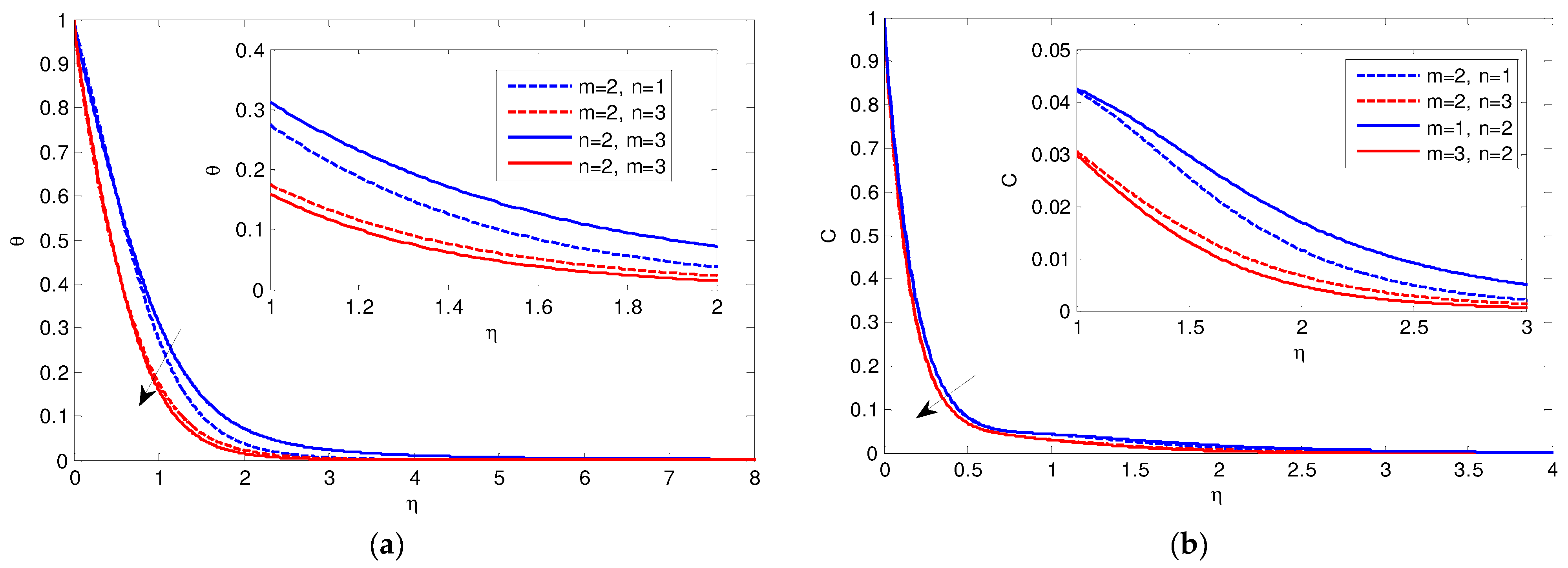
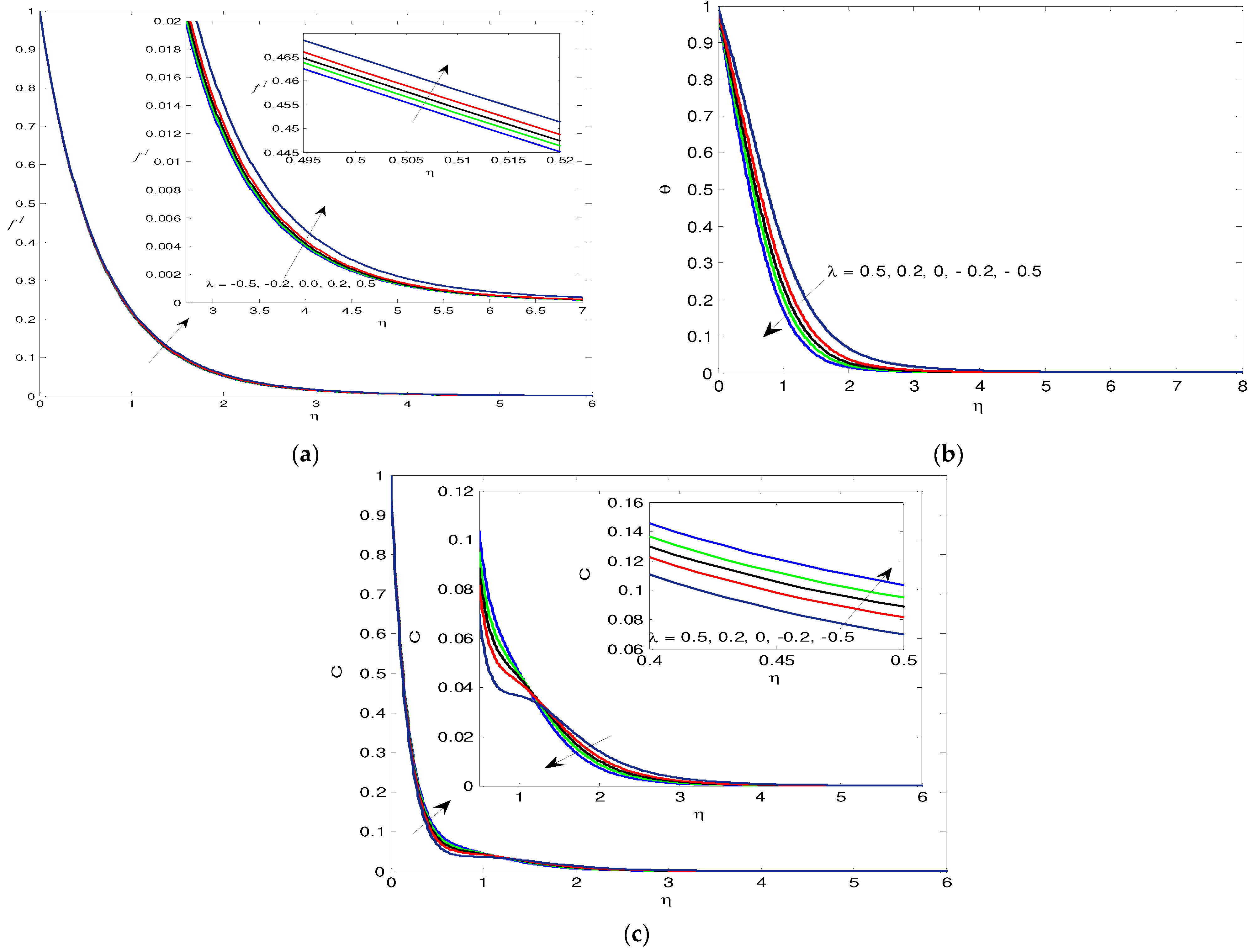

| Pr | Elbashbeshy [3] | Ishak [4] | Ibrahim [5] | Present Study | |
|---|---|---|---|---|---|
| −θ\(0) | −θ\(0) | −θ\(0) | −f\\(0) | −θ\(0) | |
| 0.72 | 0.808 | 0.8086 | 0.8095 | 1.0000 | 0.80864 |
| 1.0 | 1.0 | 1.0 | 1.0001 | 1.0000 | 1.0000 |
| 10 | 3.7207 | 3.7202 | 3.7208 | 1.0000 | 3.7207 |
| Pr = 10, β = 5, At = 0.1, Nb = 0.5, ϕ = 0.05, fw = 1, M = 1.0, Rd = 0.5, λ = 0.2, δ = 0.2, γ = 0.5, α = 0.5, Rc = 0.2, K = 0.2, Ec = 0.5, Nt = 0.5, m = 1, Sc = 10.0, n = 1. | ||||||
|---|---|---|---|---|---|---|
| γ | β | Sc | M | −f\\(0) | −θ\(0) | −C\(0) |
| 0 | 5 | 10 | 1.0 | 1.896675 | 2.256345 | 4.787477 |
| 0.5 | 1.878542 | 2.228124 | 4.715303 | |||
| 1 | 1.860481 | 2.200052 | 4.643463 | |||
| 1.5 | 1.842493 | 2.172133 | 4.571964 | |||
| 2 | 1.824579 | 2.144371 | 4.500815 | |||
| 0.5 | 0.5 | 10 | 1.0 | 1.824765 | 0.02412134 | 6.678931 |
| 1 | 1.85813 | 1.453518 | 5.398466 | |||
| 2 | 1.871352 | 1.961246 | 4.950035 | |||
| 5 | 1.878542 | 2.228124 | 4.715303 | |||
| 0.5 | 0.5 | 5 | 1.0 | 1.878542 | 2.228124 | 4.715303 |
| 10 | 1.920071 | 2.655981 | 9.524478 | |||
| 15 | 1.935082 | 2.916045 | 14.31092 | |||
| 20 | 1.942566 | 3.09288 | 19.10896 | |||
| 0.1 | 0.5 | 10 | 0.2 | 1.403348 | 1.917512 | 0.3306047 |
| 1 | 1.704235 | 1.632986 | 0.5422387 | |||
| 2 | 2.019402 | 1.273092 | 0.8306606 | |||
| 3 | 2.290982 | 0.9057231 | 1.140812 | |||
| Pr = 6.2, β = 2, A = 0.8, fw = 2, Sc = 10.0, λ = 0.5, α = 0.5, ẟ = 0.2, Rc = 0.3, K = 0.2, Ec = 0.5, Nt = 0.2, Nb = 0.1, γ = 0.5, m = 1, n = 2, M = 3 | |||||
|---|---|---|---|---|---|
| Rd | ϕ | Rc | −f\\(0) | −θ\(0) | −C\(0) |
| 0 | 0.05 | 0.3 | 2.97296 | 4.770681 | 12.2837 |
| 0.2 | 2.969926 | 4.239647 | 13.26994 | ||
| 0.8 | 2.961826 | 3.223742 | 15.12073 | ||
| 1.6 | 2.952785 | 2.49741 | 16.40074 | ||
| 0.8 | 0 | 2.699535 | 3.260639 | 15.0950 | |
| 0.05 | 2.864129 | 3.338922 | 14.9355 | ||
| 0.1 | 2.964379 | 3.494682 | 14.63177 | ||
| 0.15 | 3.004126 | 3.698014 | 14.24473 | ||
| 0.2 | 2.989678 | 3.922428 | 13.82292 | ||
| 0.8 | 0.05 | 0 | 2.86105 | 3.082186 | 15.0847 |
| 1 | 2.864047 | 3.100647 | 18.95979 | ||
| 2 | 2.86548 | 3.110097 | 21.52028 | ||
| 3 | 2.866947 | 3.120559 | 25.26479 | ||
| 4 | 2.86861 | 3.134327 | 32.89318 | ||
| Pr = 7, β = 0.5, A = 0.1, Nt = 0.5, Nb = 0.5, Sc = 10.0, Rd = 0.5, λ = 0.2, α = 0.2, Rc = 0.2, ϕ = 0.05, γ = 0.5, M = 1, fw = 0.2, K = 0.3, Ec = 0.5 | |||||||
|---|---|---|---|---|---|---|---|
| fw | K | Ec | m | n | −f\\(0) | −θ\(0) | −C\(0) |
| −0.7 | 0.3 | 0.5 | 1 | 2 | 1.166979 | 0.6896947 | 2.128103 |
| −0.5 | 1.244454 | 0.725028 | 2.530241 | ||||
| 0 | 1.466949 | 0.8371657 | 4.175094 | ||||
| 0.5 | 1.729128 | 0.9243421 | 7.03585 | ||||
| 0.7 | 1.844753 | 0.9296373 | 8.493711 | ||||
| 0.2 | −0.7 | 1.221034 | 1.467047 | 4.862795 | |||
| −0.3 | 1.369453 | 1.234478 | 4.976422 | ||||
| 0 | 1.471461 | 1.05878 | 5.069695 | ||||
| 0.3 | 1.567185 | 0.8816247 | 5.169064 | ||||
| 0.7 | 1.686805 | 0.6427757 | 5.310168 | ||||
| 0.3 | 0 | 1.586038 | 2.333166 | 4.397425 | |||
| 0.5 | 1.567185 | 1.357548 | 5.169064 | ||||
| 0.7 | 1.558611 | 0.8816247 | 5.612531 | ||||
| 1 | 1.544367 | 0.1232255 | 6.556958 | ||||
| 0.5 | 1 | 1.567185 | 0.8816247 | 5.169064 | |||
| 2 | 1.576039 | 1.405037 | 5.738256 | ||||
| 3 | 1.582981 | 1.834487 | 6.289898 | ||||
| 2 | 1 | 1.566207 | 1.088665 | 5.366438 | |||
| 2 | 1.576039 | 1.405037 | 5.738256 | ||||
| 3 | 1.582743 | 1.671965 | 6.084604 | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sedki, A.M.; Qahiti, R. Unsteady Magnetohydrodynamic Radiative Casson Nanofluid within Chemically Reactive Flow over a Stretchable Surface with Variable Thickness through a Porous Medium. Energies 2023, 16, 7776. https://doi.org/10.3390/en16237776
Sedki AM, Qahiti R. Unsteady Magnetohydrodynamic Radiative Casson Nanofluid within Chemically Reactive Flow over a Stretchable Surface with Variable Thickness through a Porous Medium. Energies. 2023; 16(23):7776. https://doi.org/10.3390/en16237776
Chicago/Turabian StyleSedki, Ahmed M., and Raed Qahiti. 2023. "Unsteady Magnetohydrodynamic Radiative Casson Nanofluid within Chemically Reactive Flow over a Stretchable Surface with Variable Thickness through a Porous Medium" Energies 16, no. 23: 7776. https://doi.org/10.3390/en16237776
APA StyleSedki, A. M., & Qahiti, R. (2023). Unsteady Magnetohydrodynamic Radiative Casson Nanofluid within Chemically Reactive Flow over a Stretchable Surface with Variable Thickness through a Porous Medium. Energies, 16(23), 7776. https://doi.org/10.3390/en16237776








