Decomposition of the Voltages in a Three-Phase Asymmetrical Circuit with a Non-Sinusoidal Voltage Source

Abstract

This article presents the concept of a mathematical description of a three-phase, four-wire asymmetrical electric circuit in decomposition into Voltages’ Physical Components (VPC), associated with distinctive physical phenomena in the load. This is an alternative method of mathematical description to the Currents’ Physical Components (CPC) still being developed since the end of the last century. According to previous studies, the improvement of the power factor in three-phase systems is possible by observing several components. Compensation for the scattered power is possible only by using a reactive compensator connected in series with the load. Thanks to the presented analytical method, it is possible to design compensators connected in series with the load. The VPC power theory opens the possibility of improving the power factor in three-phase networks for loads with asymmetry between phases. Due to the unfavorable impact of high currents on the compensator branches, the method proposed in the article can improve the energy quality in local low-power grids. However, the possibility of its practical use in high-power industrial networks is questionable.

1. Introduction

Actions that reduce the energy intensity of the power system are forced by the modern development of the energy economy. In addition to activities performed in the production and receiving area, the distribution area is also an important element. An important factor here is to take care of the appropriate reduction in the current value flowing in the power system [1]. The key tools for understanding the phenomena that cause increased current flow in an electrical circuit are mathematical methods of describing the flow of energy. Knowing the nature of the physical energy interactions in the load, it becomes possible to take action to improve the power factor. One of the basic activities is power compensation.

There are known methods of power compensation using parallel reactive compensators [2,3,4]. This is the basic method of compensation, which has many practical solutions [5,6,7,8], the essence of which is to select the appropriate value of reactance connected in parallel with the load. The optimal solution here is to use the theory of Shepherd and Zakikhani [9], but one should remember the inaccuracies of this method. The CPC theory [10,11,12,13,14], whose evolution has accelerated in recent years, can be used to determine the compensator parameters more precisely. Further references can be found in [15,16,17,18,19,20]. The description of single-phase circuits is discussed in [15,21] and for three-phase, four-wire circuits in [22,23,24]. Recent improvements in this power theory [3,7,25,26] enable a complete mathematical description of the electrical quantities in real three-phase circuits. In addition, thanks to the distribution of the current into individual components, it is possible to analyze the circuit in various situations that occur in real systems [27,28,29,30]. Based on the CPC theory, power compensation methods have been developed [31,32,33] and special attention should be paid to [34,35,36]. When changes in electrical parameters in the circuit are taken into account, adaptive algorithms [25,26] are very important. According to the conclusions presented in this literature, it is possible to improve the power factor to satisfactory values, but due to the presence of the scattered component, complete compensation cannot be achieved.

Other alternative methods for analyzing single-phase [37,38,39,40,41] and three-phase [42,43,44,45,46] electrical circuits have also been described in the literature, but due to the methodology used in them, they do not provide a solution to the problem posed in this article. These theories were also used to develop algorithms determining the parameters of the compensator [47,48,49,50,51]. The authors of [52] present a comparison of several compensation methods. A common feature of all compensation methods is the use of resonant circuits [53,54,55]. In the case of parallel compensation, current resonance is used. The classic way to compensate for reactive power in high-power industrial networks is to use a synchronous compensator—a synchronous electric machine of a rotating type with electromagnetic excitation, operating in motor mode at idle speed. However, this classical method does not compensate for load asymmetry across phases.

For the correct assessment of electrical parameters before and after compensation, an appropriate method of power measurement [56,57,58,59] should be adopted. When the analysis takes into account the nonlinearity of the load [29,60,61,62], additional analytical problems will appear. The appearance of many harmonics in current and voltage waveforms means that the compensator should be selected for all signal frequencies [63,64,65]. Then, additional voltage and current filtration [33,66,67,68] are required.

This article presents a mathematical analysis that enables a different method of power compensation, one that is able to reduce the impact of the scattered component on the efficiency of the power system. For this purpose, voltage resonance is used, i.e., power compensation is also possible in a series connection. Such a compensation method may have little practical use; however, it should be treated as an alternative to modern compensation methods and an attempt to answer the following question: is complete compensation possible in the power system?

In order to discuss the series reactive compensator, it is first necessary to decompose the electrical quantities into Voltages’ Physical Components.

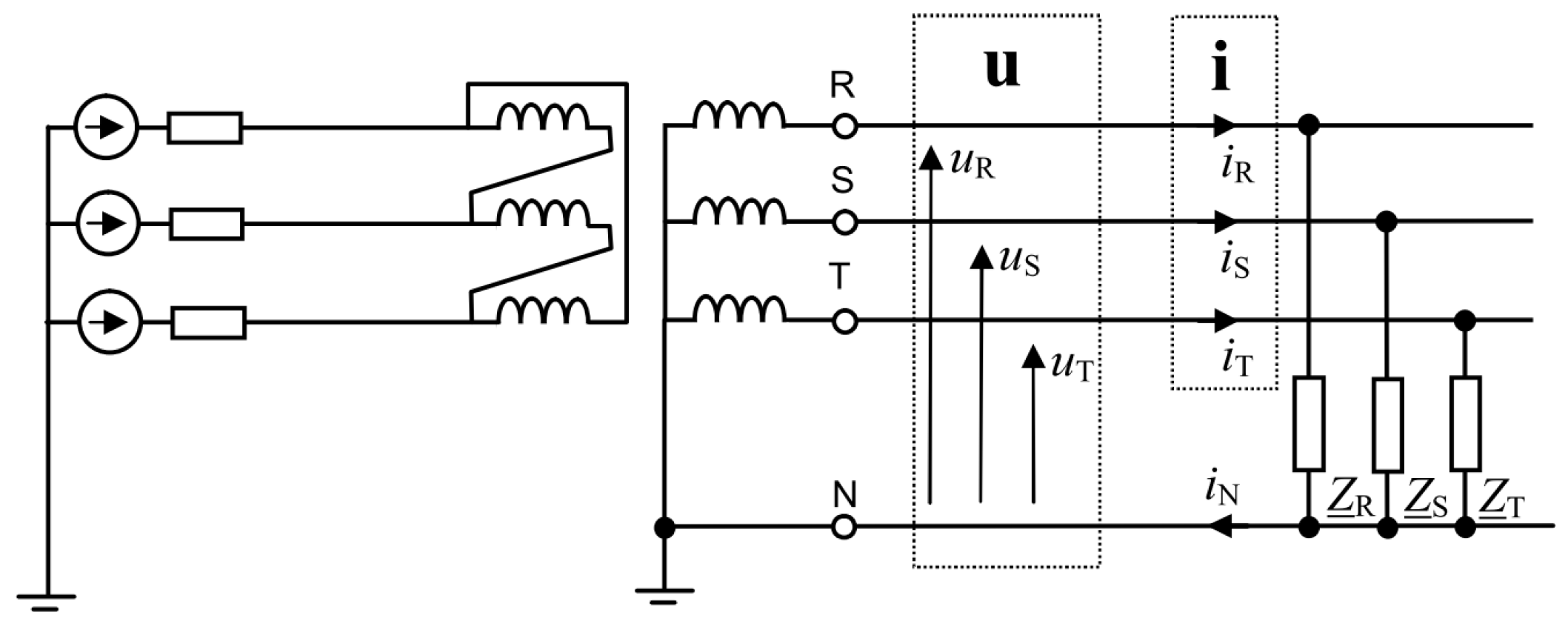
2. Decomposition into Three-Phase Voltages’ Physical Components

The only possibility to reduce the scattered component is to change the load supply conditions by adding a reactive compensator connected in series with the load. To do this, the voltage distribution should be presented analogously to the CPC theory [5,6,11].

In the case of non-sinusoidal periodic waveforms, the three-phase current and voltage quantities can be represented as the sum of individual harmonics

$$\begin{gathered} \mathbf{u}={\displaystyle \sum _n{\mathbf{u}}_{\left(n\right)}}=\sqrt{2}·\Re e{\displaystyle \sum _n{\underset{¯}{\mathbf{U}}}_{\left(n\right)}}·e^{jn{\omega }_{1}t}, \\ \mathbf{i}={\displaystyle \sum _n{\mathbf{i}}_{\left(n\right)}}=\sqrt{2}·\Re e{\displaystyle \sum _n{\underset{¯}{\mathbf{I}}}_{\left(n\right)}}·e^{jn{\omega }_{1}t}. \end{gathered}$$

(1)

This means that the vectors of currents and voltages for harmonic waveforms can be defined as follows:

$${\mathbf{u}}_{\left(n\right)}\stackrel{\mathrm{df}}{=}\left[\begin{array}{c}{u}_{\mathrm{R}\left(n\right)}\\ u_{\mathrm{S}\left(n\right)}\\ u_{\mathrm{T}\left(n\right)}\end{array}\right], {\underset{¯}{\mathbf{U}}}_{\left(n\right)}\stackrel{\mathrm{df}}{=}\left[\begin{array}{c}{\underset{¯}{U}}_{\mathrm{R}\left(n\right)}\\ {\underset{¯}{U}}_{\mathrm{S}\left(n\right)}\\ {\underset{¯}{U}}_{\mathrm{T}\left(n\right)}\end{array}\right],$$

(2)

$${\mathbf{i}}_{\left(n\right)}\stackrel{\mathrm{df}}{=}\left[\begin{array}{c}{i}_{\mathrm{R}\left(n\right)}\\ i_{\mathrm{S}\left(n\right)}\\ i_{\mathrm{T}\left(n\right)}\end{array}\right], {\underset{¯}{\mathbf{I}}}_{\left(n\right)}\stackrel{\mathrm{df}}{=}\left[\begin{array}{c}{\underset{¯}{I}}_{\mathrm{R}\left(n\right)}\\ {\underset{¯}{I}}_{\mathrm{S}\left(n\right)}\\ {\underset{¯}{I}}_{\mathrm{T}\left(n\right)}\end{array}\right].$$

(3)

Across the transformer (Figure 1) are transferred voltage harmonics with positive and negative sequences. Symmetrical harmonics of zero sequences are not transferred from the source to the secondary side of the transformer. Such harmonics will only appear on the secondary side of the transformer when zero sequence harmonics have been generated by the load.

The three-phase load (Figure 1) can be decomposed into individual components responsible for various physical phenomena occurring in this circuit. Analogously to the CPC theory [22], the three-phase vector (1) of voltage u will be decomposed into voltage components which, in the physical sense, will reflect the behavior of the system on the impact of individual phenomena.

Active component of three-phase vector of voltage u_a in the physical sense is a part of the supply voltage which, with unchanged supply current, causes unchanged active power on a three-phase resistive load. It is the most important component that describes the influence of active power on the electrical quantities supplying the load. Analogous to the equivalent conductance G_e in the CPC theory, in the voltage distribution, the equivalent resistance R_e is defined for the n-th harmonic in terms of the useful active power P at three-phase current i.

$$R_{e\left(n\right)}\stackrel{\mathrm{df}}{=}\frac{P_{\left(n\right)}}{{‖{\mathbf{i}}_{\left(n\right)}‖}^2}=\frac{1}{m}\left(R_{\mathrm{R}\left(n\right)}+R_{\mathrm{S}\left(n\right)}+R_{\mathrm{T}\left(n\right)}\right).$$

(4)

where m—multiple of active phases, e.g., for a single-phase load connected to the R phase of a three-phase network will be R_e(n) = R_R(n). If there is no resistance in a given phase, insert zero.

The equivalent resistance R_e(n) is related to the voltage component, which for the n-th harmonic is:

$${\mathbf{u}}_{\mathrm{c}\left(n\right)}=R_{\mathrm{e}\left(n\right)}·{\mathbf{i}}_{\left(n\right)}=\sqrt{2}\Re e\left\{R_{\mathrm{e}\left(n\right)}·{\underset{¯}{\mathbf{I}}}_{\left(n\right)}·e^{jn{\omega }_{1}t}\right\}.$$

(5)

The total impact of all harmonics affects the u_c component, the resultant value of which is:

$${\mathbf{u}}_{\mathrm{c}}={\displaystyle \sum _n{\mathbf{u}}_{\mathrm{c}\left(n\right)}}=\sqrt{2}\Re e{\displaystyle \sum _n{R}_{\mathrm{e}\left(n\right)}{\underset{¯}{\mathbf{I}}}_{\left(n\right)}}{e}^{jn{\omega }_{1}t}.$$

(6)

The resistance R_e(n) may vary with the order of the harmonic, in which case a scattered component of voltage will appear. A change in the R_e(n) value means a change in the real part of the equivalent impedance of the load. Such changes in the series connection of resistance and reactance cannot be observed, but when the resistance and reactance are connected in a parallel or mixed way, it is possible. The constant component, which is independent of the order of the harmonic, depends on the resultant active power and the value of the equivalent resistance R_e.

$$R_{\mathrm{e}}\stackrel{\mathrm{df}}{=}\frac{P}{{‖\mathbf{i}‖}^2}.$$

(7)

Let us define unit three-phase vectors of the positive, negative, and zero sequence

$$1^{\mathrm{p}}\stackrel{\mathrm{df}}{=}\left[\begin{array}{c}1\\ {\alpha }^{*}\\ \alpha \end{array}\right],\hspace{1em}{1}^{\mathrm{n}}\stackrel{\mathrm{df}}{=}\left[\begin{array}{c}1\\ \alpha \\ {\alpha }^{*}\end{array}\right],\hspace{1em}{1}^{\mathrm{z}}\stackrel{\mathrm{df}}{=}\left[\begin{array}{c}1\\ 1\\ 1\end{array}\right],\hspace{1em}\alpha =1e^{j\frac{2\pi }{3}},\hspace{1em}{\alpha }^{*}=1e^{-j\frac{2\pi }{3}}.$$

(8)

In the four-wire system, in the absence of symmetry, the currents in the phase conductors for the n-th harmonic are described by symmetrical components, which can be determined from the relationship:

$$\left[\begin{array}{c}{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{z}}\\ {\underset{¯}{I}}_{\left(n\right)}^{\mathrm{p}}\\ {\underset{¯}{I}}_{\left(n\right)}^{\mathrm{n}}\end{array}\right]=\frac{1}{3}\left[\begin{array}{ccc}1& 1& 1\\ 1& \alpha & {\alpha }^{*}\\ 1& {\alpha }^{*}& \alpha \end{array}\right]·\left[\begin{array}{l}{\underset{¯}{I}}_{\mathrm{R}\left(n\right)}\hfill \\ {\underset{¯}{I}}_{\mathrm{S}\left(n\right)}\hfill \\ {\underset{¯}{I}}_{\mathrm{T}\left(n\right)}\hfill \end{array}\right].$$

(9)

The active component of the voltage and its RMS value is:

$$\begin{gathered} {\mathbf{u}}_{\mathrm{a}}=\sqrt{2}\Re e{\displaystyle \sum _n{R}_{\mathrm{e}}{\underset{¯}{\mathbf{I}}}_{\left(n\right)}{e}^{jn{\omega }_{1}t}}=\sqrt{2}\Re e{\displaystyle \sum _n{R}_{\mathrm{e}}\left(1^{\mathrm{p}}{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{p}}+1^{\mathrm{n}}{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{n}}+1^{\mathrm{z}}{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{z}}\right)e^{jn{\omega }_{1}t}}, \\ ‖{\mathbf{u}}_{\mathrm{a}}‖=\frac{P}{‖\mathbf{i}‖}. \end{gathered}$$

(10)

The remaining component in u_c, which reflects changes in the equivalent resistance R_e(n) with the order of the harmonic, should be defined as the scattered component of voltage, which is:

$$\begin{array}{cc}\hfill {\mathbf{u}}_{\mathrm{s}}={\mathbf{u}}_{\mathrm{c}}-{\mathbf{u}}_{\mathrm{a}}& =\sqrt{2}\Re e{\displaystyle \sum _n{R}_{\mathrm{e}\left(n\right)}{\underset{¯}{\mathbf{I}}}_{\left(n\right)}{e}^{jn{\omega }_{1}t}}-\sqrt{2}\Re e{\displaystyle \sum _n{R}_{\mathrm{e}}{\underset{¯}{\mathbf{I}}}_{\left(n\right)}{e}^{jn{\omega }_{1}t}}=\hfill \\ & =\sqrt{2}\Re e{\displaystyle \sum _n\left(R_{\mathrm{e}\left(n\right)}-R_e\right)}·\left(1^{\mathrm{p}}{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{p}}+1^{\mathrm{n}}{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{n}}+1^{\mathrm{z}}{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{z}}\right)e^{jn{\omega }_{1}t}.\hfill \end{array}$$

(11)

The RMS value of the scattered component is:

$$‖{\mathbf{u}}_{\mathrm{s}}‖=\sqrt{{\displaystyle \sum _n{\left(R_{\mathrm{e}\left(n\right)}-R_{\mathrm{e}}\right)}^2{‖{\mathbf{i}}_{\left(n\right)}‖}^2}}.$$

(12)

The reactive nature of the circuit is described by reactive components. Knowing the reactance of the receiver, the equivalent reactance can be determined, which is:

$$X_{\mathrm{e}\left(n\right)}\stackrel{\mathrm{df}}{=}\frac{Q_{\left(n\right)}}{{‖{\mathbf{i}}_{\left(n\right)}‖}^2}=\frac{1}{m}\left(X_{\mathrm{R}\left(n\right)}+X_{\mathrm{S}\left(n\right)}+X_{\mathrm{T}\left(n\right)}\right).$$

(13)

From dependencies (4) and (13), the formula for the equivalent impedance is:

$${\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\stackrel{\mathrm{df}}{=}{R}_{\mathrm{e}\left(n\right)}+j{X}_{\mathrm{e}\left(n\right)}=\frac{1}{m}\left({\underset{¯}{Z}}_{\mathrm{R}\left(n\right)}+{\underset{¯}{Z}}_{\mathrm{S}\left(n\right)}+{\underset{¯}{Z}}_{\mathrm{T}\left(n\right)}\right).$$

(14)

The equivalent reactance (13) is associated with a component consistent with the phase sequence of the power source, which can be called the reactive component of voltage u_r:

$${\mathbf{u}}_{\mathrm{r}\left(n\right)}=\sqrt{2}\Re e\left\{j{X}_{\mathrm{e}\left(n\right)}·\left(1^{\mathrm{p}}{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{p}}+1^{\mathrm{n}}{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{n}}+1^{\mathrm{z}}{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{z}}\right)·e^{jn{\omega }_{1}t}\right\}.$$

(15)

The RMS value of the reactive component is:

$$‖{\mathbf{u}}_{\mathrm{r}}‖=\sqrt{{\displaystyle \sum _n{X}_{\mathrm{e}\left(n\right)}^2{‖{\mathbf{i}}_{\left(n\right)}‖}^2}}.$$

(16)

When the load is unbalanced, the n-th harmonic of the voltage additionally contains an unbalanced component, which is:

$${\mathbf{u}}_{\mathrm{u}\left(n\right)}={\mathbf{u}}_{\left(n\right)}-{\mathbf{u}}_{\mathrm{c}\left(n\right)}-{\mathbf{u}}_{\mathrm{r}\left(n\right)}.$$

(17)

The unbalanced component of voltage u_u (17) is asymmetric, so according to the method of symmetrical components it can be represented as a sum of vectors of symmetrical components with the following sequence: positive, negative, and zero, respectively:

$${\mathbf{u}}_{\mathrm{u}\left(n\right)}={\mathbf{u}}_{\mathrm{u}\left(n\right)}^{\mathrm{p}}+{\mathbf{u}}_{\mathrm{u}\left(n\right)}^{\mathrm{n}}+{\mathbf{u}}_{\mathrm{u}\left(n\right)}^{\mathrm{z}}.$$

(18)

From dependencies (17), (6), and (15), we obtain:

$$\begin{array}{cc}\hfill {\mathbf{u}}_{\mathrm{u}\left(n\right)}={\mathbf{u}}_{\left(n\right)}& -{\mathbf{u}}_{\mathrm{c}\left(n\right)}-{\mathbf{u}}_{\mathrm{r}\left(n\right)}=\hfill \\ & =\sqrt{2}\Re e\left\{{\mathbf{u}}_{\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\left(1^{\mathrm{p}}{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{p}}+1^{\mathrm{n}}{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{n}}+1^{\mathrm{z}}{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{z}}\right)·e^{jn{\omega }_{1}t}\right\}.\hfill \end{array}$$

(19)

The unbalanced component of voltage can be expressed by quantities describing a three-phase load. For a symmetric component with a positive sequence, it will be:

$${\mathbf{u}}_{\mathrm{u}\left(n\right)}^{\mathrm{p}}=\sqrt{2}\Re e\left\{\left[\begin{array}{l}\left({\underset{¯}{Z}}_{\mathrm{R}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\right)\hfill \\ \left({\underset{¯}{Z}}_{\mathrm{S}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\right)·{\alpha }^{*}\hfill \\ \left({\underset{¯}{Z}}_{\mathrm{T}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\right)·\alpha \hfill \end{array}\right]·{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{p}}{e}^{jn{\omega }_{1}t}\right\}$$

(20)

For a symmetric component with a negative sequence, it will be:

$${\mathbf{u}}_{\mathrm{u}\left(n\right)}^{\mathrm{n}}=\sqrt{2}\Re e\left\{\left[\begin{array}{l}\left({\underset{¯}{Z}}_{\mathrm{R}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\right)\hfill \\ \left({\underset{¯}{Z}}_{\mathrm{S}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\right)·\alpha \hfill \\ \left({\underset{¯}{Z}}_{\mathrm{T}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\right)·{\alpha }^{*}\hfill \end{array}\right]·{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{n}}{e}^{jn{\omega }_{1}t}\right\}$$

(21)

while for a symmetric component with zero sequence it will be:

$${\mathbf{u}}_{\mathrm{u}\left(n\right)}^{\mathrm{z}}=\sqrt{2}\Re e\left\{\left[\begin{array}{l}{\underset{¯}{Z}}_{\mathrm{R}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\hfill \\ {\underset{¯}{Z}}_{\mathrm{S}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\hfill \\ {\underset{¯}{Z}}_{\mathrm{T}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\hfill \end{array}\right]·{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{z}}{e}^{jn{\omega }_{1}t}\right\}$$

(22)

These vectors are mutually orthogonal, so for RMS values the following is correct:

$${‖{\mathbf{u}}_{\mathrm{u}\left(n\right)}‖}^2={‖{\mathbf{u}}_{\mathrm{u}\left(n\right)}^{\mathrm{p}}‖}^2+{‖{\mathbf{u}}_{\mathrm{u}\left(n\right)}^{\mathrm{n}}‖}^2+{‖{\mathbf{u}}_{\mathrm{u}\left(n\right)}^{\mathrm{z}}‖}^2.$$

(23)

In addition, analogously to (9), one can write:

$$\left[\begin{array}{c}{\underset{¯}{U}}_{\mathrm{u}\left(n\right)}^{\mathrm{z}}\\ {\underset{¯}{U}}_{\mathrm{u}\left(n\right)}^{\mathrm{p}}\\ {\underset{¯}{U}}_{\mathrm{u}\left(n\right)}^{\mathrm{n}}\end{array}\right]=\frac{1}{3}\left[\begin{array}{ccc}1& 1& 1\\ 1& \alpha & {\alpha }^{*}\\ 1& {\alpha }^{*}& \alpha \end{array}\right]·\left[\begin{array}{l}{\underset{¯}{U}}_{\mathrm{R}\mathrm{u}\left(n\right)}\hfill \\ {\underset{¯}{U}}_{\mathrm{S}\mathrm{u}\left(n\right)}\hfill \\ {\underset{¯}{U}}_{\mathrm{T}\mathrm{u}\left(n\right)}\hfill \end{array}\right]$$

(24)

In a three-phase circuit, harmonics can be considered in terms of symmetrical components. This means that for harmonics in a positive sequence, one gets:

$$\begin{array}{c}\left[\begin{array}{c}{\underset{¯}{U}}_{\mathrm{u}\left(n\right)}^{\mathrm{z}}\\ {\underset{¯}{U}}_{\mathrm{u}\left(n\right)}^{\mathrm{p}}\\ {\underset{¯}{U}}_{\mathrm{u}\left(n\right)}^{\mathrm{n}}\end{array}\right]=\frac{1}{3}\left[\begin{array}{ccc}1& 1& 1\\ 1& \alpha & {\alpha }^{*}\\ 1& {\alpha }^{*}& \alpha \end{array}\right]·\left[\begin{array}{l}\left({\underset{¯}{Z}}_{\mathrm{R}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\right)\hfill \\ \left({\underset{¯}{Z}}_{\mathrm{S}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\right)·{\alpha }^{*}\hfill \\ \left({\underset{¯}{Z}}_{\mathrm{T}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\right)·\alpha \hfill \end{array}\right]·{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{p}}=\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hfill \\ \hfill \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}=\frac{1}{3}\left[\begin{array}{c}{\underset{¯}{Z}}_{\mathrm{R}\left(n\right)}+{\alpha }^{*}{\underset{¯}{Z}}_{\mathrm{S}\left(n\right)}+\alpha {\underset{¯}{Z}}_{\mathrm{T}\left(n\right)}\\ 0\\ {\underset{¯}{Z}}_{\mathrm{R}\left(n\right)}+\alpha {\underset{¯}{Z}}_{\mathrm{S}\left(n\right)}+{\alpha }^{*}{\underset{¯}{Z}}_{\mathrm{T}\left(n\right)}\end{array}\right]·{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{p}}=\left[\begin{array}{c}{\underset{¯}{Z}}_{\mathrm{u}\left(n\right)}^{\mathrm{z}}\\ 0\\ {\underset{¯}{Z}}_{\mathrm{u}\left(n\right)}^{\mathrm{n}}\end{array}\right]·{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{p}},\end{array}$$

(25)

where ${\underset{¯}{Z}}_{\mathrm{u}\left(n\right)}$—the unbalanced impedance {XE “impedancja niezrównoważenia”}.

For harmonics in negative sequence, one gets:

$$\begin{array}{c}\left[\begin{array}{c}{\underset{¯}{U}}_{\mathrm{u}\left(n\right)}^{\mathrm{z}}\\ {\underset{¯}{U}}_{\mathrm{u}\left(n\right)}^{\mathrm{p}}\\ {\underset{¯}{U}}_{\mathrm{u}\left(n\right)}^{\mathrm{n}}\end{array}\right]=\frac{1}{3}\left[\begin{array}{ccc}1& 1& 1\\ 1& \alpha & {\alpha }^{*}\\ 1& {\alpha }^{*}& \alpha \end{array}\right]·\left[\begin{array}{l}\left({\underset{¯}{Z}}_{\mathrm{R}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\right)\hfill \\ \left({\underset{¯}{Z}}_{\mathrm{S}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\right)·\alpha \hfill \\ \left({\underset{¯}{Z}}_{\mathrm{T}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\right)·{\alpha }^{*}\hfill \end{array}\right]·{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{n}}=\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hfill \\ \hfill \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}=\frac{1}{3}\left[\begin{array}{c}{\underset{¯}{Z}}_{\mathrm{R}\left(n\right)}+\alpha {\underset{¯}{Z}}_{\mathrm{S}\left(n\right)}+{\alpha }^{*}{\underset{¯}{Z}}_{\mathrm{T}\left(n\right)}\\ {\underset{¯}{Z}}_{\mathrm{R}\left(n\right)}+{\alpha }^{*}{\underset{¯}{Z}}_{\mathrm{S}\left(n\right)}+\alpha {\underset{¯}{Z}}_{\mathrm{T}\left(n\right)}\\ 0\end{array}\right]·{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{n}}=\left[\begin{array}{c}{\underset{¯}{Z}}_{\mathrm{u}\left(n\right)}^{\mathrm{z}}\\ {\underset{¯}{Z}}_{\mathrm{u}\left(n\right)}^{\mathrm{p}}\\ 0\end{array}\right]·{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{n}},\end{array}$$

(26)

while for harmonics in zero sequence we get:

$$\begin{array}{c}\left[\begin{array}{c}{\underset{¯}{U}}_{\mathrm{u}\left(n\right)}^{\mathrm{z}}\\ {\underset{¯}{U}}_{\mathrm{u}\left(n\right)}^{\mathrm{p}}\\ {\underset{¯}{U}}_{\mathrm{u}\left(n\right)}^{\mathrm{n}}\end{array}\right]=\frac{1}{3}\left[\begin{array}{ccc}1& 1& 1\\ 1& \alpha & {\alpha }^{*}\\ 1& {\alpha }^{*}& \alpha \end{array}\right]·\left[\begin{array}{l}{\underset{¯}{Z}}_{\mathrm{R}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\hfill \\ {\underset{¯}{Z}}_{\mathrm{S}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\hfill \\ {\underset{¯}{Z}}_{\mathrm{T}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\hfill \end{array}\right]·{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{z}}=\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hfill \\ \hfill \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}=\frac{1}{3}\left[\begin{array}{c}0\\ {\underset{¯}{Z}}_{\mathrm{R}\left(n\right)}+\alpha {\underset{¯}{Z}}_{\mathrm{S}\left(n\right)}+{\alpha }^{*}{\underset{¯}{Z}}_{\mathrm{T}\left(n\right)}\\ {\underset{¯}{Z}}_{\mathrm{R}\left(n\right)}+{\alpha }^{*}{\underset{¯}{Z}}_{\mathrm{S}\left(n\right)}+\alpha {\underset{¯}{Z}}_{\mathrm{T}\left(n\right)}\end{array}\right]·{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{z}}=\left[\begin{array}{c}0\\ {\underset{¯}{Z}}_{\mathrm{u}\left(n\right)}^{\mathrm{p}}\\ {\underset{¯}{Z}}_{\mathrm{u}\left(n\right)}^{\mathrm{n}}\end{array}\right]·{\underset{¯}{I}}_{\left(n\right)}^{\mathrm{z}}.\end{array}$$

(27)

From these equations, it can be seen that the unbalanced impedance Z_u(n) has a different notation in each symmetrical component. In order to standardize the notation, it is convenient to define the generalized complex rotation coefficient β_(n)

$${\beta }_{\left(n\right)}\stackrel{\mathrm{df}}{=}{\left({\alpha }^{*}\right)}^n=1e^{-j\frac{2\pi ·n}{3}}=\left\{\begin{array}{cc}{\alpha }^{*},& \mathrm{dla} n=3k+1\\ \alpha ,& \mathrm{dla} n=3k+2\\ 1,& \mathrm{dla} n=3k+3\end{array}\right.$$

(28)

Using the coefficient (28), the unbalanced impedance distributed to symmetrical components in the universal notation will be:

$$\left[\begin{array}{c}{\underset{¯}{Z}}_{\mathrm{u}\left(n\right)}^{\mathrm{z}}\\ {\underset{¯}{Z}}_{\mathrm{u}\left(n\right)}^{\mathrm{p}}\\ {\underset{¯}{Z}}_{\mathrm{u}\left(n\right)}^{\mathrm{n}}\end{array}\right]=\frac{1}{3}\left[\begin{array}{c}{\underset{¯}{Z}}_{\mathrm{R}\left(n\right)}+{\beta }_{\left(n\right)}{\underset{¯}{Z}}_{\mathrm{S}\left(n\right)}+{\beta }_{\left(n\right)}^{*}{\underset{¯}{Z}}_{\mathrm{T}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\left(1+{\beta }_{\left(n\right)}+{\beta }_{\left(n\right)}^{*}\right)\\ {\underset{¯}{Z}}_{\mathrm{R}\left(n\right)}+{\beta }_{\left(n-1\right)}{\underset{¯}{Z}}_{\mathrm{S}\left(n\right)}+{\beta }_{\left(n-1\right)}^{*}{\underset{¯}{Z}}_{\mathrm{T}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\left(1+{\beta }_{\left(n-1\right)}+{\beta }_{\left(n-1\right)}^{*}\right)\\ {\underset{¯}{Z}}_{\mathrm{R}\left(n\right)}+{\beta }_{\left(n+1\right)}{\underset{¯}{Z}}_{\mathrm{S}\left(n\right)}+{\beta }_{\left(n+1\right)}^{*}{\underset{¯}{Z}}_{\mathrm{T}\left(n\right)}-{\underset{¯}{Z}}_{\mathrm{e}\left(n\right)}\left(1+{\beta }_{\left(n+1\right)}+{\beta }_{\left(n+1\right)}^{*}\right)\end{array}\right]$$

(29)

The RMS value of the unbalanced component of voltage is:

$$‖{\mathbf{u}}_{\mathrm{u}}‖=\sqrt{{\displaystyle \sum _n{‖{\mathbf{u}}_{\mathrm{u}\left(n\right)}‖}^2}}=\sqrt{{\displaystyle \sum _n\left({‖{\mathbf{u}}_{\mathrm{u}\left(n\right)}^{\mathrm{p}}‖}^2+{‖{\mathbf{u}}_{\mathrm{u}\left(n\right)}^{\mathrm{n}}‖}^2+{‖{\mathbf{u}}_{\mathrm{u}\left(n\right)}^{\mathrm{z}}‖}^2\right)}}.$$

(30)

The determined components of voltage are orthogonal, so there is a relationship between the RMS values that can be presented mathematically and graphically (Figure 2):

$${‖\mathbf{u}‖}^2={‖{\mathbf{u}}_{\mathrm{a}}‖}^2+{‖{\mathbf{u}}_{\mathrm{s}}‖}^2+{‖{\mathbf{u}}_{\mathrm{r}}‖}^2+{‖{\mathbf{u}}_{\mathrm{u}}‖}^2,$$

(31)

Thus, the RMS value of the unbalanced component of the voltage is:

$$‖{\mathbf{u}}_{\mathrm{u}}‖=\sqrt{{‖\mathbf{u}‖}^2-{‖{\mathbf{u}}_{\mathrm{a}}‖}^2-{‖{\mathbf{u}}_{\mathrm{s}}‖}^2-{‖{\mathbf{u}}_{\mathrm{r}}‖}^2}.$$

(32)

The supply voltage has been decomposed into components describing the physical properties of the circuit analogously to the CPC theory according to the relationship:

$$\mathbf{u}={\mathbf{u}}_{\mathrm{a}}+{\mathbf{u}}_{\mathrm{s}}+{\mathbf{u}}_{\mathrm{r}}+{\mathbf{u}}_{\mathrm{u}},\hspace{1em}\mathrm{where}\hspace{1em}{\mathbf{u}}_{\mathrm{u}}={\mathbf{u}}_{\mathrm{u}}^{\mathrm{p}}+{\mathbf{u}}_{\mathrm{u}}^{\mathrm{n}}+{\mathbf{u}}_{\mathrm{u}}^{\mathrm{z}}.$$

(33)

The power decomposition corresponds to the voltage decomposition (33), i.e., the apparent power S is presented as the resultant of active power P, reactive power Q_i, scattered power D_si, and unbalanced power D_ui.

$$S^2=P^2+Q_{\mathrm{i}}^2+D_{\mathrm{si}}^2+D_{\mathrm{ui}}^2.$$

(34)

The unbalanced power in a four-wire system is additionally interpreted as the resultant of three symmetrical powers:

$$D_{\mathrm{ui}}^2=D_{\mathrm{ui}}^{\mathrm{p}2}+D_{\mathrm{ui}}^{\mathrm{n}2}+D_{\mathrm{ui}}^{\mathrm{z}2},$$

(35)

whose values are determined from:

$$D_{\mathrm{u}\mathrm{i}}^{}=‖{\mathbf{u}}_{\mathrm{u}}‖·‖\mathbf{i}‖, D_{\mathrm{u}\mathrm{i}}^{\mathrm{p}}=‖{\mathbf{u}}_{\mathrm{u}}^{\mathrm{p}}‖·‖\mathbf{i}‖, D_{\mathrm{u}\mathrm{i}}^{\mathrm{n}}=‖{\mathbf{u}}_{\mathrm{u}}^{\mathrm{n}}‖·‖\mathbf{i}‖, D_{\mathrm{u}\mathrm{i}}^{\mathrm{z}}=‖{\mathbf{u}}_{\mathrm{u}}^{\mathrm{z}}‖·‖\mathbf{i}‖.$$

(36)

The reactive Q_i, scattered D_si, and unbalanced power D_ui were defined for voltage decomposition into individual components. The values of these powers are different from the values determined in the CPC theory:

$$Q_{\mathrm{i}}\ne Q,\hspace{1em}{D}_{\mathrm{si}}\ne D_{\mathrm{s}},\hspace{1em}{D}_{\mathrm{ui}}\ne D_{\mathrm{u}}.$$

(37)

The determined voltage components can be presented graphically (Figure 3).

The physical components of the voltage are related to the characteristic physical phenomena that can be dimensioned thanks to measurements at the load terminals. It should be emphasized here that these components do not exist physically—they are only the result of mathematical decomposition. So, they are more mathematical than physical quantities.

3. Numerical Illustration

The inductive load (Figure 4) was connected to a symmetrical three-phase source with parameters $u_{\mathrm{R}}=\sqrt{2}·\Re e\left\{230e^{j{\omega }_{1}t}+10e^{j5{\omega }_{1}t}\right. \mathrm{V}$, ω₁ = 2π50 rad/s. The circuit topology and numerical values were adopted in accordance with the calculation example presented in [36].

This receiver can be described as follows (

Table 1. Impedances and current.

n	ZR(n) [Ω]	Ze(n) [Ω]	I(n) [A]
1	1 + j1	1 + j1	115 − j115
5	1 + j5	1 + j5	$5/13-j25/13$

):

Active power is taken only in one phase and it is equal:

$$P=P_{\mathrm{R}}={\displaystyle \sum _n\Re e\left\{{\underset{¯}{Z}}_{\mathrm{R}\left(n\right)}\right\}·I_{\mathrm{R}\left(n\right)}^2}=26454 \mathrm{W}.$$

The current flows only in phase R, so the value of the three-phase current vector is:

$$‖\mathbf{i}‖=\sqrt{{\displaystyle \sum _n{‖{\mathbf{i}}_{\left(n\right)}^{}‖}^2}}=\sqrt{{\displaystyle \sum _n{I}_{\mathrm{R}\left(n\right)}^2}}=162.646 \mathrm{A}.$$

Thus, according to (7), the value of the equivalent resistance is:

$$R_{\mathrm{e}}=\frac{P}{{‖\mathbf{i}‖}^2}=1 \Omega$$

and this is consistent with the value of the equivalent resistance determined in a single-phase circuit.

The RMS values of the individual three-phase voltage components are equal to:

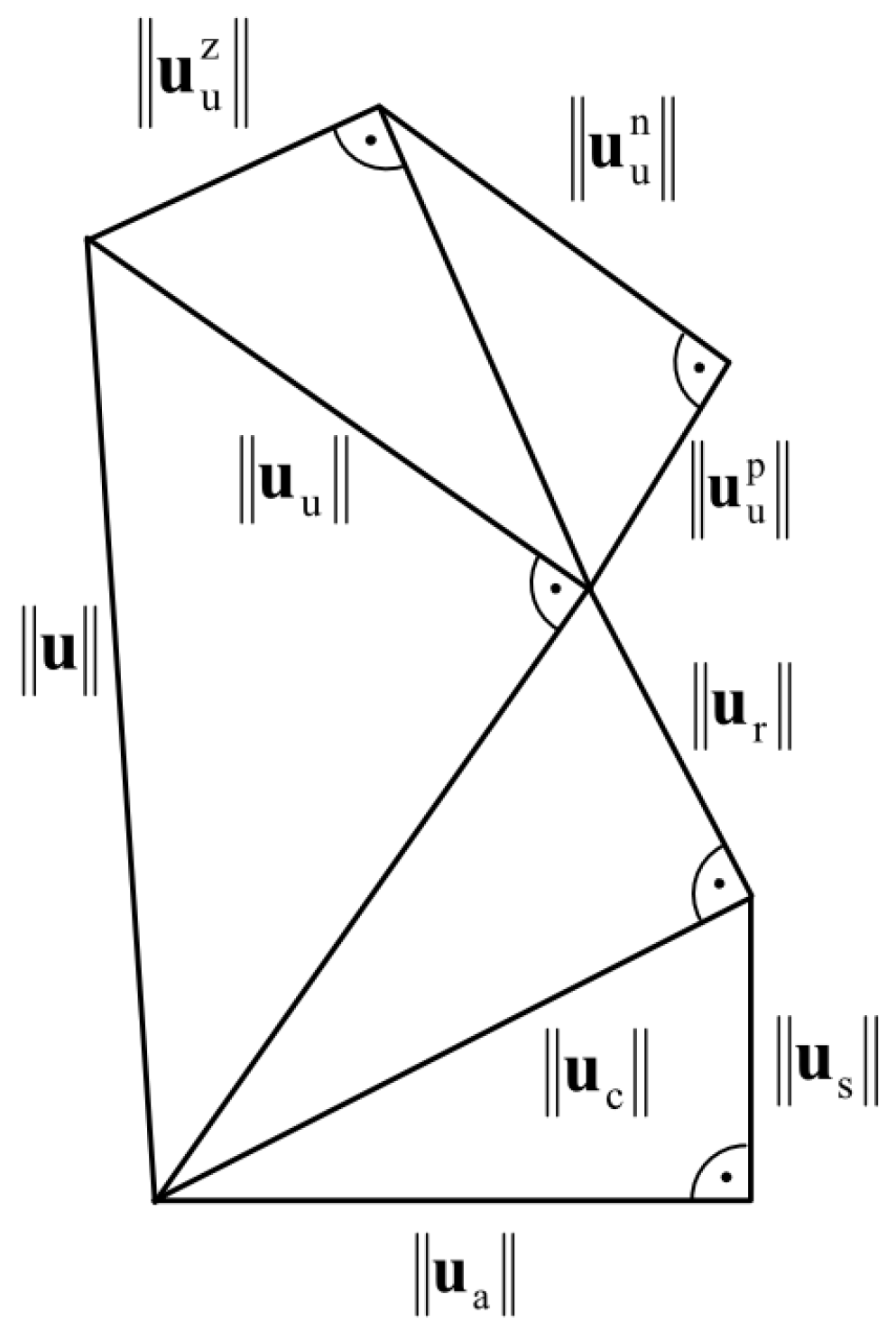
• The three-phase active voltage physical components (10).

  $$‖{\mathbf{u}}_{\mathrm{a}}‖=\raisebox{1ex}{$P$}\!\left/ \!\raisebox{-1ex}{$‖\mathbf{i}‖$}\right.=162.646 \mathrm{V}.$$
• The scattered component u_s in this example is equal to zero because, according to (12), there is no dispersion of the resistance R_e(n) around the equivalent resistance R_e for all harmonics and all phases.
• The three-phase reactive voltage physical components (16):

  $$‖{\mathbf{u}}_{\mathrm{r}}‖=\sqrt{{\displaystyle \sum _n{X}_{\mathrm{e}\left(n\right)}^2{‖{\mathbf{i}}_{\left(n\right)}‖}^2}}=162.930 \mathrm{V}.$$
• The three-phase unbalanced voltage physical components (32):

  $$‖{\mathbf{u}}_{\mathrm{u}}‖=\sqrt{{‖\mathbf{u}‖}^2-{‖{\mathbf{u}}_{\mathrm{a}}‖}^2-{‖{\mathbf{u}}_{\mathrm{s}}‖}^2-{‖{\mathbf{u}}_{\mathrm{r}}‖}^2}=\sqrt{{398.748}^2-{162.646}^2-0-{162.930}^2}=325.576 \mathrm{V}.$$

In this case, the non-zero value of the unbalanced voltage results from the lack of load symmetry and means that $u_{\mathrm{u}\mathrm{R}}=0 \mathrm{V}$,

$$\begin{gathered} u_{\mathrm{u}\mathrm{S}}=‖u_{\mathrm{S}}‖=\sqrt{{230}^2+{10}^2}=230.217 \mathrm{V}, \\ {u}_{\mathrm{u}\mathrm{T}}=‖u_{\mathrm{T}}‖=\sqrt{{230}^2+{10}^2}=230.217 \mathrm{V}. \end{gathered}$$

This is due to Kirchhoff’s voltage law, which in this case means that the entire phase’s voltage u_S and u_T translates into the value of u_uS and u_uT. From (24), we get:

$$‖{\mathbf{u}}_{\mathrm{u}}^{\mathrm{z}}‖=153.478 \mathrm{V},\hspace{1em}‖{\mathbf{u}}_{\mathrm{u}}^{\mathrm{p}}‖=‖{\mathbf{u}}_{\mathrm{u}}^{\mathrm{n}}‖=76.739 \mathrm{V}.$$

The values of power are, respectively:

• $P=26454 \mathrm{W},$
• $Q_i=\sqrt{{\displaystyle \sum _n{X}_{\mathrm{e}\left(n\right)}^2{‖{\mathbf{i}}_{\left(n\right)}‖}^4}}=26450 \mathrm{var},$
• $D_{\mathrm{si}}=0 \mathrm{VA},$
• $D_{\mathrm{u}\mathrm{i}}^{\mathrm{p}}=‖{\mathbf{u}}_{\mathrm{u}}^{\mathrm{p}}‖·‖\mathbf{i}‖=12481 \mathrm{VA},$
• $D_{\mathrm{u}\mathrm{i}}^{\mathrm{n}}=‖{\mathbf{u}}_{\mathrm{u}}^{\mathrm{n}}‖·‖\mathbf{i}‖=12481 \mathrm{VA},$
• $D_{\mathrm{u}\mathrm{i}}^{\mathrm{z}}=‖{\mathbf{u}}_{\mathrm{u}}^{\mathrm{z}}‖·‖\mathbf{i}‖=24963 \mathrm{VA},$
• $D_{\mathrm{u}\mathrm{i}}=‖{\mathbf{u}}_{\mathrm{u}}‖·‖\mathbf{i}‖=52954 \mathrm{VA}.$

According to (35), the correctness of power determination can be checked:

$$S=\sqrt{P^2+Q_{\mathrm{i}}^2+D_{\mathrm{si}}^2+D_{\mathrm{ui}}^2}=64854 \mathrm{VA}=‖\mathbf{u}‖·‖\mathbf{i}‖$$

Comparing the obtained results with the values determined in [36], in which the CPC distribution was made, one can conclude the equality of active powers and the inequality of other powers, which was noted in (37).

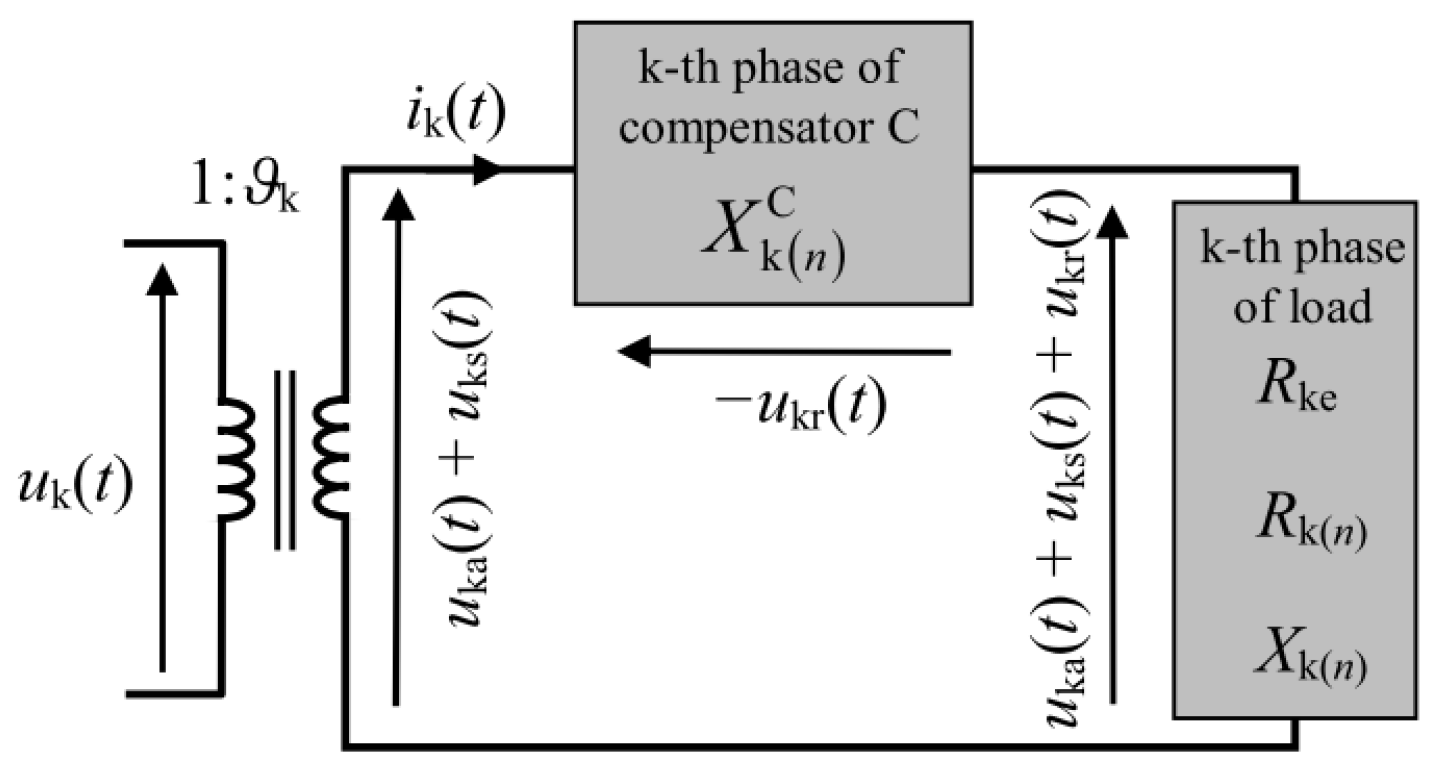
4. The Concept of Compensation of Voltage Components

The classic method of compensation, consisting in the parallel connection of the reactive compensator with the load, makes it possible to improve the power factor λ to unity only when the scattered power D_s is zero.

Reduction in the scattered power in a three-phase system is possible only by connecting a reactive compensator in series with the load (Figure 5). Therefore, the decomposition into voltage components should be carried out and the power D_si should be zeroed. When the load and the compensator are connected in series, the reactive power Q_i is also reduced.

Adding a two-wire series to a current circuit changes the voltage supplying the receiver by the voltage drop on this compensator. Therefore, this compensation method requires control of the load supply voltage in each phase individually.

The use of an ideal compensator, i.e., one that is characterized by zero resistance and non-zero reactance $X_{\mathrm{k}\left(n\right)}^{\mathrm{C}}$, when connected in series with a load with impedance ${\underset{¯}{Z}}_{\mathrm{k}\left(n\right)}=R_{\mathrm{k}\left(n\right)}+j{X}_{\mathrm{k}\left(n\right)}$ where k = R, S, T, causes a change in the load impedance of the source (Figure 6). In general, for all phases, we can write:

$${\underset{¯}{Z}}_{\mathrm{k}\left(n\right)}^{\prime }=R_{\mathrm{k}\left(n\right)}+j\left(X_{\mathrm{k}\left(n\right)}+X_{\mathrm{k}\left(n\right)}^{\mathrm{C}}\right).$$

(38)

After compensation, the resistance R_k(n) and the equivalent resistance R_e do not change. Assuming that the supply current will not change, the active power P and the scattered power D_si will not change. Only the reactive voltage will change to the value:

$$u_{\mathrm{kr}}^{\prime }=\sqrt{2}\Re e{\displaystyle \sum _{n}j\left(X_{\mathrm{k}\left(n\right)}^{\mathrm{C}}+X_{\mathrm{k}\left(n\right)}\right)·{\underset{¯}{I}}_{\mathrm{k}\left(n\right)}{e}^{jn{\omega }_{1}t}}$$

(39)

The reactive component of voltage $u_{\mathrm{kr}}^{\prime }$ reaches zero when the series compensator in each k-th phase, for each n-th harmonic, leads to the equality:

$$X_{\mathrm{k}\left(n\right)}^{\mathrm{C}}+X_{\mathrm{k}\left(n\right)}=0.$$

(40)

This type of compensation causes the source voltages to resonate with the load. The resonance, meaning the zeroing of the reactive component of voltage u_kr, causes an increase in the value of the current flowing through the load, i.e., also an increase in the active power P.

Maintaining the same energy parameters, after compensation as before, requires the use of a transformer stepping down the supply voltage, the ratio of which is:

$${\vartheta }_{\mathrm{k}}=\frac{‖u_{\mathrm{k}}^{\prime }\left(t\right)‖}{‖u_{\mathrm{k}}\left(t\right)‖}=\frac{\sqrt{{‖u_{\mathrm{ka}}\left(t\right)‖}^2+{‖u_{\mathrm{ks}}\left(t\right)‖}^2}}{\sqrt{{‖u_{\mathrm{ka}}\left(t\right)‖}^2+{‖u_{\mathrm{ks}}\left(t\right)‖}^2+{‖u_{\mathrm{kr}}\left(t\right)‖}^2}}$$

(41)

When the non-linear nature of the transformer is neglected, the scattered component will also be zero, as assumed for the load, i.e., D_si = 0. In this case, the total power compensation of Q_i means that the power factor λ becomes equal to 1.

Each stage of the compensator has different functions (Figure 7). Individual stages of the compensator affect the appropriate components. To compensate for the scattered component i_s, the operating point of the system should be changed by adding a series impedance to each phase together with a transformer with an appropriate ratio. The remaining components are compensated with two compensators, Y and Δ [36].

The basic condition for complete compensation is the lack of voltage components in the compensator C: scattered u_s and unbalanced u_u.

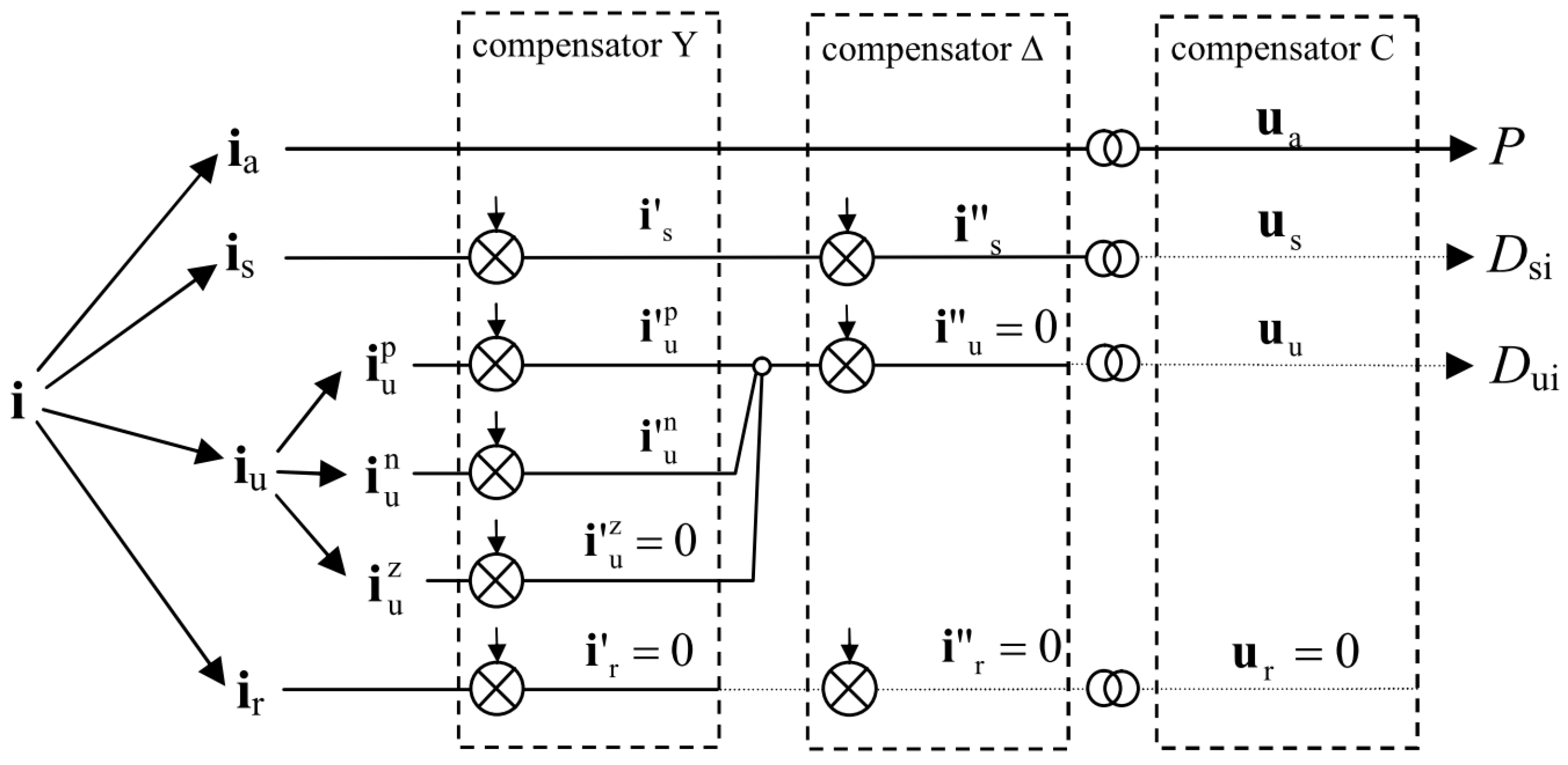
5. Evaluation of the Compensation Method Using the VPC Power Theory

First of all, it is necessary to present the limitations that the VPC method brings to the considerations.

(a)

Unidirectional energy flow in phases.

Equation (4) imposes unidirectionality for the energy flow in each phase. Changing the direction of energy flow in one of the phases relative to the others results in the inability to determine the VPC decomposition.

(b)

Modeling distributed and renewable energies.

If the load will be an electricity producer, the equations should be modified. The presented equations do not take into account voltage sources on the receiving side. To be able to use the VPC power theory in the case of distributed and renewable energy, equations must be developed that take into account voltage sources on the receiving side.

(c)

The use of VPC theory for large distribution networks.

The description of large distribution networks in accordance with the presented VPC theory is possible, but one must remember the remarks presented in items a and b. VPC decomposition for a large passive distribution network is possible; however, the idea of reactive compensation in series connection in phase lines with the flow of large currents is economically unprofitable. The presence of large banks of variable capacitances and inductances may turn out to be less advantageous in terms of economic and weight–size parameters compared to a synchronous electric machine compensator.

(d)

Analysis of the profitability of compensation in a series connection.

In the case of compensation for high currents, the profitability of using reactance in a series connection in individual phases has been discussed in the previous items. In the case of low power, the use of series compensation may be considered. A qualitative comparison of compensation methods is only possible when analyzing a specific circuit. For example, for a single-phase receiver connected to a three-phase network according to the example presented in [36]:

• Before compensation, the power factor is λ = 0.4079.
• The classical method of compensation consisting only of affecting the reactive component i_r = 0 improves the power factor to the value λ = 0.4468.
• As a result of the operation of the two-stage compensator presented in [36], it is possible to zero the unbalanced component i_u = 0, which causes the power factor to reach the value λ = 0.9992.
• Only zeroing the scattered component i_s = 0 gives the power factor λ = 1, but this is only possible as a result of the cooperation of the series compensator.

The last stage of compensation provides the least benefit and is the most expensive element to perform. In the example discussed, there is no economic indication of the profitability of using a series compensator, but thanks to theoretical considerations, it is possible to answer the following question: is total compensation possible in a three-phase, four-wire circuit?

6. Conclusions

Connecting in series the compensator with the load affects the value of the current and voltage supplying the receiver, so this method requires the use of a regulated power source. In the case of an asymmetrical system, the regulation should be carried out individually in each phase. Such a solution is rather far from the standards of modern power circuits, but such a compensation method is the only way to reduce the scattered component.

The greatest value of this study is the presentation of the concept of decomposition into Voltages’ Physical Components (VPC) as an alternative to the commonly known method of decomposition into Currents’ Physical Components (CPC). Moreover, using the VPC method, it is possible to theorize about full compensation in three-phase circuits, i.e., one in which the power factor λ is equal to one. The authors are aware that the use of the VPC theory for power compensation in power systems has little practical application; however, it enables theoretical analyses and gives alternative solutions in electrical circuits.