Numerical Investigation on Mesoscale Evolution of Hydraulic Fractures in Hydrate-Bearing Sediments
Abstract
1. Introduction
- Finite-element method (FEM): Numerous researchers have used the FEM to simulate HF in various oil and gas reservoirs, among which the cohesive zone method (CZM) based on the viscoelastic–plastic damage constitutive criterion is widely used [32,33,34,35,36]. However, the mesh needs to be constantly reconstructed to simulate the fracture growth, which greatly increases the number of calculations. Additionally, fracture propagation is disturbed by grid boundaries, thus affecting the reliability of the results.
- Extended finite-element method (XFEM): XFEM is a variation of FEM in which discontinuous enrichment functions are introduced to describe the discontinuity of fractures, thereby solving the calculation burden caused by mesh reconstruction [37]. Although the method is effective, the XFEM introduces additional difficulties such as the need for special integration techniques to resolve the stiffness matrix, blending of enriched and non-enriched elements, and ill-conditioned stiffness matrices [38]. In addition, the most suitable enrichment functions for a particular type of stress concentration are usually not known in advance. This poses new challenges when applying the XFEM to arbitrary types of singularities [39,40].
- Boundary element method (BEM): Unlike the FEM, which divides elements in the continuum domain, the BEM only meshes elements on the domain boundary [41]. The displacement discontinuity method (DDM), an indirect DEM, is widely used to simulate HF [42]. However, the BEM is not good at solving nonlinear problems and cannot accurately describe the stress and displacement fields at the fracture tip [43]. For example, it is difficult to simulate fracture propagation in heterogeneous materials and handle the coupling of fluid–solid between the rock matrix and fractures [32].
- Discrete element method (DEM): The DEM was proposed by Cundall and Strack (1979) for simulating the interaction between granular materials [44]. Unlike methods based on continuum mechanics, the DEM idealizes the material as separate particles bonded together at their contacts, and the microcracks generated by the breakage of the bonds. Since there are no precise meshing and numerous continuum assumptions, the DEM has a unique model heterogeneity and thus can simulate the HF process of discrete materials. However, limited by the number of particles and computational resources, the DEM is primarily used for small-scale simulations and is good at revealing the meso-mechanical behavior of discrete materials, whereas methods such as the unconventional fracture model [45], the simplified 3D DDM [46], and the FEM-based method [47] are capable of mine-scale simulations.
2. Methodology
2.1. HBS Simulation Methodology
2.2. Fluid–Mechanical Coupling Algorithm
2.3. Microcrack Growth and Failure Criteria
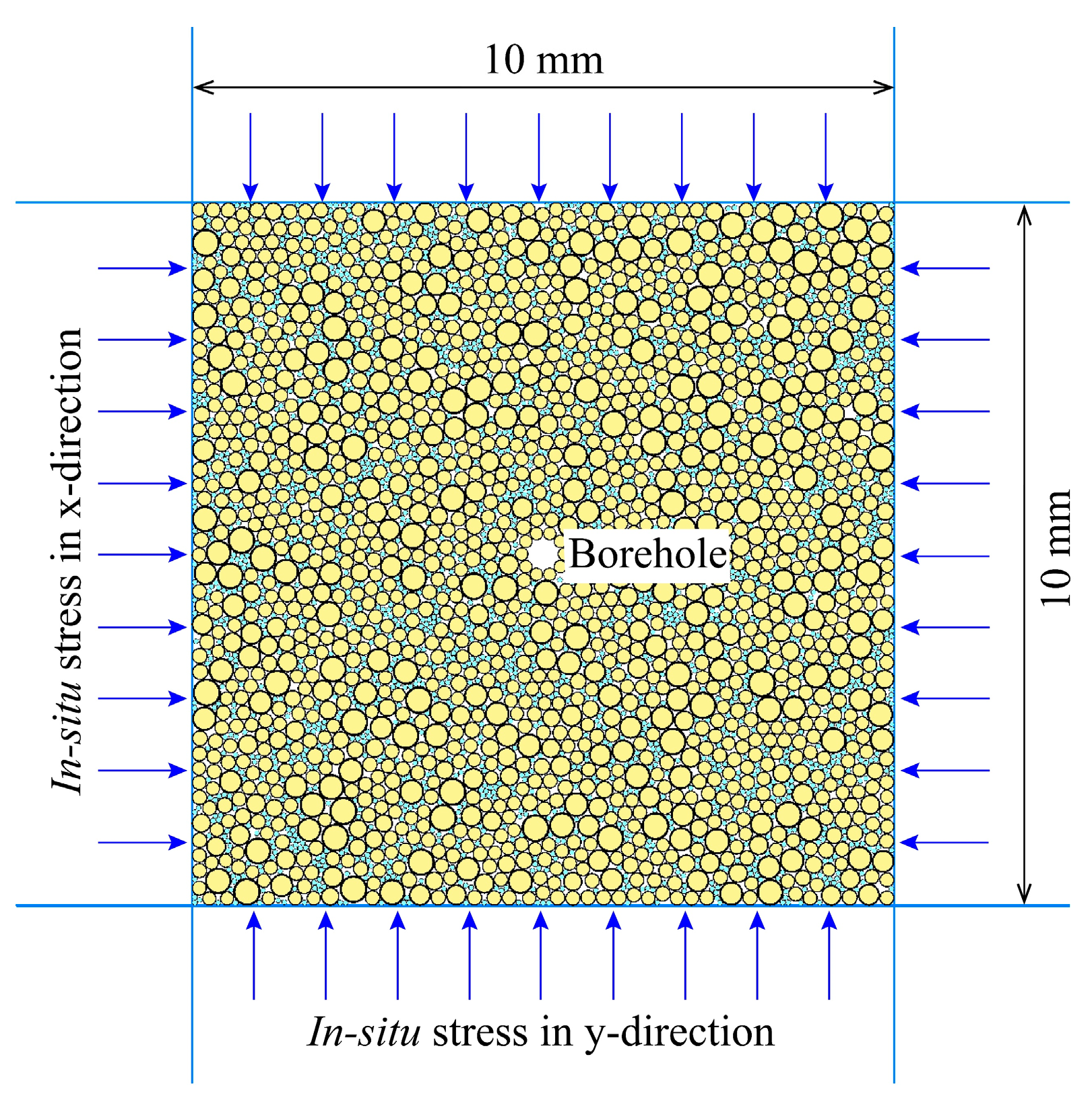
3. Modeling
3.1. HF Model
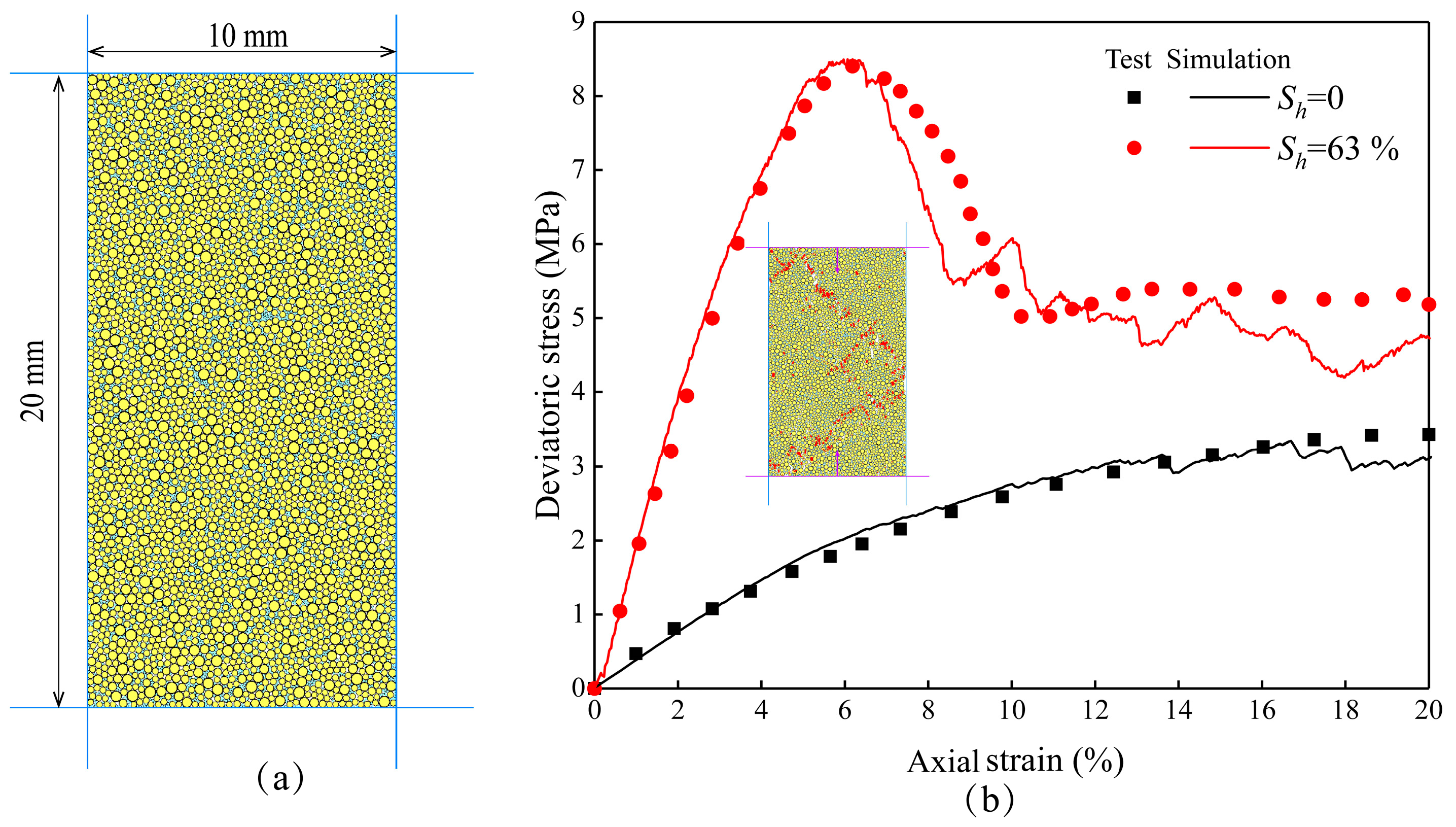
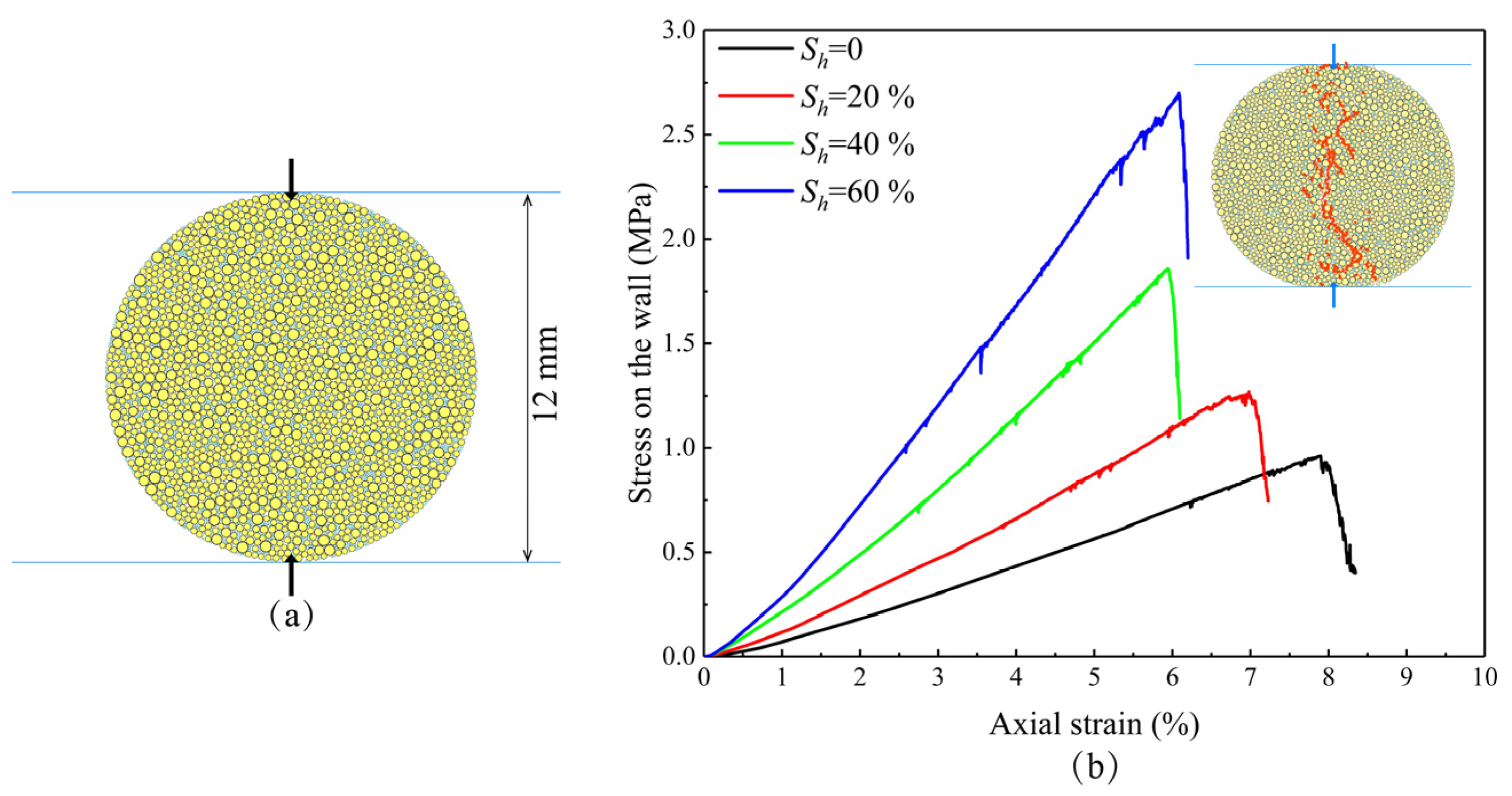
3.2. Calibration of Model Parameters
4. Results and Discussion
4.1. Effects of Hydrate Saturation
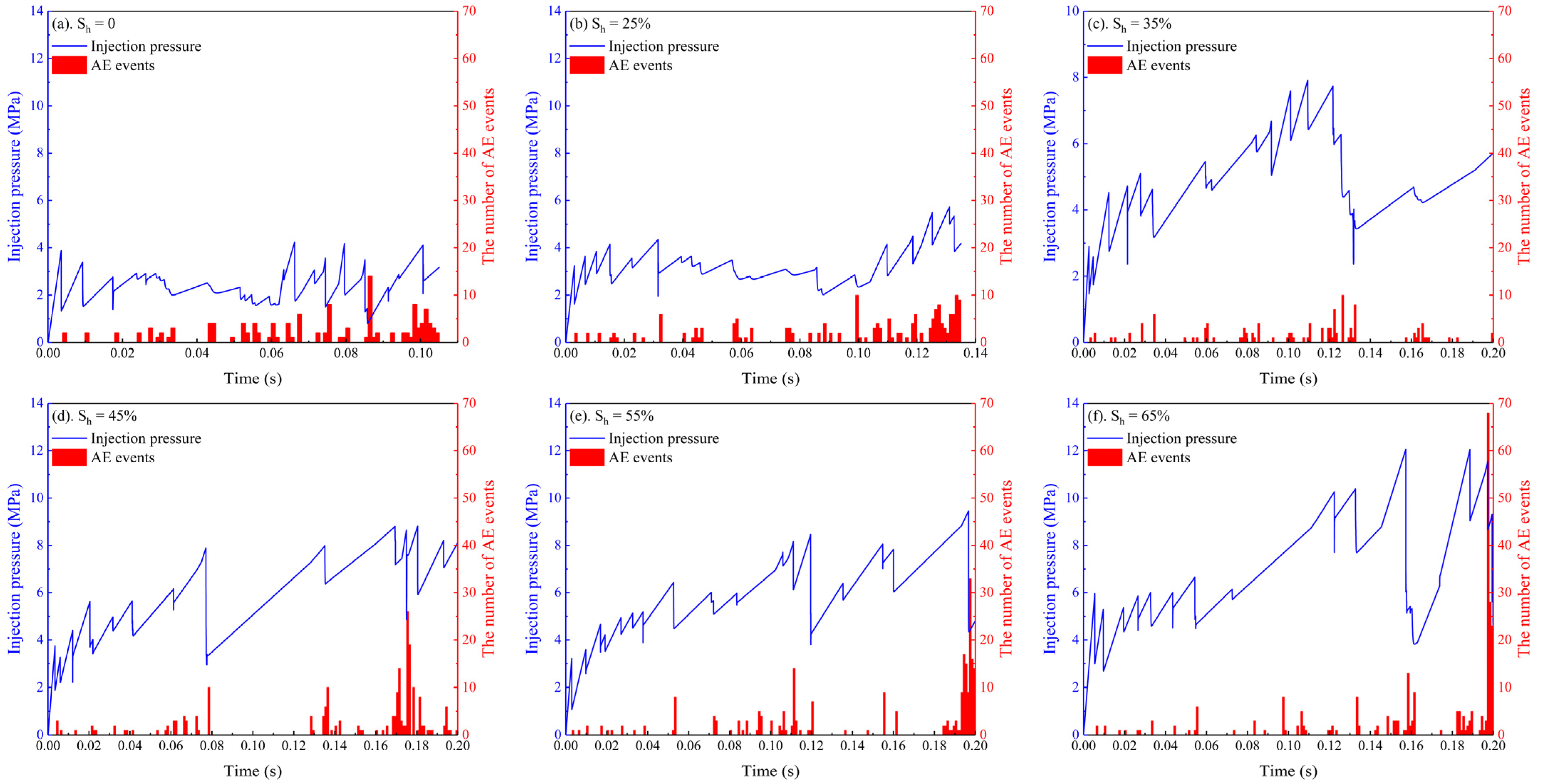
4.1.1. Injection Pressure
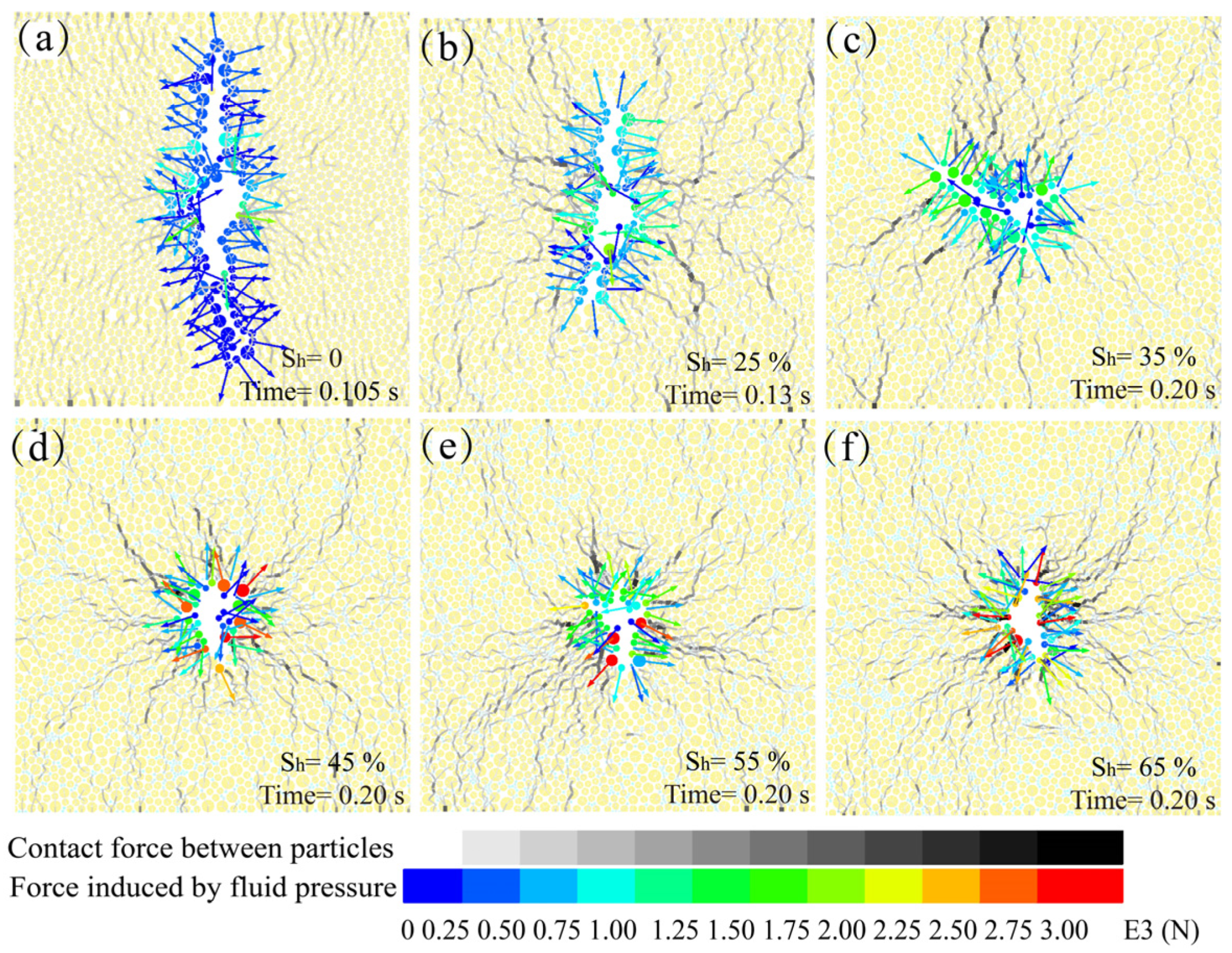
4.1.2. Contact-Force Chains and Applied Force Induced by Fluid
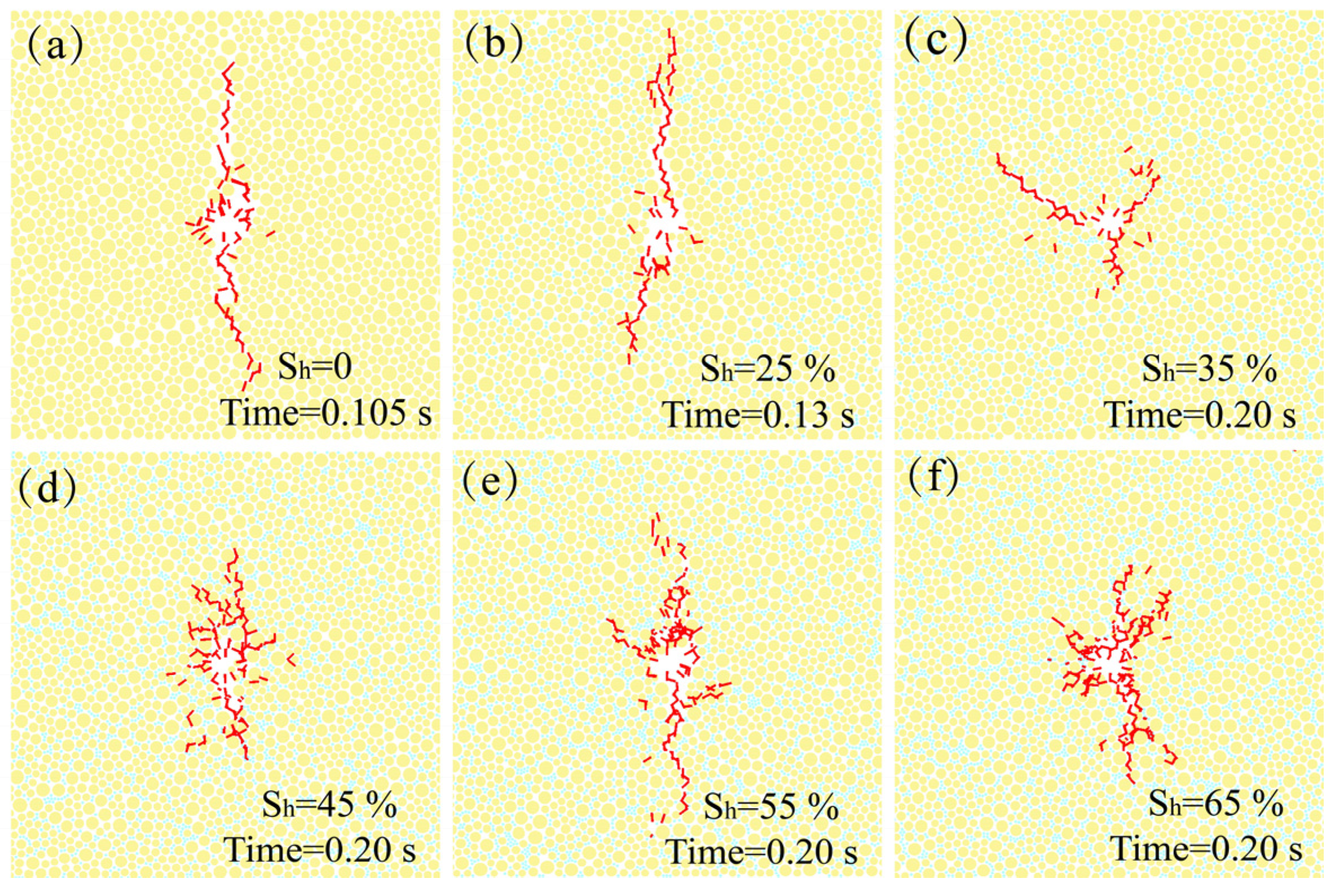
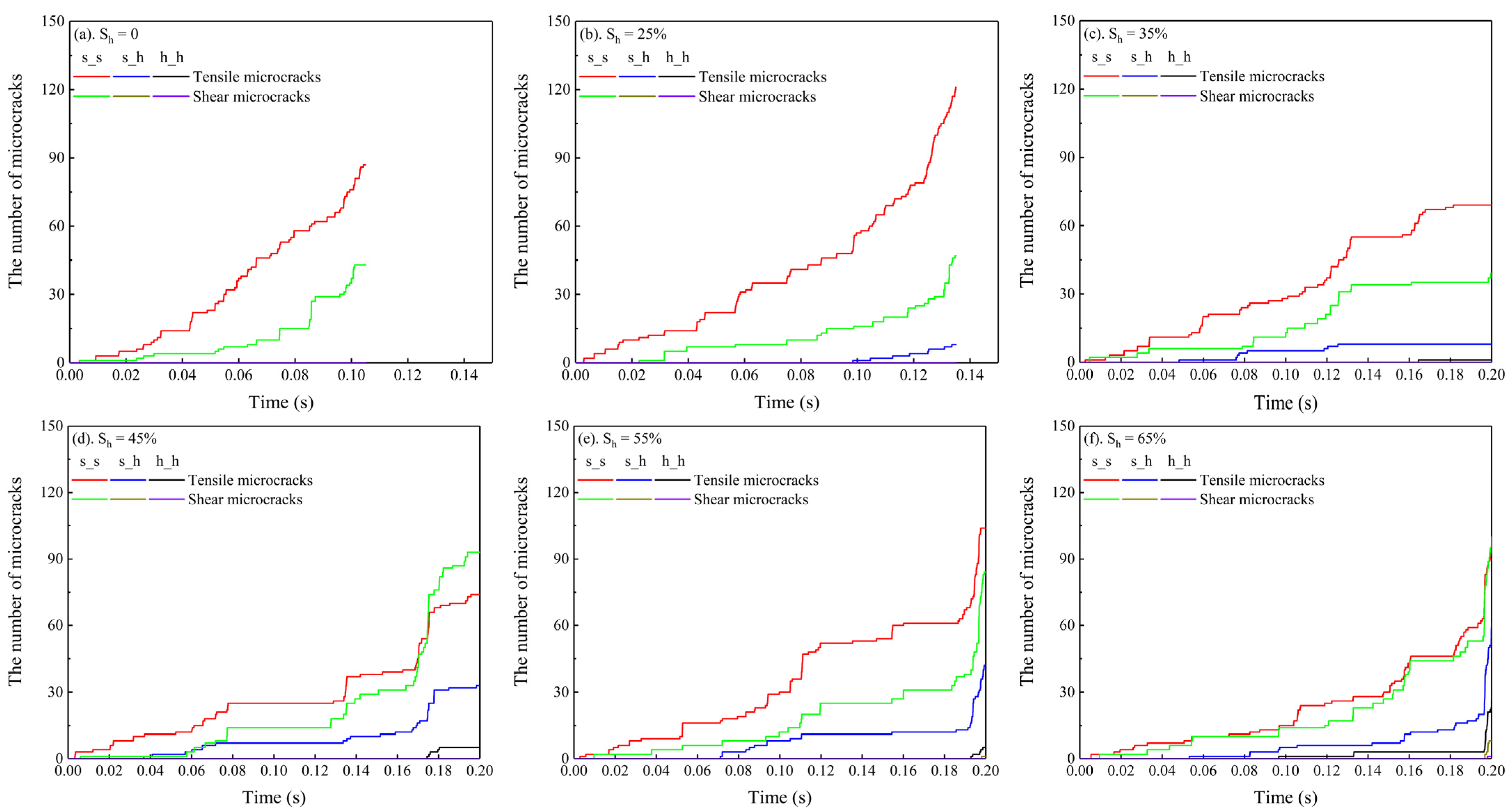
4.1.3. Evolution of Microcracks
4.2. Effects of the In Situ Stress
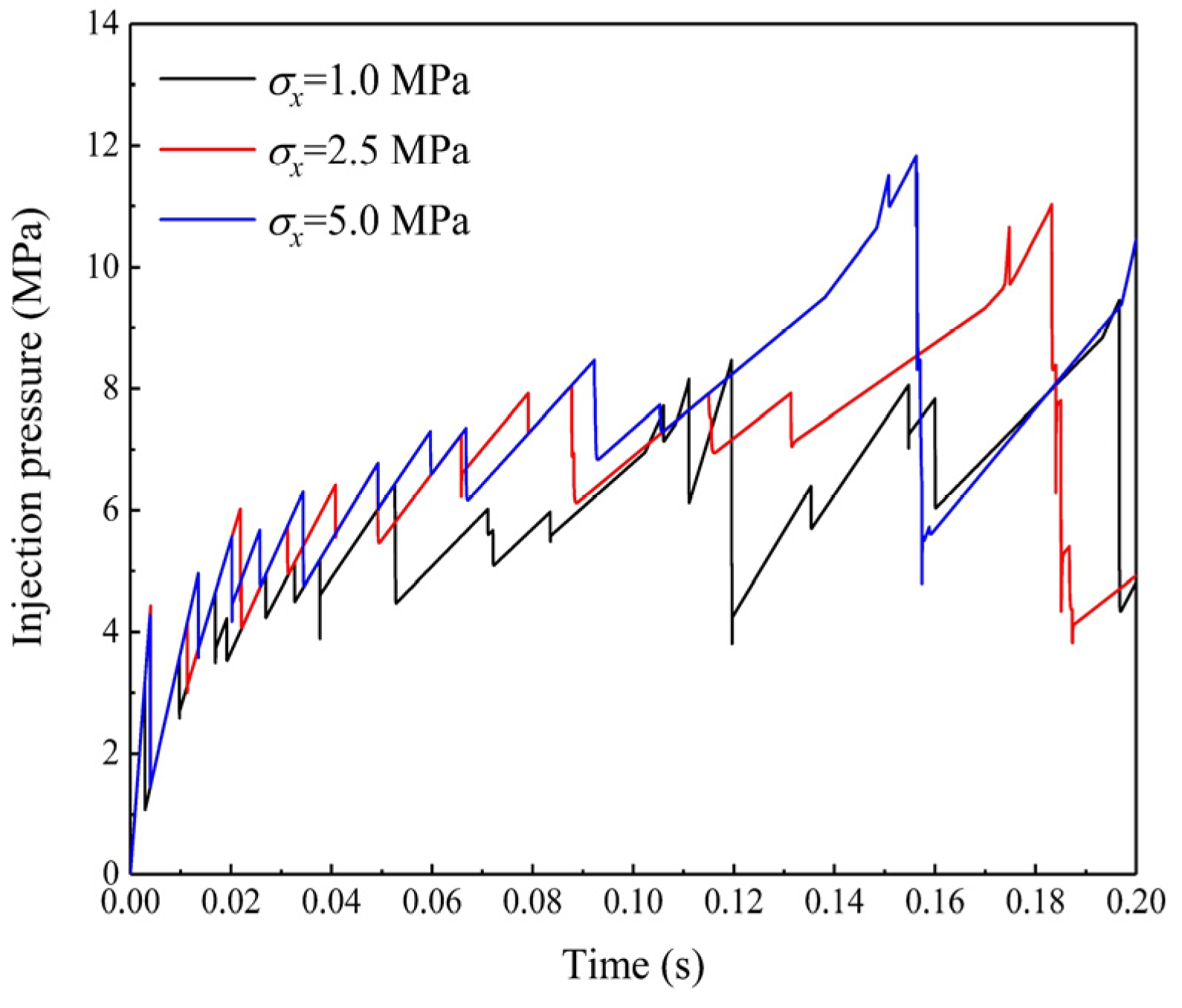
4.2.1. Injection Pressure
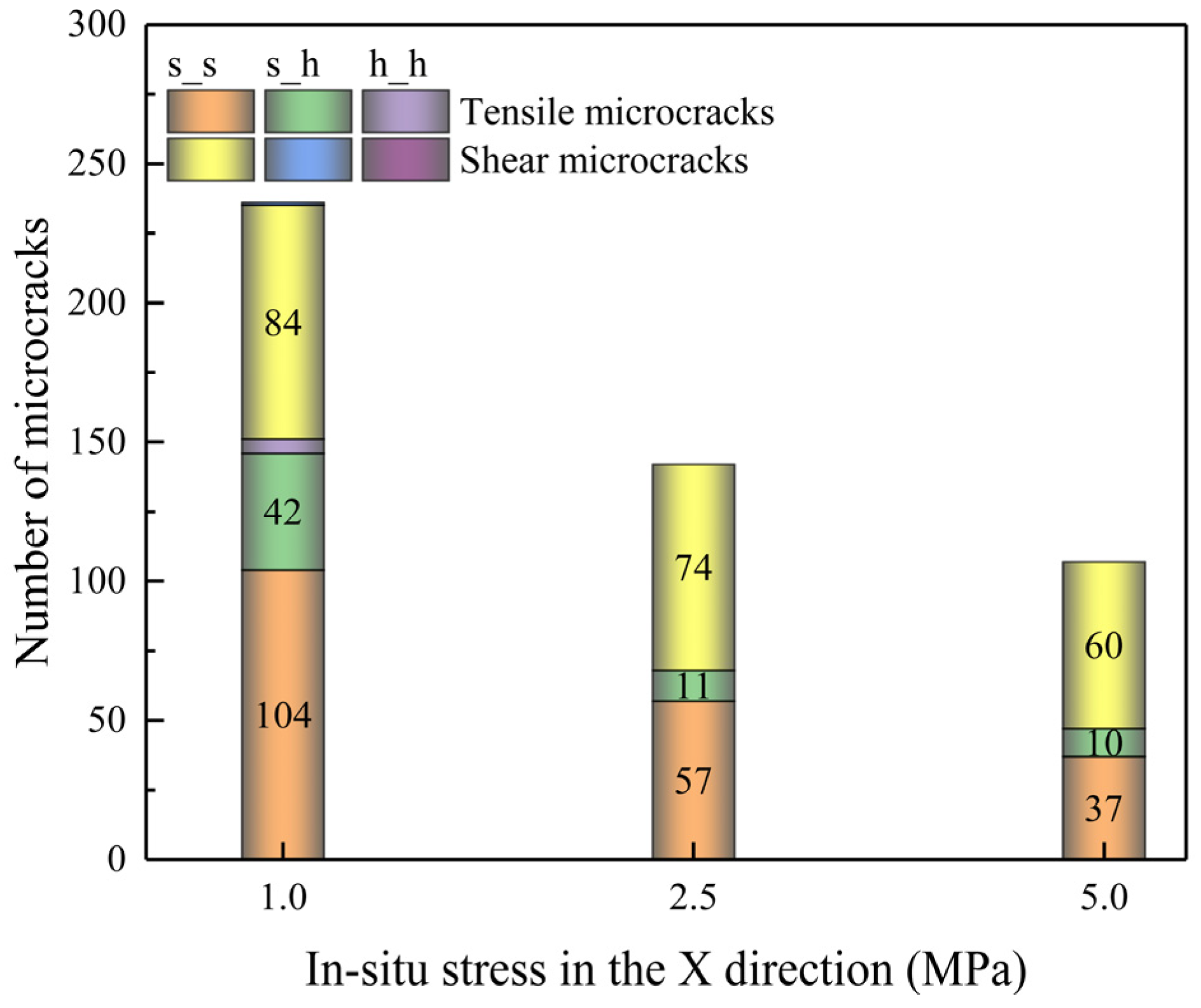
4.2.2. Distribution and Number of Microcracks
4.3. Effects of Injection Rate
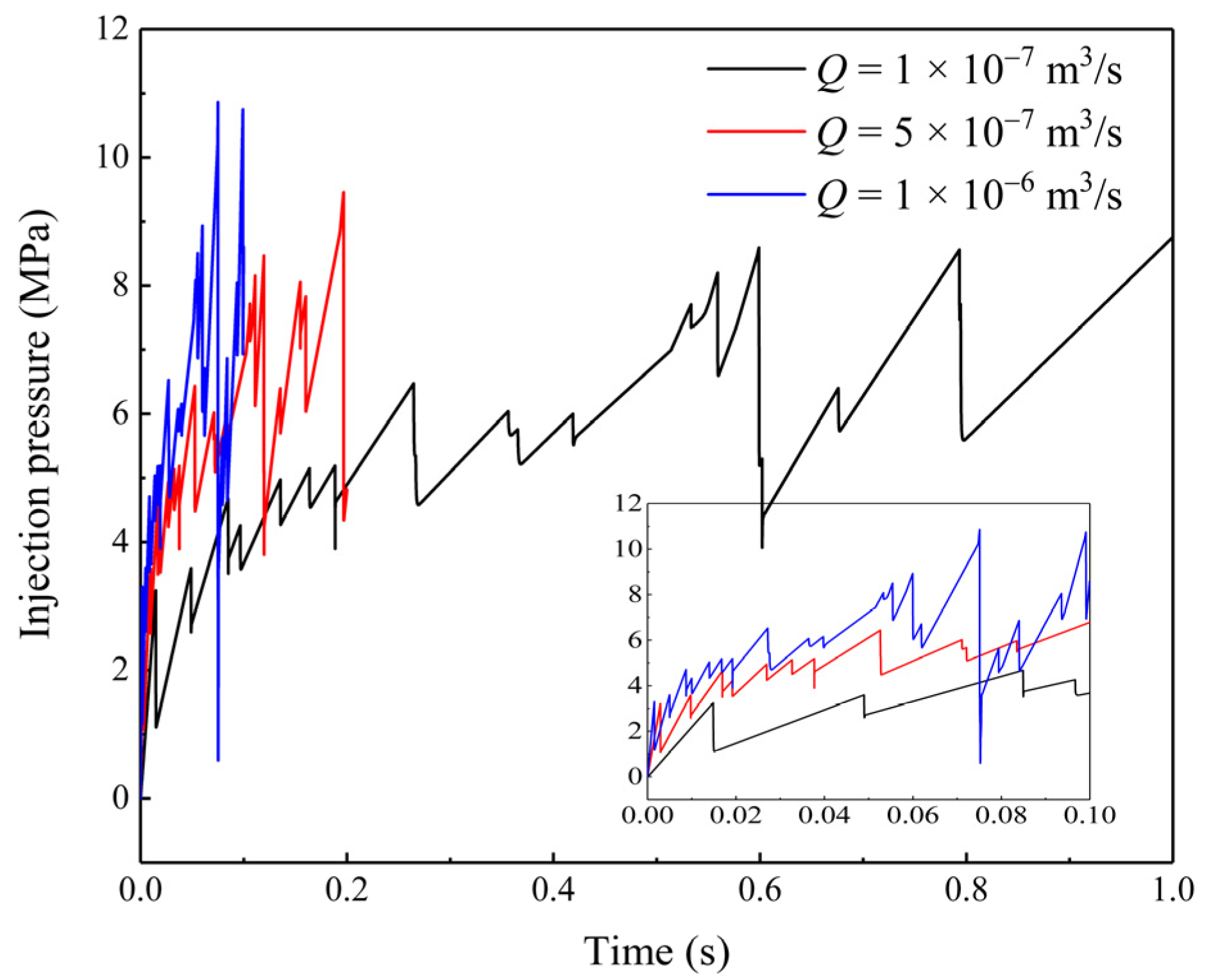
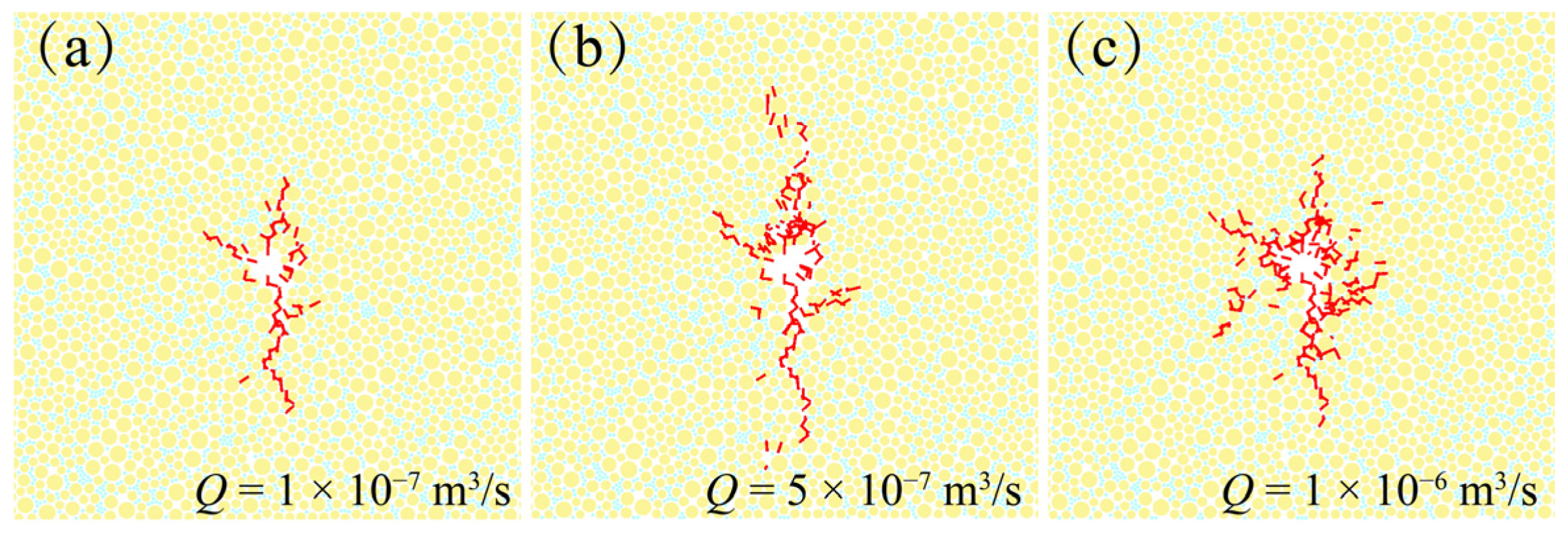
4.3.1. Injection Pressure
4.3.2. Distribution and Number of Microcracks
5. Conclusions
- Hydrate saturation has a significant effect on fracture morphology, and complex fractures tend to form in HBS with high hydrate saturation. When the hydrate saturation is lower than 25%, bi-wing fractures are formed. With the increase in hydrate saturation, the strength of HBS significantly increased due to the bond nature of hydrates, thus increasing the injection pressure and forming multiple fractures.
- The tensile and shear stresses induced by the injected fluid jointly promote the generation of hydraulic fractures, with tensile stress being dominant. Additionally, fractures tend to propagate in the weakly cemented sediments, followed by those at the interface between hydrates and sediments. Owing to the high bond strength between hydrate particles, it is difficult for fractures to pass through them.
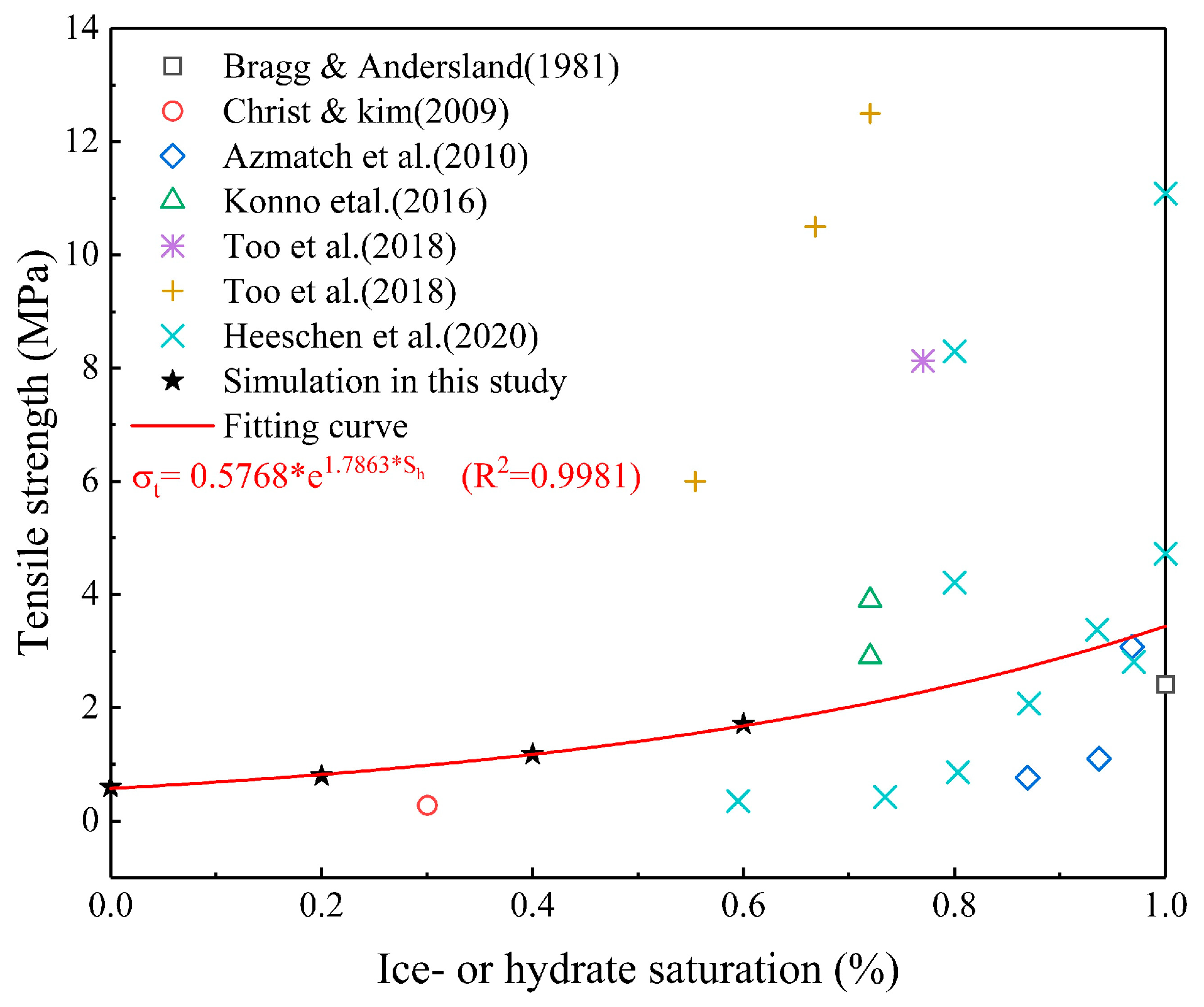
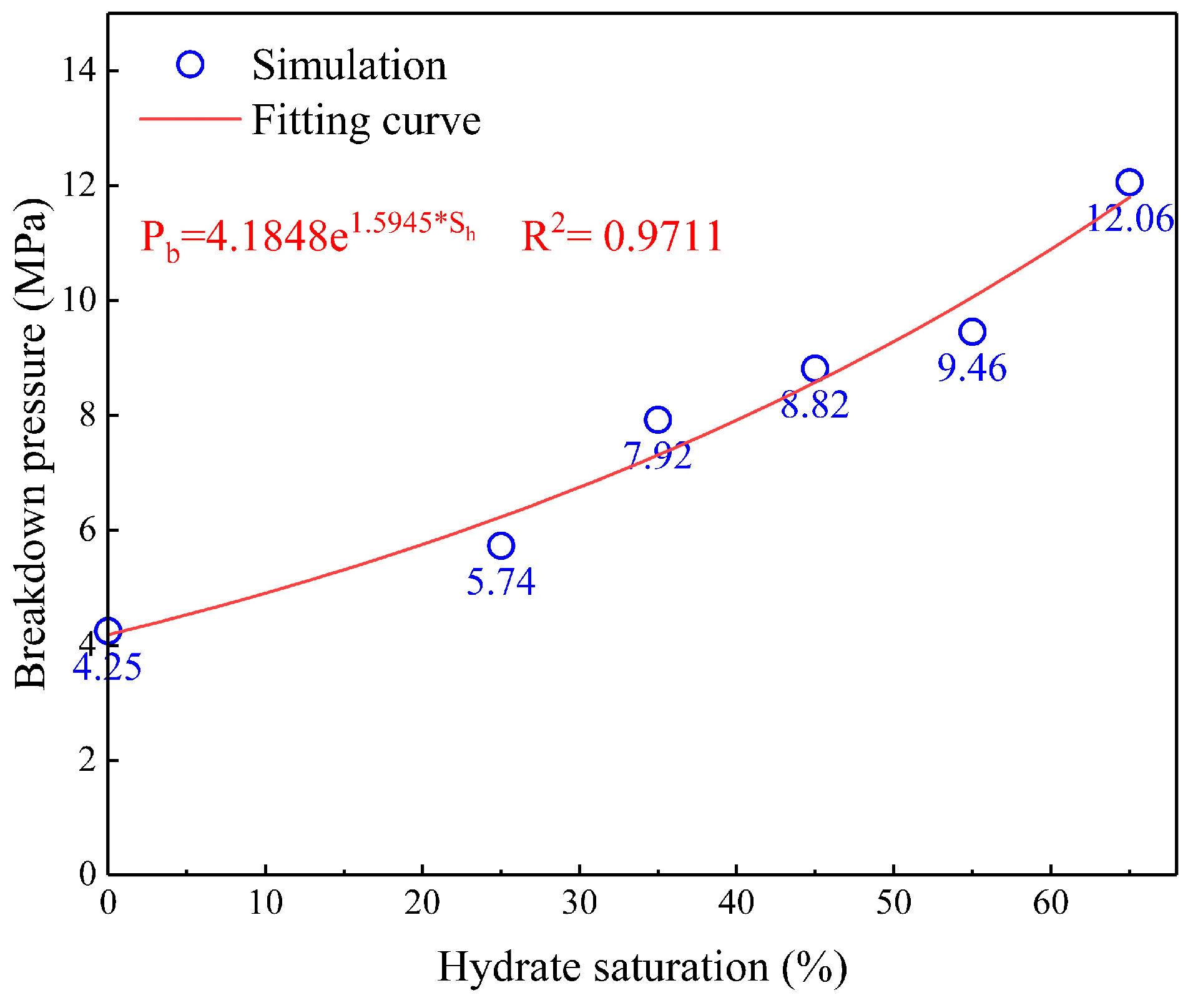
- Without considering the fracturing conditions, the breakdown pressure of HBS is governed by hydrate saturation and in situ stress. Given that the tensile strength of HBS is an exponential function of hydrate saturation, the fitted equation of the breakdown pressure of HBS is consistent with the classical Kirsch equation.
- Small in situ stress and high injection rates are conducive to the generation of microcracks, whereas an excessive injection rate causes over-reconstruction in the vicinity of the wellbore and reduces fracture penetration depth. Therefore, the shallow hydrate reservoir with elevated hydrate saturation is the “sweet spot” for fracturing, and an appropriate injection rate should be chosen for this non-diagenetic geomaterial.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sloan, E.D., Jr.; Koh, C.A. Clathrate Hydrates of Natural Gases; Taylor & Francis Group: New York, NY, USA, 2008. [Google Scholar] [CrossRef]
- Klauda, J.B.; Sandler, S.I. Global Distribution of Methane Hydrate in Ocean Sediment. Energy Fuels 2005, 19, 459–470. [Google Scholar] [CrossRef]
- Chong, Z.R.; Yang, S.H.B.; Babu, P.; Linga, P.; Li, X. Review of natural gas hydrates as an energy resource: Prospects and challenges. Appl. Energy 2016, 162, 1633–1652. [Google Scholar] [CrossRef]
- Liu, L.; Ryu, B.; Sun, Z.; Wu, N.; Cao, H.; Geng, W.; Zhang, X.; Jia, Y.; Xu, C.; Guo, L.; et al. Monitoring and research on environmental impacts related to marine natural gas hydrates: Review and future perspective. J. Nat. Gas Sci. Eng. 2019, 65, 82–107. [Google Scholar] [CrossRef]
- Liu, X.; Flemings, P.B. Dynamic multiphase flow model of hydrate formation in marine sediments. J. Geophys. Res. 2007, 112, B03101. [Google Scholar] [CrossRef]
- Buleiko, V.M.; Grigoriev, B.A.; Istomin, V.A. Capillary effects on phase behavior of liquid and gaseous propane and dynamics of hydrate formation and dissociation in porous media. Fluid Phase Equilibria 2017, 441, 64–71. [Google Scholar] [CrossRef]
- Xie, Y.; Li, R.; Wang, X.; Zheng, T.; Cui, J.; Yuan, Q.; Qin, H.; Sun, C.; Chen, G. Review on the accumulation behavior of natural gas hydrates in porous sediments. J. Nat. Gas Sci. Eng. 2020, 83, 103520. [Google Scholar] [CrossRef]
- Daigle, H.; Bangs, N.L.; Dugan, B. Transient hydraulic fracturing and gas release in methane hydrate settings: A case study from southern Hydrate Ridge. Geochem. Geophys. Geosyst. 2011, 12, Q12022. [Google Scholar] [CrossRef]
- Kayen, R.E.; Lee, H.J. Pleistocene slope instability of gas hydrate-laden sediment on the Beaufort sea margin. Mar. Geotechnol. 1991, 10, 125–141. [Google Scholar] [CrossRef]
- Handwerger, A.L.; Rempel, A.W.; Skarbek, R.M. Submarine landslides triggered by destabilization of high-saturation hydrate anomalies. Geochem. Geophys. Geosyst. 2017, 18, 2429–2445. [Google Scholar] [CrossRef]
- Brown, P.; Sanders, R.; McDonagh, E.; Henson, S.; Best, A.I.; Poulton, A.J.; Mayor, D.J. Impacts and effects of ocean warming on carbon management including methane hydrates. In Explaining Ocean Warming: Causes, Scale, Effects and Consequences; IUCN: Gland, Switzerland, 2016; p. 373. [Google Scholar] [CrossRef]
- Skarke, A.; Ruppel, C.; Kodis, M.; Brothers, D.; Lobecker, E. Widespread methane leakage from the sea floor on the northern US Atlantic margin. Nat. Geosci. 2014, 7, 657–661. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhai, W.-D. Shallow-ocean methane leakage and degassing to the atmosphere: Triggered by offshore oil-gas and methane hydrate explorations. Front. Mar. Sci. 2015, 2, 34. [Google Scholar] [CrossRef][Green Version]
- McGinnis, D.F.; Greinert, J.; Artemov, Y.; Beaubien, S.E.; Wüest, A. Fate of rising methane bubbles in stratified waters: How much methane reaches the atmosphere? J. Geophys. Res. 2006, 111, C09007. [Google Scholar] [CrossRef]
- Pillsbury, L.; Weber, T.C. Fate of methane gas bubbles emitted from the seafloor along the Western Atlantic Margin as observed by active sonar. J. Acoust. Soc. Am. 2015, 137, 2361. [Google Scholar] [CrossRef]
- Argentino, C.; Conti, S.; Fioroni, C.; Fontana, D. Evidences for Paleo-Gas Hydrate Occurrence: What We Can Infer for the Miocene of the Northern Apennines (Italy). Geosciences 2019, 9, 134. [Google Scholar] [CrossRef]
- Moridis, G.J.; Reagan, M.T.; Boyle, K.L.; Zhang, K. Evaluation of the Gas Production Potential of Some Particularly Challenging Types of Oceanic Hydrate Deposits. Transp. Porous Media 2011, 90, 269–299. [Google Scholar] [CrossRef]
- Wang, B.; Fan, Z.; Zhao, J.; Lv, X.; Pang, W.; Li, Q. Influence of intrinsic permeability of reservoir rocks on gas recovery from hydrate deposits via a combined depressurization and thermal stimulation approach. Appl. Energy 2018, 229, 858–871. [Google Scholar] [CrossRef]
- Yang, M.; Gao, Y.; Zhou, H.; Chen, B.; Li, Y. Gas production from different classes of methane hydrate deposits by the depressurization method. Int. J. Energy Res. 2019, 43, 5493–5505. [Google Scholar] [CrossRef]
- Chen, C.; Yang, L.; Jia, R.; Sun, Y.; Guo, W.; Chen, Y.; Li, X. Simulation Study on the Effect of Fracturing Technology on the Production Efficiency of Natural Gas Hydrate. Energies 2017, 10, 1241. [Google Scholar] [CrossRef]
- Yang, L.; Chen, C.; Jia, R.; Sun, Y.; Guo, W.; Pan, D.; Li, X.; Chen, Y. Influence of Reservoir Stimulation on Marine Gas Hydrate Conversion Efficiency in Different Accumulation Conditions. Energies 2018, 11, 339. [Google Scholar] [CrossRef]
- Feng, Y.; Chen, L.; Suzuki, A.; Kogawa, T.; Okajima, J.; Komiya, A.; Maruyama, S. Enhancement of gas production from methane hydrate reservoirs by the combination of hydraulic fracturing and depressurization method. Energy Convers. Manag. 2019, 184, 194–204. [Google Scholar] [CrossRef]
- Sun, J.; Ning, F.; Liu, T.; Liu, C.; Chen, Q.; Li, Y.; Cao, X.; Mao, P.; Zhang, L.; Jiang, G. Gas production from a silty hydrate reservoir in the South China Sea using hydraulic fracturing: A numerical simulation. Energy Sci. Eng. 2019, 7, 1106–1122. [Google Scholar] [CrossRef]
- Ju, X.; Liu, F.; Fu, P.; White, M.D.; Settgast, R.R.; Morris, J.P. Gas Production from Hot Water Circulation through Hydraulic Fractures in Methane Hydrate-Bearing Sediments: THC-Coupled Simulation of Production Mechanisms. Energy Fuels 2020, 34, 4448–4465. [Google Scholar] [CrossRef]
- Zhong, X.; Pan, D.; Zhai, L.; Zhu, Y.; Zhang, H.; Zhang, Y.; Wang, Y.; Li, X.; Chen, C. Evaluation of the gas production enhancement effect of hydraulic fracturing on combining depressurization with thermal stimulation from challenging ocean hydrate reservoirs. J. Nat. Gas Sci. Eng. 2020, 83, 103621. [Google Scholar] [CrossRef]
- Konno, Y.; Jin, Y.; Yoneda, J.; Uchiumi, T.; Shinjou, K.; Nagao, J. Hydraulic fracturing in methane-hydrate-bearing sand. RSC Adv. 2016, 6, 73148–73155. [Google Scholar] [CrossRef]
- Too, J.L.; Cheng, A.; Khoo, B.C.; Palmer, A.; Linga, P. Hydraulic fracturing in a penny-shaped crack. Part II: Testing the frackability of methane hydrate-bearing sand. J. Nat. Gas Sci. Eng. 2018, 52, 619–628. [Google Scholar] [CrossRef]
- Yamada, H.; Chen, L.; Okajima, J.; Komiya, A.; Maruyama, S. Visualization of the flow pattern in methane hydrate reservoir model. J. Fluid Sci. Technol. 2018, 13, JFST0028. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, W.; Qu, Z.; Guo, T.; Sun, Y.; Rabiei, M.; Cao, Q. Feasibility evaluation of hydraulic fracturing in hydrate-bearing sediments based on analytic hierarchy process-entropy method (AHP-EM). J. Nat. Gas Sci. Eng. 2020, 81, 103434. [Google Scholar] [CrossRef]
- Ma, X.; Jiang, D.; Sun, Y.; Li, S. Experimental study on hydraulic fracturing behavior of frozen clayey silt and hydrate-bearing clayey silt. Fuel 2022, 322, 124366. [Google Scholar] [CrossRef]
- Nie, S.; Zhong, X.; Song, J.; Tu, G.; Chen, C. Experimental study on hydraulic fracturing in clayey-silty hydrate-bearing sediments and fracability evaluation based on multilayer perceptron-analytic hierarchy process. J. Nat. Gas Sci. Eng. 2022, 106, 104735. [Google Scholar] [CrossRef]
- Zhai, L.; Zhang, H.; Pan, D.; Zhu, Y.; Zhu, J.; Zhang, Y.; Chen, C. Optimisation of hydraulic fracturing parameters based on cohesive zone method in oil shale reservoir with random distribution of weak planes. J. Nat. Gas Sci. Eng. 2020, 75, 103130. [Google Scholar] [CrossRef]
- Wang, D.; Dong, Y.; Sun, D.; Yu, B. A three-dimensional numerical study of hydraulic fracturing with degradable diverting materials via CZM-based FEM. Eng. Fract. Mech. 2020, 237, 107251. [Google Scholar] [CrossRef]
- Huang, C.; Chen, S. Effects of Ductility of Organic-Rich Shale on Hydraulic Fracturing: A Fully Coupled Extended-Finite-Element-Method Analysis Using a Modified Cohesive Zone Model. SPE J. 2020, 26, 591–609. [Google Scholar] [CrossRef]
- Ma, X.; Cheng, J.; Sun, Y.; Li, S. 2D Numerical Simulation of Hydraulic Fracturing in Hydrate-Bearing Sediments Based on the Cohesive Element. Energy Fuels 2021, 35, 3825–3840. [Google Scholar] [CrossRef]
- Ma, X.; Jiang, D.; Fang, X.; Wang, X. Numerical simulation of single-cluster and multi-cluster fracturing of hydrate reservoir based on cohesive element. Eng. Fract. Mech. 2022, 265, 108365. [Google Scholar] [CrossRef]
- Martínez, E.R.; de Farias, M.M.; Junior, F.E. Investigation of the notch angle in hydraulic fracturing using XFEM. J. Braz. Soc. Mech. Sci. Eng. 2019, 41, 437. [Google Scholar] [CrossRef]
- Sun, Z.; Ooi, E.T.; Song, C. Finite fracture mechanics analysis using the scaled boundary finite element method. Eng. Fract. Mech. 2015, 134, 330–353. [Google Scholar] [CrossRef]
- Bouhala, L.; Shao, Q.; Koutsawa, Y.; Younes, A.; Núñez, P.; Makradi, A.; Belouettar, S. An XFEM crack-tip enrichment for a crack terminating at a bi-material interface. Eng. Fract. Mech. 2013, 102, 51–64. [Google Scholar] [CrossRef]
- Menk, A.; Bordas, S.P.A. Numerically determined enrichment functions for the extended finite element method and applications to bi-material anisotropic fracture and polycrystals. Int. J. Numer. Methods Eng. 2010, 83, 805–828. [Google Scholar] [CrossRef]
- Zhao, Y.-L.; Xie, S.-C.; Peng, X.-L.; Zhang, L.-H. Transient pressure response of fractured horizontal wells in tight gas reservoirs with arbitrary shapes by the boundary element method. Environ. Earth Sci. 2016, 75, 1220. [Google Scholar] [CrossRef]
- Choo, L.Q.; Zhao, Z.; Chen, H.; Tian, Q. Hydraulic fracturing modeling using the discontinuous deformation analysis (DDA) method. Comput. Geotech. 2016, 76, 12–22. [Google Scholar] [CrossRef]
- Ben, Y.; Wang, Y.; Shi, G. Challenges of simulating hydraulic fracturing with DDA. In Proceedings of the ISRM SINOROCK 2013, Shanghai, China, 18–20 June 2013; pp. 607–611. [Google Scholar] [CrossRef]
- Cundall, P.A.; Strack, O.D.L. A discrete numerical model for granular assemblies. Geotechnique 1979, 29, 47–65. [Google Scholar] [CrossRef]
- Olga, K.; Xiaowei, W. Hydraulic Fracturing in Formations with Permeable Natural Fractures. In Effective and Sustainable Hydraulic Fracturing; Andrew, P.B., John, M., Rob, J., Eds.; IntechOpen: Rijeka, Croatia, 2013. [Google Scholar] [CrossRef]
- Wang, J.; Lee, H.P.; Li, T.; Olson, J.E. Three-Dimensional Analysis of Hydraulic Fracture Effective Contact Area in Layered Formations with Natural Fracture Network. In Proceedings of the 54th U.S. Rock Mechanics/Geomechanics Symposium, Physical Event Cancelled, Golden, CO, USA, 28 June–1 July 2020. [Google Scholar]
- Dahi Taleghani, A.; Gonzalez, M.; Shojaei, A. Overview of numerical models for interactions between hydraulic fractures and natural fractures: Challenges and limitations. Comput. Geotech. 2016, 71, 361–368. [Google Scholar] [CrossRef]
- Shimizu, H.; Murata, S.; Ishida, T. The distinct element analysis for hydraulic fracturing in hard rock considering fluid viscosity and particle size distribution. Int. J. Rock Mech. Min. Sci. 2011, 48, 712–727. [Google Scholar] [CrossRef]
- Wang, T.; Zhou, W.; Chen, J.; Xiao, X.; Li, Y.; Zhao, X. Simulation of hydraulic fracturing using particle flow method and application in a coal mine. Int. J. Coal Geol. 2014, 121, 1–13. [Google Scholar] [CrossRef]
- Zhou, J.; Zhang, L.; Braun, A.; Han, Z. Investigation of Processes of Interaction between Hydraulic and Natural Fractures by PFC Modeling Comparing against Laboratory Experiments and Analytical Models. Energies 2017, 10, 1001. [Google Scholar] [CrossRef]
- ICG Inc. PFC2D-Particle Flow Code in Two Dimensions. Version 4.0. User’s Manual; ICG: Minneapolis, MN, USA, 2010. [Google Scholar]
- Yan, R.T.; Wei, C.F.; Wei, H.Z.; Tian, H.H.; Wu, E.L. A generalized critical state model for gas hydrate-bearing sediments. In Constitutive Modeling of Geomaterials; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Soga, K.; Lee, S.L.; Ng, M.Y.A.; Klar, A. Characterisation and engineering properties of Methane hydrate soils. 2nd Int. Workshop Characterisation Eng. Prop. Nat. Soils 2006, 4, 1642–2591. [Google Scholar] [CrossRef]
- Jiang, M.; Liu, J.; Shen, Z. Investigating the mechanical behavior of grain-coating type methane hydrate bearing sediment in true triaxial compression tests by distinct element method. SCIENTIA SINICA Phys. Mech. Astron. 2018, 49, 034613. [Google Scholar] [CrossRef]
- Zhang, H.; Bi, J.; Luo, X. Oedometer test of natural gas hydrate-bearing sands: Particle-scale simulation. J. Nat. Gas Sci. Eng. 2020, 84, 103631. [Google Scholar] [CrossRef]
- Bruno, M.S. Micromechanics of stress-induced permeability anisotropy and damage in sedimentary rock. Mech. Mater. 1994, 18, 31–48. [Google Scholar] [CrossRef]
- Thallak, S.L.; Rothenburg; Dusseault, M. Simulation of multiple hydraulic fractures in a discrete element system. In Proceedings of the 32nd U.S. Symposium on Rock Mechanics (USRMS), Norman, Oklahoma, 10–12 July 1991. [Google Scholar]
- Han, Y.; Cundall, P.A. Lattice Boltzmann modeling of pore-scale fluid flow through idealized porous media. Int. J. Numer. Methods Fluids 2011, 67, 1720–1734. [Google Scholar] [CrossRef]
- Al-Busaidi, A. Distinct element modeling of hydraulically fractured Lac du Bonnet granite. J. Geophys. Res. 2005, 110, B06302. [Google Scholar] [CrossRef]
- Masuda, Y.; Fujinaga, Y.; Naganawa, S.; Fujita, K.; Sato, K.; Hayashi, Y. Modeling and experimental studies on dissociation of methane gas hydrates in Berea sandstone cores. In Proceedings of the International Conference on Gas Hydrates, Salt Lake City, UT, USA, 18–22 July 1999. [Google Scholar]
- Yoneda, J.; Masui, A.; Konno, Y.; Jin, Y.; Kida, M.; Katagiri, J.; Nagao, J.; Tenma, N. Pressure-core-based reservoir characterization for geomechanics: Insights from gas hydrate drilling during 2012–2013 at the eastern Nankai Trough. Mar. Pet. Geol. 2017, 86, 1–16. [Google Scholar] [CrossRef]
- Yoneda, J.; Masui, A.; Konno, Y.; Jin, Y.; Egawa, K.; Kida, M.; Ito, T.; Nagao, J.; Tenma, N. Mechanical behavior of hydrate-bearing pressure-core sediments visualized under triaxial compression. Mar. Pet. Geol. 2015, 66, 451–459. [Google Scholar] [CrossRef]
- Heeschen, K.U.; Janocha, J.; Spangenberg, E.; Schicks, J.M.; Giese, R. The impact of ice on the tensile strength of unconsolidated sand—A model for gas hydrate-bearing sands? Mar. Pet. Geol. 2020, 122, 104607. [Google Scholar] [CrossRef]
- Bragg, R.A.; Andersland, O.B. Strain rate, temperature, and sample size effects on compression and tensile properties of frozen sand. Eng. Geol. 1981, 18, 35–46. [Google Scholar] [CrossRef]
- Christ, M.; Kim, Y.-C. Experimental study on the physical-mechanical properties of frozen silt. KSCE J. Civ. Eng. 2009, 13, 317–324. [Google Scholar] [CrossRef]
- Azmatch, T.F.; Sego, D.C.; Arenson, L.U.; Biggar, K.W. Tensile Strength of Frozen Soils Using Four-point Bending Test. In Proceedings of the 63rd Canadian Geotechnical Conference and 6th Canadian Permafrost Conference, Calgary, AB, Canada, 12–16 September 2010; pp. 436–442. [Google Scholar]
- Too, J.L.; Cheng, A.; Khoo, B.C.; Palmer, A.; Linga, P. Hydraulic fracturing in a penny-shaped crack. Part I: Methodology and testing of frozen sand. J. Nat. Gas Sci. Eng. 2018, 52, 609–618. [Google Scholar] [CrossRef]
- Hubbert, M.K.; Willis, D.G. Mechanics Of Hydraulic Fracturing. Trans. AIME 1957, 210, 153–168. [Google Scholar] [CrossRef]
- Haimson, B.; Fairhurst, C. Initiation and Extension of Hydraulic Fractures in Rocks. Soc. Pet. Eng. J. 1967, 7, 310–318. [Google Scholar] [CrossRef]
- Haimson, B.C.; Cornet, F.H. ISRM Suggested Methods for rock stress estimation—Part 3: Hydraulic fracturing (HF) and/or hydraulic testing of pre-existing fractures (HTPF). Int. J. Rock Mech. Min. Sci. 2003, 40, 1011–1020. [Google Scholar] [CrossRef]
- Zhang, T.; Zhang, Y.; Katterbauer, K.; Al Shehri, A.; Sun, S.; Hoteit, I. Phase equilibrium in the hydrogen energy chain. Fuel 2022, 328, 125324. [Google Scholar] [CrossRef]
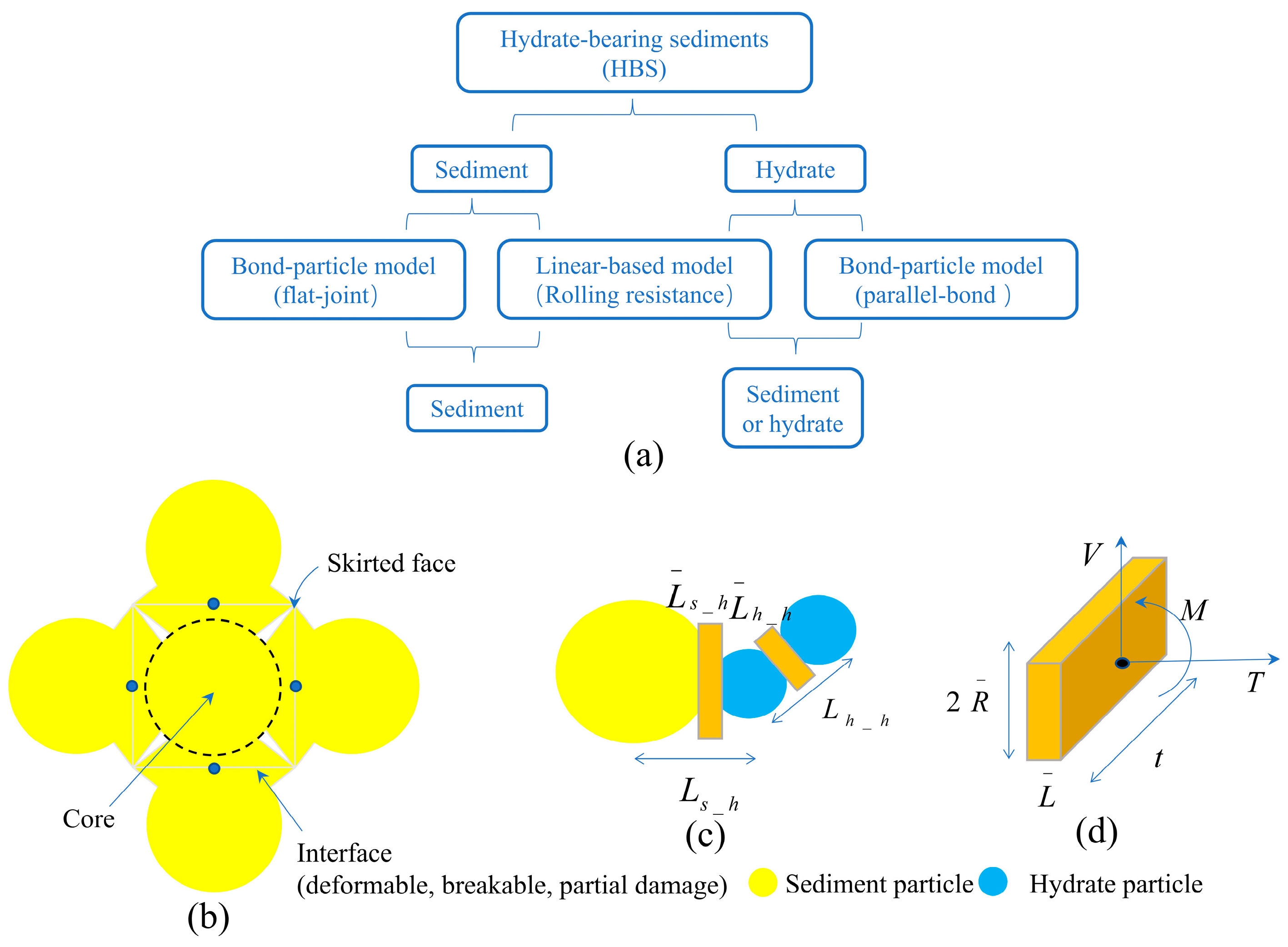
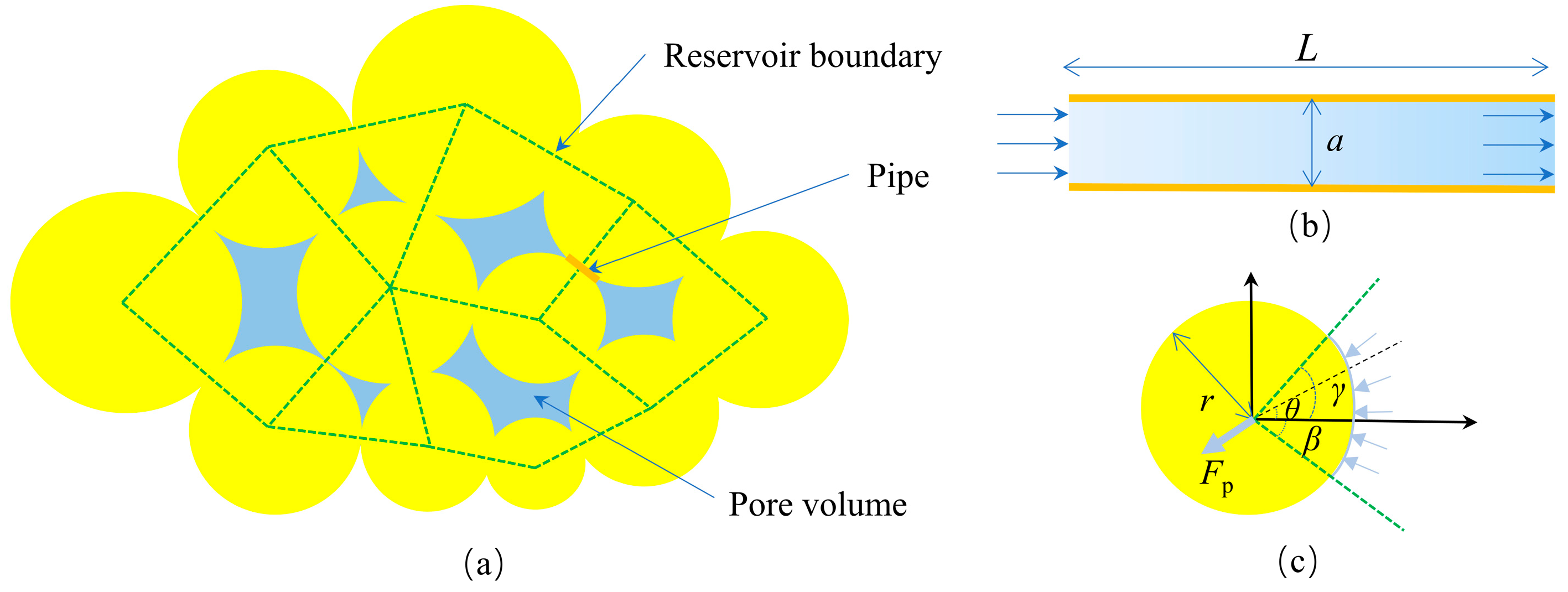
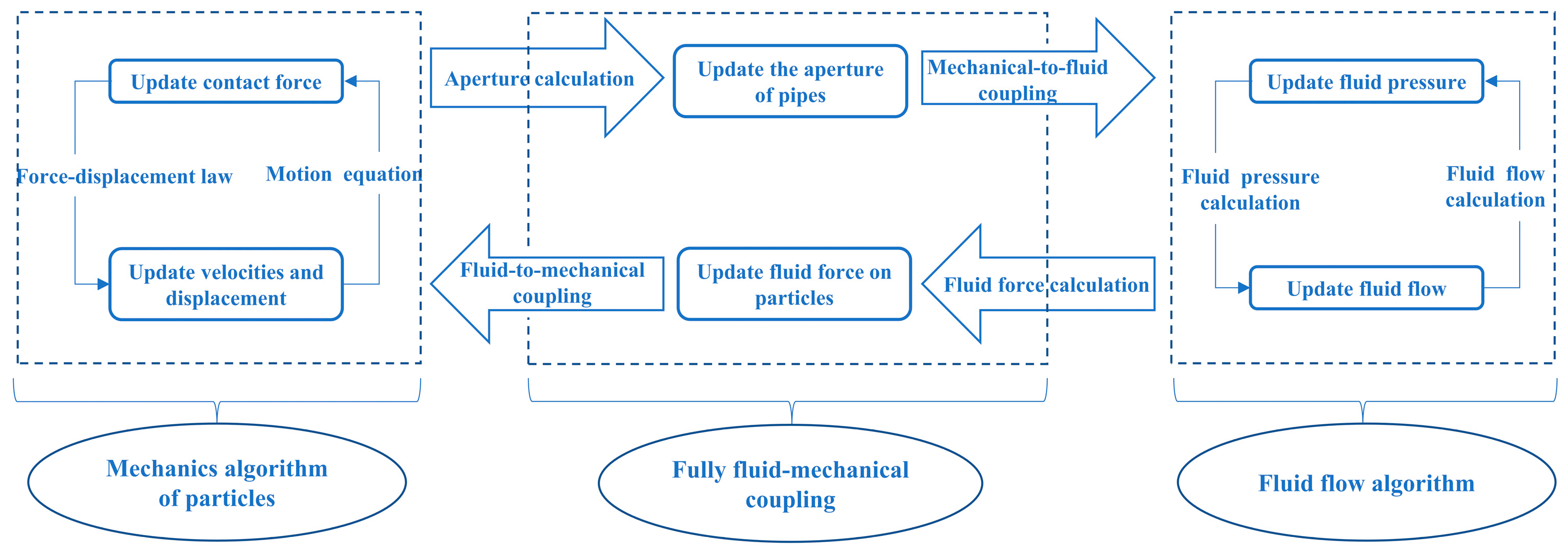
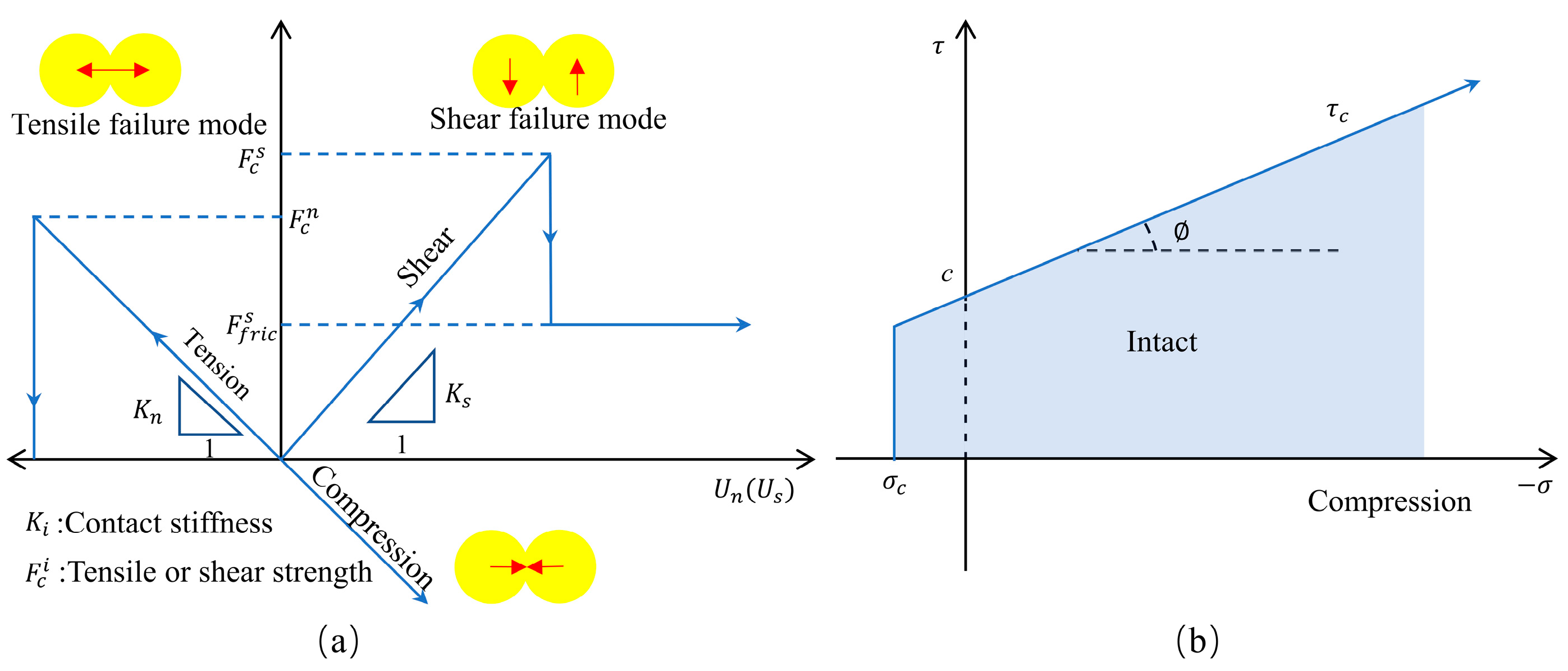


| Contact Model | Flat-Joint Model | Parallel-Bond Model | Rolling Resistance Model |
|---|---|---|---|
| Effective modulus Ec (N) | 3.8 × 107 | 4.2 × 108 | 3.8 × 107 |
| Normal-to-shear stiffness ratio kr | 1.5 | 1.5 | 1.5 |
| Friction coefficient μ | 0.25 | 0.04 | 1.0 |
| Rolling resistance coefficient μr | _ | _ | 1.0 |
| Bond effective modulus Ecb (N) | _ | 4.2 × 108 | _ |
| Bond normal-to-shear stiffness ratio krb | _ | 1.5 | _ |
| Gap interval gr (m) | 1.0 × 10−5 | 1.0 × 10−5 | 1.0 × 10−5 |
| Tensile strength of bond σcb (N) | 2.1 × 106 | 2.4 × 107 | _ |
| Cohesion cb (N) | 2.1 × 106 | 2.4 × 107 | _ |
| Friction angle ϕb (°) | 10 | 10 | _ |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liang, X.; Zhao, H.; Dang, Y.; Lei, Q.; Wang, S.; Wang, X.; Chai, H.; Jia, J.; Wang, Y. Numerical Investigation on Mesoscale Evolution of Hydraulic Fractures in Hydrate-Bearing Sediments. Energies 2023, 16, 7502. https://doi.org/10.3390/en16227502
Liang X, Zhao H, Dang Y, Lei Q, Wang S, Wang X, Chai H, Jia J, Wang Y. Numerical Investigation on Mesoscale Evolution of Hydraulic Fractures in Hydrate-Bearing Sediments. Energies. 2023; 16(22):7502. https://doi.org/10.3390/en16227502
Chicago/Turabian StyleLiang, Xiaowei, Hui Zhao, Yongchao Dang, Qihong Lei, Shaoping Wang, Xiaorui Wang, Huiqiang Chai, Jianbo Jia, and Yafei Wang. 2023. "Numerical Investigation on Mesoscale Evolution of Hydraulic Fractures in Hydrate-Bearing Sediments" Energies 16, no. 22: 7502. https://doi.org/10.3390/en16227502
APA StyleLiang, X., Zhao, H., Dang, Y., Lei, Q., Wang, S., Wang, X., Chai, H., Jia, J., & Wang, Y. (2023). Numerical Investigation on Mesoscale Evolution of Hydraulic Fractures in Hydrate-Bearing Sediments. Energies, 16(22), 7502. https://doi.org/10.3390/en16227502





