Heat Transfer and Thermal Energy Storage Enhancement by Foams and Nanoparticles
Abstract
:1. Introduction
2. Mesoscale Flow Characteristics of Fluid-Saturated Metal Foams
2.1. Use of μCT Images for Single-Phase Flow Simulations
2.2. Two-Phase Heat Transfer inside Microfinned Tubes
2.3. Metal Foams as an Engineered Periodic Structure
3. Macroscale Flow Models in Metal Foams and Comparison with Mesoscale Data
3.1. Metal Foams and Local Thermal Non-Equilibrium
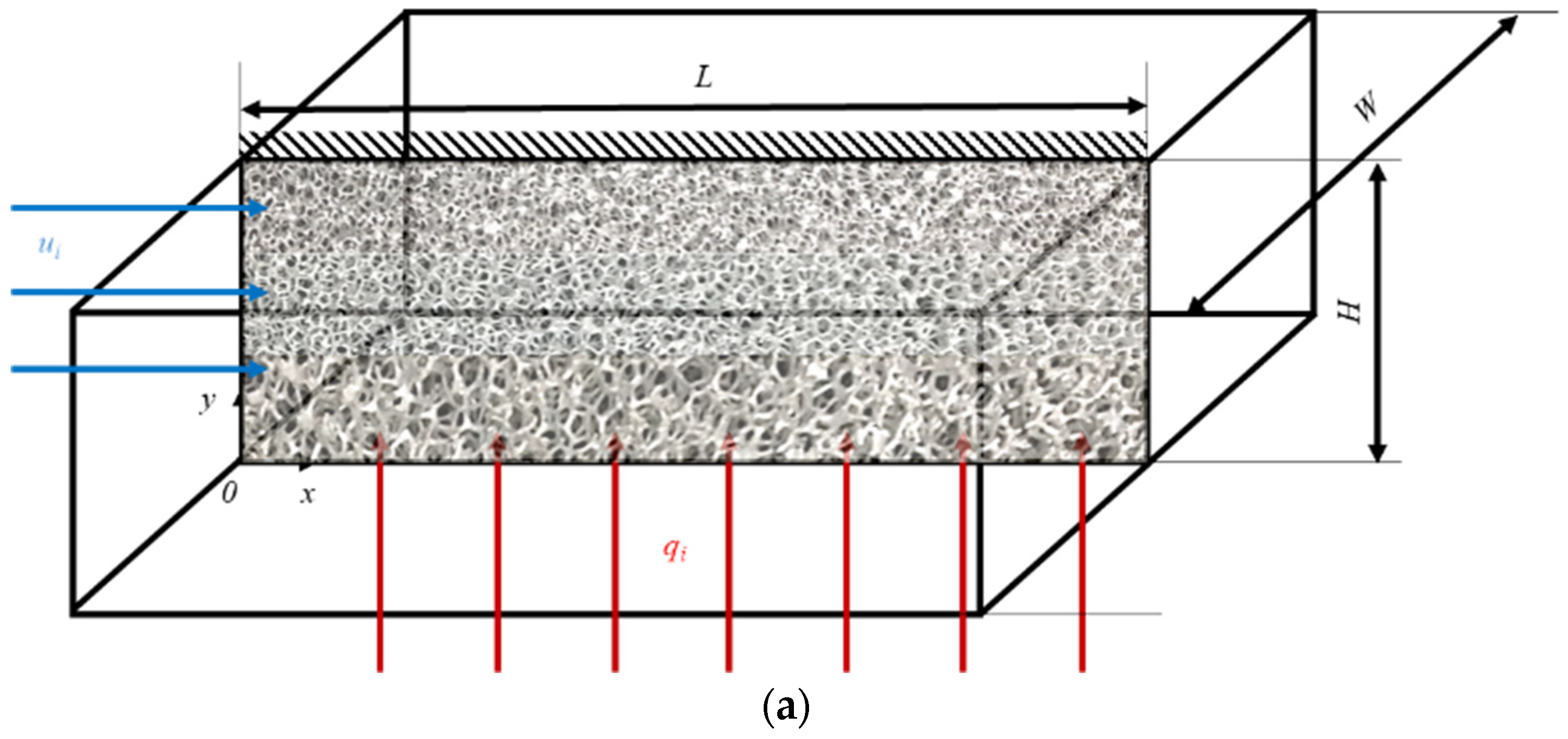
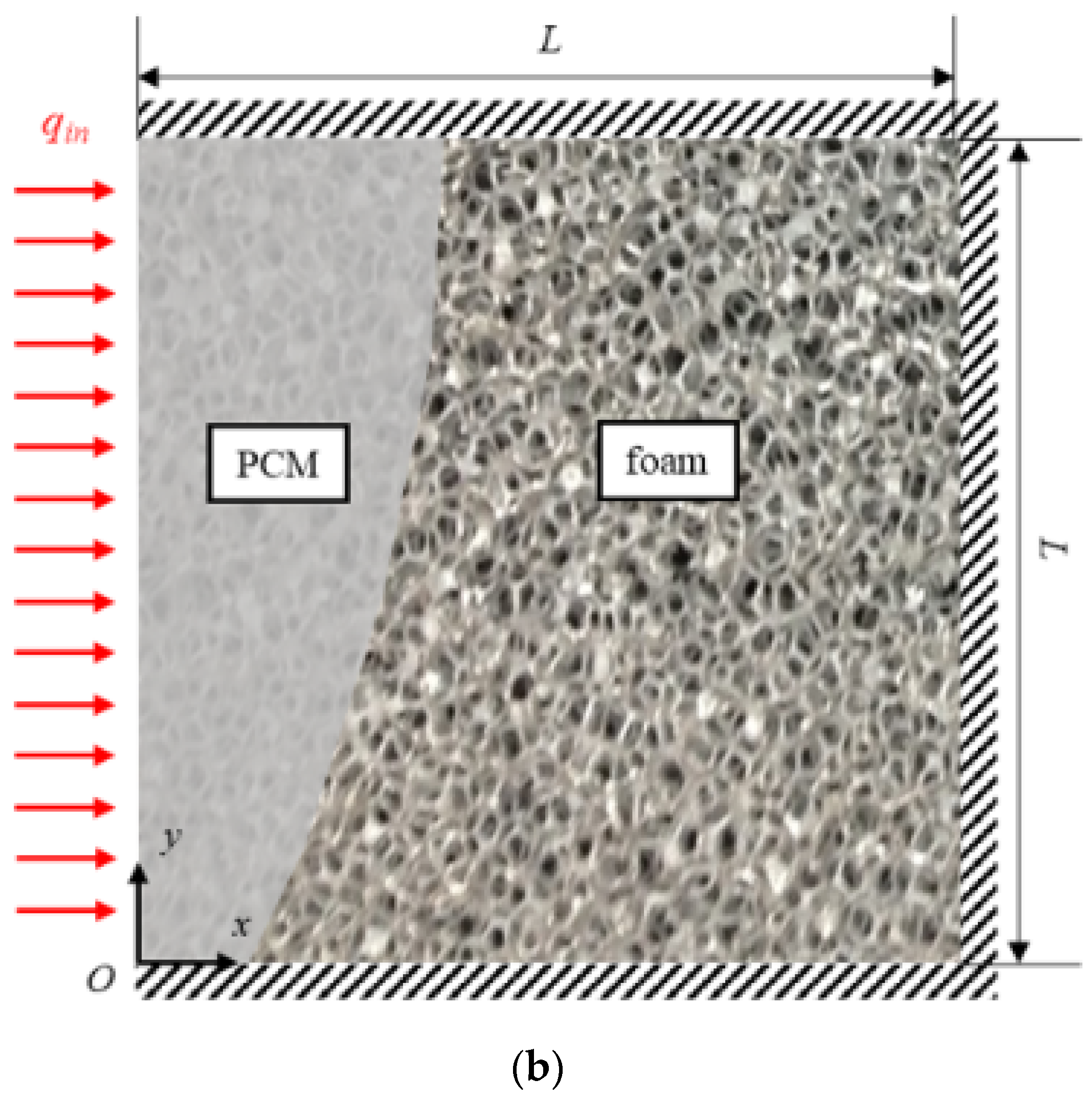
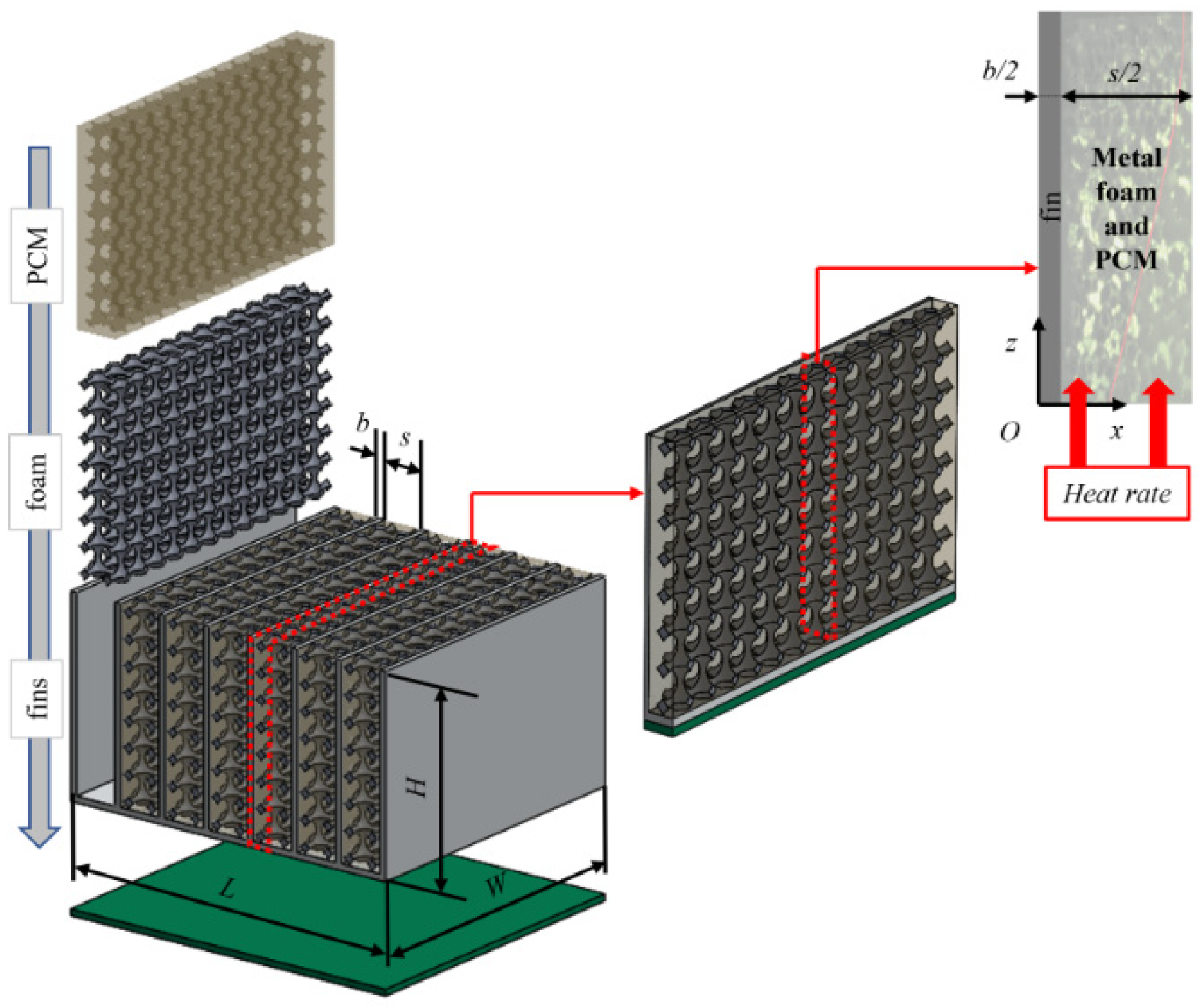
3.2. Additive-Manufactured Porous Media Impregnated with PCMs
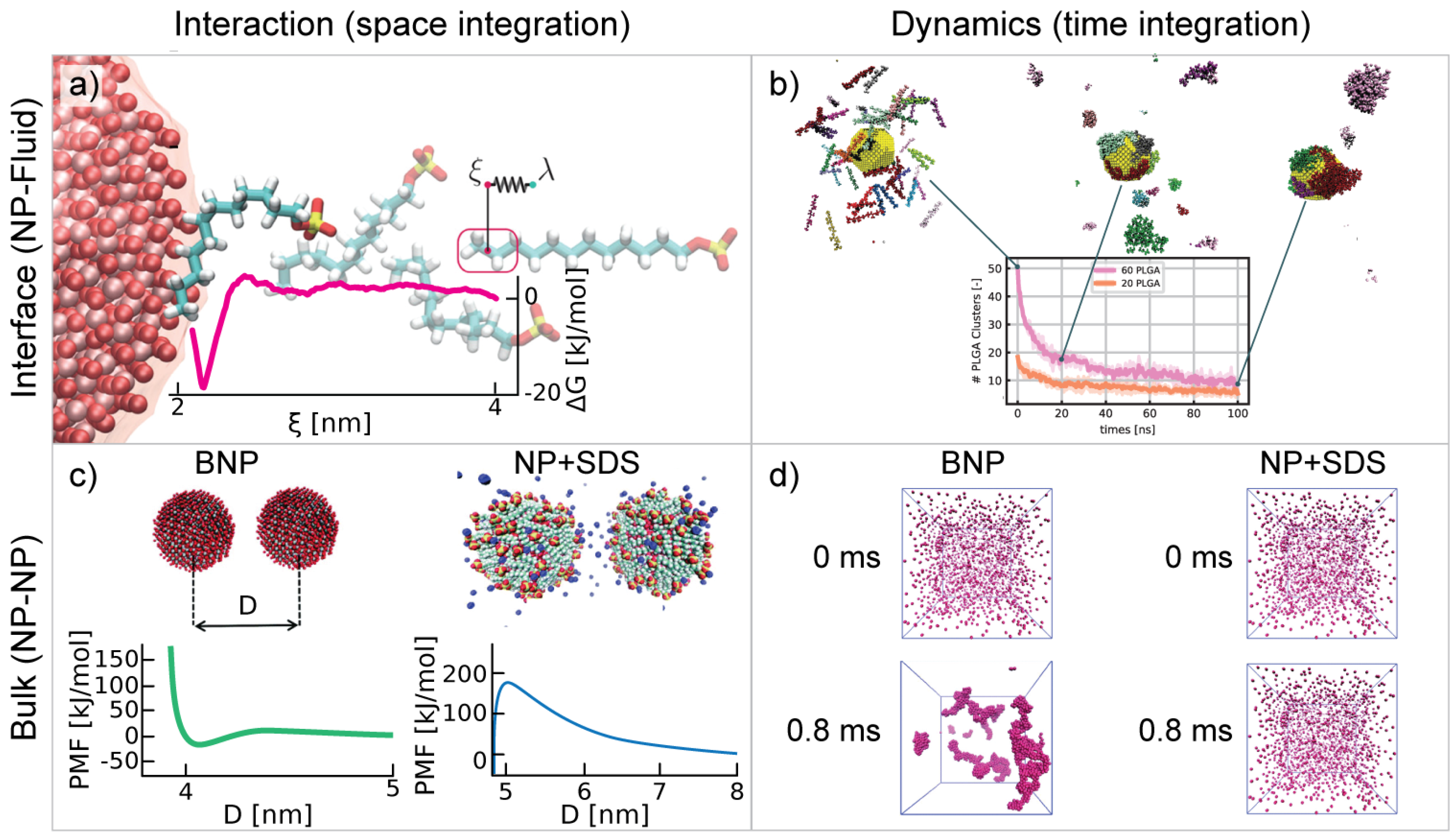
4. Nanofluids: Models and Experimental Data at Nanoscale and Macroscale
Physical Properties of Nanofluids
5. Applications of Metal Foams, Microchannels, and Nanofluids to PCM Thermal Energy Storage Devices and Heat Exchangers
5.1. Metal Foams Applications
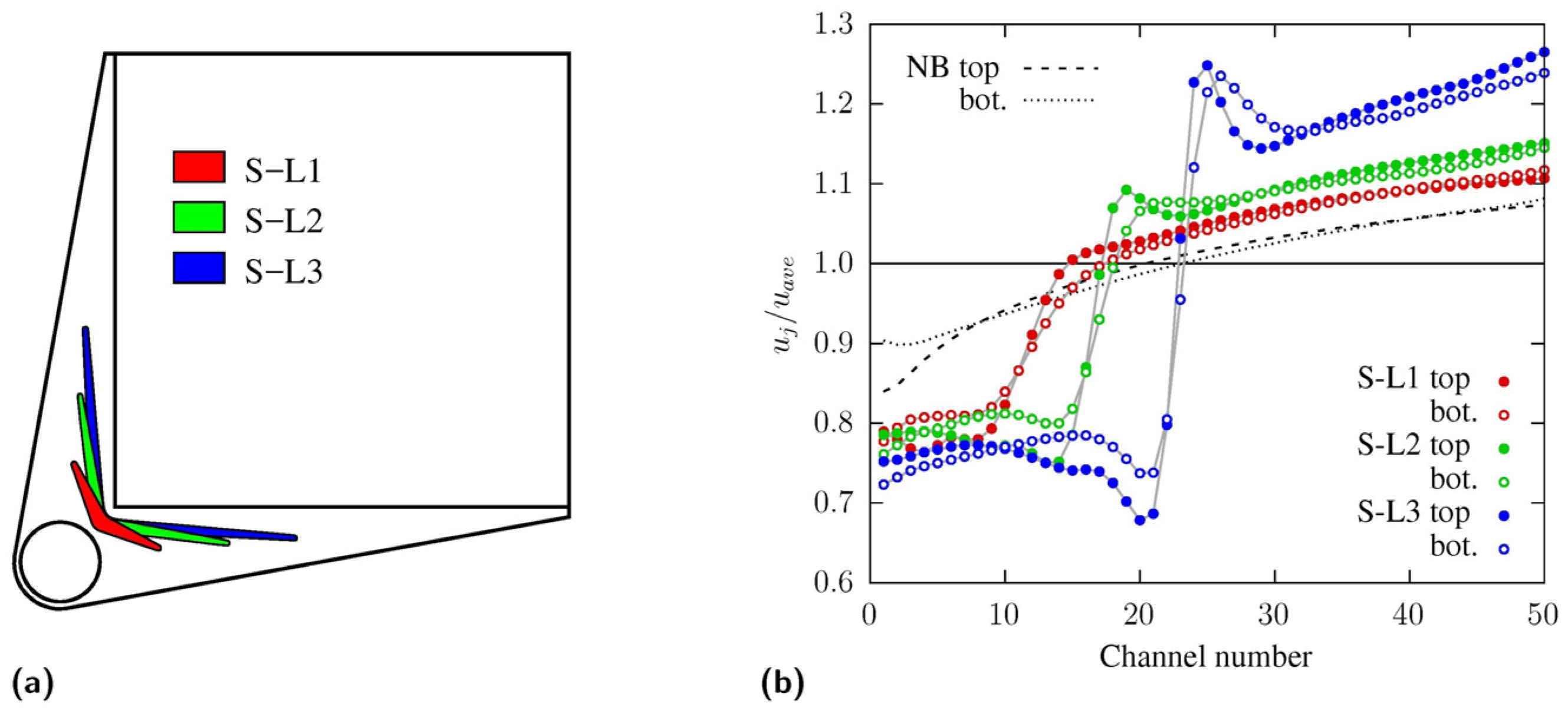
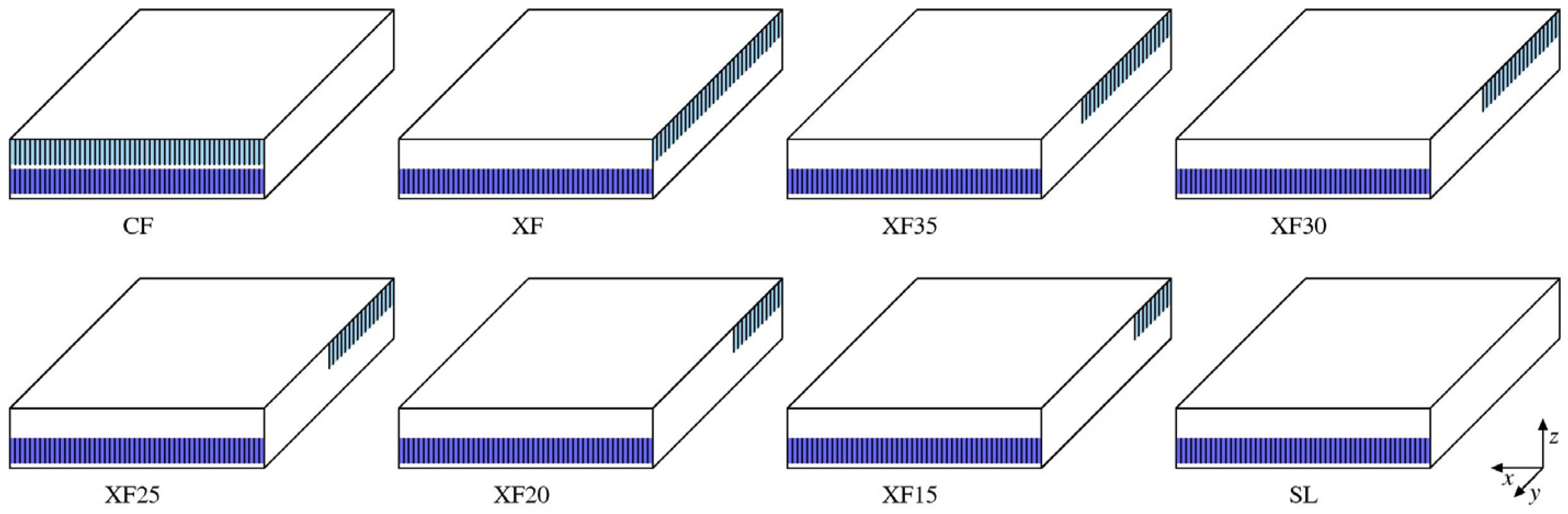
5.2. Effects of Non-Uniform Flow Distribution in Cross-Flow Double-Layered Microchannel Heat Sinks
5.3. Effects of Nanofluids in Heat Transfer Applications
5.4. Use of Phase Change Materials in Thermal Control and Thermal Energy Storage
6. Conclusions and Future Developments
Funding
Conflicts of Interest
References
- Nield, D.A.; Bejan, A. Convection in Porous Media, 4th ed.; Springer: New York, NY, USA, 2013; ISBN 978-1-4899-9822-4. [Google Scholar]
- Barletta, A. Thermal Instabilities in a Fluid Saturated Porous Medium. In Heat Transfer in Multi-Phase Materials; Öchsner, A., Murch, G.E., Eds.; Springer: Berlin/Heidelberg, Germany, 2011; pp. 381–414. ISBN 978-3-642-04403-8. [Google Scholar]
- Ambrosio, G.; Bianco, N.; Chiu, W.K.S.; Iasiello, M.; Naso, V.; Oliviero, M. The Effect of Open-Cell Metal Foams Strut Shape on Convection Heat Transfer and Pressure Drop. Appl. Therm. Eng. 2016, 103, 333–343. [Google Scholar] [CrossRef]
- Pusterla, S.; Ortona, A.; D’Angelo, C.; Barbato, M. The Influence of Cell Morphology on the Effective Thermal Conductivity of Reticulated Ceramic Foams. J. Porous Mater. 2012, 19, 307–315. [Google Scholar] [CrossRef]
- Pusterla, S.; Barbato, M.; Ortona, A.; D’Angelo, C. Numerical Study of Cell Morphology Effects on Convective Heat Transfer in Reticulated Ceramics. Int. J. Heat Mass Transf. 2012, 55, 7902–7910. [Google Scholar] [CrossRef]
- Yang, X.H.; Bai, J.X.; Yan, H.B.; Kuang, J.J.; Lu, T.J.; Kim, T. An Analytical Unit Cell Model for the Effective Thermal Conductivity of High Porosity Open-Cell Metal Foams. Transp. Porous Media 2014, 102, 403–426. [Google Scholar] [CrossRef]
- Hast, A.; Rinne, S.; Syri, S.; Kiviluoma, J. The Role of Heat Storages in Facilitating the Adaptation of District Heating Systems to Large Amount of Variable Renewable Electricity. Energy 2017, 137, 775–788. [Google Scholar] [CrossRef]
- Thaker, S.; Olufemi Oni, A.; Kumar, A. Techno-Economic Evaluation of Solar-Based Thermal Energy Storage Systems. Energy Convers. Manag. 2017, 153, 423–434. [Google Scholar] [CrossRef]
- Yang, W.; Zhang, H.; Liang, X. Experimental Performance Evaluation and Parametric Study of a Solar-Ground Source Heat Pump System Operated in Heating Modes. Energy 2018, 149, 173–189. [Google Scholar] [CrossRef]
- Dincer, I. On Thermal Energy Storage Systems and Applications in Buildings. Energy Build. 2002, 34, 377–388. [Google Scholar] [CrossRef]
- Deng, D.; Wan, W.; Qin, Y.; Zhang, J.; Chu, X. Flow Boiling Enhancement of Structured Microchannels with Micro Pin Fins. Int. J. Heat Mass Transf. 2017, 105, 338–349. [Google Scholar] [CrossRef]
- Dhumane, R.; Mallow, A.; Qiao, Y.; Gluesenkamp, K.R.; Graham, S.; Ling, J.; Radermacher, R. Enhancing the Thermosiphon-Driven Discharge of a Latent Heat Thermal Storage System Used in a Personal Cooling Device. Int. J. Refrig. 2018, 88, 599–613. [Google Scholar] [CrossRef]
- Son, K.N.; Weibel, J.A.; Kumaresan, V.; Garimella, S.V. Design of Multifunctional Lattice-Frame Materials for Compact Heat Exchangers. Int. J. Heat Mass Transf. 2017, 115, 619–629. [Google Scholar] [CrossRef]
- Mancin, S.; Diani, A.; Doretti, L.; Hooman, K.; Rossetto, L. Experimental Analysis of Phase Change Phenomenon of Paraffin Waxes Embedded in Copper Foams. Int. J. Therm. Sci. 2015, 90, 79–89. [Google Scholar] [CrossRef]
- Mallow, A.; Abdelaziz, O.; Graham, S. Thermal Charging Performance of Enhanced Phase Change Material Composites for Thermal Battery Design. Int. J. Therm. Sci. 2018, 127, 19–28. [Google Scholar] [CrossRef]
- Taylor, R.; Coulombe, S.; Otanicar, T.; Phelan, P.; Gunawan, A.; Lv, W.; Rosengarten, G.; Prasher, R.; Tyagi, H. Small Particles, Big Impacts: A Review of the Diverse Applications of Nanofluids. J. Appl. Phys. 2013, 113, 011301. [Google Scholar] [CrossRef]
- Lomascolo, M.; Colangelo, G.; Milanese, M.; de Risi, A. Review of Heat Transfer in Nanofluids: Conductive, Convective and Radiative Experimental Results. Renew. Sustain. Energy Rev. 2015, 43, 1182–1198. [Google Scholar] [CrossRef]
- Colangelo, G.; Favale, E.; Milanese, M.; de Risi, A.; Laforgia, D. Cooling of Electronic Devices: Nanofluids Contribution. Appl. Therm. Eng. 2017, 127, 421–435. [Google Scholar] [CrossRef]
- Israelachvili, J.N. (Ed.) Intermolecular and Surface Forces. In Intermolecular and Surface Forces, 3rd ed.; Academic Press: Boston, MA, USA, 2011; p. iii. ISBN 978-0-12-391927-4. [Google Scholar]
- Cardellini, A. Modelling of Multi-Scale Phenomena in Nanoparticle Suspensions. Ph.D. Thesis, Politecnico di Torino, Torino, TO, Italy, 2017. [Google Scholar]
- Liu, C.; Rao, Z.; Zhao, J.; Huo, Y.; Li, Y. Review on Nanoencapsulated Phase Change Materials: Preparation, Characterization and Heat Transfer Enhancement. Nano Energy 2015, 13, 814–826. [Google Scholar] [CrossRef]
- Ercole, D.; Manca, O.; Vafai, K. An Investigation of Thermal Characteristics of Eutectic Molten Salt-Based Nanofluids. Int. Commun. Heat Mass Transf. 2017, 87, 98–104. [Google Scholar] [CrossRef]
- Feng, S.; Shi, M.; Li, Y.; Lu, T.J. Pore-Scale and Volume-Averaged Numerical Simulations of Melting Phase Change Heat Transfer in Finned Metal Foam. Int. J. Heat Mass Transf. 2015, 90, 838–847. [Google Scholar] [CrossRef]
- Stephen, W. Advances in Theory of Fluid Motion in Porous Media. Ind. Eng. Chem. 1969, 61, 14–28. [Google Scholar] [CrossRef]
- Hill, R. Elastic Properties of Reinforced Solids: Some Theoretical Principles. J. Mech. Phys. Solids 1963, 11, 357–372. [Google Scholar] [CrossRef]
- Diani, A.; Bodla, K.K.; Rossetto, L.; Garimella, S.V. Numerical Investigation of Pressure Drop and Heat Transfer through Reconstructed Metal Foams and Comparison against Experiments. Int. J. Heat Mass Transf. 2015, 88, 508–515. [Google Scholar] [CrossRef]
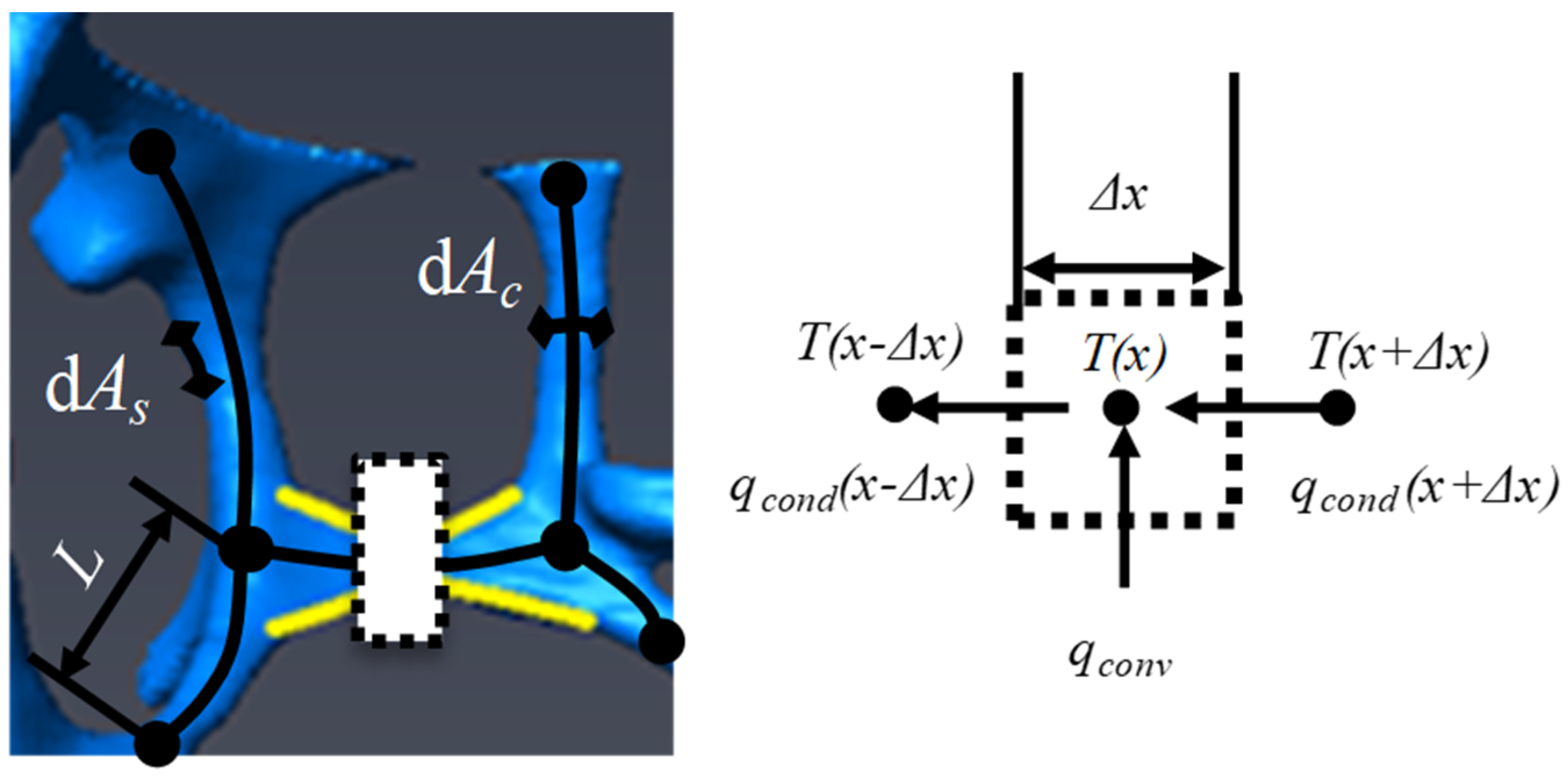
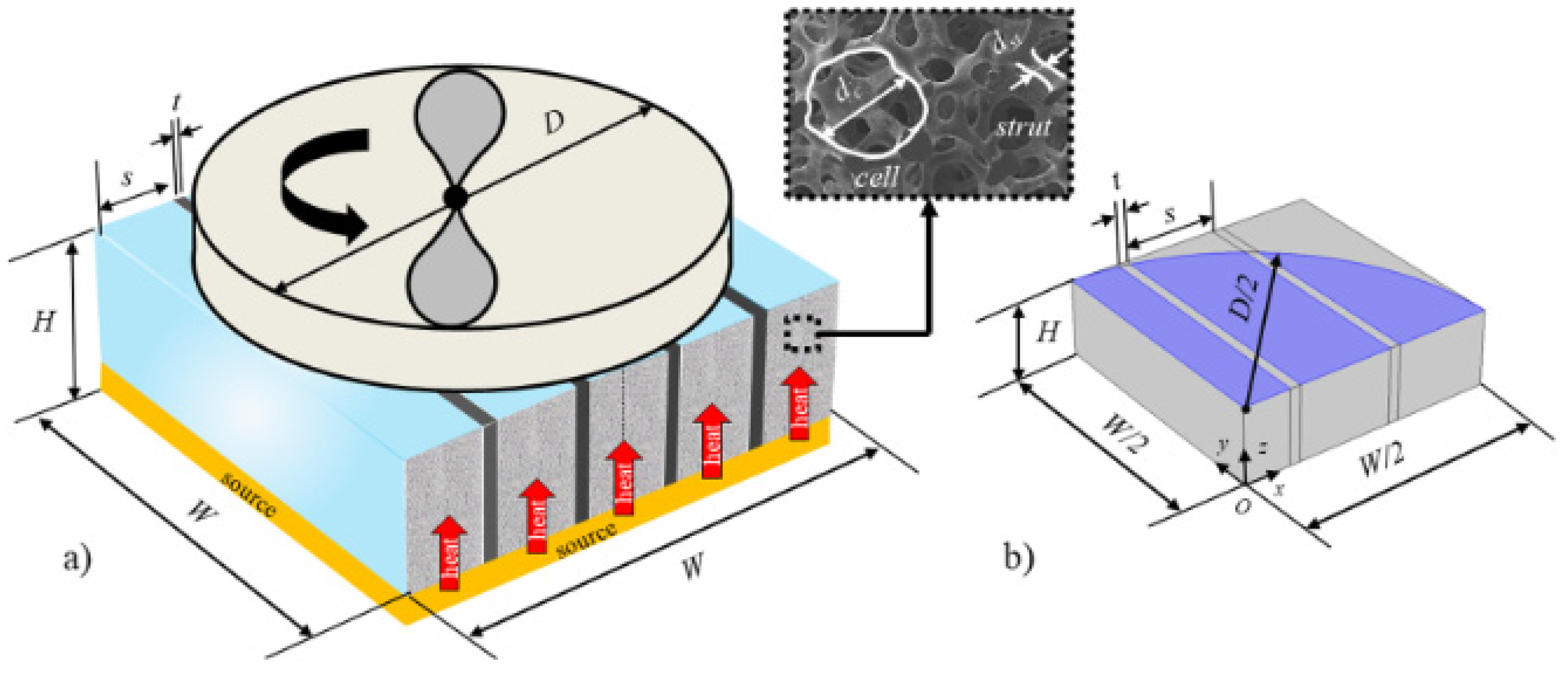
- Iasiello, M.; Savarese, C.; Damian, P.J.; Bianco, N.; Andreozzi, A.; Chiu, W.K.S.; Naso, V. Modeling Heat Conduction in Open-Cell Metal Foams by Means of the Three-Dimensional Thermal Fin Theory. J. Phys. Conf. Ser. 2019, 1224, 012009. [Google Scholar] [CrossRef]
- Nelson, G.J.; Nakajo, A.; Cassenti, B.N.; Degostin, M.B.; Bagshaw, K.R.; Peracchio, A.A.; Xiao, G.; Wang, S.; Chen, F.; Chiu, W.K.S. A Rapid Analytical Assessment Tool for Three Dimensional Electrode Microstructural Networks with Geometric Sensitivity. J. Power Sources 2014, 246, 322–334. [Google Scholar] [CrossRef]
- Iasiello, M.; Bianco, N.; Chiu, W.K.S.; Naso, V. Thermal Conduction in Open-Cell Metal Foams: Anisotropy and Representative Volume Element. Int. J. Therm. Sci. 2019, 137, 399–409. [Google Scholar] [CrossRef]
- Gibson, L.J.; Ashby, M.F. Cellular Solids: Structure and Properties, 2nd ed.; Cambridge University Press: Cambridge, UK, 2014; p. 510. [Google Scholar]
- Mancin, S.; Zilio, C.; Diani, A.; Rossetto, L. Air Forced Convection through Metal Foams: Experimental Results and Modeling. Int. J. Heat Mass Transf. 2013, 62, 112–123. [Google Scholar] [CrossRef]
- Iasiello, M.; Bianco, N.; Chiu, W.K.S.; Naso, V. Anisotropic Convective Heat Transfer in Open-Cell Metal Foams: Assessment and Correlations. Int. J. Heat Mass Transf. 2020, 154, 119682. [Google Scholar] [CrossRef]
- Iasiello, M.; Bianco, N.; Chiu, W.K.S.; Naso, V. Anisotropy Effects on Convective Heat Transfer and Pressure Drop in Kelvin’s Open-Cell Foams. J. Phys. Conf. Ser. 2017, 923, 012035. [Google Scholar] [CrossRef]
- Diani, A.; Rossetto, L. Characteristics of R513A Evaporation Heat Transfer inside Small-Diameter Smooth and Microfin Tubes. Int. J. Heat Mass Transf. 2020, 162, 120402. [Google Scholar] [CrossRef]
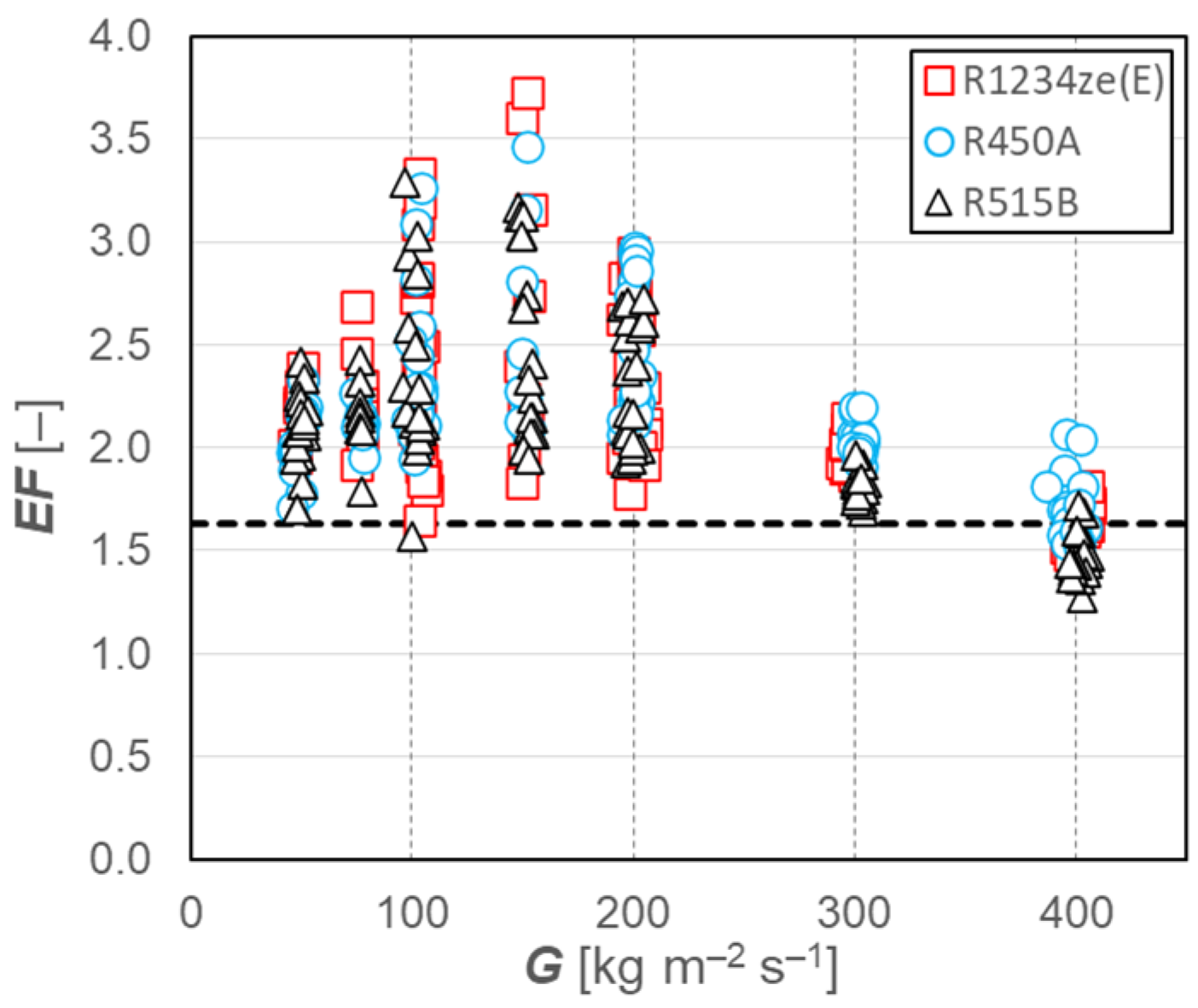
- Liu, Y.; Rossetto, L.; Diani, A. Flow Boiling of R450A, R515B, and R1234ze(E) Inside a 7.0 Mm OD Microfin Tube: Experimental Comparison and Analysis of Boiling Mechanisms. Appl. Sci. 2022, 12, 12450. [Google Scholar] [CrossRef]
- Diani, A.; Brunello, P.; Rossetto, L. R513A Condensation Heat Transfer inside Tubes: Microfin Tube vs. Smooth Tube. Int. J. Heat Mass Transf. 2020, 152, 119472. [Google Scholar] [CrossRef]
- Diani, A.; Liu, Y.; Wen, J.; Rossetto, L. Experimental Investigation on the Flow Condensation of R450A, R515B, and R1234ze(E) in a 7.0 Mm OD Micro-Fin Tube. Int. J. Heat Mass Transf. 2022, 196, 123260. [Google Scholar] [CrossRef]
- Ranut, P.; Nobile, E.; Mancini, L. High Resolution Microtomography-Based CFD Simulation of Flow and Heat Transfer in Aluminum Metal Foams. Appl. Therm. Eng. 2014, 69, 230–240. [Google Scholar] [CrossRef]
- Iasiello, M.; Cunsolo, S.; Bianco, N.; Chiu, W.K.S.; Naso, V. Developing Thermal Flow in Open-Cell Foams. Int. J. Therm. Sci. 2017, 111, 129–137. [Google Scholar] [CrossRef]
- Frazier, W.E. Metal Additive Manufacturing: A Review. J. Mater. Eng. Perform. 2014, 23, 1917–1928. [Google Scholar] [CrossRef]
- Ahmed, N.; Barsoum, I.; Abu Al-Rub, R.K. Numerical Investigation on the Effect of Residual Stresses on the Effective Mechanical Properties of 3D-Printed TPMS Lattices. Metals 2022, 12, 1344. [Google Scholar] [CrossRef]
- Novak, N.; Kytyr, D.; Rada, V.; Doktor, T.; Al-Ketan, O.; Rowshan, R.; Vesenjak, M.; Ren, Z. Compression Behaviour of TPMS-Filled Stainless Steel Tubes. Mater. Sci. Eng. A 2022, 852, 143680. [Google Scholar] [CrossRef]
- Duarte, I.; Krstulović-Opara, L.; Vesenjak, M. Characterisation of Aluminium Alloy Tubes Filled with Aluminium Alloy Integral-Skin Foam under Axial Compressive Loads. Compos. Struct. 2015, 121, 154–162. [Google Scholar] [CrossRef]
- Duarte, I.; Vesenjak, M.; Krstulović-Opara, L.; Ren, Z. Static and Dynamic Axial Crush Performance of In-Situ Foam-Filled Tubes. Compos. Struct. 2015, 124, 128–139. [Google Scholar] [CrossRef]
- Duarte, I.; Vesenjak, M.; Krstulović-Opara, L.; Ren, Z. Compressive Performance Evaluation of APM (Advanced Pore Morphology) Foam Filled Tubes. Compos. Struct. 2015, 134, 409–420. [Google Scholar] [CrossRef]
- Pulvirenti, B.; Celli, M.; Barletta, A. Flow and Convection in Metal Foams: A Survey and New CFD Results. Fluids 2020, 5, 155. [Google Scholar] [CrossRef]
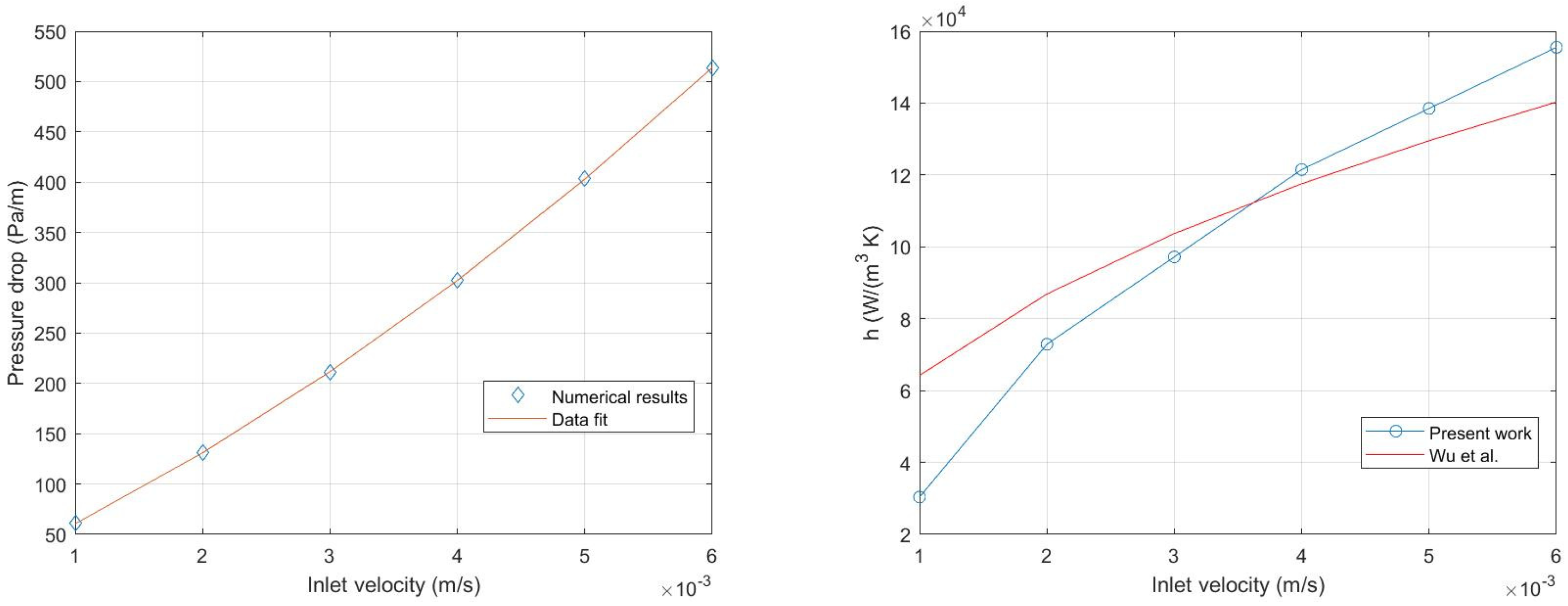
- Wu, Z.; Caliot, C.; Flamant, G.; Wang, Z. Numerical simulation of convective heat transfer between air flow and ceramic foams to optimise volumetric solar air receiver performances. Int. J. Heat Mass Transf. 2011, 54, 1527–1537. [Google Scholar] [CrossRef]
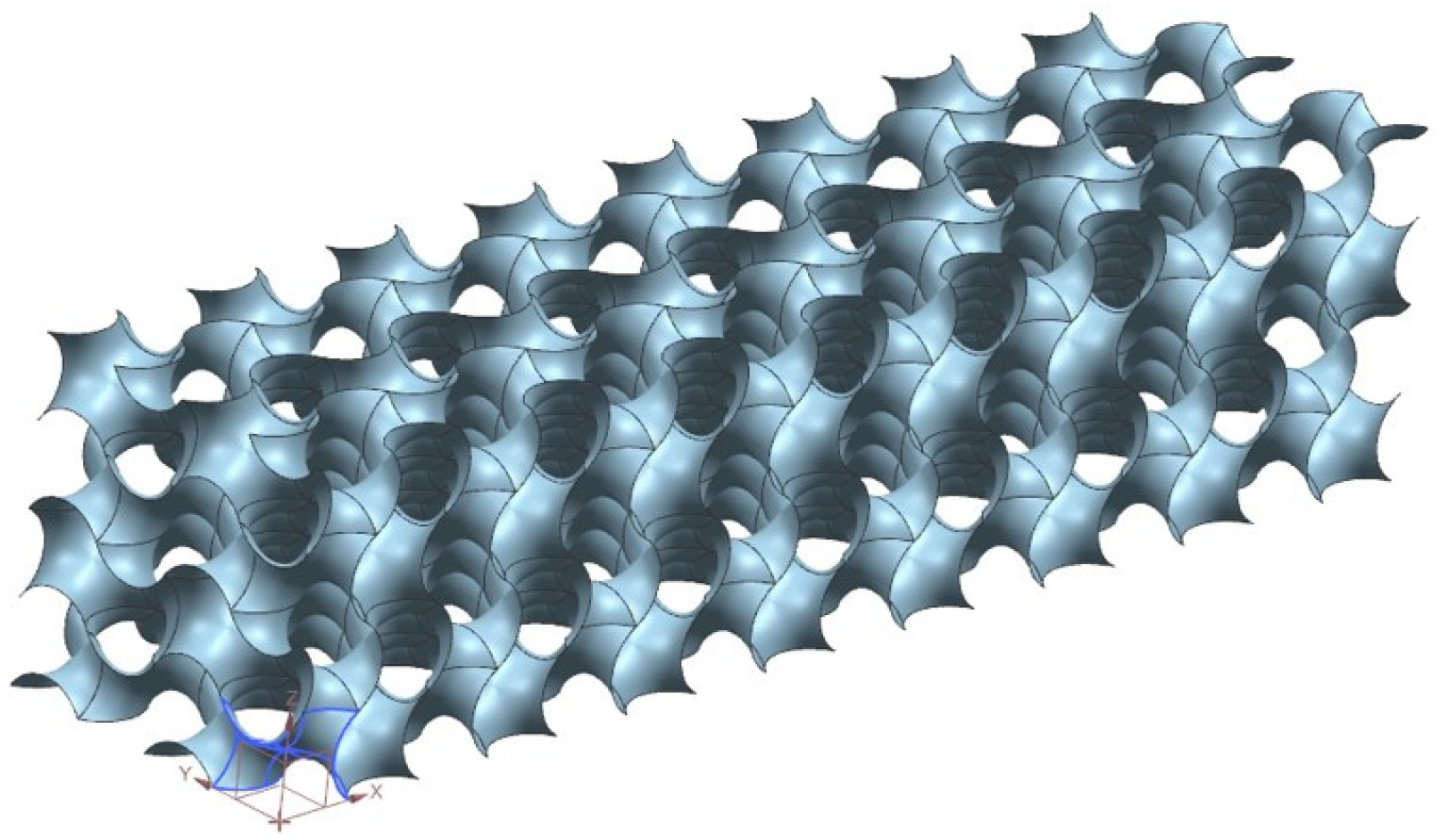
- Buonomo, B.; di Pasqua, A.; Manca, O.; Nappo, S.; Nardini, S. Analysis at Cell Scale of Porosity Effect on Forced Convection with Nanofluids in Porous Structures with Kelvin Cells. Int. J. Thermofluids 2022, 16, 100215. [Google Scholar] [CrossRef]
- Buonomo, B.; Pasqua, A.D.; Manca, O.; Sekrani, G.; Poncet, S. Numerical Analysis on Pressure Drop and Heat Transfer in Nanofluids at Pore Length Scale in Open Metal Porous Structures with Kelvin Cells. Heat Transf. Eng. 2021, 42, 1614–1624. [Google Scholar] [CrossRef]
- Buonomo, B.; di Pasqua, A.; Manca, O.; Nappo, S.; Nardini, S. Entropy Generation Analysis of Laminar Forced Convection with Nanofluids at Pore Length Scale in Porous Structures with Kelvin Cells. Int. Commun. Heat Mass Transf. 2022, 132, 105883. [Google Scholar] [CrossRef]
- Sabet, S.; Barisik, M.; Buonomo, B.; Manca, O. Thermal and Hydrodynamic Behavior of Forced Convection Gaseous Slip Flow in a Kelvin Cell Metal Foam. Int. Commun. Heat Mass Transf. 2022, 131, 105838. [Google Scholar] [CrossRef]
- Buonomo, B.; Cinquegrana, D.; Ferraiuolo, M.; Manca, O.; Nardini, S.; Plomitallo, R.E. Numerical Investigation on Thermal and Fluid Dynamic Behaviours in Ice Melting at Pore Scale. Case Stud. Therm. Eng. 2023, 47, 103117. [Google Scholar] [CrossRef]
- Mahjoob, S.; Vafai, K. A Synthesis of Fluid and Thermal Transport Models for Metal Foam Heat Exchangers. Int. J. Heat Mass Transf. 2008, 51, 3701–3711. [Google Scholar] [CrossRef]
- Freitas, R.B.; Brandão, P.V.; Alves, L.S.D.B.; Celli, M.; Barletta, A. The Effect of Local Thermal Non-Equilibrium on the Onset of Thermal Instability for a Metallic Foam. Phys. Fluids 2022, 34, 034105. [Google Scholar] [CrossRef]
- Buonomo, B.; Cascetta, F.; Manca, O.; Sheremet, M. Heat Transfer Analysis of Rectangular Porous Fins in Local Thermal Non-Equilibrium Model. Appl. Therm. Eng. 2021, 195, 117237. [Google Scholar] [CrossRef]
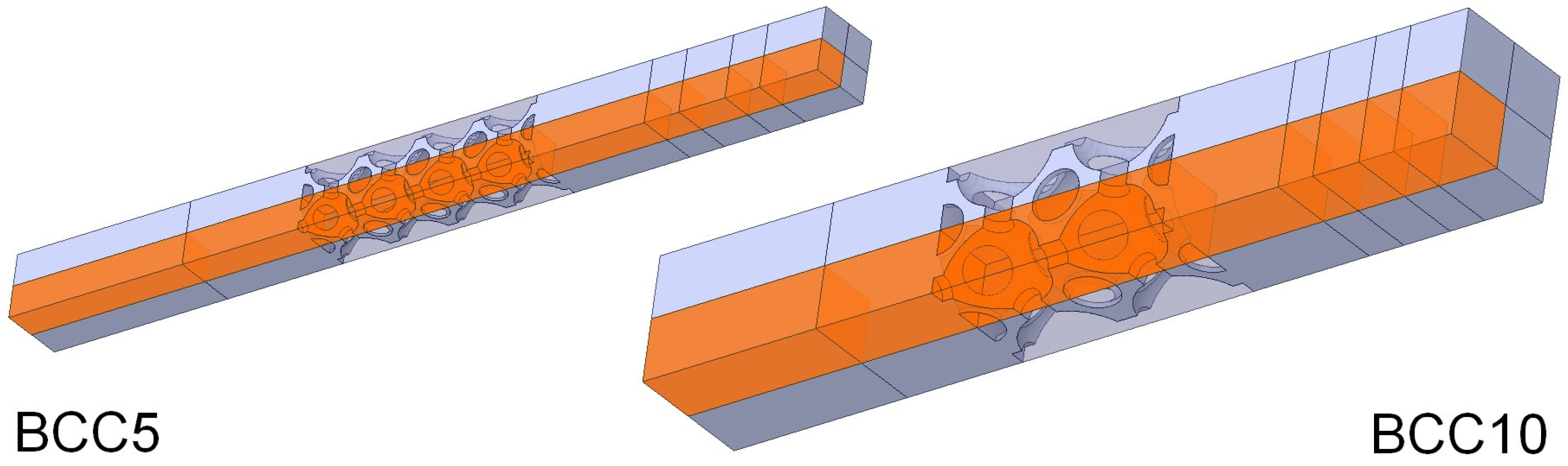
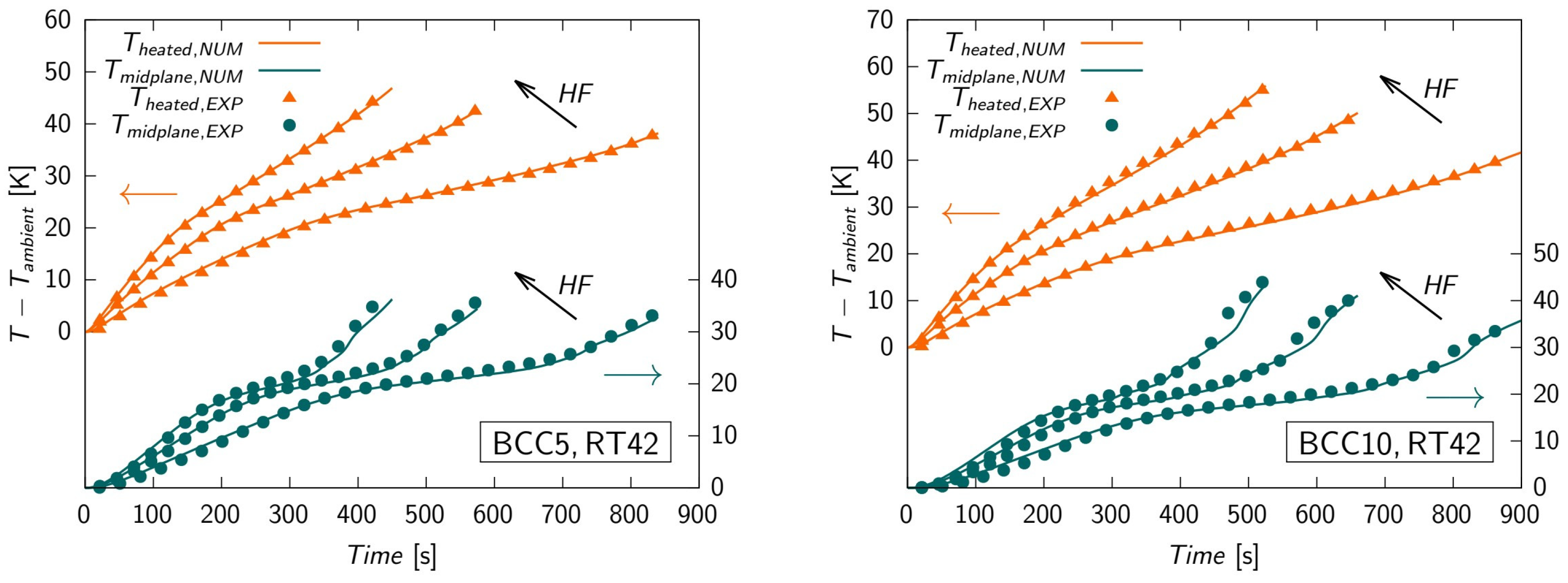
- Diani, A.; Nonino, C.; Rossetto, L. Melting of Phase Change Materials inside Periodic Cellular Structures Fabricated by Additive Manufacturing: Experimental Results and Numerical Simulations. Appl. Therm. Eng. 2022, 215, 118969. [Google Scholar] [CrossRef]
- Diani, A.; Moro, L.; Rossetto, L. Melting of Paraffin Waxes Embedded in a Porous Matrix Made by Additive Manufacturing. Appl. Sci. 2021, 11, 5396. [Google Scholar] [CrossRef]
- Nonino, C.; Diani, A.; Rossetto, L. Numerical Analysis of the Thermal Energy Storage in Cellular Structures Filled with Phase-Change Material. J. Phys. Conf. Ser. 2022, 2385, 012024. [Google Scholar] [CrossRef]
- Sabet, S.; Buonomo, B.; Sheremet, M.A.; Manca, O. Numerical Investigation of Melting Process for Phase Change Material (PCM) Embedded in Metal Foam Structures with Kelvin Cells at Pore Scale Level. Int. J. Heat Mass Transf. 2023, 214, 124440. [Google Scholar] [CrossRef]
- Misale, M.; Bocanegra, J.A.; Marchitto, A. Long-Term Experimental Study on Gravitational Sedimentation of Water Aluminum Oxide Nanofluid at Different Volumetric Concentrations. Int. J. Sediment Res. 2023, 38, 303–315. [Google Scholar] [CrossRef]
- Younes, H.; Mao, M.; Sohel Murshed, S.M.; Lou, D.; Hong, H.; Peterson, G.P. Nanofluids: Key Parameters to Enhance Thermal Conductivity and Its Applications. Appl. Therm. Eng. 2022, 207, 118202. [Google Scholar] [CrossRef]
- Bobbo, S.; Buonomo, B.; Manca, O.; Vigna, S.; Fedele, L. Analysis of the Parameters Required to Properly Define Nanofluids for Heat Transfer Applications. Fluids 2021, 6, 65. [Google Scholar] [CrossRef]
- Cardellini, A.; Fasano, M.; Bozorg Bigdeli, M.; Chiavazzo, E.; Asinari, P. Thermal Transport Phenomena in Nanoparticle Suspensions. J. Phys. Condens. Matter 2016, 28, 483003. [Google Scholar] [CrossRef]
- Cardellini, A.; Alberghini, M.; Govind Rajan, A.; Misra, R.P.; Blankschtein, D.; Asinari, P. Multi-Scale Approach for Modeling Stability, Aggregation, and Network Formation of Nanoparticles Suspended in Aqueous Solutions. Nanoscale 2019, 11, 3925–3932. [Google Scholar] [CrossRef]
- Mancardi, G.; Alberghini, M.; Aguilera-Porta, N.; Calatayud, M.; Asinari, P.; Chiavazzo, E. Multi-Scale Modelling of Aggregation of TiO2 Nanoparticle Suspensions in Water. Nanomaterials 2022, 12, 217. [Google Scholar] [CrossRef]
- De Angelis, P.; Cardellini, A.; Asinari, P. Exploring the Free Energy Landscape to Predict the Surfactant Adsorption Isotherm at the Nanoparticle-Water Interface. ACS Cent. Sci. 2019, 5, 1804–1812. [Google Scholar] [CrossRef] [PubMed]
- Cappabianca, R.; De Angelis, P.; Cardellini, A.; Chiavazzo, E.; Asinari, P. Assembling Biocompatible Polymers on Gold Nanoparticles: Toward a Rational Design of Particle Shape by Molecular Dynamics. ACS Omega 2022, 7, 42292–42303. [Google Scholar] [CrossRef] [PubMed]
- Bianco, N.; Iasiello, M.; Mauro, G.M.; Pagano, L. Multi-Objective Optimization of Finned Metal Foam Heat Sinks: Tradeoff between Heat Transfer and Pressure Drop. Appl. Therm. Eng. 2021, 182, 116058. [Google Scholar] [CrossRef]
- Feng, S.S.; Kuang, J.J.; Wen, T.; Lu, T.J.; Ichimiya, K. An Experimental and Numerical Study of Finned Metal Foam Heat Sinks under Impinging Air Jet Cooling. Int. J. Heat Mass Transf. 2014, 77, 1063–1074. [Google Scholar] [CrossRef]
- Bianco, V.; Buonomo, B.; di Pasqua, A.; Manca, O. Heat Transfer Enhancement of Laminar Impinging Slot Jets by Nanofluids and Metal Foams. Therm. Sci. Eng. Prog. 2021, 22, 100860. [Google Scholar] [CrossRef]
- Hassan, A.M.; Alwan, A.A.; Hamzah, H.K. Metallic Foam with Cross Flow Heat Exchanger: A Review of Parameters, Performance, and Challenges. Heat Transf. 2023, 52, 2618–2650. [Google Scholar] [CrossRef]
- Buonomo, B.; di Pasqua, A.; Manca, O.; Nardini, S. Evaluation of Thermal and Fluid Dynamic Performance Parameters in Aluminum Foam Compact Heat Exchangers. Appl. Therm. Eng. 2020, 176, 115456. [Google Scholar] [CrossRef]
- Buonomo, B.; Cascetta, F.; di Pasqua, A.; Manca, O. Performance Parameters Enhancement of a Thermoelectric Generator by Metal Foam in Exhaust Automotive Lines. Therm. Sci. Eng. Prog. 2023, 38, 101684. [Google Scholar] [CrossRef]
- He, Z.; Yan, Y.; Zhang, Z. Thermal Management and Temperature Uniformity Enhancement of Electronic Devices by Micro Heat Sinks: A Review. Energy 2021, 216, 119223. [Google Scholar] [CrossRef]
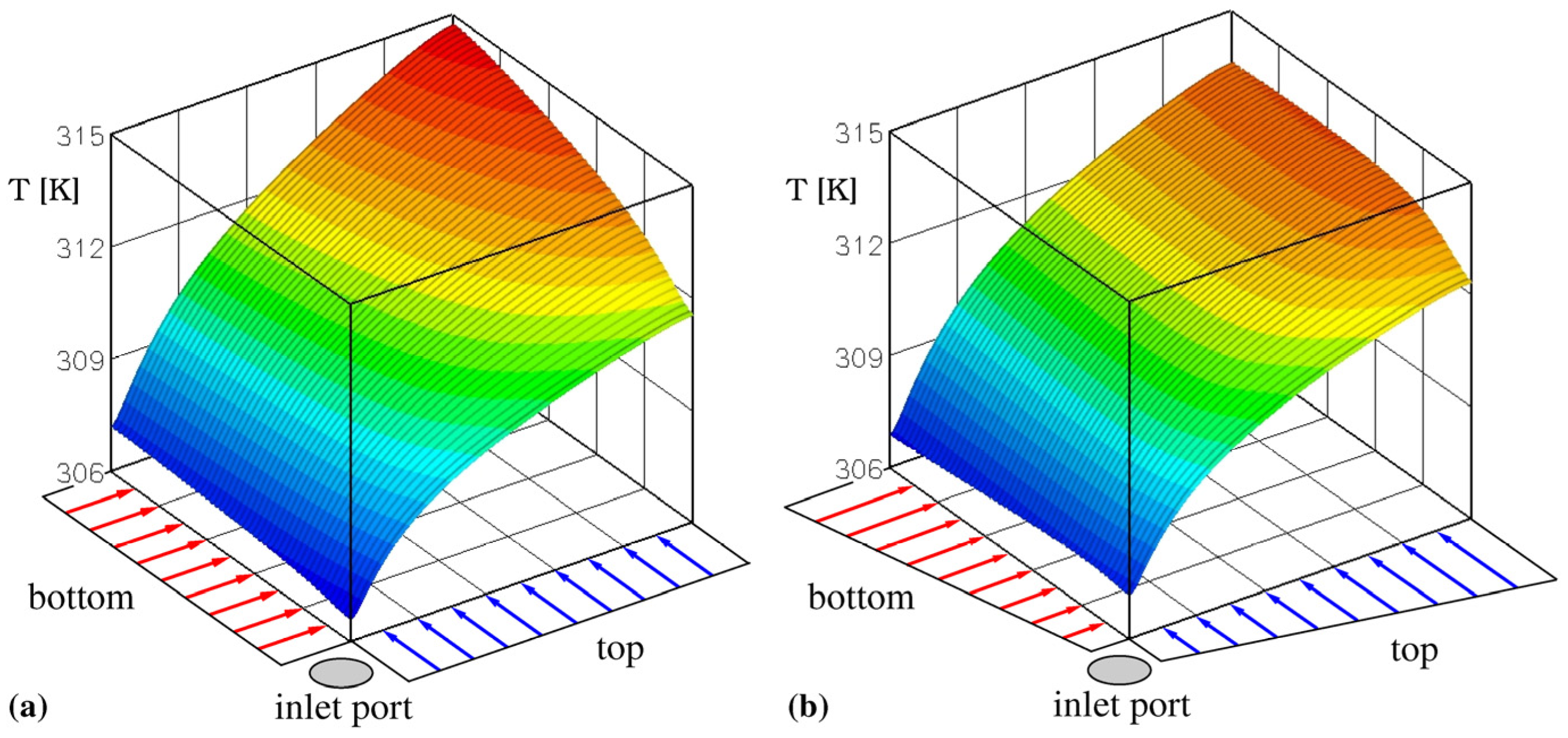
- Nonino, C.; Savino, S. Effects of Non-Uniform Flow Distribution in Double-Layered Cross-Flow Microchannel Heat Sinks. In Proceedings of the Second Pacific Rim Thermal Engineering Conference PRTEC2019, Maui, HI, USA, 13–17 December 2019; pp. 24191.1–24191.5. [Google Scholar]
- Nonino, C.; Savino, S.; Giudice, S.D. FEM for the 3-D Analysis of Conjugate Conduction-Convection Heat Transfer in Cross-Flow Micro Heat Exchangers. Int. J. Numer. Methods Heat Fluid Flow 2015, 25, 1322–1339. [Google Scholar] [CrossRef]
- Nonino, C.; Savino, S. Numerical Investigation on the Performance of Cross-Flow Micro Heat Exchangers. Int. J. Numer. Methods Heat Fluid Flow 2016, 26, 745–766. [Google Scholar] [CrossRef]
- Nonino, C.; Savino, S. Effects of Flow Maldistribution on the Thermal Performance of Cross-Flow Micro Heat Exchangers. J. Phys. Conf. Ser. 2016, 745, 032099. [Google Scholar] [CrossRef]
- Savino, S.; Nonino, C. Header Shape Effect on the Inlet Velocity Distribution in Cross-Flow Double-Layered Microchannel Heat Sinks. Fluids 2022, 7, 7. [Google Scholar] [CrossRef]
- Nonino, C.; Savino, S. Temperature Uniformity in Cross-Flow Double-Layered Microchannel Heat Sinks. Fluids 2020, 5, 143. [Google Scholar] [CrossRef]
- Bigdeli, M.B.; Fasano, M.; Cardellini, A.; Chiavazzo, E.; Asinari, P. A Review on the Heat and Mass Transfer Phenomena in Nanofluid Coolants with Special Focus on Automotive Applications. Renew. Sustain. Energy Rev. 2016, 60, 1615–1633. [Google Scholar] [CrossRef]
- Moradi, A.; Sani, E.; Simonetti, M.; Francini, F.; Chiavazzo, E.; Asinari, P. Carbon-Nanohorn Based Nanofluids for a Direct Absorption Solar Collector for Civil Application. J. Nanosci. Nanotechnol. 2015, 15, 3488–3495. [Google Scholar] [CrossRef]
- Alberghini, M.; Morciano, M.; Bergamasco, L.; Fasano, M.; Lavagna, L.; Humbert, G.; Sani, E.; Pavese, M.; Chiavazzo, E.; Asinari, P. Coffee-Based Colloids for Direct Solar Absorption. Sci. Rep. 2019, 9, 4701. [Google Scholar] [CrossRef]
- Balakin, B.V.; Struchalin, P.G. Corrigendum to “Eco-Friendly and Low-Cost Nanofluid for Direct Absorption Solar Collectors”. Mater. Lett. 2023, 340, 134211. [Google Scholar] [CrossRef]
- Ribezzo, A.; Falciani, G.; Bergamasco, L.; Fasano, M.; Chiavazzo, E. An Overview on the Use of Additives and Preparation Procedure in Phase Change Materials for Thermal Energy Storage with a Focus on Long Term Applications. J. Energy Storage 2022, 53, 105140. [Google Scholar] [CrossRef]
- Aghemo, L.; Lavagna, L.; Chiavazzo, E.; Pavese, M. Comparison of Key Performance Indicators of Sorbent Materials for Thermal Energy Storage with an Economic Focus. Energy Storage Mater. 2023, 55, 130–153. [Google Scholar] [CrossRef]
- Morciano, M.; Alberghini, M.; Fasano, M.; Almiento, M.; Calignano, F.; Manfredi, D.; Asinari, P.; Chiavazzo, E. 3D Printed Lattice Metal Structures for Enhanced Heat Transfer in Latent Heat Storage Systems. J. Energy Storage 2023, 65, 107350. [Google Scholar] [CrossRef]
- Fasano, M.; Ventola, L.; Calignano, F.; Manfredi, D.; Ambrosio, E.P.; Chiavazzo, E.; Asinari, P. Passive Heat Transfer Enhancement by 3D Printed Pitot Tube Based Heat Sink. Int. Commun. Heat Mass Transf. 2016, 74, 36–39. [Google Scholar] [CrossRef]
- Ventola, L.; Robotti, F.; Dialameh, M.; Calignano, F.; Manfredi, D.; Chiavazzo, E.; Asinari, P. Rough Surfaces with Enhanced Heat Transfer for Electronics Cooling by Direct Metal Laser Sintering. Int. J. Heat Mass Transf. 2014, 75, 58–74. [Google Scholar] [CrossRef]
- Chiavazzo, E.; Ventola, L.; Calignano, F.; Manfredi, D.; Asinari, P. A Sensor for Direct Measurement of Small Convective Heat Fluxes: Validation and Application to Micro-Structured Surfaces. Exp. Therm. Fluid Sci. 2014, 55, 42–53. [Google Scholar] [CrossRef]
- Ventola, L.; Scaltrito, L.; Ferrero, S.; Maccioni, G.; Chiavazzo, E.; Asinari, P. Micro-Structured Rough Surfaces by Laser Etching for Heat Transfer Enhancement on Flush Mounted Heat Sinks. J. Phys. Conf. Ser. 2014, 525, 012017. [Google Scholar] [CrossRef]
- Alberghini, M.; Morciano, M.; Giardino, M.; Perrucci, F.; Scaltrito, L.; Janner, D.; Chiavazzo, E.; Fasano, M.; Asinari, P. Textured and Rigid Capillary Materials for Passive Energy-Conversion Devices. Adv. Mater. Interfaces 2022, 9, 2200057. [Google Scholar] [CrossRef]
- Bianco, N.; Busiello, S.; Iasiello, M.; Mauro, G.M. Finned Heat Sinks with Phase Change Materials and Metal Foams: Pareto Optimization to Address Cost and Operation Time. Appl. Therm. Eng. 2021, 197, 117436. [Google Scholar] [CrossRef]
- Mauro, G.M.; Iasiello, M.; Bianco, N.; Chiu, W.K.S.; Naso, V. Mono-and Multi-Objective CFD Optimization of Graded Foam-Filled Channels. Materials 2022, 15, 968. [Google Scholar] [CrossRef]
- Iasiello, M.; Mameli, M.; Filippeschi, S.; Bianco, N. Metal Foam/PCM Melting Evolution Analysis: Orientation and Morphology Effects. Appl. Therm. Eng. 2021, 187, 116572. [Google Scholar] [CrossRef]
- Xu, Z.G.; Zhao, C.Y. Experimental Study on Pool Boiling Heat Transfer in Gradient Metal Foams. Int. J. Heat Mass Transf. 2015, 85, 824–829. [Google Scholar] [CrossRef]
- Webb, R.L.; Eckert, E.R.G. Application of Rough Surfaces to Heat Exchanger Design. Int. J. Heat Mass Transf. 1972, 15, 1647–1658. [Google Scholar] [CrossRef]
- Yilmaz, M.; Comakli, O.; Yapici, S.; Sara, O.N. Performance Evaluation Criteria for Heat Exchangers Based on First Law Analysis. J. Enhanc. Heat Transf. 2005, 12, 121–157. [Google Scholar] [CrossRef]
- Sehrawat, R.; Sahdev, R.K.; Tiwari, S. Heat Storage Material: A Hope in Solar Thermal. Environ. Sci. Pollut. Res. 2022, 30, 11175–11198. [Google Scholar] [CrossRef] [PubMed]
- Buonomo, B.; Manca, O.; Nardini, S.; Plomitallo, R.E. Numerical Study on Latent Heat Thermal Energy Storage System with PCM Partially Filled with Aluminum Foam in Local Thermal Equilibrium. Renew. Energy 2022, 195, 1368–1380. [Google Scholar] [CrossRef]
- Buonomo, B.; Manca, O.; Nardini, S.; Plomitallo, R.E. Numerical Investigation on Shell and Tube Latent Heat Thermal Energy Storage with External Heat Losses Partially Filled with Metal Foam. J. Phys. Conf. Ser. 2022, 2385, 012023. [Google Scholar] [CrossRef]
- Buonomo, B.; Golia, M.R.; Manca, O.; Nardini, S. A Numerical Study on an Integrated Solar Chimney with Latent Heat Thermal Energy Storage in Various Arrangements. Int. J. Sustain. Dev. Plan. 2022, 17, 1693–1698. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Andreozzi, A.; Asinari, P.; Barletta, A.; Bianco, V.; Bocanegra, J.A.; Brandão, P.V.; Buonomo, B.; Cappabianca, R.; Celli, M.; Chiavazzo, E.; et al. Heat Transfer and Thermal Energy Storage Enhancement by Foams and Nanoparticles. Energies 2023, 16, 7421. https://doi.org/10.3390/en16217421
Andreozzi A, Asinari P, Barletta A, Bianco V, Bocanegra JA, Brandão PV, Buonomo B, Cappabianca R, Celli M, Chiavazzo E, et al. Heat Transfer and Thermal Energy Storage Enhancement by Foams and Nanoparticles. Energies. 2023; 16(21):7421. https://doi.org/10.3390/en16217421
Chicago/Turabian StyleAndreozzi, Assunta, Pietro Asinari, Antonio Barletta, Vincenzo Bianco, Johan Augusto Bocanegra, Pedro Vayssière Brandão, Bernardo Buonomo, Roberta Cappabianca, Michele Celli, Eliodoro Chiavazzo, and et al. 2023. "Heat Transfer and Thermal Energy Storage Enhancement by Foams and Nanoparticles" Energies 16, no. 21: 7421. https://doi.org/10.3390/en16217421
APA StyleAndreozzi, A., Asinari, P., Barletta, A., Bianco, V., Bocanegra, J. A., Brandão, P. V., Buonomo, B., Cappabianca, R., Celli, M., Chiavazzo, E., De Angelis, P., Diani, A., Filippeschi, S., Iasiello, M., Manca, O., Nardini, S., Nonino, C., & Rossetto, L. (2023). Heat Transfer and Thermal Energy Storage Enhancement by Foams and Nanoparticles. Energies, 16(21), 7421. https://doi.org/10.3390/en16217421