Shielding Strategies for Electric Field Minimization in Current Transformers: A Finite Element Combined Design of Experiment Approach
Abstract
:1. Introduction
2. Establishment of Transient Field Finite Element Model
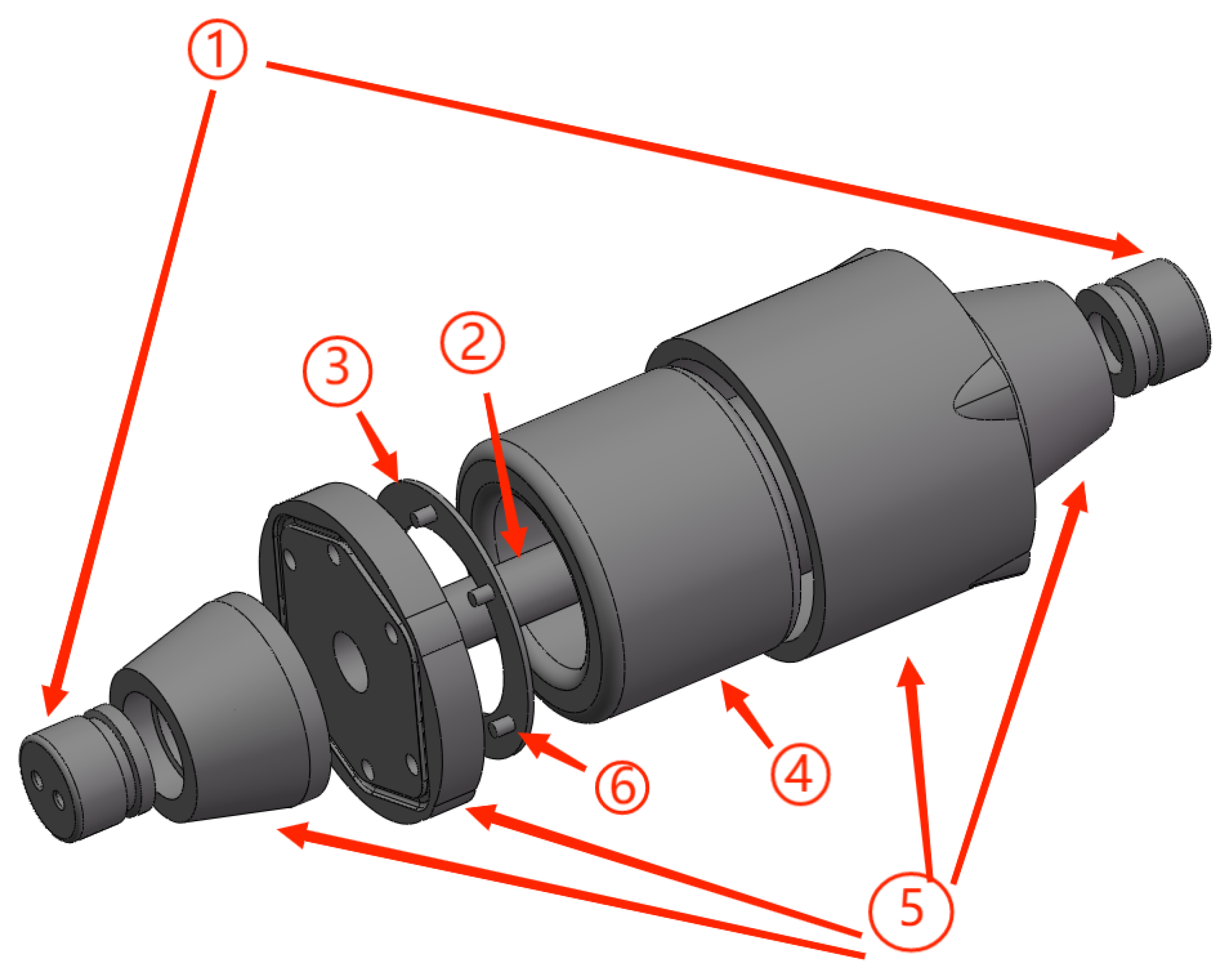
Physical Model
3. Simulation Results and Analysis
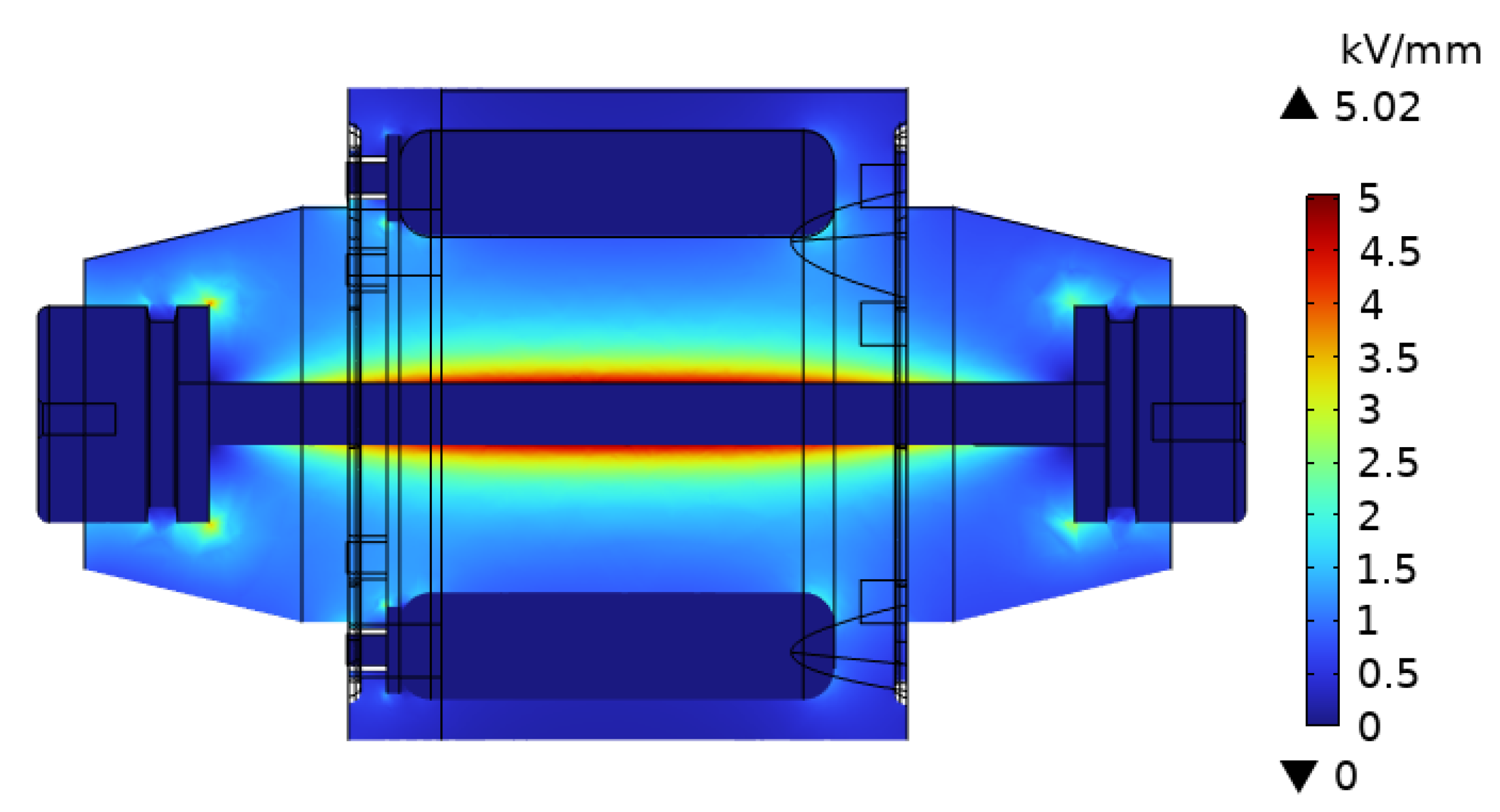
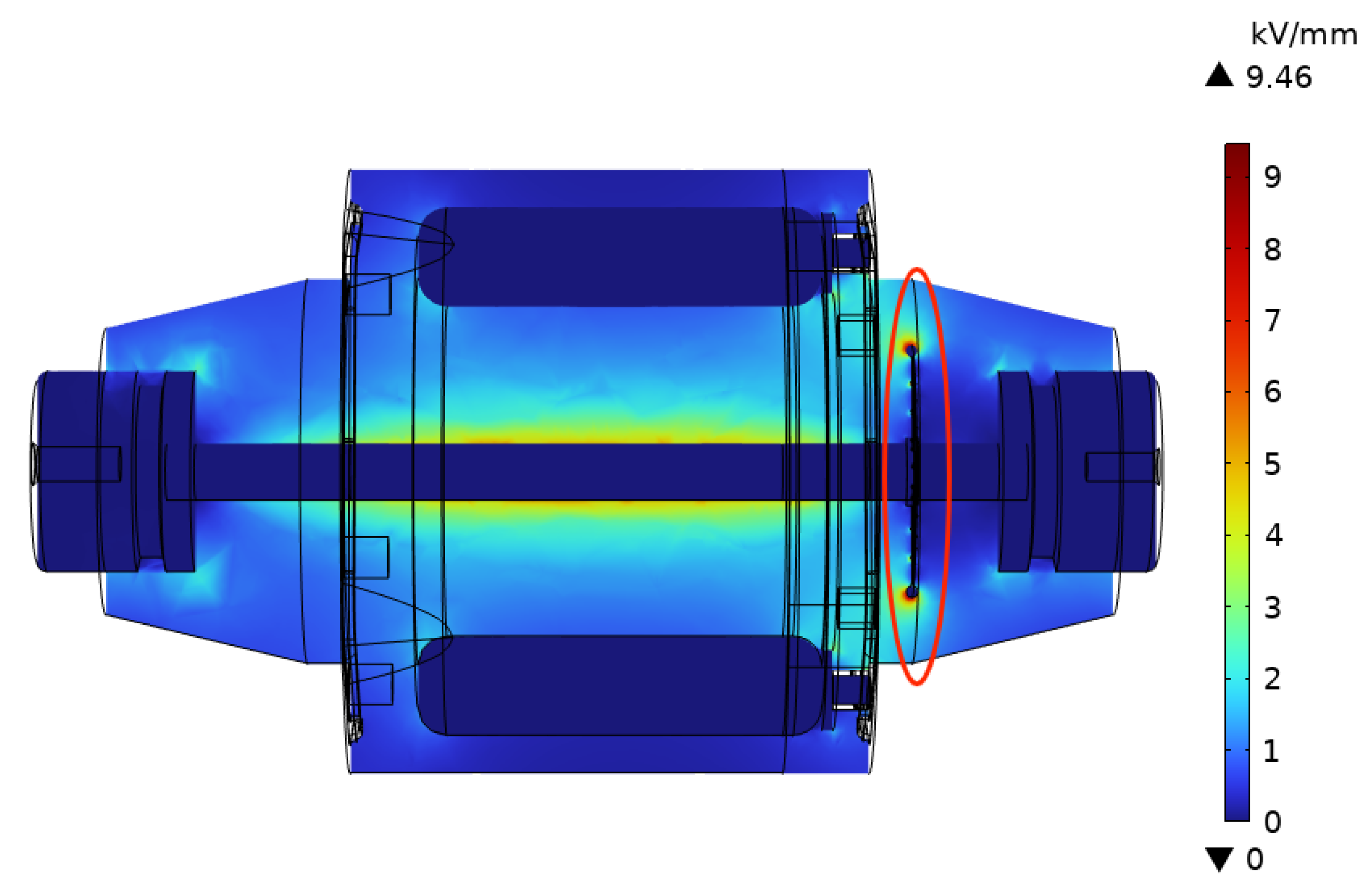
3.1. Electric-Field Distribution of Current Transformer
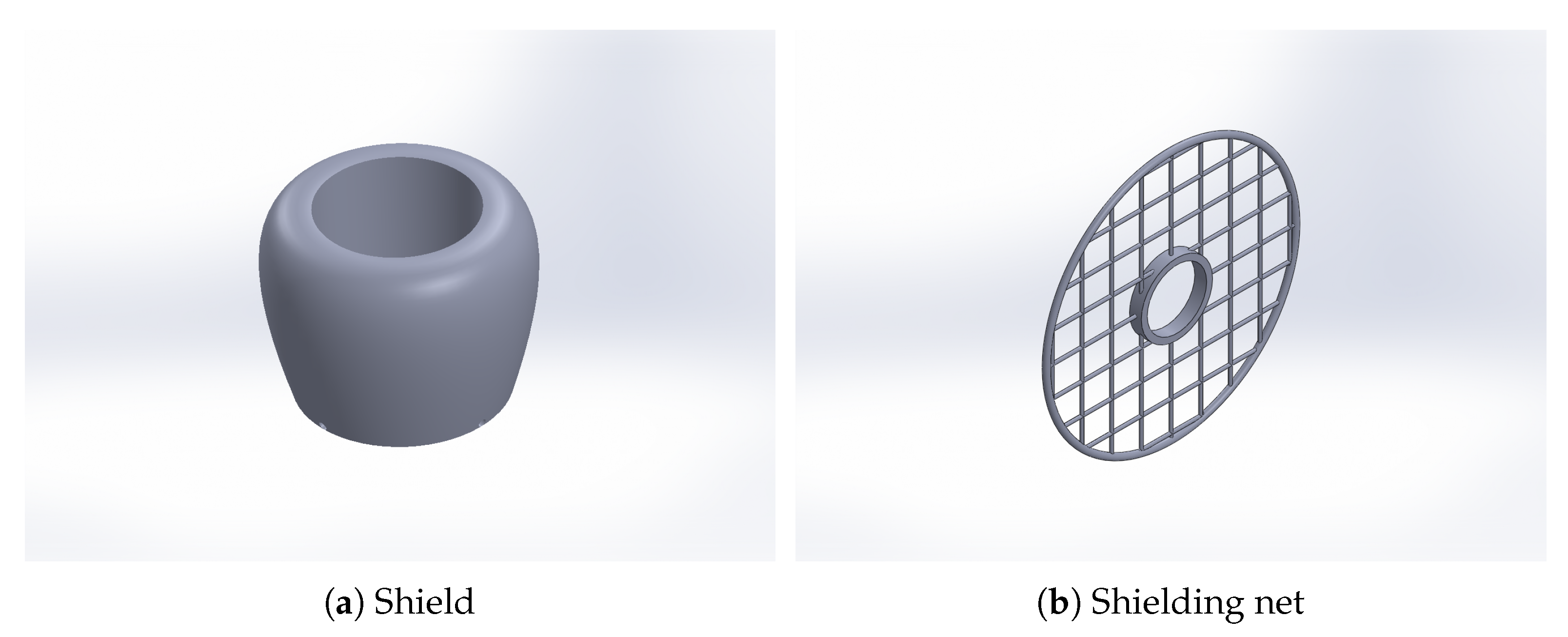
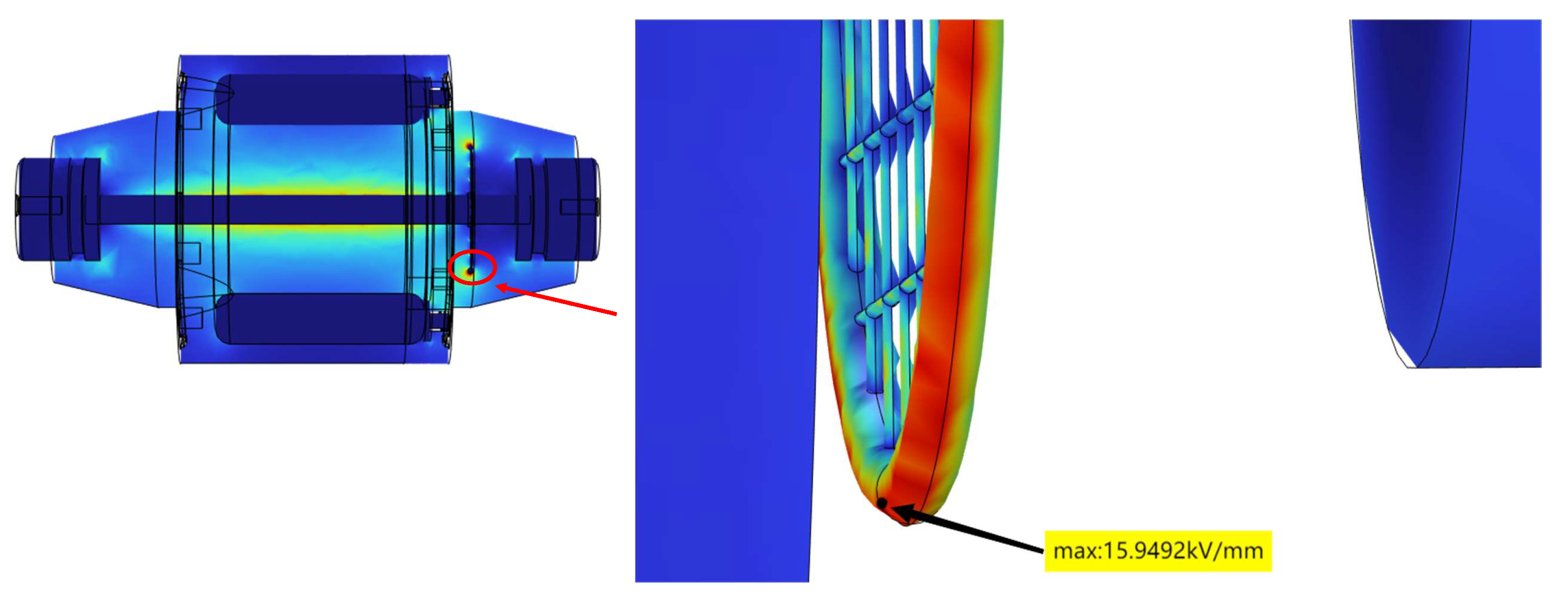
3.2. Optimization Scheme
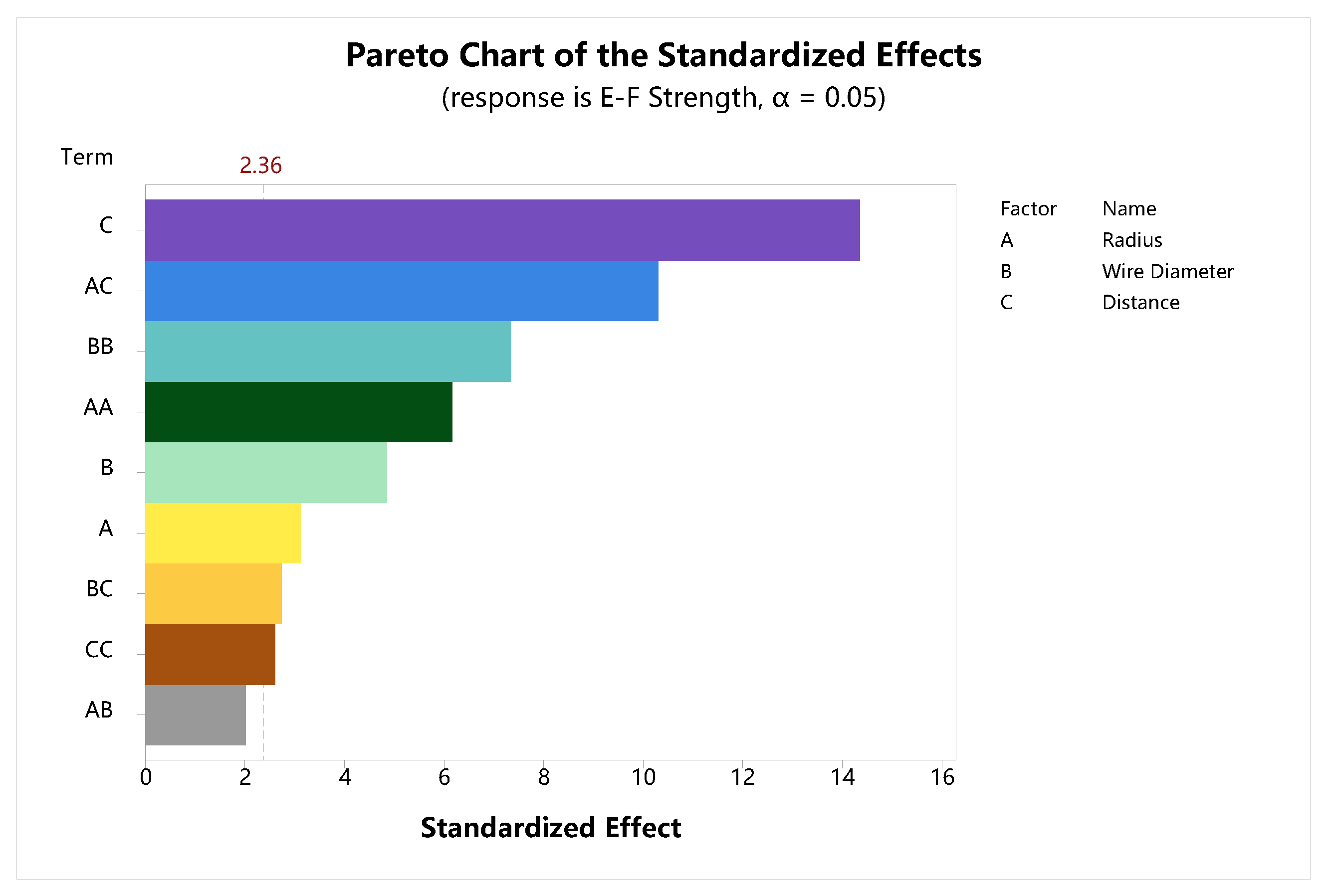
4. Design of Experiment and Response Surface
4.1. Selection of Factors
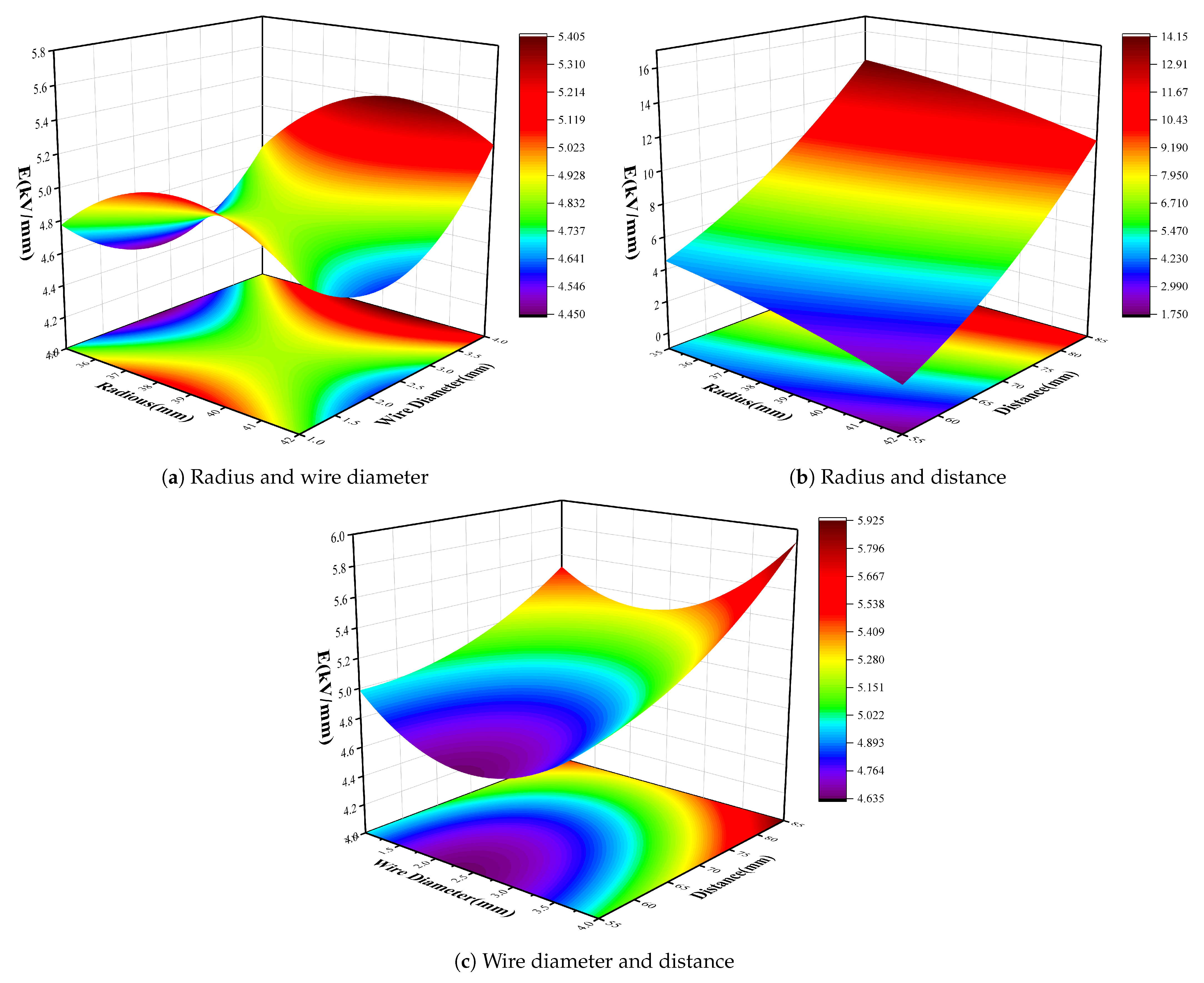
4.2. Establishment of Response Surface
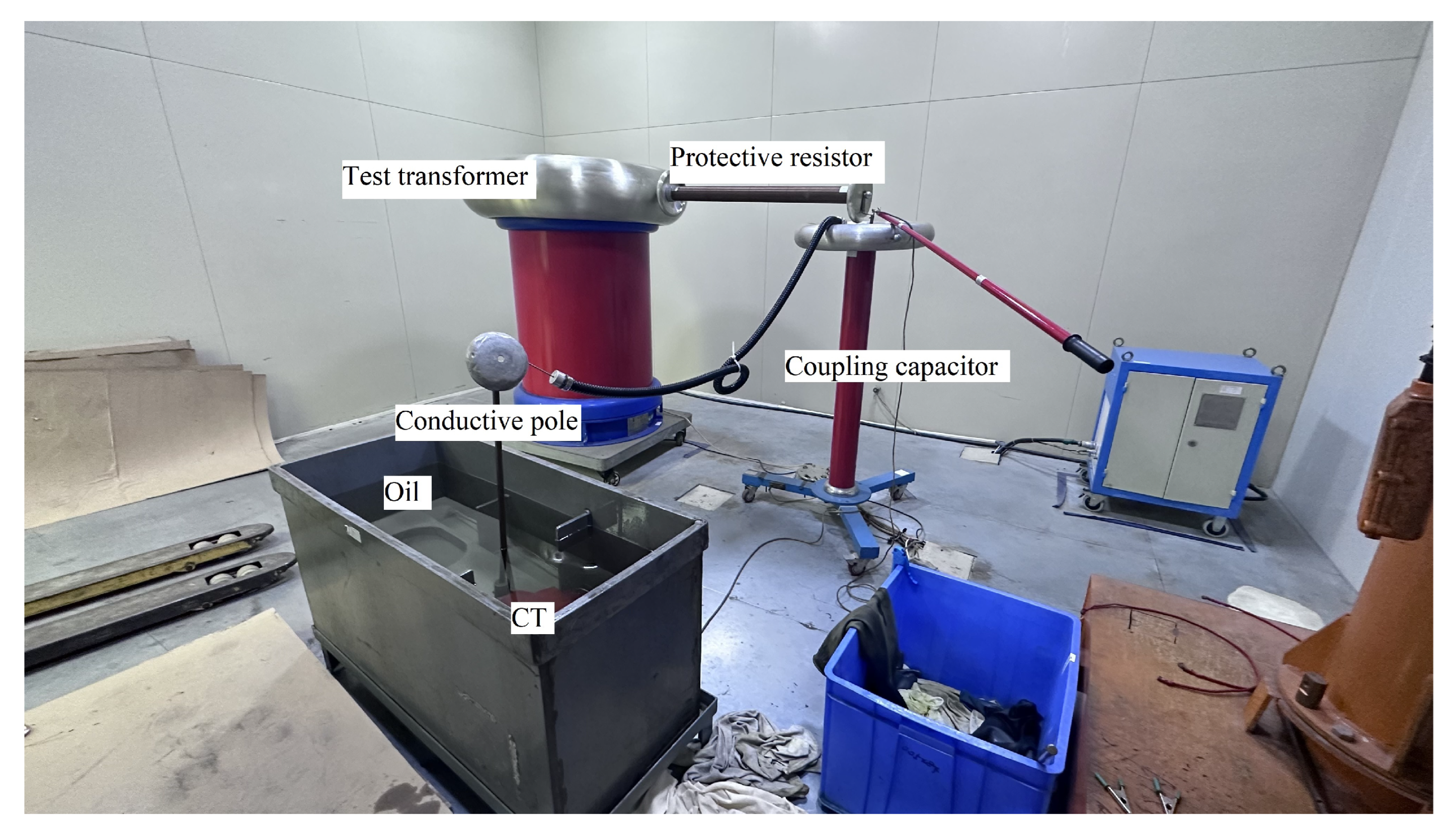
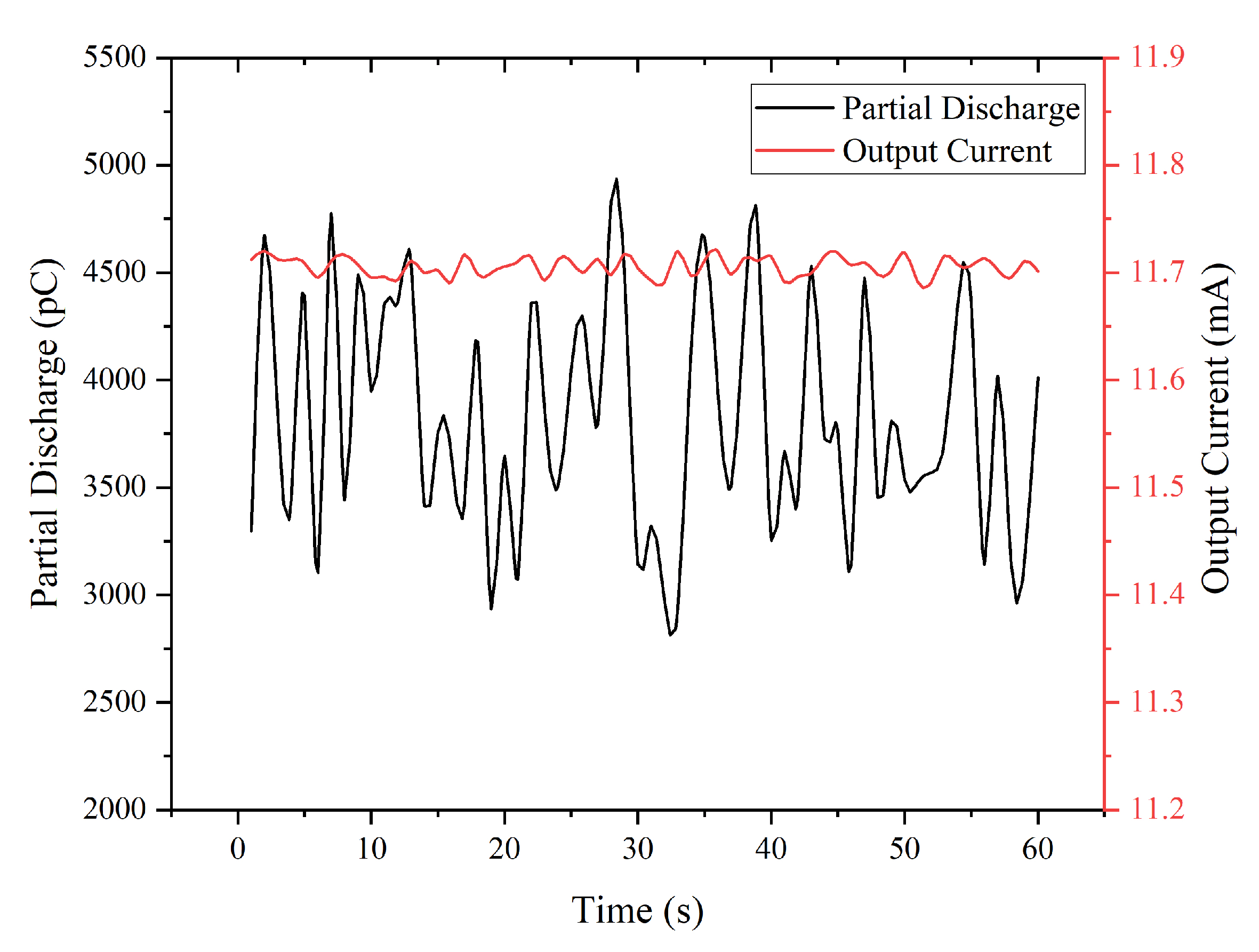
5. Partial Discharge Test
6. Conclusions
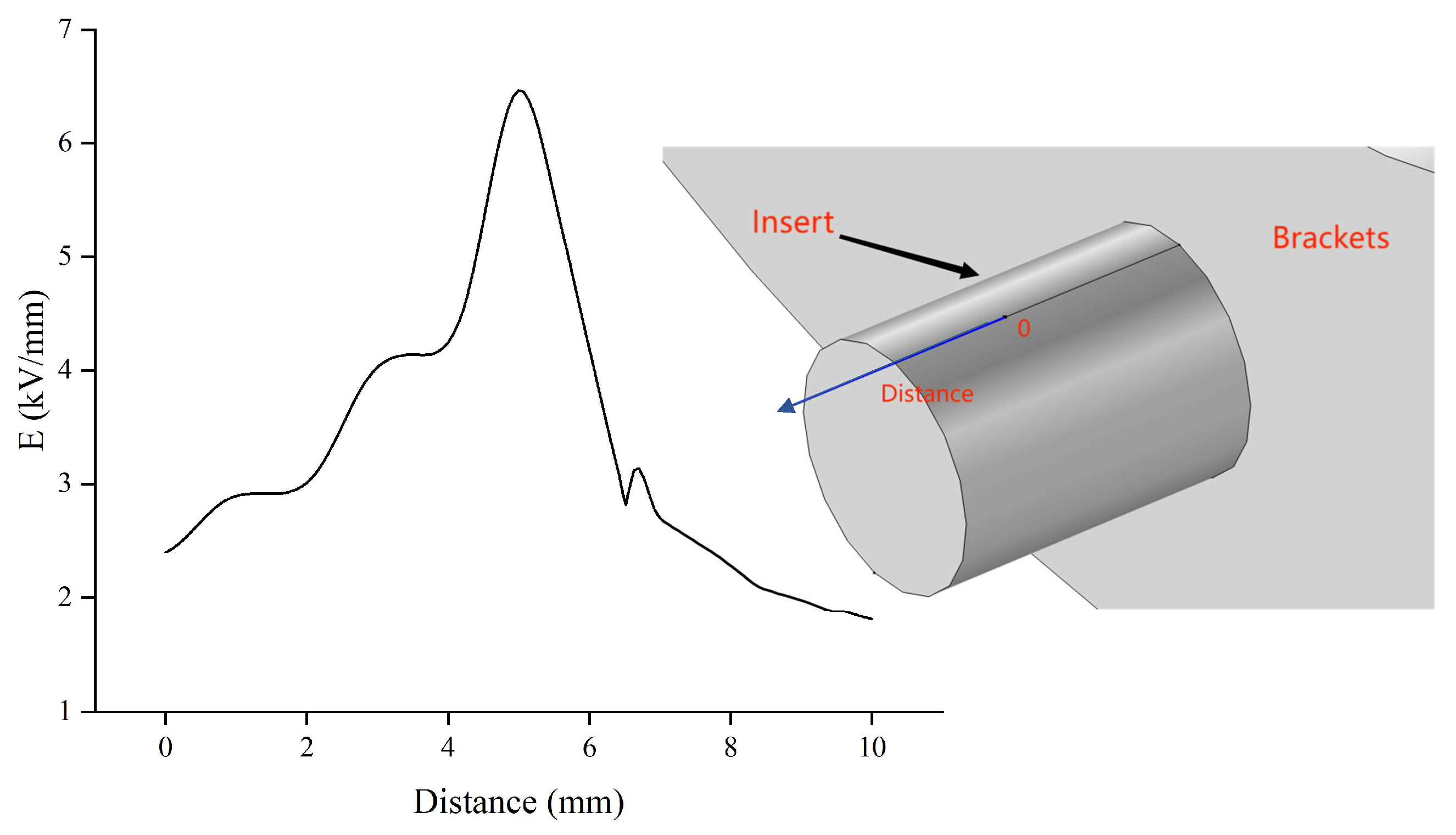
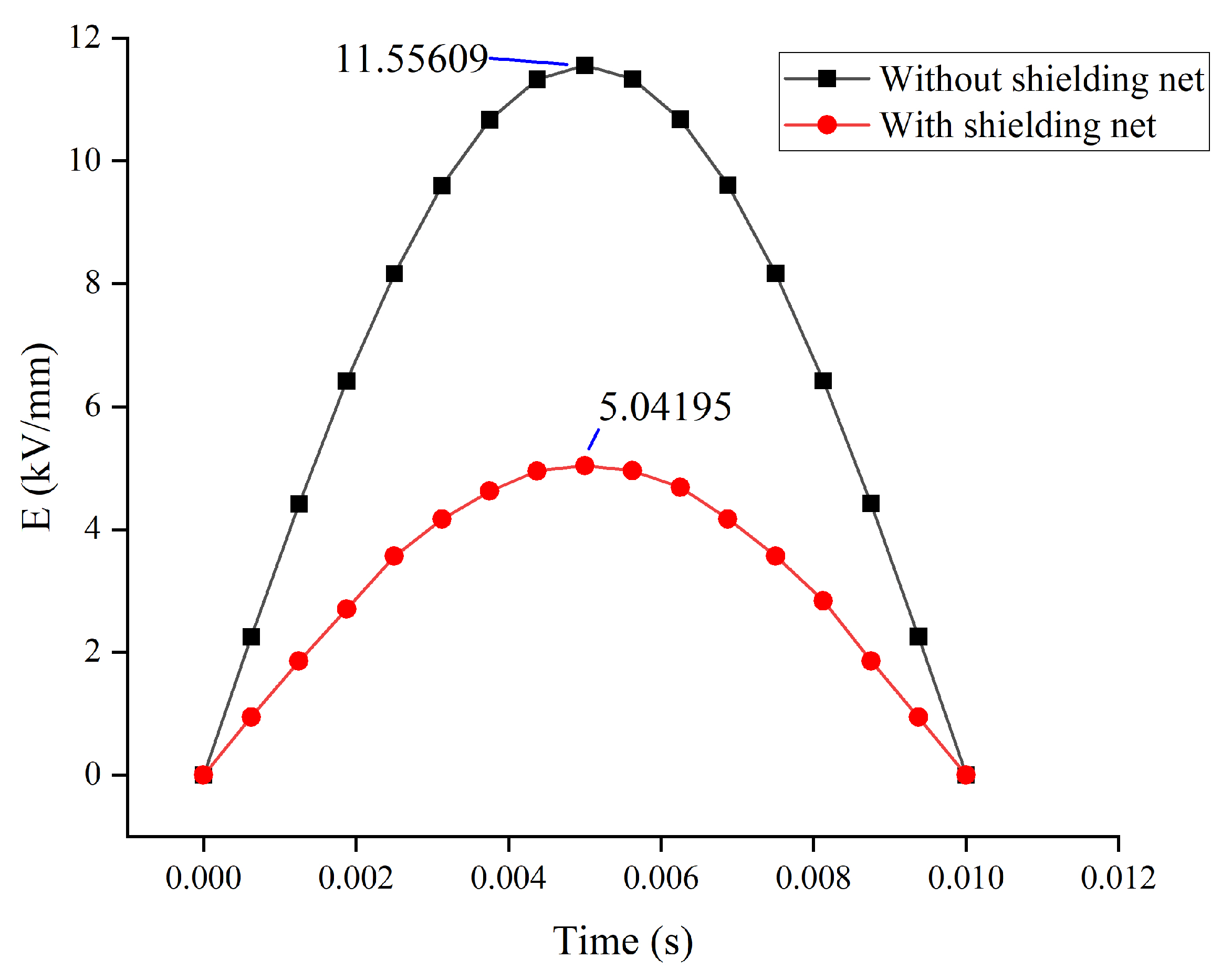
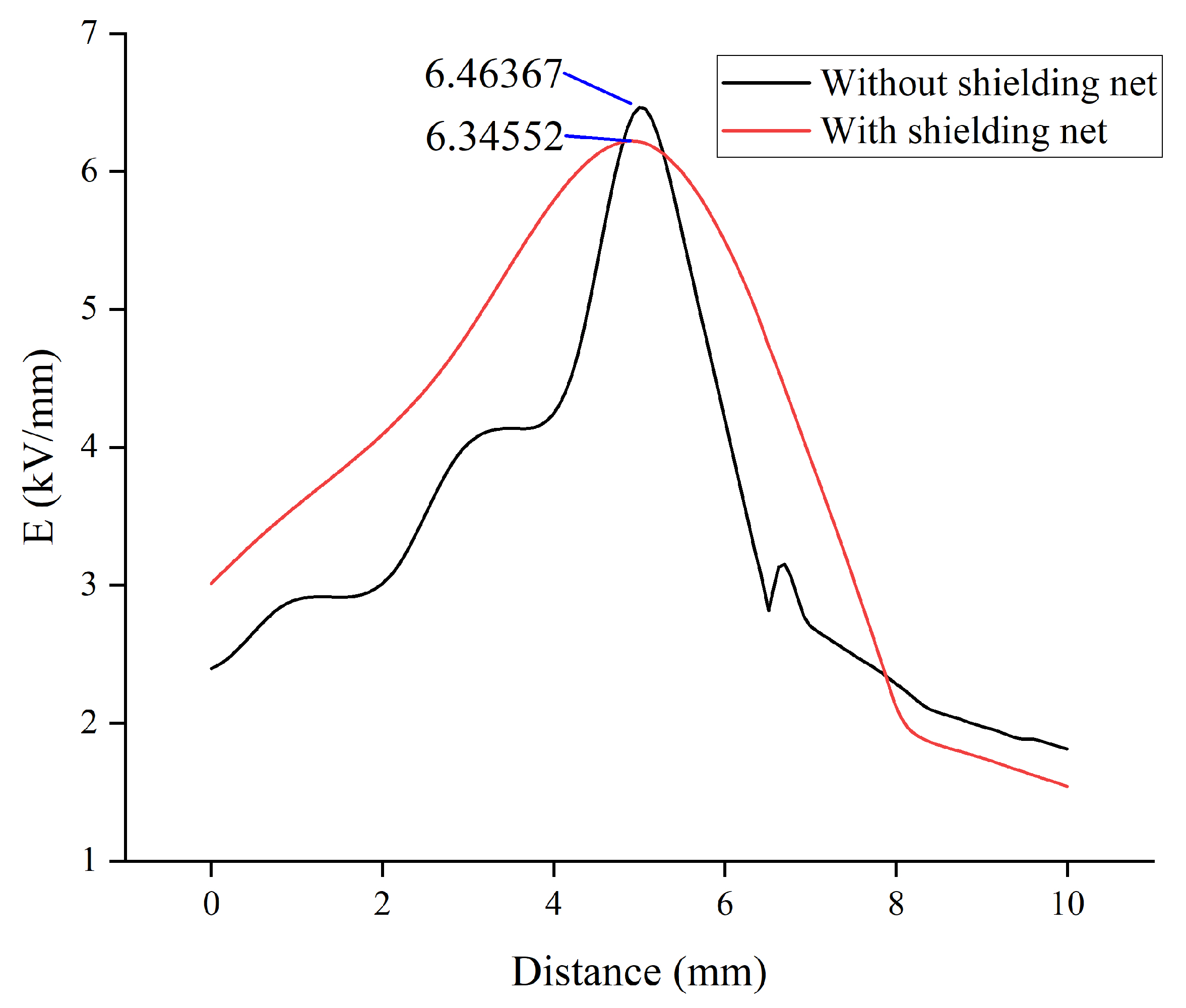
- The weak area of the electric field distribution in the CT is found near the metal insert of the bracket. When the input voltage is at its maximum, the electric field strength is 11.5561 kV/mm. At , the electric field on the surface of the insert distributes unevenly along the axial direction.
- After optimization, the maximum electric field in the current transformer is located on the shielding net, with a value of 15.9492 kV/mm. The electric field strength at is reduced to 5.0419 kV/mm, which is a decrease of 56.37%.
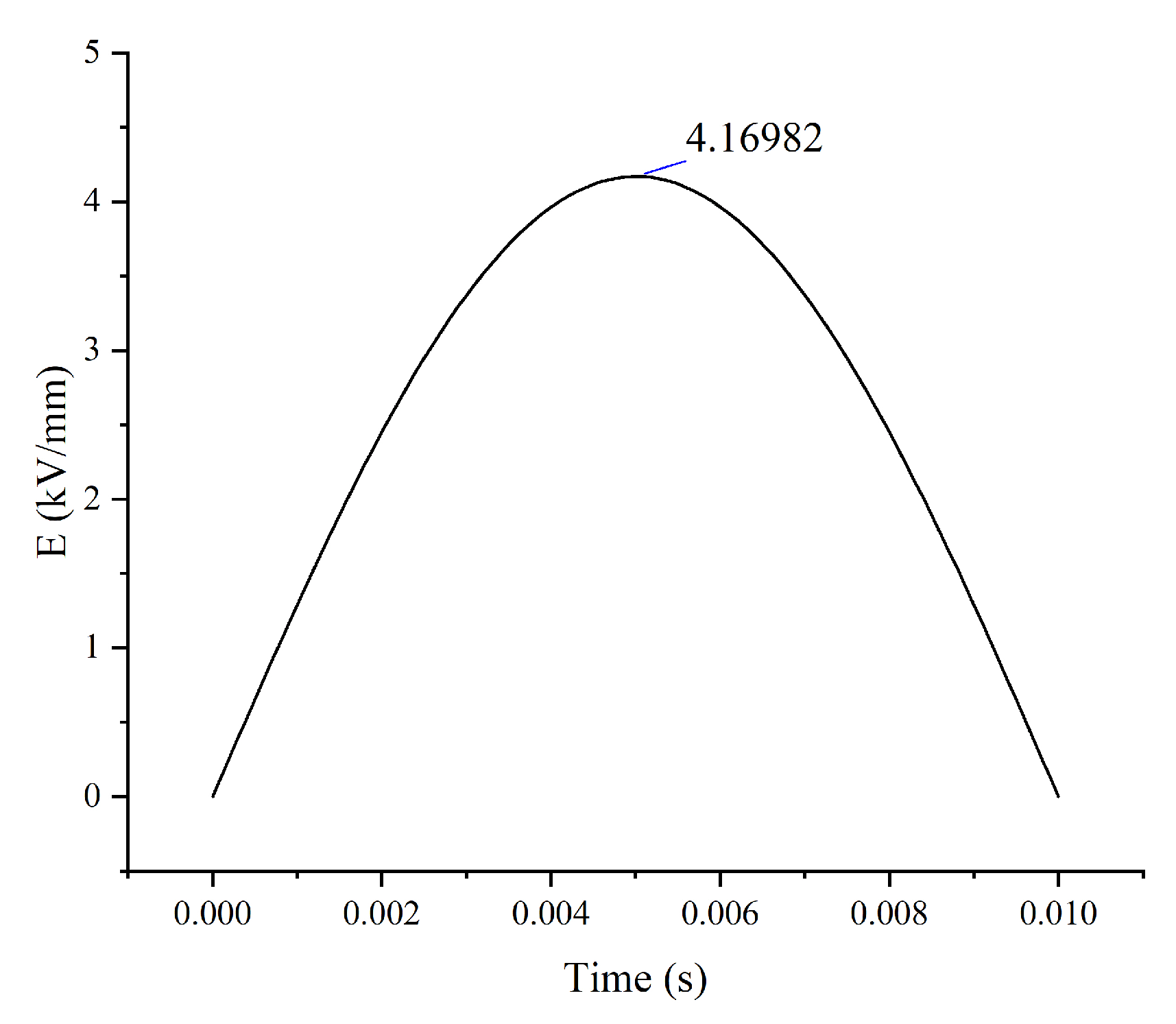
- The response surfaces predict the minimum electric field strength is 4.053 kV/mm when the radius is 42 mm, the wire diameter is 2.2727 mm, and the position is 55 mm. The simulation result is 4.16982 kV/mm.
- The optimization effect is obvious when the improved CT is subjected to partial discharge test. Without a shielding net, the CT withstand voltage test at 62 kV appears obviously discharging. After optimization, it can withstand up to 85 kV in 1 min without discharging.
- In this paper, a finite element combined design of experiment approach is used for optimization. Future research could try to use a more systematic algorithm, such as neural network combined with genetic algorithm, to select more factors that may affect the electric field for optimization and compare it with the method in this paper.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Feng, Y. Study on the Relationship between Surface Discharge and Decomposition Gas of Basin Insulators for SF6 Current Transformers. Ph.D. Thesis, Shandong University, Jinan, China, 2021. [Google Scholar]
- Xiuke, Y.; Yang, S.; Cunzhan, Y.; Dexin, X. Electric Field Analysis and Optimal Design of Main Insulation for Oil-Immersed Inverted Current Transformers. Trans. China Electrotech. Soc. 2014, 29, 37–43. [Google Scholar]
- Thango, B.A.; Bokoro, P.N. Defining and Specifying Design Considerations for Distribution Transformers in Large-Scale Solar Photovoltaic Plants. Energies 2022, 15, 2773. [Google Scholar] [CrossRef]
- Dameng, H.; Nianping, Y.; Zhongyi, W.; Junxin, T.; Jiancang, M. Electric Field Analysis and Experimental Study of Defective Basin Insulators Within SF6- Gas-Insulated Current Transformers of 500 kV. Electr. Power 2014, 47, 27–32. [Google Scholar]
- Guo, L.; Yu, Z.; Bo, L.; Tao, D.; Yongyi, Z. Optimal design of insulation of current transformers cast by epoxy resin at high altitude. High Volt. Electr. Appl. 2010, 46, 57–60. [Google Scholar]
- Ana, D.; Bojan, T. Transformer electric field calculation using BEM and FEM. Procedia Eng. 2017, 202, 312–318. [Google Scholar]
- Dalibor, F.G.; Božidar, F.G.; Miroslav, P. Electric field at sharp edge as a criterion for dimensioning condenser-type insulation systems. Electr. Power Syst. Res. 2017, 152, 485–492. [Google Scholar]
- Dong, B.; Gu, Y.; Gao, C.; Zhang, Z.; Wen, T.; Li, K. Three-Dimensional Electro-Thermal Analysis of a New Type Current Transformer Design for Power Distribution Networks. Energies 2021, 14, 1792. [Google Scholar]
- Jia, J.; Xu, J.; Wen, Z. Electric Field Distribution in SF 6 Gas of Converter Transformer Bushing under Complex Voltages. In Proceedings of the IEEE International Conference on High Voltage Engineering and Application, Beijing, China, 6–10 September 2020. [Google Scholar]
- Xiaodong, W.; Wenwen, G.; Shudi, Z.; Gaolin, W.; Qian, W.; Xiaoping, L.; Huixian, H. Finite element analysis of electric field and structural optimization design of 10 kV cast strut type current transformer. J. Southwest Univ. 2019, 41, 134–140. [Google Scholar]
- Qingyu, W.; Peng, L.; Huidong, T.; Gengsheng, X.; Zongren, P.; Xi, Y. Research on the dynamic characteristics of electric field distribution of the 1100 kV Ultra high voltage converter transformer valve-side bushing using weakly ionised gas conductance model. High Volt. 2022, 7, 288–301. [Google Scholar]
- Ricardo, M.A.V.; Jennifer, V.M.L. Current transformer failure caused by electric field associated to circuit breaker and pollution in 500kV substations. Eng. Fail. Anal. 2018, 92, 163–181. [Google Scholar]
- Lesniewska, E.E.; Olak, J. Improvement of the Insulation System of Unconventional Combined Instrument Transformer Using 3-D Electric-Field Analysis. IEEE Trans. Power Deliv. 2018, 33, 2582–2589. [Google Scholar] [CrossRef]
- Wei, W.; Hanbo, Z.; Jinhua, H.; Xiaogang, L.; Yuquan, L.A. 500kV current transformer flashover failure cause analysis. Transformer 2016, 53, 64–67. [Google Scholar]
- Benshuai, Z.; Fenfang, W.; Bo, W.; Haocun, C.; Jiatao, L. ANSYS finite element software simulation and analysis of 145 kV SF6 current transformer. Telecom Power Technol. 2020, 37, 87–89. [Google Scholar]
- Anguraja, R.; Dixit, P. Electric Field Distribution of 800 kV OIP Transformer Bushing. Lect. Notes Electr. Eng. 2022, 783, 1784–1792. [Google Scholar]
- Huang, K.; Cheng, J.; Wang, S.; Bao, L.; La, Y.; Zhang, Y. Field-Circuit Coupled Simulation of Core Grounding Current of a VSC Transformer. In Proceedings of the 2022 IEEE International Conference on High Voltage Engineering and Applications (ICHVE), Chongqing, China, 25–29 September 2022. [Google Scholar]
- Yang, S. Analysis and Optimization Design of Main Insulation Electric Field of Oil-Immersed Inverted Current Transformer. Ph.D. Thesis, Shenyang University of Technology, Shenyang, China, 2012. [Google Scholar]
- Xuan, L.; Shipu, W.; Ping, W. Design and performance verification of ultra-high voltage small-capacity capacitive voltage transformer with series damping elements. High Volt. Technol. 2018, 44, 1844–1852. [Google Scholar]
- Jaafar, M.J.; Muhamad, N.A.; Jamil, M.K.M.; Rosle, N. Electric Field and Potential Changes Studies on Cast-Resin Dry-Type Power Transformer Having Misalignment. In Proceedings of the 13th IEEE International Conference on the Properties and Applications of Dielectric Materials, Virtual, 12–14 July 2021. [Google Scholar]
- Mansour, D.E.A.; Kojima, H.; Hayakawa, N.; Hanai, M.; Okubo, H. Physical mechanisms of partial discharges at nitrogen filled delamination in epoxy cast resin power apparatus. IEEE Trans. Dielectr. Electr. Insul. 2013, 20, 454–461. [Google Scholar] [CrossRef]
- Yongsheng, W.; Mingbin, P.; Wenyong, J.; Tongyu, L.; Yanan, W. Feasibility study on substitution of SF6 insulated current transformer with environment-friendly natural gas. Energy Rep. 2023, 9, 1784–1792. [Google Scholar]
- Kieffel, Y.; Irwin, T.; Ponchon, P.; Owens, J. Green gas to replace SF6 in electrical grids. IEEE Power Energy Mag. 2016, 14, 32–39. [Google Scholar] [CrossRef]
- Hu, F. Study of Stress and Electric Field Simulation of Internal Defects in GIS Tub Insulators and Their Detection Methods. Ph.D. Thesis, North China Electric Power University, Beijing, China, 2017. [Google Scholar]
- GB/T 50150–2016; Electric Equipment Installation Engineering-Standard for Hand-Over Test of Electric Equipment. Standardization Administration of the People’s Republic of China: Beijing, China, 2016.
- Montgomery, D.C. Design and Analysis of Experiments; John Wiley & Sons: Hoboken, NJ, USA, 2020. [Google Scholar]





| Components | Materials | Electrical Conductivity (S/m) | Relative Dielectric Constant |
|---|---|---|---|
| Primary terminals | Copper | 1 | |
| Conductive poles | Copper | 1 | |
| Brackets | Iron | 1 | |
| Secondary Coil Core | Iron | 1 | |
| Pouring body | Epoxy resin | 3.5 | |
| Oil | 2.2 |
| Factor | Low Level | High Level |
|---|---|---|
| Radius (mm) | 35 | 42 |
| Wire diameter (mm) | 1 | 4 |
| Distance (mm) | 55 | 85 |
| Std Order 1 | Run Order | Pt Type 2 | Radius (mm) | Wire Diameter (mm) | Distance (mm) | E-F Strength (kV/mm) |
|---|---|---|---|---|---|---|
| 10 | 1 | 1 | 0 | 0 | 4.56949 | |
| 7 | 2 | 1 | 1 | 1 | 5.27290 | |
| 9 | 3 | 0 | 0 | 4.45780 | ||
| 3 | 4 | 1 | 1 | 4.80860 | ||
| 6 | 5 | 1 | 1 | 1 | 5.67650 | |
| 8 | 6 | 1 | 1 | 1 | 1 | 6.09160 |
| 4 | 7 | 1 | 1 | 1 | 4.60530 | |
| 2 | 8 | 1 | 1 | 4.29080 | ||
| 12 | 9 | 0 | 1 | 0 | 5.40000 | |
| 15 | 10 | 0 | 0 | 0 | 0 | 4.91300 |
| 17 | 11 | 0 | 0 | 0 | 0 | 4.90000 |
| 11 | 12 | 0 | 0 | 5.04776 | ||
| 13 | 13 | 0 | 0 | 4.63900 | ||
| 5 | 14 | 1 | 1 | 4.87040 | ||
| 1 | 15 | 1 | 4.97230 | |||
| 16 | 16 | 0 | 0 | 0 | 0 | 4.90410 |
| 14 | 17 | 0 | 0 | 1 | 5.31000 |
| Radius (mm) | Wire Diameter (mm) | Distance (mm) | Prediction of Electric Field Size (kV/mm) |
|---|---|---|---|
| 42 | 2.2727 | 55 | 4.053 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yin, X.; Zeng, J.; Guan, Q. Shielding Strategies for Electric Field Minimization in Current Transformers: A Finite Element Combined Design of Experiment Approach. Energies 2023, 16, 7247. https://doi.org/10.3390/en16217247
Yin X, Zeng J, Guan Q. Shielding Strategies for Electric Field Minimization in Current Transformers: A Finite Element Combined Design of Experiment Approach. Energies. 2023; 16(21):7247. https://doi.org/10.3390/en16217247
Chicago/Turabian StyleYin, Xuzhen, Jianbin Zeng, and Qingbo Guan. 2023. "Shielding Strategies for Electric Field Minimization in Current Transformers: A Finite Element Combined Design of Experiment Approach" Energies 16, no. 21: 7247. https://doi.org/10.3390/en16217247
APA StyleYin, X., Zeng, J., & Guan, Q. (2023). Shielding Strategies for Electric Field Minimization in Current Transformers: A Finite Element Combined Design of Experiment Approach. Energies, 16(21), 7247. https://doi.org/10.3390/en16217247





