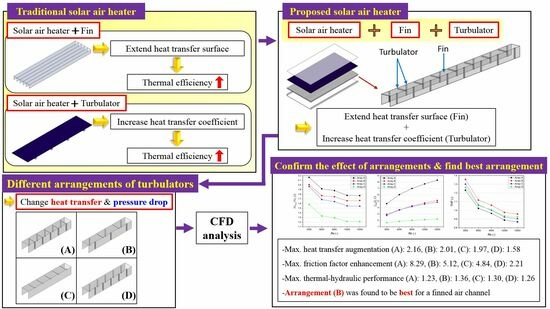
Heat Transfer Augmentation and Friction Factor Due to the Arrangement of Rectangular Turbulators in a Finned Air Channel of a Solar Air Heater
Abstract
:1. Introduction
2. Methodology
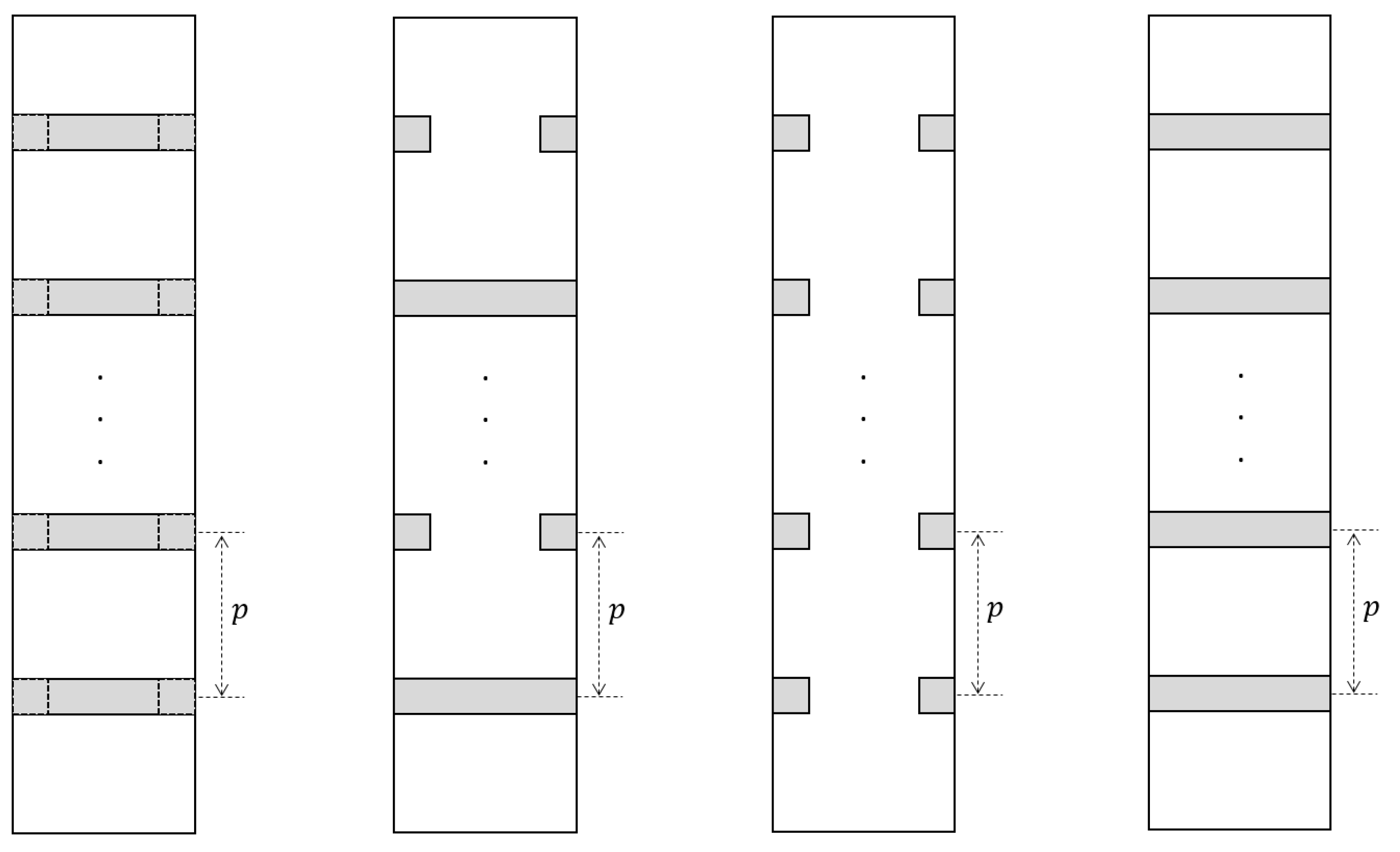
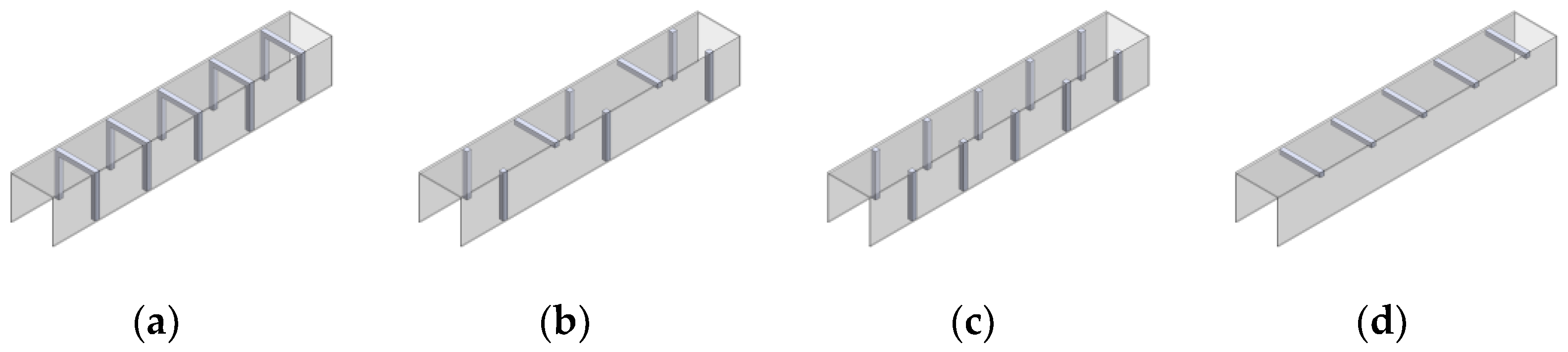
2.1. 3D Model Description
2.2. Governing Equations
2.3. Boundary Condition and Solution Method
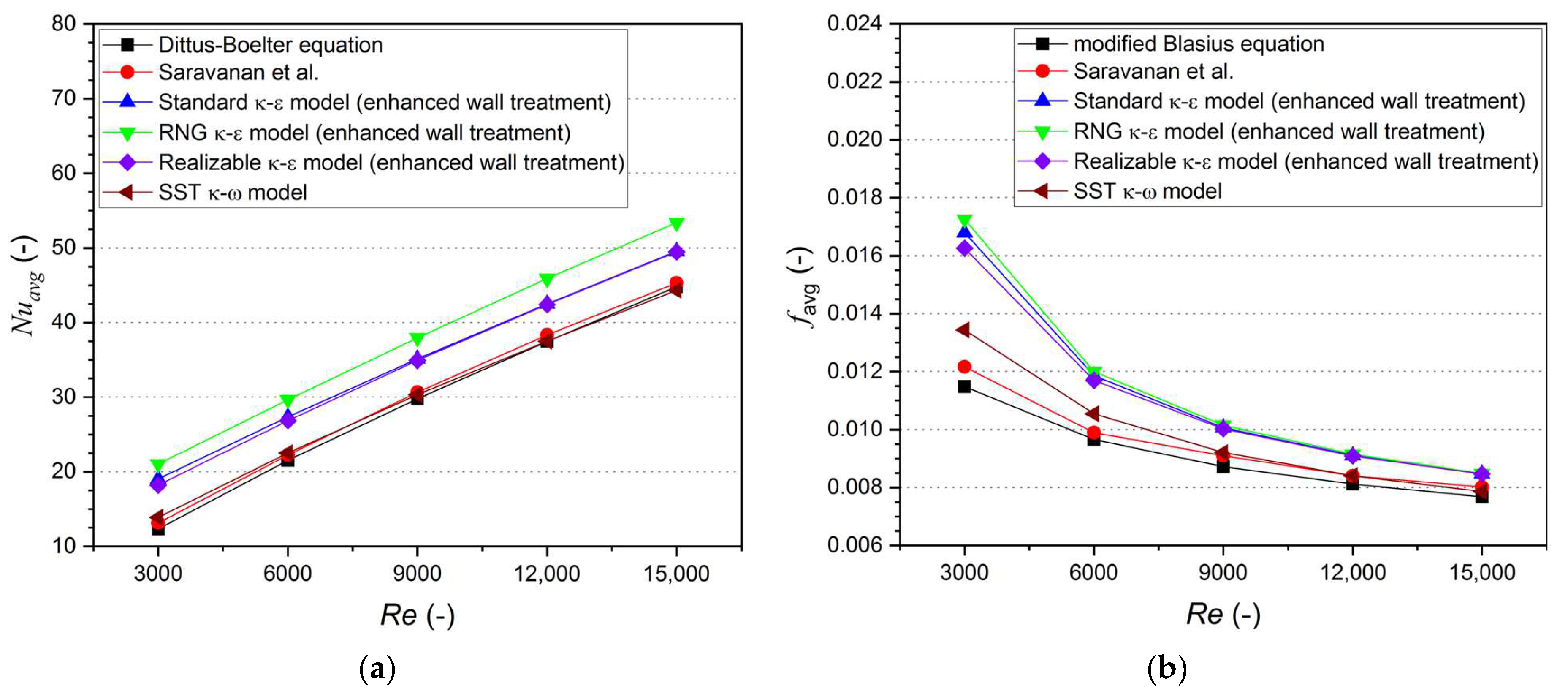
2.4. Validation and Selection of Turbulence Model
2.5. Grid Independence Test
3. Performance Indicators
4. Results and Discussion
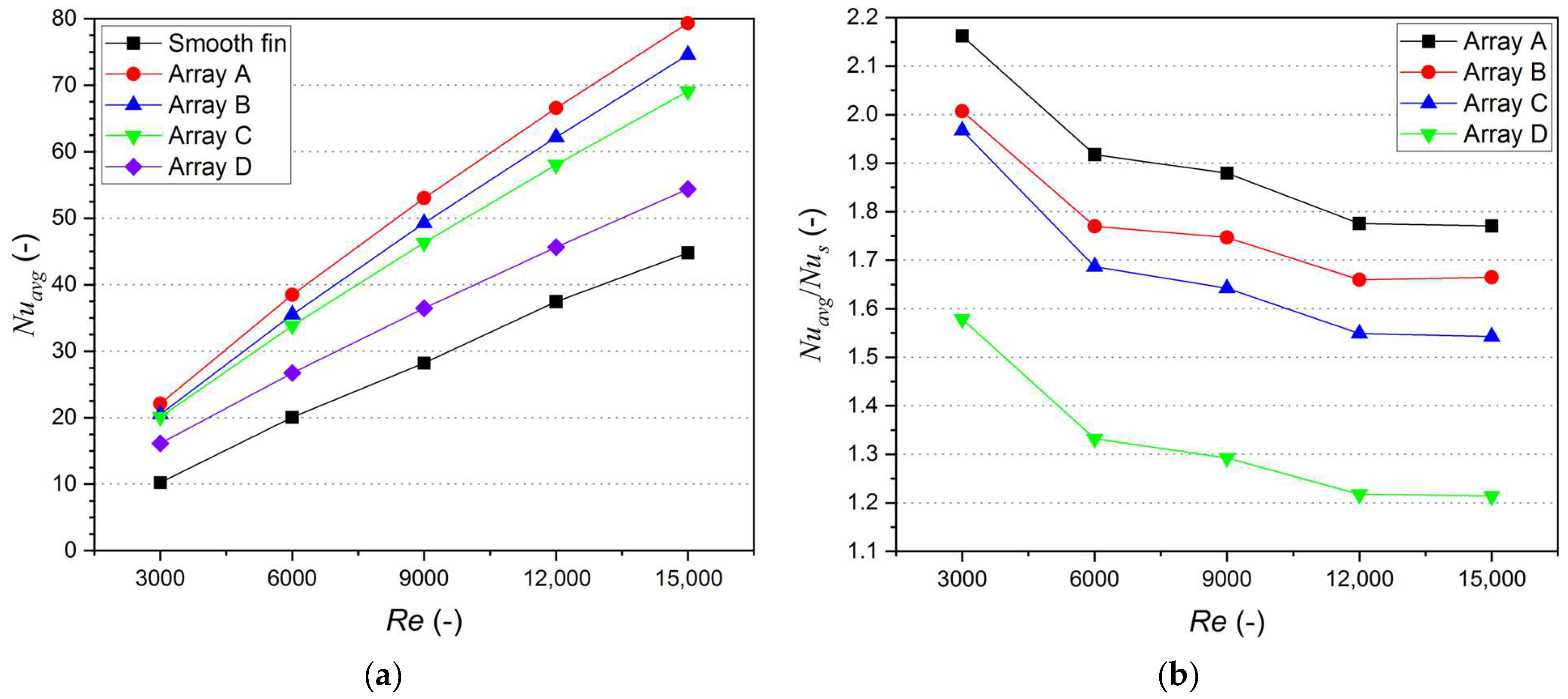
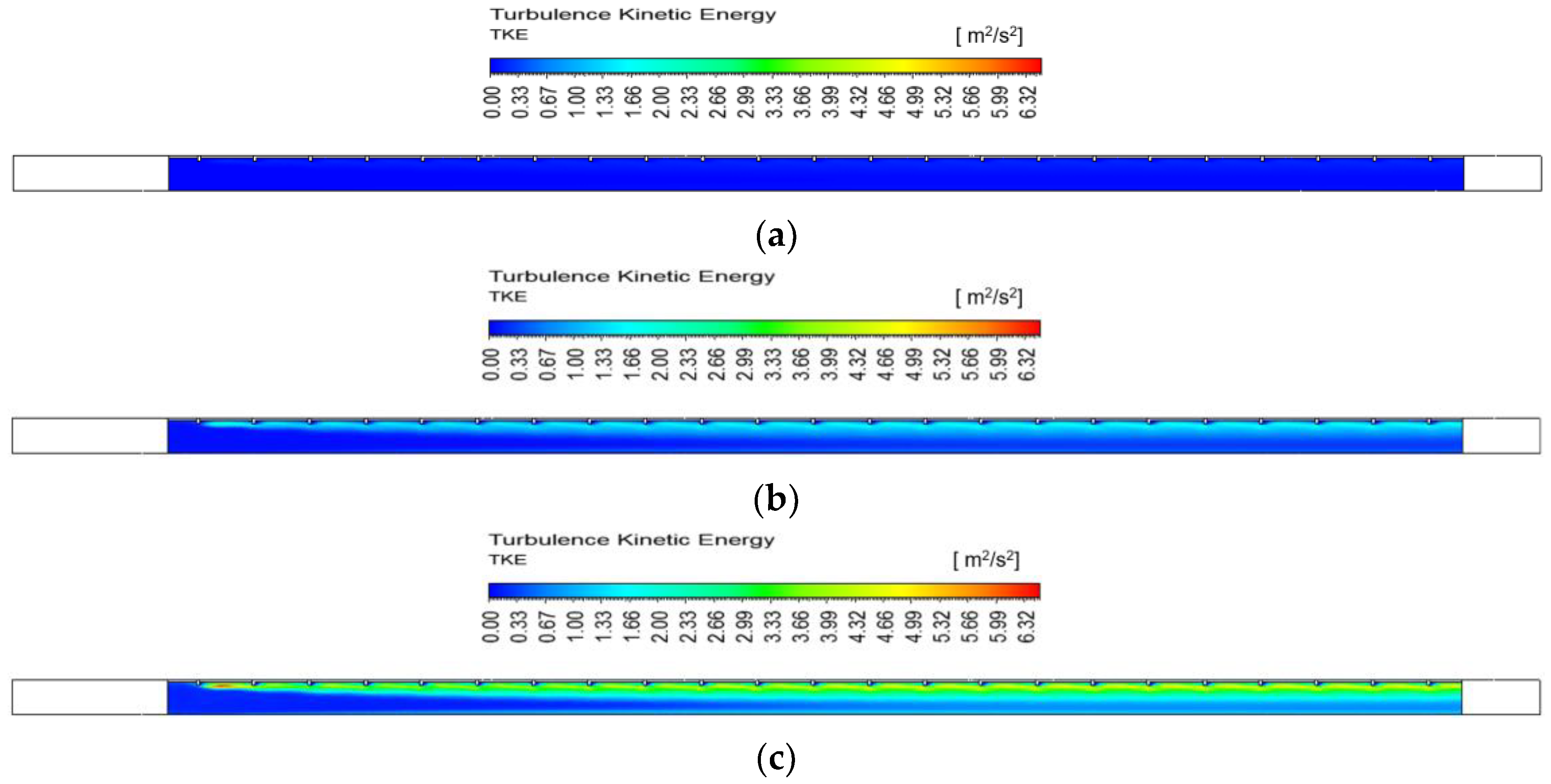
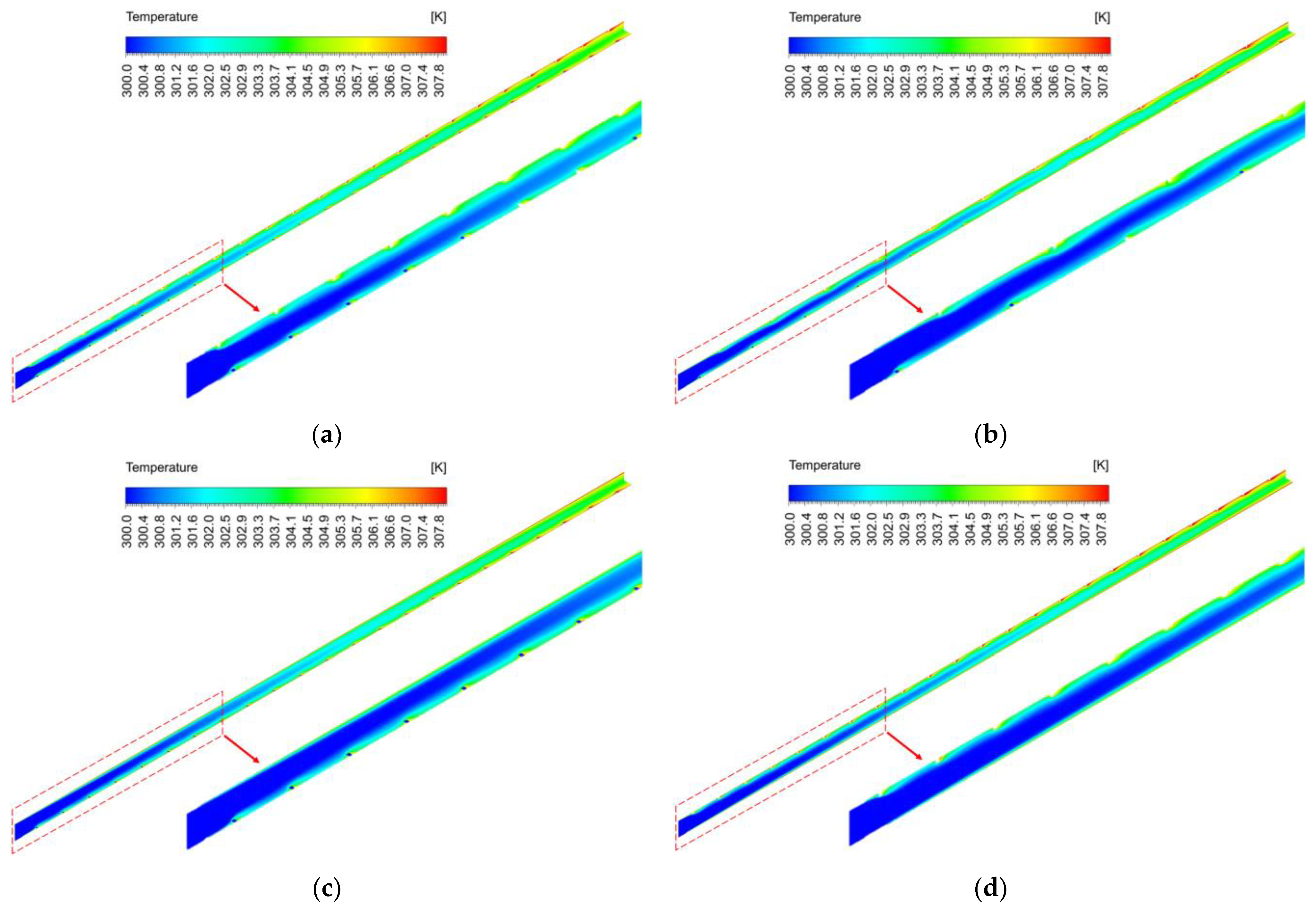
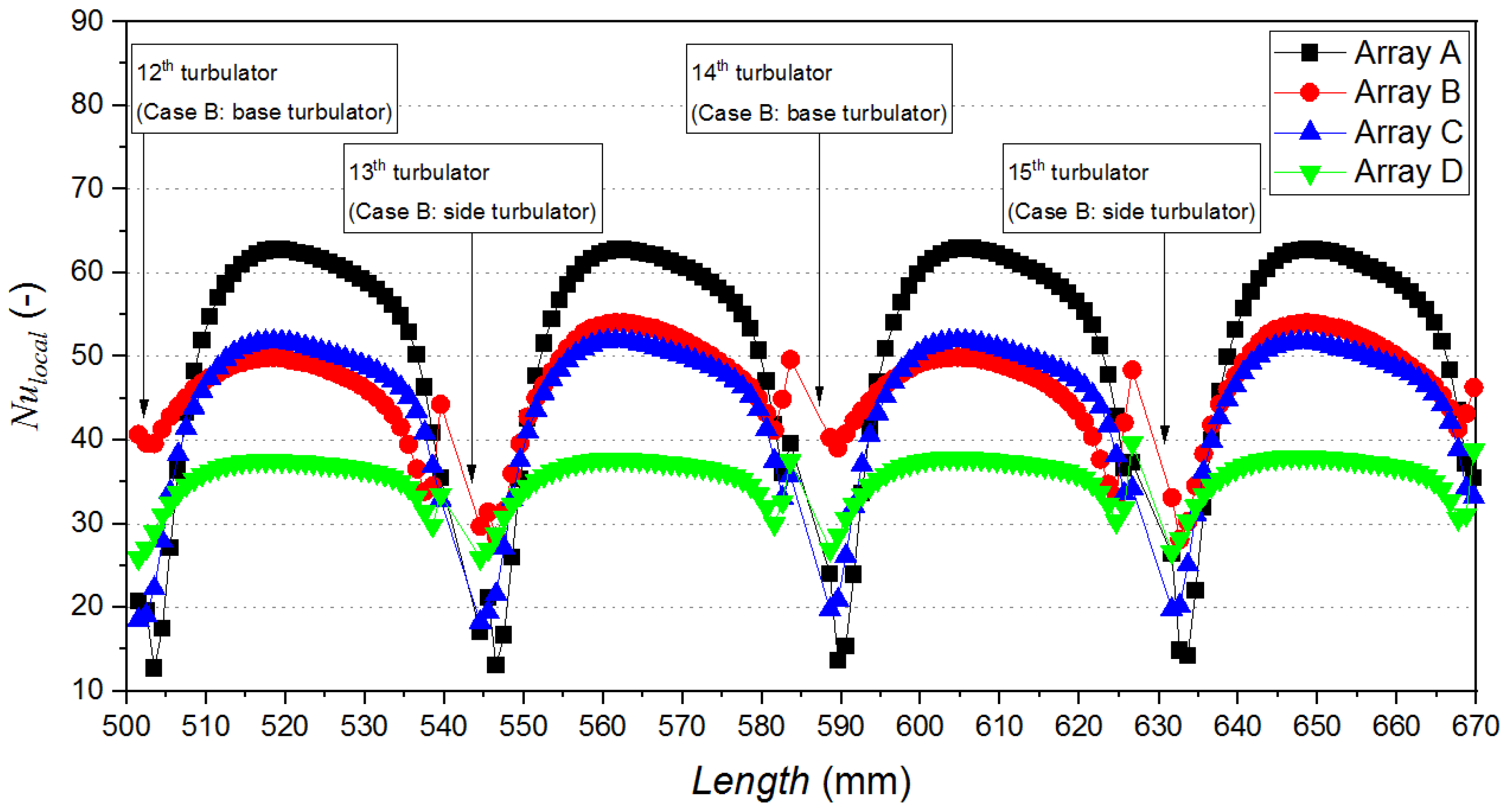
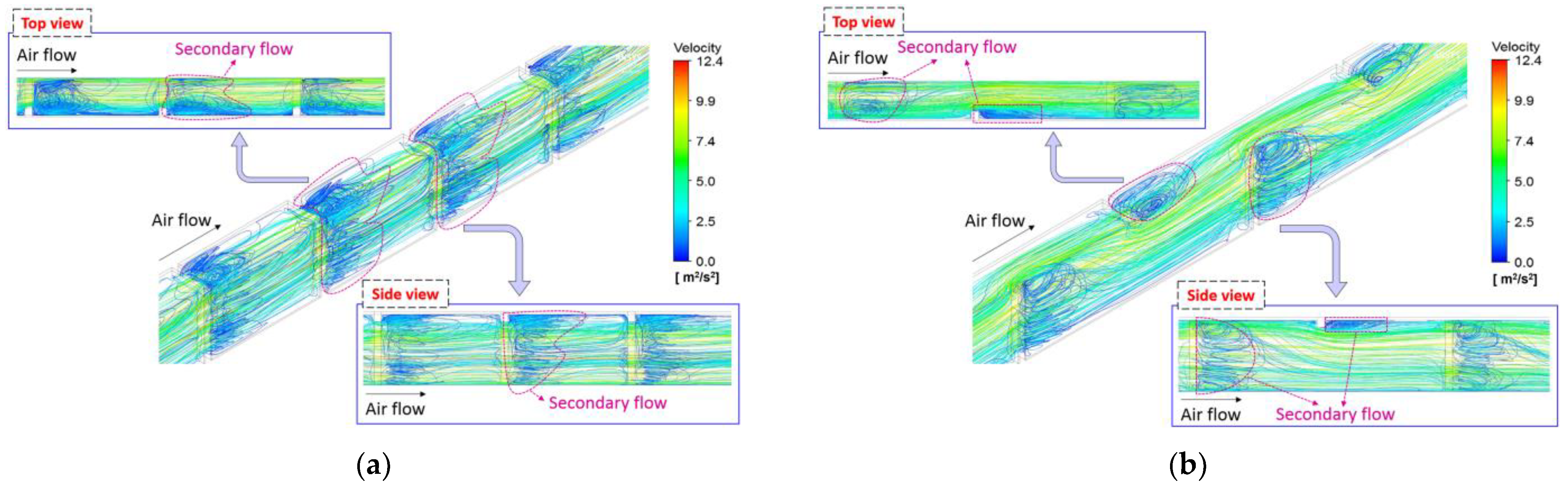
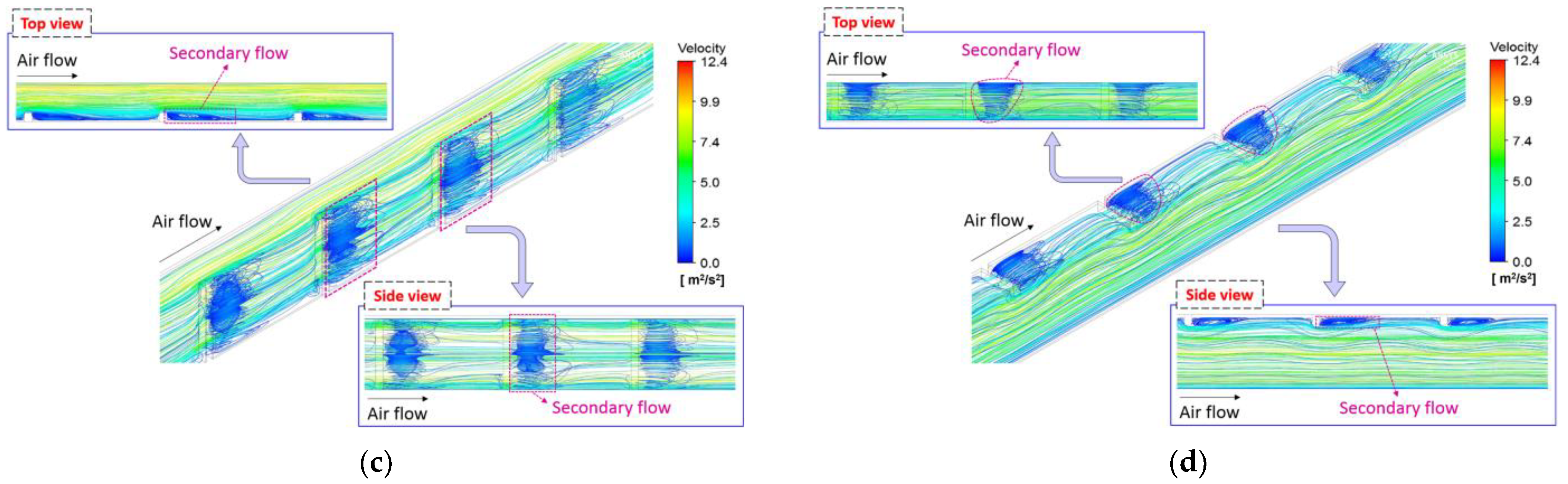
4.1. Heat Transfer
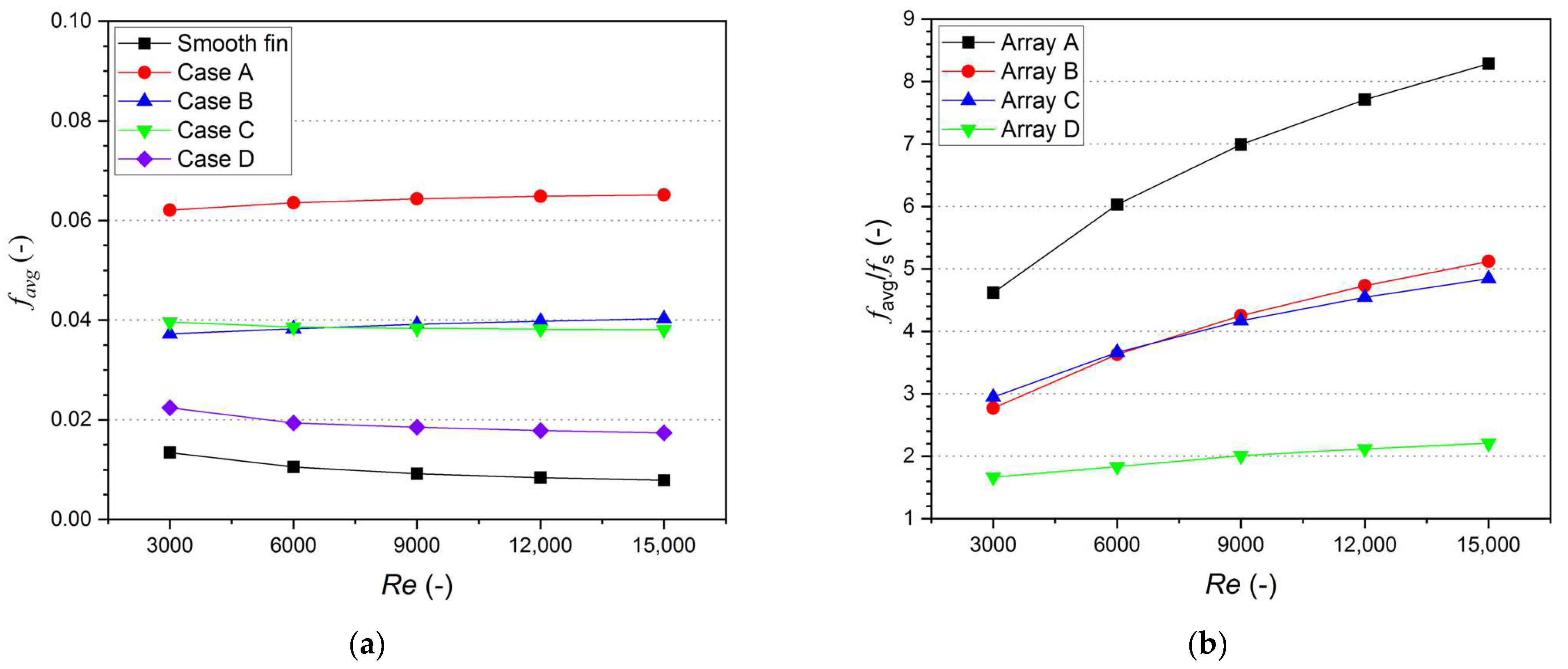
4.2. Friction Factor
4.3. Thermo-Hydraulic Performance
5. Conclusions
- (i)
- The installation of the turbulator enhanced heat transference from the fin to flowing air. Differing Nusselt number augmentation was observed depending on the arrangement methods of the turbulators due to the different turbulence kinetic energy. Among the investigated arrangements, the highest augmentation in the Nusselt number was observed in Array A with a value of 2.16, while Array B, Array C, and Array D had maximum Nusselt numbers of 2.01, 1.97, and 1.58, respectively.
- (ii)
- The different arrangements of the turbulator resulted in different friction factors in a finned air channel of an SAH. The most significant enhancement in the friction factor was observed in Array A, with a value of 8.29, followed by Array B, Array C, and Array D, with maximum friction factor enhancements of 5.12, 4.84, and 2.21, respectively.
- (iii)
- The THP was in the range of 0.87 to 1.23, 0.96 to 1.36, 0.90 to 1.30, and 0.92 to 1.26 for Array A, Array B, Array C, and Array D, respectively. The highest THP was found in Array B with a value of 1.36 and Array B has higher THP than those of other arrangements for all Reynolds numbers.
- (iv)
- In the investigated arrangements, Array B is considered the most appropriate and effective arrangement for the finned air channel of an SAH due to its highest THP value. On the other hand, Array A is considered unsuitable despite its high Nusselt number augmentation due to the significant increase in friction factor.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Area (m2) | |
| Hydraulic diameter (m) | |
| thermal conductivity (W/m·K) | |
| Convection heat transfer coefficient (W/m2·K) | |
| Air channel length (m) | |
| Pressure drop (Pa) | |
| Heat transfer rate (W) | |
| Velocity (m/s) | |
| Nusselt number | |
| Prandtl number | |
| Reynolds Number | |
| Greek symbols | |
| Density (kg/m3) | |
| Subscripts | |
| Air | |
| average | |
| smooth | |
| Fin | |
| Total | |
References
- Chamoli, S.; Lu, R.; Xu, D.; Yu, P. Thermal Performance Improvement of a Solar Air Heater Fitted with Winglet Vortex Generators. Sol. Energy 2018, 159, 966–983. [Google Scholar] [CrossRef]
- Markam, B.; Maiti, S. Artificial Enhancer for Small-Scale Solar Air Heater—A Comprehensive Review Aspect Ratio. Clean. Energy Syst. 2023, 4, 100046. [Google Scholar] [CrossRef]
- Mahanand, Y.; Senapati, J.R. Implementation of Hybrid Rib-Turbulators on the Thermal Performance of Solar Air Heater Duct: A Collective Review. Sustain. Energy Technol. Assess. 2022, 52, 102345. [Google Scholar] [CrossRef]
- Yadav, A.S.; Bhagoria, J.L. A CFD (Computational Fluid Dynamics) Based Heat Transfer and Fluid Flow Analysis of a Solar Air Heater Provided with Transverse Wire Rib Roughness on the Absorber Plate. Energy 2013, 55, 1127–1142. [Google Scholar] [CrossRef]
- Yadav, A.S.; Bhagoria, J.L. A Numerical Investigation of Square Sectioned Transverse Rib Roughened Solar Air Heater. Int. J. Therm. Sci. 2014, 79, 111–131. [Google Scholar] [CrossRef]
- Yadav, A.S.; Bhagoria, J.L. A CFD Based Thermo-Hydraulic Performance Analysis of an Artificially Roughened Solar Air Heater Having Equilateral Triangular Sectioned Rib Roughness on the Absorber Plate. Int. J. Heat Mass Transf. 2014, 70, 1016–1039. [Google Scholar] [CrossRef]
- Gawande, V.B.; Dhoble, A.S.; Zodpe, D.B.; Chamoli, S. Experimental and CFD Investigation of Convection Heat Transfer in Solar Air Heater with Reverse L-Shaped Ribs. Sol. Energy 2016, 131, 275–295. [Google Scholar] [CrossRef]
- Kumar, S.; Verma, S.K. Three-Dimensional Simulation and Experimental Validation of Solar Air Heater Having Sinusoidal Rib. Energy Sources Part A Recover. Util. Environ. Eff. 2020, 12, 1–19. [Google Scholar] [CrossRef]
- Kumar, R.; Goel, V.; Kumar, A. Investigation of Heat Transfer Augmentation and Friction Factor in Triangular Duct Solar Air Heater Due to Forward Facing Chamfered Rectangular Ribs: A CFD Based Analysis. Renew. Energy 2018, 115, 824–835. [Google Scholar] [CrossRef]
- Kumar, R.; Kumar, A.; Goel, V. Performance Improvement and Development of Correlation for Friction Factor and Heat Transfer Using Computational Fluid Dynamics for Ribbed Triangular Duct Solar Air Heater. Renew. Energy 2019, 131, 788–799. [Google Scholar] [CrossRef]
- Kumar, R.; Goel, V. Unconventional Solar Air Heater with Triangular Flow-Passage: A CFD Based Comparative Performance Assessment of Different Cross-Sectional Rib-Roughnesses. Renew. Energy 2021, 172, 1267–1278. [Google Scholar] [CrossRef]
- Pandey, N.K.; Bajpai, V.K. Varun Experimental Investigation of Heat Transfer Augmentation Using Multiple Arcs with Gap on Absorber Plate of Solar Air Heater. Sol. Energy 2016, 134, 314–326. [Google Scholar] [CrossRef]
- Singh, I.; Vardhan, S.; Singh, S.; Singh, A. Experimental and CFD Analysis of Solar Air Heater Duct Roughened with Multiple Broken Transverse Ribs: A Comparative Study. Sol. Energy 2019, 188, 519–532. [Google Scholar] [CrossRef]
- Thao, P.B.; Truyen, D.C.; Phu, N.M. Cfd Analysis and Taguchi-Based Optimization of the Thermohydraulic Performance of a Solar Air Heater Duct Baffled on a Back Plate. Appl. Sci. 2021, 11, 4645. [Google Scholar] [CrossRef]
- Bhuvad, S.S.; Azad, R.; Lanjewar, A. Thermal Performance Analysis of Apex-up Discrete Arc Ribs Solar Air Heater-an Experimental Study. Renew. Energy 2022, 185, 403–415. [Google Scholar] [CrossRef]
- Verma, S.K.; Prasad, B.N. Investigation for the Optimal Thermohydraulic Performance of Artificially Roughened Solar Air Heaters. Renew. Energy 2000, 20, 19–36. [Google Scholar] [CrossRef]
- Bhagoria, J.L.; Saini, J.S.; Solanki, S.C. Heat Transfer Coefficient and Friction Factor Correlations for Rectangular Solar Air Heater Duct Having Transverse Wedge Shaped Rib Roughness on the Absorber Plate. Renew. Energy 2002, 25, 341–369. [Google Scholar] [CrossRef]
- Thakur, D.S.; Khan, M.K.; Pathak, M. Performance Evaluation of Solar Air Heater with Novel Hyperbolic Rib Geometry. Renew. Energy 2017, 105, 786–797. [Google Scholar] [CrossRef]
- Thakur, S.; Thakur, N.S. Impact of Multi-Staggered Rib Parameters of the ‘W’ Shaped Roughness on the Performance of a Solar Air Heater Channel. Energy Sources Part A Recover. Util. Environ. Eff. 2020, 12, 1–20. [Google Scholar] [CrossRef]
- Mahanand, Y.; Senapati, J.R. Thermo-Hydraulic Performance Analysis of a Solar Air Heater (SAH) with Quarter-Circular Ribs on the Absorber Plate: A Comparative Study. Int. J. Therm. Sci. 2021, 161, 106747. [Google Scholar] [CrossRef]
- Kumar, S.; Verma, S.K. Heat Transfer and Fluid Flow Analysis of Sinusoidal Protrusion Rib in Solar Air Heater. Int. J. Therm. Sci. 2022, 172, 107323. [Google Scholar] [CrossRef]
- Singh, V.P.; Jain, S.; Karn, A.; Kumar, A.; Dwivedi, G.; Meena, C.S.; Cozzolino, R. Mathematical Modeling of Efficiency Evaluation of Double-Pass Parallel Flow Solar Air Heater. Sustainability 2022, 14, 535. [Google Scholar] [CrossRef]
- Nidhul, K.; Yadav, A.K.; Anish, S.; Arunachala, U.C. Thermo-Hydraulic and Exergetic Performance of a Cost-Effective Solar Air Heater: CFD and Experimental Study. Renew. Energy 2022, 184, 627–641. [Google Scholar] [CrossRef]
- Singh, V.P.; Jain, S.; Karn, A.; Kumar, A.; Dwivedi, G.; Meena, C.S.; Dutt, N.; Ghosh, A. Recent Developments and Advancements in Solar Air Heaters: A Detailed Review. Sustainability 2022, 14, 12149. [Google Scholar] [CrossRef]
- Fakoor Pakdaman, M.; Lashkari, A.; Basirat Tabrizi, H.; Hosseini, R. Performance Evaluation of a Natural-Convection Solar Air-Heater with a Rectangular-Finned Absorber Plate. Energy Convers. Manag. 2011, 52, 1215–1225. [Google Scholar] [CrossRef]
- Fudholi, A.; Sopian, K.; Ruslan, M.H.; Othman, M.Y. Performance and Cost Benefits Analysis of Double-Pass Solar Collector with and without Fins. Energy Convers. Manag. 2013, 76, 8–19. [Google Scholar] [CrossRef]
- Priyam, A.; Chand, P. Thermal and Thermohydraulic Performance of Wavy Finned Absorber Solar Air Heater. Sol. Energy 2016, 130, 250–259. [Google Scholar] [CrossRef]
- Priyam, A.; Chand, P. Effect of Wavelength and Amplitude on the Performance of Wavy Finned Absorber Solar Air Heater. Renew. Energy 2018, 119, 690–702. [Google Scholar] [CrossRef]
- Bhattacharyya, T.; Anandalakshmi, R.; Srinivasan, K. Heat Transfer Analysis on Finned Plate Air Heating Solar Collector for Its Application in Paddy Drying. Energy Procedia 2017, 109, 353–360. [Google Scholar] [CrossRef]
- Kabeel, A.E.; Hamed, M.H.; Omara, Z.M.; Kandeal, A.W. Influence of Fin Height on the Performance of a Glazed and Bladed Entrance Single-Pass Solar Air Heater. Sol. Energy 2018, 162, 410–419. [Google Scholar] [CrossRef]
- Saravanan, A.; Murugan, M.; Reddy, M.S.; Ranjit, P.S.; Elumalai, P.V.; Kumar, P.; Sree, S.R. Thermo-Hydraulic Performance of a Solar Air Heater with Staggered C-Shape Finned Absorber Plate. Int. J. Therm. Sci. 2021, 168, 107068. [Google Scholar] [CrossRef]
- Chand, S.; Chand, P.; Kumar Ghritlahre, H. Thermal Performance Enhancement of Solar Air Heater Using Louvered Fins Collector. Sol. Energy 2022, 239, 10–24. [Google Scholar] [CrossRef]
- Karwa, R. Thermo-Hydraulic Performance of Solar Air Heater with Finned Absorber Plate Forming Multiple Rectangular Air Flow Passages in Parallel under Laminar Flow Conditions. Appl. Therm. Eng. 2023, 221, 119673. [Google Scholar] [CrossRef]
- Nagaraj, M.; Reddy, M.K.; Honnesara Sheshadri, A.K.; Karanth, K.V. Numerical Analysis of an Aerofoil Fin Integrated Double Pass Solar Air Heater for Thermal Performance Enhancement. Sustainability 2023, 15, 591. [Google Scholar] [CrossRef]
- Alam, M.W.; Souayeh, B. Parametric Cfd Thermal Performance Analysis of Full, Medium, Half and Short Length Dimple Solar Air Tube. Sustainability 2021, 13, 6462. [Google Scholar] [CrossRef]
- ANSYS. ANSYS Fluent 12.0 User’s Guide; ANSYS Inc.: Canonsburg, PA, USA, 2009. [Google Scholar]
- Wongcharee, K.; Changcharoen, W.; Eiamsa-Ard, S. Numerical Investigation of Flow Friction and Heat Transfer in a Channel with Various Shaped Ribs Mounted on Two Opposite Ribbed Walls. Int. J. Chem. React. Eng. 2011, 9, 2560. [Google Scholar] [CrossRef]
- Alam, T.; Kim, M.H. Heat Transfer Enhancement in Solar Air Heater Duct with Conical Protrusion Roughness Ribs. Appl. Therm. Eng. 2017, 126, 458–469. [Google Scholar] [CrossRef]
- Nidhul, K.; Yadav, A.K.; Anish, S.; Arunachala, U.C. Efficient Design of an Artificially Roughened Solar Air Heater with Semi-Cylindrical Side Walls: CFD and Exergy Analysis. Sol. Energy 2020, 207, 289–304. [Google Scholar] [CrossRef]
- Patel, S.S.; Lanjewar, A. Experimental Investigation of Solar Air Heater Duct with Discrete V-Rib Integrated with Staggered Elements. Int. J. Sustain. Eng. 2021, 14, 162–171. [Google Scholar] [CrossRef]
- Chaube, A.; Sahoo, P.K.; Solanki, S.C. Analysis of Heat Transfer Augmentation and Flow Characteristics Due to Rib Roughness over Absorber Plate of a Solar Air Heater. Renew. Energy 2006, 31, 317–331. [Google Scholar] [CrossRef]
- Boulemtafes-Boukadoum, A.; Benzaoui, A. CFD Based Analysis of Heat Transfer Enhancement in Solar Air Heater Provided with Transverse Rectangular Ribs. Energy Procedia 2014, 50, 761–772. [Google Scholar] [CrossRef]
- Choi, H.U.; Moon, K.A.; Kim, S.B.; Choi, K.H. CFD Analysis of the Heat Transfer and Fluid Flow Characteristics Using the Rectangular Rib Attached to the Fin Surface in a Solar Air Heater. Sustainability 2023, 15, 5382. [Google Scholar] [CrossRef]
- Choi, H.U.; Choi, K.H. CFD Analysis on the Heat Transfer and Fluid Flow of Solar Air Heater Having Transverse Triangular Block at the Bottom of Air Duct. Energies 2020, 13, 1099. [Google Scholar] [CrossRef]
- Singh, I.; Singh, S. CFD Analysis of Solar Air Heater Duct Having Square Wave Profiled Transverse Ribs as Roughness Elements. Sol. Energy 2018, 162, 442–453. [Google Scholar] [CrossRef]
- Gupta, A.D.; Varshney, L. Performance Prediction for Solar Air Heater Having Rectangular Sectioned Tapered Rib Roughness Using CFD. Therm. Sci. Eng. Prog. 2017, 4, 122–132. [Google Scholar] [CrossRef]

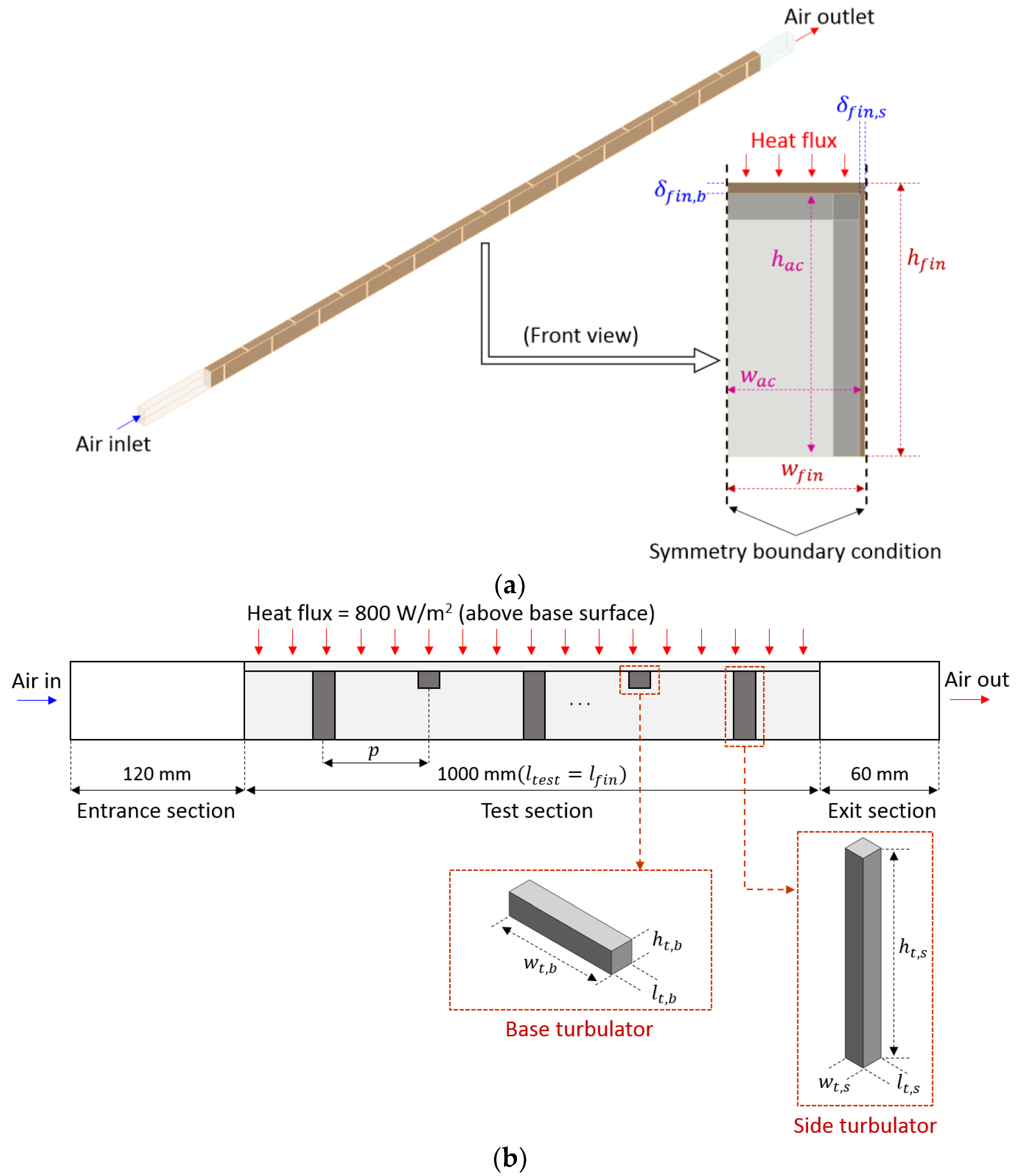




| Parameter | Value |
|---|---|
| Entrance region length (mm), | 120 |
| Test region length (mm), | 1000 |
| Exit region length (mm), | 60 |
| Air channel height (mm), | 24 |
| Air channel width (mm), | 12 |
| Fin height (mm), | 25 |
| Fin width (mm), | 12.5 |
| Fin length (mm), | 1000 |
| Fin thickness at the base surface (mm), | 1 |
| Fin thickness at the side surface (mm), | 0.5 |
| Turbulator height at the side surface (mm), | 24 |
| Turbulator width at the side surface (mm), | 2.4 |
| Turbulator length at the side surface (mm), | 2.4 |
| Turbulator height at the base surface (mm), | 2.4 |
| Turbulator width at the base surface (mm), | 12 |
| Turbulator length at the base surface (mm), | 2.4 |
| Turbulator pitch (mm), | 43.2 |
| Boundary | Conditions | Values |
|---|---|---|
| Air inlet | Velocity (m/s) | 1.83, 3.65, 5.48, 7.30, 9.13 |
| Reynolds number (-) | 3000, 6000, 9000, 12,000, 15,000 | |
| Air outlet | Constant pressure (Pa) | 101,325 |
| At the top of fin’s base surface | Uniform heat flux (W/m2) | 800 |
| Fin’s side surface | Symmetry | - |
| Center of air channel | Symmetry | - |
| Other walls | Adiabatic | - |
| Cell Number | (-) | (%) | (-) | (%) |
|---|---|---|---|---|
| 4,086,513 | 64.02 | - | 0.0810 | - |
| 5,105,280 | 65.25 | 1.93 | 0.0838 | 3.41 |
| 6,354,043 | 65.88 | 0.96 | 0.0857 | 2.30 |
| 7,516,107 | 65.65 | −0.34 | 0.0846 | −1.32 |
| 8,329,500 | 65.92 | 0.40 | 0.0847 | 0.15 |
| 9,096,384 | 65.78 | 0.20 | 0.0843 | −0.47 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
An, B.-H.; Choi, K.-H.; Choi, H.-U. Heat Transfer Augmentation and Friction Factor Due to the Arrangement of Rectangular Turbulators in a Finned Air Channel of a Solar Air Heater. Energies 2023, 16, 6891. https://doi.org/10.3390/en16196891
An B-H, Choi K-H, Choi H-U. Heat Transfer Augmentation and Friction Factor Due to the Arrangement of Rectangular Turbulators in a Finned Air Channel of a Solar Air Heater. Energies. 2023; 16(19):6891. https://doi.org/10.3390/en16196891
Chicago/Turabian StyleAn, Byeong-Hwa, Kwang-Hwan Choi, and Hwi-Ung Choi. 2023. "Heat Transfer Augmentation and Friction Factor Due to the Arrangement of Rectangular Turbulators in a Finned Air Channel of a Solar Air Heater" Energies 16, no. 19: 6891. https://doi.org/10.3390/en16196891
APA StyleAn, B.-H., Choi, K.-H., & Choi, H.-U. (2023). Heat Transfer Augmentation and Friction Factor Due to the Arrangement of Rectangular Turbulators in a Finned Air Channel of a Solar Air Heater. Energies, 16(19), 6891. https://doi.org/10.3390/en16196891