Optimal Configuration of Power-to-Heat Equipment Considering Peak-Shaving Ancillary Service Market
Abstract
:1. Introduction
2. Deep Peak-Shaving Income of the CHP Plant
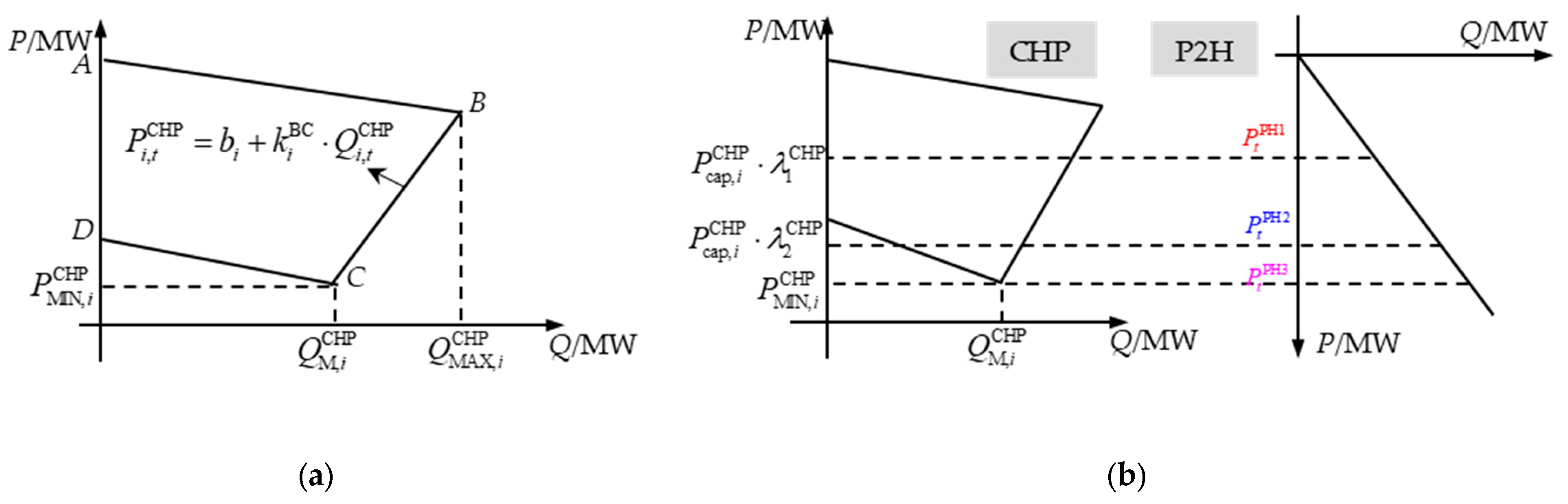
2.1. Deep Peak-Shaving Capacity Provided by the CHP Plant
2.2. Deep Peak-Shaving Capacity Required by the Power Grid
| Scenario 1 (S1): | |
| Scenario 2 (S2): | |
| Scenario 3 (S3): | |
| Scenario 4 (S4): | |
| Scenario 5 (S5): |
2.3. Deep Peak-Shaving Income of the CHP Plant
3. Optimal Configuration of the P2H Equipment
3.1. Total Fixed Cost of the P2H Equipment
3.2. Operation Income of the P2H Equipment
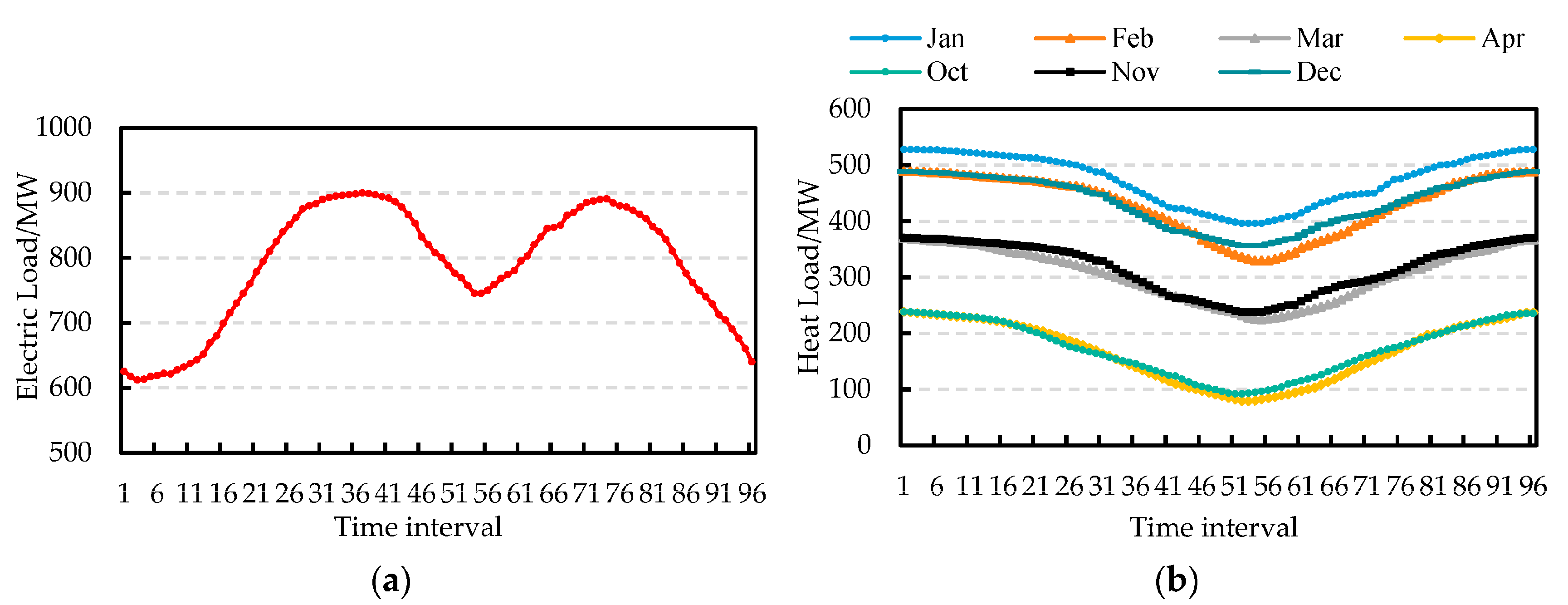
4. Case Studies
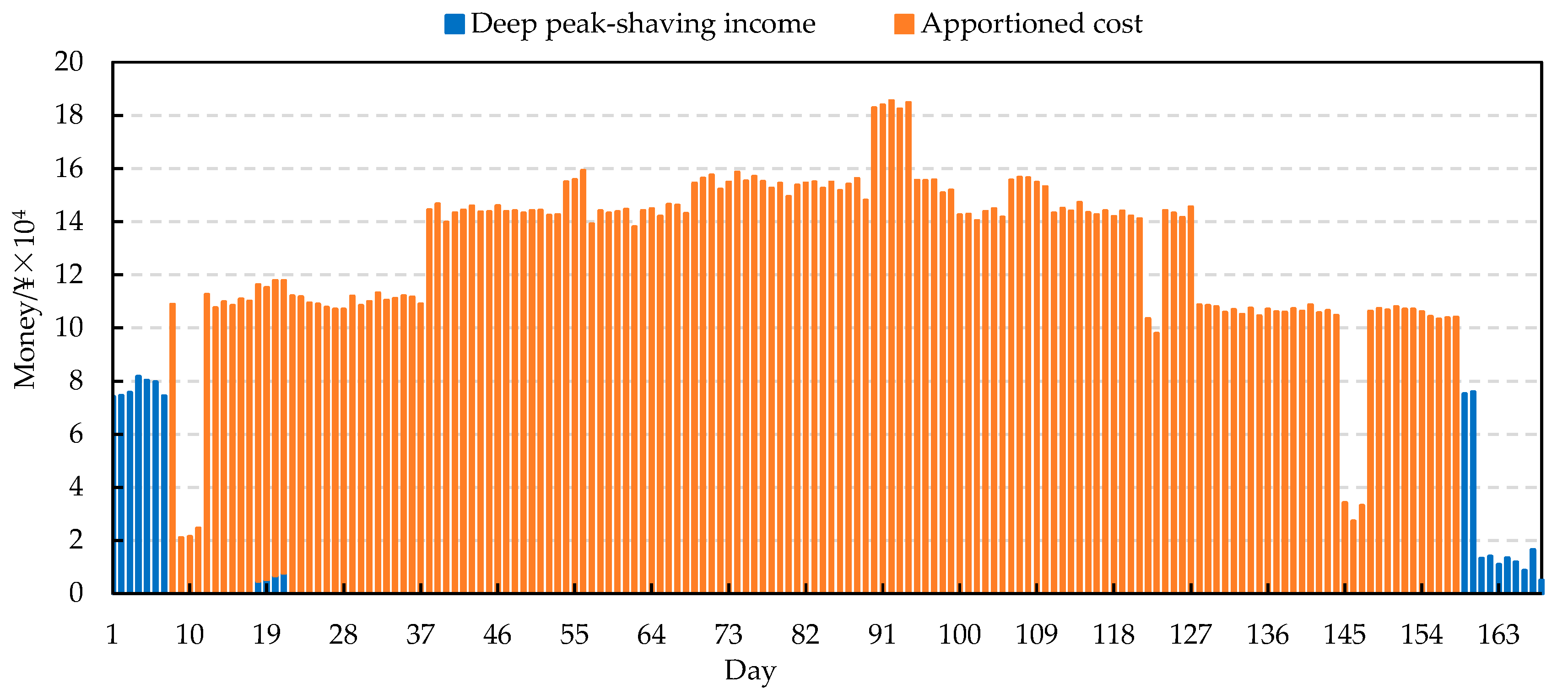
4.1. Analysis of the Deep Peak-Shaving Ability of the CHP Plant without the P2H Equipment
4.2. Analysis of Economic Benefits with Different P2H Equipment
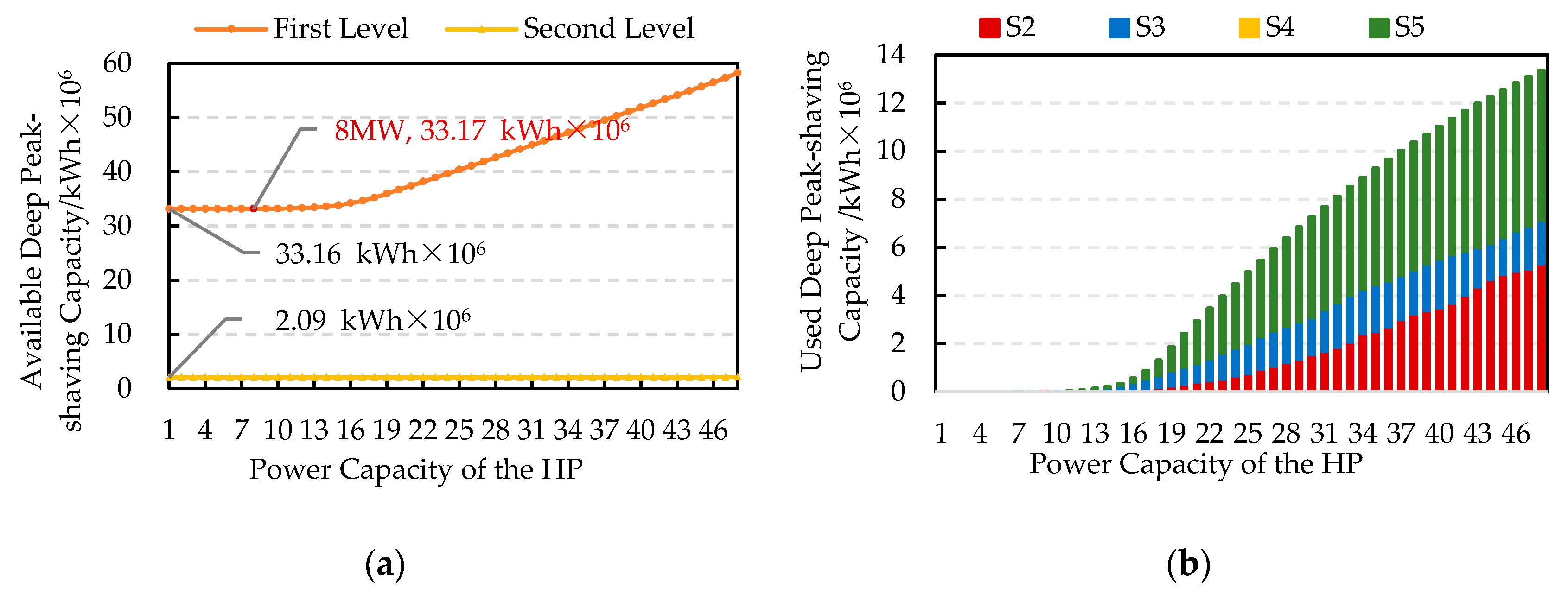
4.3. Analysis of Deep Peak-Shaving Ability of the CHP Plant with an HP
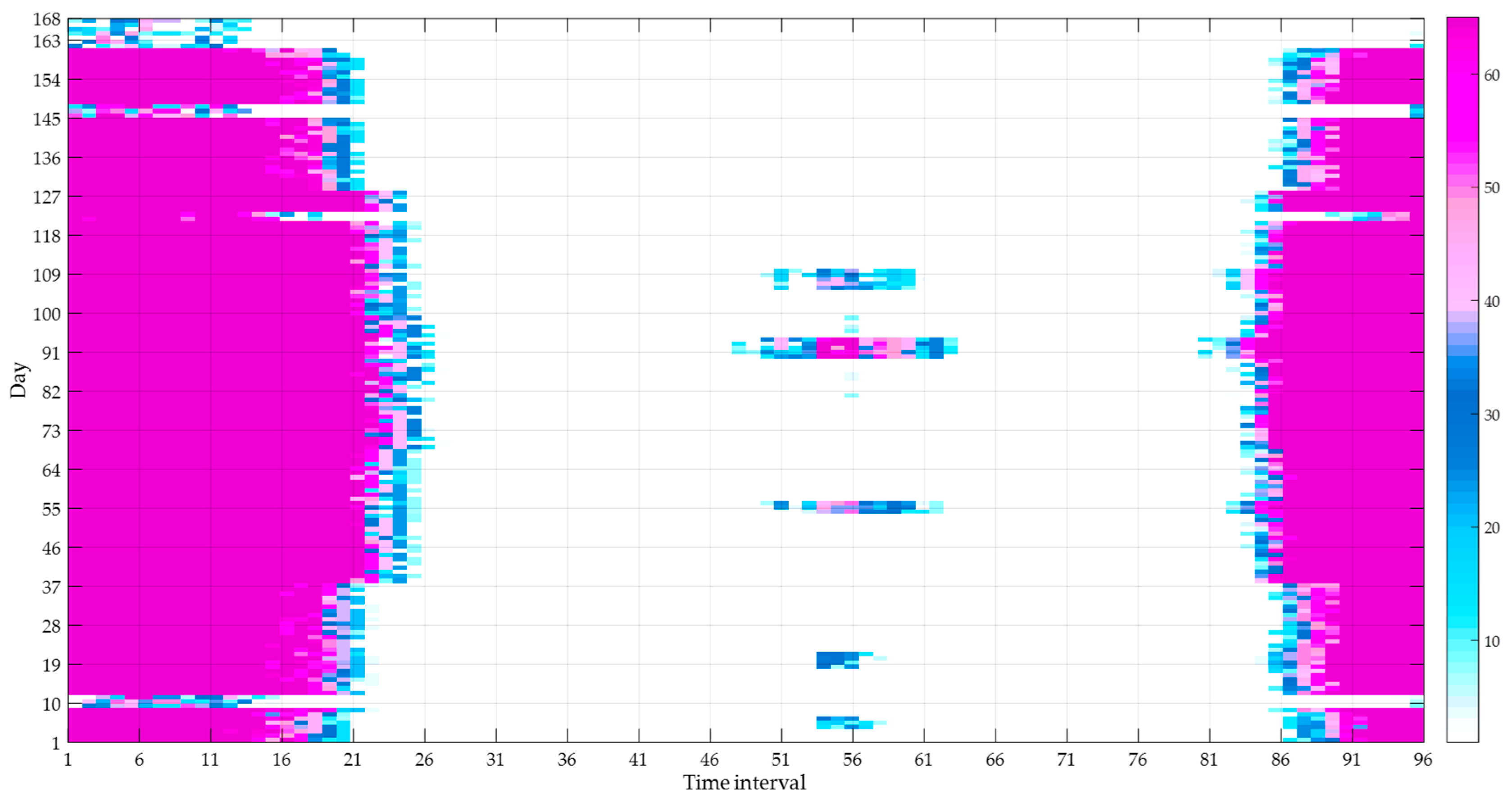
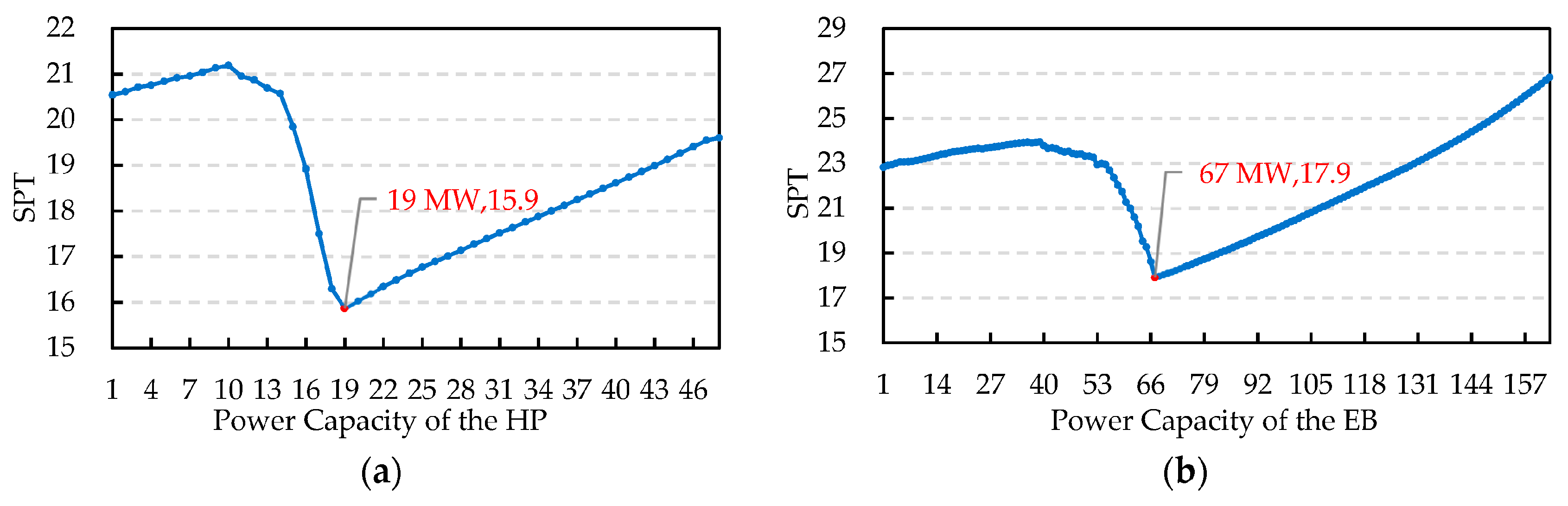
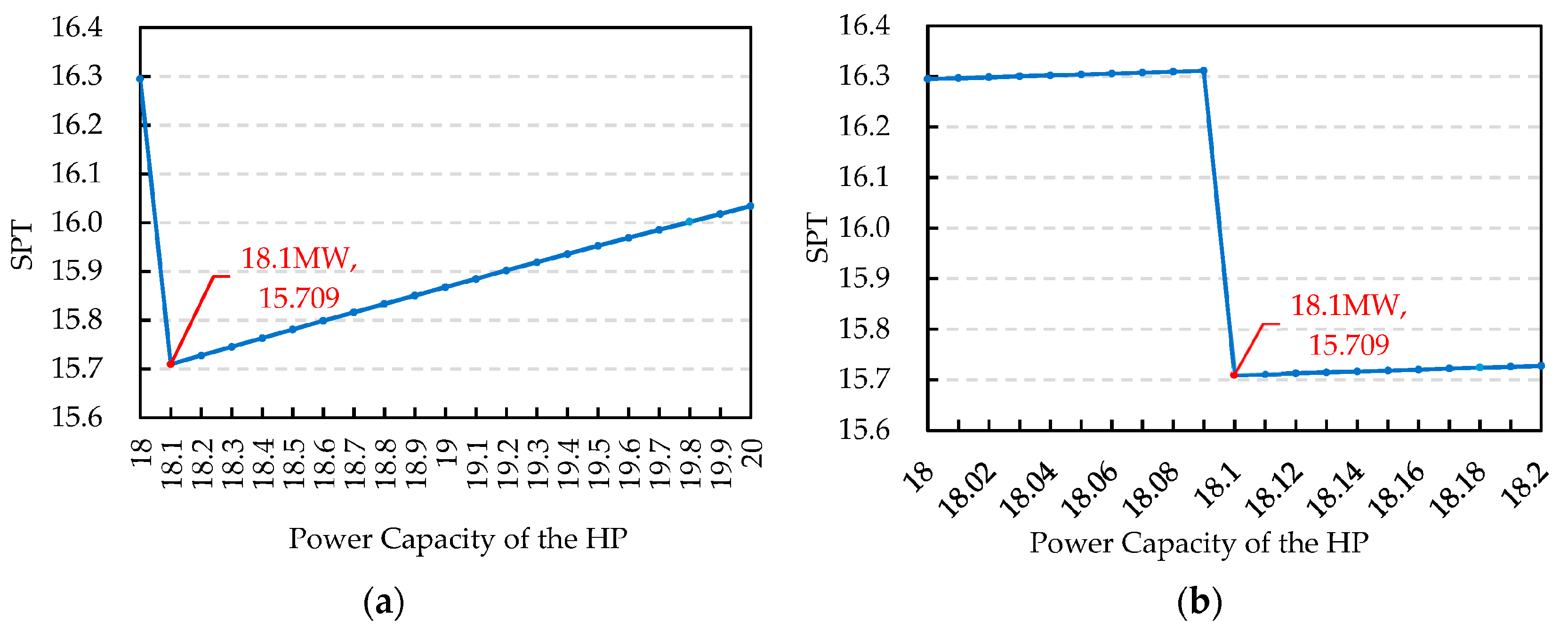
4.4. Analysis of Influence Factors of the Optimal P2H Equipment Capacity
5. Conclusions
- (1)
- It is necessary for the CHP plant to invest in P2H equipment in order to improve the peak-shaving ability of the CHP units.
- (2)
- It is beneficial for the CHP plant to invest in P2H equipment considering the economic benefits that may be obtained in the ancillary service market.
- (3)
- Due to higher energy conversion efficiency of the HP, investing in the HP has better economic benefits than investing in the EB. Furthermore, with the trend of continuous growth in wind power scale, the CHP plant will achieve higher investment returns.
- (4)
- The optimal capacity of the HP will not be affected by the deviation between historical and real-time data of quotation value, power load, and wind power. The optimization model proposed in this study is feasible and stable.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| thermal power output of the i-th CHP unit, MW | |
| maximum thermal power output of the i-th CHP unit, MW | |
| maximum thermal power output of the i-th CHP when the unit is operated at the minimum running power, MW | |
| running power of the P2H equipment, MW | |
| maximum running power of the P2H equipment, MW | |
| power capacity of the P2H equipment, MW | |
| heat load, MW | |
| generated electric power of the i-th CHP unit, MW | |
| minimum electric power output of the i-th CHP unit, MW | |
| power capacity of the i-th CHP unit, MW | |
| running power of the P2H equipment when CHP units running at the first base line of deep peak-shaving, MW | |
| running power of the P2H equipment when CHP units running at the second base line of deep peak-shaving, MW | |
| running power of the P2H equipment when CHP units running at the minimum power output, MW | |
| deep peak-shaving capacity at the first level available from the CHP plant, MW | |
| deep peak-shaving capacity at the second level available from the CHP plant, MW | |
| deep peak-shaving capacity required by the power grid, MW | |
| generated wind power, MW | |
| minimum power output of non-coal-fired units, MW | |
| power capacity of the j-th CON unit, MW | |
| electric power load, MW | |
| deep peak-shaving capacity at the first level available from the CON plant, MW | |
| first level of deep peak-shaving capacity actually provided by CHP units, MW | |
| second level of deep peak-shaving capacity actually provided by CHP units, MW | |
| transaction price of the power capacity provided by the P2H equipment on the d-th day in heating season, ¥/kWh | |
| transaction price of the deep peak-shaving capacity at the first level, ¥/kWh | |
| transaction price of the deep peak-shaving capacity at the second level, ¥/kWh | |
| daily modified power production value of wind power generators, MW | |
| daily modified power production value of solar power generators, MW | |
| daily modified power production value of nuclear power generators, MW | |
| compensation cost apportioned by the CHP plant with the P2H equipment, ¥ | |
| deep peak-shaving income of the CHP plant with the P2H equipment, ¥ | |
| minimum power generated by the CON plant, MW | |
| t | index of scheduling time interval |
| N1 | total number of CHP units |
| coefficient of performance | |
| expression coefficient | |
| expression coefficient | |
| approximate value of the electrothermal ratio | |
| first base line of deep peak-shaving set for the CHP unit | |
| second base line of deep peak-shaving set for the CHP unit | |
| N2 | total number of CHP units |
| first base line of deep peak-shaving set for the CON unit | |
| second base line of deep peak-shaving set for the CON unit | |
| D | total days of the heating season |
| lifetime of the P2H equipment | |
| ratio of maintenance cost | |
| carbon emission coefficient of standard coal | |
| correction factor | |
| deep peak-shaving income of the CHP plant, ¥ | |
| daily income of the P2H equipment, ¥ | |
| total fixed cost of the of the P2H equipment, ¥ | |
| unit price of the P2H equipment, ¥/MW | |
| reduced coal cost, ¥ | |
| reduced carbon trading cost, ¥ | |
| reduced compensation cost of deep peak-shaving apportioned by the CHP plant, ¥ | |
| increased deep peak-shaving income, ¥ | |
| unit price of coal, ¥/ton | |
| unit price of carbon trading, ¥/ton | |
| reduced coal for thermal power production, ton | |
| daily modified power production value of CHP units, MW | |
| compensation cost apportioned by the CHP plant without the P2H equipment, ¥ | |
| deep peak-shaving income of the CHP plant without the P2H equipment, ¥ |
References
- Jin, T.; Chen, X.; Wen, J.; Wu, Q.; Bai, L.; Liu, Y.; Cao, Y. Improved ramping and reserve modeling of combined heat and power in integrated energy systems for better renewable integration. IEEE Trans. Sustain. Energy 2021, 13, 683–692. [Google Scholar] [CrossRef]
- Chen, H.; Chen, J.; Han, G.; Gui, Q. Winding down the wind power curtailment in China: What made the difference? Renew. Sustain. Energy Rev. 2022, 167, 112725. [Google Scholar] [CrossRef]
- Yu, Y.; Chen, H.; Chen, L.; Chen, C.; Wu, J. Optimal operation of the combined heat and power system equipped with power-to-heat devices for the improvement of wind energy utilization. Energy Sci. Eng. 2019, 7, 1605–1620. [Google Scholar] [CrossRef]
- Zhao, P.; Gou, F.; Xu, W.; Shi, H.; Wang, J. Multi-objective optimization of a hybrid system based on combined heat and compressed air energy storage and electrical boiler for wind power penetration and heat-power decoupling purposes. J. Energy Storage 2023, 58, 106353. [Google Scholar] [CrossRef]
- Ma, Y.F.; Yang, Y.; Mi, Z.Q. Accommodation of curtailed wind power by electric boilers equipped in different locations of heat-supply network for power system with CHPs. J. Mod. Power Syst. Clean Energy 2021, 9, 930–939. [Google Scholar] [CrossRef]
- Tian, L.; Xie, Y.; Hu, B.; Liu, X.; Deng, T.; Luo, H.; Li, F. A deep peak regulation auxiliary service bidding strategy for CHP units based on a risk-averse model and district heating network energy storage. Energies 2019, 12, 3314. [Google Scholar] [CrossRef]
- Zhang, Z.; Cong, W.; Liu, S.; Li, C.; Qi, S. Auxiliary service market model considering the participation of pumped-storage power stations in peak shaving. Front. Energy Res. 2022, 10, 745. [Google Scholar] [CrossRef]
- Yu, Y.J.; Chen, H.K.; Chen, L. Comparative study of electric energy storages and thermal energy auxiliaries for improving wind power integration in the cogeneration system. Energies 2018, 11, 263. [Google Scholar] [CrossRef]
- Zhang, D.; Hu, Y.; Gao, Y. Flexibility improvement of CHP unit for wind power accommodation. J. Mod. Power Syst. Clean Energy 2021, 10, 731–742. [Google Scholar] [CrossRef]
- Zhao, S.; Ge, Z.; Sun, J.; Ding, Y.; Yang, Y. Comparative study of flexibility enhancement technologies for the coal-fired combined heat and power plant. Energy Convers. Manag. 2019, 184, 15–23. [Google Scholar] [CrossRef]
- Yan, R.; Wang, J.; Huo, S.; Zhang, J.; Tang, S.; Yang, M. Comparative study for four technologies on flexibility improvement and renewable energy accommodation of combined heat and power system. Energy 2023, 263, 126056. [Google Scholar] [CrossRef]
- Lyu, Q.; Gong, H.; Yang, N.; Xu, X.; Zhang, N.; Wang, H. An evaluation method of wind power integration in power systems with flexible combined heat and power plant. Energies 2019, 12, 4129. [Google Scholar] [CrossRef]
- Lei, Z.; Wang, G.; Li, T.; Cheng, S.; Yang, J.; Cui, J. Strategy analysis about the active curtailed wind accommodation of heat storage electric boiler heating. Energy Rep. 2021, 7, 65–71. [Google Scholar] [CrossRef]
- Lepiksaar, K.; Mašatin, V.; Latõšov, E.; Siirde, A.; Volkova, A. Improving CHP flexibility by integrating thermal energy storage and power-to-heat technologies into the energy system. Smart Energy 2021, 2, 100022. [Google Scholar] [CrossRef]
- Han, X.; Wang, F.; Tian, C.; Xue, K.; Zhang, J. Economic evaluation of actively consuming wind power for an integrated energy system based on game theory. Energies 2018, 11, 1476. [Google Scholar] [CrossRef]
- Liu, B.; Li, J.; Zhang, S.; Gao, M.; Ma, H.; Li, G.; Gu, C. Economic dispatch of combined heat and power energy systems using electric boiler to accommodate wind power. IEEE Access 2020, 8, 41288–41297. [Google Scholar] [CrossRef]
- Bloess, A.; Schill, W.P.; Zerrahn, A. Power-to-heat for renewable energy integration: A review of technologies, modeling approaches, and flexibility potentials. Appl. Energy 2018, 212, 1611–1626. [Google Scholar] [CrossRef]
- Walden, J.V.; Bähr, M.; Glade, A.; Gollasch, J.; Tran, A.P.; Lorenz, T. Nonlinear operational optimization of an industrial power-to-heat system with a high temperature heat pump, a thermal energy storage and wind energy. Appl. Energy 2023, 344, 121247. [Google Scholar] [CrossRef]
- Wang, J.; Zhou, Z.; Zhao, J.; Zheng, J. Improving wind power integration by a novel short-term dispatch model based on free heat storage and exhaust heat recycling. Energy 2018, 160, 940–953. [Google Scholar] [CrossRef]
- Dengiz, T.; Jochem, P.; Fichtner, W. Demand response with heuristic control strategies for modulating heat pumps. Appl. Energy 2019, 238, 1346–1360. [Google Scholar] [CrossRef]
- Blarke, M.B. Towards an intermittency-friendly energy system: Comparing electric boilers and heat pumps in distributed cogeneration. Appl. Energy 2012, 91, 349–365. [Google Scholar] [CrossRef]
- Liu, M.; Wang, S.; Yan, J. Operation scheduling of a coal-fired CHP station integrated with power-to-heat devices with detail CHP unit models by particle swarm optimization algorithm. Energy 2021, 214, 119022. [Google Scholar] [CrossRef]
- Treshcheva, M.; Anikina, I.; Sergeev, V.; Skulkin, S.; Treshchev, D. Selection of heat pump capacity used at thermal power plants under electricity market operating conditions. Energies 2021, 14, 226. [Google Scholar] [CrossRef]
- Liu, M.; Ma, G.; Wang, S.; Wang, Y.; Yan, J. Thermo-economic comparison of heat–power decoupling technologies for combined heat and power plants when participating in a power-balancing service in an energy hub. Renew. Sustain. Energy Rev. 2021, 152, 111715. [Google Scholar] [CrossRef]
- Wang, Z.; Gu, Y.; Lu, S.; Zhao, Z. Optimization of thermocline heat storage tank capacity for combined heat and power plant based on environmental benefits: Scenarios for China. J. Energy Storage 2023, 57, 106303. [Google Scholar] [CrossRef]
- Østergaard, P.A.; Andersen, A.N. Optimal heat storage in district energy plants with heat pumps and electrolysers. Energy 2023, 275, 127423. [Google Scholar] [CrossRef]
- Liu, D.; Zhang, G.; Huang, B.; Liu, W. Optimum electric boiler capacity configuration in a regional power grid for a wind power accommodation scenario. Energies 2016, 9, 144. [Google Scholar] [CrossRef]
- Yi, Z.K.; Xu, Y.L.; Li, Z.M. Optimal configuration of distributed heat sources to promote the wind power penetration in combined heat and power system. J. Renew. Sustain. Energy 2019, 11, 036302. [Google Scholar] [CrossRef]
- Gou, X.; Chen, Q.; Hu, K.; Ma, H.; Chen, L.; Wang, X.-H.; Qi, J.; Xu, F.; Min, Y. Optimal planning of capacities and distribution of electric heater and heat storage for reduction of wind power curtailment in power systems. Energy 2018, 160, 763–773. [Google Scholar] [CrossRef]
- Li, L.; Wang, Y.; Wu, Q.; Li, J.; Wang, D.; Han, M. Analysis on optimal capacity and parameter configuration of electrode type boiler for improving wind power consumption capacity. In Proceedings of the 2022 IEEE 4th International Conference on Power, Intelligent Computing and Systems (ICPICS), Shenyang, China, 29–31 July 2022; pp. 768–772. [Google Scholar]
- Nielsen, M.G.; González, J.M.M.; Madsen, H.; Zugno, M.; Boldt, J.; Engberg, T.; Nielsen, H.A. Probabilistic forecasting and optimization in CHP systems. Master’s Thesis, Department of Applied Mathematics and Computer Science, Technical University of Denmark, Lyngby, Denmark, 2014. [Google Scholar]
- Lv, Q.; Du, S.Y.; Liu, L. Economic Analysis on Peak Shaving of Combined Heat and Power Plant with Electric Boilers in Auxiliary Service Market of Northeast China. Autom. Electr. Power Syst. 2019, 20, 49–56. [Google Scholar]
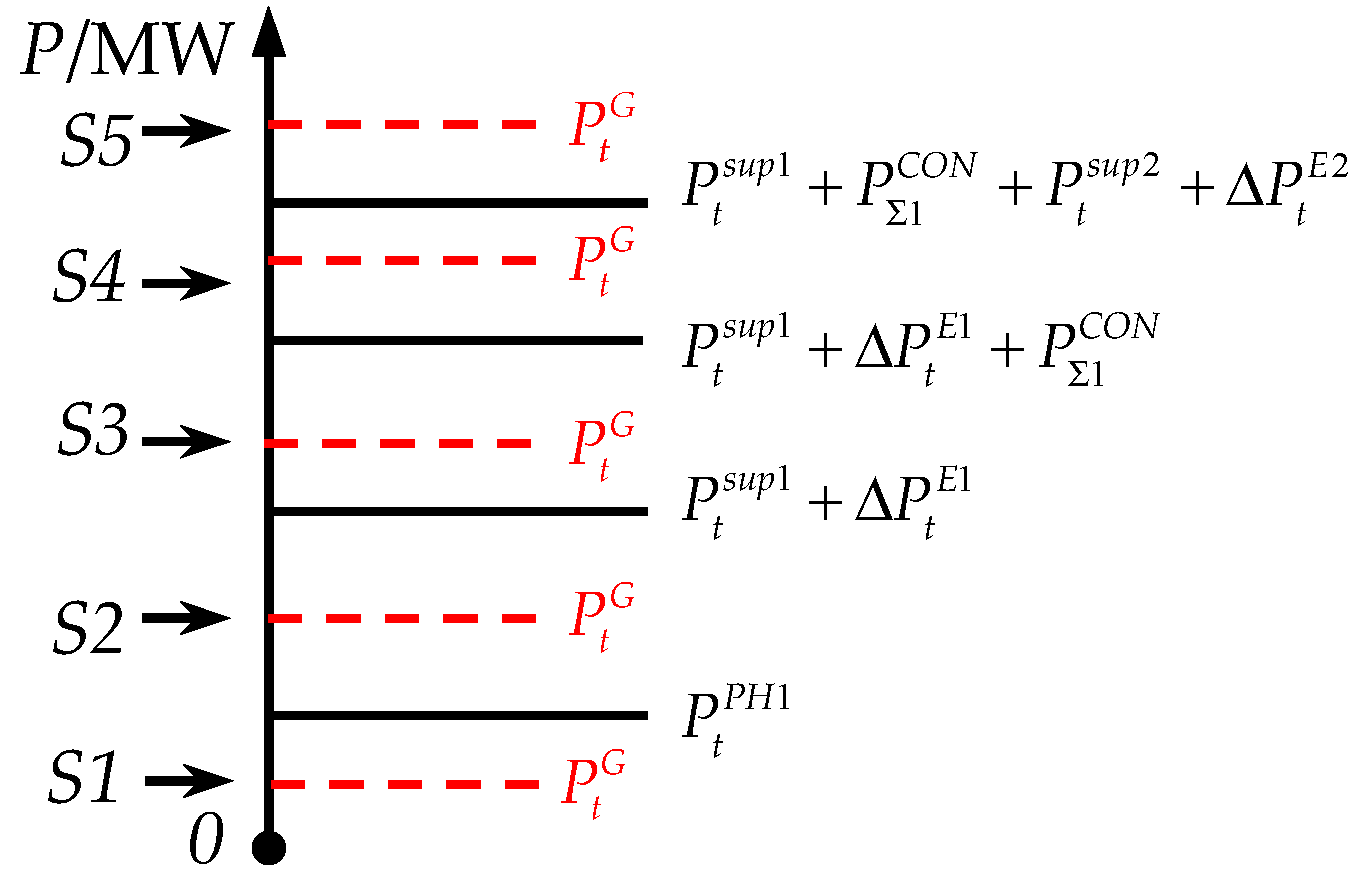
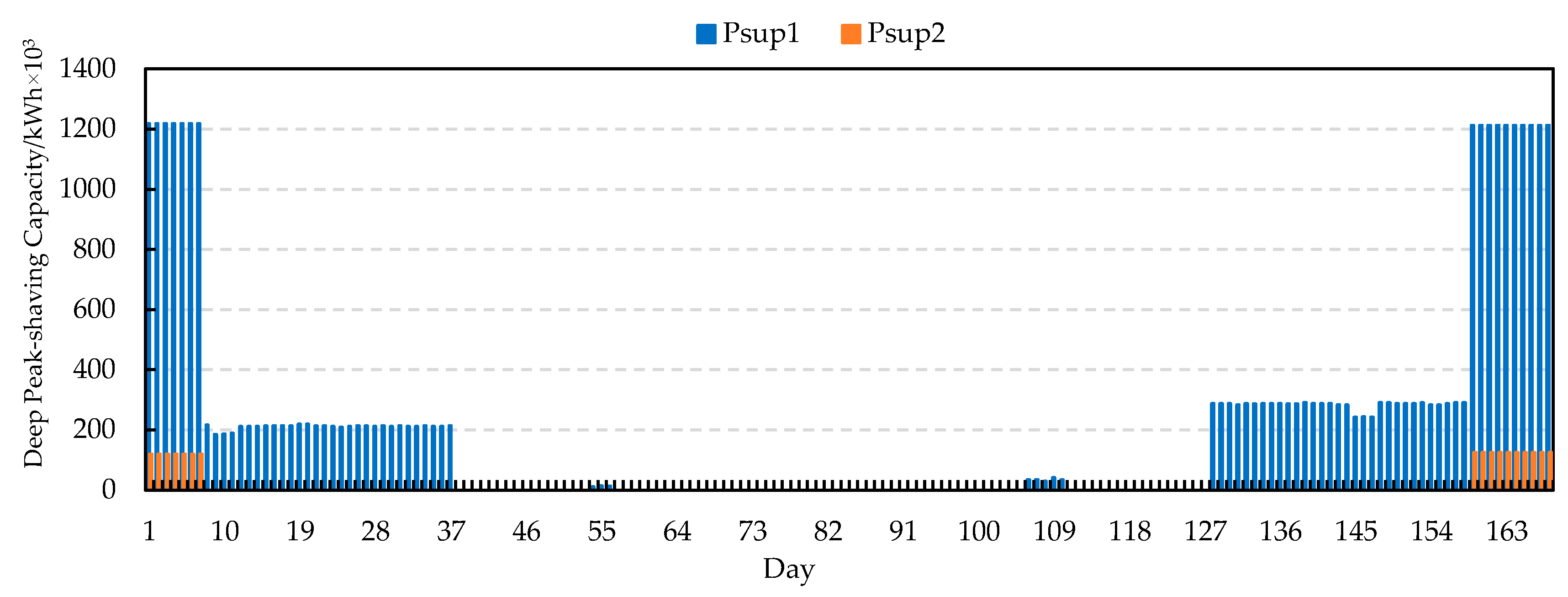

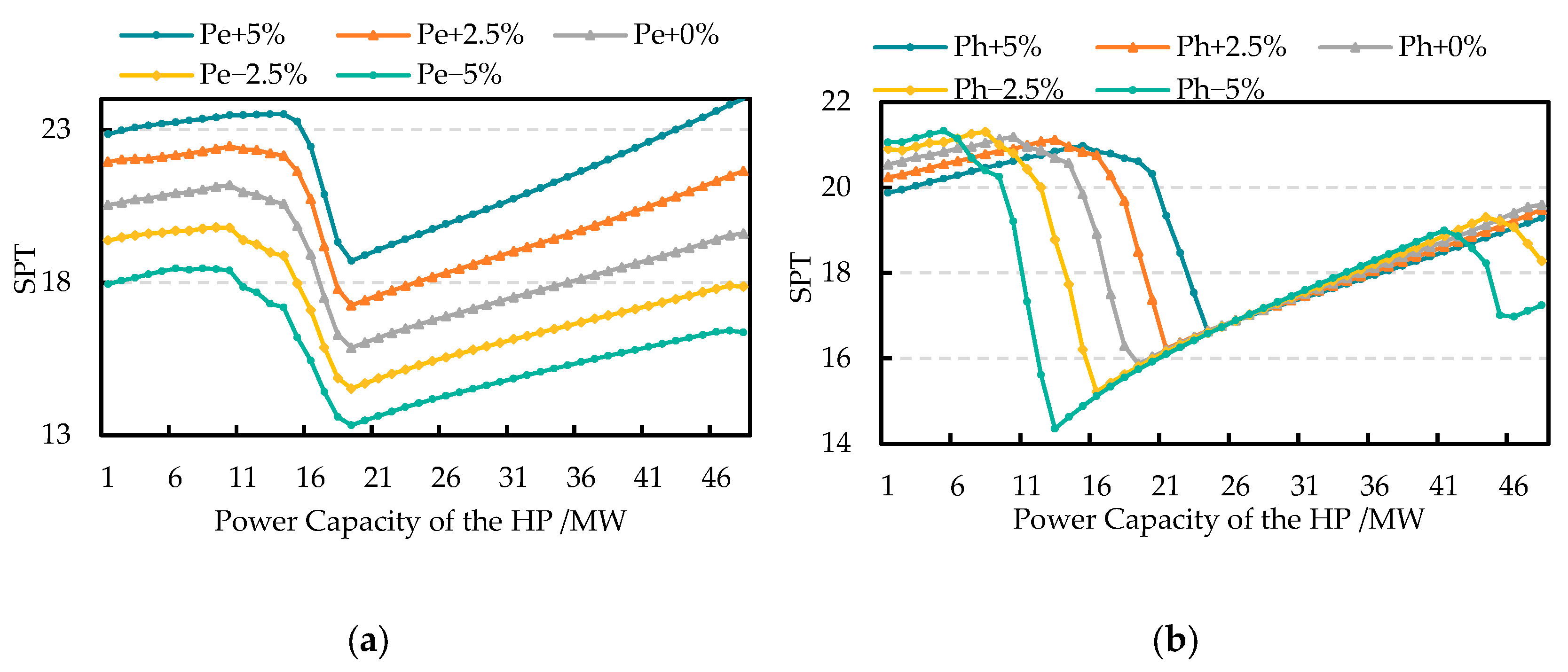

| Parameters | Ucoal | Qcoal | kav | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Value | 114 MW | 105 MW | 56 MW | 73.4 MW | 840 ¥/ton | 135 kg/kWh | 56 ¥/ton | 2.66 | 0.45 |
| Parameters | η | yPH | cPH | |
|---|---|---|---|---|
| EB | 95% | 20 years | 30% | 980 k¥/MW |
| HP | 3.5 | 20 years | 15% | 4500 k¥/MW |
| Parameters | /¥ × 104 | /¥ × 104 | /¥ × 104 | /¥ × 104 | /¥ × 104 | /¥ × 104 |
|---|---|---|---|---|---|---|
| Without P2H | 81.03 | 1952.36 | 0 | 0 | 0 | 0 |
| EB = 67 MW | 563.15 | 787.18 | 780.33 | 138.38 | 2566.01 | 45,962 |
| HP = 19 MW | 293.91 | 1074.30 | 904.04 | 160.32 | 2155.30 | 34,200 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, Y.; Zhou, G.; Wu, K.; Chen, C.; Bian, Q. Optimal Configuration of Power-to-Heat Equipment Considering Peak-Shaving Ancillary Service Market. Energies 2023, 16, 6860. https://doi.org/10.3390/en16196860
Yu Y, Zhou G, Wu K, Chen C, Bian Q. Optimal Configuration of Power-to-Heat Equipment Considering Peak-Shaving Ancillary Service Market. Energies. 2023; 16(19):6860. https://doi.org/10.3390/en16196860
Chicago/Turabian StyleYu, Yanjuan, Guohua Zhou, Kena Wu, Cheng Chen, and Qiang Bian. 2023. "Optimal Configuration of Power-to-Heat Equipment Considering Peak-Shaving Ancillary Service Market" Energies 16, no. 19: 6860. https://doi.org/10.3390/en16196860
APA StyleYu, Y., Zhou, G., Wu, K., Chen, C., & Bian, Q. (2023). Optimal Configuration of Power-to-Heat Equipment Considering Peak-Shaving Ancillary Service Market. Energies, 16(19), 6860. https://doi.org/10.3390/en16196860







