DC Voltage Source Based on a Battery of Supercapacitors with a Regulator in the Form of an Isolated Boost LCC Resonant Converter
Abstract
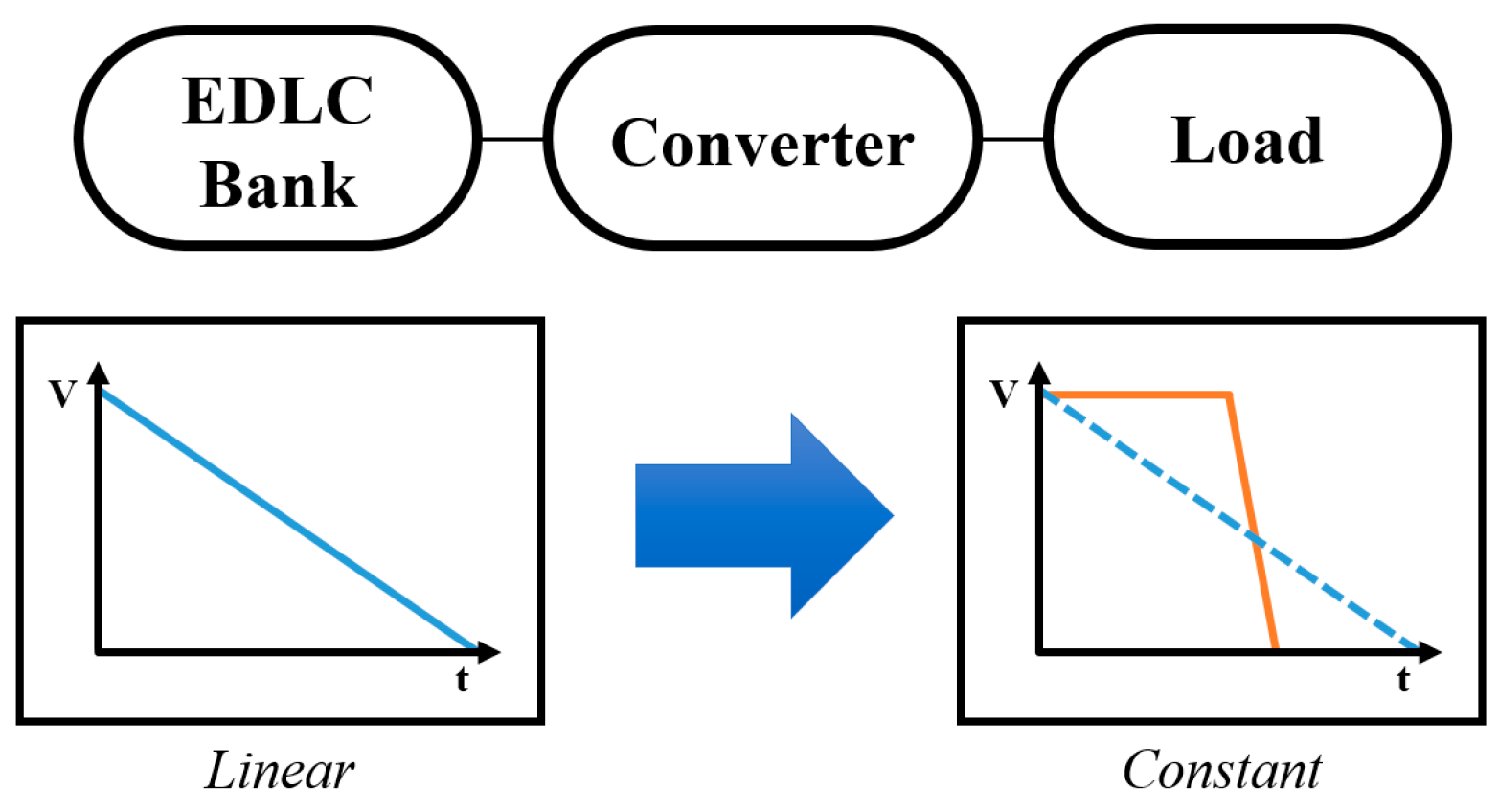
:1. Introduction
2. 64.8-V, 400-F EDLC Stand-Alone System Design and Analysis
2.1. Supercapacitor Bank
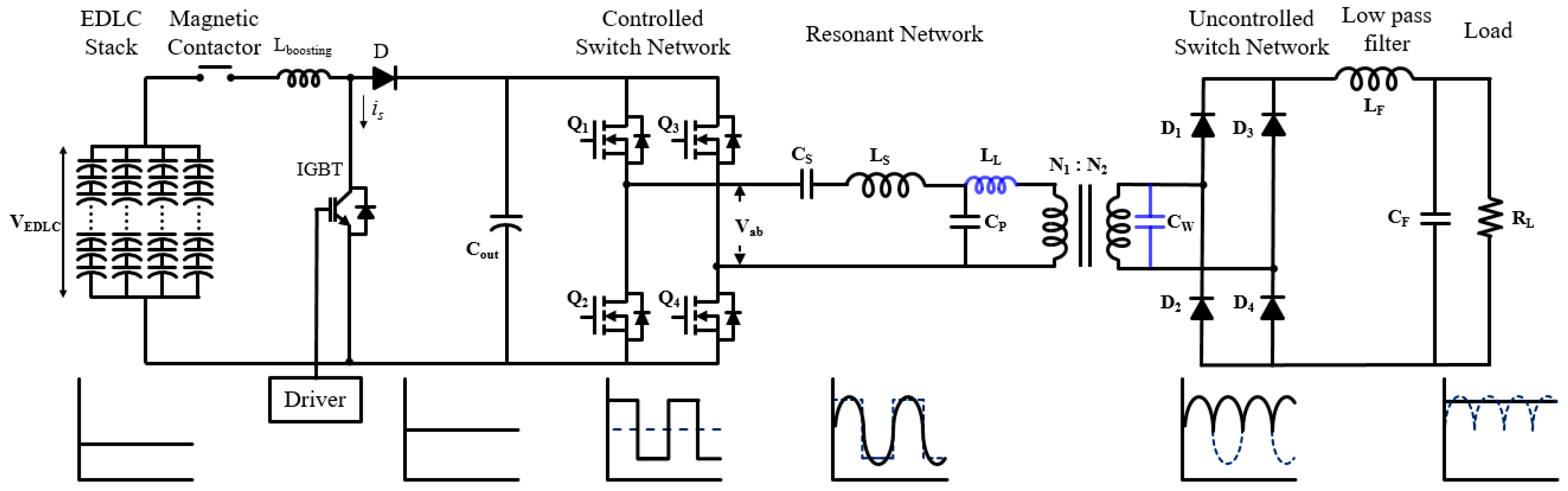
2.2. Isoltaed Boost LCC Series-Parallel Resonant Converter
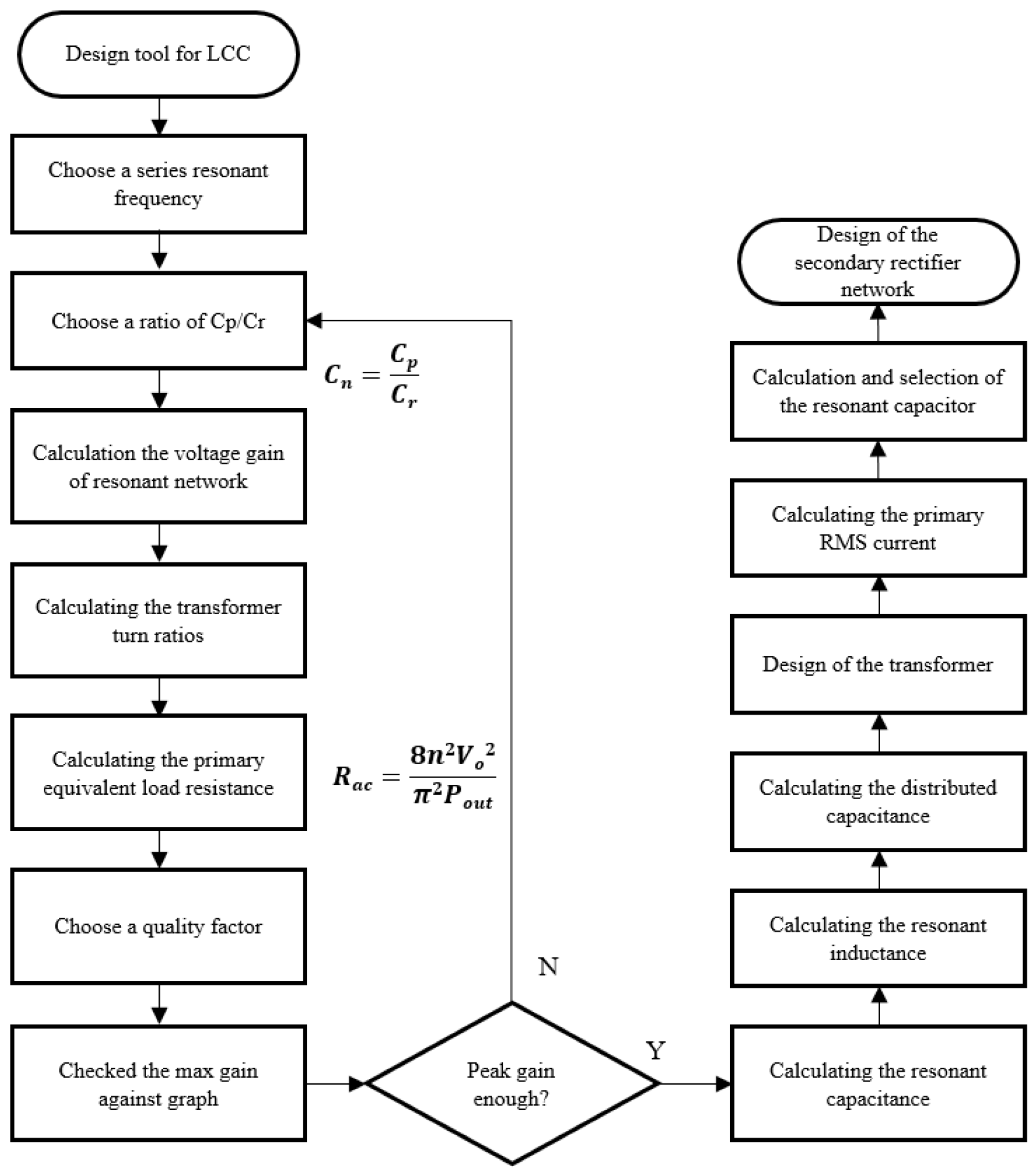
2.3. Isoltaed Boost LCC Series-Parallel Resonant Converter Design and Analysis
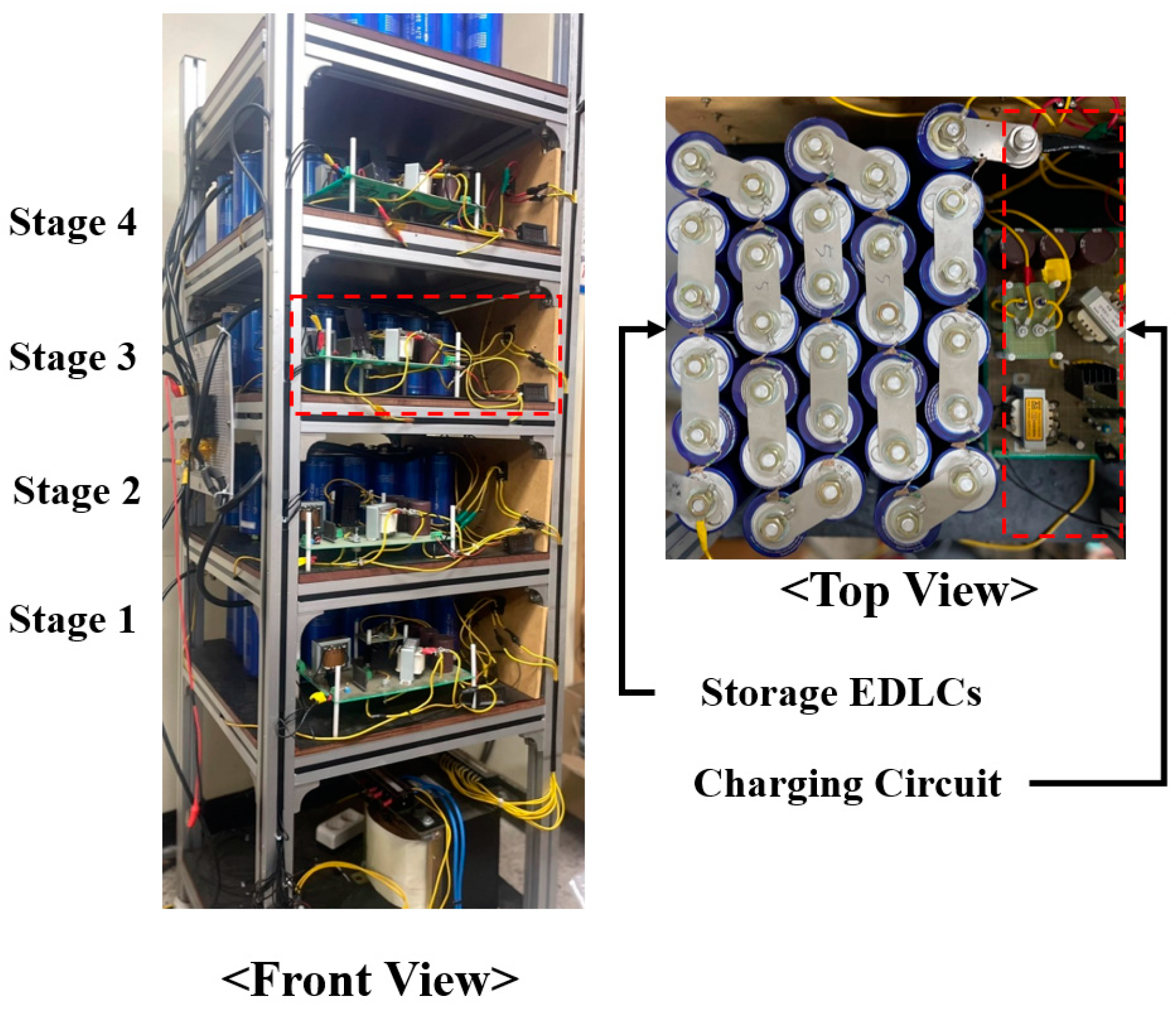
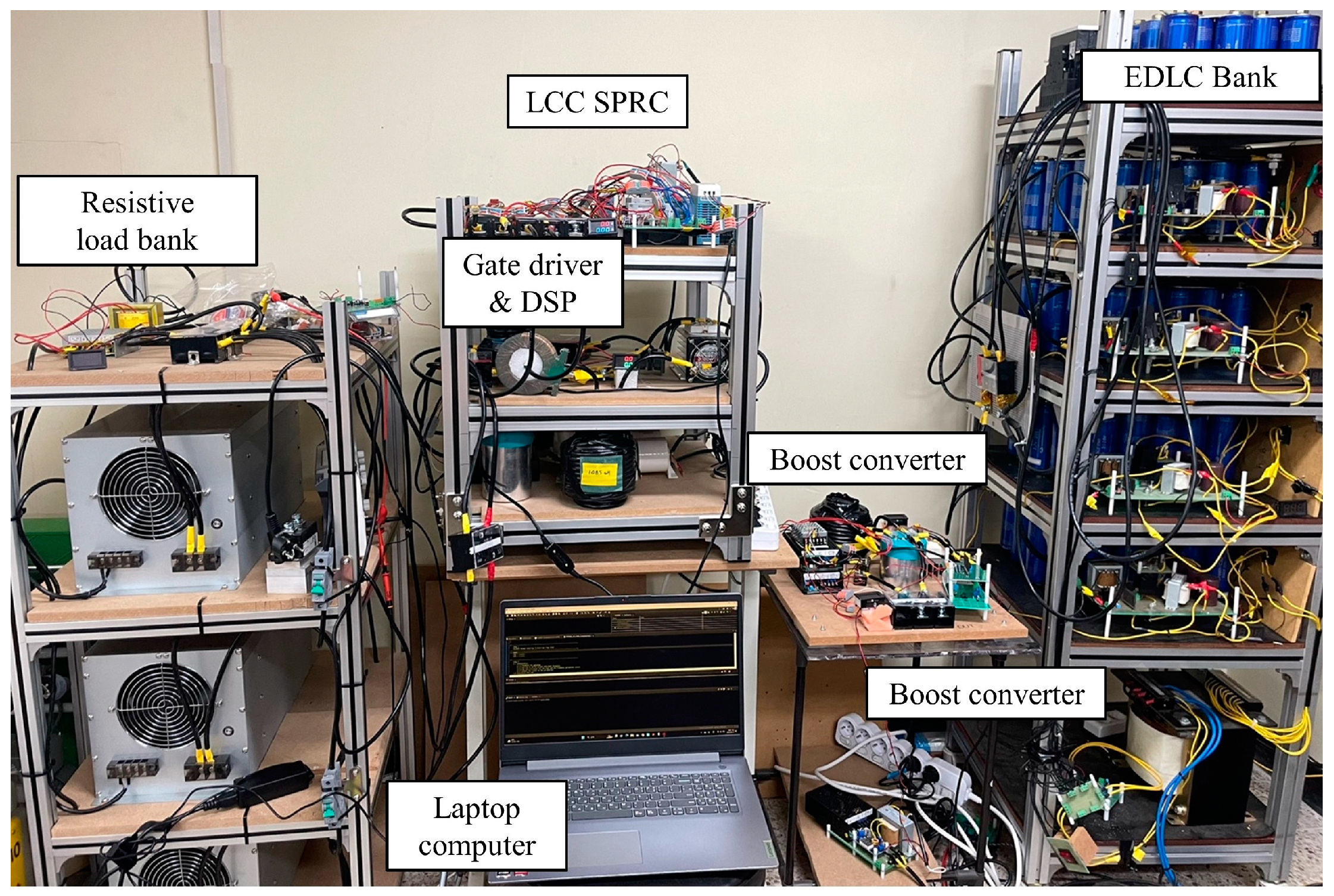
3. Description of the Experimental System Setup
3.1. 233-Wh EDLC Bank
3.2. Isolated Boost Series-Parallel Reosnant Converter
4. Experimental Results
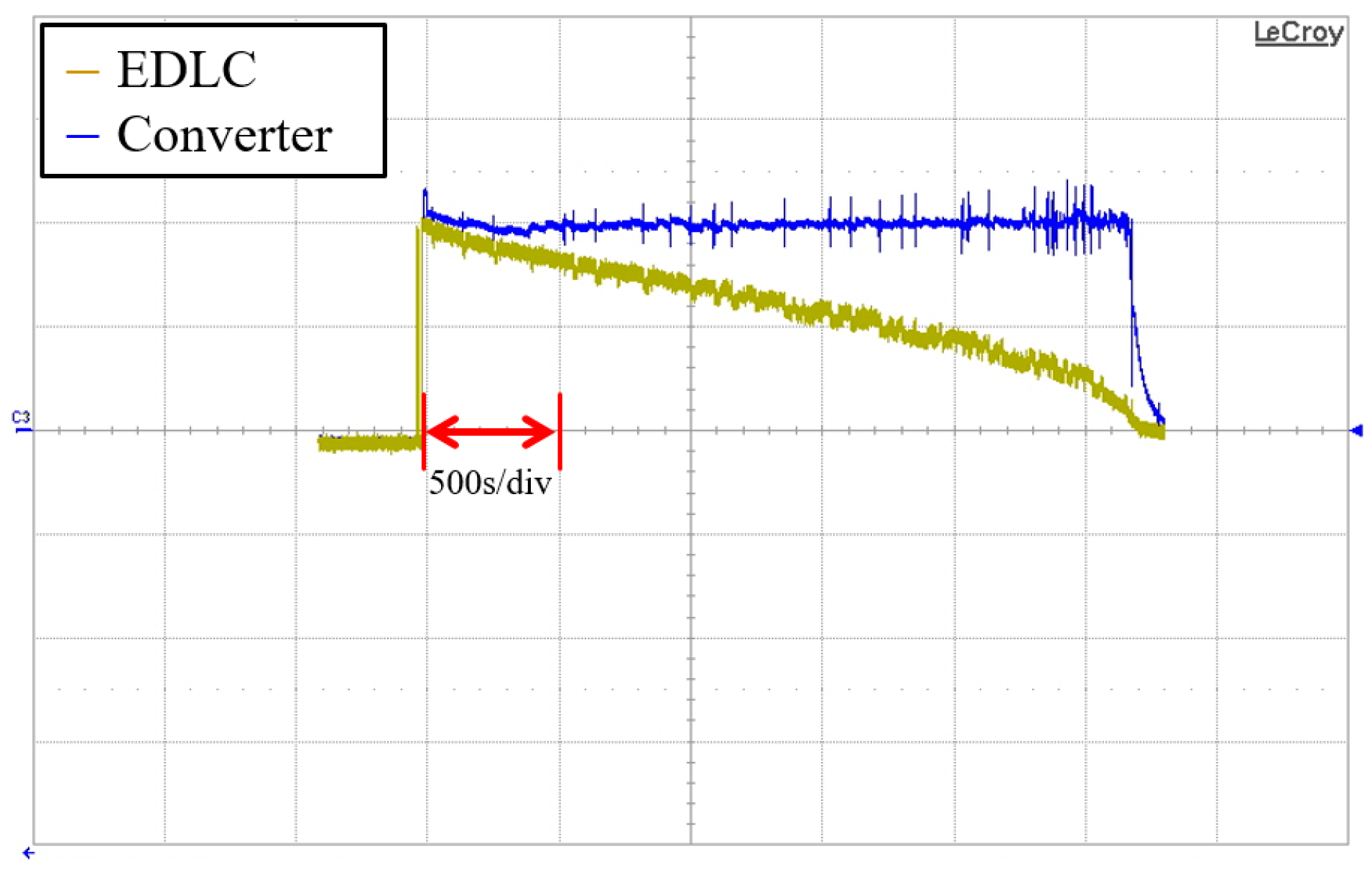
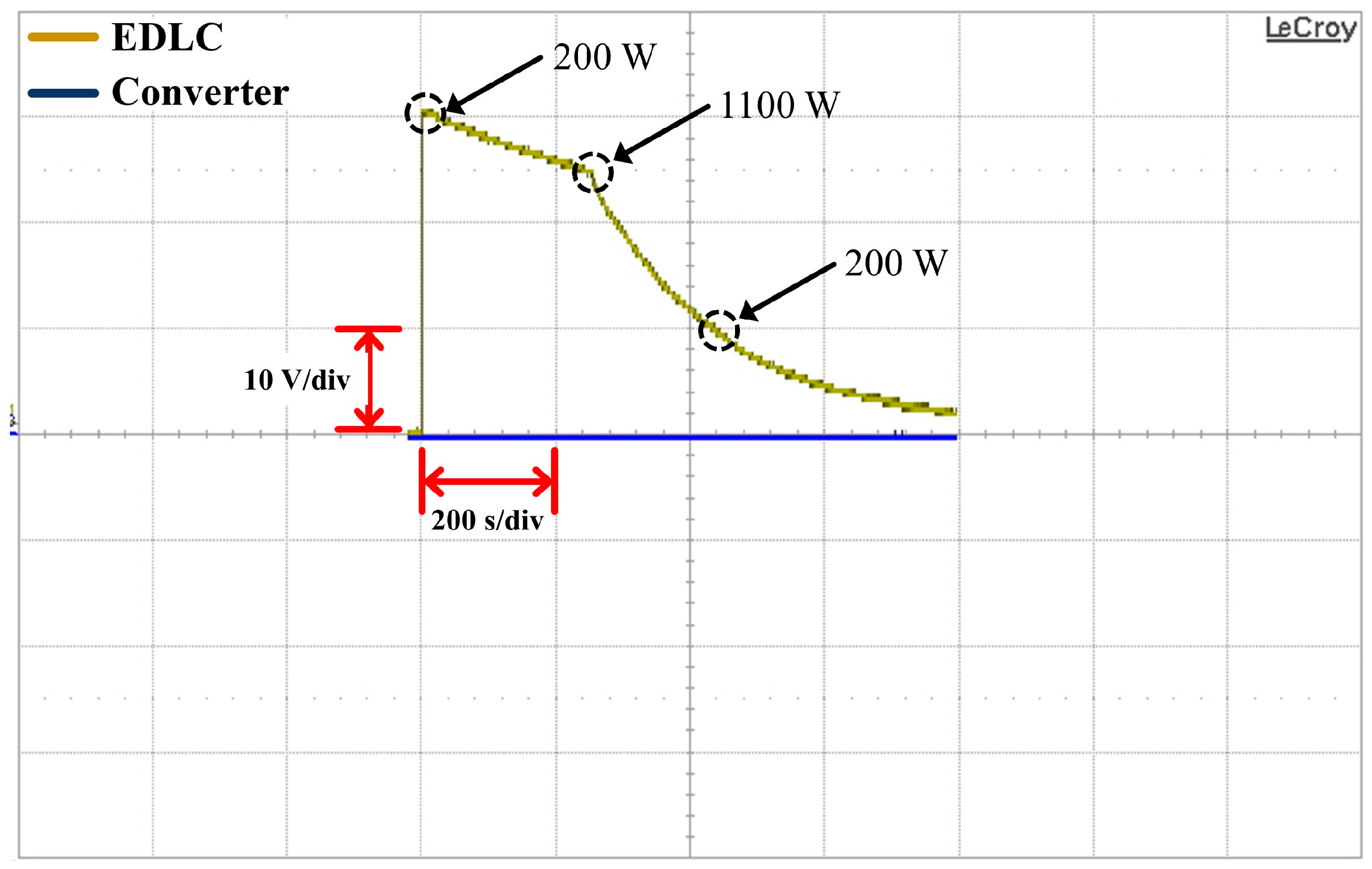
4.1. Constant Voltage Output with Supercapacitor Bank
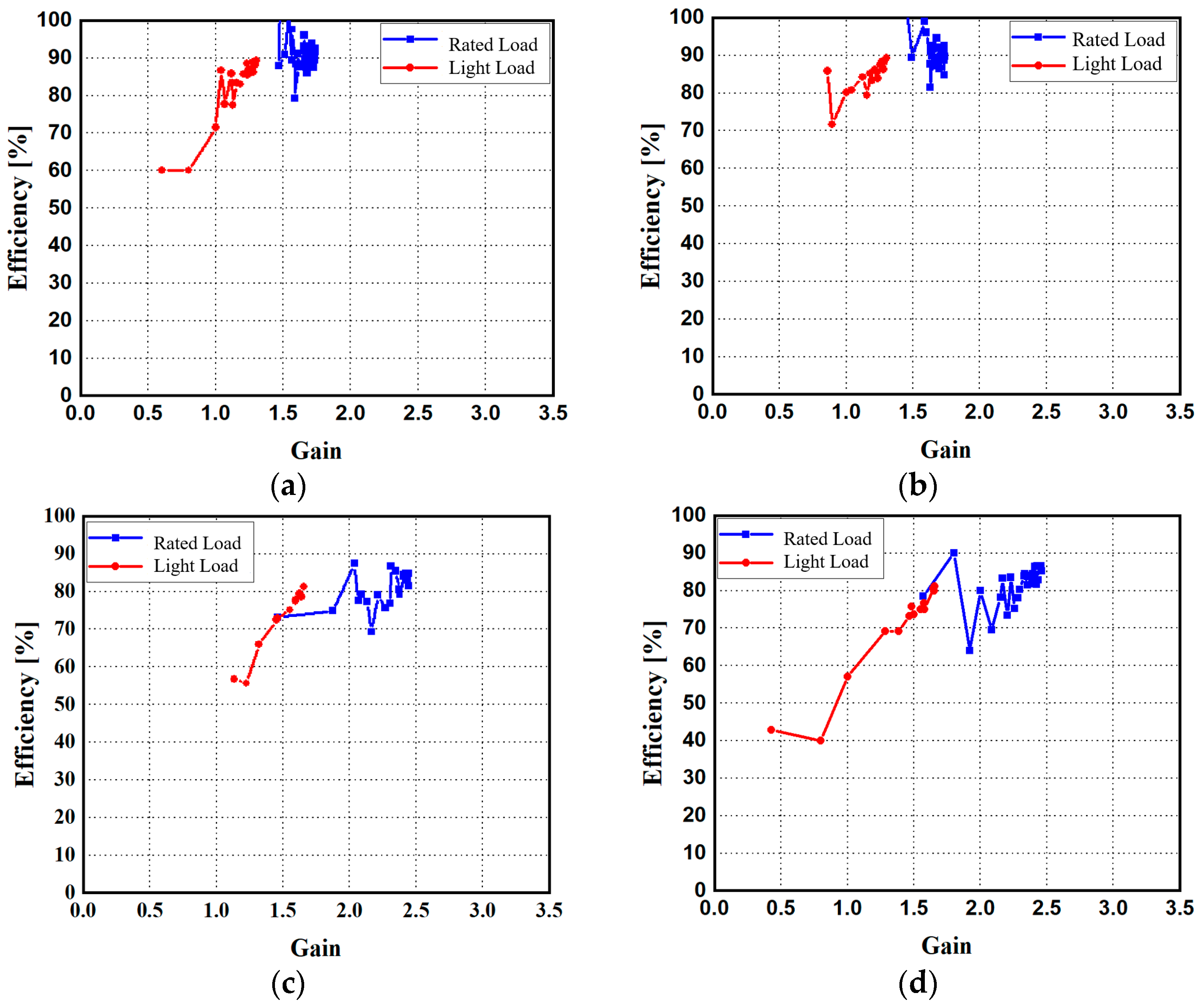
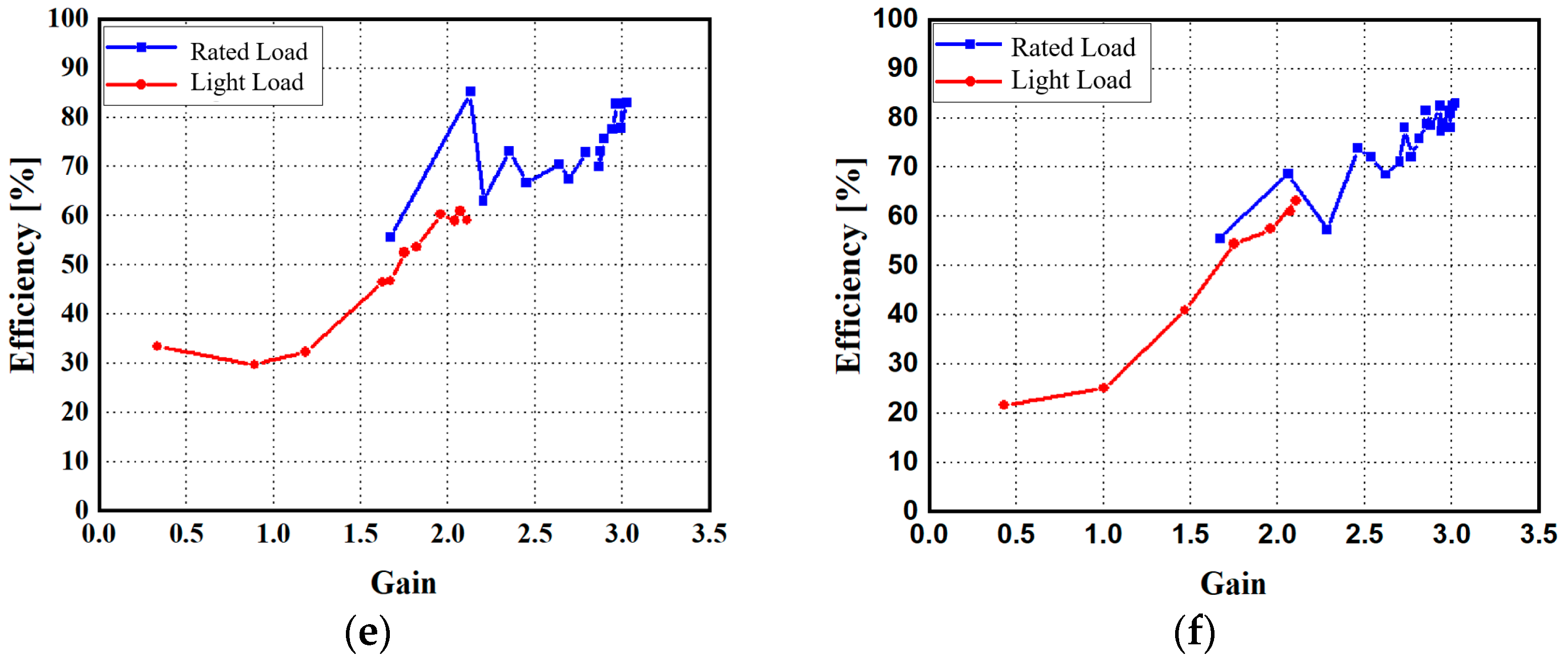
4.2. Efficiency Comparison and Analysis
5. Conclusions
- The converter ensures a constant output with a supercapacitor bank.
- The isolated boost LCC series-parallel resonant converter operates at high frequency and employs a frequency modulation technique.
- ZVS (Zero Voltage Switching) conditions for the inverter-side MOSFET can be achieved through resonance parameters.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Rani, J.R.; Thangavel, R.; Oh, S.-I.; Woo, J.M.; Chandra Das, N.; Kim, S.-Y.; Lee, Y.-S.; Jang, J.-H. High volumetric energy density hybrid supercapacitors based on reduced graphene oxide scrolls. ACS Appl. Mater. Interfaces 2017, 9, 22398–22407. [Google Scholar] [CrossRef]
- Liu, C.; Li, F.; Ma, L.P.; Cheng, H.M. Advanced materials for energy storage. Adv. Mater. 2010, 22, E28–E62. [Google Scholar] [CrossRef]
- Miller, J.R.; Simon, P. Electrochemical capacitors for energy management. Science 2008, 321, 651–652. [Google Scholar] [CrossRef] [PubMed]
- Simon, P.; Gogotsi, Y. Materials for electrochemical capacitors. In Nanoscience and Technology: A Collection of Reviews from Nature Journals; World Scientific: Singapore, 2010; pp. 320–329. [Google Scholar]
- Chidembo, A.; Aboutalebi, S.H.; Konstantinov, K.; Salari, M.; Winton, B.; Yamini, S.A.; Nevirkovets, I.P.; Liu, H.K. Globular reduced graphene oxide-metal oxide structures for energy storage applications. Energy Environ. Sci. 2012, 5, 5236–5240. [Google Scholar] [CrossRef]
- Cheng, X.; Li, T.; Ruan, X.; Wang, Z. Thermal runaway characteristics of a large format lithium-ion battery module. Energies 2019, 12, 3099. [Google Scholar] [CrossRef]
- Kaempgen, M.; Chan, C.K.; Ma, J.; Cui, Y.; Gruner, G. Printable thin film supercapacitors using single-walled carbon nanotubes. Nano Lett. 2009, 9, 1872–1876. [Google Scholar] [CrossRef]
- Chen, Z.; Wen, J.; Yan, C.; Rice, L.; Sohn, H.; Shen, M.; Cai, M.; Dunn, B.; Lu, Y. High-performance supercapacitors based on hierarchically porous graphite particles. Adv. Energy Mater. 2011, 1, 551–556. [Google Scholar] [CrossRef]
- Chen, M.; Sun, Q.; Li, Y.; Wu, K.; Liu, B.; Peng, P.; Wang, Q. A thermal runaway simulation on a lithium titanate battery and the battery module. Energies 2015, 8, 490–500. [Google Scholar] [CrossRef]
- Tian, X.; Yi, Y.; Fang, B.; Yang, P.; Wang, T.; Liu, P.; Qu, L.; Li, M.; Zhang, S. Design strategies of safe electrolytes for preventing thermal runaway in lithium ion batteries. Chem. Mater. 2020, 32, 9821–9848. [Google Scholar] [CrossRef]
- Liu, X.; Zhou, Z.; Wu, W.; Gao, L.; Li, Y.; Huang, H.; Huang, Z.; Li, Y.; Song, Y. Three-dimensional modeling for the internal shorting caused thermal runaway process in 20AH lithium-ion battery. Energies 2022, 15, 6868. [Google Scholar] [CrossRef]
- Qian, F.; Wang, H.; Li, M.; Li, C.; Shen, H.; Wang, J.; Li, Y.; Ouyang, M. Thermal Runaway vent gases from high-capacity energy storage LiFePO4 lithium iron. Energies 2023, 16, 3485. [Google Scholar] [CrossRef]
- Wang, D.; Zheng, L.; Li, X.; Du, G.; Zhang, Z.; Feng, Y.; Jia, L.; Dai, Z. Effects of overdischarge rate on thermal runaway of NCM811 Li-ion batteries. Energies 2020, 13, 3885. [Google Scholar] [CrossRef]
- Ghiji, M.; Novozhilov, V.; Moinuddin, K.; Joseph, P.; Burch, I.; Suendermann, B.; Gamble, G. A review of lithium-ion battery fire suppression. Energies 2020, 13, 5117. [Google Scholar] [CrossRef]
- Ehsani, M.; Gao, Y.; Miller, J.M. Hybrid electric vehicles: Architecture and motor drives. Proc. IEEE 2007, 95, 719–728. [Google Scholar] [CrossRef]
- Tan, N.M.; Inoue, S.; Kobayashi, A.; Akagi, H. Voltage balancing of a 320-V, 12-F Electric Double-Layer Capacitor bank combined with a 10-kW bidirectional isolated DC--DC converter. IEEE Trans. Power Electron. 2008, 23, 2755–2765. [Google Scholar] [CrossRef]
- Ali, A.; Shakoor, R.; Raheem, A.; Muqeet, H.A.U.; Awais, Q.; Khan, A.A.; Jamil, M. Latest energy storage trends in multi-energy standalone electric vehicle charging stations: A comprehensive study. Energies 2022, 15, 4727. [Google Scholar] [CrossRef]
- Ibanez, F.M. Analyzing the need for a balancing system in supercapacitor energy storage systems. IEEE Trans. Power Electron. 2017, 33, 2162–2171. [Google Scholar] [CrossRef]
- Forouzandeh, P.; Kumaravel, V.; Pillai, S.C. Electrode materials for supercapacitors: A review of recent advances. Catalysts 2020, 10, 969. [Google Scholar] [CrossRef]
- Atmaja, T.D.; Mirdanies, M. Electric vehicle mobile charging station dispatch algorithm. Energy Procedia 2015, 68, 326–335. [Google Scholar] [CrossRef]
- Cui, S.; Zhao, H.; Chen, H.; Zhang, C. The mobile charging vehicle routing problem with time windows and recharging services. Comput. Intell. Neurosci. 2018, 2018, 5075916. [Google Scholar] [CrossRef]
- Afshar, S.; Macedo, P.; Mohamed, F.; Disfani, V. A literature review on mobile charging station technology for electric vehicles. In Proceedings of the 2020 IEEE Transportation Electrification Conference & Expo (ITEC), Chicago, IL, USA, 23–26 June 2020; pp. 1184–1190. [Google Scholar]
- Ye, B.; Jiang, J.; Miao, L.; Yang, P.; Li, J.; Shen, B. Feasibility study of a solar-powered electric vehicle charging station model. Energies 2015, 8, 13265–13283. [Google Scholar] [CrossRef]
- Saboori, H.; Jadid, S.; Savaghebi, M. Optimal management of mobile battery energy storage as a self-driving, self-powered and movable charging station to promote electric vehicle adoption. Energies 2021, 14, 736. [Google Scholar] [CrossRef]
- Gomez Vidales, A.; Sridhar, D.; Meunier, J.-L.; Omanovic, S. Nickel oxide on directly grown carbon nanofibers for energy storage applications. J. Appl. Electrochem. 2020, 50, 1217–1229. [Google Scholar] [CrossRef]
- Gomez Vidales, A.; Kim, J.; Omanovic, S. Ni0.6−xMo0.4−xIrx-oxide as an electrode material for supercapacitors: Investigation of the influence of iridium content on the charge storage/delivery. J. Solid State Electrochem. 2019, 23, 2129–2139. [Google Scholar] [CrossRef]
- Pernía, A.M.; Prieto, M.J.; Villegas, P.J.; Díaz, J.; Martín-Ramos, J.A. LCC resonant multilevel converter for X-ray applications. Energies 2017, 10, 1573. [Google Scholar] [CrossRef]
- Martín-Ramos, J.A.; Sáiz, P.J.V.; Pernía, A.M.; Díaz, J.; Martínez, J.A. Optimal control of a high-voltage power supply based on the PRC-LCC topology with a capacitor as output filter. IEEE Trans. Ind. Appl. 2013, 49, 2323–2329. [Google Scholar] [CrossRef]
- Ivensky, G.; Kats, A.; Ben-Yaakov, S. An RC load model of parallel and series-parallel resonant DC-DC converters with capacitive output filter. IEEE Trans. Power Electron. 1999, 14, 515–521. [Google Scholar] [CrossRef]
- Diaz, J.; Saiz, P.J.V.; Martin-Ramos, J.A.; Martin-Pernia, A.; Martinez, J.A. A high-voltage AC/DC resonant converter based on PRC with single capacitor as an output filter. IEEE Trans. Ind. Appl. 2010, 46, 2134–2142. [Google Scholar] [CrossRef]
- Kobaku, T.; Jeyasenthil, R.; Sahoo, S.; Ramchand, R.; Dragicevic, T. Quantitative feedback design-based robust PID control of voltage mode controlled DC-DC boost converter. IEEE Trans. Circuits Syst. II Express Briefs 2020, 68, 286–290. [Google Scholar] [CrossRef]
- Merai, M.; Naouar, M.W.; Slama-Belkhodja, I. An improved DC-link voltage control strategy for grid connected converters. IEEE Trans. Power Electron. 2017, 33, 3575–3582. [Google Scholar] [CrossRef]
- Wang, L.; Wu, Q.; Tao, Y.; Tang, W. Switching control of buck converter based on energy conservation principle. IEEE Trans. Control Syst. Technol. 2016, 24, 1779–1787. [Google Scholar] [CrossRef]
- Baek, J.-E.; Rhee, J.-H.; Ko, K.-C. Hybrid analog and digital control of a high current converter based on an EDLC Bank for rapidly decreasing input voltage. IEEE Trans. Plasma Sci. 2018, 47, 542–548. [Google Scholar] [CrossRef]
- Zhang, L.; Li, H.; Guo, Q.; Xie, S.; Yang, Y. Research on constant voltage/current output of LCC–S envelope modulation wireless power transfer system. Energies 2022, 15, 1562. [Google Scholar] [CrossRef]
- Yang, J.; Zhang, X.; Zhang, K.; Cui, X.; Jiao, C.; Yang, X. Design of LCC-S compensation topology and optimization of misalignment tolerance for inductive power transfer. IEEE Access 2020, 8, 191309–191318. [Google Scholar] [CrossRef]
- Feng, X.; Ouyang, M.; Liu, X.; Lu, L.; Xia, Y.; He, X. Thermal runaway mechanism of lithium ion battery for electric vehicles: A review. Energy Storage Mater. 2018, 10, 246–267. [Google Scholar] [CrossRef]
- Ruiz, V.; Pfrang, A.; Kriston, A.; Omar, N.; Van den Bossche, P.; Boon-Brett, L. A review of international abuse testing standards and regulations for lithium ion batteries in electric and hybrid electric vehicles. Renew. Sustain. Energy Rev. 2018, 81, 1427–1452. [Google Scholar] [CrossRef]
- Wang, Q.; Ping, P.; Zhao, X.; Chu, G.; Sun, J.; Chen, C. Thermal runaway caused fire and explosion of lithium ion battery. J. Power Source 2012, 208, 210–224. [Google Scholar] [CrossRef]
- Steigerwald, R.L. A comparison of half-bridge resonant converter topologies. IEEE Trans. Power Electron. 1988, 3, 174–182. [Google Scholar] [CrossRef]
- Biela, J.; Kolar, J.W. Using transformer parasitics for resonant converters-a review of the calculation of the stray capacitance of transformers. In Proceedings of the Fourtieth IAS Annual Meeting. Conference Record of the 2005 Industry Applications Conference, Hong Kong, China, 2–6 October 2005; pp. 1868–1875. [Google Scholar]
- Kolahian, P.; Grimm, F.; Baghdadi, M. A comprehensive review on planar magnetics and the structures to reduce the parasitic elements and improve efficiency. Energies 2023, 16, 3254. [Google Scholar] [CrossRef]
- Li, H.; Zhang, Z.; Wang, S.; Tang, J.; Ren, X.; Chen, Q. A 300-kHz 6.6-kW SiC bidirectional LLC onboard charger. IEEE Trans. Ind. Electron. 2019, 67, 1435–1445. [Google Scholar] [CrossRef]
- Kundu, U.; Yenduri, K.; Sensarma, P. Accurate ZVS analysis for magnetic design and efficiency improvement of full-bridge LLC resonant converter. IEEE Trans. Power Electron. 2016, 32, 1703–1706. [Google Scholar] [CrossRef]




| Devices | Cell Voltage (V) | Energy Density (Wh/kg) | Power Density (Wh/kg) | Cycle Life (Times) |
|---|---|---|---|---|
| SC | 2.5/2.7 | 2–30 | 4–10 | More than 1,000,000 |
| Lead Acid | 2 | 30–40 | 0.18 | No more than 800 |
| Li-Ion | 3.6 | 80–170 | 0.8–2 | No more than 1200 |
| Li-Polymer | 3.7 | 130/200 | 1–2.8 | No more than 1000 |
| LCC Resonant Network with High-Frequency Transformer | |||||
|---|---|---|---|---|---|
| LS | CS | CP | LL | CW | Fmin |
| 11 uH | 2 uF | 1 uF | 1.8 uH | 200 pF | 50 kHz |
| Components | Specifications |
|---|---|
| EDLC | DH5U308W60138 |
| IGBT | 1MBH 75D-060 |
| Driver | VLA517-01R |
| Rcharge Lfilter | 10 Ω |
| 50 μH | |
| Rectifier Diode | DL212-25N16 |
| HF Diode | VS40HFLR60S02 |
| Components | Specifications |
|---|---|
| Primary boost circuit | |
| IGBT | SKM200GM12T4 |
| Driver | VLA517-01R |
| Diode | DA2F100P12S |
| Lboosting | 110 μH |
| Coutput | 650 μF |
| Secondary boost circuit | |
| MOSFET | G3R20MT12N |
| Driver | BM60212FV-C |
| DSP | TMS320F28335 |
| Signal Isolator | MAX14937 |
| Rectifier Diode | MFQ150U6NH5 |
| LF | 305 μH |
| CF | 650 μH |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kang, H.-W.; Lee, H.-S.; Rhee, J.-H.; Lee, K.-A. DC Voltage Source Based on a Battery of Supercapacitors with a Regulator in the Form of an Isolated Boost LCC Resonant Converter. Energies 2023, 16, 6721. https://doi.org/10.3390/en16186721
Kang H-W, Lee H-S, Rhee J-H, Lee K-A. DC Voltage Source Based on a Battery of Supercapacitors with a Regulator in the Form of an Isolated Boost LCC Resonant Converter. Energies. 2023; 16(18):6721. https://doi.org/10.3390/en16186721
Chicago/Turabian StyleKang, Hyung-Wook, Hyun-Seong Lee, Jae-Ho Rhee, and Kun-A Lee. 2023. "DC Voltage Source Based on a Battery of Supercapacitors with a Regulator in the Form of an Isolated Boost LCC Resonant Converter" Energies 16, no. 18: 6721. https://doi.org/10.3390/en16186721
APA StyleKang, H.-W., Lee, H.-S., Rhee, J.-H., & Lee, K.-A. (2023). DC Voltage Source Based on a Battery of Supercapacitors with a Regulator in the Form of an Isolated Boost LCC Resonant Converter. Energies, 16(18), 6721. https://doi.org/10.3390/en16186721






