A Test Method for Shielding Effectiveness of Materials against Electromagnetic Pulse Based on Coaxial Flange
Abstract
1. Introduction
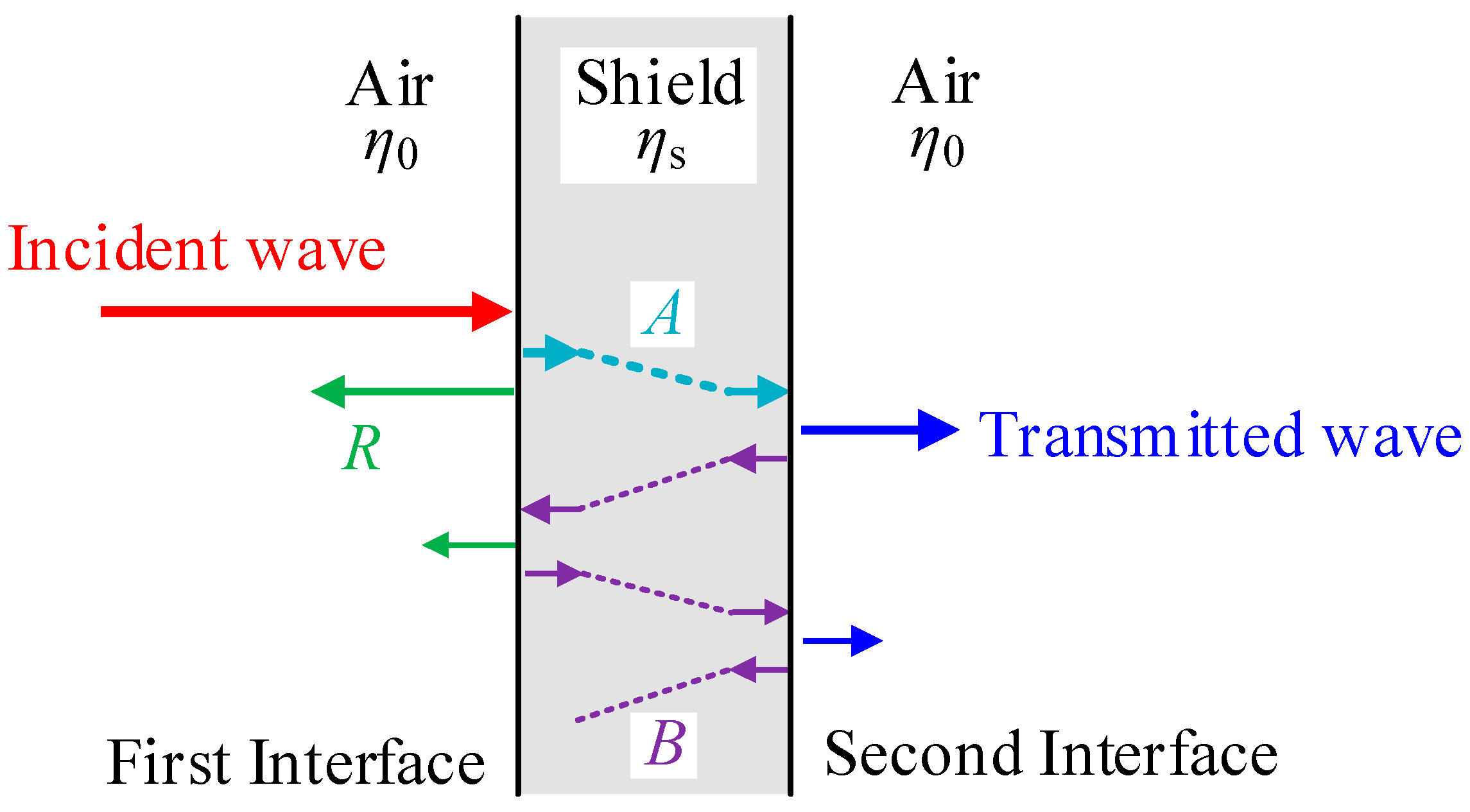
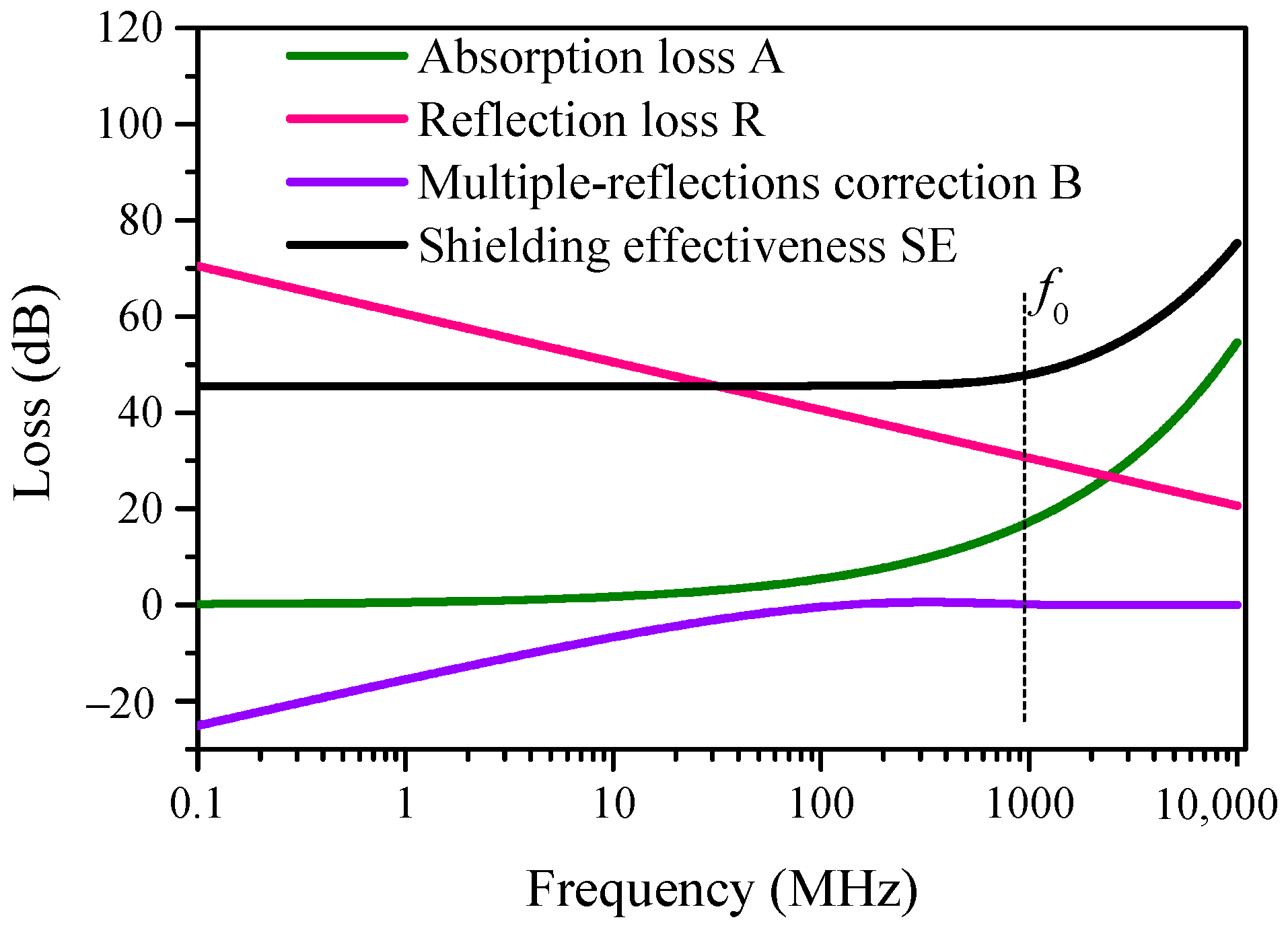
2. Basic Shielding Theory
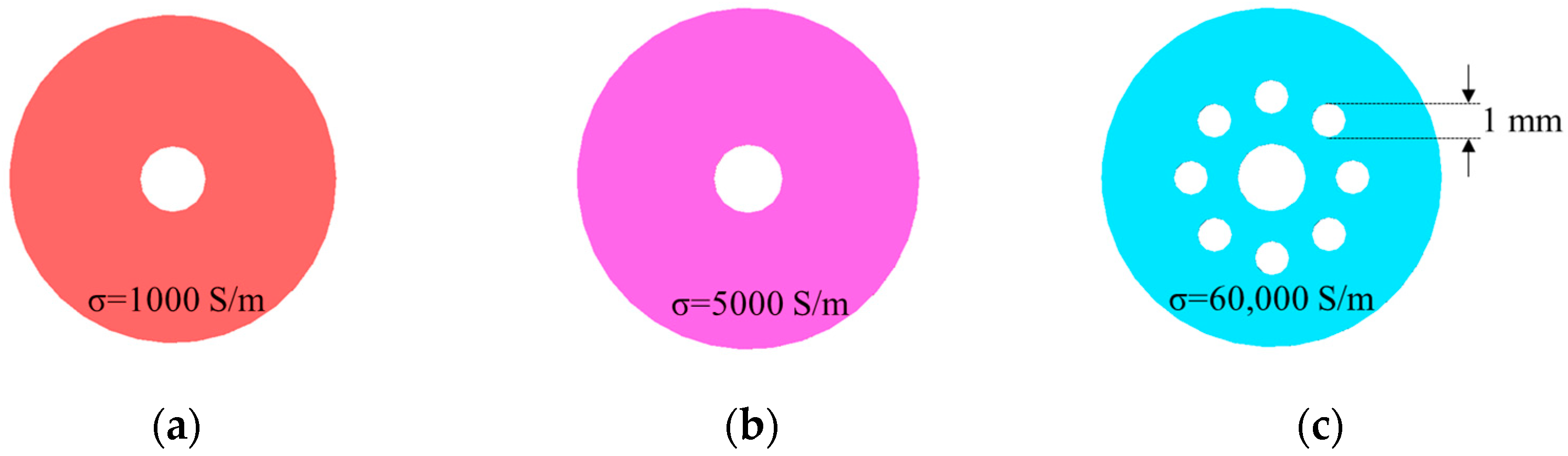
3. Time Domain SE Simulation
- Peak value reduction SE
- Energy density reduction SE
4. Time Domain SE Test
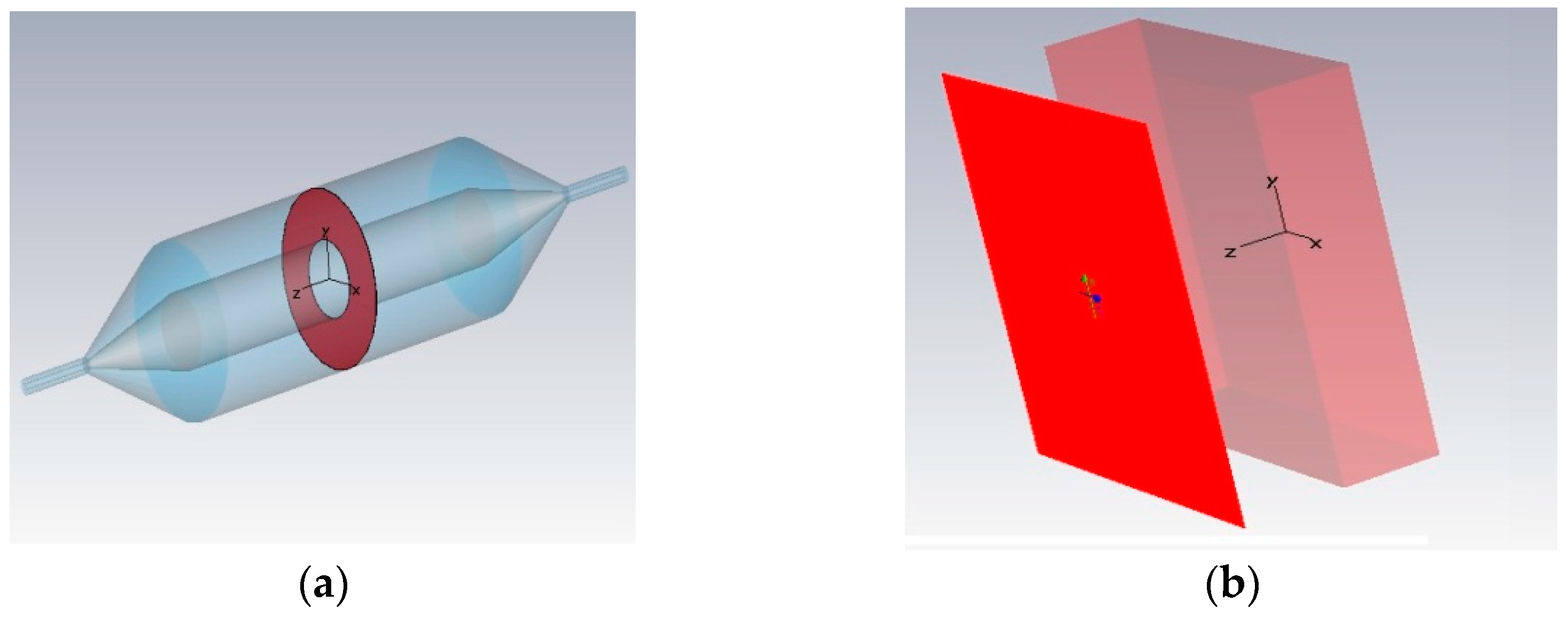
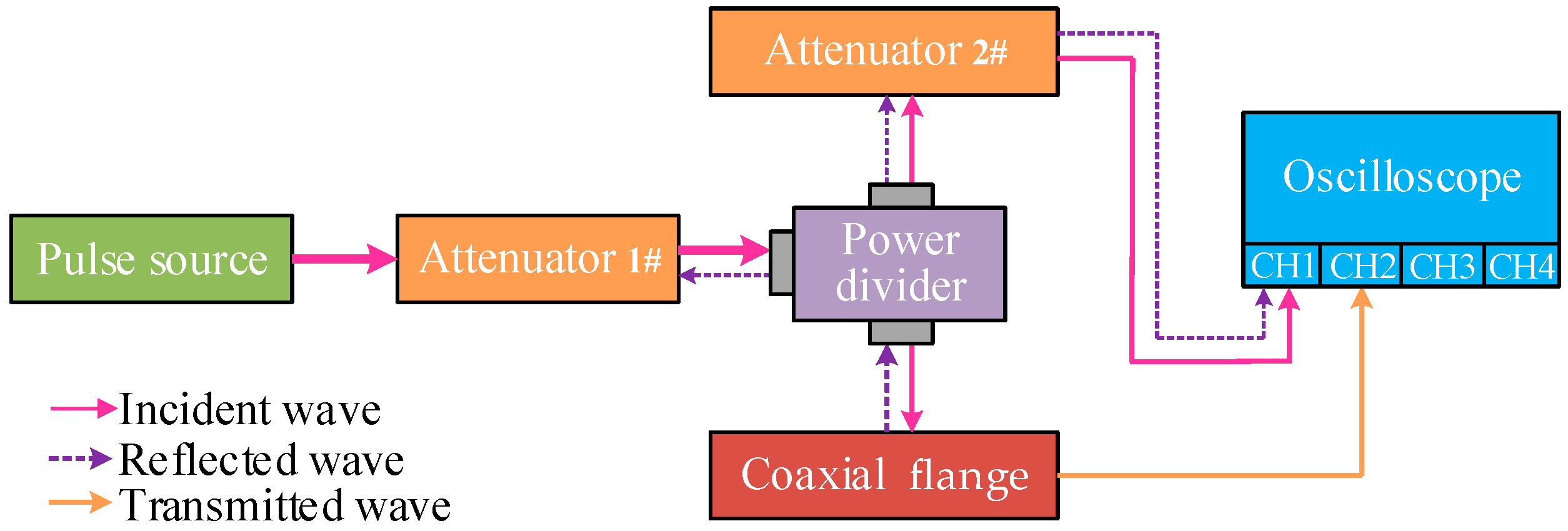
4.1. Test Method
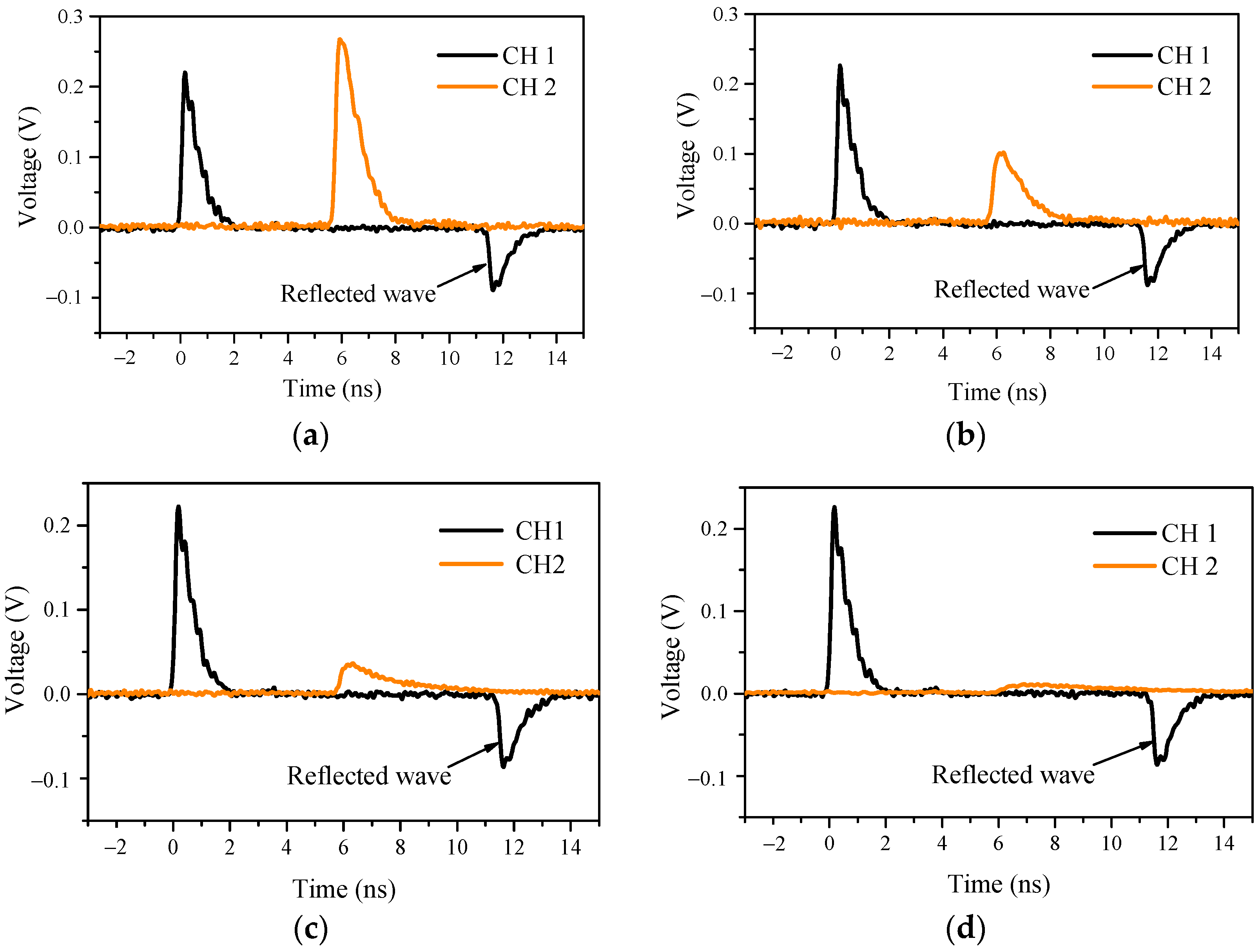
4.2. Experimental Test
4.3. Result Analysis
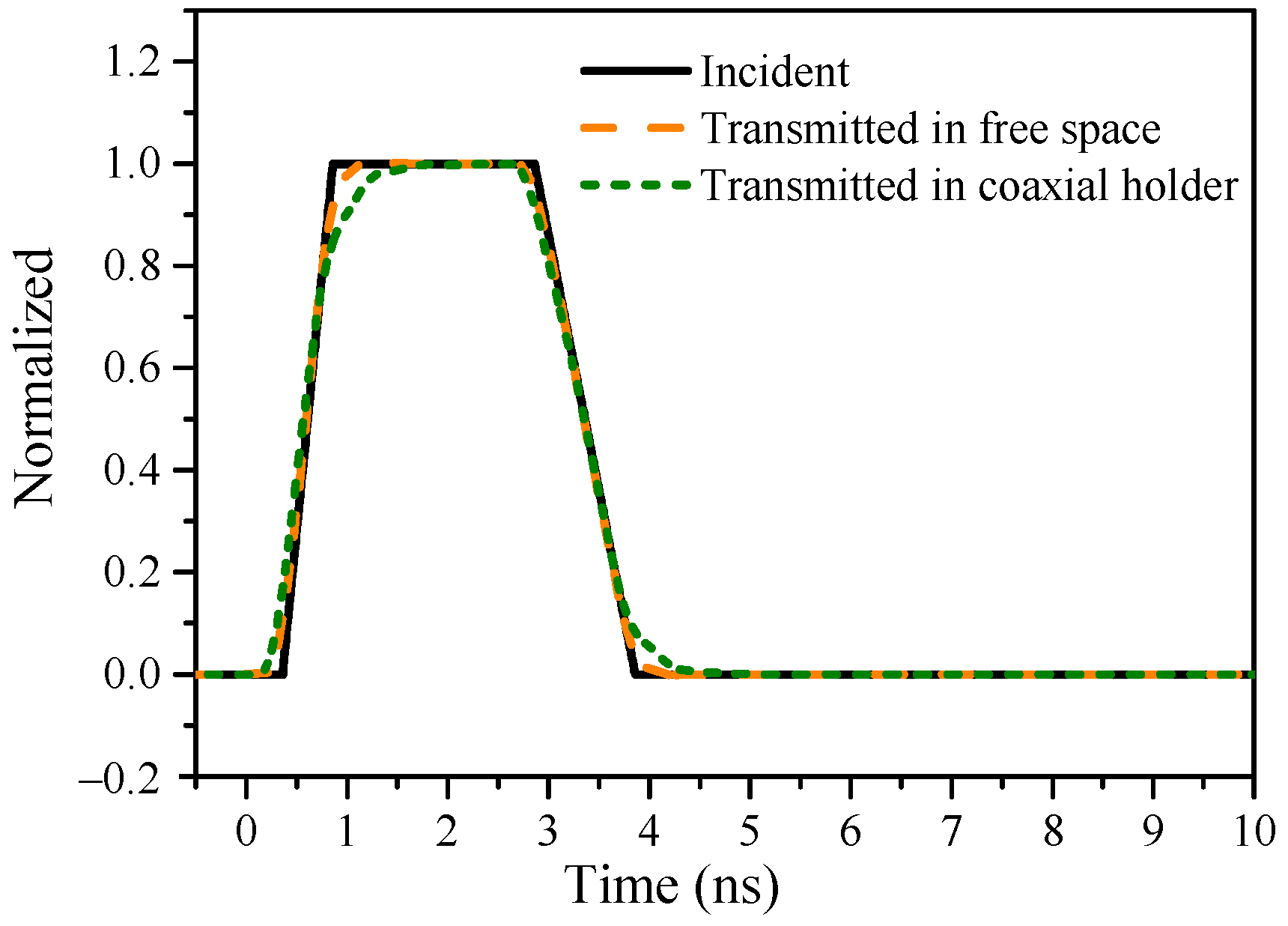
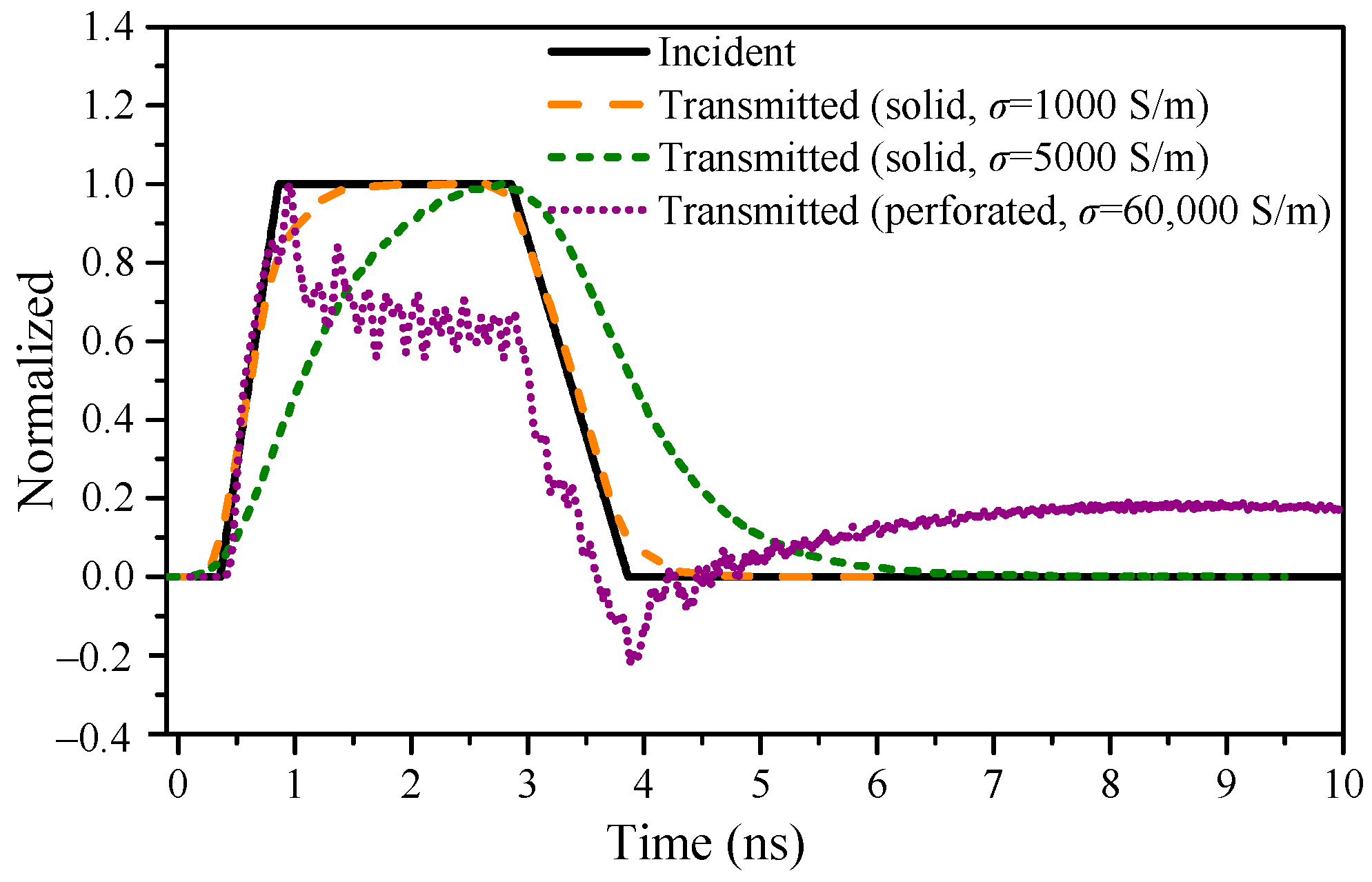
4.3.1. Waveform Characteristics
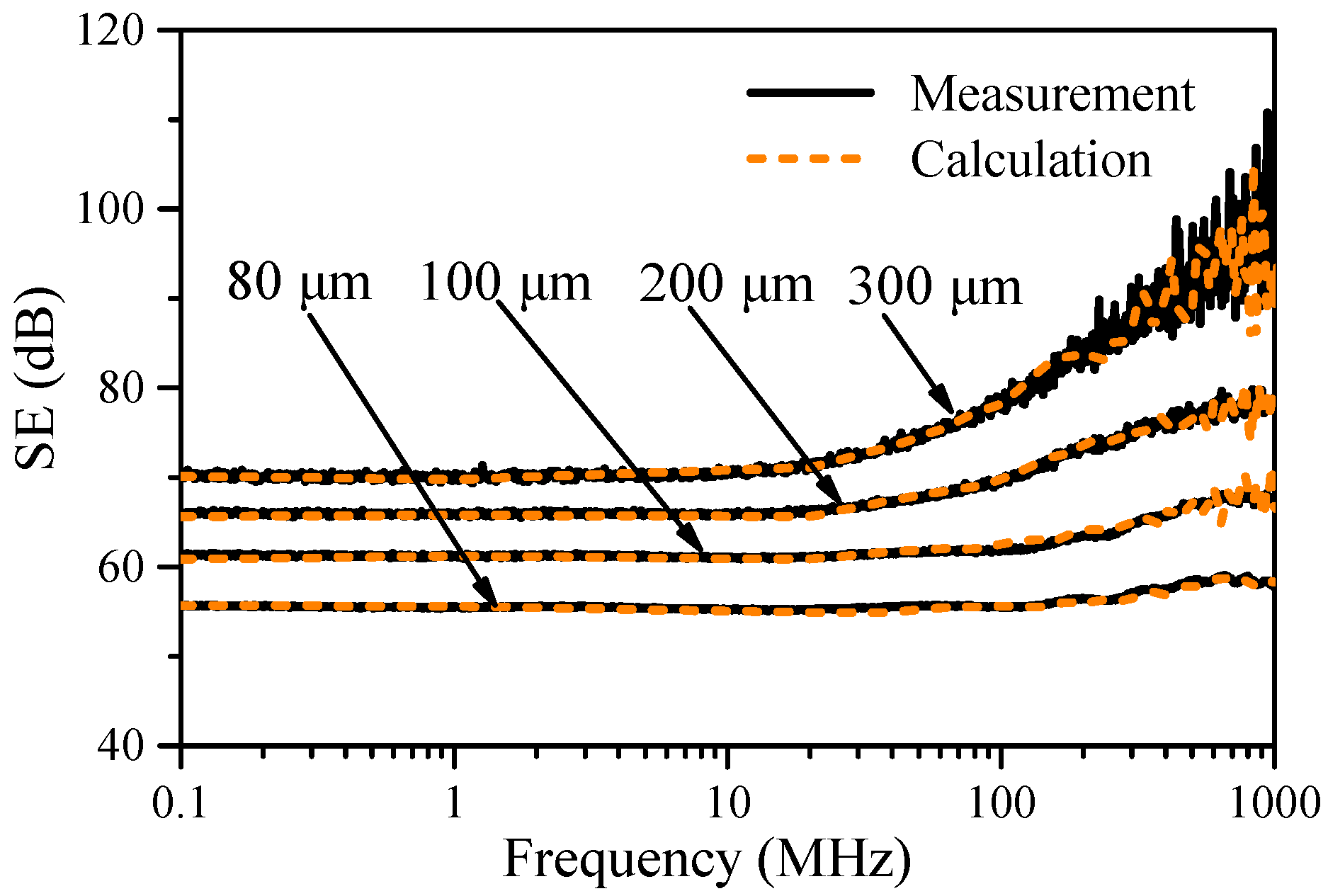
4.3.2. SE Calculation
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhou, B.H.; Shi, L.H.; Wang, J.B. Electromagnetic Pulse and Its Engineering Protection, 2nd ed.; National Defense Industry Press: Beijing, China, 2019; pp. 1–7. [Google Scholar]
- Du, C.; Cui, Z.; Mao, C. Risk Analysis of HPEM threats for linear RF channel with pyramid horn antenna based on system-Level SPICE modelling. Energies 2022, 15, 6142. [Google Scholar] [CrossRef]
- Du, C.; Liu, Z.; Mao, C.; Cui, Z.; Sun, D.; Qin, F. Statistical snalysis comparison of HEMP transient response of monopole antenna from facility and burst views. J. Electromagn. Waves Appl. 2021, 35, 1663–1677. [Google Scholar] [CrossRef]
- Ryu, J.; Lee, J. An integrated antenna-source system of very high ultra wide-band gain for radiating high-power wide-band pulses. IEEE Trans. Plasma Sci. 2012, 4, 1019–1026. [Google Scholar] [CrossRef]
- Kim, K.; Min, K.; Park, Y. A Pilot Experiment to develop a lightweight non-nuclear EMP shelter applying civil-military cooperation in a sustainability policy. Sustainability 2020, 24, 10669. [Google Scholar] [CrossRef]
- Deng, J.Q.; Hao, C. Research on powerful electromagnetic pulse coupling and power source protection. J. Microw. 2017, 33, 85–89. [Google Scholar]
- Jiang, L.J.; Hao, J.H.; Gong, Y.F. Electromagnetic coupling to an apertured enclosure via a penetrated transmission line based on electromagnetic topology. J. Electromagn. Waves Appl. 2017, 32, 606–623. [Google Scholar] [CrossRef]
- Henry, W. Electromagnetic Compatibility Engineering; John Wiley & Sons: Hoboken, NJ, USA, 2009; pp. 238–260. [Google Scholar]
- Geetha, S.; Satheesh, K.; Rao, C. EMI shielding: Methods and materials-a review. J. Appl. Polym. Sci. 2009, 4, 2073–2086. [Google Scholar] [CrossRef]
- Munalli, D.; Dimitrakis, G.; Chronopoulos, D. Electromagnetic shielding effectiveness of carbon fibre reinforced composites. Compos. B 2019, 173, 106906. [Google Scholar] [CrossRef]
- Zhou, B.H.; Gao, C. The definition of EMP shielding effectiveness. In Proceedings of the Asia-Pacific Conference on Environmental Electromagnetics, Hangzhou, China, 4–7 November 2003. [Google Scholar]
- Wang, J.; Cui, Z.; Chen, Z. Machine learning based prediction for the response of gas discharge tube to damped sinusoid signal. Energies 2022, 15, 2622. [Google Scholar] [CrossRef]
- Singh, A.S. A review of porous lightweight composite materials for electromagnetic interference shielding. Compos. B 2018, 149, 188–197. [Google Scholar] [CrossRef]
- Tesche, F. On the use of the Hilbert transform for processing measured CW data. IEEE Trans. Electromagn. Compat. 1992, 3, 259–266. [Google Scholar] [CrossRef]
- Lu, Y.; Yan, M.; Gao, Y. Mechanism and development of electromagnetic shielding materials. Value Eng. 2019, 1, 159–162. [Google Scholar]
- Zhang, L.; Wei, G.; Hu, X. Shielding effectiveness testing method of pulse electric field based on GTEM cell. J. Phys. Conf. Ser. 2013, 418, 012080. [Google Scholar] [CrossRef]
- Herlemann, H.; Koch, M. Measurement of the transient shielding effectiveness of enclosures using UWB pulses inside an open TEM waveguide. Adv. Radio Sci. 2007, 5, 75–79. [Google Scholar] [CrossRef][Green Version]
- Cui, M.; Wei, M.; Chen, X. Testing of materials shielding effectiveness against electromagnetic pulse. In Proceedings of the Cross Strait Quad-Regional Radio Science and Wireless Technology Conference, Chengdu, China, 21–25 July 2013. [Google Scholar]
- Chen, X.; Chen, Y.; Wei, M. Time domain testing methods of material’s shielding effectiveness of electromagnetic pulse using flange coaxial. High Volt. Eng. 2012, 3, 594–660. [Google Scholar]
- Liu, Z.; Bai, G.; Huang, Y. Reflection and absorption contributions to the electromagnetic interference shielding of single-walled carbon nanotube/polyurethane composites. Carbon 2007, 45, 821. [Google Scholar] [CrossRef]
- Lameirinhas, R.A.M.; Torres, J.P.N.; Baptista, A. A new method to analyse the role of surface plasmon polaritons on dielectric-metal interfaces. IEEE Photonics J. 2022, 4, 2236409. [Google Scholar] [CrossRef]
- Lameirinhas, R.A.M.; Torres, J.P.N.; Baptista, A. A new method to determine the response of Kretschmann’s structure-based biosensors. IEEE Sens. J. 2022, 21, 20421–20429. [Google Scholar] [CrossRef]
- Weber, H. The Fresnel equations for lossy dielectrics and conservation of energy. J. Mod. Opt. 2014, 15, 1219–1224. [Google Scholar] [CrossRef]
- Schulz, R.B.; Plantz, V.C.; Brush, D.R. Shielding theory and practice. IEEE Trans. Electromagn. Compat. 1988, 3, 187–201. [Google Scholar] [CrossRef]
- Jia, X.; Li, Y.; Shen, B. Evaluation, fabrication and dynamic performance regulation of green EMI-shielding materials with low reflectivity: A review. Compos. B 2022, 233, 109652. [Google Scholar] [CrossRef]
- Jia, Z.L.; Zhang, H.H.; Ding, D.Z. Time-domain shielding effectiveness analysis based on DGTD method accelerated by local time-stepping and parallel techniques. IEEE Trans. Electromagn. Compat. 2023, 3, 900–911. [Google Scholar] [CrossRef]
- ASTM D4935-10; Standard Test Method for Measuring the Electromagnetic Shielding Effectiveness of Planar Materials. ASTM International: West Conshohocken, PA, USA, 2010.
- Sarto, M.S.; Tamburrano, A. Innovative test method for the shielding effectiveness measurement of conductive thin films in a wide frequency range. IEEE Trans. Electromagn. Compat. 2006, 48, 331–341. [Google Scholar] [CrossRef]
- Chen, X.; Chen, Y.; Wei, M. Broadband coaxial holder with continuous-conductor used for shielding effectiveness of materials against electromagnetic pulse. Electron. Lett. 2013, 8, 532–534. [Google Scholar] [CrossRef]
- Guo, J.; Ma, L.; Cheng, Y. Design of a sub-nanosecond solid-state pulse generator. Mod. Appl. Phys. 2019, 4, 040402. [Google Scholar]


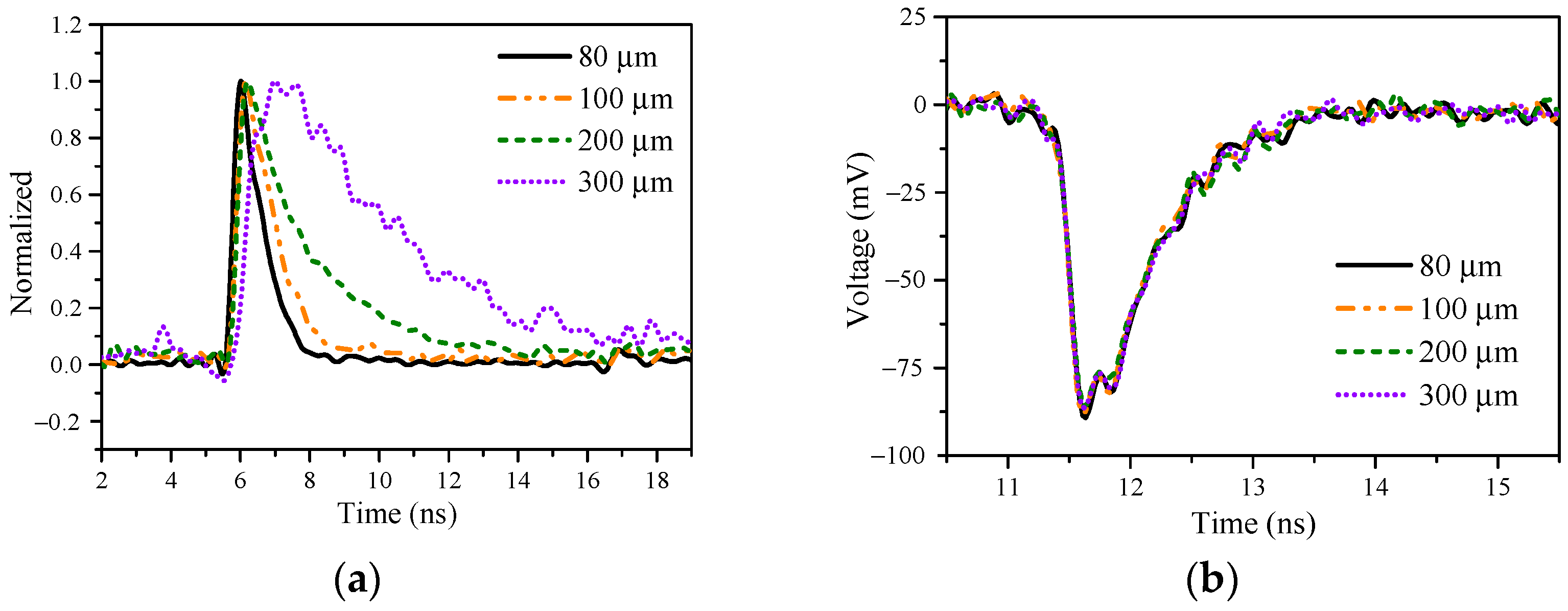

| 80 μm | 100 μm | 200 μm | 300 μm | |
|---|---|---|---|---|
| Rise time (ps) | 280 | 320 | 350 | 860 |
| Half-width (ps) | 890 | 1150 | 1620 | 3950 |
| 80 μm | 100 μm | 200 μm | 300 μm | |
|---|---|---|---|---|
| SEpeak (dB) | 58.3 | 67.0 | 75.7 | 85.9 |
| SEenergy (dB) | 54.5 | 60.5 | 65.0 | 67.3 |
| Δp-e (dB) | 3.8 | 6.5 | 10.7 | 18.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Wu, W.; Chen, X.; Nie, X.; Zhao, M.; Jia, R.; Li, J. A Test Method for Shielding Effectiveness of Materials against Electromagnetic Pulse Based on Coaxial Flange. Energies 2023, 16, 6701. https://doi.org/10.3390/en16186701
Liu Y, Wu W, Chen X, Nie X, Zhao M, Jia R, Li J. A Test Method for Shielding Effectiveness of Materials against Electromagnetic Pulse Based on Coaxial Flange. Energies. 2023; 16(18):6701. https://doi.org/10.3390/en16186701
Chicago/Turabian StyleLiu, Yifei, Wei Wu, Xiang Chen, Xin Nie, Mo Zhao, Rui Jia, and Jinxi Li. 2023. "A Test Method for Shielding Effectiveness of Materials against Electromagnetic Pulse Based on Coaxial Flange" Energies 16, no. 18: 6701. https://doi.org/10.3390/en16186701
APA StyleLiu, Y., Wu, W., Chen, X., Nie, X., Zhao, M., Jia, R., & Li, J. (2023). A Test Method for Shielding Effectiveness of Materials against Electromagnetic Pulse Based on Coaxial Flange. Energies, 16(18), 6701. https://doi.org/10.3390/en16186701





