High-Temperature Heat Pump Using CO2-Based Mixture for Simultaneous Heat and Cold Energy Reservation
Abstract
:1. Introduction
2. Methodology
2.1. System Description
2.2. Thermodynamic Model
2.3. Assumptions
- (1)
- Each component is considered to be steady-state and steady-flow.
- (2)
- Pressure losses and heat losses in all heat exchangers and pipelines are neglected.
- (3)
- Overall composition of the mixture in each component keeps constant.
- (4)
- Only counter-flow heat exchangers are used in the system.
- (5)
- The environmental temperature is equal to the inlet temperature of the TES.
- (6)
- The leakage of working fluid from the components are negligible.
- (7)
- The evaporating temperature for mixtures refers to dew point temperature.
3. Results and Discussion
3.1. Parametric Influence Analysis of Cycle
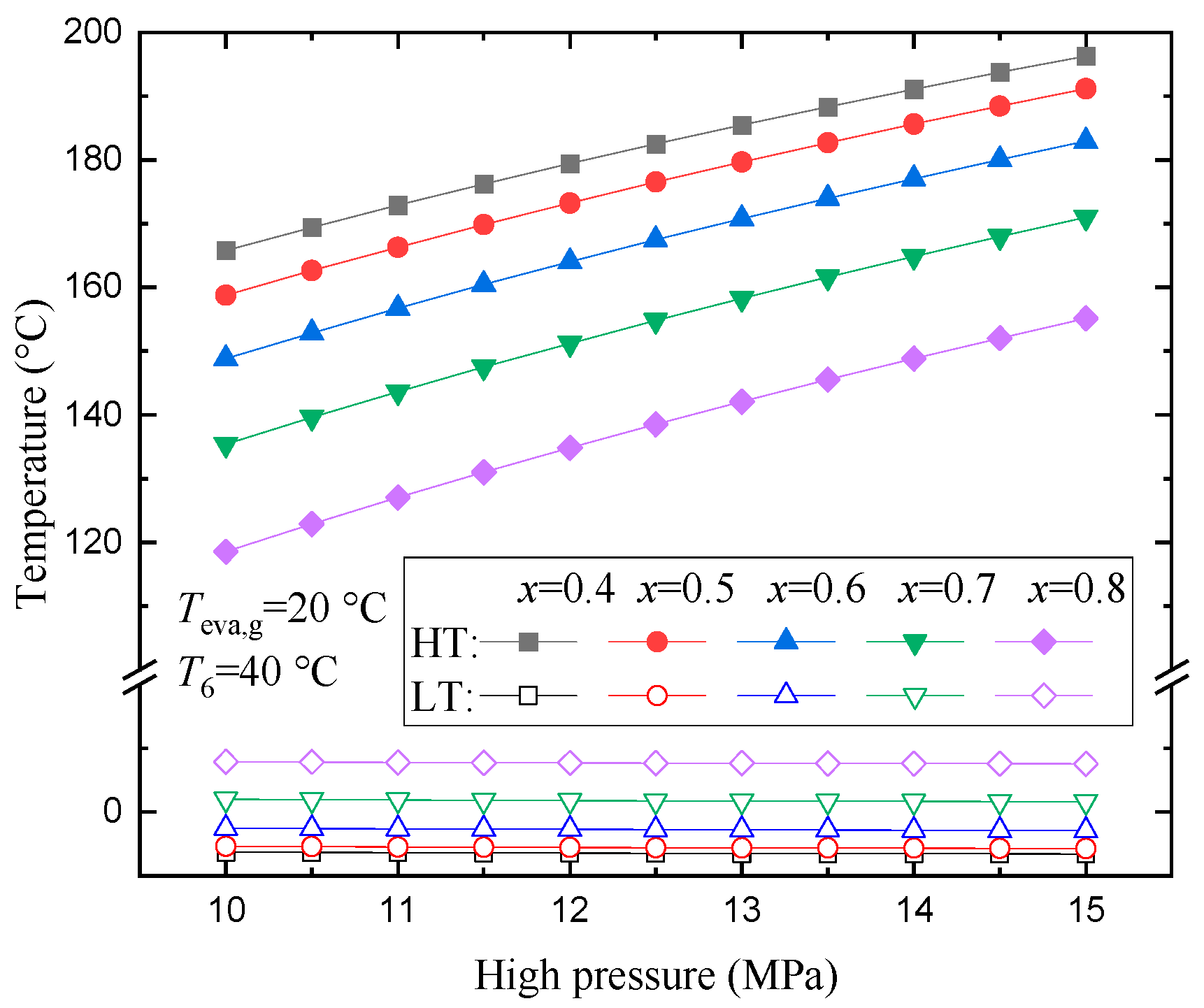
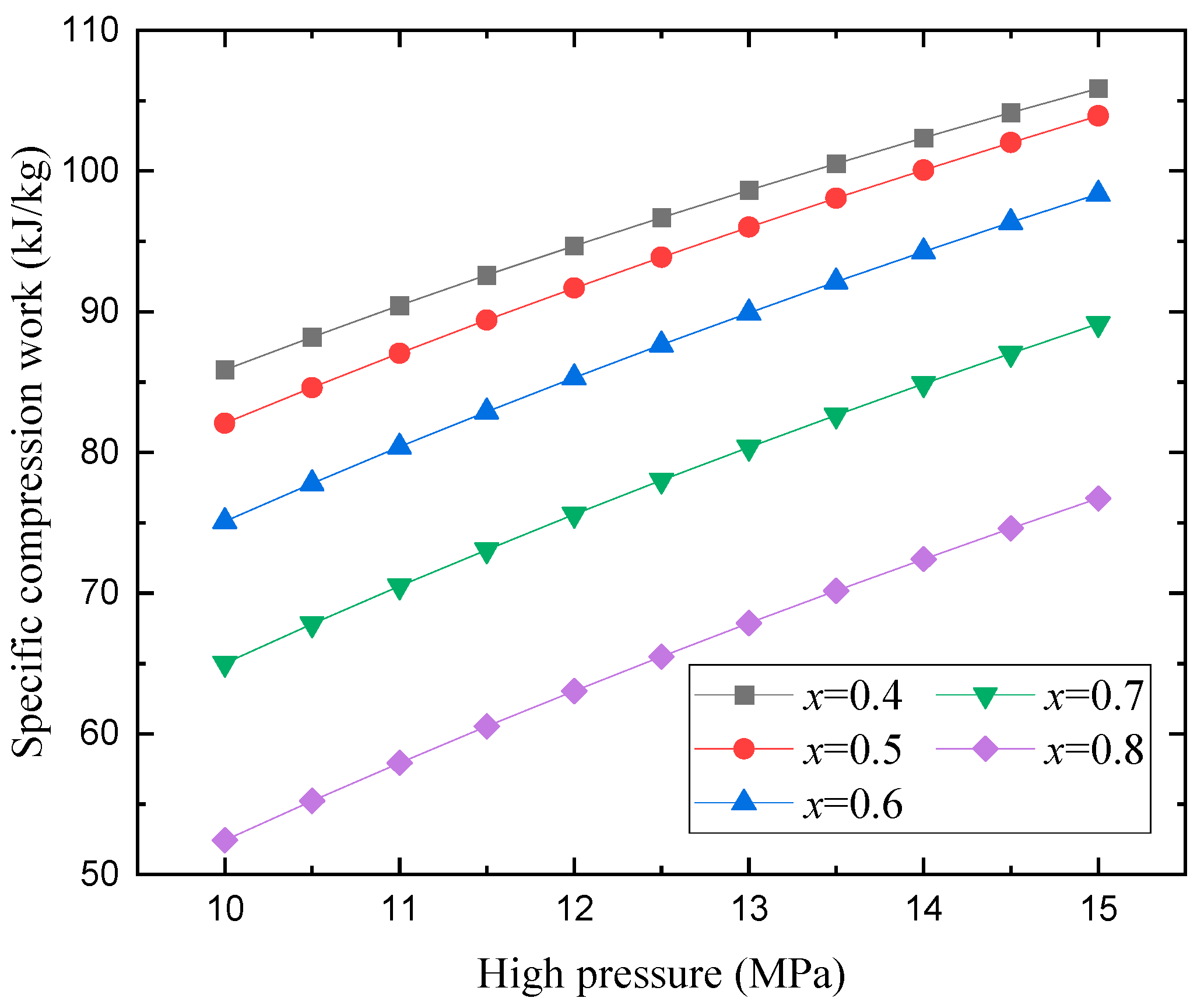
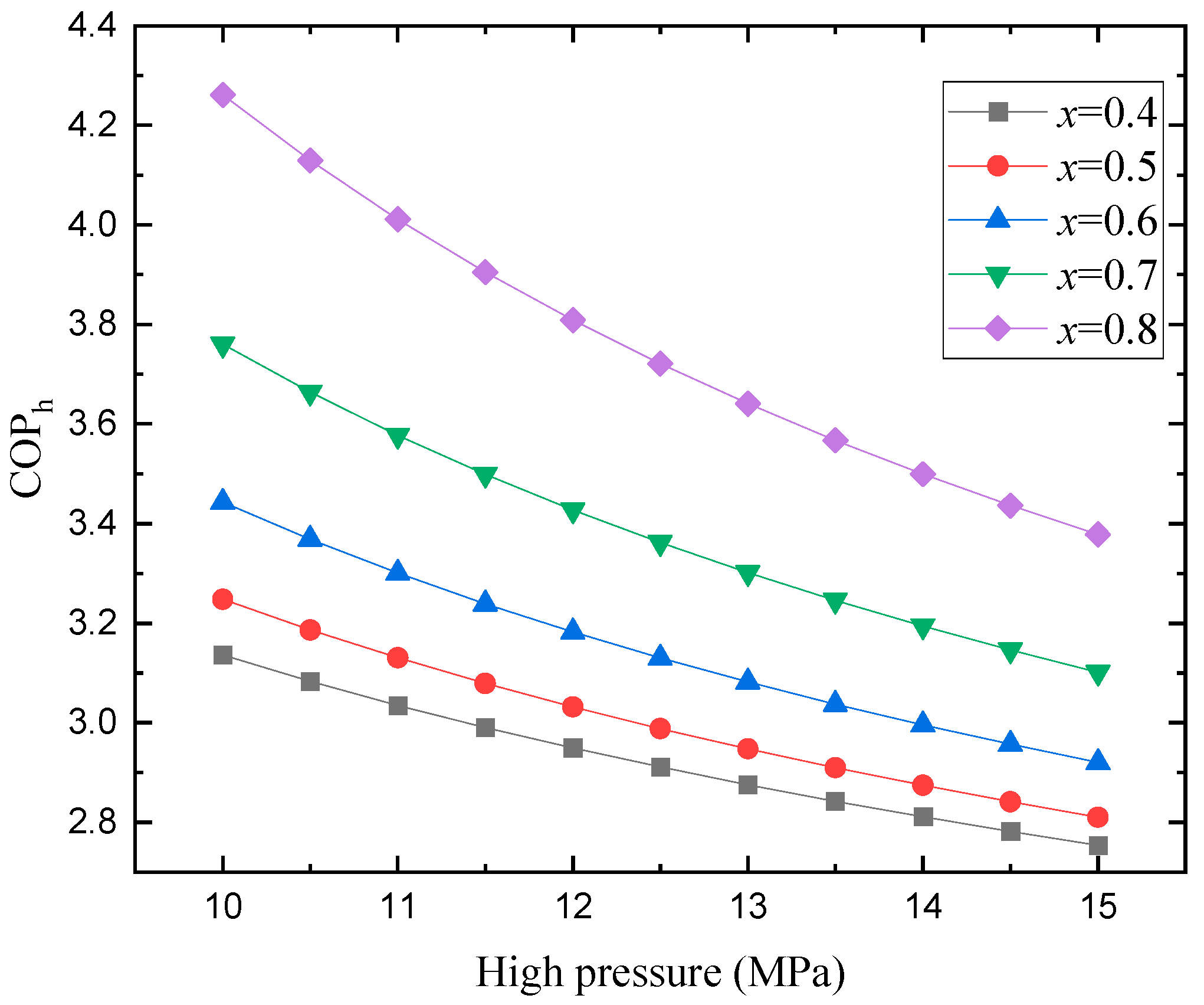
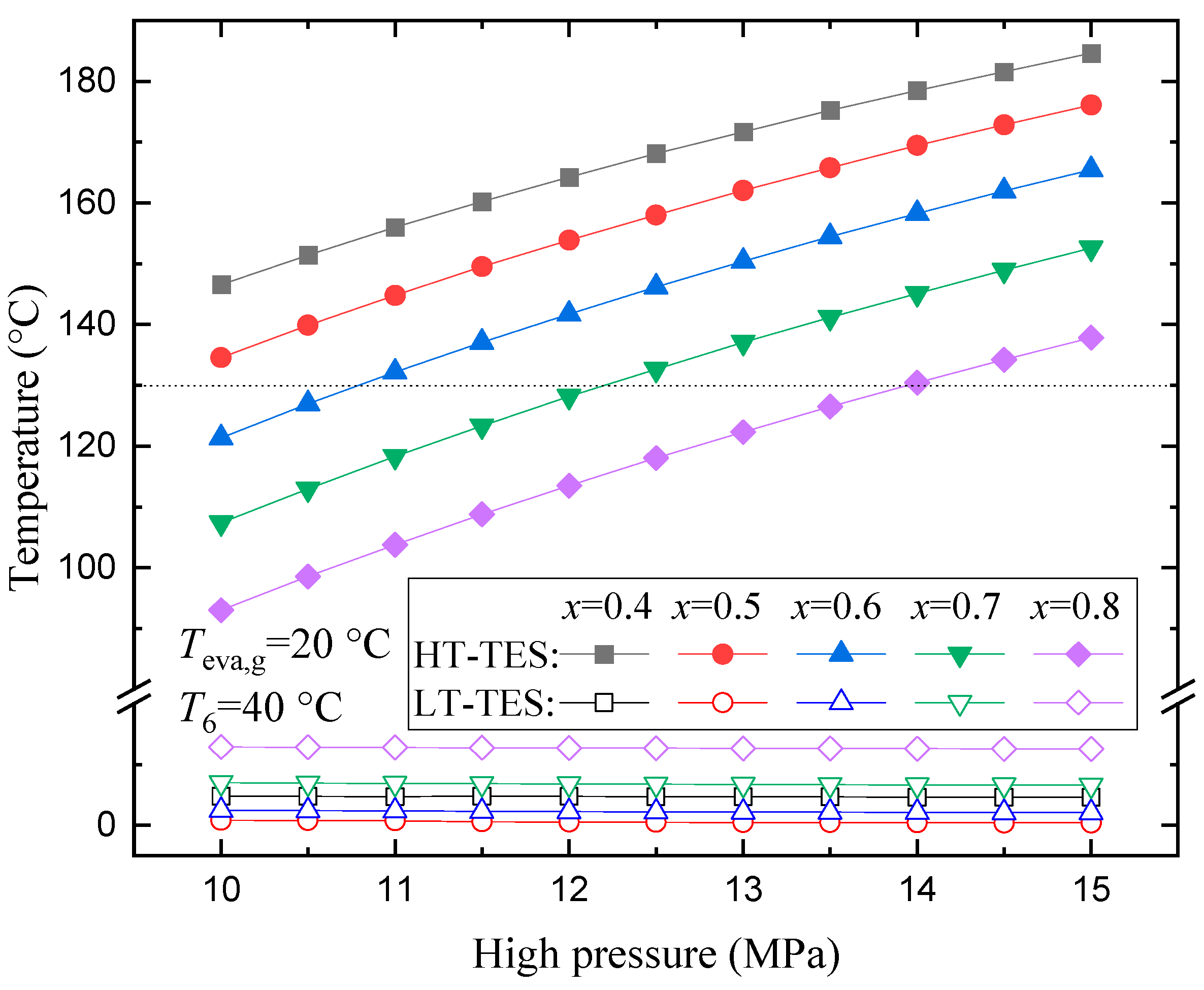
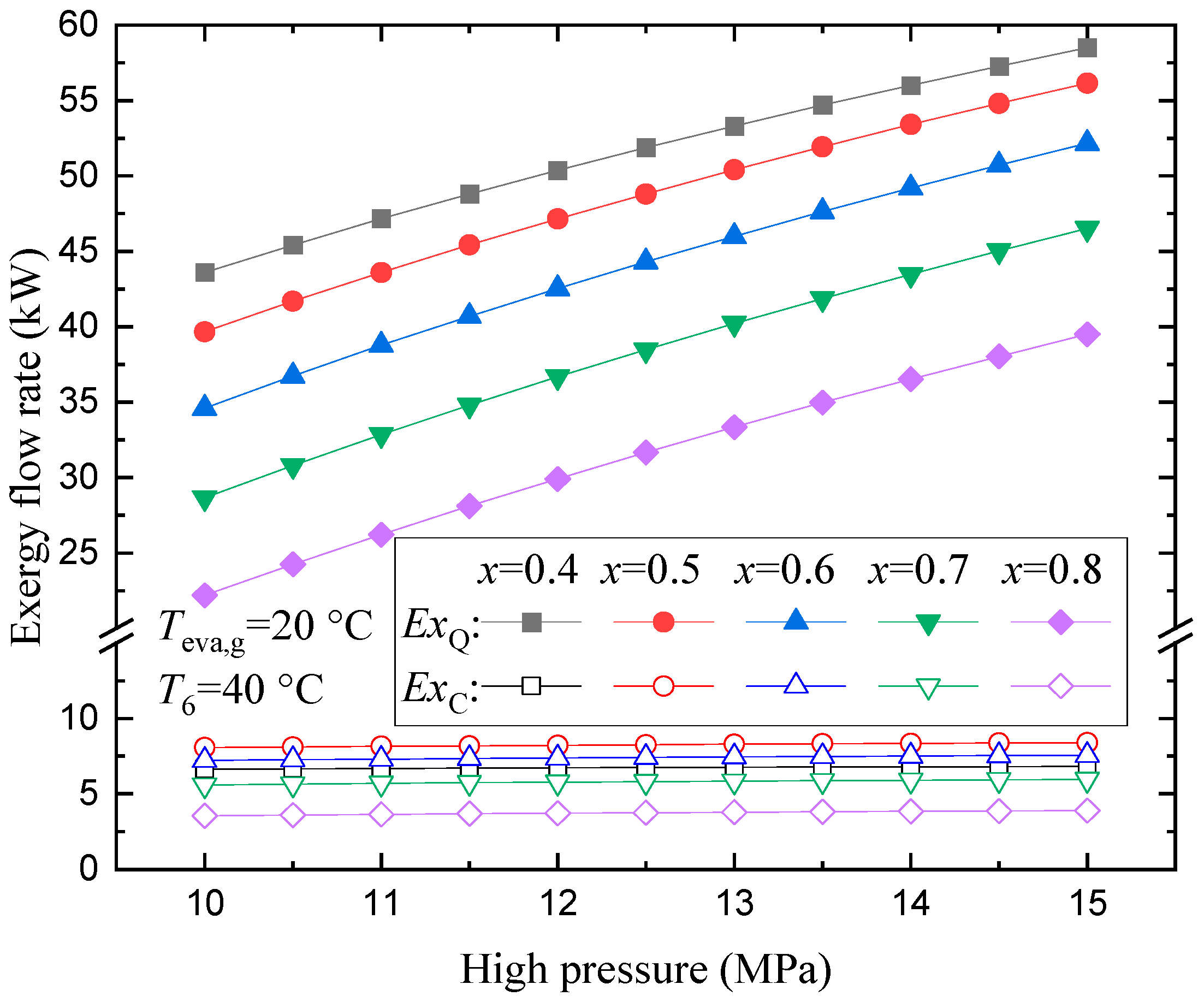
3.1.1. Effects of High Pressure
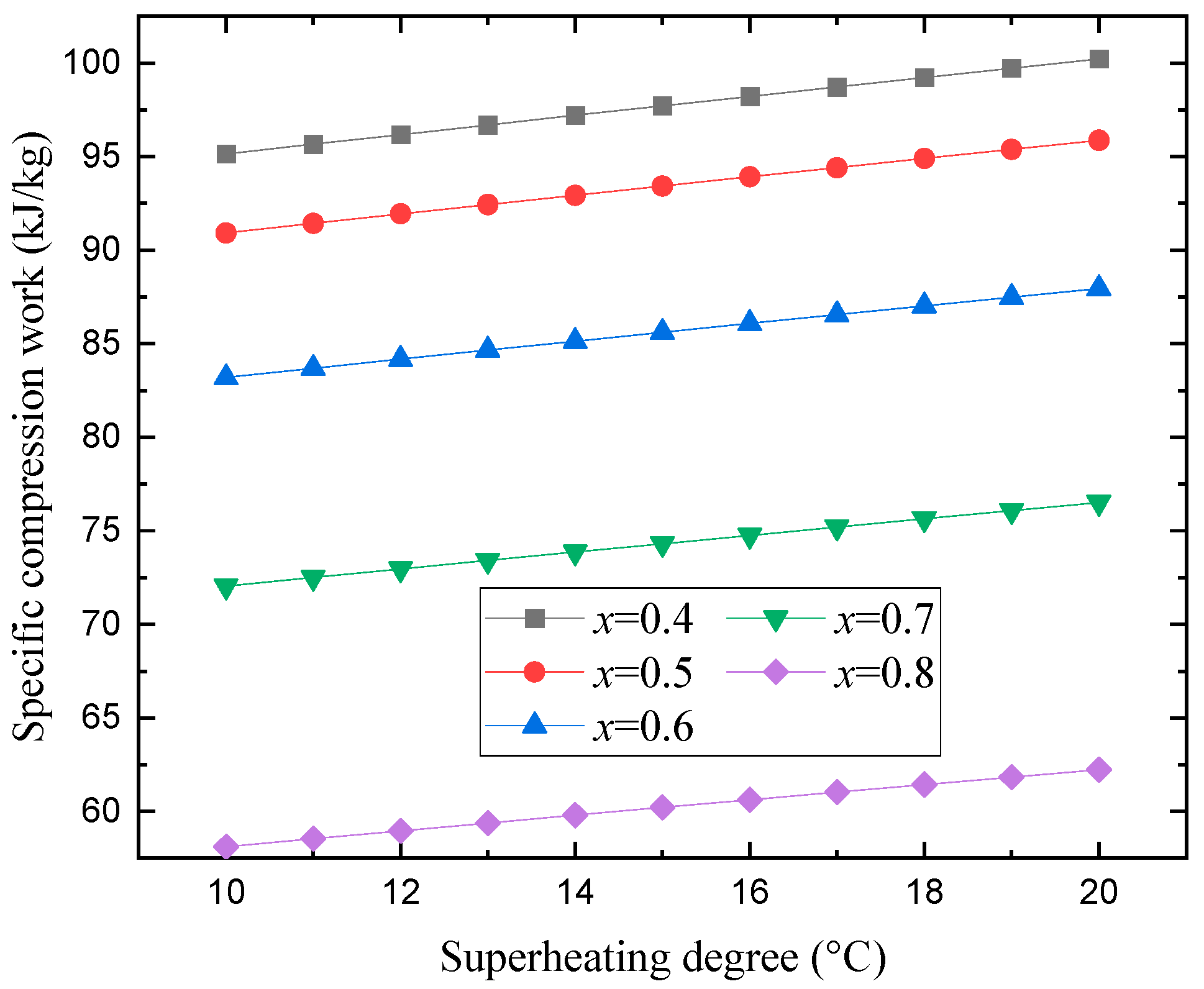
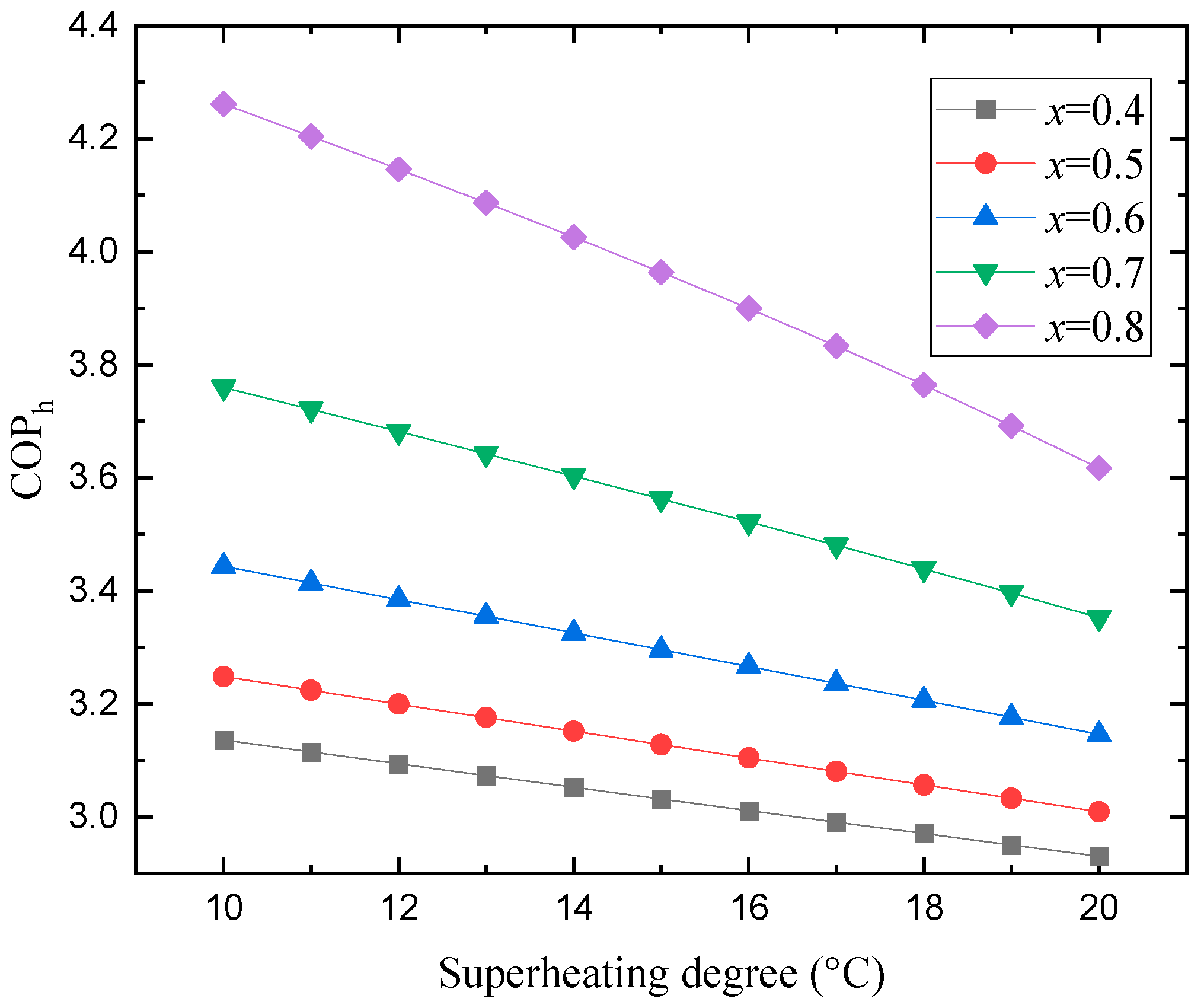
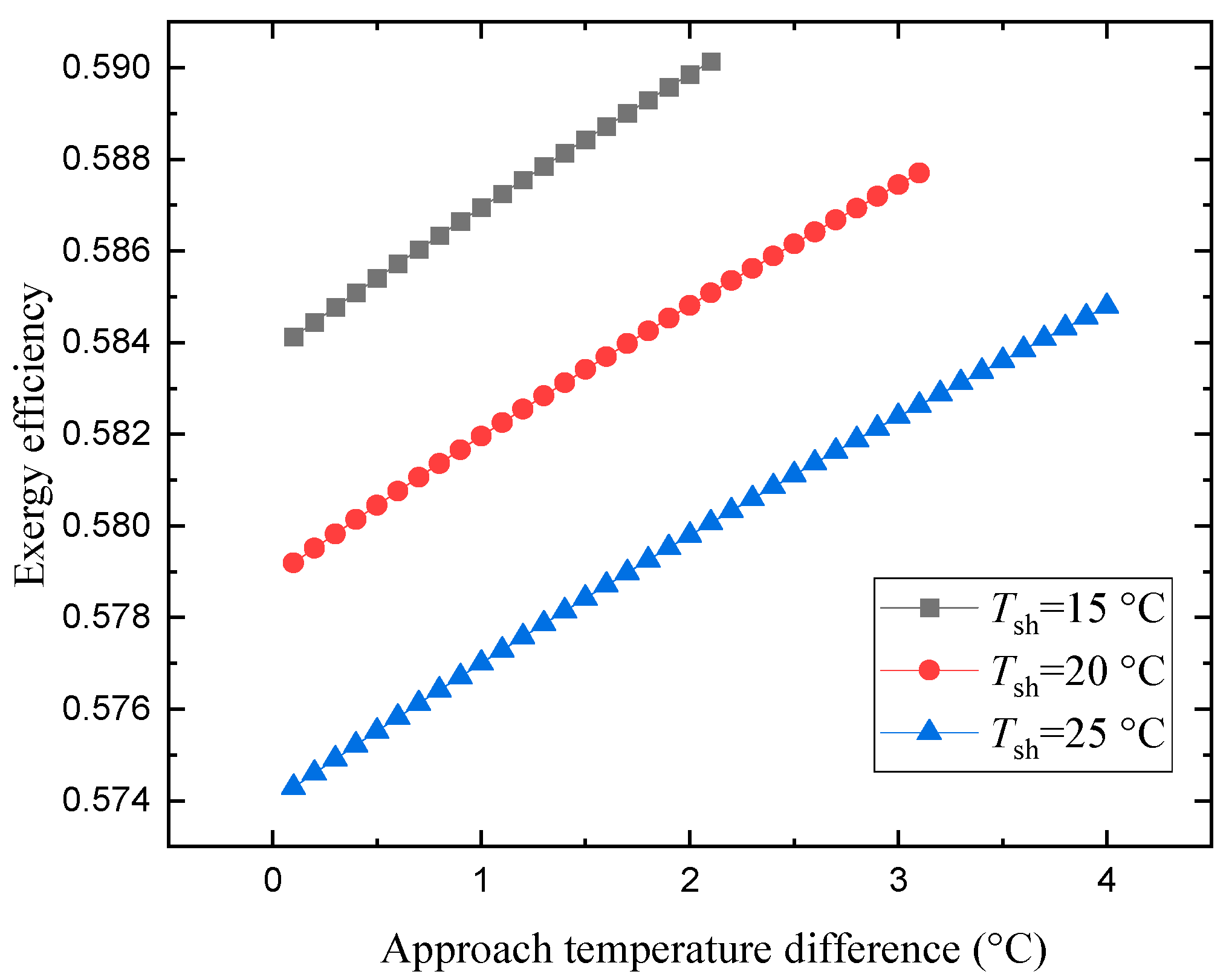
3.1.2. Effects of Superheating Degree
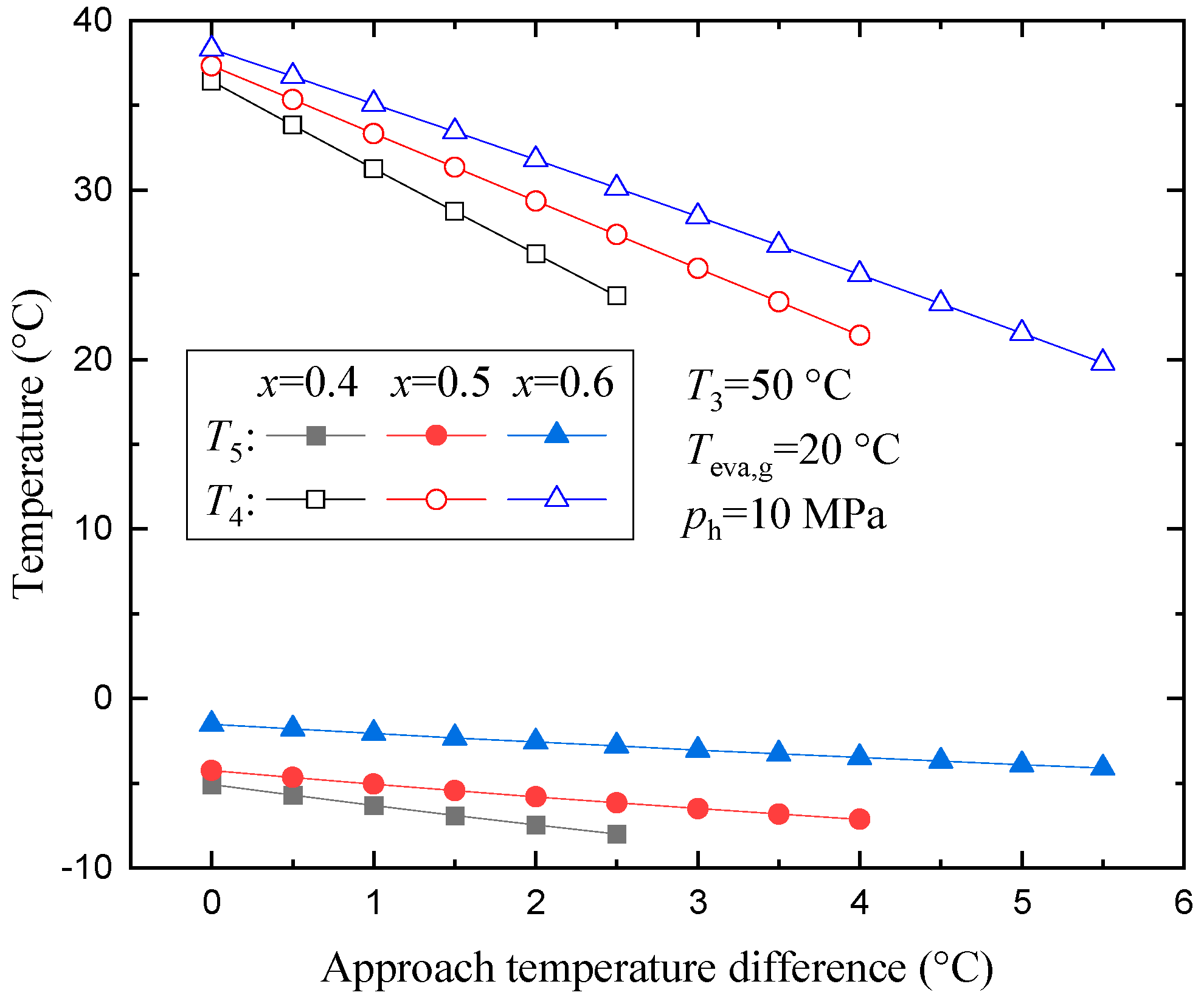
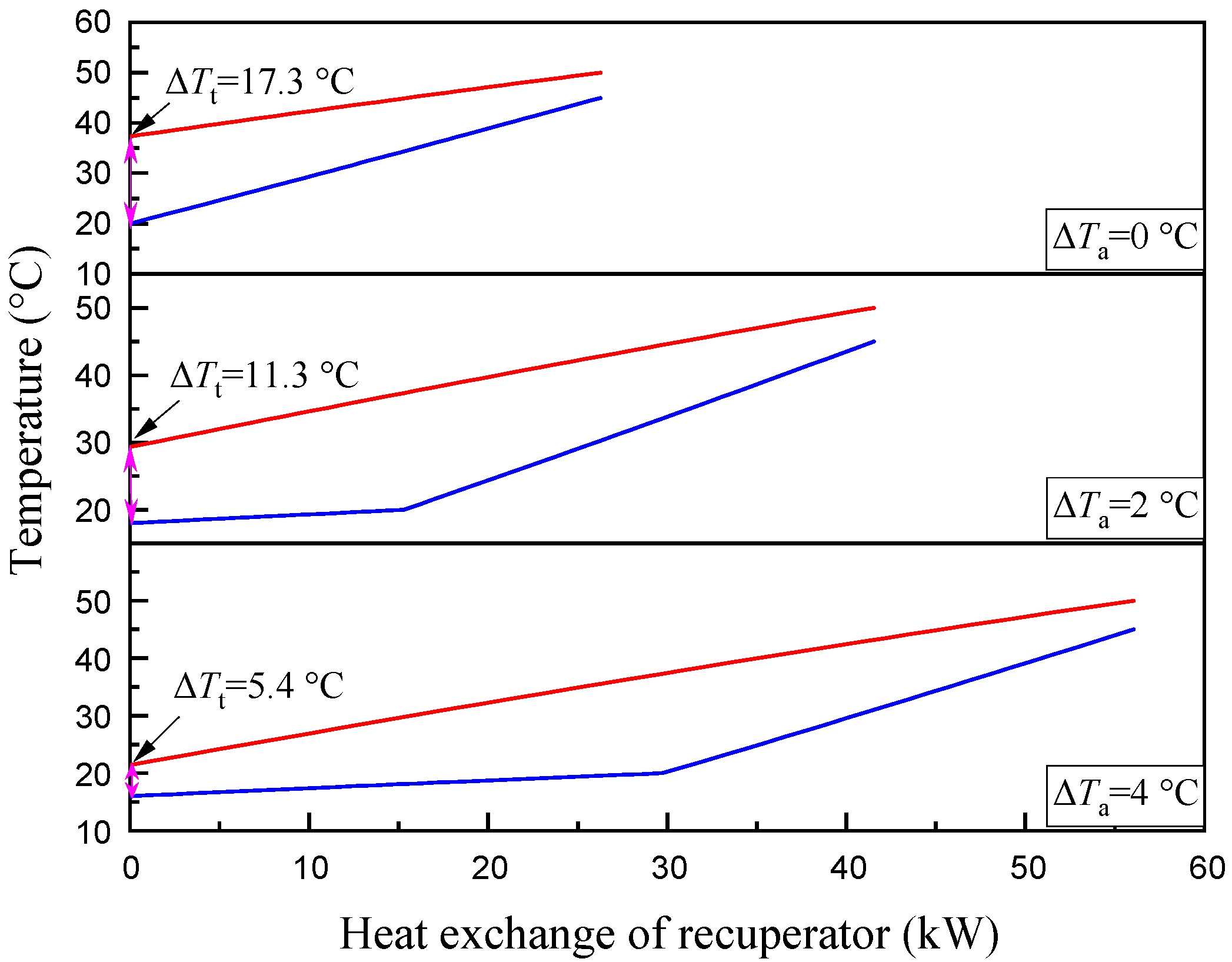
3.1.3. Effects of Recuperator
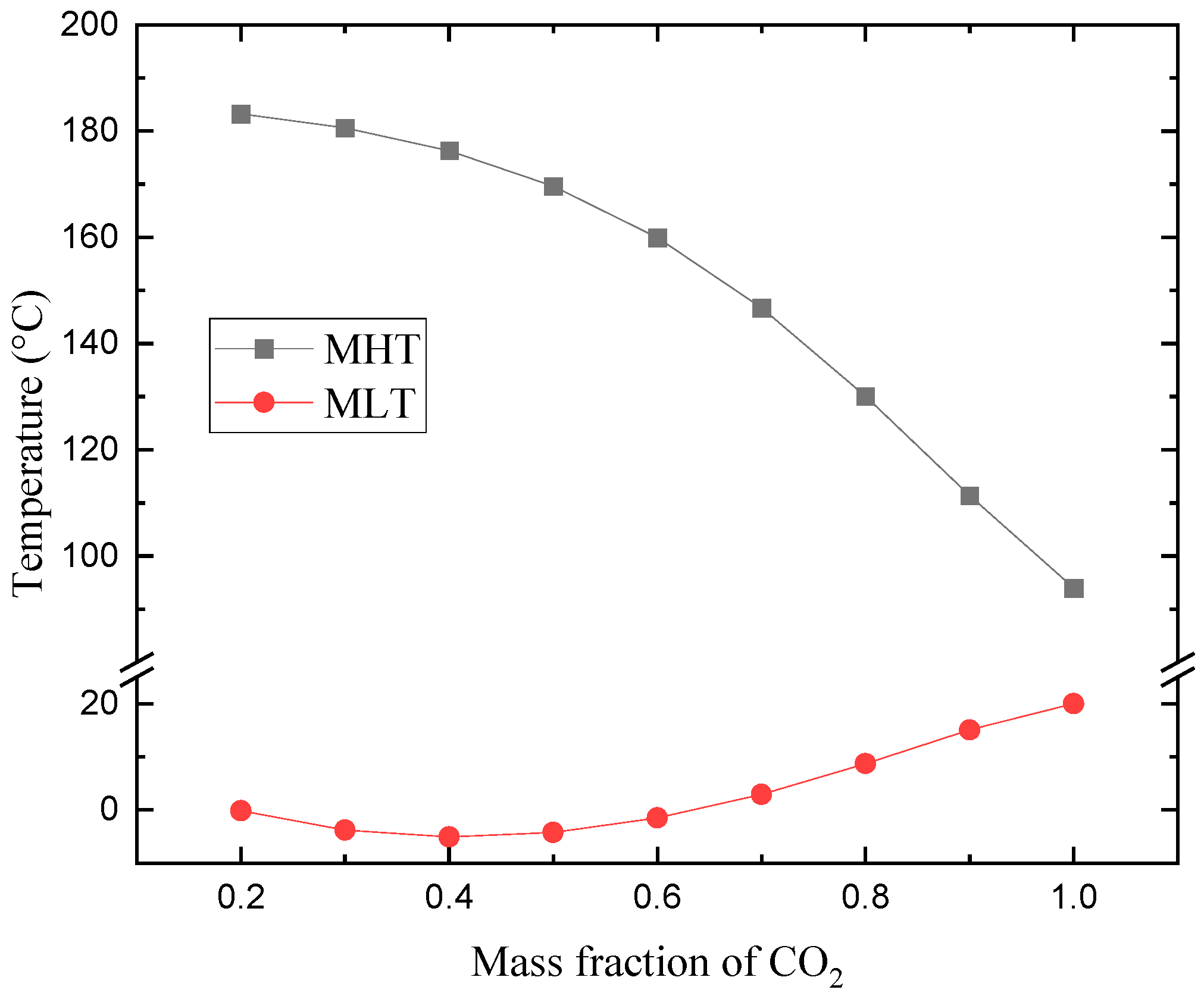
3.1.4. Effects of Refrigerant Fraction
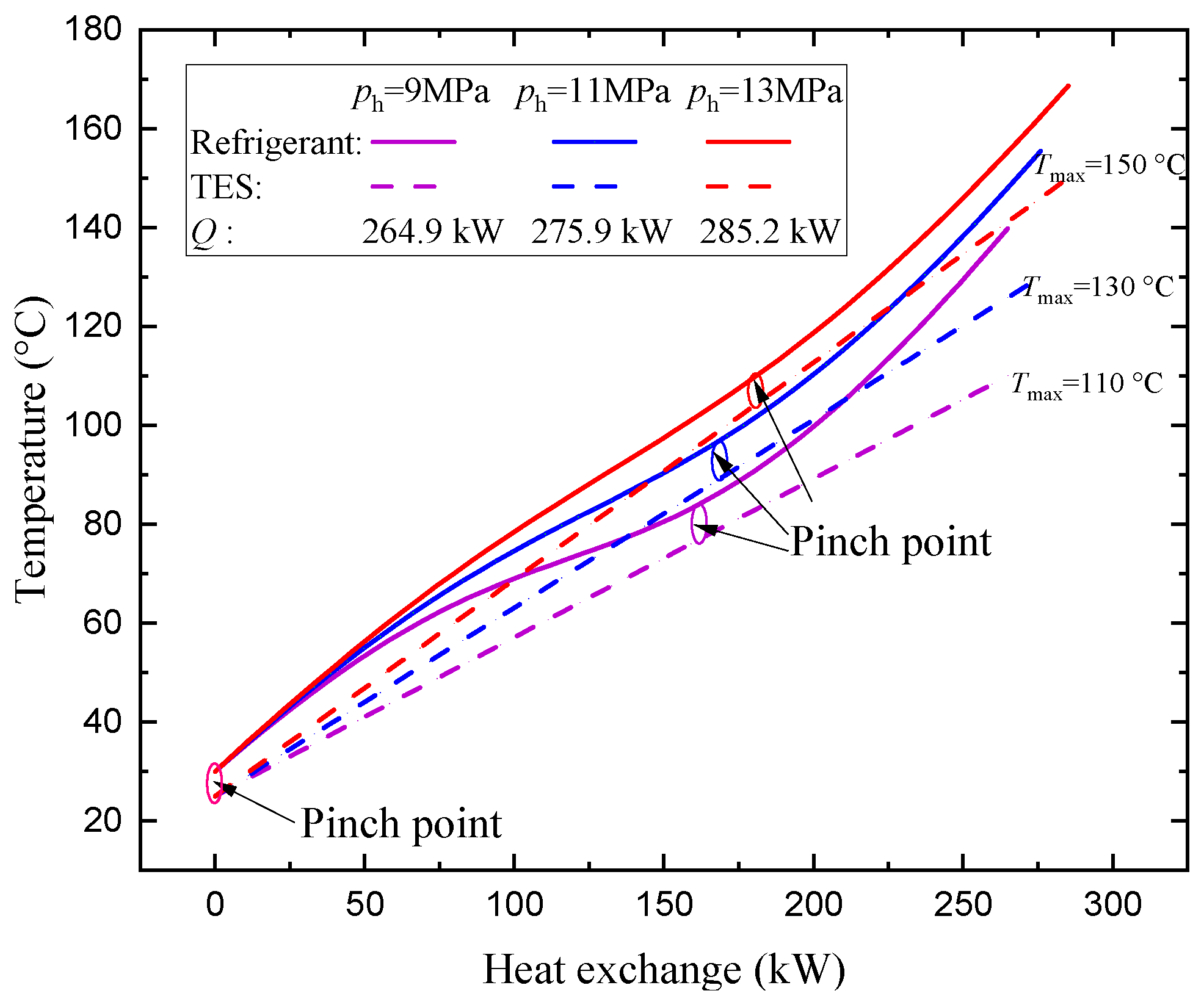
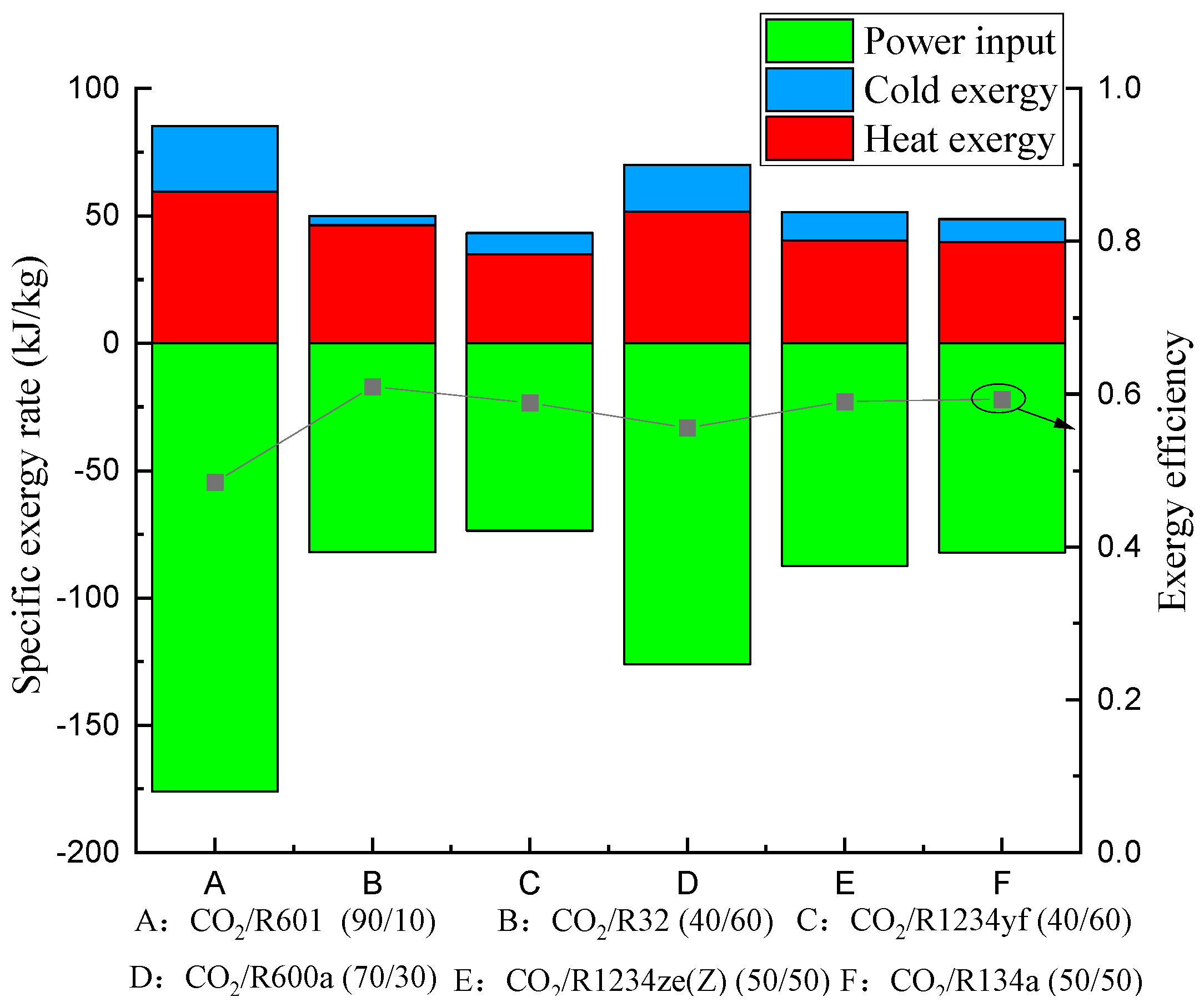
3.2. Coupling of Cycle with Hot and Cold Energy Storage Materials
3.3. Optimization and Comparison
4. Conclusions
- (1)
- The minimum cycle temperature is mainly affected by refrigerant composition, with little influence from high pressure. The maximum cycle temperature benefits from higher pressure, a higher superheating degree, and a lower CO2 mass fraction, while COPh shows an opposite relationships with these parameters.
- (2)
- By utilizing latent heat of two-phase refrigerant for recuperation, the refrigerant achieves a lower temperature after throttling, without additional energy consumption. However, the utilization of latent heat is restricted by the cold terminal temperature difference of the recuperator.
- (3)
- The temperature of TESs is affected by the cycle operation condition, as well as thermal matching between the refrigerant and the TES material. An internal pinch point in the gas cooler induces larger terminal difference in the hot end. Exergy efficiency commonly increases as high pressure increases.
- (4)
- In the condition of a fixed HT-TES temperature of 130 °C, CO2/R32 (90/10) produces the maximum exergy efficiency of 61.0% among mixtures under optimization. A larger evaporating temperature glide is suggested to output higher cold exergy and lower LT-TES temperature. CO2/R600a produces the largest cold exergy proportion of 30.1%, as well as the lowest temperature of −32.4 °C for LT-TES.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| exergy rate, kW | |
| h | specific enthalpy, kJ/kg |
| mass flow rate, kg/s | |
| p | pressure, MPa |
| heat flow rate, kW | |
| s | specific entropy, kJ/(kg·K) |
| T | temperature, °C |
| power consumed, kW | |
| Abbreviations | |
| COPh | heating coefficiency of performance |
| HT | high temperature |
| LT | low temperature |
| TES | thermal energy storage |
| VCHP | vapor compression heat pump |
| Subscripts | |
| 0 | ambient state |
| 1, 2, 3… | states in cycle |
| C | cold |
| H | heat |
| in | inlet |
| net | net |
| out | outlet |
| pp | pinch point |
| t | turbine |
| tot | total |
| Greek symbols | |
| ηex | exergy efficiency |
References
- Ayachi, F.; Tauveron, N.; Tartière, T.; Colasson, S.; Nguyen, D. Thermo-Electric Energy Storage involving CO2 transcritical ground heat storage. Appl. Therm. Eng. Des. Process. Equip. Econ. 2016, 108, 1418–1428. [Google Scholar] [CrossRef]
- Mercangoez, M.; Hemrle, J.; Kaufmann, L.; Z’Graggen, A.; Ohler, C. Electrothermal energy storage with transcritical CO2 cycles. Energy 2012, 45, 407–415. [Google Scholar] [CrossRef]
- Carro, A.; Chacartegui, R.; Ortiz, C.; Carneiro, J.; Becerra, J.A. Integration of energy storage systems based on transcritical CO2: Concept of CO2 based electrothermal energy and geological storage. Energy 2022, 238, 121665. [Google Scholar] [CrossRef]
- Belazreg, A.; Abderrahmane, A.; Qasem, N.A.; Sene, N.; Mohammed, S.; Younis, O.; Guedri, K.; Nasajpour-Esfahani, N.; Toghraie, D. Effect of Y-shaped fins on the performance of shell-and-tube thermal energy storage unit. Case Stud. Therm. Eng. 2022, 40, 102485. [Google Scholar] [CrossRef]
- Miansari, M.; Nazari, M.; Toghraie, D.; Akbari, O.A. Investigating the thermal energy storage inside a double-wall tank utilizing phase-change materials (PCMs). J. Therm. Anal. Calorim. 2020, 139, 2283–2294. [Google Scholar] [CrossRef]
- Frate, G.F.; Antonelli, M.; Desideri, U. A novel Pumped Thermal Electricity Storage (PTES) system with thermal integration. Appl. Therm. Eng. Des. Process. Equip. Econ. 2017, 121, 1051–1058. [Google Scholar] [CrossRef]
- Frate, G.F.; Ferrari, L.; Desideri, U. Multi-criteria investigation of a pumped thermal electricity storage (PTES) system with thermal integration and sensible heat storage. Energy Convers. Manag. 2020, 208, 112530. [Google Scholar] [CrossRef]
- Zhang, M.; Shi, L.; Hu, P.; Pei, G.; Shu, G. Carnot battery system integrated with low-grade waste heat recovery: Toward high energy storage efficiency. J. Energy Storage 2023, 57, 106234. [Google Scholar] [CrossRef]
- Dumont, O.; Frate, G.F.; Pillai, A.; Lecompte, S.; Lemort, V. Carnot battery technology: A state-of-the-art review. J. Energy Storage 2020, 32, 101756. [Google Scholar] [CrossRef]
- Obara, S. Energy storage device based on a hybrid system of a CO2 heat pump cycle and a CO2 hydrate heat cycle. Renew. Sustain. Energy Rev. 2023, 179, 113290. [Google Scholar] [CrossRef]
- Hakkaki-Fard, A.; Aidoun, Z.; Ouzzane, M. Applying refrigerant mixtures with thermal glide in cold climate air-source heat pumps. Appl. Therm. Eng. 2014, 62, 714–722. [Google Scholar] [CrossRef]
- Guo, H.; Gong, M.; Qin, X. Performance analysis of a modified subcritical zeotropic mixture recuperative high-temperature heat pump. Appl. Energy 2019, 237, 338–352. [Google Scholar] [CrossRef]
- Cui, Q.; Wang, C.; Gao, E.; Zhang, X. Pinch point characteristics and performance evaluation of CO2 heat pump water heater under variable working conditions. Appl. Therm. Eng. 2022, 207, 118208. [Google Scholar] [CrossRef]
- Wang, X.; Li, H.; Wang, Y.; Zhao, J.; Zhu, J.; Zhong, S.; Li, Y. Energy, exergy, and economic analysis of a data center energy system driven by the CO2 ground source heat pump: Prosumer perspective. Energy Convers. Manag. 2021, 232, 113877. [Google Scholar] [CrossRef]
- White, S.D.; Yarrall, M.G.; Cleland, D.J.; Hedley, R.A. Modelling the performance of a transcritical CO2 heat pump for high temperature heating (Reprinted). Int. J. Refrig. 2002, 25, 479–486. [Google Scholar] [CrossRef]
- Sun, Z.; Cui, Q.; Wang, Q.; Ning, J.; Xu, Y. Experimental study on CO2/R32 blends in a water-to-water heat pump system. Appl. Therm. Eng. 2019, 162, 114303. [Google Scholar] [CrossRef]
- Dai, B.; Dang, C.; Li, M.; Tian, H.; Ma, Y. Thermodynamic performance assessment of carbon dioxide blends with low-global warming potential (GWP) working fluids for a heat pump water heater. Int. J. Refrig. 2015, 56, 1–14. [Google Scholar] [CrossRef]
- Ganesan, P.; Eikevik, T.M. New zeotropic CO2-based refrigerant mixtures for cascade high-temperature heat pump to reach heat sink temperature up to 180 °C. Energy Convers. Manag. X 2023, 20, 100407. [Google Scholar] [CrossRef]
- Gómez-Hernández, J.; Grimes, R.; Briongos, J.V.; Marugán-Cruz, C.; Santana, D. Carbon dioxide and acetone mixtures as refrigerants for industry heat pumps to supply temperature in the range 150–220 °C. Energy 2023, 269, 126821. [Google Scholar] [CrossRef]
- Zhang, F.; Zhu, Y.; Li, C.; Jiang, P. Thermodynamic optimization of heat transfer process in thermal systems using CO2 as the working fluid based on temperature glide matching. Energy 2018, 151, 376–386. [Google Scholar] [CrossRef]
- Zühlsdorf, B.; Jensen, J.K.; Cignitti, S.; Madsen, C.; Elmegaard, B. Analysis of temperature glide matching of heat pumps with zeotropic working fluid mixtures for different temperature glides. Energy 2018, 153, 650–660. [Google Scholar] [CrossRef]
- Frate, G.F.; Ferrari, L.; Desideri, U. Rankine Carnot Batteries with the Integration of Thermal Energy Sources: A Review. Energ. 2020, 13, 4766. [Google Scholar] [CrossRef]
- Rodríguez-deArriba, P.; Crespi, F.; Sánchez, D.; Muñoz, A.; Sánchez, T. The potential of transcritical cycles based on CO2 mixtures: An exergy-based analysis. Renew. Energy 2022, 199, 1606–1628. [Google Scholar] [CrossRef]
- Binbin, Y.U.; Yang, J.; Wang, D.; Shi, J.; Chen, J. Modeling and theoretical analysis of a CO2-propane autocascade heat pump for electrical vehicle heating. Int. J. Refrig. 2018, 95, 146–155. [Google Scholar]
- Li, C.; Pan, L.; Wang, Y. Thermodynamic optimization of Rankine cycle using CO2-based binary zeotropic mixture for ocean thermal energy conversion. Appl. Therm. Eng. 2020, 178, 115617. [Google Scholar] [CrossRef]
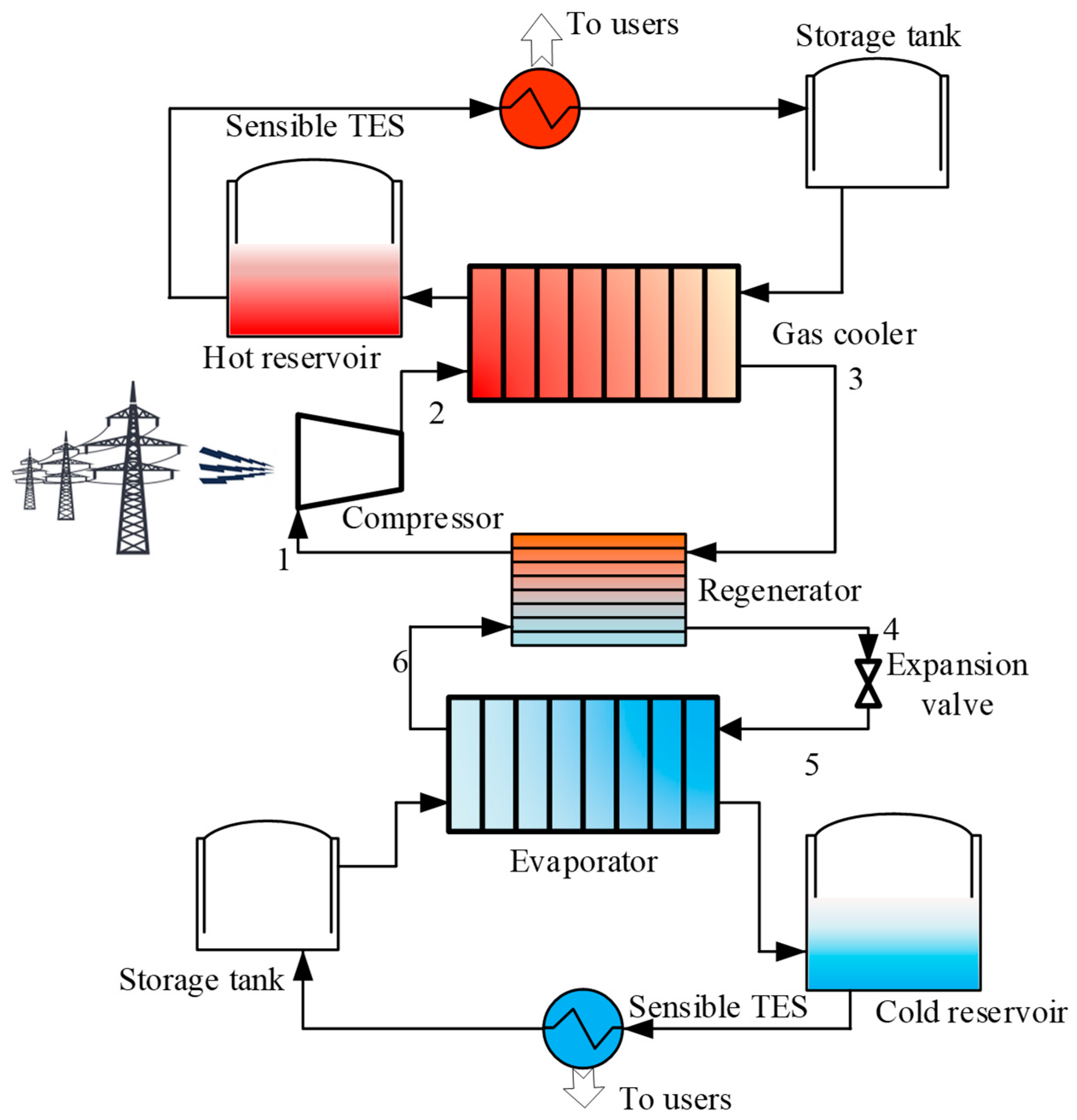






| Parameter | Value |
|---|---|
| Heat pump cycle | |
| High pressure, ph (MPa) | ≥1.1 pcrit |
| Evaporating temperature, Teva (°C) | 20 |
| Mass flow rate of refrigerant, (kg/s) | 1 |
| Pinch point temperature difference, ∆Tpp (°C) | 5 |
| Approach temperature difference, ∆Tap (°C) | 0–5 |
| Superheating degree, ∆Tsh (°C) | 5–20 |
| Compressor isentropic efficiency, ηcom | 0.85 |
| Storage | |
| Hot storage max temperature, Ths,max (°C) | 130 |
| Cold storage temperature, Tcs (°C) | Variable |
| Ambient temperature, Tamb (°C) | 25 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, C.; Wang, Y.; Guo, Q.; Wang, Y.; Chen, H. High-Temperature Heat Pump Using CO2-Based Mixture for Simultaneous Heat and Cold Energy Reservation. Energies 2023, 16, 6587. https://doi.org/10.3390/en16186587
Li C, Wang Y, Guo Q, Wang Y, Chen H. High-Temperature Heat Pump Using CO2-Based Mixture for Simultaneous Heat and Cold Energy Reservation. Energies. 2023; 16(18):6587. https://doi.org/10.3390/en16186587
Chicago/Turabian StyleLi, Chengyu, Yongzhen Wang, Qiang Guo, Youtang Wang, and Hu Chen. 2023. "High-Temperature Heat Pump Using CO2-Based Mixture for Simultaneous Heat and Cold Energy Reservation" Energies 16, no. 18: 6587. https://doi.org/10.3390/en16186587
APA StyleLi, C., Wang, Y., Guo, Q., Wang, Y., & Chen, H. (2023). High-Temperature Heat Pump Using CO2-Based Mixture for Simultaneous Heat and Cold Energy Reservation. Energies, 16(18), 6587. https://doi.org/10.3390/en16186587






