Transient Simulation and Parameter Sensitivity Analysis of Godiva Experiment Based on MOOSE Platform
Abstract
:1. Introduction
2. Simulation Model and Methods
2.1. Reactivity Feedback Effect
2.1.1. Point Reactor Kinetic Method
2.1.2. Neutron Flux Calculation
2.1.3. Heat Source Loading
2.1.4. Quench Coefficient and Reactivity Temperature Coefficient
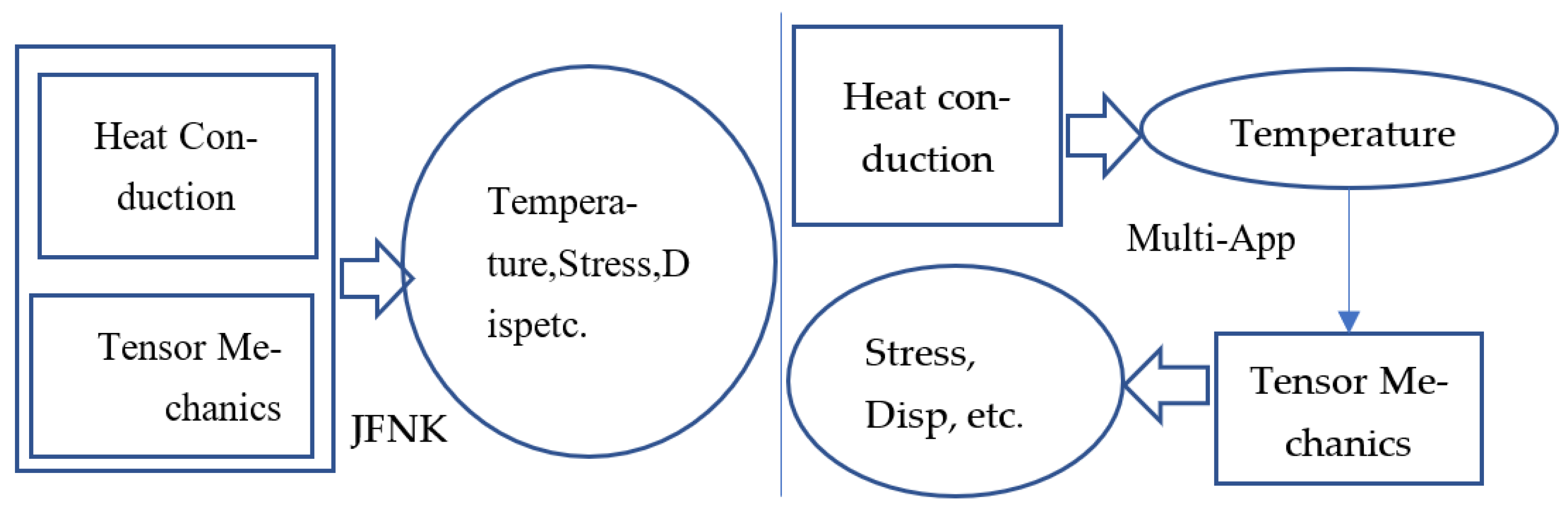
2.1.5. Thermal-Mechanical Calculation
2.2. Inertia Effect
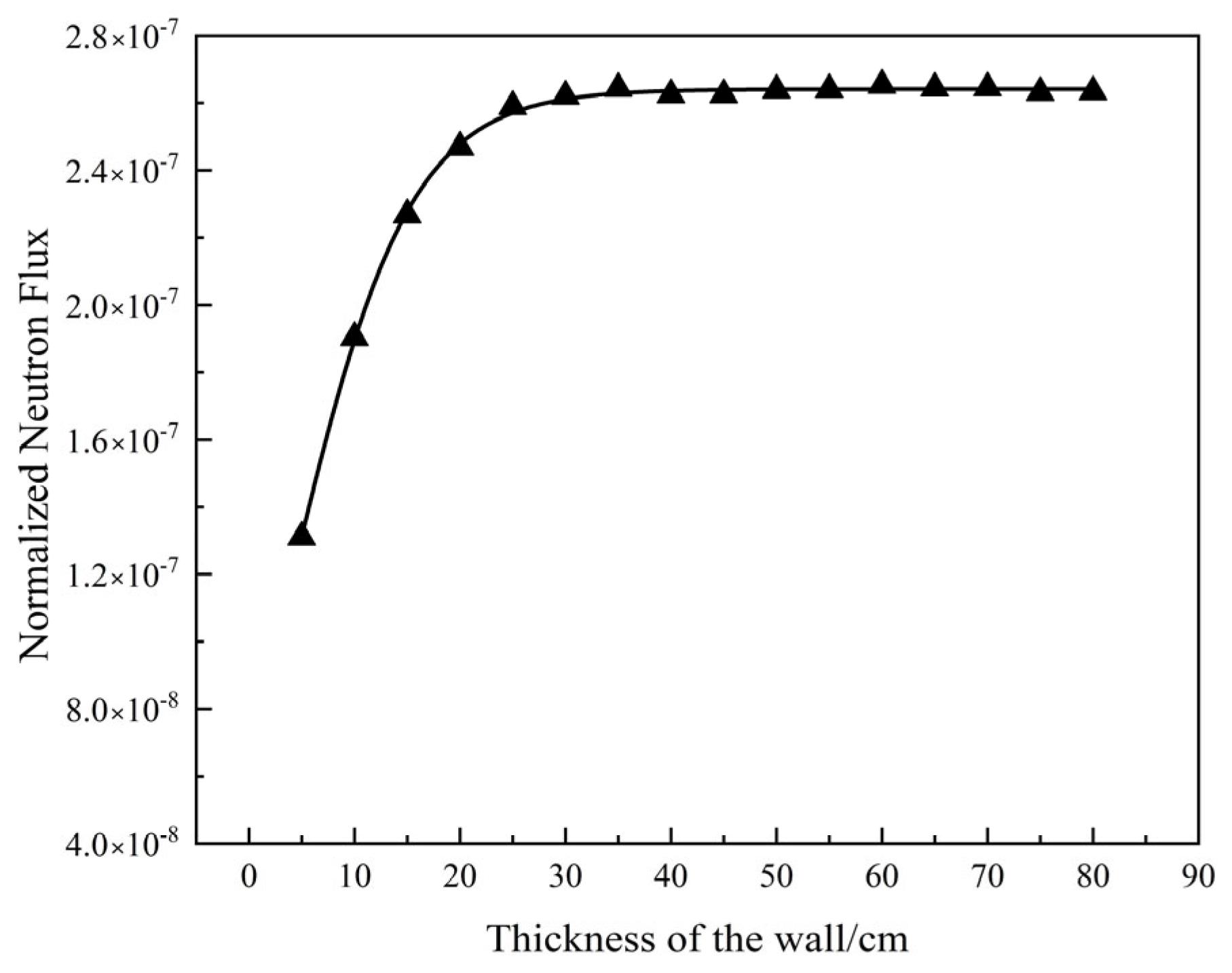
2.3. Wall-Reflected Neutrons Effect
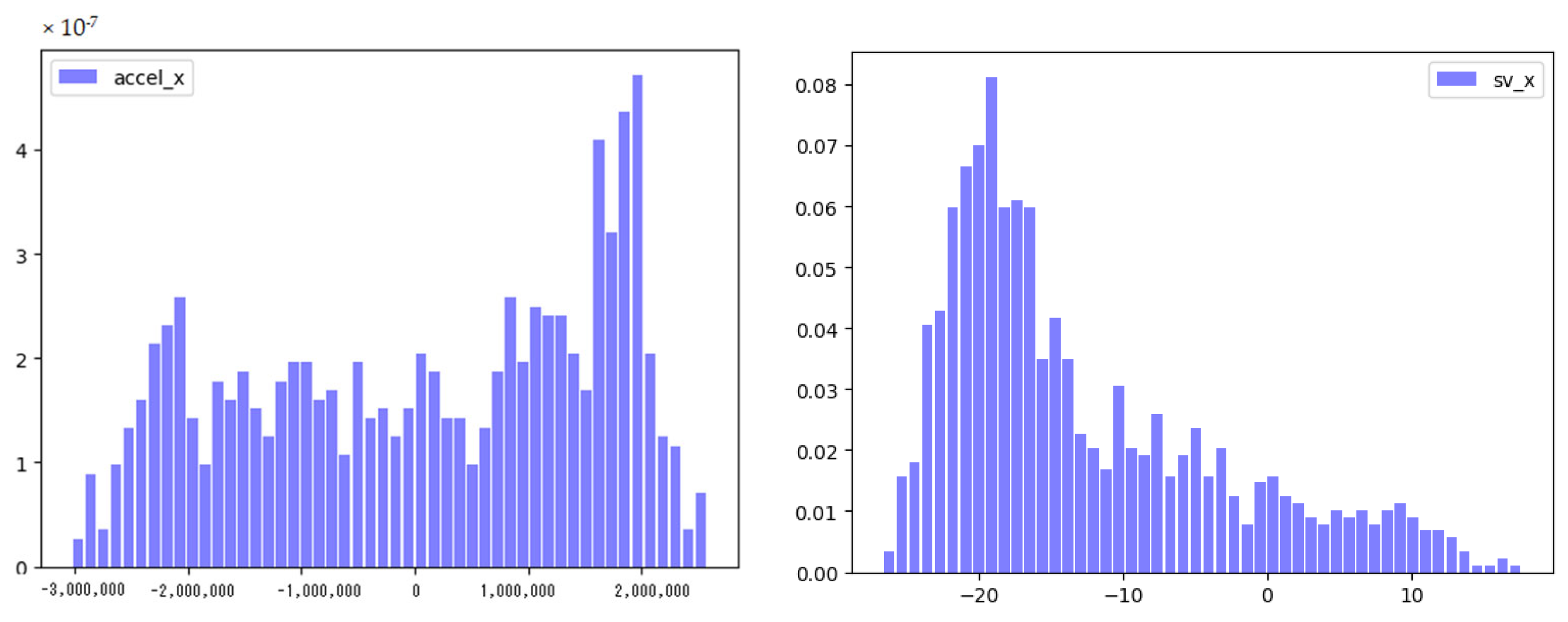
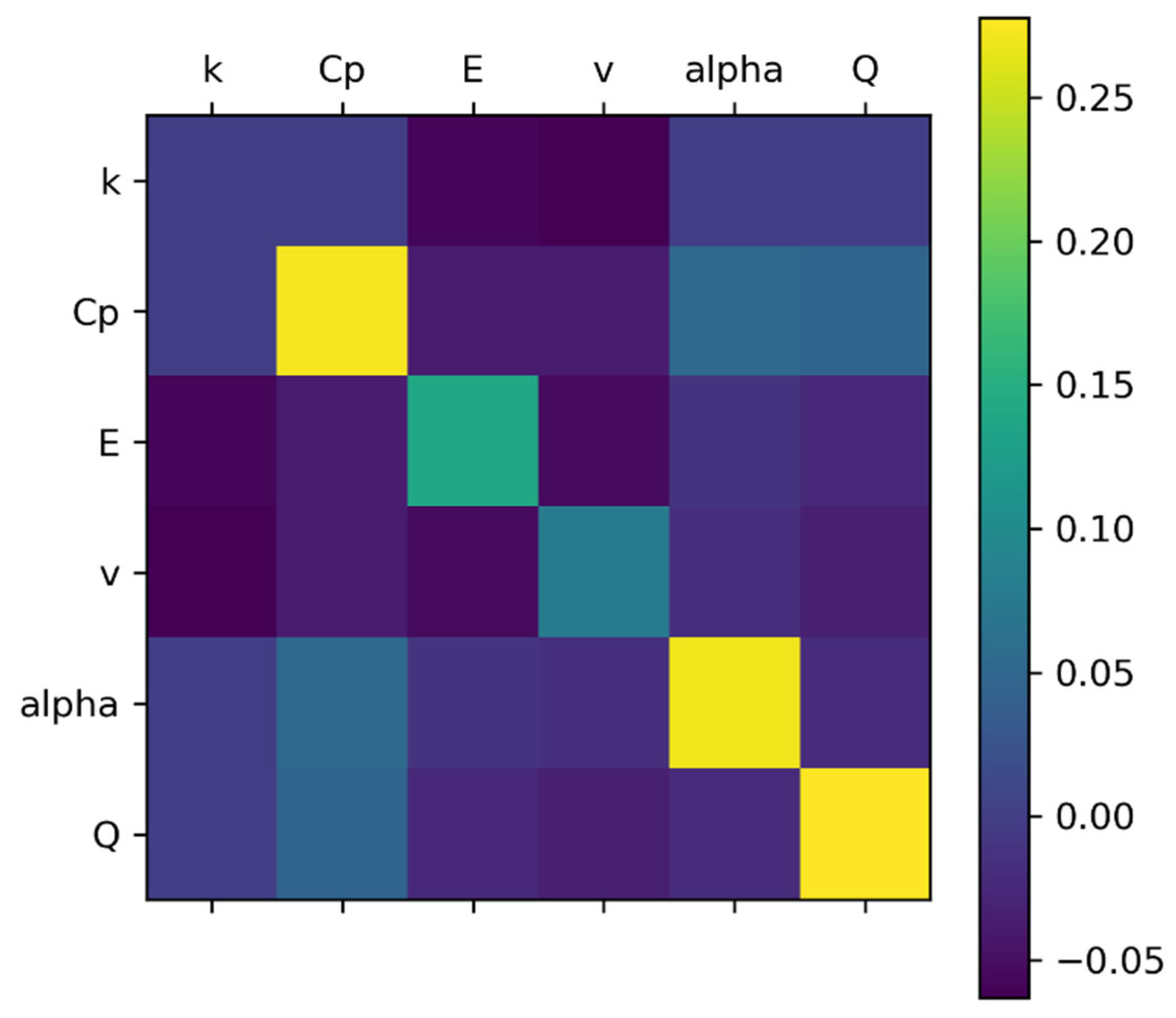
2.4. Sensitivity Analysis
3. Results and Discussion
3.1. Reactivity Feedback Effect
3.1.1. Steady-State Reactivity Temperature Coefficient
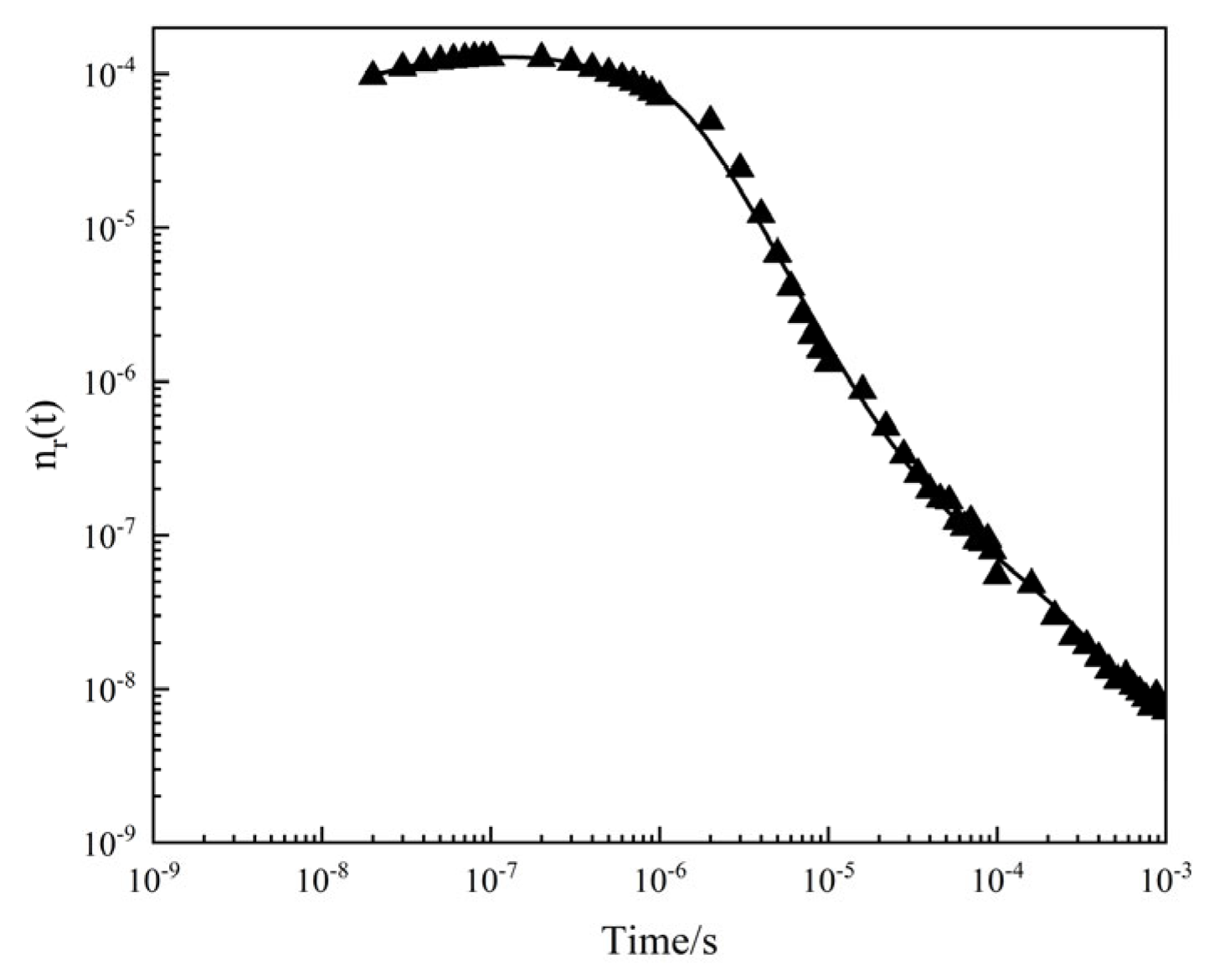
3.1.2. Transient Reactivity
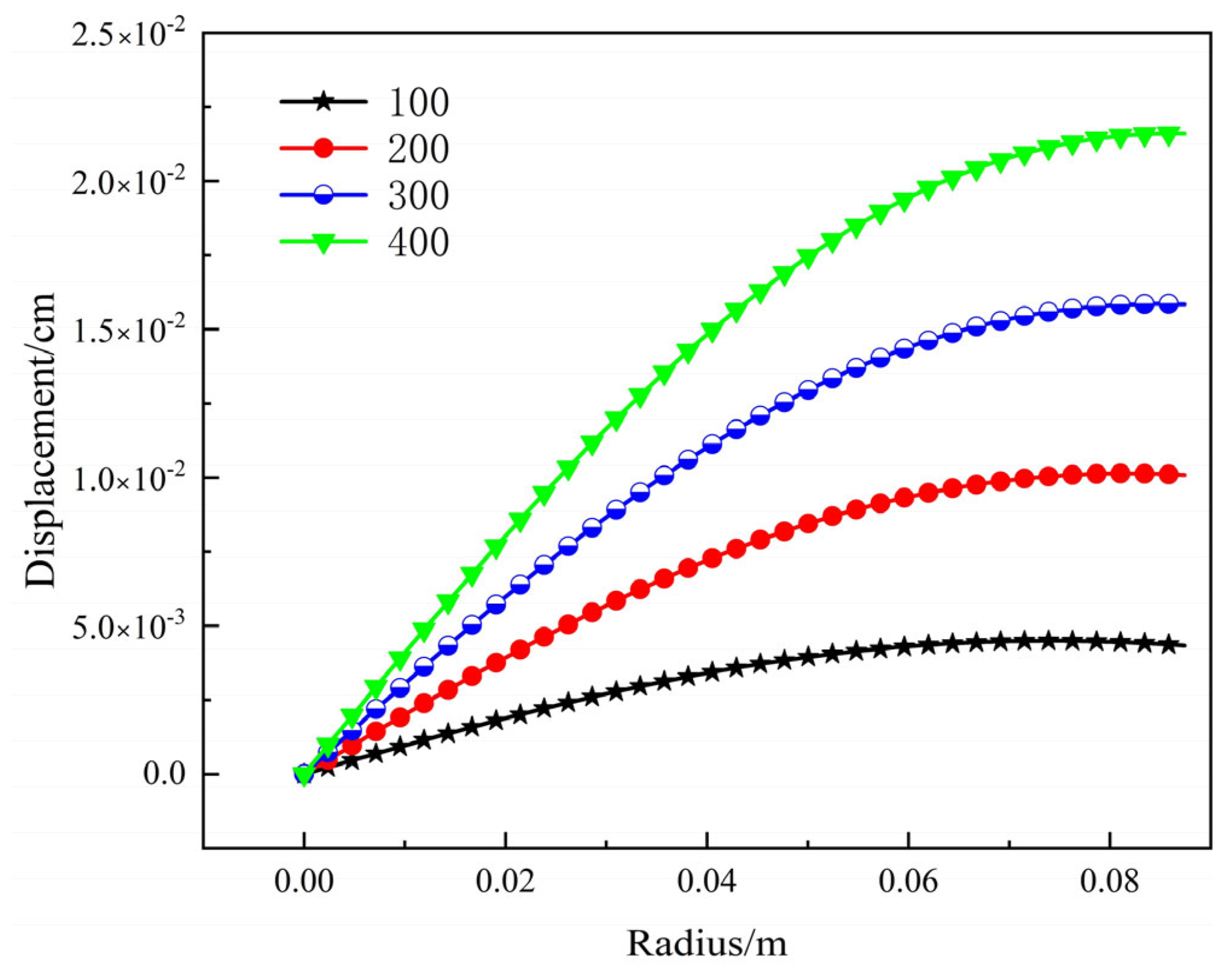
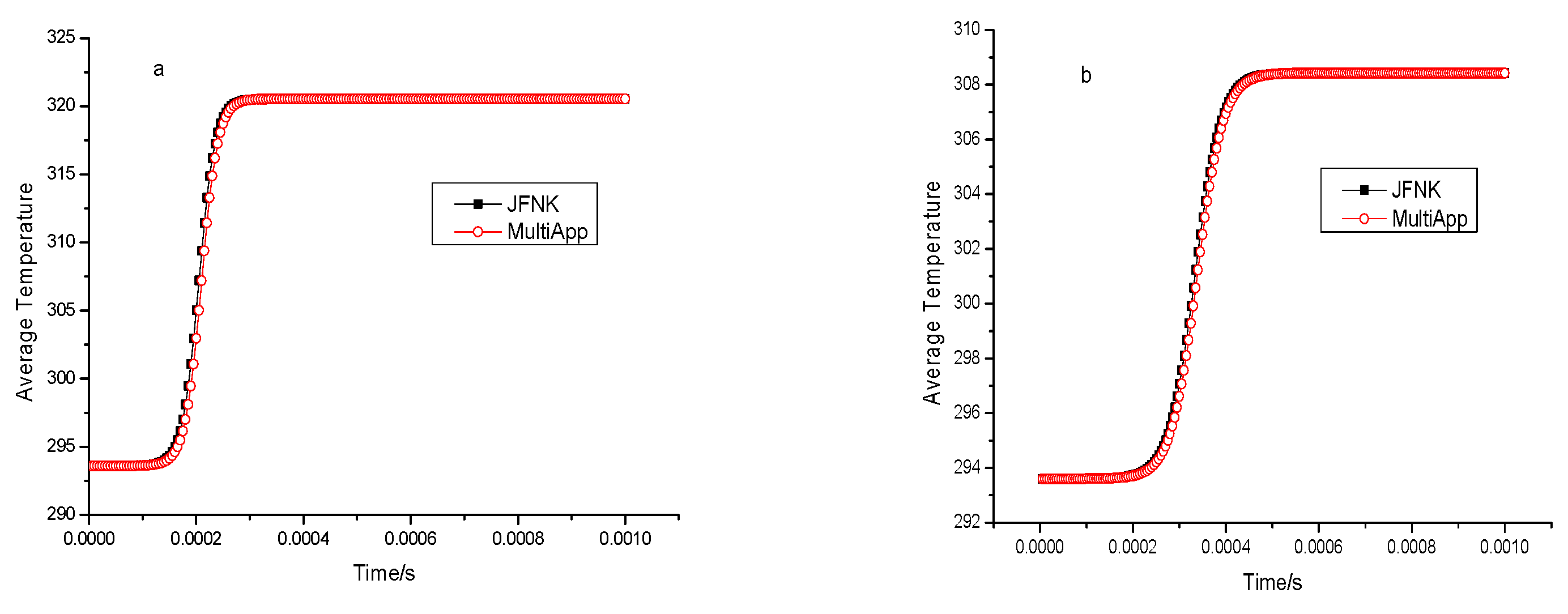
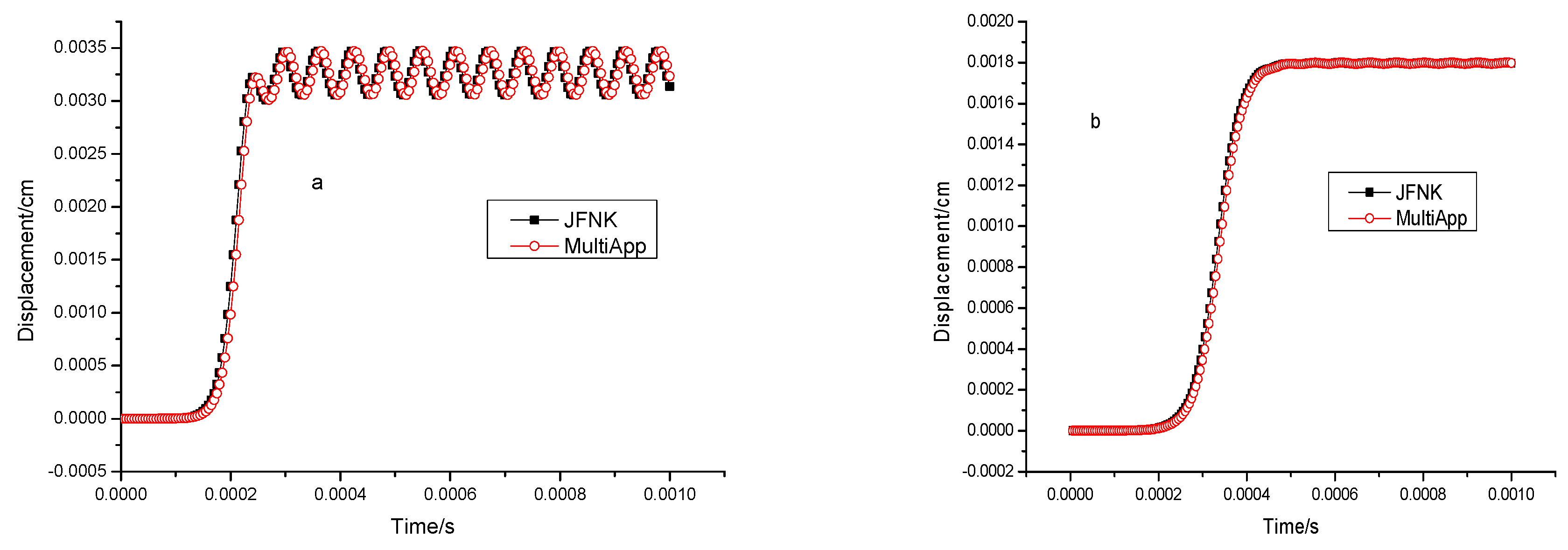
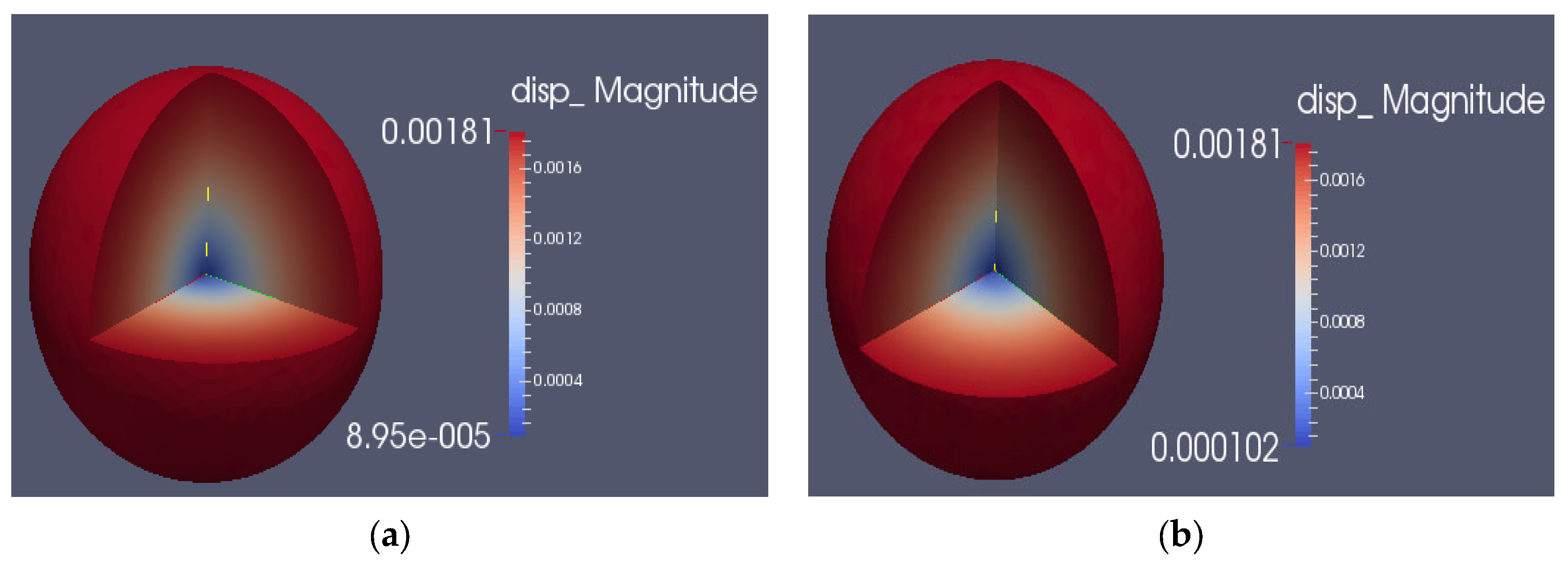
3.1.3. Transient Thermal-Mechanical Calculation
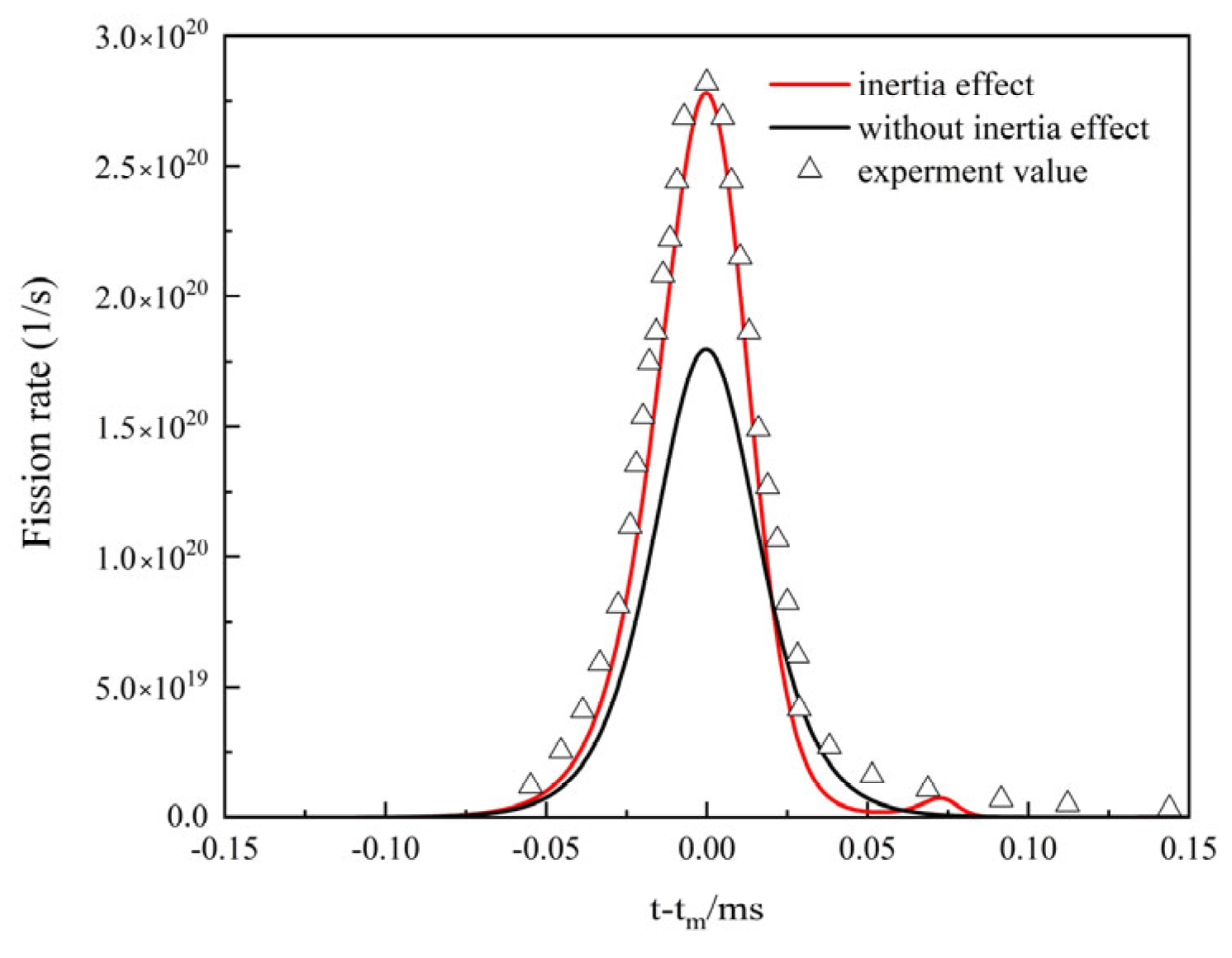
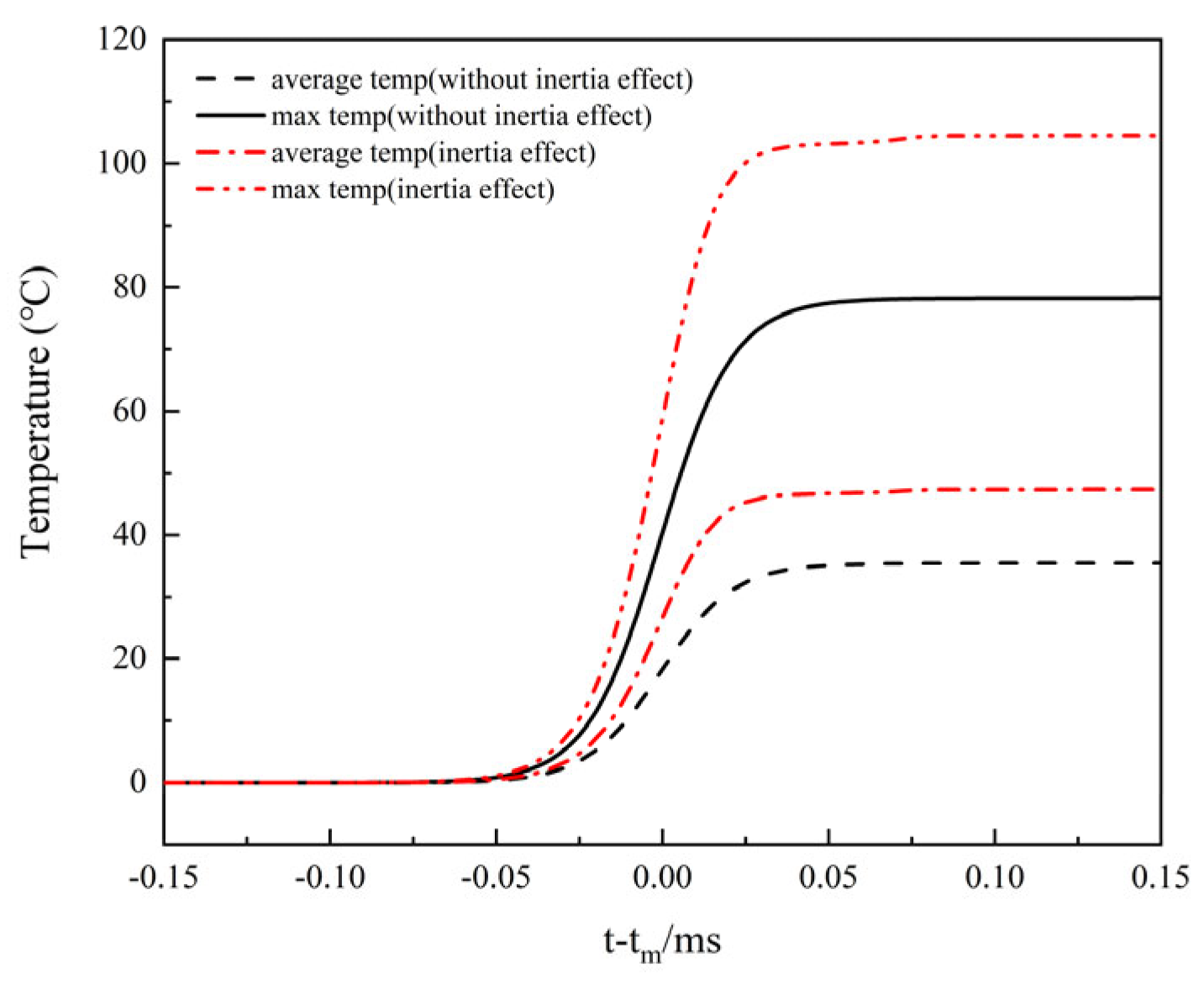
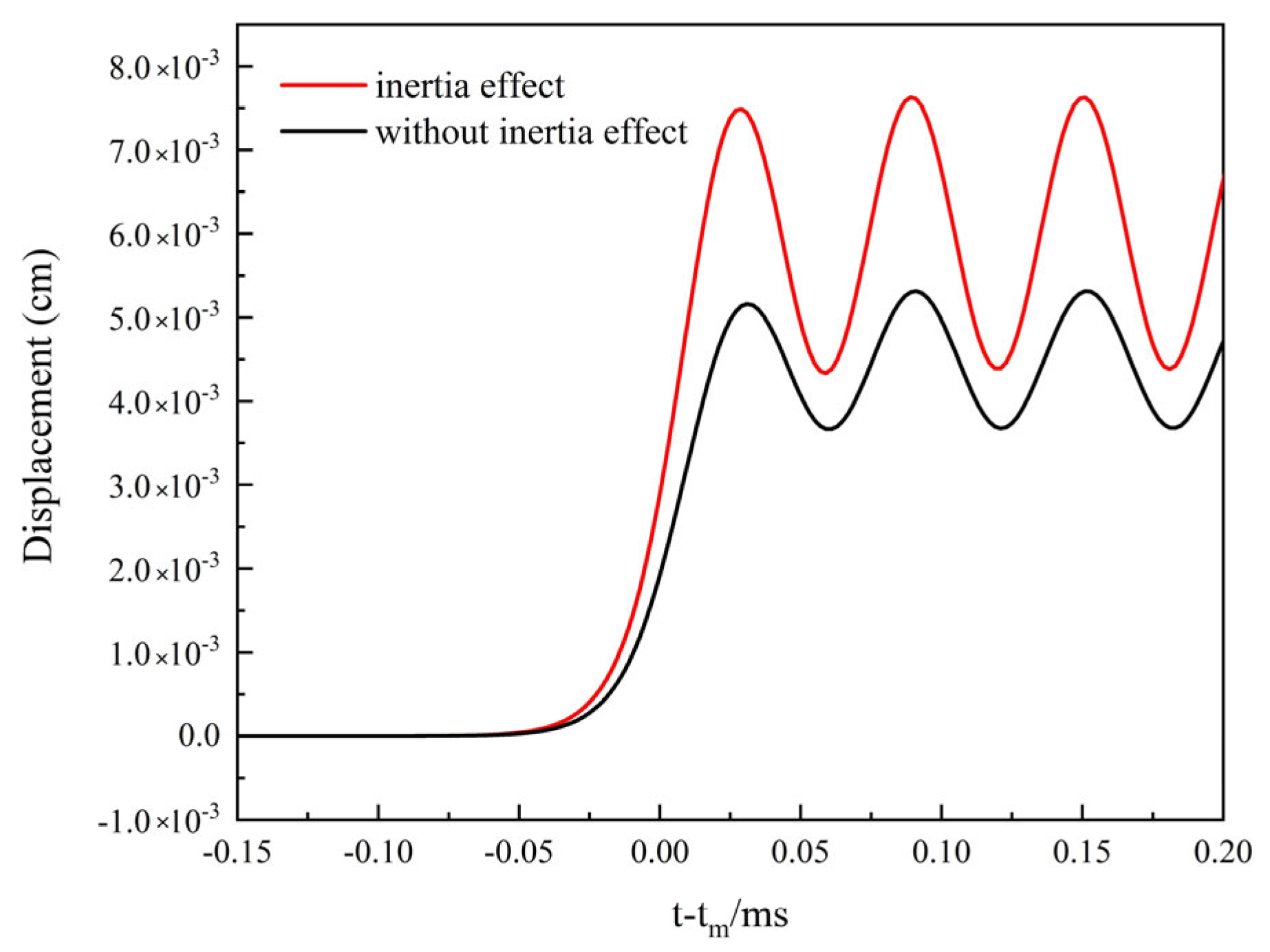
3.2. Inertia Effect
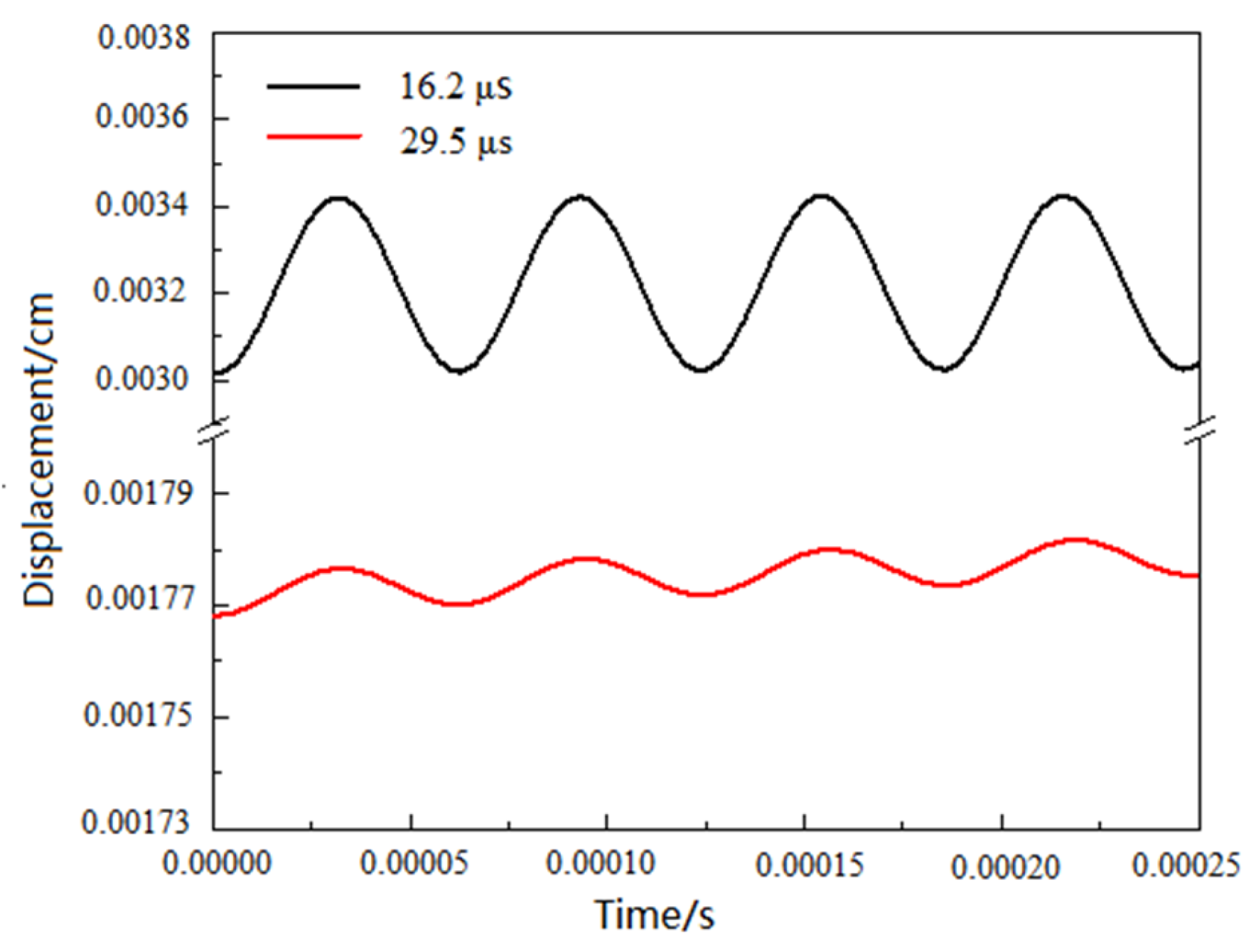
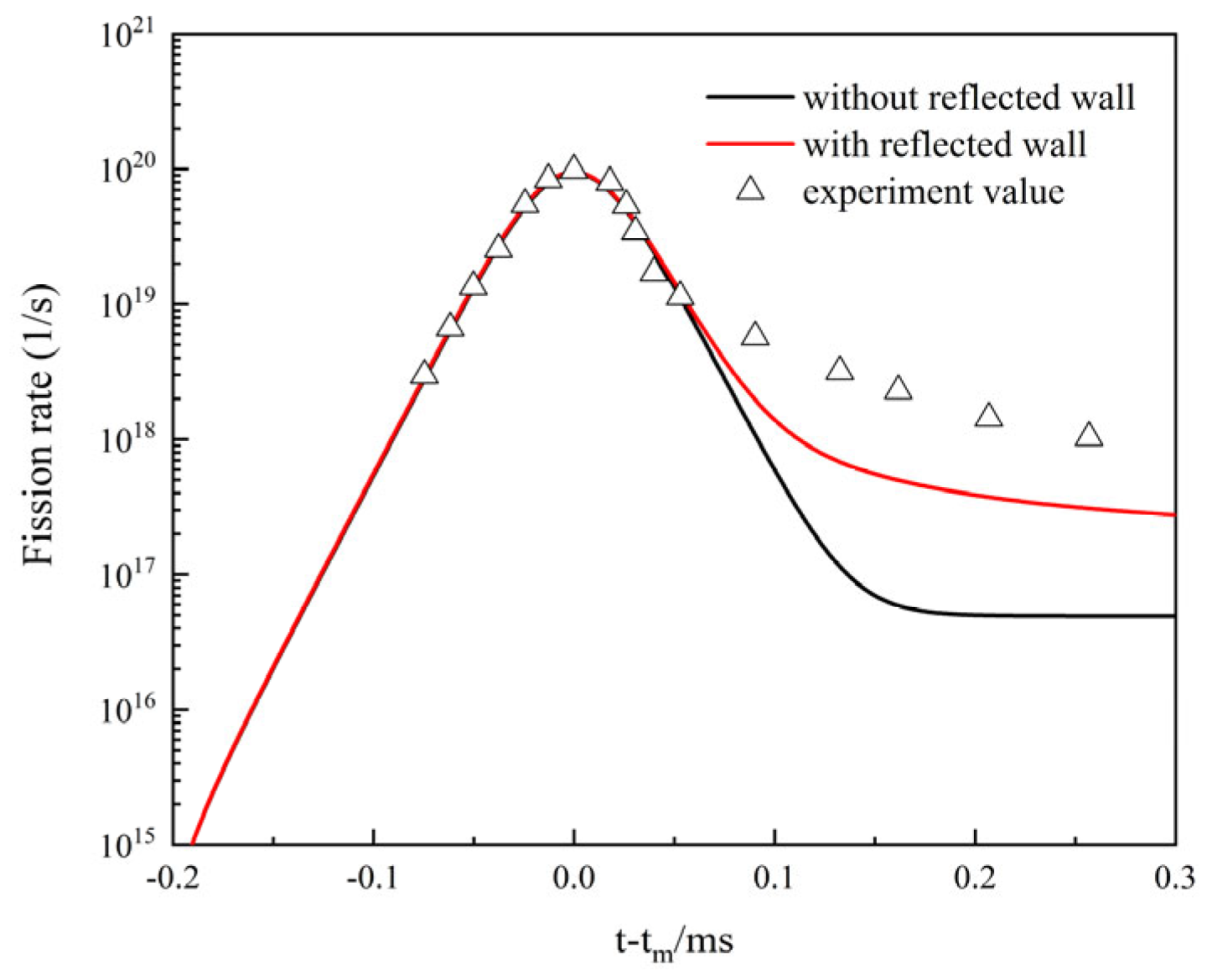
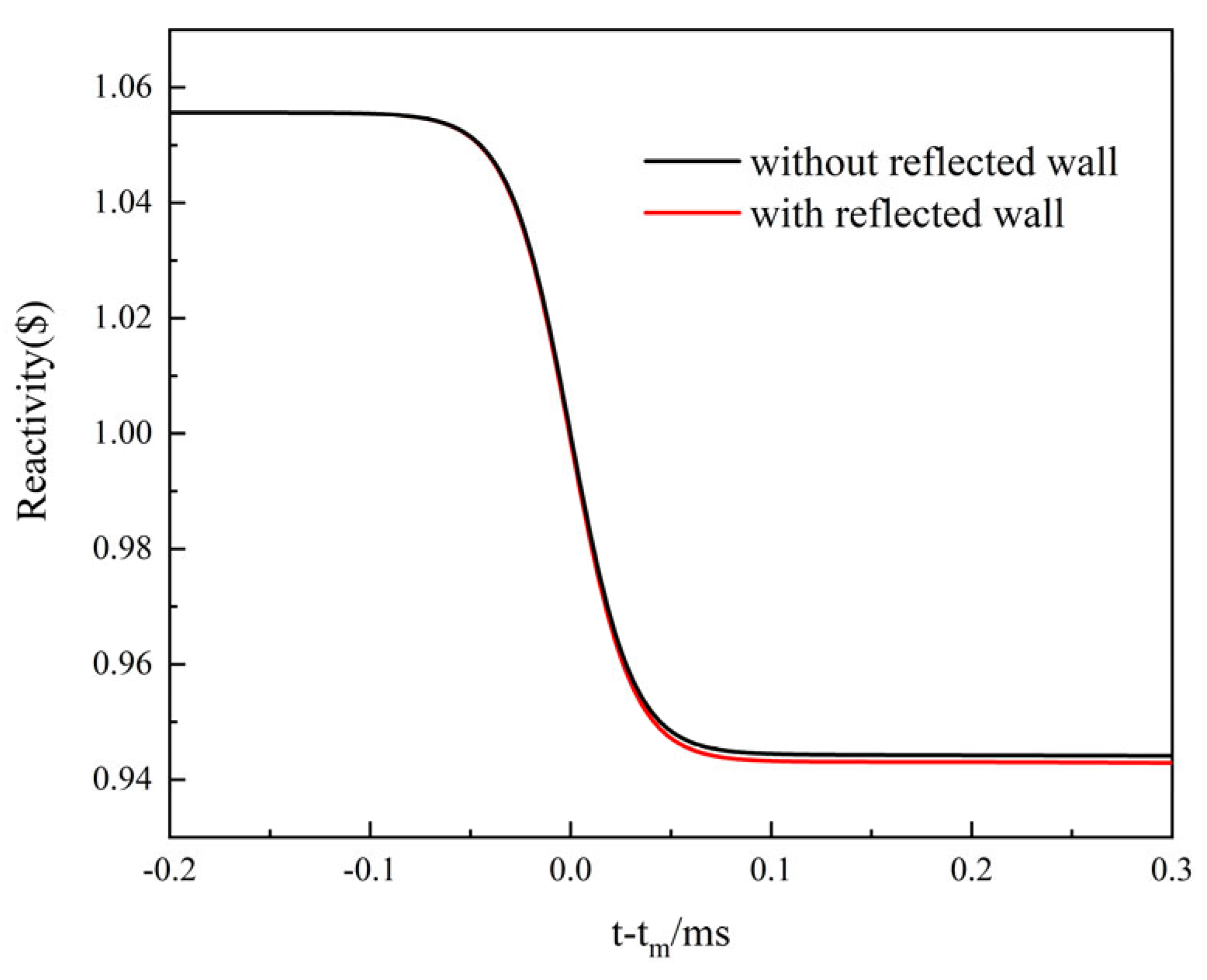
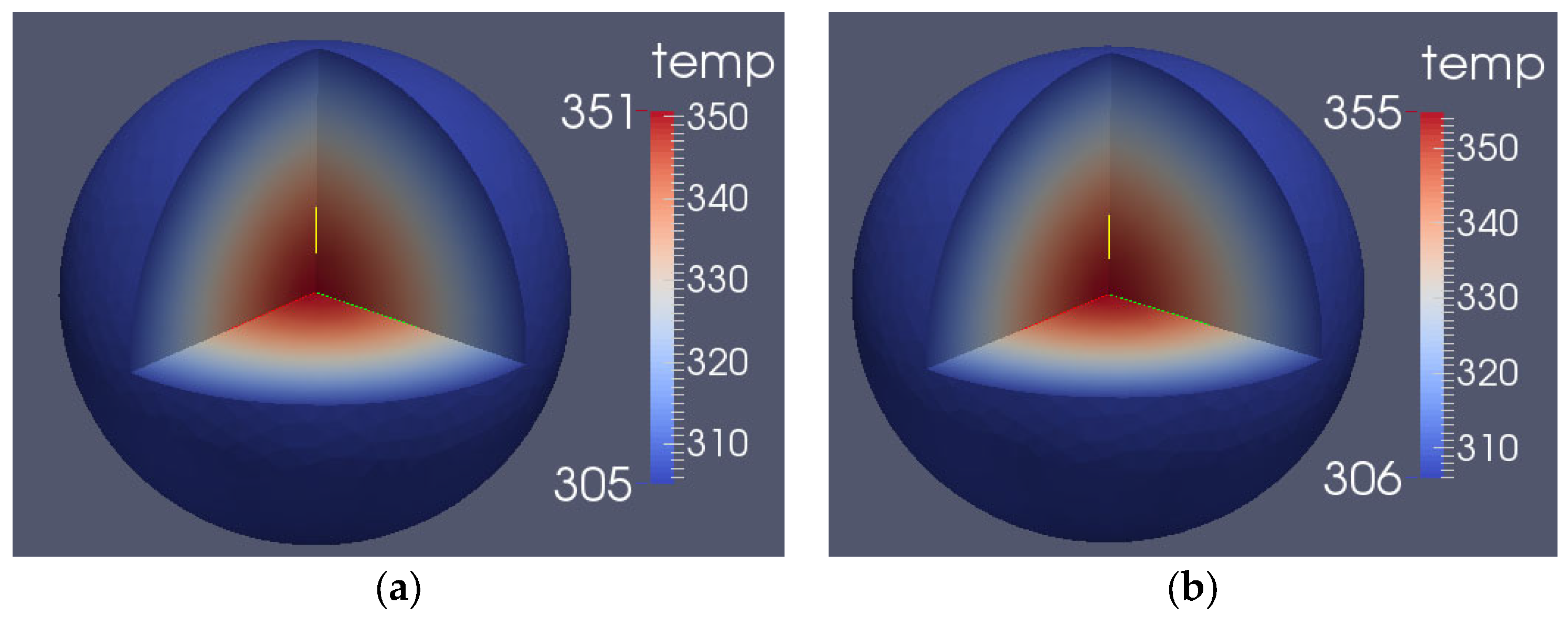
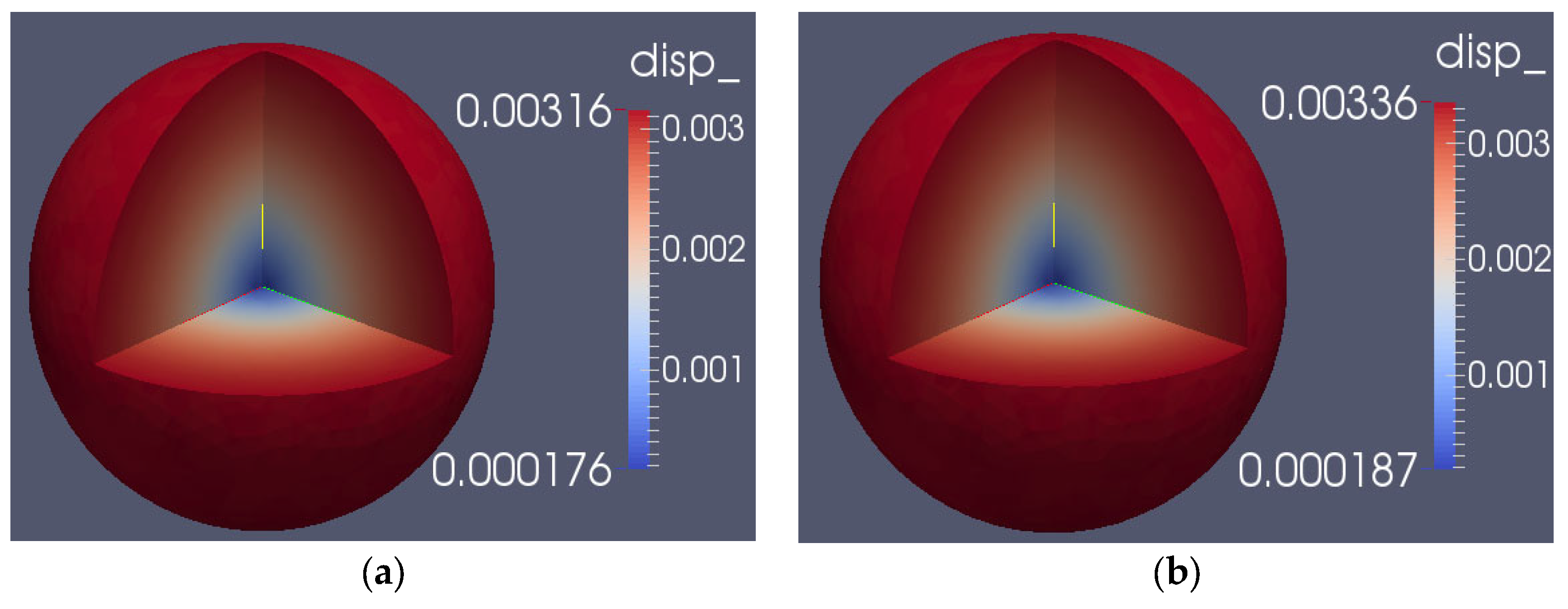
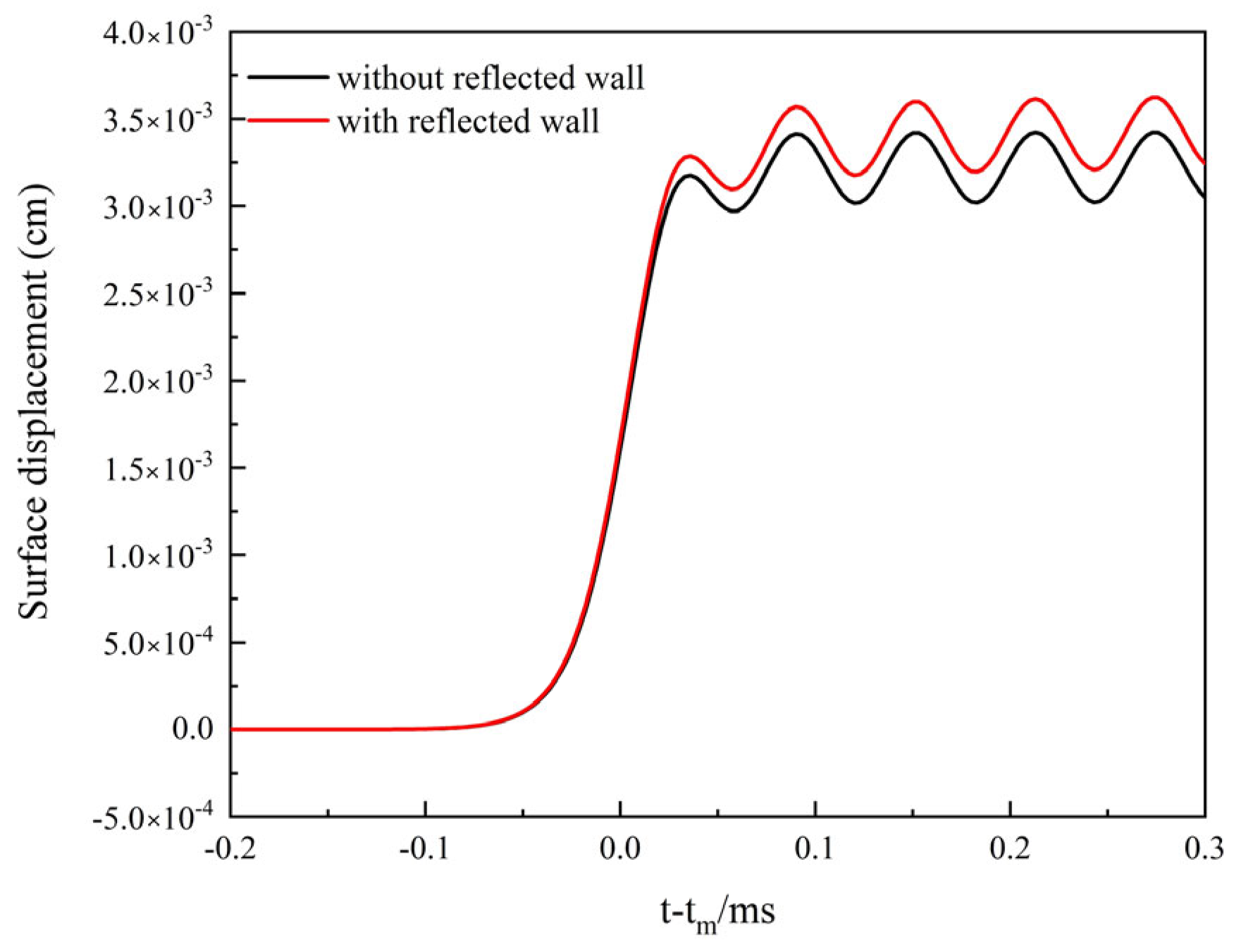
3.3. Reflected Neutrons of Wall
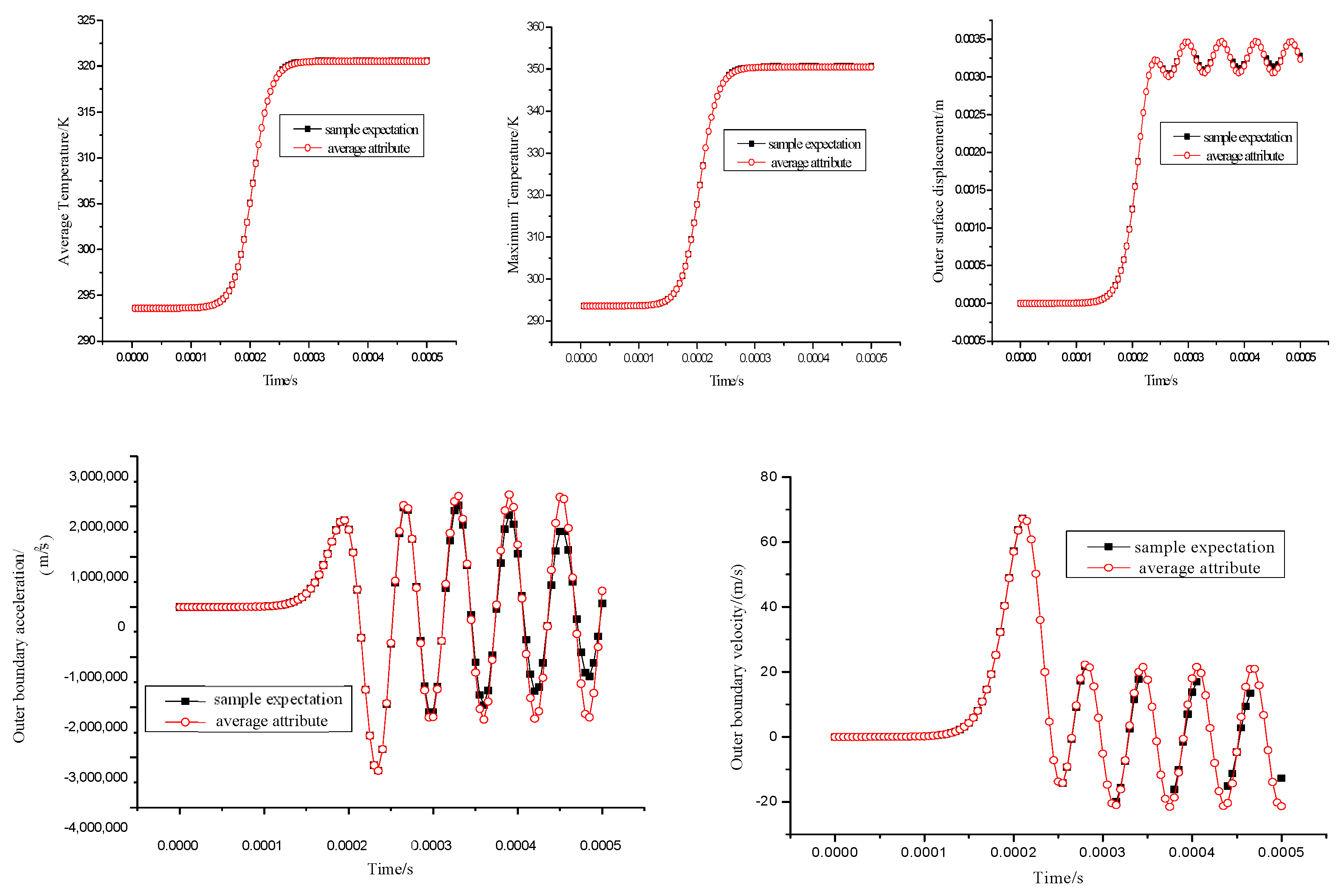
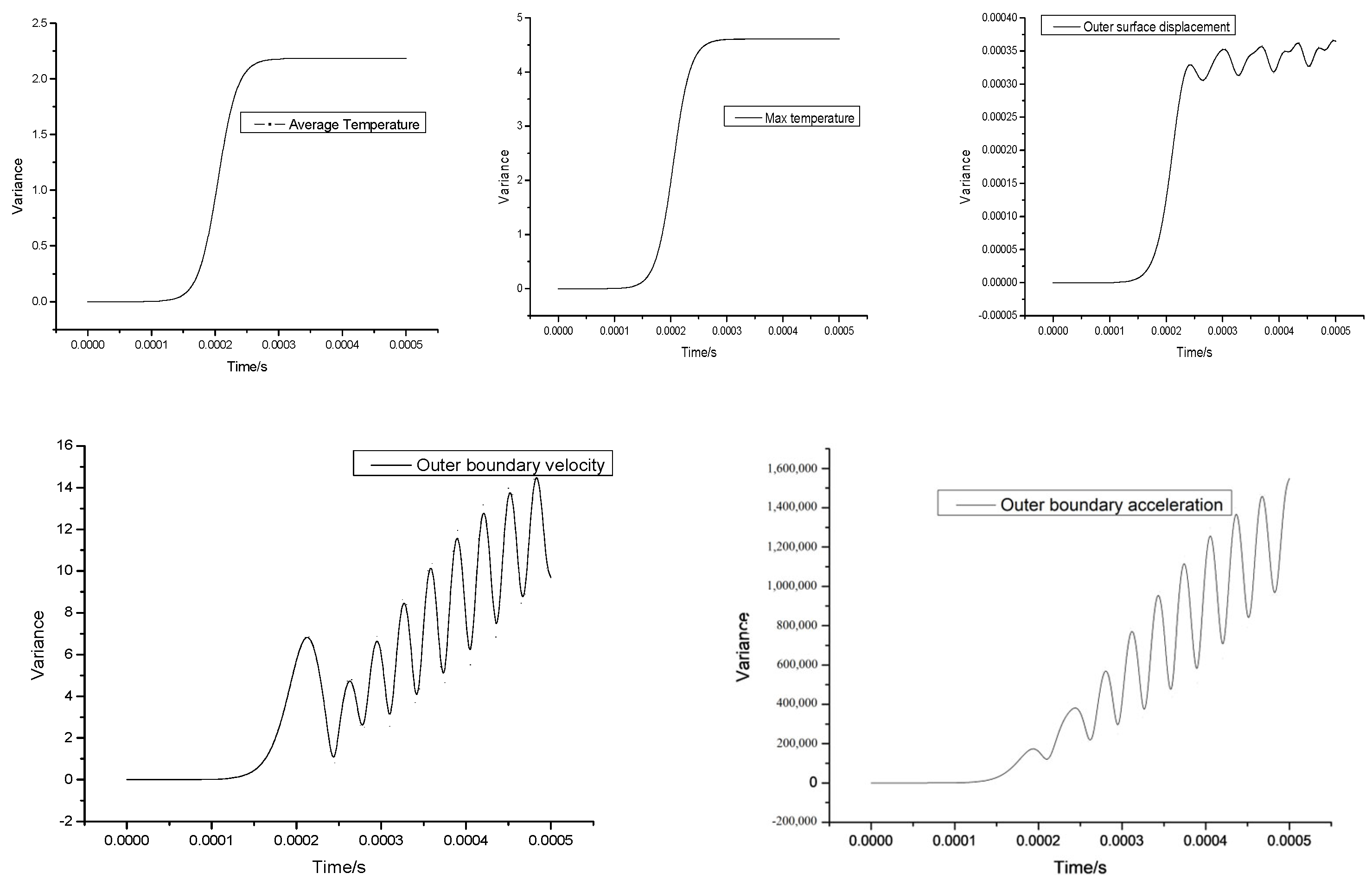
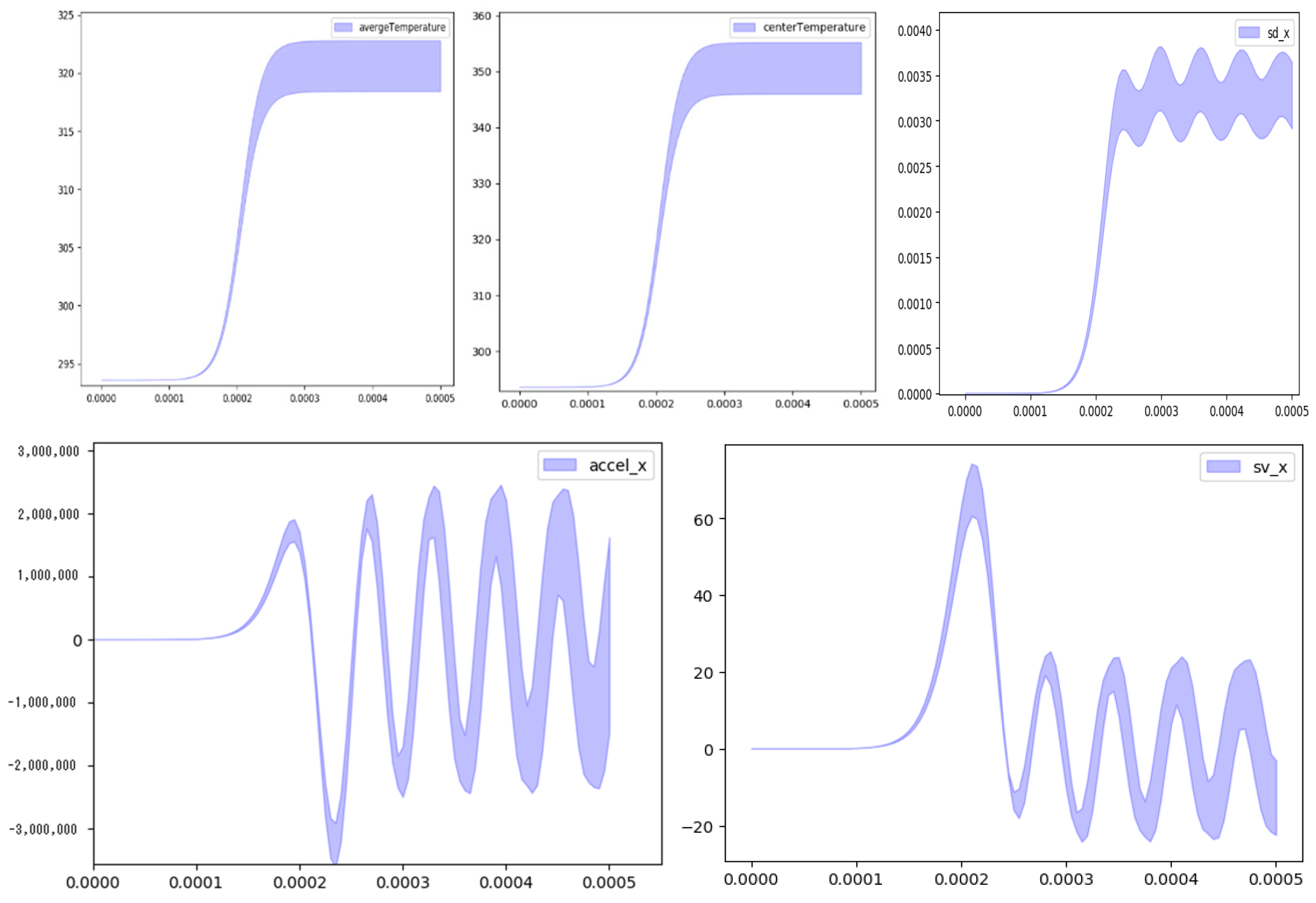
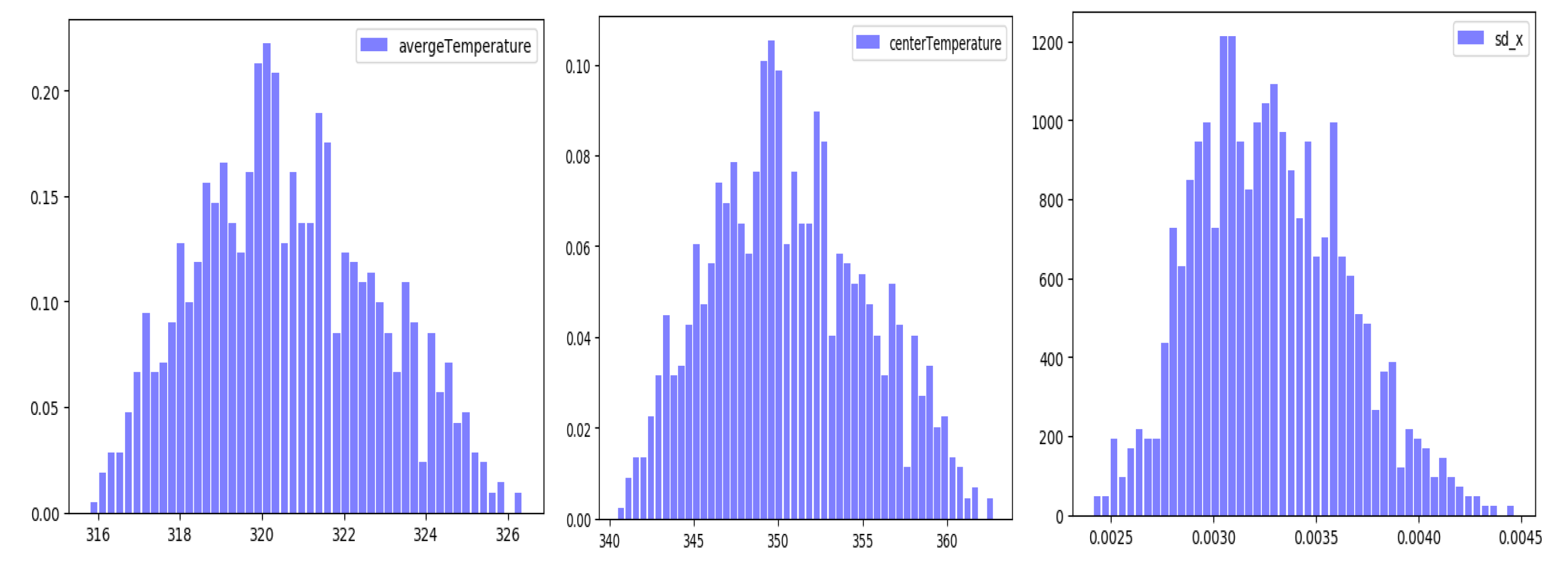
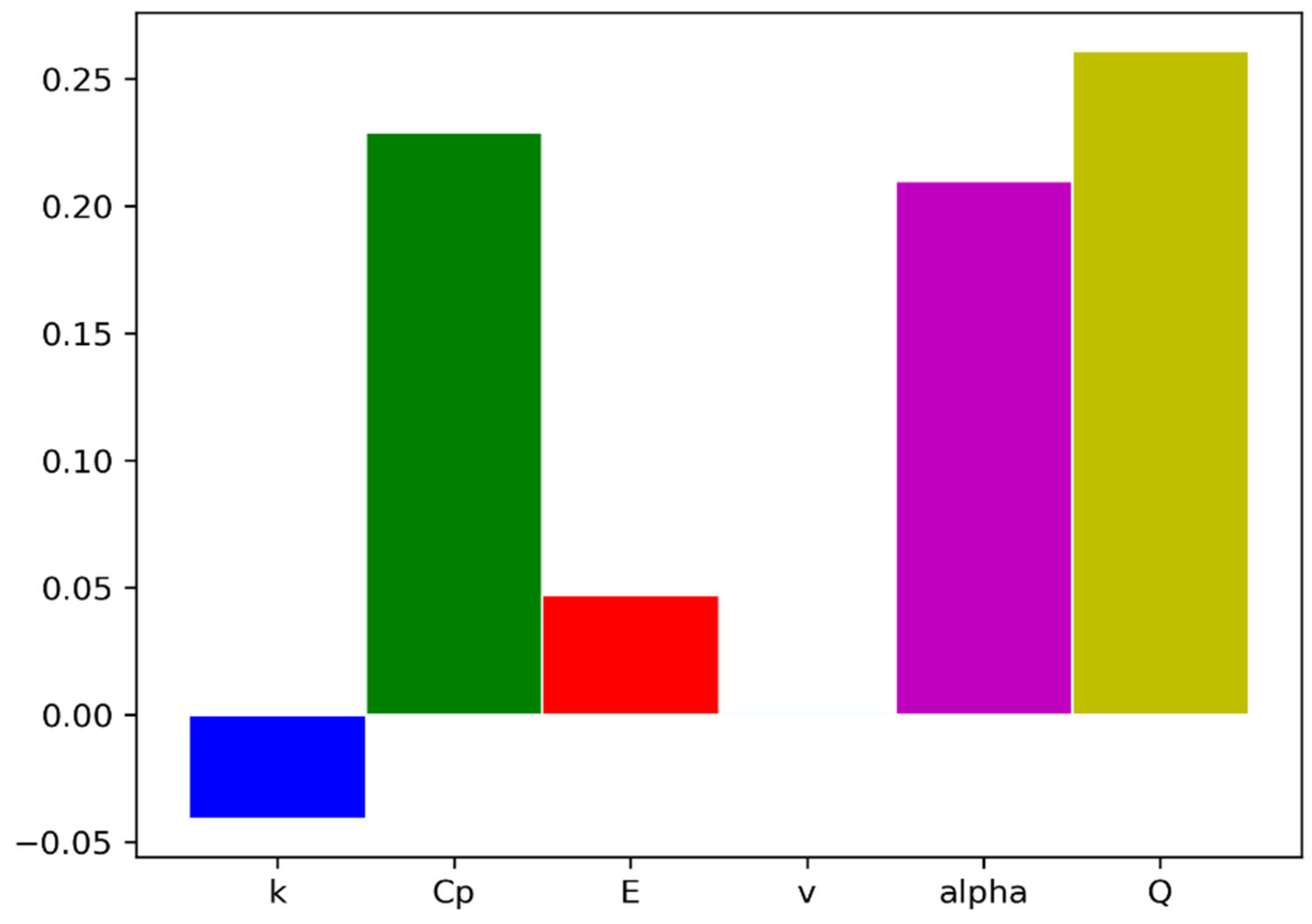
3.4. Sensitivity Analysis
4. Discussion
5. Conclusions
- (1)
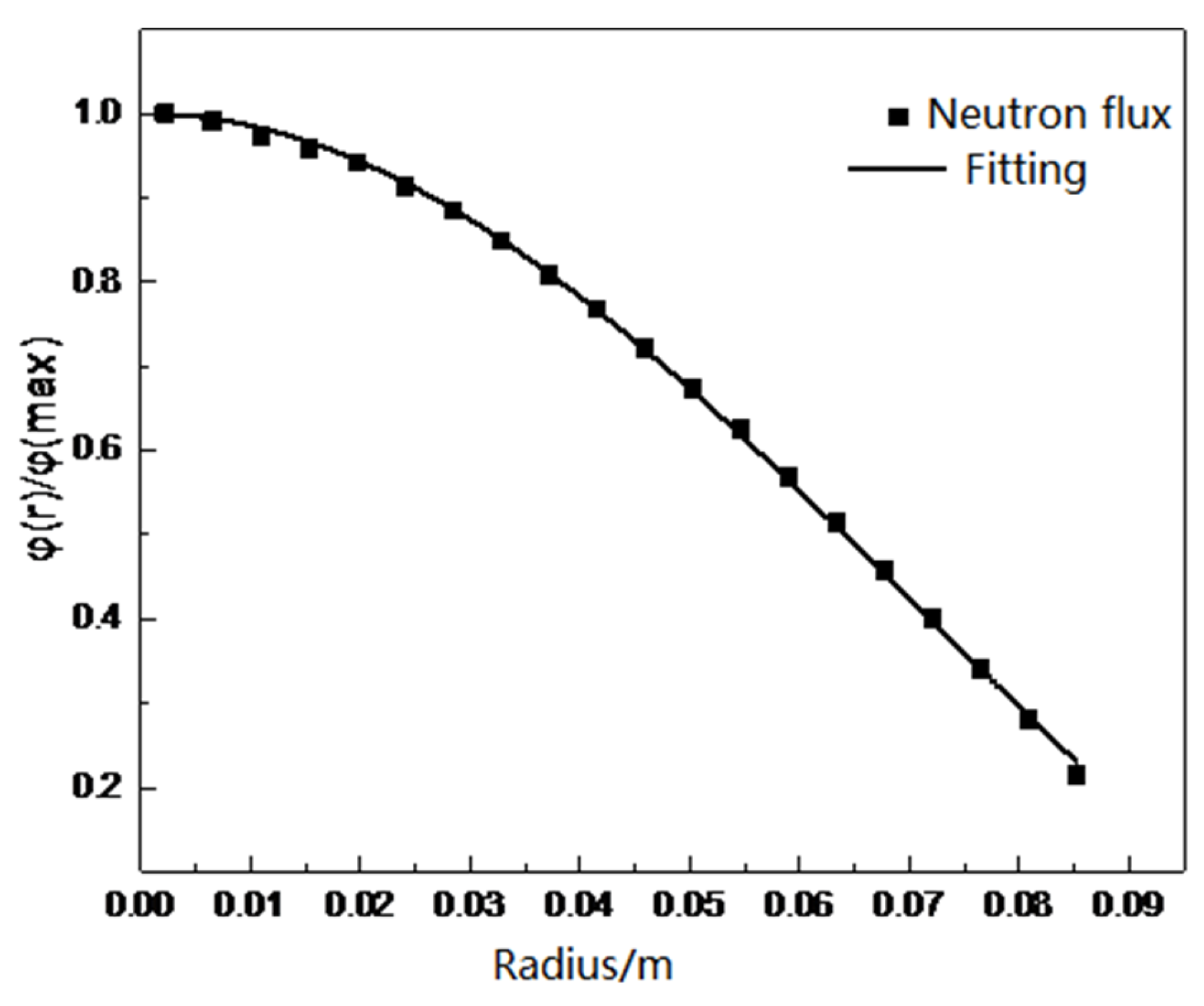
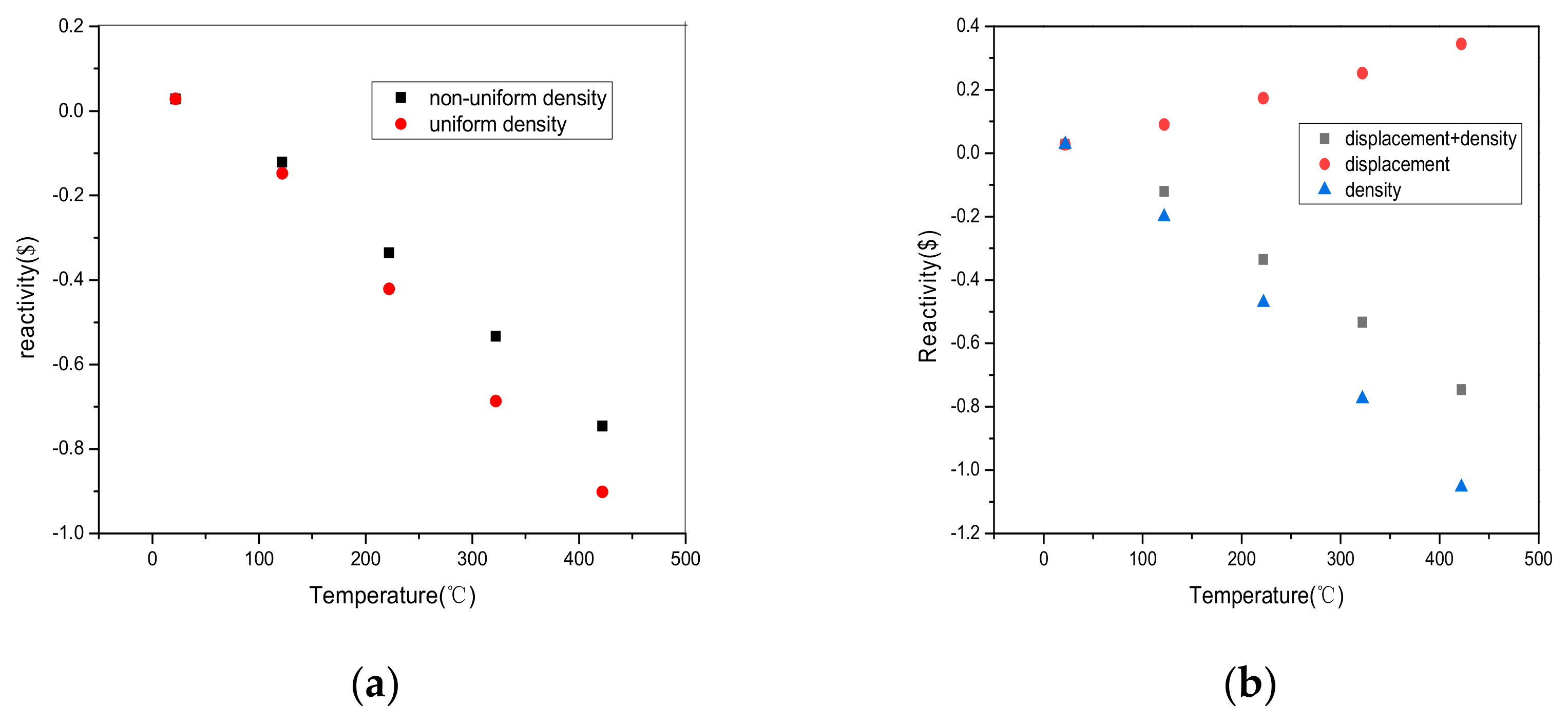
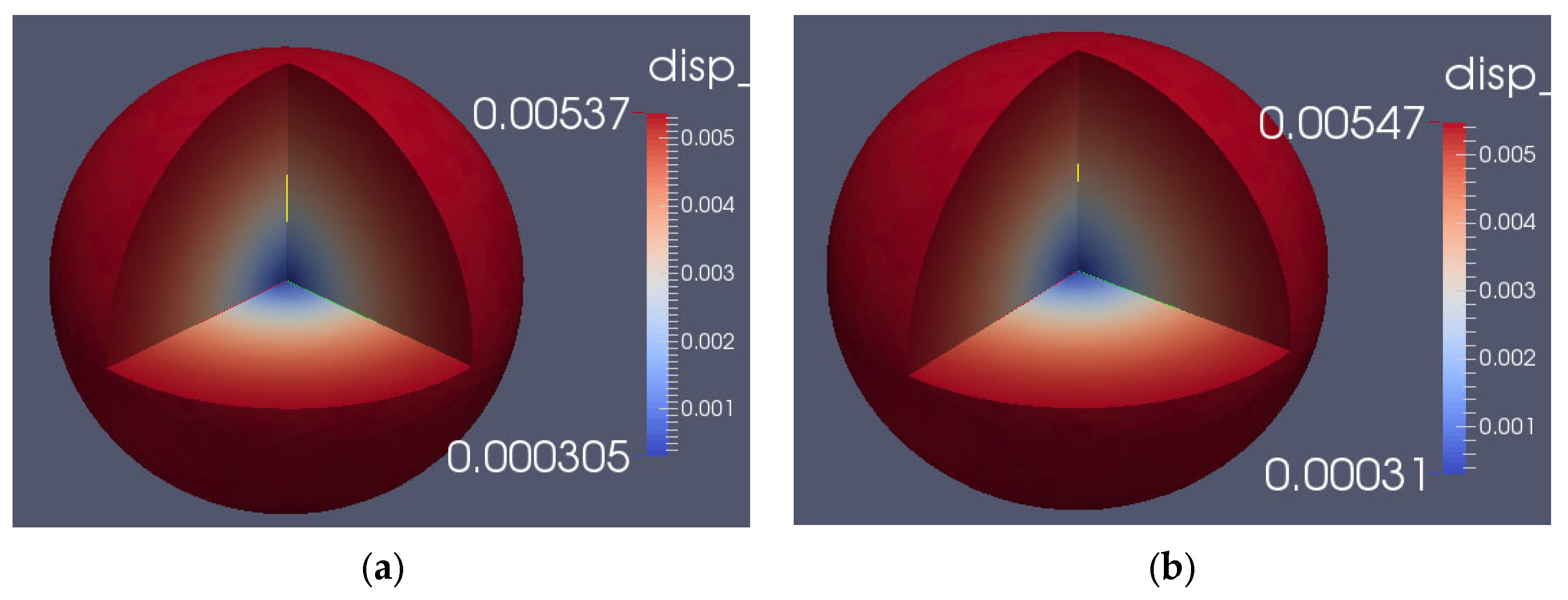
- The calculation of reactivity feedback effects showed that the neutron flux in the core followed a distribution trend with the highest flux in the core and the lowest at the surface. The surface flux was about 21.5% of the center flux. After considering displacement and density, the relative deviation of the steady-state reactivity temperature coefficient was found to be 1.27%. In transient calculations, the relative deviation of the peak fission rate was less than 5%. Additionally, the temperature rises in the core, as calculated using the Multi-App and JFNK methods, were almost the same in transient thermal-mechanical calculations. The displacement of the core increased gradually from the core to the surface, with the vibration trend changing over time. The JFNK method was found to have less time and memory consumption, making it more realistic due to its consideration of the coupling between the temperature field and the stress field.
- (2)
- The inertia effect calculation shows that when the initial period is 11.6 μs, the peak value of the fission rate is 2.78 × 1020/s, and the relative deviation of the experimental value is −1.35%; the average temperature rise and the maximum temperature rise of the core are 47.34 °C and 104.53 °C, which is about 1.3 times of that without considering the inertia effect; The maximum displacement on the outer surface is 7.63 × 10−3 cm which is about 1.43 times without considering the inertia effect. In conclusion, the inertia effect can significantly increase the core fission yield and increase the temperature and displacement of the core.
- (3)
- The wall-reflected neutrons effect calculation shows that when the initial period is 16.2 us, the temperature calculated by considering the reflected neutron effect is higher than that of the unconsidered, and the maximum displacement of the outer surface of the reactor is 3.63 × 10−3 cm, 1.06 times of that without considering the reflected neutrons. The wall-reflected neutrons significantly change the pulse waveform and raise the pulse trailing edge, which in turn raises the fission yield, resulting in elevated core temperature rise and displacement.
- (4)
- The analysis of uncertainty and sensitivity reveals that the external surface displacement is most affected by the specific heat capacity, thermal expansion coefficient, and heat source magnitude factor.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wimett, T.F. Fast Burst Reactors in the USA; Los Alamos Scientific Laboratory: Los Alamos, NM, USA, 1965.
- Paxton, H.C. History of Critical Experiments at Pajarito Site; Los Alamos National Laboratory: Los Alamos, NM, USA, 1983.
- Wang, J.; Liang, W.; Chen, S.; Zhou, H.; Xie, Q.; Fan, X.; Qian, D. Dynamic behavior in fast burst reactor with three-dimensional coupled multiphysics method. Nucl. Eng. Des. 2018, 338, 16–22. [Google Scholar] [CrossRef]
- Wang, J.M.; Gao, H.; Xie, Q.L.; Fan, X.Q.; Qian, D.Z. Development of a display reactivity feedback model for dynamic behavior simulation in fast burst reactor. Nucl. Sci. Technol. 2019, 30, 10. [Google Scholar] [CrossRef]
- He, R.; Deng, M. Experimental Physics of Fast Neutron Critical Devices and Pulse Reactors; National Defense Industry Press: Beijing, China, 2012; pp. 34–37. (In Chinese) [Google Scholar]
- Zhang, C.; Zhou, Q.; Zhu, Q.F.; Xia, Z.D.; Zheng, J.Y. Research on Transient Characteristics Analysis of Metal Nuclear Fuel Systems. At. Energy Sci. Technol. 2016, 50, 2170–2174. (In Chinese) [Google Scholar]
- McLaughlin, T.P. A Review of Criticality Accountants 2000 Revision; Los Alamos National Laboratory: Los Alamos, NM, USA, 2000.
- Liang, W.F.; Xie, Q.L.; Gao, H.; Qiu, D.; Yang, C.D. Coupled calculation of fast neutron pulse reactors based on waveform approximation. At. Energy Sci. Technol. 2016, 50, 2018–2022. (In Chinese) [Google Scholar]
- Wilson, S.C.; Biegalski, S.R.; Coats, R.L. Computational Modeling of Coupled Thermomechanical and Neutron Transport Behavior in a Godiva Like Nuclear Assembly. Nucl. Sci. Eng. 2007, 157, 344–353. [Google Scholar] [CrossRef]
- Liang, W.; Qiu, D.; Yang, C.; Fan, X.; Xie, Q.; Yin, Y. Dynamic Response Simulation of Fuel Elements under Thermal Shock Based on ANSYS. Sci. Technol. Eng. 2014, 14, 51–55. (In Chinese) [Google Scholar]
- Wimett, T.F. Dynamics and power prediction in session bursts. Nucl. Sci. Eng. 1992, 3, 209–236. [Google Scholar] [CrossRef]
- Li, B.; Fan, X.; Deng, M. Dynamic character research of fast neutron burst reactor. Nucl. Electron. Detect. Technol. 2001, 21, 277–280. (In Chinese) [Google Scholar]
- Zhong, L.; Gao, H.; Liang, W.; Li, J. Calculation of inertia effect on metal burst reactors. High Power Laser Part. Beams 2017, 29, 146–149. (In Chinese) [Google Scholar]
- Gao, H.; Song, L.-L.; Li, B. Influence of reflected neutrons of wall on waveform of burst reactors. Acta Phys. Sin. 2018, 67, 172801. (In Chinese) [Google Scholar] [CrossRef]
- Aufiero, M.; Fiorina, C.; Laureau, A.; Rubiolo, P.; Valtavirta, V. Serpent-OpenFOAM coupling in transient model of a Godiva prompt critical burst. J. Perio-Dontal Res. 2015, 37, 154–160. [Google Scholar]
- Wang, Y.; Schunert, S.; Ortensi, J.; Gleicher, F.N.; Laboure, V.M.; Baker, B.A.; De Hart, M.; Martineau, R.C. Demonstration of MAMMOTH Fully Coupled Simulation with the Godiva Benchmark Problem. In Proceedings of the International Conference on Mathematics & Computational Methods Applied to Nuclear Science & Engineering, Niagara Falls, ON, Canada, 13–17 August 2017. [Google Scholar]
- Peterson, R.E. Lady Godiva: An Un-Reflected Uranium-235 Critical Assembly; Los Alamos National Laboratory: Los Alamos, NM, USA, 1953.
- Wimett, T.F.; Engle, L.B.; Graves, G.A.; Keepin, G.R., Jr.; Orndoff, J.D. Time Behavior of Godiva through Prompt Critical; Los Alamos National Laboratory: Los Alamos, NM, USA, 1956; pp. 1–39.
- Gaston, D.; Newman, C.; Hansen, G.; Lebrun-Grandie, D. MOOSE: A parallel computational framework for coupled systems of nonlinear equations. Nucl. Eng. Des. 2009, 239, 1768–1778. [Google Scholar] [CrossRef]
- Shabalin, E.P. Fast Pulsed and Burst Reactor; Pergamon Press: New York, NY, USA, 1979. [Google Scholar]
- Stratton, W.R. Analysis of Prompt Excursions in Simple Systems on Idealized Fast Reactors; Proceeding and International Conference on Peacefue Uses of Atomic Energy; Los Alamos National Laboratory: Los Alamos, NM, USA, 1958.
- Zhang, X. Theoretical calculation of fast-neutron pulsed reactor. J. Chang. Teach. Coll. Tecnol. 1995, 1, 1–12. (In Chinese) [Google Scholar]
- Cohn, C.E. Reflected-reactor kinetics. Nucl. Sci. Eng. 1962, 1, 12–17. [Google Scholar] [CrossRef]
- Li, B.; Lu, Y.; Gao, H. Kinetic effect of scattering neutrons on super-prompt critical system. High Power Laser Part. Beams 2016, 28, 056001. (In Chinese) [Google Scholar]
- Zhang, X.; Zhang, L.; Jiang, X.; He, B.; Wang, L.; Guo, H.; Jiang, D.; Zhu, Y. Study on Influence of Depletion on Neutron Generation Time for Xi’an Pulsed Reactor. Nucl. Sci. Eng. 2019, 39, 357–362. (In Chinese) [Google Scholar]
- Slaughter, A.E.; Prince, Z.M.; German, P.; Halvic, I.; Jiang, W.; Spencer, B.W.; Dhulipala, S.L.N.; Gaston, D.R. MOOSE Stochastic Tools: A module for performing parallel, memory-efficient in situ stochastic simulations. SoftwareX 2023, 22, 101345. [Google Scholar] [CrossRef]
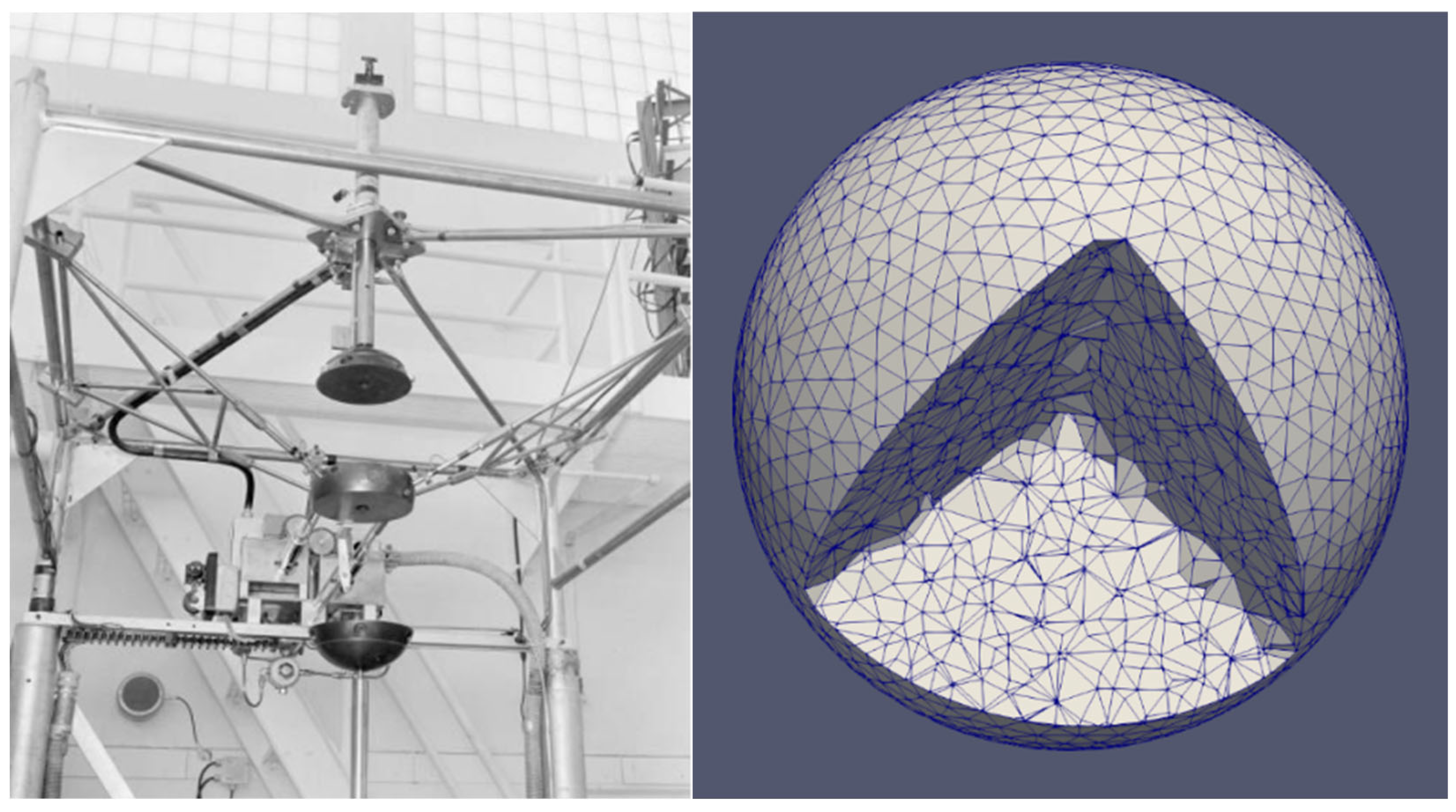









| Parameters | Value | Unit |
|---|---|---|
| Density (ρ) | 18.7398 | g/cm3 |
| Poisson’s ratio (v) | 0.23 | |
| Young’s modulus (E) | 208 | GPa |
| Thermal conductivity (k) | 27.5 | W·m−1·K−1 |
| Thermal expansion coefficient (α) | 1.39 × 10−5 | K−1 |
| Specific heat capacity (c) | 117.72 | J·Kg−1·K−1 |
| Energy Spectrum (MeV) | (%) | Energy Spectrum (MeV) | (%) |
|---|---|---|---|
| 0–0.005 | 0.0103 | 3–4 | 7.1324 |
| 0.005–0.01 | 0.0272 | 4–5 | 3.8565 |
| 0.01–0.02 | 0.1030 | 5–6 | 2.0459 |
| 0.02–0.05 | 0.6152 | 6–7 | 1.0504 |
| 0.05–0.1 | 1.7876 | 7–8 | 0.5047 |
| 0.1–0.2 | 5.1520 | 8–9 | 0.2416 |
| 0.2–0.5 | 17.7245 | 9–10 | 0.1173 |
| 0.5–1 | 22.7261 | 10–15 | 0.1096 |
| 1–2 | 24.0981 | 15–20 | 0.0024 |
| 2–3 | 12.6952 |
| Parameters (Unit) | Value Range | Average Value | Distribution Type |
|---|---|---|---|
| Coefficient (W/m·K) | [0.2475, 0.3025] | 27.5 | Gaussian |
| Specific heat capacity (W/m·K) | [0.105948, 0.129492] | 0.118877 | Gaussian |
| Young’s modulus (Pa) | [1.872 × 105,2.288 × 105] | 2.1008 × 105 | Gaussian |
| Poisson’s ratio | [0.207, 0.253] | 0.2323 | Gaussian |
| Thermal expansion coefficient (1/K) | [1.251 × 10−5, 1.529 × 10−5] | 1.4039 × 10−5 | Gaussian |
| Heat source factor | [0.9, 1.1] | 1.01 | Gaussian |
| Method | Initial Reactivity Period of 16.2 μs | Initial Reactivity Period of 29.5 μs | ||
|---|---|---|---|---|
| Runtime | Memory | Runtime | Memory | |
| JFNK | 252.33 s | 91 MB | 348.25 s | 90 MB |
| Multi-App | 270.08 s | 112 MB | 263.09 s | 118 MB |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, L.; Guo, S.; Hu, T.; Jiang, D.; Zhang, X.; Cao, L.; Jiang, X. Transient Simulation and Parameter Sensitivity Analysis of Godiva Experiment Based on MOOSE Platform. Energies 2023, 16, 6575. https://doi.org/10.3390/en16186575
Wang L, Guo S, Hu T, Jiang D, Zhang X, Cao L, Jiang X. Transient Simulation and Parameter Sensitivity Analysis of Godiva Experiment Based on MOOSE Platform. Energies. 2023; 16(18):6575. https://doi.org/10.3390/en16186575
Chicago/Turabian StyleWang, Lipeng, Shuwei Guo, Tianliang Hu, Duoyu Jiang, Xinyi Zhang, Lu Cao, and Xinbiao Jiang. 2023. "Transient Simulation and Parameter Sensitivity Analysis of Godiva Experiment Based on MOOSE Platform" Energies 16, no. 18: 6575. https://doi.org/10.3390/en16186575
APA StyleWang, L., Guo, S., Hu, T., Jiang, D., Zhang, X., Cao, L., & Jiang, X. (2023). Transient Simulation and Parameter Sensitivity Analysis of Godiva Experiment Based on MOOSE Platform. Energies, 16(18), 6575. https://doi.org/10.3390/en16186575






