Abstract
Proton Exchange Membrane (PEM) water electrolysers have gained attention as efficient and sustainable hydrogen production devices. Understanding their electrical behavior is crucial for optimizing their performance and control strategies. This comprehensive review analyzes both the static and dynamic electrical models, emphasizing the often-neglected dynamic models. Static models reveal steady-state behavior, offering insights into voltage-current characteristics, polarization phenomena, and overall efficiency. On the other hand, dynamic models capture transient responses, enabling an analysis of time-dependent performance and system reactions to input variations, such as flow rate, temperature, pressure, and electrical potential. The review also addresses overlooked diffusion phenomena, crucial for accurate mass transport dynamics and enhancing dynamic model accuracy. Furthermore, the article highlights challenges and research possibilities in electrical modeling, offering insight into future research subjects.
1. Introduction
One of the most serious issues confronting humanity today is the need to reduce greenhouse gas emissions and prevent the effects of climate change while meeting the increasing need for energy to support economic growth [].
Supported by evidence [], it is a proven fact that pollution has led to climate change, culminating in escalated pollution levels and the phenomenon of global warming. The emission of carbon and hazardous gases from vehicles and industries significantly contributes to the progression of global warming []. This environmental shift poses a potential threat to our planet and gives rise to various issues, including health concerns, increased occurrences of floods, and more frequent droughts. As the world grapples with the urgent need to mitigate the greenhouse effect in the coming years, renewable energy sources (RES) have emerged as a promising substitute for carbon-based fuels. Given their intermittent nature, RES, such as ocean energy, wind, solar, thermal, and geothermal power [], possess an inherent unreliability. Furthermore, as the depletion of fossil fuels becomes more pronounced, the necessity for transitioning from nonrenewable to renewable energy sources becomes increasingly imperative.
To address the issue of energy shortage, extra electricity generated during off-peak hours must be stored. Due to their high energy density, high power density, and extended lifespan, electrochemical energy storage (EES) systems, such as rechargeable batteries, fuel cells, supercapacitors (SCs), and hybrid capacitors, have emerged as power sources for electronic devices and vehicles. Batteries, a major energy storage equipment, have been thought to have the capacity to manage and mitigate environmental impact []. SCs provide specific advantages, including increased power density, faster charging and discharging rates (in seconds), and a longer cycle life.
Green hydrogen (GH2) generation utilizing RES-powered electrolysers has increased in recent years due to its benefits over conventional techniques (such as batteries and ultra-capacitors) for long-term storage and delivery. Because of their faster start-up speeds and dynamic load-changing capability, proton exchange membrane (PEM)-based electrolysers are better suited for coupling with RES than alkaline electrolysers []. The intermittent nature of RES has an impact on the performance and operating dynamics of the PEM electrolyser, which must be evaluated and studied in order to make these systems more reliable and safer to use [].
In this context, hydrogen energy has emerged as a possible candidate for easing the transition to a low-carbon society [,]. Hydrogen is a clean fuel with a high energy density and no pollution emissions, making it an appealing substitute for fossil fuels []. Its adaptability extends to a variety of applications, particularly as a transportation fuel [,,]. However, the increasing integration of renewable energy sources into the European electrical network faces a supply and demand mismatch concern [,]. To address this issue effectively, one practical solution is to connect intermittent renewable sources to an electrolysis system, which can convert and store excess electrical energy as hydrogen []. Water electrolysis technology, powered by low-carbon electricity, currently stands as one of the most advanced methods for producing low-carbon hydrogen. Consequently, electrolysis offers a viable approach for large-scale green hydrogen production and electrical energy storage. To solve the challenges associated with connecting intermittent electrical sources to electrolysis systems, modeling plays a crucial and influential role. It helps in understanding the underlying phenomena, designing control strategies, sizing, managing energy, and performing optimization. Therefore, this article aims to provide a comprehensive and detailed review of previous research on the electrical modeling of PEM electrolysis systems available in the literature. The criteria considered for the modeling approach include analytical models, which are based on equations derived from physical laws and have parameters with a physical meaning. These models are also known as semi-empirical models, unlike empirical models that rely on parametric equations derived from mathematical processing of experimental data, and whose parameters lack a physical interpretation.
This article has been structured into different sections. Section 2 focuses on a comprehensive exploration of the fundamental principles of water electrolysis. Moving forward, Section 3 looks into an extensive investigation of various models found in the existing literature, encompassing both static and dynamic models. Within this section, a detailed explanation is provided for all factors involved in the polarization curve, namely reversible, activation, ohmic, and diffusion over-voltages considering both the static and dynamic models. Furthermore, Section 3 includes an examination of Faraday’s efficiency.
Indeed, in reference [], researchers have examined both static and dynamic models. However, the authors of this study only proposed a single equation to illustrate the activation and diffusion over-voltages. In contrast, our paper presents various formulations for these phenomena. Additionally, their dynamic modeling encompassed only a simple equivalent circuit (a simple RC circuit), neglecting diffusion phenomena. In our article, we introduce four distinct dynamic modeling approaches that comprehensively account for these phenomena.
Reference [] presents a compilation of published models focusing on the main equations used to predict cell voltage, including reversible voltage, activation losses, ohmic losses, and mass transport losses. This review aims to provide the main guidelines for beginners on PEM electrolyser modeling, focusing only on the static models without presenting any dynamic models while in our article, both static and dynamic models can be found.
Reference [] provides a thorough examination of current electrochemical, thermal, mass transfer, and fluid models for low-temperature electrolysis setups. While this review is undoubtedly all-encompassing, it does not differentiate between static and dynamic models, and it does not clarify the concepts of dynamic activation and diffusion phenomena.
In Section 4, the exploration of various topics that may draw readers’ interest and contribute to future research, such as discussions on control methodologies and algorithms, diagnosis methodologies, the integration of these technologies with renewable energy sources, and recent challenges in electrical modeling, can be found.
Finally, Section 5 presents the conclusion of this work. It should be underlined that the Appendix A, Appendix B contains valuable material regarding diffusion impedance and diffusion phenomena, enabling readers to develop a comprehensive understanding of this phenomenon. Additionally, the Appendix C provides a specific range of values for physical parameters such as the charge transfer coefficient, exchange current density, and membrane surface that have been used in the literature.
2. Fundamental Principles of Water Electrolysis
Proton Exchange Membrane Water Electrolysis (PEMWE) is an electrochemical process that uses electricity to split water into hydrogen and oxygen. This process is considered to be one of the most environmentally friendly methods for producing hydrogen and oxygen with zero emissions.
The fundamental concept behind water electrolysis can be described using the following equation []:
External energy is required to break the molecular bonds in water and separate its components. The energy required or released during the breaking or building up of chemical bonds is known as the reaction enthalpy, represented by . In general, this quantity is calculated from the sum of the enthalpies of the formation of the products minus the sum of enthalpies of formation of the reactants []:
where:
- change in the reaction enthalpy for this endothermic reaction [;
- Stoichiometric factor for product and reactant;
- : the stoichiometric factors;
- enthalpies of formation of the products [;
- enthalpies of formation of the reactants [.
The process of water splitting is facilitated by the input of electrical and thermal energy. The enthalpy change () for this reaction can be expressed as the sum of the contributions of these driving forces:
where:
- change in Gibbs free energy or free enthalpy of reaction [];
- T: system temperature [;
- molar change in entropy [];
At standard conditions (P = 1 atm, T = 298.15 K), the Gibbs free energy change of the reaction, , is (the reaction is considered as a non-spontaneous process).
We feed water as a liquid reactant to the PEM electrolysis cell, as it is usually operated below the boiling point of water. The overall electrolysis reaction is the combination of two electrochemical half-reactions occurring at the electrodes in an acidic environment, as described by the following equations:
To overcome the limited self-ionization of pure water, an acid is added as an electrolyte, and electrocatalysts are used to decrease the activation energy required for the reaction. To begin the process, a direct current (DC) power source is connected to two inert metal plates, such as platinum or iridium, which are submerged in the aqueous electrolyte as shown in Figure 1. The decomposition of water starts when a DC voltage exceeding the thermodynamic reversible potential is applied to the electrodes.
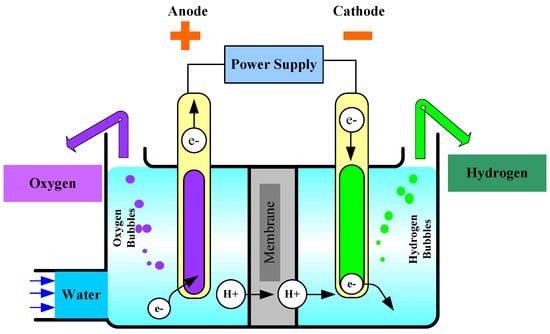
Figure 1.
General principle of a water electrolysis cell.
3. Electrical Models
3.1. Steady-State Methodology
The electric response of an electrolysis cell, and by extension of an electrolysis stack, is typically characterized by a relatively fast response time (e.g., 50 for a PEM stack []) compared to the response time of the overall system []. As a result, the static approach is commonly used to model the electrochemical response of an electrolysis cell. In this approach, it is assumed that the electrical equilibrium of the cell is established instantaneously for each time step of the simulation. Therefore, an electrolysis cell can be modeled as voltage source in series with a nonlinear resistive element. This involves the mathematical description of current–voltage cell (and stack) characteristics, commonly known as polarization curves, which can be described using analytical or empirical models.
3.1.1. Semi-Empirical Models
The mathematical models used to describe the polarization curve of an electrolysis cell are based on physical laws and empirical equations and involve parameters with physical meaning. The equations used in these models are designed to describe each term of the polarization curve, including the reversible potential (), as well as the overvoltage resulting from activation overvoltage (), ohmic overvoltage (), and diffusion overvoltage ().
where:
- cell voltage [V];
- reversible voltage [V];
- : activation overvoltage [];
- ohmic overvoltage [V];
- : diffusion Overvoltage [].
In the following sections, each of these terms will be examined in detail.
Reversible Voltage
The reversible potential is the minimum potential required to induce the water dissociation reaction between the two electrodes of an electrolysis cell. The first term () of Equation (7) corresponds to the reversible voltage under standard operating conditions while the second term accounts for deviations from these standard operating conditions.
where:
- F: Faraday’s constant [];
- reversible voltage at standard conditions [V];
- universal gas constant (8.314) [];
- hydrogen pressure [bar];
- oxygen pressure [bar];
- reference pressure [bar];
- n: number of moving electrons in the chemical reaction (n = 2);
- Gibbs Free Energy at standard condition [].
The standard reversible potential at 298.15 K is . It is important to note that this potential is temperature-dependent and, therefore, can vary at different operating temperatures. Additionally, various correlations based on empirical or thermodynamic tables and Kirchhoff’s laws can be found in different electrolysis models. Table 1 presents various equations that can be used to calculate the standard reversible potential.

Table 1.
Different reversible voltage relationship in the literature.
Table 1.
Different reversible voltage relationship in the literature.
| Reversible Voltage | Equation | Ref. |
|---|---|---|
| (9) | [,,,,,,,] | |
| (10) | [,,] | |
| (11) | [] | |
| (12) | [,] | |
| (13) | [,,,,,,] |
where:
- T: system temperature [];
- reversible voltage (temperature-dependent equations) [V].
In electrical circuit models, the overall reversible potential of the cell is modeled by a voltage source, which depends on the temperature and pressure of the gases. (Figure 2).
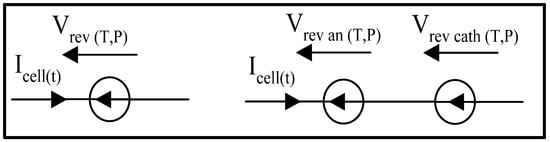
Figure 2.
Electrical model for reversible voltage of cell and electrodes.
In a similar manner, the potential of each electrode can be represented as a voltage source that is influenced by both temperature and pressure. These voltage sources are inherently connected in series. Equations (14)–(19) demonstrate the standard reversible voltage of every electrode.
where:
- Gibbs Free Energy at standard condition [];
- n: number of moving electrons in the chemical reaction (n = 2);
- anode reversible voltage [V];
- cathode reversible voltage [V];
- anode Gibbs Free Energy at standard condition () [];
- cathode Gibbs Free Energy at standard condition () [].
Activation Overvoltage
The activation overpotential in a PEM electrolyser corresponds to the excess voltage required to initiate proton transfer to drive the electrochemical kinetics within the system. When chemical reactions occur at the electrodes, a fraction of the applied voltage is consumed in the process of transferring electrons to or from the electrodes []. The activation overvoltage arises from the kinetics of the electronic charge transfer reactions that take place at the reaction sites on the electrodes []. Various expressions for the activation overpotential can be found in the literature. They are all based on the Butler–Volmer equation, which is a fundamental equation in electrochemistry that describes the relationship between the reaction rate and the applied potential in an electrode–electrolyte interface. Table 2 presents some of these expressions.

Table 2.
Different activation overvoltage relationships found in the literature.
Table 2.
Different activation overvoltage relationships found in the literature.
| Activation Overvoltage | Equation | Ref. |
|---|---|---|
| (20) | [,,] | |
| (21) | - | |
| (22) | [,,,,] | |
| (23) | [,,,,,,] | |
| (24) | [,,,,,] | |
| (25) | [,,] | |
| (26) | [] |
Where:
- cell current density [];
- the leakage current density due to crossover [];
- exchange current density at electrodes [];
- charge transfer coefficient [];
- activation overvoltage at both electrodes [V];
- T: system Temperature [];
- F: Faraday’s constant [];
- k: refers to anode (an) or cathode (cath);
- concentrations of the reaction product and reactant in the vicinity of the electrode [];
- concentrations of the reaction product and reactant in the solution [].
It should be noted that in the preceding Equations (20)–(26), the current density might be replaced by the current since the relationship between these two quantities is , where is the current of the cell [A] and A is active (membrane-electrode) surface area [].
The Butler–Volmer equation can be derived by applying the activated complex theory to the two half-reactions as Equation (20). To obtain a simpler expression for this overvoltage, some assumptions are made. The first assumption is that diffusion is not a limiting process during the reaction. Accordingly, the concentrations in the bulk and near the interface are assumed to be equal (as shown in Equation (21)).
Several hypotheses can be made based on Equation (21) to express the activation overvoltage as a simpler function of the other variables.
One such hypothesis is to consider the charge transfer coefficient to be equal to 0.5, assuming symmetry in the electronic transfer processes and equal distribution of charges as shown in Equation (22). When the activation overvoltage is sufficiently high, the second term of Equation (18) is assumed to be negligible. This results in the Tafel equation, shown in Equation (23) [].
As a result, it is important to note that the validity domain of the Tafel equation is limited to high enough current densities. However, at low current densities, the Tafel equation does not accurately describe the electrochemical behavior, and other equations must be used.
Two other expressions derived from the Butler–Volmer equation are also commonly used to model the activation overvoltage, as shown in Equations (24) and (25), respectively.
By considering the most frequent form of the activation overpotential (Equation (23)), in the static condition, the losses from activation can only be modeled by a controlled voltage source or by a non-linear resistance, which follows a law, . Figure 3 shows the electrical static model of activation phenomena with dissociated electrodes.

Figure 3.
Electrical static models with dissociated electrodes of activation phenomena.
In the case of the model with non-dissociated electrodes, it is possible to take a generalized Butler–Volmer law, which considers the contribution of the two electrodes. The approach consists of first making Tafel’s hypothesis for each electrode, i.e., in considering only the preponderant exponential from the Equation (21). We will have:
with:
where:
- global transfer coefficient [];
- total activation overvoltage [];
- anode charge transfer coefficient [];
- cathode charge transfer coefficient [].
The static model for non-dissociated electrodes is obtained by Equation (27) and can be seen in Figure 4:
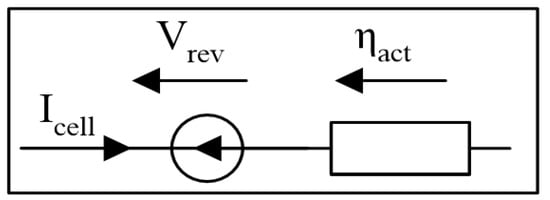
Figure 4.
Electrical Static models with non-dissociated electrodes of activation phenomena.
Ohmic Overvoltage
The ohmic overvoltage arises from non-infinite conductivity of electrolysis cells. Various factors contribute to the sources of ohmic overvoltage, including the electrolyte, electrodes, and contact resistances.
The conductivity of a proton exchange membrane () as can be seen in the Table 3 can be determined based on its temperature (T) and water content (λ) using an empirical expression (Equation (30)). This expression was initially developed in [] and has since been utilized in several models of PEM electrolysis.

Table 3.
Conductivity expression used in different articles.
Table 3.
Conductivity expression used in different articles.
| Conductivity | Equation | Ref. |
|---|---|---|
| (30) | [,,,,,,,,,] |
Ohmic overpotential can be modeled by implementing the following equations (the most frequently used equations):
where:
- membrane overvoltage [V];
- electronic overvoltage [V];
- electronic resistance [];
- L: length of the path of the electrons [cm];
- : electrode material resistivity [m];
- the electrode cross-sectional area [];
- Ionic resistance [];
- : thickness of the membrane [cm];
- : conductivity of the membrane [];
- T: temperature of the cell [K];
- : hydration rate [−];
- active surface area [].
In some of the literature [], it can be seen that the hydration rate is temperature-dependent:
The most common approach for modeling the ohmic overpotential in the literature is to use a linear resistance, as seen in Figure 5.
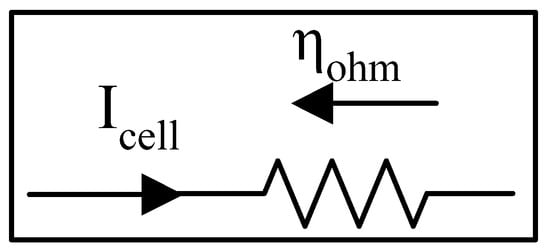
Figure 5.
Circuit model of ohmic overvoltage.
Diffusion Overvoltage
At high current densities, the reaction is no longer solely influenced by electronic transfer, but it is also affected by the movement of matter. This phenomenon is referred to as diffusion overvoltage, and it can significantly impact the shape of the polarization curve. Appendix A contains further material about the notion of diffusion. Table 4 and Table 5 provide us with the summary of the relationships between the concentration ratio and current in one layer and two layers diffusion phenomena.

Table 4.
Relationships between concentration ratio and current in one-layer diffusion phenomena.
Table 4.
Relationships between concentration ratio and current in one-layer diffusion phenomena.
| Situation | Species | Formula | Equation |
|---|---|---|---|
| Case of a species x produced and evacuated from the electrolyser | and | (35) | |
| Case of a species y introduced and consumed within the electrolyser | (36) |
Where:
- stoichiometric coefficients of the species x and y. [];
- concentrations of species x and y, at the catalytic sites (cat is stand for catalytic);
- diffusion length [m];
- diffusion coefficients of the species x or y ;
- cell current [];
- A: active surface area [];
- diffusion limit currents [A];
- concentrations in the channel [], determined from the ideal gas law. [].

Table 5.
Relationship between concentration ratio and current in two-layers diffusion phenomena.
Table 5.
Relationship between concentration ratio and current in two-layers diffusion phenomena.
| Situation | Species | Formula | Equation |
|---|---|---|---|
| Case of a species x produced and evacuated from the electrolyser | and | | (37) |
| Case of a species y introduced and consumed within the electrolyser | | (38) |
Where:
- diffusion length of zones A and B [m];
- , diffusion limit currents effective respectively in zones A and B [A];
- diffusion limit current effective in zone A in the case of operation of the electrolyser in the opposite mode if there was only one zone [A];
- effective diffusion coefficients for zones A and B [].
More information can be found in Appendix A.
Impact on the Voltage
To investigate the influence of diffusion on the cell voltage, we consider the general equation of the Butler–Volmer Equation (20), and by focusing on the dominant term of this equation (the first term) and based on Equation (36), the relationship between anode current and overvoltage can be represented as:
We only consider the exponential of the dominant reaction by considering = and setting k = an.
By convention, the currents entering the electrodes are counted positively and the currents leaving negatively. In the case of the electrolyser, the current at the cathode is outgoing: it will be counted negatively. The current at the anode is entering: it will be counted positively.
We demonstrate the diffusion overvoltage:
When modeling static diffusion phenomena, the literature typically considers matter transport phenomena as global, without explicitly determining the individual contributions of each electrode.
Steady-state diffusion phenomena are modeled with a non-linear component, as shown in Figure 6.
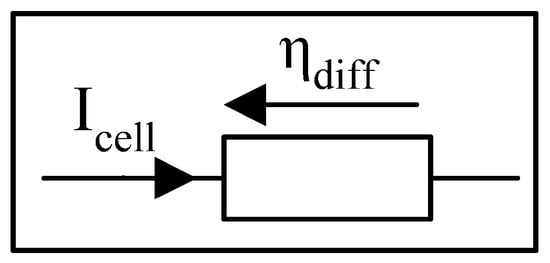
Figure 6.
Circuit model of steady-state diffusion phenomena.
Table 6 shows various descriptions of this phenomenon can be found in the literature.

Table 6.
Various descriptions of diffusion overvoltage.
Table 6.
Various descriptions of diffusion overvoltage.
| Formula | Equation | Ref. |
|---|---|---|
| (43) | [,] | |
| (44) | [] | |
| (45) | [,,,,] |
Summary of the Static Approach
The voltage of a PEM cell can be given in steady-state by:
Figure 7 shows a circuit model of a static PEM cell with non-dissociated electrodes.
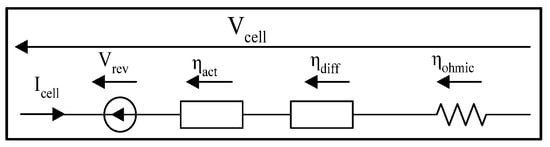
Figure 7.
Static circuit model with non-dissociated electrodes of a PEM Electrolyser.
When dissociating the electrodes, the cell voltage can be expressed by:
Table 7 shows the equations that can be used for different voltages in Equations (46) and (47):

Table 7.
Guidelines for using the equations presented from the “Reversible Voltage” section to the “Summary of the Static Approach” section.
The diffusion losses and the ohmic losses are given by the same expressions as in the model with non-dissociated electrodes. Figure 8 presents a dissociated electrode circuit model of a static PEM cell.
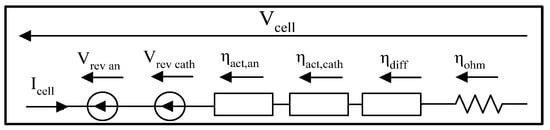
Figure 8.
Static circuit model with dissociated electrodes of a PEM Electrolyser.
3.1.2. Empirical Models
The electrochemical response of an electrolysis cell can be modeled using static modeling approaches, which can be either analytical or empirical. Analytical models have been reviewed extensively, but there are also empirical models available. Empirical models provide a mathematical description of the polarization curve using parameters that are empirically fitted and may not have a physical meaning. Although their structures are similar to analytical models, they are considered empirical because of their non-physical parameters. One of the most well-known empirical models is Ulleberg’s model []. This model involves fitting several parameters, which allows for the impact of temperature on the current–voltage characteristic of the cell to be considered.
where:
- Fitting parameters.
In the first instance, this model was initially developed to describe an alkaline electrolysis stack. Subsequently, several authors have used it to describe both PEM and alkaline electrolysers. Table 8 presents different empirical electrochemical models in the literature.

Table 8.
Various empirical electrochemical models.
Table 8.
Various empirical electrochemical models.
| Empirical Electrochemical Models | Electrolysis Technology | Equation | Ref. |
|---|---|---|---|
| Alkaline | (49) | [,] | |
| Alkaline | (50) | [] | |
| Alkaline | (51) | [] |
Where:
- ,, ,, s, , and are constants obtained from the experimental data;
- P is the pressure of system [bar];
- is the cell current [A];
- temperature dependence of the parameters and was gained by fitting with a second-order polynomial.
3.2. Faraday Efficiency
An electrolysis system is primarily responsible for converting electrical energy into chemical energy under the form of hydrogen. Electrochemical models form the foundation of electrolysis system modeling, allowing us to establish a relationship between input electrical power and output hydrogen flow. By applying matter balances to an electrolysis cell, we can demonstrate that the amount of hydrogen produced by the cell is proportional to the current passing through it []. This equation, known as Faraday’s Law, relates the molar flow of hydrogen () with the current , the number of charges (n = 2), and the Faraday constant (F):
To account for the fact that not all electrons participate in the electrolysis reaction due to factors such as leak currents and parasitic reactions, this equation can be modified by introducing the concept of Faraday efficiency [] or current efficiency () as:
where:
- molar flow of hydrogen [];
- current efficiency [−].
Table 9 shows the various representations of Faraday efficiency that have been used in the literature.

Table 9.
Different Faraday efficiency expression found in the literature.
Where:
- A: cell area [];
- empirical coefficients.
3.3. Dynamic Methodology
The electrical response of an electrolysis cell, while fast, is not instantaneous because of internal storage phenomena, such as double layer capacitance and species diffusion limitation and accumulation [,,]. To model these phenomena, electrolysis cells are described using an equivalent electrical circuit, and impedance parameters are fitted to reproduce their electrical behavior. These models are useful to study the overall impedance of the cell and to link internal phenomena, such as species diffusion and double-layer capacitance, to their impact on the electrical response. Additionally, these models can be used to study the coupling of electrolysis stacks with converters. Numerous models have been developed [,,,,,,] to model these internal phenomena.
Various types of equivalent electrical dynamic models can be found in the literature for both non-dissociated and dissociated electrodes.
These models include:
- The Large signal dynamic model [,];
- The Small signal (Impedance model) [];
- The Warburg and Randles circuits [];
- The Simple equivalent electrical model [,,].
In the upcoming sections, we will discuss each model. However, it is crucial to first provide a clarification on the distinction between the large signal model and the small signal model.
It is worth mentioning that the circuit models discussed here differ significantly from the impedance models typically obtained using impedance spectroscopy methods. The impedance model obtained through such methods is a small signal model that is only valid around a specific operating point. In contrast, the electrical circuit presented here is a large signal model that can handle high amplitudes or high-power levels.
3.3.1. Large Signal Dynamic Models
Refs. [,] proposed the large signal dynamic models based on the equivalent circuits shown in the Figure 9 and Figure 10.
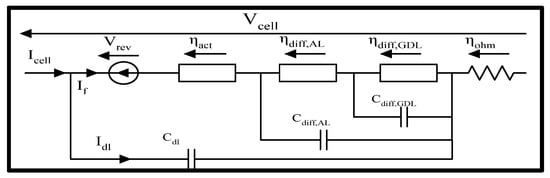
Figure 9.
Large signal dynamic model with non-dissociated electrodes and two diffusion layers.
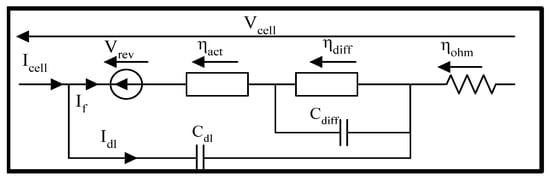
Figure 10.
Large signal dynamic model with non-dissociated electrodes and one diffusion layer.
In the following figures two layers can be seen regarding diffusion phenomena, Gas Diffusion Layer (GDL) and another for the Activation Layer (AL). More details can be found in the Section 3.3.3.
More specifically, ref. [] utilized the circuit diagram shown Figure 11, which incorporates the diffusion of hydrogen into the model.

Figure 11.
Large signal dynamic model of an electrolyser with hydrogen diffusion.
Examining the double layer and diffusion phenomena is crucial for a better understanding of the model. We will explain both phenomena in the following section.
3.3.2. Dynamics of Activation Phenomena
In every electrochemical device, there exists a spontaneous phenomenon known as the electrochemical double layer at each electrode/electrolyte interface. This phenomenon involves the accumulation of charges with opposite signs on either side of the interface, forming a true capacitor in the electrostatic sense of the term.
To model this phenomenon for each electrode, a capacitor C is connected in parallel with the activation and diffusion processes. This capacitor C represents the storage of electrical potential energy and plays a crucial role in determining the dynamics of the activation overvoltage.
Figure 12 shows a dynamic model with non-dissociated electrodes of activation and electrochemical double layer phenomena. is the current delivered to the double layer phenomena, and is the voltage across the capacitor . It should be noted that, for examining the dynamic of activation phenomena, the effect of two others phenomena have been neglected.

Figure 12.
Circuit model of coupling activation and double layer phenomena.
The relation between the current and voltage will be calculated:
where:
- : capacitive current of the electrochemical double layer [A];
- : double layer capacitance [F].
Figure 13 shows the dynamic model with dissociated electrodes of activation and electrochemical double layer phenomena.
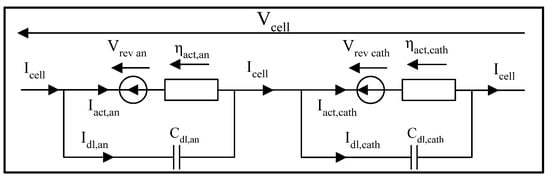
Figure 13.
Dynamic model with dissociated electrodes of activation and electrochemical double layer phenomena.
The capacitance of the double layer in an electrolyser can be approximated by []:
where
- d: distance between the electrode and the electrolyte [m];
- ε: electrical permittivity [];
- : active surface of electrode [].
This expression assumes that the double layer behaves as a parallel plate capacitor, with the electrode and the electrolyte acting as two plates. The capacitance depends on the electrical permittivity, the surface area of the electrode, and the distance between the electrode and the electrolyte. This expression provides an approximate value for the capacitance, as the behavior of the double layer is complex and depends on many factors such as the type of electrolyte, the electrode material, pores geometry, electrolyte concentrations [] and the applied voltage.
We have never yet encountered in the literature the consideration of an internal resistance for the double layer capacitor in the case of electrolysers. The definition of this electrochemical double layer capacitor can exclude the existence of an internal resistance.
As shown in Figure 14, the electrochemical double layer “encompasses” the activation phenomena. Indeed, according to [], the total current, when an electrochemical reaction takes place at the electrode–electrolyte interface, is equal to the sum of the activation current and the capacitive current of the double layer.
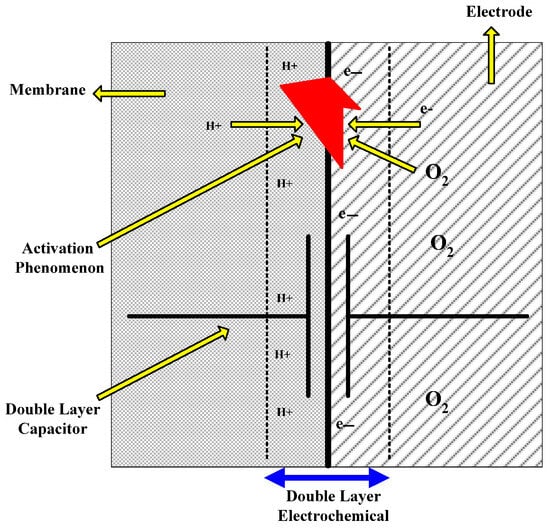
Figure 14.
Phenomena of activation and electrochemical double layer at the cathode.
According to this description of the activation and double layer phenomena, the elements modeling, respectively, the reversible potential and the losses by activation will be in parallel with the double layer capacitor. Given that the element modeling the reversible potential of the electrodes is a voltage source, the element modeling the losses from activation cannot be a voltage source. This element can be a driven current source or a nonlinear resistor. This element must not impose the voltage: this is the role of the double layer capacitor.
3.3.3. Dynamics of Diffusion Phenomena
Several authors have adopted the approach proposed in [] to associate dynamics with diffusion phenomena. Fontes [] demonstrated that for a fuel cell, starting from the small signal impedance modeling of a diffusion phenomenon, it is possible to arrive at a large signal pattern. By starting from an dipole, an dipole is obtained, which associates a voltage-controlled current source I_diff with a C_diff pseudo-capacitor in parallel as can be seen in Figure 15. The term “pseudo-capacitor” is used because it is a fluidic/electrical analogy. The pseudo-capacitor provides an image, in the electrical domain, of the volumetric storage of the concerned species in the zone of interest [].
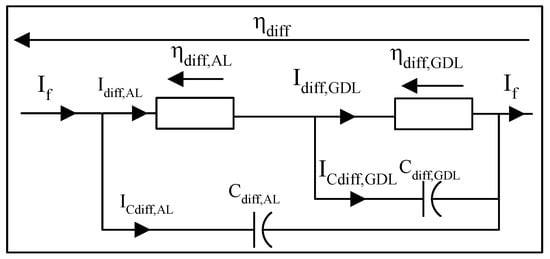
Figure 15.
Electrical model nesting activation layer and gas diffusion layer.
In terms of nesting the circuit models of slow diffusion phenomena and fast diffusion phenomena, many studies maintain the proposal made in [], namely to nest the dipole in the dipole.
In the literature, there are two main dynamic models for diffusion phenomena:
- The first model adopts a single equivalent diffusion layer.
- The second model adopts two distinct diffusion layers, one for the Gas Diffusion Layer and another for the Activation Layer.
Dynamic model of diffusion phenomena with a single equivalent layer
In Appendix A, the explanation of the diffusion phenomena has been provided, but in this section, we will concentrate solely on the dynamic aspect of diffusion. The dynamic circuit model of diffusion phenomena with an equivalent layer is developed in Figure 16.
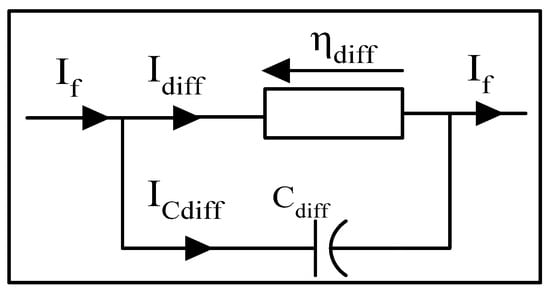
Figure 16.
Diffusion overvoltage models.
Based on the equations presented in Impact on the Voltage section, we can write:
where:
- identified experimentally ;
- diffusion resistance ;
- capacitor of the diffusion phenomena [F];
- : diffusion curent [A];
- : effective diffusion coefficient [];
- diffusion length [m].
Remark 1:
Remark: How the resistance and capacitor of the diffusion phenomena is obtained can be found in Appendix B.
Dynamic model of diffusion phenomena with double equivalent layer
The diffusion overpotential in this case can be calculated by []:
The capacitor and resistance of the activation layer and gas diffusion layer can be expressed as:
The diffusion phenomena in the activation and diffusion zones present a strong coupling. Following a variation of the current, the concentration profile varies first in the activation layer AL, then in the layer GDL. The diffusion models of the respective layers are, therefore, nested as shown in Figure 17.
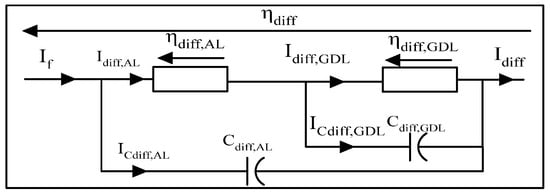
Figure 17.
Diffusion phenomena with double equivalent layer.
3.3.4. Summary of Large Signal Dynamic Models
In the literature, different types of large signal dynamic models can be found based on:
- The dissociated or non-dissociated electrodes;
- The proton transfer;
- The number of diffusion layers (AL, GDL);
- The consideration of parasitic phenomena.
In this context, Table 10 can provide us with the complete information regarding to the mentioned classification.

Table 10.
Comparison of two references in terms of large signal classification.
3.3.5. Small Signal Dynamic Model
The large signal dynamic models are quite complex to manipulate. Therefore, the literature often resorts to use the small-signal dynamic models or impedance models. Essentially, this involves operating around a specific operating point and studying the tangent behavior, which is equivalent to deriving the large dynamic signal model.
By placing the electrolyser around an operating point, the circuit shown in Figure 18 can be obtained []. Finally, an inductance, , can be seen, in the model which translates the inductive behavior that is systematically found at high frequencies during experimental impedance measurements.
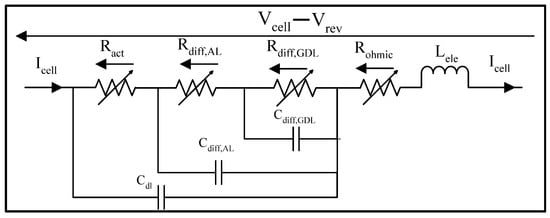
Figure 18.
Small-signal dynamic model of an electrolyser directly derived from the high-signal dynamic model.
The existing small signal and large signal models are effective for medium and low frequencies, but they are not as effective at high frequencies due to the dynamic behavior of the electrodes in an electrolyser.
The impedance of an electrolyser can be modified to include non-integer powers (nip) [] in order to create a more general expression, which is given as:
Remark 2:
The capacitors and resistances of the model can be found in the Equations (67) and (68).
To set orders of magnitude []:
- is typically 0.5;
- : takes values between 0.7 and 1;
- is typically 1;
- take quite varied values between 0.3 and 1.
3.3.6. Warburg and Randles Circuits
An equivalent electrical model based on the Randles–Warburg cell can be found in the literature []. These models consist of electrical components, such as:
- The membrane resistance, which accounts for the ohmic losses;
- The charge transfer resistance, which reflects the activation losses;
- The double layer capacitance, which represents the capacitance created by the applied electric field across the collector plates of the electrolyser;
- The Warburg impedance, which accounts for the concentration losses.
In order to model the cell voltage described in Equation (6), we can use an electric circuit represented in Figure 19a. This circuit consists of a series of Randles models [], each model comprising a combination of electrical components. The anode and cathode regions of the cell are associated with Warburg impedances, represented by and , respectively, which account for the diffusion of gas in these regions. The charge transfer resistances in the anode and cathode regions are denoted as and , respectively. Additionally, the anode and cathode regions are characterized by their respective double layer capacities, and . Finally, the resistance of the membrane separating the anode and cathode regions is represented as . The simplified model is shown in Figure 19b.
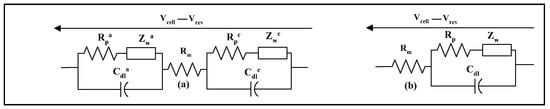
Figure 19.
Electrolyser electric models: (a) complete model; (b) simplified model.
The Warburg impedance, defined as []:
can be conveniently approximated using an RC-impedance transfer function:
with:
This approximation is illustrated in Figure 20, which shows the approximated Warburg impedance denoted as . To model , we need to consider four parameters:, , , and . These parameters correspond to the dimensionless Warburg coefficients, , , , and , respectively.

Figure 20.
Equivalent circuit: Randles–Warburg cell.
From Equations (74) and (75), it is seen that:
where:
- is the diffusion resistance ];
- is the diffusion capacitor [F];
- is the diffusion time constant [s];
- is the Warburg impedance ];
- The coefficients , , , and were estimated by parameter fitting and were computed by means of a Levenberg–Marquardt algorithm.
3.3.7. Simple Equivalent Electrical Model
In recent years, some authors [,,] developed a method to represent the electrolyser as a basic electric circuit, including two resistance-capacitor branches to model the dynamics both at the anode and cathode (activation over-potential) and one resistance to model the membrane (ohmic overpotential), A DC voltage source (reversible potential), as illustrated in Figure 21.
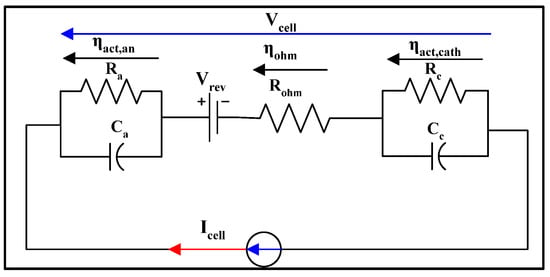
Figure 21.
Simple Equivalent circuit for a PEM Electrolyser.
They used the following equation for the stack voltage of the electrolyser:
The dynamic equations for the activation overvoltage in both electrodes can be expressed by:
The time constants and governing the dynamics are varying according to the operating conditions at the input of the electrolyser.
The researchers in reference [] have shown that the reaction speed of the PEM electrolyser is slower compared to a PEM fuel cell. Specifically, the equivalent capacitor in the PEM electrolyser was determined to be 37 F, whereas a value of 3 F was obtained for the PEM fuel cell. Ultimately, the dynamics of the PEM electrolyser are predominantly influenced by the anode reaction, which exhibits a slower dynamic response in contrast to the cathode reaction, as stated in []. In this type of model, the reversible overpotential usually is considered as a constant value (1.29 V) and ohmic overpotential can be calculated by Equations (30)–(33).
4. Discussion
As we move forward, several exciting avenues of research emerge that can contribute to a deeper understanding of PEM electrolysers and their integration into dynamic energy systems. This section will discuss the future prospects and existing obstacles in electrical modeling for PEM electrolysis.
The development of sophisticated diagnosis procedures aiming at comprehensively monitoring the health conditions of PEM electrolysers is a topic ripe for future research. These techniques could encompass real-time sensing of various performance metrics, such as temperature distribution, membrane integrity, catalyst degradation, and gas crossover. By implementing such diagnostics, researchers can gain valuable insights into the operational conditions that lead to performance degradation or system failures. This proactive approach could pave the way for predictive maintenance strategies, enhancing the longevity and reliability of PEM electrolysers within dynamic energy environments. Several researchers are conducting innovative studies to design non-intrusive technologies for monitoring and assessing the health of high-power electrolysers. The focus is on using the power converter’s capabilities to closely monitor the electrochemical reaction while minimizing intrusiveness. One possibility is to extract critical information about the electrolyser’s state by exploiting the power conversion system’s inherent monitoring potential without interfering with its normal operation. This may contribute to the development of more efficient and robust diagnostic methodologies for advanced PEM electrolyser technologies.
To fully realize the promise of PEM electrolysers in dynamic energy systems, future research must focus on control techniques and algorithms. Integrating dynamic models into control frameworks opens up possibilities for improving the performance, response, and overall efficiency of PEM electrolysis systems.
Furthermore, the development of predictive control algorithms holds promise for optimizing hydrogen production while maintaining system stability. These algorithms can leverage historical data and predictive dynamic models to anticipate future system behavior and adjust operating parameters preemptively. Such an approach can minimize overpotentials, manage transient states, and improve overall energy conversion efficiency [,].
In addition, as the integration of PEM electrolysers with renewable energy sources becomes more common [,], it becomes imperative to investigate advanced hybrid control systems. These strategies can dynamically switch between different operational modes, such as maximizing hydrogen production during periods of excess renewable energy and shifting to power-saving modes during low-energy periods. Such flexibility can contribute not only to efficient hydrogen production but also to grid stability and energy management [,].
Offshore hydrogen might be mentioned in terms of integrating hydrogen production systems with renewable energy sources. Many countries have met their climate goals and are on track to be completely carbon neutral. In this context, offshore hydrogen could enable a rapid, mass-scale transition to climate-neutral energy. Some of the wind farms instead sit in clusters more than 100 km out at sea. They are highly automated production islands that directly convert wind energy to hydrogen. In these clusters, the wind turbines are integrated with electrolysers that generate hydrogen from desalinated seawater. Chemical plants on dedicated platforms then process part of the hydrogen, combining it with nitrogen to make ammonia or with carbon dioxide to produce substitutes for fossil fuels [].
As a result, the combination of renewable energy sources, energy storage, and PEM electrolysers has an enormous potential for achieving long-term hydrogen generation. These hybrid systems help to increase energy resilience, reduce emissions, and accelerate the transition to a greener energy future. Ongoing research aims to address technical challenges and optimize system design and operation for practical implementation.
The integration of PEM electrolysers into the larger hydrogen supply chain is a topic of interest in the field of renewable energy and sustainable hydrogen production. Although such a network does not exist yet, dynamic electrical models, which are computational tools used to simulate the behavior of electrical systems over time, can be used to improve the operation and performance of individual electrolysers within the larger system.
When it comes to the challenges of modeling the electrolyser, PEM electrolysis involves complex electrochemical reactions occurring at various interfaces; accurately modeling these processes requires a thorough understanding of the electro-chemical kinetics and transport phenomena involved. The static model, which is basic and unsuitable of representing the true nonlinear behavior of the electrolyser, is the main emphasis of the literature in this context.
Another difficult issue is that the electrical modeling of PEM electrolysis needs to account for multiple physical phenomena, including electrochemical reactions, heat transfer, mass transport of reactants and products, and fluid dynamics. Integrating these aspects into a comprehensive model can be challenging.
To summarize, the field of electrical modeling for PEM electrolysis faces challenges related to system complexity, nonlinearity, and validation. However, there are numerous exciting research opportunities to enhance the accuracy, versatility, and applicability of these models. Modeling advances will be critical in accelerating the development and adoption of PEM electrolysis for sustainable hydrogen production.
5. Conclusions
This comprehensive review has provided a thorough analysis of static and dynamic electrical models used in PEM water electrolysers. While static models have received significant attention, this review emphasizes the importance of exploring dynamic models to capture the transient behavior of PEM electrolysers. The fast response time of PEM electrolysers, which is essential for coupling them with dynamic renewable energy sources, underscores the need for exploring dynamic models that capture the time-dependent performance of these systems.
In this article, different forms of voltage, including reversible, activation, ohmic, and diffusion, that contribute significantly to the electrolyser’s voltage cell, have been thoroughly researched. This review also considered Faraday efficiency, which is important in estimating the rate of hydrogen production. Large signal dynamic models, Small signal (Impedance model), Warburg and Randles circuits, and Simple equivalent electrical models have all been investigated. Furthermore, the review highlights the often-neglected diffusion phenomena within PEM electrolysers, emphasizing their significance in accurately modeling mass transport dynamics. Therefore, the dynamics of diffusion phenomena for both layers (Activation Layer and Gas Diffusion Layer) have been explained individually, and all relations and equations have been brought to a thorough demonstration. Understanding diffusion is vital for enhancing the accuracy of dynamic models.
By providing a comprehensive and detailed review of previous research on electrical modeling in PEM electrolysis systems, this article serves as a valuable resource for researchers and practitioners in the field.
Author Contributions
Conceptualization, investigation, writing—original draft preparation: H.M.; methodology: H.M., F.A.; writing—review and editing: H.M., F.A, J.-C.O.; supervision, project administration: F.A, J.-C.O. All authors have read and agreed to the published version of the manuscript.
Funding
This research received no external funding.
Data Availability Statement
Not applicable.
Conflicts of Interest
The authors declare no conflict of interest.
Parameters
| change in the reaction enthalpy for this endothermic reaction [] | the conductor cross-sectional area [] | ||
| Stoichiometric factor for product and reactant. | Ionic resistance [] | ||
| The stoichiometric factors are: [] | : | is the thickness of the membrane [cm] | |
| enthalpies of formation of the products [] | : | is the conductivity of the membrane [] | |
| enthalpies of formation of the reactants [] | : | is the hydration rate [-] | |
| Change in Gibbs Free Energy or Free enthalpy of reaction [] | diffusion coefficient of the species X or Y | ||
| T: | Temperature of the cell [] | : | The diffusion limit current [A] |
| : | Molar change in Entropy [] | The diffusion limit current density [] | |
| Anode reversible voltage [V] | Concentration in the channel [] | ||
| : | Cathode reversible voltage [V] | Diffusion length of zone A and B. [m] | |
| : | Cell voltage [V] | , | are the diffusion limit currents [A] effective respectively in zones A and B. |
| Reversible voltage (V) | β: | coefficient considering the contribution of all diffusion overvoltage [-]. This parameter will be identified experimentally. | |
| : | Anode activation over voltage [] | [-] | |
| Cathode activation overvoltage [] | P: | Pressure [bar] | |
| : | Ohmic overvoltage [V] | : | Molar flow of hydrogen [] |
| : | Diffusion Overvoltage [] | Current efficiency [-] | |
| F: | Faraday’s constant [] | A: | Cell area [] |
| Reversible voltage at standard condition [V] | Empiric coefficients | ||
| : | Universal gas constant [8.314 ] | The capacitive current of the electrochemical double layer [A] | |
| : | Hydrogen pressure [bar] | : | the double layer capacitance [F] |
| : | Oxygen pressure [bar] | electrical permittivity [] | |
| : | References Pressure [bar] | : | Active surface of electrode |
| n: | the number of moving electrons in the chemical reaction | d: | is the distance between the electrode and the electrolyte [m]. |
| [n = 2] | : | Electrode current [A] | |
| Gibbs Free Energy at standard condition [] | The faradic current (current linked to activation phenomena) [A] | ||
| Cell current density [] | Diffusion resistance | ||
| Exchange current density at electrodes [] | Capacitor of the diffusion phenomena [F] | ||
| The concentrations of the reaction product and reactant in the vicinity of the electrode [] | : | The diffusion curent [A] | |
| The concentration of the reaction product and reactant in the solution [] | Diffusion resistance ] | ||
| Charge transfer coefficient [-] | Diffusion time constant [s] | ||
| Activation overvoltage at both electrodes [V] | Warburg impedance ] | ||
| k: | refer to anode, cathode [-] | and : | are the capacitors for anode and cathode (F) |
| the leakage current density due to crossover [] | and : | are the resistances for anode and cathode (F) | |
| : | is the global transfer coefficient [] | and | Time constant (s) |
| is the total activation overvoltage [] | Molar flow of species [] | ||
| Anode charge transfer coefficient [-] | Concentration of species X or Y in and at time t | ||
| Cathode charge transfer coefficient [-] | is the coordinate [m] on the axis [) | ||
| Cell current [] | Time [s] | ||
| A: | is the surface of the cell [] | ||
| : | The membrane overvoltage [V] | ||
| The electronic overvoltage [V] | |||
| Electronic resistance [] | |||
| L: | the length of the path of the electrons [cm] | ||
| : | electrode material resistivity [m] | ||
Appendix A
Appendix A.1. Description of Convective Diffusion Phenomenon
Figure A1 presents a stationary concentration profile in the case of the anode. This is the Nernst convective diffusion model. This model assumes the existence in contact with the reactive site of a layer where only diffusion ensures the transport of the species. Beyond that, a convection regime ensures that the concentration of the species is constant and independent of the distance to the reactive [] site.
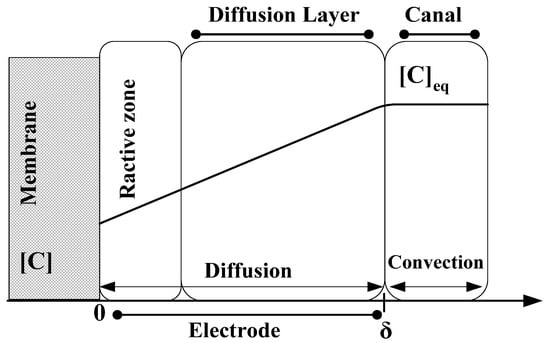
Figure A1.
Stationary concentration profile in the case of the convective diffusion model [].
We will be discussing the diffusion of a species through a layer, as depicted in Figure A2. It is important to distinguish between two cases:
- One where the species is produced and evacuated from the electrolyser ( and );
- One where the species is brought in and consumed within the electrolyser ( for the latter case).
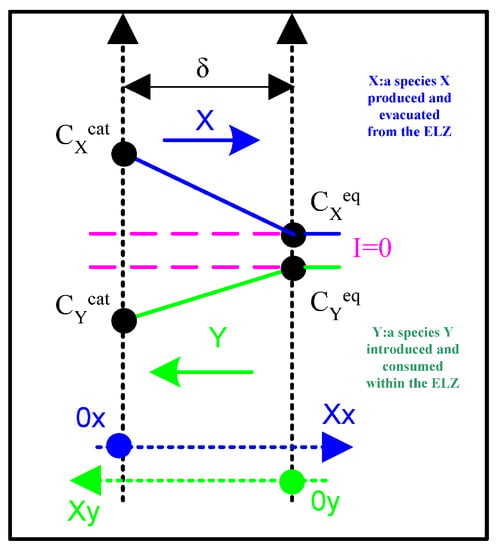
Figure A2.
Conventions and notation adopted for the study of diffusion through a layer [].
For each species, we can apply Fick’s law by considering only a diffusion in a single direction (axis [) perpendicular to the surface of the component):
In steady-state, this equation becomes:
We obtain intermediately:
Finally, we will have:
where:
- molar flow of species X or Y [mol/s];
- concentration of species X or Y in and at time t ;
- the coordinate [m] on the axis [);
- time [s].
This equation (Equation (A4)) is general and can be applied to each species.
Appendix A.2. First Case: Species Produced and Evacuated from the Component ( and )
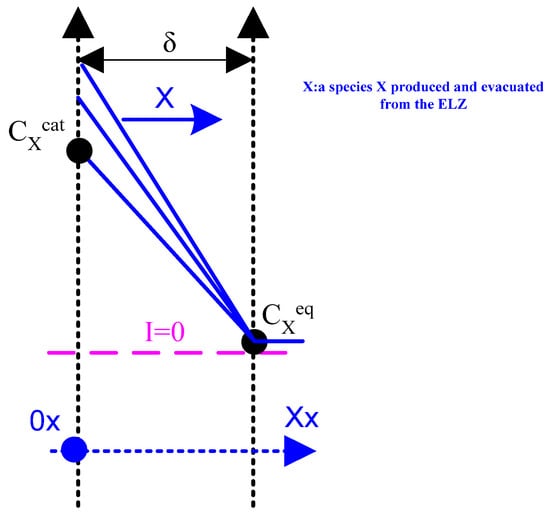
Figure A3.
Conventions and notation adopted for a species X produced and evacuated from the electrolyser through a layer [].
The general formula (A4) is applicable for this first case study. We apply it here to species X:
The origin of the axis [Ox) is taken at the level of the place where the species is produced. According to the boundary condition:
Or:
We deduce the value of the concentration in x = 0 place where the species is produced:
As a result:
Appendix A.3. Second Case: Species Introduced and Consumed within the Component (O)
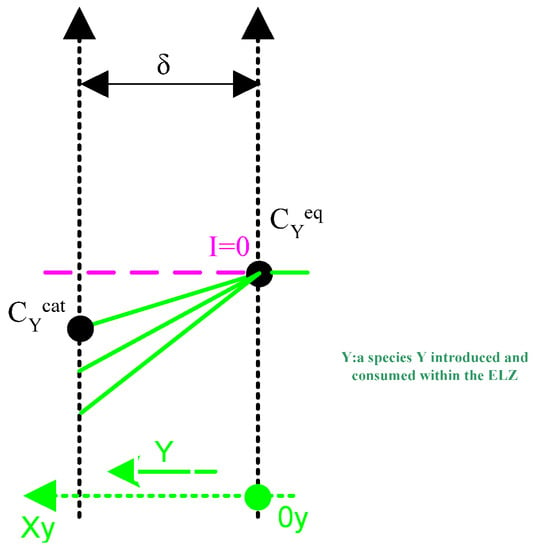
Figure A4.
Conventions and notation adopted for a species X introduced and consumed within the electrolyser through a layer [].
Similar to the previous case we will have:
According to the boundary condition:
Or:
We deduce the value of the concentration in x = δ at the place where the species is consumed:
As a result:
A brief description of the diffusion of the double layer is provided in the following section. This approach distinctly considers diffusion phenomena in the diffusion and activation zones. As shown in Figure A5, the activation layer is the place of faster diffusion than in the diffusion layer. On the other hand, the activation layer generates a greater drop in concentration than in the diffusion layer.
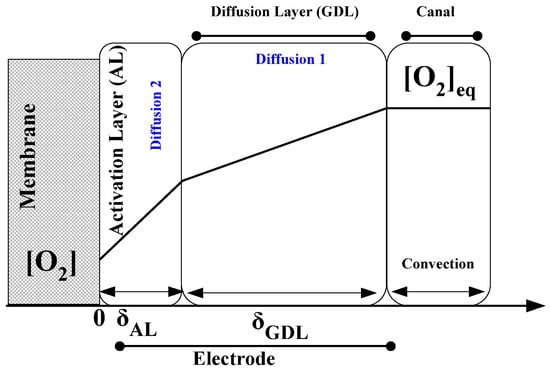
Figure A5.
Double layer diffusion model [].
Like the single-layer phenomenon, we will investigate the diffusion of a species in two consecutive layers, which we will refer to as “zone A” and “zone B” (Figure A6). There are two scenarios we will consider:
- One where the species is produced and evacuated from the component ( and );
- Another where the species is introduced and consumed within the component ().
Typically, zone A represents the active layer while zone B is a diffusive zone.
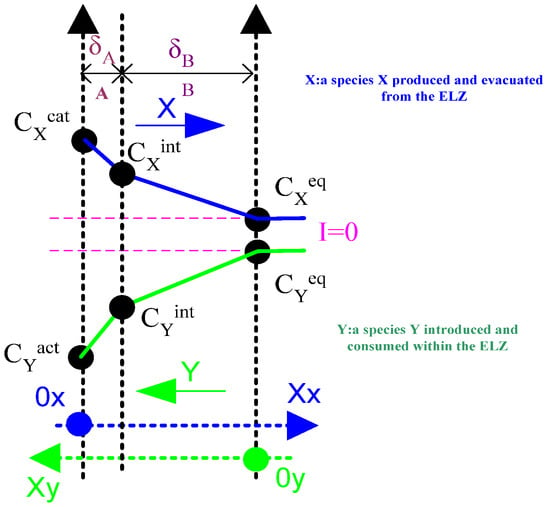
Figure A6.
Conventions and notation adopted for the study of diffusion through two layers [].
Appendix B
Appendix B.1. Calculation of Diffusion Impedance
This appendix details the calculation of the diffusion impedances. The approach consists of calculating the cathodic faradic impedance, including the diffusion impedances. We will use the same methods as []. Consider the following redox reaction:
The principle of impedance spectroscopy is placing ourselves around an operating point and applying low-amplitude disturbances; under these conditions, the faradic impedance is obtained by:
The faradic current is a function of time, electrode potential, and concentrations of oxidizing and reducing species at a given temperature:
where:
- E (0, t): electrode potential [V];
- [Ox] (0, t): concentration of oxidizing species [];
- [Red] (0, t): concentration of reducing species [].
The variation of the faradic current around an operating point can be written as:
Using the formalism of Laplace:
This last relation makes it possible to calculate the faradaic impedance. The faradic impedance depends on three terms:
- the charge transfer or activation resistance [];
- ZOx(p): the concentration or diffusion impedance of the oxidizing species [].
- ZRed(p): the concentration or diffusion impedance of the reducing species [].
Now, we are calculating the partial derivatives of in the steady-state condition. can be express as:
- Potential of electrode [V];
- rate constant of oxidation and reduction;
- transfer coefficient (), which indicate in which direction the reaction is favored. If > 0.5, the reaction in the direction of oxidation (anodic direction) is favored; if > 0.5, the reaction in the reduction direction (cathode direction) is favoured.
We can deduce:
We can rewrite the terms of the faradaic impedance:
Let us now determine the variations of the concentrations [Ox] and [Red] with respect to the variations of the faradaic current around an operating point. According to the second law of Fick for the species X:
where:
- diffusion coefficient [].
Solving the above equation in Laplace domain:
With:
Equation (A30) is a second order differential equation, and the general solution is:
where the constants A and B depend on the boundary conditions. In the case of the Nernst hypothesis, the boundary condition is written: , where u is the diffusion length of the species X. According to Equation (A32):
We will have:
By reinjecting these values into 115, we obtain:
The diffusion flow of species X ([]) (Equation (A3)) can then be expressed:
Finally, with the Equation (A33):
Now, we can define the time constant of the diffusion of the species X in the diffusion layer:
The faradic current can be expressed as a function of diffusion flow of the oxidizing and reducing species:
where:
- diffusion flow in the direction of oxidation [];
- diffusion flow in the direction of reduction [].
We can deduce the variation of species concentration:
Then, the impedance of oxidation and reduction can be deduced:
Therefore, the impedance of diffusion can be introduced as:
Since we are looking for the RC dipole approximation for diffusion impedance, using the Taylor series and by letting x tend towards 0:
Therefore, the diffusion impedance can be expressed as:
And the capacitor of diffusion is:
Appendix C

Table A1.
A review on the physical parameters.
Table A1.
A review on the physical parameters.
| Ref. | Membrane Thickness | Charge Transfer (-) | ) | Membrane Surface Area | ||||
|---|---|---|---|---|---|---|---|---|
| Nafion 115 | Nafion 117 | etc. | Anode | Cathode | Anode | Cathode | ||
| [] | 178 | 0.5 | 0.5 | Pt: Pt-Ir: | Pt: | |||
| [] | 0.05–0.2 mm | 0.5 | 0.5 | 0.01–10 | 160 | |||
| [] | 0.7178 | 0.6395 | ||||||
| [] | 0.18–0.42 | |||||||
| [] | 178 | 0.18–0.42 | 0.5 | |||||
| [] | 178 | 0.1–0.6 | 0.5 | 86.4 (20 cells) | ||||
| [] | Nafion 110 | 0.5 | 0.5 | Pt: Pt-Ir: | 160 | |||
| [] | 100 | 0.5 | 0.5 | 1 | ||||
| [] | Nafion 112 | 0.5 | 0.5 | 50 of active area-2 cells | ||||
| [] | 178 | 0.5 | 0.5 | 5 | ||||
| [] | 0.0254 cm | 0.8 | 0.25 | 160 | ||||
| [] | 200 | Electrode surface area 1 cm2 | ||||||
Remark A1:
- Literature values for i0 are also greatly dispersed in a range between ;
- In practice, the thickness of the electrolyte membrane of a PEM electrolyser can range from 50 to 200 .
References
- AR5 Climate Change 2013: The Physical Science Basis—IPCC. Available online: https://www.ipcc.ch/report/ar5/wg1/ (accessed on 13 March 2023).
- Liu, Y.; Jiang, S.P.; Shao, Z. Intercalation pseudocapacitance in electrochemical energy storage: Recent advances in fundamental understanding and materials development. Mater. Today Adv. 2020, 7, 100072. [Google Scholar] [CrossRef]
- Sood, S. Multiphysics Modelling for Online Diagnosis and Efficiency Tracking: Application to Green H2 Production. Ph.D. Thesis, Université de Lille, Lille, France, 2021. Available online: https://theses.hal.science/tel-03675228 (accessed on 17 June 2023).
- Veziro, T.N.; Barbir, F. Hydrogen: The wonder fuel. Int. J. Hydrogen Energy 1992, 17, 391–404. [Google Scholar] [CrossRef]
- Yilanci, A.; Dincer, I.; Ozturk, H.K. A review on solar-hydrogen/fuel cell hybrid energy systems for stationary applications. Prog. Energy Combust. Sci. 2009, 35, 231–244. [Google Scholar] [CrossRef]
- Singh, S.; Jain, S.; Venkateswaran, P.S.; Tiwari, A.K.; Nouni, M.R.; Pandey, J.K.; Goel, S. Hydrogen: A sustainable fuel for future of the transport sector. Renew. Sustain. Energy Rev. 2015, 51, 623–633. [Google Scholar] [CrossRef]
- Gahleitner, G. Hydrogen from renewable electricity: An international review of power-to-gas pilot plants for stationary applications. Int. J. Hydrogen Energy 2013, 38, 2039–2061. [Google Scholar] [CrossRef]
- Albadi, M.H.; El-Saadany, E.F. Overview of wind power intermittency impacts on power systems. Electr. Power Syst. Res. 2010, 80, 627–632. [Google Scholar] [CrossRef]
- Zhang, G.; Wan, X. A wind-hydrogen energy storage system model for massive wind energy curtailment. Int. J. Hydrogen Energy 2014, 39, 1243–1252. [Google Scholar] [CrossRef]
- Nitsch, J.; Klaiss, H.; Meyer, J. The contribution of hydrogen in the development of renewable energy sources. Int. J. Hydrogen Energy 1992, 17, 651–663. [Google Scholar] [CrossRef]
- Hernández-Gómez, Á.; Ramirez, V.; Guilbert, D. Investigation of PEM electrolyzer modeling: Electrical domain, efficiency, and specific energy consumption. Int. J. Hydrogen Energy 2020, 45, 14625–14639. [Google Scholar] [CrossRef]
- Falcão, D.S.; Pinto, A.M.F.R. A review on PEM electrolyzer modelling: Guidelines for beginners. J. Clean. Prod. 2020, 261, 121184. [Google Scholar] [CrossRef]
- Olivier, P.; Bourasseau, C.; Bouamama, P.B. Low-temperature electrolysis system modelling: A review. Renew. Sustain. Energy Rev. 2017, 78, 280–300. [Google Scholar] [CrossRef]
- Bessarabov, D.G.; Wang, H.H.; Li, H.; Zhao, N. (Eds.) PEM Electrolysis for Hydrogen Production: Principles and Applications; CRC Press: Boca Raton, FL, USA; London, UK, 2016. [Google Scholar]
- Rozain, C. Développement de Nouveaux Matériaux d’Electrodes pour la Production d’Hydrogène par Electrolyse de l’eau. Ph.D. Thesis, Université Paris Sud-Paris XI, Bures-sur-Yvette, France, 2013. Available online: https://theses.hal.science/tel-00923169 (accessed on 13 March 2023).
- Kauranen, P.S.; Lund, P.D.; Vanhanen, J.P. Development of a self-sufficient solar-hydrogen energy system. Int. J. Hydrogen Energy 1994, 19, 99–106. [Google Scholar] [CrossRef]
- Görgün, H. Dynamic modelling of a proton exchange membrane (PEM) electrolyzer. Int. J. Hydrogen Energy 2006, 31, 29–38. [Google Scholar] [CrossRef]
- Shen, M.; Bennett, N.; Ding, Y.; Scott, K. A concise model for evaluating water electrolysis. Int. J. Hydrogen Energy 2011, 36, 14335–14341. [Google Scholar] [CrossRef]
- Choi, P.; Bessarabov, D.G.; Datta, R. A simple model for solid polymer electrolyte (SPE) water electrolysis. Solid State Ion. 2004, 175, 535–539. [Google Scholar] [CrossRef]
- Zhang, H.; Su, S.; Lin, G.; Chen, J. Efficiency Calculation and Configuration Design of a PEM Electrolyzer System for Hydrogen Production. Int. J. Electrochem. Sci. 2012, 7, 4143–4157. [Google Scholar] [CrossRef]
- Abdin, Z.; Webb, C.J.; Gray, E.M. Modelling and simulation of a proton exchange membrane (PEM) electrolyser cell. Int. J. Hydrogen Energy 2015, 40, 13243–13257. [Google Scholar] [CrossRef]
- Kang, Z.; Alia, S.M.; Young, J.L.; Bender, G. Effects of various parameters of different porous transport layers in proton exchange membrane water electrolysis. Electrochim. Acta 2020, 354, 136641. [Google Scholar] [CrossRef]
- Lefranc, O. Développement d’un outil de Conception de Micro-Réseaux Energétiques”. Ph.D. Thesis, Toulouse, INPT, Toulouse, France, 2021. Available online: https://theses.hal.science/tel-04186359 (accessed on 17 June 2023).
- Hammoudi, M.; Henao, C.; Agbossou, K.; Dubé, Y.; Doumbia, M.L. New multi-physics approach for modelling and design of alkaline electrolyzers. Int. J. Hydrogen Energy 2012, 37, 13895–13913. [Google Scholar] [CrossRef]
- Henao, C.; Agbossou, K.; Hammoudi, M.; Dubé, Y.; Cardenas, A. Simulation tool based on a physics model and an electrical analogy for an alkaline electrolyser. J. Power Sources 2014, 250, 58–67. [Google Scholar] [CrossRef]
- da Costa Lopes, F.; Watanabe, E.H. Experimental and theoretical development of a PEM electrolyzer model applied to energy storage systems. In Proceedings of the 2009 Brazilian Power Electronics Conference, Bonito-Mato Grosso do Sul, Brazil, 27 September–1 October 2009; pp. 775–782. [Google Scholar] [CrossRef]
- Dale, N.V.; Mann, M.D.; Salehfar, H. Semiempirical model based on thermodynamic principles for determining 6 kW proton exchange membrane electrolyzer stack characteristics. J. Power Sources 2008, 185, 1348–1353. [Google Scholar] [CrossRef]
- García-Valverde, R.; Espinosa, N.; Urbina, A. Simple PEM water electrolyser model and experimental validation. Int. J. Hydrogen Energy 2012, 37, 1927–1938. [Google Scholar] [CrossRef]
- Agbli, K.S.; Péra, M.C.; Hissel, D.; Rallières, O.; Turpin, C.; Doumbia, I. Multiphysics simulation of a PEM electrolyser: Energetic Macroscopic Representation approach. Int. J. Hydrogen Energy 2011, 36, 1382–1398. [Google Scholar] [CrossRef]
- Ursúa, A.; Sanchis, P. Static–dynamic modelling of the electrical behaviour of a commercial advanced alkaline water electrolyser. Int. J. Hydrogen Energy 2012, 37, 18598–18614. [Google Scholar] [CrossRef]
- Marangio, F.; Santarelli, M.; Calì, M. Theoretical model and experimental analysis of a high pressure PEM water electrolyser for hydrogen production. Int. J. Hydrogen Energy 2009, 34, 1143–1158. [Google Scholar] [CrossRef]
- Roy, A.; Watson, S.; Infield, D. Comparison of electrical energy efficiency of atmospheric and high-pressure electrolysers. Int. J. Hydrogen Energy 2006, 31, 1964–1979. [Google Scholar] [CrossRef]
- Rallières, O. Modélisation et Caractérisation de Piles a Combustible et Electrolyseurs PEM. Ph.D. Thesis, Toulouse, INPT, Toulouse, France, 2011. Available online: https://www.theses.fr/2011INPT0128 (accessed on 13 March 2023).
- Rabih, S. Contribution à la Modélisation de Systèmes Réversibles de Types Electrolyseur et Pile à Hydrogène en vue de leur Couplage aux Générateurs Photovoltaïques. Ph.D. Thesis, Toulouse, INPT, Toulouse, France, 2008. Available online: http://ethesis.inp-toulouse.fr/archive/00000677/ (accessed on 13 March 2023).
- Igourzal, A.; Auger, F.; Olivier, J.-C.; Retière, C. Electrical and thermal modelling of PEMFCs for naval applications. Math. Comput. Simul. 2023, 206, S0378475423001817. [Google Scholar] [CrossRef]
- Ni, M.; Leung, M.K.H.; Leung, D.Y.C. Energy and exergy analysis of hydrogen production by a proton exchange membrane (PEM) electrolyzer plant. Energy Convers. Manag. 2008, 49, 2748–2756. [Google Scholar] [CrossRef]
- Sawada, S.; Yamaki, T.; Maeno, T.; Asano, M.; Suzuki, A.; Terai, T.; Maekawa, Y. Solid polymer electrolyte water electrolysis systems for hydrogen production based on our newly developed membranes, Part I: Analysis of voltage–current characteristics. Prog. Nucl. Energy 2008, 50, 443–448. [Google Scholar] [CrossRef]
- Grigoriev, S.A.; Kalinnikov, A.A.; Millet, P.; Porembsky, V.I.; Fateev, V.N. Mathematical modeling of high-pressure PEM water electrolysis. J. Appl.Electrochem. 2010, 40, 921–932. [Google Scholar] [CrossRef]
- Lee, B.; Park, K.; Kim, H.-M. Dynamic Simulation of PEM Water Electrolysis and Comparison with Experiments. Int. J. Electrochem. Sci. 2013, 8, 235–248. [Google Scholar] [CrossRef]
- Lebbal, M.E.; Lecœuche, S. Identification and monitoring of a PEM electrolyser based on dynamical modelling. Int. J. Hydrogen Energy 2009, 34, 5992–5999. [Google Scholar] [CrossRef]
- Awasthi, A.; Scott, K.; Basu, S. Dynamic modeling and simulation of a proton exchange membrane electrolyzer for hydrogen production. Int. J. Hydrogen Energy 2011, 36, 14779–14786. [Google Scholar] [CrossRef]
- Kim, H.; Park, M.; Lee, K.S. One-dimensional dynamic modeling of a high-pressure water electrolysis system for hydrogen production. Int. J. Hydrogen Energy 2013, 38, 2596–2609. [Google Scholar] [CrossRef]
- Ulleberg, Ø. Modeling of advanced alkaline electrolyzers: A system simulation approach. Int. J. Hydrogen Energy 2003, 28, 21–33. [Google Scholar] [CrossRef]
- Fontes, G. Modélisation et Caractérisation de la pile PEM pour l’étude des Interactions Avec les Convertisseurs Statiques. Ph.D. Thesis, Toulouse, INPT, Toulouse, France, 2005. Available online: https://www.theses.fr/2005INPT020H (accessed on 13 March 2023).
- Springer, T.E.; Zawodzinski, T.A.; Gottesfeld, S. Polymer Electrolyte Fuel Cell Model. J. Electrochem. Soc. 1991, 138, 2334. [Google Scholar] [CrossRef]
- Brown, T.M.; Brouwer, J.; Samuelsen, G.S.; Holcomb, F.H.; King, J. Dynamic first principles model of a complete reversible fuel cell system. J. Power Sources 2008, 182, 240–253. [Google Scholar] [CrossRef]
- Han, B.; Steen, S.M.; Mo, J.; Zhang, F.-Y. Electrochemical performance modeling of a proton exchange membrane electrolyzer cell for hydrogen energy. Int. J. Hydrogen Energy 2015, 40, 7006–7016. [Google Scholar] [CrossRef]
- Maruf-ul-Karim, M.; Iqbal, M.T. Dynamic modeling and simulation of alkaline type electrolyzers. In Proceedings of the 2009 Canadian Conference on Electrical and Computer Engineering, St. John’s, NL, Canada, 3–6 May 2009; pp. 711–715. [Google Scholar] [CrossRef]
- Sánchez, M.; Amores, E.; Rodríguez, L.; Clemente-Jul, C. Semi-empirical model and experimental validation for the performance evaluation of a 15 kW alkaline water electrolyzer. Int. J. Hydrogen Energy 2018, 43, 20332–20345. [Google Scholar] [CrossRef]
- Hug, W.; Bussmann, H.; Brinner, A. Intermittent operation and operation modeling of an alkaline electrolyzer. Int. J. Hydrogen Energy 1993, 18, 973–977. [Google Scholar] [CrossRef]
- Baurens, P.; Serre-Combe, P.; Poirot-Crouvezier, J.-P. Fuel Cells. In Low Emission Power Generation Technologies and Energy Management; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2013; pp. 179–262. [Google Scholar] [CrossRef]
- Barbir, F. PEM electrolysis for production of hydrogen from renewable energy sources. Sol. Energy 2005, 78, 661–669. [Google Scholar] [CrossRef]
- Artuso, P.; Gammon, R.; Orecchini, F.; Watson, S.J. Alkaline electrolysers: Model and real data analysis. Int. J. Hydrogen Energy 2011, 36, 7956–7962. [Google Scholar] [CrossRef]
- Atlam, O.; Kolhe, M. Equivalent electrical model for a proton exchange membrane (PEM) electrolyser. Energy Convers. Manag. 2011, 52, 2952–2957. [Google Scholar] [CrossRef]
- Martinson, C.; van Schoor, G.; Uren, K.; Bessarabov, D. Equivalent electrical circuit modelling of a Proton Exchange Membrane electrolyser based on current interruption. In Proceedings of the 2013 IEEE International Conference on Industrial Technology (ICIT), Cape Town, South Africa, 25–28 February 2013; pp. 716–721. [Google Scholar] [CrossRef]
- Rozain, C.; Millet, P. Electrochemical characterization of Polymer Electrolyte Membrane Water Electrolysis Cells. Electrochim. Acta 2014, 131, 160–167. [Google Scholar] [CrossRef]
- Martinson, C.A.; van Schoor, G.; Uren, K.R.; Bessarabov, D. Characterisation of a PEM electrolyser using the current interrupt method. Int. J. Hydrogen Energy 2014, 39, 20865–20878. [Google Scholar] [CrossRef]
- Guilbert, D.; Vitale, G. Experimental Validation of an Equivalent Dynamic Electrical Model for a Proton Exchange Membrane Electrolyzer. In Proceedings of the 2018 IEEE International Conference on Environment and Electrical Engineering and 2018 IEEE Industrial and Commercial Power Systems Europe (EEEIC/I&CPS Europe), Palermo, Italy, 12–15 June 2018; pp. 1–6. [Google Scholar] [CrossRef]
- Hernández-Gómez, Á.; Ramirez, V.; Guilbert, D.; Saldivar, B. Development of an adaptive static-dynamic electrical model based on input electrical energy for PEM water electrolysis. Int. J. Hydrogen Energy 2020, 45, 18817–18830. [Google Scholar] [CrossRef]
- Macdonald, J.R.; Johnson, W.B. Fundamentals of Impedance Spectroscopy. In Impedance Spectroscopy; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2005; pp. 1–26. [Google Scholar] [CrossRef]
- Serna, Á.; Yahyaoui, I.; Normey-Rico, J.E.; De Prada, C.; Tadeo, F. Predictive control for hydrogen production by electrolysis in an offshore platform using renewable energies. Int. J. Hydrogen Energy 2017, 42, 12865–12876. [Google Scholar] [CrossRef]
- Abdelghany, M.B.; Shehzad, M.F.; Liuzza, D.; Mariani, V.; Glielmo, L. Optimal operations for hydrogen-based energy storage systems in wind farms via model predictive control. Int. J. Hydrogen Energy 2021, 46, 29297–29313. [Google Scholar] [CrossRef]
- Nasser, M.; Megahed, T.F.; Ookawara, S.; Hassan, H. A review of water electrolysis–based systems for hydrogen production using hybrid/solar/wind energy systems. Environ. Sci. Pollut. Res. 2022, 29, 86994–87018. [Google Scholar] [CrossRef]
- Wang, H.; Sun, M.; Ren, J.; Yuan, Z. Circumventing Challenges: Design of Anodic Electrocatalysts for Hybrid Water Electrolysis Systems. Adv. Energy Mater. 2023, 13, 2203568. [Google Scholar] [CrossRef]
- Zhou, T.; Francois, B. Modeling and control design of hydrogen production process for an active hydrogen/wind hybrid power system. Int. J. Hydrogen Energy 2009, 34, 21–30. [Google Scholar] [CrossRef]
- Nafchi, F.M.; Baniasadi, E.; Afshari, E.; Javani, N. Performance assessment of a solar hydrogen and electricity production plant using high temperature PEM electrolyzer and energy storage. Int. J. Hydrogen Energy 2018, 43, 5820–5831. [Google Scholar] [CrossRef]
- Wind-to-Hydrogen Tech Goes to Sea-IEEE Spectrum. Available online: https://spectrum.ieee.org/green-hydrogen-2663997448 (accessed on 26 August 2023).
- Toghyani, S. Optimization of operating parameters of a polymer exchange membrane electrolyzer. Int. J. Hydrogen Energy 2019, 44, 6403–6414. [Google Scholar] [CrossRef]
- Biaku, C.; Dale, N.; Mann, M.; Salehfar, H.; Peters, A.; Han, T. A semiempirical study of the temperature dependence of the anode charge transfer coefficient of a 6kW PEM electrolyzer. Int. J. Hydrogen Energy 2008, 33, 4247–4254. [Google Scholar] [CrossRef]
- Ahn, J.; Holze, R. Bifunctional electrodes for an integrated water-electrolysis and hydrogen-oxygen fuel cell with a solid polymer electrolyte. J. Appl. Electrochem. 1992, 22, 1167–1174. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).