A Coupled Poro-Elastic Fluid Flow Simulator for Naturally Fractured Reservoirs
Abstract
:1. Introduction
2. Derivation of Multiphase Flow Equations
2.1. Mass Conversation Equation
2.2. Momentum Balance Equation
2.3. Finite Element Discretization
2.4. Evaluation of Non-Linear Coefficients and Computational Procedure
3. Results and Discussion
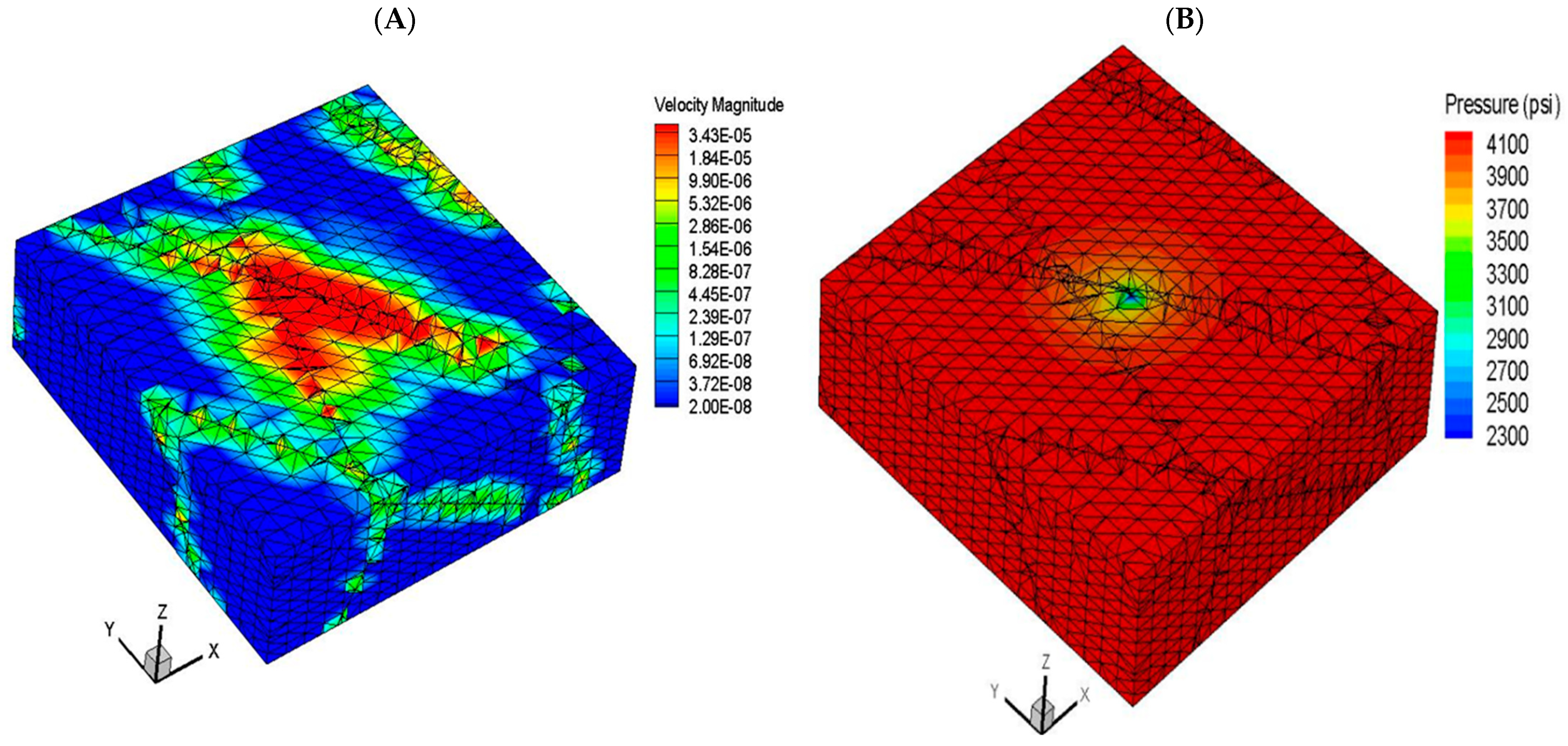
3.1. Validation Using Kirsch’s Problem in Poroelasticity
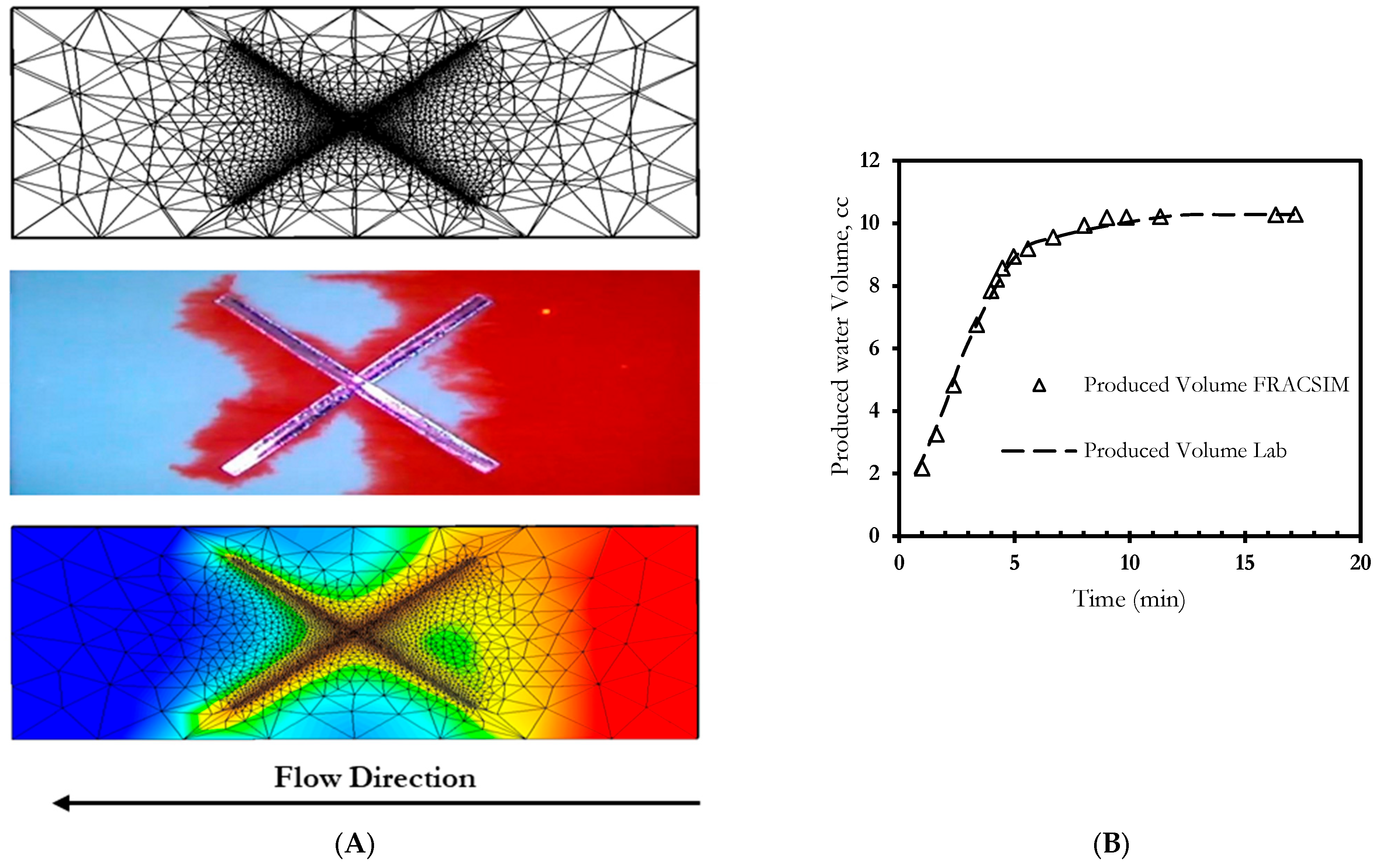
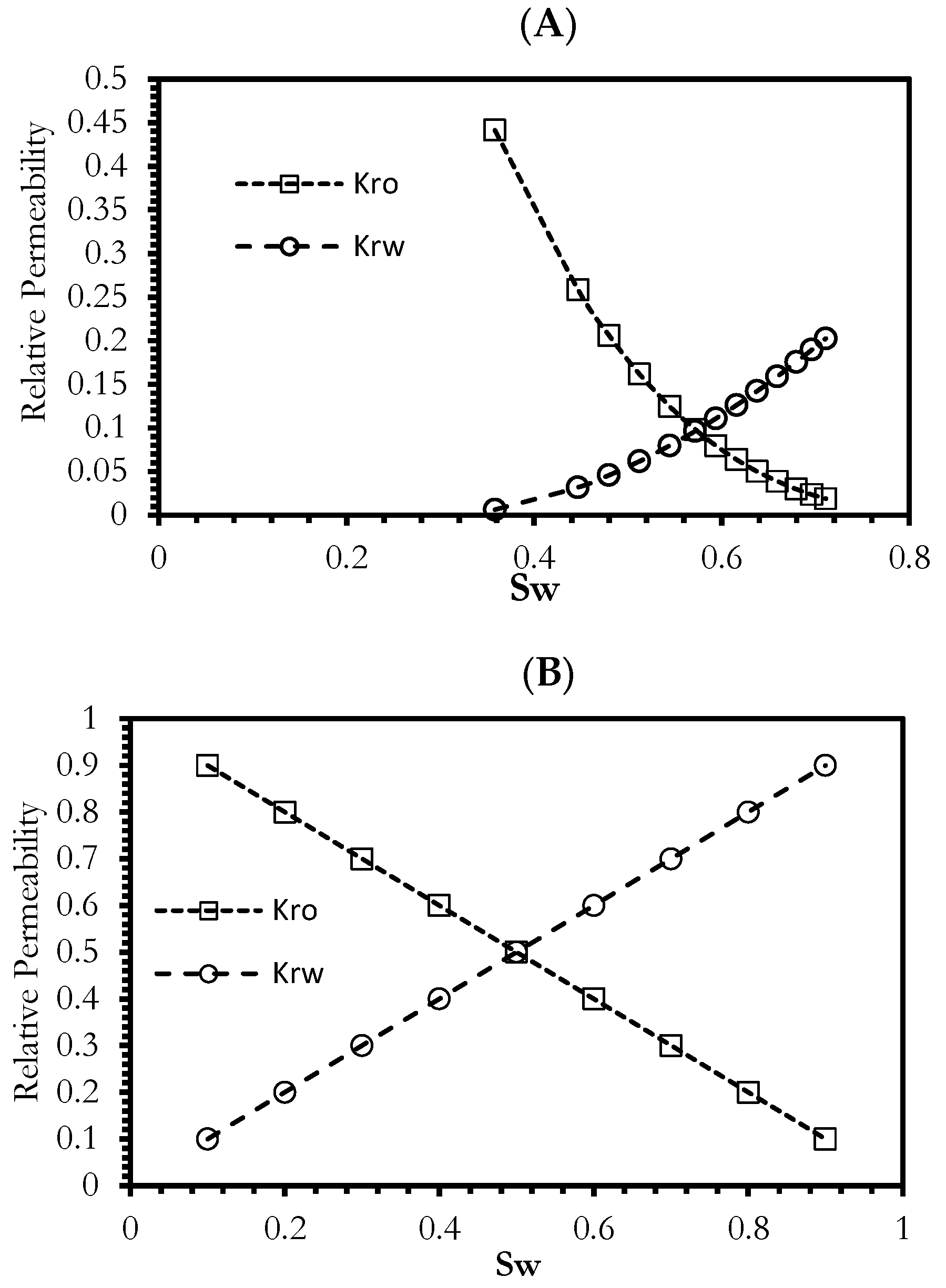
3.2. History Matching of Two-Phase Flow Real Data—Laboratory Scale
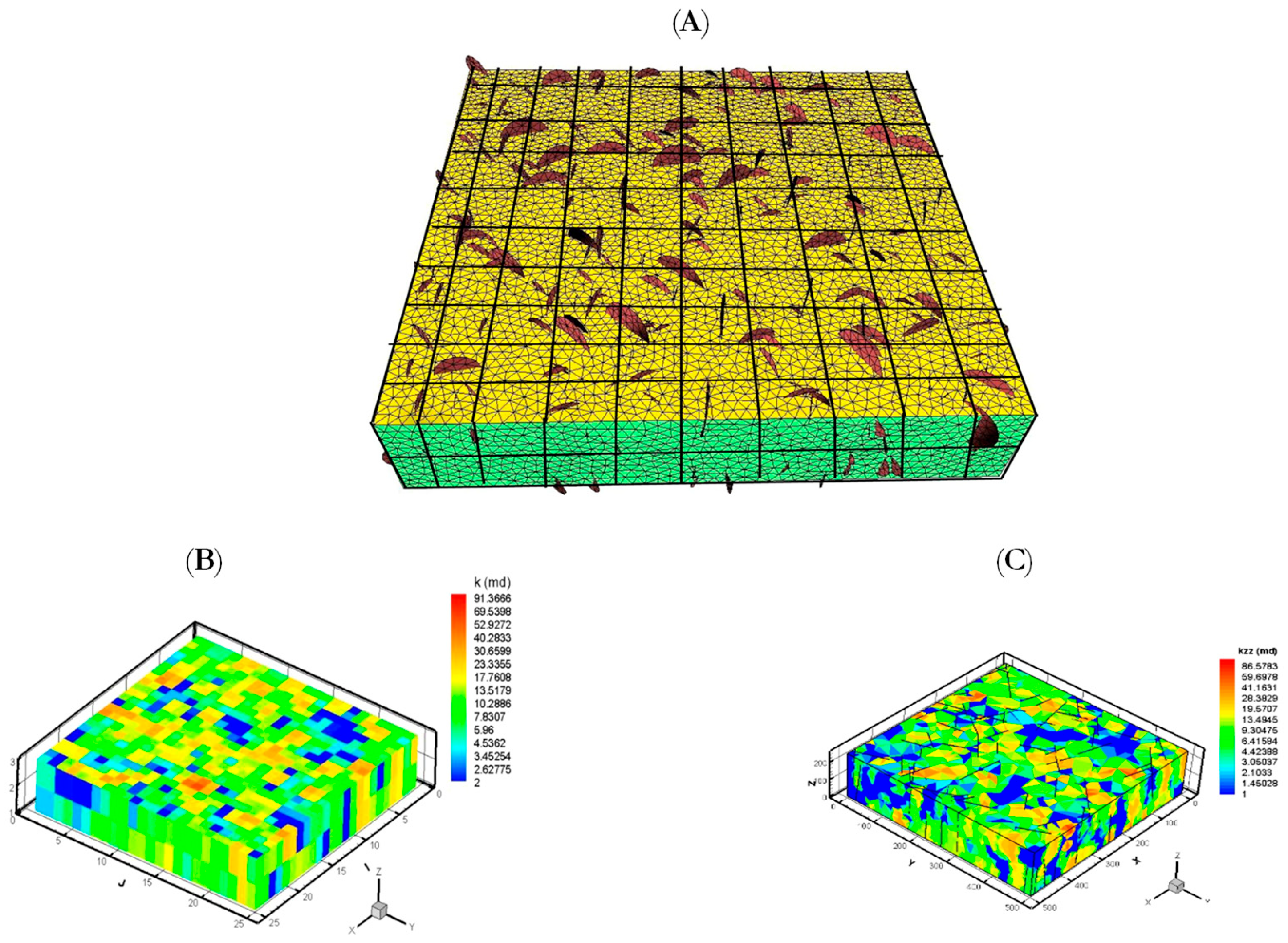
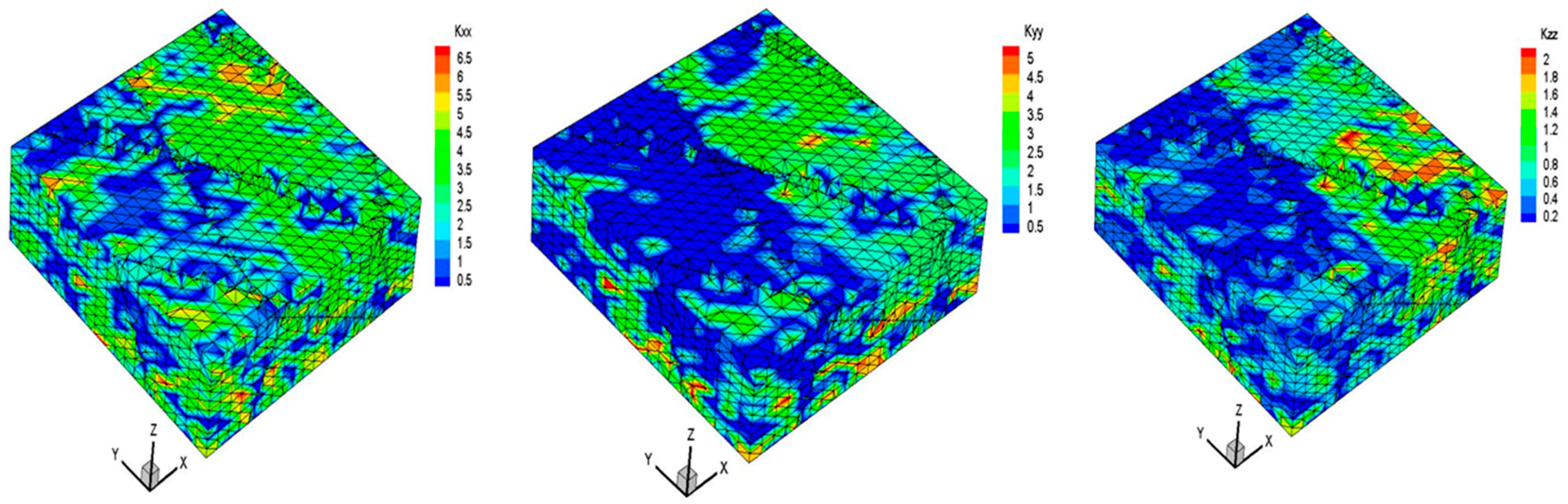
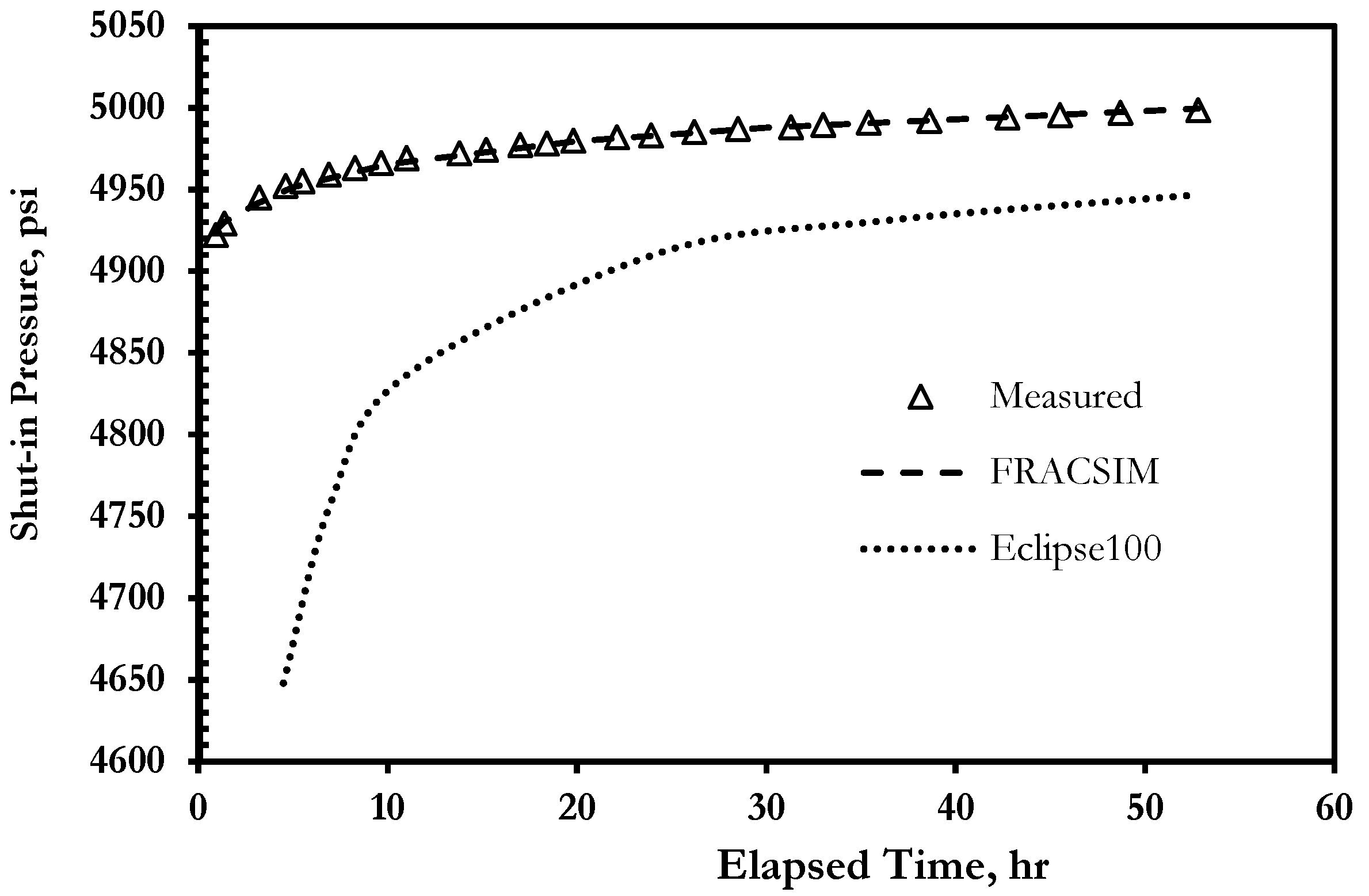
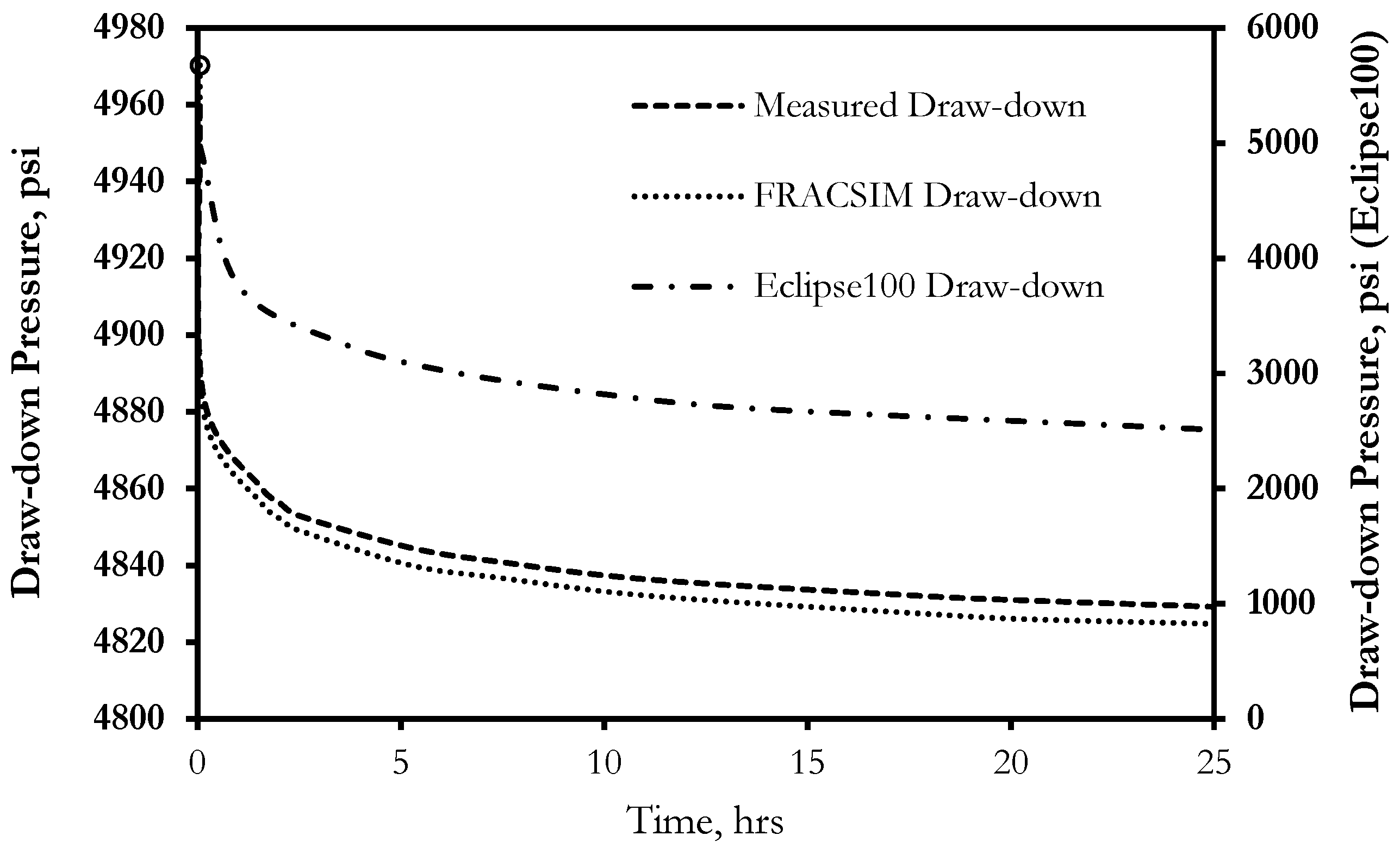
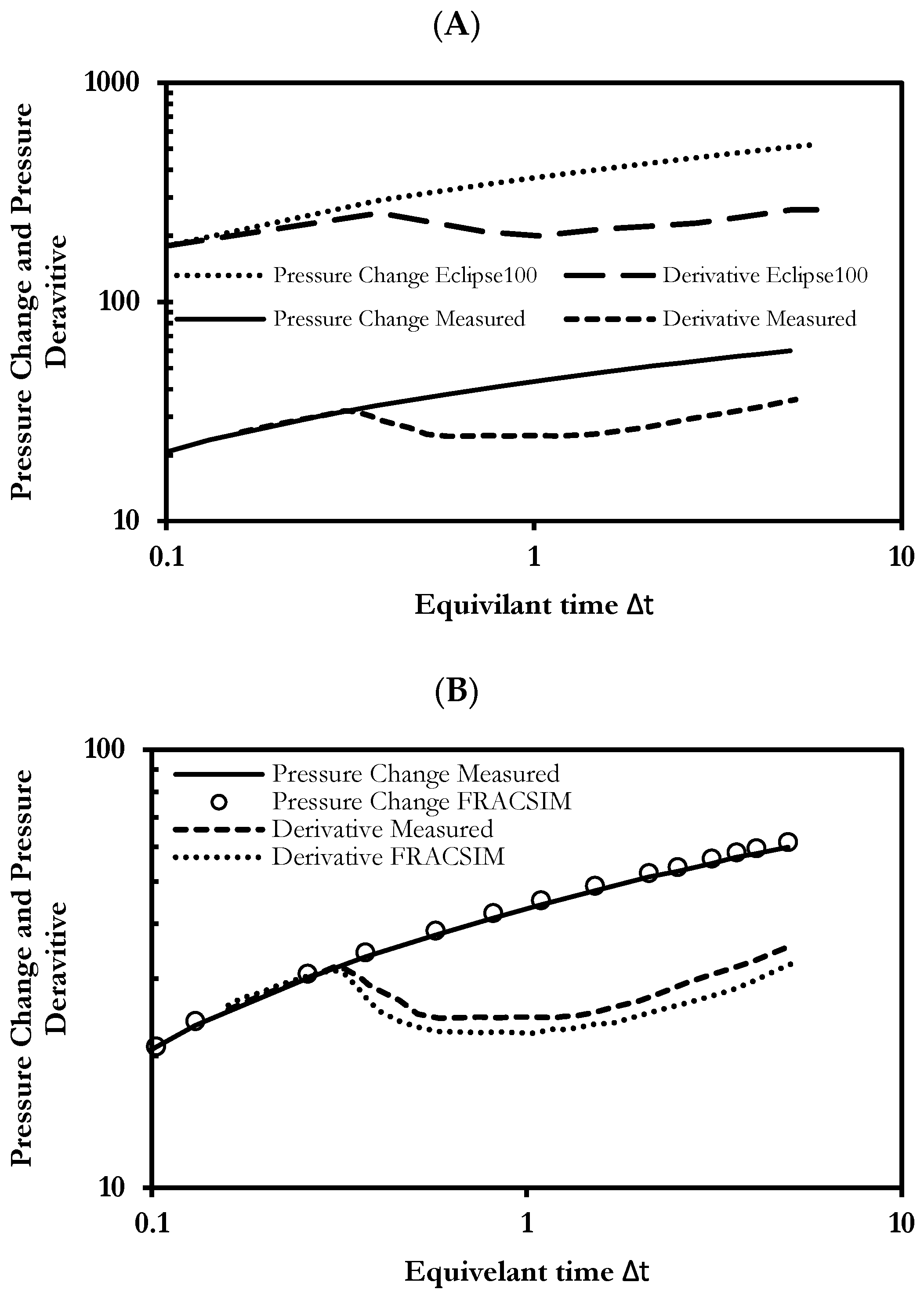
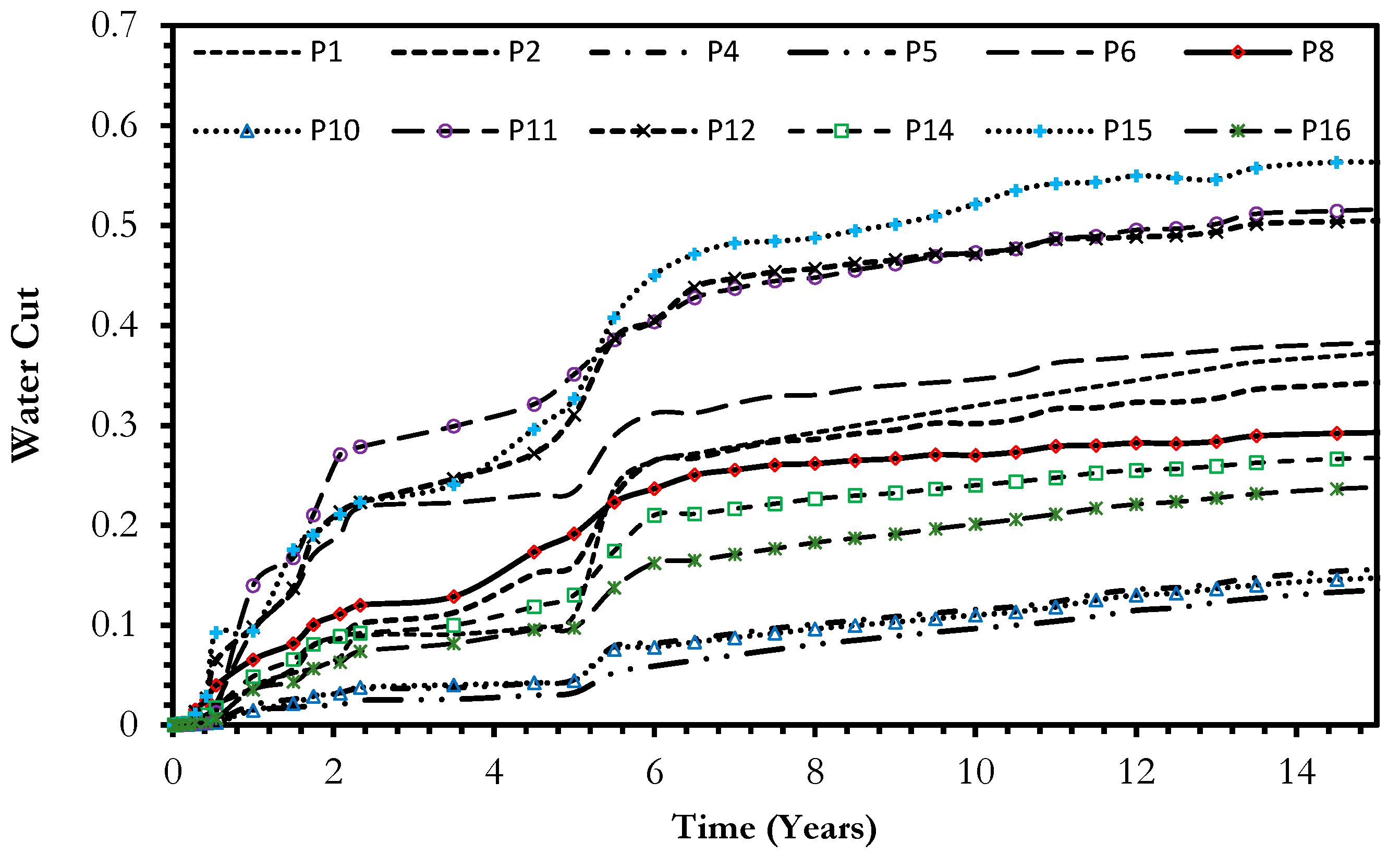
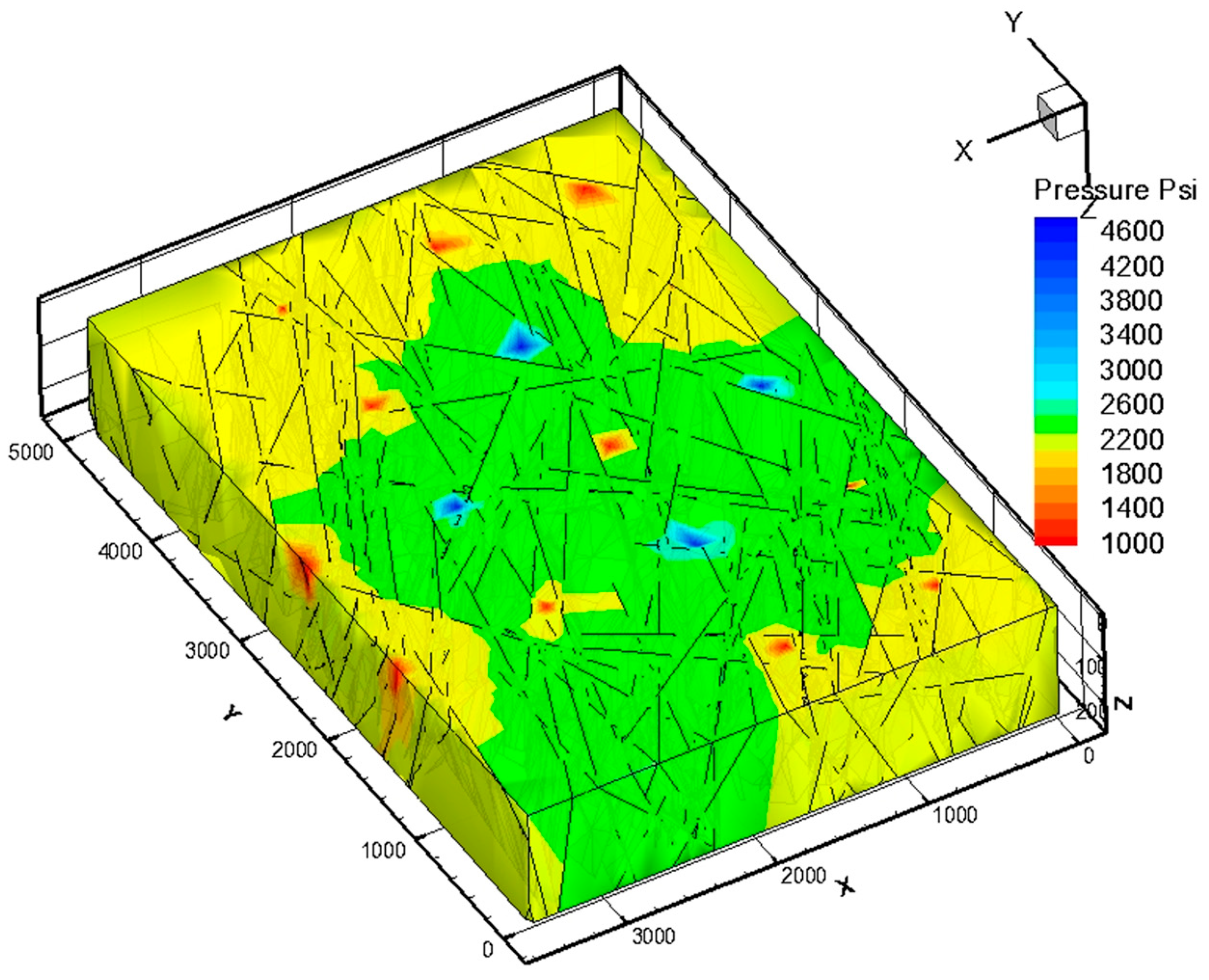
3.3. History Matching of Real Dynamic Data—Field Scale
- Generate the subsurface fracture realization using field data based on Doonechaly and Rahman (2012) approach [34].
- Utilizing periodic boundary conditions Durlofsky (1991) [35], calculate the block-based permeability tensor of the single continuum taking into account the short fractures.
- Couple the block-based permeability tensor with the discrete fracture network of long fractures using the in-house mesh generator (hybrid approach).
- Start FRACSIM in-house model to simulate pressure build-up and draw-down cycles.
- Compare the FRACSIM results with that of the measured well test data to estimate the error.
- If the error from step 5 is less than the predefined error threshold, then stop and report the optimum fracture realization with the pressure change and pressure derivative results. Otherwise, go back to step 1 to modify the fracture attribute and generate a new fracture realization. The simulated well-test results are compared with that from the build-up and draw-down test data for each realization until the error is minimized.
3.4. Sensitivity Study of Full Field Development Plan
3.5. Limitation and Future Work Recommendation
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Barenblatt, G.; Zheltov, I.; Kochina, I. Basic concepts in the theory of seepage of homogeneous liquids in fissured rocks [strata]. J. Appl. Math. Mech. 1960, 24, 1286–1303. [Google Scholar] [CrossRef]
- Warren, J.; Root, P. The Behavior of Naturally Fractured Reservoirs. Soc. Pet. Eng. J. 1963, 3, 245–255. [Google Scholar] [CrossRef]
- Kazemi, H. Pressure Transient Analysis of Naturally Fractured Reservoirs with Uniform Fracture Distribution. Soc. Pet. Eng. J. 1969, 9, 451–462. [Google Scholar] [CrossRef]
- Snow, D.T. Anisotropie Permeability of Fractured Media. Water Resour. Res. 1969, 5, 1273–1289. [Google Scholar] [CrossRef]
- Thomas, L.K.; Dixon, T.N.; Pierson, R.G. Fractured Reservoir Simulation. Soc. Pet. Eng. J. 1983, 23, 42–54. [Google Scholar] [CrossRef]
- Noorishad, J.; Mehran, M. An Upstream Finite Element Method for Solution of Transient Transport Equation in Fractured Porous Media. Water Resour. Res. 1982, 18, 588–596. [Google Scholar] [CrossRef]
- Baca, R.G.; Arnett, R.C.; Langford, D.W. Modelling fluid flow in fractured-porous rock masses by finite-element techniques. Int. J. Numer. Methods Fluids 1984, 4, 337–348. [Google Scholar] [CrossRef]
- Pruess, K. A practical method for modeling fluid and heat flow in fractured porous media. Soc. Pet. Eng. J. 1985, 25, 14–26. [Google Scholar] [CrossRef]
- Wu, Y.-S.; Pruess, K. A multiple-porosity method for simulation of naturally fractured petroleum reservoirs. SPE Reserv. Eng. 1988, 3, 327–336. [Google Scholar] [CrossRef]
- Sudicky, E.A.; McLaren, R.A. The Laplace transform Galerkin technique for large-scale simulation of mass transport in discretely fractured porous formations. Water Resour. Res. 1992, 28, 499–514. [Google Scholar] [CrossRef]
- Kim, J.G.; Deo, M.D. Finite Element, Discrete-Fracture Model for Multiphase Flow in Porous Media. AIChE J. 2000, 46, 1120–1130. [Google Scholar] [CrossRef]
- Karimi-Fard, M.; Firoozabadi, A. Numerical Simulation of Water Injection in Fractured Media Using The Discrete-Fracture Model and The Galerkin Method. SPEREE 2003, 6, 117–126. [Google Scholar] [CrossRef]
- Niessner, J.; Helmig, R.; Jakobs, H.; Roberts, J. Interface condition and linearization schemes in the newton iterations for two-phase flow in heterogeneous porous media. Adv. Water Resour. 2005, 28, 671–687. [Google Scholar] [CrossRef]
- Hoteit, H.; Firoozabadi, A. Numerical Modeling of two Phase Flow in Heterogeneous Permeable media with Different capillarity Pressures. Adv. Water Resour. J. 2007, 31, 56–73. [Google Scholar] [CrossRef]
- Wang, Q.; Chen, X.; Jha, A.N.; Rogers, H. Natural gas from shale formation–The evolution, evidences and challenges of shale gas revolution in United States. Renew. Sustain. Energy Rev. 2014, 30, 1–28. [Google Scholar] [CrossRef]
- Wang, Q.; Li, R. Research status of shale gas: A review. Renew. Sustain. Energy Rev. 2017, 74, 715–720. [Google Scholar] [CrossRef]
- Wang, Q.; Li, S.; Li, R.; Ma, M. Forecasting U.S. shale gas monthly production using a hybrid ARIMA and metabolic nonlinear grey model. Energy 2018, 160, 378–387. [Google Scholar] [CrossRef]
- Wang, Q.; Li, S. Shale gas industry sustainability assessment based on WSR methodology and fuzzy matter-element extension model: The case study of China. J. Clean. Prod. 2019, 226, 336–348. [Google Scholar] [CrossRef]
- Berre, I.; Doster, F.; Keilegavlen, E. Flow in fractured porous media: A review of conceptual models and discretization approaches. Transp. Porous Media 2019, 130, 215–236. [Google Scholar] [CrossRef]
- Wong, D.L.Y.; Doster, F.; Geiger, S.; Francot, E.; Gouth, F. Fluid flow characterization framework for naturally fractured reservoirs using small-scale fully explicit models. Transp. Porous Media 2020, 134, 399–434. [Google Scholar] [CrossRef]
- Kazemi, H.; Merrill, L., Jr.; Porterfield, K.; Zeman, P. Numerical simulation of water–oil flow in naturally fractured reservoirs. Soc. Petrol. Eng. J. 1976, 16, 317–326. [Google Scholar] [CrossRef]
- Quandalle, P.; Sabathier, J.C. Typical features of a multipurpose reservoir simulator. SPE Reserv. Eng. 1989, 4, 475–480. [Google Scholar] [CrossRef]
- Ramirez, B.; Kazemi, H.; Al-Kobaisi, M.; Ozkan, E.; Atan, S. A critical review for proper use of water/oil/gas transfer functions in dual-porosity naturally fractured reservoirs: Part I. SPE Reserv. Eval. Eng. 2009, 12, 200–210. [Google Scholar] [CrossRef]
- Bourbiaux, B. Fractured reservoir simulation: A challenging and rewarding issue. Oil Gas Sci. Technol.–Rev. De L’institut Français Du Pétrole 2010, 65, 227–238. [Google Scholar] [CrossRef]
- Lough, M.; Lee, S.; Kamath, J. An efficient boundary integral formulation for flow through fractured porous media. J. Comput. Phys. 1998, 143, 462–483. [Google Scholar] [CrossRef]
- Park, Y.C.; Sung, W.M. Development of a FEM Reservoir Model Equipped with an Effective Permeability Tensor and its Application to Naturally Fractured Reservoirs. 2000. Available online: https://www.tandfonline.com/doi/abs/10.1080/00908310290086545 (accessed on 9 June 2023).
- Gupta, A.; Penuela, G.; Avila, R. An Integrated Approach to the Determination of Permeability Tensors for Naturally Fractured Reservoirs. J. Can. Pet. Technol. 2001, 40. [Google Scholar] [CrossRef]
- Teimoori, A.; Chen, Z.; Rahman, S.S.; Tran, T. Effective Permeability Calculation Using Boundary Element Method in Naturally Fractured Reservoirs. Pet. Sci. Technol. 2004, 23, 693–709. [Google Scholar] [CrossRef]
- Hussain, S.T.; Rahman, S.S.; Azim, R.A.; Haryono, D.; Regenauer-Lieb, K. Multiphase fluid flow through fractured porous media supported by innovative laboratory and numerical methods for estimating relative permeability. Energy Fuels Am. Chem. Soc. J. 2021, 35, 17372–17388. [Google Scholar] [CrossRef]
- Abdelazim, R. Integration of static and dynamic reservoir data to optimize the generation of subsurface fracture map. J. Pet. Explor. Prod. Technol. 2016, 6, 691–703. [Google Scholar] [CrossRef]
- Kirsch, G. Theory of Elasticity and Application in Strength of Materials. Z. Des Ver. Dtsch. Ingenieure 1898, 42, 797–807. [Google Scholar]
- Detournay, E.; Cheng, A.-D. Poroelastic response of a borehole in a non-hydrostatic stress field. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1988, 25, 171–182. [Google Scholar] [CrossRef]
- Fahad, M. Simulation of Fluid Flow and Estimation of Production from Naturally Fractured Reservoirs. Ph.D. Thesis, UNSW Sydney, Kensington, Australia, 2013. [Google Scholar]
- Doonechaly, N.G.; Rahman, S. 3D hybrid tectono-stochastic modeling of naturally fractured reservoir: Application of finite element method and stochastic simulation technique. Tectonophysics 2012, 541, 43–56. [Google Scholar] [CrossRef]
- Durlofsky, L.J. Numerical calculation of equivalent grid block permeability tensors for heterogeneous porous media. Water Resour. Res. 1991, 27, 699–708. [Google Scholar] [CrossRef]

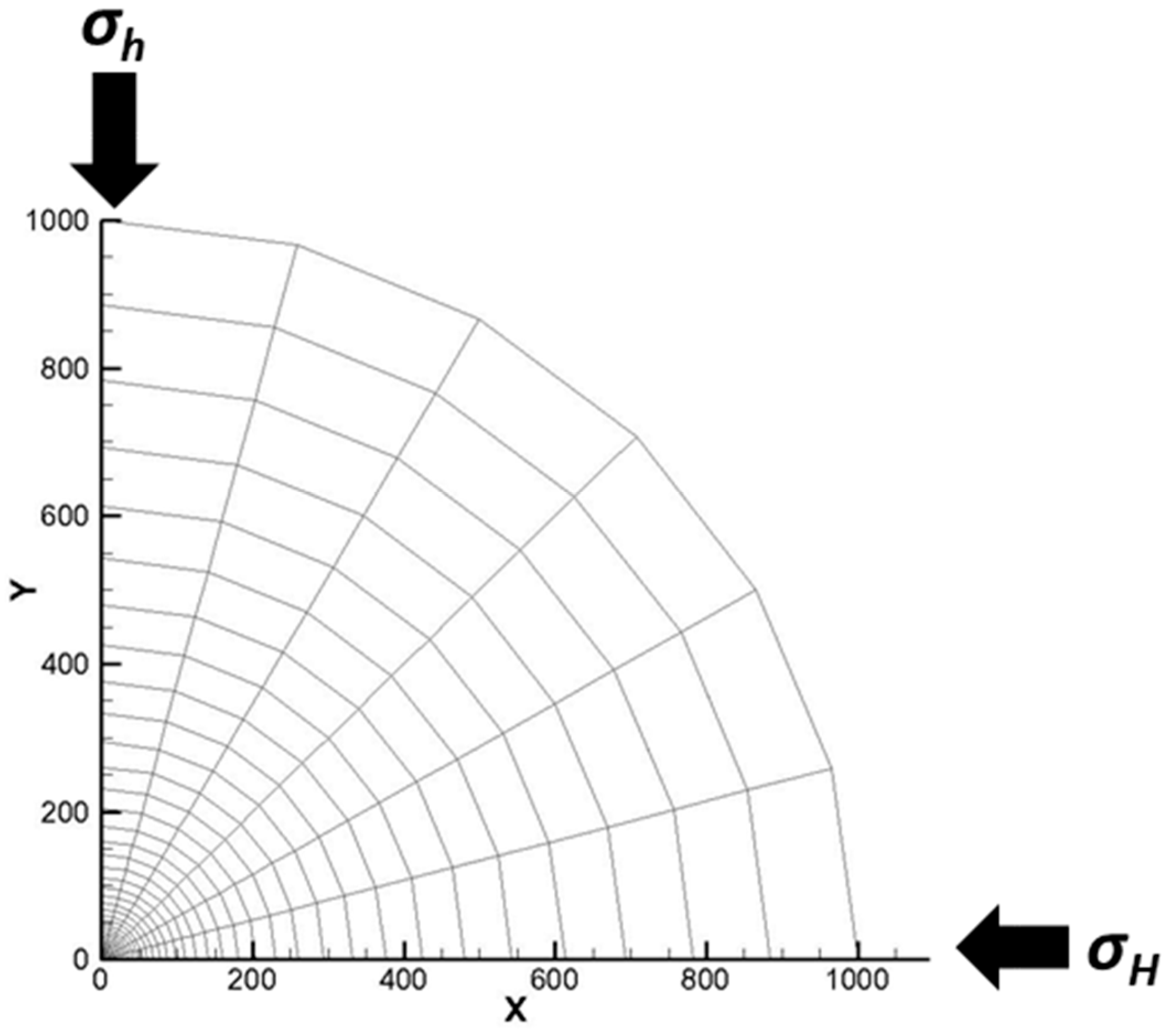
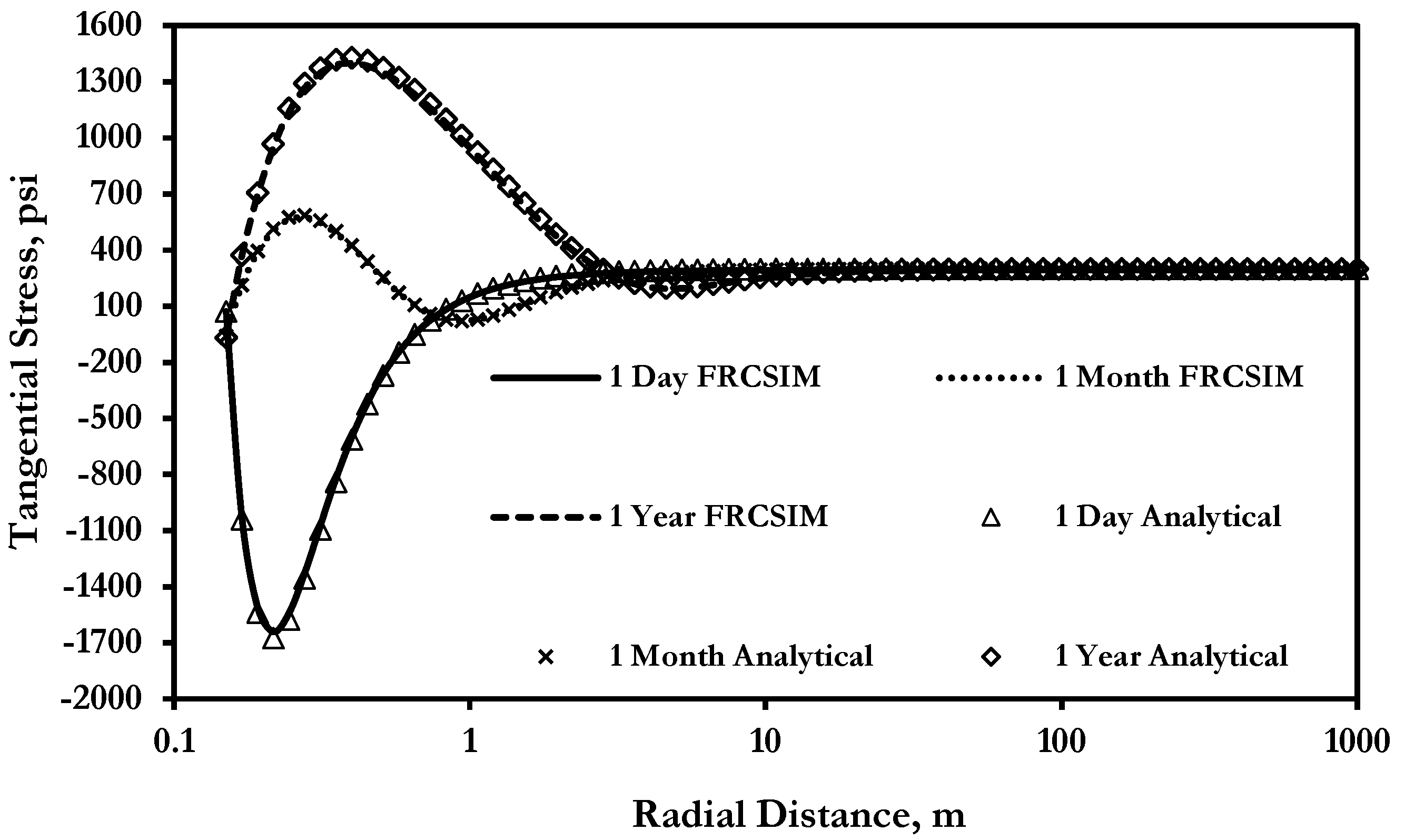
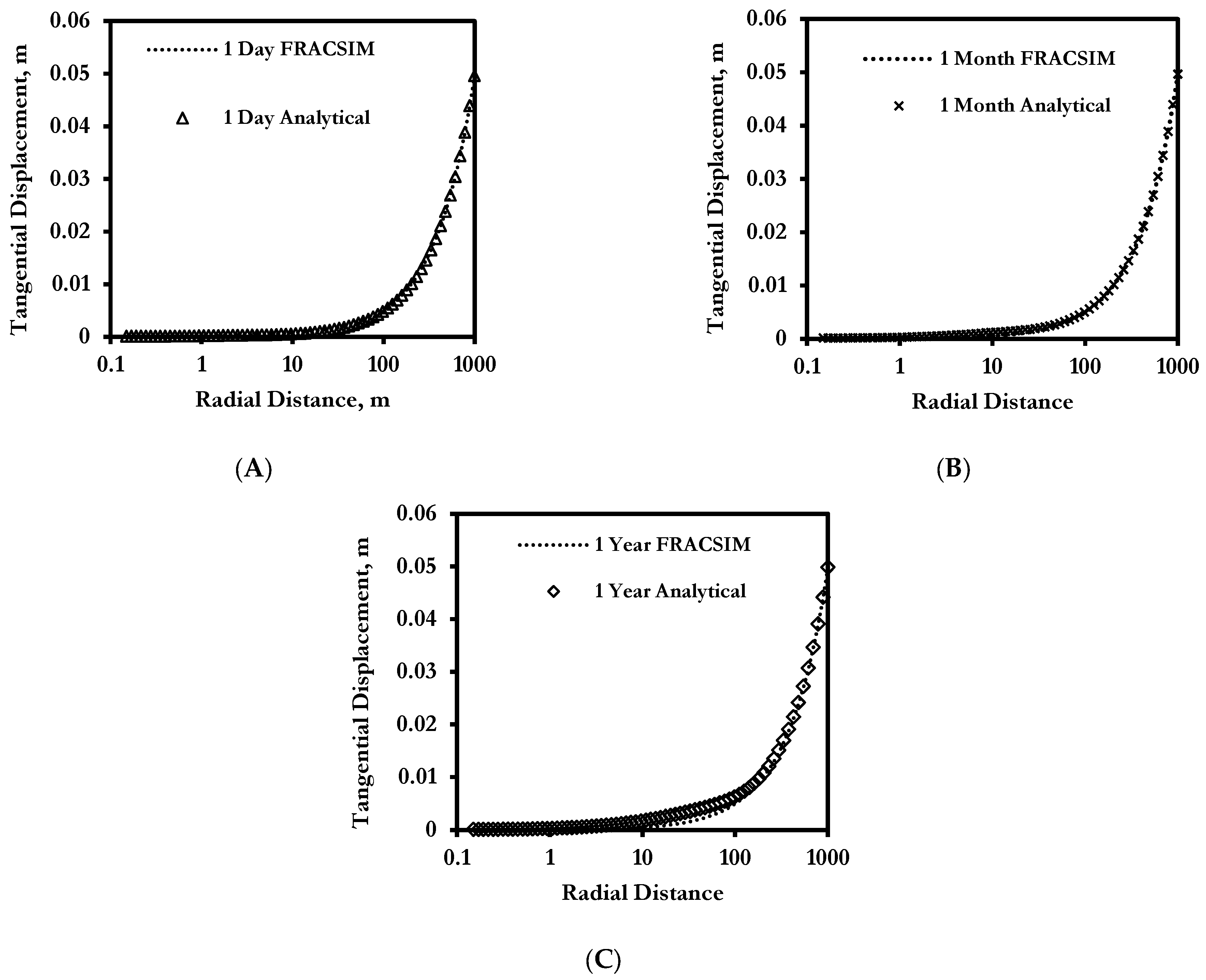
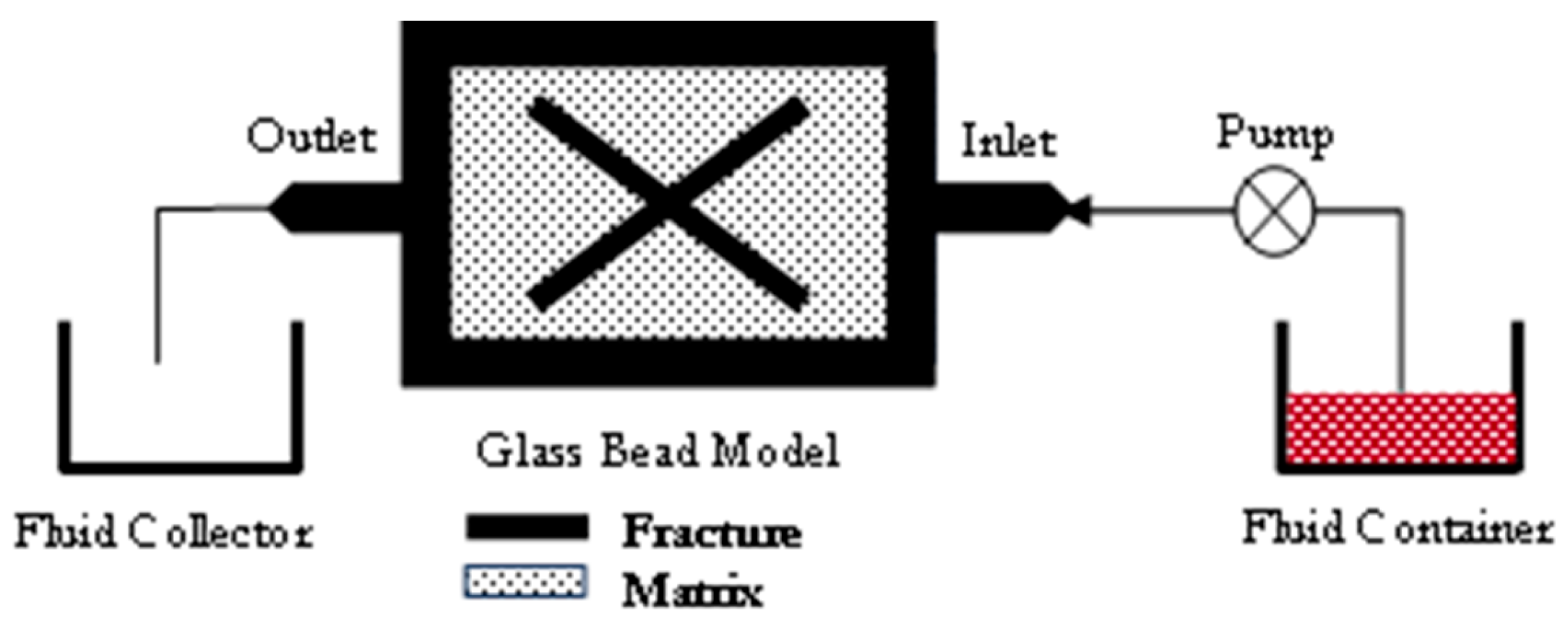


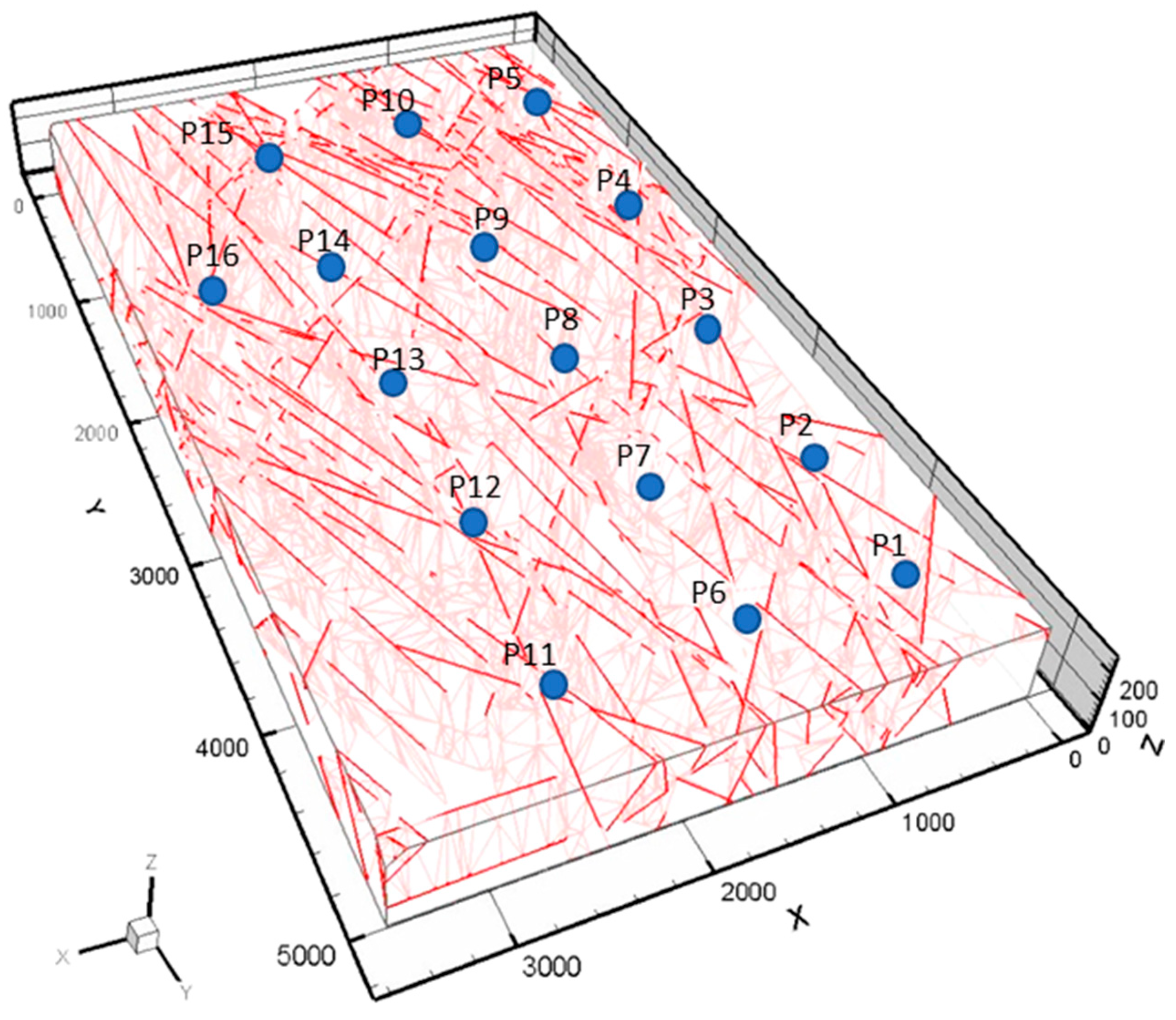

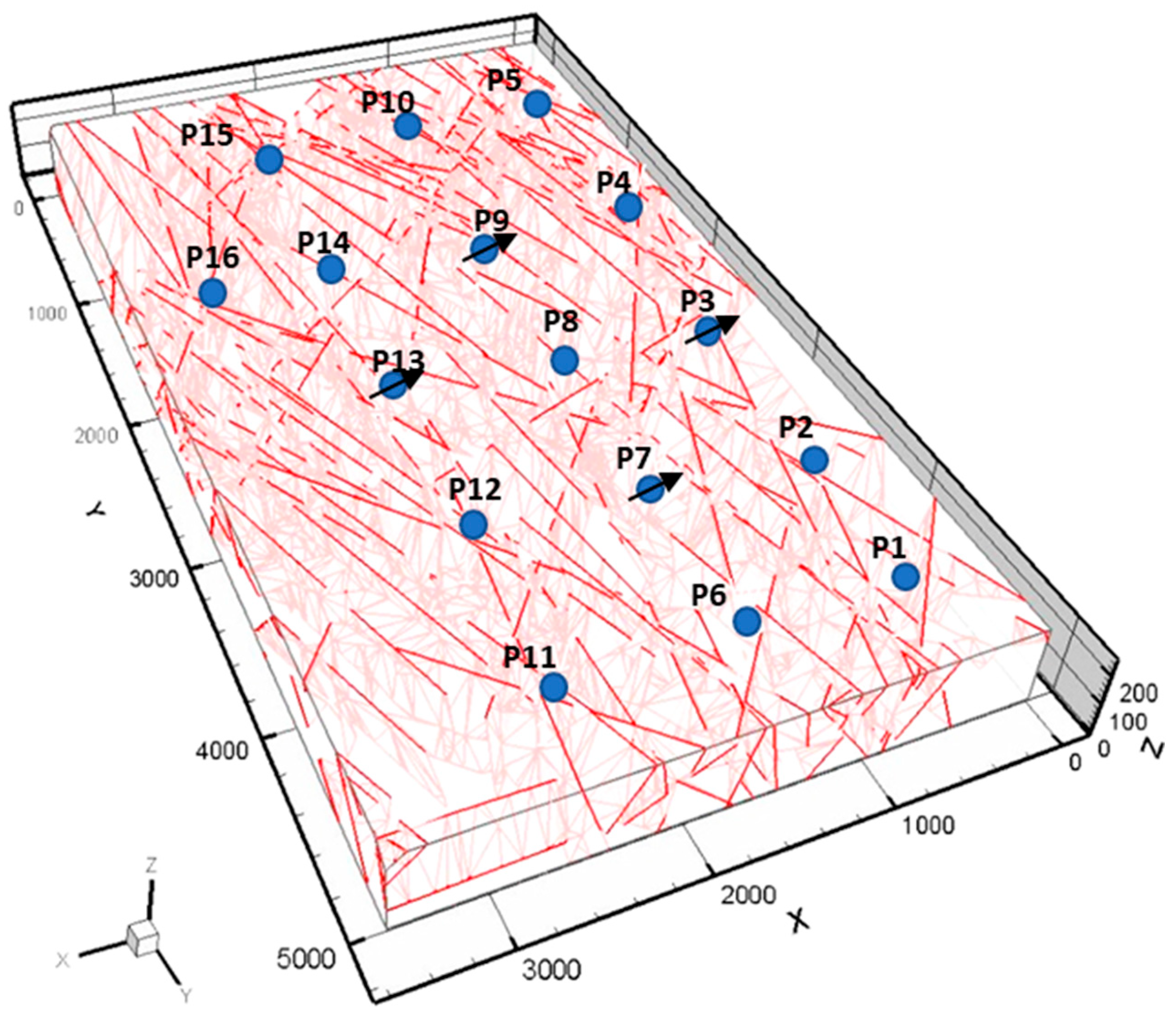


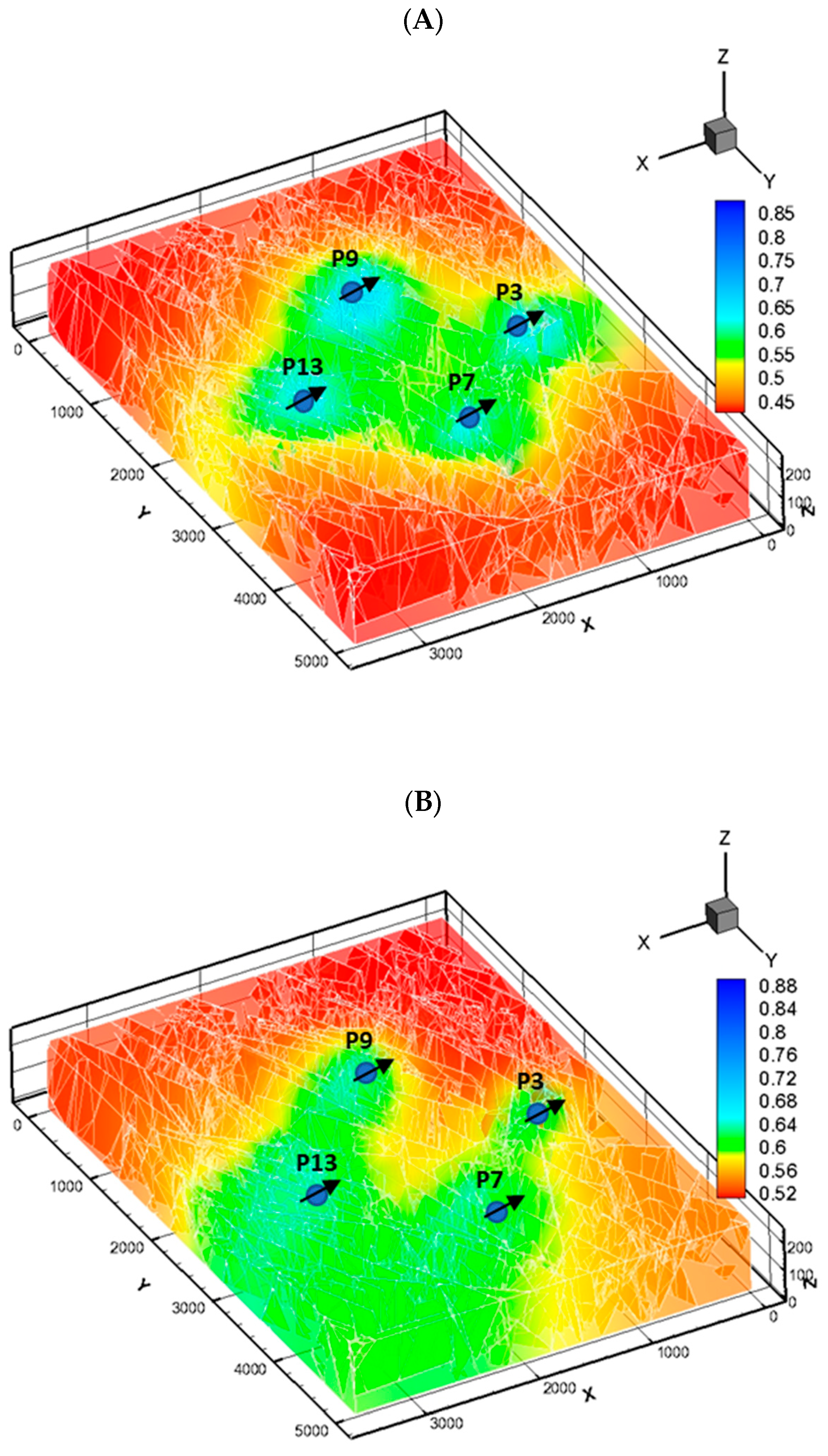
| Parameter | Value |
|---|---|
| Young’s modulus | 40 GPa |
| Poisson’s ratio | 0.2 |
| Porosity ϕ | 0.1 |
| Water compresibility Cw | 1.0 × 10−4 psi−1 |
| Water viscosity µw | 0.1 cp |
| Biot’s coefficient | 1.0 |
| Maximum stress | 5800 psi |
| Minimum stress | 5500 psi |
| Initial reservoir pressure | 5500 psi |
| Wellbore pressure | 1000 psi |
| Formation permeability Kx | 0.01 md |
| Formation permeability Ky | 0.01 md |
| Wellbore radius rw | 0.1 m |
| Reservoir drainage radius re | 1000 m |
| Parameter | Value |
|---|---|
| Reservoir dimensions | 500 m × 500 m × 250 m |
| Wellbore entry | partially penetration (90 m) |
| Matrix permeability | 0.0095 mD |
| Matrix porosity | 0.02 |
| Initial water saturation | 0.34 |
| Fracture aperture | 7.06 × 10−3 mm |
| Initial fracture intensity | 0.15 m−1 |
| Fractal dimension (D) | 1.25 |
| Fracture permeability | 100 D |
| Fracture porosity | 0.1 |
| Initial reservoir pressure | 4200 psi |
| Horizontal stresses | 4400 psi |
| Vertical stress | 6000 psi |
| Fluid viscosity | 1.38 cp |
| Fluid compressibility | 10−5 psi−1 |
| Production time before shut in (tp) | 72 h |
| Production flow rate before shut in | 5571 bbl/d |
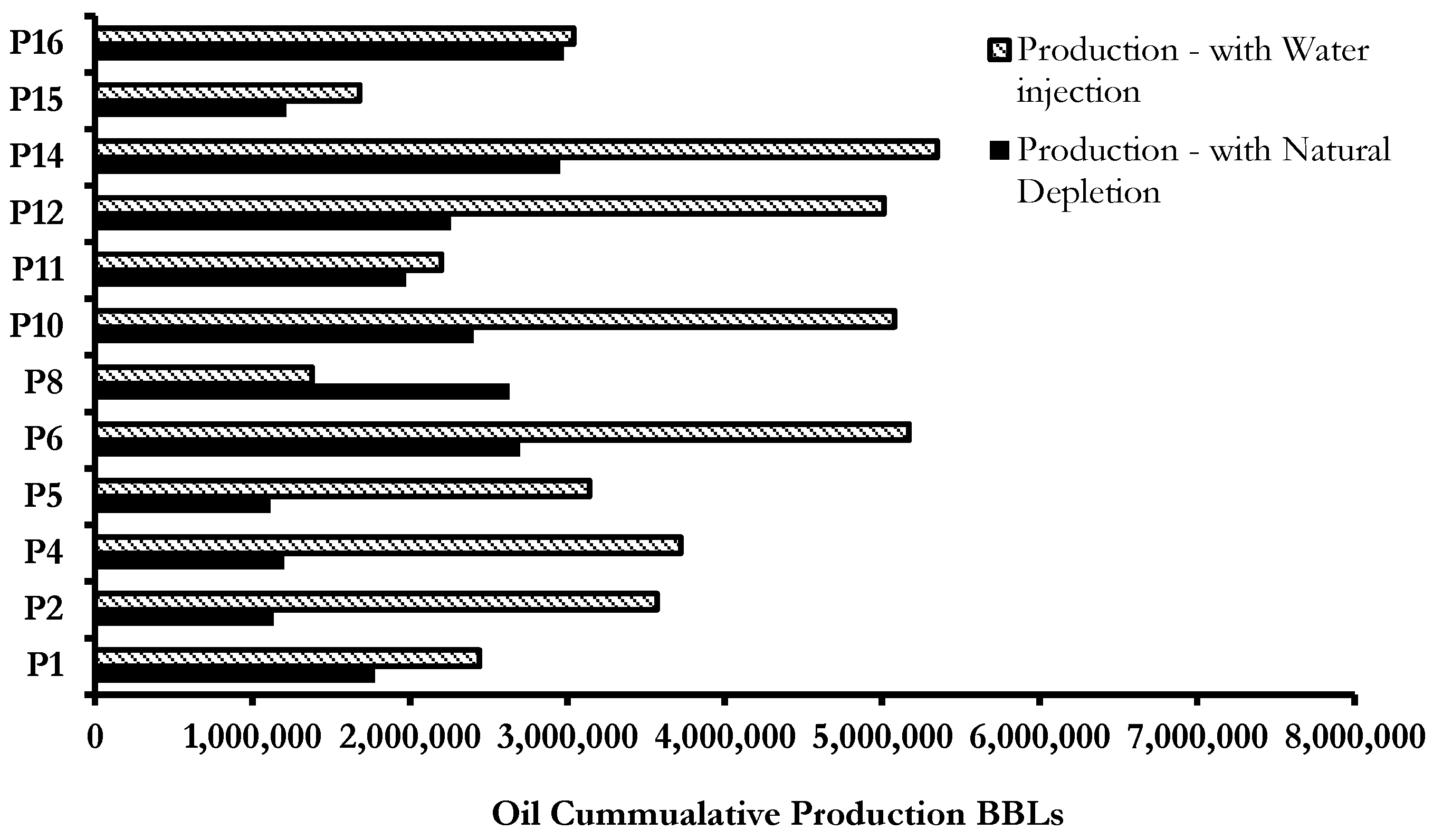
| Well # | Cumulative Oil Production—Natural Depletion | Cumulative Oil Production—Water Flooding | Difference % |
|---|---|---|---|
| P1 | 1.78 × 106 | 2.44 × 106 | 37.43 |
| P2 | 1.13 × 106 | 3.57 × 106 | 214.89 |
| P4 | 1.20 × 105 | 3.72 × 106 | 210.00 |
| P5 | 1.11 × 106 | 3.14 × 106 | 182.23 |
| P6 | 2.70 × 106 | 5.17 × 106 | 91.49 |
| P8 | 2.63 × 106 | 1.38 × 106 | −47.55 |
| P10 | 2.40 × 106 | 5.08 × 106 | 111.28 |
| P11 | 1.98 × 106 | 2.20 × 106 | 11.37 |
| P12 | 2.26 × 106 | 5.01 × 106 | 121.77 |
| P14 | 2.95 × 106 | 5.35 × 106 | 81.06 |
| P15 | 1.21 × 106 | 1.68 × 106 | 38.42 |
| P16 | 2.98 × 106 | 3.04 × 106 | 2.06 |
| Total | 2.41 × 107 | 4.18 × 107 | 73.51 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abdel Azim, R.; Alatefi, S.; Alkouh, A. A Coupled Poro-Elastic Fluid Flow Simulator for Naturally Fractured Reservoirs. Energies 2023, 16, 6476. https://doi.org/10.3390/en16186476
Abdel Azim R, Alatefi S, Alkouh A. A Coupled Poro-Elastic Fluid Flow Simulator for Naturally Fractured Reservoirs. Energies. 2023; 16(18):6476. https://doi.org/10.3390/en16186476
Chicago/Turabian StyleAbdel Azim, Reda, Saad Alatefi, and Ahmad Alkouh. 2023. "A Coupled Poro-Elastic Fluid Flow Simulator for Naturally Fractured Reservoirs" Energies 16, no. 18: 6476. https://doi.org/10.3390/en16186476
APA StyleAbdel Azim, R., Alatefi, S., & Alkouh, A. (2023). A Coupled Poro-Elastic Fluid Flow Simulator for Naturally Fractured Reservoirs. Energies, 16(18), 6476. https://doi.org/10.3390/en16186476








