A Novel Smart Charging Method to Mitigate Voltage Fluctuation at Fast Charging Stations
Abstract
:1. Introduction
1.1. Motivation
1.2. Related Works
1.3. Contribution
- Investigation of the quality of the service voltage in the presence of FCSs in distribution grids;
- Proposal of a novel smart charging approach that quantifies and mitigates the impact of an FCS on voltage fluctuation and light flicker;
- Development of a novel set of smart charging constraints that offers more flexibility to customers than those currently in the literature, and by which customer can select the charging power according to priority of time or cost.
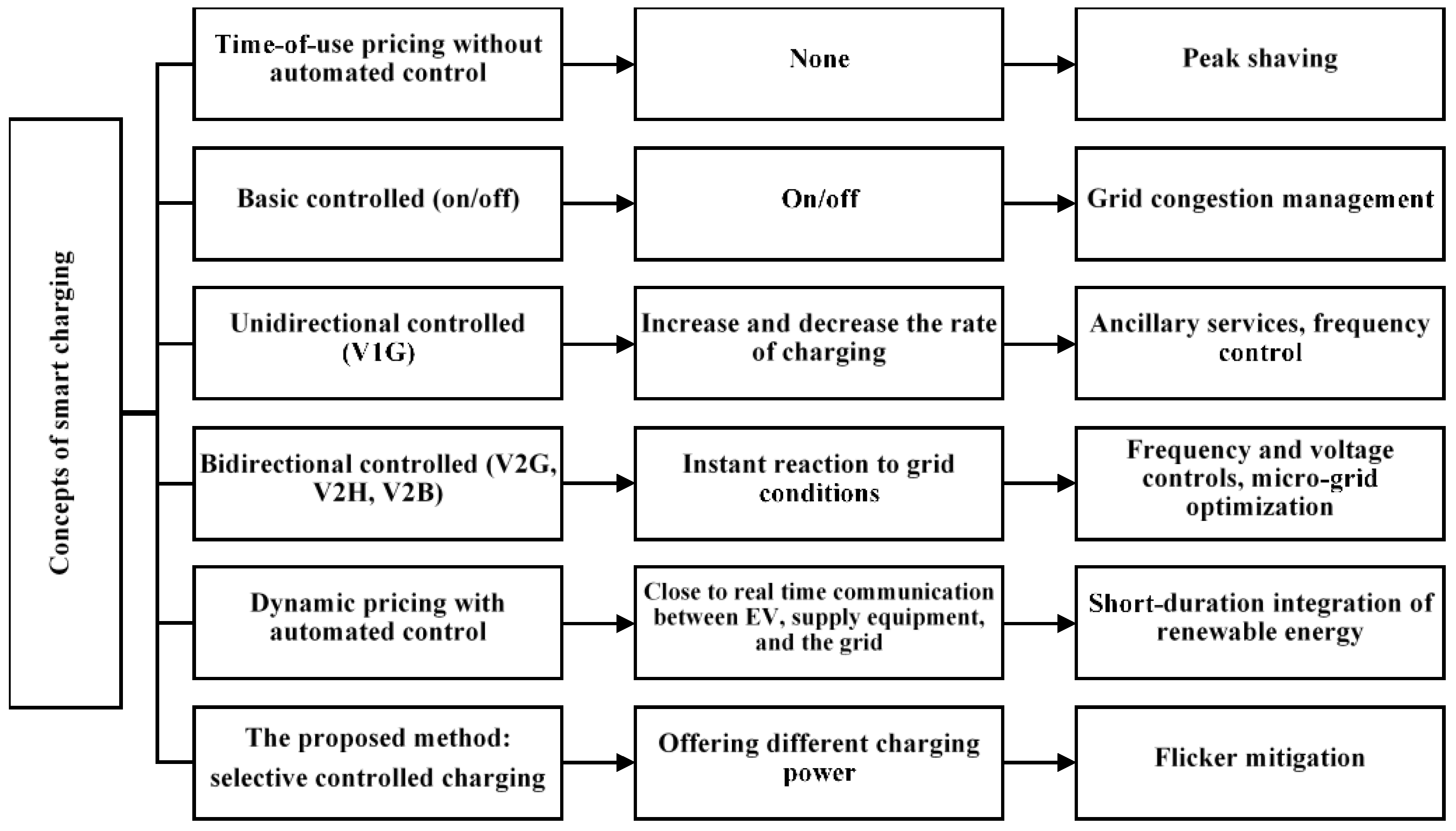
2. Input Data Required for a Vehicle Charging Model
2.1. Number of BPEVs
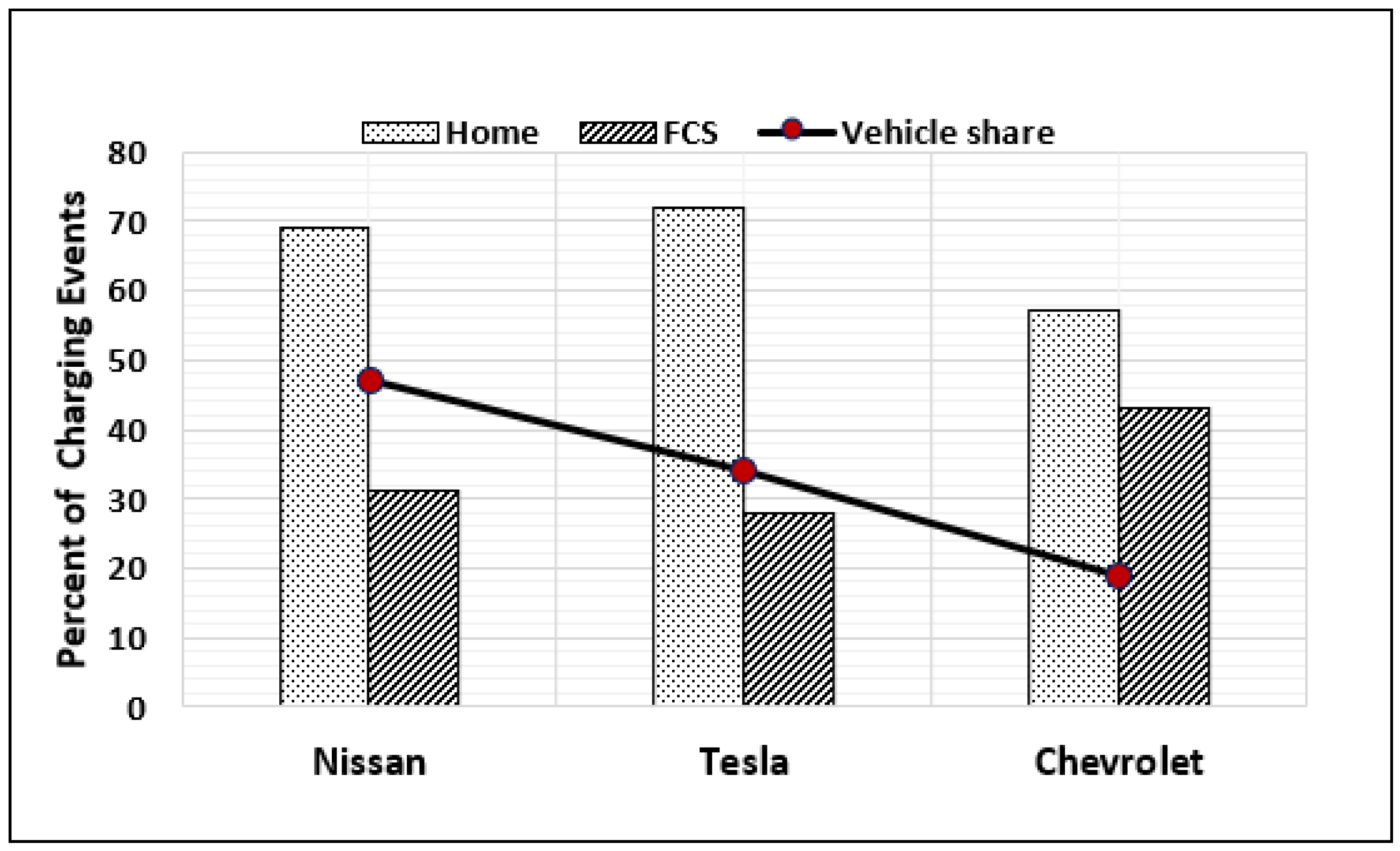
2.2. Types of BPEVs, Their Shares, and Locations
- Three different BPEVs are utilized in the system because of their high market shares representing more than 50% [25]. These vehicles are the Tesla Model S, the Chevy Bold, and the Nissan Leaf;
- The Nissan Leaf is assumed to represent the group of BPEVs with small battery capacity, ;
- The group of BPEVs with medium battery capacity is represented by the Chevy Bolt.
- The Tesla Model S is utilized to represent the group of BPEVs with large battery capacity, .
- As battery prices decrease, the battery capacity increases, thus it would not be sufficient (if there were a willingness and need) to charge the vehicle from slow charging stations;
- The EV market share is forecast to be 50% by 2030 [27]. As the BPEV market share increases, public charging stations need to meet the increased share by increasing the utilization rate. This is not achievable by assuming slow charging stations;
- Considering the value of charging time, especially for ride-hailing EVs, as well as commercial and automated car-sharing EVs, FCSs are extremely important in reducing downtime due to charging.
2.3. BPEV Arrival Rate at FCSs
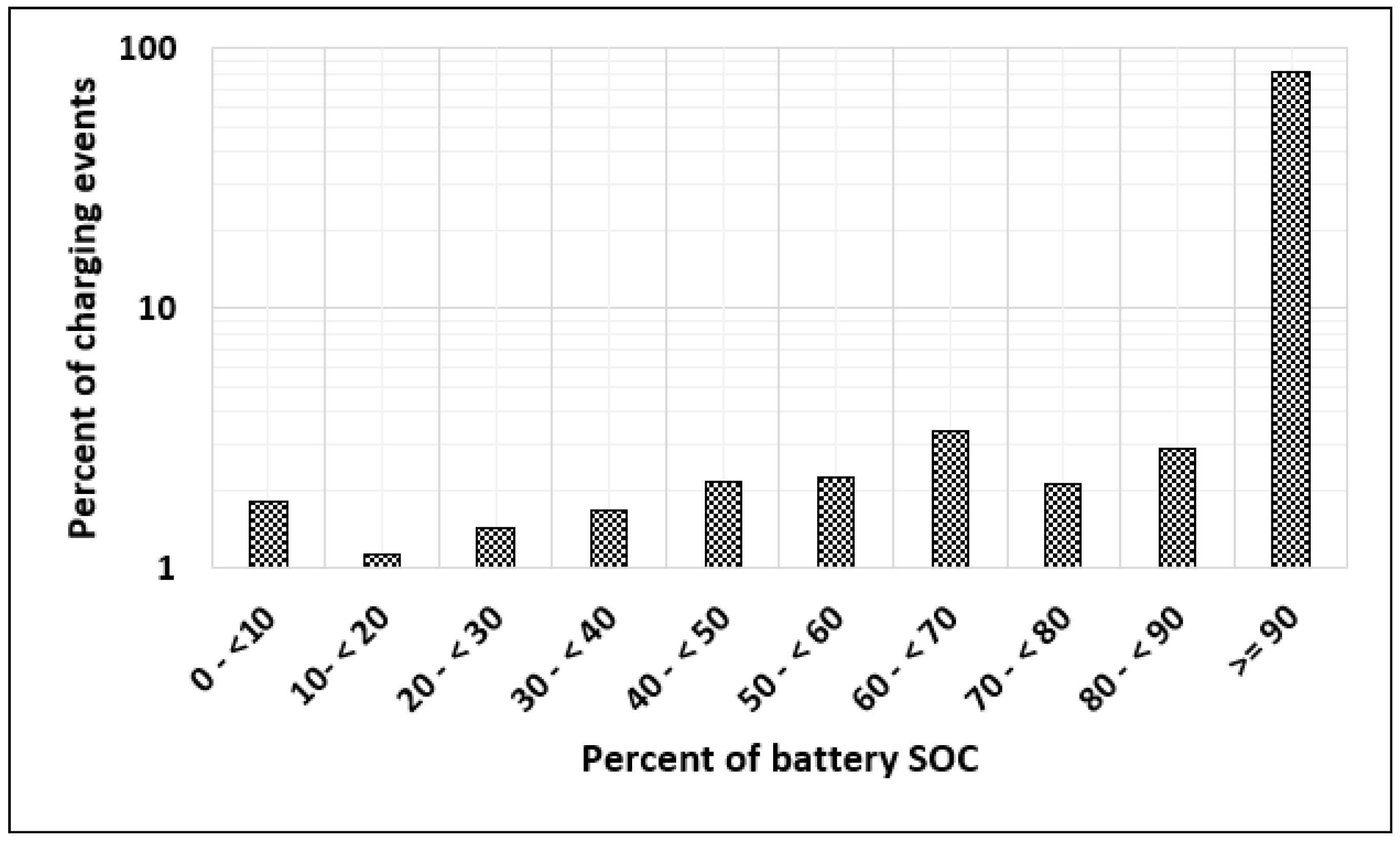
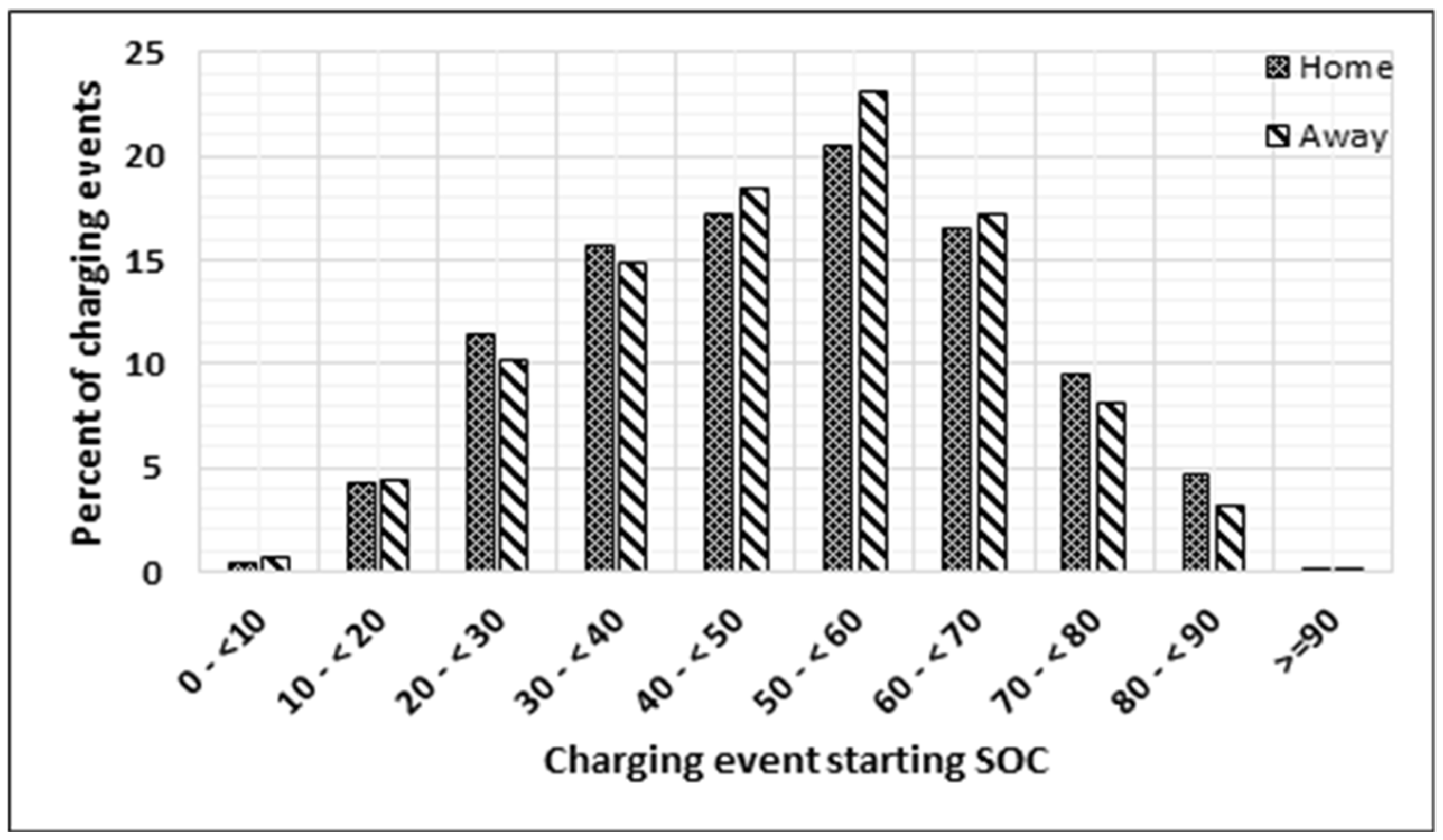
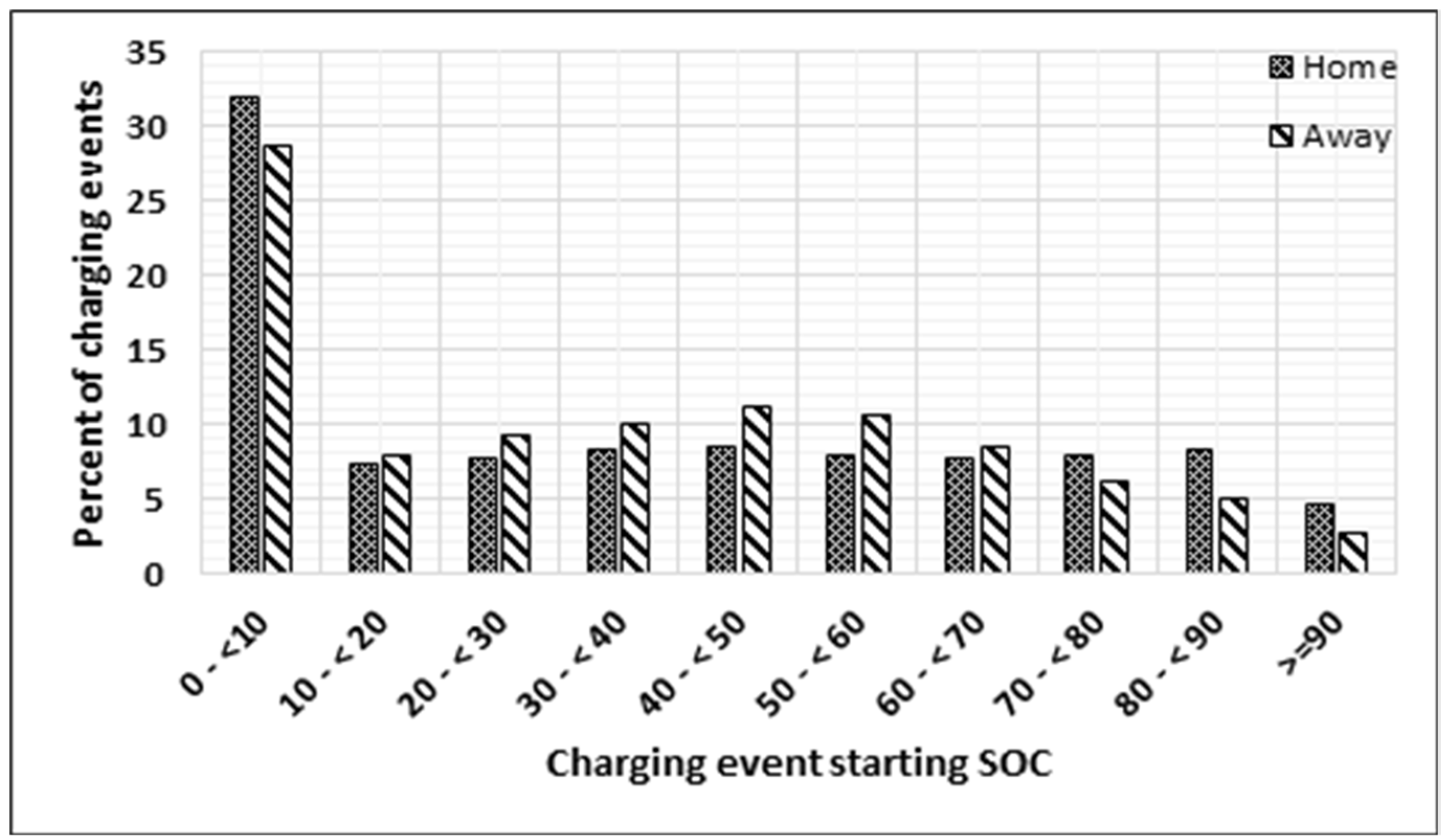
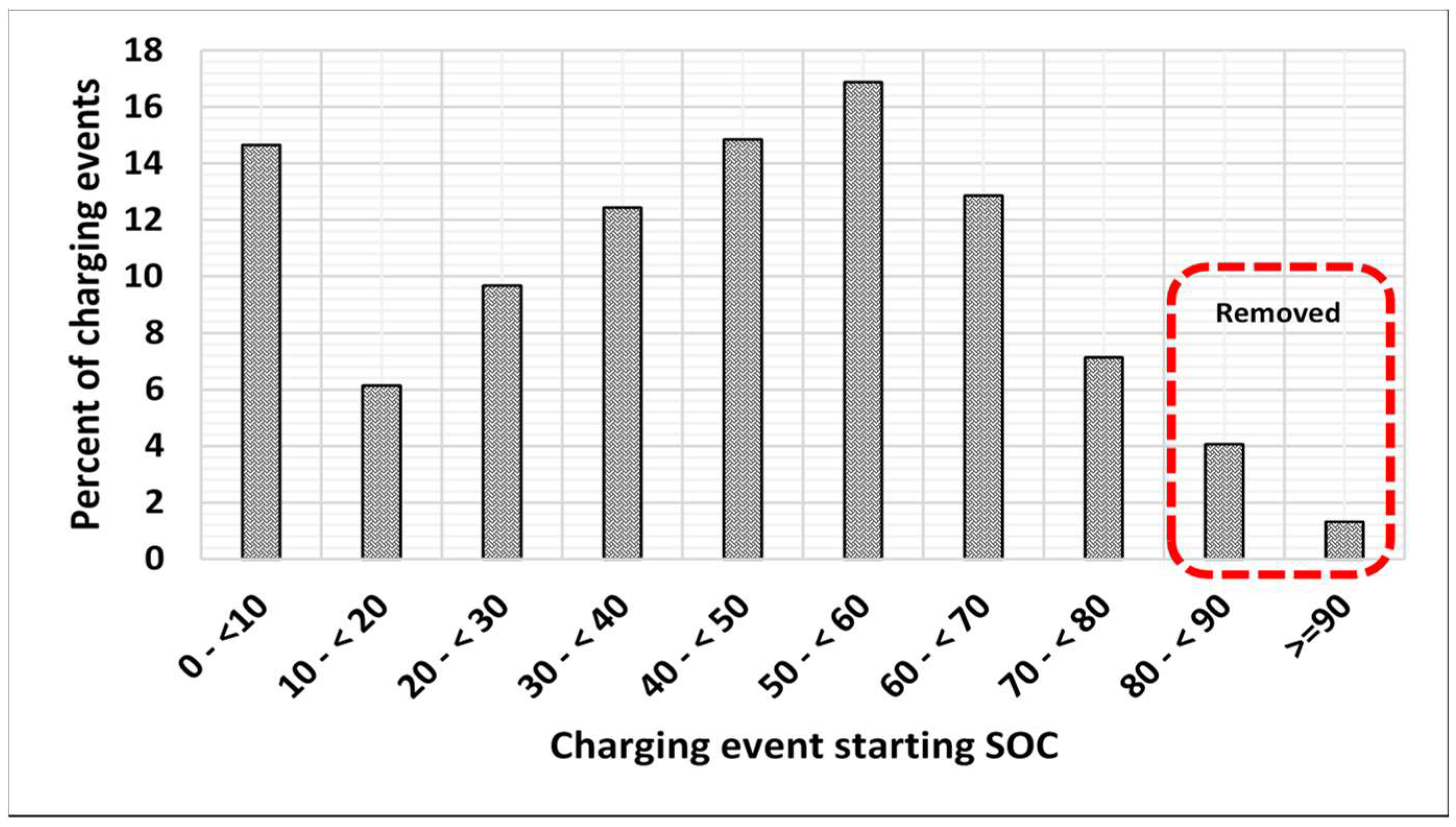
2.4. Level of Batteries Charged Form an FCS
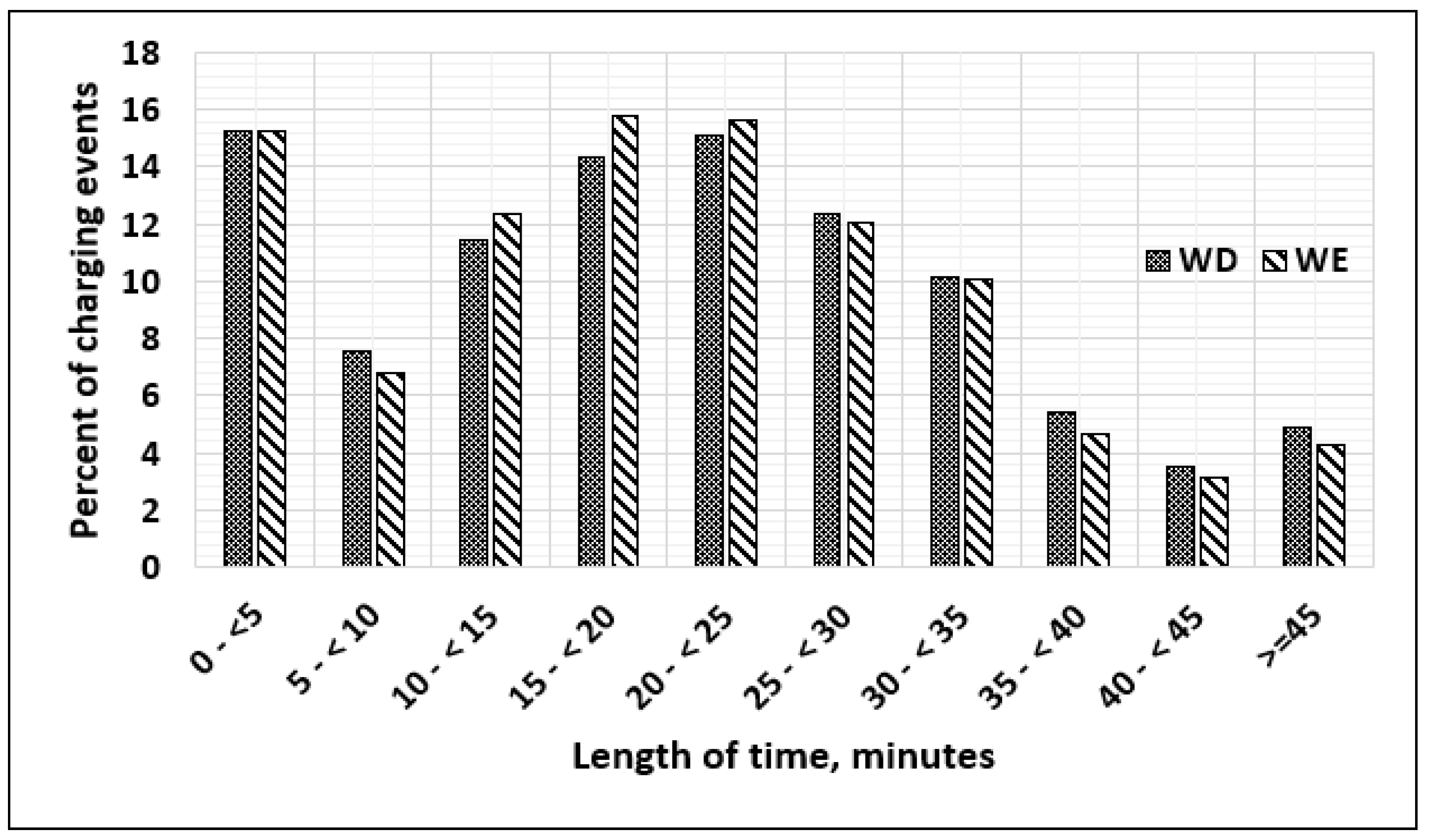
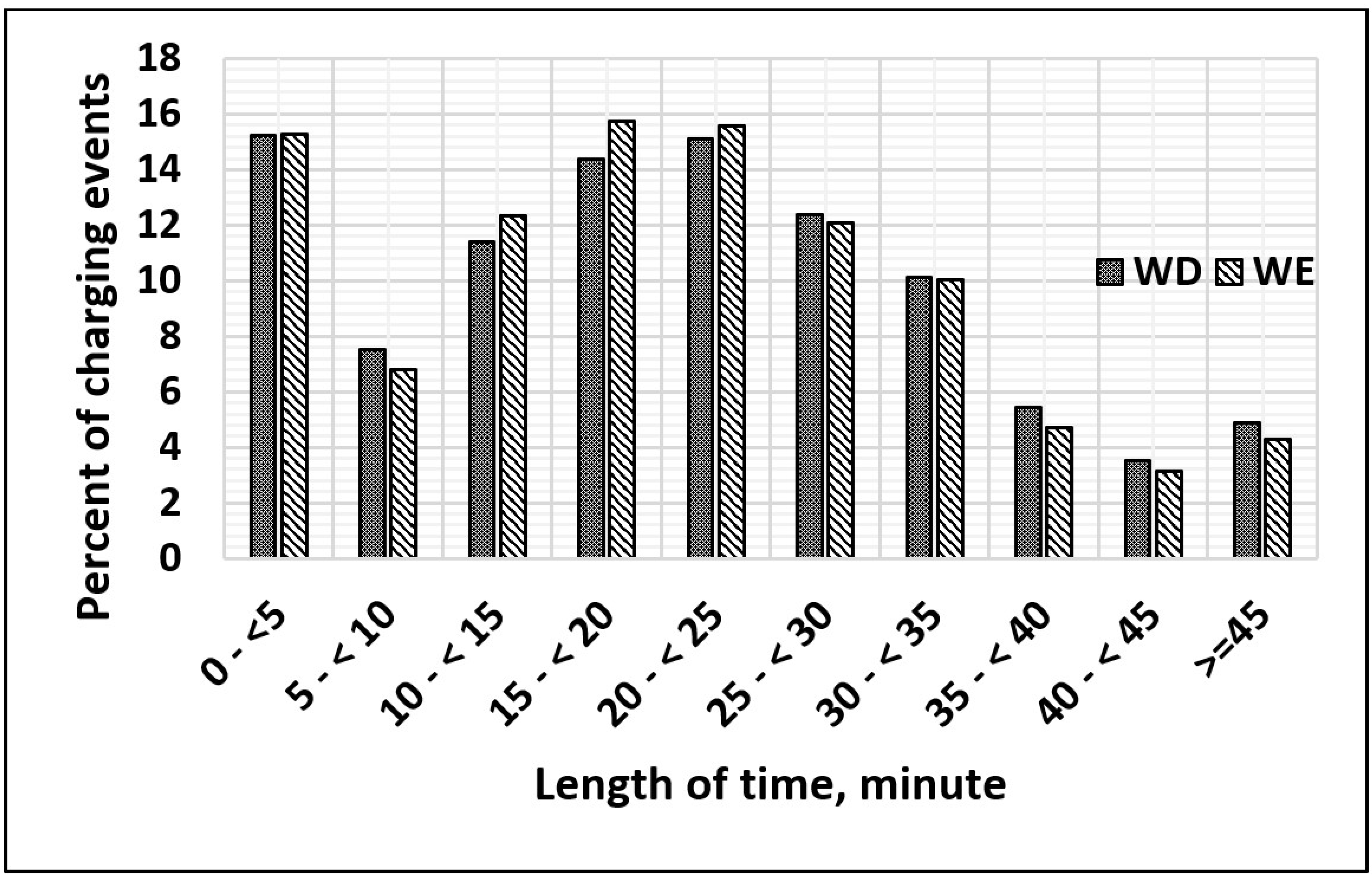
2.5. Length of Time for BPEVs to Draw Power Form an FCS
2.6. Length of Time for BPEVs to Be Connected to an FCS
3. Proposed Smart Charge and Mathematical Models
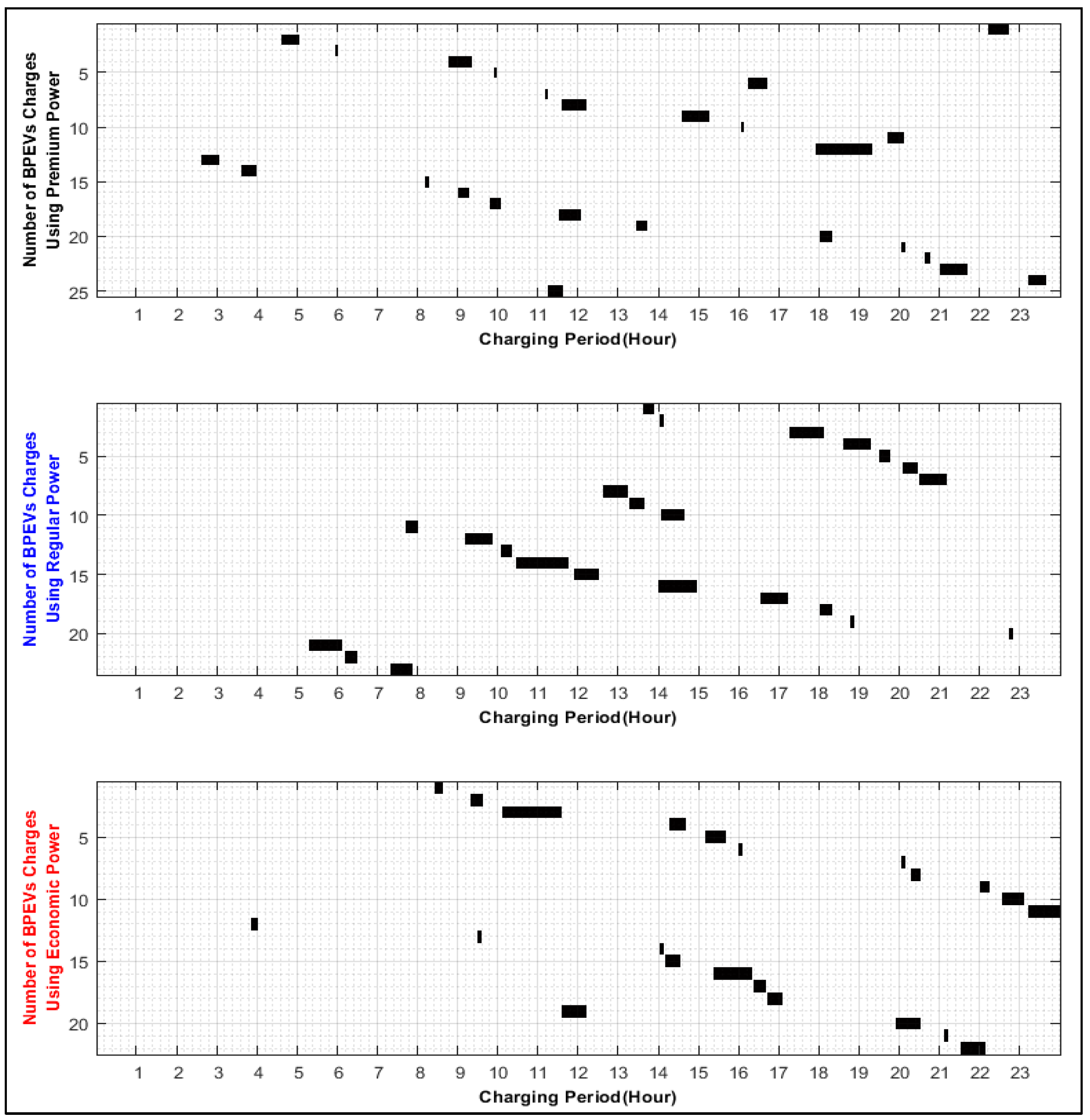
3.1. Charging Power
- Premium;
- Regular;
- Economic.
- Small battery capacity, i.e., the Nissan Leaf ();
- Medium battery capacity, i.e., the Chevrolet Bolt ();
- Large battery capacity, i.e., the Tesla Model S ().
3.2. Smart Charging Constraints
3.3. Flicker Assessment and Relation to Output Power of the FCS
- Determining the maximum relative voltage change, , caused by the offending load (FCS);
- Computing the corresponding flicker severity raised by that change;
- Adding flicker severity from all fluctuating loads.
4. Results and Discussion
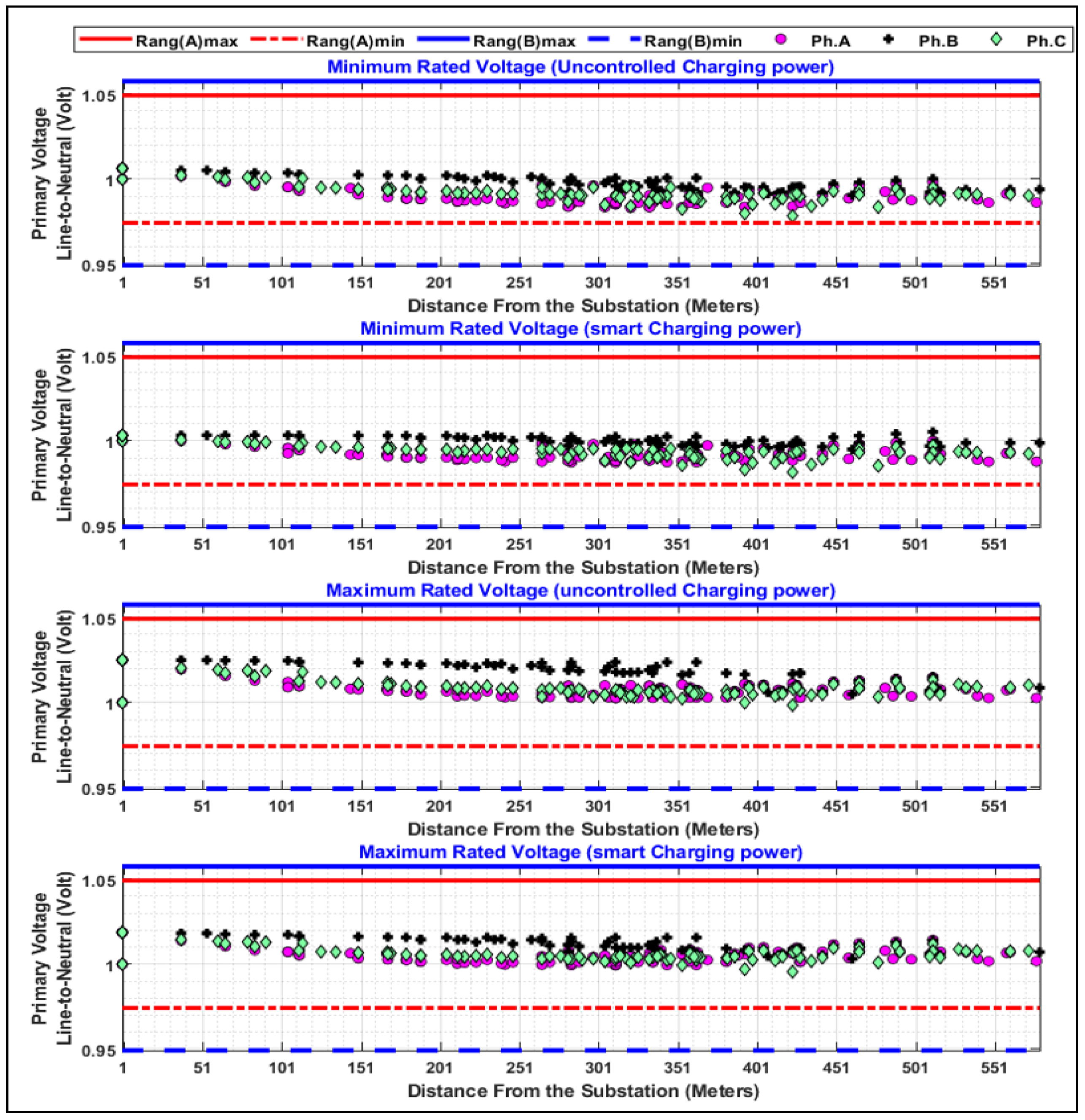
4.1. Test System
| Algorithm 1. Estimation and Assessment of Smart and Uncontrolled Charging Profiles. |
| 1: Start |
| 2: Input: |
| Number of EVs in the system |
| Share of Evs with respect of vehicles in the system |
| Number of residential houses in the system |
| Number of vehicles per house |
| Share of BPEVs charges from fcs |
| Share of BPEVs with small battery capacity that charge from FCS |
| Share of BPEVs with medium battery capacity that charge from FCS |
| Share of BPEVs with large battery capacity that charge from FCS |
| Probability distribution of vehicle arrival rate and time at the FCS |
| Probability distribution of battery state-of-charge at the start of charging event at an fcs. |
| Probability distribution of length of time with BPEV drawing power from an FCS |
| Probability distribution of battery state-of-charge at end of charging from an FCS The maximum output power per each port of the FCS The upper limits of premium, regular, and economic charging power The lower limits of premium, regular, and economic charging power FCS efficiency |
| 3: For each BPEV do |
| 4: Generate a sample representing BPEV arrival rate and time 5: Determine vehicle’s battery capacity 6: Generate a sample representing BPEV state-of-charge at the start of charging 7: Generate a sample representing BPEV state-of-charge at the end of charging |
| 8: If the port is not available and |
| 9: PBEV may or may not wait until the port becomes available, based on a random |
| number uniformly distribution |
| 10: else |
| 11: BPEV will wait until the port becomes available |
| 12: End If |
| 13: If smart charging is selected |
| 14: Determine the charging power, premium, regular, or economic |
| 15: Consider the corresponding upper and lower limits 16: Determine the required state-of-charge accordingly |
| 17: Determine the departure time and then the charging duration is estimated 18: End If 19: Affix the estimated duration to the daily demand profile of smart charging starting from the hour in which arrival time was estimated |
| 20: If uncontrolled charging is selected |
| 21: Utilize the maximum charging power |
| 22: Determine the required state-of-charge accordingly |
| 23: Determine the departure time and then the charging duration is estimated 24: End If 25: Affix the estimated duration to the daily demand profile of uncontrolled charging starting from the hour in which arrival time was estimated |
| 26: End For 27: Affix the generated daily profile to the IEEE 123 test system to the node in which FCS is connected 28: Run the power flow analysis and report the voltages at all buses for 24 h 29: Assess the flicker at point-of-common coupling in which the FCS is installed |
| 30: End |
4.2. Charging Methods
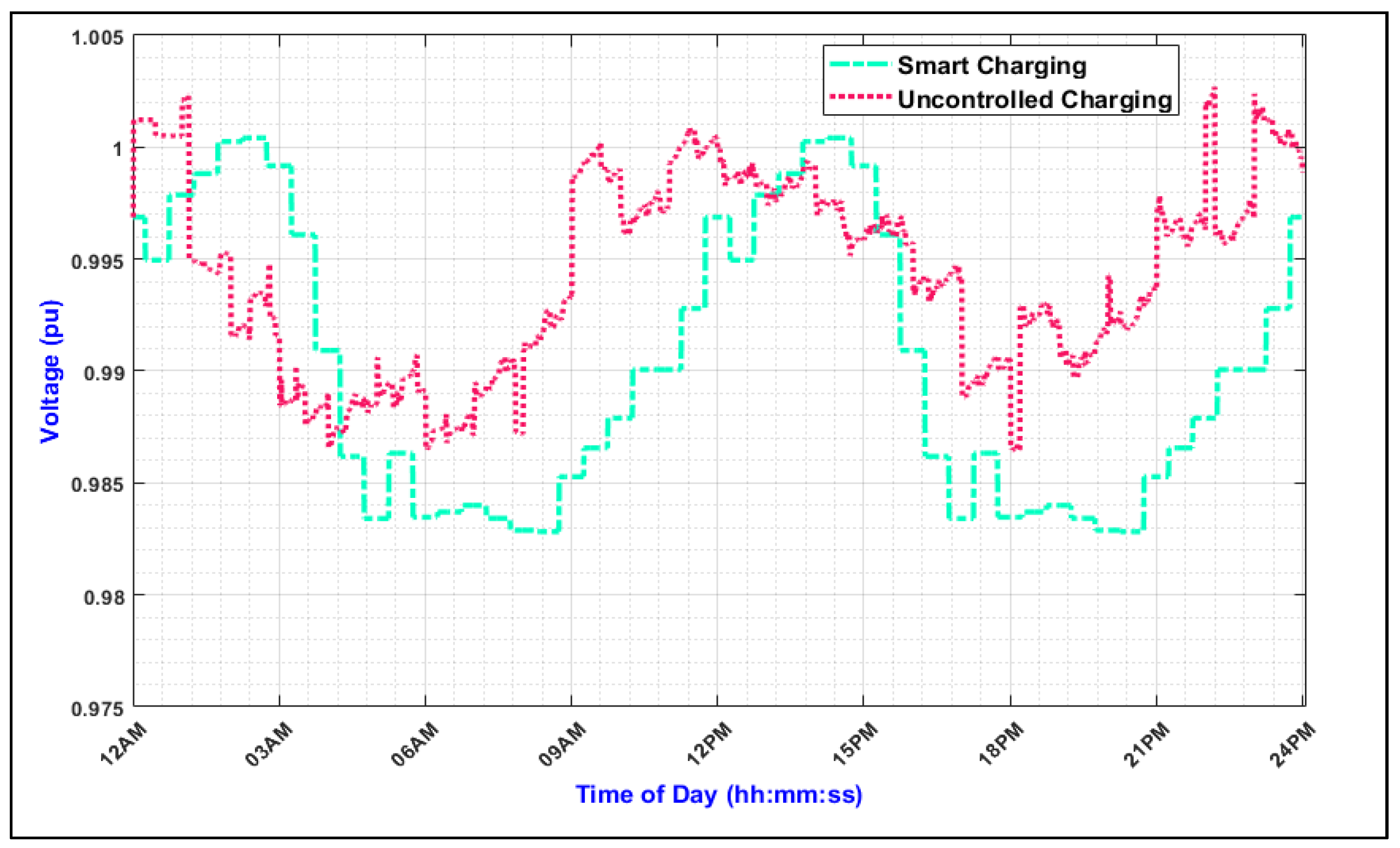
4.2.1. Uncontrolled Charging
4.2.2. Smart Charging
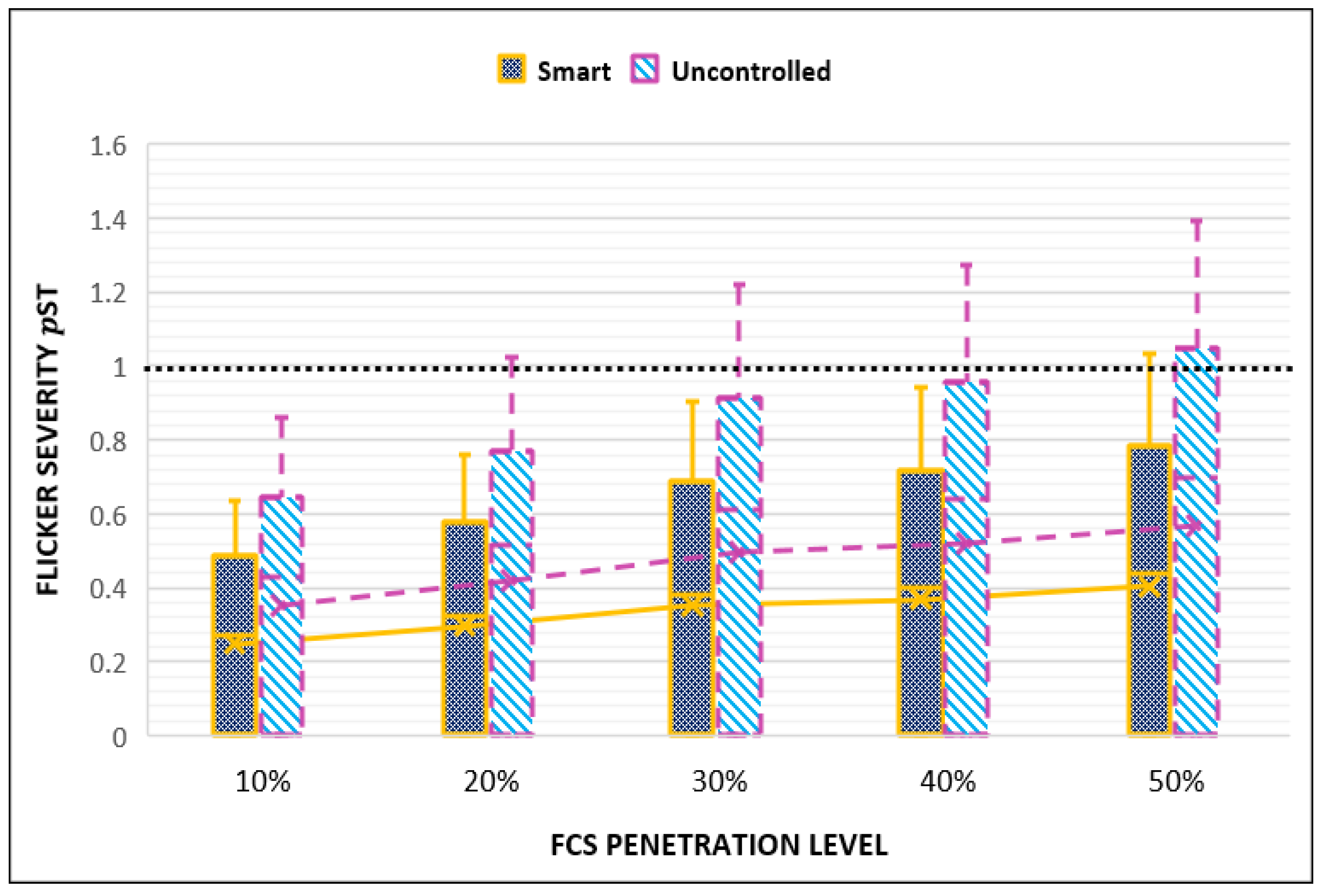
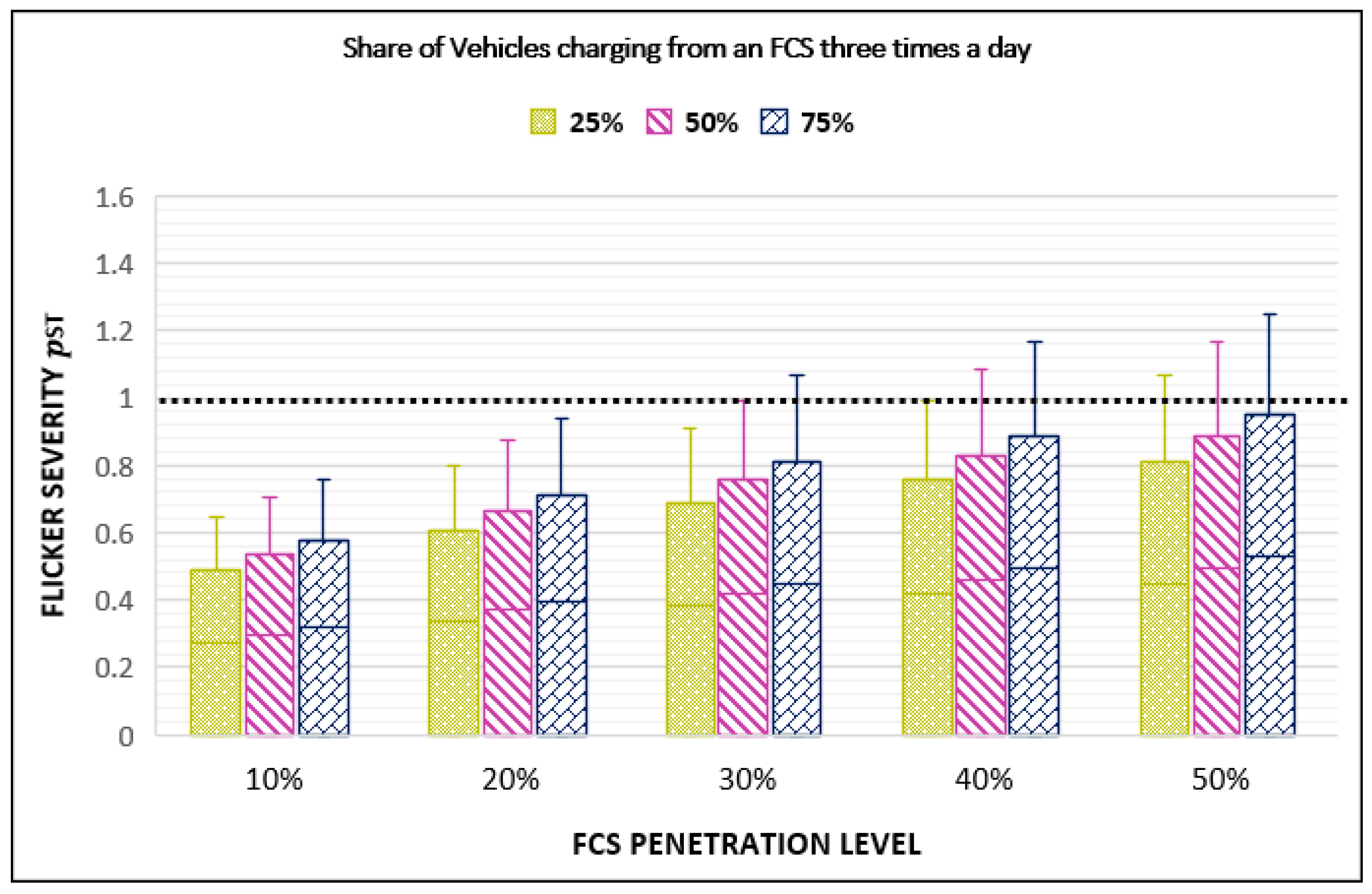
4.3. Effect of Uncontrolled and Smart Charging on Flicker Seversity
4.4. Effect of Full Battery Charging on Flicker Severity
4.5. Effect of Flicker Enverlope
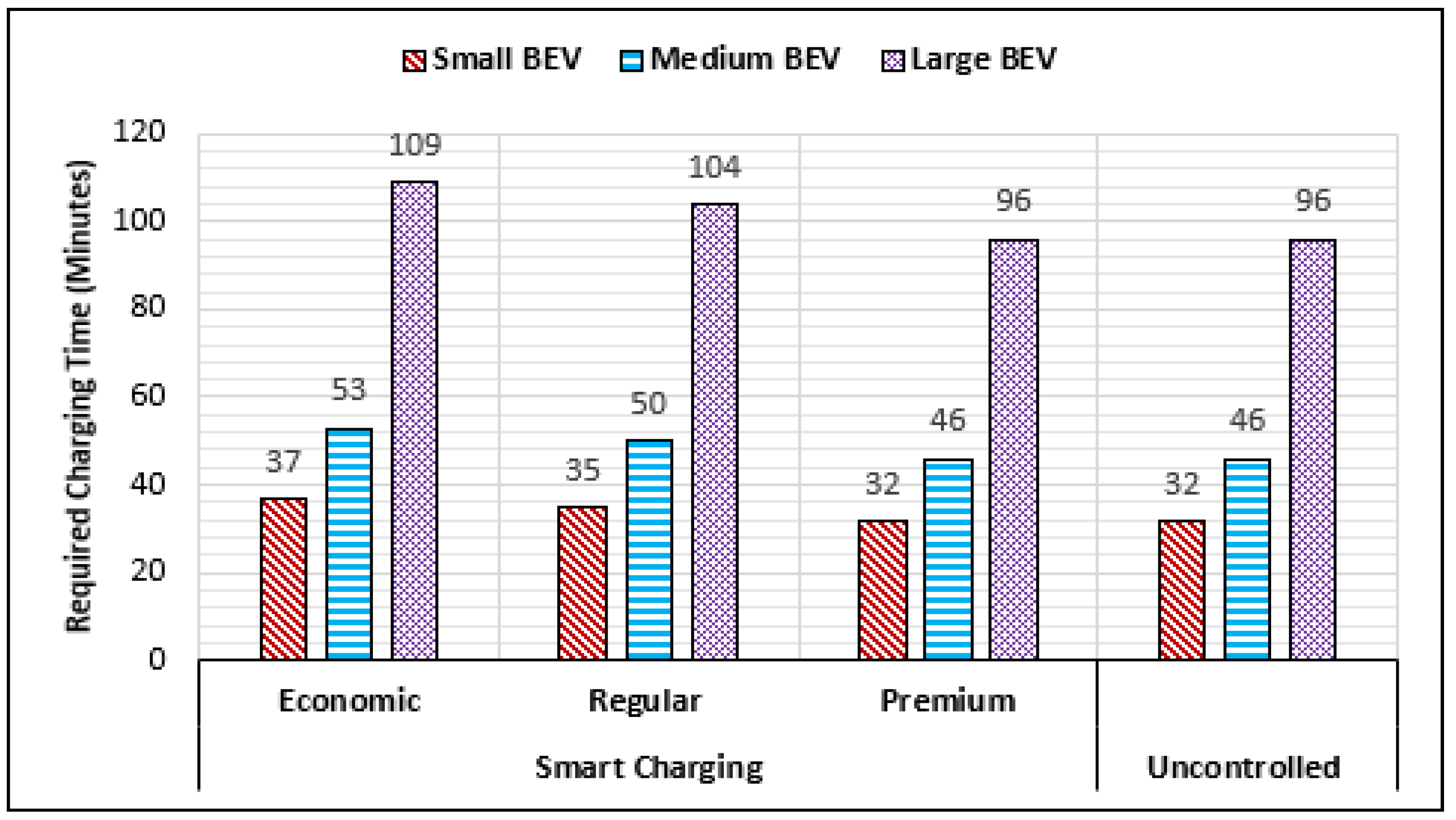
4.6. Effect of Smart Charging on Charging Duration
4.7. Comparative Study
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Number of BPEV in the system | |
| Share of electric vehicles with respect to the total number of vehicles in the system | |
| Number of residential houses in the system, 750 houses | |
| Number of vehicles per house, 2 vehicles/house | |
| Battery capacity of vehicle , kwh | |
| Capacity of small battery, | |
| Capacity of medium battery, | |
| Capacity of large battery, | |
| A set of PEVS with small battery capacity | |
| A set of PEVS with medium battery capacity | |
| A set of PEVS with large battery capacity | |
| A set of PEVS uses the home chargers | |
| A set of PEVS uses the FCS | |
| Energy required for vehicle from FCS, kwh | |
| Length of time with vehicle connected to the fast charger, minute | |
| Efficiency of the fast charger, % | |
| Factor to convert hour into minutes | |
| Maximum charging power per port, kw | |
| Output power of fast-charging station, kw | |
| Uncontrolled charging power, kw | |
| Premium charging power, kw | |
| Regular charging power, kw | |
| Economic charging power, kw | |
| Number of PEV that charging using premium charging power at time | |
| Number of PEV that charging using regular charging power at time | |
| Number of PEV that charging using economic charging power at time | |
| Factor to set the upper limits of premium charging power, % | |
| Factor to set the lower limits of premium charging power, % | |
| Factor to set the upper limits of regular charging power, % | |
| Factor to set the lower limits of regular charging power, % | |
| Factor to set the upper limits of economic charging power, % | |
| Factor to set the lower limits of economic charging power, % | |
| Capacity of the FCS, kw | |
| Number of ports in the fast-charging station | |
| Share of BPEV with small battery capacity charges at the FCS at time , | |
| Share of BPEV with medium battery capacity charges at the FCS at time , | |
| Share of BPEV with large battery capacity charges at the FCS at time , | |
| Share of BPEV that have battery capacity charged using premium charging power at FCS at time and required departure time of , | |
| Share of BPEV that have battery capacity charged using regular charging power at time and required departure time of , | |
| Share of BPEV that have battery capacity charged using economic charging power at time and required departure time of , | |
| Share of BPEV that uses FCS, | |
| A set of BPEV uses the premium charging power | |
| A set of BPEV uses the regular charging power | |
| A set of BPEV uses the economic charging power | |
| Number of PEV with small battery capacity uses the FCS | |
| Number of PEV with medium battery capacity uses the FCS | |
| Number of PEV with large battery capacity uses the FCS | |
| State-of-charge of vehicle at time | |
| State-of-charge of vehicle at time | |
| Minimum energy required from the FCS | |
| Desired state-of-charge at the departure time of vehicle , % | |
| Maximum state-of-charge at the departure time of vehicle , % | |
| Departure time of vehicle | |
| Relative voltage changes caused by FCS at point-of-common, % | |
| Voltage variation at point-of-common coupling, | |
| Nominal voltage at point-of-common, | |
| Apparent power variation at point-of-common coupling, | |
| Active power load change at point-of-common coupling, | |
| Reactive power load change at point-of-common coupling, | |
| Current load change at point-of-common coupling, | |
| Network impedance angle, degree | |
| Network reactance at point-of-common, | |
| Network resistance at point-of-common, | |
| Flicker time represents the flicker impression of a single voltage change, second | |
| Factor represents the waveform shape of voltage fluctuation, rectangular change | |
| Factor to comply with the flicker curve | |
| Coefficient to describe the source of disturbance | |
| Summation of all flicker times, minute | |
| Number of voltage dips per minute | |
| Limit of short-term flicker severity |
References
- Gao, Z.; Mao, D.; Wang, J. Distribution grid response monitor. IET Gener. Transm. Distrib. 2019, 13, 4374–4381. [Google Scholar] [CrossRef]
- Dominion Energy. 2017 Blue Book; Dominion Energy Virginia and Dominion Energy: Elizabeth City, NC, USA, 2019. [Google Scholar]
- Duke Energy Midwest Duke Energy Midwest Transmission Systems Facility Connection Requirements. Available online: https://dms.psc.sc.gov/Attachments/Matter/af2591fc-cdb8-af85-a7faf2d347bd0c65 (accessed on 11 December 2019).
- Engineering Recommendation P28 Voltage Fluctuations and the Connection of Disturbing Equipment to Transmission Systems and Distribution Networks in the United Kingdom. Available online: https://www.nienetworks.co.uk/documents/d-code/ena_erec_p28_issue-2_(2019).aspx/ (accessed on 4 May 2019).
- Alhazmi, Y.A.; Salama, M.M.A. Economical staging plan for implementing electric vehicle charging stations. Sustain. Energy Grids Netw. 2017, 10, 12–25. [Google Scholar] [CrossRef]
- Sharma, I.; Canizares, C.; Bhattacharya, K. Smart charging of PEVs penetrating into residential distribution systems. IEEE Trans. Smart Grid 2014, 5, 1196–1209. [Google Scholar] [CrossRef]
- Humayd, A.S.B.; Bhattacharya, K. Design of optimal incentives for smart charging considering utility-customer interactions and distribution systems impact. IEEE Trans. Smart Grid 2017, 10, 1521–1531. [Google Scholar] [CrossRef]
- Hafez, O.; Bhattacharya, K. Integrating EV charging stations as smart loads for demand response provisions in distribution systems. IEEE Trans. Smart Grid 2016, 9, 1096–1106. [Google Scholar] [CrossRef]
- Moghaddam, Z.; Habibi, D. Smart Charging Strategy for Electric Vehicle Charging Stations. IEEE Trans. Transp. Electrif. 2018, 4, 13. [Google Scholar] [CrossRef]
- van der Kam, M.; van Sark, W. Smart charging of electric vehicles with photovoltaic power and vehicle-to-grid technology in a microgrid; a case study. Appl. Energy 2015, 152, 20–30. [Google Scholar] [CrossRef] [Green Version]
- Humayd, A.S.B.; Lami, B.; Bhattacharya, K. The effect of PEV uncontrolled and smart charging on distribution system planning. In Proceedings of the 2016 IEEE Electrical Power and Energy Conference (EPEC), Ottawa, ON, Canada, 12–14 October 2016; IEEE: New York City, NY, USA, 2016; pp. 1–6. [Google Scholar]
- Almutairi, A.; Salama, M.M.A. Assessment and Enhancement Frameworks for System Reliability Performance Using Different PEV Charging Models. IEEE Trans. Sustain. Energy 2018, 9, 1969–1984. [Google Scholar] [CrossRef]
- Alshareef, S.M.; Morsi, W.G. Impact of fast charging stations on the voltage flicker in the electric power distribution systems. In Proceedings of the 2017 IEEE Electrical Power and Energy Conference (EPEC), Saskatoon, SK, Canada, 22–25 October 2017; pp. 1–6. [Google Scholar]
- Diaz, C.; Ruiz, F.; Patino, D. Smart charge of an electric vehicles station: A model predictive control approach. In Proceedings of the 2018 IEEE Conference on Control Technology and Applications (CCTA), Copenhagen, Denmark, 21–24 August 2018; IEEE: New York City, NY, USA, 2018; pp. 54–59. [Google Scholar]
- Marei, M.I.; El-Saadany, E.F.; Salama, M.M. Envelope tracking techniques for FlickerMitigation and Voltage regulation. IEEE Trans. Power Deliv. 2004, 19, 1854–1861. [Google Scholar] [CrossRef]
- Marei, M.I.; Salama, M.M.A. Advanced Techniques for Voltage Flicker Mitigation. In Proceedings of the 2006 IEEE International Power Electronics Congress, Puebla, Mexico, 16–18 October 2006; IEEE: New York City, NY, USA, 2006; pp. 1–5. [Google Scholar]
- Elnady, A.; Salama, M.M. Unified approach for mitigating voltage sag and voltage flicker using the DSTATCOM. IEEE Trans. Power Deliv. 2005, 20, 992–1000. [Google Scholar] [CrossRef]
- Perera, D.; Meegahapola, L.; Perera, S.; Ciufo, P. Characterisation of flicker emission and propagation in distribution networks with bi-directional power flows. Renew. Energy 2014, 63, 172–180. [Google Scholar] [CrossRef] [Green Version]
- Eghtedarpour, N.; Farjah, E.; Khayatian, A. Effective Voltage Flicker Calculation Based on Multiresolution S-Transform. IEEE Trans. Power Deliv. 2012, 27, 521–530. [Google Scholar] [CrossRef]
- Robert, A.; Couvreur, M. Recent experience of connection of big arc furnaces with reference to flicker level. In Proceedings of the International Conference on Large High Voltage Electric Systems, Interlaken, Switzerland, 24–29 July 1994; pp. 36–305. [Google Scholar]
- Arlt, D.; Stark, M.; Eberlein, C. Examples of international flicker requirements in high voltage networks and real world measurements. In Proceedings of the 2007 9th International Conference on Electrical Power Quality and Utilisation, Barcelona, Spain, 9–10 October 2007; IEEE: Barcelona, Spain, 2007; pp. 1–4. [Google Scholar]
- Perera, S.; Robinson, D.; Elphick, S.; Geddey, D.; Browne, N.; Smith, V.; Gosbell, V. Synchronized flicker measurement for flicker transfer evaluation in power systems. IEEE Trans. Power Deliv. 2006, 21, 1477–1482. [Google Scholar] [CrossRef]
- Irena Innovation Outlook Smart Charging for Electric Vehicles. Available online: https://www.irena.org/publications/2019/May/Innovation-Outlook-Smart-Charging/ (accessed on 5 January 2019).
- IRENA Innovation Landscape Brief: Electric-Vehicle Smart Charging. Available online: https://irena.org/-/media/Files/IRENA/Agency/Publication/2019/Sep/IRENA_EV_smart_charging_2019.pdf?la=en&hash=E77FAB7422226D29931E8469698C709EFC13EDB2 (accessed on 5 January 2019).
- Alshareef, S.M.; Morsi, W.G. Probabilistic Modeling of Plug-in Electric Vehicles Charging from Fast Charging Stations. In Proceedings of the 2019 IEEE Electrical Power and Energy Conference (EPEC), Montreal, QC, Canada, 16–18 October 2019; IEEE: Montréal, QU, Canada, 2019; pp. 1–6. [Google Scholar]
- Axsen, J.; Goldberg, S.; Bailey, J.; Kamiya, G.; Langman, B.; Cairns, J.; Wolinetz, M.; Miele, A. Electrifying Vehicles: Insights from the Canadian Plug-in Electric Vehicle Study. Simon Fraser University, Vancouver, Canada. Available online: https://sustainabletransport.ca/the-canadian-plug-in-electric-vehicle-study-cpevs/ (accessed on 12 March 2019).
- Duvall, M.; Knipping, E.; Alexander, M.; Tonachel, L.; Clark, C. Environmental assessment of plug-in hybrid electric vehicles, Volume 1: Nationwide greenhouse gas emissions. Electr. Power Res. Inst. Palo Alto CA 2007, 1015325. Available online: https://www.energy.gov/sites/prod/files/oeprod/DocumentsandMedia/EPRI-NRDC_PHEV_GHG_report.pdf (accessed on 4 May 2020).
- evCloud | FleetCarma. Available online: https://www.fleetcarma.com/evCloud (accessed on 19 December 2018).
- Gjelaj, M.; Træholt, C.; Hashemi, S.; Andersen, P.B. DC Fast-charging stations for EVs controlled by a local battery storage in low voltage grids. In Proceedings of the PowerTech, 2017 IEEE Manchester, Manchester, UK, 18–22 June 2017; IEEE: Manchester, UK, 2017; pp. 1–6. [Google Scholar]
- Francfort, J.; Brion Bennett, R. Plug-in Electric Vehicle and Infrastructure Analysis. Available online: https://inldigitallibrary.inl.gov/sites/sti/sti/6799570.pdf (accessed on 13 October 2019).
- Vehicle Technologies Office Avta: Arra ev Project Chevrolet Volt Data Summary Reports. Available online: https://www.energy.gov/eere/vehicles/downloads/avta-arra-ev-project-chevrolet-volt-data-summary-reports (accessed on 7 July 2020).
- Petro-Canada Reveals $0.33 per Minute EV Charging Cost in Ontario. Available online: https://mobilesyrup.com/2020/01/22/petro-canada-33-cents-per-minute-ev-cost/ (accessed on 11 September 2020).
- IEC/TR 61000-3-7; Electromagnetic Compatibility (EMC). Part 3: Limits—Section 7: Assessment of Emission Limits for Fluctuating Loads in MV and HV Power Systems. International Electrotechnical Commission: Geneva, Switzerland, 1996; Volume 61, pp. 1–10.
- IEC 61000-3-3:2013; Electromagnetic Compatibility (EMC). Part 3-3, Limits–Limitation of Voltage Changes, Voltage Fluctuations and Flicker in Public Low-Voltage Supply Systems, for Equipment with Rated Current≤ 16 A per Phase and Not Subject to Conditional Connection. International Electrotechnical Commission: Geneva, Switzerland, 2013; pp. 61000–61003.
- Baggini, A. Handbook of Power Quality; John Wiley & Sons: Hoboken, NJ, USA, 2008; ISBN 0-470-75423-0. [Google Scholar]
- IEEE Std 1453-2015; IEEE Recommended Practice–Adoption of IEC 61000-4-15:2010, Electromagnetic Compatibility (EMC)–Testing and Measurement Techniques–Flickermeter–Functional and Design Specifications. IEEE: New York City, NY, USA, 2019. Available online: https://ieeexplore.ieee.org/document/6053977 (accessed on 17 February 2022).
- IEEE PES AMPS DSAS Test Feeder Working Group Resources. PES Test Feeder. Available online: http://sites.ieee.org/pes-testfeeders/resources/ (accessed on 11 March 2019).
- MATLAB Release 2020b; The MathWorks, Inc.: Natick, MA, USA, 1992.
- EPRI, Smart Grid Resource Center. Simulation Tool—OpenDSS. Available online: https://smartgrid.epri.com/SimulationTool.aspx (accessed on 17 February 2022).
- Smart, J. Charging Infrastructure for Shared and Autonomous EVs. In Proceedings of the Future of Electric Vehicle Infrastructure in the U.S. Webinar, Online, 2 May 2019. [Google Scholar]
- Wood, E.; Rames, C.; Kontou, E.; Motoaki, Y.; Smart, J.; Zhou, Z. Analysis of Fast Charging Station Network for Electrified Ride-Hailing Services. In Proceedings of the SAE World Congress Experience, Detroit, MI, USA, 10–12 April 2018. SAE Technical Paper 2018-01-0667. [Google Scholar]
- Rosnan, T.D.; Murray, M.A. Voltage fluctuation monitoring in an ESB Transmission station. In Irish Colloquium on DSP & Control; University of Limerick: Dublin, Ireland, 1993. [Google Scholar]
- Brosnan, T.D.; Murray, M.A. Flicker Severity Measurements on an Electric Arc Furnace during Switched Changes in a Transmission Network. In Proceedings of the First International Conference on Power Quality, Paris, France, 15–18 October 1991. [Google Scholar]
- Arlt, D.; Eberlein, C. Network disturbances caused by Ultra High Power electric arc furnaces and possible reduction methods. In Proceedings of the Conference Electrical Power Quality and Utilisation, Cracow, Poland, 23–25 September 1997; pp. 23–25. [Google Scholar]
- d’études, C.; Arlt, D.; Papič, I. C4, C. international des grands réseaux électriques. In Review of Flicker Objectives for LV, MV, and HV Systems: Cigre/Cired; CIGRÉ: Paris, France, 2011; ISBN 978-2-85873-138-1. [Google Scholar]




| Reference | Charging Power | Charging Type | Flicker | |||
|---|---|---|---|---|---|---|
| Slow | Fast | Uncontrolled | Smart | Analysis | Mitigation | |
| [7,9,10,11] | ✓ | × | × | ✓ | × | × |
| [12] | ✓ | × | ✓ | ✓ | × | × |
| [13] | × | ✓ | ✓ | × | × | × |
| [14] | ✓ | ✓ | × | ✓ | × | × |
| [8] | × | ✓ | ✓ | ✓ | × | × |
| [15,16,17] | × | × | × | × | ✓ | ✓ |
| [18,19,20,21,22] | × | × | × | × | ✓ | × |
| [6] | ✓ | × | × | ✓ | × | × |
| Proposed Smart Charging | × | ✓ | ✓ | ✓ | ✓ | ✓ |
| Slow Charging | Fast Charging | ||||
|---|---|---|---|---|---|
| Uncontrolled | Smart | Uncontrolled | with Battery | Smart | |
| Electricity demand | + | + | + | + | + |
| Peak demand | ++ | + | ++ | + | + |
| Distribution grids | ++ | + | ++ | + | + |
| Light flicker | × | × | ✓ | ✓ | × |
| Penetration Level (Increase of ) | Number of PBEVs in the System () | Number of PBEVs Using the FCS () | Dips per Minute () | |
|---|---|---|---|---|
| in 24 Hours | at 4:00 p.m. | |||
| 10% | 150 | 51 | 4 | 0.14 |
| 20% | 300 | 102 | 8 | 0.28 |
| 30% | 450 | 153 | 12 | 0.42 |
| 40% | 600 | 204 | 16 | 0.56 |
| 50% | 750 | 255 | 20 | 0.7 |
| Reference | Reported Flicker Value | |
|---|---|---|
| Previous work | [21] | 2 |
| [20] | 1.7 | |
| [42] | 1.05 | |
| [43] | 1.4 | |
| [44] | 1.26 | |
| [22] | 2.78 | |
| [45] | 2.6 | |
| Current study | Uncontrolled FCS, at 10% up to 40% of FCS utilization rate | 0.9–1.3 |
| FCS with smart charging, at 10% up to 40% of FCS utilization rate | 0.6–0.9 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alshareef, S.M. A Novel Smart Charging Method to Mitigate Voltage Fluctuation at Fast Charging Stations. Energies 2022, 15, 1746. https://doi.org/10.3390/en15051746
Alshareef SM. A Novel Smart Charging Method to Mitigate Voltage Fluctuation at Fast Charging Stations. Energies. 2022; 15(5):1746. https://doi.org/10.3390/en15051746
Chicago/Turabian StyleAlshareef, Sami M. 2022. "A Novel Smart Charging Method to Mitigate Voltage Fluctuation at Fast Charging Stations" Energies 15, no. 5: 1746. https://doi.org/10.3390/en15051746
APA StyleAlshareef, S. M. (2022). A Novel Smart Charging Method to Mitigate Voltage Fluctuation at Fast Charging Stations. Energies, 15(5), 1746. https://doi.org/10.3390/en15051746






