Energy Harvesting and Water Saving in Arid Regions via Solar PV Accommodation in Irrigation Canals
Abstract
1. Introduction
2. Materials and Methods
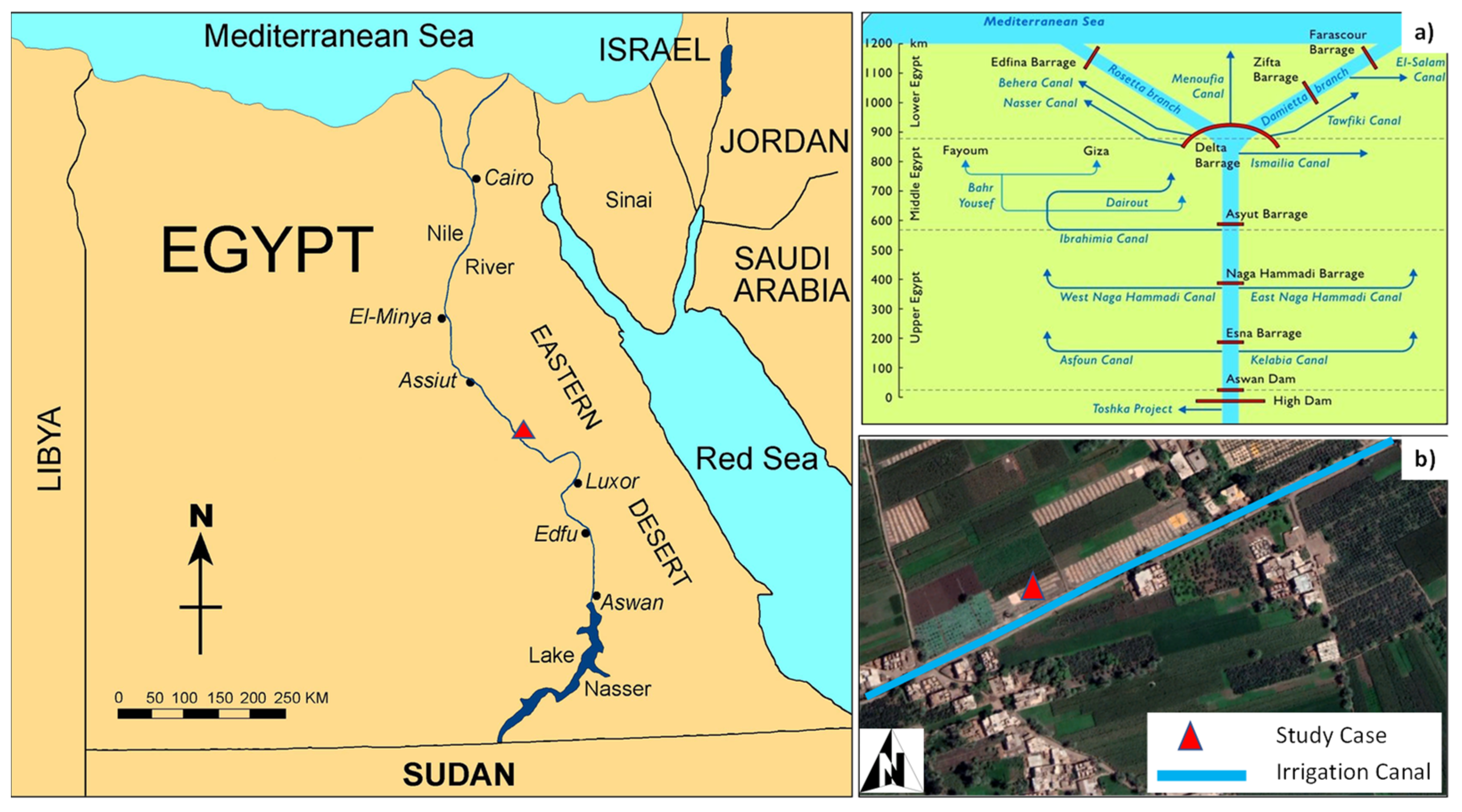
2.1. Case Study
2.2. Problem Statement
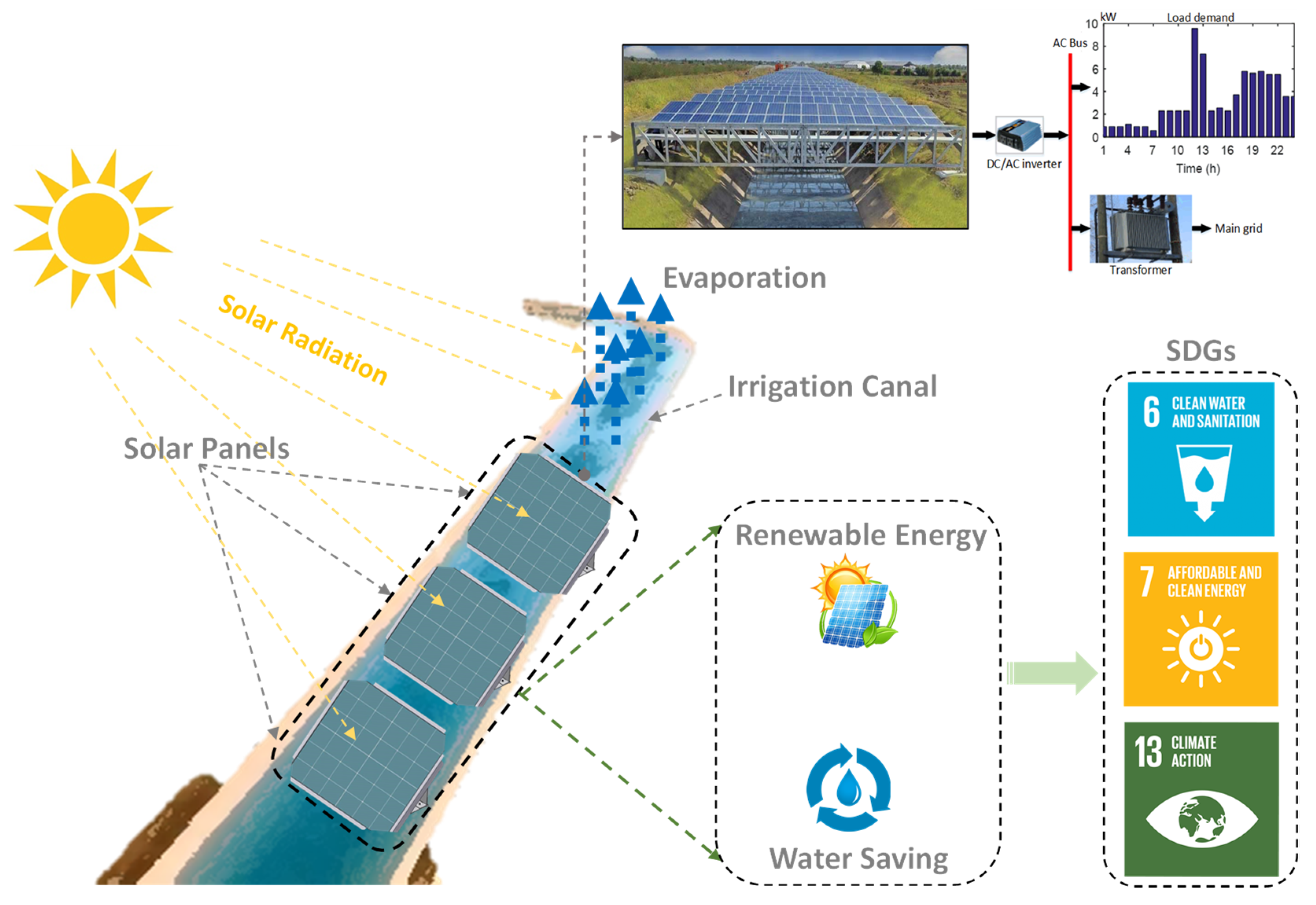
2.3. Canal-Top PV Accommodation
2.4. System Modeling
2.4.1. Canal-Top PV
2.4.2. DC/AC Inverter
2.4.3. Load Demand Profile
2.5. Metaheuristic Optimizers
2.5.1. Particle Swarm Optimization (PSO)
2.5.2. Genetic Algorithm (GA)
- The algorithm randomly starts reading the LCOE, and then an initial population (POPo) is assumed.
- The constraints in (POPo) are checked. The solutions that are outside the constraints are eliminated with a large penalty.
- The objective function (i.e., LCOE) is checked and evaluated at (POPo), and a new solution is generated (POPk).
- Another population is generated (POPk+1) via GA elitism, selection, crossover, and mutation.
- The constraints are checked at a solution (POPk+1). The simulation is stopped for a previously determined number of iterations. Ultimately, the best solution is printed.
2.5.3. Cuckoo Search (CS)
- The number of host nests is decided. Adult cuckoo birds choose random nests to lay the eggs. An adult cuckoo bird can lay an egg one at a time.
- Only eggs with better quality that the host parents cannot discover are moved to the upcoming generation.
- Provided that the probability (Pa) lies between 0 and 1, the host nest can delineate a cuckoo egg according to the Pa. Cuckoos’ eggs discovered by the host birds are thrown away. Sometimes, the host bird might abandon the nest.
3. Problem Formulation
3.1. GHG Emissions
3.2. Loss of Power Supply Probability (LPSP)
3.3. Constraints
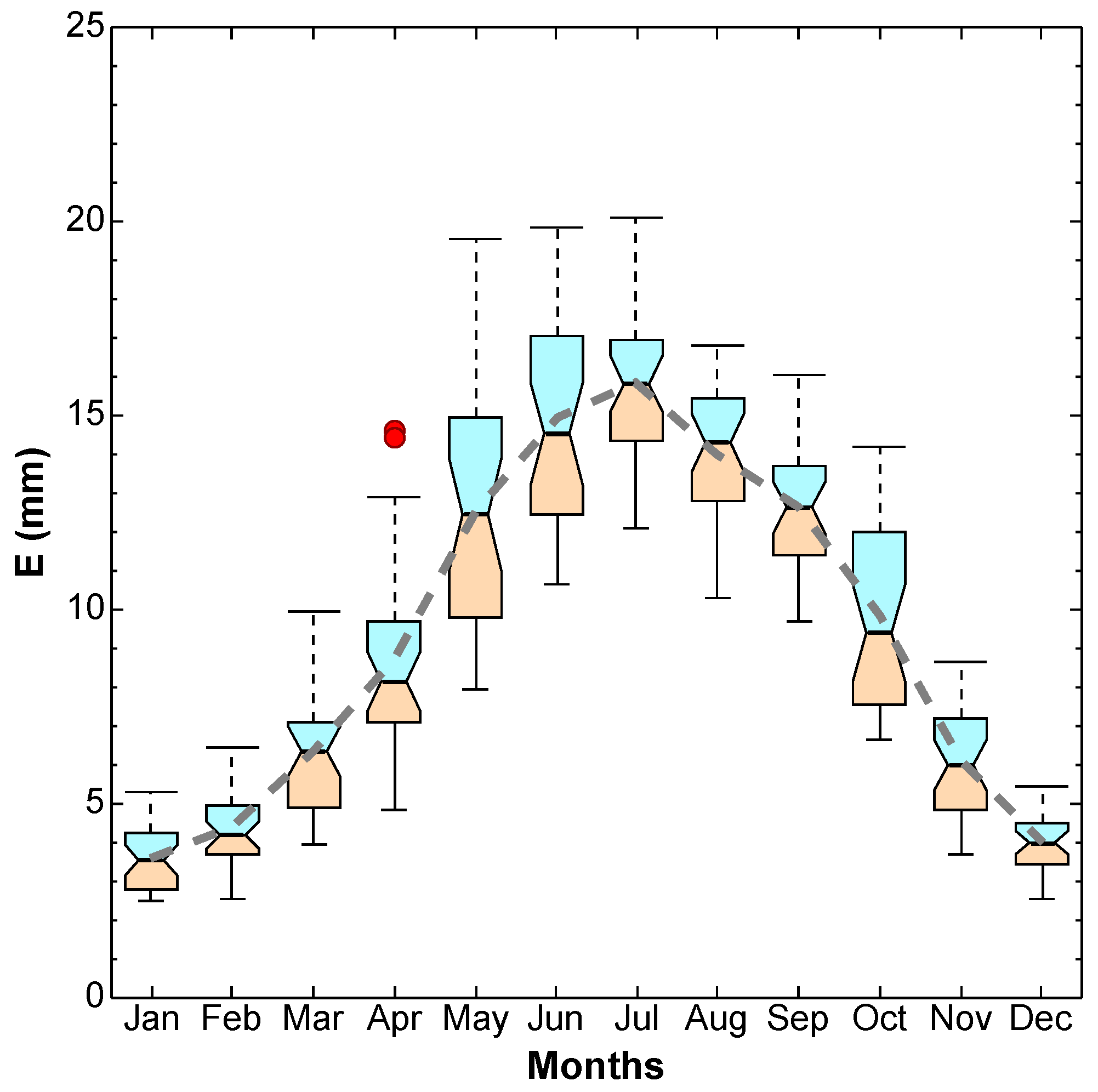
3.4. Evaporation Estimation
3.5. Optimizers Implementation
- For satisfactory evaporation reduction and environmental improvement, the PVs have a higher priority than the primary grid to meet the load demands,
- Canal-top PVs can provide the load demands; consequently, the excess energy is sold to the primary grid, as demonstrated in Figure 4, and
- Canal-top PVs cannot meet load demands. The deficit load energy is purchased from the primary grid.
4. Results and Discussion
4.1. Water Loss Due to Evaporation
4.2. Energy Production and Profitability
4.2.1. Scenario 1: Impact of Tilt Angle
4.2.2. Scenario 2: Economical Solution
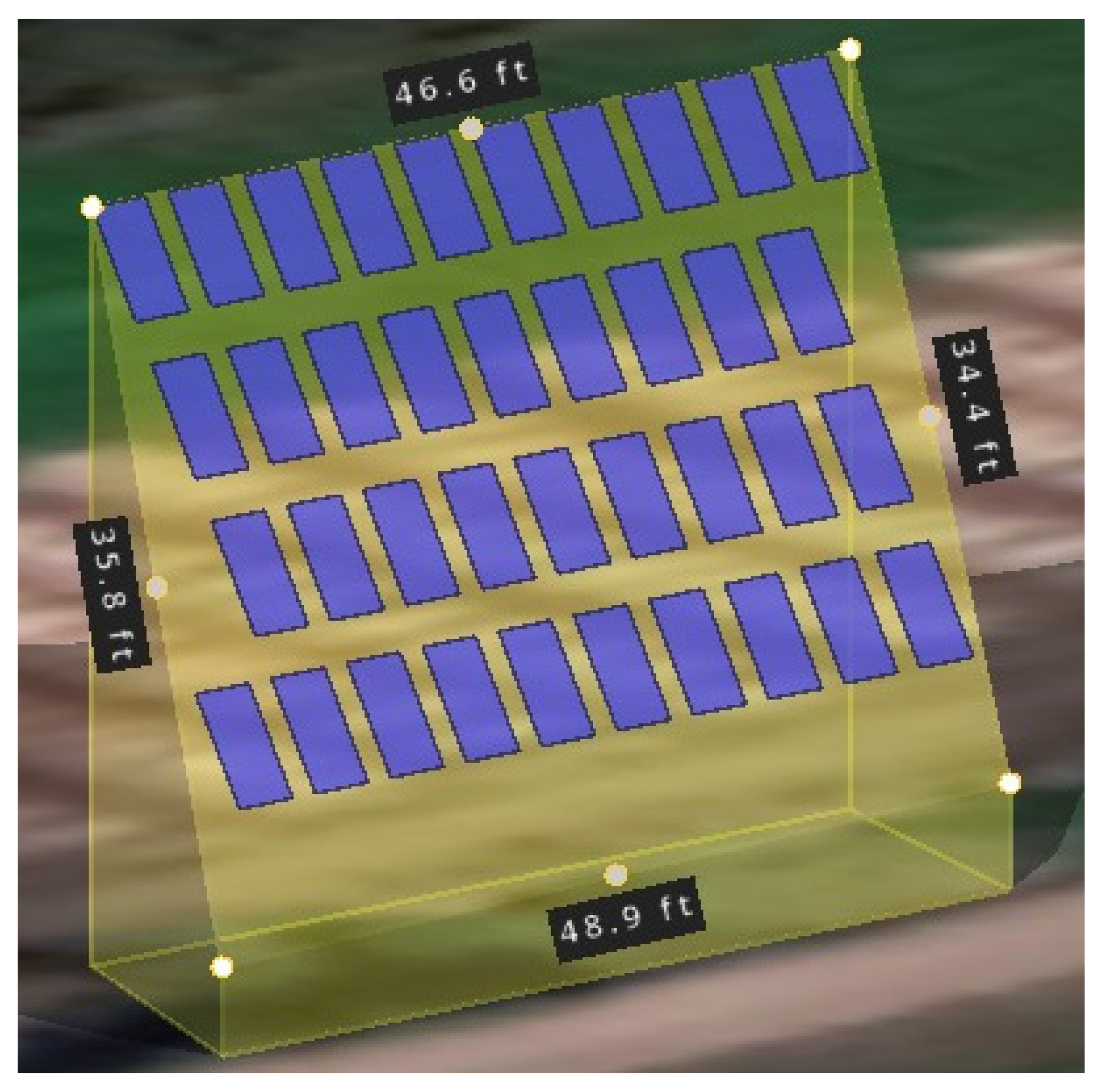
4.2.3. Scenario 3: GIS Investigations
4.2.4. Scenario 4: Sensitivity of the Top Canal PV Accommodation
5. Discussions
- -
- The developed CS optimizer is validated through an impartial comparison with prior research, as demonstrated in Table 3. In [56], several battery-mix technologies were employed via a hybrid PSO-GOA algorithm. In [34], a bi-objective ant colony was conducted. Despite the figures in Table 3 depending on location, meteorological data, initial costs, microgrid configuration, salvage market, and the optimizer’s ability to find a near-optimal solution, the developed CS seems to be competitive the other algorithms in the literature.
| Algorithm | LCOE ($/kWh) | GHG (ton) | TNPC (k$) |
| PSOGOA [56] | 0.658 | 141.8 | 118.8 |
| BOACA [34] | 1.082 | 118.3 | 121.6 |
| HOMER [34] | 0.809 | - | 30,033 |
| NGSA [37] | 0.19–0.25 | 8.87 | - |
| GWO [57] | 0.78–1.59 | - | - |
| Decision making | 0.12–0.17 | 100.3–130 | - |
| CS | 0.707 | 67.3 | 140.6 |
- -
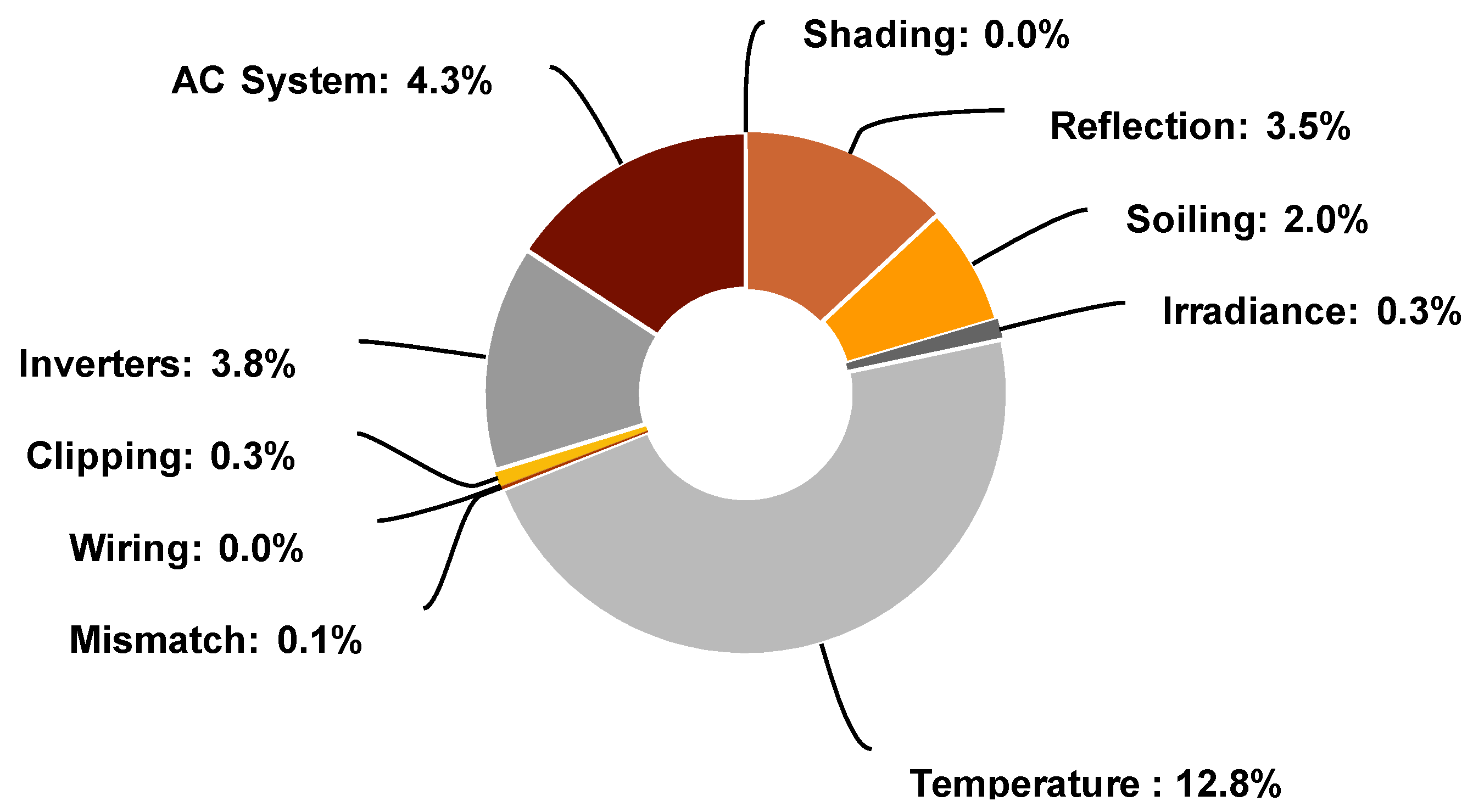
- According to the Helioscope investigation, the ambient temperature might significantly affect the canal-top PV accommodation performance, as in Figure 13. Contrarily, the soil in such agricultural areas has a negligible influence.
- -
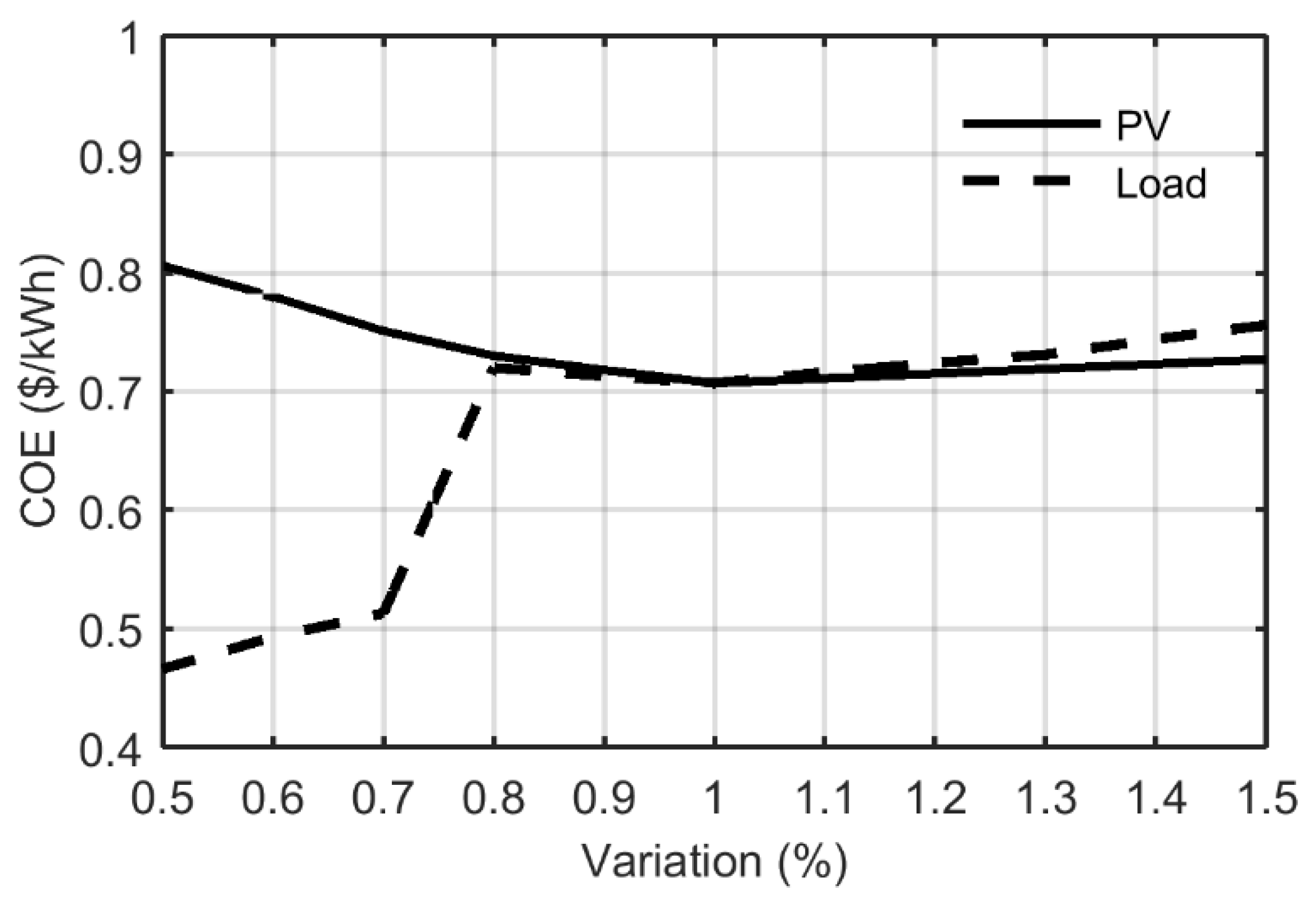
- The canal-top PV rating has a negligible influence compared to the load impact on the LCOE, as in Figure 14.
- -
- The simulated results show that the PVs are superior to the main grid to meet the load demand, as illustrated in the annual energy share in Figure 11.
- -
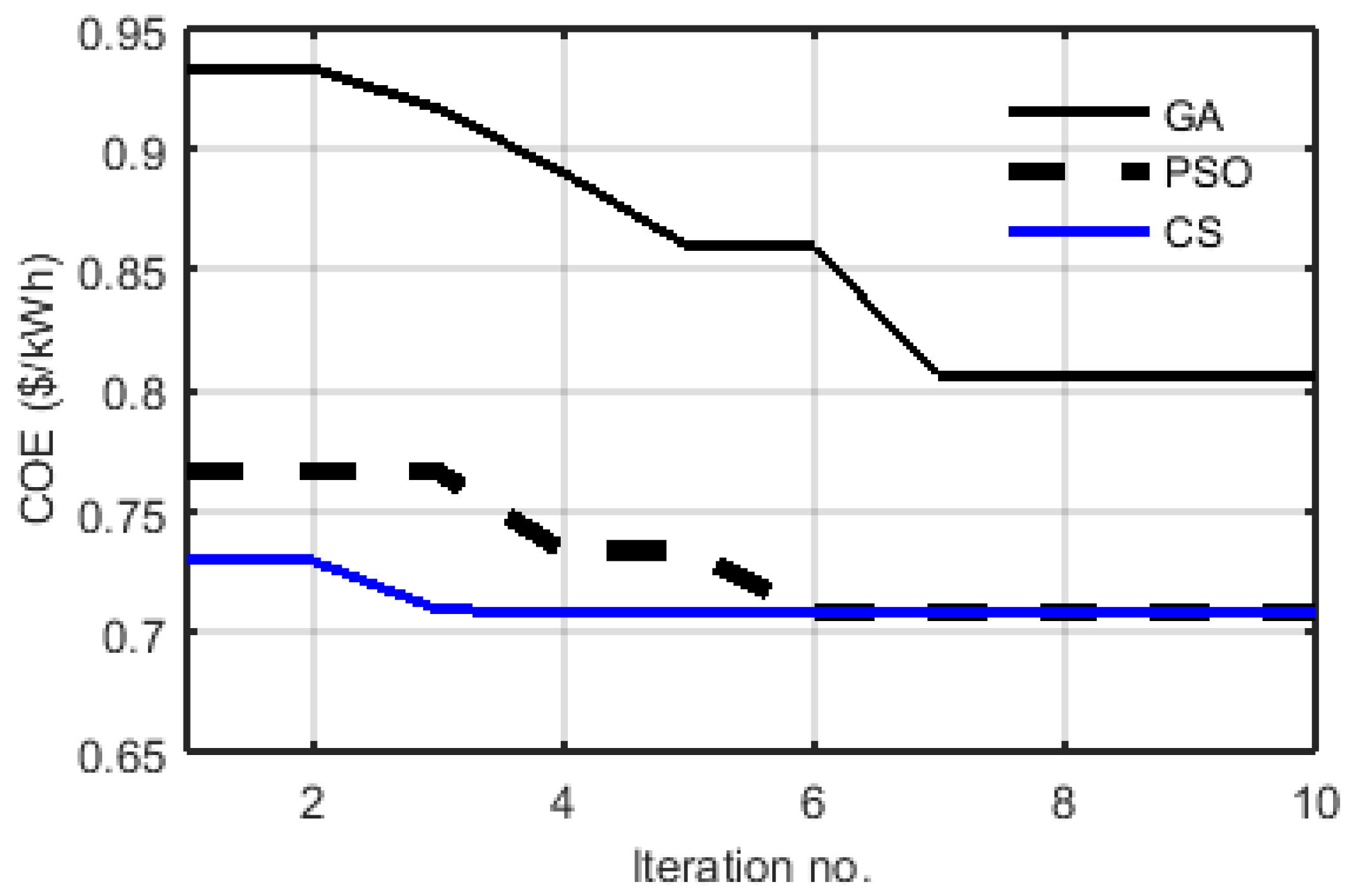
- The developed CS outperforms the other algorithms (Figure 10) as it relaxed fast towards the optimal solution.
- -
- Water-saving through canal-top PV is a significant benefit; however, a quantitative analysis of the actual evaporation reduction and the quantity of the water saved will be part of future research.
- -
- Another issue of the current work is related to the nature of the renewables, which could influence the utility grid frequency [58]. The modern controllers are to be designed to handle the canal-top PV injected energy and the main grid frequency oscillations.
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| CS | Cuckoo Search |
| D | Saturation Deficit |
| DHI | Diffuse Horizontal Irradiation |
| E | Evaporation |
| GA | Genetic Algorithm |
| GHG | Greenhouse Gas Emissions |
| GHI | Global Horizontal Irradiation |
| GIS | Graphical Information System |
| HMG | Hybrid Microgrids |
| LCOE | Levelized Cost of Energy |
| LPSP | Loss of Power Supply Probability |
| NPC | Net Present Costs |
| PSO | Particle Swarm Optimization |
| PV | Photovoltaic |
| Rh | Relative humidity |
| SDGs | Sustainable Development Goals |
| Ta | Air temperature |
| V | Wind speed |
Appendix A
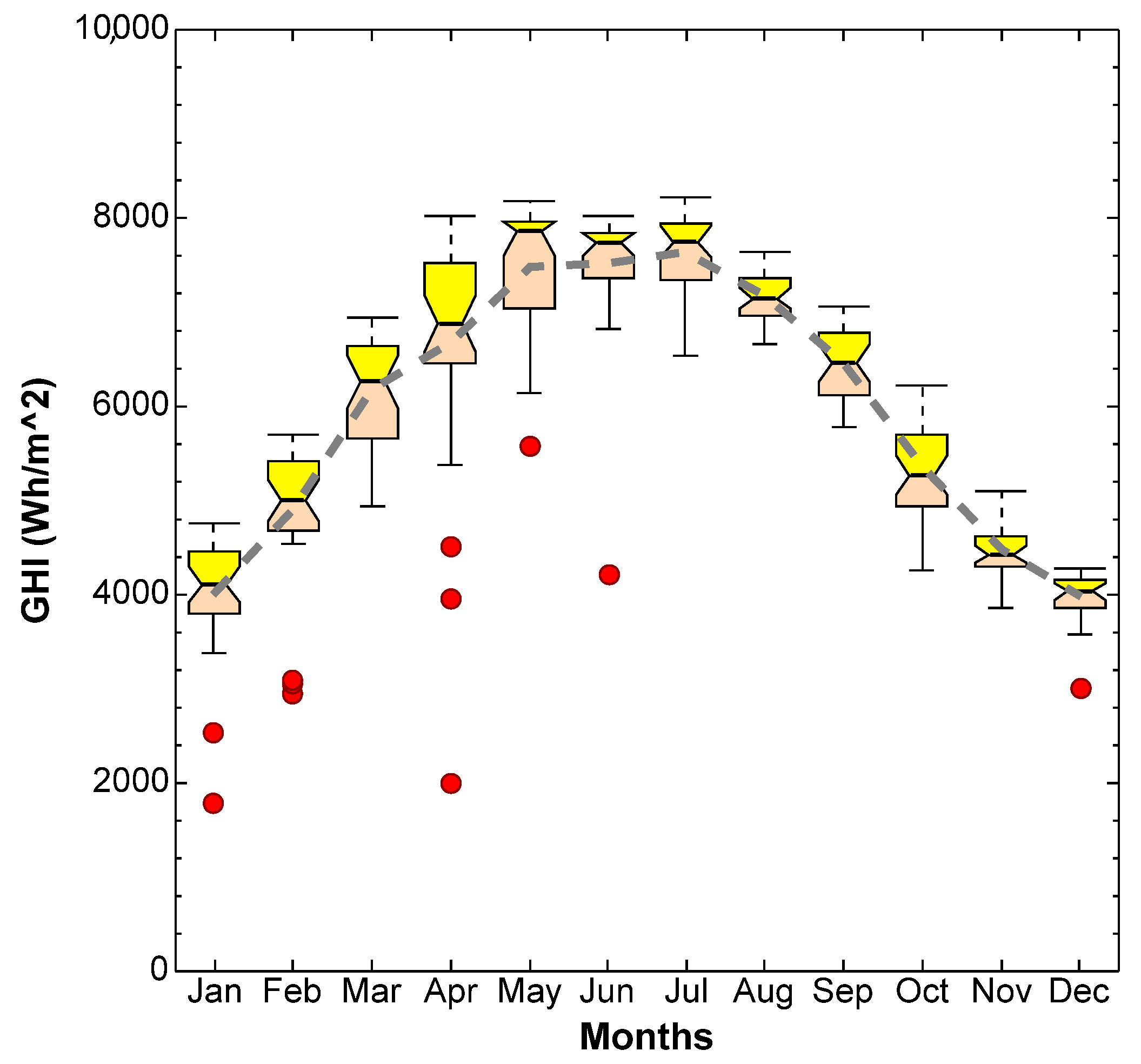
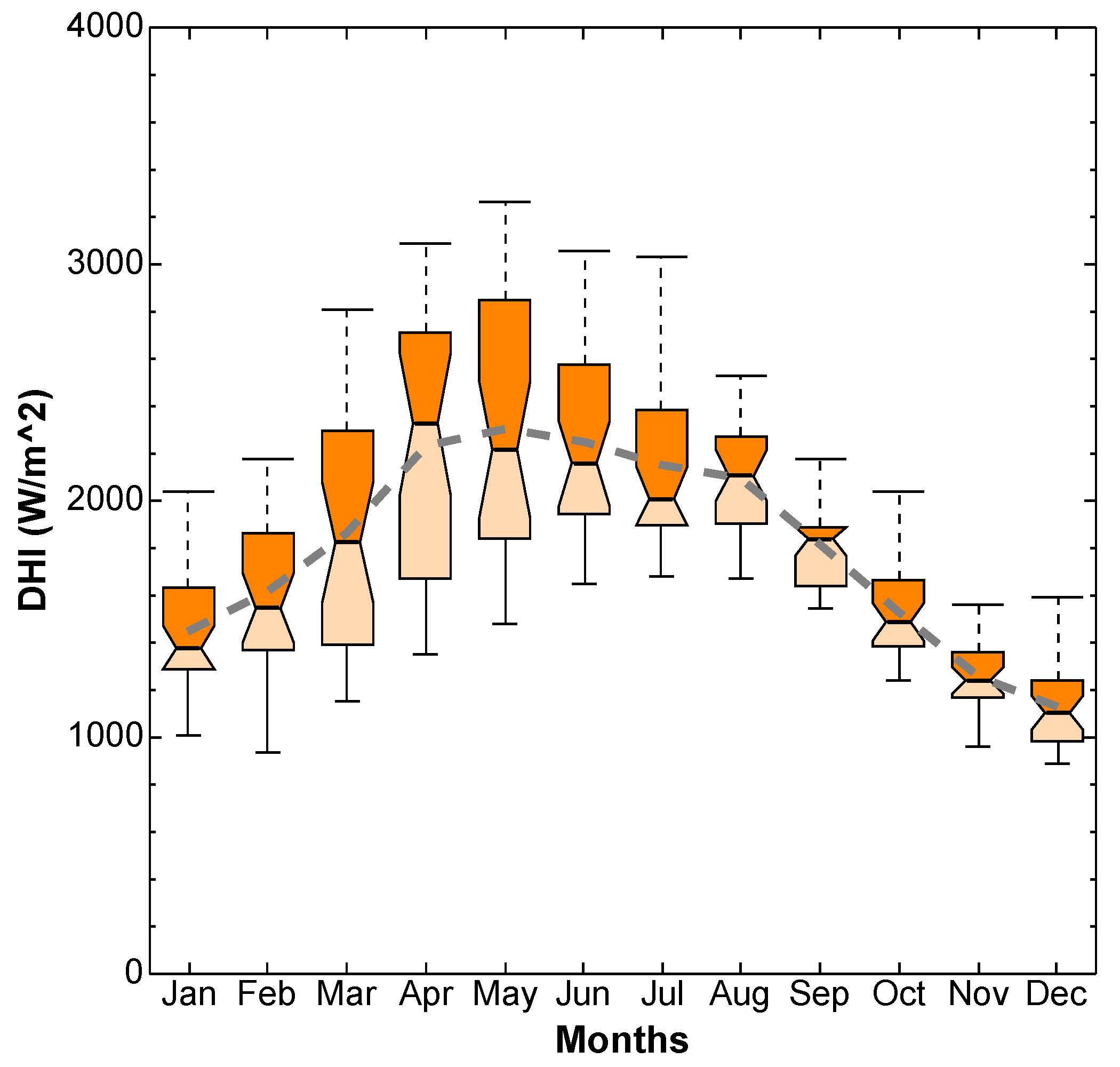
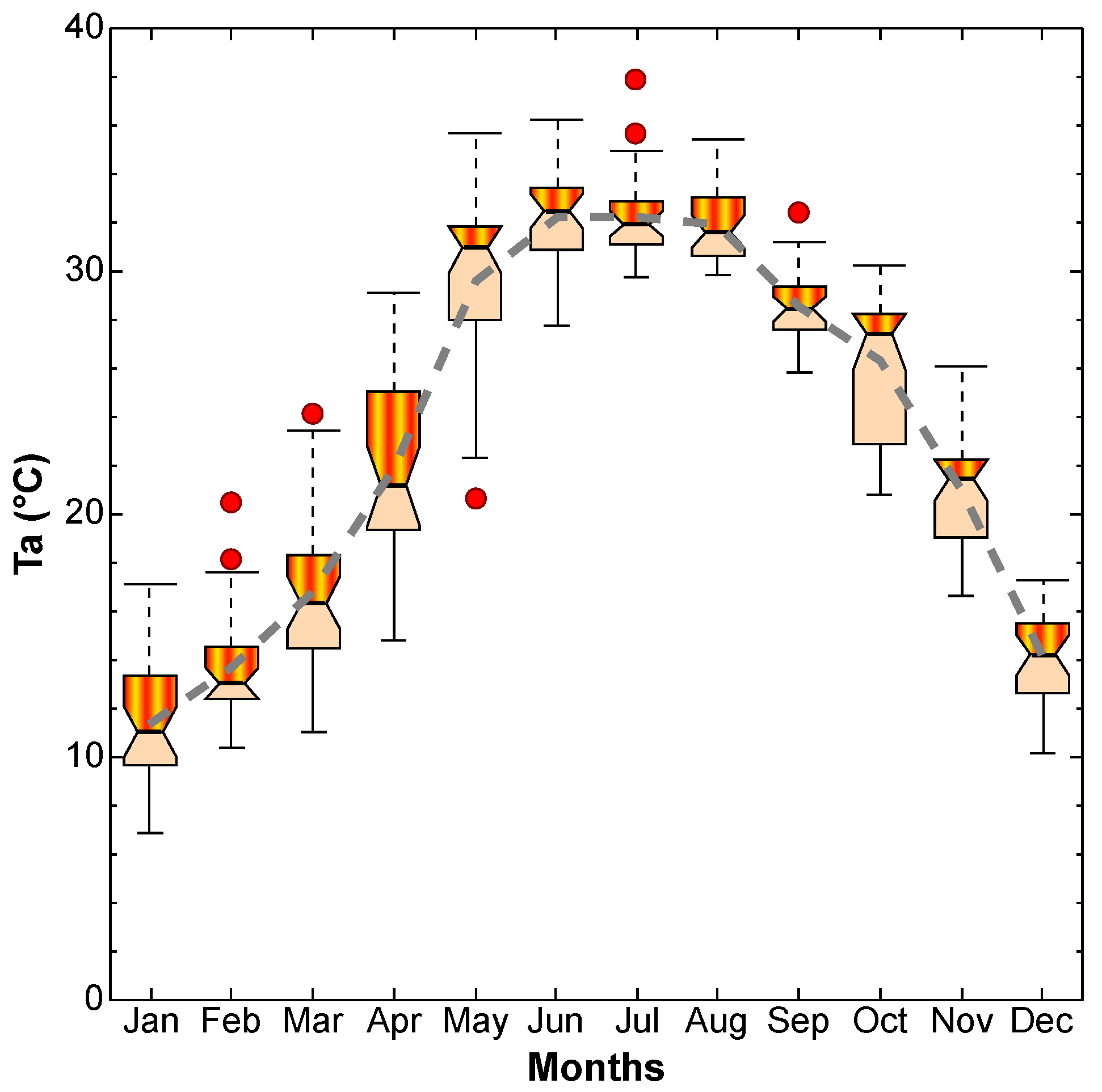

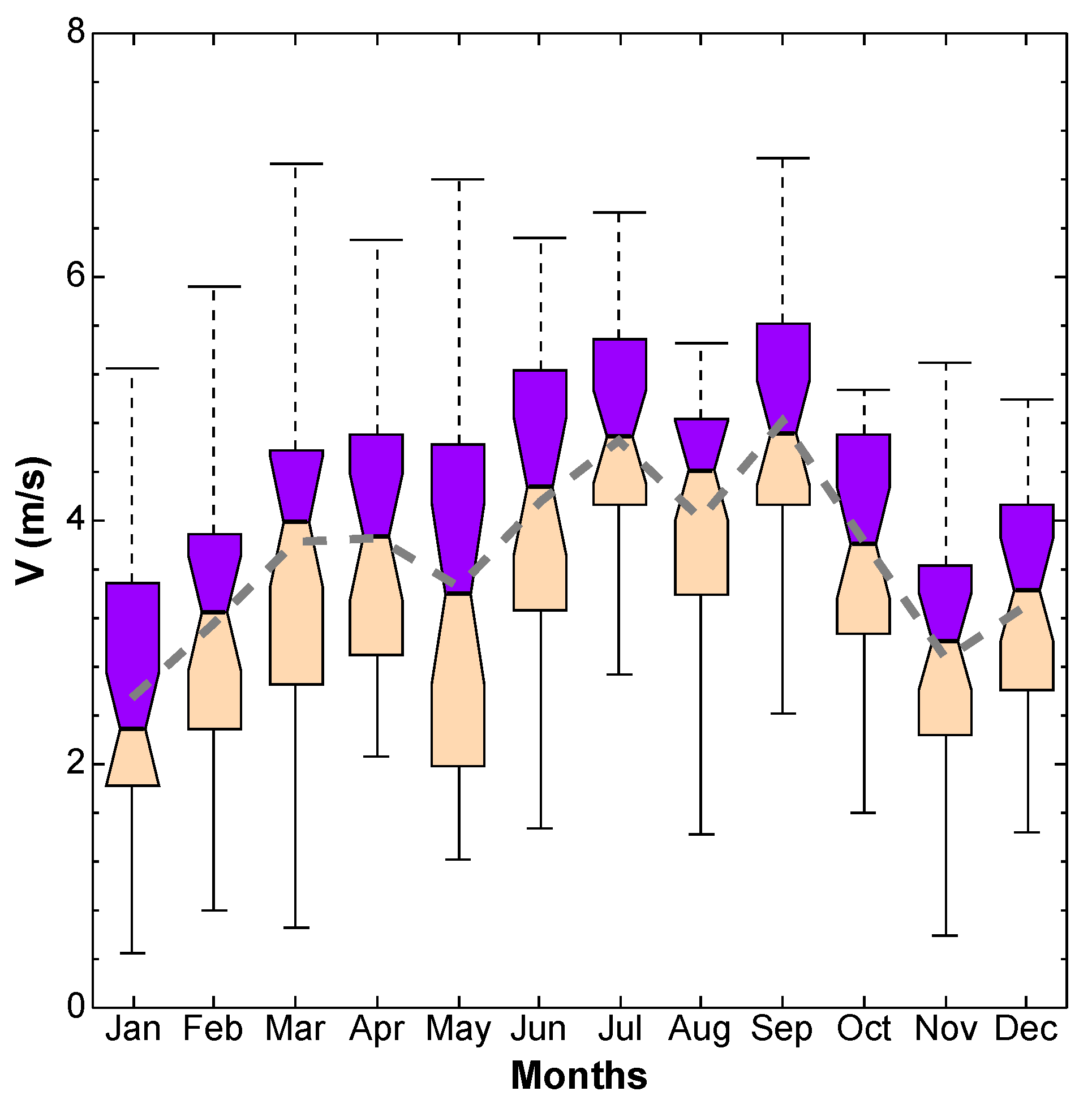
Appendix B
- CS parameters: number of iterations = 10, number of host nests = 25, number of generations = 25, Pa = 0.25.
- PSO parameters: number of iterations = 10, population = 25, wo = 0.1, c1 = 0.25, c2 = 0.99, r1 = 0.3, r2 = 0.45.
- GA parameters: number of iterations = 10, population = 25, crossover probability = 0.6, crossover probability = 0.5.
- The technical and economic parameters of system compounds are given in Table A1.
| Type | Value | Unit |
|---|---|---|
| PV | ||
| Lifetime | 20 | year |
| Initial cost | 600 | $/kW |
| Operational and maintenance cost | 0.01 | $/kW |
| CO2 emissions | 0.0225 | Kg/kWh |
| Grid | ||
| CO2 emissions | 0.143 | Kg/kWh |
| Converter | ||
| Lifetime | 20 | Years |
| Initial costs | 515 | $/kWh |
| Efficiency | 95 | % |
| Others | ||
| Project lifetime | 20 | Years |
| Interest rate | 13 | % |
| Inflation rate | 5 | % |
References
- Salem, M.; Tsurusaki, N.; Divigalpitiya, P. Land use/land cover change detection and urban sprawl in the peri-urban area of greater Cairo since the Egyptian revolution of 2011. J. Land Use Sci. 2020, 15, 592–606. [Google Scholar] [CrossRef]
- Salem, M.; Tsurusaki, N.; Divigalpitiya, P. Remote sensing-based detection of agricultural land losses around Greater Cairo since the Egyptian revolution of 2011. Land Use Policy 2020, 97, 104744. [Google Scholar] [CrossRef]
- Konapala, G.; Mishra, A.K.; Wada, Y.; Mann, M.E. Climate change will affect global water availability through compounding changes in seasonal precipitation and evaporation. Nat. Commun. 2020, 11, 1–10. [Google Scholar] [CrossRef]
- Luo, P.; Sun, Y.; Wang, S.; Wang, S.; Lyu, J.; Zhou, M.; Nakagami, K.; Takara, K.; Nover, D. Historical assessment and future sustainability challenges of Egyptian water resources management. J. Clean. Prod. 2020, 263, 121154. [Google Scholar] [CrossRef]
- Adnan, R.M.; Chen, Z.; Yuan, X.; Kisi, O.; El-Shafie, A.; Kuriqi, A.; Ikram, M. Reference Evapotranspiration Modeling Using New Heuristic Methods. Entropy 2020, 22, 547. [Google Scholar] [CrossRef] [PubMed]
- Abdelhafez, A.A.; Metwalley, S.M.; Abbas, H.H. Irrigation: Water Resources, Types and Common Problems in Egypt. In Springer Water; Springer: Cham, Switzerland, 2020; pp. 15–34. [Google Scholar]
- Fawzy, H.E.-D.; Sakr, A.; El-Enany, M.; Moghazy, H.M. Spatiotemporal assessment of actual evapotranspiration using satellite remote sensing technique in the Nile Delta, Egypt. Alex. Eng. J. 2021, 60, 1421–1432. [Google Scholar] [CrossRef]
- Sharma, V.; Chandel, S. Performance analysis of a 190 kWp grid interactive solar photovoltaic power plant in India. Energy 2013, 55, 476–485. [Google Scholar] [CrossRef]
- Baradei, S.E.; Sadeq, M.A. Effect of Solar Canals on Evaporation, Water Quality, and Power Production: An Optimization Study. Water 2020, 12, 2103. [Google Scholar] [CrossRef]
- Kumar, M.; Chandel, S.; Kumar, A. Performance analysis of a 10 MWp utility scale grid-connected canal-top photovoltaic power plant under Indian climatic conditions. Energy 2020, 204, 117903. [Google Scholar] [CrossRef]
- Gorjian, S.; Sharon, H.; Ebadi, H.; Kant, K.; Scavo, F.B.; Tina, G.M. Recent Technical Advancements, Economics and Environmental Impacts of Floating Photovoltaic Solar Energy Conversion Systems. J. Clean. Prod. 2021, 278, 124285. [Google Scholar] [CrossRef]
- Sairam, P.M.; Aravindhan, A. Canal top solar panels: a unique nexus of energy, water, and land. Mater. Today Proc. 2020, 33, 705–710. [Google Scholar] [CrossRef]
- Cazzaniga, R.; Cicu, M.; Rosa-Clot, M.; Tina, G.; Ventura, C. Floating photovoltaic plants: Performance analysis and design solutions. Renew. Sustain. Energy Rev. 2018, 81, 1730–1741. [Google Scholar] [CrossRef]
- Santafé, M.R.; Gisbert, P.S.F.; Romero, F.J.S.; Soler, J.B.T.; Gozálvez, J.J.F.; Gisbert, C.M.F. Implementation of a photovoltaic floating cover for irrigation reservoirs. J. Clean. Prod. 2014, 66, 568–570. [Google Scholar] [CrossRef]
- Colmenar-Santos, A.; Buendia-Esparcia, Á.; de Palacio-Rodríguez, C.; Borge-Diez, D. Water canal use for the implementation and efficiency optimization of photovoltaic facilities: Tajo-Segura transfer scenario. Sol. Energy 2016, 126, 168–194. [Google Scholar] [CrossRef]
- Yadav, N.; Gupta, M.; Sudhakar, K. Energy assessment of floating photovoltaic system. In Proceedings of the 2016 International Conference on Electrical Power and Energy Systems (ICEPES), Bhopal, India, 14–16 December 2016; pp. 264–269. [Google Scholar]
- Kougias, I.; Bódis, K.; Jäger-Waldau, A.; Monforti-Ferrario, F.; Szabó, S. Exploiting existing dams for solar PV system installations. Prog. Photovolt. Res. Appl. 2016, 24, 229–239. [Google Scholar] [CrossRef]
- Lee, Y.-G.; Joo, H.-J.; Yoon, S.-J. Design and installation of floating type photovoltaic energy generation system using FRP members. Sol. Energy 2014, 108, 13–27. [Google Scholar] [CrossRef]
- Chrysochoidis-Antos, N.; Chrysochoidis, C. Benefits from PV system integration and drainage infrastructure: Case study for Thessaloniki-Imathia-Pella plain in Greece. In Proceedings of the 33rd European Photovoltaic Energy Conference and Exhibition, Thessaloniki, Greece, 14 December 2017; pp. 2151–2159. [Google Scholar]
- Kougias, I.; Bódis, K.; Jäger-Waldau, A.; Moner-Girona, M.; Monforti-Ferrario, F.; Ossenbrink, H.; Szabó, S. The potential of water infrastructure to accommodate solar PV systems in Mediterranean islands. Sol. Energy 2016, 136, 174–182. [Google Scholar] [CrossRef]
- Available online: https://www.firstpost.com (accessed on 24 March 2021).
- Kumar, M.; Kumar, A. Performance assessment and degradation analysis of solar photovoltaic technologies: A review. Renew. Sustain. Energy Rev. 2017, 78, 554–587. [Google Scholar] [CrossRef]
- Kumar, M.; Kumar, A.; Gupta, R. Comparative degradation analysis of different photovoltaic technologies on experimentally simulated water bodies and estimation of evaporation loss reduction. Prog. Photovolt. Res. Appl. 2021, 29, 357–378. [Google Scholar] [CrossRef]
- Meziani, A.; Remini, B.; Boutoutaou, D. Estimating Evaporation from Dam-Reservoirs in Arid and Semi Arid Regions Case of Algeria. J. Eng. Appl. Sci. 2020, 15, 2097–2107. [Google Scholar]
- Bradei, S.E.; Alsadeq, M. Impact of covering irrigation canals on evaporation rates in arid areas. In Proceedings of the Response Design and Delivery of the Constructed Project; ISEC Press, American University of Beirut: Lebanon, IN, USA, 2018; pp. 1–6. [Google Scholar]
- AbdElrehim, H.A.M. Techno-economic feasibility of PV Irrigation in Egypt. In Proceedings of the International Scientific Conference Micro Perspectives for Decentralized Energy Supply; Technishe Universitat Berlin, Micro Systems: Bangalore, India, 2015; pp. 1–31. [Google Scholar]
- Dai, L.; Mao, J.; Wang, Y.; Dai, H.; Zhang, P.; Guo, J. Optimal operation of the Three Gorges Reservoir subject to the ecological water level of Dongting Lake. Environ. Earth Sci. 2016, 75, 1111. [Google Scholar] [CrossRef]
- Kumar, M.; Kumar, A. Experimental validation of performance and degradation study of canal-top photovoltaic system. Appl. Energy 2019, 243, 102–118. [Google Scholar] [CrossRef]
- Perraki, V.; Kounavis, P. Effect of temperature and radiation on the parameters of photovoltaic modules. J. Renew. Sustain. Energy 2016, 8, 013102. [Google Scholar] [CrossRef]
- Rawat, R.; Kaushik, S.; Sastry, O.; Singh, Y.K.; Bora, B.J. Energetic and exergetic performance analysis of CdS/CdTe based photovoltaic technology in real operating conditions of composite climate. Energy Convers. Manag. 2016, 110, 42–50. [Google Scholar] [CrossRef]
- Kumar, M.; Kumar, A. Performance Assessment of Different Photovoltaic Technologies for Canal-Top and Reservoir Applications in Subtropical Humid Climate. IEEE J. Photovolt. 2019, 9, 722–732. [Google Scholar] [CrossRef]
- Singh, R.; Sharma, M.; Rawat, R.; Banerjee, C. An assessment of series resistance estimation techniques for different silicon based SPV modules. Renew. Sustain. Energy Rev. 2018, 98, 199–216. [Google Scholar] [CrossRef]
- Kirmani, S.; Kalimullah, M. Degradation Analysis of a Rooftop Solar Photovoltaic System—A Case Study. Smart Grid Renew. Energy 2017, 8, 212–219. [Google Scholar] [CrossRef]
- Abo-Elyousr, F.K.; Elnozahy, A. Bi-objective economic feasibility of hybrid micro-grid systems with multiple fuel options for islanded areas in Egypt. Renew. Energy 2018, 128, 37–56. [Google Scholar] [CrossRef]
- Borowy, B.S.; Salameh, Z.M. Methodology for optimally sizing the combination of a battery bank and PV array in a wind/PV hybrid system. IEEE Trans. Energy Convers. 1996, 11, 367–375. [Google Scholar] [CrossRef]
- Chedid, R.; Rahman, S. Unit sizing and control of hybrid wind-solar power systems. IEEE Trans. Energy Convers. 1997, 12, 79–85. [Google Scholar] [CrossRef]
- Abdelshafy, A.M.; Jurasz, J.; Hassan, H.; Mohamed, A.M. Optimized energy management strategy for grid connected double storage (pumped storage-battery) system powered by renewable energy resources. Energy 2020, 192, 116615. [Google Scholar] [CrossRef]
- Maleki, A. Design and optimization of autonomous solar-wind-reverse osmosis desalination systems coupling battery and hydrogen energy storage by an improved bee algorithm. Desalination 2018, 435, 221–234. [Google Scholar] [CrossRef]
- Heydari, A.; Askarzadeh, A. Optimization of a biomass-based photovoltaic power plant for an off-grid application subject to loss of power supply probability concept. Appl. Energy 2016, 165, 601–611. [Google Scholar] [CrossRef]
- Erdinc, O.; Uzunoglu, M. Optimum design of hybrid renewable energy systems: Overview of different approaches. Renew. Sustain. Energy Rev. 2012, 16, 1412–1425. [Google Scholar] [CrossRef]
- Kaur, R.; Krishnasamy, V.; Kandasamy, N.K.; Kumar, S. Discrete Multiobjective Grey Wolf Algorithm Based Optimal Sizing and Sensitivity Analysis of PV-Wind-Battery System for Rural Telecom Towers. IEEE Syst. J. 2020, 14, 729–737. [Google Scholar] [CrossRef]
- Makhdoomi, S.; Askarzadeh, A. Optimizing operation of a photovoltaic/diesel generator hybrid energy system with pumped hydro storage by a modified crow search algorithm. J. Energy Storage 2020, 27, 101040. [Google Scholar] [CrossRef]
- Abo-Elyousr, F.K.; Abdelshafy, A.M.; Abdelaziz, A.Y. MPPT-Based Particle Swarm and Cuckoo Search Algorithms for PV Systems. In Smart and Sustainable Planning for Cities and Regions; Springer: Berlin/Heidelberg, Germany, 2020; pp. 379–400. [Google Scholar]
- Edathil, S.L.; Singh, S.P. ACO and CS-based hybrid optimisation method for optimum sizing of the SHES. IET Renew. Power Gener. 2019, 13, 1789–1801. [Google Scholar] [CrossRef]
- Nguyen, T.T.; Vo, D.N.; Quynh, N.V.; Van Dai, L. Modified Cuckoo Search Algorithm: A Novel Method to Minimize the Fuel Cost. Energies 2018, 11, 1328. [Google Scholar] [CrossRef]
- Abdrabbo, A.A.K. Crop Water Productivity as Influenced by Irrigation Improvement in the Nile Delta. In Proceedings of the 2009 Reno, Reno, Nevada, 21–24 June 2009. [Google Scholar]
- Wackernagel, M.; Hanscom, L.; Lin, D. Making the Sustainable Development Goals Consistent with Sustainability. Front. Energy Res. 2017, 5, 5. [Google Scholar] [CrossRef]
- Stafford-Smith, M.; Griggs, D.; Gaffney, O.; Ullah, F.; Reyers, B.; Kanie, N.; Stigson, B.; Shrivastava, P.; Leach, M.; O’Connell, D. Integration: The key to implementing the Sustainable Development Goals. Sustain. Sci. 2017, 12, 911–919. [Google Scholar] [CrossRef] [PubMed]
- Yang, X.-S. Engineering Optimization: An Introduction with Metaheuristic Applications; John Wiley & Sons: Hoboken, NJ, USA, 2010. [Google Scholar]
- Mohamad, A.B.; Zain, A.M.; Bazin, N.E.N. Cuckoo Search Algorithm for Optimization Problems—A Literature Review and its Applications. Appl. Artif. Intell. 2014, 28, 419–448. [Google Scholar] [CrossRef]
- Bulatović, R.R.; Đorđević, S.R.; Đorđević, V.S. Cuckoo Search algorithm: A metaheuristic approach to solving the problem of optimum synthesis of a six-bar double dwell linkage. Mech. Mach. Theory 2013, 61, 1–13. [Google Scholar] [CrossRef]
- Lang, D.; Zheng, J.; Shi, J.; Liao, F.; Ma, X.; Wang, W.; Chen, X.; Zhang, M. A Comparative Study of Potential Evapotranspiration Estimation by Eight Methods with FAO Penman–Monteith Method in Southwestern China. Water 2017, 9, 734. [Google Scholar] [CrossRef]
- Todorovic, M.; Karic, B.; Pereira, L.S. Reference evapotranspiration estimate with limited weather data across a range of Mediterranean climates. J. Hydrol. 2013, 481, 166–176. [Google Scholar] [CrossRef]
- Eshra, N.M.; Zobaa, A.F.; Aleem, S.H.A. Assessment of mini and micro hydropower potential in Egypt: Multi-criteria analysis. Energy Rep. 2021, 7, 81–94. [Google Scholar] [CrossRef]
- Nagananthini, R.; Nagavinothini, R. Investigation on floating photovoltaic covering system in rural Indian reservoir to minimize evaporation loss. Int. J. Sustain. Energy 2021, 10, 1–25. [Google Scholar] [CrossRef]
- Elnozahy, A.; Ramadan, H.; Abo-Elyousr, F.K. Efficient Metaheuristic Utopia-based Multi-Objective Solutions of Optimal Battery-Mix Storage for Microgrids. J. Clean. Prod. 2021, 303, 127038. [Google Scholar] [CrossRef]
- Kaabeche, A.; Bakelli, Y. Renewable hybrid system size optimization considering various electrochemical energy storage technologies. Energy Convers. Manag. 2019, 193, 162–175. [Google Scholar] [CrossRef]
- Abo-Elyousr, F.K.; Abbas, H.S.; Yousef, A.M.; Quynh, N.V.; Ali, Z.M.; Nazir, M.S. Oscillation Damping for Wind Energy Conversion System with Doubly Fed Induction Generator Association with Synchronous Generator. Energies 2020, 13, 5067. [Google Scholar] [CrossRef]


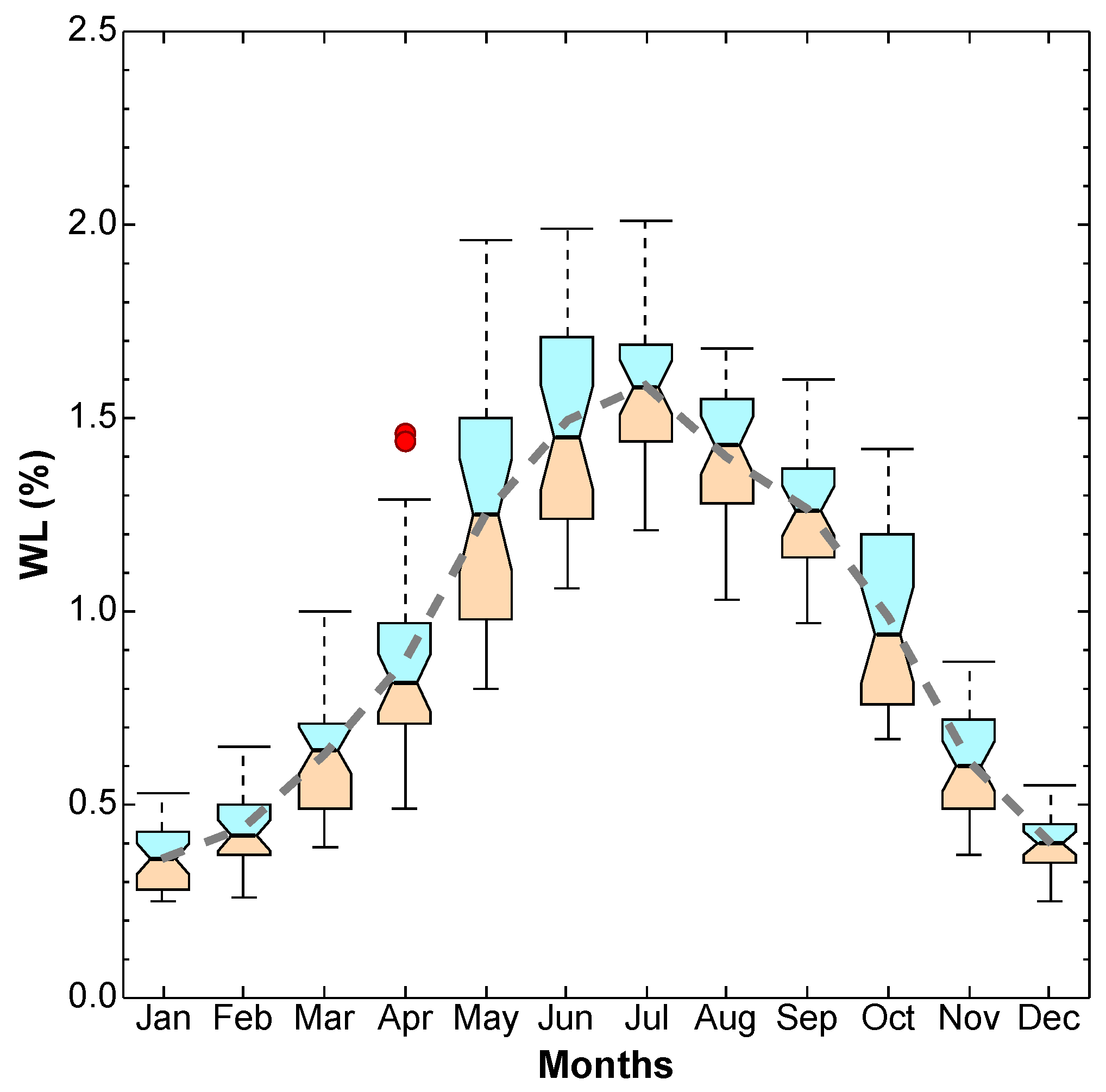
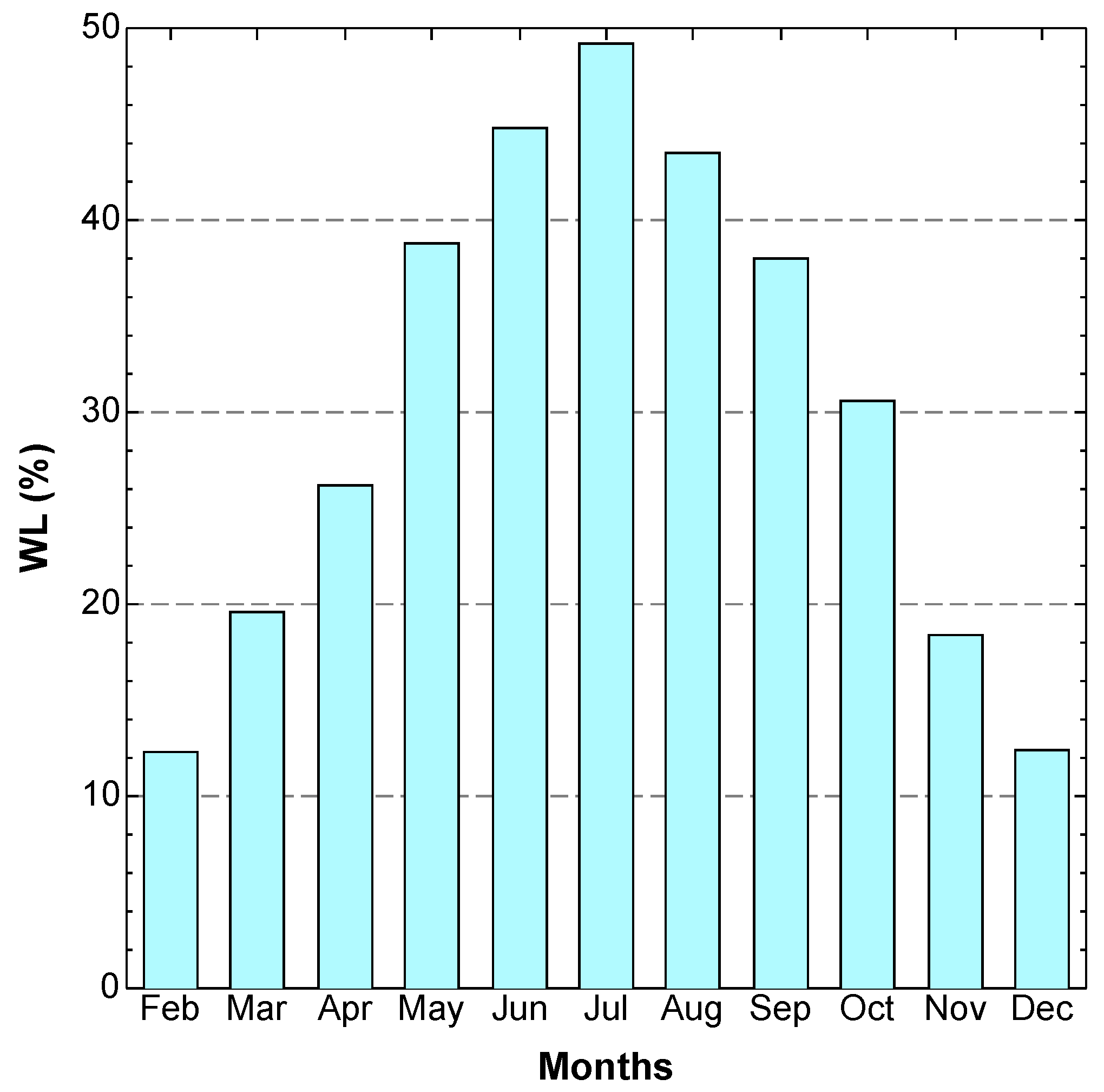
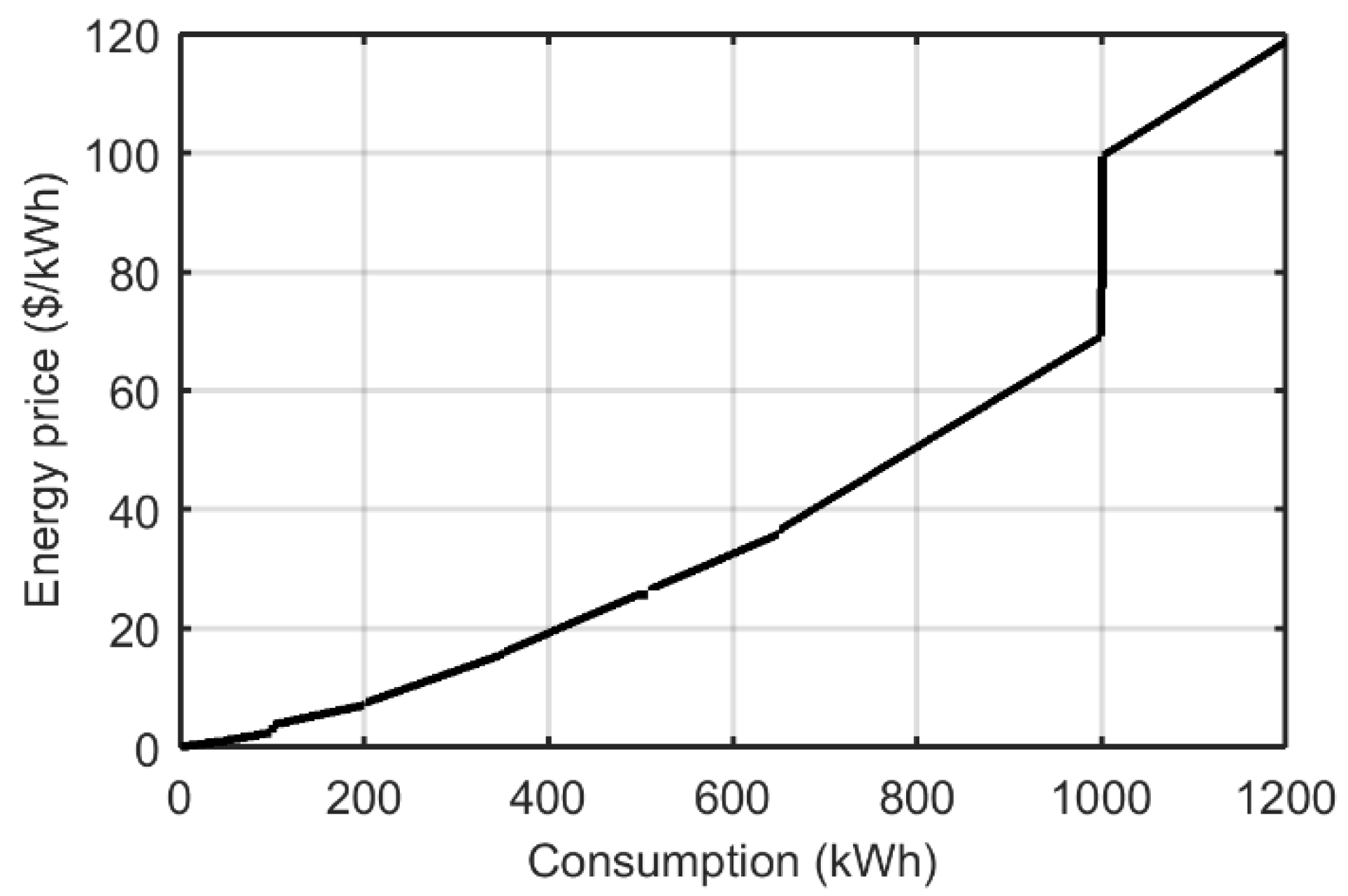
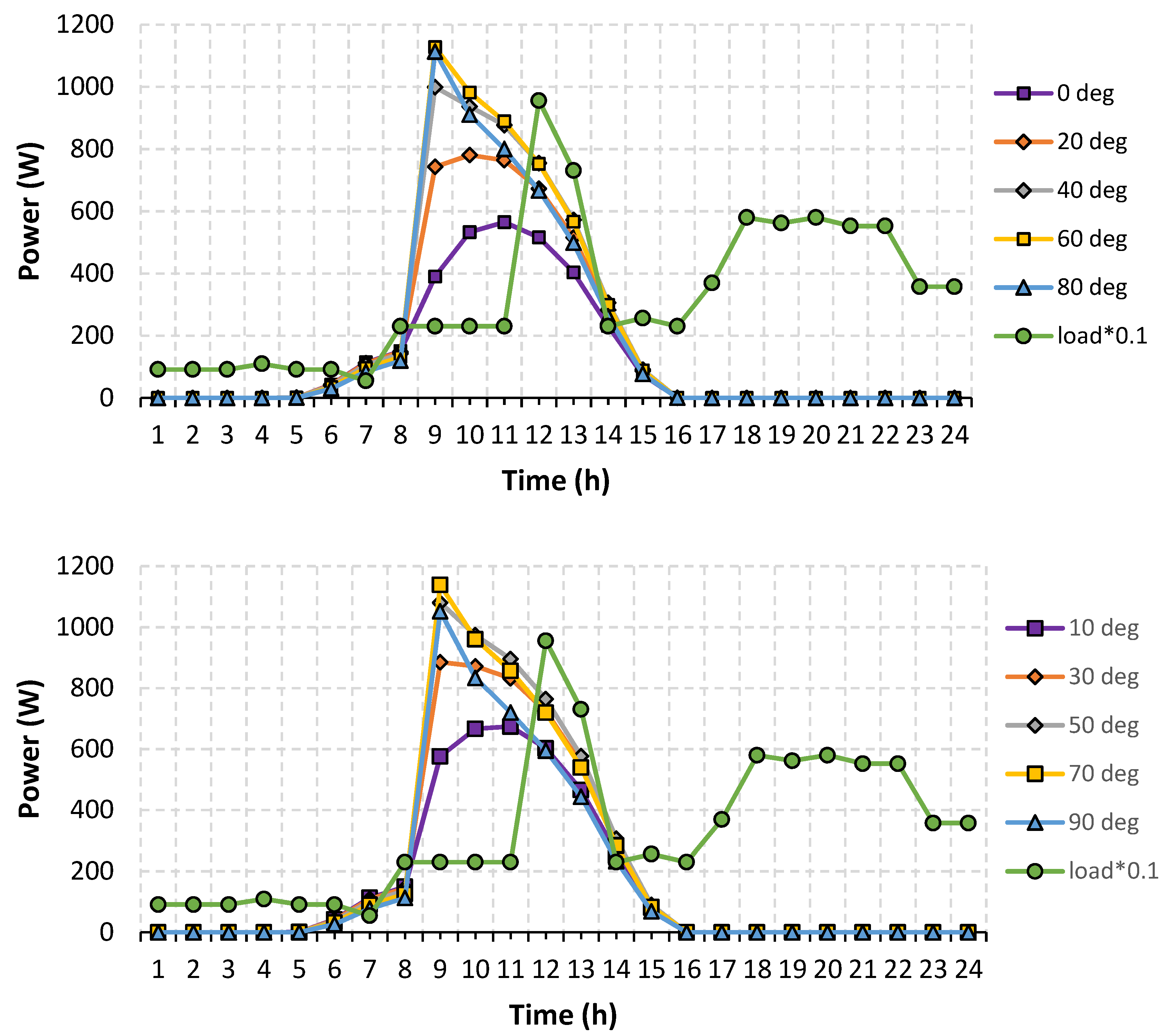
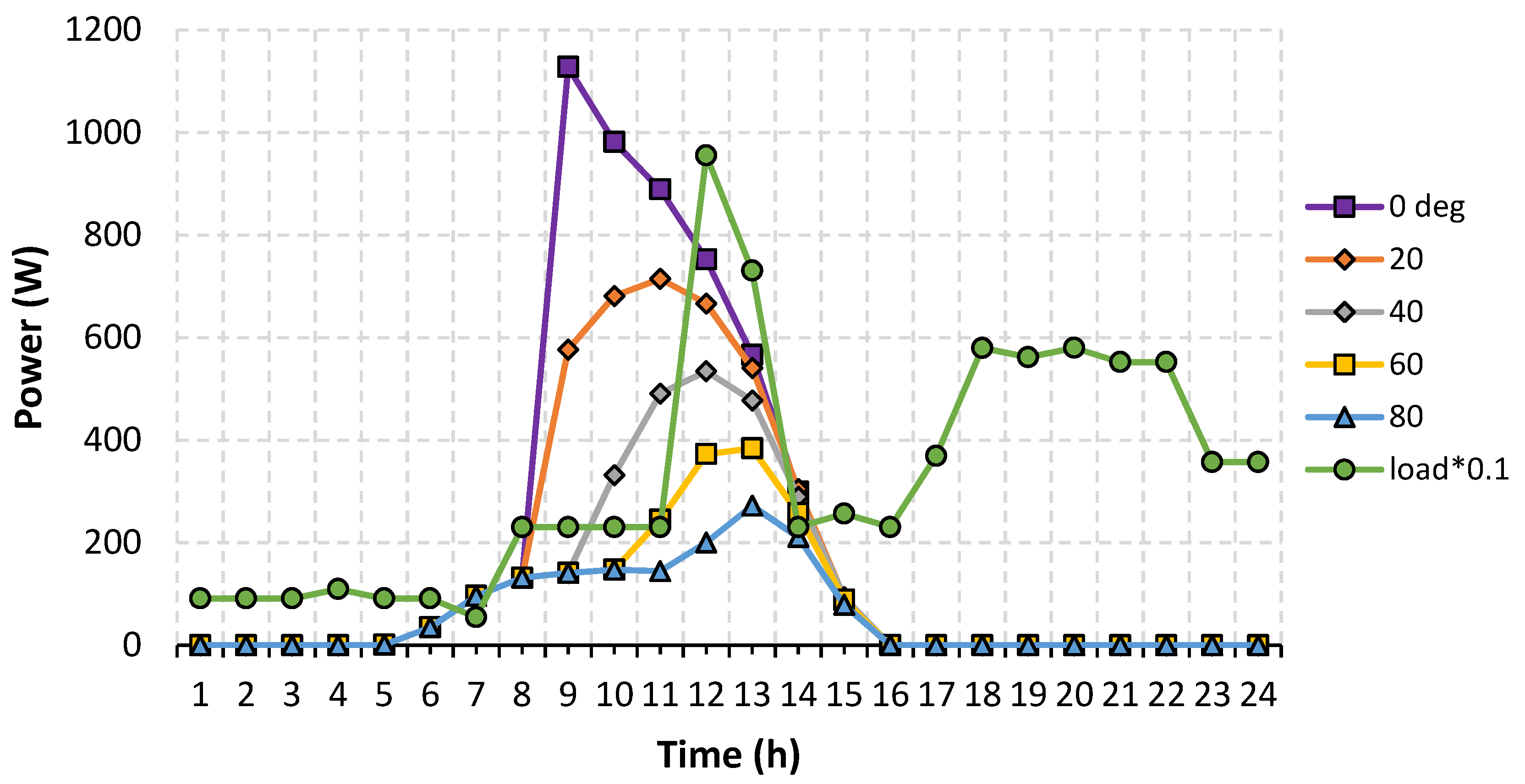

| Month | Avg. GHI [Wh/m2] | Avg. DHI [W/m2] | Avg. Temp. (°C) | Avg. Rh (%) | Avg. Wind Speed (m/s) | Avg. E (mm) |
|---|---|---|---|---|---|---|
| Jan | 4003.6 | 6.91 | 11.4 | 38.5 | 2.6 | 3.6 |
| Feb | 4905.1 | 10.4 | 13.7 | 40.8 | 3.1 | 4.4 |
| Mar | 6189.4 | 11 | 16.8 | 31.2 | 3.8 | 6.3 |
| Apr | 6684.4 | 14.7 | 21.9 | 25.6 | 3.9 | 8.7 |
| May | 7482.4 | 20.7 | 29.6 | 15.2 | 3.5 | 12.5 |
| Jun | 7515.3 | 27.8 | 32.2 | 21.6 | 4.2 | 14.9 |
| Jul | 7637.2 | 29.8 | 32.2 | 23.2 | 4.7 | 15.9 |
| Aug | 7174.5 | 29.8 | 31.9 | 24.4 | 4.0 | 14.0 |
| Sep | 6461.1 | 25.9 | 28.6 | 31.0 | 4.8 | 12.7 |
| Oct | 5351.7 | 20.8 | 26.3 | 32.0 | 3.8 | 9.9 |
| Nov | 4477.5 | 16.6 | 21.0 | 37.1 | 2.9 | 6.1 |
| Dec | 3979.2 | 10.2 | 14.1 | 50.8 | 3.3 | 4.0 |
| Algorithm | Canal-top PV (kW) | LCOE ($/kWh) | GHG (ton) | TNPC (k$) | LPSP | Yearly Evaporation * (m3) |
|---|---|---|---|---|---|---|
| GA | 6.1 | 0.811 | 64.9 | 161.1 | 0 | 6.9 |
| PSO | 10.4 | 0.707 | 67.3 | 140.6 | 0 | 6.9 |
| CS | 10.4 | 0.707 | 67.3 | 140.6 | 0 | 6.9 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alhejji, A.; Kuriqi, A.; Jurasz, J.; Abo-Elyousr, F.K. Energy Harvesting and Water Saving in Arid Regions via Solar PV Accommodation in Irrigation Canals. Energies 2021, 14, 2620. https://doi.org/10.3390/en14092620
Alhejji A, Kuriqi A, Jurasz J, Abo-Elyousr FK. Energy Harvesting and Water Saving in Arid Regions via Solar PV Accommodation in Irrigation Canals. Energies. 2021; 14(9):2620. https://doi.org/10.3390/en14092620
Chicago/Turabian StyleAlhejji, Ayman, Alban Kuriqi, Jakub Jurasz, and Farag K. Abo-Elyousr. 2021. "Energy Harvesting and Water Saving in Arid Regions via Solar PV Accommodation in Irrigation Canals" Energies 14, no. 9: 2620. https://doi.org/10.3390/en14092620
APA StyleAlhejji, A., Kuriqi, A., Jurasz, J., & Abo-Elyousr, F. K. (2021). Energy Harvesting and Water Saving in Arid Regions via Solar PV Accommodation in Irrigation Canals. Energies, 14(9), 2620. https://doi.org/10.3390/en14092620









