Investigation on Bubble Diameter Distribution in Upward Flow by the Two-Fluid and Multi-Fluid Models
Abstract
:1. Introduction
2. Model Description and Numerical Dispersion
2.1. Governing Equation of the Two-Fluid Model
2.2. Governing Equation of the Multi-Fluid Model
- (1)
- The fluid phase is an incompressible fluid, the gas phase (dispersed phase) is a continuous fluid, and the physical properties of each phase are constant;
- (2)
- The coalescence and fragmentation of bubbles are not considered;
- (3)
- The flow field is isothermal and adiabatic, there is no heat exchange with the external environment, and the phase transition and heat transfer are not considered.
2.3. Coupling and Numerical Dispersion
2.4. Turbulent Model
3. Numerical Configurations and Grid Independence Test
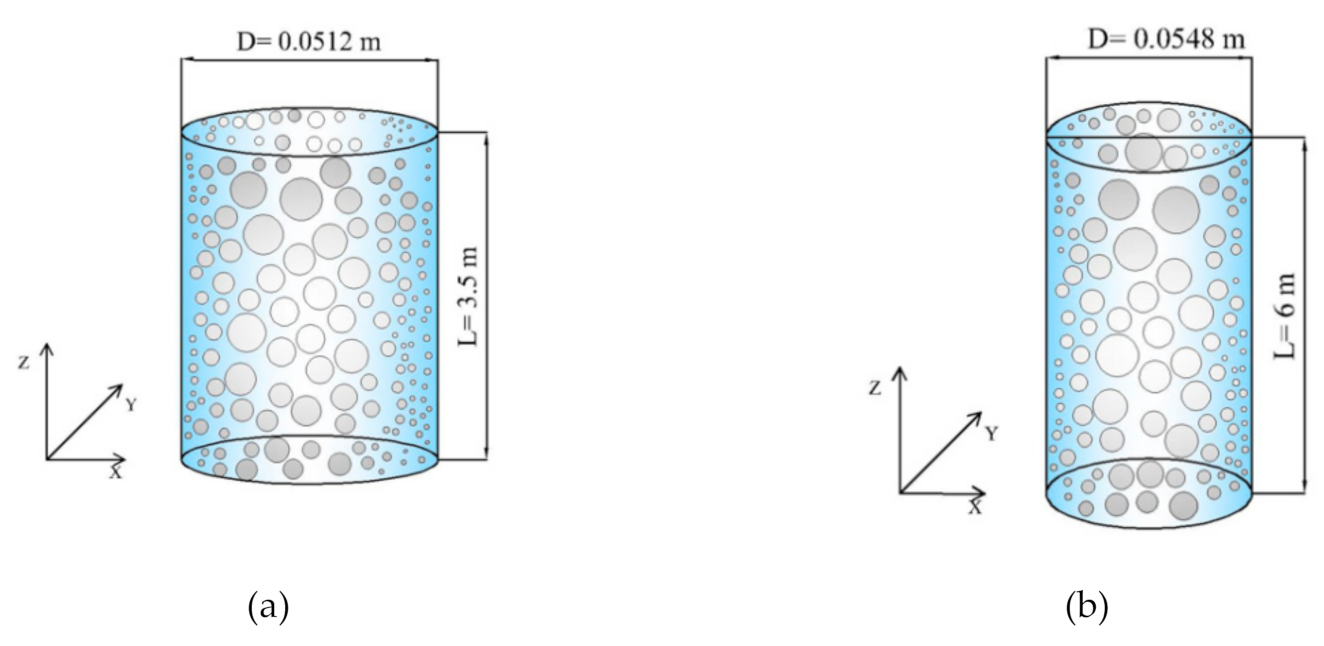
3.1. Numerical Configurations
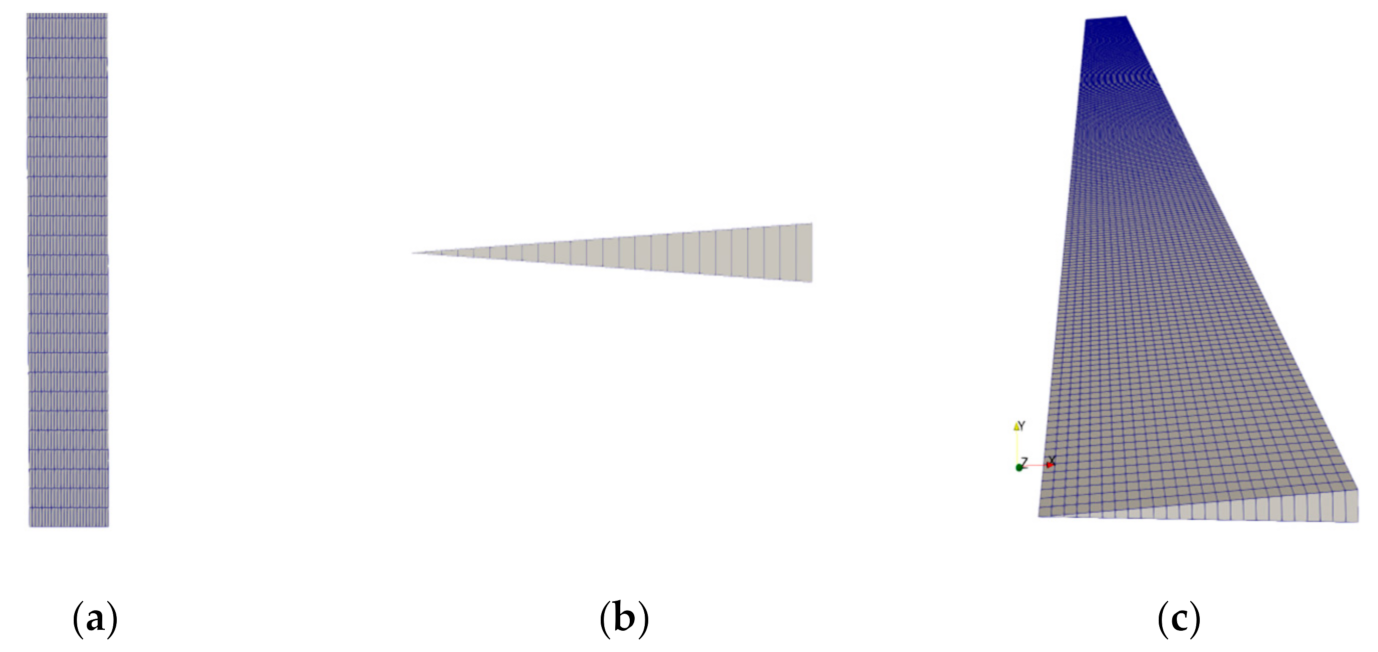
3.2. Computational Domain and Grid Independence Test
Boundary Condition Setting
3.3. Comparison of Different Drag Models
4. Results and Discussion
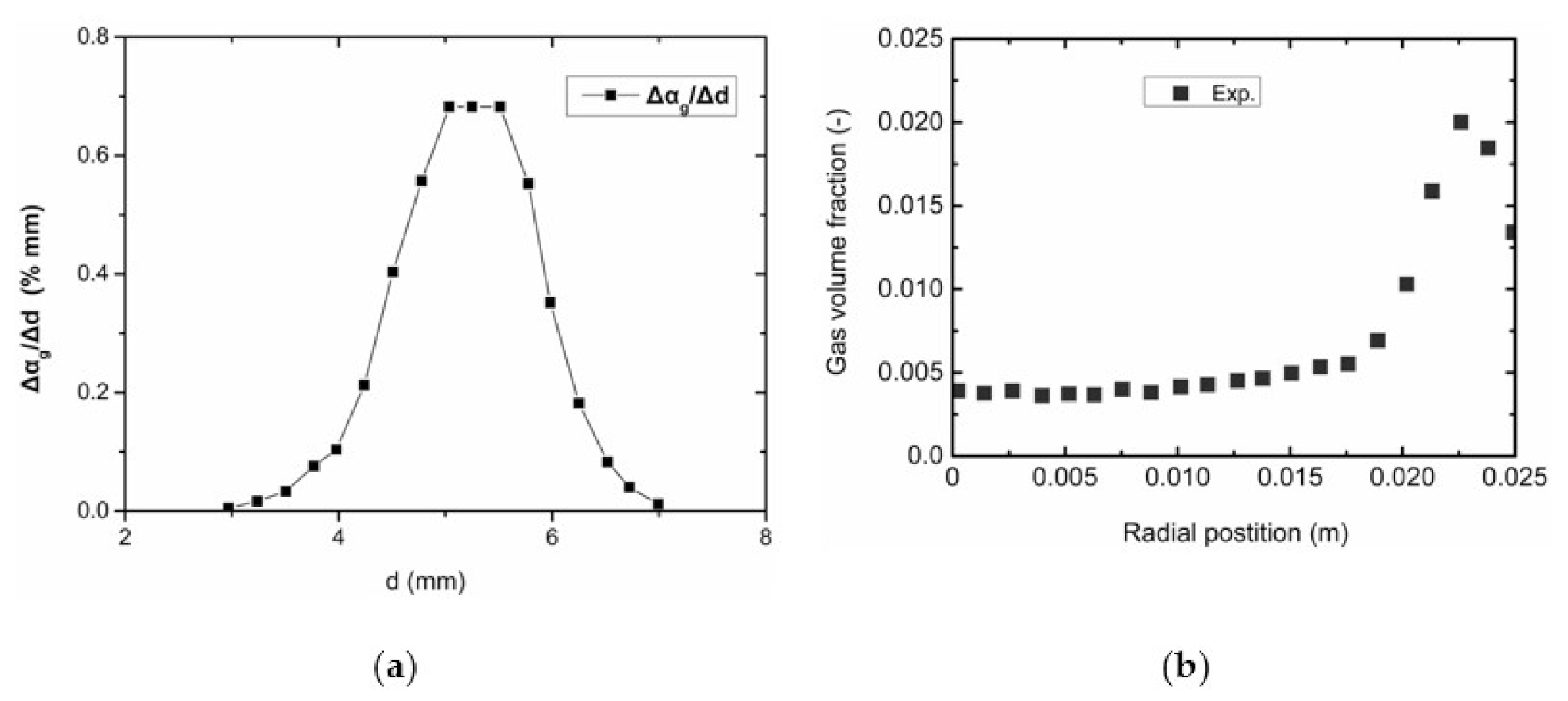
4.1. Experimental Verification Case 1 (Unimodal Distribution)
4.1.1. Influence of Group Number of the Dispersed Phase on the Calculation Results
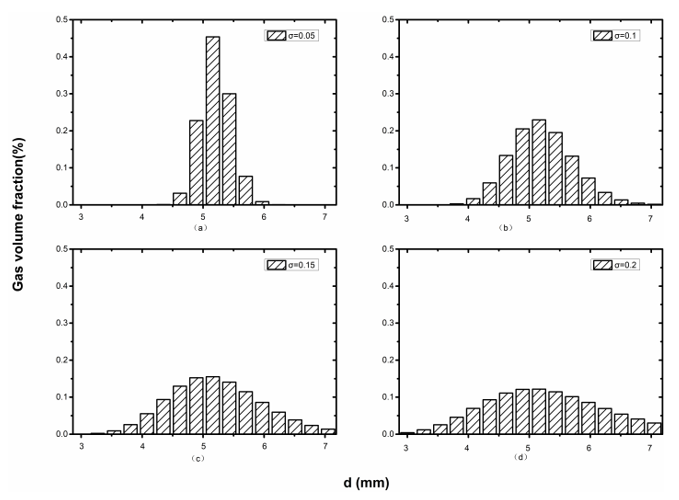
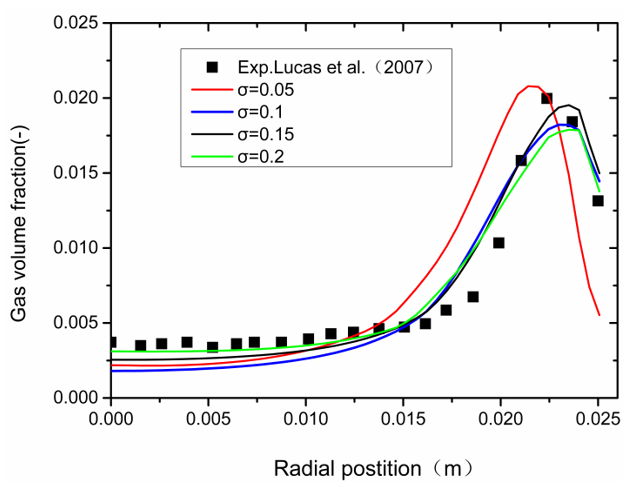
4.1.2. Influence of Parameter σ on the Calculation Results
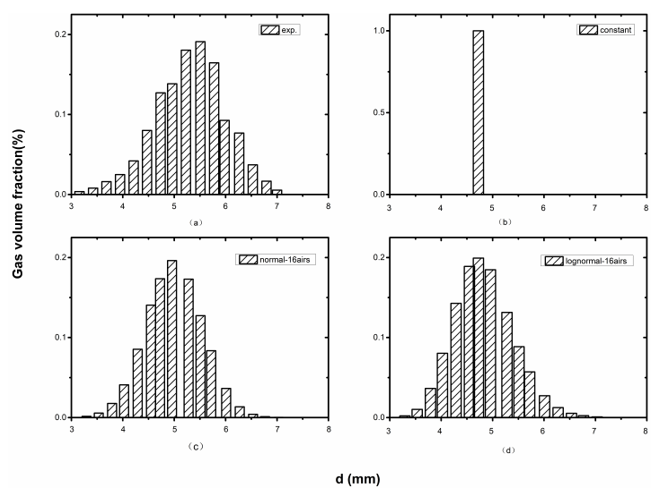
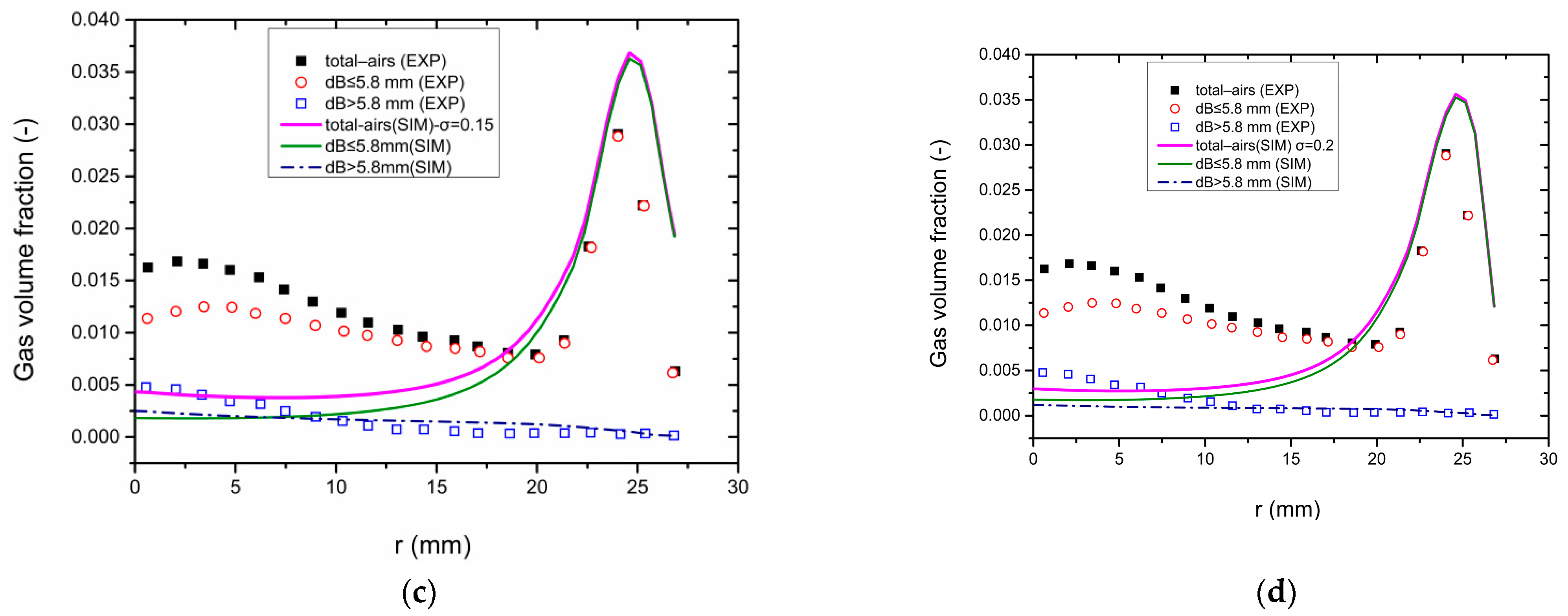
4.1.3. Influence of Different Distribution Functions on the Calculation Results
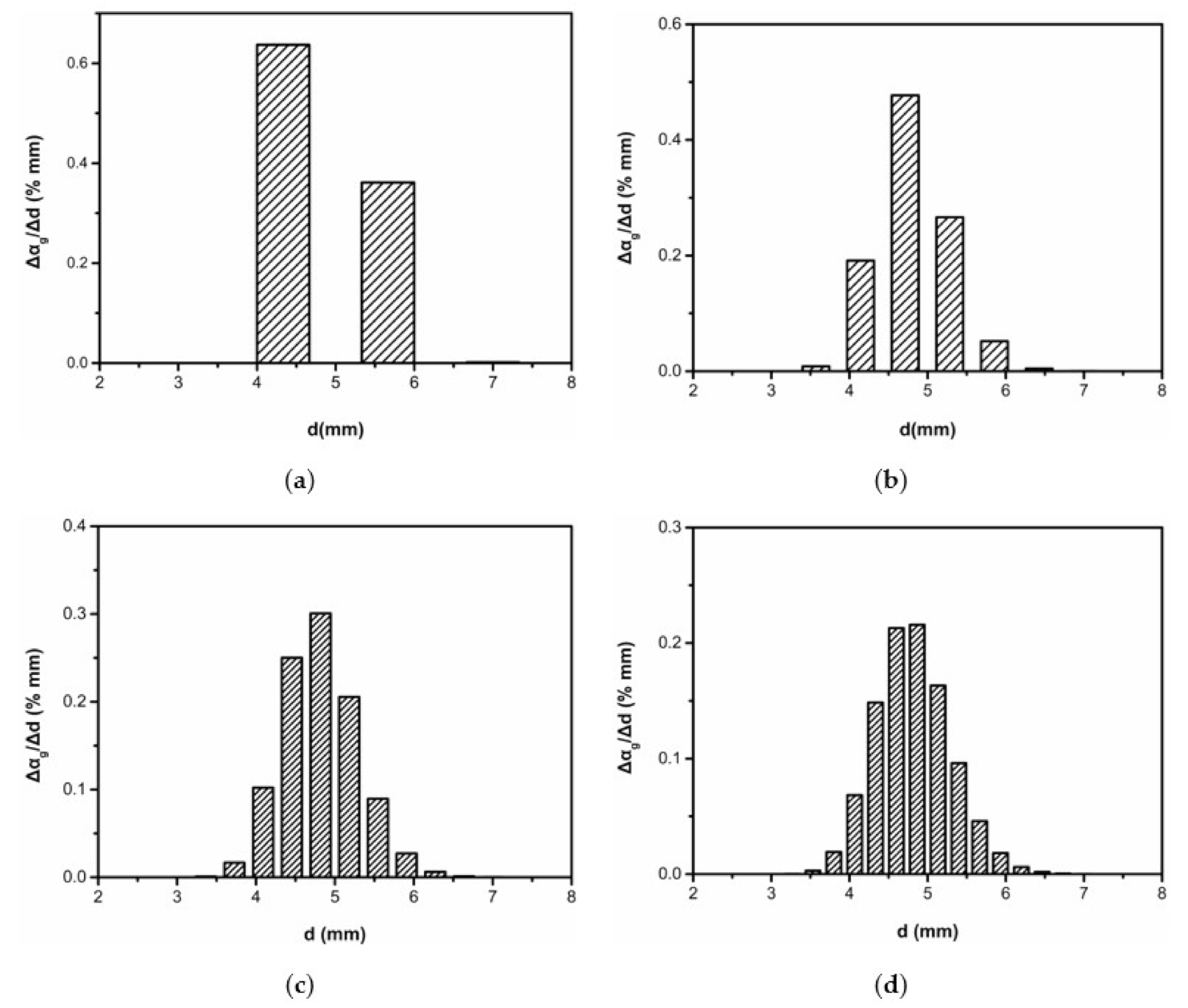
4.2. Experimental Verification Case 2 (Bimodal Distribution)
4.2.1. Calculation Results of E-E Two-Fluid Model
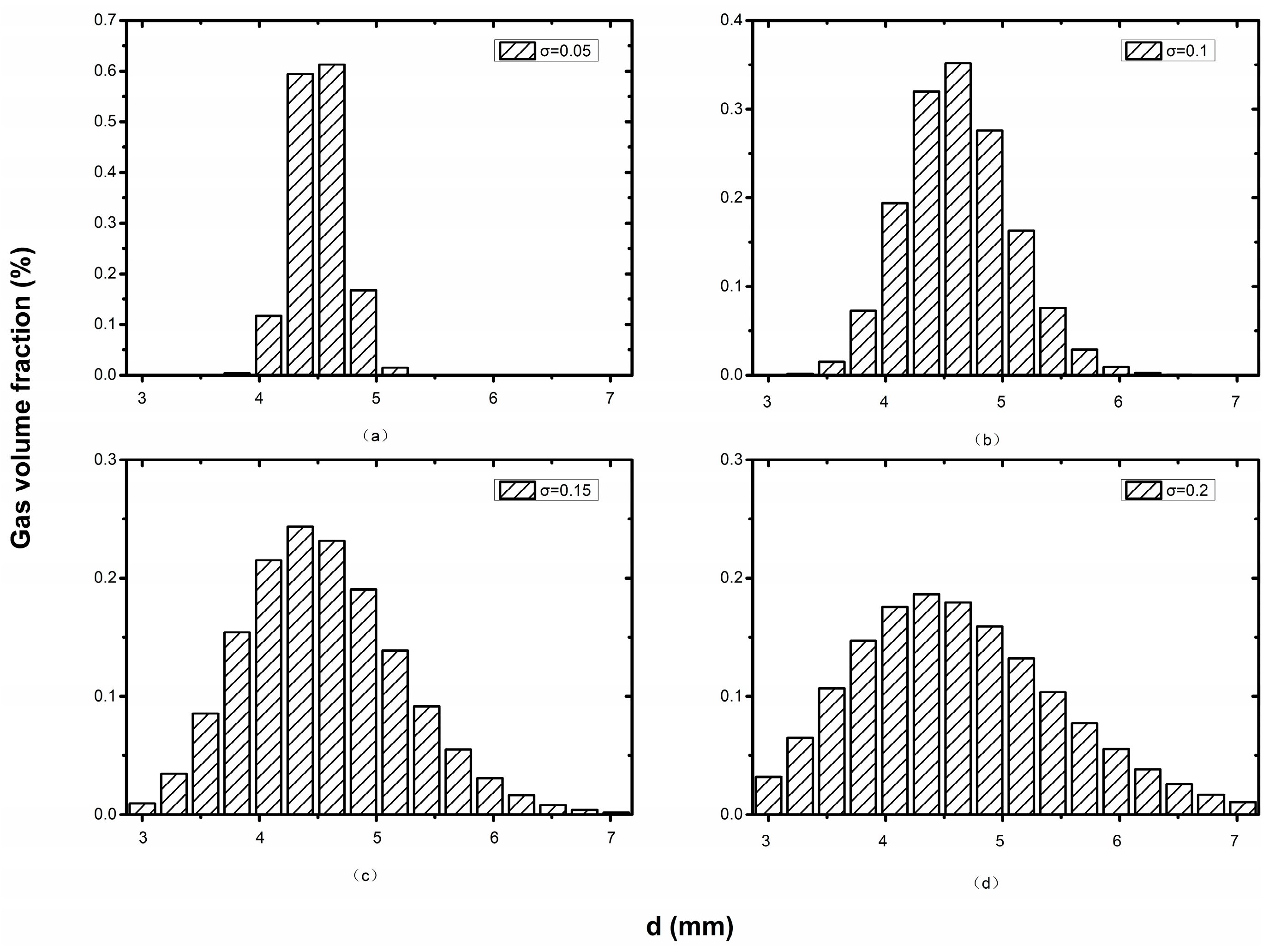
4.2.2. Calculation Results of Multi-Fluid Model with Unimodal and Bimodal Lognormal Distribution of Bubbles Diameters
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Delhaye, J.M. Basic Equations for Two-Phase Flow Modeling. In Two-Phase Flow, and Heat Transfer in the Power and Process Industries; Hemisphere: New York, NY, USA, 1981. [Google Scholar]
- Bankoff, S.G. Handbook of Multiphase Systems. Am. Sci. 1983, 71. Available online: https://www.jstor.org/stable/i27851891 (accessed on 5 September 2021).
- Krepper, E.; Lucas, D.; Prasser, H. On the modelling of bubbly flow in vertical pipes. Nucl. Eng. Des. 2005, 235, 597–611. [Google Scholar] [CrossRef]
- Colombo, M.; Fairweather, M. Accuracy of Eulerian–Eulerian, two-fluid CFD boiling models of subcooled boiling flows. Int. J. Heat Mass Transfer 2016, 103, 28–44. [Google Scholar] [CrossRef]
- Panicker, N.; Passalacqua, A.; Fox, R.O. On the hyperbolicity of the twofluid model for gas–liquid bubbly flows. Appl. Math. Model. 2018, 57, 432–447. [Google Scholar] [CrossRef] [Green Version]
- Legendre, D.; Magnaudet, J. A note on the lift force on a spherical bubble or drop in a low-Reynolds-number shear flow. Phys. Fluids 1997, 9, 3572. [Google Scholar] [CrossRef] [Green Version]
- Legendre, D.; Magnaudet, J. The lift-force on a spherical bubble in a viscous linear shear flow. J. Fluid Mech. 1998, 368, 81. [Google Scholar] [CrossRef]
- Hibiki, T.; Ishii, M. Lift force in bubbly flow systems. Chem. Eng. Sci. 2007, 62, 6457–6474. [Google Scholar] [CrossRef]
- Rzehak, R.; Krepper, E.; Lifante, C. Comparative study of wall-force models for the simulation of bubbly flows. Nucl. Eng. Des. 2012, 253, 41–49. [Google Scholar] [CrossRef]
- Lopez de Bertodano, M.; Lahey, R.T.; Jones, O.C. Phase distribution in bubbly two-phase flow in vertical ducts. Int. J. Multiph. Flow 1994, 20, 805–818. [Google Scholar] [CrossRef]
- Kataoka, I.; Serizawa, A.; Besnard, D.C. Prediction of turbulence suppression and turbulence modeling in bubbly two-phase flow. Nucl. Eng. Des. 1993, 141, 145–158. [Google Scholar] [CrossRef]
- Sato, Y.; Sekoguchi, K. Liquid velocity distribution in two-phase bubbly flow. Int. J. Multiph. Flow 1975, 2, 79–95. [Google Scholar] [CrossRef]
- Tomiyama, A.; Matsuoka, T.; Fukuda, T.; Sakaguchi, T. A Simple Numerical Method for Solving an Incompressible Two-Fluid Model in a General Curvilinear Coordinate System, Advances in Multiphase Flow; Elsevier: Amsterdam, The Netherlands, 1995; Volume 241. [Google Scholar]
- Tomiyama, A.; Shimada, N. A Numerical Method for Bubbly Flow Simulation based on a Multi-Fluid Model. ASME J. Press. Vessel Technol. 2001, 123, 510. [Google Scholar] [CrossRef]
- Tomiyama, A. Modeling and hybrid simulation of bubbly flow. Multiph. Sci. Technol. 2005, 17, 445–482. [Google Scholar] [CrossRef]
- Laın, S.; Bröder, D.; Sommerfeld, M.; Göz, M.F. Modelling hydrodynamics and turbulence in a bubble column using the Euler-Lagrange procedure. Int. J. Multiph. Flow 2002, 28, 1381–1407. [Google Scholar] [CrossRef]
- McGraw, R. Description of aerosol dynamics by the quadrature method of moments. Aerosol. Sci. Tech. 1997, 27, 255–265. [Google Scholar] [CrossRef]
- Dorao, C.A.; Jakobsen, H.A. A least squares method for the solution of population balance problems. Comput. Chem. Eng. 2006, 30, 535–547. [Google Scholar] [CrossRef]
- Petitti, M.; Vanni, M.; Marchisio, D.L.; Buffo, A.; Podenzani, F. Simulation of coalescence, break-up and mass transfer in a gas–liquid stirred tank with CQMOM. Chem. Eng. J. 2013, 228, 1182–1194. [Google Scholar] [CrossRef]
- Lo, S. Application of the MUSIG model to bubbly flows AEAT-1096. AEA Technol. 1996, 230, 8216–8246. [Google Scholar]
- Krepper, E.; Lucas, D.; Frank, T.; Prasser, H.; Zwart, P. The inhomogeneous MUSIG model for the simulation of polydispersed flows. Nucl. Eng. Des. 2008, 238, 1690–1702. [Google Scholar] [CrossRef]
- Lucas, D.; Rzehak, R.; Krepper, E.; Ziegenhein, T.; Liao, Y.; Kriebitzsch, S.; Apanasevich, P. A strategy for the qualification of multi-fluid approaches for nuclear reactor safety. Nucl. Eng. Des. 2016, 299, 2–11. [Google Scholar] [CrossRef]
- Xiao, Q.; Wang, J.; Yang, N.; Li, J. Simulation of the multiphase flow in bubble columns with stability-constrained multi-fluid CFD models. Chem. Eng. J. 2017, 329, 88–99. [Google Scholar] [CrossRef]
- Li, D.; Li, Z.; Gao, Z. Compressibility induced bubble size variation in bubble column reactors: Simulations by the CFD-PBE. Chin. J. Chem. Eng. 2018, 26, 13–17. [Google Scholar] [CrossRef]
- Li, D.; Buffo, A.; Podgórska, W.; Marchisio, D.L.; Gao, Z. Investigation of droplet breakup in liquid–liquid dispersions by CFD–PBM simulations: The influence of the surfactant type. Chin. J. Chem. Eng. 2017, 25, 1369–1380. [Google Scholar] [CrossRef]
- Zhang, X.B.; Zheng, R.Q.; Luo, Z.H. CFD-PBM Simulation of Bubble Columns: Effect of Parameters in the Class Method for Solving PBEs. Chem. Eng. Sci. 2020, 226, 115853. [Google Scholar] [CrossRef]
- An, M.; Guan, X.; Yang, N. Modeling the Effects of Solid Particles in CFD-PBM Simulation of Slurry Bubble Columns. Chem. Eng. Sci. 2020, 223, 115743. [Google Scholar] [CrossRef]
- Li, D.; Marchisio, D.; Hasse, C.; Lucas, D. Comparison of Eulerian QBMM and classical Eulerian–Eulerian method for the simulation of polydisperse bubbly flows. AIChE J. 2019, 65, e16732. [Google Scholar] [CrossRef]
- Li, D.; Marchisio, D.; Hasse, C.; Lucas, D. twoWayGPBEFoam: An open-source Eulerian QBMM solver for monokinetic bubbly flows. Comput. Phys. Commun. 2019, 250, 107036. [Google Scholar] [CrossRef]
- Ishii, M. Thermo-fluid dynamic theory of two-phase flow. NASA Sti/Recon Tech. Rep. A 1976, 75, 29657. [Google Scholar]
- Drew, D.A. Continuum Modeling of Two-Phase Flows. In Theory of Dispersed Multiphase Flow; Academic Press: Cambridge, MA, USA, 1983; pp. 173–190. [Google Scholar]
- Jackson, R. Locally averaged equations of motion for a mixture of identical spherical particles and a Newtonian fluid. Chem. Eng. Sci. 1997, 52, 2457–2469. [Google Scholar] [CrossRef]
- Tomiyama, A.; Kataoka, I.; Zun, I.; Sakaguchi, T. Drag coe8cients of single bubbles under normal and micro gravity conditions. JSME Int. J. Ser. B 1998, 41, 472–479. [Google Scholar] [CrossRef]
- Tomiyama, A.; Tamai, H.; Zun, I.; Hosokawa, S. Transverse migration of single bubbles in simple shear flows. Chem. Eng. Sci. 2002, 57, 1849–1858. [Google Scholar] [CrossRef]
- Hosokawa, S.; Tomiyama, A.; Misaki, S.; Hamada, T. Lateral migration of single bubbles due to the presence of wall. In Proceedings of the ASME 2002 Joint US-European Fluids Engineering Division Conference, Montreal, QC, Canada, 14–18 June 2002; American Society of Mechanical Engineers: Montreal, QC, Canada, 2002. [Google Scholar]
- Biesheuvel, A.; Gorissen, W.C.M. Void fraction disturbances in a uniform bubbly fluid. Int. J. Multiph. Flow 1990, 16, 211–231. [Google Scholar] [CrossRef] [Green Version]
- Li, D.; Christian, H. Simulation of bubbly flows with special numerical treatments of the semi-conservative and fully conservative two-fluid model. Chem. Eng. Sci. 2017, 174, 25–39. [Google Scholar] [CrossRef]
- Rusche, H. Computational Fluid Dynamics of Dispersed Two-Phase Flows at High Phase Fractions. Ph.D. Thesis, University of London, London, UK, 2003. [Google Scholar]
- Sharma, S.L.; Hibiki, T.; Ishii, M.; Schlegel, J.P.; Buchanan, J.R., Jr.; Hogan, K.J.; Guilbert, P.W. An interfacial shear term evaluation study for adiabatic dispersed air–water two-phase flow with the twofluid model using CFD. Nucl. Eng. Des. 2017, 312, 389–398. [Google Scholar] [CrossRef]
- Bhusare, V.H.; Dhiman, M.K.; Kalaga, D.V.; Roy, S.; Joshi, J.B. CFD simulations of a bubble column with and without internals by using OpenFOAM. Chem. Eng. J. 2017, 317, 157–174. [Google Scholar] [CrossRef]
- Lahey, R.T., Jr. The simulation of multidimensional multiphase flows. Nucl. Eng. Des. 2005, 235, 1043–1060. [Google Scholar] [CrossRef]
- Song, Q.; Luo, R.; Yang, X.Y.; Wang, Z. Phase distributions for upward laminar dilute bubbly flows with non-uniform bubble sizes in a vertical pipe. Int. J. Multiph. Flow 2001, 27, 379–390. [Google Scholar] [CrossRef]
- Kashinsky, O.N.; Timkin, L. Slip velocity measurements in an upward bubbly flow by combined LDA and electrodiffusional techniques. Exp. Fluids 1999, 26, 305–314. [Google Scholar] [CrossRef]
- Mohseni, K.; Shkoller, S.; Kosović, B.; Marsden, J.E. Numerical simulations of the lagrangian averaged navier-stokes (lans-α) equations for forced homogeneous isotropic turbulence. American institute of aeronautics & astronautics. Phys. Fluids 2003, 15, 524. [Google Scholar] [CrossRef]
- Lahey, R.T.; Drew, D.A. The analysis of two-phase flow and heat transfer using a multidimensional, four field, two-fluid model. Nucl. Eng. Des. 2001, 204, 29–44. [Google Scholar] [CrossRef]
- Ma, T.; Santarelli, C.; Ziegenhein, T.; Lucas, D.; Fröhlich, J. Direct numerical simulation–based Reynolds-averaged closure for bubble-induced turbulence. Phys. Rev. Fluids 2017, 2, 034301. [Google Scholar] [CrossRef]
- Issa, R.I.; Gosman, A.D.; Watkins, A.P. The computation of compressible and incompressible recirculating flows by a non-iterative implicit scheme. J. Comput. Phys. 1986, 62, 66–82. [Google Scholar] [CrossRef]
- Ishii, M.; Mishima, K. Two-fluid model and hydrodynamic constitutive relations. Nucl. Eng. Des. 1984, 82, 107–126. [Google Scholar] [CrossRef]
- Ahmadi-Befrui, B.; Gosman, A.D.; Issa, R.I.; Watkins, A.P. EPISO—An implicit non-iterative solution procedure for the calculation of flows in reciprocating engine chambers. Comput. Methods Appl. Mech. Eng. 1990, 79, 249–279. [Google Scholar] [CrossRef]
- Tomiyama, A. Struggle with computational bubble dynamics. Multiph. Sci. Technol. 1998, 10, 369–405. [Google Scholar]
- Lucas, D.; Krepper, E.; Prasser, H.-M. Use of models for lift, wall and turbulent dispersion forces acting on bubbles for poly-disperse flows. Chem. Eng. Sci. 2007, 62, 4146–4157. [Google Scholar] [CrossRef]
- Banowski, M.; Hampel, U.; Krepper, E.; Beyer, M.; Lucas, D. Experimental investigation of two-phase pipe flow with ultrafast X-ray tomography and comparison with state-of-the-art CFD simulations. Nucl. Eng. Des. 2017, 336, 90–104. [Google Scholar] [CrossRef]
- Prasser, H.-M.; Beyer, M.; Böttger, A.; Carl, H.; Lucas, D.; Schaffrath, A.; Schütz, P.; Weiß, F.-P.; Zschau, J. Influence of the pipe diameter on the structure of the gas-liquid interface in a vertical two-phase pipe flow. Nucl. Technol. 2005, 152, 3–22. [Google Scholar] [CrossRef]











| Momentum Closure Models | References and Other Parameters Are Cited |
|---|---|
| Drag model | Tomiyama et al. [33] |
| Lift model | Tomiyama et al. [34] |
| Wall model | Hosokawa et al. [35] |
| Turbulent dispersion model | Biesheuvel et al. [36] |
| Geometry | Cylindrical pipe |
| Measurements available | α distribution, liquid velocity |
| Comparison of the Content | The Model Name | |
|---|---|---|
| E-E Multi-Fluid | E-E Two-Fluid | |
| Continuous phase | Navier–Stokes equation | Navier–Stokes equation |
| Dispersed phase | Navier–Stokes equation | Navier–Stokes equation |
| Advantages | Bubbles of different diameters have different speeds The gas volume fraction distribution of bubbles with different diameters can be calculated | Bubbles in the flow field are all considered to have the same velocity and the same diameter |
| Disadvantages | High demand on computing resources | Low demand on computing resources |
| Grid Name | All-Grid-1 | All-Grid-2 | All-Grid-3 | All-Grid-4 |
|---|---|---|---|---|
| Number of mesh nodes along the flow direction | 40 | 105 | 360 | 450 |
| Number of section grids | 1548 | 1548 | 2052 | 2052 |
| Total number of grids | 61,920 | 162,540 | 738,720 | 923,400 |
| Variable | Value of Inlet | Property of Inlet | Value of Outlet | Property of Outlet |
|---|---|---|---|---|
| Gas phase volume fraction G | 0.01 | Fixed Value | zeroGradient | Zero Gradient |
| Liquid volume fraction 1-G | 0.99 | Fixed Value | zeroGradient | Zero Gradient |
| Gas phase velocity (m/s) | 0.96 | Fixed Value | $internalField | No slip |
| Liquid phase velocity (m/s) | 1.0167 | Fixed Value | $internalField | No slip |
| Pressure (pa) | 101,325 | Zero Gradient | $internalField | Fixed Value |
| Liquid turbulent kinetic energy | 0.0041 | Fixed Value | $internalField | Standard wall function |
| Kinetic energy dissipation rate of liquid phase turbulence | 0.188 | Fixed Value | $internalField | Standard wall function |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zeng, Y.; Xu, W. Investigation on Bubble Diameter Distribution in Upward Flow by the Two-Fluid and Multi-Fluid Models. Energies 2021, 14, 5776. https://doi.org/10.3390/en14185776
Zeng Y, Xu W. Investigation on Bubble Diameter Distribution in Upward Flow by the Two-Fluid and Multi-Fluid Models. Energies. 2021; 14(18):5776. https://doi.org/10.3390/en14185776
Chicago/Turabian StyleZeng, Yongzhong, and Weilin Xu. 2021. "Investigation on Bubble Diameter Distribution in Upward Flow by the Two-Fluid and Multi-Fluid Models" Energies 14, no. 18: 5776. https://doi.org/10.3390/en14185776
APA StyleZeng, Y., & Xu, W. (2021). Investigation on Bubble Diameter Distribution in Upward Flow by the Two-Fluid and Multi-Fluid Models. Energies, 14(18), 5776. https://doi.org/10.3390/en14185776






