Second-Order Approximation of the Seismic Reflection Coefficient in Thin Interbeds
Abstract
:1. Introduction
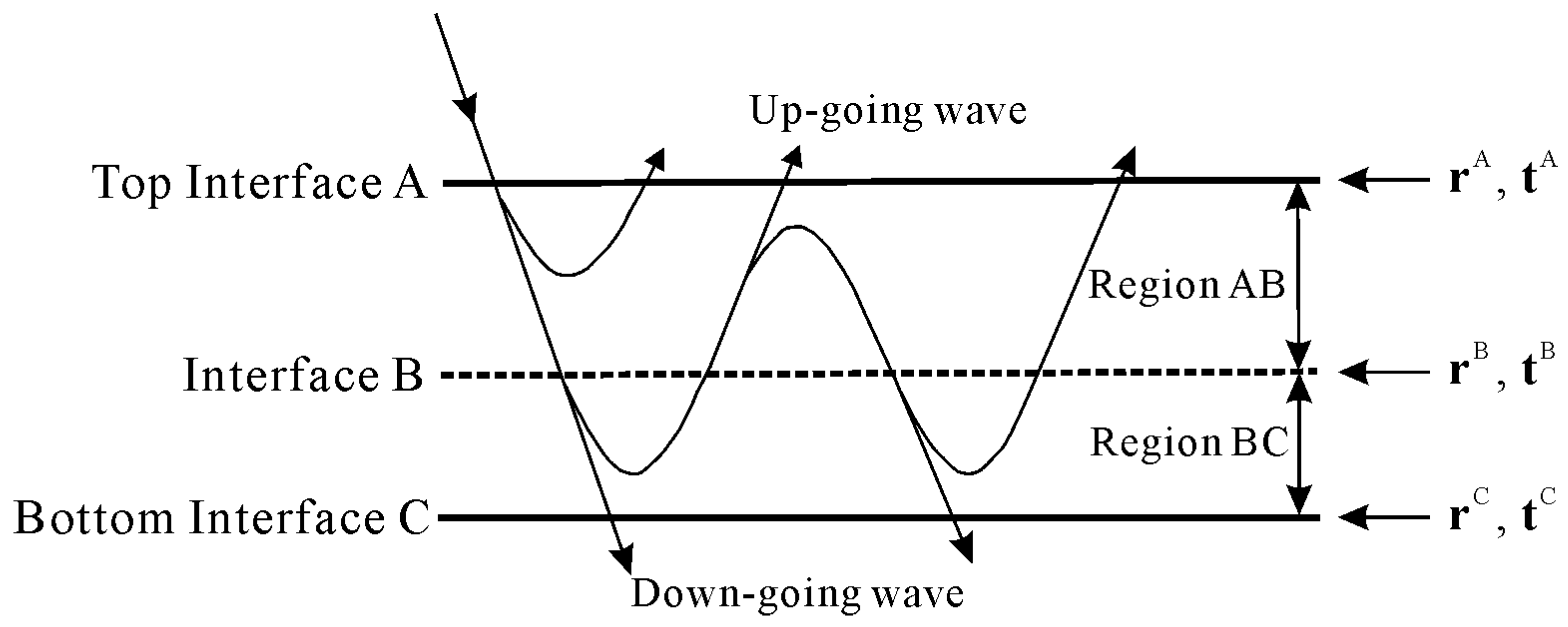
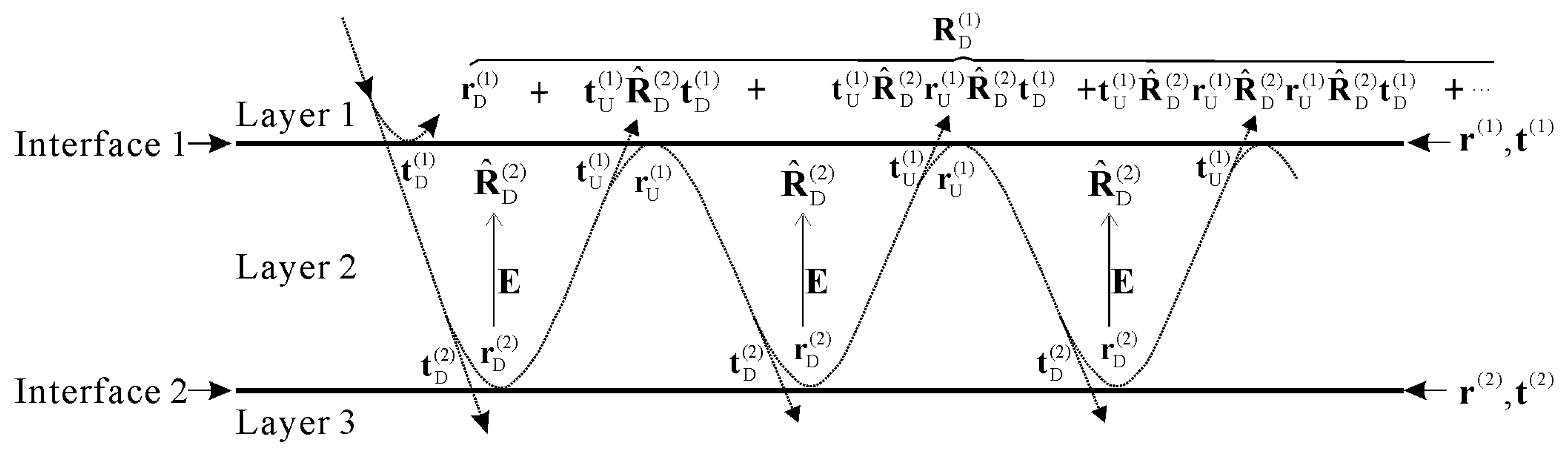
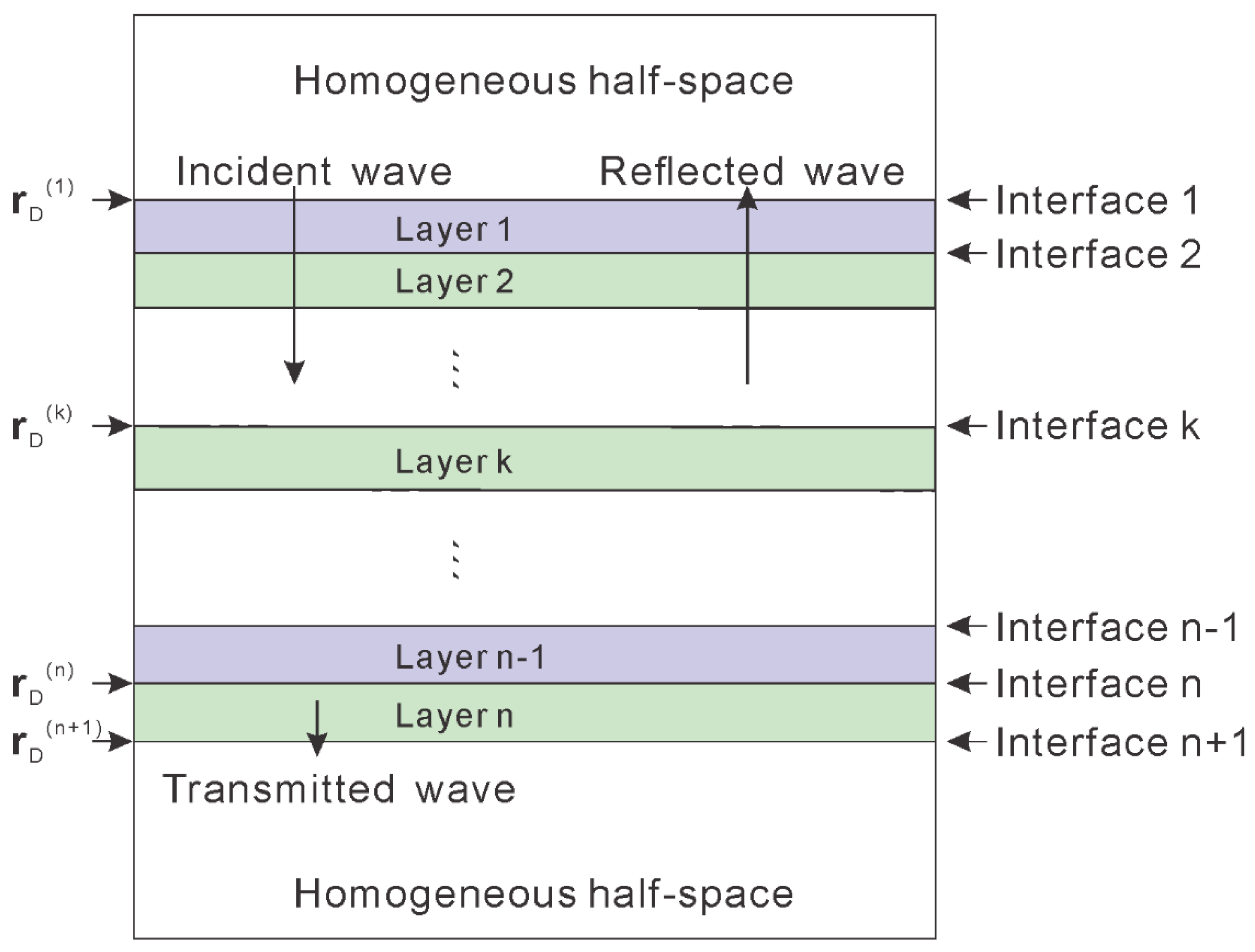
2. Exact Reflection Coefficient Equation in Stratified Media
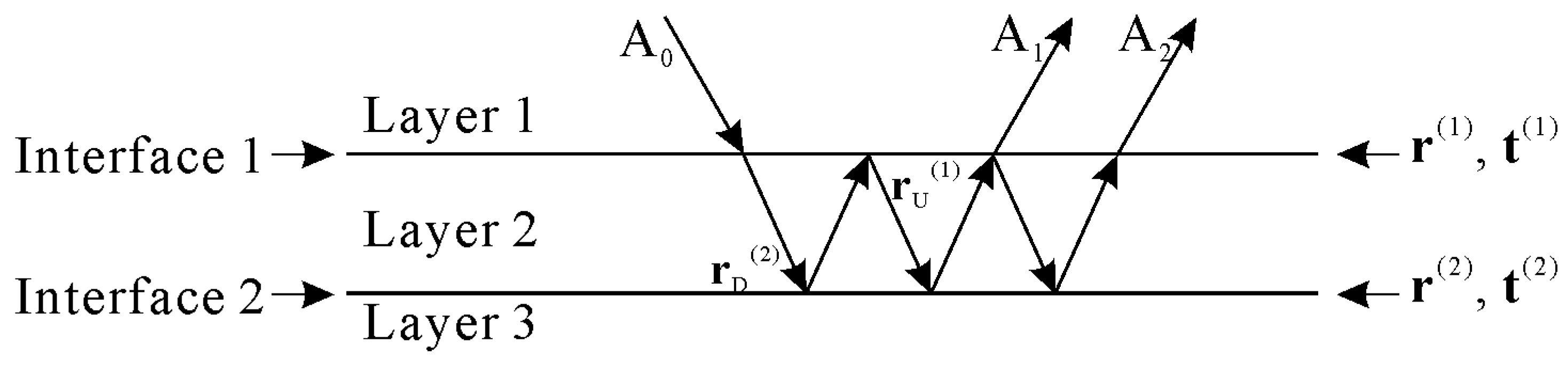
3. Second-Order Approximate Reflection of Single Thin-Bed Media
3.1. Assumption of Small to Medium Impedance Contrast
- (1)
- : the reflection coefficient at the top interface (Interface 1) of the thin bed.
- (2)
- : the primary reflection coefficient at the bottom interface (Interface 2) of the thin bed, in which the down-going and up-going wave transmission losses ( and ) at Interface 1 are considered;
- (3)
- : the first-order multiple reflection coefficient of the thin-bed. An additional interaction in the thin-bed is introduced based on the primary reflection.
- (4)
- : the second-order multiple reflection coefficient of the thin-bed. An additional interaction in the thin-bed is added based on the first-order multiple reflections.
- (1)
- Slowness to incident angle
- (2)
- Frequency domain to time domain
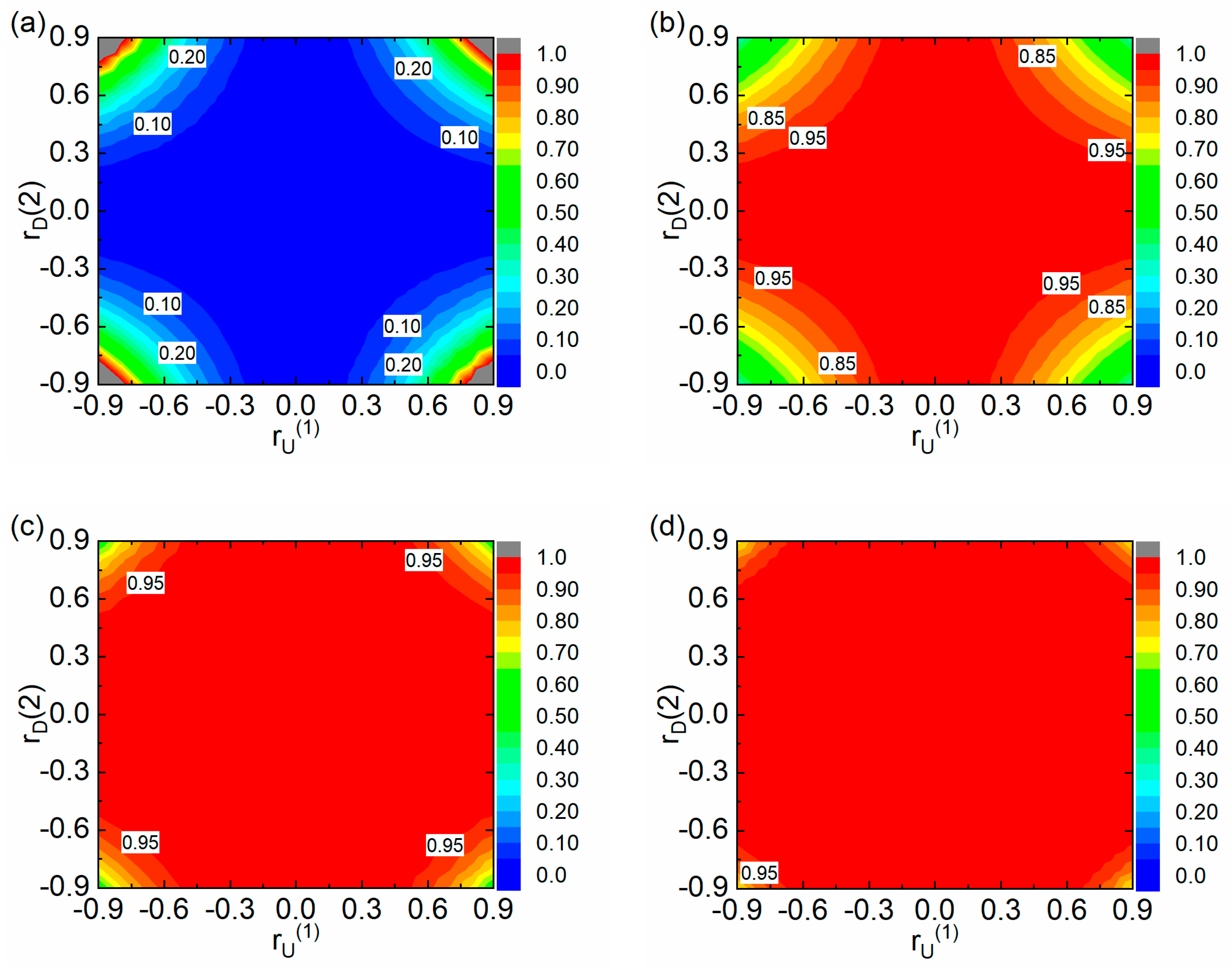
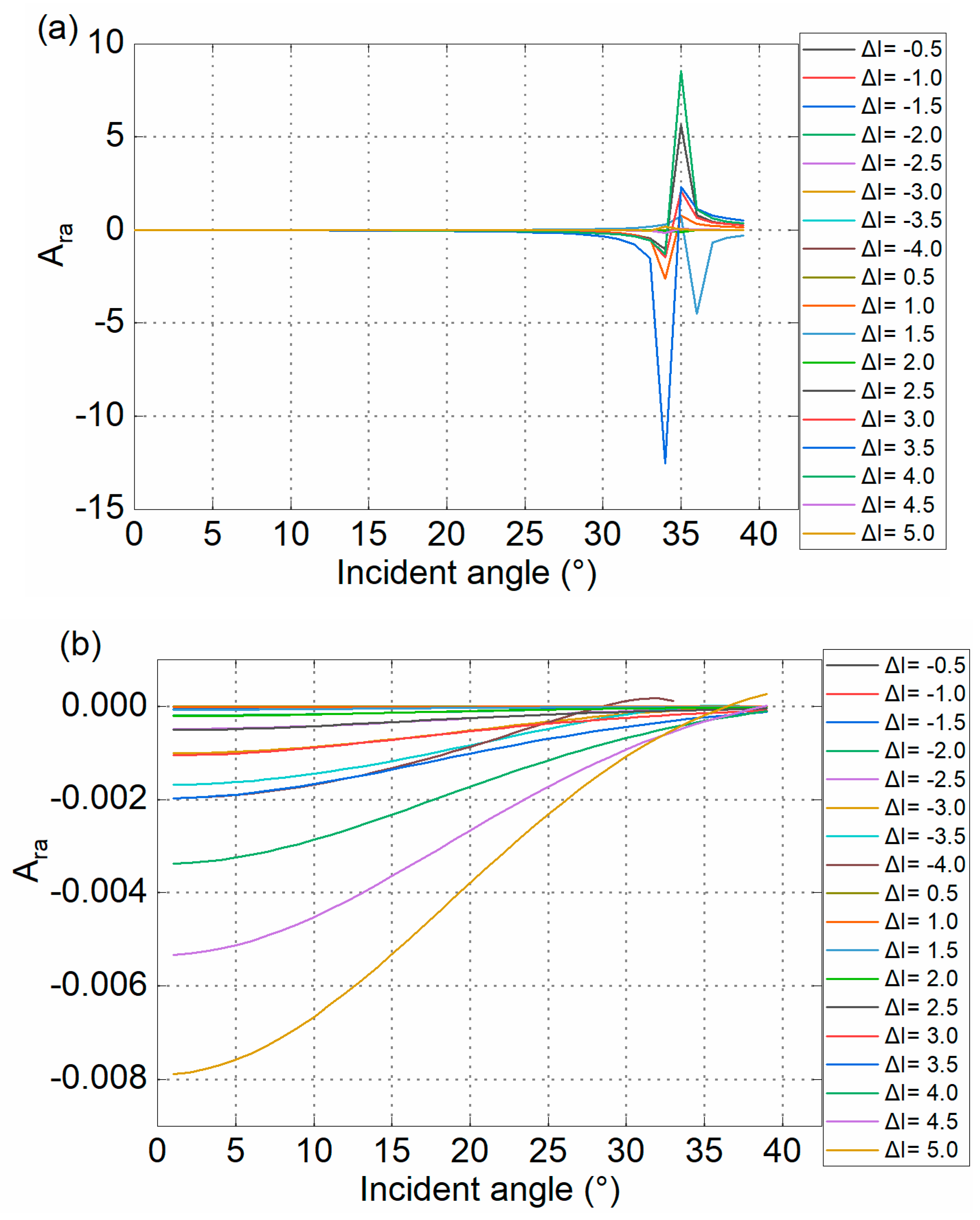
3.2. Accuracy Analysis of Second-Order Approximation
- (1)
- With the increase of impedance contrasts, Ara also tends to increase.
- (2)
- If the incident angle exceeds 30°, the error will rapidly increase when using the second-order approximation equation to calculate the PP-wave reflection coefficient. For the PP-wave, initially, Ara is small and increases very slowly with the increasing angle, but after the angle exceeds 30°, Ara increases rapidly.
- (3)
- The Ara of the PS-wave decreases as the incident angle increases, which shows that the second-order approximation equation is suitable for calculating PS reflections at large angles. However, if the PP- and PS-wave are jointly used for seismic inversion, the applicable angle range of the second-order approximate equation needs to take the intersection of PP- and PS-waves.
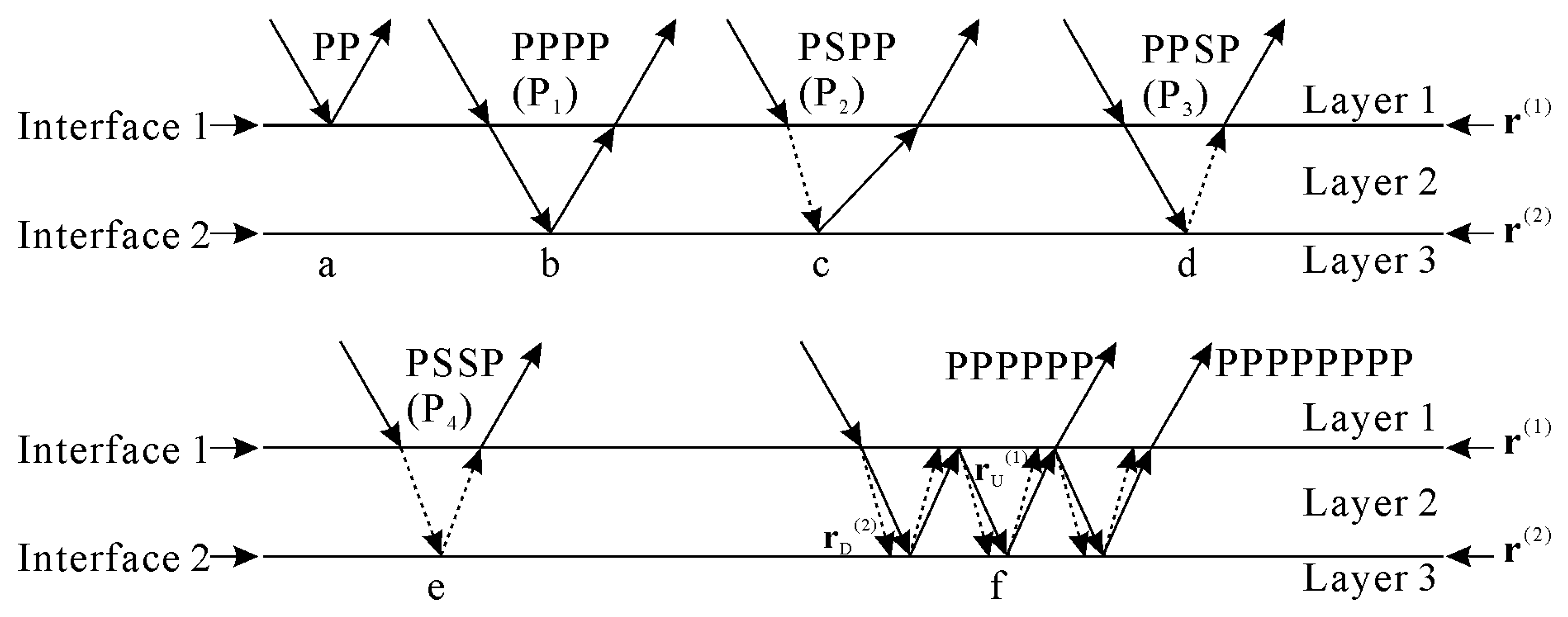
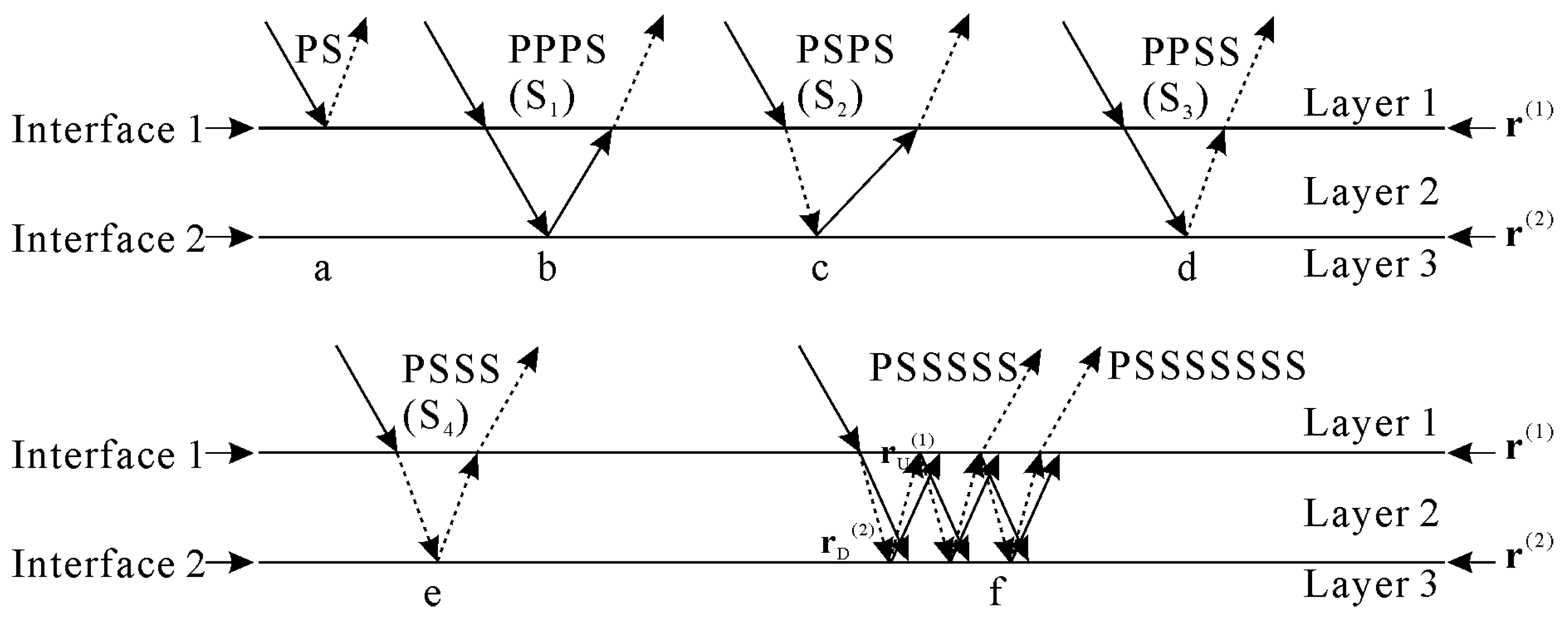
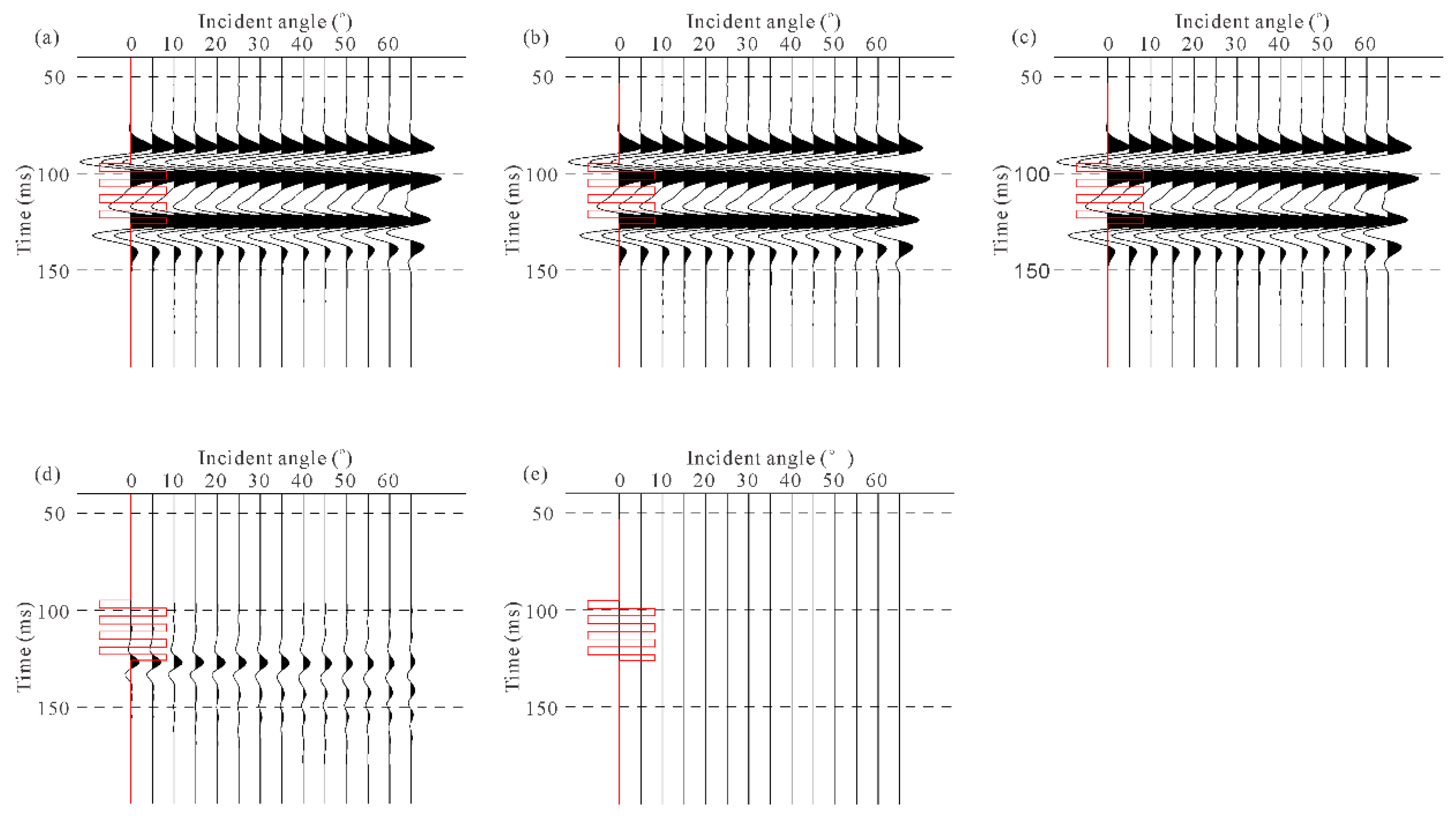
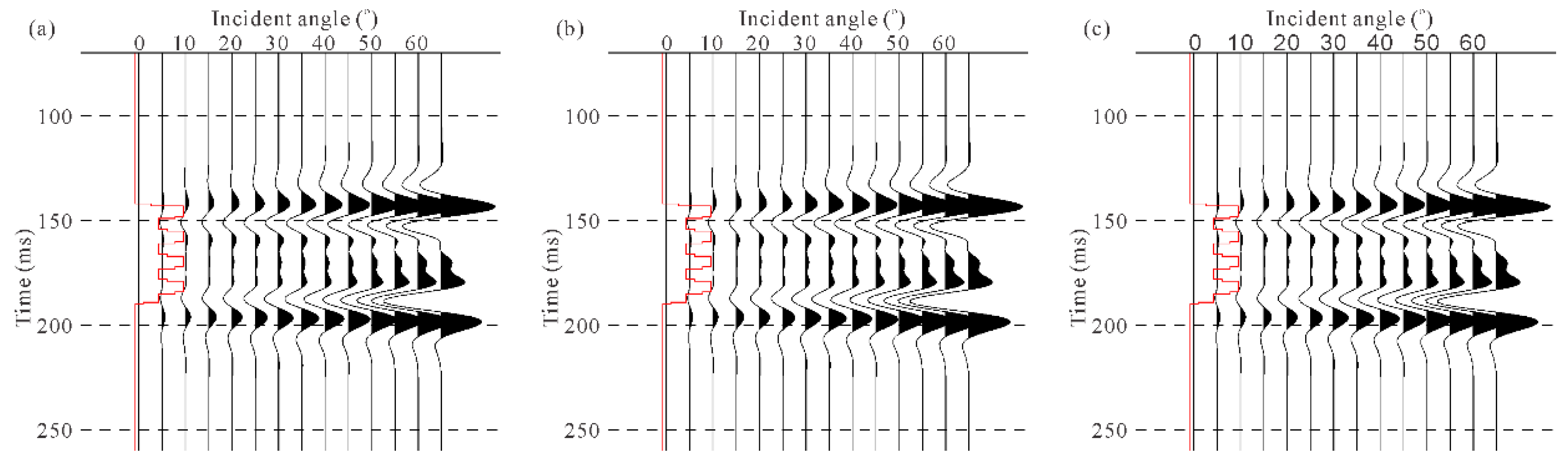
4. Second-Order Approximate Reflection of Thin-Interbed Media
4.1. Assumption of Small to Medium Impedance Contrast
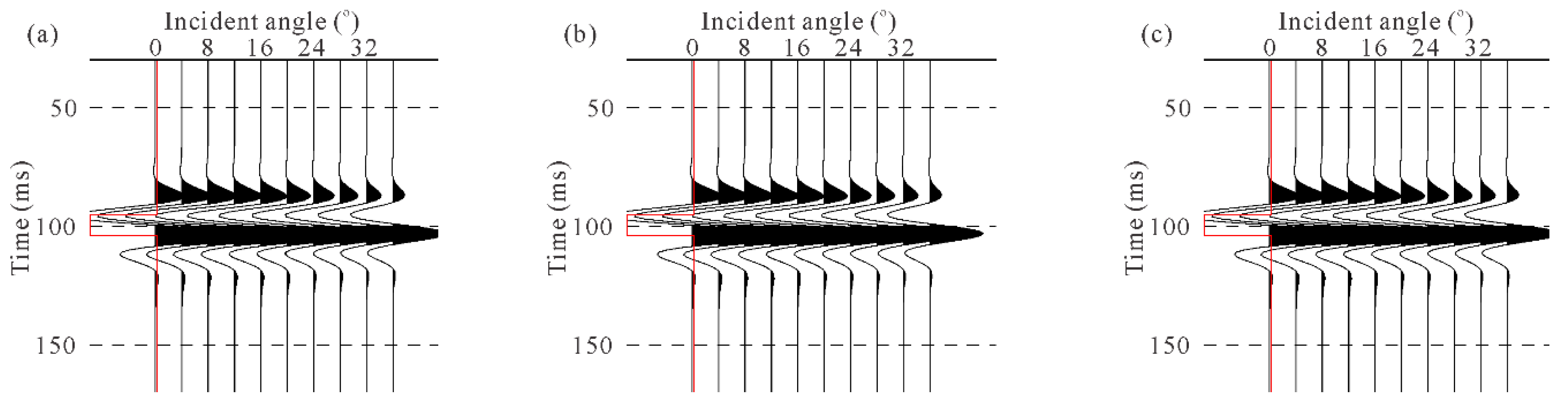
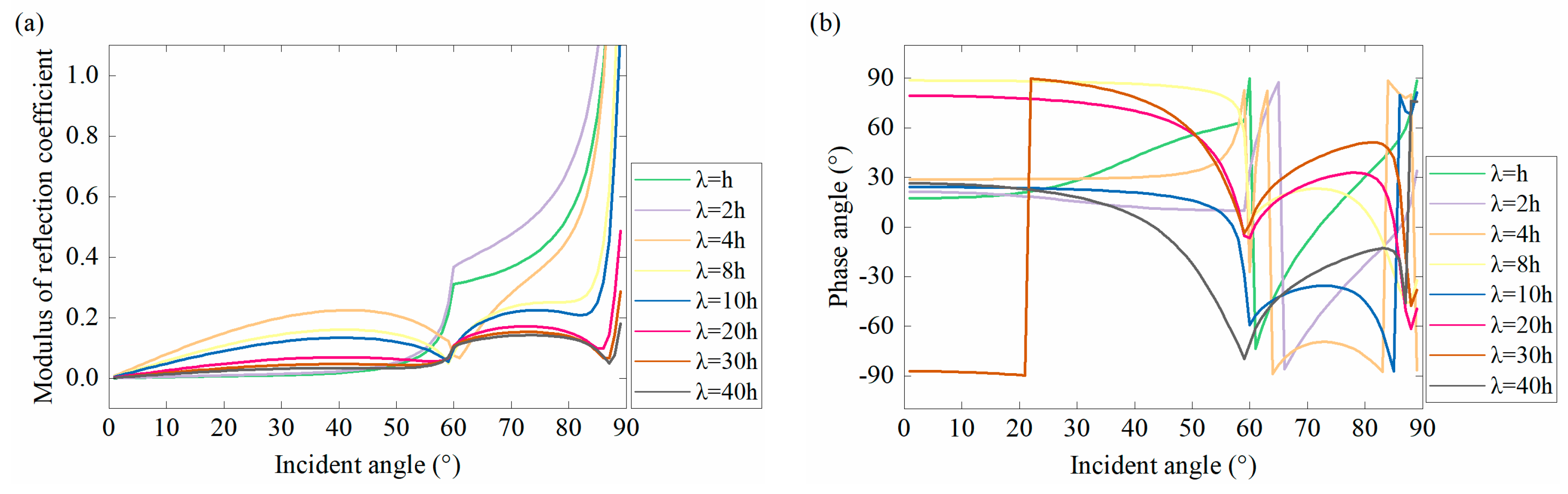
4.2. Accuracy Analysis of Second-Order Approximation
- (1)
- (2)
- The second-order approximation equation effectively calculates the internal multiples (Figure 13d and Figure 14d), and these multiples are aliased together with the primary wave. It is difficult for existing technology to eliminate these internal multiples without affecting the energy of primary reflections.
- (3)
- As can be seen from Figure 13d and Figure 14d, for PP-waves, the internal multiples have a more significant effect on the reflection coefficient at small to medium angles, and the effect decreases as the incident angle increases. For PS-waves, the internal multiples have a large effect on the reflection coefficient at medium to large angles, and the effect becomes more significant as the incident angle increases.
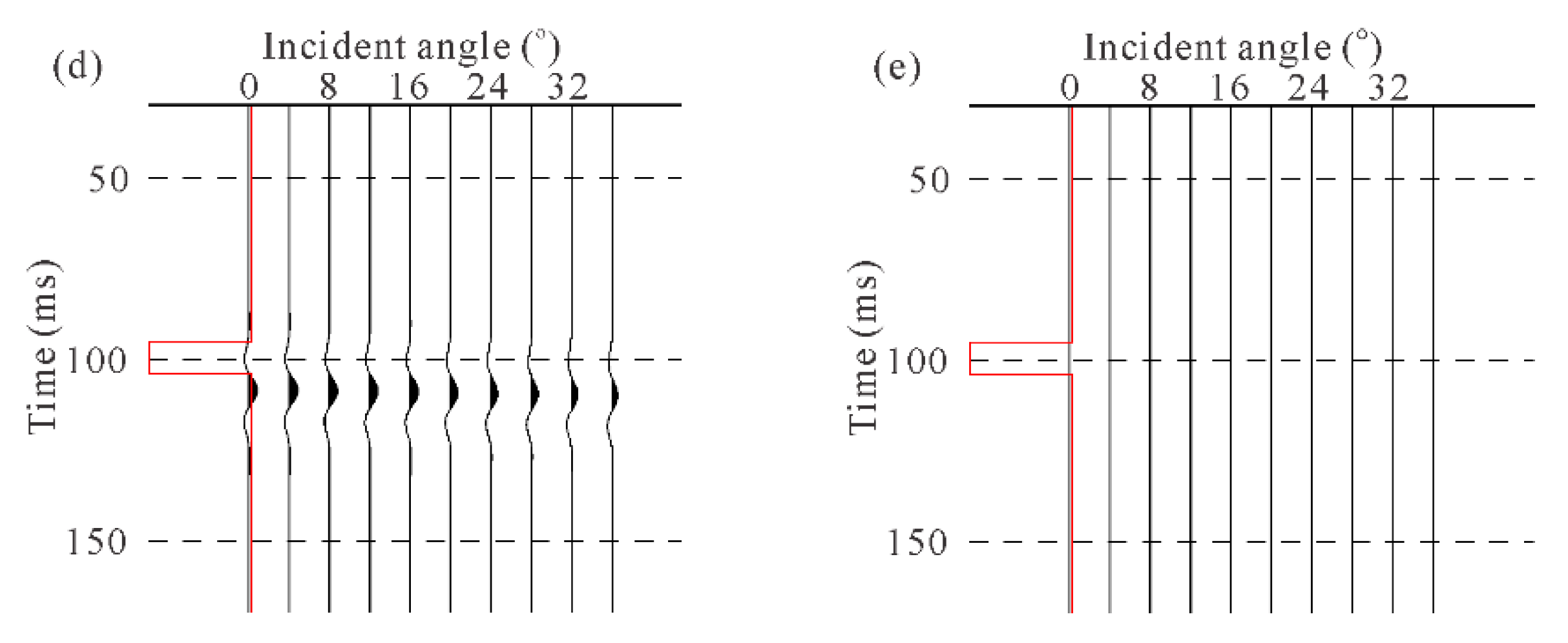
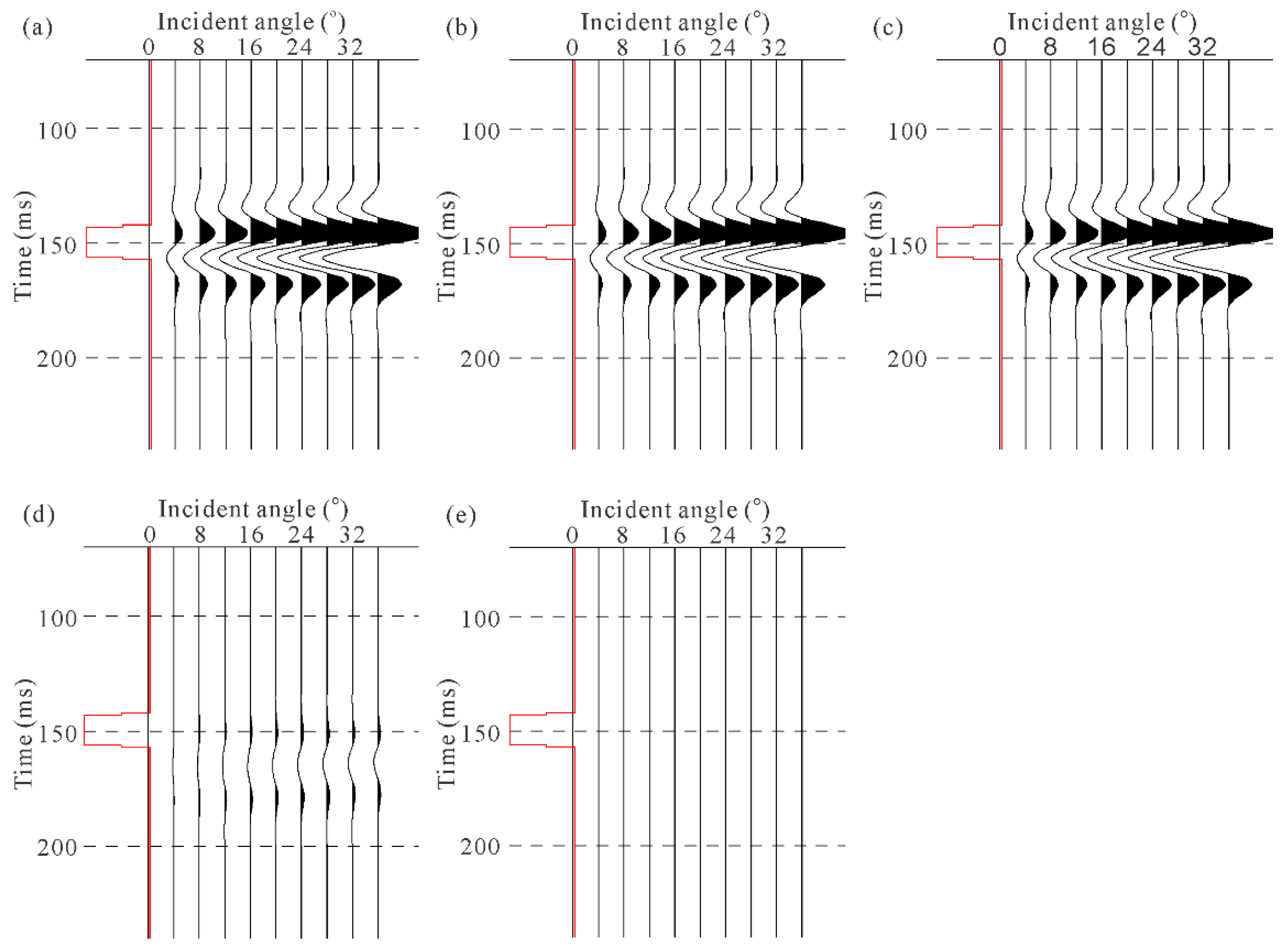
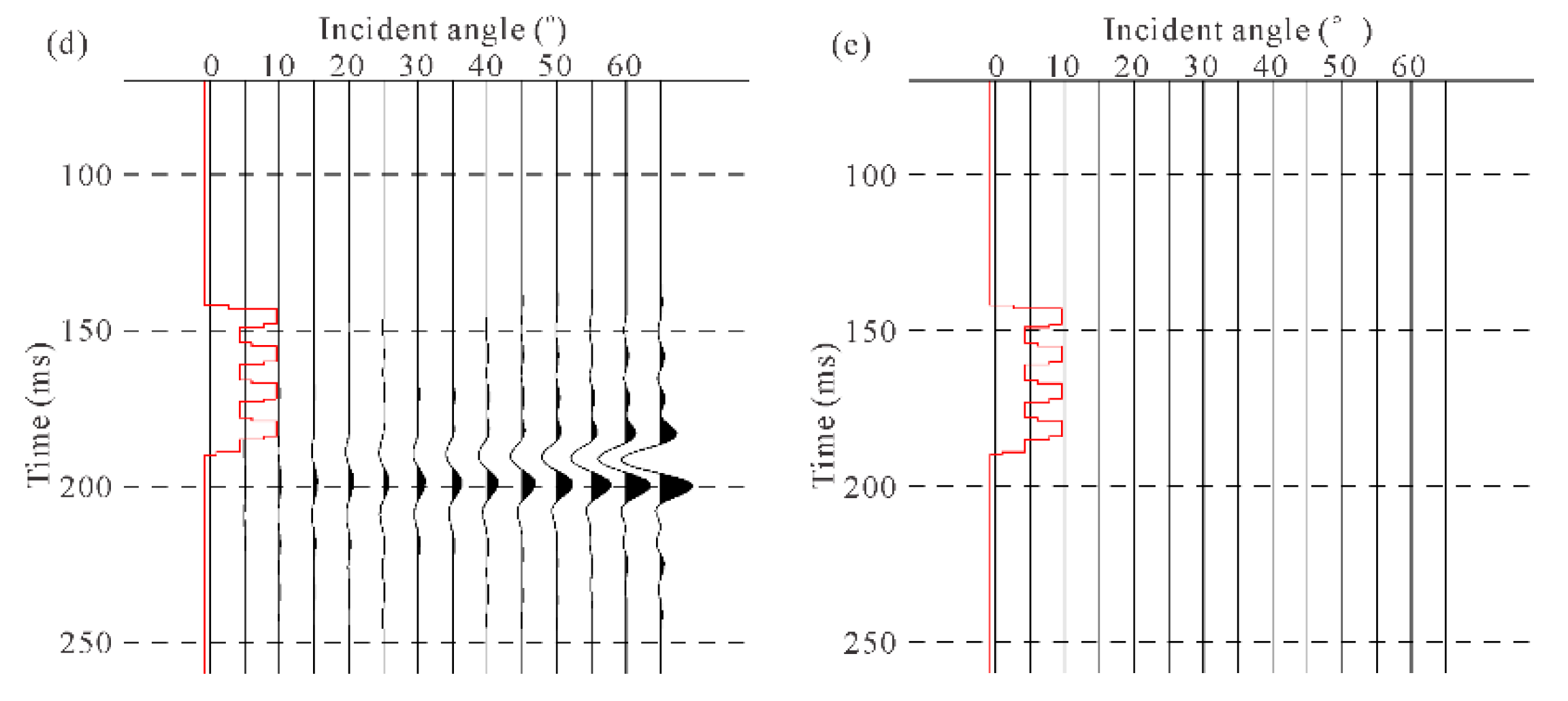
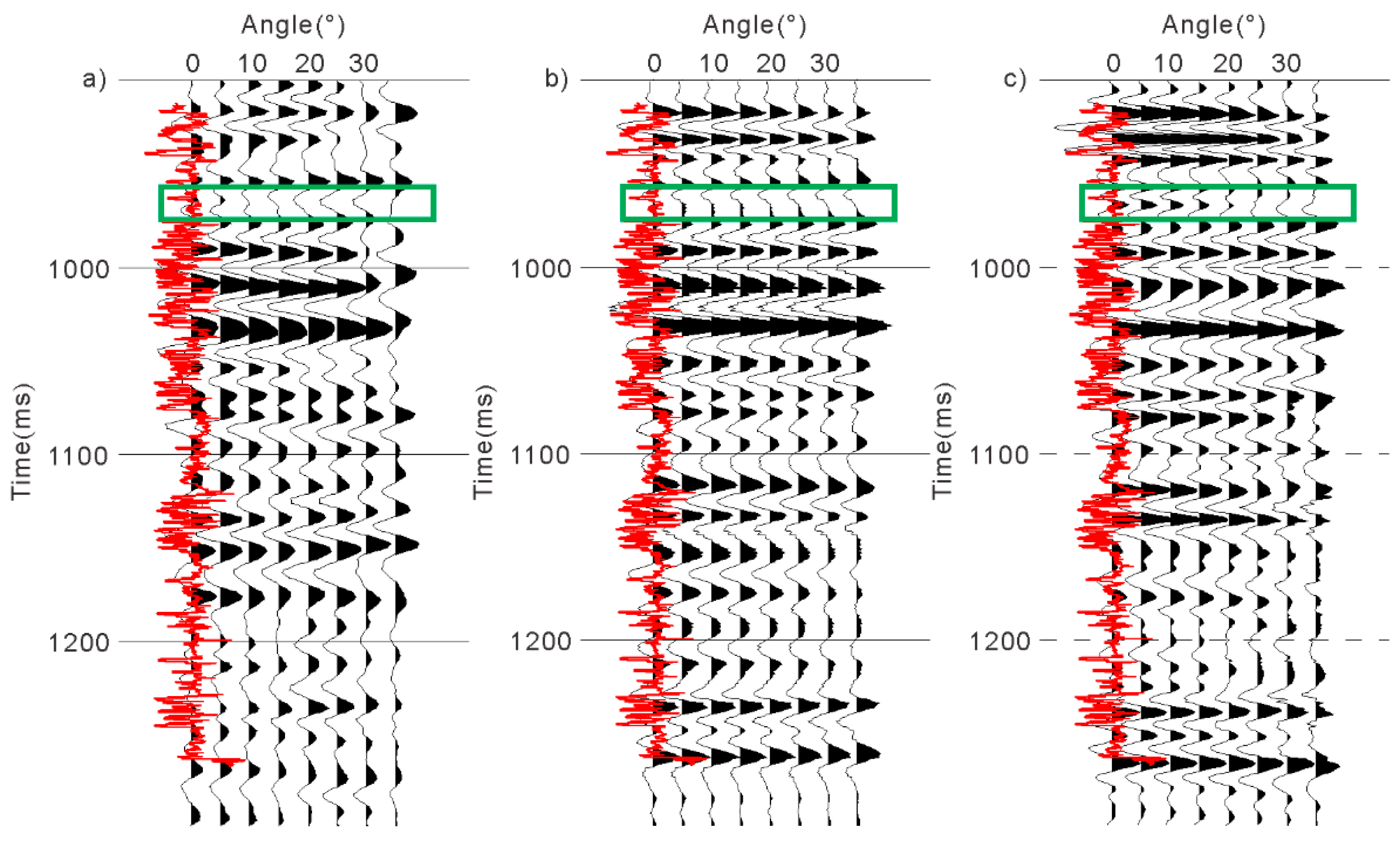
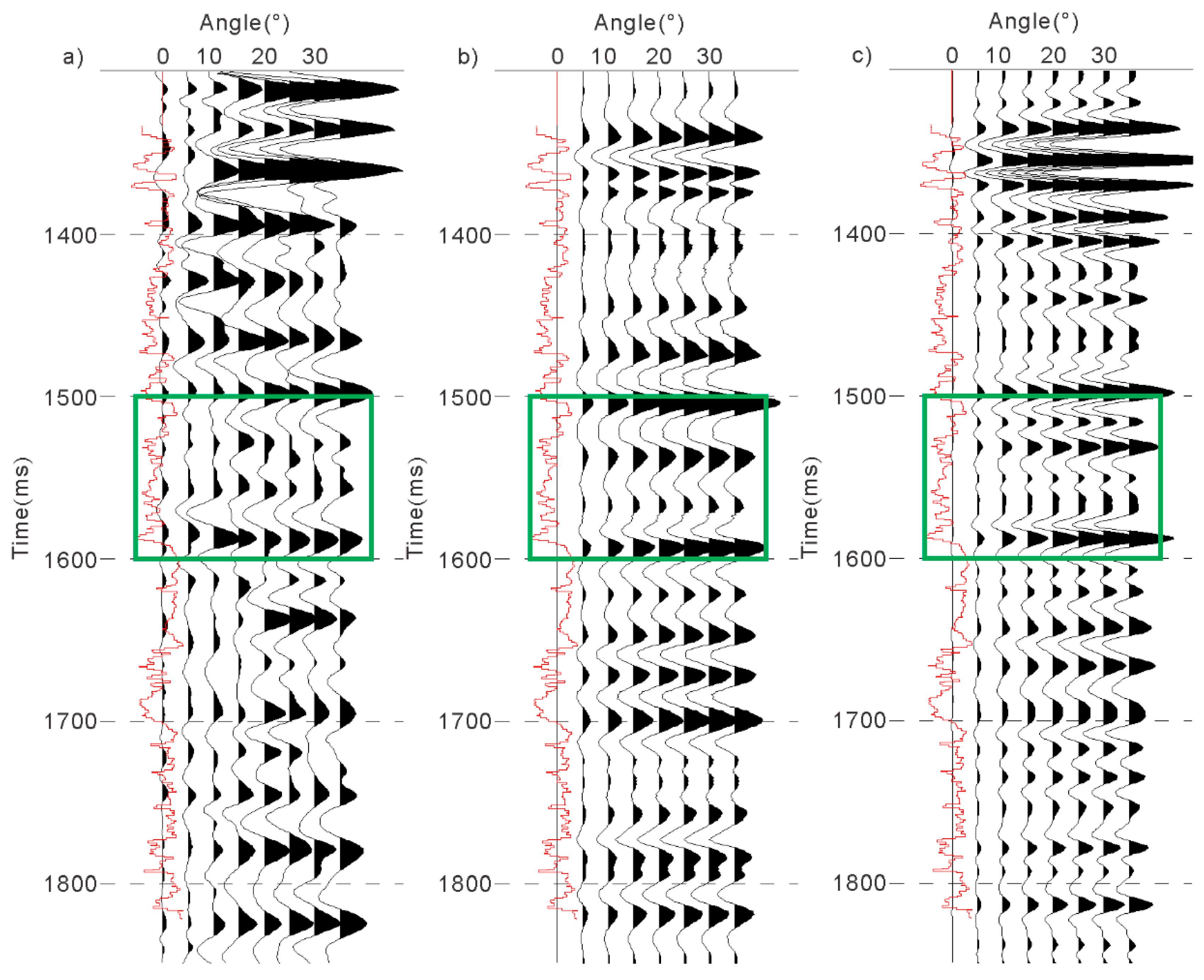
4.3. Comparison With Field Multicomponent Data
- (1)
- The simulated angle gathers based on the second-order approximate equation will be more similar to the field data. For example, the field angle gather in the green box in Figure 15a has a phase inversion phenomenon, but this phenomenon only appears in the simulated angle gather based on the second-order approximate equation.
- (2)
- For thin interbeds, the simulation results of the second-order approximate equation contain richer information. As shown in Figure 16, the strata in the green box is thin interbeds. Moreover, the simulation results based on the second-order approximate equation contain internal multiples and are more similar to the field data.
5. Discussion
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Ostrander, W.J. Plane-wave reflection coefficients for gas sands at nonnormal angles of incidence. SEG Tech. Progr. Expand. Abstr. 1982, 216–218. [Google Scholar] [CrossRef]
- Ostrander, W.J. Plane-wave reflection coefficients for gas sands at non-normal angles of incidence. Geophysics 1984, 49, 1637–1648. [Google Scholar] [CrossRef]
- Hilterman, F.J. Seismic Amplitude Interpretation; SEG and EAGE: Houton, TX, USA, 2001; ISBN 1-56080-109-3. [Google Scholar]
- Lu, J.; Meng, X.; Wang, Y.; Yang, Z. Prediction of coal seam details and mining safety using multicomponent seismic data: A case history from China. Geophysics 2016, 81, B149–B165. [Google Scholar] [CrossRef]
- Xu, Z.; Tu, H.; Wu, Q. Reflection coefficient of planar wave and AVO technique. Geophys. Prospect. Pet. 1991, 30, 1–21. [Google Scholar]
- Pan, W.; Innanen, K.A. AVO/AVF analysis of thin-bed in elastic media. SEG Techn. Progr. Expand. Abstr. 2013, 373–377. [Google Scholar] [CrossRef]
- Yang, C.; Wang, Y.; Lu, J. Weak impedance difference approximations of thin-bed PP-wave reflection responses. J. Geophys. Eng. 2017, 14, 1010–1019. [Google Scholar] [CrossRef]
- Widess, M.B. How thin is a thin bed? Geophysics 1973, 38, 1176–1180. [Google Scholar] [CrossRef]
- Lu, J.M.; Wang, Y. The Principle of Seismic Exploration; China Petroleum University Press: Beijing, China, 2011; ISBN 978-7-5636-2822-3. [Google Scholar]
- Brekhovoskikh, L.M. Waves in Layered Media; Academic Process: Tamil Nadu, India, 1960. [Google Scholar]
- Brekhovskikh, L.M. Waves in Layered Media, 2nd ed.; Academic Press: New York, NY, USA, 1980; ISBN 0-12-130560-0. [Google Scholar]
- Kennett, B.L.N. Reflections, rays, and reverberations. Bull. Seismol. Soc. Am. 1974, 64, 1685–1696. [Google Scholar]
- Kennett, B.L.N. Seismic Wave Propagation in Stratified Media; Cambridge University Press: Cambridge, UK, 1983. [Google Scholar]
- Kennett, B.L.N. Seismic Wave Propagation in Stratified Media, 2nd ed.; ANUE Press: Canberra, Australia, 2009. [Google Scholar] [CrossRef]
- Liu, Y.; Schmitt, D.R. Amplitude and AVO responses of a single thin bed. Geophysics 2003, 68, 1161–1168. [Google Scholar] [CrossRef]
- Yang, C.; Wang, Y.; Wang, Y.H. Reflection and transmission coefficients of a thin bed. Geophysics 2016, 81, N31–N39. [Google Scholar] [CrossRef] [Green Version]
- Yang, C.; Wang, Y.; Lu, J.; Chen, B.C.; Shi, L. A Low-Order Series Approximation of Thin-Bed PP-Wave Reflections. Appl. Sci. 2019, 9, 709. [Google Scholar] [CrossRef] [Green Version]
- Lu, J.; Wang, Y.; Chen, J.; An, Y. Joint anisotropic amplitude variation with offset inversion of PP and PS seismic data. Geophysics 2018, 83, N31–N50. [Google Scholar] [CrossRef]
- Aki, K.; Richards, P.G. Quantitative Seismology, 2nd ed.; Integrated Book technology: Mill Valley, CA, USA, 2009; ISBN 978-1-891389-63-4. [Google Scholar]
- Mavko, G.; Mukerji, T.; Dvorkin, J. The Rock Physics Handbook: Tools for Seismic Analysis of Porous Media; Cambridge University Press: Cambridge, UK, 2009; ISBN -13. [Google Scholar]
- Fryer, G.J. A slowness approach to the reflectivity method of seismogram synthesis. Geophys. J. Int. 1980, 63, 747–758. [Google Scholar] [CrossRef] [Green Version]
- Castagna, J.P.; Batzle, M.L.; Kan, T.K. Rock physics—The link between rock properties and AVO response. In Offset-Dependent Reflectivity—Theory and Practice of AVO Analysis; Castagna, J.P., Backus, M., Eds.; Investigations in Geophysics, Society of Exploration Geophysicists: Tulsa, OK, USA, 1993; pp. 135–171. [Google Scholar]
- Gardner, G.H.F.; Gardner, L.W.; Gregory, A.R. Formation velocity and density—The diagnostic basics for stratigraphic traps. Geophysics 1974, 39, 770–780. [Google Scholar] [CrossRef] [Green Version]
- Lu, J.; Yang, Z.; Wang, Y.; Shi, Y. Joint PP and PS AVA seismic inversion using exact Zoeppritz equations. Geophysics 2015, 80, R239–R250. [Google Scholar] [CrossRef] [Green Version]


| ΔI (km/s∙g/cm3) | α2 (km/s) | β2 (km/s) | ρ2 (g/cm3) |
|---|---|---|---|
| 5 | 4.817 | 2.981 | 2.579 |
| 4.5 | 4.662 | 2.846 | 2.558 |
| 4 | 4.505 | 2.711 | 2.536 |
| 3.5 | 4.346 | 2.574 | 2.514 |
| 3 | 4.186 | 2.437 | 2.490 |
| 2.5 | 4.025 | 2.298 | 2.466 |
| 2 | 3.862 | 2.157 | 2.441 |
| 1.5 | 3.697 | 2.015 | 2.414 |
| 1 | 3.531 | 1.871 | 2.386 |
| 0.5 | 3.362 | 1.726 | 2.357 |
| −0.5 | 3.018 | 1.430 | 2.295 |
| −1 | 2.842 | 1.371 | 2.261 |
| −1.5 | 2.664 | 1.286 | 2.224 |
| −2 | 2.483 | 1.198 | 2.185 |
| −2.5 | 2.298 | 1.109 | 2.144 |
| −3 | 2.109 | 1.019 | 2.098 |
| −3.5 | 1.916 | 0.926 | 2.048 |
| −4 | 1.719 | 0.831 | 1.993 |
| α (km/s) | β (km/s) | ρ (g/cm3) | h (m) |
|---|---|---|---|
| 3.094 | 1.515 | 2.400 | 150 |
| 3.048 | 1.595 | 2.230 | 6 |
| 3.146 | 1.554 | 2.410 | 6 |
| 3.048 | 1.595 | 2.230 | 6 |
| 3.146 | 1.554 | 2.410 | 6 |
| 3.048 | 1.595 | 2.230 | 6 |
| 3.146 | 1.554 | 2.410 | 6 |
| 3.048 | 1.595 | 2.230 | 6 |
| 3.146 | 1.554 | 2.410 | 6 |
| 3.094 | 1.515 | 2.400 | 150 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, Z.; Lu, J. Second-Order Approximation of the Seismic Reflection Coefficient in Thin Interbeds. Energies 2020, 13, 1465. https://doi.org/10.3390/en13061465
Yang Z, Lu J. Second-Order Approximation of the Seismic Reflection Coefficient in Thin Interbeds. Energies. 2020; 13(6):1465. https://doi.org/10.3390/en13061465
Chicago/Turabian StyleYang, Zhen, and Jun Lu. 2020. "Second-Order Approximation of the Seismic Reflection Coefficient in Thin Interbeds" Energies 13, no. 6: 1465. https://doi.org/10.3390/en13061465
APA StyleYang, Z., & Lu, J. (2020). Second-Order Approximation of the Seismic Reflection Coefficient in Thin Interbeds. Energies, 13(6), 1465. https://doi.org/10.3390/en13061465





