1. Introduction
Numerical models based on the use of the finite element method have become an indispensable tool in the design of electrical machines. As is well known, the most accurate approach for overall consideration of physical phenomena in an analyzed converter is the use of time-domain models. Unfortunately, this approach usually involves significant computational cost, sometimes making its practical application impossible. For that reason, it is justified to explore methods which allow, particularly in the analysis of steady states, to achieve similar results, but in a much shorter time. While analyzing induction machines, one of such methods is the use of the multi-harmonic field-circuit model [
1,
2,
3,
4,
5,
6,
7,
8,
9]. The results presented in [
1,
2,
9] prove that this type of the field-circuit model can be particularly useful when analyzing steady states of the special constructions of induction machines (single-phase machines, high-speed solid-rotor machines). As demonstrated by Garbiec et al., with an appropriate modification of the coupling scheme between individual sub-models, the multi-harmonic field-circuit model makes it possible to achieve comparable calculation results with those obtained using the time-domain model, at a calculation cost comparable to the monoharmonic model. The multi-harmonic model in this case is understood as the De-Gersem type model formulated using the magnetic vector potential, where permeance slot harmonics of the magnetic field distribution in the air gap are extracted via Fourier transform of an air-gap magnetic field. These harmonics can affect the power losses in the rotor and the electromagnetic torque developed by the machine. The magnetic field in the stator in these models is considered as a function of the fundamental time harmonic only.
The application of the above-mentioned approach essentially involves the problem of modeling the nonlinearity of the magnetic materials. Taking this into account in the monoharmonic field models based on the use of the complex magnetic vector potential method is not a new task and it has been described to date in several works, including [
10,
11,
12,
13,
14,
15,
16]. They propose several different methods of defining the so-called effective magnetic permeability based, among others, on the development of nonlinear waveforms in the Fourier series [
14,
16], averaging the magnetic reluctivity [
10,
15,
16] or equivalence of energy stored in ferromagnetic components [
10,
11,
12,
13]. However, analyzing the conclusions drawn in the mentioned works, it is not possible to clearly state which method of defining the effective magnetic permeability will be most appropriate when using the multi-harmonic model with a strong coupling, as proposed by Garbiec et al. Some of them assume the sinusoidal variation of the magnetic flux density in calculating the effective magnetic permeability (which facilitates the calculations using the magnetic vector potential) [
12,
13,
14,
15], while others assume that it should be determined assuming sinusoidal variation of the magnetic field strength, or that it is insignificant for the accuracy of the calculations [
10,
11,
14]. The latter approach was adopted in the authors’ previous work, and satisfactory results were achieved [
9]. It was based on the results of the earlier work of the authors, where the effective magnetic permeability defined with the assumption of the sinusoidal variation of the magnetic field strength provided correct results, modeling a solid-rotor induction machine with the use of the multi-harmonic field-circuit model with a weak coupling [
17]. To the best of authors’ knowledge, the research providing an analysis of the accuracy of definitions of the effective magnetic permeability on the results of computations using multi-harmonic field-circuit models was not reported.
This paper is a continuation of works by Garbiec et al. that aim at an in-depth verification of the De-Gersem type multi-harmonic complex model of induction machines from the point of view of their application in designing and optimization of real machines. The authors focus on the analysis of the impact of formulating the effective magnetic permeability. For that purpose, the basic operating characteristics of an exemplary machine were calculated for seven different methods of formulating the effective magnetic permeability. The first six methods use existing definitions derived in literature. The available formulas use averaging of magnetic reluctivity in terms of mean or RMS value. Also, the Fourier series truncated to the fundamental component and assuming sinusoidal variation of the magnetic flux density or magnetic field strength is used [
14,
15,
16]. The seventh method considered in this paper is a new approach proposed by the authors that relies upon formulating the so-called multidimensional effective magnetic permeability. The results of calculations were compared with those obtained with the time-domain model and with the results of measurements performed on the physical model under one of the previous works where the efficient computational method for fast determination of the performance characteristics of solid-rotor induction machines was presented [
17].
3. Calculation Results
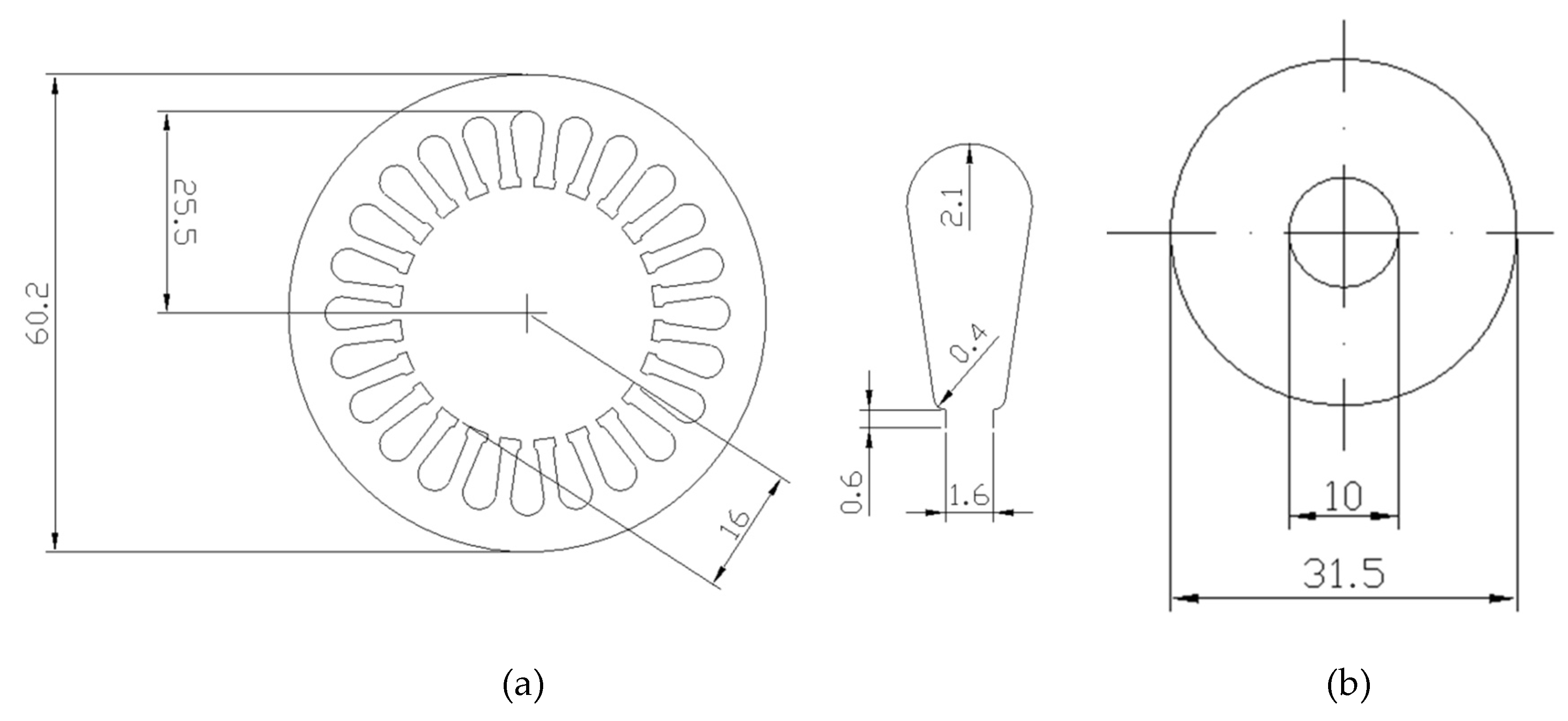
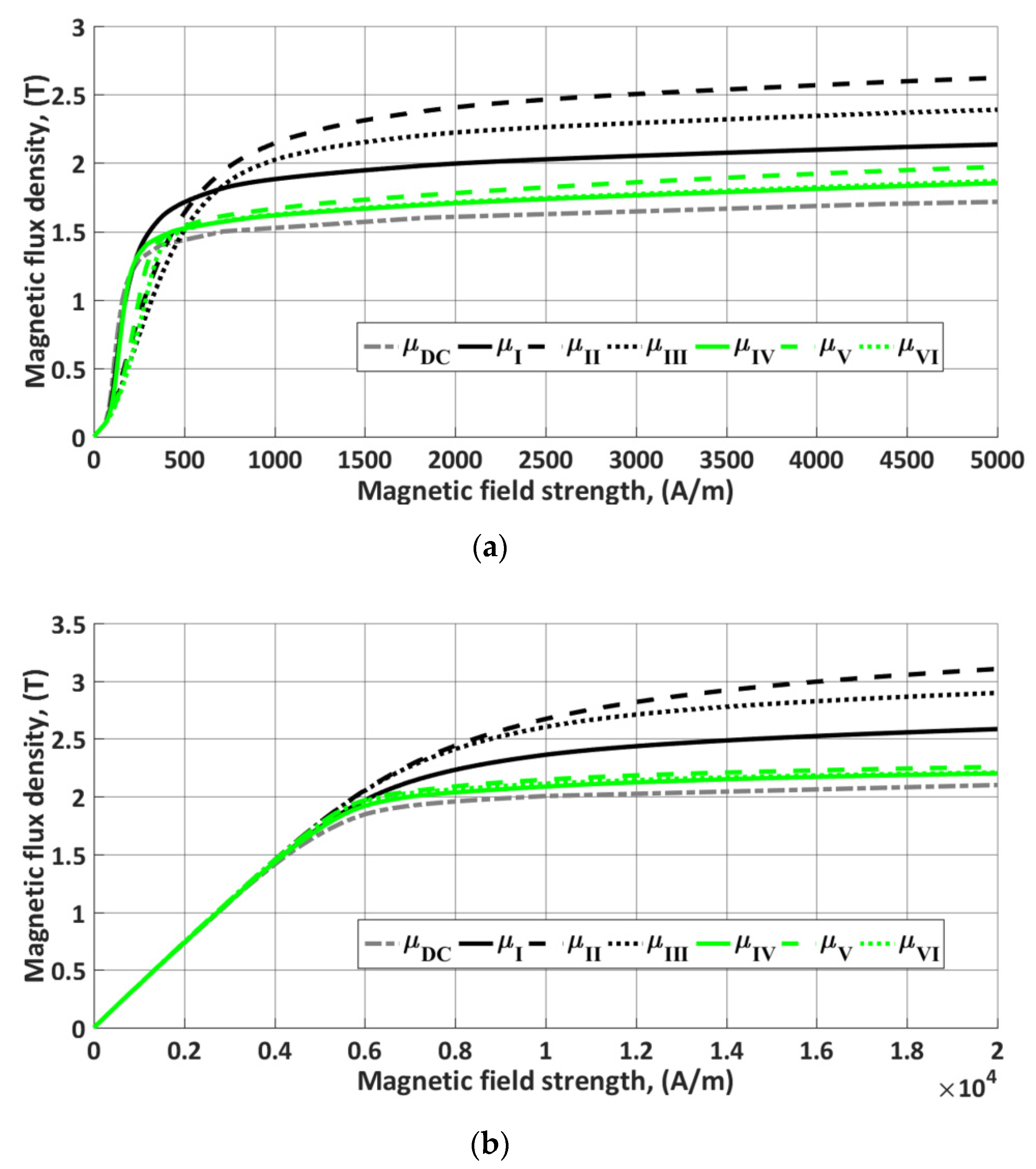
The magnetization curves shown in
Figure 2 were used to determine three basic operational characteristics of the machine (electromagnetic torque, RMS phase current, power losses in the rotor) vs. rotational speed at two different supply voltages (phase voltages), i.e., 50 V (rated) and 75 V (150% of the rated value) without changing the frequency of power supply. Note that the effective magnetic permeability for the rotor models is determined in each iteration based on the knowledge of the magnetic field strength distribution (expressions (1)–(3)) or magnetic flux density ((4)–(6)) only for the rotor model associated with the fundamental harmonic of the magnetic field distribution. The characteristics calculated were compared with the results of calculations using time-domain model, as described in
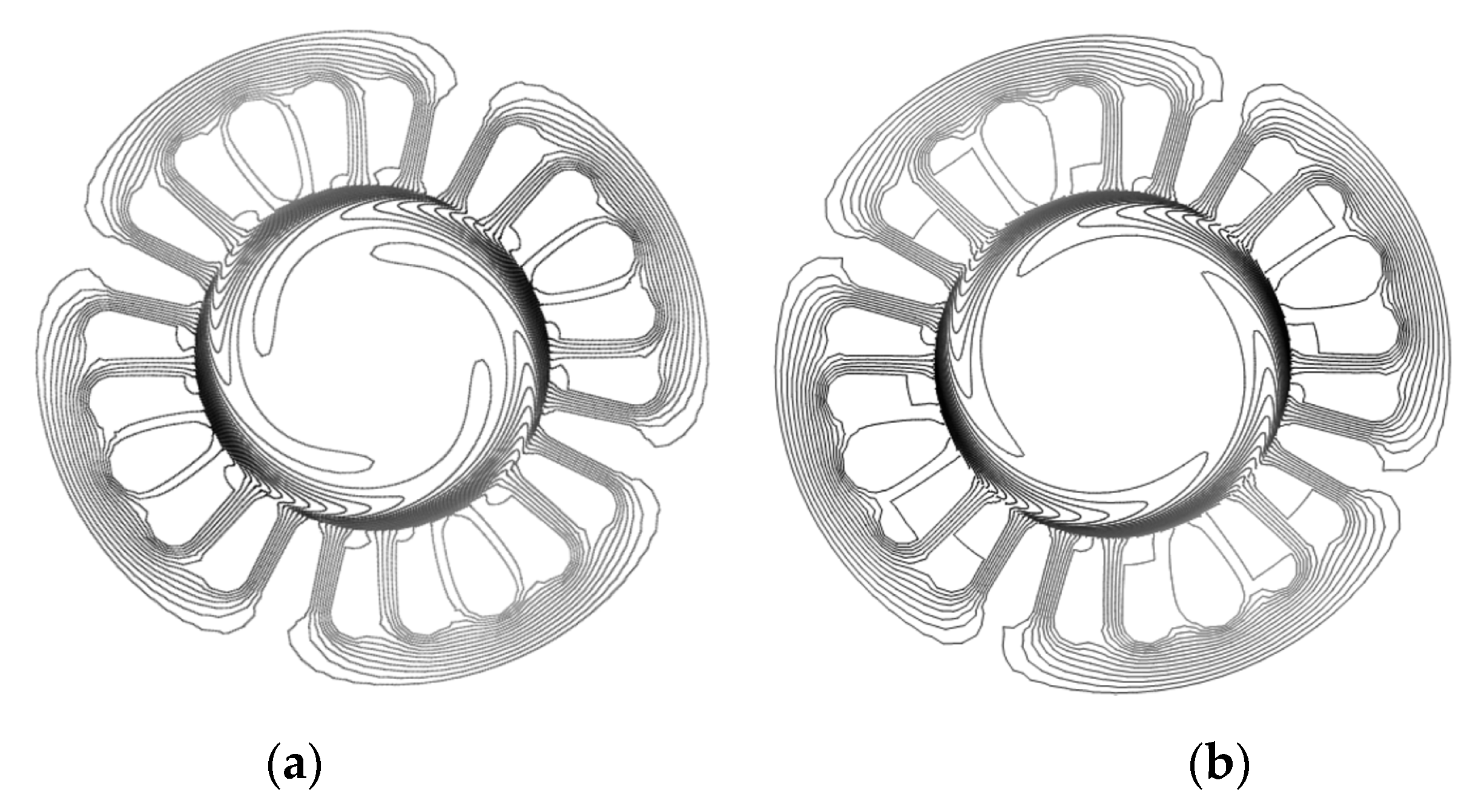
Section 2. It should be kept in mind that the considered multi-harmonic model, despite the associated mathematical burden, is a simplified approach. This simplification can be observed in e.g., the obtained steady-state distributions of the magnetic flux over the machine cross-section shown in (
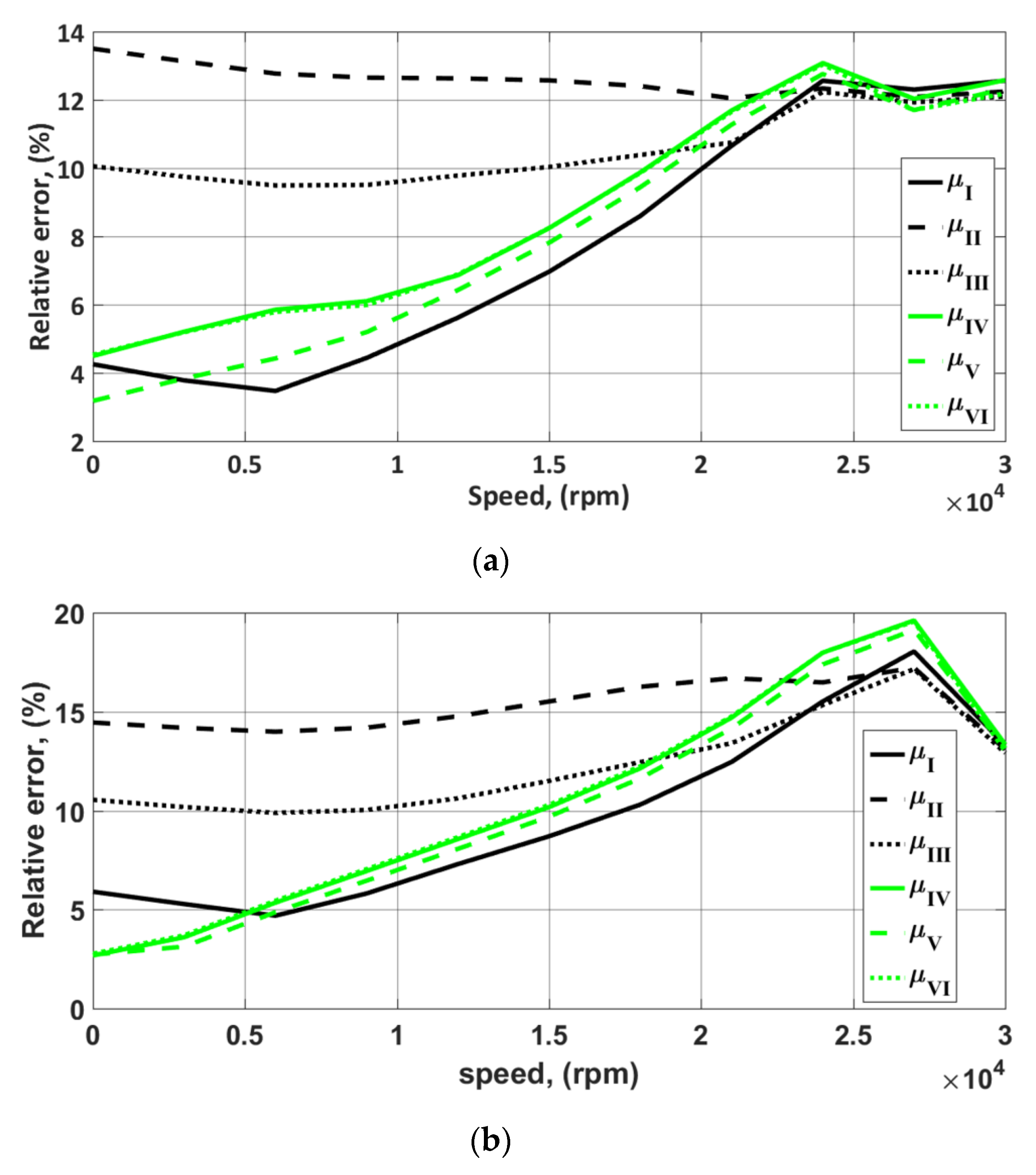
Figure 3). Based on the results of that comparison, two groups of characteristics were created (
Figure 4) showing the total relative percentage calculation error for the three determined quantities (electromagnetic torque, RMS phase current, power loss in the rotor) in relation to the calculation results obtained from the model (8):
where:
,
,
,
,
—respectively, electromagnetic torque, stator RMS phase current, power loss in the rotor calculated with the model formulated in the time domain,
—respectively, electromagnetic torque, stator RMS phase current, and power loss in the rotor calculated using the multi-harmonic model.
Table 2 shows the averaged values of that indicator against the entire speed range considered.
Out of the definitions of the effective magnetic permeability considered, noticeably the best results were achieved using μ
I (originally used in calculations). Slightly worse results (except for the locked rotor state) can be obtained with the definitions assuming the sinusoidal variation of the magnetic flux density. On the other hand, an interesting conclusion is that the method how the nonlinearities are taken into account has practically no impact on the results of calculations for near-synchronous rotational speeds. It is caused by a much lower saturation of the rotor surface, crucial from the point of view of the machine properties, and consequently use by the time-harmonic model (7) similar values of effective permeability (see
Figure 2). An increased error in the range of nominal operation point (21–27 krpm) is an adverse trend observed. However, note that the waveforms of the global error indicator shown in
Figure 4 provide information on the discrepancy in the results of calculations of power losses in the rotor (
). The results of those calculations are distinguished by the greatest discrepancy (for
μI and
N = 11 considered points of the curve
= 15.42% at 50 V and 19.32% at 75 V), thus overestimate the global error indicator. In the rotational speed range from 21,000 rpm to 27,000 rpm, the average electromagnetic torque error and the RMS current error is 7.56% and 1.43% at 50 V, 10.93% and 1.67% at 75 V, respectively.
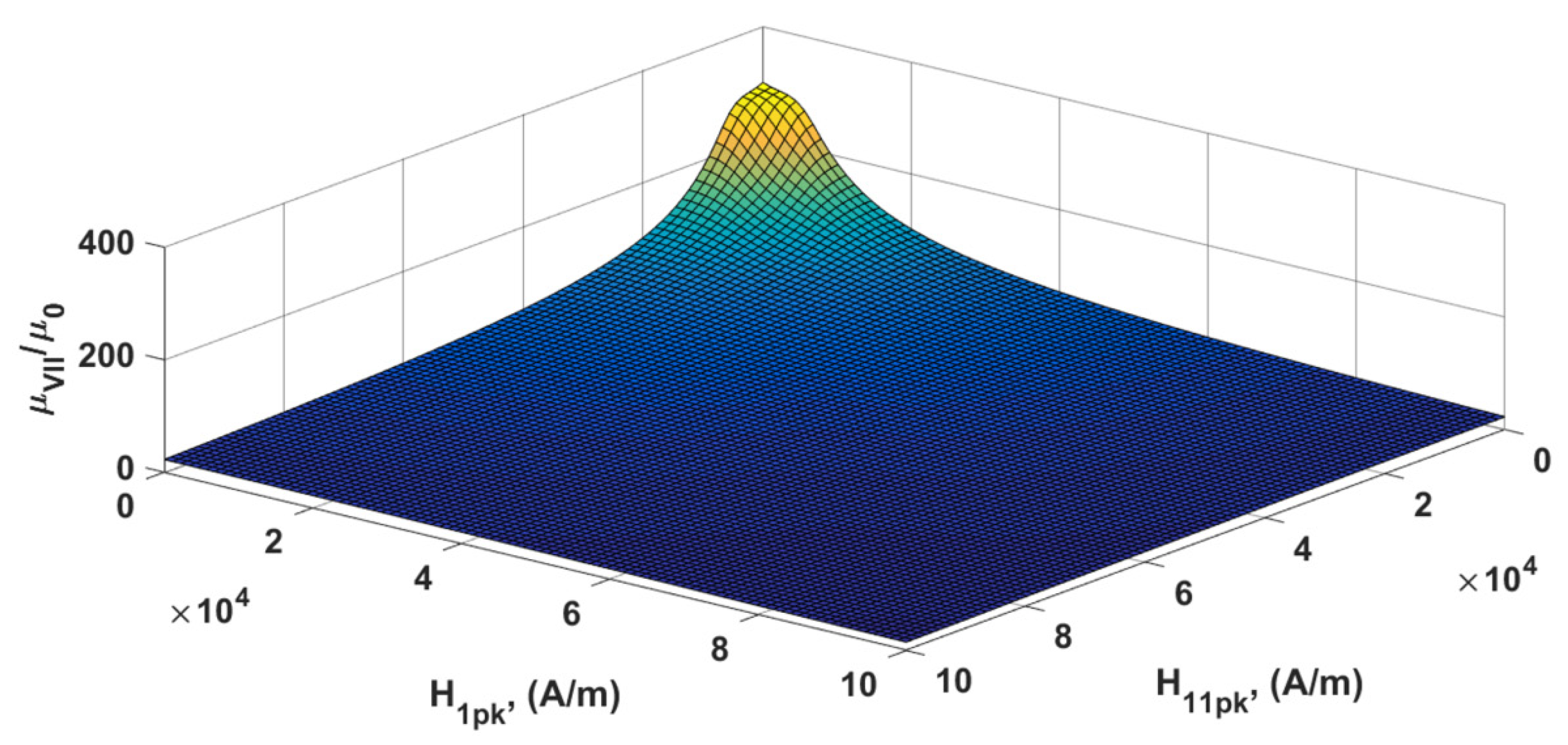
Calculating the effective magnetic permeability based on the distribution of the magnetic field strength or magnetic flux density, determined only for the model associated with the fundamental field harmonic in the machine air gap, can raise doubts. To take into account the impact of the higher harmonics and to assess the error related to this assumption, a new definition of the multidimensional effective magnetic permeability was proposed:
where
,
,
are the magnetic field strength amplitudes related to the fundamental, eleventh and thirteenth harmonics of the field and
For zero values of the higher harmonics, the definition (10) is equivalent to (1). This approach only slightly complicates the model implementation (in each algorithm iteration the distribution of the magnetic field strength in all considered rotor models needs to be obtained). An advantage of this expression, however, is the fact that effective permeability can be expressed before starting the calculations, without knowing the individual amplitudes. For the expression (10), it is possible since
does not depend on the phase angles of individual harmonics. In general, this occurs when the frequencies of the higher harmonics are the multiples of the fundamental harmonic. The value of the multidimensional effective permeability is determined only once as a multidimensional table for the assumed variability ranges of individual harmonics of the magnetic field strength.
Figure 5 shows the determined distribution of this quantity assuming that
= 0 A/m. The multidimensional permeability determined was used again for calculations and compared with the results obtained for (1). The result of that comparison is shown in
Table 3. As can be seen, the application of the multidimensional effective permeability brings only slight improvement to the accuracy of calculation results. This is due to relatively low magnetic field strength values in the areas of the models associated with the slot harmonics, as illustrated in
Figure 6.
4. Physical Validation
The analysis demonstrated that, from a practical point of view, it is sufficient to assume the first definition of the effective magnetic permeability, both due to the accuracy of calculations, and ease of implementation. It should be mentioned that, when adopting the sinusoidal variation of the magnetic field strength in determining the effective magnetic permeability, the fixed point method is applied in the multi-harmonic model to solve the nonlinearity problem, which is easy to implement, and it is distinguished by good convergence quality in case of time-harmonic magnetic fields. In the latter case, it is recommended to use the Newton–Raphson method [
16].
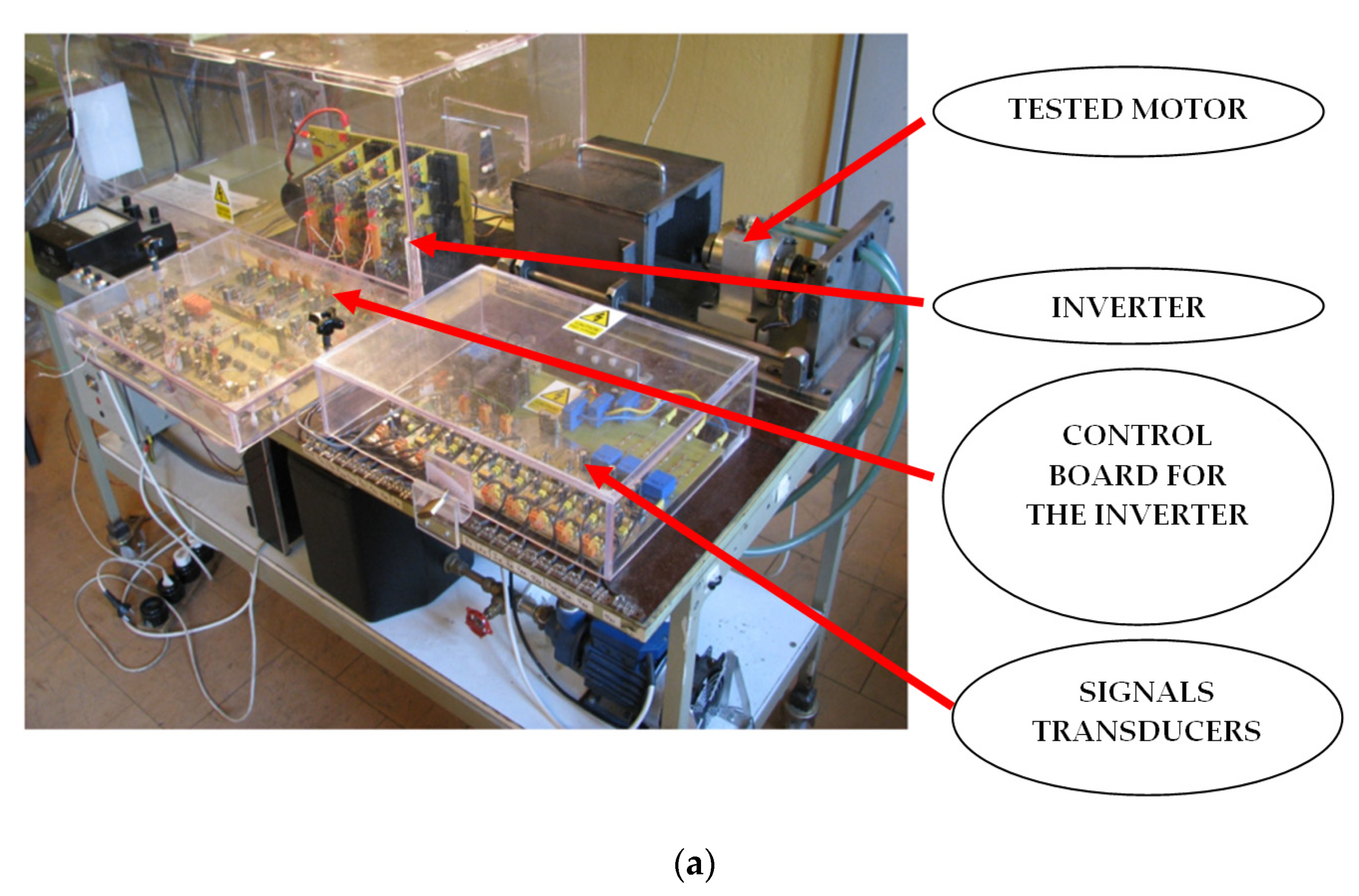
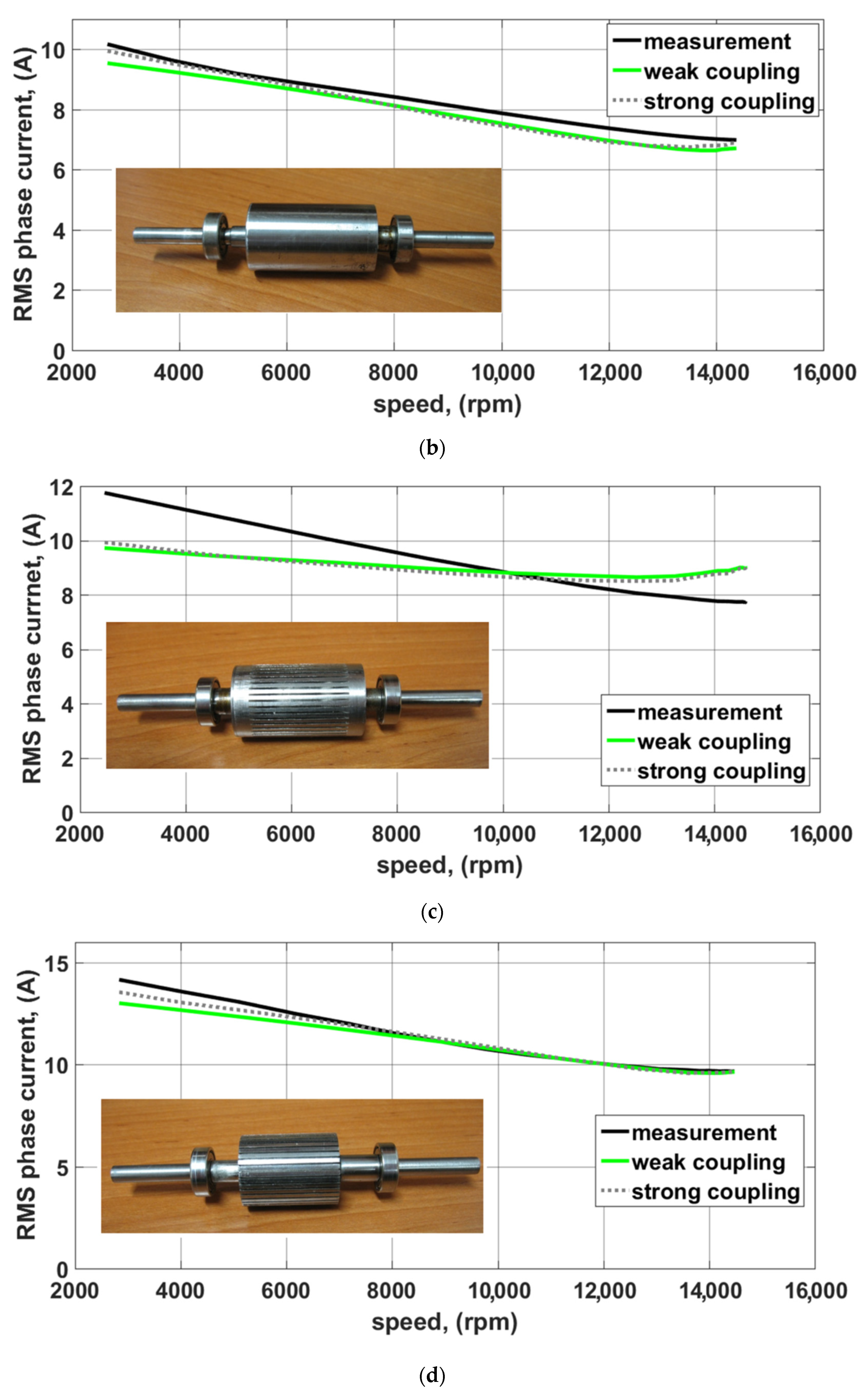
The model developed, together with the first definition of the effective magnetic permeability, was applied to calculate the RMS current of a real machine (supplied via a quasi-square wave voltage inverter, fundamental frequency of supplying voltage equal to 500 Hz) with three different solid rotors tested by Garbiec and to compare the results with the calculations performed using a model with a weak coupling the same definition of the effective magnetic permeability applied (see
Figure 7).
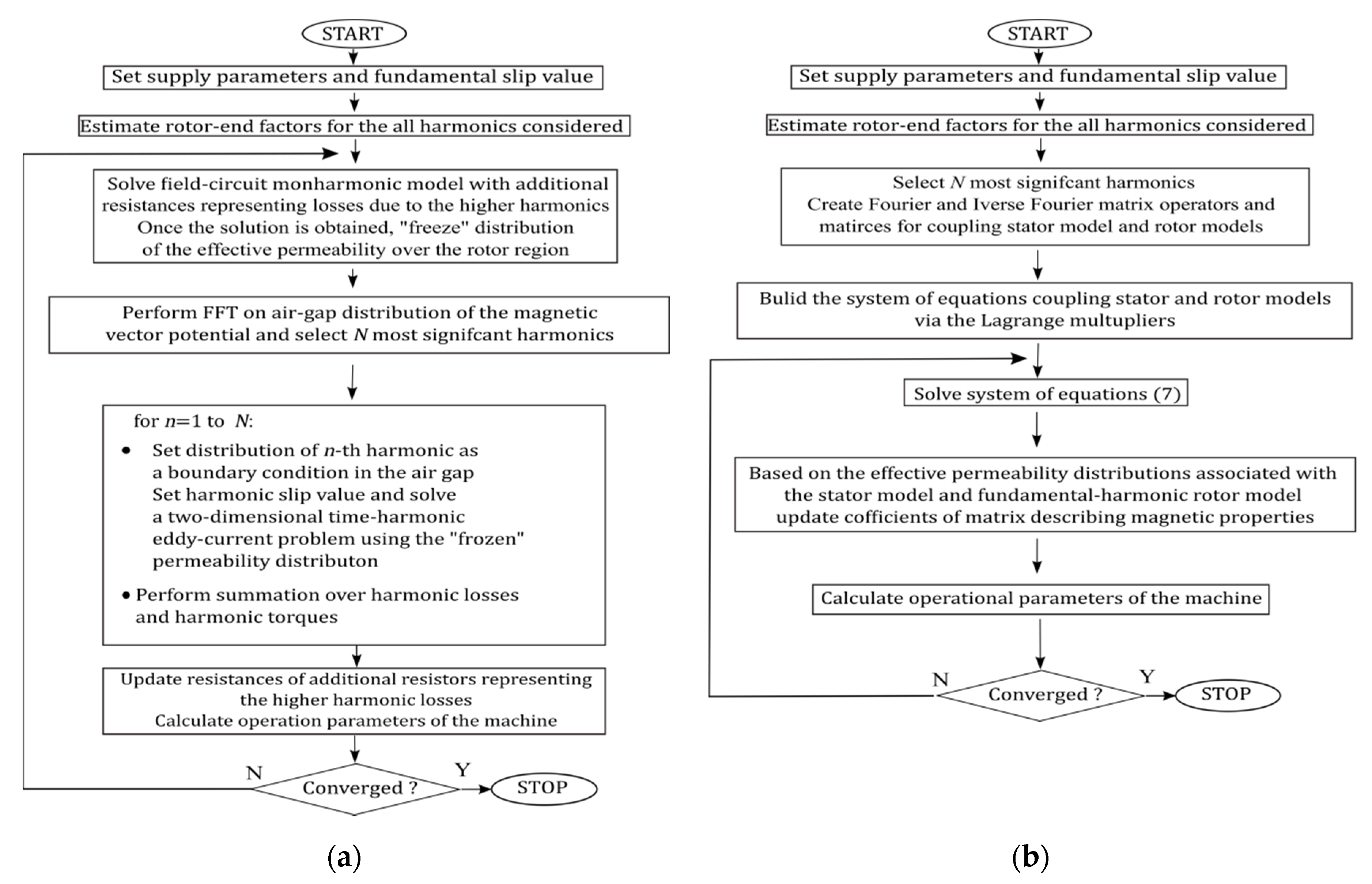
As it can be seen in the figure the idea of using the model with the strong coupling is to simultaneously solve the problem composed of a few submodels (the stator model and rotor models associated with the considered harmonics) coupled by appropriate boundary conditions imposed on the interface boundary in the air gap. This formulation results in one system of equations and determination of the magnetic vector potential distribution for all models via single solution of (7). The interface conditions in the air-gap are formulated with the use of matrix operators of the Fourier transform and the inverse Fourier transform, as shown in Garbiec at. al, enforce the propagation of only the selected harmonics of the air-gap field distribution between the stator model and the considered rotor models. All rotor models act via the same constraint on the stator model. In the considered model with the weak coupling, the monoharmonic field-circuit model is used. The power loss due to slot harmonics is estimated by means of extraction of these harmonics from the calculated distribution of the magnetic vector potential. In this way it is possible to solve the field problem limited to only the area of rotor. In this approach the power factor, torque and stator current can be appropriately corrected, although there is no backward relationship, i.e., the air-gap field remains unaffected by these effects. To this end, it was also necessary to take into account the rotor-end effect [
18].
The physical test-stand and result of the comparison of computed and measured data for the machine with different rotors is shown in
Figure 8. With the application of the multi-harmonic model with the strong coupling and with the modified sub-model coupling scheme, it was possible to achieve practically identical calculation results, yet in a shorter time by approx. 30%. The errors found result mainly from supplying the physical model via a quasi-square wave voltage inverter (in numerical models, the pure sine-wave voltage supply with an RMS value equal to the RMS value of the measured voltage is assumed).
5. Conclusions
The comparison of various methods for calculating the effective magnetic permeability for a multi-harmonic model of a solid-rotor induction machine carried out in the study proved that, from a practical point of view, the best results are obtained using the basic definition, described by Equation (1). Determining the effective magnetic permeability as the ratio of the magnitudes of the fundamental harmonics of the magnetic flux density and the magnetic field strength calculated assuming the sinusoidal variation of the latter allows for an easy implementation of the algorithm which is important from practical point of view. Furthermore, it was demonstrated that, in the studied case, the most influential magnetic saturation effects come from the fundamental harmonic of the magnetic field which means that the slot harmonics of the air-gap magnetic flux density do not have to be computed in that process. For the same definition of the effective magnetic permeability and an appropriate modification of the coupling scheme, it is possible to obtain practically the same results as in the case of the multi-harmonic model with the weak coupling, but yet in a shorter time. Another step to take will be to conduct detailed research using the developed model to analyze the magnetic saturation effects in the squirrel cage induction machines with both three- and single-phase windings.