Numerical Study of the Turbulent Flow from a Steam Dumping Pressurizer Relief Tank
Abstract
1. Introduction
2. The Theory and Numerical Techniques
2.1. Governing Equations
2.2. RANS Turbulence Models
2.2.1. SSTKW
2.2.2. LRR
2.3. LES Turbulence Models
SL-LES
2.4. Numerical Algorithm
| Algorithm 1: The numerical algorithm of PRTFOAM, which adopt the PIMPLE algorithm for the velocity and pressure coupling problem. |
 |
3. Geometry and Configurations
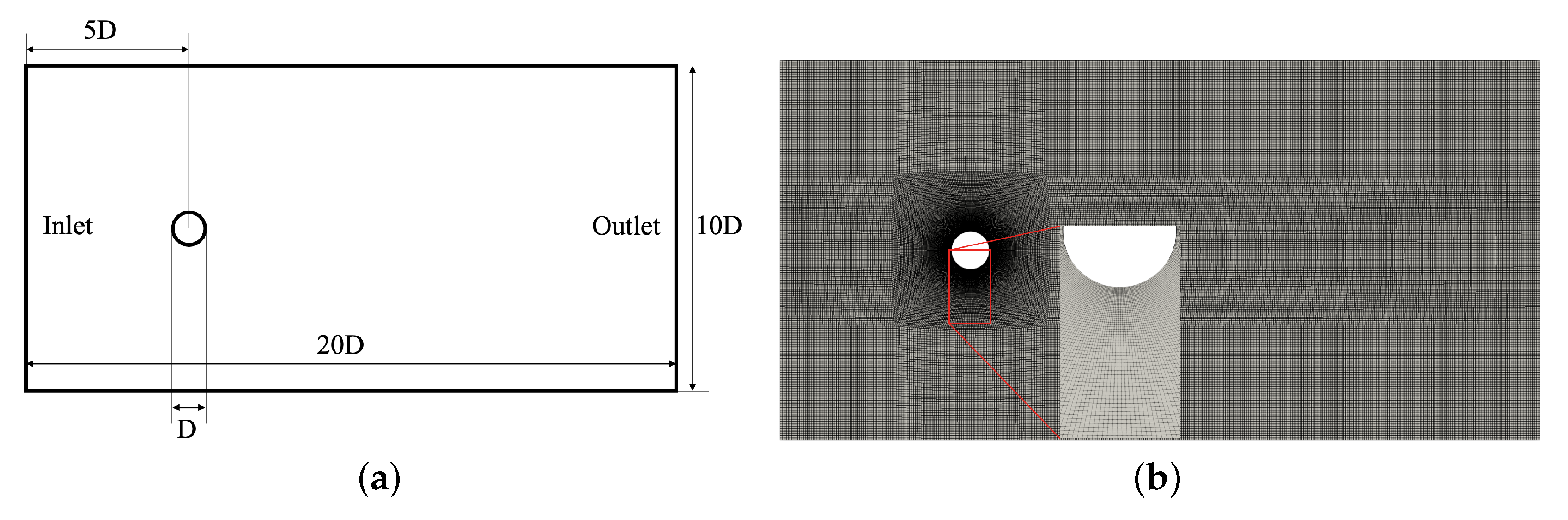
3.1. Flow past a Circular Cylinder (Re = 3900)
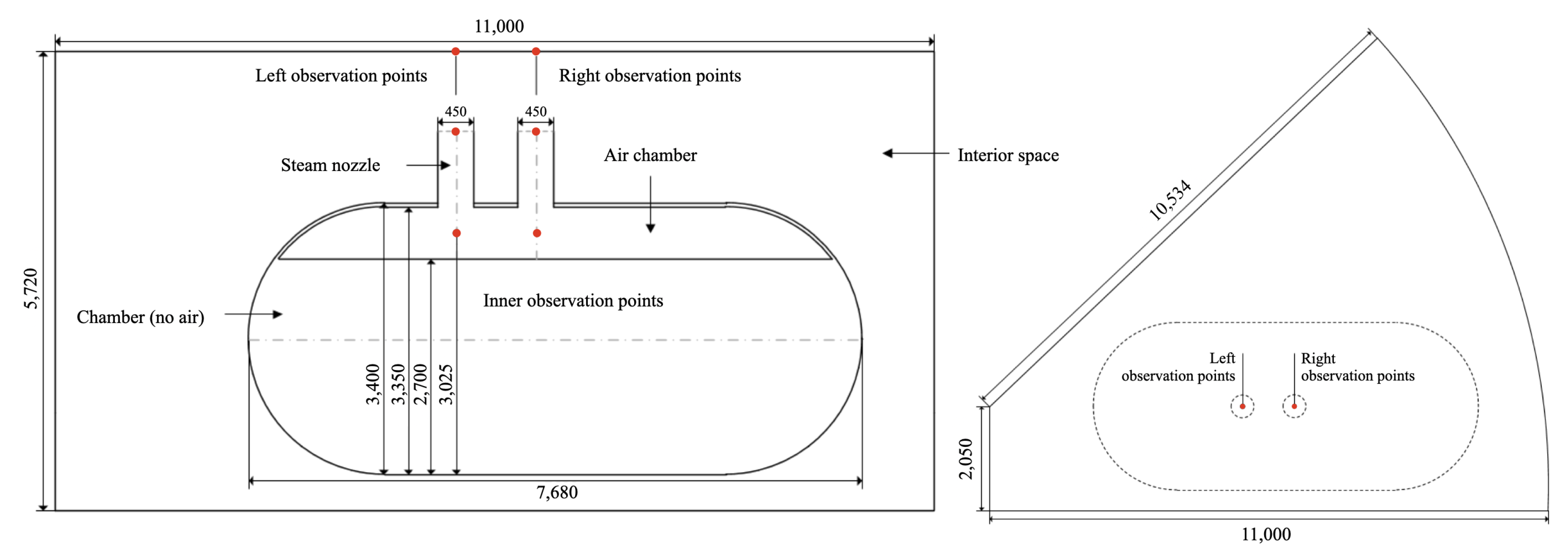
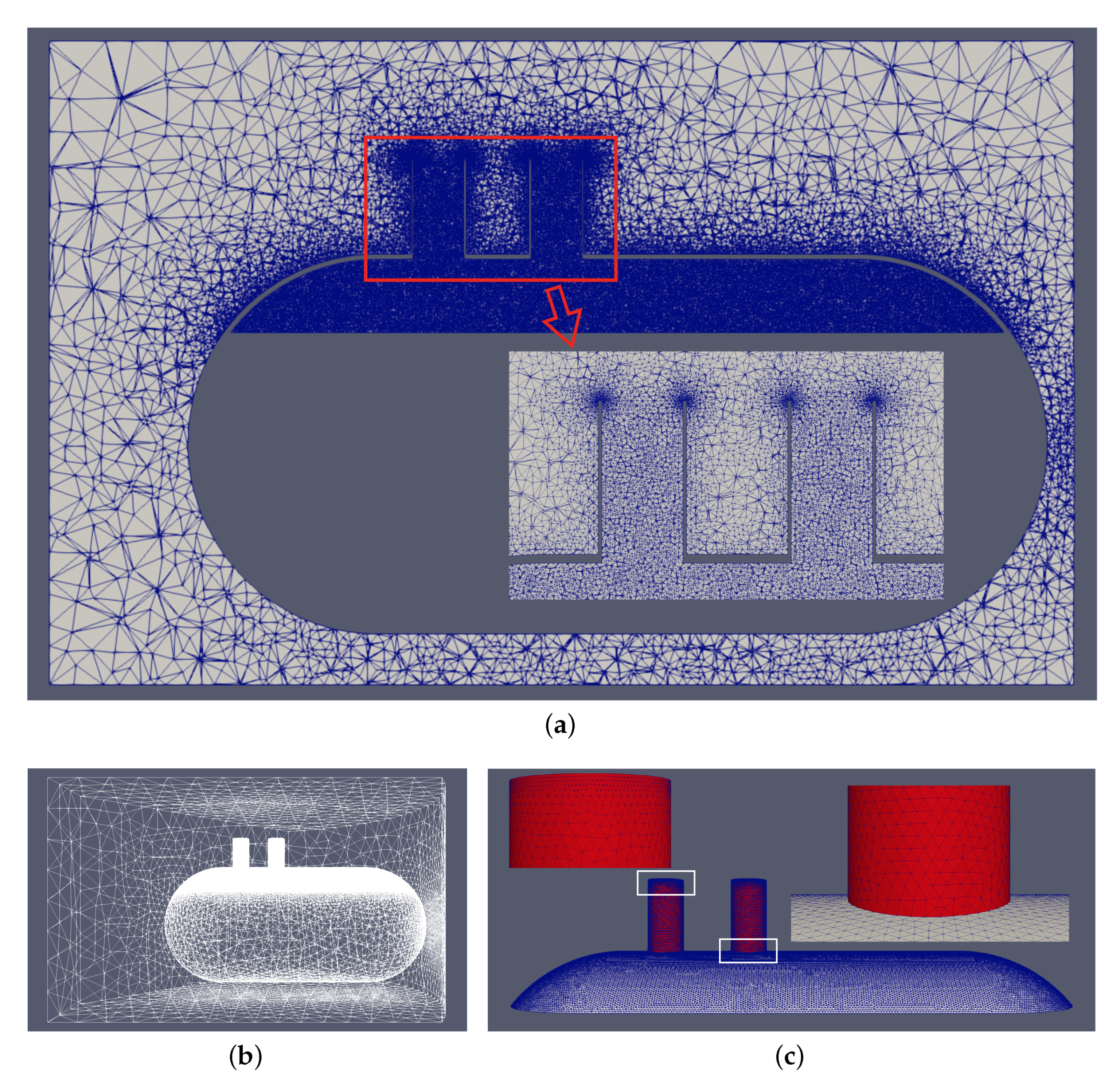
3.2. The PRT Steam Dumping Process
4. Results and Discussion
4.1. Flow Past a Circular Cylinder (Re = 3900)
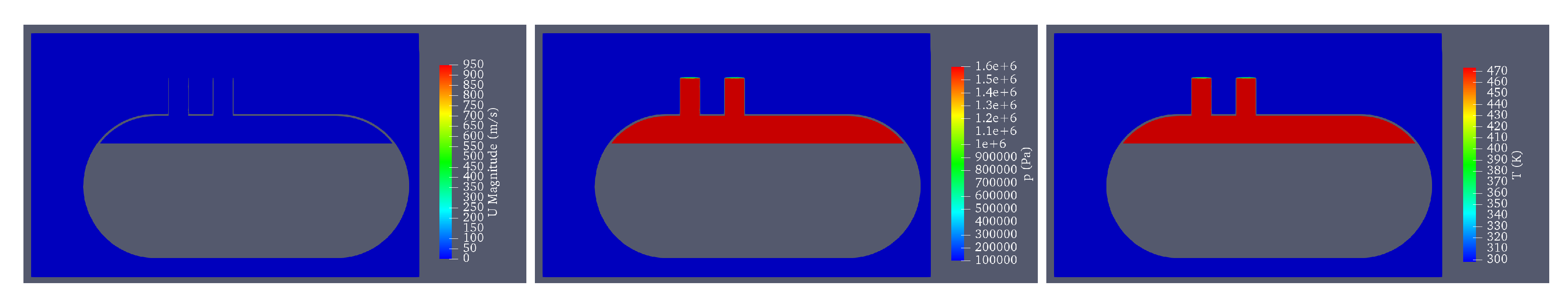
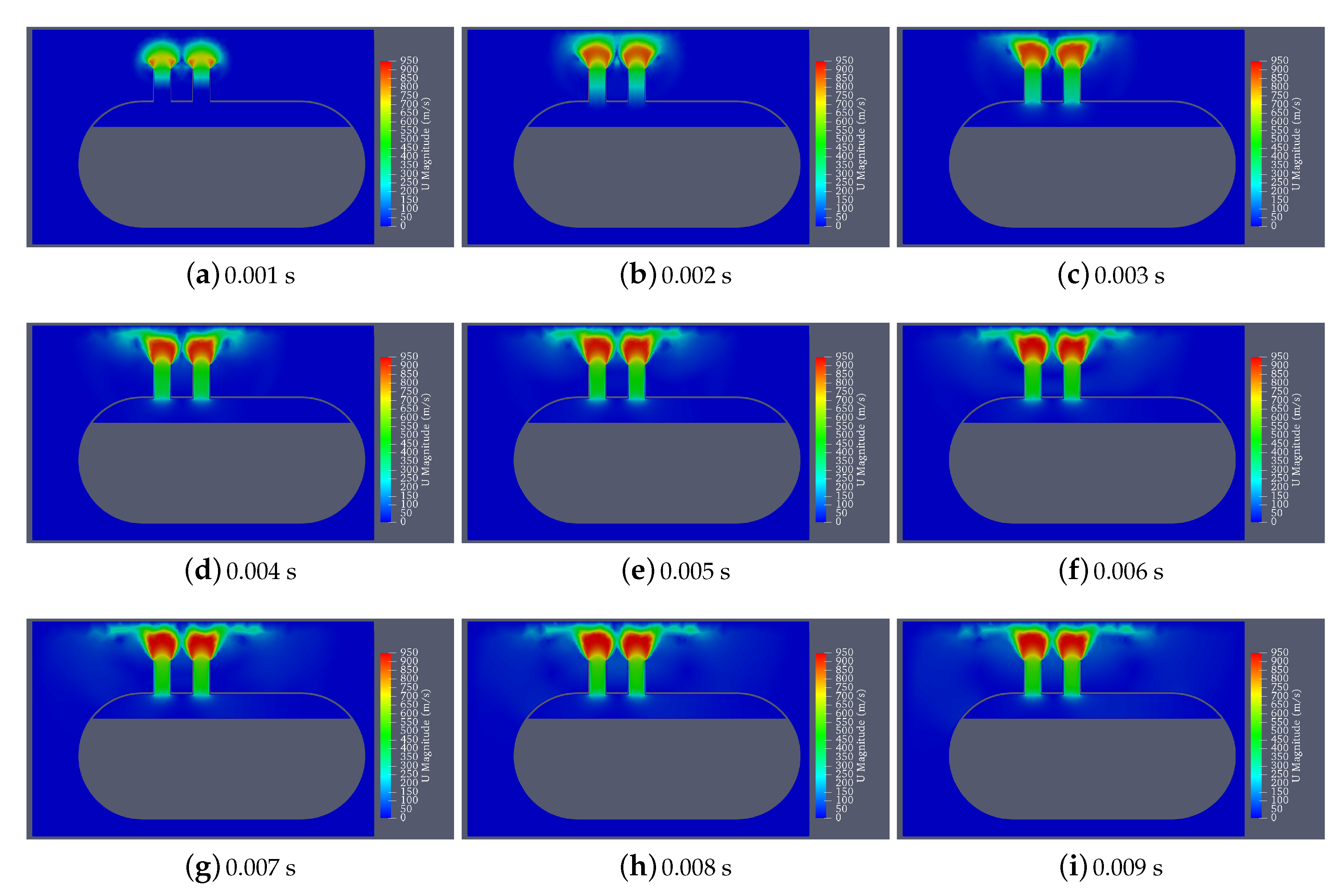
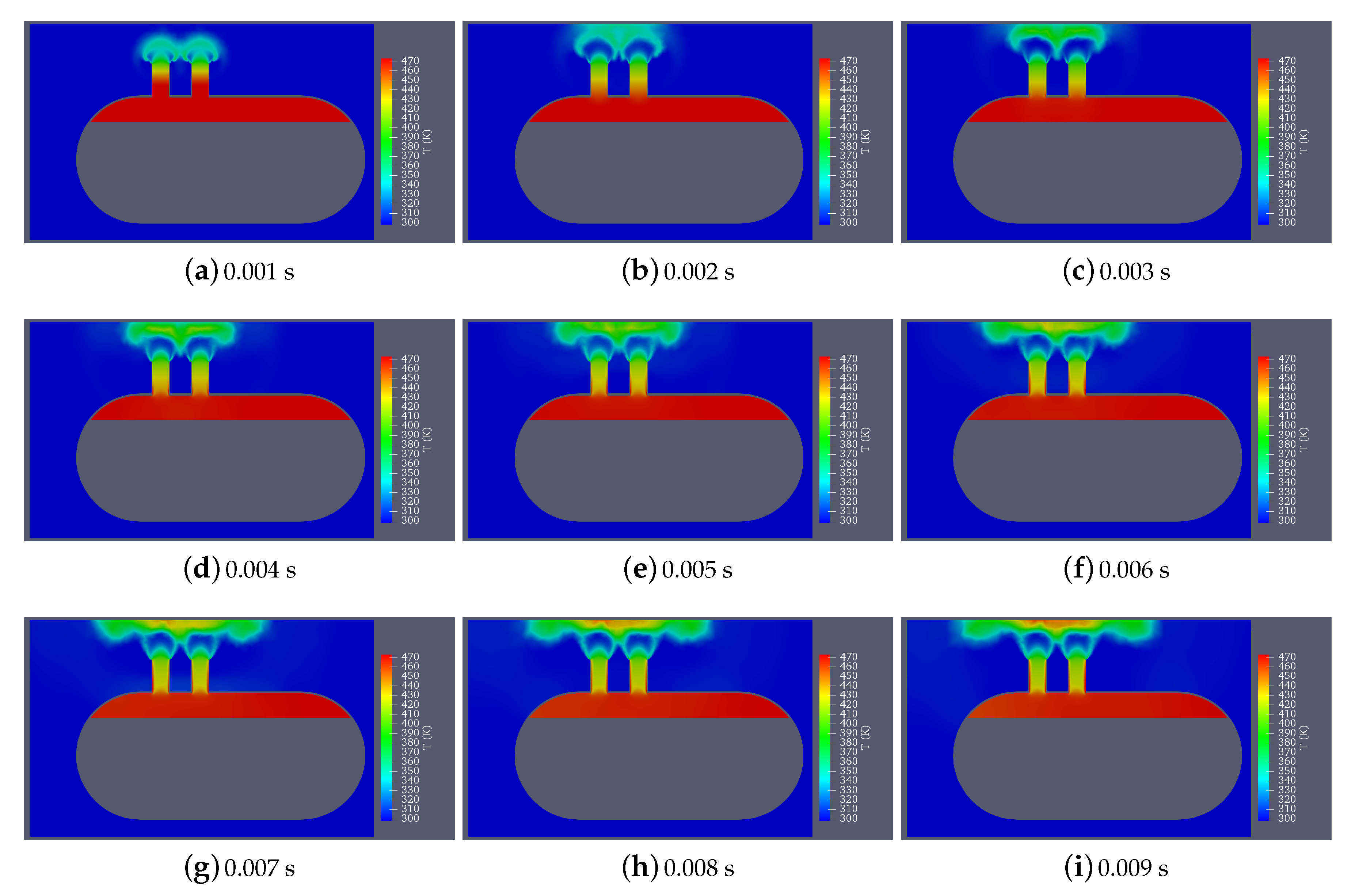
4.2. PRT Steam Dumping Process
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
| Symbol/Acronym | Meaning |
|---|---|
| PRT | Pressurizer Relief Tank |
| LWR | Light Water Reactors |
| PWR | Pressurized Water Reactors |
| DNS | Direct Numerical Simulation |
| RANS | Reynolds-averaged Navier-Stokes |
| LES | Large Eddy Simulation |
| Re | Reynolds number |
| SSTKW | the SST k- model proposed by Menter [24,25] |
| LRR | the Reynolds stress model based on Launder et al. [23] |
| SL-LES | the Smagorinsky–Lilly SGS model base LES approach [19,26] |
| SGS | Sub-grid-scale |
| Velocity | |
| Pressure | |
| Density | |
| Temperature | |
| () | components of velocity vector in x-, y- and z-direction |
| & | the source terms |
| the coefficient of thermal conductivity | |
| the dissipation function | |
| the second viscosity | |
| e | specific internal energy |
| specific heat capacity | |
| Reynolds stresses | |
| k | the turbulent kinetic energy |
| the dissipation rate of turbulent kinetic energy | |
| the specific dissipation rate | |
| the kinematic eddy viscosity | |
| the convective term | |
| the production term | |
| the rotational term | |
| the diffusion term | |
| the dissipation rate | |
| the pressure–strain term | |
| the SGS viscosity | |
| Sutherland coefficient | |
| enthalpy change | |
| reference temperature | |
| lift coefficient | |
| drag coefficient | |
| Strouhal number |
References
- Zvirin, Y. A review of natural circulation loops in pressurized water reactors and other systems. Nucl. Eng. Des. 1982, 67, 203–225. [Google Scholar] [CrossRef]
- Guo, X.W.; Xu, X.H.; Wang, Q.; Li, H.; Ren, X.G.; Xu, L.; Yang, X.J. A Hybrid Decomposition Parallel Algorithm for Multi-scale Simulation of Viscoelastic Fluids. In Proceedings of the 2016 IEEE International Parallel and Distributed Processing Symposium (IPDPS), Chicago, IL, USA, 23–27 May 2016; pp. 443–452. [Google Scholar] [CrossRef]
- Liu, Y.; Guo, X.W.; Li, C.; Yang, C.; Gan, X.; Zhang, P.; Wang, Y.; Zhao, R.; Fan, S. The Communication- Overlapped Hybrid Decomposition Parallel Algorithm for Multi-Scale Fluid Simulations. In Proceedings of the 48th International Conference on Parallel Processing. Association for Computing Machinery, Kyoto, Japan, 5–8 August 2019. [Google Scholar] [CrossRef]
- Zhang, J.; Shi, W.; Zhou, L.; Gong, R.; Wang, L.; Zhou, H. A Low-Power and High-PSNR Unified DCT/IDCT Architecture Based on EARC and Enhanced Scale Factor Approximation. IEEE Access 2019, 7, 165684–165691. [Google Scholar] [CrossRef]
- Baraldi, D.; Heitsch, M.; Wilkening, H. CFD simulations of hydrogen combustion in a simplified EPR containment with CFX and REACFLOW. Nucl. Eng. Des. 2007, 237, 1668–1678. [Google Scholar] [CrossRef]
- Hata, K.; Liu, Q.; Nakajima, T. Natural convection heat transfer from a vertical single cylinder with helical wire spacer in liquid sodium. Nucl. Eng. Des. 2019, 341, 73–90. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhang, R.; Tian, W.; Su, G.H.; Qiu, S. Numerical prediction of CHF based on CFD methodology under atmospheric pressure and low flow rate. Appl. Therm. Eng. 2019, 149, 881–888. [Google Scholar] [CrossRef]
- Min Baek, S.; Cheon No, H.; Park, I.Y. A nonequilibrium three-region model for transient analysis of pressurized water reactor pressurizer. Nucl. Technol. 1986, 74, 260–266. [Google Scholar] [CrossRef]
- Qiang, G.; Yaodong, C. Hydrogen concentration distribution simulation during severe accidents in pressurizer relief tank compartment on NPP containment. At. Energy Sci. Technol. 2012, 46, 32–36. [Google Scholar]
- Gong, A.C.; Zhang, B.; Gao, Y.F. Thermal Design and Calculation of Pressurizer Relief Tanks. J. Chin. Soc. Power Eng. 2012, 7, 577–580. [Google Scholar]
- Wang, X.; Wang, M.; Zhang, J.; Wu, Y.; Tian, W.; Su, G.; Qiu, S. Study on the water seal formation process in advanced PWR pressurizer using CFD method. Ann. Nucl. Energy 2020, 135, 106949. [Google Scholar] [CrossRef]
- Wang, Y.; Guo, X.W.; Liu, D.; Wu, G.; Li, C.; Chen, L.; Zhao, R.; Yang, C. A 3D Numerical Study of Supersonic Steam Dumping Process of the Pressurizer Relief Tank. Energies 2019, 12, 2276. [Google Scholar] [CrossRef]
- Zou, S.; Yuan, X.F.; Yang, X.; Yi, W.; Xu, X. An integrated lattice Boltzmann and finite volume method for the simulation of viscoelastic fluid flows. J. Non-Newton. Fluid Mech. 2014, 211, 99–113. [Google Scholar] [CrossRef]
- Xu, C.; Deng, X.; Zhang, L.; Fang, J.; Wang, G.; Jiang, Y.; Cao, W.; Che, Y.; Wang, Y.; Wang, Z.; et al. Collaborating CPU and GPU for large-scale high-order CFD simulations with complex grids on the TianHe-1A supercomputer. J. Comput. Phys. 2014, 278, 275–297. [Google Scholar] [CrossRef]
- Giacomelli, F.; Biferi, G.; Mazzelli, F.; Milazzo, A. CFD modeling of the supersonic condensation inside a steam ejector. Energy Procedia 2016, 101, 1224–1231. [Google Scholar] [CrossRef]
- Sirisup, S.; Karniadakis, G.; Saelim, N.; Rockwell, D. DNS and experiments of flow past a wired cylinder at low Reynolds number. Eur. J. Mech.-B/Fluids 2004, 23, 181–188. [Google Scholar] [CrossRef]
- Ma, X.; Karamanos, G.S.; Karniadakis, G. Dynamics and low-dimensionality of a turbulent near wake. J. Fluid Mech. 2000, 410, 29–65. [Google Scholar] [CrossRef]
- Boussinesq, J. Theorie de l’ecoulement tourbillant. Mem. Acad. Sci. 1877, 23, 46. [Google Scholar]
- Smagorinsky, J. General circulation experiments with the primitive equations: I. The basic experiment. Mon. Weather. Rev. 1963, 91, 99–164. [Google Scholar] [CrossRef]
- Nicoud, F.; Ducros, F. Subgrid-scale stress modelling based on the square of the velocity gradient tensor. Flow Turbul. Combust. 1999, 62, 183–200. [Google Scholar] [CrossRef]
- Deardorff, J.W. A numerical study of three-dimensional turbulent channel flow at large Reynolds numbers. J. Fluid Mech. 1970, 41, 453–480. [Google Scholar] [CrossRef]
- Komen, E.; Camilo, L.; Shams, A.; Geurts, B.J.; Koren, B. A quantification method for numerical dissipation in quasi-DNS and under-resolved DNS, and effects of numerical dissipation in quasi-DNS and under-resolved DNS of turbulent channel flows. J. Comput. Phys. 2017, 345, 565–595. [Google Scholar] [CrossRef]
- Launder, B.E.; Reece, G.J.; Rodi, W. Progress in the development of a Reynolds-stress turbulence closure. J. Fluid Mech. 1975, 68, 537–566. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Menter, F.R. Eddy viscosity transport equations and their relation to the k-ε model. J. Fluids Eng. 1997, 119, 876–884. [Google Scholar] [CrossRef]
- Lilly, D.K. On the application of the eddy viscosity concept in the inertial sub-range of turbulence. NCAR Manuscr. 1966, 123. [Google Scholar] [CrossRef]
- Yakhot, V.; Orszag, S.A. Renormalization group analysis of turbulence. I. Basic theory. J. Sci. Comput. 1986, 1, 3–51. [Google Scholar] [CrossRef]
- WILCOX, D. Progress in hypersonic turbulence modeling. In Proceedings of the 22nd Fluid Dynamics, Plasma Dynamics and Lasers Conference, Honolulu, HI, USA, 24–26 June 1991; p. 1785. [Google Scholar]
- Menter, F.R.; Kuntz, M.; Langtry, R. Ten years of industrial experience with the SST turbulence model. Turbul. Heat Mass Transf. 2003, 4, 625–632. [Google Scholar]
- Patankar, S.V.; Spalding, D.B. A calculation procedure for heat, mass and momentum transfer in three-dimensional parabolic flows. In Numerical Prediction of Flow, Heat Transfer, Turbulence and Combustion; Elsevier: Amsterdam, The Netherlands, 1983; pp. 54–73. [Google Scholar]
- Issa, R.I. Solution of the implicitly discretised fluid flow equations by operator-splitting. J. Comput. Phys. 1986, 62, 40–65. [Google Scholar] [CrossRef]
- Khan, N.B.; Jameel, M.; Badry, A.B.B.M.; Ibrahim, Z.B. Numerical study of flow around a smooth circular cylinder at Reynold number = 3900 with large eddy simulation using CFD code. In Proceedings of the ASME 2016 35th International Conference on Ocean, Offshore and Arctic Engineering. American Society of Mechanical Engineers, Busan, Korea, 19–24 June 2016. [Google Scholar]
- Norberg, C. An experimental investigation of the flow around a circular cylinder: Influence of aspect ratio. J. Fluid Mech. 1994, 258, 287–316. [Google Scholar] [CrossRef]
- Tremblay, F.; Manhart, M.; Friedrich, R. LES of flow around a circular cylinder at a subcritical Reynolds number with cartesian grids. In Advances in LES of Complex Flows; Springer: Berlin/Heidelberg, Germany, 2002; pp. 133–150. [Google Scholar]
- Lourenco, L.; Shih, C. Characteristics of the plane turbulent near wake of a circular cylinder: A particle image velocity study (private communication), reported by Kravchenko, AG, Moin, P., 2000. Numerical studies of flow over a circular cylinder at ReD = 3900. Phys. Fluids 1993, 12, 403–417. [Google Scholar]
- Ong, L.; Wallace, J. The velocity field of the turbulent very near wake of a circular cylinder. Exp. Fluids 1996, 20, 441–453. [Google Scholar] [CrossRef]
- Beaudan, P.; Moin, P. Numerical Experiments on the Flow Past a Circular Cylinder At Sub-Critical Reynolds Number; Technical Report; Stanford Univ Ca Thermosciences Div: Stanford, CA, USA, 1994. [Google Scholar]
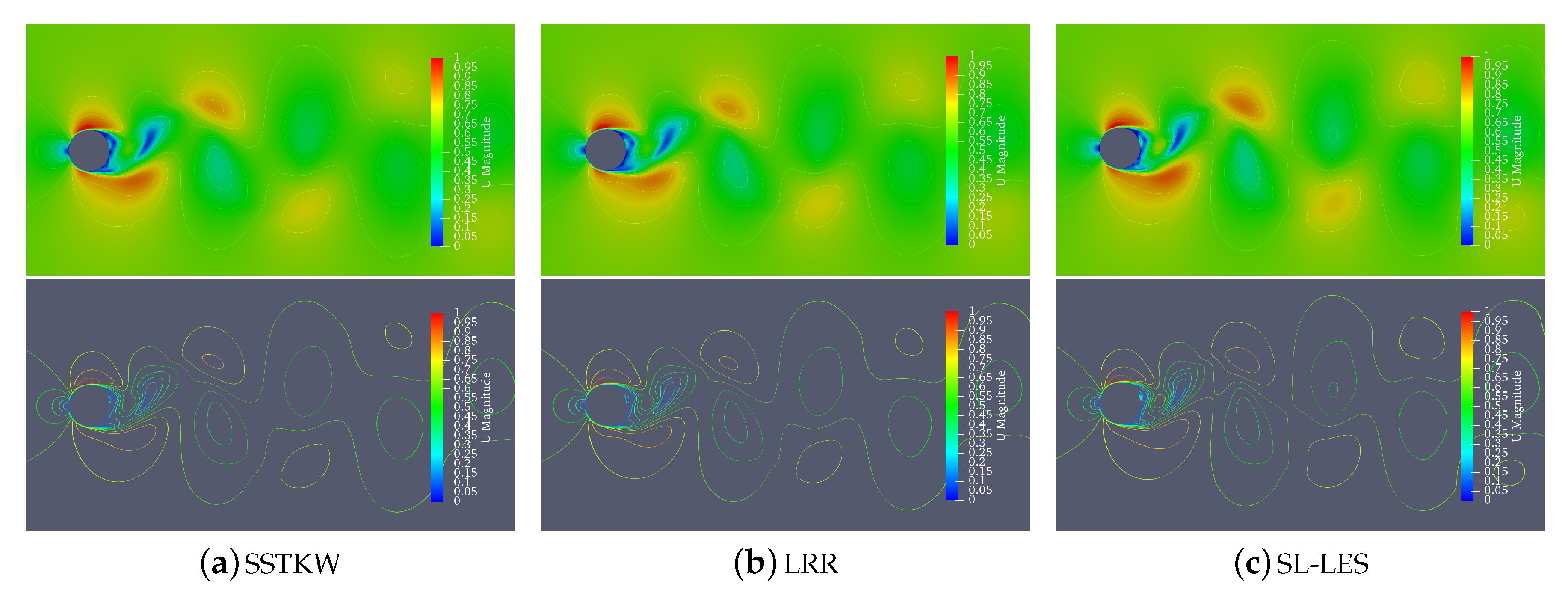
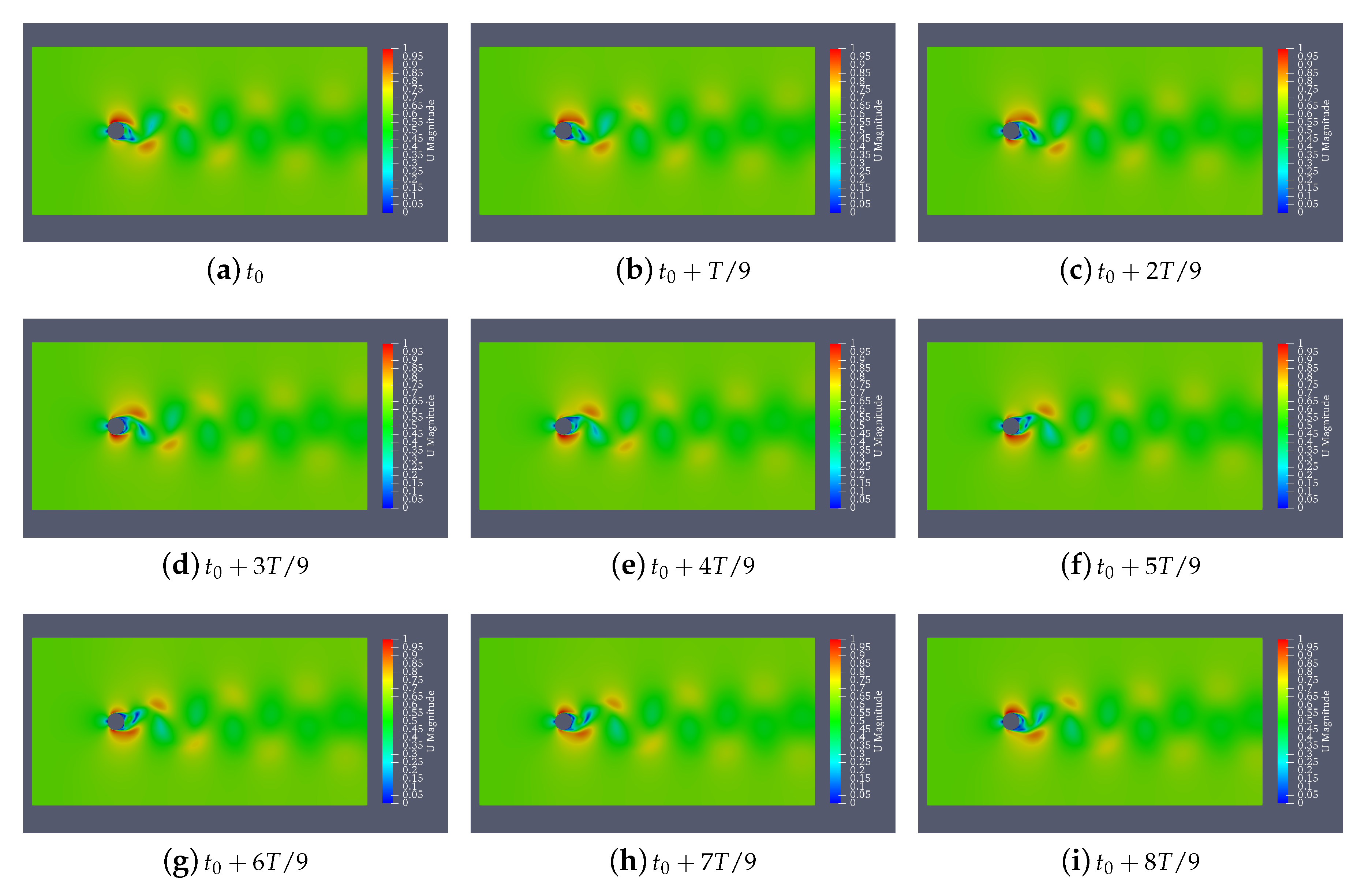
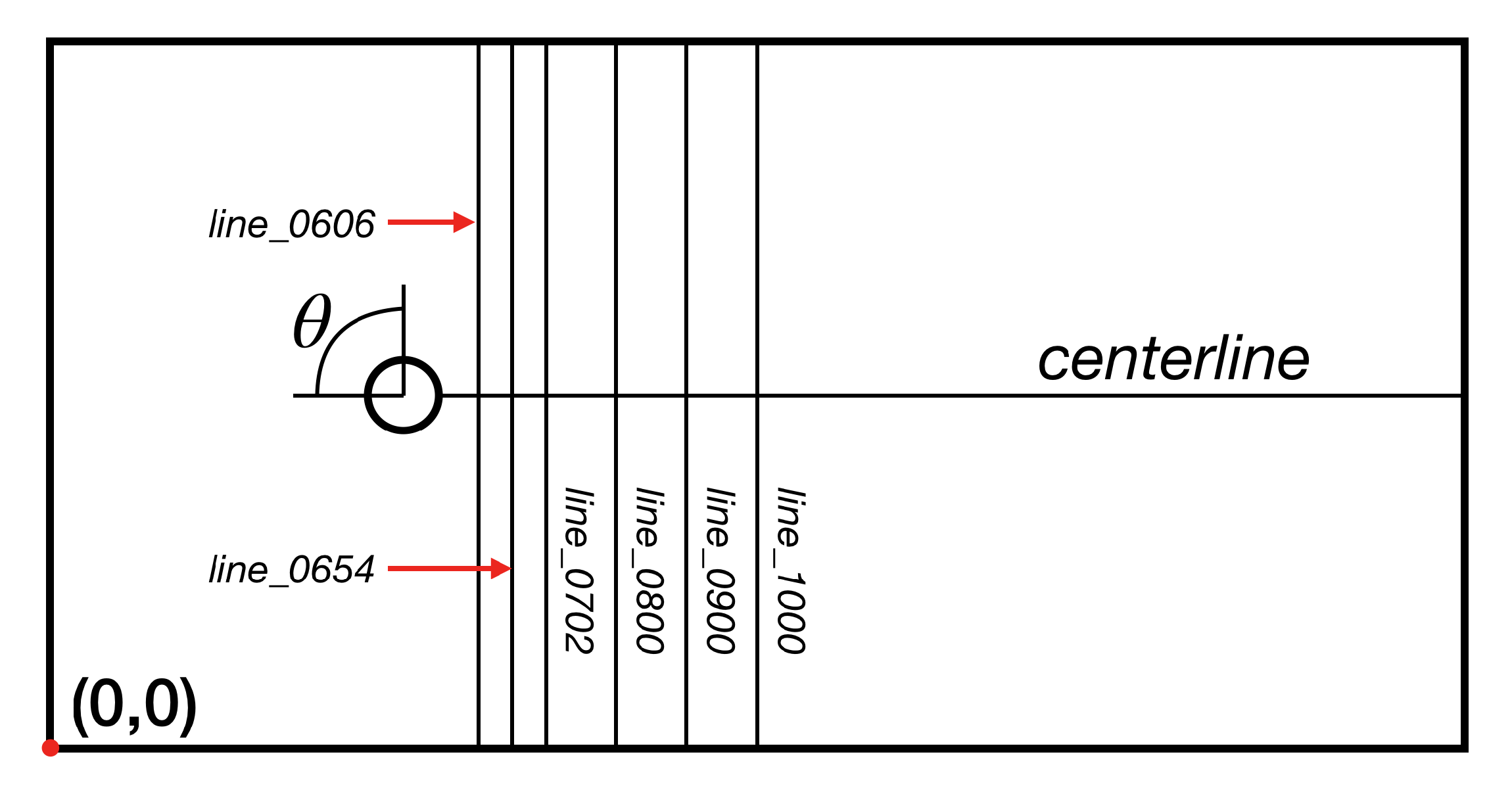
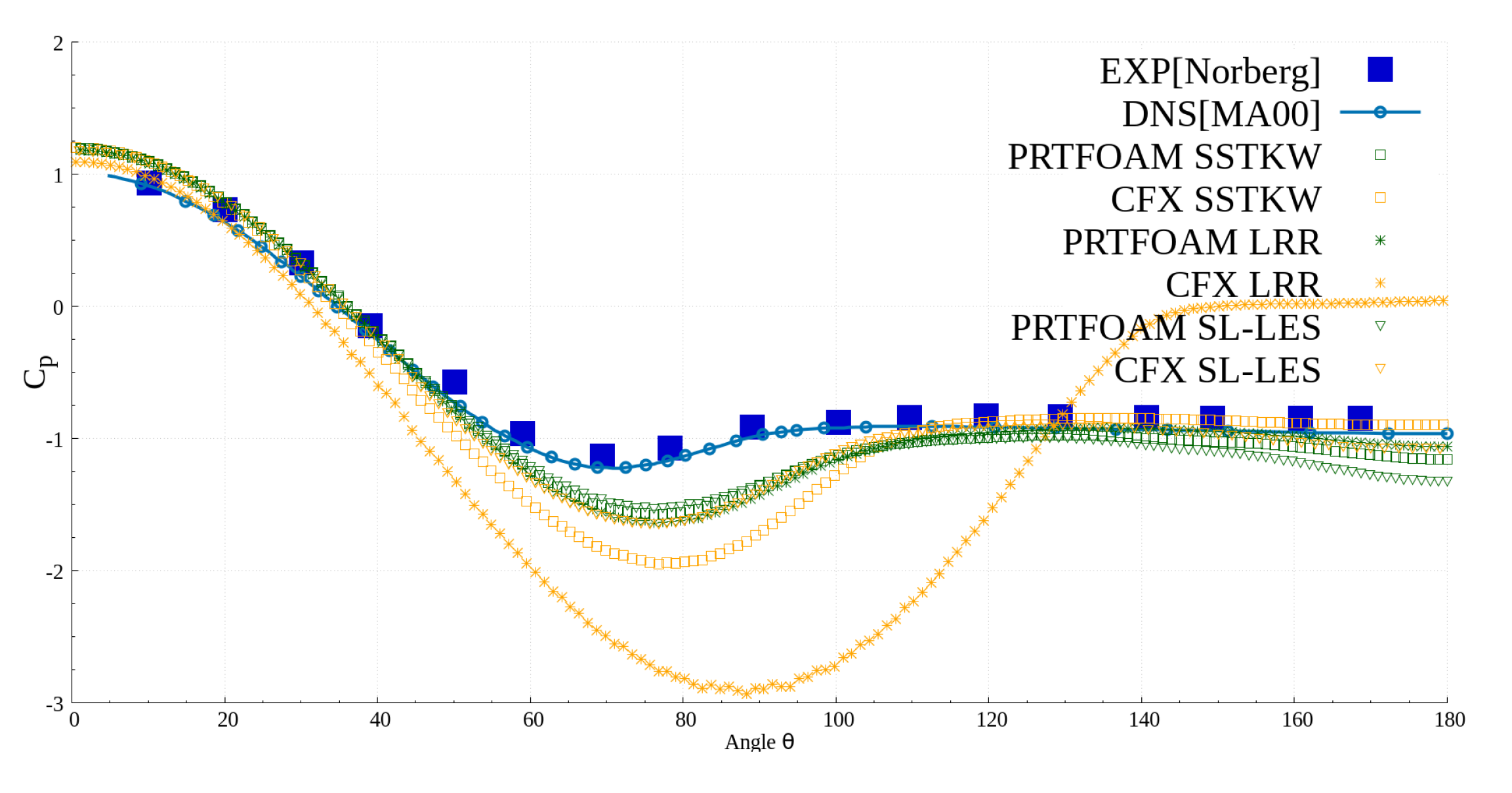
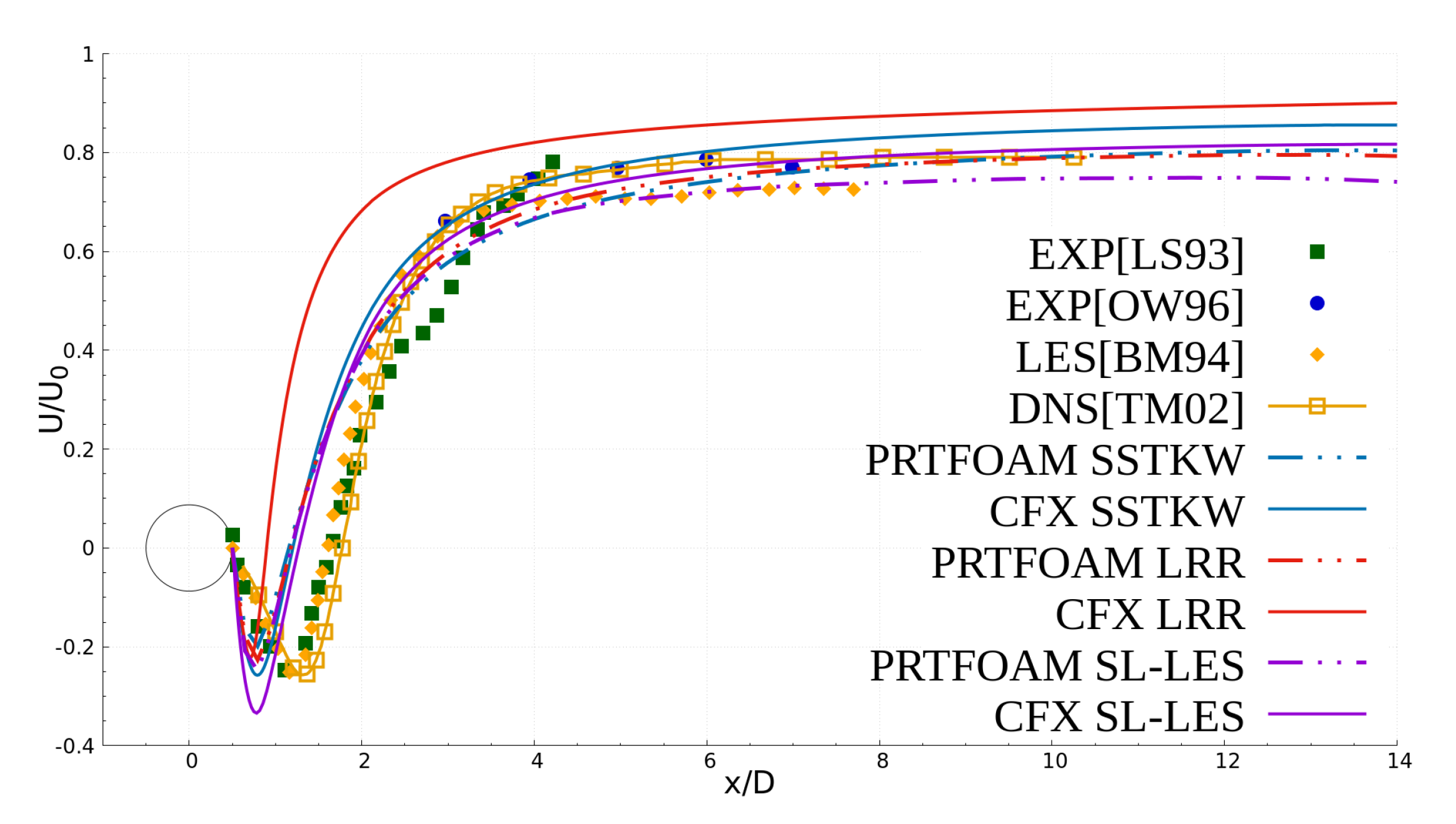
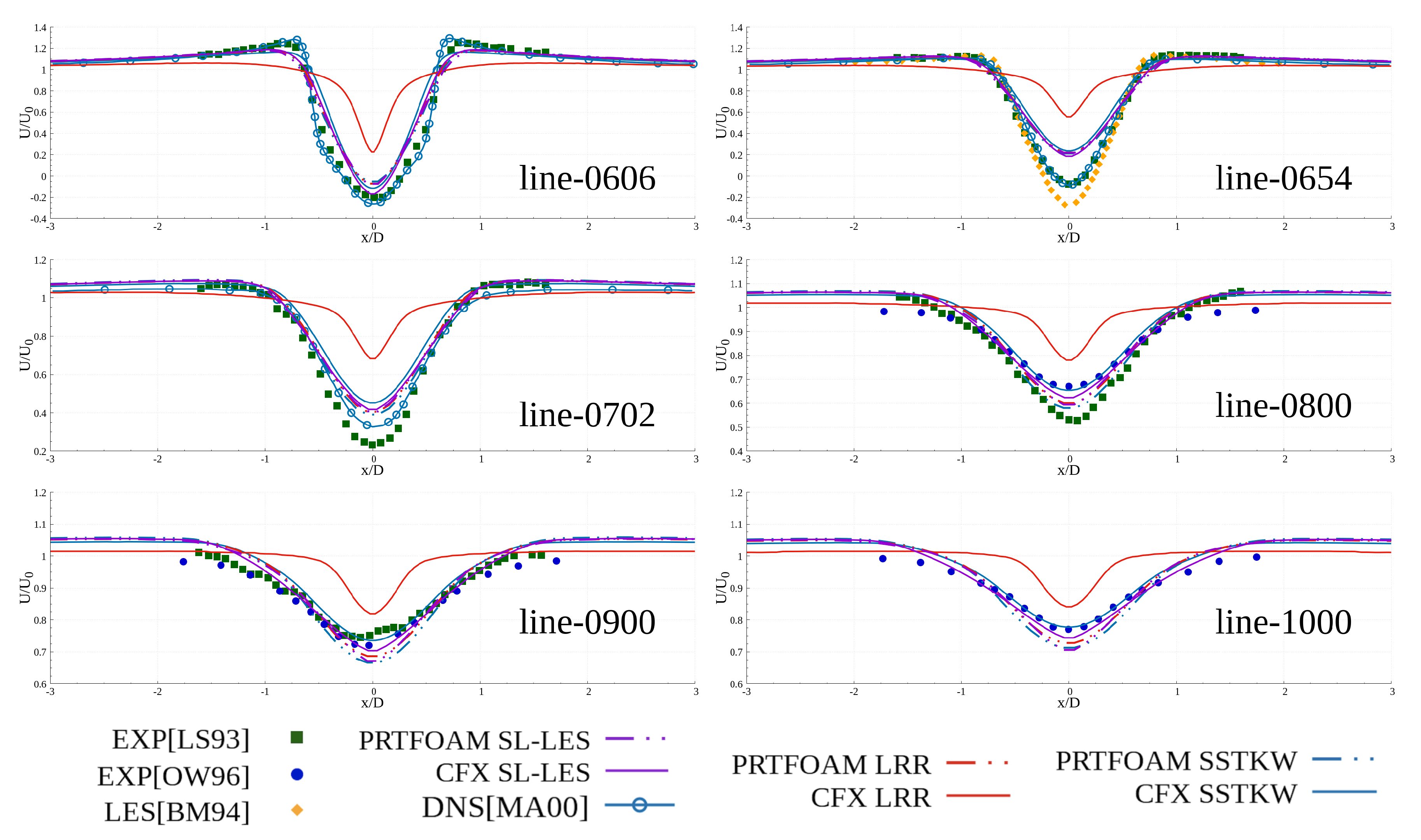


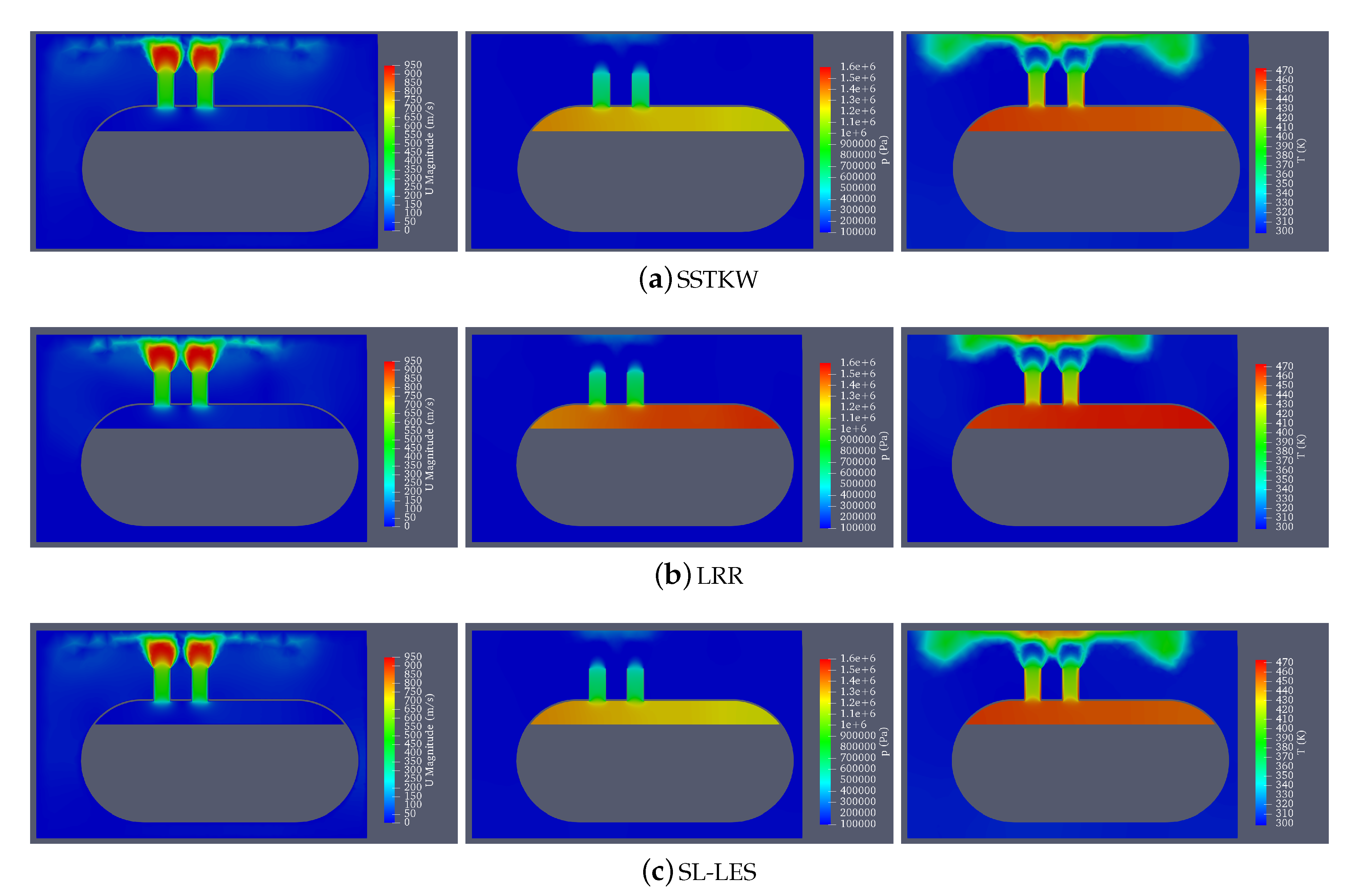

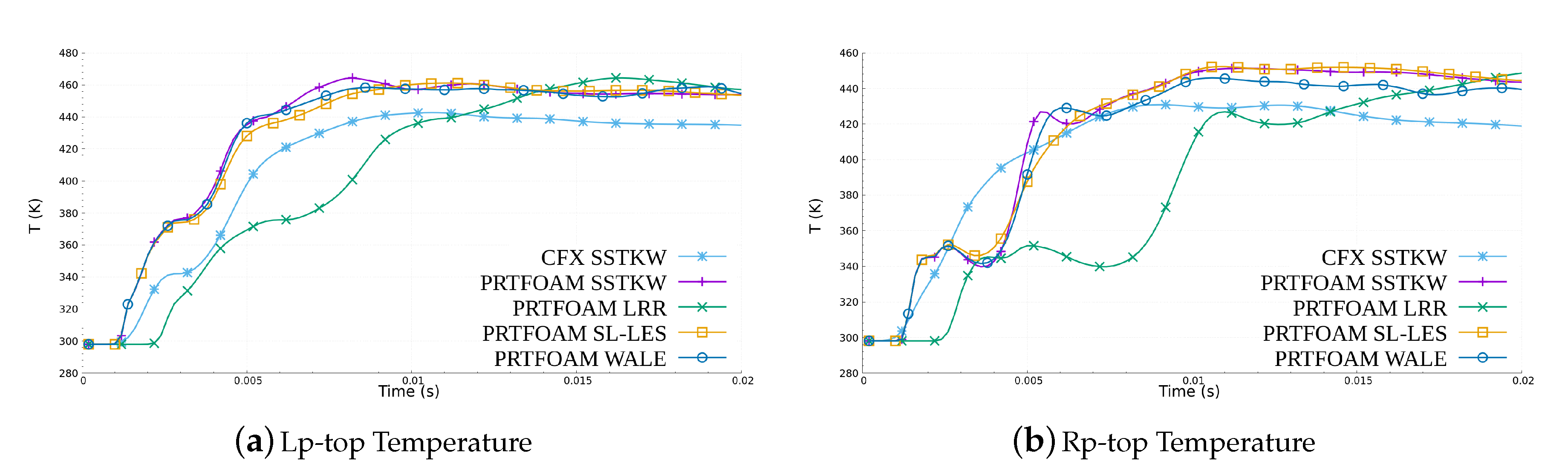
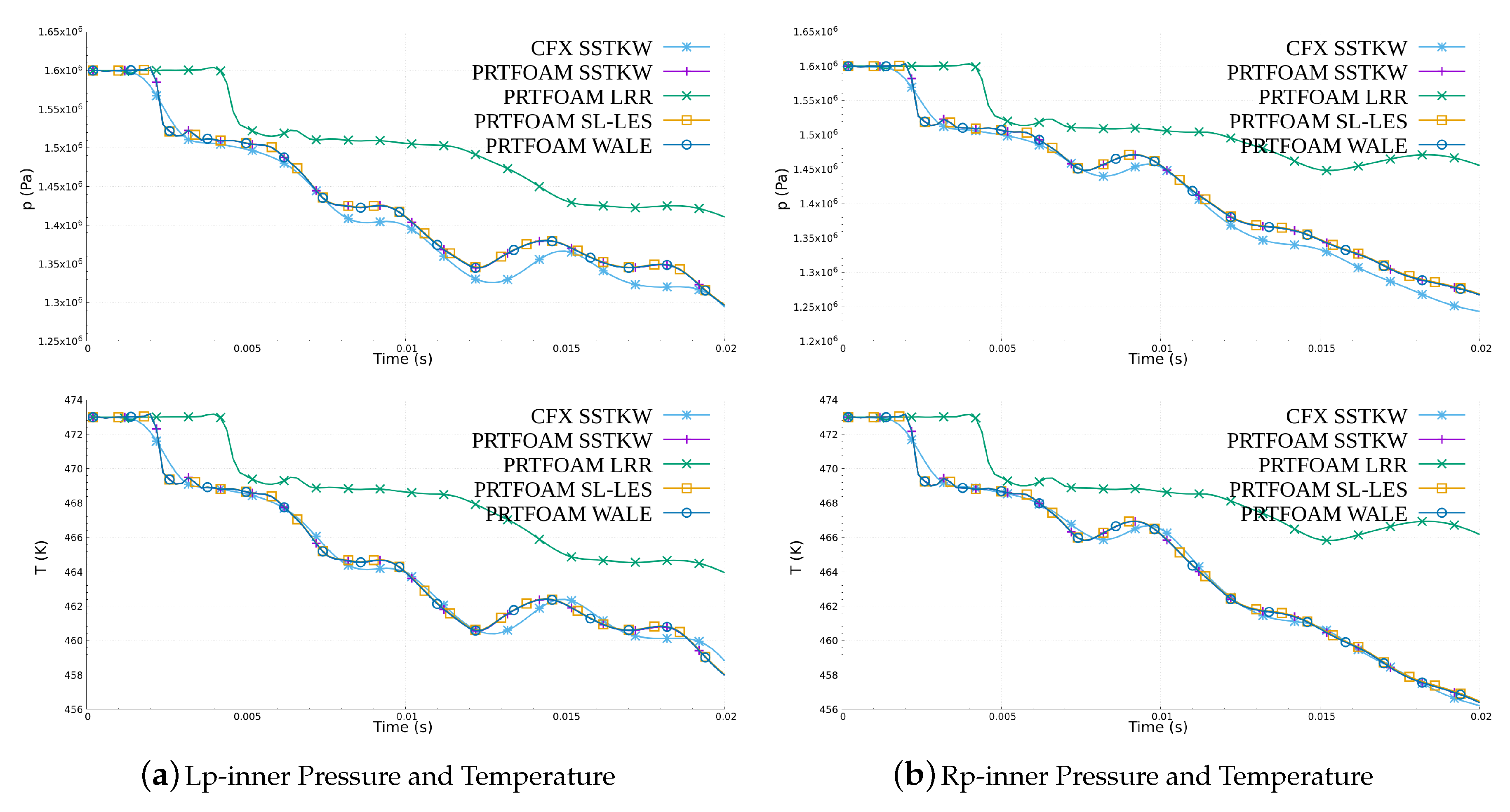


| Parameter * | (m/s) | (kg/m·s) | (kg/m3) | (m2/s2) | (m2/s3) | (s−1) |
|---|---|---|---|---|---|---|
| Value | (0.6, 0, 0) | 1.6 × 10−5 | 1.04 | 0.0017 | 0.0016 | 10.7539 |
| Parameter * | molWeight (kg/mol) | (K) | (m2/s3) | (m2/s2) | (s−1) | |||
|---|---|---|---|---|---|---|---|---|
| Value | 18.02 | 273 | 1.5 | 4.79 | 35.49 | 1 × 10−5 | 3011 | 0 |
| Models | Drag Coefficient | Strouhal Number * | ||
|---|---|---|---|---|
| PRTFOAM | CFX | PRTFOAM | CFX | |
| SSTKW | 1.3291 | 0.9611 | 0.2063 (1.8%) | 0.2160 (2.9%) |
| LRR | 1.2195 | 0.2341 | 0.2137 (1.8%) | - |
| SL-LES | 1.4298 | 1.1974 | 0.2116 (0.8%) | 0.1970 (6.2%) |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, S.; Guo, X.-W.; Li, C.; Liu, Y.; Zhao, R.; Yang, C. Numerical Study of the Turbulent Flow from a Steam Dumping Pressurizer Relief Tank. Energies 2020, 13, 4059. https://doi.org/10.3390/en13164059
Zhang S, Guo X-W, Li C, Liu Y, Zhao R, Yang C. Numerical Study of the Turbulent Flow from a Steam Dumping Pressurizer Relief Tank. Energies. 2020; 13(16):4059. https://doi.org/10.3390/en13164059
Chicago/Turabian StyleZhang, Sen, Xiao-Wei Guo, Chao Li, Yi Liu, Ran Zhao, and Canqun Yang. 2020. "Numerical Study of the Turbulent Flow from a Steam Dumping Pressurizer Relief Tank" Energies 13, no. 16: 4059. https://doi.org/10.3390/en13164059
APA StyleZhang, S., Guo, X.-W., Li, C., Liu, Y., Zhao, R., & Yang, C. (2020). Numerical Study of the Turbulent Flow from a Steam Dumping Pressurizer Relief Tank. Energies, 13(16), 4059. https://doi.org/10.3390/en13164059





