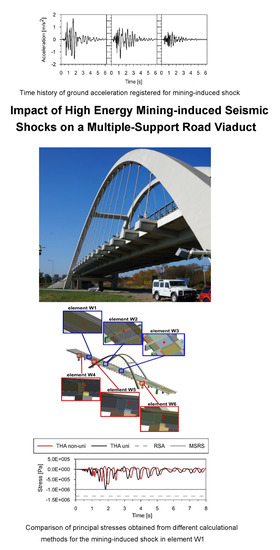
Impact of High Energy Mining-Induced Seismic Shocks from Different Mining Activity Regions on a Multiple-Support Road Viaduct
Abstract
:1. Introduction
- Proposing the models of non-uniform kinematic excitation of mining origin;
- Adopting the multiple-support response spectrum method for assessing the dynamic performance of a multiple-support structure under mining-triggered shock; and
- Performing comprehensive research on the dynamic performance of a multiple-support structure exposed to non-uniform kinematic excitations of mining origin.
2. Materials and Methods
2.1. Non-Uniformity of Kinematic Excitation of Multiple-Support Structures
2.2. Data on the Mining-Induces Shocks Registered in the Selected Mining Activity Regions
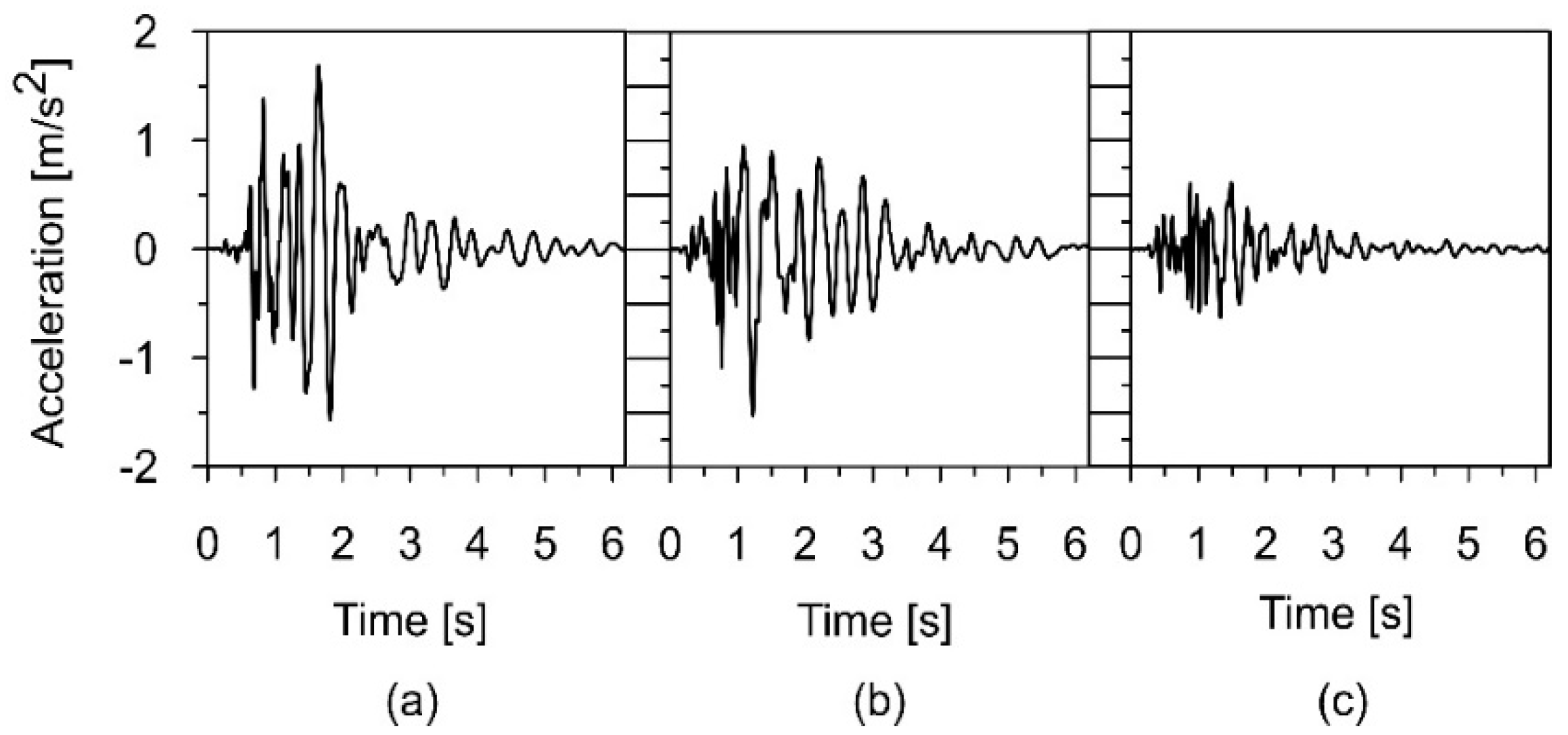
2.2.1. The Mining-Induced Shock Registered in the Upper Silesian Coal Basin
2.2.2. The Mining-Induced Shock Registered in the Legnica-Glogow Copper District
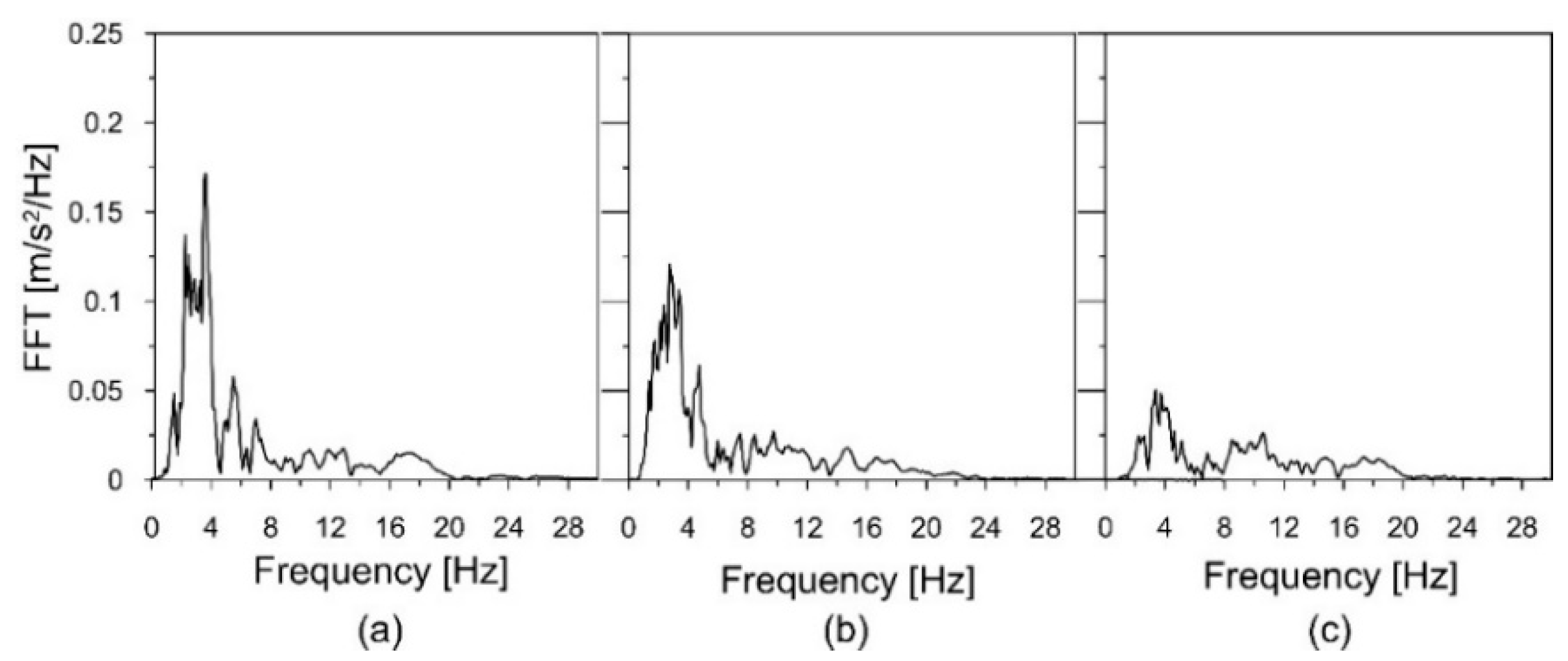
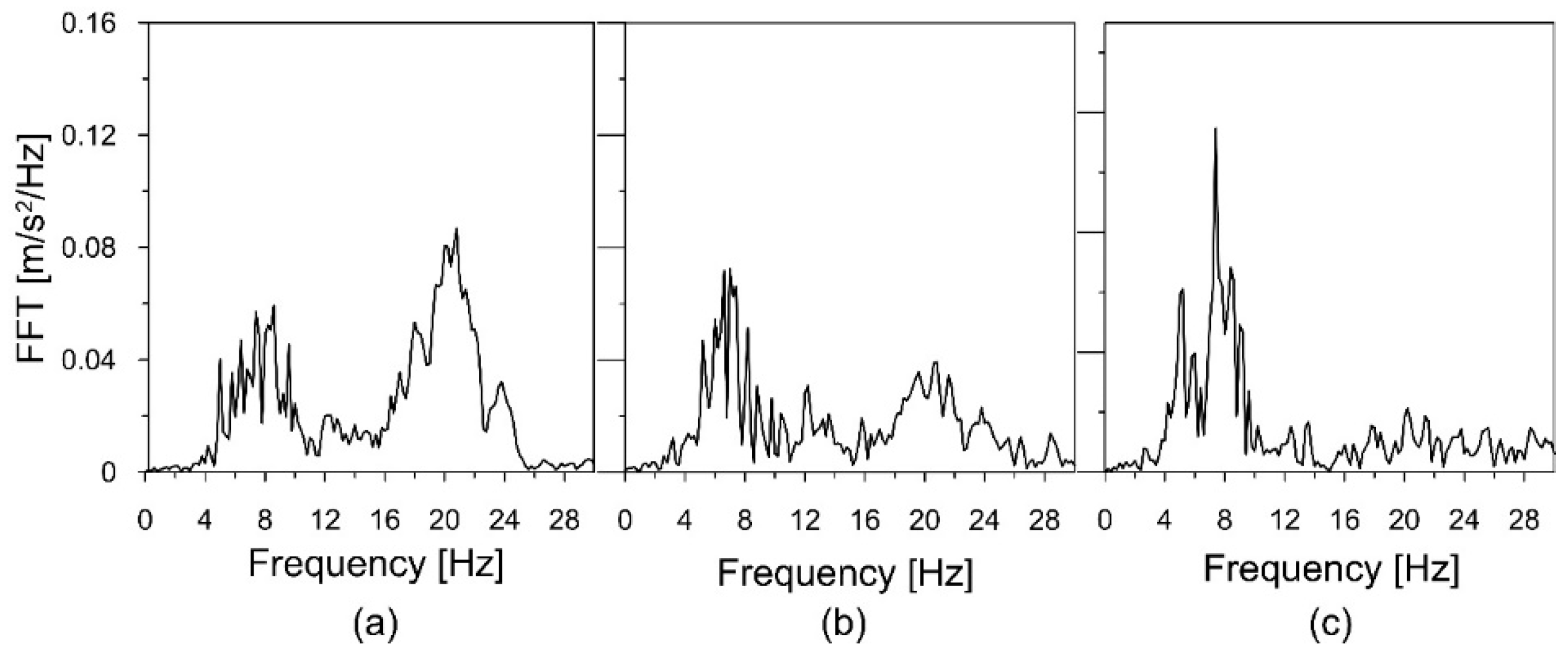
2.2.3. The Comparison of Frequency Spectra of the Selected Shocks
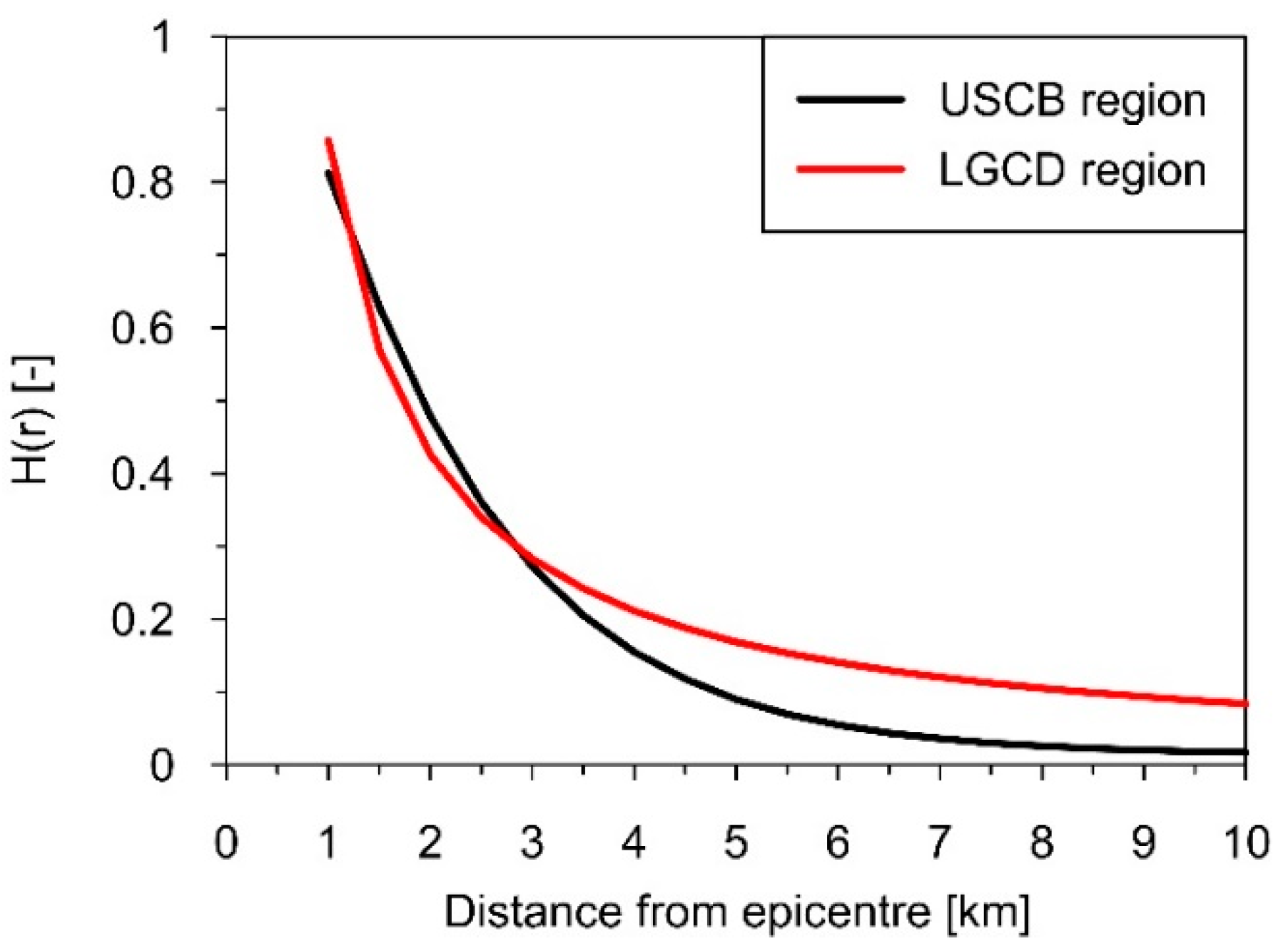
2.2.4. Amplitude Decay with Distance for the Selected Mining Activity Regions
2.3. Calculation Methods of the Dynamic Response of a Structure to Kinematic Excitation
2.3.1. Time History Analysis (THA)
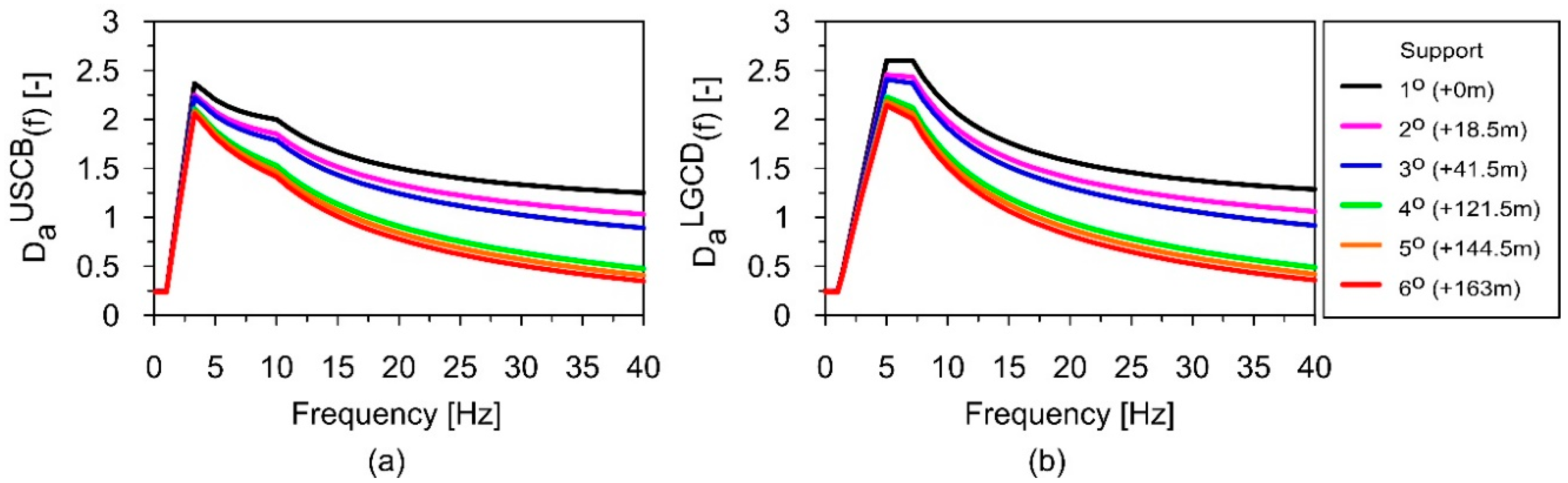
2.3.2. Response Spectrum Analysis (RSA) with Spectral Curves for the Selected Mining Regions
2.3.3. Multiple Support Response Spectrum (MSRS) Analysis
2.3.4. Comparison of the Introduced Calculational Methods of the Dynamic Behavior of Structures
- The THA is more precise than methods based on the modal analysis (RSA and MSRS). It allows for finding the time-histories of structure response measures (stresses, strains, displacements) at any element. It also enables incorporating different problem nonlinearities like complex material behavior, large displacements or strains, or unilateral contact conditions.
- The methods based on system eigenmodes are much more cost-effective than direct time integration of all the degrees of freedom of the system in the dynamic analysis performed with THA.
- Taking into account the non-uniformity of ground motion, both in THA or by adopting MSRS method may play an important role in the analysis of the dynamic response of large-scale structures to seismic shocks. However, one should bear in mind that the correct definition of the non-uniform kinematic excitation model requires the knowledge of local soil conditions and in situ tests. Such a model for the current analysis is presented in Section 3.2.
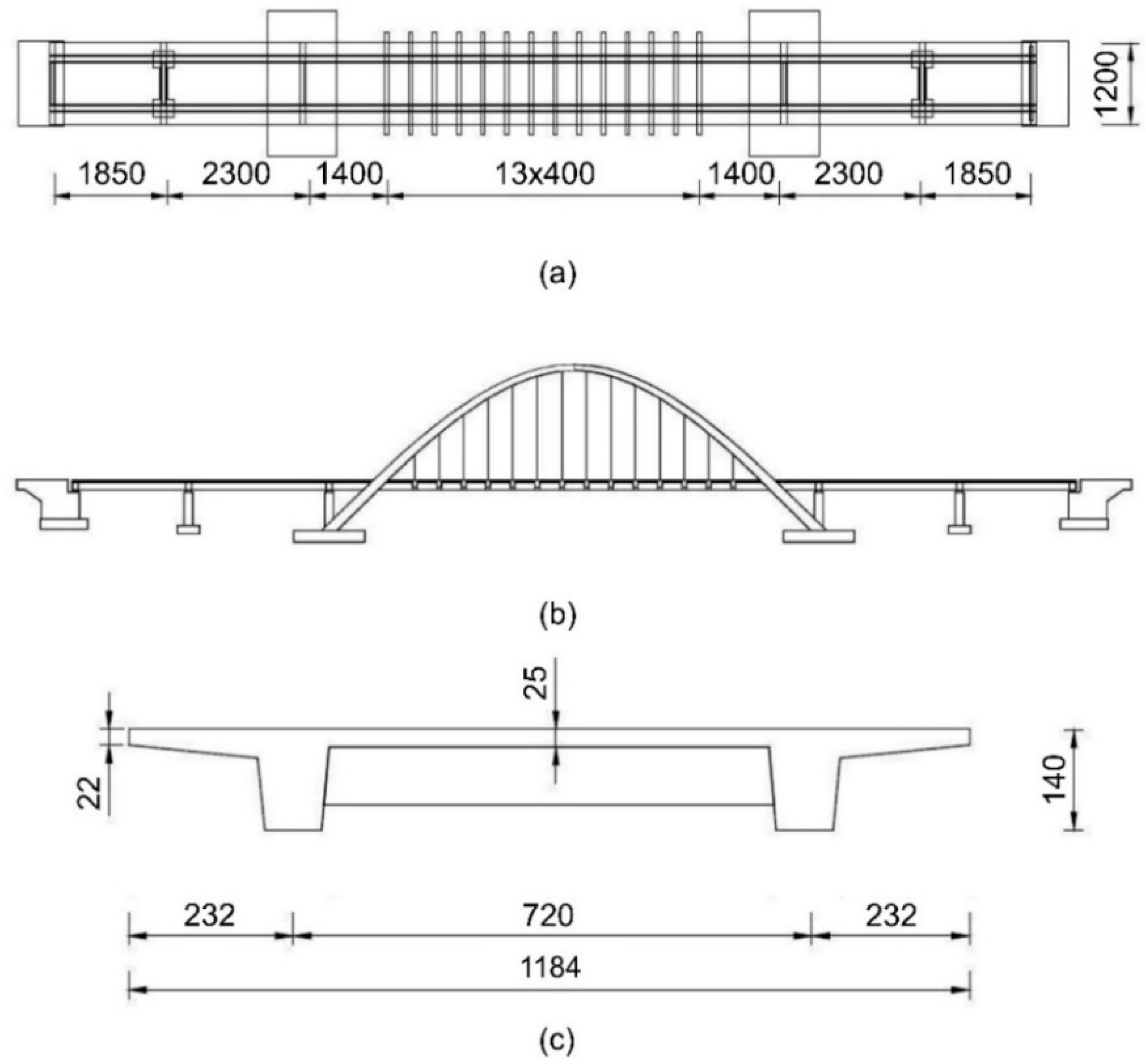
2.4. The Description of the Analyzed Road Viaduct
2.4.1. Structural Layout and Material Data of the Viaduct
2.4.2. The Numerical Model of the Viaduct
3. Results
3.1. The Natural Frequencies and Modes of Vibration of the Viaduct
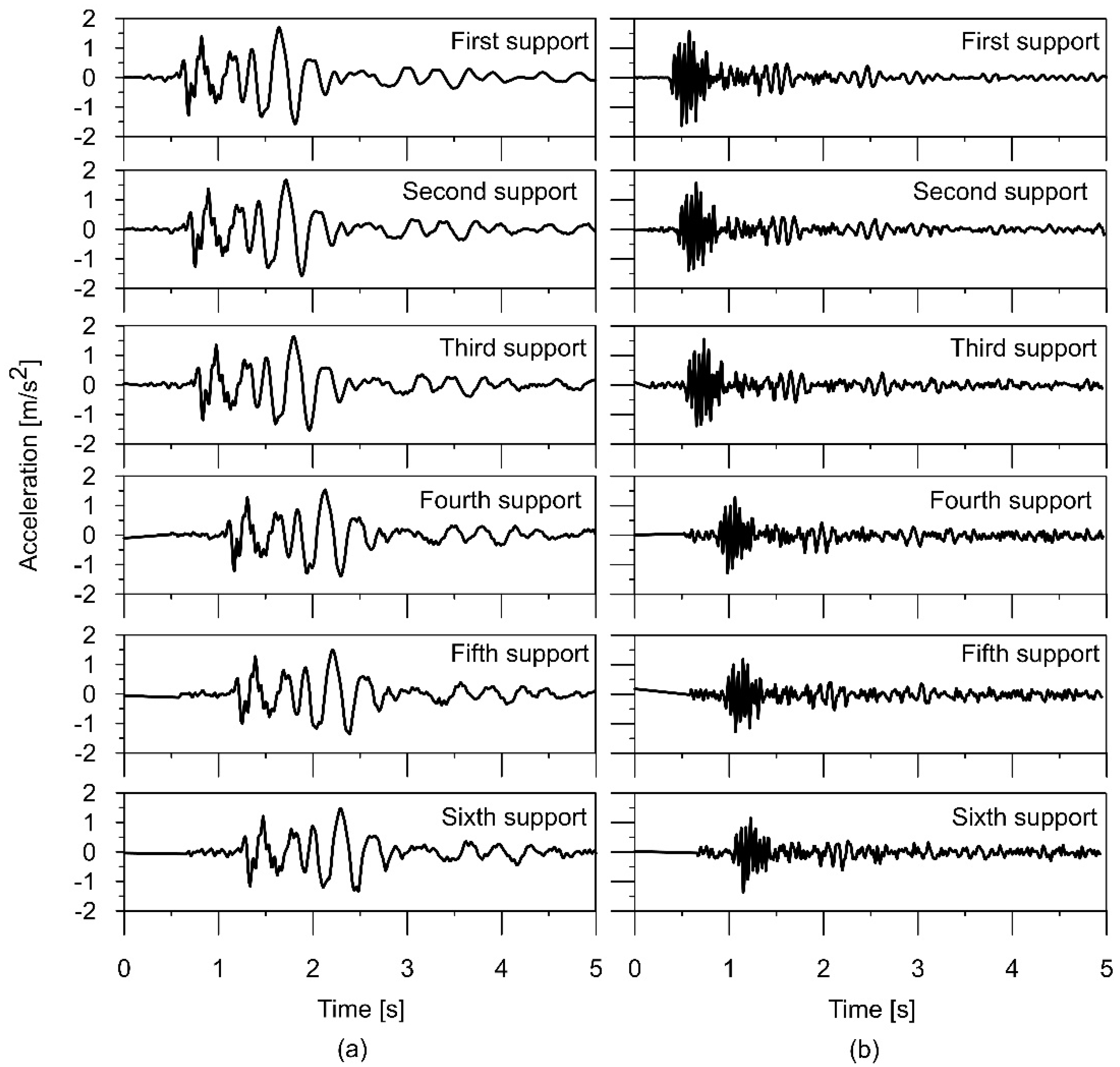
3.2. The Proposed Model of Non-Uniform Mining-Induces Excitation Used for the Time History Analysis
3.2.1. The Effect of Incoherence
3.2.2. The Wave Passage Effect
3.2.3. The Attenuation Effect
3.2.4. Resultant Data of the Non-Uniform Mining-Induced Excitation for the Selected Mining Regions
3.3. Adoption of the Multiple-Support Response Spectrum (MSRS) Method for the Assessment of the Dynamic Performance of the Viaduct under Mining-Induced Excitations
4. Discussion
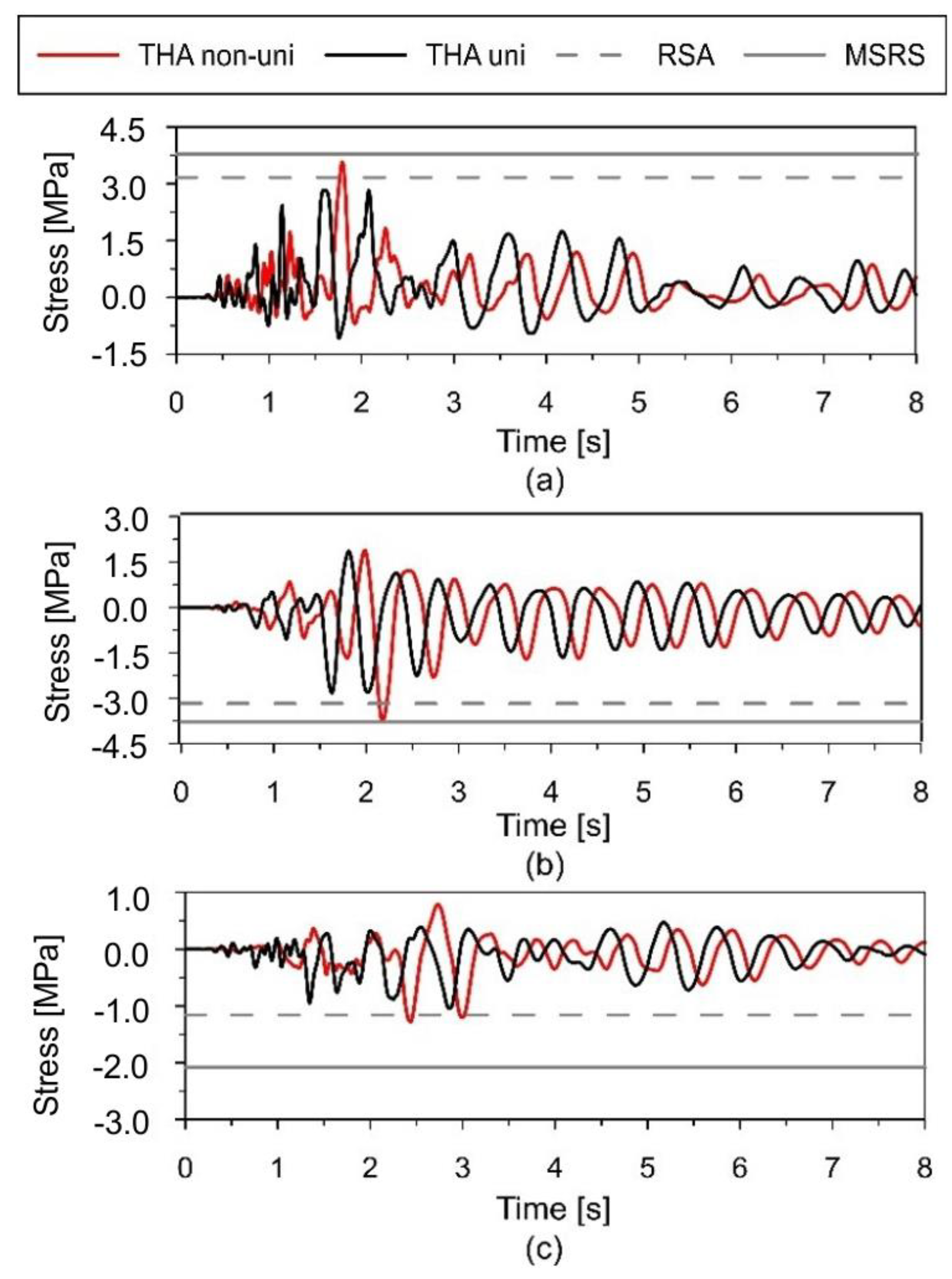
4.1. Comparative Analysis of the Dynamic Responses of the Viaduct to Mining-Induced Shocks
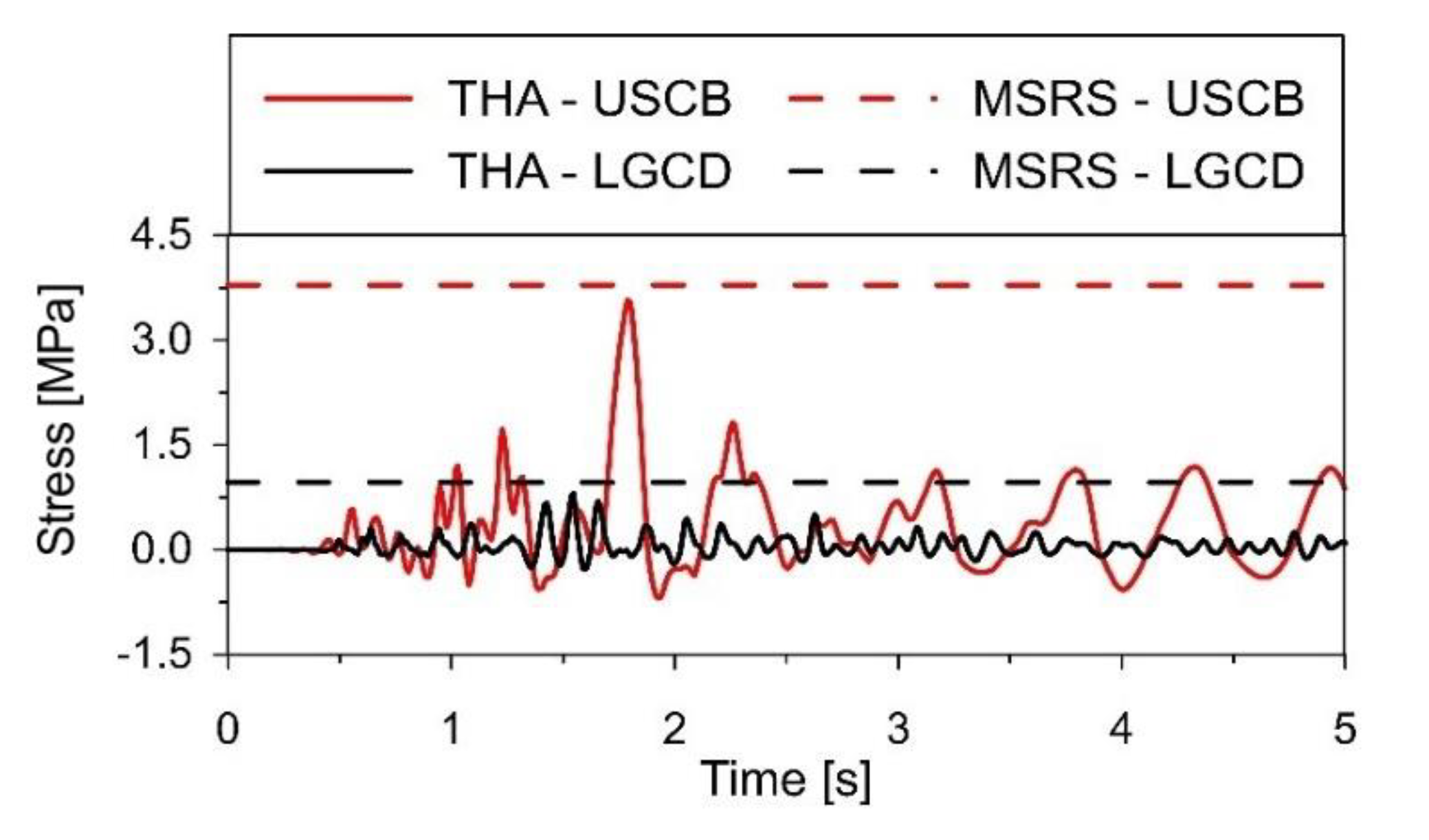
4.2. Assessment of the Dynamic Performance of the Viaduct Subjected to the Mining-Induced Events from Different Mining Activity Regions
5. Conclusions
- The dynamic response of some parts of the structure, like the support zones, obtained using the non-uniform excitation model was meaningfully greater than that calculated for the uniform excitation. The research proved that some members of a structure may be strongly affected by pseudo-static effects resulting from the non-uniformity of kinematic excitation. Hence, in the assessment of the dynamic response of large multiple-support structures to mining-induced events, the effect of spatial variation of ground motion seems to be vital and should be accounted for.
- The commonly used RSA method may lead to an underestimation of the dynamic response of a structure to non-uniform mining-induced kinematic excitation. Hence, as nonconservative, the RSA cannot be recommended as an appropriate method in determining the dynamic response of large-scale structures that undergo non-uniform mining-induced excitation.
- The performed investigation demonstrated that the dynamic response obtained from the multiple support response spectrum analysis was greater than the response received from the THA analyses. The MSRS method, which takes into account the non-uniformity of ground motion, is a conservative approximation and provides a safe upper estimation of the full-dynamic analysis results of large-scale structures under mining-induced tremors.
- In the dynamic analyses of multiple-support structures subjected to spatially varying ground motion, an adequate model of non-uniform mining-induced kinematic excitation should be applied, taking into account features characterizing events arising in mining zones. In the proposed models the main aspects of ground motion non-uniformity, specific for quakes of mining origin, were accounted for. The parameters of the models, like the velocity of seismic wave propagation, coherence, and attenuation functions, should be obtained experimentally for the local soil condition in mining activity zones.
- Mining activity regions usually differ in geological structure and topography, which results in discrepancies in frequency contents of mining-induced shocks in these zones. The presented research indicated that the dynamic performance of a structure strongly depends on a frequency range attributed to the specific mining region. The dynamic performance of identical engineering structures located in various mining regions under tremors of similar maximal amplitudes may differ significantly, due to the dissimilarity of frequency contents.
Author Contributions
Funding
Conflicts of Interest
References
- Cai, Y.; Li, X.; Xiao, W.; Zhang, W. Simulation of mining-induced ground damage using orthogonal experiments to determine key parameters of super-large coalface: A case study in Shendong Coalfield in China. Appl. Sci. 2020, 10, 2258. [Google Scholar] [CrossRef] [Green Version]
- Zhironkin, S.; Selyukov, A.; Gasanov, M. Parameters of transition from deepening longitudinal to continuous lateral surface mining methods to decrease environmental damage in coal clusters. Energies 2020, 13, 3305. [Google Scholar] [CrossRef]
- Cehlár, M.; Janočko, J.; Šimková, Z.; Pavlik, T.; Tyulenev, M.; Zhironkin, S.; Gasanov, M. Mine sited after mine activity: The Brownfields Methodology and Kuzbass Coal Mining case. Resources 2019, 8, 21. [Google Scholar] [CrossRef] [Green Version]
- Pachla, F. Influence of Mining Shocks on Surface Structures; Cracow University of Technology Publishing House: Cracow, Poland, 2019. (In Polish) [Google Scholar]
- Cai, Y.; Verdel, T.; Deck, O. Using plane frame structural models to assess building damage at a large scale in a mining subsidence area. Eur. J. Environ. Civ. Eng. 2020, 24, 283–306. [Google Scholar] [CrossRef]
- Franza, A.; Deck, O.; DeJong, M.J. Charts for the mining-induced deflection of buildings. Can. Geotech. J. 2020, 1–7. [Google Scholar] [CrossRef]
- Liu, Z.; Cui, B.; Liang, Y.; Guo, H.; Li, Y. Study on foundation deformation of buildings in mining subsidence area and surface subsidence prediction. Geotech. Geol. Eng. 2019, 37, 1755–1764. [Google Scholar] [CrossRef]
- Foulger, G.; Wilson, M.; Gluyas, J.; Julian, B.; Davies, R. Global review of human-induced earthquakes. Earth-Sci. Rev. 2018, 178, 438–514. [Google Scholar] [CrossRef] [Green Version]
- Kuzniar, K.; Stec, K.; Tatara, T. Comparison of approximate assessments of the harmfulness of mining shocks using ground and building foundation vibrations. J. Meas. Eng. 2018, 6, 218–225. [Google Scholar] [CrossRef]
- Tatara, T. An Influence of Surface Mining-Related Vibration on Low-Rise Buildings; Scientific Notebooks of Cracow University of Technology: Cracow, Poland, 2002. (In Polish) [Google Scholar]
- Salajka, V.; Kalab, Z.; Kala, J.; Hradil, P. Response of the residential buildings structure on load technical seismicity due to mining activities. World Acad. Sci. Eng. Technol. 2009, 50, 61–69. [Google Scholar]
- Malinowska, A.; Hejmanowski, R. Building damage risk assessment on mining terrains in Poland with GIS application. Int. J. Rock. Mech. Min. 2010, 47, 238–245. [Google Scholar] [CrossRef]
- Pachla, F.; Tatara, T. Dynamic resistance of residential masonry building with structural irregularities. In Seismic Behaviour and Design of Irregular and Complex Civil Structures III. Geotechnical, Geological and Earthquake Engineering; Springer: Berlin/Heidelberg, Germany, 2020; Volume 48. [Google Scholar]
- EN 1998-1:2004. Eurocode 8: Design of Structures for Earthquake Resistance–Part 1: General Rules, Seismic Actions and Rules for Buildings; CEN: Brussels, Belgium, 2005. [Google Scholar]
- EN 1998-2. Eurocode 8: Design of Structures for Earthquake Resistance—Part 2: Bridges; CEN: Brussels, Belgium, 2005. [Google Scholar]
- PN-B-02170:2016. Assessment of the Harmfulness of Vibrations Transmitted through Ground to Buildings; Polish Standard. (In Polish); Polish Committee for Standardization: Warsaw, Poland, 2016.
- Pachla, F. The impact of predicted vibrations from mining shocks on the viaduct–case study. Vib. Proced. 2019, 23, 93–98. [Google Scholar] [CrossRef] [Green Version]
- Ayuddin, A. Global structural analysis of high-rise hospital building using earthquake resistant design approach. Sinergi 2020, 24, 95–108. [Google Scholar] [CrossRef]
- Świdziński, W.; Korzec, A.; Woźniczko, K. Stability analysis of Żelazny Most tailings dam loaded by mining-induced earthquakes. In Seismic Behaviour and Design of Irregular and Complex Civil Structures II, Geotechnical, Geological and Earthquake Engineering; Springer: Berlin/Heidelberg, Germany, 2016; Volume 40. [Google Scholar]
- Zerva, A. Spatial Variation of Seismic Ground Motions: Modeling and Engineering Applications; CRC Press/Balkema–Taylor & Francis Group: Boca Raton, FL, USA, 2009. [Google Scholar]
- Lupoi, G.; Franchin, P.; Lupoi, A.; Pinto, P. Seismic fragility analysis of structural system. In Proceedings of the 13th World Conference on Earthquake Engineering, Vancouver, BC, Canada, 1–6 August 2004. Paper No. 4008. [Google Scholar]
- Zong, Z.; Zhou, R.; Huang, X. Seismic response study on a multi-span cable-stayed bridge scale model under multi-support excitations. Part I: Shaking table tests. J. Zheijang Univ.-Sci. 2014, 15, 351–363. [Google Scholar] [CrossRef] [Green Version]
- Leger, P.; Idé, M.I.; Paultre, P. Multiple-support seismic analysis of large structures. Comput. Struct. 1990, 36, 1153–1158. [Google Scholar] [CrossRef]
- Burdette, N.J.; Elnashai, A.S.; Lupoi, A.; Sextos, A.G. Effect of asynchronous earthquake motion on complex bridges. I: Methodology and input motion. J. Bridge Eng. 2008, 13, 158–165. [Google Scholar] [CrossRef]
- Sextos, A.G.; Kappos, A.J. Evaluation of seismic response of bridges under asynchronous excitation and comparisons with Eurocode 8–2 provisions. Bull. Earthq. Eng. 2009, 7, 519–545. [Google Scholar] [CrossRef]
- Zembaty, Z. Vibrations of bridge structure under kinematic wave excitations. J. Struct. Eng. 1997, 123, 479–487. [Google Scholar] [CrossRef]
- Sextos, A.G.; Karakostas, C.; Lekidis, V.; Papadopoulos, S. Multiple support seismic excitation of the Evripos bridge based on free-field and on-structure recordings. Struct. Infrastruct. Eng. 2015, 11, 1510–1523. [Google Scholar] [CrossRef]
- Drygala, I.J.; Dulinska, J.M.; Polak, M.A. Seismic assessment of footbridges under spatial variation of earthquake ground motion (SVEGM): Experimental testing and finite element analyses. Sensors 2020, 20, 1227. [Google Scholar] [CrossRef] [Green Version]
- Miao, Y.; Yao, E.; Ruan, B.; Zhuang, H. Seismic response of shield tunnel subjected to spatially varying earthquake ground motions. Tunn. Undergr. Space Technol. 2018, 77, 216–226. [Google Scholar] [CrossRef]
- Davoodii, M.; Jafari, M.K.A.; Sadrolddini, S.M. Effect of multi support excitation on seismic response of embankment dams. Int. J. Civ. Eng. 2013, 11, 19–28. [Google Scholar]
- Akbari, M.; Hariri-Ardebili, M.; Mirzabozorg, H. Nonlinear response of high arch dams to nonuniform seismic excitation considering joint effects. J. Eng. 2013, 2013, 912830. [Google Scholar] [CrossRef] [Green Version]
- Tsinidis, G.; Di Sarno, L.; Sextos, A.; Furtner, P. A critical review on the vulnerability assessment of natural gas pipelines subjected to seismic wave propagation. Part 2: Pipe analysis aspects. Tunn. Undergr. Space Technol. 2019, 92, 103056. [Google Scholar] [CrossRef]
- Dulinska, J.M. Cooling tower shell under asynchronous kinematic excitation using concrete damaged plasticity model. Key Eng. Mater. 2013, 535–536, 469–472. [Google Scholar] [CrossRef]
- Boroń, P.; Dulińska, J.M. Assessing the dynamic response of a steel pipeline to a strong vertical mining tremor using the multiple support response spectrum method. Trans. Tech. 2019, 2, 97–108. [Google Scholar] [CrossRef]
- Burkacki, D.; Wójcik, M.; Jankowski, R. Numerical investigation on behaviour of cylindrical steel tanks during mining tremors and moderate earthquakes. Earthq. Struct. 2020, 18, 97–111. [Google Scholar]
- Der Kiureghian, A.; Neuenhofer, A. Response spectrum method for multi-support seismic excitations. Earthq. Eng. Struct. Dyn. 1992, 8, 713–740. [Google Scholar] [CrossRef]
- Savor Novak, M.; Lazarevic, D.; Atalic, J.; Uros, M. Influence of multiple-support excitation on seismic response of reinforced concrete arch bridges. Appl. Sci. 2020, 10, 17. [Google Scholar] [CrossRef] [Green Version]
- Shen, J.; Li, R.; Shi, J.; Zhou, G. Modified Multi-Support Response Spectrum Analysis of structures with multiple supports under incoherent ground excitation. Appl. Sci. 2019, 9, 1744. [Google Scholar] [CrossRef] [Green Version]
- Mutke, G.; Stec, K. Seismicity in the Upper Silesian Coal Basin, Poland: Strong regional seismic events. In Rockbursts and Seismicity in the Mines; A.A.Balkema: Rotterdam/Brookfield, The Netherlands, 1997; pp. 213–219. [Google Scholar]
- Chopra, A.K. Dynamics of Structures, 4th ed.; University of California at Berkeley: Berkeley, CA, USA, 2012; Pearson. [Google Scholar]
- Czerwionka, L.; Tatara, T. Standard response spectra from chosen mining regions at Upper Silesian Coalfield. Trans. Tech. 2007, 2–B, 11–18. [Google Scholar]
- Workbench User’s Guide. Release 18.2; ANSYS, Inc: Canonsburg, PA, USA, 2017.
- Sipple, J.; Sanayei, M. Full-Scale Bridge Finite-Element Model Calibration Using Measured Frequency-Response Functions. J. Bridge Eng. 2014, 20, 04014103. [Google Scholar] [CrossRef] [Green Version]
- Bednarski, Ł.; Sieńko, R.; Howiacki, T. Estimation of the value and the variability of elastic modulus of concrete in existing structure on the basis of continuous in situ measurements. Cem. Wapno Beton 2014, 6, 396–404. [Google Scholar]
- Boron, P. Dynamic Response Analysis of the Multi-Support Structure to Mining Shocks Using Multiple Support Response Spectrum. Ph.D. Thesis, Cracow University of Technology, Cracow, Poland, June 2019. (In Polish). [Google Scholar]
- Van Overshee, P.; de Moor, B. Subspace Identification for Linear System–Theory–Implementation–Applications; Kluwer Academic Publishers: Boston, MA, USA, 1996. [Google Scholar]
- Daniotti, N.; Cheynet, E.; Jakobsen, J.B.; Snæbjörnsson, J. Damping estimation from full-scale traffic-induced vibrations of a suspension bridge. J. Comput. Civ. Eng. 2019, 2019, 171–179. [Google Scholar]
- Ewins, D.J. Modal Testing: Theory, Practice and Application, 2nd ed.; Research Studies Press Ltd.: Philadelphia, PA, USA, 2000. [Google Scholar]
- Jankowski, R. Numerical simulation of space-time conditional random fields of ground motions. Comput. Sci. 2006, 3993, 56–59. [Google Scholar]
- Jankowski, R.; Wilde, K. A simple method of conditional random field simulation of ground motions for long structures. Eng. Struct. 2000, 22, 552–561. [Google Scholar] [CrossRef]
- Vanmarcke, E.; Fenton, G. Conditioned simulation of local fields of earthquake ground motion. Struct. Saf. 1991, 10, 247–264. [Google Scholar] [CrossRef]







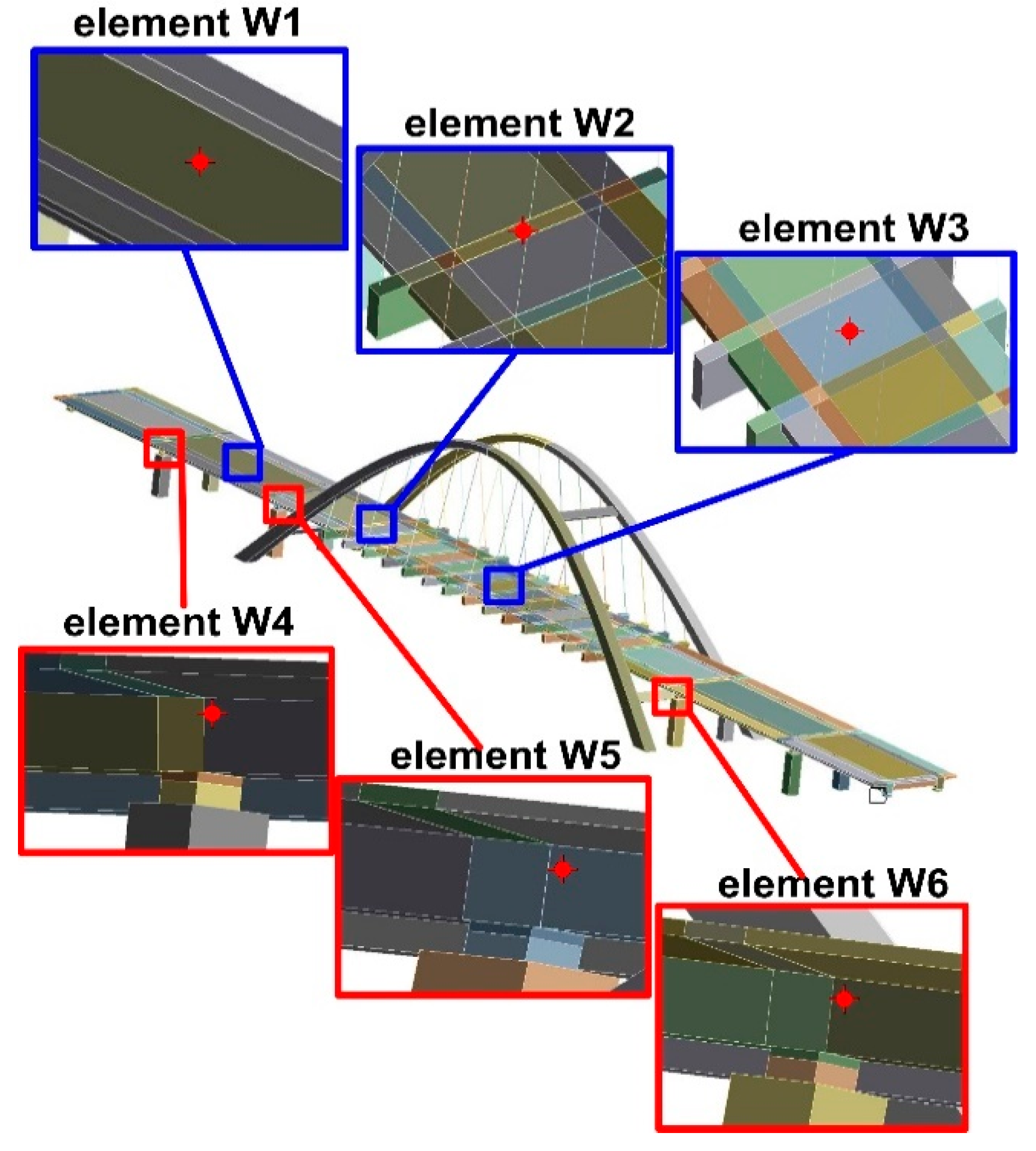



| Element | Ρ (kg/m3) | E (GPa) | Υ (-) |
|---|---|---|---|
| Concrete (supports) | 2600 | 32 | 0.15 |
| Concrete (arch) | 2600 | 37 | 0.15 |
| Concrete (girder) | 2400 | 42 | 0.15 |
| Structural steel (hangers) | 7800 | 200 | 0.30 |
| Mode | Natural Frequency (Hz) | Differences [%] | ||
|---|---|---|---|---|
| FE Analysis | OMA | |||
| 1 | 1.62 | 1.49 | 8.7 | 0.803 |
| 2 | 2.27 | 2.48 | 8.4 | 0.998 |
| 3 | 3.55 | 3.36 | 5.8 | 0.873 |
| 4 | 14.47 | 14.26 | 1.5 | 0.883 |
| 5 | 16.1 | 14.72 | 9.4 | 0.873 |
| Supports No | 1 | 2 | 3 | 4 | 5 | 6 |
|---|---|---|---|---|---|---|
| Amplitude reduction for USCB region | 1.00 | 0.991 | 0.983 | 0.942 | 0.936 | 0.928 |
| Amplitude reduction for LGCB region | 1.00 | 0.997 | 0.991 | 0.972 | 0.967 | 0.963 |
| Element | Maximum Stress Obtained for Region (MPa): | Stress Ratio (-) | |
|---|---|---|---|
| USCB | LGCD | ||
| W1 | 1.00 | 0.27 | 3.7 |
| W2 | 0.80 | 0.17 | 5.9 |
| W3 | 1.27 | 0.32 | 4.0 |
| W4 | 3.85 | 0.97 | 4.0 |
| W5 | 3.80 | 0.65 | 5.5 |
| W6 | 2.09 | 0.46 | 4.5 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Boroń, P.; Dulińska, J.M.; Jasińska, D. Impact of High Energy Mining-Induced Seismic Shocks from Different Mining Activity Regions on a Multiple-Support Road Viaduct. Energies 2020, 13, 4045. https://doi.org/10.3390/en13164045
Boroń P, Dulińska JM, Jasińska D. Impact of High Energy Mining-Induced Seismic Shocks from Different Mining Activity Regions on a Multiple-Support Road Viaduct. Energies. 2020; 13(16):4045. https://doi.org/10.3390/en13164045
Chicago/Turabian StyleBoroń, Paweł, Joanna Maria Dulińska, and Dorota Jasińska. 2020. "Impact of High Energy Mining-Induced Seismic Shocks from Different Mining Activity Regions on a Multiple-Support Road Viaduct" Energies 13, no. 16: 4045. https://doi.org/10.3390/en13164045
APA StyleBoroń, P., Dulińska, J. M., & Jasińska, D. (2020). Impact of High Energy Mining-Induced Seismic Shocks from Different Mining Activity Regions on a Multiple-Support Road Viaduct. Energies, 13(16), 4045. https://doi.org/10.3390/en13164045