Suitability of Sm3+-Substituted SrTiO3 as Anode Materials for Solid Oxide Fuel Cells: A Correlation between Structural and Electrical Properties
Abstract
1. Introduction
2. Materials and Methods
3. Results and Discussion
3.1. Structural Studies
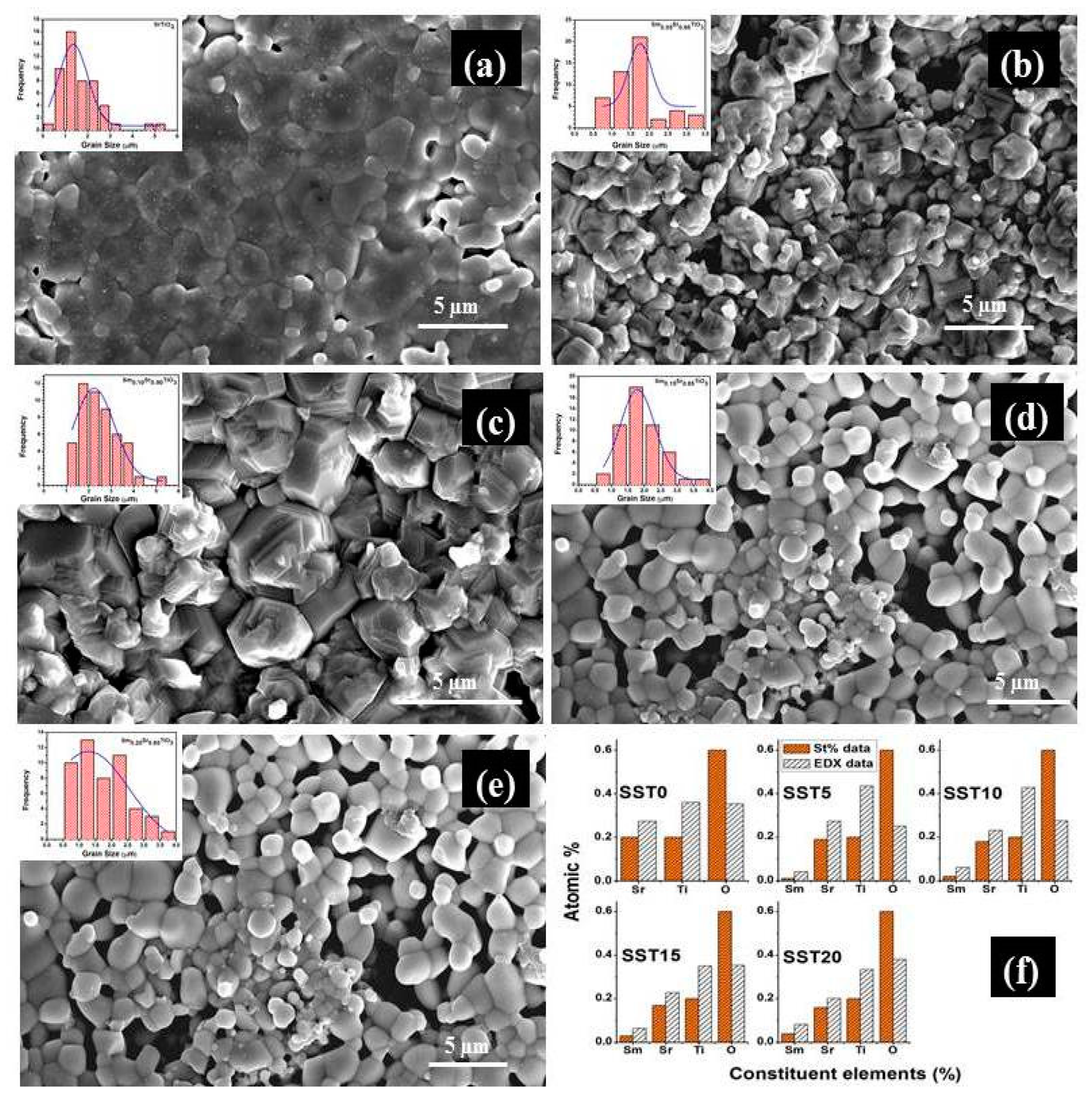
3.2. Microstructural Analysis
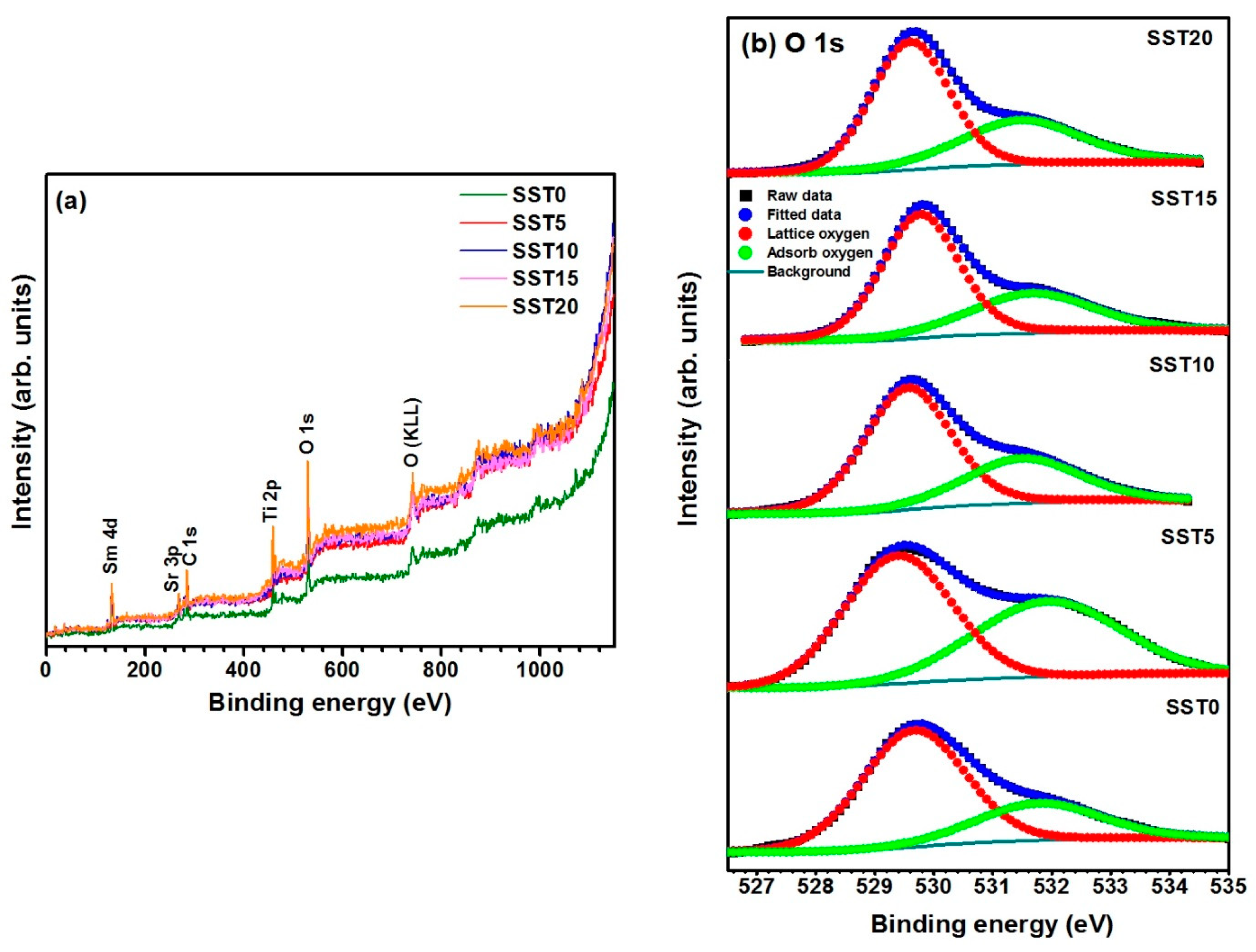
3.3. XPS Analysis
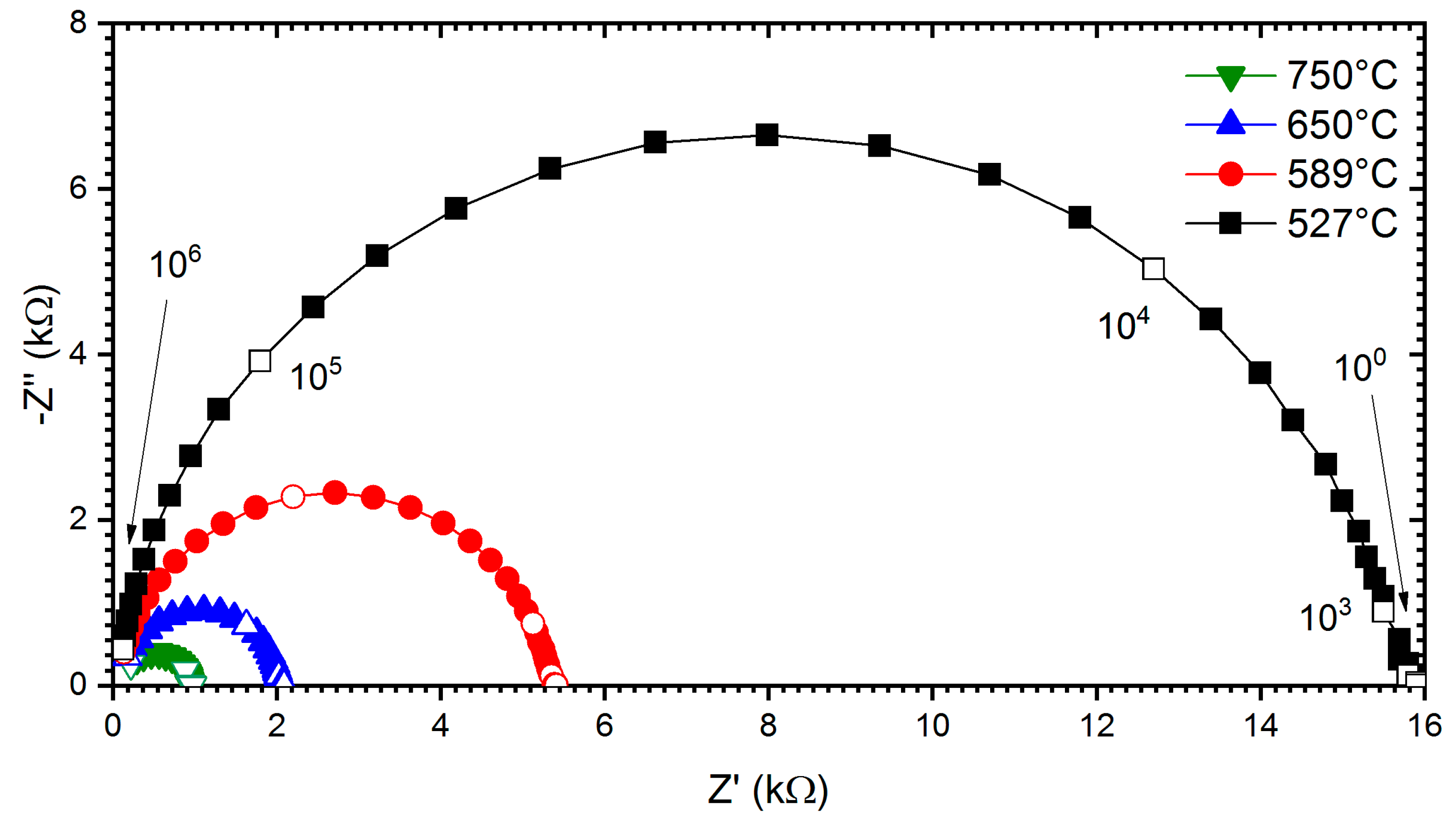
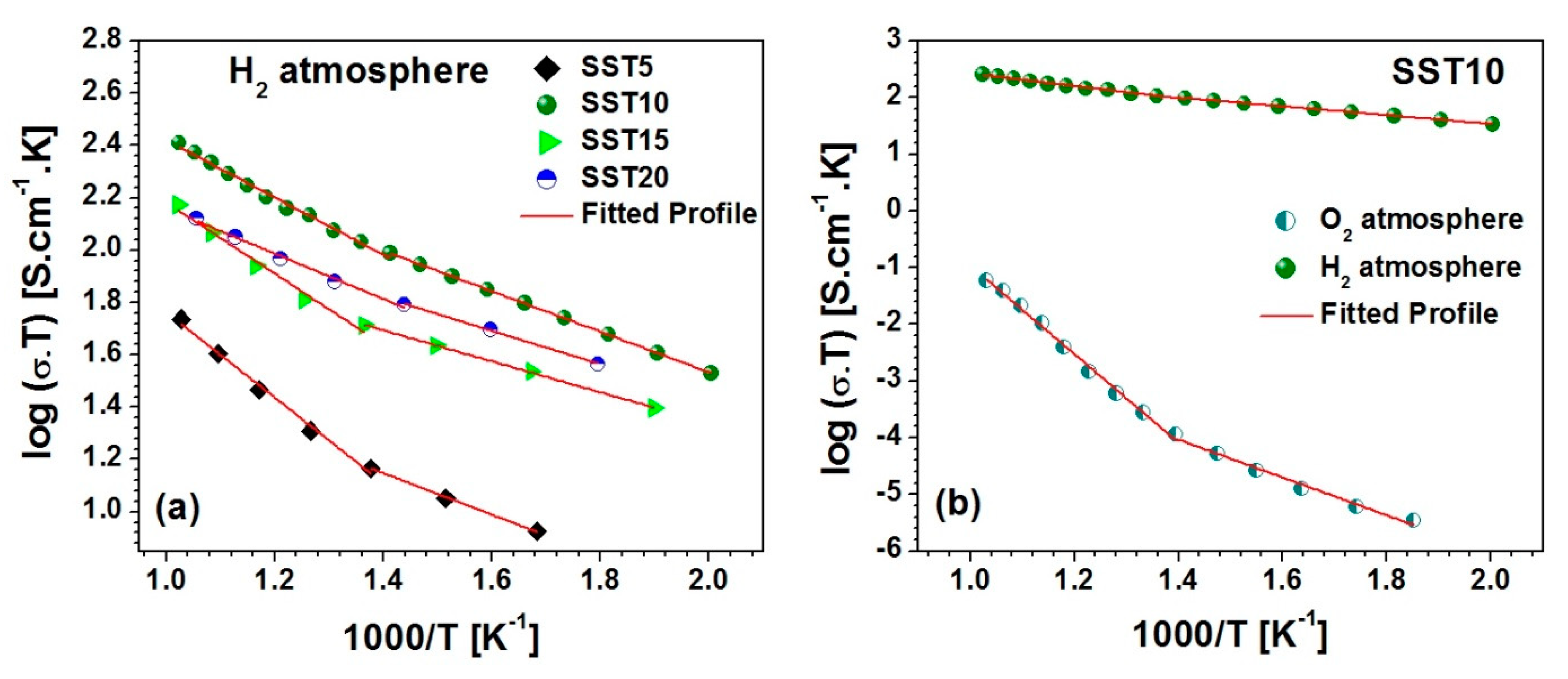
3.4. Electrical Conductivity and Chemical Stability
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- De Lorenzo, G.; Fragiacomo, P. Electrical and thermal analysis of an intermediate temperature IIR-SOFC system fed by biogas. Energy Sci. Eng. 2018, 6, 60–72. [Google Scholar] [CrossRef]
- Fragiacomo, P.; Corigliano, O.; De Lorenzo, G.; Mirandola, F.A. Experimental Activity on a 100-W IT-SOFC Test Bench Fed by Simulated Syngas. J. Energy Eng. 2018, 144, 04018006. [Google Scholar] [CrossRef]
- Lo Faro, M.; Trocino, S.; Zignani, S.C.; Italiano, C.; Vita, A.; Aricò, A.S. Study of a solid oxide fuel cell fed with n-dodecane reformate. Part II: Effect of the reformate composition. Int. J. Hydrog. Energy 2017, 42, 1751–1757. [Google Scholar] [CrossRef]
- Lo Faro, M.; Trocino, S.; Campagna Zignani, S.; Machado Reis, R.; Monforte, G.; Ticianelli, E.A.; Arico, A.S. Ni-based Alloys as Protective Layer for a Conventional Solid Oxide Fuel Cell Fed with Biofuels. ECS Trans. 2015, 68, 2653–2658. [Google Scholar] [CrossRef]
- Yang, C.; Ren, C.; Yu, L.; Jin, C. High performance intermediate temperature micro-tubular SOFCs with Ba0.9Co0.7Fe0.2Nb0.1O3−δ as cathode. Int. J. Hydrog. Energy 2013, 38, 15348–15353. [Google Scholar] [CrossRef]
- Miyake, M.; Matsumoto, S.; Iwami, M.; Nishimoto, S.; Kameshima, Y. Electrochemical performances of Ni1−xCux/SDC cermet anodes for intermediate-temperature SOFCs using syngas fuel. Int. J. Hydrog. Energy 2016, 41, 13625–13631. [Google Scholar] [CrossRef]
- Fragiacomo, P.; De Lorenzo, G.; Corigliano, O. Performance Analysis of an Intermediate Temperature Solid Oxide Electrolyzer Test Bench under a CO2-H2O Feed Stream. Energies 2018, 11, 2276. [Google Scholar] [CrossRef]
- Milewski, J.; Wołowicz, M.; Lewandowski, J. Comparison of SOE/SOFC system configurations for a peak hydrogen power plant. Int. J. Hydrog. Energy 2017, 42, 3498–3509. [Google Scholar] [CrossRef]
- Steele, B.C.H.; Heinzel, A. Materials for fuel-cell technologies. Nature 2001, 414, 345–352. [Google Scholar] [CrossRef]
- Zhang, X.; Liu, L.; Zhao, Z.; Tu, B.; Ou, D.; Cui, D.; Wei, X.; Chen, X.; Cheng, M. Enhanced Oxygen Reduction Activity and Solid Oxide Fuel Cell Performance with a Nanoparticles-Loaded Cathode. Nano Lett. 2015, 15, 1703–1709. [Google Scholar] [CrossRef]
- Da Silva, F.S.; de Souza, T.M. Novel materials for solid oxide fuel cell technologies: A literature review. Int. J. Hydrog. Energy 2017, 42, 26020–26036. [Google Scholar] [CrossRef]
- Rashid, N.; Samat, A.; Jais, A.; Somalu, M.; Muchtar, A.; Baharuddin, N.; Isahak, W. Review on zirconate-cerate-based electrolytes for proton-conducting solid oxide fuel cell. Ceram. Int. 2019, 45, 6605–6615. [Google Scholar] [CrossRef]
- Presto, S.; Barbucci, A.; Viviani, M.; Ilhan, Z.; Ansar, S.A.; Soysal, D.; Thorel, A.; Abreu, J.; Chesnaud, A.; Politova, T.; et al. IDEAL-cell, an innovative dual membrane fuel-cell: Fabrication and electrochemical testing of first prototypes. ECS Trans. 2009, 25, 773–782. [Google Scholar]
- Singh, R.K.; Singh, P. Electrical conductivity of LSGM–YSZ composite materials synthesized via coprecipitation route. J. Mater. Sci. 2014, 49, 5571–5578. [Google Scholar]
- Artini, C.; Carnasciali, M.M.; Viviani, M.; Presto, S.; Plaisier, J.R.; Costa, G.A.; Pani, M. Structural properties of Sm-doped ceria electrolytes at the fuel cell operating temperatures. Solid State Ion. 2018, 315, 85–91. [Google Scholar] [CrossRef]
- Brunaccini, G.; Lo Faro, M.; La Rosa, D.; Antonucci, V.; Aricò, A.S. Investigation of composite Ni-doped perovskite anode catalyst for electrooxidation of hydrogen in solid oxide fuel cell. Int. J. Hydrog. Energy 2008, 33, 3150–3152. [Google Scholar] [CrossRef]
- Presto, S.; Barbucci, A.; Carpanese, M.P.; Viviani, M.; Marazza, R. Electrochemical performance of Ni-based anodes for solid oxide fuel cells. J. Appl. Electrochem. 2009, 39, 2257–2264. [Google Scholar] [CrossRef]
- Li, X.; Zhao, H.; Zhou, X.; Xu, N.; Xie, Z.; Chen, N. Electrical conductivity and structural stability of La-doped SrTiO3 with A-site deficiency as anode materials for solid oxide fuel cells. Int. J. Hydrog. Energy 2010, 35, 7913–7918. [Google Scholar] [CrossRef]
- Verbraeken, M.C.; Ramos, T.; Agersted, K.; Ma, Q.; Savaniu, C.D.; Sudireddy, B.R.; Irvine, J.T.S.; Holthappels, P.; Tietz, F. Modified strontium titanates: From defect chemistry to SOFC anodes. RSC Adv. 2015, 5, 1168–1180. [Google Scholar] [CrossRef]
- Moos, R.; Härdtl, K.H. Electronic transport properties of Sr1−xLaxTiO3 ceramics. J. Appl. Phys. 1996, 80, 393–400. [Google Scholar] [CrossRef]
- Slater, P.R.; Fagg, D.P.; Irvine, J.T.S. Synthesis and electrical characterisation of doped perovskite titanates as potential anode materials for solid oxide fuel cells. J. Mater. Chem. 1997, 7, 2495–2498. [Google Scholar] [CrossRef]
- Hui, S.; Petric, A. Electrical Properties of Yttrium-Doped Strontium Titanate under Reducing Conditions. J. Electrochem. Soc. 2002, 149, J1–J10. [Google Scholar] [CrossRef]
- Li, X.; Zhao, H.; Gao, F.; Zhu, Z.; Chen, N.; Shen, W. Synthesis and electrical properties of Co-doped Y0.08Sr0.92TiO3−δ as a potential SOFC anode. Solid State Ion. 2008, 179, 1588–1592. [Google Scholar] [CrossRef]
- Shannon, R.D. Revised effective ionic radii and systematic studies of interatomic distances in halides and chalcogenides. Acta Crystallogr. Sect. A 1976, 32, 751–767. [Google Scholar] [CrossRef]
- Wang, Y.; Lee, K.H.; Hyuga, H.; Kita, H.; Inaba, K.; Ohta, H.; Koumoto, K. Enhancement of Seebeck coefficient for SrO(SrTiO3)2 by Sm substitution: Crystal symmetry restoration of distorted TiO6 octahedra. Appl. Phys. Lett. 2007, 91, 242102. [Google Scholar] [CrossRef]
- Panahi, S.N.; Samadi, H.; Nemati, A. Effect of Samarium Oxide on the Electrical Conductivity of Plasma-Sprayed SOFC Anodes. JOM 2016, 68, 2569–2573. [Google Scholar] [CrossRef]
- Goldschmidt, V.M. Die Gesetze der Krystallochemie. Die Nat. 1926, 21, 477–485. [Google Scholar] [CrossRef]
- Goldschmidt, V.M. Crystal structure and chemical composition. Ber. Dtsch. Chem. Ges. 1927, 60, 1263–1268. [Google Scholar] [CrossRef]
- Maletic, S.; Popovic, D.; Dojcilovic, J. Dielectric measurements, Raman scattering and surface studies of Sm-doped SrTiO3 single crystal. J. Alloy. Compd. 2010, 496, 388–392. [Google Scholar] [CrossRef]
- Ma, Q.; Tietz, F.; Stöver, D. Nonstoichiometric Y-substituted SrTiO3 materials as anodes for solid oxide fuel cells. Solid State Ion. 2011, 192, 535–539. [Google Scholar] [CrossRef]
- Johnson, D.W.; Cross, L.E.; Hummel, F.A. Dielectric Relaxation in Strontium Titanates Containing Rare–Earth Ions. J. Appl. Phys. 1970, 41, 2828–2833. [Google Scholar] [CrossRef]
- Eror, N.G.; Balachandran, U. Self-compensation in lanthanum-doped strontium titanate. J. Solid State Chem. 1981, 40, 85–91. [Google Scholar] [CrossRef]
- Bamberger, C.E. Homogeneity Ranges of Phases Sr4−xLn2x/3Ti4O12 (Ln = Sm to Lu) and Sr1−yEuy∥TiO3. J. Am. Ceram. Soc. 2005, 80, 1024–1026. [Google Scholar] [CrossRef]
- Shimomura, S.; Wakabayashi, N.; Kuwahara, H.; Tokura, Y. X-Ray Diffuse Scattering due to Polarons in a Colossal Magnetoresistive Manganite. Phys. Rev. Lett. 1999, 83, 4389–4392. [Google Scholar] [CrossRef]
- Ohon, N.; Stepchuk, R.; Blazhivskyi, K.; Vasylechko, L. Structural Behaviour of Solid Solutions in the NdAlO3-SrTiO3 System. Nanoscale Res. Lett. 2017, 12, 148. [Google Scholar] [CrossRef][Green Version]
- Prabhu, Y.T.; Rao, K.V.; Kumar, V.S.S.; Kumari, B.S. X-ray Analysis by Williamson-Hall and Size-Strain Plot Methods of ZnO Nanoparticles with Fuel Variation. World. J. Nano Sci. Eng. 2014, 4, 21–28. [Google Scholar] [CrossRef]
- Mannella, N.; Yang, W.L.; Tanaka, K.; Zhou, X.J.; Zheng, H.; Mitchell, J.F.; Zaanen, J.; Devereaux, T.P.; Nagaosa, N.; Hussain, Z.; et al. Polaron coherence condensation as the mechanism for colossal magnetoresistance in layered manganites. Phys. Rev. B 2007, 76, 233102. [Google Scholar] [CrossRef]
- Nasar, R.S.; Cerqueira, M.; Longo, E.; Varela, J.A. Sintering mechanisms of ZrO2·MgO with addition of TiO2 and CuO. Ceram. Int. 2004, 30, 571–577. [Google Scholar] [CrossRef]
- Singh, P. Influence of Bi2O3 additive on the electrical conductivity of calcia stabilized zirconia solid electrolyte. J. Eur. Ceram. Soc. 2015, 35, 1485–1493. [Google Scholar]
- Kumar, P.; Presto, S.; Sinha, A.S.K.; Varma, S.; Viviani, M.; Singh, P. Effect of samarium (Sm3+) doping on structure and electrical conductivity of double perovskite Sr2NiMoO6 as anode material for SOFC. J. Alloys Compd. 2017, 725, 1123–1129. [Google Scholar] [CrossRef]
- Aziz, S.B.; Woo, T.J.; Kadir, M.F.Z.; Ahmed, H.M. A conceptual review on polymer electrolytes and ion transport models. J. Sci. Adv. Mater. Devices 2018, 3, 1–17. [Google Scholar] [CrossRef]
- Praharaj, S.; Rout, D. Study of electrical properties of Pb(Yb0.5Ta0.5)O3 by impedance spectroscopy. IOP Conf. Ser. Mater. Sci. Eng. 2016, 149, 12181. [Google Scholar] [CrossRef]
- Dhahri, A.; Dhahri, E.; Hlil, E.K. Electrical conductivity and dielectric behaviour of nanocrystalline La0.6Gd0.1Sr0.3Mn0.75Si0.25O3. RSC. Adv. 2018, 8, 9103–9111. [Google Scholar] [CrossRef]
- Jonscher, A.K. Dielectric relaxation in solids. J. Phys. D Appl. Phys. 1999, 32, R57–R70. [Google Scholar] [CrossRef]
- Dwivedi, R.K.; Kumar, D.; Parkash, O. Valence compensated perovskite oxide system Ca1−xLaxTi1−xCrxO3 Part I Structure and dielectric behaviour. J. Mater. Sci. 2001, 36, 3641–3648. [Google Scholar] [CrossRef]
- Peláiz-Barranco, A.; Guerra, J.D.S.; López-Noda, R.; Araújo, E.B. Ionized oxygen vacancy-related electrical conductivity in (Pb1−xLax)(Zr0.90Ti0.10)1−x/4O3 ceramics. J. Phys. D. Appl. Phys. 2008, 41, 215503. [Google Scholar] [CrossRef]
- Ghosh, A.; Pan, A. Scaling of the Conductivity Spectra in Ionic Glasses: Dependence on the Structure. Phys. Rev. Lett. 2000, 84, 2188–2190. [Google Scholar] [CrossRef]
- Sen, S.; Choudhary, R.N.; Pramanik, P. Structural and electrical properties of Ca2+-modified PZT electroceramics. Phys. B Condens. Matter 2007, 387, 56–62. [Google Scholar] [CrossRef]
- Katiliute, R.M.; Seibutas, P.; Ivanov, M.; Grigalaitis, R.; Stanulis, A.; Banys, J.; Kareiva, A. Dielectric and Impedance Spectroscopy of BaSnO3 and Ba2 SnO4. Ferroelectrics 2014, 464, 49–58. [Google Scholar] [CrossRef]
- Liu, J.; Wang, C.L.; Li, Y.; Su, W.B.; Zhu, Y.H.; Li, J.C.; Mei, L.M. Influence of rare earth doping on thermoelectric properties of SrTiO3 ceramics. J. Appl. Phys. 2013, 114, 223714. [Google Scholar] [CrossRef]
- Li, G.; Liu, H.; Wang, Z.; Hao, H.; Yao, Z.; Cao, M.; Yu, Z. Dielectric properties and relaxation behaviour of Sm substituted SrTiO3 ceramics. J. Mater. Sci. Mater. Electron. 2014, 25, 4418–4424. [Google Scholar] [CrossRef]
- Gao, F.; Zhao, H.; Li, X.; Cheng, Y.; Zhou, X.; Cui, F. Preparation and electrical properties of yttrium-doped strontium titanate with B-site deficiency. J. Power Sources 2008, 185, 26–31. [Google Scholar] [CrossRef]
- Lai, Y.W.; Wei, W.C. Synthesis and Study on Ionic Conductive (Bi1−x,Vx)O1.5-δ Materials with a Dual-Phase Microstructure. Materials 2016, 9, 863. [Google Scholar] [CrossRef]
- Singh, S.; Singh, P.; Viviani, M.; Presto, S. Dy doped SrTiO3: A promising anodic material in solid oxide fuel cells. Int. J. Hydrog. Energy 2018, 43, 19242–19249. [Google Scholar] [CrossRef]
- Singh, S.; Jha, P.A.; Presto, S.; Viviani, M.; Sinha, A.S.K.; Varma, S.; Singh, P. Structural and electrical conduction behaviour of yttrium doped strontium titanate: Anode material for SOFC application. J. Alloy. Compd. 2018, 748, 637–644. [Google Scholar] [CrossRef]
- Kolodiazhnyi, T.; Petric, A. The Applicability of Sr-deficient n-type SrTiO3 for SOFC Anodes. J. Electroceramics 2005, 15, 5–11. [Google Scholar] [CrossRef]
- Liu, J.; Wang, C.L.; Peng, H.; Su, W.B.; Wang, H.C.; Li, J.C.; Zhang, J.L.; Mei, L.M. Thermoelectric Properties of Dy-Doped SrTiO3 Ceramics. J. Electron. Mater. 2012, 41, 3073–3076. [Google Scholar] [CrossRef][Green Version]
- Presto, S.; Barbucci, A.; Carpanese, M.; Han, F.; Costa, R.; Viviani, M. Application of La-Doped SrTiO3 in Advanced Metal-Supported Solid Oxide Fuel Cells. Crystals 2018, 8, 134. [Google Scholar] [CrossRef]
- Buscaglia, M.T.; Viviani, M.; Buscaglia, V.; Bottino, C.; Nanni, P. Incorporation of Er3+ into BaTiO3. J. Am. Ceram. Soc. 2002, 85, 1569–1575. [Google Scholar] [CrossRef]
- Beltrán Rodríguez, R.; Landínez Téllez, D.; Roa-Rojas, J. Chemical Stability and Crystallographic Analysis of the the Sr2HoNbO6 Cubic Perovskite as Potential Substrate for YBa2Cu3O7−δ Superconducting Films. Mater. Res. 2016, 19, 877–888. [Google Scholar] [CrossRef]
- Bernuy-Lopez, C.; Høydalsvik, K.; Einarsrud, M.A.; Grande, T. Effect of A-Site Cation Ordering on Chemical Stability, Oxygen Stoichiometry and Electrical Conductivity in Layered LaBaCo2O5+δ Double Perovskite. Materials 2016, 9, 154. [Google Scholar] [CrossRef]
- Barison, S.; Battagliarin, M.; Cavallin, T.; Doubova, L.; Fabrizio, M.; Mortalò, C.; Boldrini, S.; Malavasi, L.; Gerbasi, R. High conductivity and chemical stability of BaCe1−x-yZrxYyO3−δ proton conductors prepared by a sol–gel method. J. Mater. Chem. 2008, 18, 5120. [Google Scholar] [CrossRef]

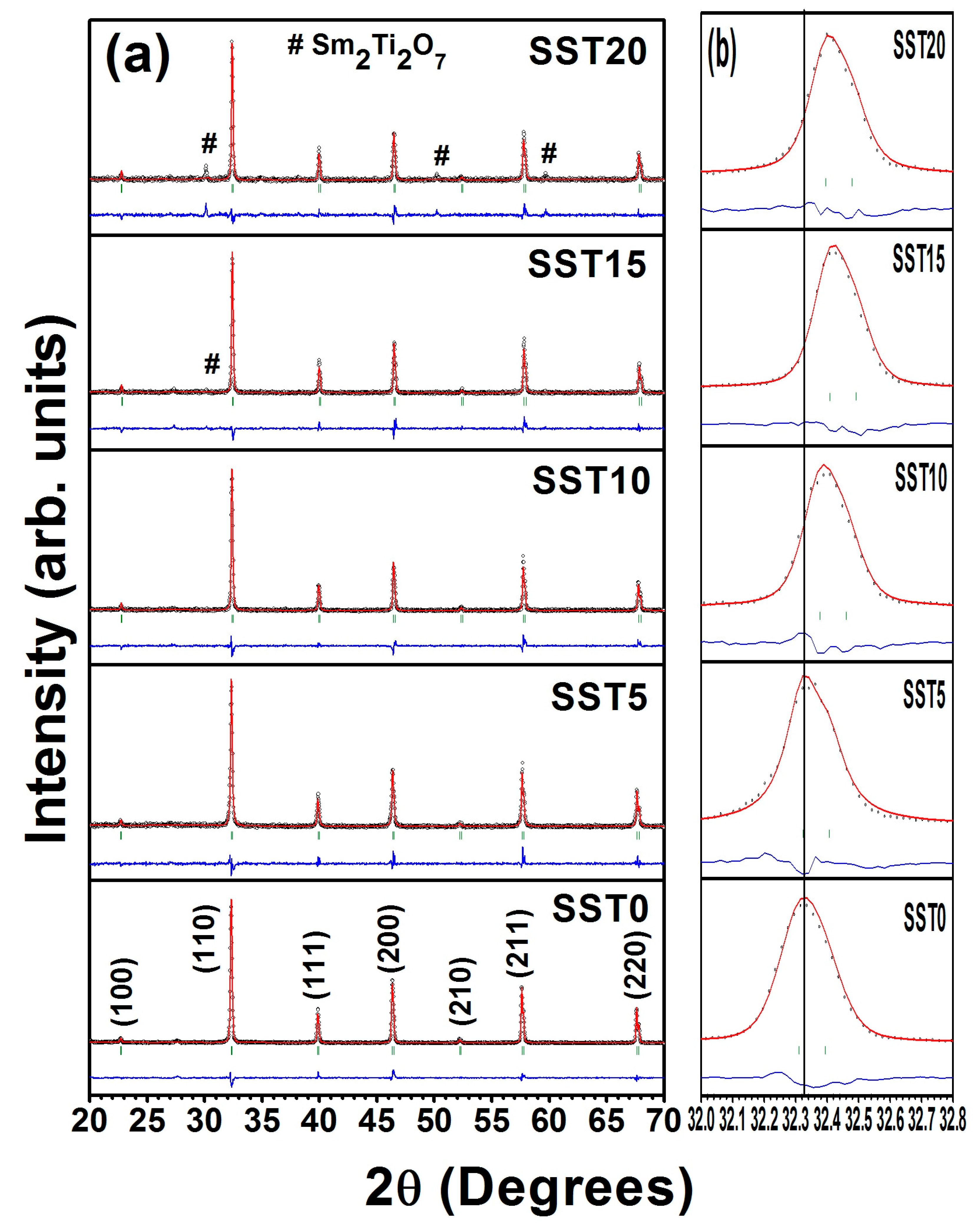
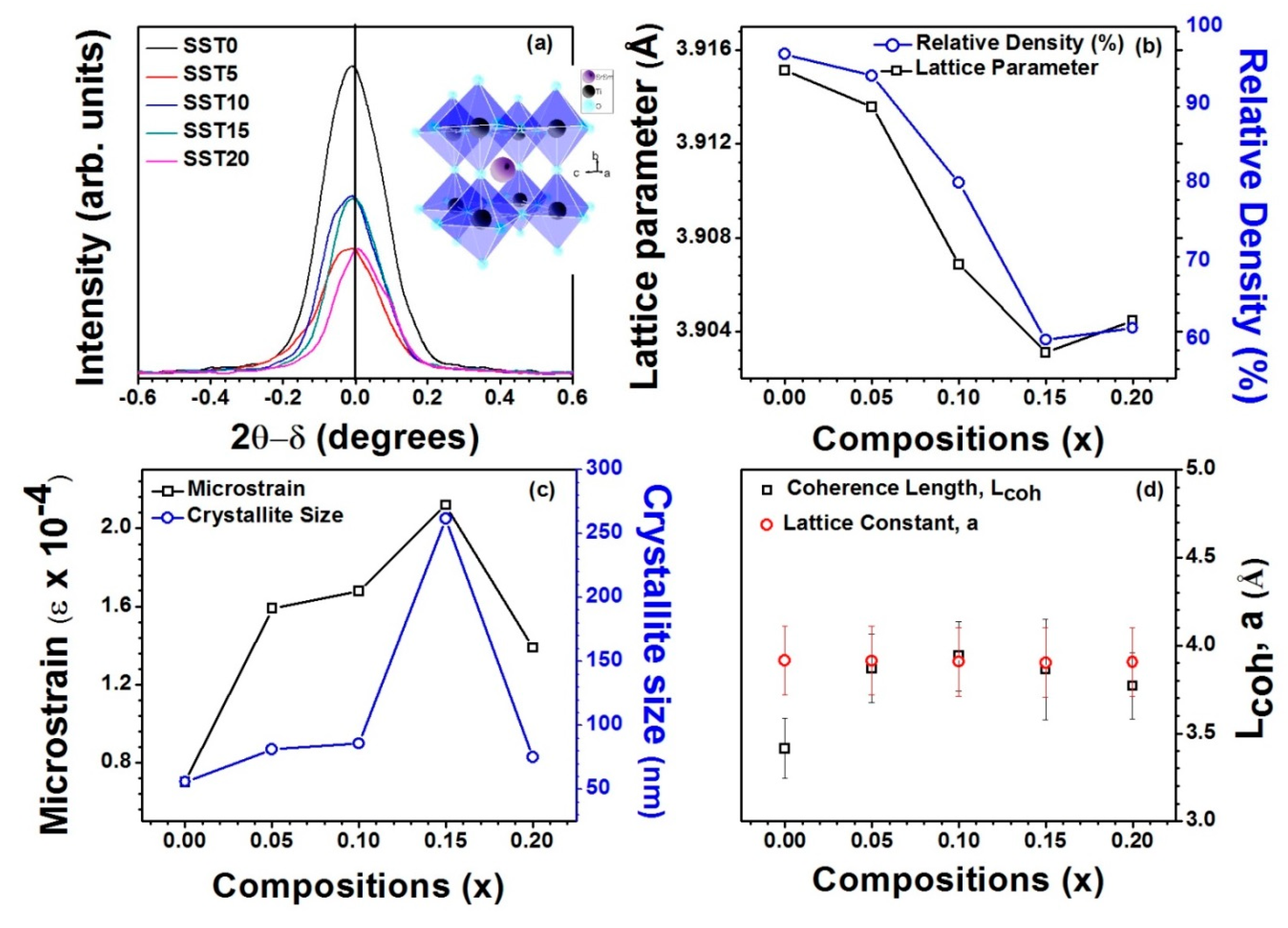


| Sample | Lattice Parameter (Å) | Cell Volume (Å3) | χ2 | Re | RB | RF | Rp | Rwp | Porosity (%) |
|---|---|---|---|---|---|---|---|---|---|
| SST0 | 3.9151 | 60.011 | 5.81 | 9.21 | 4.61 | 2.93 | 23.0 | 22.2 | 3.5 |
| SST5 | 3.9136 | 59.942 | 5.14 | 13.6 | 5.90 | 4.13 | 39.3 | 32.2 | 6.3 |
| SST10 | 3.9069 | 59.634 | 5.45 | 13.1 | 6.46 | 4.97 | 41.9 | 31.3 | 19.9 |
| SST15 | 3.9031 | 59.461 | 5.67 | 13.6 | 7.11 | 5.39 | 47.5 | 32.9 | 40.0 |
| SST20 | 3.9045 | 59.525 | 5.92 | 15.9 | 5.86 | 5.70 | 59.1 | 39.4 | 38.6 |
| Sample | Ea in H2 200–450 °C | Ea in H2 450–700 °C | Lattice Oxygen (%) |
|---|---|---|---|
| SST5 | 0.16 | 0.33 | 55.8 |
| SST10 | 0.15 | 0.22 | 66.6 |
| SST15 | 0.12 | 0.27 | 64.7 |
| SST20 | 0.13 | 0.17 | 62.4 |
| Sample | σ (Scm−1) @ 650°C | Reduction T (°C)/Time (h) | Reference |
|---|---|---|---|
| Sm0.1Sr0.9TiO3−σ | 2.7 x10−1 | 700/24 | this work |
| Dy0.08Sr0.92TiO3−σ | 1.4 x10−1 | 700/24 | [54] |
| Y0.08Sr0.92TiO3−σ | 6.8 x10−2 | 700/24 | [55] |
| Y0.08Sr0.88TiO3−σ | 1.0 x10−1 | 850/24 | [56] |
| Y0.07Sr0.93TiO3−σ | 5.0 x100 | 1400/5 | [30] |
| Dy0.1Sr0.9TiO3−σ | 7.1 x101 | 1450/4 | [57] |
| La0.1Sr0.9TiO3−σ | 7.0 x101 | 1450/12 | [58] |
| Y0.08Sr0.92TiO3−σ | 5.5 x101 | 1500/10 | [52] |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Singh, S.; Pandey, R.; Presto, S.; Carpanese, M.P.; Barbucci, A.; Viviani, M.; Singh, P. Suitability of Sm3+-Substituted SrTiO3 as Anode Materials for Solid Oxide Fuel Cells: A Correlation between Structural and Electrical Properties. Energies 2019, 12, 4042. https://doi.org/10.3390/en12214042
Singh S, Pandey R, Presto S, Carpanese MP, Barbucci A, Viviani M, Singh P. Suitability of Sm3+-Substituted SrTiO3 as Anode Materials for Solid Oxide Fuel Cells: A Correlation between Structural and Electrical Properties. Energies. 2019; 12(21):4042. https://doi.org/10.3390/en12214042
Chicago/Turabian StyleSingh, Saurabh, Raghvendra Pandey, Sabrina Presto, Maria Paola Carpanese, Antonio Barbucci, Massimo Viviani, and Prabhakar Singh. 2019. "Suitability of Sm3+-Substituted SrTiO3 as Anode Materials for Solid Oxide Fuel Cells: A Correlation between Structural and Electrical Properties" Energies 12, no. 21: 4042. https://doi.org/10.3390/en12214042
APA StyleSingh, S., Pandey, R., Presto, S., Carpanese, M. P., Barbucci, A., Viviani, M., & Singh, P. (2019). Suitability of Sm3+-Substituted SrTiO3 as Anode Materials for Solid Oxide Fuel Cells: A Correlation between Structural and Electrical Properties. Energies, 12(21), 4042. https://doi.org/10.3390/en12214042








