1. Introduction
As the population grows, the energy demand increases at a similar rate. Worldwide primary energy consumption in 2016 was 8.12 × 10
19 BTU (British Thermal Units) (8.57 × 10
22 J (Joules)) and it was 1.08 × 10
9 BTU (1.14 × 10
12 J) in Mexico [
1]. In Mexico the most representatives sources of the energetic mix are as follows: natural gas 52.75%, fuel oil 10.20%, coal 14.9%, nuclear power 4.49%, hydropower 4.57%, geothermic 5.52%, wind 0.05%, solar 0.003% [
2].
In 2015 the Mexican government reformed the energy laws and created the Energy Transition Law with the intent to increase the amount of electricity generated from non-conventional sources, including nuclear energy, to 35% by 2024 and 50% by 2050. The law also set a national goal to reduce greenhouse gas emissions by 30% by the end of the decade [
3].
Considering solar energy, an interesting statistic described in [
4] states that the global average solar radiation, per m
2 and per year, can produce the same amount of energy as a barrel of oil, 200 kg of coal, or 140 m
3 of natural gas.
Although there have been several studies on Stand-Alone Photovoltaic Systems (SAPVSs) most of them are focused on isolated areas.
Although there have been several studies on stand-alone photovoltaic systems (SAPVSs), most of them are focused on isolated areas. SAPVSs have usually been used in remote areas to harvest the solar resource. Because without doubt energy storage could define the future of SAPVSs, this should be mandatory because can provide grid stability and reduce the environmental impact [
5].
There are different studies about SAPVSs in isolated areas. For example, the authors in [
6] present a comparative study of the performances of various stand-alone solar photovoltaic (PV), grid-connected PV and hybrid renewable energy systems (HRESs) across the globe; the study presented in [
7] designed a methodology for highly reliable and sustainable stand-alone photovoltaic power systems (SPPSs). The approach consists of the following processes: prediction of the load demand, characterization of the PV performance on site, sizing the SPPS considering different storage technologies, and forming the most effective design.
Geographic Information Systems (GIS) have been used to determine the most suitable zones with solar resources, as done in [
8,
9,
10,
11]. However, SAPVSs in urban areas are uncommon, as the stand-alone PV design assumes that the system is not embedded in a building, unlike the building integrated design in [
12]. According to [
13], SAPVSs represent a cost-effective and eco-friendly alternative to conventional high cost diesel-fired generators, particularly in developing countries where most of the population lives in rural and isolated areas. The authors in [
14] mentioned that the only distinguishing feature between the stand-alone and building-integrated designs is the associated costs of land which is included in stand-alone design analysis; thus, making the unit cost of energy produced higher relative that obtained from a building-integrated design. Another constraint is the design of SAPVSs, PV power systems must be designed optimally to provide a feasible system that covers the desired load demand at a defined level of availability [
15].
There are currently several works on optimal sizing for SAPVSs [
12,
16,
17,
18]. Different sizing methods have been proposed in previous research works to obtain an optimum size of stand-alone PV systems and can be broadly classified as follows: numerical methods [
19,
20,
21,
22]; analytical methods [
23,
24,
25,
26] and intuitive methods [
27,
28].
In Mexico there have been several studies on SAPVSs. The authors of [
29] found out in the state of Aguascalientes, in central Mexico, some characteristics improved the conversion efficiency of Direct Current (DC) and Alternating Current (AC) in photovoltaic inverters. These characteristics are: climate, ratio of the inverter nominal power and DC input voltage and orientation of PV modules. The study presented in [
9] assessed solar irradiance in urban communities in the Baja California Peninsula using meteorological data and determined in this area an average irradiance of 5.5 kWh/m
2/day. The authors in [
30] forecasted the supply curve of non-conventional renewable technologies. They found out that Mexico would require an investment of 4.56 billion United States Dollars (USD), equivalent to 0.29% of Mexico’s Gross Domestic Product (GDP).
There are studies about technical-economic assessment for SAPVSs in houses connected to the electrical grid, like the one the authors carried out in [
31]. They performed a study in Mexico about the profitability of grid-connected photovoltaic systems (GCPVSs) for electricity users falling within the so called Domestic High Consumption Tariff (DHCT). These users do not receive any subsidy and make a GCPVS feasible due to the following double mechanism: on the one hand, the reduced amount of electricity that is drawn from the grid, and on the other, a consumption reclassification which makes them pass from the DHCT to a lower consumption tariff which benefits from state subsidies. It is also shown that the utilization of GCPVSs would lead to economic benefits for the electric power sector, which in consequence accounts for a social benefit. In this paper, a broad study on planning and optimization of SAPVSs in an urban area, and load profiles of Mexican urban areas are considered. A novel perspective is proposed considering the characteristics of the electric demand showing the positive impact of these systems in reducing the high electrical consumption from the grid and an economic profit for the user over 20 years comparing between a storage system and electrical fees. An average load profile in South Mexico is applied for SAPVSs and simulated it’s the RETScreen software as done in [
32,
33,
34,
35]. RETScreen gives as output the optimal system configuration that is required for a reliable system operation. The optimization result also gives the total cost and the cost of energy for a microgrid setup.
2. Materials and Methods
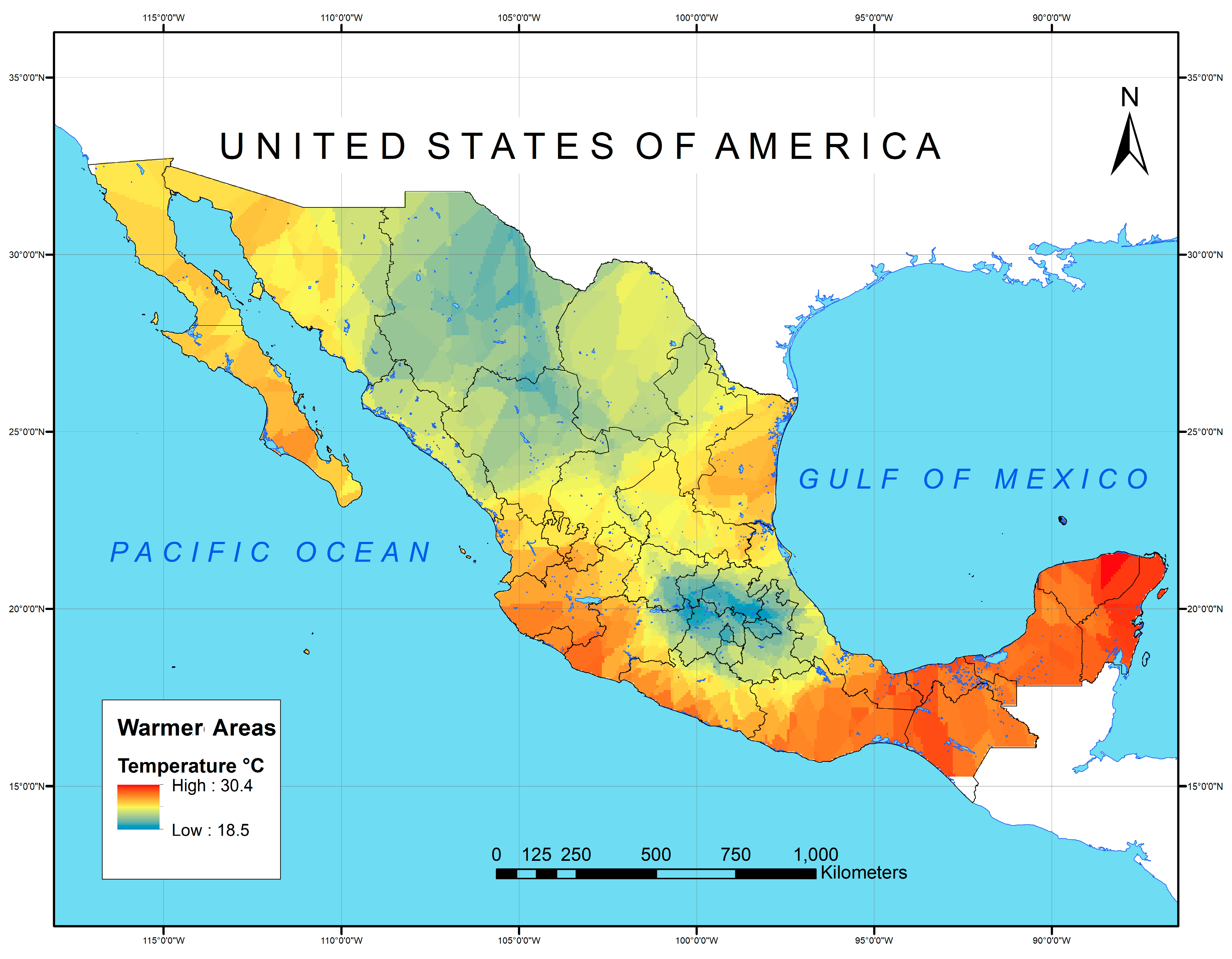
The population dynamics of Mexico’s coastal zones follow global trends, which indicate a movement of human populations towards these areas. In 2010, the population of the coastal states was 51,900,847 inhabitants, 4.5 million more than in 2005 and 7.25 million more than in 2000. It is expected that by 2030 it will increase to 55 million [
36]. Warmer areas in Mexico usually are next to the sea, in the desert and rainforest. To identify the warmer zones in Mexico, temperature and relative humidity data were taken from [
37] and they are presented in
Figure 1.
According
Figure 1, the warmer zones in Mexico are in the south and southeast of the country. These zones are characterized for having an irradiance ranging from 4.4 to 5.5 kWh/m
2/day [
38]. It is necessary to consider that the population of coastal municipalities, following the definition of Mexican policy, grew in the period 2000–2010 by 18.53% [
39].
The proposed SAPVS has been designed for warmer urban areas in Mexico, it is widely known that variation of irradiance depends on the geographic location, in this case, the selected city is Coatzacoalcos located at the southeast of Mexico, its geographical coordinates are 18°08′09″ N, 94°27′48″ W and 10 m height above sea level.
On average, during a year, the city has 75% relative humidity and a 28 °C temperature, its area is around 309 km
2 and its population is 332,464 inhabitants, 2327 of which or 441 households are without electricity [
39]. During this period an irradiation of 5.3 kWh/m
2/day has been recorded [
40].
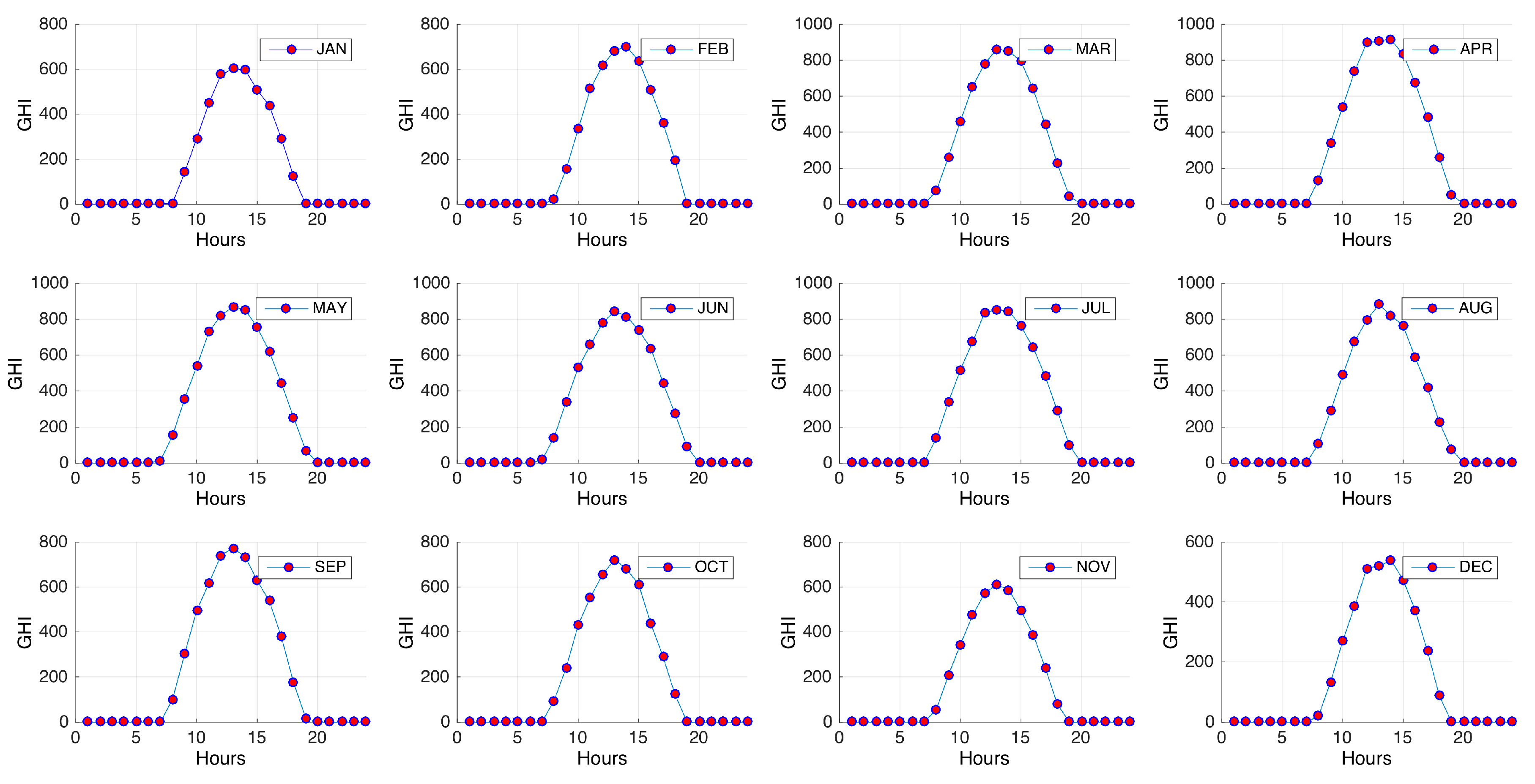
The output power and energy generated have been calculated with solar irradiance data recorded every 10 min from Automatic Meteorological Stations (AMSs) located at Coatzacoalcos [
41].
Figure 2 shows monthly Global Horizontal Irradiance (GHI) over Coatzacoalcos (Veracruz, Mexico).
2.1. Modelling Energy Generation
RETScreen is a Clean Energy Management Software system for energy efficiency, renewable energy and cogeneration project feasibility analysis as well as ongoing energy performance analysis. The software identifies, assesses and optimizes technical and financial viability clean energy projects potential [
42]. Even though, RETScreen software has its own database, it allows the addition of new data from other databases.
2.2. Stand-Alone PV Model
The solar irradiance could be considered the most important climatic variable that determines the correct performance of a PV. A photovoltaic system (PVS) is an alternative energy generation technique that takes advantage of solar irradiation to produce electrical energy through the photoelectric principle and can be used in two main ways: direct consumption and energy storage into a battery bank of deep cycle, this allows to have energy availability at any time, can be delivered either as Direct Current (DC) or Alternating Current (AC) using an inverter.
A SAPVS consists mainly of the following elements: a photovoltaic panel, which is responsible for transforming the solar irradiation into DC electrical energy; a load regulator whose function is to regulate the voltage generated either to charge a DC load or to perform a charge cycle to a deep cycle battery; a deep cycle battery, which is responsible for storing the energy produced by the photovoltaic panel, and finally, protection systems, which protect both the user and the system itself. The schematic system with its components is shown in
Figure 3. The methodology to obtain the optimum angle is proposed in [
43] and used in [
12].
The latitude angle (φ) is the angle forming according to the equator center. The north of the equator is positive and the south of the equator is negative and it varies between −90° ≤ φ ≤ 90°. The longitude and the latitude angles are used to define the any location on the surface of the Earth.
Declination angle (
δ) is the angle between the sunlight and the equator plane. The declination angle occurs due to the 23.45 degree angle between Earth’s rotational angle and the orbital plane. It is positive in the north and varies between −23.45° ≤
δ ≤ 23.45°. Declination angle is at its highest point on 21 June (23.45°) while it is at its lowest point (−23.45°) on 22 December in winter. Sunlight falls on the equator with a steep angle twice a year. This condition is called the equinox. The vernal equinox is on 20 March and the autumnal equinox is on 23 September. Daytime and night-time durations are equal on equinox dates and the declination angle is 0. The declination angle is calculated by Equation (1), where
n represents a Julian day.
The optimum angle (
β) is the angle between the panels and the horizontal plane. This angle is south oriented in the Northern Hemisphere and north oriented in the Southern Hemisphere. Tilt angle varies between 0° ≤
β ≤ 180°. When a plane is rotated about a horizontal east-west axis with a single daily adjustment, the tilt angle of the surface will be fixed for each day and is calculated by Equation (2):
In order to define the power output of a PV module for a certain region, it depends on ambient conditions such as module operating temperature and its nominal output efficiency
ηref; which determine the optimal output
ηPV of PV array. According to the Solar Synthesis of Mexico [
44], the efficiency of a PV module
ηPV is a function of the reference efficiency
ηref, the temperature correction factor/power temperature coefficient of module
βref (normally taken between −0.3 and −0.6% per °C for crystalline silicon [
45]), the cell temperature
Tc, and the standard testing temperature
Tstc which are provided in the manufacturers’ data sheet. The efficiency of a PV module can then be calculated using Equation (3):
The cell temperature
Tc, which depends on the air temperature of location
Ta and the solar radiation
Itilt, is calculated with Equation (4), where
NOCT is the nominal operating cell temperature:
The power output of a PV module can be calculated by Equation (5):
where
Pmax,stc is the maximum power of the cell under standard test conditions and
Gstc is the irradiance of 1 kW/m
2 at standard test conditions.
2.3. Batteries
The energy from SAPVS is stored in batteries and there are several types of them according to the Technical Specifications for Photovoltaic System of Sustainable Energy. For all the Americas there are four types of batteries: electrolyte absorbed amid fiberglass (AGM) and gelled electrolyte (GEL) for small systems; Stationary armored plate liquid (OPzS) liquid electrolyte type and flat plate liquid electrolyte for bigger systems. Recently the OPzS batteries, which refers to a flooded type of tubular-plated, lead acid and deep cycle batteries, have reached 20 years of service life at 20 °C.
Some aspects must considered like battery banks, service life, autonomy days, input and output charge voltage, efficiency and deep cycle discharge. To calculate the autonomy of a battery bank, using Equation (6), it is necessary to know the voltage at which it will work at critical power or maximum consumption during the year:
where
Ecrit daily critical energy consumption,
ta days of autonomy and
Vsrate rate system voltage.
Equation (7) shows the rate capacity by hours, in order to determine the capacity that the batteries bank needs to supply during the hours of night consumption or its shared use:
where
Ah is the rate capacity by hours,
I is the current and
T is the time in hours.
The life cycle, deep discharge and efficiency parameters depend of the load demand, model and type of battery as well as the temperature where the battery bank is operated.
Load voltage and battery banks are in function of type of connection of the system, charger output voltage and the batteries’ input voltage. In a SAPVS the voltage of the system is given by the battery bank voltage. To calculate the number of batteries in a parallel connection, which is required for systems where the current demand is greater than a battery with the same voltage, Equation (8) is used:
where
AD is the average daily amperes-hour consumption,
TA are days of autonomy,
Dl is the discharge limit and
BAh is the capacity in amperes-hour of the battery.
2.4. Inverter
In order to convert DC to AC, an inverter becomes necessary. Currently, there are exclusive inverter models for isolated systems which already have an integrated battery charger and controller. Depending on the AC voltage and power consumption, the voltage of the charger and the same voltage of the inverter vary from 12, 24 or 48 V. There are also inverters for isolated systems with voltages that exceed 48 V.
Because these models already include the charger and controller inside the battery bank, to calculate the maximum AC load current (from the controller) Equation (9) is used:
where
PTDC is the total power of the system in DC and the voltage in DC,
VDC. To determine the short circuit current of the system, Equation (10) is used:
where
Ispscc is the solar panel short circuit current;
MP are the modules in parallel and the 1.25 is the constant of the controller short circuit current.
3. Results and Discussion
The model presented is a SAPVS located in a warm urban area where the irradiation is around 5.3 kWh/m
2/day [
40] and an average GHI of 800 W/m
2. This model was designed for a residential house and can be applied for any location where data are known. Although in Mexico 99.6% of the urban areas are electrified [
46], this SAPVS show technical and economic benefits. For the application of the model, the city of Coatzacoalcos was selected because it has been named as a Special Economic Zone and represents an opportunity for future investments [
47]. 20.75° is the optimum angle for Coatzacoalcos and it was obtained using the methodology proposed in [
43]. With this value it can be assumed that SAPVS is an option of electrification on the already existing flat roofs in the area. The electrical load proposed is typical in Coatzacoalcos, and the data are presented in
Table 1.
The characteristics of the loads presented in
Table 1 are the consumption average of a housing type DHCT, which are the ones that pay the highest electricity rates. All the loads are in AC. It can be seen the useful hours of TV are 5 for 3 days, it has been considered that population are focused in mobile devices, so TV or PCs are increasingly less used.
In SAPVS the power supply must be regulated.
Table 1 shows a column called intermittent resource-load correlation, which means its power-load correlation can be determined as follows: (−) negative: if the load is to be supplied by the battery bank system; (0) zero: if the load is to be supplied by both the solar panel systems and the battery bank; (+) positive: if the supply depends on the SAPVS.
For three days of autonomy, Equation (6) was used, where using the power critical consumption in a year and 24 VDC for DC system of 5.91 kW, the capacity obtained was 738.6 Ah in three days (720 h).
To provide the consumption of current in Ah and a voltage of 24 V with the intention of maintain the same voltage in the battery bank, the batteries are connected in parallel in six battery modules. Each battery supports 4 kW for three days of autonomy, which is equivalent to 150 Ah at 20 °C, in a parallel 900 Ah connection.
Based on power consumption, voltage and current of the system, the annual peak power consumption (PP) is 5.91 kW, 3.092 kW of constant power (PC), 230 V and a current of 14.83 A (PP), 7.763 A (PC) and the maximum current in the system is determined by the protection. According to the mexican standard NOM-001-SEDE-2012 published by the National Consultative Committee for the Standardization of Electrical Installations and by the General Directorate of Electric Power Distribution and Supply in June of 2012 the current is 37.082 A, resulting a power of 14.7 kW multiplied by a factor of 2.5, which is consumed at the moment that the system in AC is protected. For DC, Equations (9) and (10) are used to obtain the protection current. The maximum current of the DC system that is detected by the internal inverter IMaxAC is 167 A (Equation (9)). Equation (10) is useful to determine the short circuit current of the PV system, which is 109.35 A.
There are inverters for stand-alone systems designed to support currents even higher to 200 A with constant power, according to voltage, temperature and current up to 15 kW.
For the conditions of the system in AC, the inverter must be designed with a capacity of 6.8 kW in PC and 16 kW in PP. In this case the controller and charger are integrated into the inverter, and according to Equation (9) the maximum current in AC of the controller is 155 A and applying Equation (10) the short circuit current in DC is 109.35 A. As the inverter includes a control system this has a current detection function, which is divided in two sections, in the first one has a capacity of 100 A designed to continue consumption and the second one if the demand increased the capacity as well as 200 A.
The SAPVS characteristics are: 12 modules of 310 W, a rated power of 3.72 kW and generating 5.41 MWh annually, an efficiency of 16%. A 0.505 tCO
2/MWh of greenhouse gases (GHG) emission factor was obtained. Based on the power system, the reduction of net annual GHG would be 11 tCO
2 and a financial revenue of 36.951 × 10
3 $/tCO
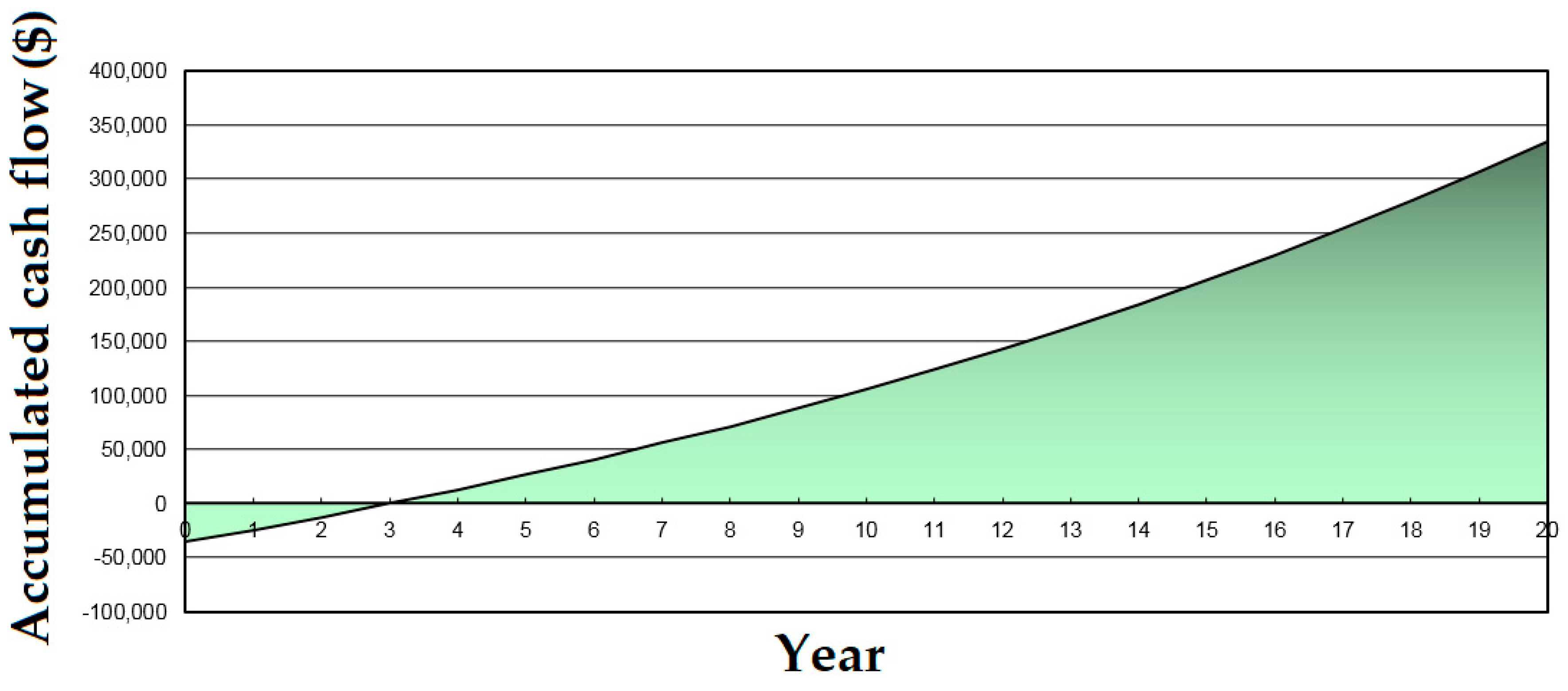
2 would be obtained. Financial parameters such as an Internal Rate Return (IRR) of 36.3%, simple payback of 3.4 years and equity payback of 3 years show the financial viability in this investment; simple payback represents the length of time that it takes for a proposed project to recoup its own initial cost, out of the income or savings it generates and equity payback represents the length of time that it takes for the owner of a project to recoup the initial investment (equity) out of the project cash flows generated. In
Figure 4 we can see the cash flow during the service life of the project.
Table 2 presents the DHCT-type prices by month.
With the prices presented in
Table 2 the annual cost can be calculated as follows: this value is given by taking the average consumption (59.79 kWh) of a base case (see
Table 3), multiplied by the days of the month and a share of energy. Then, a fixed monthly charge is added, e.g., 59.79 kWh × 31 (Jan.) × 0.193 + 4.7835. This calculation is performed for the 12 months of the year and the result is
$4437.7 USD, if this value were to be constant during 20 years, the total obtained would be
$88,755.5 USD with a 0.50 USD/kWh of cost per kWh. If the initial cost is
$38,027 USD and the NPV of the annual cost is
$5321 USD for 20 years with 10% of interest, the
$/kWh price is 0.187. The authors in [
12] consider that SAPVSs are an opportunity to generate electricity for developing countries, however, this analysis suggests that SAPVSs is far from the economic possibilities of developing countries due to the high investment costs.
The model has been validated using different arrays on RETScreen, where changes were made on the characteristics of solar panels, inverters and batteries. The best configuration is with 12 PV of 310 W, inverter of 8 kVA at 25 °C and 6.8 kW with input voltage of 9.5 to 66 DC and output voltage 230 AC (+/−2%) maximum current of 200 A, a controller and charger integrated, a battery bank of six OPzS solar batteries of 24 VDC at 150 Ah 72 h each, 900 Ah for the batteries bank equivalent to 3 days of autonomy and a service life of 20 years at 20 °C, satisfying the power consumption in peak power of 5.91 kW and constant power of 3.09 kW, 230 V, a short circuit current in peak consumption of 37.08 A, 738.6 Ah for 3 days of autonomy and 206.77 Ah for 14 h shared with the battery bank of the PV system, with capacity to work with 24 VDC and supporting an input power of the PV system of 3.72 kW at 20 °C and 4 kWh.
4. Conclusions
SAPVSs in urban areas in Mexico depend on two analyses, the economic and technical. For the first one, the electricity consumption has to be DHCT type, because is the highest rate of consumption for housing; the income per house must be greater than $4437.7 USD per year. For the technical analysis, the SAPVS has to satisfy the electricity consumption of peak load; the system should be designed for future loads for 20 years; to obtain the maximum service life, its installation must be standardized.
In this study, batteries represented 77% of the total cost, and with 12 PV panels of 310 W could export 5.41 MWh to the grid and an inverter an integrated controller and charger was selected, thus decreasing the initial cost. The city of Coatzacoalcos was chosen because the average annual temperature is 28°, an average relative humidity of 75% and an average irradiance of 5.3 kWh/m2/day and because this year the city has been named a Special Economic Zone and thus represents an opportunity for future investments. In this city, 98% of the houses have air conditioners with 12,000 BTU of power. The Mexican government has introduced an energy program called Escrow for Electric Energy Savings (EEES), which finances part of solar projects. Among its objectives are: finance programs and projects for energy efficiency, electric and thermal, cogeneration and distributed generation with renewable sources in industries, shops, services and housing; to contribute in the strengthening of the culture of saving and the efficient use of energy among the population; and incursion in applied research and technological innovation in savings, efficient use of energy and distributed generation with renewable sources.
The service life of the batteries could be the main disadvantage of SAPVSs, even though there are batteries with 20 years of service life, this is affected for the temperature factor, which is the ambient temperature and the temperature that is produced in the battery itself (during charging and discharging). The batteries are designed to work at 20 °C and if this temperature changes by 10 °C the service life decreases, i.e., at 30 °C the service life of the batteries will be only 10 years.
Currently, there are proposals for the development of SAPVSs for remote places and cities in Mexico. The first one, with the purpose of generate the electrical energy necessary for the communities that do not have access to it; the second one, to reduce the electricity supply generated by fossil fuels, as emergency systems, so the isolated systems help in the management of a greater efficiency of generation of electric power and its transmission for urban areas.
In recent years, the development of energy sustainability has been sought in order to include the environment as one of the elements of competition that contribute to the economic and social development of the population. Hence, there is a clear commitment, derived from the Energy Reform: to foresee the gradual increase of the participation of Renewable Energies in the Electricity Industry, in order to comply with the established goals in terms of clean energy generation and emission reduction.
Mexico has created the Energy Transition Law, which states that the electricity sector must be transformed so that by 2024 a maximum of 65% of electricity comes from fossil fuels. This goal is ratified in the General Law on Climate Change that stipulates that 35% of electricity generation comes from clean energy for the same year.