Mathematical Modeling of Non-Premixed Laminar Flow Flames Fed with Biofuel in Counter-Flow Arrangement Considering Porosity and Thermophoresis Effects: An Asymptotic Approach
Abstract
:1. Introduction
2. Theoretical Modeling
2.1. Lycopodium Characteristics
2.2. Porosity of Lycopodium Particles
2.3. Thermophoretic Force
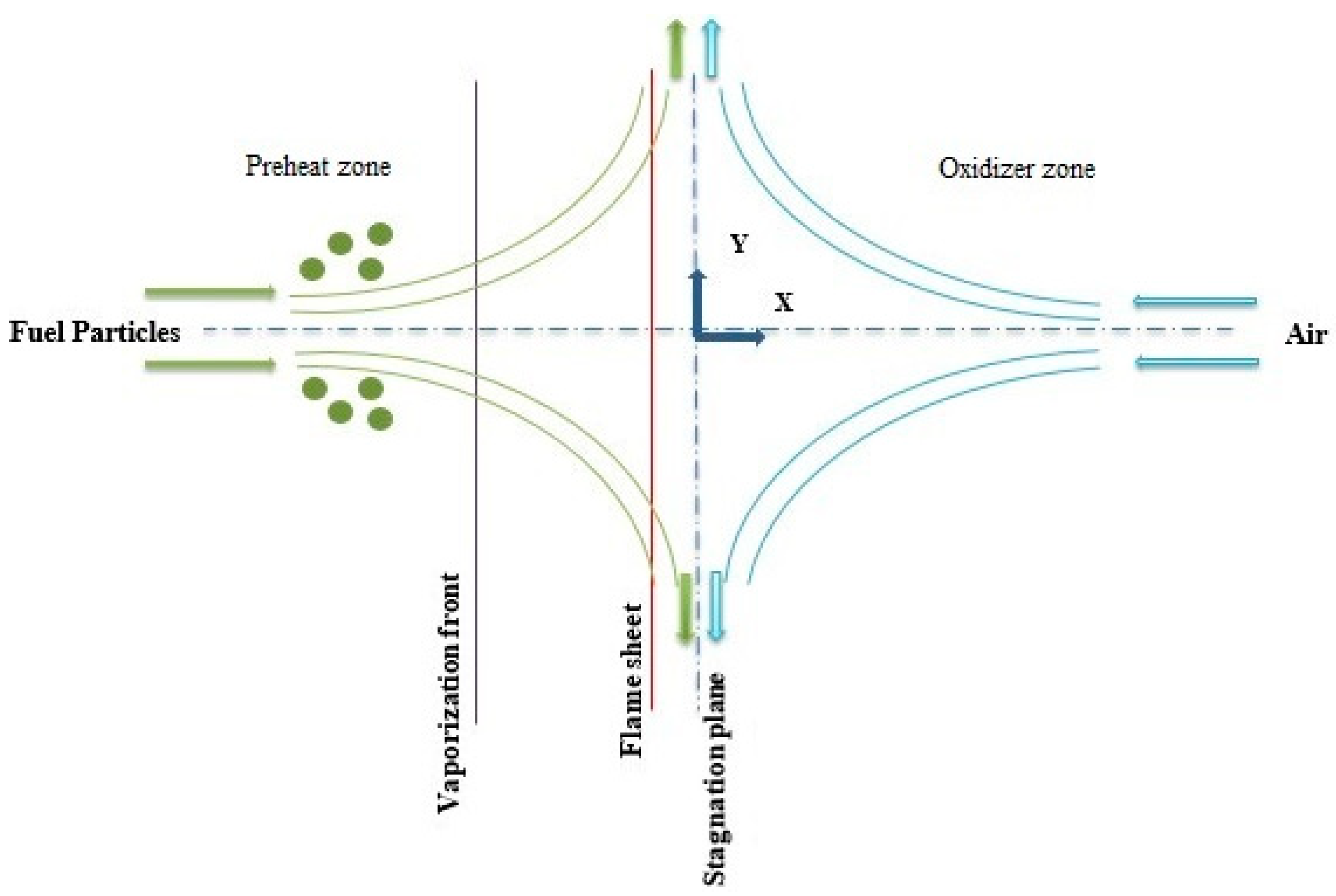
2.4. The Flame Structure
2.5. Mathematical Modeling of the Flame
- For simplicity, it was assumed that values of density and specific heat were constant and the momentum of the fuel and oxidizer streams were the same.
- The vaporization process occurred in a very thin zone (asymptotic limit).
- Thermal radiation and heat losses were disregarded.
- Lycopodium particles were uniformly dispersed. In other words, the size and shape of the particles were assumed to be the same.
- A large Zeldowich number was presumed. Thus, the thickness of the flame zone would be too small.
- The ambient temperature was assumed to be 300 K.
- In order to analytically solve the coupled complex conservation equations of mass and energy in the considered zones, it was assumed that a gaseous fuel with a certain chemical composition evolved from the asymptotic vaporization of the lycopodium particles. Therefore, pyrolysis was disregarded as clearly considered in References [11,20,43].
- No chemical interaction occurred between the solid particles before the vaporization front.
2.5.1. Dimensionalized Governing Equations
2.5.2. Normalization of the Governing Equations
2.5.3. Boundary and Jump Conditions
| Preheat zone: | |
| Post vaporization zone: | |
| Oxidizer zone: |
2.5.4. Solution of the Governing Equations
2.6. Flame Zone Analysis
2.7. Calculation of the Thermophoretic Force
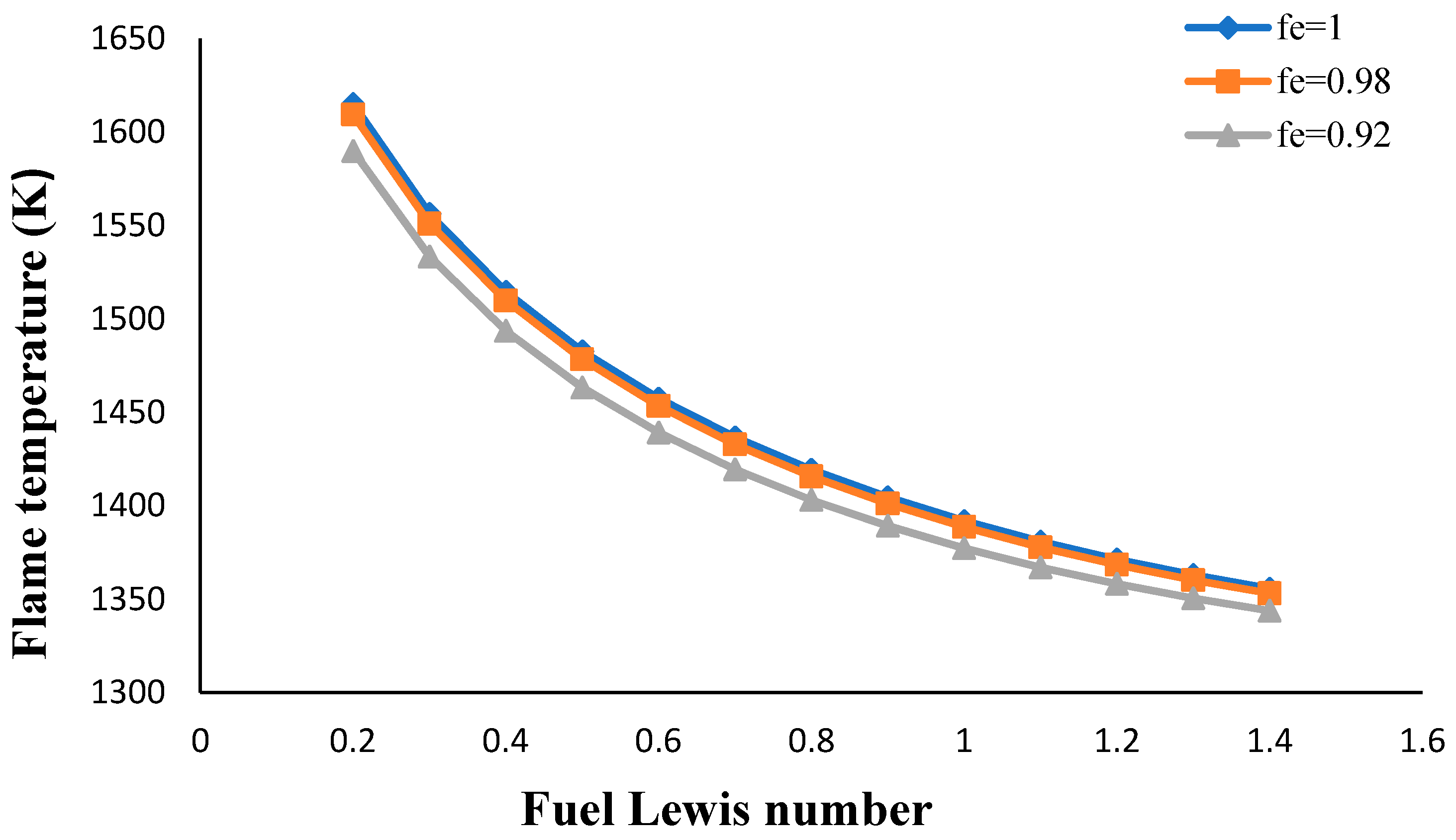
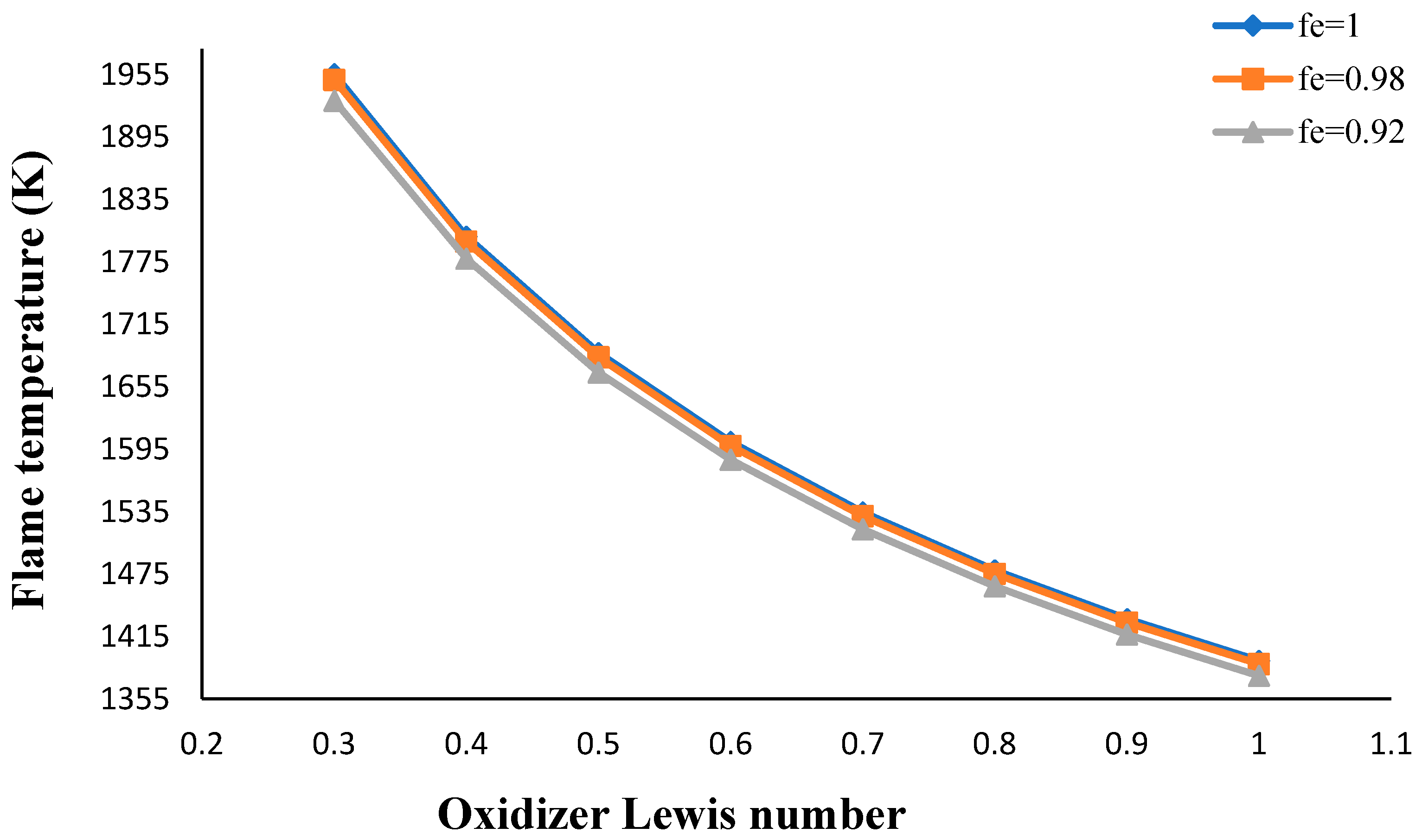
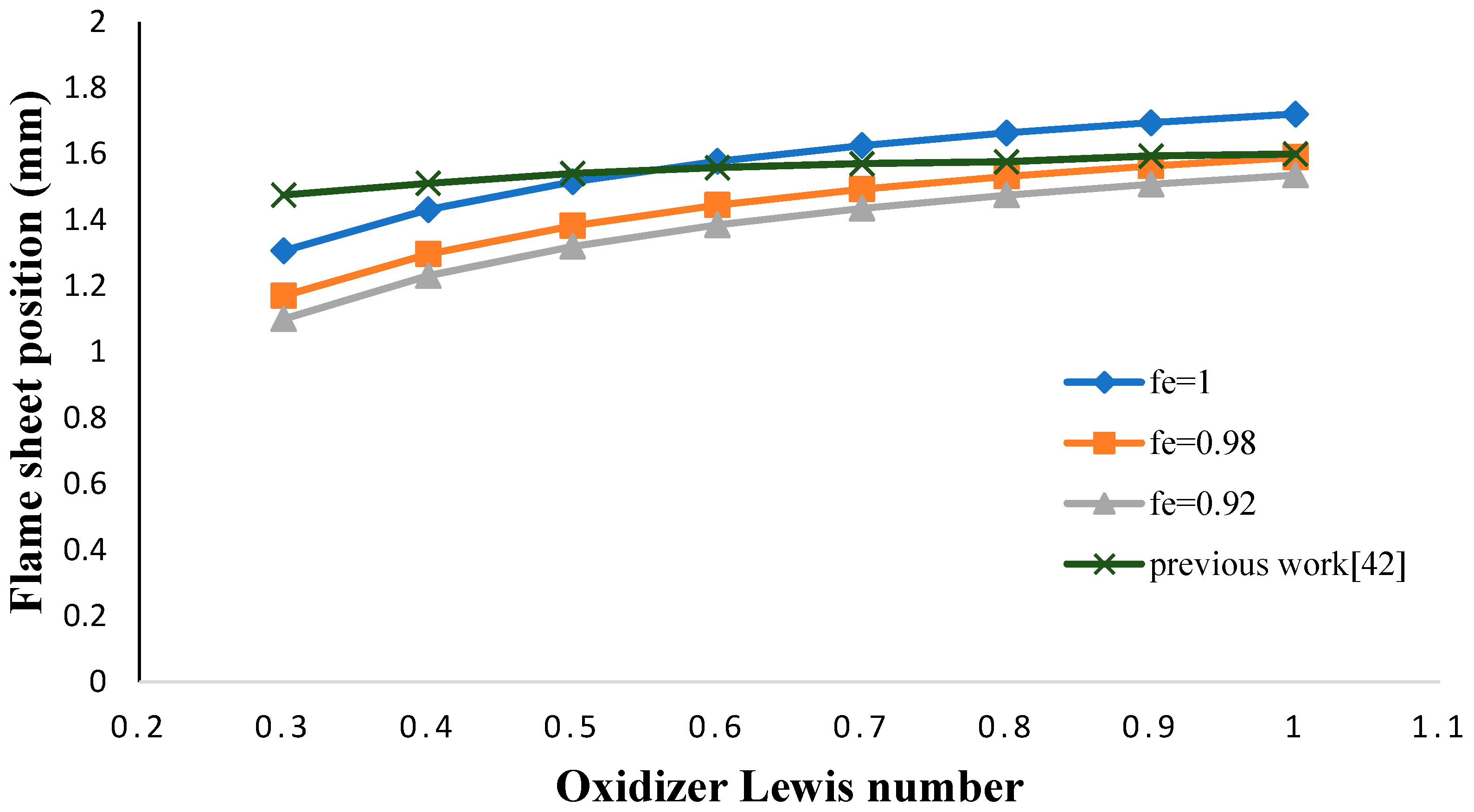
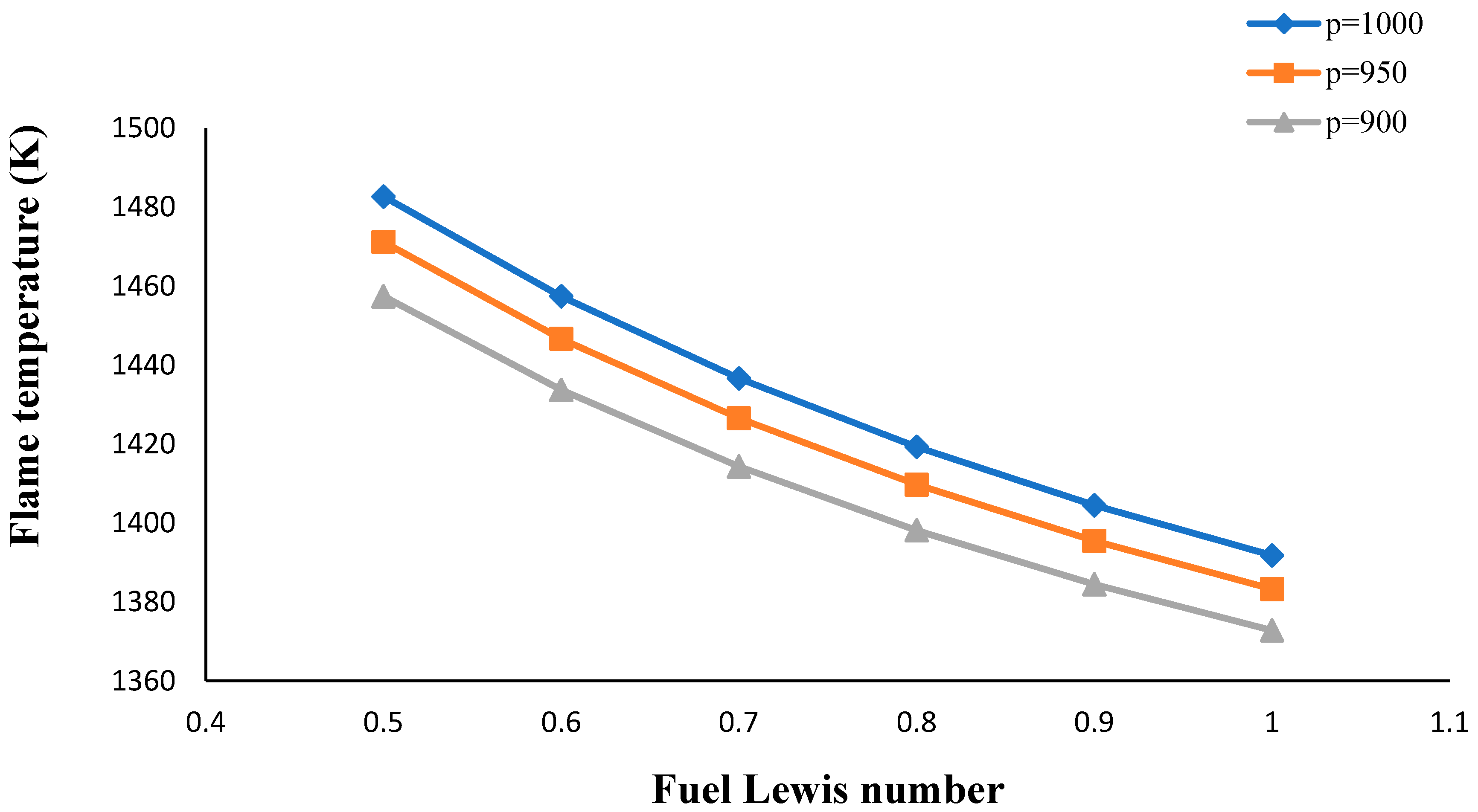
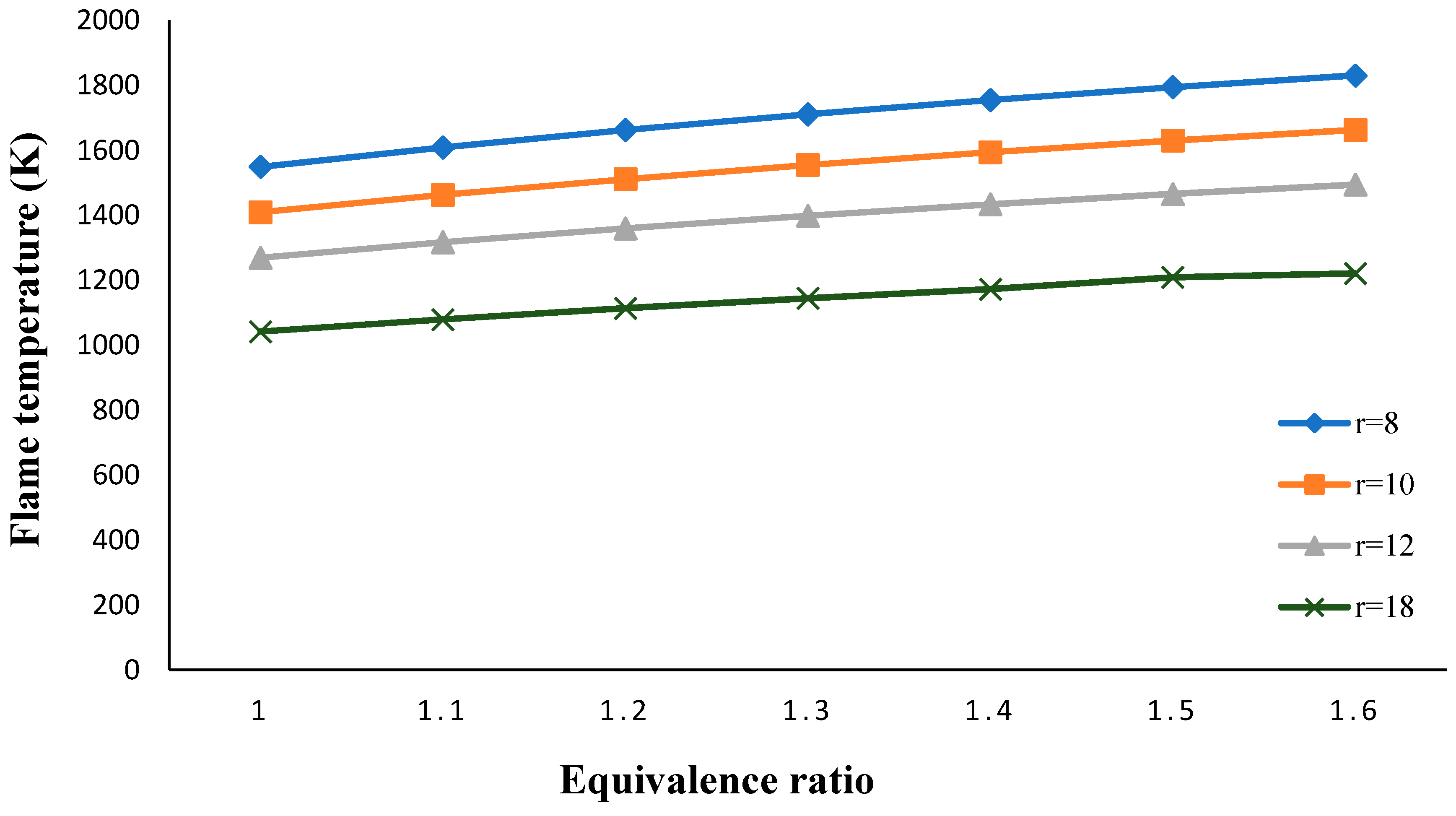
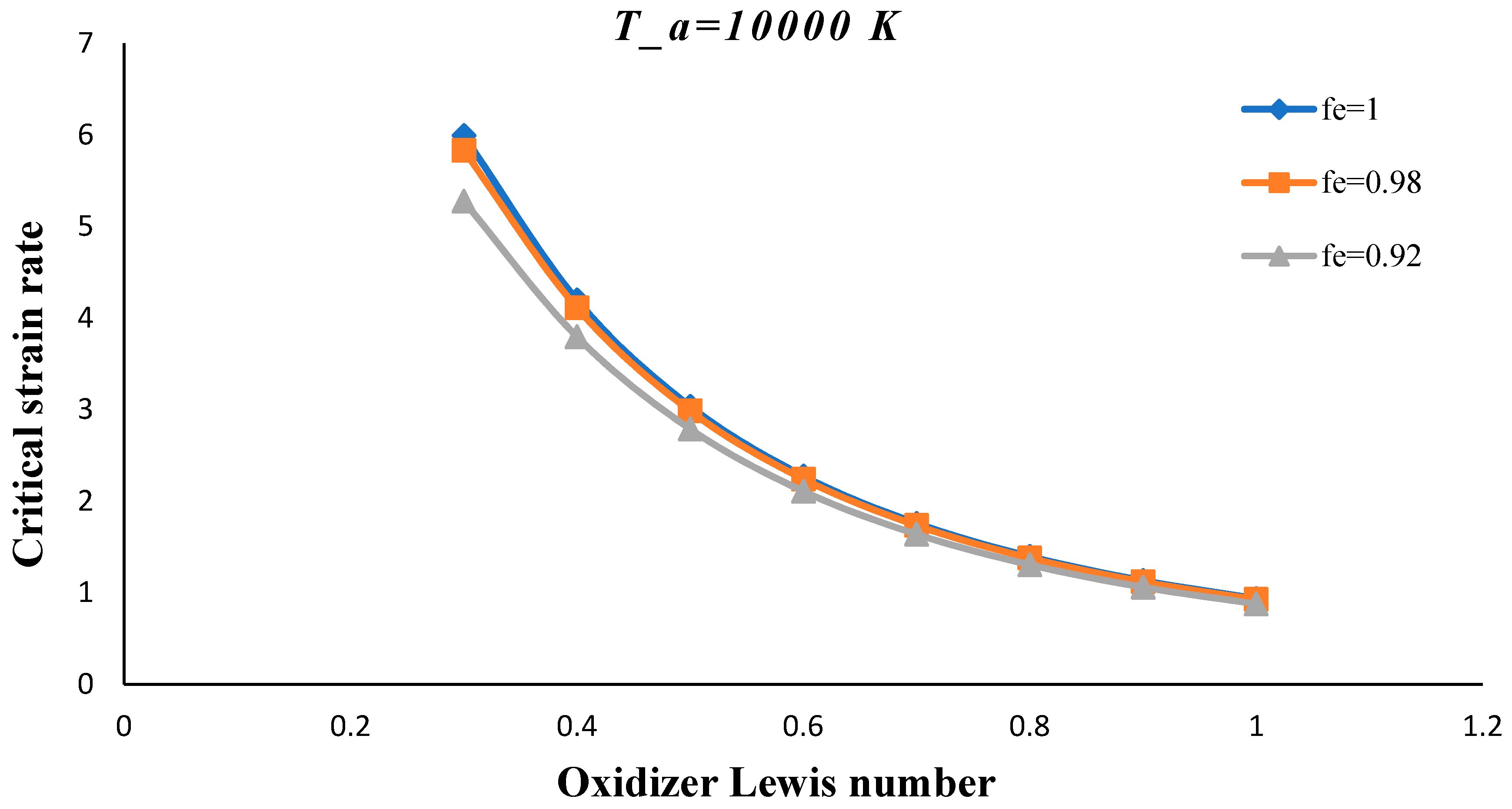
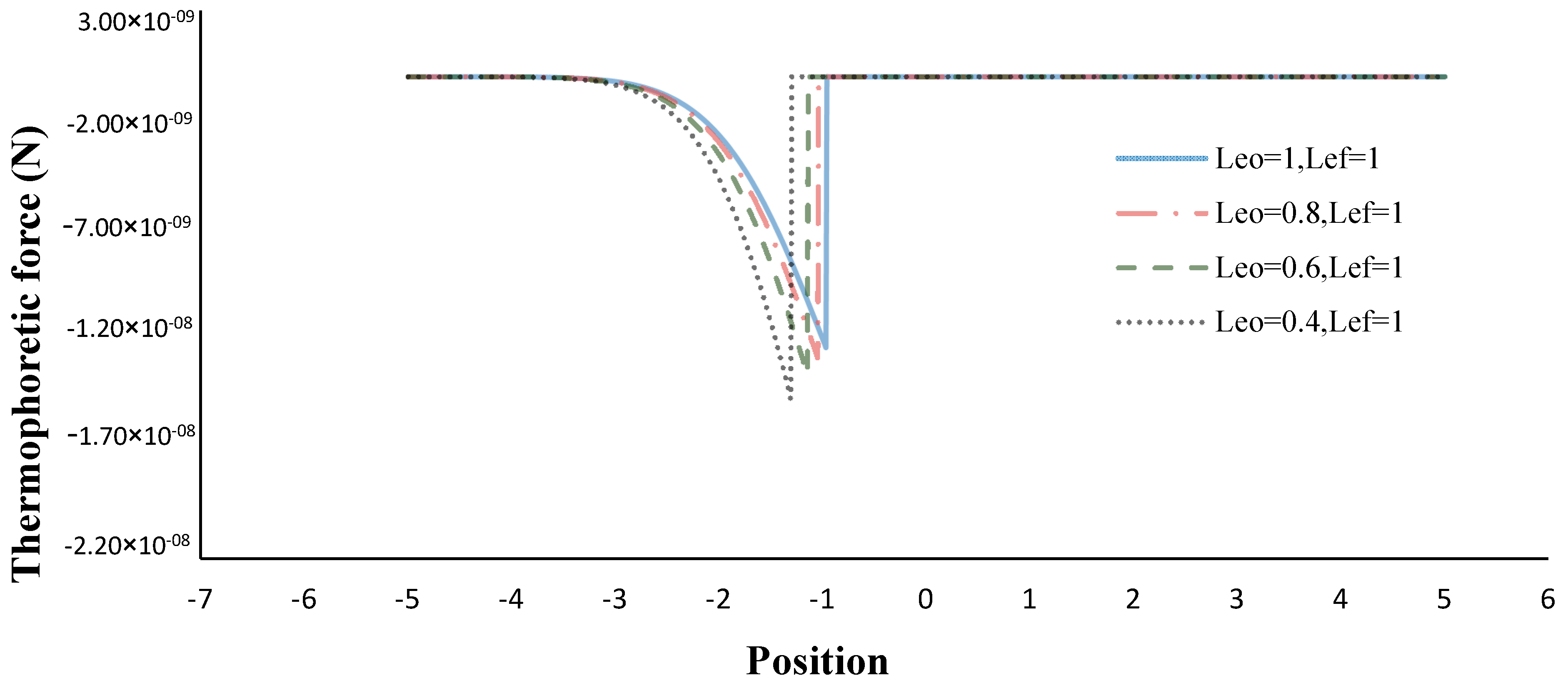
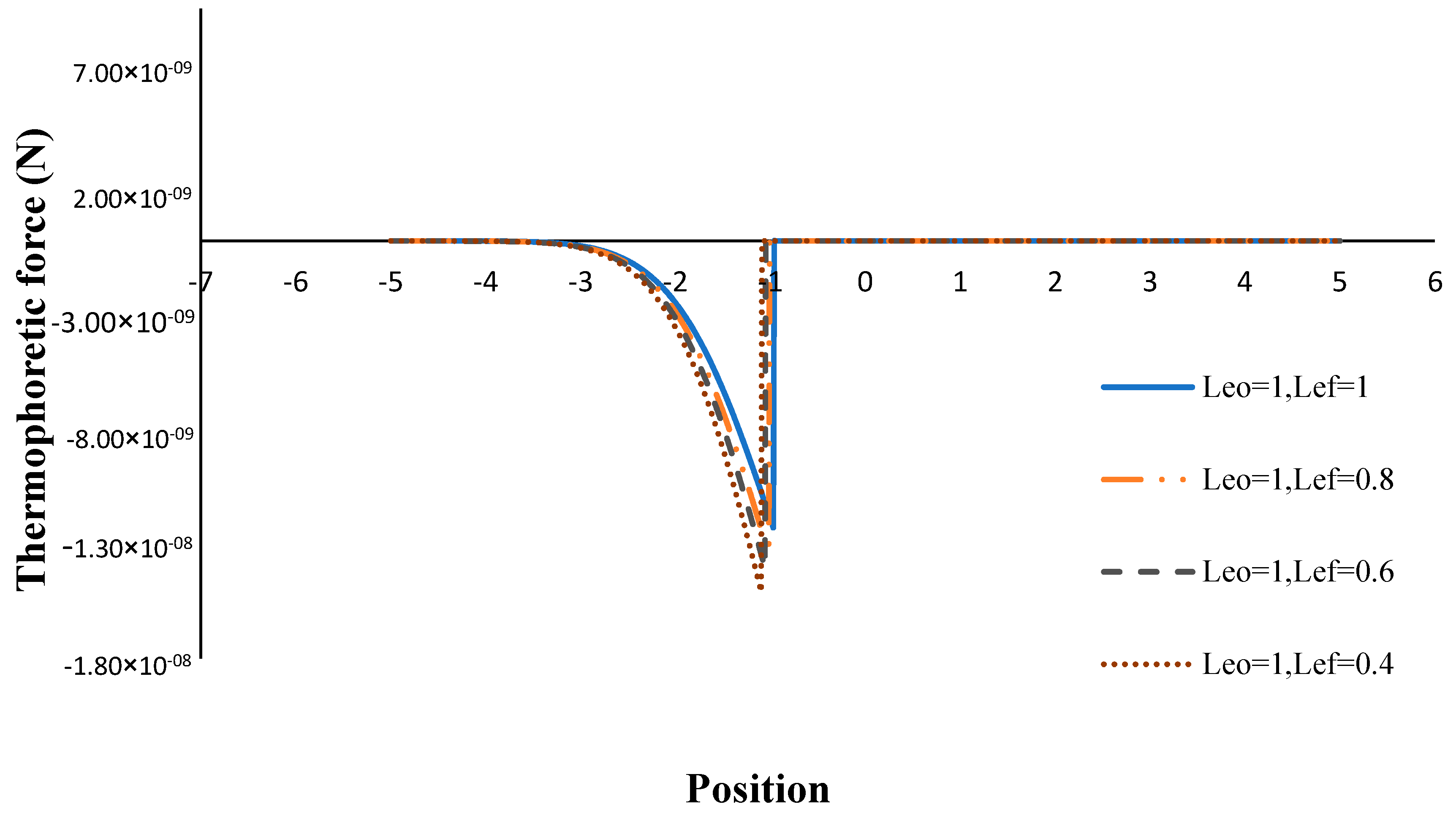
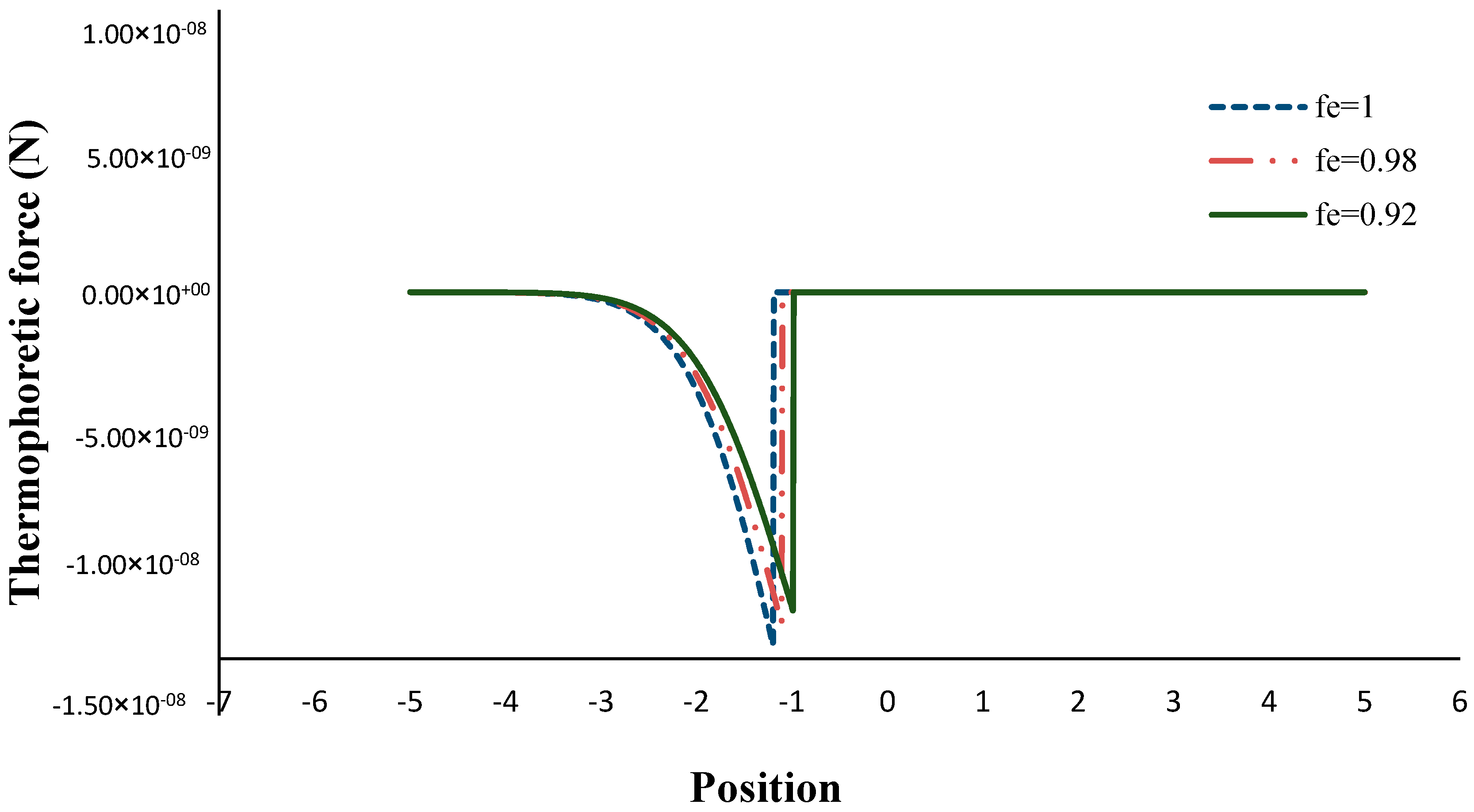
3. Results and Discussion
4. Conclusions
- The flame temperature increased by decreasing the volume porosity, fuel and oxidizer Lewis numbers.
- The flame sheet position moved toward the fuel nozzle with an increasing volume porosity and fuel Lewis number.
- The flame sheet position shifted toward the fuel nozzle by decreasing the oxidizer Lewis number.
- The thermophoretic force increased by decreasing the volume porosity, fuel and oxidizer Lewis numbers.
- The critical strain rate increased by decreasing the volume porosity, fuel and oxidizer Lewis numbers.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Strain rate | |
| Mean thermal velocity of gas molecular | |
| gaseous phase specific heat | |
| temperature jump coefficient | |
| Solid particle specific heat | |
| Temperature creep coefficient | |
| Velocity jump coefficient | |
| Gas velocity discontinuities coefficient | |
| Damkohler number | |
| Mass diffusivity coefficient of gaseous fuel (m2/s) | |
| Mass diffusivity coefficient of oxidizer (m2/s) | |
| Critical Damkohler number | |
| Thermal diffusivity coefficient (m2/s) | |
| activation energy (kj) | |
| Error function | |
| Porosity factor | |
| Heavi side function | |
| Gas thermal conductivity | |
| Lycopodium thermal conductivity | |
| Constant Defined in Equation (4) | |
| Mean free path | |
| Lewis number | |
| Mixture molecular mass () | |
| Fuel molecular mass () | |
| Oxygen molecular mass () | |
| Number of particle per volume unit | |
| Reaction heat per unit of fuel mass | |
| Dimensionless heat | |
| Universal constant of gases | |
| Particle radius | |
| Fuel temperature | |
| activation temperature (K) | |
| Flame temperature (K) | |
| Particle Start temperature of vaporization | |
| molecular weight of fuel | |
| Dimension length | |
| Flame position | |
| Vaporization front position | |
| Gaseous fuel mass fraction | |
| Oxidizer mass fraction | |
| Particle mass fraction | |
| Dimensionless fuel mass fraction | |
| Dimensionless oxidizer mass fraction | |
| Dimensionless mass fraction of solid particles | |
| Secondary coordinate axis | |
| Zeldovich number | |
| Greek symbols | |
| Initial mass fraction of oxidizer | |
| Volume porosity | |
| Θ | Dimensionless Temperature |
| Thermal conductivity of fuel or oxidizer | |
| Dynamic viscosity | |
| Fuel stoichiometric coefficient | |
| oxidizer stoichiometric coefficient | |
| Product stoichiometric coefficient | |
| Density | |
| Gaseous phase density | |
| Density of Solid particle | |
| constant time characteristic of vaporization | |
| Particle vaporization rate | |
| Rate of chemical reaction | |
| Constant and equal to 0.941 |
References
- Caputo, A.C.; Palumbo, M.; Pelagagge, P.M.; Scacchia, F. Economics of biomass energy utilization in combustion and gasification plants: Effects of logistic variables. Biomass Bioenergy 2005, 28, 35–51. [Google Scholar] [CrossRef]
- Wahlund, B.; Yan, J.; Westermark, M. Increasing biomass utilisation in energy systems: A comparative study of CO2 reduction and cost for different bioenergy processing options. Biomass Bioenergy 2004, 26, 531–544. [Google Scholar] [CrossRef]
- Demirbas, A.H.; Demirbas, I. Importance of rural bioenergy for developing countries. Energy Convers. Manag. 2007, 48, 2386–2398. [Google Scholar] [CrossRef]
- Hall, D.O.; Scrase, J.I. Will biomass be the environmentally friendly fuel of the future? Biomass Bioenergy 1998, 15, 357–367. [Google Scholar] [CrossRef]
- Hu, Y.; Kurose, R. Nonpremixed and premixed flamelets LES of partially premixed spray flames using a two-phase transport equation of progress variable. Combust. Flame 2018, 188, 227–242. [Google Scholar] [CrossRef]
- Bhoi, P.R.; Channiwala, S.A. Emission characteristics and axial flame temperature distribution of producer gas fired premixed burner. Biomass Bioenergy 2009, 33, 469–477. [Google Scholar] [CrossRef]
- Akbarzadeh, M.; Birouk, M. Effect of Fuel Nozzle Geometry on the Stability of Non-Premixed Turbulent Methane Flame. In Proceedings of the 23rd ICDERS, Irvine, CA, USA, 24–29 July 2011. [Google Scholar]
- Josh, N.D.; Berlad, A.L. Gravitational effects on stabilized, premixed, lycopodium-air flames. Combust. Sci. Technol. 1986, 47, 55–68. [Google Scholar] [CrossRef]
- Berlad, A.L.; Joshi, N.D. Gravitational effects on the extinction conditions for premixed flames. Acta Astronaut. 1985, 12, 539–545. [Google Scholar] [CrossRef]
- Berlad, A.L.; Tangirala, V.; Facca, L.R. Radiative structures of lycopodium-air flames in low gravity. J. Propuls. Power 1991, 7, 5–8. [Google Scholar] [CrossRef]
- Seshadri, K.; Berlad, A.L.; Tangirala, V. The structure of premixed particle-cloud flames. Combust. Flame 1992, 89, 333–342. [Google Scholar] [CrossRef]
- Han, O.S.; Yashima, M.; Matsuda, T.; Matsui, H.; Miyake, A.; Ogawa, T. Behavior of flames propagating through lycopodium dust clouds in a vertical duct. J. Loss Prev. Process. Ind. 2000, 13, 449–457. [Google Scholar] [CrossRef]
- Han, O.S.; Yashima, M.; Matsuda, T.; Matsui, H.; Miyake, A.; Ogawa, T. A study of flame propagation mechanisms in lycopodium dust clouds based on dust particles’ behavior. J. Loss Prev. Process. Ind. 2001, 14, 153–160. [Google Scholar] [CrossRef]
- Xi, Z.; Fu, Z.; Hu, X.; Sabir, S.; Jiang, Y. An experimental investigation on flame pulsation for a swirl non-premixed combustion. Energies 2018, 11, 1757. [Google Scholar] [CrossRef]
- Proust, C. Flame propagation and combustion in some dust-air mixtures. J. Loss Prev. Process. Ind. 2006, 19, 89–100. [Google Scholar] [CrossRef]
- Skjold, T.; Olsen, K.L.; Castellanos, D. A constant pressure dust explosion experiment. J. Loss Prev. Process. Ind. 2013, 26, 562–570. [Google Scholar] [CrossRef]
- Shamooni, A.; Cuoci, A.; Faravelli, T.; Sadiki, A. Prediction of Combustion and Heat Release Rates in Non-Premixed Syngas Jet Flames Using Finite-Rate Scale Similarity Based Combustion Models. Energies 2018, 11, 2464. [Google Scholar] [CrossRef]
- Rahbari, A.; Shakibi, A.; Bidabadi, M. A two-dimensional analytical model of laminar flame in lycopodium dust particles. Korean J. Chem. Eng. 2015, 32, 1798–1803. [Google Scholar] [CrossRef]
- Bidabadi, M.; Esmaeilnejad, A. An analytical model for predicting counterflow flame propagation through premixed dust micro particles with radiative heat loss. J. Loss Prev. Process. Ind. 2015, 35, 182–199. [Google Scholar] [CrossRef]
- Bidabadi, M.; Ramezanpour, M.; Poorfar, A.K.; Monteiro, E.; Rouboa, A. Mathematical Modeling of a Non-premixed Organic Dust Flame in a Counterflow Configuration. Energy Fuels 2016, 30, 9772–9782. [Google Scholar] [CrossRef]
- Xi, Z.; Fu, Z.; Hu, X.; Sabir, S.W.; Jiang, Y. An Investigation on Flame Shape and Size for a High-Pressure Turbulent Non-Premixed Swirl Combustion. Energies 2018, 11, 930. [Google Scholar] [CrossRef]
- Li, X.; Zhang, J.; Yang, H.; Jiang, L.; Wang, X.; Zhao, D. Combustion characteristics of non-premixed methane micro-jet flame in coflow air and thermal interaction between flame and micro tube. Appl. Therm. Eng. 2017, 112, 296–303. [Google Scholar] [CrossRef]
- Bidabadi, M.; Harati, M.; Afzalabadi, A.; Rahbari, A. Effect of Thermal Resistance on the Random Combustion of Micro-Organic Dust Particles. J. Energy Eng. 2017, 144, 04017073. [Google Scholar] [CrossRef]
- Spijker, C.; Raupenstrauch, H. Numerical investigation on inner particle effects in Lycopodium/Air dust deflagrations. J. Loss Prev. Process. Ind. 2017, 49, 870–879. [Google Scholar] [CrossRef]
- Chen, L.; Xia, Y.F.; Li, B.W.; Shi, J.R. Flame front inclination instability in the porous media combustion with inhomogeneous preheating temperature distribution. Appl. Therm. Eng. 2018, 128, 1520–1530. [Google Scholar] [CrossRef]
- Bidabadi, M.; Ebrahimi, F.; Bordbar, V. Modeling multi regional counter flow combustion of lycopodium dust cloud with considering radiative heat loss. J. Cent. South Univ. 2017, 24, 2638–2648. [Google Scholar] [CrossRef]
- Ji, W.; Yu, J.; Yu, X.; Yan, X. Experimental investigation into the vented hybrid mixture explosions of lycopodium dust and methane. J. Loss Prev. Process. Ind. 2018, 51, 102–111. [Google Scholar] [CrossRef]
- Di Benedetto, A.; Di Sarli, V.; Russo, P. On the determination of the minimum ignition temperature for dust/air mixtures. Chem. Eng. Trans. 2010, 19, 189–194. [Google Scholar]
- Sanchirico, R.; Russo, P.; Saliva, A.; Doussot, A.; Di Sarli, V.; Di Benedetto, A. Explosion of lycopodium-nicotinic acid–methane complex hybrid mixtures. J. Loss Prev. Process. Ind. 2015, 36, 505–508. [Google Scholar] [CrossRef]
- Sanchirico, R.; Russo, P.; Di Sarli, V.; Di Benedetto, A. On the explosion and flammability behavior of mixtures of combustible dusts. Process. Saf. Environ. Prot. 2015, 94, 410–419. [Google Scholar] [CrossRef]
- Sanchirico, R.; Di Sarli, V.; Russo, P.; Di Benedetto, A. Effect of the nozzle type on the integrity of dust particles in standard explosion tests. Powder Technol. 2015, 279, 203–208. [Google Scholar] [CrossRef]
- Kassebaum, J.L.; Chelliah, H.K. Oxidation of isolated porous carbon particles: Comprehensive numerical model. Combust. Theory Model. 2009, 13, 143–166. [Google Scholar] [CrossRef]
- Comtois, P. John Tyndall and the floating matter of the air. Aerobiologia 2001, 17, 193–202. [Google Scholar] [CrossRef]
- Davies, C.N. Aerosol Science; London Academic Press: London, UK, 1966. [Google Scholar]
- Braun, D.; Libchaber, A. Trapping of DNA by thermophoretic depletion and convection. Phys. Rev. Lett. 2002, 89, 188103. [Google Scholar] [CrossRef] [PubMed]
- Talbot, L.R.; Cheng, R.K.; Schefer, R.W.; Willis, D.R. Thermophoresis of particles in a heated boundary layer. J. Fluid Mech. 1980, 101, 737–758. [Google Scholar] [CrossRef]
- Bakanov, S.P. The thermophoresis of solids in gases. J. Appl. Math. Mech. 2005, 5, 767–772. [Google Scholar] [CrossRef]
- Rahbari, A.; Wong, K.F.; Vakilabadi, M.A.; Poorfar, A.K.; Afzalabadi, A. Theoretical investigation of particle behavior on flame propagation in lycopodium dust cloud. J. Energy Resour. Technol. 2017, 139, 012202. [Google Scholar] [CrossRef]
- Bidabadi, M.; Natanzi, A.H.; Mostafavi, S.A. Thermophoresis effect on volatile particle concentration in micro-organic dust flame. Powder Technol. 2012, 217, 69–76. [Google Scholar] [CrossRef]
- Haghiri, A.; Bidabadi, M. Modeling of laminar flame propagation through organic dust cloud with thermal radiation effect. Int. J. Therm. Sci. 2010, 49, 1446–1456. [Google Scholar] [CrossRef]
- Seshadri, K.; Trevino, C. The influence of the Lewis numbers of the reactants on the asymptotic structure of counterflow and stagnant diffusion flames. Combust. Sci. Technol. 1989, 64, 243–261. [Google Scholar] [CrossRef]
- Wang, H.Y.; Chen, W.H.; Law, C.K. Extinction of counterflow diffusion flames with radiative heat loss and nonunity Lewis numbers. Combust. Flame 2007, 148, 100–116. [Google Scholar] [CrossRef]
- Bidabadi, M.; Panahifar, P.; Sadeghi, S. Analytical development of a model for counter-flow non-premixed flames with volatile biofuel particles considering drying and vaporization zones with finite thicknesses. Fuel 2018, 231, 172–186. [Google Scholar] [CrossRef]
- Linan, A. The asymptotic structure of counterflow diffusion flames for large activation energies. Acta Astronaut. 1974, 1, 1007–1039. [Google Scholar] [CrossRef] [Green Version]
- Fendell, F.E. Ignition and extinction in combustion of initially unmixed reactants. J. Fluid Mech. 1965, 21, 281–303. [Google Scholar] [CrossRef]
- Huang, Y.; Risha, G.A.; Yang, V.; Yetter, R.A. Effect of particle size on combustion of aluminum particle dust in air. Combust. Flame 2009, 156, 5–13. [Google Scholar] [CrossRef]
- Wichman, I.S.; Yang, M. Double-spray counterflow diffusion flame model. Strain 1998, 2, 373–398. [Google Scholar] [CrossRef]
- Rockwell, S.R.; Rangwala, A.S. Modeling of dust air flames. Fire Saf. J. 2013, 59, 22–29. [Google Scholar] [CrossRef]
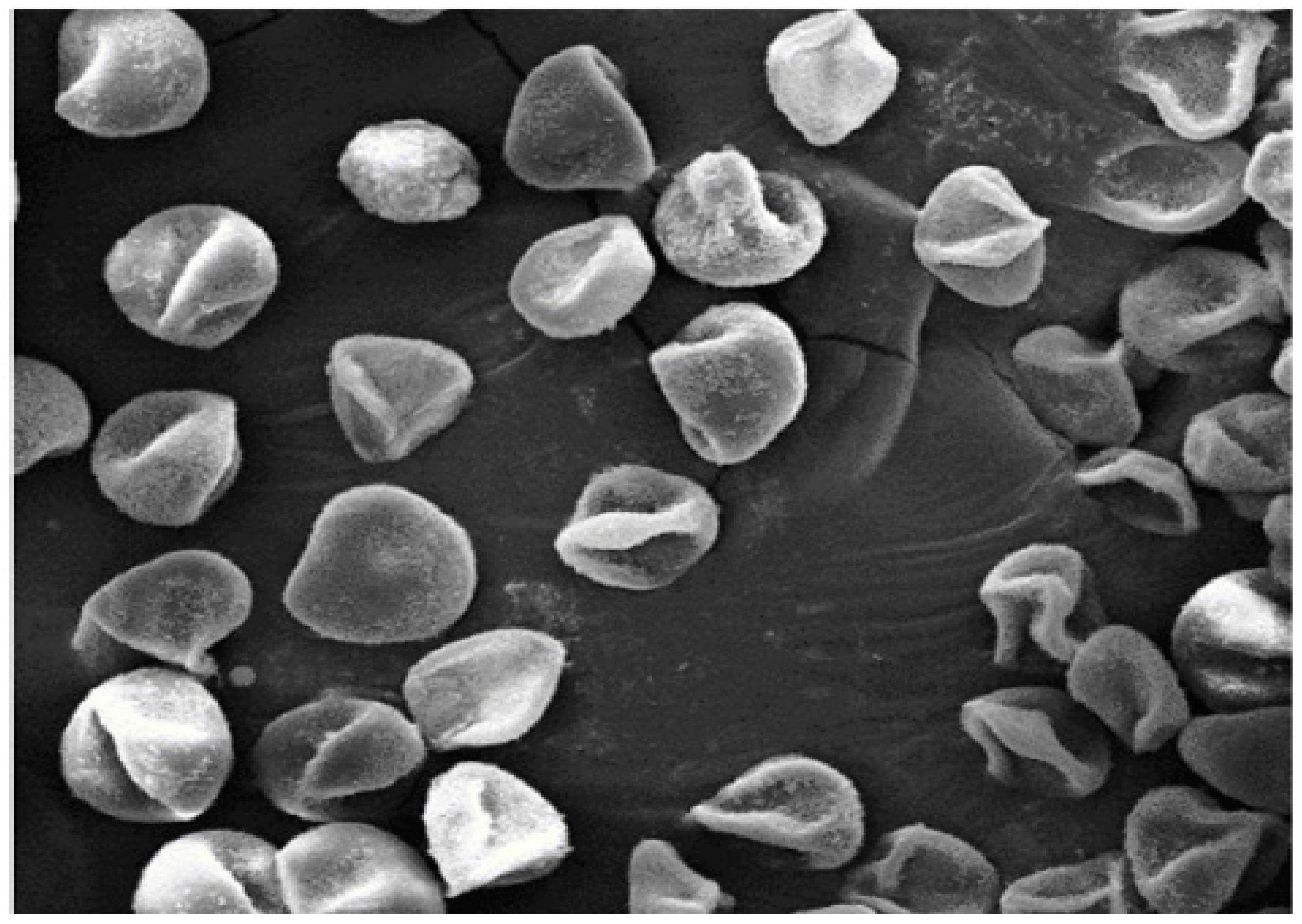






| Parameter | Value |
|---|---|
| 1.446538 × | |
| 0.3468 × | |
| 1.147 | |
| 2.2 | |
| 1.146 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bidabadi, M.; Ghashghaei Nejad, P.; Rasam, H.; Sadeghi, S.; Shabani, B. Mathematical Modeling of Non-Premixed Laminar Flow Flames Fed with Biofuel in Counter-Flow Arrangement Considering Porosity and Thermophoresis Effects: An Asymptotic Approach. Energies 2018, 11, 2945. https://doi.org/10.3390/en11112945
Bidabadi M, Ghashghaei Nejad P, Rasam H, Sadeghi S, Shabani B. Mathematical Modeling of Non-Premixed Laminar Flow Flames Fed with Biofuel in Counter-Flow Arrangement Considering Porosity and Thermophoresis Effects: An Asymptotic Approach. Energies. 2018; 11(11):2945. https://doi.org/10.3390/en11112945
Chicago/Turabian StyleBidabadi, Mehdi, Peyman Ghashghaei Nejad, Hamed Rasam, Sadegh Sadeghi, and Bahman Shabani. 2018. "Mathematical Modeling of Non-Premixed Laminar Flow Flames Fed with Biofuel in Counter-Flow Arrangement Considering Porosity and Thermophoresis Effects: An Asymptotic Approach" Energies 11, no. 11: 2945. https://doi.org/10.3390/en11112945
APA StyleBidabadi, M., Ghashghaei Nejad, P., Rasam, H., Sadeghi, S., & Shabani, B. (2018). Mathematical Modeling of Non-Premixed Laminar Flow Flames Fed with Biofuel in Counter-Flow Arrangement Considering Porosity and Thermophoresis Effects: An Asymptotic Approach. Energies, 11(11), 2945. https://doi.org/10.3390/en11112945






