Gas–Liquid Two-Phase Upward Flow through a Vertical Pipe: Influence of Pressure Drop on the Measurement of Fluid Flow Rate

Abstract

The accurate estimation of pressure drop during multiphase fluid flow in vertical pipes has been widely recognized as a critical problem in oil wells completion design. The flow of fluids through the vertical tubing strings causes great losses of energy through friction, where the value of this loss depends on fluid flow viscosity and the size of the conduit. A number of friction factor correlations, which have acceptably accurate results in large diameter pipes, are significantly in error when applied to smaller diameter pipes. Normally, the pressure loss occurs due to friction between the fluid flow and the pipe walls. The estimation of the pressure gradients during the multiphase flow of fluids is very complex due to the variation of many fluid parameters along the vertical pipe. Other complications relate to the numerous flow regimes and the variabilities of the fluid interfaces involved. Accordingly, knowledge about pressure drops and friction factors is required to determine the fluid flow rate of the oil wells. This paper describes the influences of the pressure drop on the measurement of the fluid flow by estimating the friction factor using different empirical friction correlations. Field experimental work was performed at the well site to predict the fluid flow rate of 48 electrical submersible pump (ESP) oil wells, using the newly developed mathematical model. Using Darcy and Colebrook friction factor correlations, the results show high average relative errors, exceeding ±18.0%, in predicted liquid flow rate (oil and water). In gas rate, more than 77% of the data exceeded ±10.0% relative error to the predicted gas rate. For the Blasius correlation, the results showed the predicted liquid flow rate was in agreement with measured values, where the average relative error was less than ±18.0%, and for the gas rate, 68% of the data showed more than ±10% relative error.

1. Introduction

In the oil and gas industry, multiphase flow in vertical pipes often occurs. The flow of fluids through the vertical pipe string causes a loss of energy through friction losses, where the value of this loss depends on the fluid flow viscosity and the size of the conduit. Often, the friction loss is an important part of the oil well completion design [1]. The pressure drop occurs as a result of the changes in potential and kinetic energy of the fluid due to the friction on the pipe walls [2]. Generally, the total pressure drop in the vertical conduit is basically related to four main components: frictional, hydrostatic, acceleration, and pressure drop. Among these four components, calculation of the pressure drop is the most complex component and has received extensive attention by researchers [3,4]. Many researchers have attempted to determine the two-phase frictional pressure drop over the whole range of flow patterns through a vertical pipe. A substantial number of experiments have been carried out to determine fluid flow friction losses in both Newtonian [1,2,5,6,7,8,9,10,11] and non-Newtonian systems [5,12]. A large number of experimental works was made in short tubes. Consequently, a lot of engineering problems come up when efforts are made to extend these experimental results to real oil field conditions where a longer pipe is used. In those experiments, the data shows only a limited number of variables, and as a result, imprecisions are introduced when the friction correlations are applied outside the limitations of the experimental data. As a consequence of the limited amount of data available for these experiments, the effects of some significant variables were ignored in the early studies [13,14,15,16,17]. The accuracy of the pressure drop prediction in flowing wells has a significant influence on the fluid flow measurement. There are many particular solutions, but they are valid only for some specific conditions. This is due to the complexity of two-phase flow analysis. In some conditions, the gas travels at a much higher velocity than the liquid. Accordingly, the flowing density of the gas–liquid mixture is higher than the corresponding density. Moreover, the liquid’s velocity inside the pipe wall can be different over a short distance and can cause a variable friction loss. The difference in velocity and flow regime of the two phases strongly affect pressure drop computations [13], meaning that slippage is a consequence of the difference between the combined velocities of the two phases, which is caused by the physical properties of the fluids involved. For single-phase flow, the frictional pressure losses do not normally increase with a decrease in the tubing size or an increase in well production flow rate. This refers to the existence of a gas phase, which tends to slip by the liquid phase without essentially contributing to its lift. Many researchers have tried to show a relationship between the slippage losses and the friction losses [15,16,17,18]. A method for the estimation of gas–liquid flow rates in the vertical pipe has been proposed [19]. The method was used to calibrate a differential pressure sensor to predict the flow rates of both phases in air–water flow. The estimations were in good agreement with real flow rate measurements. A study by Daev and Kairakbaev [20] proposed a new model of the liquid flow through pipes that incorporated flow straighteners. The prediction of the flow rate of liquid was studied and the parameters affecting the process of measuring the flow rate of liquid were considered. An experimental study of the two-phase flow regime and frictional pressure drop inside the pipe was done by Cai et al. [21]. The flow patterns were defined and recorded by a high-speed camera. A new empirical correlation was proposed based on the experimental results to predict the liquid multiplier factor of the test channel. A two-phase flow measurement applying a resistive void fraction meter combined to a venturi, or orifice plate, was suggested by Oliveira et al. [22]. This method was applied to determine the fluid mass flow rates using an air–water experimental apparatus. The results showed that the flow path has no important effect on the meters in relation to the frictional pressure drop in the experimental process range. The outcomes of the experimental work displayed a mean slip ratio of less than 1.1, when slug and bubbly flow patterns were lower than 70%.

This research work aims to evaluate the influence of a pressure drop on the measurement of the fluid flow rate in ESP oil wells. A new mathematical model was developed to determine the fluid flow rate of the oil wells through the prediction of multiphase flow parameter variations inside a vertical pipe based on local temperature and pressure changes with depth and applying multiphase flow physics equations and empirical correlations. The objective of this study was to obtain data from well tests conducted in a long vertical pipe and utilize this data to evaluate the effects of slippage and friction factor, in different flow regimes, on the calculation accuracies of the fluid flow rate of the oil wells. The approach measured the liquid hold-up along the conduit and used different friction correlations such as Blasius, Darcy, and Colebrook friction factor correlations to compare the predicted fluid flow rate with the measured fluid flow rate for each oil well. Generally, the results show that any errors in pressure drop calculation will generate inaccuracies in the prediction of fluid flow rate.

2. Experimental Arrangements and Measurement Procedure

The experiments conducted in the present study were carried out for two-phase flow through a vertical pipe of 48 oil wells using ESP pumps. A schematic of the experimental system is shown in Figure 1. The flow measurement starts at the surface wellhead, and then down to the bubble point pressure location depth in the well. Wellhead flowing pressure was measured at normal production conditions before and after the wing valve shut-in, leaving the ESP pump running, to measure the build-up of pressure at the wellhead. The total shut-in time period of the wellhead valve was then recorded. The first free gas bubbles started liberating from the bubble point location depth inside the tubing string. This occurred in the production flowing well before and after the wellhead wing valve shut-in, and the changes of flow patterns inside the pipe were reallocated once again, due to variations of temperature and pressure along the conduit. As a consequence, the liberated gas was dissolved in the oil phase, and the location depth of the bubble point pressure relocated to another position after the wellhead wing valve shut-in. The column of liquid that replaced the liberated gas column space, during the shut-in time period, was the difference between the first and second bubble point location depths. Figure 2 shows the bubble point location depths before and after the wellhead wing valve shut-in. A conceptual basis of physics for prediction of fluid flow rate in the conduit was employed along with multiphase empirical correlations to compute the variations of fluid flow parameters inside the tubing.

Several assumptions were made to conduct the calculations such as: assumed one-dimensional flow in the conduit, assumed uniform cross-sectional area of the pipe, the phase’s properties varied with depth, the frictional factor varied along the conduit, and the effect of the liquid compressibility was neglected.

2.1. Required Input Data

The input data required were the well parameters data and physical properties data of the fluid, as seen in

Table 1. Well and physical properties of the fluid.

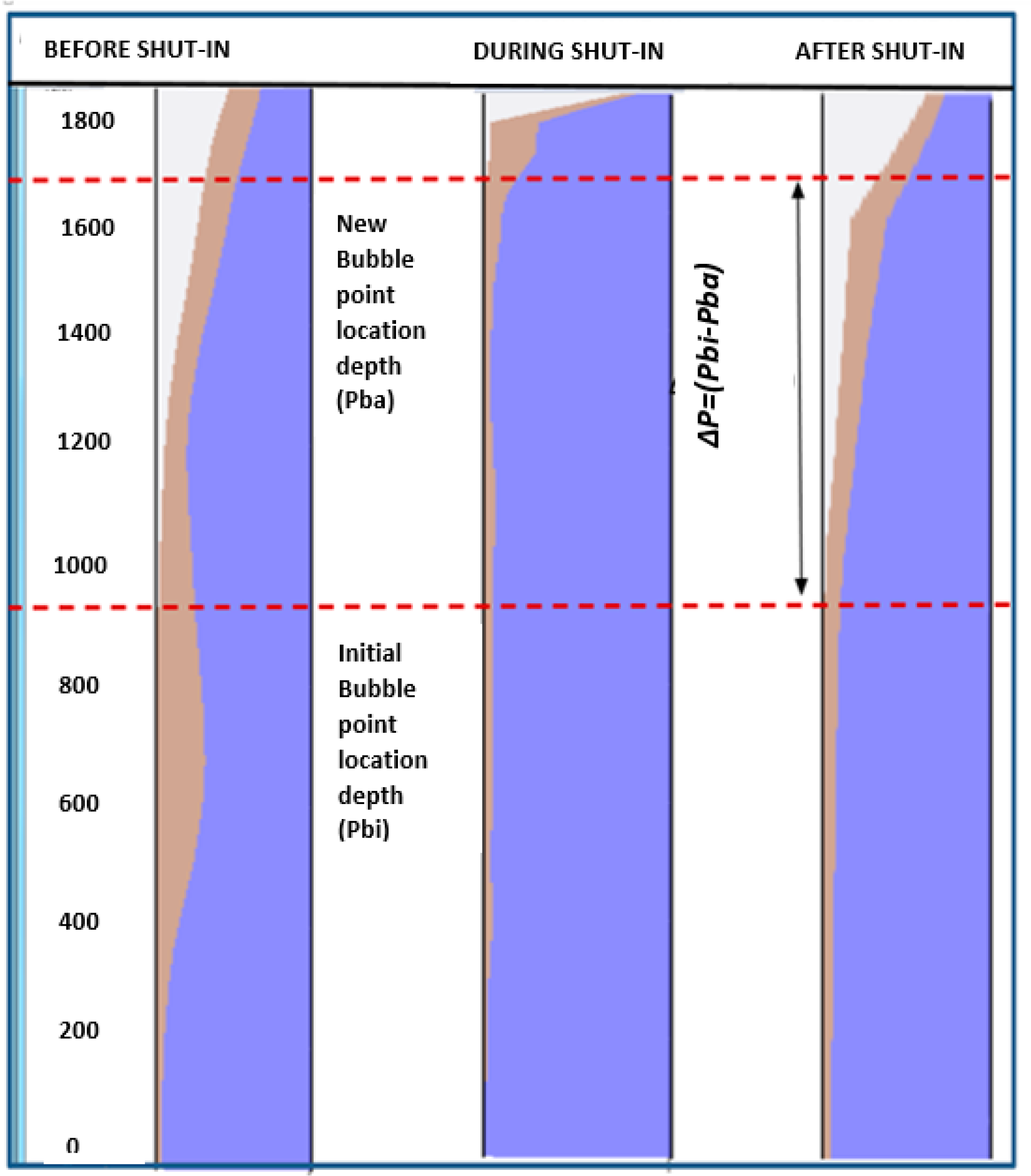
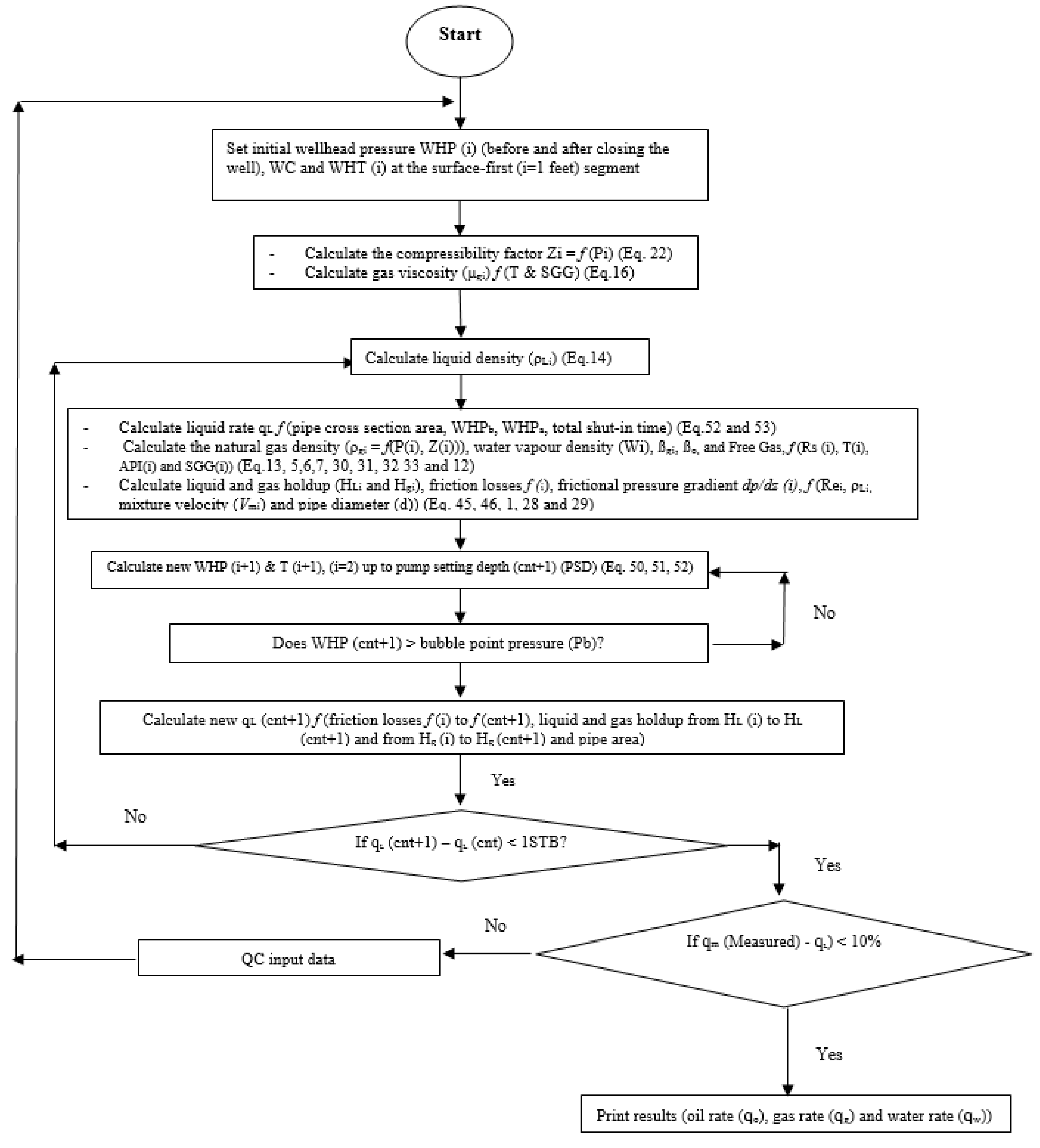
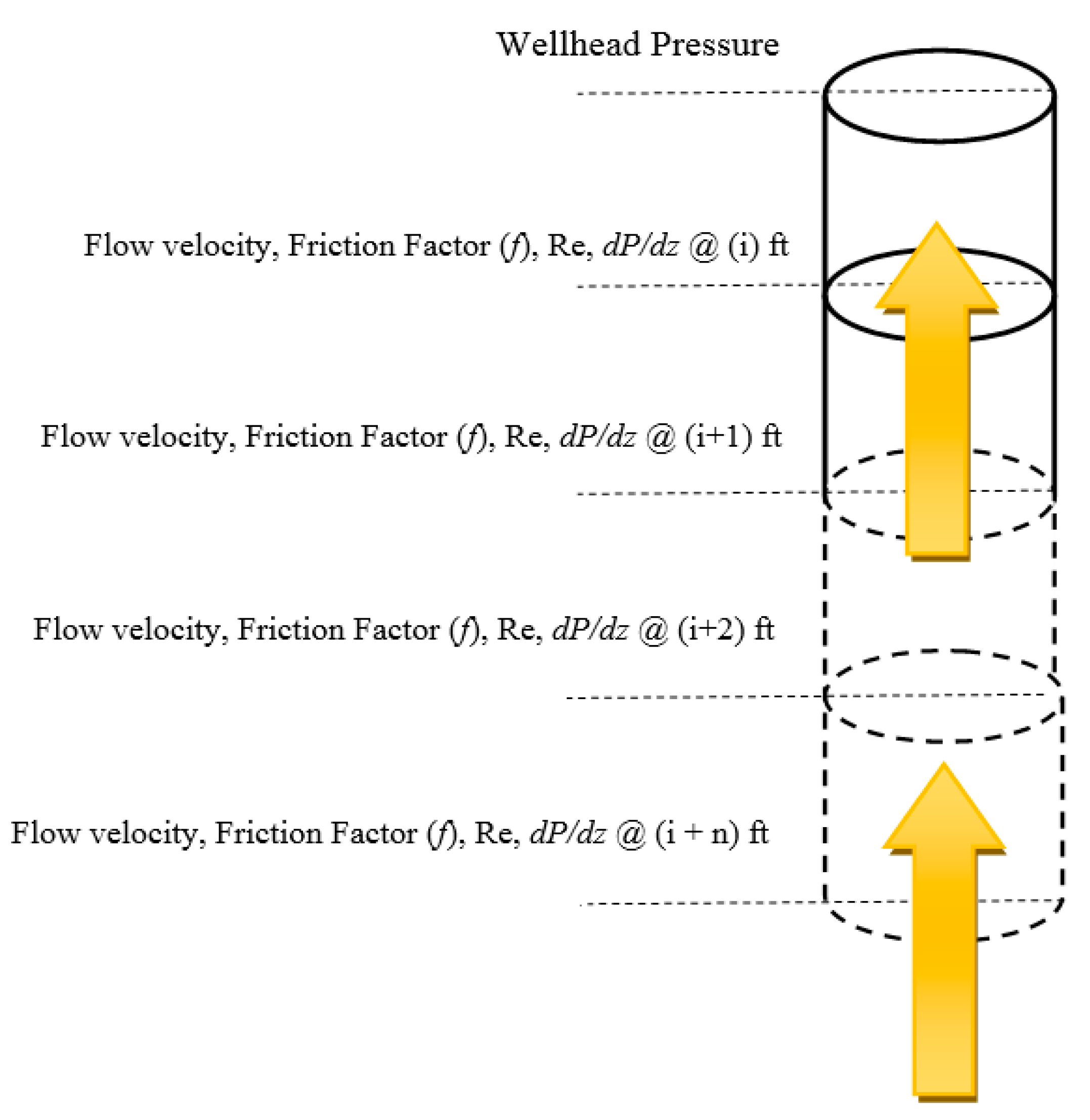
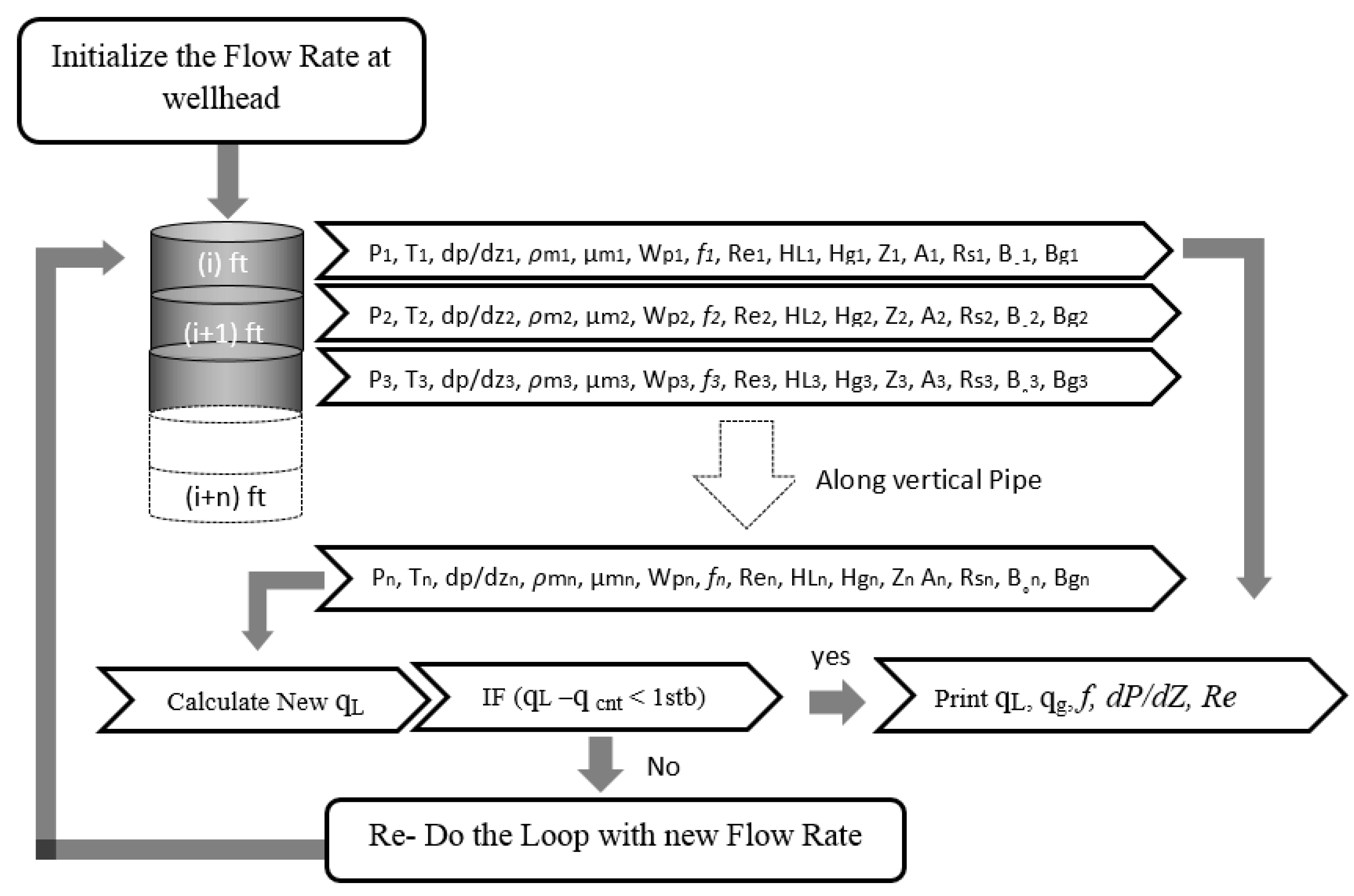
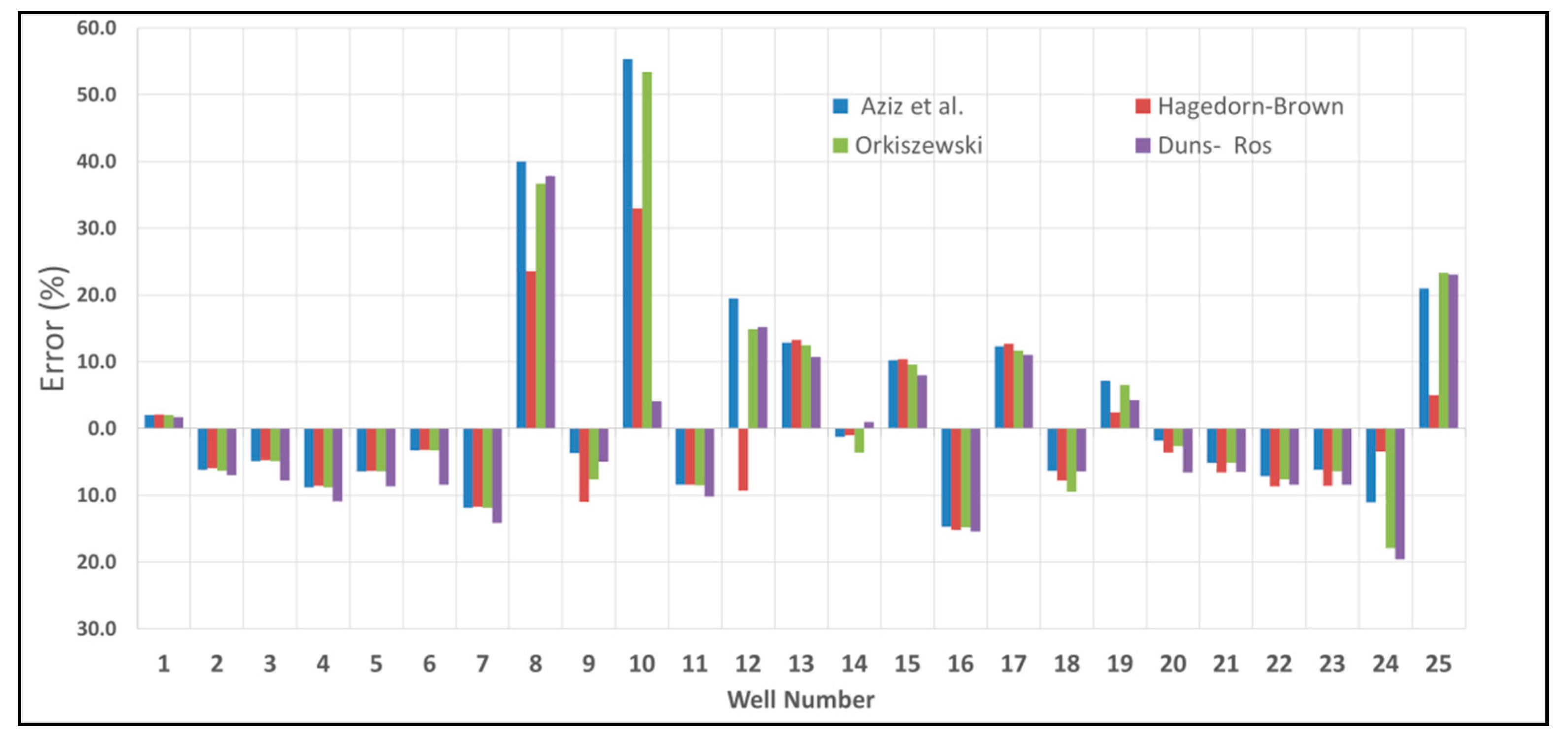
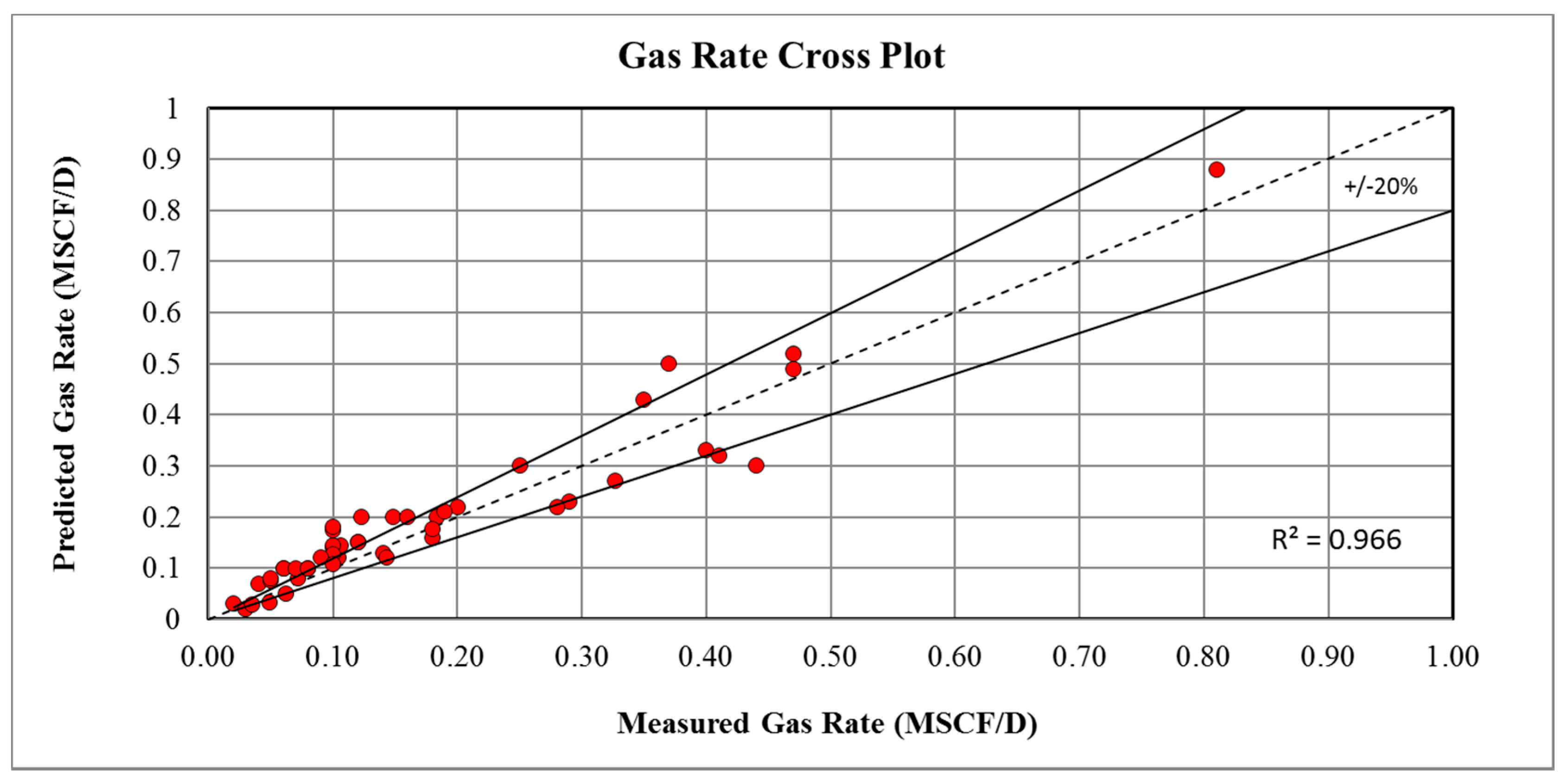
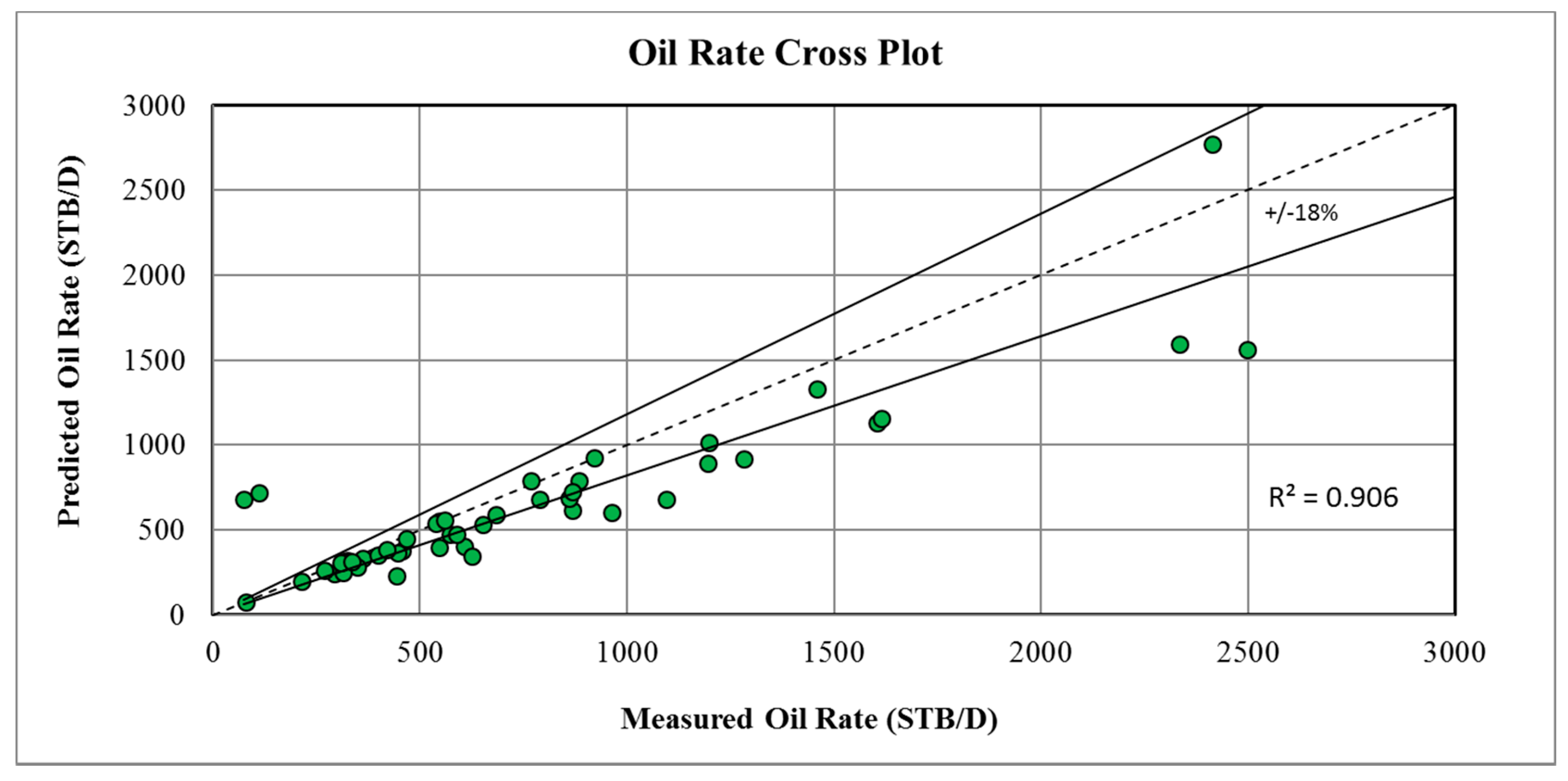
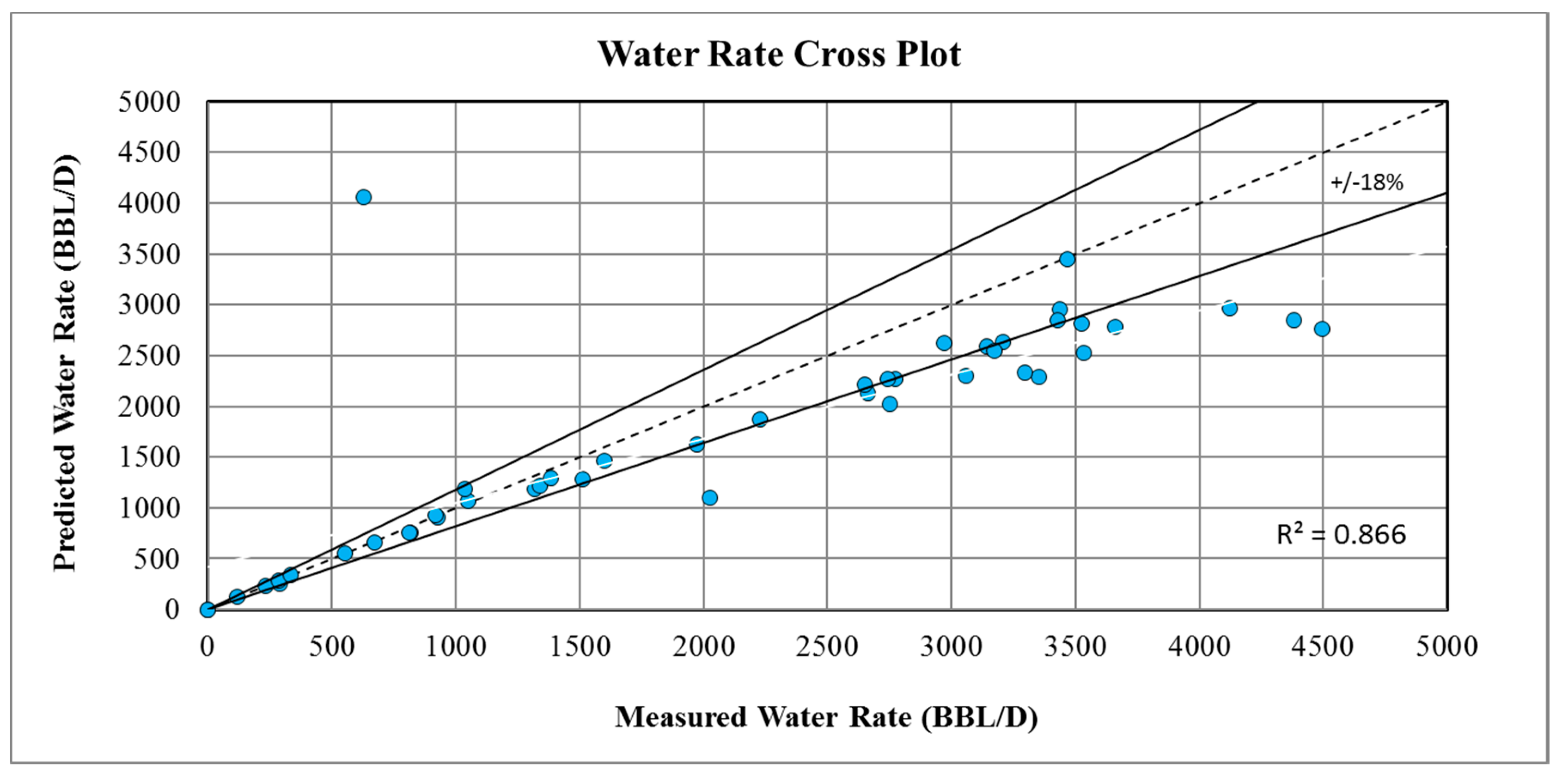
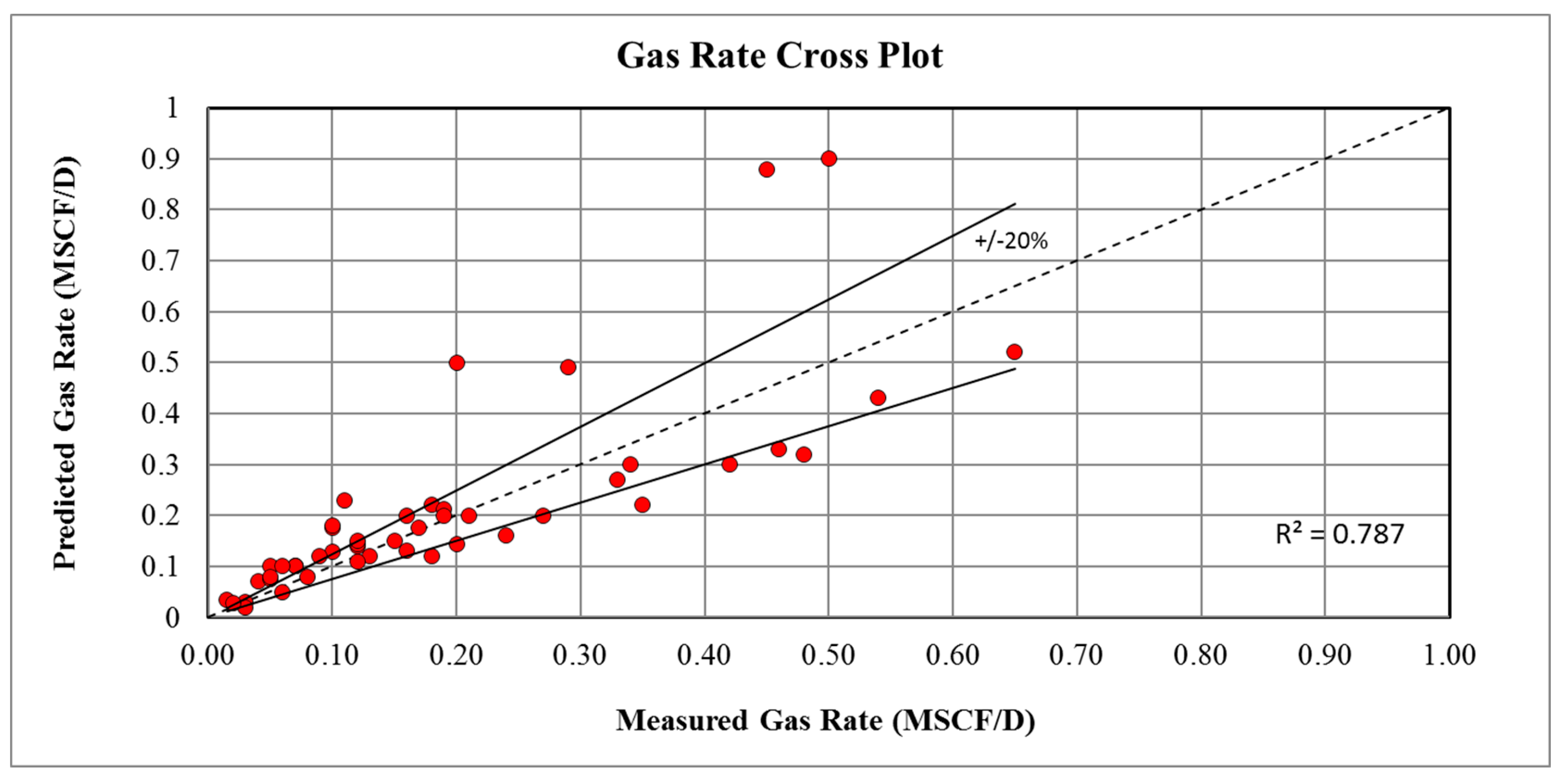
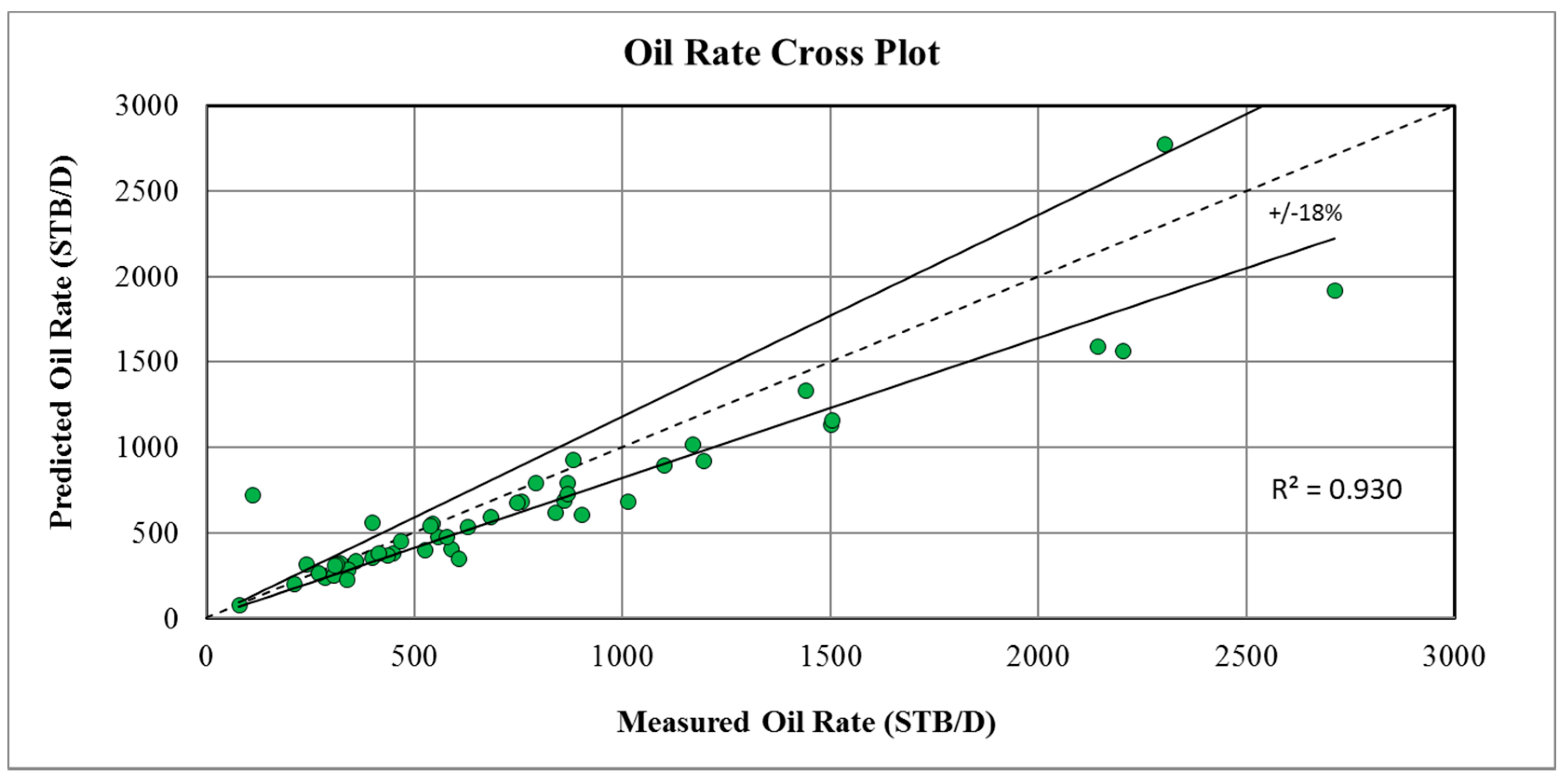
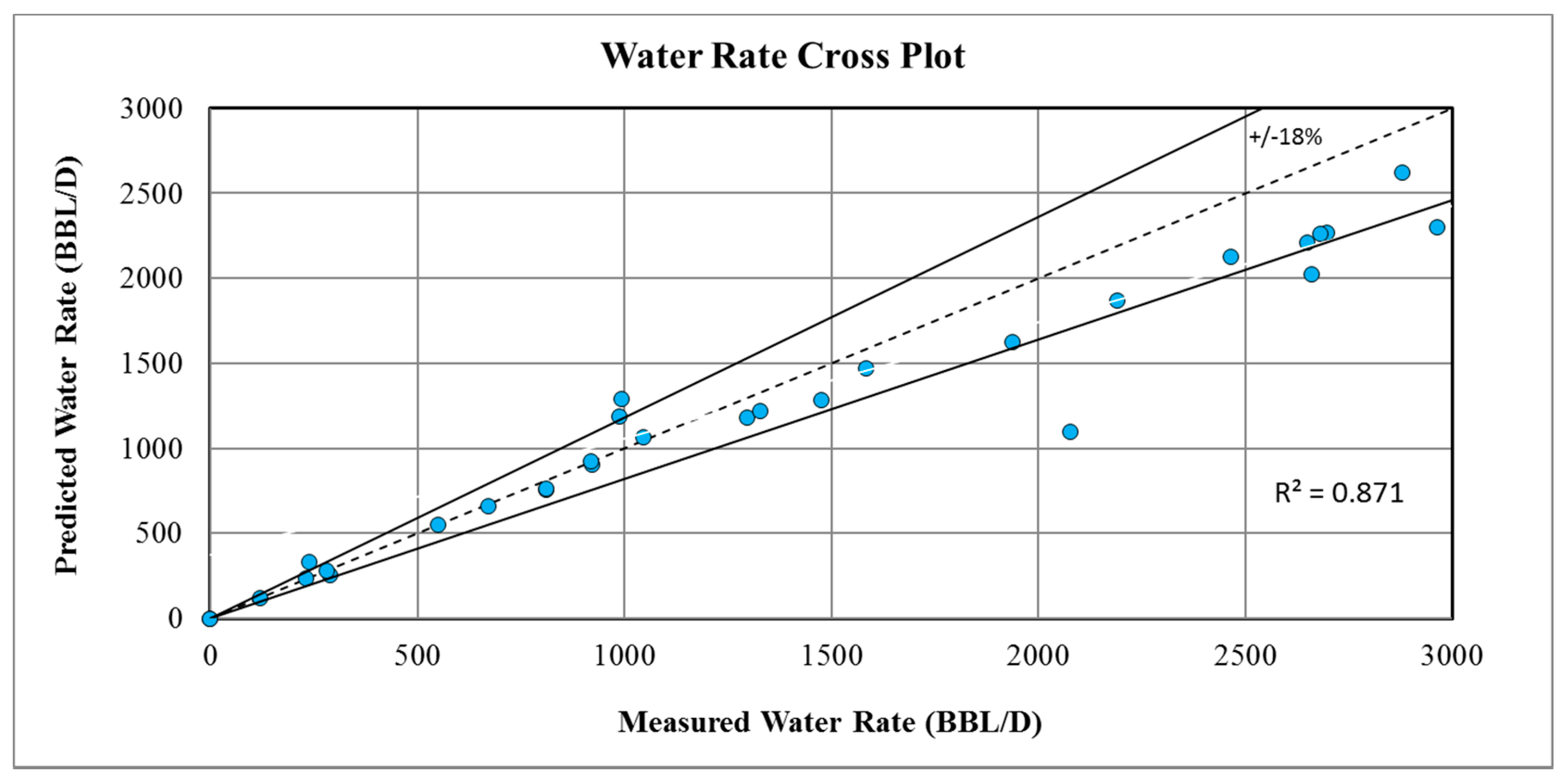
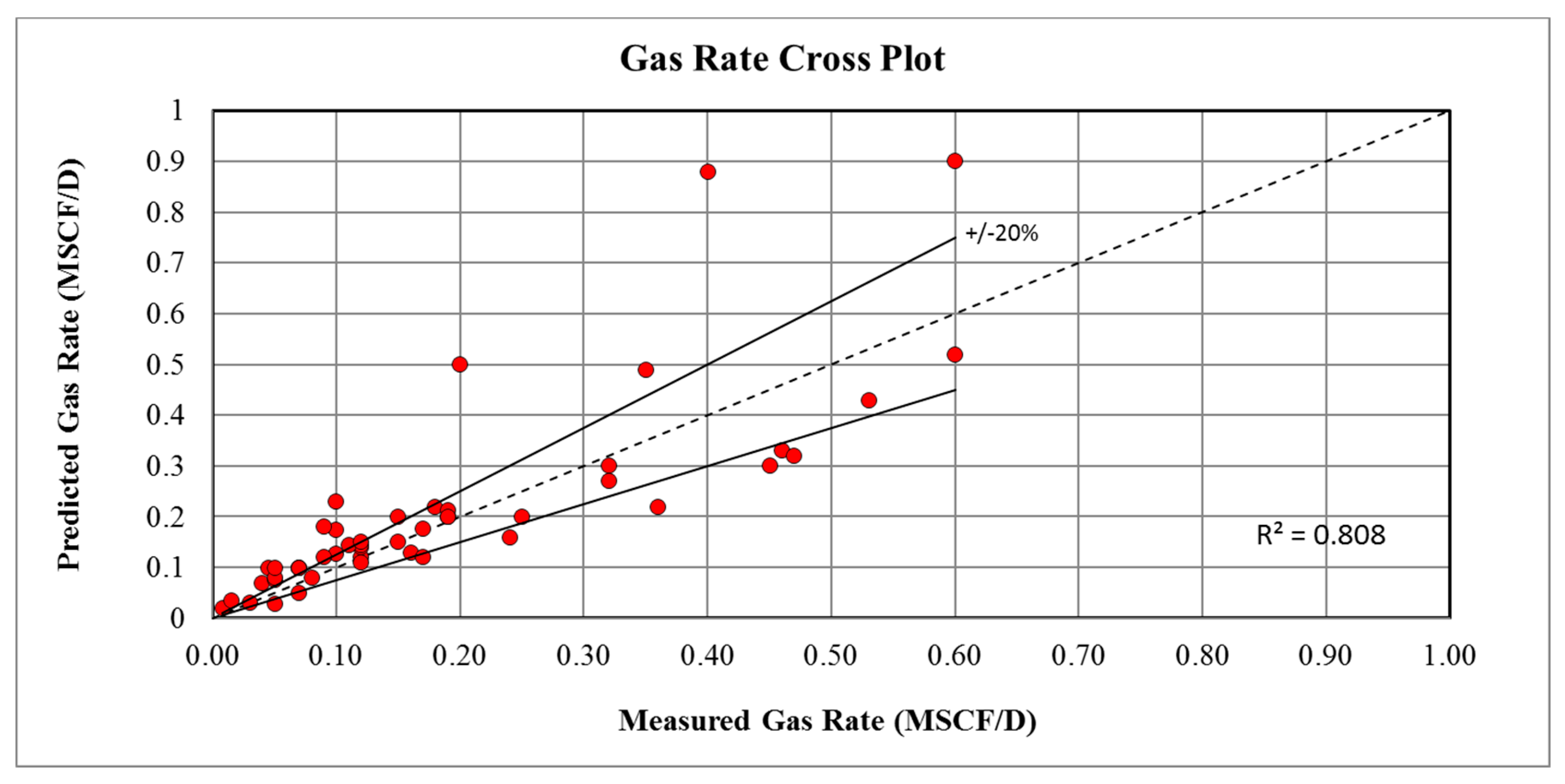
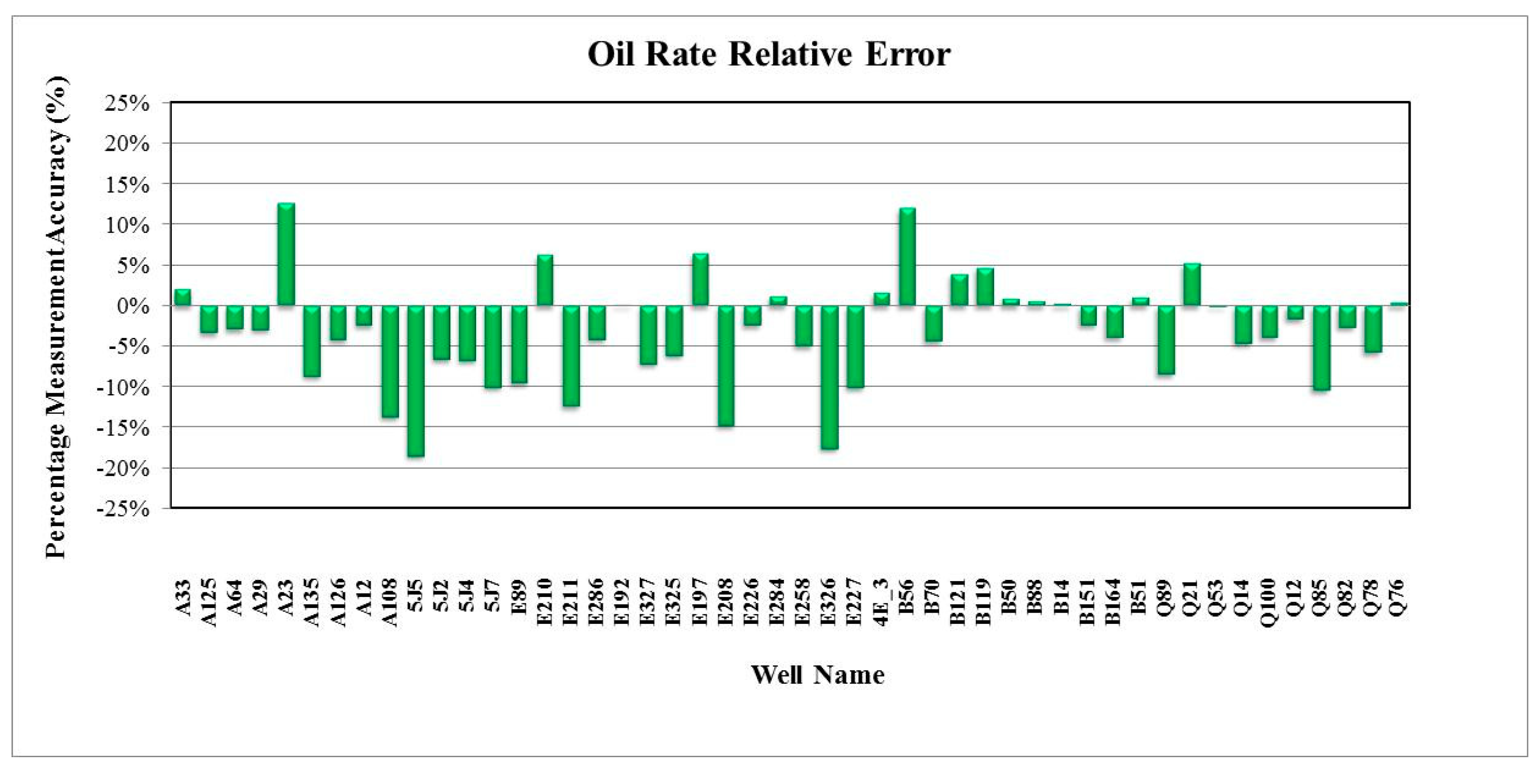
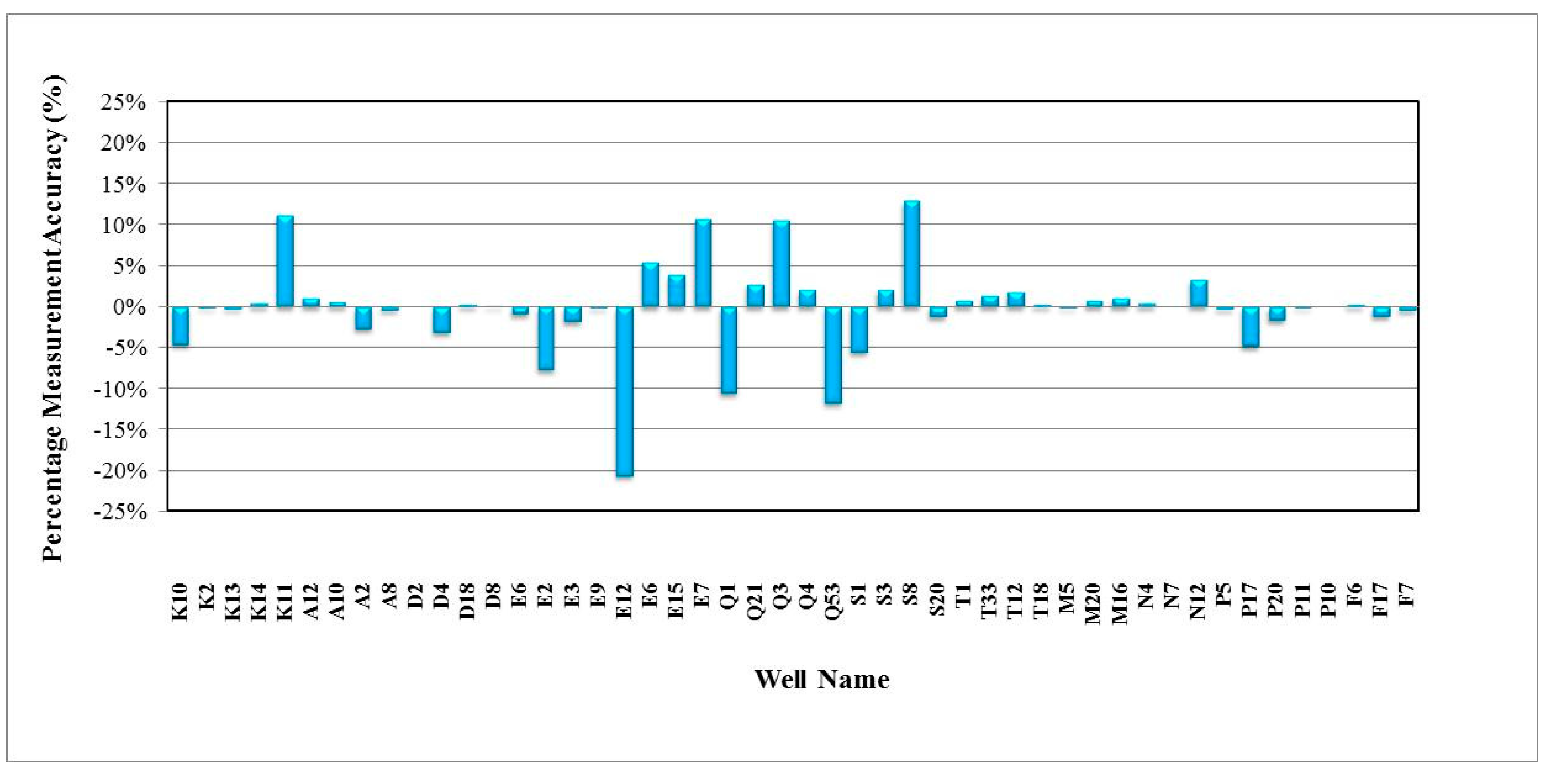
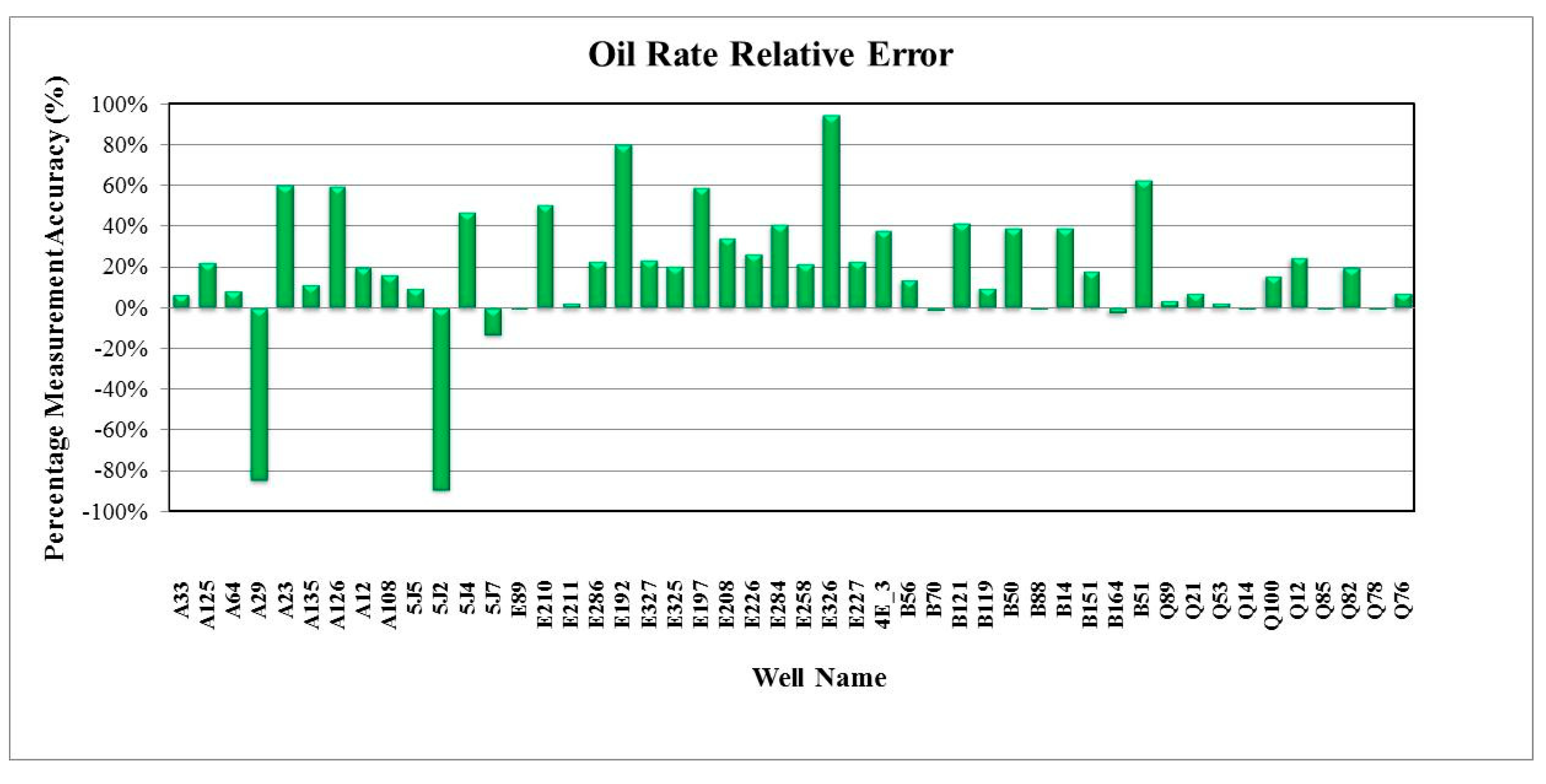
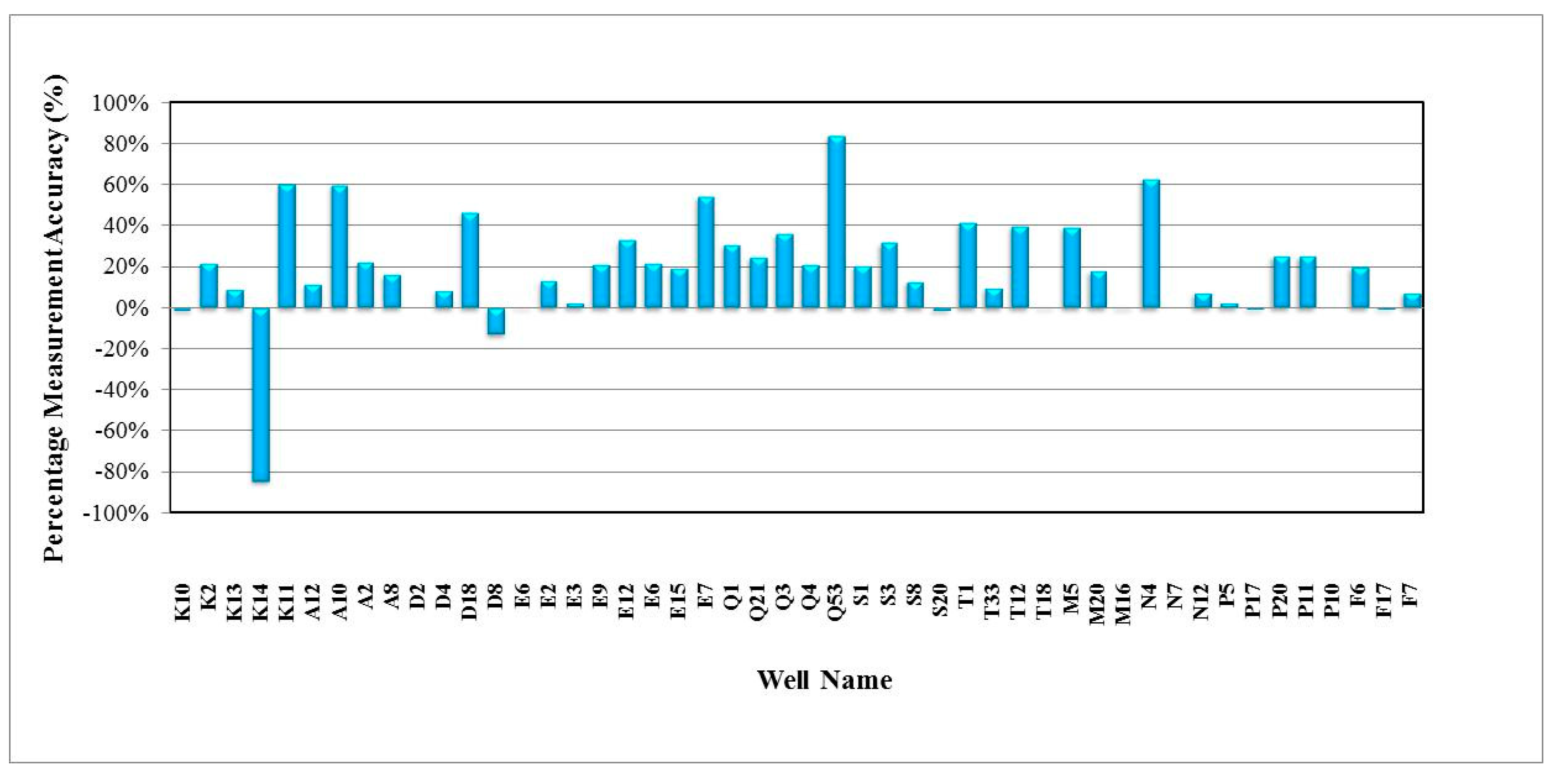
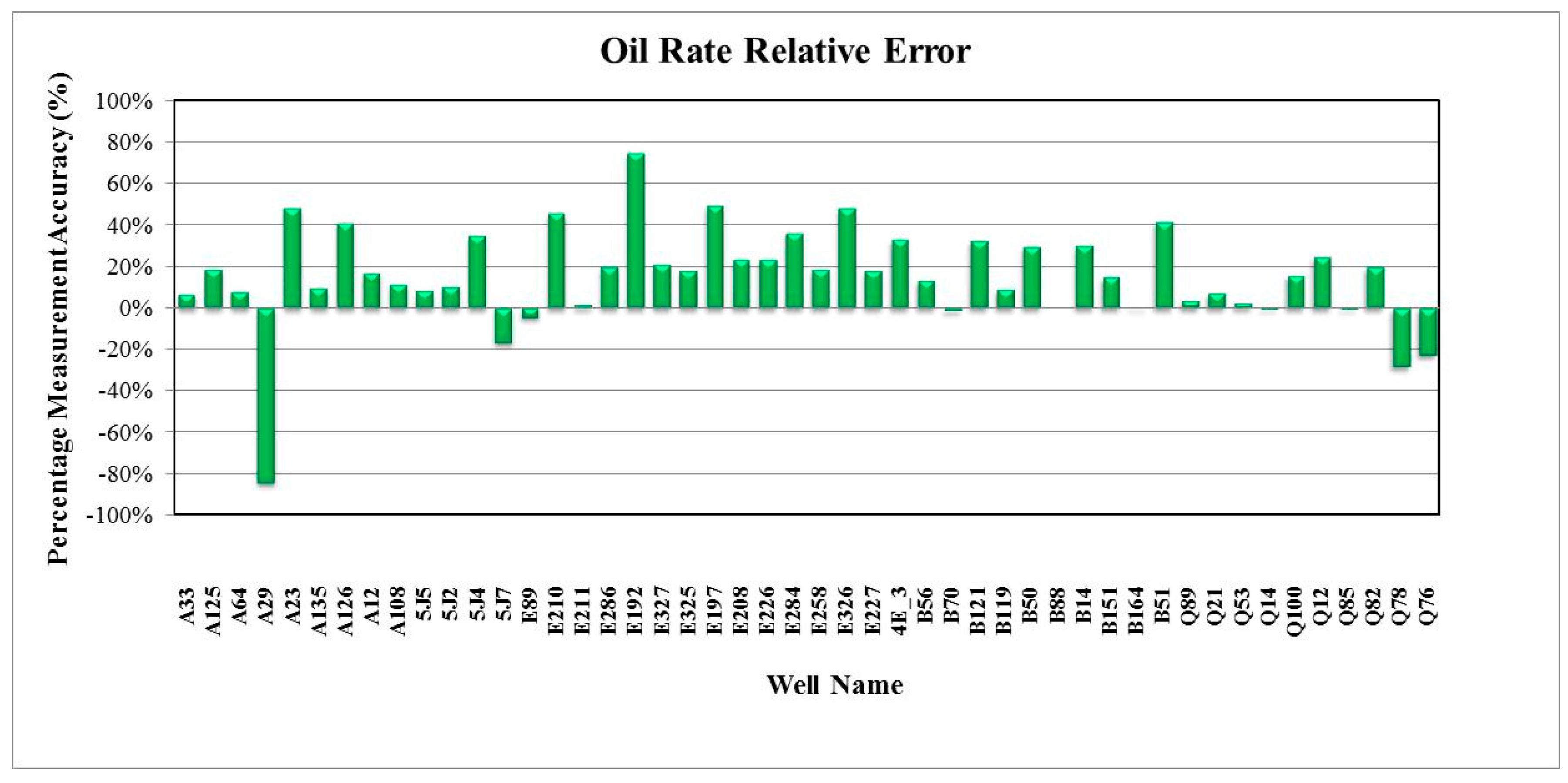
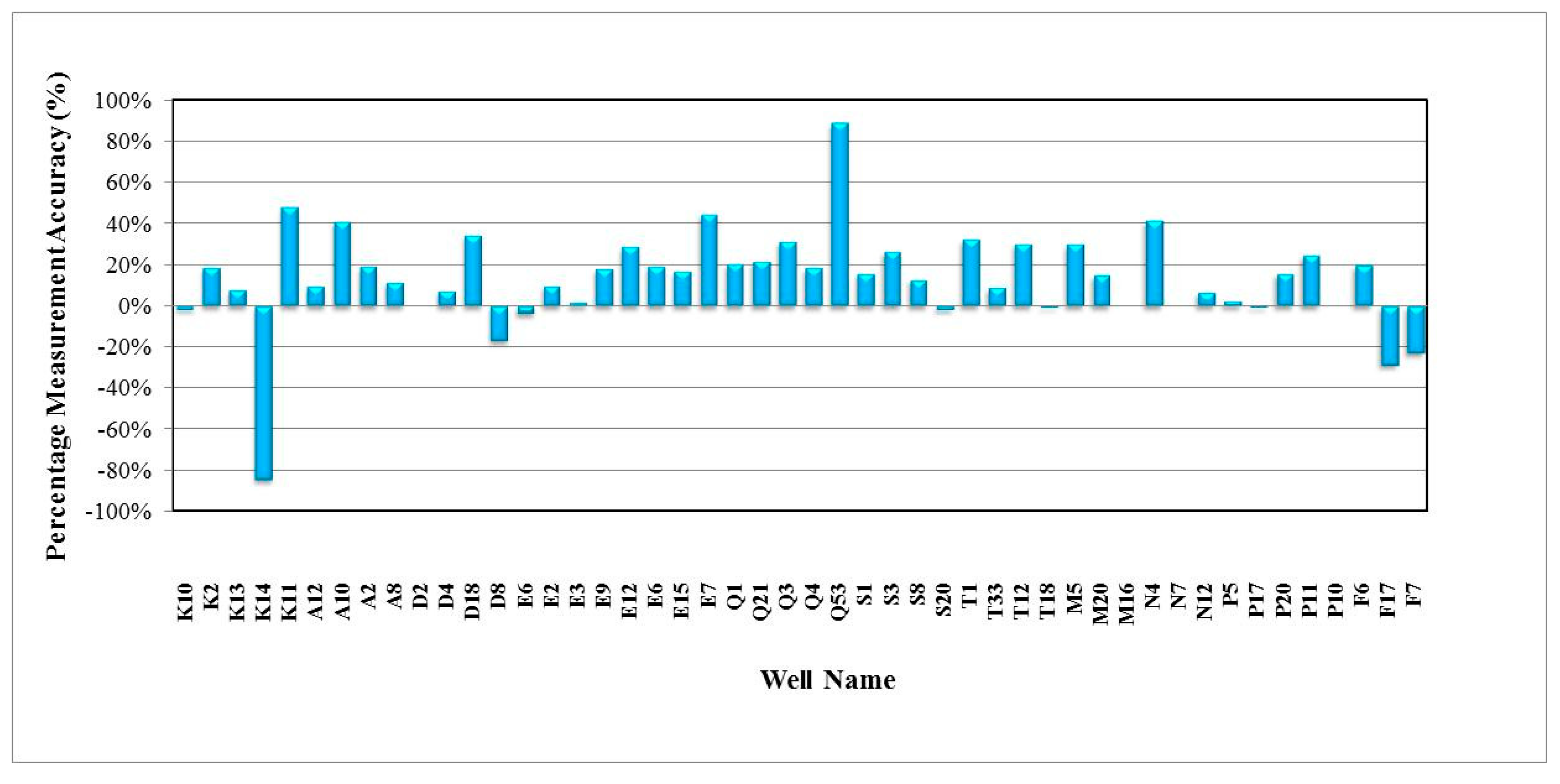
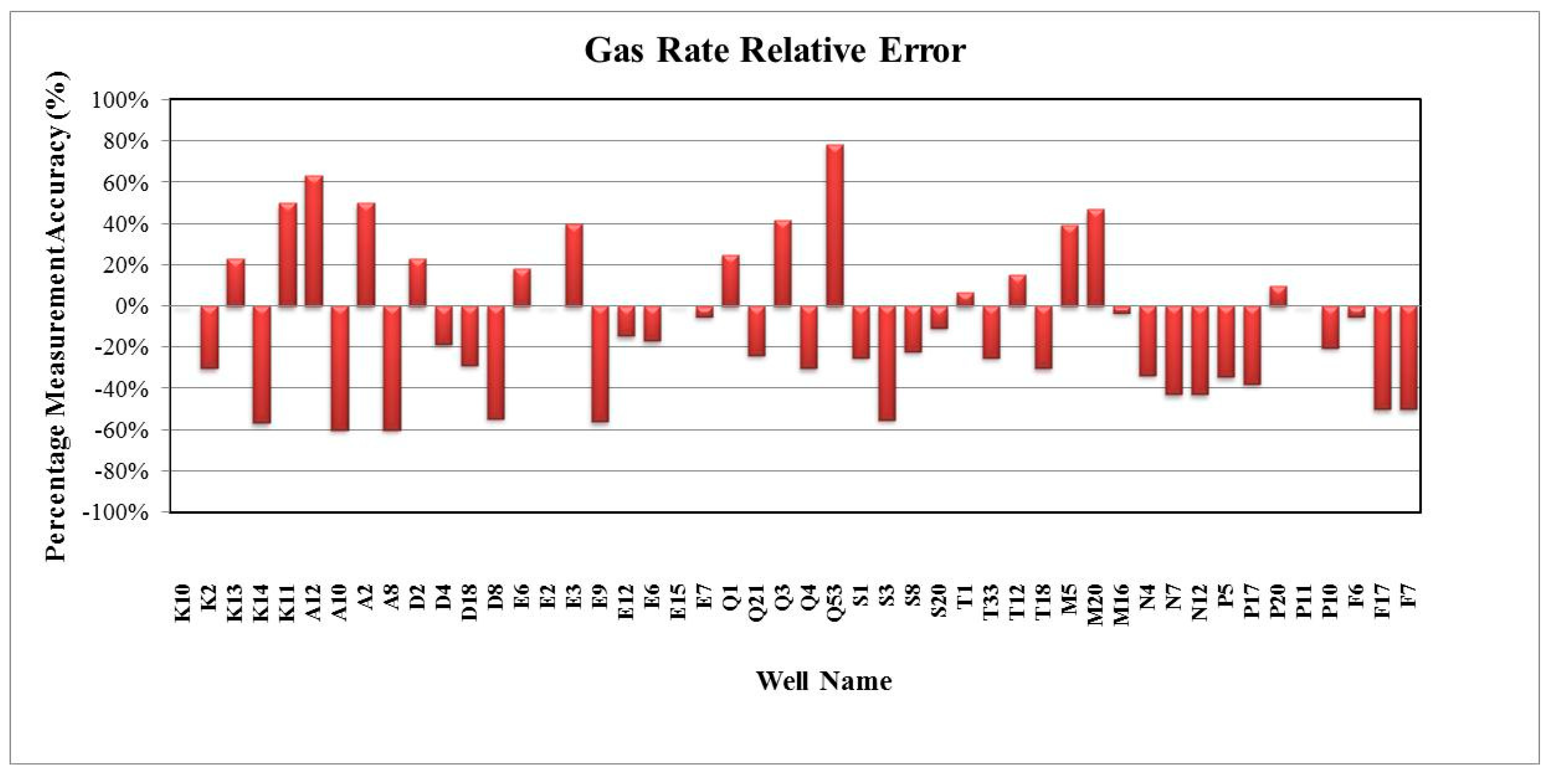
Well Name	WHPb	WHPa	WHT	GOR	WC	Total Shut-in Time
	(PSIA)	(PSIA)	(F)	(SCE/STB)	(%)	(min)
A33	140	200	98	360.92	93	1.55
A125	100	200	95	360.92	91.62	0.83
A64	180	250	107	360.92	81.52	1.06
A29	250	270	107	360.92	84.88	0.80
A23	210	260	127.7	360.92	82.11	0.24
A135	210	250	100	360.92	59.88	0.56
A126	250	300	98	360.92	66.91	0.20
A12	175	270	107	360.92	82.9	0.84
A108	260	300	97	360.92	81.31	0.28
5J5	150	300	95	360.92	4	3.36
5J2	100	170	101	360.92	52	1.39
5J4	250	300	101.6	360.92	58.95	0.27
5J7	250	300	98	360.92	30	0.47
E89	150	190	140	300	79	0.27
E210	80	120	110	300	83	0.33
E211	80	120	129	300	74	0.96
E286	70	100	146	300	90	0.25
E192	80	110	146	300	83	0.24
E327	80	110	115.5	300	77	0.35
E325	70	110	124.6	300	83	0.44
E197	90	110	146.1	300	82	0.11
E208	95	110	146.8	300	81	0.07
E226	80	110	138.4	300	91	0.25
E284	80	120	124.5	300	76	0.33
E258	65	90	142	300	86	0.23
E326	60	100	113	300	82	0.48
E227	100	150	142	300	84	0.36
4E_3	130	300	146	300	87	1.18
B56	120	170	120	384	42	2.3
B70	160	230	120	384	29.9	2.9
B121	100	160	120	364	67.29	0.5
B119	100	160	120	364	76.18	1.1
B50	180	250	110	364	68.65	0.55
B88	100	160	110	364	63.59	2.1
B14	250	270	110	364	76.28	0.15
B151	180	230	110	364	55.78	0.66
B164	100	170	120	364	26.3	2.1
B51	240	310	120	364	59.05	0.44
Q89	100	150	120	364	0	3.7
Q21	80	150	120	364	79.22	2.1
Q53	80	150	120	364	71.41	2.3
Q14	75	130	120	364	74.83	1.4
Q100	80	130	110	364	78.27	0.55
Q12	80	150	110	364	80.33	0.58
Q85	100	150	110	364	18.18	2.5
Q82	100	150	110	364	75.3	0.5
Q78	80	150	120	364	37.27	2.5
Q76	80	150	120	364	80.5	1.3

. To carry out this study, 48 ESP oil wells were selected where the wells were producing from four different reservoirs using same production pipe diameter. Also, these reservoirs had almost the same reservoir fluid properties: the bubble point pressure ranged from 924 psi to 1124 psi, the American Petroleum Institute (API) oil gravity ranged from 36 to 37 @ 60 °F, the oil viscosity ranged from 0.784 cP to 1.0119 cP, and the reservoir temperature ranged from 157 °F to 186 °F. Furthermore, Figure 3 classifies the input data required.

2.2. Computational Algorithm

Figure 4 shows the algorithm steps to evaluate the mathematical model. The algorithm classified all the main stages and sub-steps in the model. In this process, the calculations were performed to obtain the bubble point pressure location depth before and after the wellhead wing valve shut-in. The fluid flowing pressure gradient could be calculated anywhere inside the pipe. All the variables needed to be identified to correctly evaluate the physics interactions between all the fluid parameters using the suitable multi-physics equations and empirical correlations.

The calculation starts at the surface wellhead and then down to the location depth of the bubble point pressure as a function of temperature and pressure variations with depth. To consider the fact that flow regimes vary depending on the in situ flow rates of gas/liquid, the model calculates, at each foot along the vertical pipe, the variations of supercritical velocities, viscosities, and densities for both phases (liquid and gas). The in situ flow rate can also be calculated by the mathematical model at any flow regime at any depth. As shown in Figure 5 and Figure 6, the calculation iteration can stop at any depth (i, ..., i + n) using all the equations (from Equation (1) to Equation (56)), where there is a different flow regime along the vertical pipe.

The following are the physics equations and the correlations applied to determine each independent variable at every single foot.

Total pressure losses expressed as

$$\Delta P_{Total}=\Delta P_{HH}+\Delta P_{Frictional}$$

(1)

Hydrostatic head is expressed as

$$\Delta P_{HH}=\frac{{\rho }_{m}g\Delta Z}{144g_c}$$

(2)

Darcy–Weisbach equation [23] was used to calculate the frictional pressure loss

$$\Delta P=f\frac{L}{D}\frac{\rho V^2}{2}$$

(3)

Reynolds number is given by

$$Re=\frac{2.2\times {10}^{-2}{m}_t}{D{\mu }_L{}^{H_L}{\mu }_g{}^{\left(1-H_L\right)}}$$

(4)

Three different friction factor correlations were applied to evaluate the impact of the friction on the computation of the fluid flow rate. The first correlation is the Blasius empirical correlation for turbulent flow [24].

$$f=0.316{\left(Re\right)}^{-0.25}$$

(5)

The second friction correlation applied is Darcy correlation [23]

$$f=\frac{64}{Re}$$

(6)

The third friction factor correlation applied is from Colebrook [25]

$$\frac{1}{\surd f}=2lo{g}_{10}\left(\frac{\epsilon /D_h}{3.7}+\frac{2.51}{R_{e\surd f}}\right)$$

(7)

for Re <≈ 2300 and Re >≈ 4000.

The gas density is expressed as

$${\rho }_g=\frac{m_g}{V_R}=\frac{M_{g}P}{ZRT}$$

(8)

The density at wellbore condition, is given by

$${\rho }_g=\frac{{\rho }_{gs}}{B_g}$$

(9)

The oil density is expressed as

$${\rho }_o=\frac{62.428{\gamma }_o+0.014{\gamma }_g{R}_s}{B_o}$$

(10)

where

$${\gamma }_o={\rho }_o/{\rho }_w$$

(11)

$${\gamma }_g=\frac{{\rho }_g}{{\rho }_{air}}=\frac{{\rho }_g}{0.077}$$

(12)

$${\rho }_g=0.077{\gamma }_g$$

(13)

Liquid density is given by

$${\rho }_L={\rho }_{W}WC+{\rho }_o\left(1-WC\right)$$

(14)

Mixture density is expressed as

$${\rho }_m={\rho }_L{H}_L+{\rho }_g\left(1-H_L\right)$$

(15)

The gas viscosity is determined by the following equation [26]:

$${\mu }_g=K_{1}exp\left(X{\rho }^Y\right)$$

(16)

where

$$\rho =\frac{p{M}_g}{zRT}=0.0015\frac{p{M}_g}{zT}$$

(17)

$$K_1=\frac{\left(0.001+2\times {10}^{-6}{M}_g\right)T^{1.5}}{\left(209+19M_g+T\right)}$$

(18)

$$X=3.5+\frac{986}{T}+0.01M_g$$

(19)

$$Y=2.4-0.2X$$

(20)

Mixture viscosity is given by

$${\mu }_m={\mu }_L^{H_L}+{\mu }_g^{\left(1-H_L\right)}$$

(21)

Beggs and Brill equation [27] was applied to estimate the gas compressibility factor (Z)

$$Z=A+\frac{\left(1-A\right)}{e^B}+CP{r}^D$$

(22)

Using Standing and Katz equations [28] to obtain the pseudo critical temperature and pressure of the gas mixture

$$Pr=688.634-21.983{\gamma }_g-13.886{\gamma }_g^2$$

(23)

$$Tr=158.01+342.12{\gamma }_g-16.04{\gamma }_g^2$$

(24)

and

$$A=1.39{\left(Tr-0.92\right)}^{0.5}-0.36Tr-0.101$$

(25)

$$B=\left(0.62-0.23Tr\right)Pr+\left(\frac{0.066}{Tr-0.86}-0.037\right)P{r}^2+\frac{0.32}{{10}^{9\left(Tr-1\right)}}P{r}^2$$

(26)

$$C=0.132-0.32log\left(Tr\right)$$

(27)

$$D={10}^{\left(0.302-0.49Tr+0.182T{r}^2\right)}$$

(28)

Superficial gas velocity is expressed as

$$V_{sg}=\frac{4q_g{B}_g}{\pi D^2}$$

(29)

Superficial liquid velocity is expressed as

$$V_{sL}=\frac{4q_L}{\pi D^2}$$

(30)

The water vapor density using the Sloan correlation [29] is expressed as

$$W=exp\left(c_1+\frac{c_2}{T}+c_{3}ln\left(p\right)+\frac{c_4}{T^2}+\frac{c_{5}ln\left(P\right)}{T}+c_6{\left(ln\left(P\right)\right)}^2\right)$$

(31)

where the values of constants c₁ to c₆ are shown in

Table 2. Constants c₁ to c₆.

Constants	Value
c1	28.911
c2	−9668.146
c3	−1.663
c4	−130,823.5
c5	205.323
c6	0.0385

.

The gas formation volume factor is expressed as

$$B_g=\frac{P_{sc}ZT}{T_{sc}P}=0.028\frac{ZT}{P}$$

(32)

Using the Vasquez and Beggs equation [30] to obtain the oil formation volume factor

$$B_{ob}=1+C_1{R}_{sb}+C_2\left(T-60\right)\left(\frac{{\gamma }_{API}}{{\gamma }_g}\right)+C_3{R}_{sb}\left(T-60\right)\left(\frac{{\gamma }_{API}}{{\gamma }_g}\right)$$

(33)

and oil gas ratio

$$R_{sb}=\frac{{\gamma }_{g}P{b}^{C_2}}{C_1}{10}^{\left(\frac{C_3{\gamma }_{API}}{T+459.67}\right)}$$

(34)

where the coefficients C₁, C₂ and C₃ are given by

Coefficient	°API ≤ 30	°API ≥ 30
C1	27.64	56.060
C2	1.0937	1.187
C3	11.172	10.393

To make sure that the obtained liquid and gas hold-up is accurate, some popular correlations, used by the industry and are included in almost every commercial software package, were considered to predict the liquid and gas hold-up inside each well. The correlations considered in this study are the ones developed by Hagedorn and Brown [31], Duns and Ros [32], Orkiszewski [33], and Aziz et al. [34]. The statistical results for the various prediction methods when applied to all 25 well tests are shown in Figure 7 and

Table 3. Statistical results for the various prediction correlations.

Prediction Method	Average Error	Standard Deviation
	(%)	(%)
Duns and Ros	−1.06	13.06
Hagedorn and Brown	−0.86	11.57
Orkiszewski	1.8	16.52
Aziz et al.	2.9	16.66

. These results indicate that the Hagedorn and Brown correlation seems to predict liquid and gas hold-up better than the other correlations selected in this study. However, the overall results show minor differences between the different correlations. This is because each correlation was developed based on certain assumption and for a particular range of data.

Using the Hagedorn-Brown empirical correlation [31] to obtain liquid and gas hold-up (H_L and H_g)

$$N_{Lv}=1.938{\nu }_{sL}\frac{\sqrt[4]{\rho }}{\sigma }$$

(35)

$$N_{gv}=1.938{\nu }_{sg}\sqrt[4]{\frac{{\rho }_L}{\sigma }}$$

(36)

$$N_d=120.872D\frac{\sqrt{{\rho }_L}}{\sigma }$$

(37)

$$N_L=0.157{\mu }_L\sqrt[4]{\frac{1}{{\rho }_L{\sigma }^3}}$$

(38)

$$Y=-2.699+0.158X_1-0.551X_1{}^2+0.548X_1{}^3-0.122X_1{}^4$$

(39)

where

$$X_1=log\left(N_L+3\right)$$

(40)

$$C{N}_L={10}^Y$$

(41)

$$\frac{H_L}{\psi }=-0.103+0.618\left(log{X}_2+6\right)-0.633{\left(log{X}_2+6\right)}^2+0.296{\left(log{X}_2+6\right)}^3-0.04{\left(log{X}_2+6\right)}^4$$

(42)

where

$$X_2=\frac{N_{VL}{P}^{0.1}C{N}_L}{N_{Vg}{}^{0.575}P{a}^{0.1}{N}_D}$$

(43)

$$\psi =0.912-4.822X_3+1232.25X_3^2-22253.6X_3^3+116174.3X_3^4$$

(44)

where

$$X_3=\frac{N_{Vg}{N}_L{}^{0.38}}{N_D{}^{2.14}}$$

(45)

The liquid hold-up is

$$H_L=\psi \left(\frac{H_L}{\psi }\right)$$

(46)

and

$$H_g=\left(1-H_L\right)$$

(47)

The liquid flow rate is expressed as

$$q_L=\frac{\Delta H·A}{t}$$

(48)

the cross section of the conduit is given by

$$A=\frac{\pi r^2}{4}$$

(49)

$$\Delta H=H_2-H_1$$

(50)

as

$$\Delta P=\Delta H·{\rho }_L$$

(51)

then

$$\Delta H=\frac{\Delta P}{{\rho }_L}$$

(52)

and

$$\Delta P=WH{P}_a-WH{P}_b$$

(53)

then

$$q_L=\Delta P\frac{A}{{\rho }_L}t$$

(54)

The flow rates for gas, oil, and water are expressed as

$$q_o=q_L\left(1-WC\right)$$

(55)

$$q_g=q_{o}Rs$$

(56)

$$q_w=q_{o}WC$$

(57)

3. Results and Discussion

The experiments were run on 48 ESP oil wells from four different reservoirs. For each friction factor correlation, the measured oil flow rate values for each oil well were compared against the predicted flow rate values. It should be noted that as the points near the dotted straight line drawn at 45° (i.e., $y=x$) in the graph, the more accurate the prediction was. The results show that the pressure drop value was the significant parameter that had the main influence on the fluid flow rate computation. Indeed, any errors in pressure drop values would lead to high uncertainty errors of fluid flow rate prediction. For this reason, the properties of independent variables needed to be considered. Likewise, the interactions between each phase needed to be taken into account along with mixture properties and in situ volume fractions of oil and gas inside the conduit. Each multiphase flow correlation found the friction factor differently. Typically, each friction correlation made its own assumptions and modifications to make them useable to multiphase conditions. The prediction of frictional pressure drop in two-phase flow was usually complicated due to pressure and temperature variations along the flow path. When estimating the friction factor, there were a number of methods for calculating the Reynolds number depending on how much of the two-phase flow mixture was defined. Therefore, the oil and water were considered as a single liquid phase while the gas was considered as a separate phase.

By using the Blasius friction factor correlation, the differences between the predicted flow rate and the measured flow rate were very small. R-squared (R²) explained exactly how the data points were fitted close to the regression line ($y=x$). Figure 8, Figure 9 and Figure 10 displayed the regression model for oil, water, and gas flow rate measurements. It can be seen that the plots show that most data points lie on or close to the unit slope line (e.g., best fit line), indicating that the predicted and actual values were in excellent agreement and illustrated an accurate flow rate prediction for oil, water, and gas with good correlating coefficients of 0.994, 0.993, and 0.966, respectively. This means that 99.4%, 99.3%, and 96.6% of the variance in the oil, water, and gas data, respectively, was explained by the line and 0.6%, 0.7%, and 3.4% of the variance was due to unexplained effects. The figures show that the predicted wells flow rates fell within the accepted uncertainty when compared with the measured flow rates.

By using the Darcy friction factor correlation, the differences between the predicted fluid flow rates with the measured flow rates were larger than those of the Blasius correlation. Figure 11, Figure 12 and Figure 13 displayed the regression model for oil, water, and gas flow rate measurements. From these figures, one can easily recognize that the data plotted is under-estimated for oil and water flow rates and scattered around the best fit line for gas flow rates. This discrepancy was more evident for high flow rates where the correlation coefficients for oil, water, and gas flow rates accounted for 90.6%, 86.6%, and 78.7% of the variance, respectively. The figures show that the predicted well flow rates did not fall within the accepted uncertainty when compared with the measured flow rates.

By using the Colebrook friction factor correlation, the differences between the predicted fluid flow rates with the measured flow rates were slightly better than the Darcy correlation performance, but still less than the Blasius correlation performance. Figure 14, Figure 15 and Figure 16 displays the data fitting for oil, water, and gas flow rate measurements. Similar to the performance of the Darcy correlation, one can easily recognize that the data plotted is under-estimated for oil and water flow rates and scattered around the best fit line for gas flow rates. This discrepancy was more evident for high flow rates where the correlation coefficients for oil, water, and gas flow rates accounted for 93.0%, 87.1%, and 80.8% of the variance, respectively. The figures showed that the predicted wells flow rates did not fall within the accepted uncertainty when compared with the measured flow rates.

In general, the validation results of the predicted fluid flow rates were satisfactory when using the Blasius correlation rather than the Darcy or Colebrook correlations, where 96% and 98% of the predicted fluid flow rates were in good agreement with the real measured oil and water flow rate, respectively. Furthermore, the relative errors were less than ±18%, which were still within the reasonable uncertainty, as shown in Figure 17 and Figure 18. For the predicted and measured gas rates, 68% of the wells showed about ±10% relative errors, as shown in Figure 19. By using the Darcy correlation, 63% and 70% of the wells were not in good agreement with the predicted and measured oil and water rate, respectively, with more than ±18% for relative errors, as shown in Figure 20 and Figure 21. For predicted and measured gas rates, 79% of the wells showed more than ±10% relative errors, as shown in Figure 22. By using the Colebrook correlation, 67% and 75% of the wells were not in good agreement with the predicted and measured oil and water rate, respectively, with more than ±18% relative errors, as shown in Figure 23 and Figure 24. For predicted and measured gas rates, 77% of the wells showed more than ±10% relative errors, as shown in Figure 25.

The results showed high relative errors in gas rate prediction which can happen due to the oil separator meters being insufficiently accurate. Also, these errors may occur due to fixed orifice plate meters used to measure the gas flow rate despite the fact that orifice plates are not appropriate to measure low gas rates. Besides, wear and corrosion can increase the orifice size and cause excessive loss.

4. Summary and Conclusions

The prediction of the fluid flow rate of oil wells using the new mathematical model has been made and validated with experimentally measured fluid flow rate data. To evaluate the influence of the frictional pressure drop value on the measurement of fluid flow rate of oil wells, Blasius, Darcy, and Colebrook friction correlations were applied. Using the Blasius correlation, the analysis showed that the predicted fluid flow rate values were in accord with the measured values, while by using the Darcy and Colebrook friction correlations, the results were not in good agreement with the measured values. This discrepancy was due to the fact that each friction correlation found the friction factor differently. To determine the friction factor, many expressions were used to compute the Reynolds number. Essentially, each empirical correlation states its own assumptions and modifications to defend the variable components in order to be applicable to multiphase conditions. The two-phase flow significantly complicated the pressure drop calculations, where any errors in determining the frictional pressure drop values would generate some inaccuracies in predicting the fluid flow rate of the oil wells. Consequently, mixture properties and the interactions between the existing phase’s properties must be considered. Therefore, the gas and liquid volume fractions throughout the conduit needed to be determined. Overall, the performance of the new mathematical model indicated that the selection of the appropriate friction factor correlation would lead to predicting the gas and liquid flow rate within the acceptable accuracy. However, the friction loss dominated only with very high flow rates. For relatively small flow rates, the hydrostatic pressure played the key role in the overall pressure drop in the vertical tubing. Thus, different multiphase flow models, either empirical or mechanistic model, used in the computation would output different predictions. That being said, the Blasius equation may be superior to other models coupled with the Hagedorn-Brown empirical correlation, as it has been shown in this work. Indeed, a very reasonable average relative error of 4.6% was observed between the predicted and measured flow rates. However, it may not be as good as it is when coupled with other mechanistic models that may further reduce this error. Further research is needed to further validate the developed model by accounting for other sophisticated multiphase models.