Mixed Finite Element Simulation with Stability Analysis for Gas Transport in Low-Permeability Reservoirs
Abstract
:1. Introduction
2. Modeling and Formulation
3. Method of Solution
3.1. Preliminaries
3.2. Mixed Finite Element Approximation
3.3. Numerical Algorithm
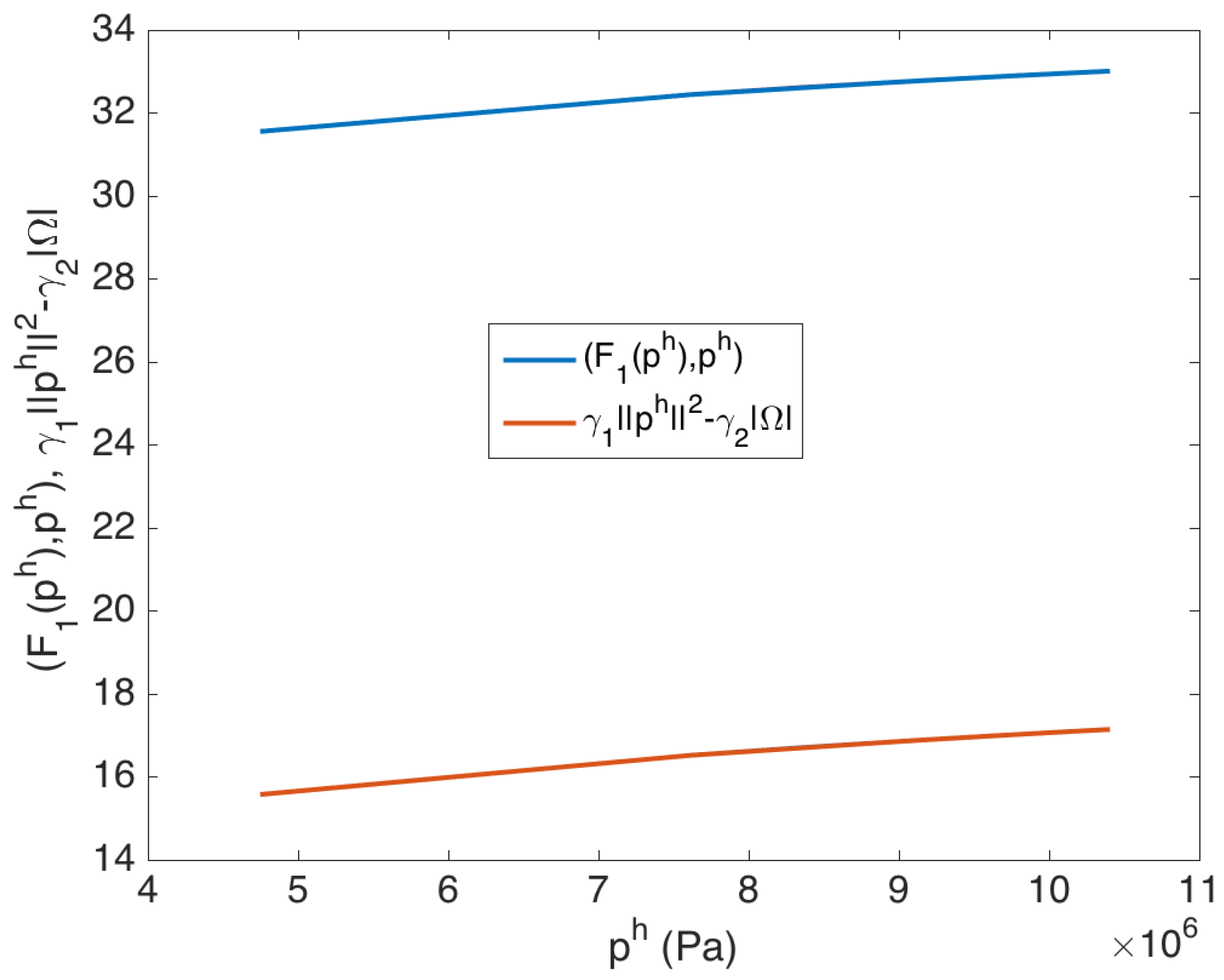
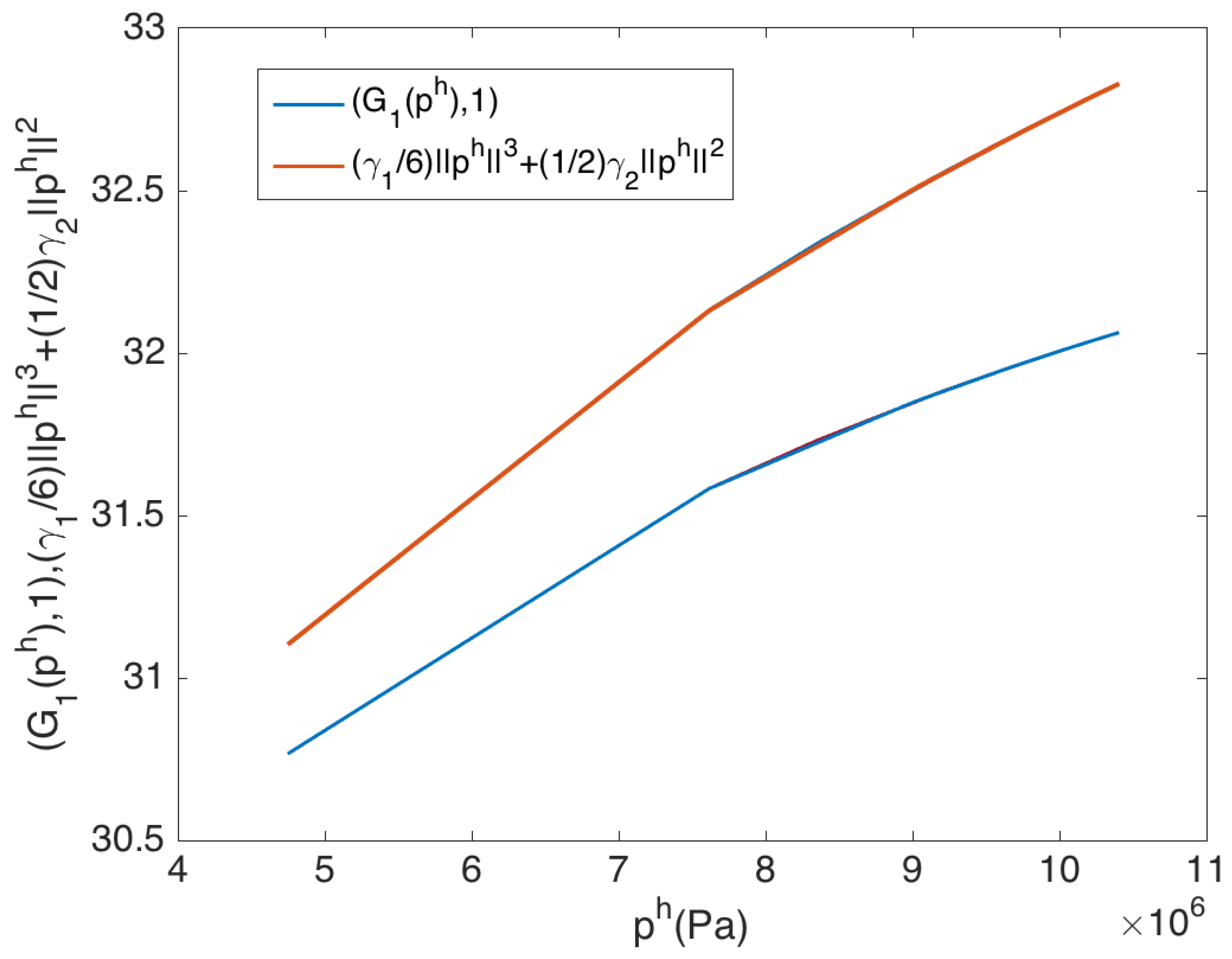
4. Stability Analysis
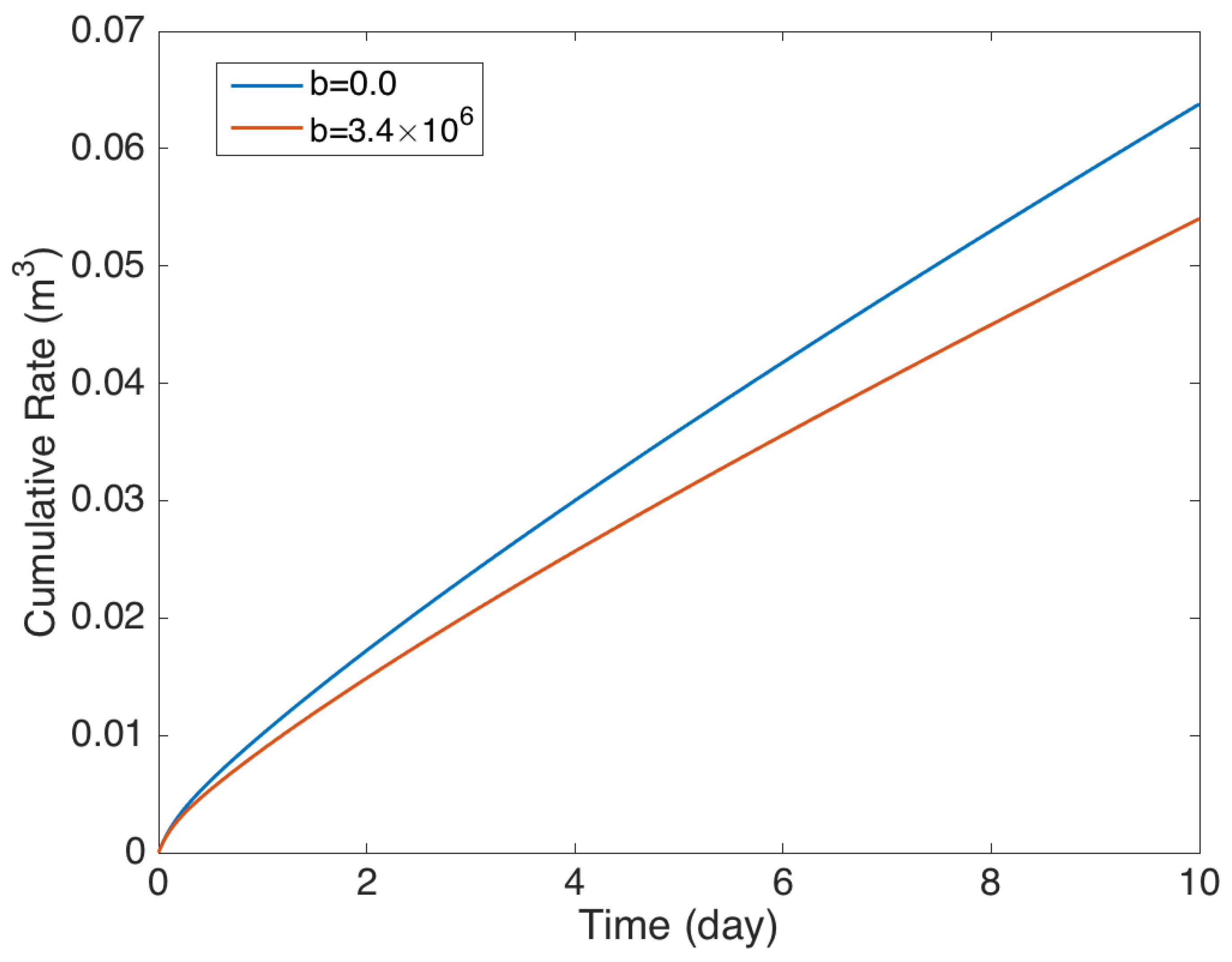
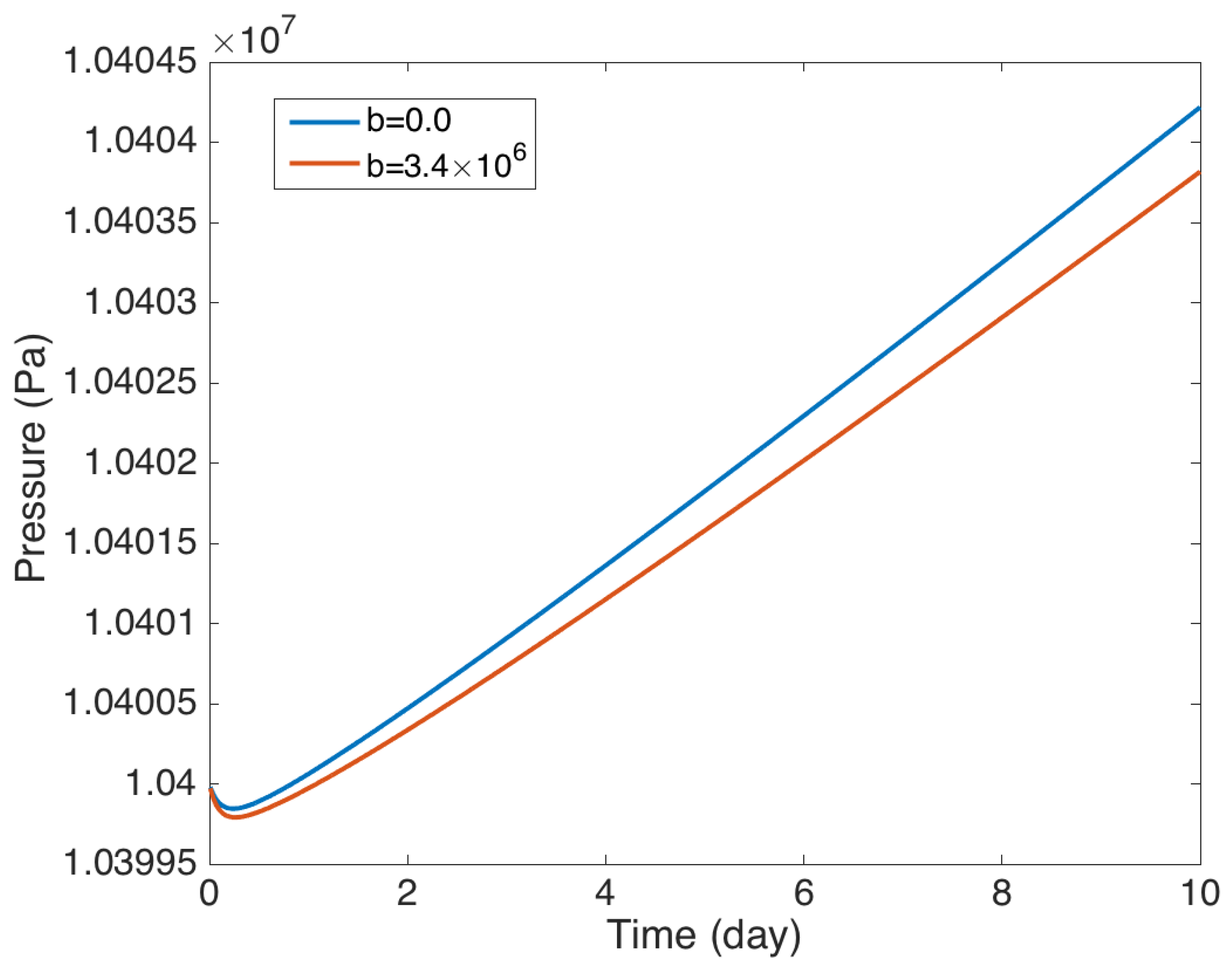
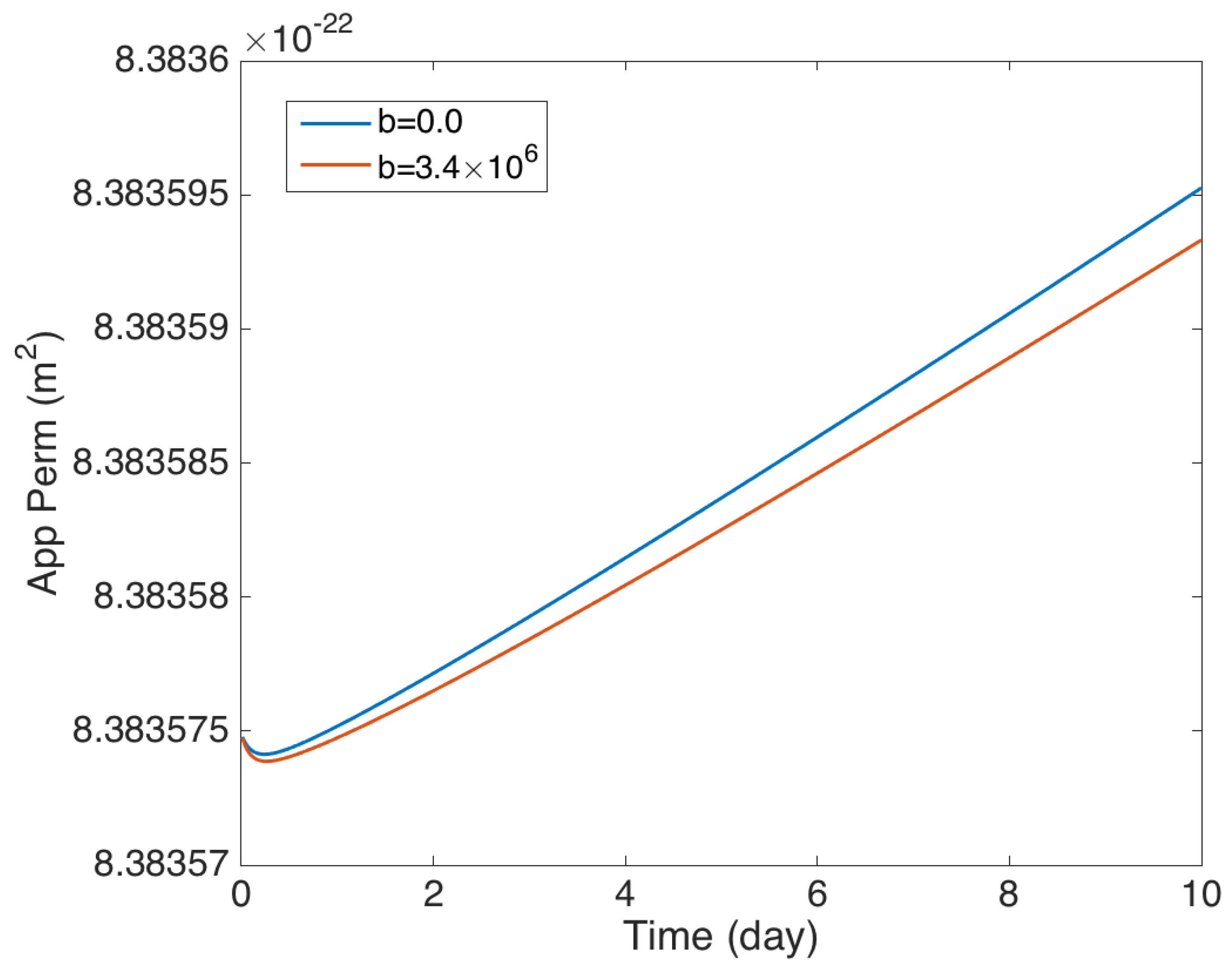
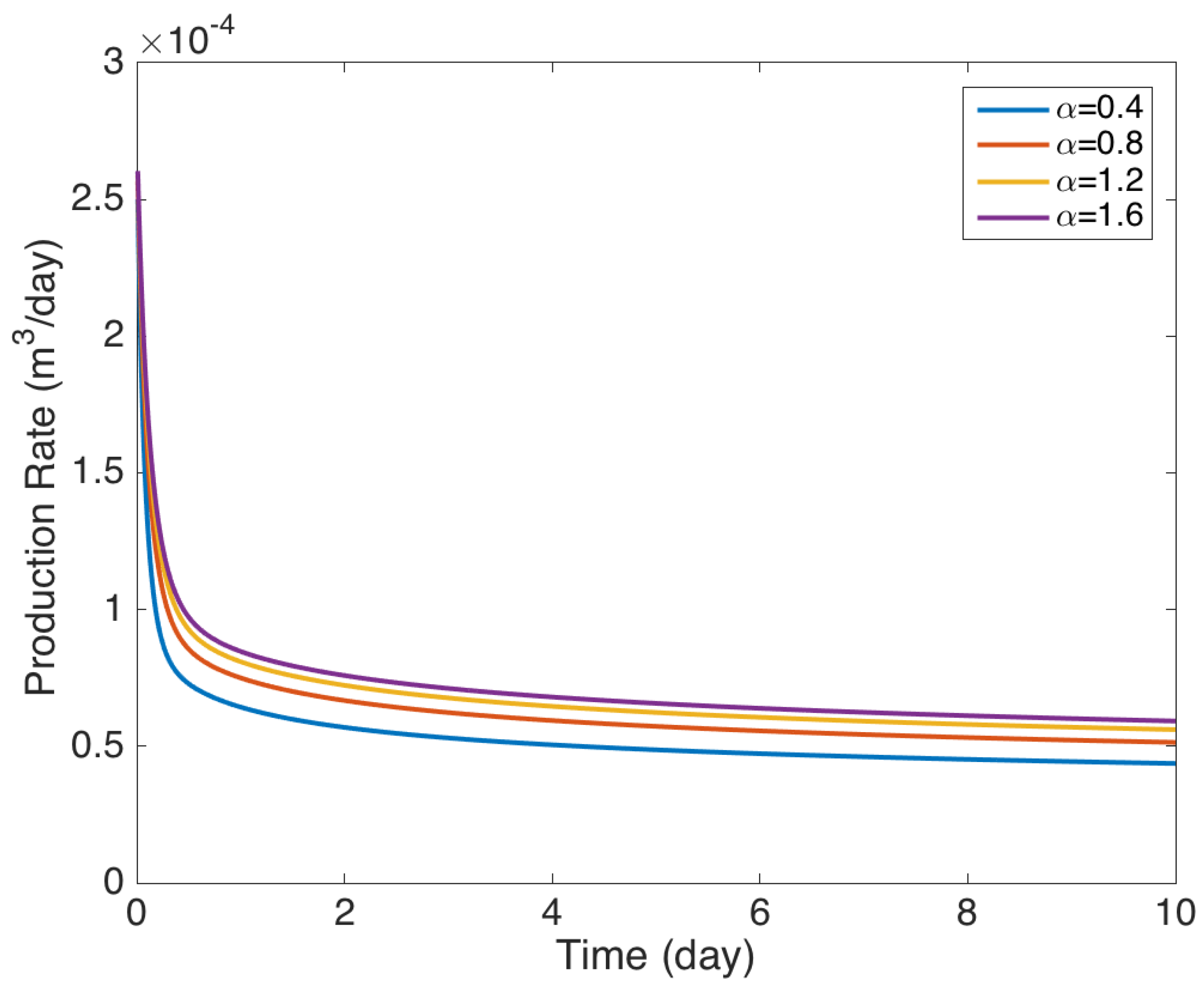
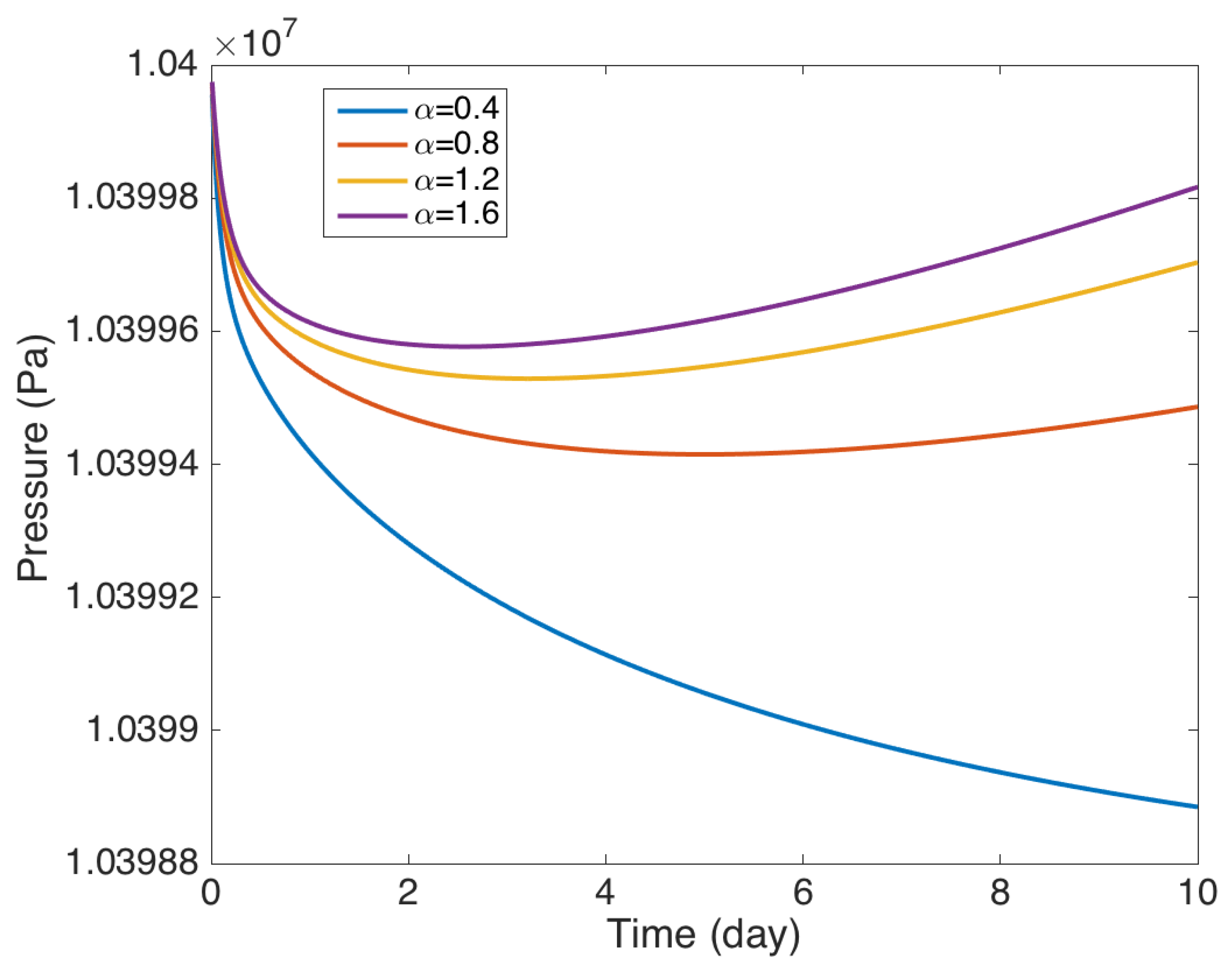
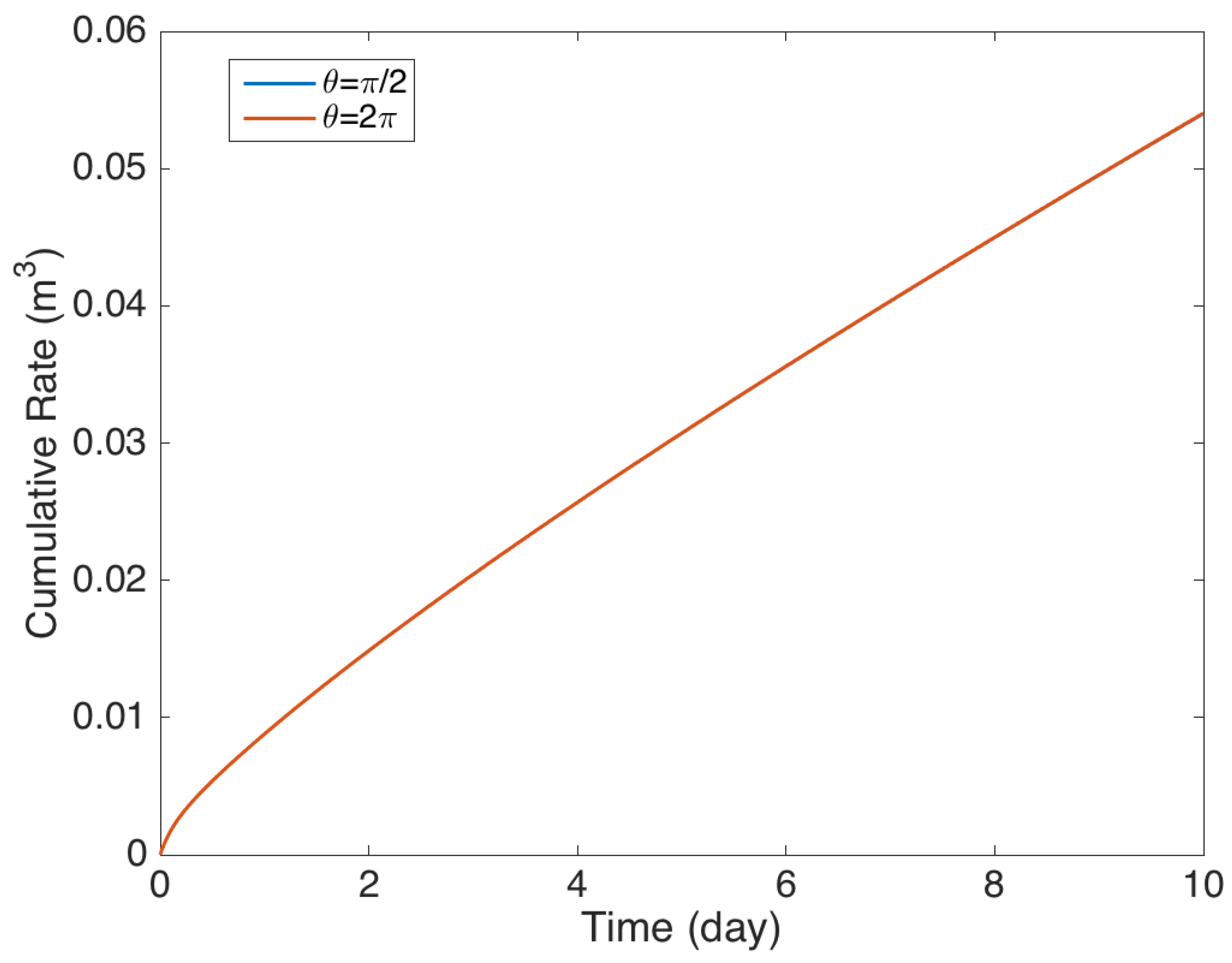
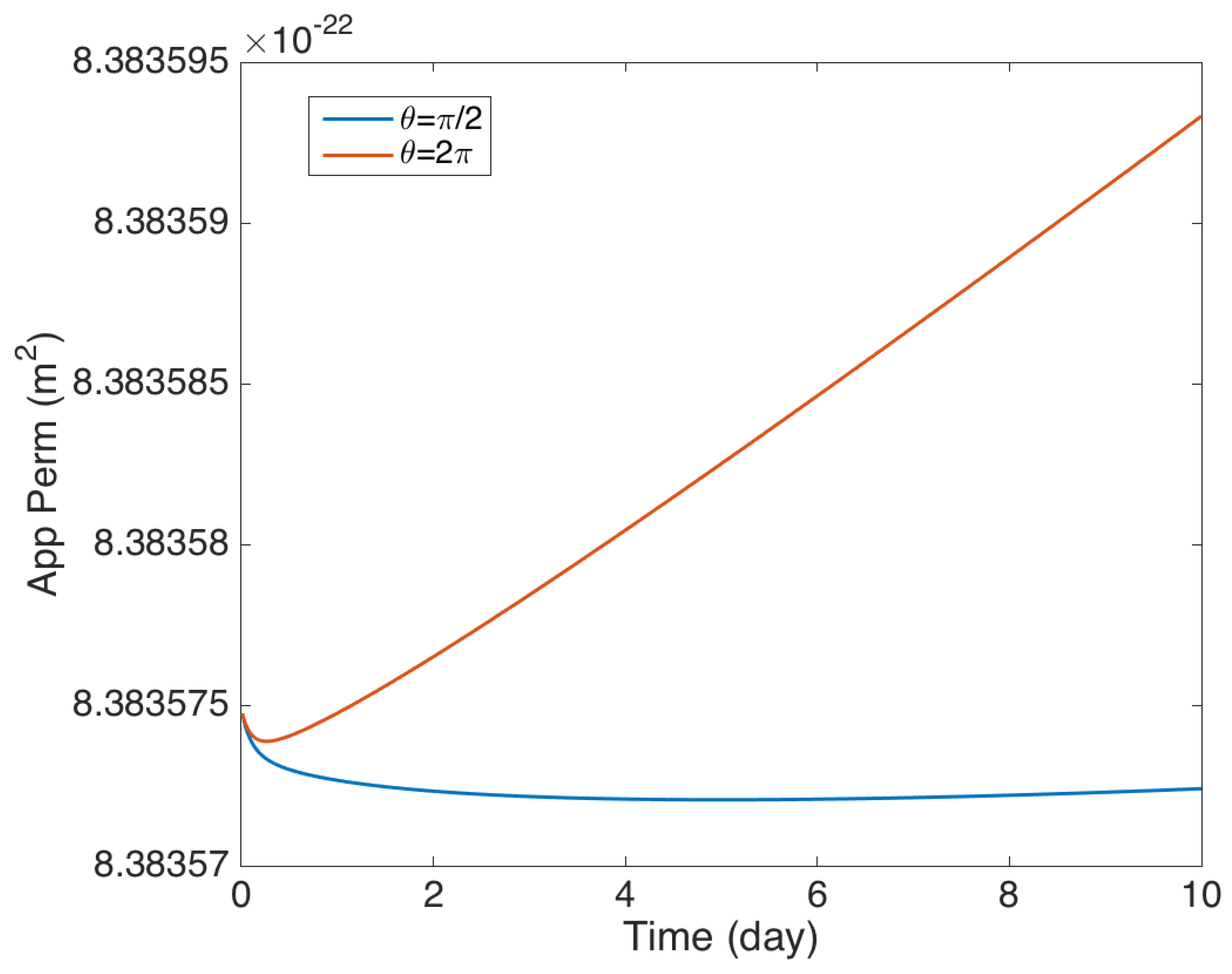
5. Numerical Tests
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Klinkenberg, L.J. The permeability of porous media to liquids and gases. In Drilling and Production Practice, American Petroleum Institute; American Petroleum Institute: Washington, DC, USA, 1941. [Google Scholar]
- McPhee, C.A.; Arthur, K.G. Klinkenberg permeability measurements: Problems and practical solutions. In Advances in Core Evaluation II: Reservoir Appraisal: Reviewed Proceedings; Edinburg Petroleum Services Ltd.: Edinburg, UK, 1991; pp. 371–392. [Google Scholar]
- Tanikawa, W.; Shimamoto, T. Comparison of Klinkenberg-corrected gas permeability and water permeability in sedimentary rocks. Int. J. Rock Mech. Min. Sci. 2009, 46, 229–238. [Google Scholar] [CrossRef]
- Wu, Y.; Pruess, K.; Persoff, P. Gas flow in porous media with Klinkenberg effects. Trans. Porous Media 1998, 32, 117–137. [Google Scholar] [CrossRef]
- Pazos, F.A.; Bhaya, A.; Compan, A.L.M. Calculation of Klinkenberg permeability, slip factor and turbulence factor of core plugs via nonlinear regression. J. Pet. Sci. Eng. 2009, 67, 159–167. [Google Scholar] [CrossRef]
- Esmaili, S.; Mohaghegh, S.D. Full field reservoir modeling of shale assets using advanced data-driven analytics. Geosci. Front. 2016, 7, 11–20. [Google Scholar] [CrossRef]
- Javadpour, F.; Fisher, D.; Unsworth, M. Nanoscale gas flow in shale gas sediments. J. Can. Pet. Technol. 2007, 46. [Google Scholar] [CrossRef]
- Javadpour, F. Nanopores and apparent permeability of gas flow in mudrocks (shales and siltstone). J. Can. Pet. Technol. 2009, 48, 16–21. [Google Scholar] [CrossRef]
- Cui, X.; Bustin, A.M.M.; Bustin, R.M. Measurements of gas permeability and diffusivity of tight reservoir rocks: different approaches and their applications. Geofluids 2009, 9, 208–223. [Google Scholar] [CrossRef]
- Shabro, V.; Torres-Verdin, C.; Javadpour, F. Numerical simulation of shale-gas production: From pore-scale modeling of slip-flow, Knudsen diffusion, and Langmuir desorption to reservoir modeling of compressible fluid. In Proceedings of the North American Unconventional Gas Conference and Exhibition, The Woodlands, TX, USA, 14–16 June 2011. [Google Scholar]
- Civan, F.; Rai, C.S.; Sondergeld, C.H. Shale-gas permeability and diffusivity inferred by improved formulation of relevant retention and transport mechanisms. Trans. Porous Media 2011, 86, 925–944. [Google Scholar] [CrossRef]
- Freeman, C.; Moridis, G.; Michael, G. Measurement, modeling, and diagnostics of flowing gas composition changes in shale gas well. In Proceedings of the Latin America and Caribbean Petroleum Engineering Conference, Mexico City, Mexico, 16–18 April 2012. [Google Scholar]
- Singh, H.; Cai, J. Screening improved recovery methods in tight-oil formations by injecting and producing through fractures. Int. J. Heat Mass Transf. 2018, 116, 977–993. [Google Scholar] [CrossRef]
- Ge, X.; Fan, Y.; Cao, Y.; Li, J.; Cai, J.; Liu, J.; Wei, S. Investigation of organic related pores in unconventional reservoir and its quantitative evaluation. Energy Fuels 2016, 30, 4699–4709. [Google Scholar] [CrossRef]
- Qi, Y.; Ju, Y.; Jia, T.; Zhu, H.; Cai, J. Nanoporous structure and gas occurrence of organic-rich shales. J. Nanosci. Nanotechnol. 2017, 17, 6942–6950. [Google Scholar] [CrossRef]
- Salama, A.; El-Amin, M.F.; Kumar, K.; Sun, S. Flow and transport in tight and shale formations. Geofluids 2017, 2017, 4251209. [Google Scholar] [CrossRef]
- El-Amin, M.F.; Amir, S.; Salama, A.; Urozayev, D.; Sun, S. Comparative study of shale-gas production using single- and dual-continuum approaches. J. Pet. Sci. Eng. 2017, 157, 894–905. [Google Scholar] [CrossRef]
- El-Amin, M.F. Analytical solution of the apparent-permeability gas-transport equation in porous media. Eur. Phys. J. 2017, 132, 129–135. [Google Scholar] [CrossRef]
- El-Amin, M.F.; Radwan, A.; Sun, S. Analytical solution for fractional derivative gas-flow equation in porous media. Res. Phys. 2017, 7, 2432–2438. [Google Scholar] [CrossRef]
- Raviart, P.A.; Thomas, J.M. A mixed finite element method for 2nd order elliptic problems, Mathematical aspects of finite element methods. Lect. Notes Math. 1977, 606, 292–315. [Google Scholar]
- Brezzi, F.; Douglas, J.; Marini, L.D. Two families of mixed finite elements for second order elliptic problems. Numer. Math. 1985, 47, 217–235. [Google Scholar] [CrossRef]
- Brezzi, F.; Fortin, V. Mixed and Hybrid Finite Element Methods; Springer: Berlin, Germany, 1991. [Google Scholar]
- Nakshatrala, K.B.; Turner, D.Z.; Hjelmstad, K.D.; Masud, A. A stabilized mixed finite element formulation for Darcy flow based on a multiscale decomposition of the solution. Comp. Meth. App. Mech. Eng. 2006, 195, 4036–4049. [Google Scholar] [CrossRef]
- El-Amin, M.F.; Kou, J.; Sun, S.; Salama, A. Adaptive time-splitting scheme for two-phase flow in heterogeneous porous media. Adv. Geo-Energy Res. 2017, 1, 182–189. [Google Scholar]
- Kou, J.; Sun, S. Unconditionally stable methods for simulating multi-component two-phase interface models with Peng-Robinson equation of state and various boundary conditions. J. Comput. Appl. Math. 2016, 291, 158–182. [Google Scholar] [CrossRef]
- Brown, G.P.; Dinardo, A.; Cheng, G.K.; Sherwood, T.K. The flow of gases in pipes at low pressures. J. Appl. Phys. 1946, 17, 802–813. [Google Scholar] [CrossRef]
- Roy, S.; Raju, R. Modeling gas flow through microchannels and nanopores. J. Appl. Phys. 2003, 93, 4870–4879. [Google Scholar] [CrossRef]





| Parameter | Value | Unit | Description |
|---|---|---|---|
| md | Permeability | ||
| 0.05 | – | Porosity | |
| R | 8.314 | m Pa mol K | Gas constant |
| 10.4 | MPa | Initial reservoir pressure | |
| 3.45 | MPa | Bottom hole pressure | |
| 0.016 | kg mol | Molecular weight of methane | |
| 0.0224 | m mol | Standard gas volume | |
| 2.07 | MPa | Langmuir pressure | |
| m kg | Langmuir volume | ||
| 2550 | kg m | Shale rock density | |
| Pa s | Initial gas viscosity | ||
| 0.1 | m | Wellbore radius | |
| 0.14 | – | Constant |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
El-Amin, M.F.; Kou, J.; Sun, S. Mixed Finite Element Simulation with Stability Analysis for Gas Transport in Low-Permeability Reservoirs. Energies 2018, 11, 208. https://doi.org/10.3390/en11010208
El-Amin MF, Kou J, Sun S. Mixed Finite Element Simulation with Stability Analysis for Gas Transport in Low-Permeability Reservoirs. Energies. 2018; 11(1):208. https://doi.org/10.3390/en11010208
Chicago/Turabian StyleEl-Amin, Mohamed F., Jisheng Kou, and Shuyu Sun. 2018. "Mixed Finite Element Simulation with Stability Analysis for Gas Transport in Low-Permeability Reservoirs" Energies 11, no. 1: 208. https://doi.org/10.3390/en11010208
APA StyleEl-Amin, M. F., Kou, J., & Sun, S. (2018). Mixed Finite Element Simulation with Stability Analysis for Gas Transport in Low-Permeability Reservoirs. Energies, 11(1), 208. https://doi.org/10.3390/en11010208







