Decentralized Framework for Optimal Price-Based Power System Operation Using Feedback Control Mechanism
Abstract
:1. Introduction
2. Optimal Power Flow
3. Structure of Decentralized Framework
3.1. Substructure for Marginal Price
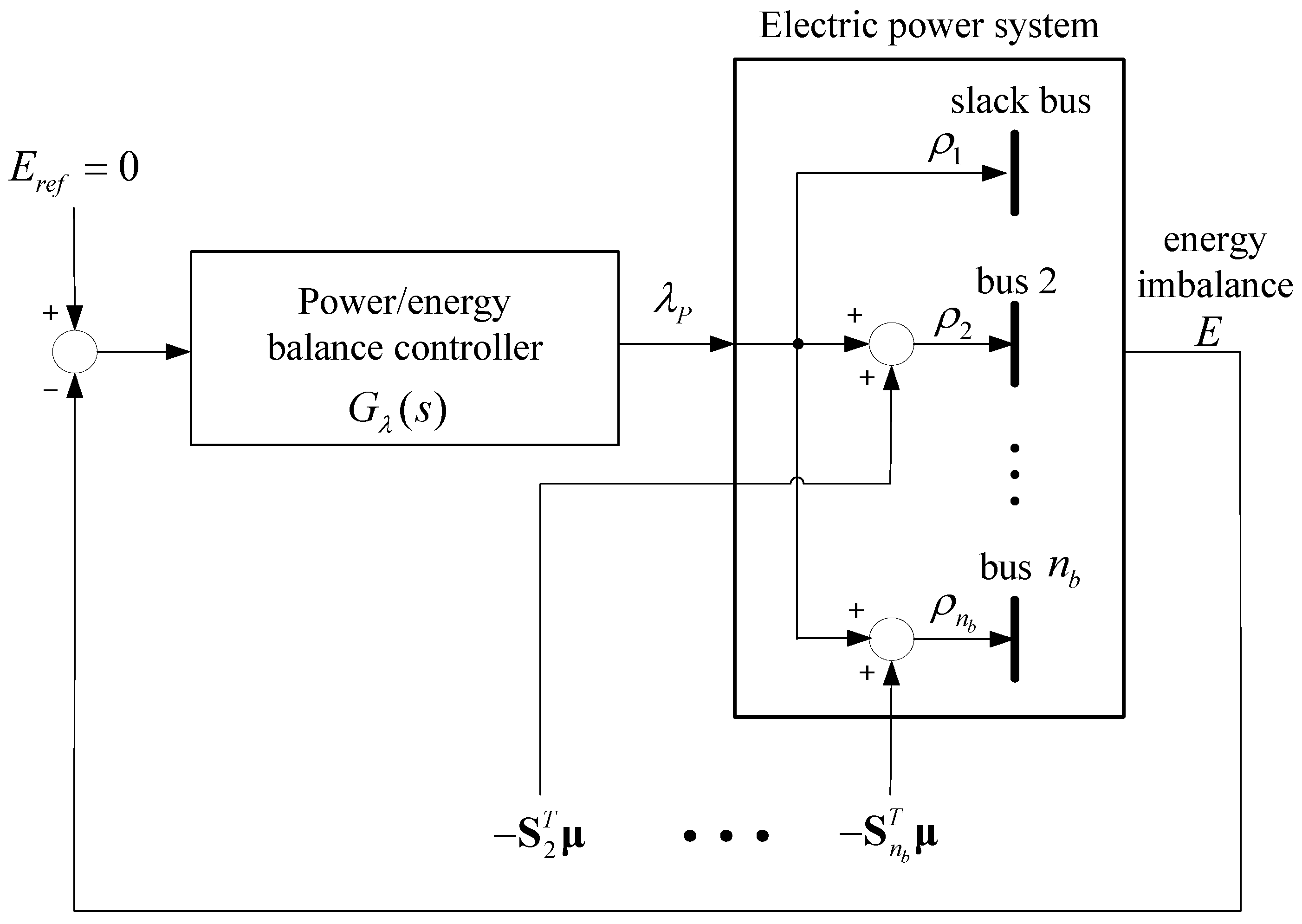
3.2. Substructure for Balancing Power and Energy
3.3. Substructure for Nodal Prices
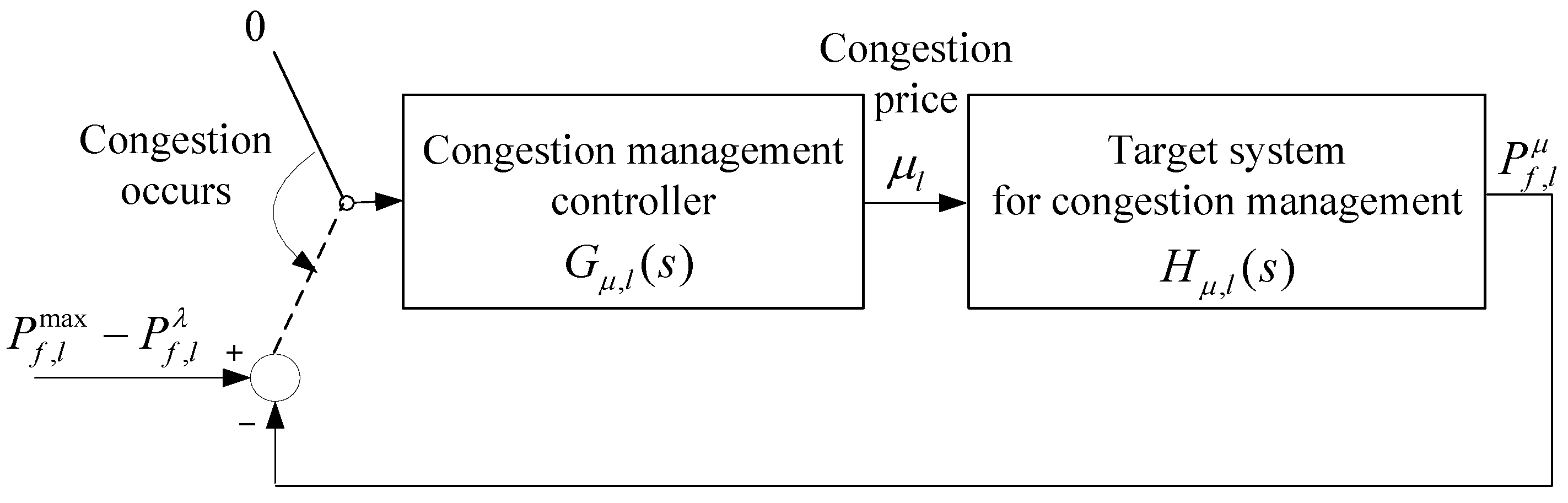
3.4. Substructure for Line Congestion Management
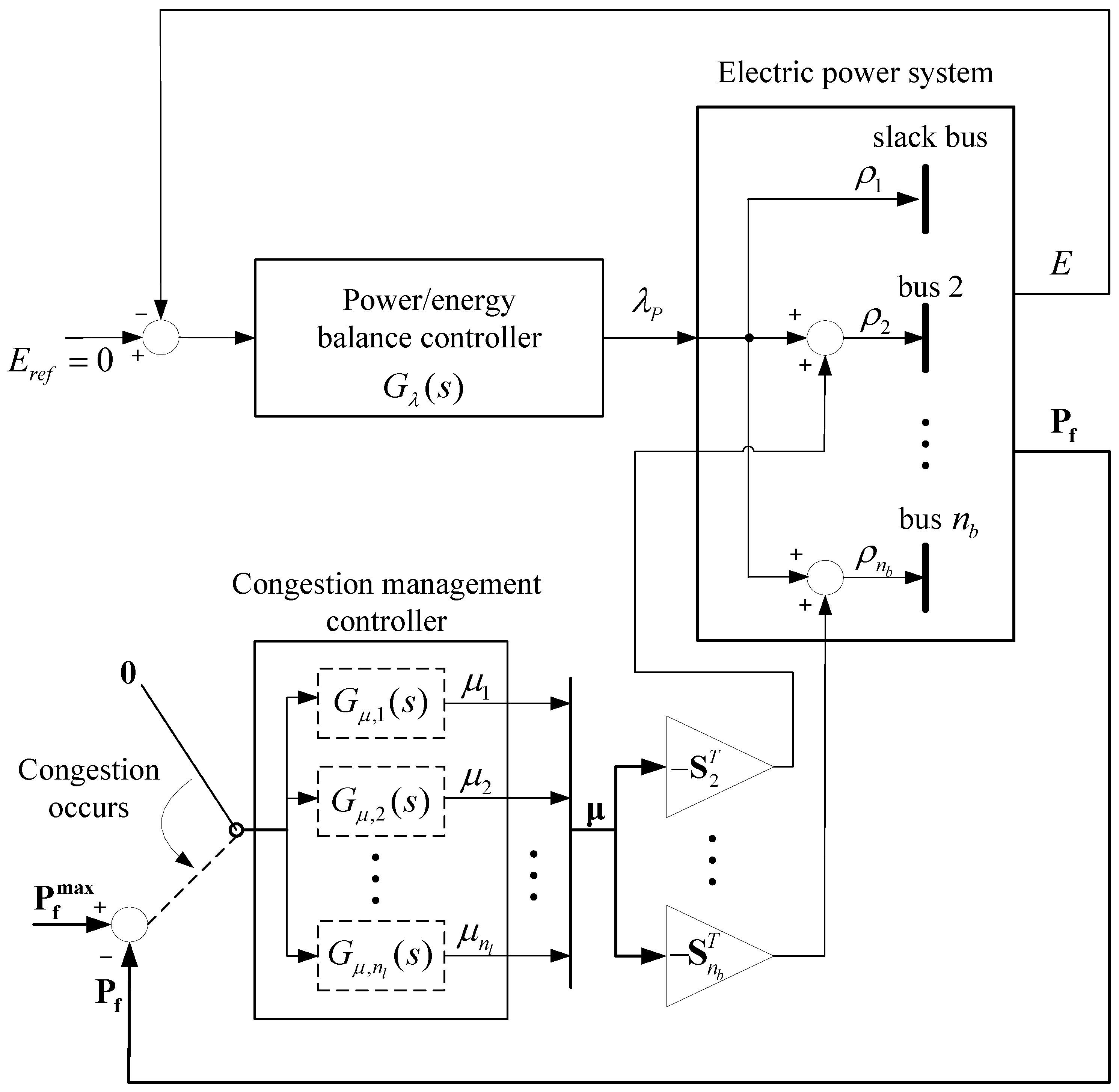
4. Overall Structure of the Framework
5. Simulations and Verification
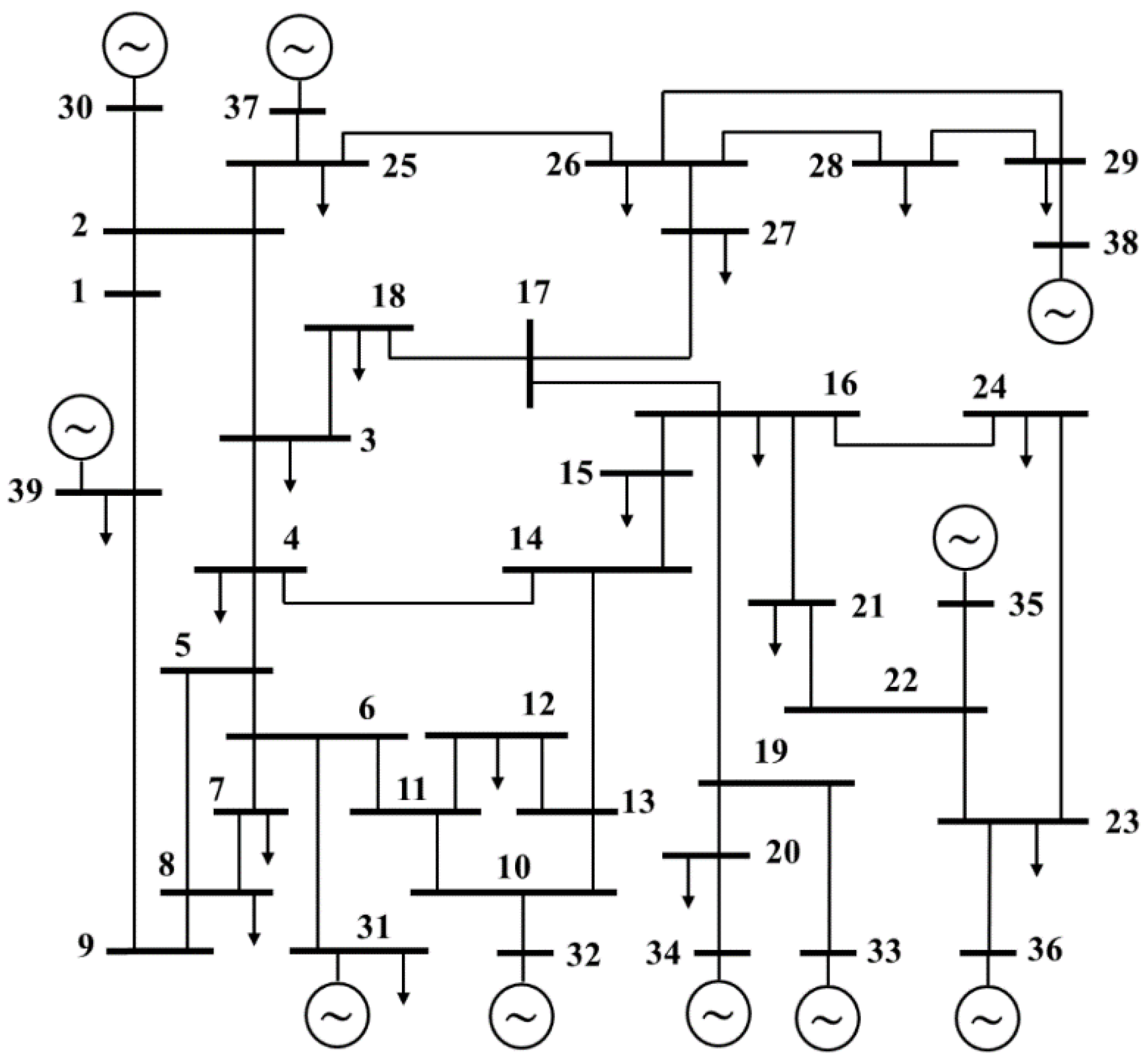
5.1. Simulation Settings
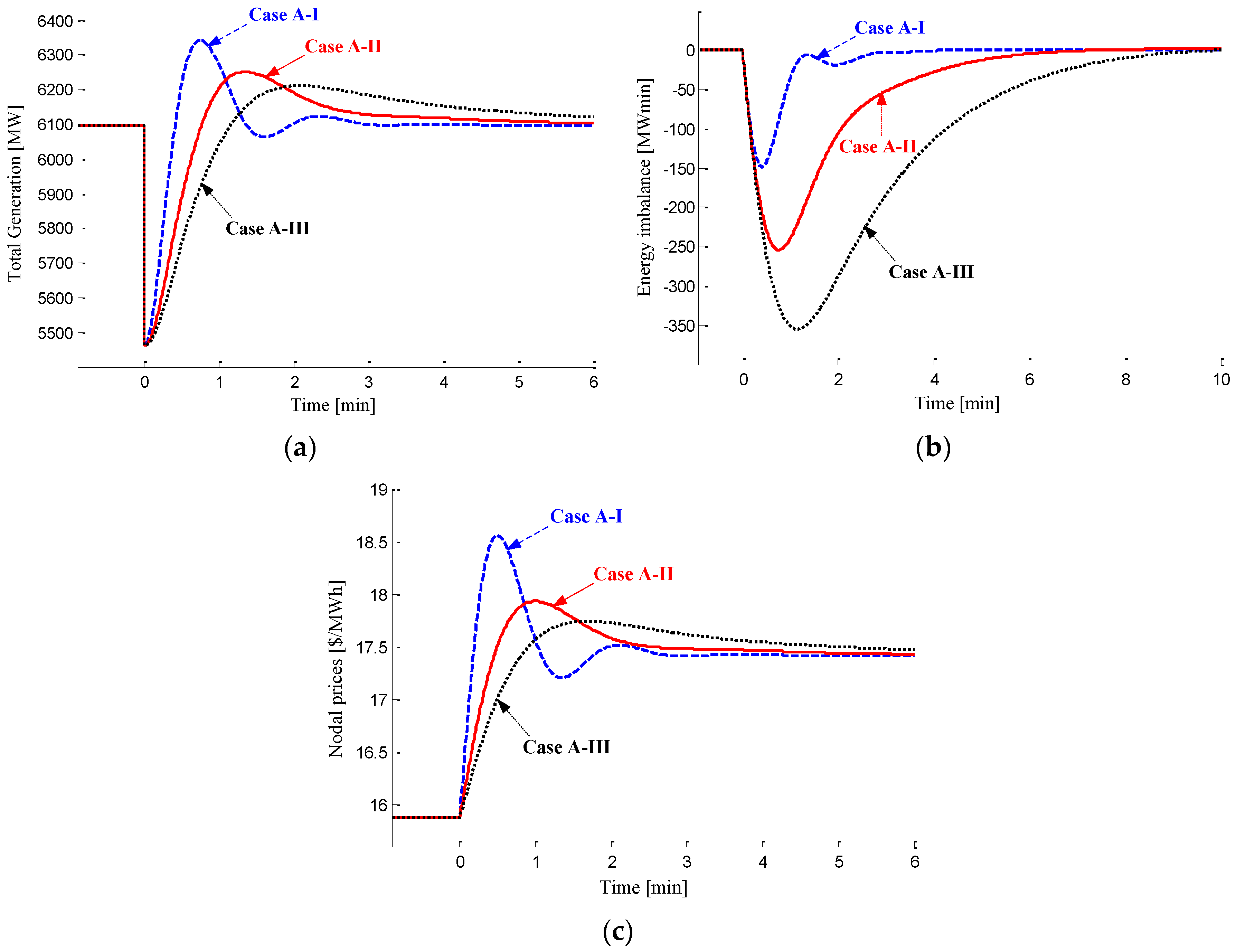
5.2. Simulation Results for a Generation Failure Scenario
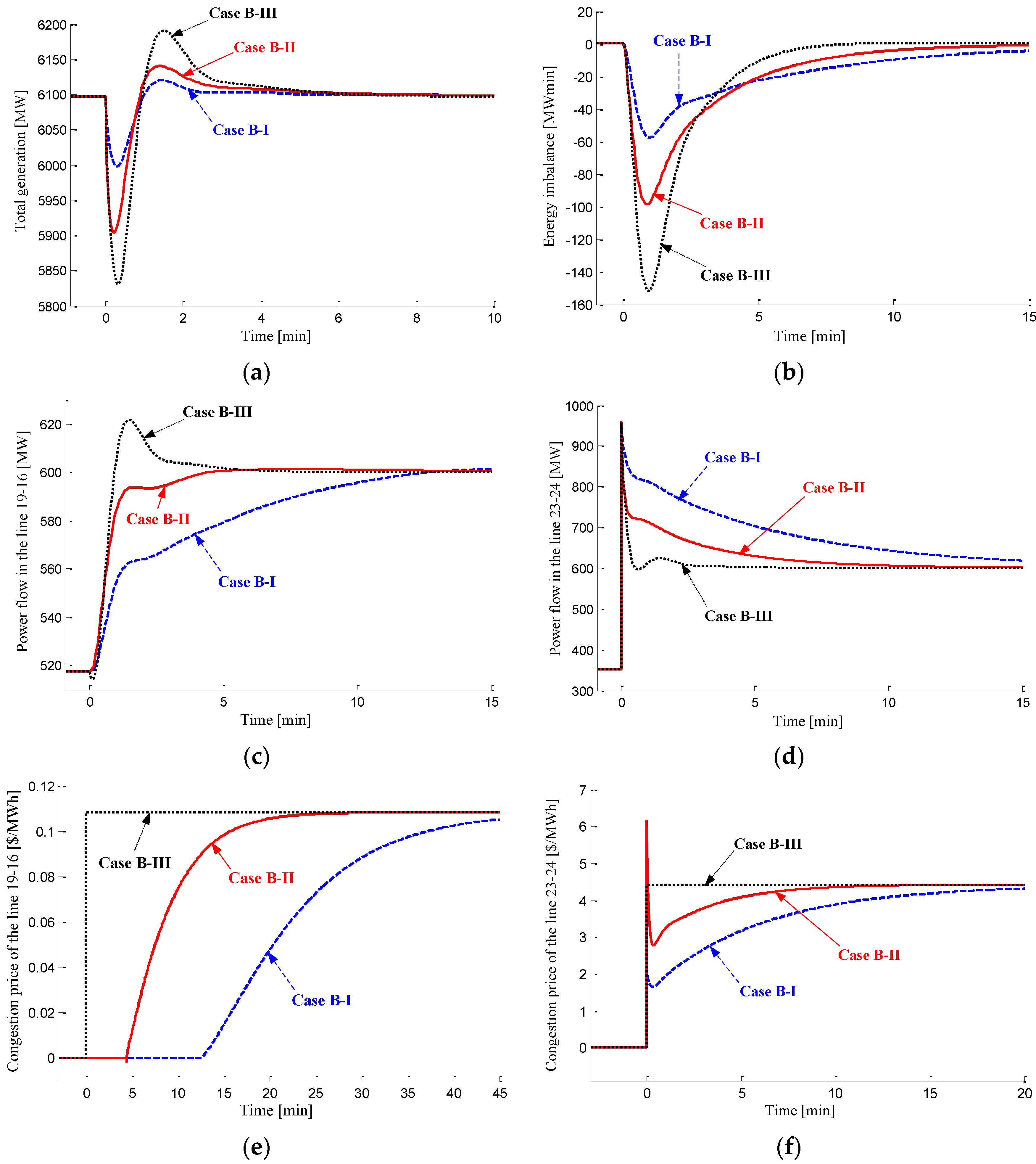
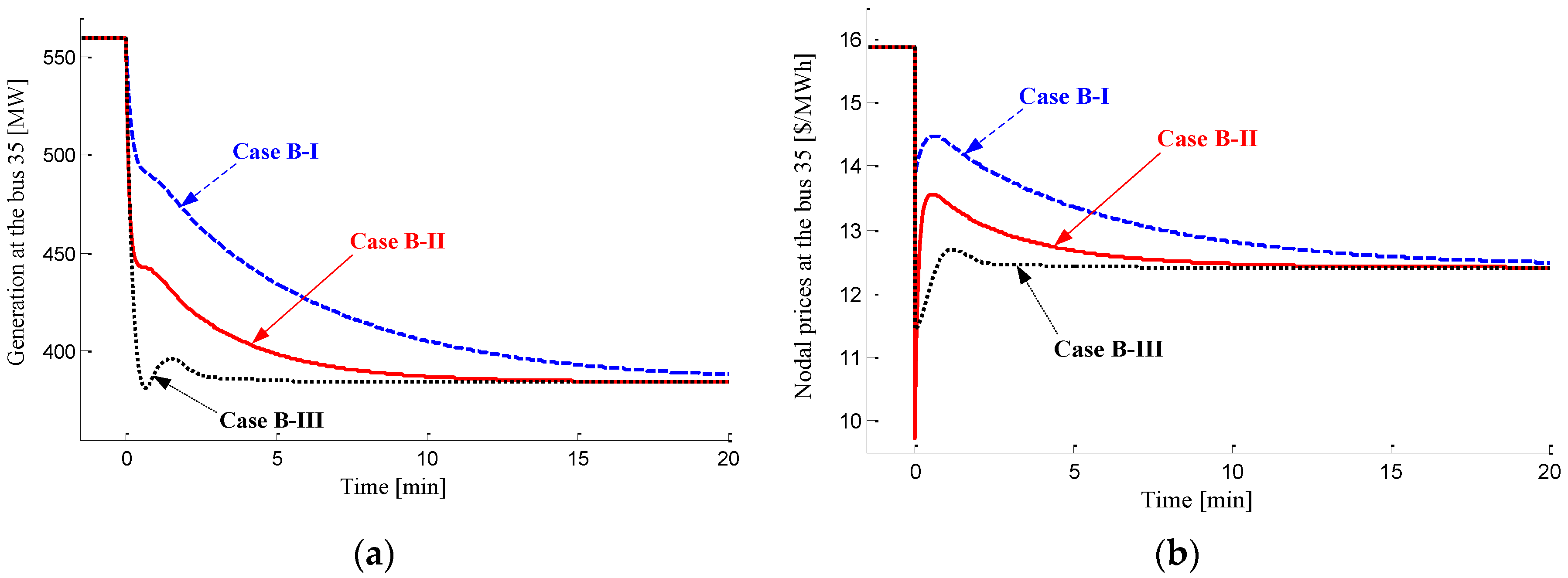
5.3. Simulation Results for a Line Trip Scenario
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Nomenclature
| total cost function | |
| cost function of generation at the bus k. | |
| utility or benefit function of consumption at the bus k | |
| number of the buses | |
| number of transmission lines | |
| amount of power generation | |
| amount of power consumption | |
| net power injection | |
| line susceptance matrix for DC power flow, matrix | |
| k-th column of line susceptance matrix B | |
| modified line susceptance matrix composed by eliminating the column of slack bus from B, matrix | |
| modified line susceptance matrix composed by eliminating the row and the column of slack bus B, matrix. | |
| relative phase angle to the slack bus assumed to be bus 1 | |
| active power flow | |
| active power flow limit | |
| active power flow from bus i to bus j | |
| inductive reactance in p.u. of transmission line connecting buses i and j | |
| line susceptance matrix | |
| branch-to-node incidence matrix | |
| Lagrange multiplier associated with equality constraint for power balance | |
| Lagrange multipliers associated with the equality constraint for power flow, vector. | |
| Lagrange multipliers associated with the equality constraint for power flow except for the slack bus, vector. | |
| , | Lagrange multipliers associated with the inequality constraints for power flow limit |
| matrix of power transfer distribution factors | |
| sensitivity of the power flow in the line l to power at the bus k | |
| set of nodal prices, vector | |
| set of nodal prices except for the slack bus, vector | |
| marginal cost of generation at the bus k | |
| marginal benefit of consumption at the bus k. | |
| , | time constants of dynamic equations for generation and consumption at the bus k |
| energy imbalance or accumulated power imbalance | |
| output disturbance added to energy imbalance | |
| target system for tuning in terms of power and energy balance | |
| target system for tuning in terms of congestion management of the line l | |
| controller for balancing power and energy | |
| controller for congestion management in the line l |
References
- Wood, A.; Wollenberg, B. Power Generation, Operation and Control, 2nd ed.; Wiley: New York, NY, USA, 1996. [Google Scholar]
- Global Wind Report−Annual Market Update 2015. Global Wind Energy Council. Available online: http://www.gwec.net/wp-content/uploads/vip/GWEC-Global-Wind-2015-Report_April-2016_22_04.pdf (accessed on 1 November 2015).
- Karimi, M.; Mokhlis, H.; Naidu, K.; Uddin, S.; Bakar, A.H.A. Photovoltaic penetration issues and impacts in distribution network—A review. Renew. Sustain. Energy Rev. 2016, 53, 594–605. [Google Scholar] [CrossRef]
- Walling, R.A.; Saint, R.; Dugan, R.C.; Burke, J.; Kojovic, L.A. Summary of distributed resources impact on power delivery systems. IEEE Trans. Power Deliv. 2008, 23, 1636–1644. [Google Scholar] [CrossRef]
- De Martini, P.; Kristov, L. Distribution Systems in a High Distributed Energy Resources Future: Planning, Market Design, Operation and Oversight; Lawrence Berkeley Laboratory: Berkeley, CA, USA, 2015. [Google Scholar]
- Kok, K.; Widergren, S. A society of devices. PES Mag. 2016, 14, 34–45. [Google Scholar]
- Schweppe, F.C.; Tabors, R.D.; Kirtley, H.R., Jr.; Outhred, H.R.; Pickel, F.H.; Cox, A.J. Homeostatic utility control. IEEE Trans. Power Appar. Syst. 1980, PAS-99, 1151–1163. [Google Scholar] [CrossRef]
- Schweppe, F.C.; Caramanis, M.C.; Tabors, R.D.; Bohn, R.D. Spot Pricing of Electricity; Kluwer Academic Publishers: Hingham, MA, USA, 1988. [Google Scholar]
- Alvarado, F.L. The Dynamics of Power System Markets; University of Wisconsin-Madison: Madison, WI, USA, 1997. [Google Scholar]
- Alvarado, F.L. The stability of power system markets. IEEE Trans. Power Syst. 1999, 14, 505–511. [Google Scholar] [CrossRef]
- Jokic, A.; Wittebol, E.H.M.; Van den Bosch, P.P.J. Dynamic market behavior of autonomous network-based power systems. Eur. Trans. Electr. Power 2006, 16, 533–544. [Google Scholar] [CrossRef]
- Christie, R.D.; Wollenberg, B.F.; Wangensteen, I. Transmission management in the deregulated environment. Proc. IEEE 2000, 88, 170–195. [Google Scholar] [CrossRef]
- Glatvitsch, H.; Alvarado, F. Management of multiple congested conditions in unbundled operation of a power system. IEEE Trans. Power Syst. 1998, 13, 1013–1019. [Google Scholar] [CrossRef]
- Alvarado, F.L. Controlling power systems with price signals. Decis. Support Syst. 2005, 40, 495–504. [Google Scholar] [CrossRef]
- Jokic, A.; Lazar, M.; Van den Bosch, P.P.J. Real-time control of power systems using nodal prices. Int. J. Electr. Power Energy Syst. 2009, 31, 522–530. [Google Scholar] [CrossRef]
- Nyeng, P.; Ostergaard, J. Information and communications systems for control-by-price of distributed energy resources and flexible demand. IEEE Trans. Smart Grid 2011, 2, 334–341. [Google Scholar] [CrossRef]
- Corradi, O.; Ochsenfeld, H.; Madsen, H.; Pinson, P. Controlling electricity consumption by forecasting its response to varying prices. IEEE Trans. Power Syst. 2013, 28, 421–429. [Google Scholar] [CrossRef]
- Jin, Y.G.; Lee, S.Y.; Kim, S.W.; Yoon, Y.T. Designing rule for price-based operation with reliability enhancement by reducing the frequency deviation. IEEE Trans. Power Syst. 2013, 28, 4365–4372. [Google Scholar] [CrossRef]
- Moradi, H.; Abtahi, A.; Esfahanian, M. Optimal operation of a multi-source microgrid to achieve cost and emission targets. In Proceedings of the IEEE Power and Energy Conference, Champaign, IL, USA, 19–20 February 2016; pp. 1–6.
- Boyd, S.; Vandenberghe, L. Convex Optimization; Cambridge University Press: Cambridge, NY, USA, 2004. [Google Scholar]
- Bergen, A.; Vittal, V. Power System Analysis, 2nd ed.; Prentice-Hall: Upper Saddle River, NJ, USA, 2000. [Google Scholar]
- Baldick, R. Electricity market equilibrium models: The effect of parametrization. IEEE Trans. Power Syst. 2002, 17, 1170–1176. [Google Scholar] [CrossRef]
- Gomez-Exposito, A.; Conejo, A.J.; Canizares, C. Electric Energy Systems: Analysis and Operation; CRC Press: Boca Raton, FL, USA, 2008. [Google Scholar]
- Pai, M.A. Energy Function Analysis for Power System Stability; Kluwer Academic Publishers: Hingham, MA, USA, 1989. [Google Scholar]
- Nguyen, T.B.; Pai, M.A. Dynamic security-constrained rescheduling of power systems using trajectory sensitivities. IEEE Trans. Power Syst. 2003, 18, 848–854. [Google Scholar] [CrossRef]
- Zimmerman, R.D.; Murillo-Sánchez, C.E.; Thomas, R.J. Matpower: Steady-state operations, planning and analysis tools for power systems research and education. IEEE Trans. Power Syst. 2011, 26, 12–19. [Google Scholar] [CrossRef]
- Zhong, Z. Power Systems Frequency Dynamic Monitoring System Design and Applications. Ph.D. Thesis, Virginia Polytechnic Institute and State University, Blacksburg, VA, USA, July 2005. [Google Scholar]
- Cai, H.R.; Chung, C.Y.; Wong, K.P. Application of differential evolution algorithm for transient stability constrained optimal power flow. IEEE Trans. Power Syst. 2008, 23, 719–728. [Google Scholar] [CrossRef]
- Skogestad, S. Simple analytic rules for model reduction and PID controller tuning. J. Process Control 2003, 13, 291–309. [Google Scholar] [CrossRef]

| Bus | Maximum Generation (MW) | (min) | ($/MW2h) | ($/MWh) |
|---|---|---|---|---|
| 30 | 350 | 0.6 | 0.0386 | 6.9 |
| 31 | 650 | 1.1 | 0.0222 | 3.7 |
| 32 | 800 | 1.2 | 0.0208 | 2.8 |
| 33 | 750 | 1.4 | 0.0176 | 4.7 |
| 34 | 650 | 1.0 | 0.0256 | 2.8 |
| 35 | 750 | 1.3 | 0.0188 | 3.7 |
| 36 | 750 | 1.3 | 0.0198 | 4.8 |
| 37 | 700 | 1.1 | 0.0226 | 3.6 |
| 38 | 900 | 1.8 | 0.0142 | 3.7 |
| 39 | 1200 | 2.0 | 0.0128 | 3.9 |
| Bus | Generation (MW) |
|---|---|
| 30 | 232.45 |
| 31 | 548.32 |
| 32 | 628.50 |
| 33 | 634.81 |
| 34 | 510.65 |
| 35 | 647.49 |
| 36 | 559.23 |
| 37 | 543.04 |
| 38 | 857.23 |
| 39 | 935.37 |
| Case Name | (min) | Power/Energy Balance Controller |
|---|---|---|
| Case A-I | 0.2 (fast) | |
| Case A-II | 0.4 (normal) | |
| Case A-III | 0.6 (slow) |
| Case Name | Power/Energy Balance Controller | Congestion Management Controller |
|---|---|---|
| Case B-I | ||
| Case B-II | ||
| Case B-III |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jin, Y.G.; Kim, S.W.; Lee, S.Y.; Yoon, Y.T. Decentralized Framework for Optimal Price-Based Power System Operation Using Feedback Control Mechanism. Energies 2017, 10, 291. https://doi.org/10.3390/en10030291
Jin YG, Kim SW, Lee SY, Yoon YT. Decentralized Framework for Optimal Price-Based Power System Operation Using Feedback Control Mechanism. Energies. 2017; 10(3):291. https://doi.org/10.3390/en10030291
Chicago/Turabian StyleJin, Young Gyu, Seung Wan Kim, Si Young Lee, and Yong Tae Yoon. 2017. "Decentralized Framework for Optimal Price-Based Power System Operation Using Feedback Control Mechanism" Energies 10, no. 3: 291. https://doi.org/10.3390/en10030291
APA StyleJin, Y. G., Kim, S. W., Lee, S. Y., & Yoon, Y. T. (2017). Decentralized Framework for Optimal Price-Based Power System Operation Using Feedback Control Mechanism. Energies, 10(3), 291. https://doi.org/10.3390/en10030291






