Aftereffects of Saccades Explored in a Dynamic Neural Field Model of the Superior Colliculus
Abstract
Introduction
Saccades and the Superior Colliculus
Computational Explorations of Saccade Initiation
Why Are Return Saccades Slower to Initiate than Forward Saccades
“Because oculomotor responses are likely initiated by a winner-take-all algorithm mediated by lateral inhibition (and implemented in the superior colliculus), any asymmetric preparation would result in inhibition of the least prepared saccades.”.(p. 351)
Model Architecture
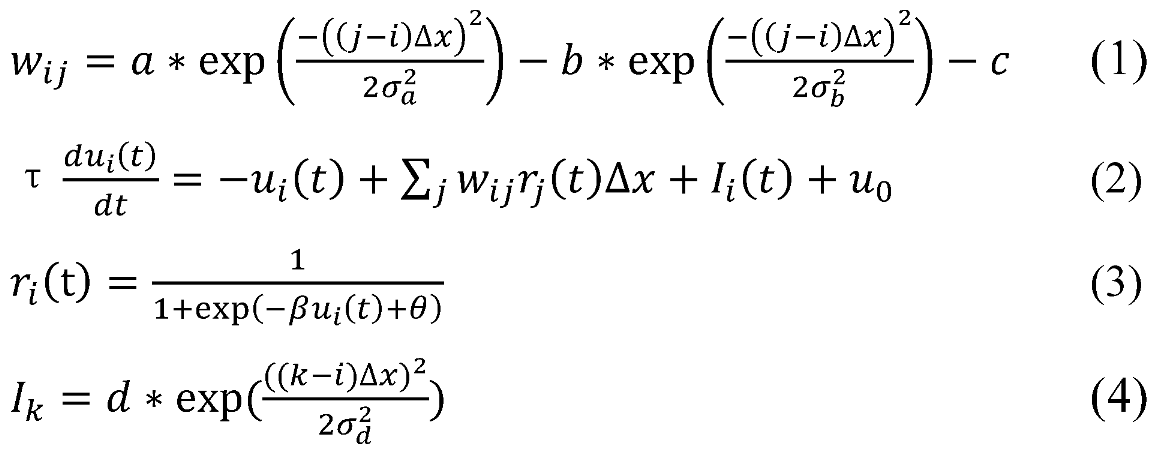
Simulations
Residual Activity after Saccades
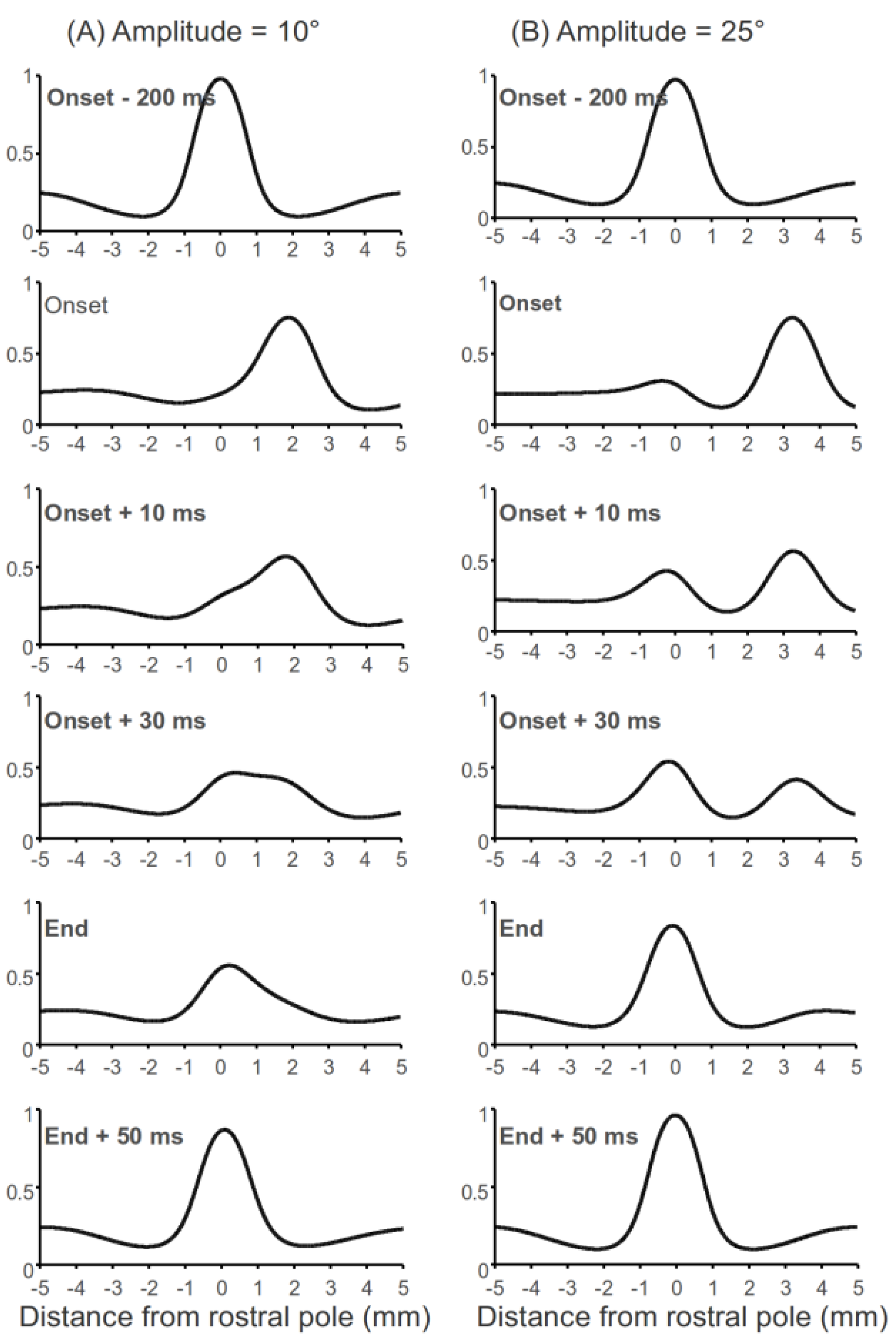
Simulation of Behavioral Findings
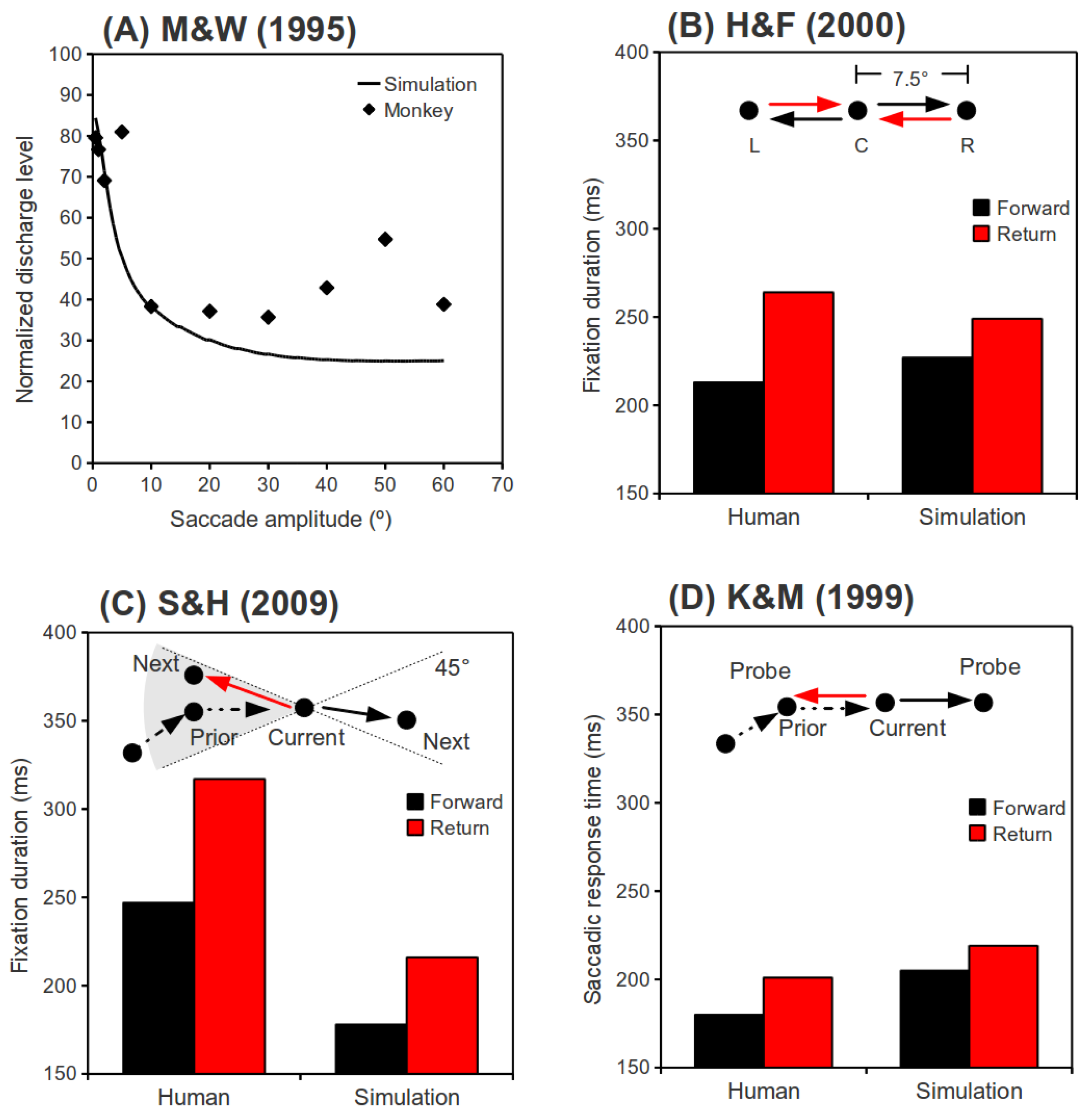
Spatio-temporal Characteristics
General Discussion
Why Are Forward Saccades Initiated More Rapidly?
Another IOR mechanism?
IOR in Saccade-Saccade Paradigms
IOR in Visual Search
Limitations of the Present Study
Implications for Future Studies
Acknowledgments
Appendix: Moving Hill or Jumping Hill?
References
- Amari, S. 1977. Dynamics of pattern formation in lateral-inhibition type neural fields. Biological Cybernetics 27, 2: 77–87. [Google Scholar] [CrossRef] [PubMed]
- Anderson, A., H. Yadav, and R. Carpenter. 2008. Directional prediction by the saccadic system. Current Biology 18, 8: 614–618. [Google Scholar] [CrossRef] [PubMed]
- Anderson, R. W., E. L. Keller, N. J. Gandhi, and S. Das. 1998. Two-dimensional saccade-related population activity in superior colliculus in monkey. Journal of Neurophysioogy 80, 2: 798–817. [Google Scholar] [CrossRef]
- Arai, K., E. L. Keller, and J. A. Edelman. 1994. Two-dimensional neural network model of the primate saccadic system. Neural Networks 7, 6–7: 1115–1135. [Google Scholar] [CrossRef]
- Bergeron, A., S. Matsuo, and D. Guitton. 2003. Superior colliculus encodes distance to target, not saccade amplitude, in multi-step gaze shifts. Nature Neuroscience 6, 4: 404. [Google Scholar] [CrossRef]
- Carpenter, R. H. 1988. Movements of the Eyes, 2nd ed. London: Pion Ltd. [Google Scholar]
- Choi, W. Y., and D. Guitton. 2009. Firing patterns in superior colliculus of head-unrestrained monkey during normal and perturbed gaze saccades reveal short-latency feedback and a sluggish rostral shift in activity. Journal of Neurophysioogyl 29, 22: 7166–7180. [Google Scholar] [CrossRef]
- Chu, L., and C. R. S. Kaneko. 1995. Do the saccadic system and the vestibulo-ocular reflex of monkeys share a common neural integrator? Neuroscience Letters 187, 3: 193–196. [Google Scholar] [CrossRef]
- Das, S., E. L. Keller, and K. Arai. 1996. A distributed model of the saccadic system: The effects of internal noise. Neurocomputing 11, 2–4: 245–269. [Google Scholar] [CrossRef]
- Dodd, M. D., S. Van der Stigchel, and A. Hollingworth. 2009. Novelty is not always the best policy: Inhibition of return and facilitation of return as a function of visual task. Psychological Science 20, 3: 333–339. [Google Scholar] [CrossRef]
- Dorris, M. C., R. M. Klein, S. Everling, and D. P. Munoz. 2002. Contribution of the primate superior colliculus to inhibition of return. Journal of Cognitive Neuroscience 14, 8: 1256–1263. [Google Scholar] [CrossRef]
- Dorris, M. C., M. Pare, and D. P. Munoz. 1997. Neuronal activity in monkey superior colliculus related to the initiation of saccadic eye movements. The Journal of Neuroscience 17, 21: 8566–8579. [Google Scholar] [CrossRef] [PubMed]
- Dorris, M. C., T. L. Taylor, R. M. Klein, and D. P. Munoz. 1999. Influence of previous visual stimulus or saccade on saccadic reaction times in monkey. Journal of Neurophysiology 81, 5: 2429–2436. [Google Scholar] [CrossRef][Green Version]
- Dukewich, K., and S. Boehnke. 2008. Cue repetition increases inhibition of return. Neuroscience Letters 448, 3: 231–235. [Google Scholar] [CrossRef] [PubMed]
- Dukewich, K. R. 2009. Reconceptualizing inhibition of return as habituation of the orienting response. Psychonomic Bulletin & Review 16, 2: 238–251. [Google Scholar]
- Fecteau, J. H., and D. P. Munoz. 2005. Correlates of capture of attention and inhibition of return across stages of visual processing. Journal of Cognitive Neuroscience 17, 11: 1714–1727. [Google Scholar] [CrossRef]
- Findlay, J. M., and R. Walker. 1999. A model of saccade generation based on parallel processing and competitive inhibition. The Behavioral and Brain Sciences 22, 4: 661–674; discussion 674–721. [Google Scholar] [CrossRef]
- Hooge, I. T. C., E. A. B. Over, R. J. A. van Wezel, and M. A. Frens. 2005. Inhibition of return is not a foraging facilitator in saccadic search and free viewing. Vision Research 45, 14: 1901–1908. [Google Scholar] [CrossRef]
- Hooge, I., and M. Frens. 2000. Inhibition of saccade return (ISR): Spatio-temporal properties of saccade programming. Vision Research 40, 24: 3415–3426. [Google Scholar] [CrossRef]
- Horowitz, T., and J. Wolfe. 1998. Visual search has no memory. Nature 394, 6693: 575–577. [Google Scholar] [CrossRef]
- Itti, L., and C. Koch. 2001. Computational modelling of visual attention. Nature Reviews Neuroscience 2, 3: 194–203. [Google Scholar] [CrossRef]
- Jiang, H., B. E. Stein, and J. G. McHaffie. 2003. Opposing basal ganglia processes shape midbrain visuomotor activity bilaterally. Nature 423, 6943: 982–986. [Google Scholar] [CrossRef] [PubMed]
- Klein, R. 1988. Inhibitory tagging system facilitates visual search. Nature 334, 6181: 430–431. [Google Scholar] [CrossRef] [PubMed]
- Klein, R. M., and J. W. MacInnes. 1999. Inhibition of return is a foraging facilitator in visual search. Psychological Science 10, 4: 346–352. [Google Scholar] [CrossRef]
- Koch, C., and S. Ullman. 1985. Shifts in selective visual attention: Towards the underlying neural circuitry. Human Neurobiology 4, 4: 219–27. [Google Scholar]
- Kopecz, K. 1995. Saccadic reaction times in gap/overlap paradigms: a model based on integration of intentional and visual information on neural, dynamic fields. Vision Research 35, 20: 2911–2925. [Google Scholar] [CrossRef]
- Kopecz, K., and G. Schöner. 1995. Saccadic motor planning by integrating visual information and pre-information on neural dynamic fields. Biological Cybernetics 73, 1: 49–60. [Google Scholar] [CrossRef]
- MacInnes, J. W., and R. M. Klein. 2003. Inhibition of return biases orienting during the search of complex scenes. The Scientific World Journal 3: 75–86. [Google Scholar] [CrossRef]
- Mathôt, S., and J. Theeuwes. 2010. Gradual remapping results in early retinotopic and late spatiotopic inhibition of return. Psychological Science 21, 12: 1793–1798. [Google Scholar] [CrossRef]
- Maylor, E. A., and R. Hockey. 1985. Inhibitory component of externally controlled covert orienting in visual space. Journal of Experimental Psychology: Human Perception and Performance 11, 6: 777–787. [Google Scholar] [CrossRef]
- Meeter, M., S. Van der Stigchel, and J. Theeuwes. 2010. A competitive integration model of exogenous and endogenous eye movements. Biological Cybernetics 102, 4: 271–291. [Google Scholar] [CrossRef][Green Version]
- Munoz, D. P., and R. H. Wurtz. 1993. Fixation cells in monkey superior colliculus. I. Characteristics of cell discharge. Journal of Neurophysiology 70, 2: 559–575. [Google Scholar] [CrossRef] [PubMed]
- Munoz, D. P., and R. H. Wurtz. 1995a. Saccade-related activity in monkey superior colliculus. II. Spread of activity during saccades. Journal of Neurophysiology 73, 6: 2334–2348. [Google Scholar] [CrossRef] [PubMed]
- Munoz, D. P., and R. H. Wurtz. 1995b. Saccade-related activity in monkey superior colliculus. I. Characteristics of burst and buildup cells. Journal of Neurophysiology 73, 6: 2313–2333. [Google Scholar] [CrossRef] [PubMed]
- Munoz, D. P., D. Pelisson, and D. Guitton. 1991. Movement of neural activity on the superior colliculus motor map during gaze shifts. Science 251, 4999: 1358–1360. [Google Scholar] [CrossRef]
- Munoz, D. P., and J. D. Schall. 2003. Edited by W. C. Hall and A. K. Moschovakis. Concurrent, distributed control of saccade initiation in the frontal eye field and superior colliculus. In The superior colliculus: New approaches for studying sensorimotor integration. New York: CRC Press: pp. 55–82. [Google Scholar]
- Munoz, D. P., and J. H. Fecteau. 2002. Vying for dominance: Dynamic interactions control visual fixation and saccadic initiation in the superior colliculus. In The Brain’s eye: Neurobiological and clinical aspects of oculomotor research. Elsevier: Vol. 140, pp. 3–19. [Google Scholar]
- Rafal, R., R. Egly, and D. Rhodes. 1994. Effects of inhibition of return on voluntary and visually guided saccades. Canadian Journal of Experimental Psychology 48, 2: 284–300. [Google Scholar] [CrossRef]
- Robinson, D. A. 1972. Eye movements evoked by collicular stimulation in the alert monkey. Vision Research 12, 11: 1795–1808. [Google Scholar] [CrossRef] [PubMed]
- Satel, J., Z. Wang, T. P. Trappenberg, and R. M. Klein. 2011. Modeling inhibition of return as short-term depression of early sensory input to the superior colliculus. Vision Research 51, 9: 987–996. [Google Scholar] [CrossRef][Green Version]
- Smith, T. J., and J. M. Henderson. 2009. Facilitation of return during scene viewing. Visual Cognition 17, 6: 1083–1108. [Google Scholar] [CrossRef]
- Soetedjo, R., C. R. S. Kaneko, and A. F. Fuchs. 2002. Evidence against a moving hill in the superior colliculus during saccadic eye movements in the monkey. Journal of Neurophysiology 87, 6: 2778–2789. [Google Scholar] [CrossRef]
- Souto, D., and D. Kerzel. 2009. Involuntary cueing effects during smooth pursuit: Facilitation and inhibition of return in oculocentric coordinates. Experimental Brain Research 192, 1: 25–31. [Google Scholar] [CrossRef]
- Taylor, T., and R. Klein. 1998. On the causes and effects of inhibition of return. Psychonomic Bulletin & Review 5, 4: 625–643. [Google Scholar]
- Taylor, T. L., and R. M. Klein. 2000. Visual and motor effects in inhibition of return. Journal of Experimental Psychology: Human Perception and Performance 26, 5: 1639–1656. [Google Scholar] [CrossRef]
- Trappenberg, T., M. Dorris, D. Munoz, and R. Klein. 2001. A model of saccade initiation based on the competitive integration of exogenous and endogenous signals in the superior colliculus. Journal of Cognitive Neuroscience 13, 2: 256–271. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z., and R. M. Klein. 2010. Searching for inhibition of return in visual search: A review. Vision Research 50, 2: 220–228. [Google Scholar] [CrossRef]
- von Wartburg, R., P. Wurtz, T. Pflugshaupt, T. Nyffeler, M. Lüthi, and R. M. Müri. 2007. Size matters: Saccades during scene perception. Perception 36, 3: 355–365. [Google Scholar] [CrossRef] [PubMed]
- Wilimzig, C., S. Schneider, and G. Schoener. 2006. The time course of saccadic decision making: Dynamic field theory. Neural Networks 19, 8: 1059–1074. [Google Scholar] [CrossRef]
- Wilson, H. R., and J. D. Cowan. 1973. A mathematical theory of the functional dynamics of cortical and thalamic nervous tissue. Biological Cybernetics 13, 2: 55–80. [Google Scholar] [CrossRef]

Copyright © 2011. This article is licensed under a Creative Commons Attribution 4.0 International License.
Share and Cite
Wang, Z.; Satel, J.; Trappenberg, T.P.; Klein, R.M. Aftereffects of Saccades Explored in a Dynamic Neural Field Model of the Superior Colliculus. J. Eye Mov. Res. 2011, 4, 1-16. https://doi.org/10.16910/jemr.4.2.1
Wang Z, Satel J, Trappenberg TP, Klein RM. Aftereffects of Saccades Explored in a Dynamic Neural Field Model of the Superior Colliculus. Journal of Eye Movement Research. 2011; 4(2):1-16. https://doi.org/10.16910/jemr.4.2.1
Chicago/Turabian StyleWang, Zhiguo, Jason Satel, Thomas P. Trappenberg, and Raymond M. Klein. 2011. "Aftereffects of Saccades Explored in a Dynamic Neural Field Model of the Superior Colliculus" Journal of Eye Movement Research 4, no. 2: 1-16. https://doi.org/10.16910/jemr.4.2.1
APA StyleWang, Z., Satel, J., Trappenberg, T. P., & Klein, R. M. (2011). Aftereffects of Saccades Explored in a Dynamic Neural Field Model of the Superior Colliculus. Journal of Eye Movement Research, 4(2), 1-16. https://doi.org/10.16910/jemr.4.2.1


