Environmental, Social and Governance-Valued Portfolio Optimization and Dynamic Asset Pricing
Abstract
1. Introduction
To achieve this, we develop an asset valuation model based on considering the ESG score as a variable independent of both expected return and risk measure. While this approach can be considered axiomatic relative to our goal, it is, in fact, based upon three considerations. First, we note that the plethora of rating agencies and subsequent ESG-rating methodologies (not all of which are publicly available or fully disclosed), each involving a large number of input factors, argues in favor of independence. A second consideration, emerging from a review of the literature, is that ESG investors are motivated by concerns that place a high value on societal benefit, and not just on financial return. Thirdly, we are wary of incorporating any implicit bias that presupposes “higher ESG ratings translate to higher financial return” (or the opposite). Specifically, we are wary of presupposing that ESG ratings and financial return must be correlated.1 For example, the recent work by Berg et al. (2021) suggests that Refinitiv’s reworking of their ESG scoring methodology in 2020, which was then used to rewrite their ESG ratings for previous years, may, in fact, reflect such a bias.2 (See also the discussion in Section 2 of the literature related to potential ESG valuation bias.) As revealed by the discussion in Section 2, the academic literature (as well as reporting through financial news, social media and blog outlets) suggests there are too many factors determining what elements of ESG are taken into consideration by individual investors and investment firms to support a presupposition of ESG score-to-financial return correlation.In this regard, we clarify from the beginning that, unlike many of the ESG-based articles referenced in Section 2, our goal in this work is not to contend or prove that ESG investing leads to better financial performance. Rather, we want an ESG-based financial model that will allow unbiased determination as to when, and to what extent, this may be true.
2. ESG Research Overview and ESG Approaches to Portfolio Optimization
3. ESG-Valued Returns
3.1. Scaling of ESG Values
4. ESG-Valued Portfolio Optimization
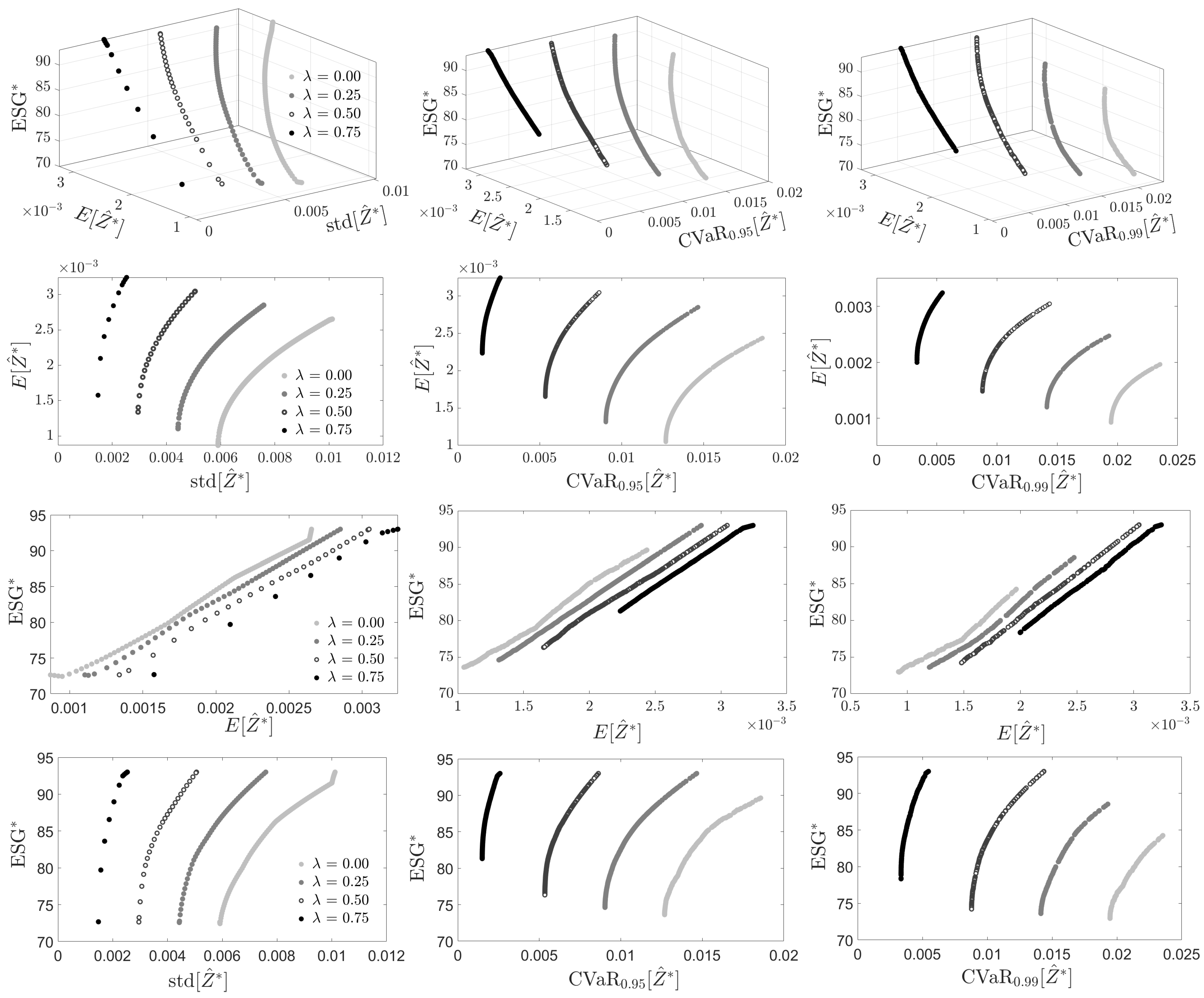
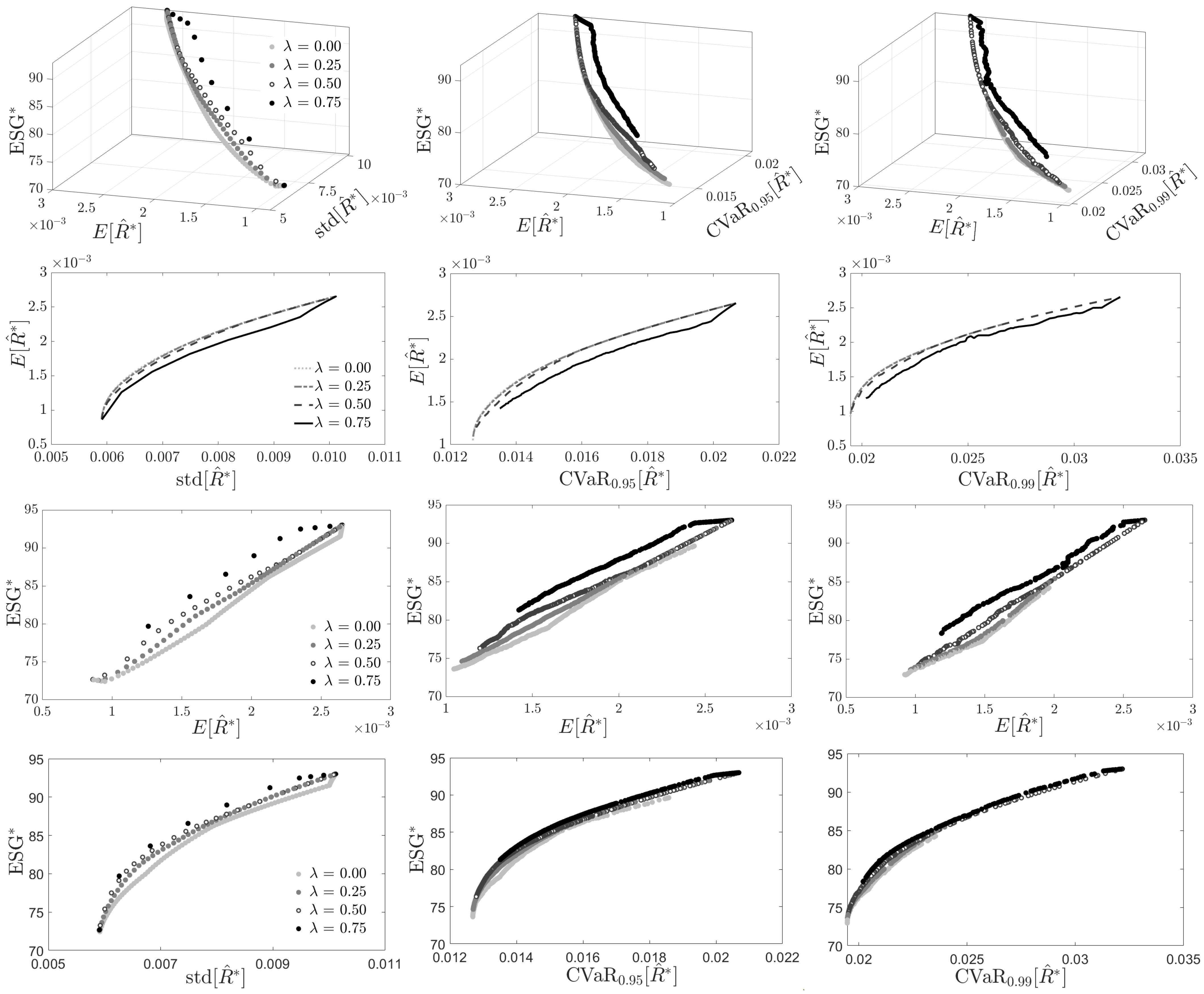
4.1. ESG-Efficient Frontier
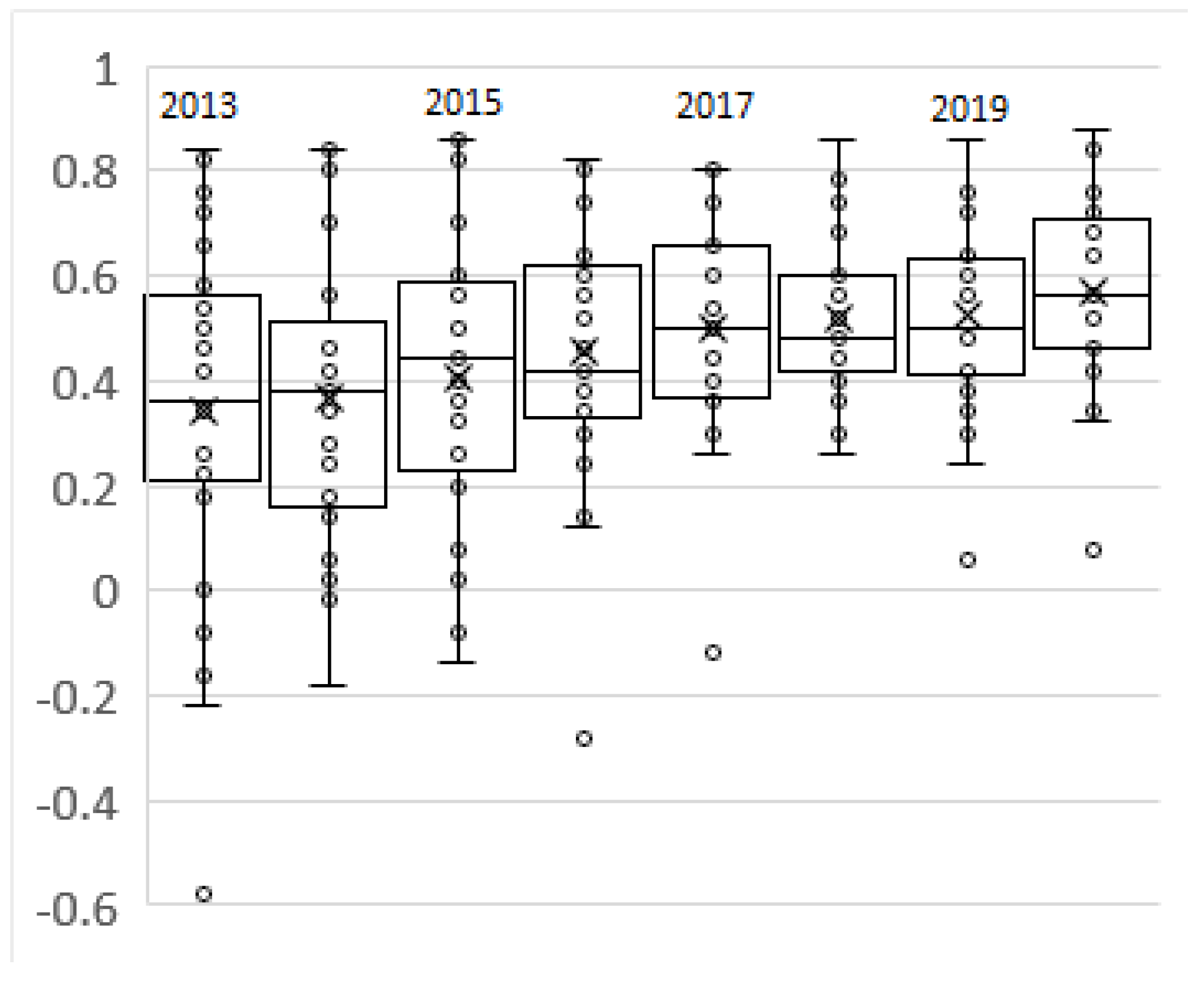
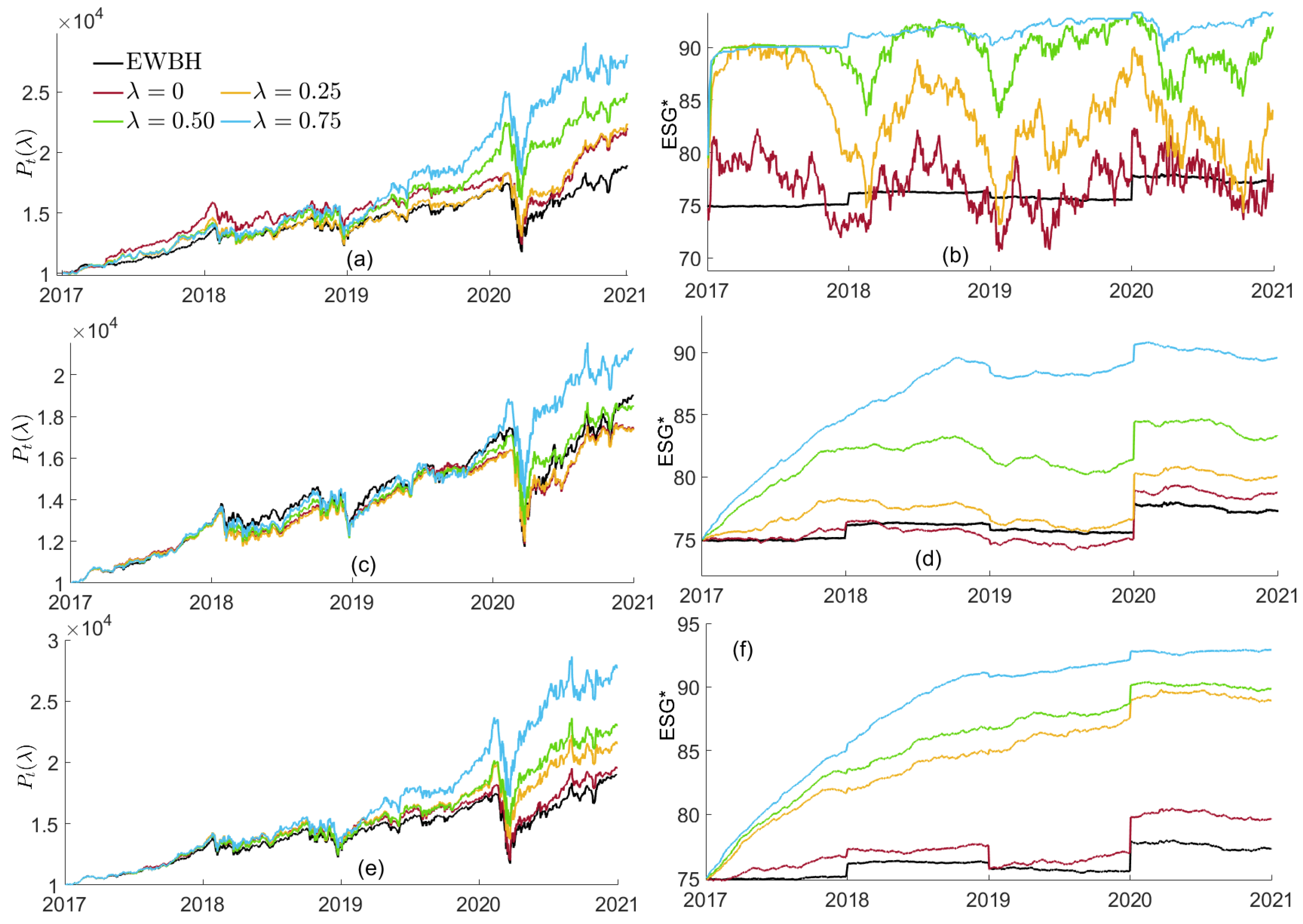
5. Performance over Time
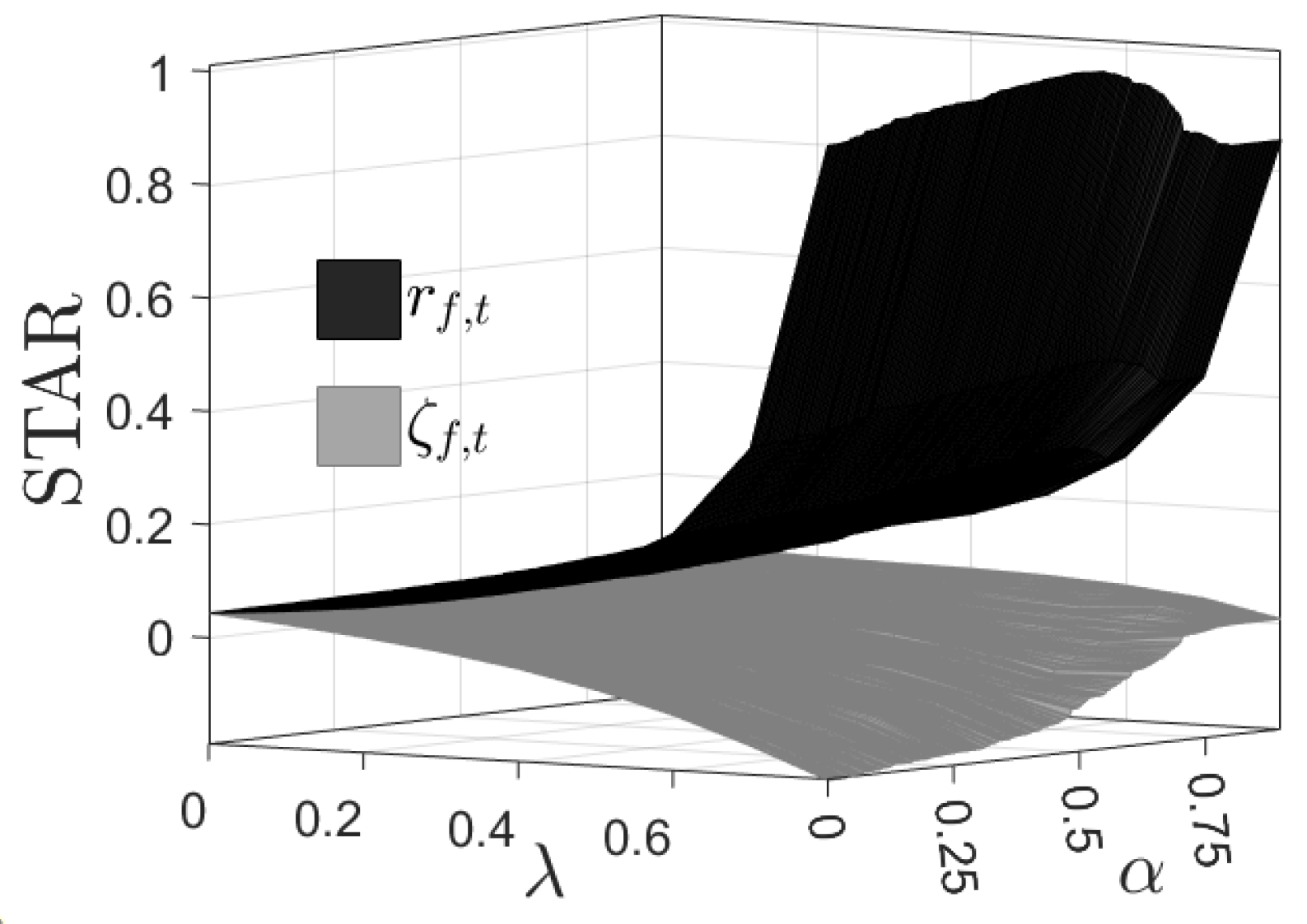
5.1. ESG-Valued Reward–Risk Ratios
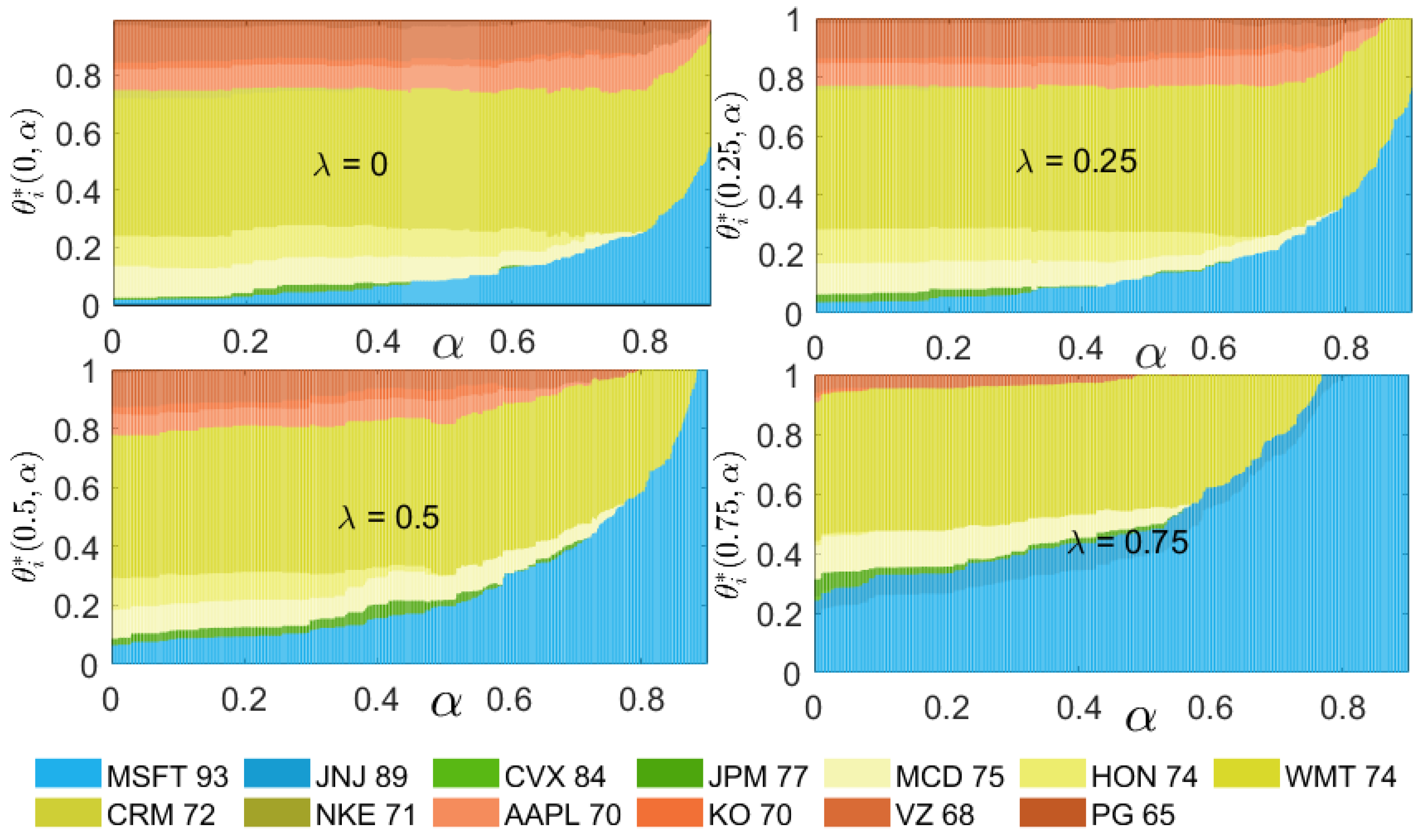
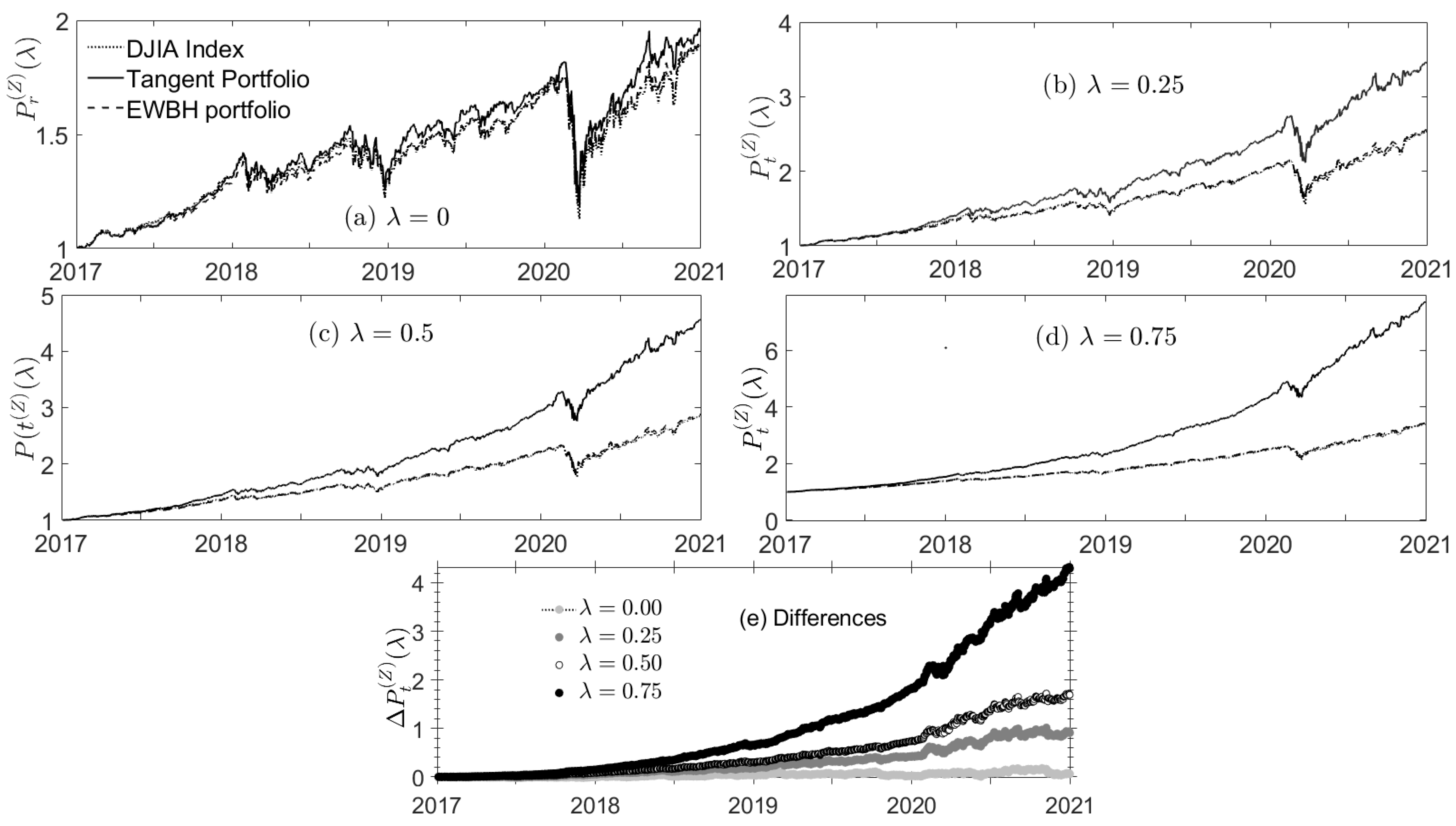
6. ESG-Valued Tangent Portfolios
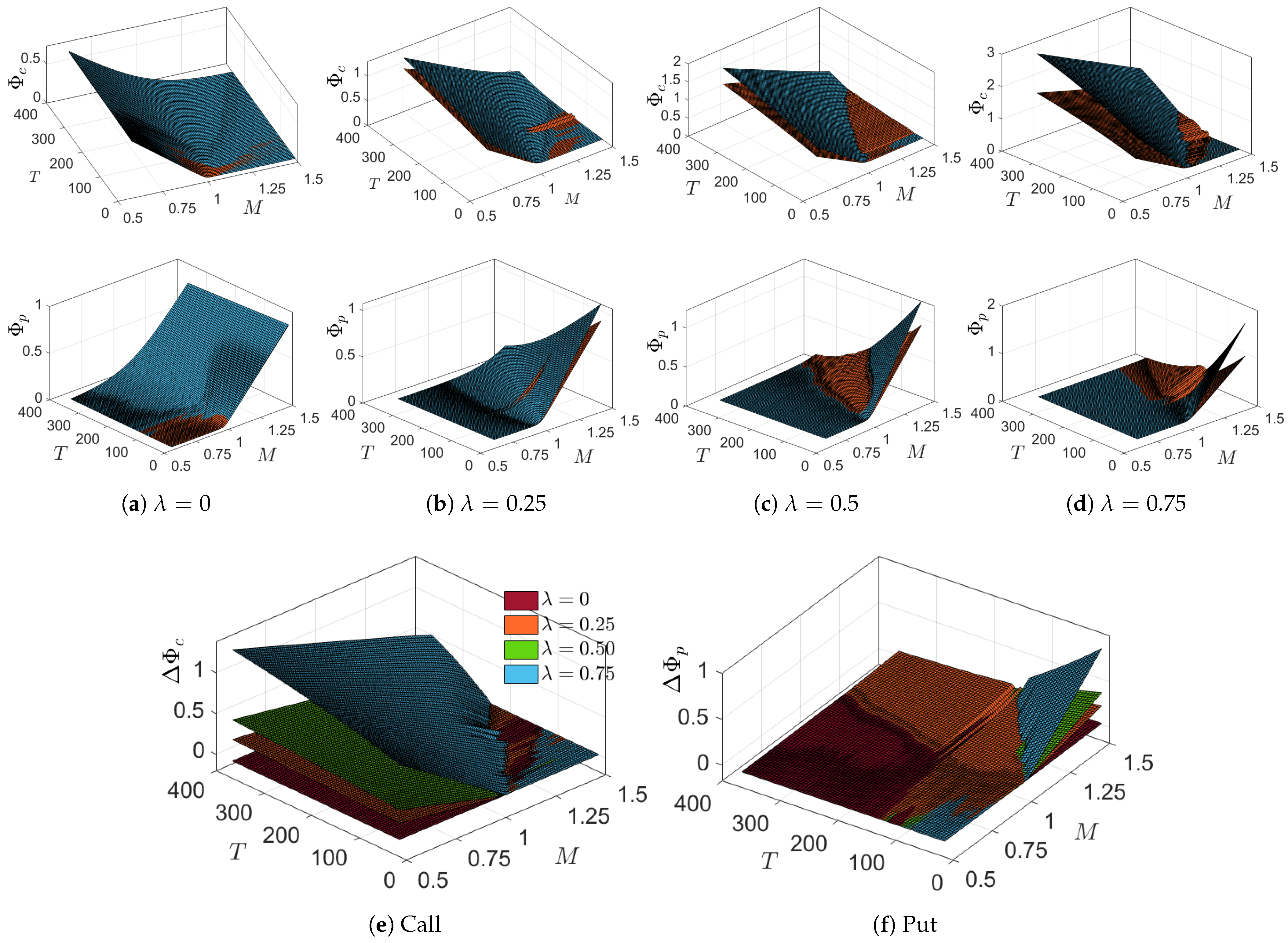
7. ESG-Valued Option Pricing
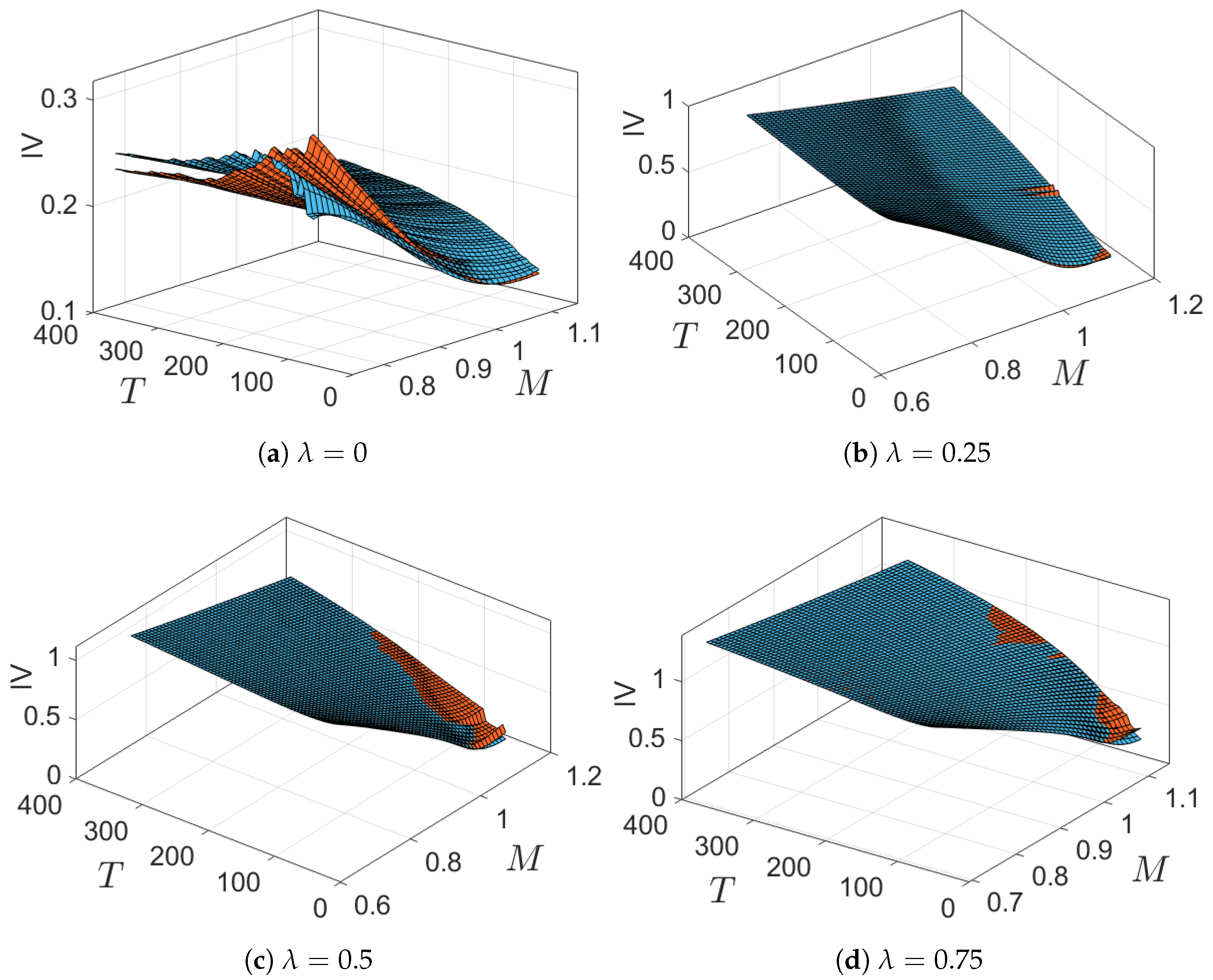
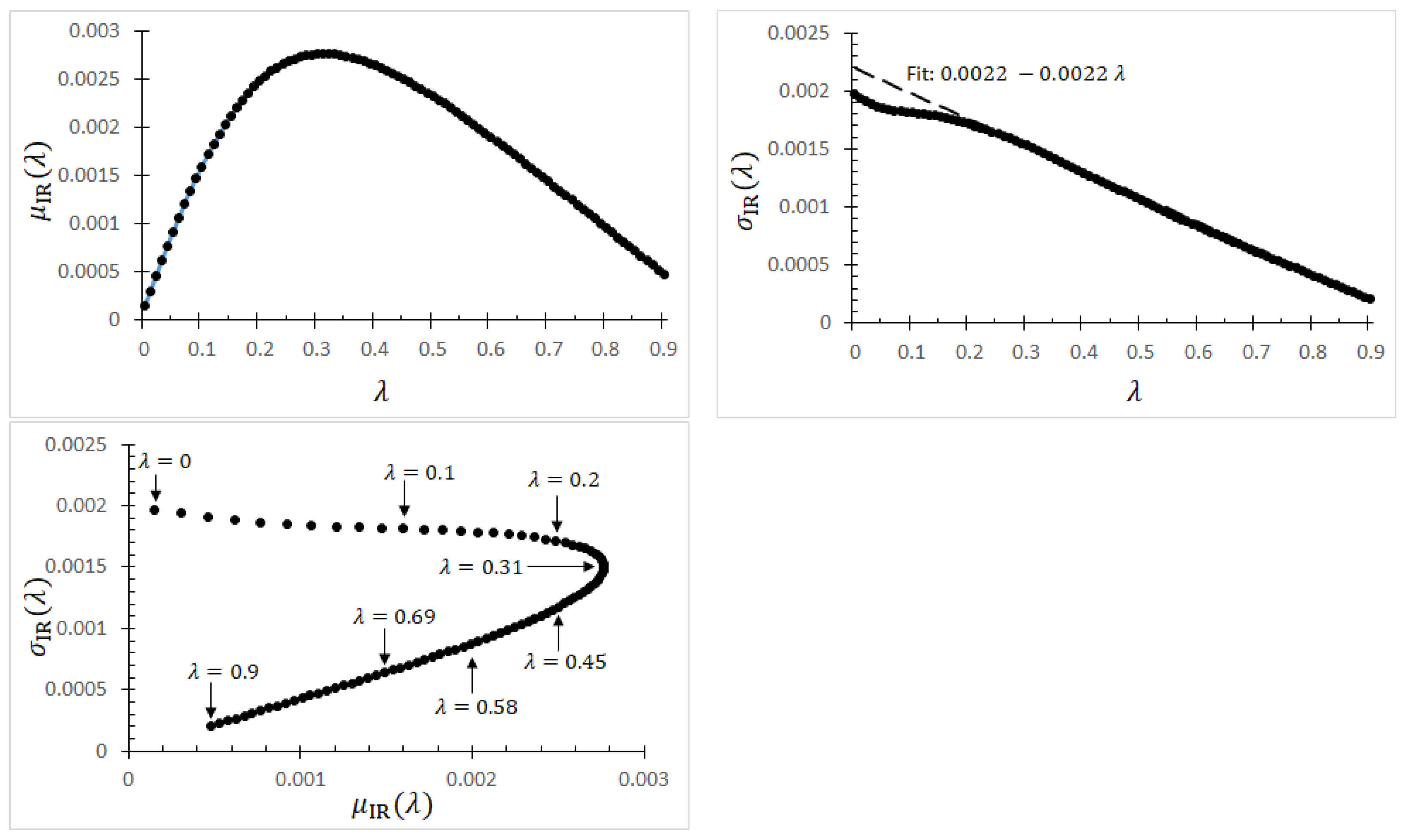
8. ESG-Valued Shadow Riskless Rate
9. Variation in Agency-Provided ESG Scores
10. Discussion
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. MV and mCVaRβ ESG-Valued Optimization
Appendix B. ESG-Valued Option Pricing: Discrete Model
Appendix C. Shadow Riskless Rate
| 1 | Extensive literature reviews, such as those conducted by Friede et al. (2015) and Brooks and Oikonomou (2018), have revealed less-than-conclusive results regarding the strength of correlation between ESG scores and financial performance. |
| 2 | “It is also possible that Refinitiv ESG adjusted the methodology to take into account certain factors that happen to be correlated with firms’ stock price performance.”, Berg et al. (2021). |
| 3 | In the notation of Pástor et al., their ESG-valued return for investor i is expressed as , where is (a vector of) the excess financial returns for the assets under consideration, is the risk aversion coefficient for investor i, g is a (vector of) the “greeness” of each asset and is the “ESG taste”. Unlike our model, there is no constraint between the financial and ESG contributions to their return. |
| 4 | Due to the non-Gaussian nature of our portfolio optimization, we hesitate to postulate a capital asset pricing model based upon this capital market line. |
| 5 | In fact, their optimization can only depend on the ratios of two of these parameters relative to the third. |
| 6 | The determination of the resultant equilibrium model will not be straightforward, given the rich one-step ahead predictive environment involving ARMA-GARCH with NIG returns used in our portfolio optimization model. |
| 7 | Sections S1–S9, Tables S1–S13, and Figures S1–S11 refer to the Supplementary Material supplied as an online resource for this paper. |
| 8 | e.g., ESG Funds Resist Worst of Downturn But Investors Are Spooked (Bloomberg, 18 June 2022), https://www.bloomberg.com/news/articles/2022-06-18/esg-funds-are-losing-less-in-the-market-slump-so-far (accessed on 14 July 2022); ESG Backlash Has Fund Clients Demanding Proof It Works (Bloomberg, 31 May 2022), https://www.bloomberg.com/news/articles/2022-05-31/esg-fund-clients-demand-proof-strategy-works-amid-backlash (accessed on 14 July 2022); Sustainable Investing Failed Its First Big Test. A Reckoning Is Coming. (Barrons, 17 April 2022), |
| 9 | ESG Can’t Square with Fiduciary Duty. (Wall Street Journal, 7 July 2022), |
| 10 | We develop our formalism for daily trading. |
| 11 | This normalization is discussed in Section 3.1. |
| 12 | We note that ESG ratings are currently effectively updated by most rating agencies once a year. |
| 13 | Some literature has focused on the higher returns provided by portfolios based on certain ESG criteria. Pedersen et al. (2021) found that governance factors are more linked to financial performance than those related to environmental impact. |
| 14 | Although some providers distribute monthly scores, these data are usually expensive and are not provided on a regular basis. |
| 15 | Environmental, Social and Governance Scores from Refinitiv; accessed on 22 February 2021. |
| 16 | Equation (3) assumes the difference between daily log-return values and arithmetic return values is negligibly small. We continue this assumption throughout the paper. |
| 17 | Artzner et al. (1999) proposed an axiomatic approach to define the minimum set of properties that should satisfy to be a coherent measure of risk. Frittelli and Rosazza Gianin (2002) have specified requirements to define a convex risk measure. |
| 18 | Due to its shorter span of existence, stock from DOW Inc is not included in the portfolio. |
| 19 | Persistence was found to be both stock and time dependent; even for the same stock, the presence of persistence could change over time. |
| 20 | Settling on a nomenclature for for this article has not been easy. The phrase “ESG-valued numeraire” is awkward. The shorter “ESG value” leads to confusion between values of and values of ESG score. The phrase “ESG-valued price” or “ESG price” runs the risk of continuing to blur the distinction between and . Finding nothing better, we run that risk and will refer to as the ESG-valued price (ESG price for brevity). |
| 21 | For this portfolio, there is essentially no change in the optimized solutions over the range . |
| 22 | As a consequence of the non-linear spacing of the efficient frontiers, the curves in Figure 3d–f have been delineated through solid lines rather than points in order to be able to differentiate those corresponding to smaller values of . |
| 23 | Do ESG Ratings Impact Stock Price Volatility?, ISS LiquidMetrix report, 12 July 2022. https://www.issgovernance.com/library/do-esg-ratings-impact-stock-price-volatility/ (accessed on 14 July 2022). |
| 24 | The relative difference in the portfolio ESG score is defined in the usual manner as (ESG(out-of-sample) − ESG(in-sample))/ESG(in-sample). |
| 25 | With reference to Equations (7) and (8), the EWBH portfolio is defined as follows. ; the stock for Dow Inc. remains excluded. No scenario sets are generated; no optimization is performed. At , . For , the number of shares of each stock remains fixed at ; thus , where is the price of the asset at the close of trading day t. |
| 26 | Tot Rtn, Ann Rtn, ETL95, ETR95 and MDD are all computed on the financial returns. |
| 27 | Also known as conditional value-at-risk or expected shortfall. |
| 28 | To compute the STAR ratio, we used the 10-year U.S. treasury yield rate to determine daily values for . Computation of the expectation and ETL in the STAR ratio is performed over the ensemble of ESG-valued returns generated for day based upon the information available at day t. |
| 29 | Here we define the Sharpe, Sortino and STAR ratios using only the portfolio returns and not the excess returns relative to a benchmark. |
| 30 | While this approach can be used to formulate an ESG-valued capital asset pricing model and the two-fund separation theorem (Tobin, 1958), as noted in the introduction we prefer to derive the equilibrium formulation from this model based upon firmer principles. |
| 31 | The analysis of ESG factors associated with a fixed income security, especially one issued by a government entity, involves dealing with complex methodological and technical implications (Badía et al., 2019; Inderst & Stewart, 2018). |
| 32 | Another possibility is to use an ESG score for the appropriate country for a government bond. Some agencies are beginning to provide such scores, though not necessarily in the same scale used for companies. |
| 33 | It is critical to emphasize that the tangent portfolios are identified using the efficient frontiers computed in space and not in space. The optimization is performed in the former space, not the latter. As can be seen by comparing Figure 2 and Figure 3, convexity of the efficient frontiers can only be guaranteed in the former space. |
| 34 | With reference to Equations (7) and (8), the DJIA index is defined as follows: . No scenario sets are generated; where is the DJIA published weight for component i and is the published weight for Dow Inc., which is excluded from our benchmark index. We utilized the published DJIA weights applicable to 30/12/2020 in composing our index. |
| 35 | For , strike values in this ESG formulation are not traditional strike prices. |
| 36 | There is currently no theory governing identification of the standard deviation parameters from the elements of the variance-covariance matrix of asset returns. The Cholesky decomposition approach used here is ad hoc. |
| 37 | We perform the SRR computations on the DJIA index rather than the optimized portfolios because the Dow Index is conventionally recognized as an indicator of the general health of the US stock market (though there are valid arguments against this). |
| 38 | As noted in Section 4, we consider long-only portfolio optimization. |
| 39 | For the computations in Section 6, we used , . |
| 40 | More complex pricing models have been considered (Rachev et al., 2017); here, we employ the basic Brownian motion model. |
| 41 | This follows directly from Itô’s formula. |
References
- Abate, G., Basile, I., & Ferrari, P. (2021). The level of sustainability and mutual fund performance in Europe: An empirical analysis using ESG ratings. Corporate Social Responsibility and Environmental Management, 28(5), 1446–1455. [Google Scholar] [CrossRef]
- Amel-Zadeh, A., & Serafeim, G. (2018). Why and how investors use ESG information: Evidence from a global survey. Financial Analysts Journal, 74(3), 87–103. [Google Scholar] [CrossRef]
- Artzner, P., Delbaen, F., Eber, J.-M., & Heath, D. (1999). Coherent measures of risk. Mathematical Finance, 9(3), 203–228. [Google Scholar] [CrossRef]
- Avellaneda, M., Buff, R., Friedman, C., Grandechamp, N., Kruck, L., & Newman, J. (2001). Weighted Monte Carlo: A new technique for calibrating asset-pricing models. International Journal of Theoretical and Applied Finance, 4(1), 91–119. [Google Scholar] [CrossRef]
- Badía, G., Pina, V., & Torres, L. (2019). Financial performance of government bond portfolios based on environmental, social and governance criteria. Sustainability, 11(9), 2514. [Google Scholar] [CrossRef]
- Barndorff-Nielsen, O. E. (1977). Exponentially decreasing distributions for the logarithm of particle size. Proceedings of The Royal Society A: Mathematical, Physical and Engineering Sciences, 353(1674), 401–419. [Google Scholar]
- Barndorff-Nielsen, O. E. (1997). Normal inverse Gaussian distributions and stochastic volatility modelling. Scandinavian Journal of Statistics, 24(1), 1–13. [Google Scholar] [CrossRef]
- Basel Committee on Bank Supervision. (2019). Explanatory note on the minimum capital requirements for market risk. Tech. Rep. Bank for International Settlements. Available online: https://www.bis.org/bcbs/publ/d457_note.pdf (accessed on 26 April 2024).
- Berg, F., Fabisik, K., & Sautner, Z. (2021). Rewriting history II: The (un)predictable past of ESG ratings. Tech. Rep. ECGI Working Paper Series in Finance 708/2020. Available online: https://papers.ssrn.com/sol3/papers.cfm?abstract_id=3722087 (accessed on 26 April 2024).
- Berg, F., Kölbel, J. F., & Rigobon, R. (2022). Aggregate confusion: The divergence of ESG ratings. Review of Finance, 26(6), 1315–1344. [Google Scholar] [CrossRef]
- Berry, T. C., & Junkus, J. C. (2013). Socially responsible investing: An investor perspective. Journal of Business Ethics, 112(4), 707–720. [Google Scholar] [CrossRef]
- Bialkowski, J., & Starks, L. T. (2016). SRI funds: Investor demand, exogenous shocks and ESG profiles. Working Papers in Economics No. 16/11. University of Canterbury, Department of Economics and Finance. Available online: https://ideas.repec.org/p/cbt/econwp/16-11.html (accessed on 14 July 2022).
- Bilbao-Terol, A., Arenas-Parra, M., Cañal-Fernández, V., & Bilbao-Terol, C. (2013). Selection of socially responsible portfolios using hedonic prices. Journal of Business Ethics, 115(3), 515–529. [Google Scholar] [CrossRef]
- Billio, M., Costola, M., Hristova, I., Latino, C., & Pelizzon, L. (2021). Inside the ESG ratings: (Dis)agreement and performance. Corporate Social Responsibility and Environmental Management, 28(5), 1426–1445. [Google Scholar] [CrossRef]
- Black, F. (1972). Capital market equilibrium with restricted borrowing. The Journal of Business, 45(3), 444–455. [Google Scholar] [CrossRef]
- Black, F. (1995). Interest rates as options. The Journal of Finance, 50(5), 1371–1376. [Google Scholar] [CrossRef]
- Breedt, A., Ciliberti, S., Gualdi, S., & Seager, P. (2019). Is ESG an equity factor or just an investment guide. The Journal of Investing, 28(2), 32–42. [Google Scholar] [CrossRef]
- Brooks, C., & Oikonomou, I. (2018). The effects of environmental, social and governance disclosures and performance on firm value: A review of the literature in accounting and finance. The British Accounting Review, 50(1), 1–15. [Google Scholar] [CrossRef]
- Cesarone, F., Martino, M. L., & Carleo, A. (2022). Does ESG impact really enhance portfolio profitability? Sustainability, 14(4), 2050. [Google Scholar] [CrossRef]
- Chatterji, A. K., Durand, R., Levine, D. I., & Touboul, S. (2016). Do ratings of firms converge? Implications for managers, investors and strategy researchers. Strategic Management Journal, 37(8), 1597–1614. [Google Scholar] [CrossRef]
- Cheklov, A., Uryasev, S., & Zabarankin, M. (2005). Drawdown measure in portfolio optimization. International Journal of Theoretical and Applied Finance, 8(1), 13–58. [Google Scholar] [CrossRef]
- Chen, L., Zhang, L., Huang, J., Xiao, H., & Zhou, Z. (2021). Social responsibility portfolio optimization incorporating ESG criteria. Journal of Management Science and Engineering, 6(1), 75–85. [Google Scholar] [CrossRef]
- Chen, M., & Mussalli, G. (2020). An integrated approach to quantitative ESG investing. Journal of Portfolio Management, 46, 65–74. [Google Scholar] [CrossRef]
- Cheridito, P., & Kromer, E. (2013). Reward-risk ratios. Journal of Investment Strategies, 3, 3–18. [Google Scholar] [CrossRef]
- Chorro, C., Guégan, D., & Lelpo, F. (2010). Option pricing for GARCH-type models with generalized hyperbolic innovations. Quantitative Finance, 12, 1079–1094. [Google Scholar] [CrossRef]
- Christensen, D. M., Serafeim, G., & Sikochi, A. (2021). Why is corporate virtue in the eye of the beholder? The case of ESG ratings. The Accounting Review, 97(1), 147–175. [Google Scholar] [CrossRef]
- Clarke, R., de Silva, H., & Thorley, S. (2002). Portfolio constraints and the fundamental law of active management. Financial Analysts Journal, 58(5), 48–66. [Google Scholar] [CrossRef]
- Daugaard, D. (2020). Emerging new themes in environmental, social and governance investing: A systematic literature review. Accounting & Finance, 60(2), 1501–1530. [Google Scholar]
- Delbaen, F., & Schachermayer, W. (1994). A general version of the fundamental theorem of asset pricing. Mathematische Annalen, 300(1), 463–520. [Google Scholar] [CrossRef]
- Duffie, D. (2001). Dynamic asset pricing theory (3rd ed.). Princeton Univ. Press. [Google Scholar]
- Fama, E., & French, K. (2004). The capital asset pricing model: Theory and evidence. Journal of Economic Perspectives, 18, 25–46. [Google Scholar] [CrossRef]
- Friede, G., Busch, T., & Bassen, A. (2015). ESG and financial performance: Aggregated evidence from more than 2000 empirical studies. Journal of Sustainable Finance & Investment, 5(4), 210–233. [Google Scholar]
- Frittelli, M., & Rosazza Gianin, E. (2002). Putting order in risk measures. Journal of Banking & Finance, 26(7), 1473–1486. [Google Scholar]
- Gasser, S. M., Rammerstorfer, M., & Weinmayer, K. (2017). Markowitz revisited: Social portfolio engineering. European Journal of Operational Research, 258(3), 1181–1190. [Google Scholar] [CrossRef]
- Geczy, C. C., & Guerard, J. (2023). ESG and expected returns on equities: The case of environmental ratings. In P. B. Hammond, R. Maurer, & O. Mitchell (Eds.), Pension funds and sustainable investment: Challenges and opportunities. Oxford Academic. [Google Scholar] [CrossRef]
- Ghalanos, A. (2024). Introduction to the rugarch package (Version 1.4-3) [Computer software manual]. Available online: https://cran.r-project.org/web/packages/rugarch/vignettes/Introduction_to_the_rugarch_package.pdf (accessed on 3 October 2024).
- Gibson Brandon, R., Krueger, P., & Schmidt, P. S. (2021). ESG rating disagreement and stock returns. Financial Analysts Journal, 77(4), 104–127. [Google Scholar] [CrossRef]
- Giese, G., Lee, L.-E., Melas, D., Nagy, Z., & Nishikawa, L. (2019). Foundations of ESG investing: How ESG affects equity valuation, risk, and performance. The Journal of Portfolio Management, 45(5), 69–83. [Google Scholar] [CrossRef]
- Görgen, M., Jacob, A., Nerlinger, M., Riordan, R., Rohleder, M., & Wilkens, M. (2020). Carbon risk. Environmental Economics eJournal. Available online: https://api.semanticscholar.org/CorpusID:219367357 (accessed on 14 July 2022). [CrossRef]
- Hartzmark, S. M., & Sussman, A. B. (2019). Do investors value sustainability? A natural experiment examining ranking and fund flows. The Journal of Finance, 74(6), 2789–2837. [Google Scholar] [CrossRef]
- Hirschberger, M., Steuer, R. E., Utz, S., Wimmer, M., & Qi, Y. (2013). Computing the nondominated surface in tri-criterion portfolio selection. Operations Research, 61(1), 169–183. [Google Scholar] [CrossRef]
- Höchstädter, A. K., & Scheck, B. (2015). What’s in a name: An analysis of impact investing understandings by academics and practitioners. Journal of Business Ethics, 132(2), 449–475. [Google Scholar] [CrossRef]
- Hu, Y., Lindquist, W. B., & Rachev, S. T. (2024). Sustainability-valued discrete option pricing in complete markets. Journal of Sustainable Finance & Investment, 1–35. [Google Scholar] [CrossRef]
- Inderst, G., & Stewart, F. (2018). Incorporating environmental, social and governance (ESG) factors into fixed income investment. Tech. Rep. World Bank Group. [Google Scholar]
- Kirby, C., & Ostdiek, B. (2012). It’s all in the timing: Simple active portfolio strategies that outperform naïve diversification. The Journal of Financial and Quantitative Analysis, 47(2), 437–467. [Google Scholar] [CrossRef]
- Kolm, P. N., Tütüncü, R., & Fabozzi, F. J. (2014). 60 Years of portfolio optimization: Practical challenges and current trends. European Journal of Operational Research, 234(2), 356–371. [Google Scholar] [CrossRef]
- Konno, H., & Yamazaki, H. (1991). Mean-absolute deviation portfolio optimization model and its applications to Tokyo stock market. Management Science, 37(5), 519–531. [Google Scholar] [CrossRef]
- Krüger, P., Sautner, Z., & Starks, L. T. (2020). The importance of climate risks for institutional investors. The Review of Financial Studies, 33(3), 1067–1111. [Google Scholar] [CrossRef]
- Lwin, K. T., Qu, R., & MacCarthy, B. L. (2017). Mean-VaR portfolio optimization: A nonparametric approach. European Journal of Operational Research, 260(2), 751–766. [Google Scholar] [CrossRef]
- Mansini, R., Ogryczak, W., & Speranza, M. G. (2007). Conditional value at risk and related linear programming models for portfolio optimization. Annals of Operations Research, 152(1), 227–256. [Google Scholar] [CrossRef]
- Markowitz, H. (1952). Portfolio selection. The Journal of Finance, 7(1), 77–91. [Google Scholar]
- Markowitz, H. (1956). The optimization of a quadratic function subject to linear constraints. Naval Research Logistics Quarterly, 3(1–2), 111–133. [Google Scholar] [CrossRef]
- Martin, R. D., Rachev, S. T., & Siboulet, F. (2003). Phi-alpha optimal portfolios and extreme risk management. Wilmott, 2003, 70–83. [Google Scholar] [CrossRef]
- Michaud, R. O. (1989). The Markowitz optimization enigma: Is ‘optimized’ optimal? Financial Analysts Journal, 45(1), 31–42. [Google Scholar] [CrossRef]
- Pan, Y., Pikulina, E. S., Siegel, S., & Wang, T. Y. (2022). Do equity markets care about income inequality? Evidence from pay ratio disclosure. The Journal of Finance, 77(2), 1371–1411. [Google Scholar] [CrossRef]
- Pástor, L., Stambaugh, R., & Taylor, L. (2021). Sustainable investing in equilibrium. Journal of Financial Economics, 142, 550–571. [Google Scholar] [CrossRef]
- Pedersen, L. H., Fitzgibbons, S., & Pomorski, L. (2021). Responsible investing: The ESG-efficient frontier. Journal of Financial Economics, 142(2), 572–597. [Google Scholar] [CrossRef]
- Prol, J. L., & Kim, K. (2022). Risk-return performance of optimized ESG equity portfolios in the NYSE. Finance Research Letters, 50, 103312. [Google Scholar] [CrossRef]
- Rachev, S. T., Asare Nyarko, N., Omotade, B., & Yegon, P. (2024). Bachelier’s market model for ESG asset pricing. Journal of Risk and Financial Management, 17, 553. [Google Scholar] [CrossRef]
- Rachev, S. T., Stoyanov, S. V., & Fabozzi, F. J. (2017). Financial markets with no riskless (safe) asset. International Journal of Theoretical and Applied Finance, 20(8), 1750054. [Google Scholar] [CrossRef]
- Rockafellar, R. T., & Uryasev, S. (2000). Optimization of conditional value-at-risk. Journal of Risk, 2, 21–42. [Google Scholar] [CrossRef]
- Sandberg, J., Juravle, C., Hedesström, T. M., & Hamilton, I. (2008). The heterogeneity of socially responsible investment. Journal of Business Ethics, 87(4), 519. [Google Scholar] [CrossRef]
- Sautner, Z., & Starks, L. T. (2023). ESG and downside risks: Implications for pension funds. Oxford University Press. [Google Scholar]
- Schmidt, A. B. (2022). Optimal ESG portfolios: An example for the Dow Jones Index. Journal of Sustainable Finance & Investment, 12, 529–535. [Google Scholar]
- Starks, L. T., Venkat, P., & Zhu, Q. (2017). Corporate ESG profiles and investor horizons. Available online: https://ssrn.com/abstract=3049943 (accessed on 14 July 2022).
- Tobin, J. (1958). Liquidity preference as behavior towards risk. Review of Economic Studies, 25(2), 65–86. [Google Scholar] [CrossRef]
- Utz, S., Wimmer, M., & Steuer, R. E. (2015). Tri-criterion modeling for constructing more-sustainable mutual funds. European Journal of Operational Research, 246(1), 331–338. [Google Scholar] [CrossRef]
- van Duuren, E., Plantinga, A., & Scholtens, B. (2016). ESG integration and the investment management process: Fundamental investing reinvented. Journal of Business Ethics, 138, 525–533. [Google Scholar] [CrossRef]
- Weibel, M., Luethi, D., & Breymann, W. (2013). ghyp: Generalized hyperbolic distribution and its special cases. R Package. [Google Scholar]
- Widyawati, L. (2020). A systematic literature review of socially responsible investment and environmental social governance metrics. Business Strategy and the Environment, 29(2), 619–637. [Google Scholar] [CrossRef]
- Wigglesworth, R. (2018, April 11). How a volatility virus infected Wall Street. The Financial Times. [Google Scholar]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lauria, D.; Lindquist, W.B.; Mittnik, S.; Rachev, S.T. Environmental, Social and Governance-Valued Portfolio Optimization and Dynamic Asset Pricing. J. Risk Financial Manag. 2025, 18, 153. https://doi.org/10.3390/jrfm18030153
Lauria D, Lindquist WB, Mittnik S, Rachev ST. Environmental, Social and Governance-Valued Portfolio Optimization and Dynamic Asset Pricing. Journal of Risk and Financial Management. 2025; 18(3):153. https://doi.org/10.3390/jrfm18030153
Chicago/Turabian StyleLauria, Davide, W. Brent Lindquist, Stefan Mittnik, and Svetlozar T. Rachev. 2025. "Environmental, Social and Governance-Valued Portfolio Optimization and Dynamic Asset Pricing" Journal of Risk and Financial Management 18, no. 3: 153. https://doi.org/10.3390/jrfm18030153
APA StyleLauria, D., Lindquist, W. B., Mittnik, S., & Rachev, S. T. (2025). Environmental, Social and Governance-Valued Portfolio Optimization and Dynamic Asset Pricing. Journal of Risk and Financial Management, 18(3), 153. https://doi.org/10.3390/jrfm18030153








