Finite Difference Simulation of Nonlinear Convection in Magnetohydrodynamic Flow in the Presence of Viscous and Joule Dissipation over an Oscillating Plate
Abstract
:1. Introduction
2. Mathematical Modeling
3. Numerical Methodology (FTCS)
4. Results and Discussion
5. Closing Remarks
- The velocity field diminishes with the increase in the magnetic parameter and Prandtl number.
- Reverse impacts are observed with the non-convective flow parameter; the Eckert number and Grashof number show increments, i.e., when these parameters increase, the velocity also increases.
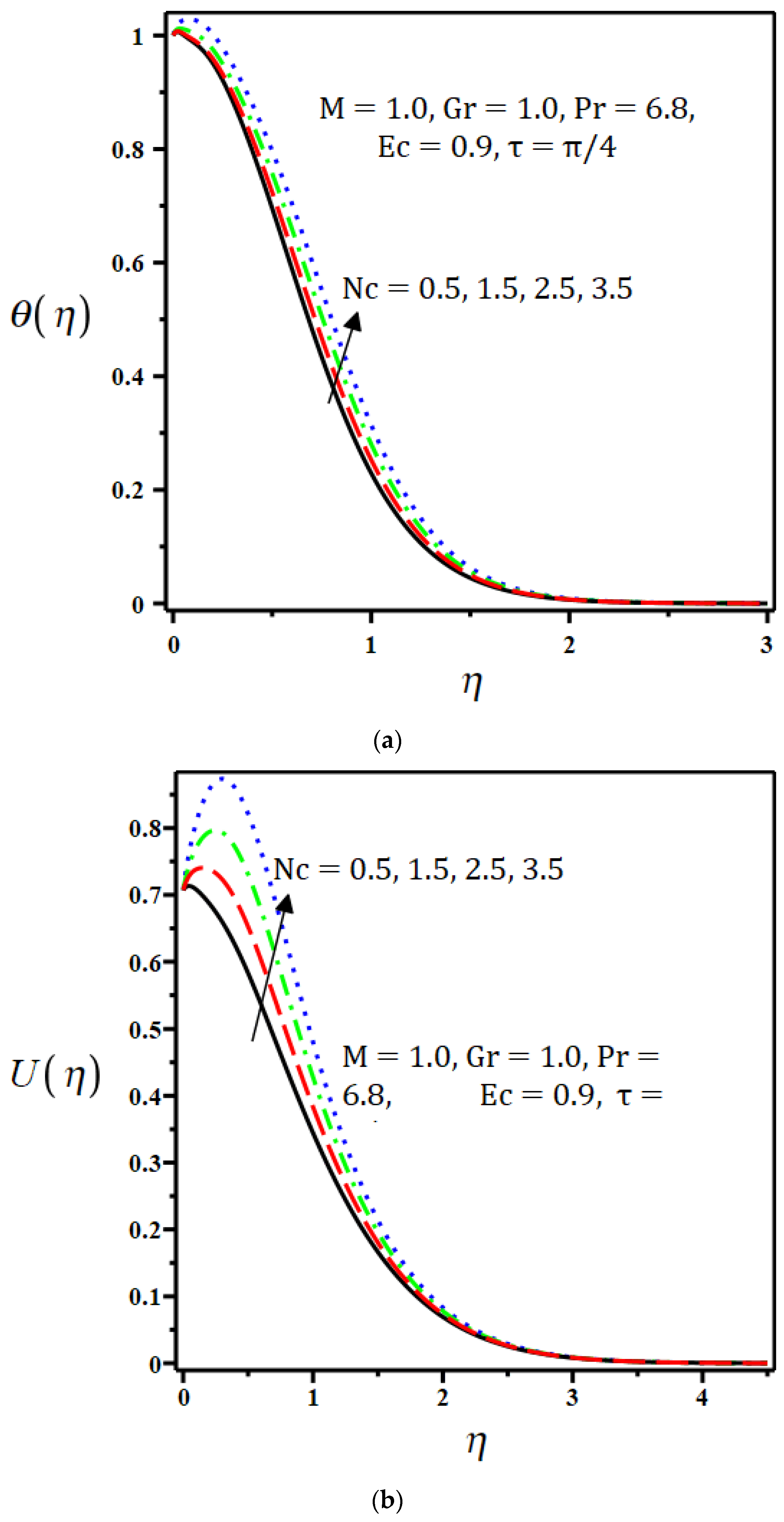
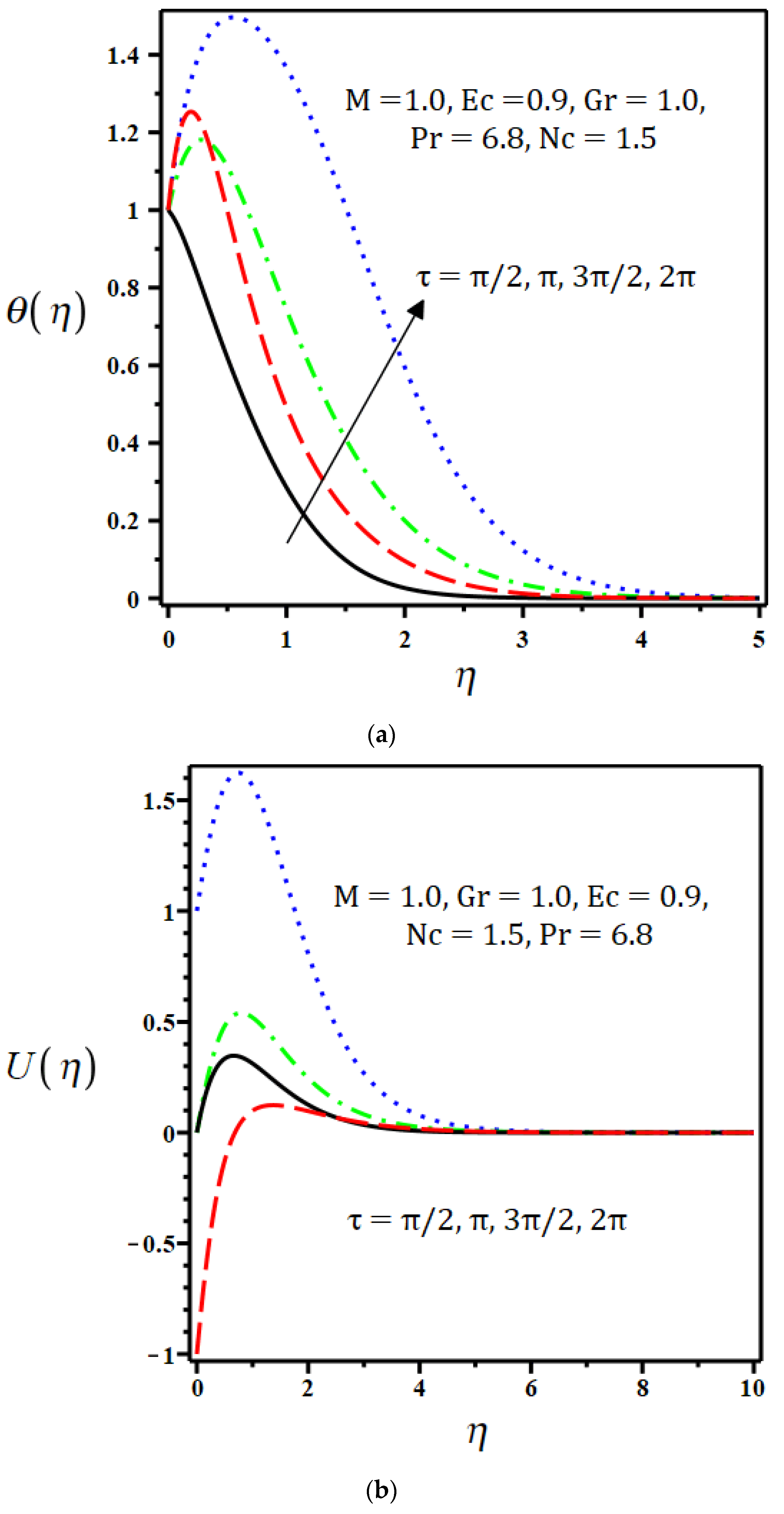
- With preceding time, as the values of Eckert number, magnetic parameter, nonlinear convective flow parameter, and Grashof number increase, the temperature profile shows increasing behavior.
- The temperature field decrements in value as the Prandtl number increases.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ajibade, A.O.; Umar, A.M. Effects of viscous dissipation and wall conduction on steady mixed convection Couette flow of heat generation/absorbing fluid. Int. J. Appl. Mech. Eng. 2019, 24, 12–35. [Google Scholar] [CrossRef]
- Reddy, M.G.; Padmab, P.; Shankarc, B. Effects of viscous dissipation and heat source on unsteady MHD flow over a stretching sheet. Ain Shams Eng. J. 2015, 6, 1195–1201. [Google Scholar] [CrossRef]
- Yusof, Z.M.; Soid, S.K.; Aziz, A.S.A.; Kechil, S.A. Radiation effect on unsteady MHD flow over a stretching surface World. Acad. Sci. Eng. Technol. 2012, 6, 12–27. [Google Scholar]
- Srinivasacharya, D.; Jagadeeshwar, P. Effect of Joule heating on the flow over an exponentially stretching sheet with convective thermal condition. Math. Sci. 2019, 13, 201–211. [Google Scholar] [CrossRef]
- Ganesh Kumar, K.; Ramesh, G.K.; Gireesha, B.J.; Gorlac, R.S.R. Characteristics of Joule heating and viscous dissipation on three-dimensional flow of Oldroyd B nanofluid with thermal radiation. Alex. Eng. J. 2018, 57, 2139–2149. [Google Scholar] [CrossRef]
- Swain, B.K.; Parida, B.C.; Kar, S.; Senapatic, N. Viscous dissipation and joule heating effect on MHD flow and heat transfer past a stretching sheet embedded in a porous medium. Heliyon 2020, 10, e05338. [Google Scholar] [CrossRef]
- Afridi, M.I.; Qasim, M. Entropy generation in three-dimensional flow of dissipative fluid. Int. J. Appl. Comput. Math. 2018, 4, 16. [Google Scholar] [CrossRef]
- Jaber, K.K. Joule Heating and Viscous Dissipation on Effects on MHD Flow over a Stretching Porous Sheet Subjected to Power Law Heat Flux in Presence of Heat Source. Open J. Fluid Dyn. 2016, 6, 156–165. [Google Scholar] [CrossRef]
- Afridi, M.I.; Ashraf, M.U.; Qasim, M.; Wakif, A. Numerical simulation of entropy transport in the oscillating fluid flow with transpiration and internal fluid heating by GGDQM. Waves Random Complex Media 2022. [Google Scholar] [CrossRef]
- Afridi, M.I.; Wakif, A.; Alanazi, A.K.; Chen, Z.M.; Ashraf, M.U.; Qasim, M. A comprehensive entropic scrutiny of dissipative flows over a thin needle featured by variable thermophysical properties. Waves Random Complex Media 2022. [Google Scholar] [CrossRef]
- Lu, D.; Afridi, M.I.; Allauddin, U.; Farooq, U.; Qasim, M. Entropy Generation in a Dissipative Nanofluid Flow under the Influence of Magnetic Dissipation and Transpiration. Energies 2020, 13, 5506. [Google Scholar] [CrossRef]
- Qasim, M.; Afridi, M.I.; Wakif, A.; Thoiand, T.N.; Hussanan, A. Second Law Analysis of Unsteady MHD Viscous Flow over a Horizontal Stretching Sheet Heated Non-Uniformly in the Presence of Ohmic Heating: Utilization of Gear-Generalized Differential Quadrature Method. Entropy 2019, 21, 240. [Google Scholar] [CrossRef]
- Afridi, M.I.; Qasim, M.; Makinde, O.D. Entropy generation due to heat and mass transfer in a flow of dissipative elastic fluid through porous medium. J. Heat Transf. 2019, 141, 022002. [Google Scholar] [CrossRef]
- Veera Krishna, M.; Ameer Ahammad, N.; Chamkha, A.J. Radiative MHD flow of Casson hybrid nanofluid over an infinite exponentially accelerated vertical porous surface. Case Stud. Therm. Eng. 2021, 27, 101229. [Google Scholar] [CrossRef]
- Veera Krishna, M.; Ameer Ahamad, N.; Chamkha, A.J. Radiation absorption on MHD convective flow of nanofluids through vertically travelling absorbent plate. Ain Shams Eng. J. 2021, 12, 3043–3056. [Google Scholar] [CrossRef]
- Alqahtani, A.; Khan, I. Time-dependent MHD flow of non-Newtonian generalized Burger’s fluid (GBF) over a suddenly moved plate with generalized Darcy’s law. Front. Phys. 2020, 7, 214. [Google Scholar] [CrossRef]
- Alamri, S.; Khan, A.; Azeez, M.; Ellahi, R. Effects of mass transfer on MHD second grade fluid towards stretching cylinder: A novel perspective of Cattaneo-Christov heat flux model. Phys. Lett. A 2019, 383, 276–281. [Google Scholar] [CrossRef]
- Stone, J.M. Astrophysical magnetohydrodynamics. Bull. Astron. Soc. India 2011, 39, 129–143. [Google Scholar]
- Jonathan, B. MHD: Astrophysical Contexts. In Essential Fluid Dynamics for Scientists; Morgan & Claypool Publishers: Berlin, Germany, 2017. [Google Scholar]
- Megahed, A.M.; Ghoneim, N.I.; Reddy, M.G.; El-Khatib, M. Magnetohydrodynamic Fluid Flow due to an Unsteady Stretching Sheet with Thermal Radiation, Porous Medium, and Variable Heat Flux. Adv. Astron. 2021, 2021, 6686883. [Google Scholar] [CrossRef]
- Athira, P.R.; Mahanthesh, B.; Gireesha, B.J.; Makinde, O.D. Non-Linear Convection in Chemically Reacting Fluid with an Induced Magnetic Field across a Vertical Porous Plate in the Presence of Heat Source/Sink. In Defect and Diffusion Forum; Trans Tech Publications Ltd.: Zurich, Switzerland, 2018; Volume 387. [Google Scholar]
- Mahanthesh, B.; Gireesha, B.J.; Thammanna, G.T.; Shehzad, S.A.; Abbasi, F.M.; Gorla, R.S.R. Nonlinear convection in nano Maxwell fluid with nonlinear thermal radiation: A three-dimensional study. Alex. Eng. J. 2018, 57, 1927–1935. [Google Scholar] [CrossRef]
- Vasu, B.; RamReddy, C.; Murthy, P.; Gorla, R.S.R. Entropy generation analysis in nonlinear convection flow of thermally stratified fluid in saturated porous medium with convective boundary condition. J. Heat Transf. 2017, 139, 091701. [Google Scholar] [CrossRef]
- Hayat, T.; Qasim, M. Influence of thermal radiation and Joule heating on MHD flow of a Maxwell fluid in the presence of thermophoresis. Int. J. Heat Mass Transf. 2010, 53, 4780–4788. [Google Scholar] [CrossRef]
- Qayyum, T.; Hayat, S.; Shehzad, A.; Alsaedi, A. Nonlinear convective flow of Powell-Erying magneto nanofluid with newtonian heating. Results Phys. 2017, 7, 2933–2940. [Google Scholar] [CrossRef]
- Qayyum, S.; Hayat, T.; Alsaedi, A. Chemical reaction and heat generation/absorption aspects in magnetohydrodynamic nonlinear convective flow of third grade nanofluid over a nonlinear stretching sheet with variable thickness. Results Phys. 2017, 7, 2752–2761. [Google Scholar] [CrossRef]
- Adesanya, S.O.; Ogunseye, H.; Lebelo, R.; Moloi, K.; Adeyemi, O. Second law analysis for nonlinear convective flow of a reactive couple stress fluid through a vertical channel. Heliyon 2018, 4, e00907. [Google Scholar] [CrossRef]
- Hayat, T.; Qayyum, S.; Shehzad, S.A.; Alsaedi, A. Magnetohydrodynamic three-dimensional nonlinear convection flow of Oldroyd-B nanoliquid with heat generation/absorption. J. Mol. Liq. 2017, 230, 641–651. [Google Scholar] [CrossRef]
- Hayat, T.; Qayyum, S.; Alsaedi, A.; Ahmad, B. Nonlinear convective flow with variable thermal conductivity and Cattaneo-Christov heat flux. Neural Comput. Appl. 2019, 31, 295–305. [Google Scholar] [CrossRef]
- Hayat, T.; Qayyum, S.; Alsaedi, A.; Ahmad, B. Magnetohydrodynamic nonlinear convective flow of Walters-B nanofluid over a nonlinear stretching sheet with variable thickness. Int. J. Heat Mass Transf. 2017, 110, 506–514. [Google Scholar] [CrossRef]
- Yusuf, T.A.; Mabood, F.; Gbadeyan, J.A.; Adesanya, S.O. Nonlinear Convective Flow of Magnetohydrodynamic Oldroyd 8-Constant Fluid in a Channel with Chemical Reaction and Convective Boundary Condition. J. Therm. Sci. Eng. Appl. 2020, 12, 051022. [Google Scholar] [CrossRef]
- Ibrahim, W.; Zemedu, C. Non-linear convection flow of micro polar nanofluid past an isothermal sphere. J. Phys. Commun. 2019, 3, 11. [Google Scholar] [CrossRef]
- Saeed, A.; Kumam, P.; Nasir, S.; Gul, T.; Kumam, W. Non-linear convective flow of the thin film nanofluid over an inclined stretching surface. Sci. Rep. 2021, 11, 18410. [Google Scholar] [CrossRef]
- Huang, Y.-H.; Chen, F.; Chung, C.-A. Convective flow generated by lateral heating on a vertically stable solute gradient. Phys. Rev. Fluids 2021, 6, 053502. [Google Scholar] [CrossRef]
- Hussain, A.; Haider, Q.; Rehman, A.; Abdussattar, A.; Y Malik, M. A New Heat Dissipation Model and Convective Two-Phase Nanofluid in Brittle Medium Flow over a Cone. Appl. Math. Stat. Mech. Appl. 2021, 2021, 6688747. [Google Scholar] [CrossRef]
- Ali, Z.; Qasim, M.; Ashraf, M.U. Thermodynamic analysis of nonlinear convection in peristaltic flow. Int. Commun. Heat Mass Transf. 2021, 129, 105686. [Google Scholar] [CrossRef]
- Wakif, A.; Qasim, M.; Afridi, M.I.; Saleem, S.; Al-Qarni, M.M. Numerical Examination of the Entropic Energy Harvesting in a Magnetohydrodynamic Dissipative Flow of Stokes Second Problem: Utilization of the Gear-Generalized Differential Quadrature Method. J. Non-Equilib. Thermodyn. 2019, 44, 385–403. [Google Scholar] [CrossRef]
- Suja, C.; Kar, A.; Cates, W.; Remmert, S.M.; Savage, P.D.; Fuller, G.G. Evaporation-induced foam stabilization in lubricating oils. Proc. Natl. Acad. Sci. USA 2018, 115, 7919–7924. [Google Scholar] [CrossRef]
- Velev, O.D.; Gurkov, T.D.; Borwankar, R.P. Spontaneous cyclic dimpling in emulsion films due to surfactant mass transfer between the phases. J. Colloid Interface Sci. 1993, 159, 497–501. [Google Scholar] [CrossRef]
- Ishfaq, N.; Khan, W.A.; Khan, Z.H. The Stokes’ second problem for nanofluids. J. King Saud Univ.—Sci. 2019, 31, 61–65. [Google Scholar] [CrossRef]
- Uddin, M.; Murad, A. Stokes’ Second Problem and Oscillatory Couette Flow for a Two-Layer Fluid: Analytical Solutions. Alex. Eng. J. 2022, 61, 10197–10218. [Google Scholar] [CrossRef]
- Roy, N.C.; Pop, I. Exact solutions of Stokes’ second problem for hybrid nanofluid flow with a heat source. Phys. Fluids 2021, 33, 063603. [Google Scholar] [CrossRef]
- Hussanan, A.; Anwar, M.I.; Ali, F.; Khan, I.; Shafie, S. Natural convection flow past an oscillating plate with Newtonian heating. Heat Transf. Res. 2014, 45, 119–135. [Google Scholar] [CrossRef]
- Khan, I.; Abro, K.A.; Mirbhar, M.N.; Tlili, I. Thermal analysis in Stokes’ second problem of nanofluid: Applications in thermal engineering. Case Stud. Therm. Eng. 2018, 12, 271–275. [Google Scholar] [CrossRef]
- Khan, I.; Ali, F.; Shafie, S. Stokes’ second problem for magnetohydrodynamics flow in a Burgers’ fluid: The cases γ = λ2/4 and γ > λ2/4. PLoS ONE 2013, 8, e61531. [Google Scholar] [CrossRef] [Green Version]
- Hoffmann, K.A.; Chiang, S.T. Computational Fluid Dynamics; Engineering Edition System: Wichita, KS, USA, 2000. [Google Scholar]
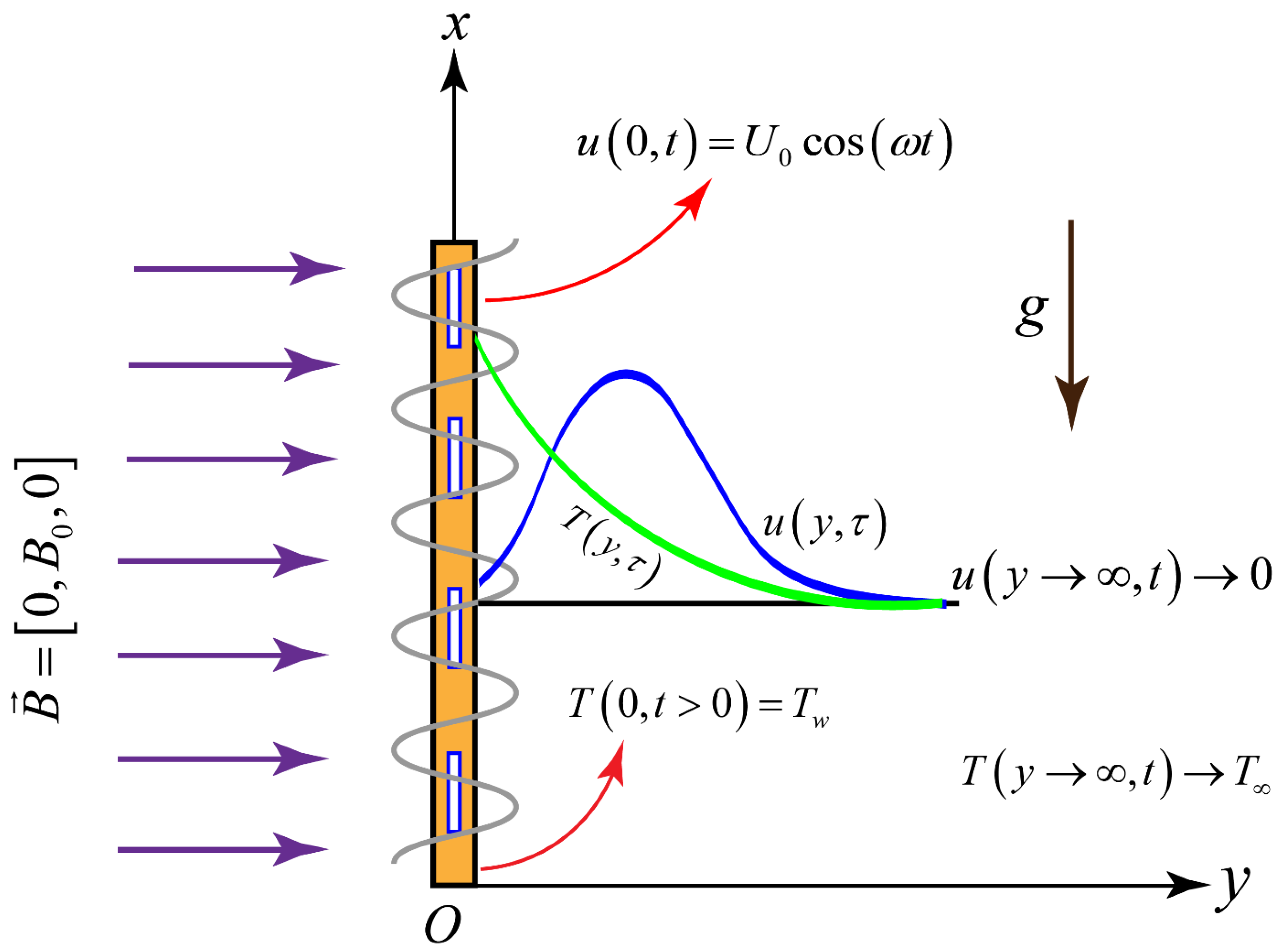
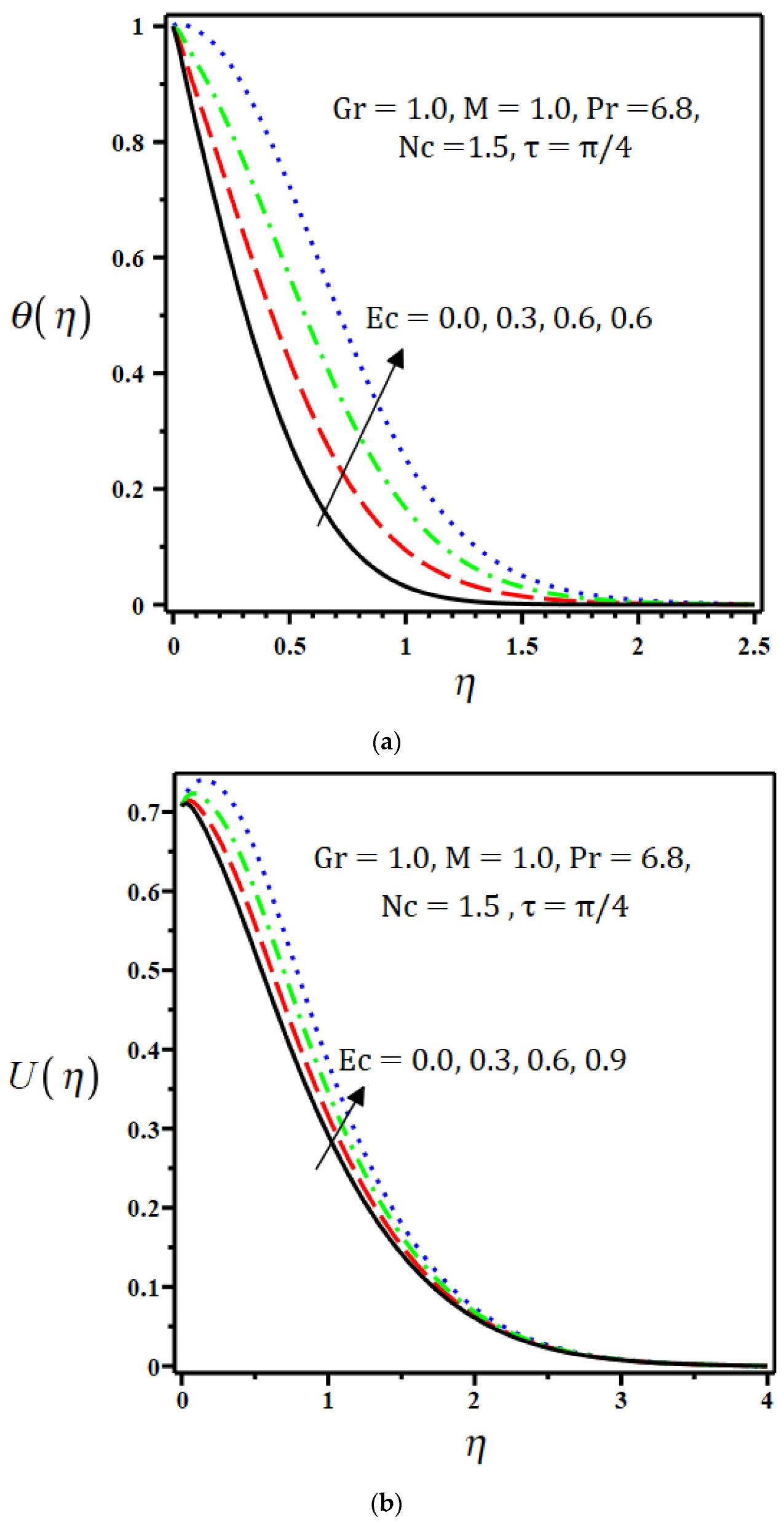
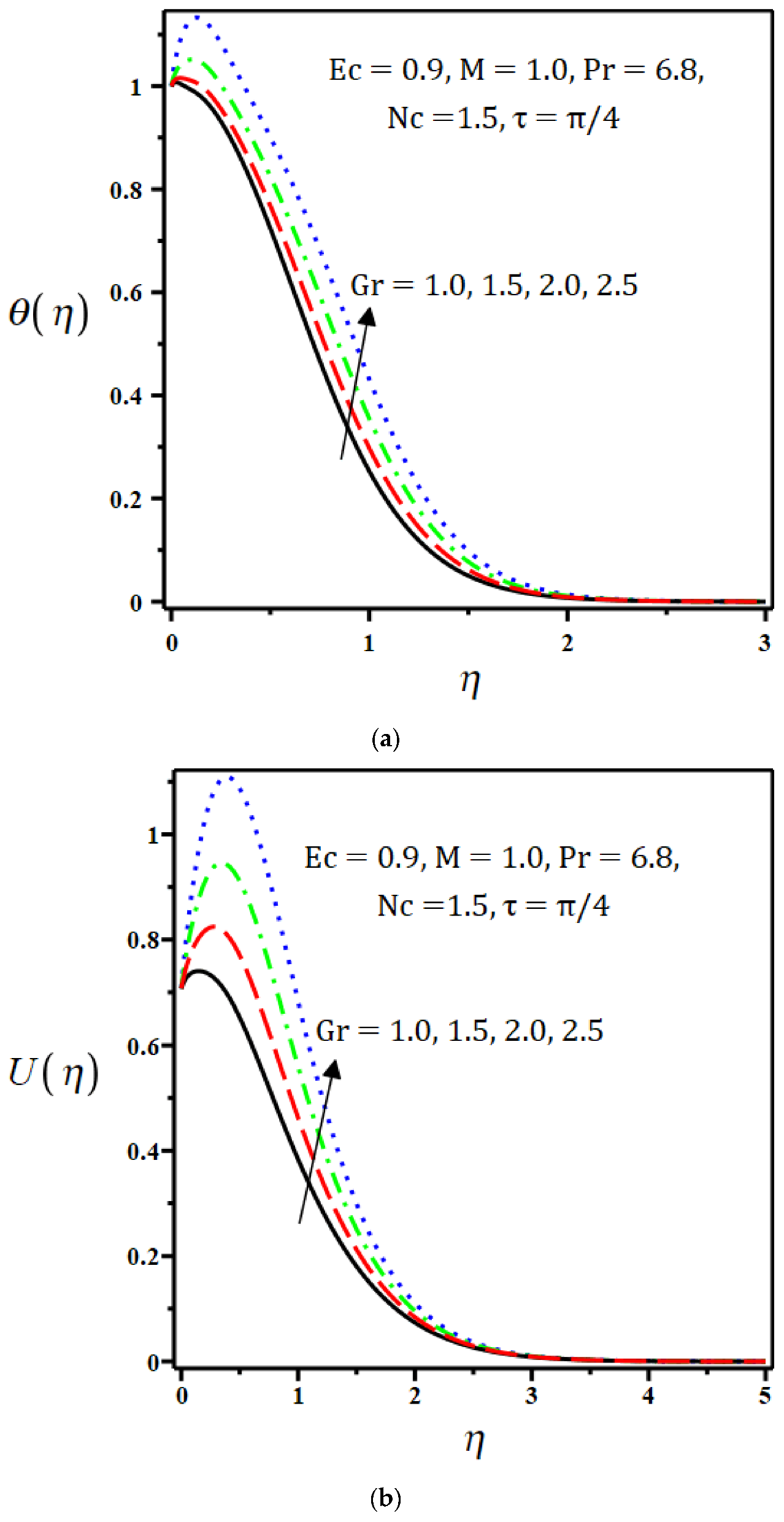
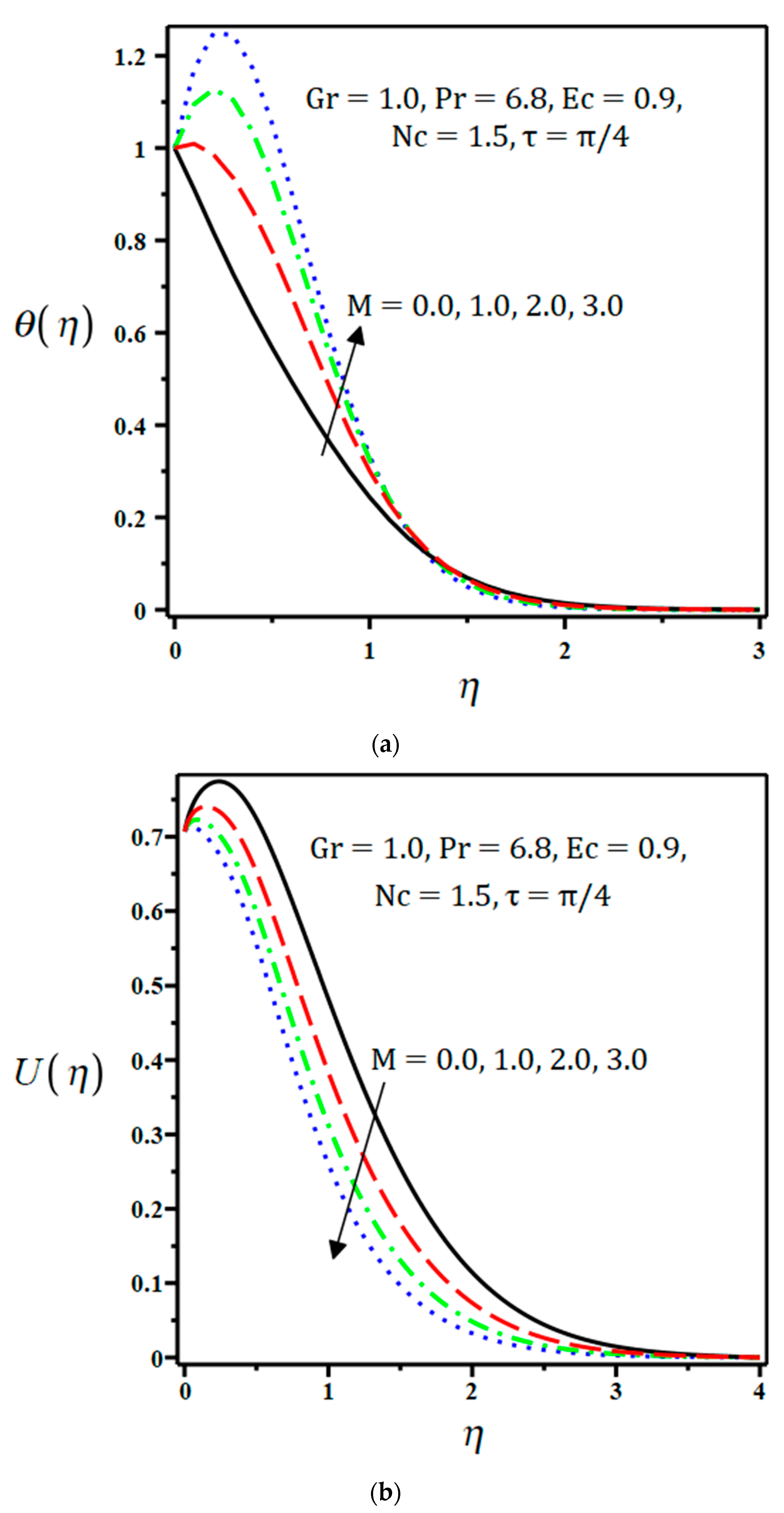

| τ | Cf | |
|---|---|---|
| Exact Solution | FDM | |
| 0.1 | −0.6329813065 | −0.6329813064 |
| 0.2 | −0.5525312920 | −0.5525312920 |
| 0.3 | −0.4665605675 | −0.4665605676 |
| 0.4 | −0.3759281240 | −0.3759281240 |
| 0.5 | −0.2815395311 | −0.2815395311 |
| 0.6 | −0.1843378880 | −0.1843378882 |
| 0.7 | −0.08529440190 | −0.08529440189 |
| 0.8 | 0.01460131775 | 0.01460131773 |
| 0.9 | 0.1143511457 | 0.1143511455 |
| 1.0 | 0.2129584152 | 0.2129584151 |
| τ | Cf | Nu | ||
|---|---|---|---|---|
| * GGDQM [37] | ** FDM | GGDQM [37] | FDM | |
| 0.1 | −1.77812702 | −1.77812702 | 4.72034872 | 4.72034871 |
| 0.2 | −1.21946930 | −1.21946929 | 3.33779059 | 3.33779061 |
| 0.3 | −0.93773608 | −0.93773608 | 2.72529460 | 2.72529460 |
| 0.4 | −0.73915669 | −0.73915670 | 2.36017436 | 2.36017438 |
| τ | Cf | Nu | ||
|---|---|---|---|---|
| GGDQM [37] | FDM | GGDQM [37] | FDM | |
| 0.1 | −1.67115676 | −1.67115677 | 1.78412411 | 1.78412412 |
| 0.2 | −1.06843318 | −1.06843319 | 1.26156626 | 1.26156625 |
| 0.3 | −0.75321389 | −0.75321390 | 1.03006454 | 1.03006456 |
| 0.4 | −0.52680054 | −0.52680051 | 0.89206205 | 0.89206205 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khan, M.S.; Siddiqui, M.A.; Afridi, M.I. Finite Difference Simulation of Nonlinear Convection in Magnetohydrodynamic Flow in the Presence of Viscous and Joule Dissipation over an Oscillating Plate. Symmetry 2022, 14, 1988. https://doi.org/10.3390/sym14101988
Khan MS, Siddiqui MA, Afridi MI. Finite Difference Simulation of Nonlinear Convection in Magnetohydrodynamic Flow in the Presence of Viscous and Joule Dissipation over an Oscillating Plate. Symmetry. 2022; 14(10):1988. https://doi.org/10.3390/sym14101988
Chicago/Turabian StyleKhan, Muhammad Samad, Mubashir Ali Siddiqui, and Muhammad Idrees Afridi. 2022. "Finite Difference Simulation of Nonlinear Convection in Magnetohydrodynamic Flow in the Presence of Viscous and Joule Dissipation over an Oscillating Plate" Symmetry 14, no. 10: 1988. https://doi.org/10.3390/sym14101988
APA StyleKhan, M. S., Siddiqui, M. A., & Afridi, M. I. (2022). Finite Difference Simulation of Nonlinear Convection in Magnetohydrodynamic Flow in the Presence of Viscous and Joule Dissipation over an Oscillating Plate. Symmetry, 14(10), 1988. https://doi.org/10.3390/sym14101988







