Mathematical Models of Blood-Brain Barrier Transport of Monoclonal Antibodies Targeting the Transferrin Receptor and the Insulin Receptor
Abstract
1. Introduction
2. Results
2.1. Transferrin Receptor (TfR) Model
2.1.1. TfR Model Input Parameters
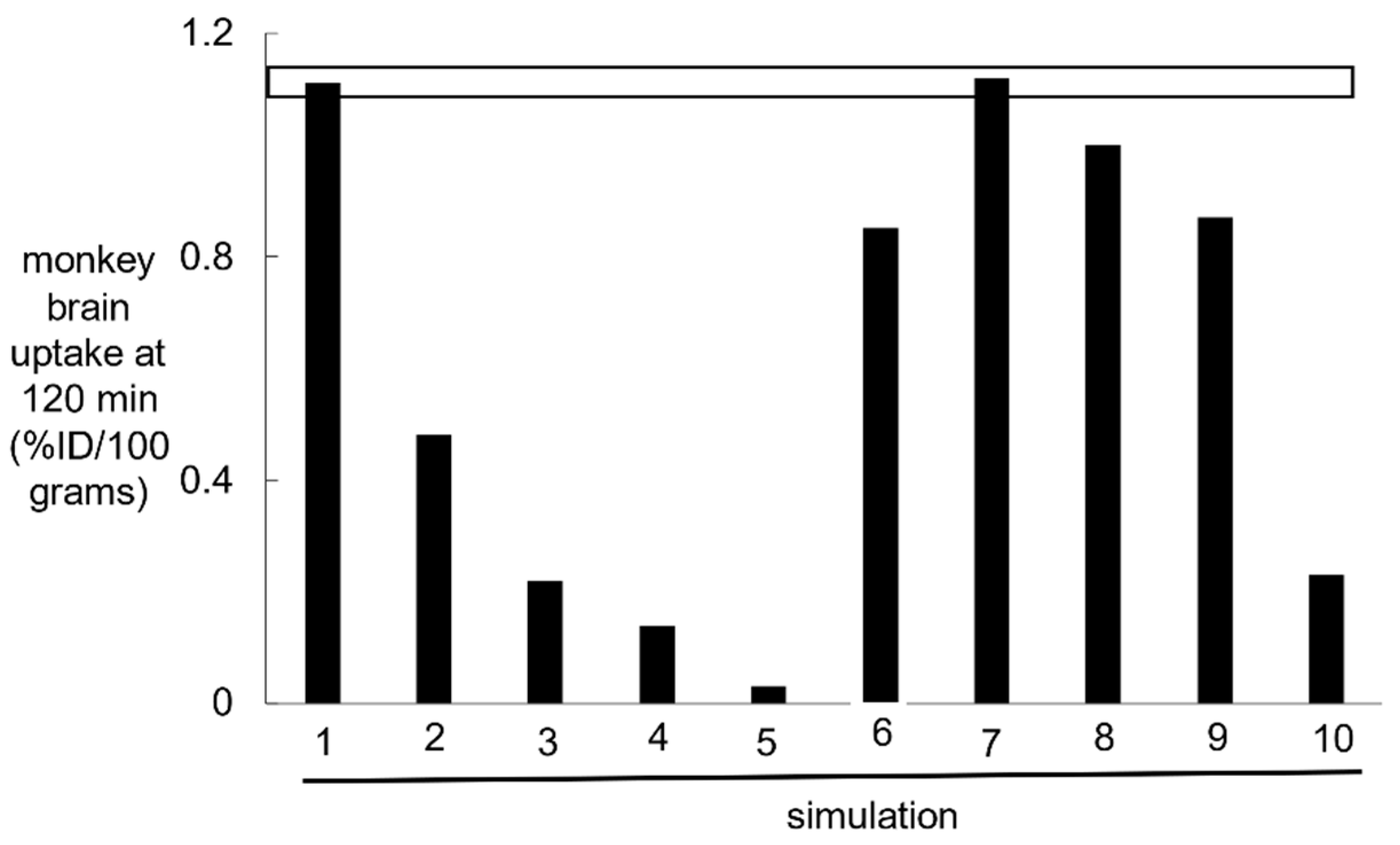
2.1.2. TfR Model Simulations of TfRMAb Uptake by Brain in the Rhesus Monkey
2.1.3. TfR Model Simulations of the Brain Uptake of a TfRMAb with a High, Moderate, and Low Affinity for the TfR and with Different Association Rate Constants
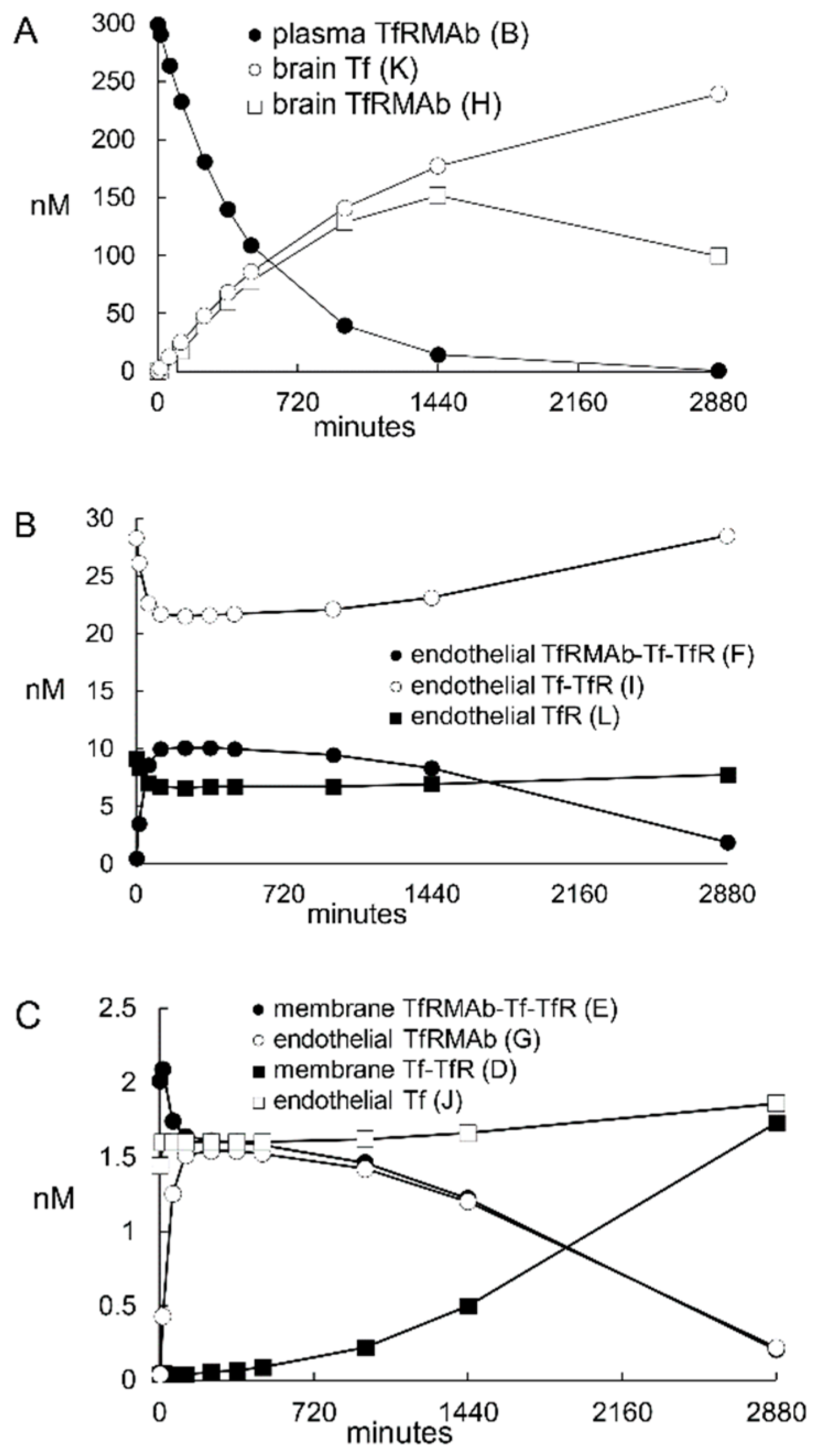
2.1.4. TfR Model Simulations of the Time Course of All Model Variables
2.2. Insulin Receptor (IR) Model
2.2.1. IR Model Input Parameters
2.2.2. IR Model Simulations of HIRMAb-IDUA Uptake by Brain in the Rhesus Monkey
2.2.3. IR Model Simulations of the Time Course of All Model Variables
3. Discussion
4. Methods
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
References
- Pardridge, W.M.; Boado, R.J. Reengineering biopharmaceuticals for targeted delivery across the blood-brain barrier. Methods Enzymol. 2012, 503, 269–292. [Google Scholar] [CrossRef]
- Giugliani, R.; Giugliani, L.; de Oliveira Poswar, F.; Donis, K.C.; Corte, A.D.; Schmidt, M.; Boado, R.J.; Nestrasil, I.; Nguyen, C.; Chen, S.; et al. Neurocognitive and somatic stabilization in pediatric patients with severe Mucopolysaccharidosis Type I after 52 weeks of intravenous brain-penetrating insulin receptor antibody-iduronidase fusion protein (valanafusp alpha): An open label phase 1–2 trial. Orphanet J. Rare Dis. 2018, 13, 110. [Google Scholar] [CrossRef]
- Okuyama, T.; Eto, Y.; Sakai, N.; Nakamura, K.; Yamamoto, T.; Yamaoka, M.; Ikeda, T.; So, S.; Tanizawa, K.; Sonoda, H.; et al. A Phase 2/3 Trial of Pabinafusp Alfa, IDS Fused with Anti-Human Transferrin Receptor Antibody, Targeting Neurodegeneration in MPS-II. Mol. Ther. 2021, 29, 671–679. [Google Scholar] [CrossRef]
- Ullman, J.C.; Arguello, A.; Getz, J.A.; Bhalla, A.; Mahon, C.S.; Wang, J.; Giese, T.; Bedard, C.; Kim, D.J.; Blumenfeld, J.R.; et al. Brain delivery and activity of a lysosomal enzyme using a blood-brain barrier transport vehicle in mice. Sci. Transl. Med. 2020, 12. [Google Scholar] [CrossRef] [PubMed]
- Boado, R.J.; Zhang, Y.; Zhang, Y.; Xia, C.F.; Pardridge, W.M. Fusion antibody for Alzheimer’s disease with bidirectional transport across the blood-brain barrier and abeta fibril disaggregation. Bioconjug. Chem. 2007, 18, 447–455. [Google Scholar] [CrossRef] [PubMed]
- Yu, Y.J.; Zhang, Y.; Kenrick, M.; Hoyte, K.; Luk, W.; Lu, Y.; Atwal, J.; Elliott, J.M.; Prabhu, S.; Watts, R.J.; et al. Boosting brain uptake of a therapeutic antibody by reducing its affinity for a transcytosis target. Sci. Transl. Med. 2011, 3, 84ra44. [Google Scholar] [CrossRef] [PubMed]
- Yu, Y.J.; Atwal, J.K.; Zhang, Y.; Tong, R.K.; Wildsmith, K.R.; Tan, C.; Bien-Ly, N.; Hersom, M.; Maloney, J.A.; Meilandt, W.J.; et al. Therapeutic bispecific antibodies cross the blood-brain barrier in nonhuman primates. Sci. Transl. Med. 2014, 6, 261ra154. [Google Scholar] [CrossRef]
- Niewoehner, J.; Bohrmann, B.; Collin, L.; Urich, E.; Sade, H.; Maier, P.; Rueger, P.; Stracke, J.O.; Lau, W.; Tissot, A.C.; et al. Increased brain penetration and potency of a therapeutic antibody using a monovalent molecular shuttle. Neuron 2014, 81, 49–60. [Google Scholar] [CrossRef] [PubMed]
- Webster, C.I.; Caram-Salas, N.; Haqqani, A.S.; Thom, G.; Brown, L.; Rennie, K.; Yogi, A.; Costain, W.; Brunette, E.; Stanimirovic, D.B. Brain penetration, target engagement, and disposition of the blood-brain barrier-crossing bispecific antibody antagonist of metabotropic glutamate receptor type 1. FASEB J. 2016, 30, 1927–1940. [Google Scholar] [CrossRef]
- Karaoglu Hanzatian, D.; Schwartz, A.; Gizatullin, F.; Erickson, J.; Deng, K.; Villanueva, R.; Stedman, C.; Harris, C.; Ghayur, T.; Goodearl, A. Brain uptake of multivalent and multi-specific DVD-Ig proteins after systemic administration. mAbs 2018, 10, 765–777. [Google Scholar] [CrossRef]
- Kariolis, M.S.; Wells, R.C.; Getz, J.A.; Kwan, W.; Mahon, C.S.; Tong, R.; Kim, D.J.; Srivastava, A.; Bedard, C.; Henne, K.R.; et al. Brain delivery of therapeutic proteins using an Fc fragment blood-brain barrier transport vehicle in mice and monkeys. Sci. Transl. Med. 2020, 12. [Google Scholar] [CrossRef]
- Do, T.M.; Capdevila, C.; Pradier, L.; Blanchard, V.; Lopez-Grancha, M.; Schussler, N.; Steinmetz, A.; Beninga, J.; Boulay, D.; Dugay, P.; et al. Tetravalent Bispecific Tandem Antibodies Improve Brain Exposure and Efficacy in an Amyloid Transgenic Mouse Model. Mol. Ther. Methods Clin. Dev. 2020, 19, 58–77. [Google Scholar] [CrossRef] [PubMed]
- Hultqvist, G.; Syvanen, S.; Fang, X.T.; Lannfelt, L.; Sehlin, D. Bivalent Brain Shuttle Increases Antibody Uptake by Monovalent Binding to the Transferrin Receptor. Theranostics 2017, 7, 308–318. [Google Scholar] [CrossRef] [PubMed]
- Meier, S.R.; Sehlin, D.; Hultqvist, G.; Syvanen, S. Pinpointing Brain TREM2 Levels in Two Mouse Models of Alzheimer’s Disease. Mol. Imaging Biol. 2021. [Google Scholar] [CrossRef] [PubMed]
- Pardridge, W.M.; Boado, R.J.; Patrick, D.J.; Ka-Wai Hui, E.; Lu, J.Z. Blood-Brain Barrier Transport, Plasma Pharmacokinetics, and Neuropathology Following Chronic Treatment of the Rhesus Monkey with a Brain Penetrating Humanized Monoclonal Antibody Against the Human Transferrin Receptor. Mol. Pharm. 2018, 15, 5207–5216. [Google Scholar] [CrossRef] [PubMed]
- Boado, R.J.; Pardridge, W.M. Brain and Organ Uptake in the Rhesus Monkey in vivo of Recombinant Iduronidase Compared to an Insulin Receptor Antibody-Iduronidase Fusion Protein. Mol. Pharm. 2017, 14, 1271–1277. [Google Scholar] [CrossRef]
- Schmaier, A.H. Transferrin: A blood coagulation modifier. Cell Res. 2020, 30, 101–102. [Google Scholar] [CrossRef]
- Bar, R.S.; Gorden, P.; Roth, J.; Kahn, C.R.; De Meyts, P. Fluctuations in the affinity and concentration of insulin receptors on circulating monocytes of obese patients: Effects of starvation, refeeding, and dieting. J. Clin. Investig. 1976, 58, 1123–1135. [Google Scholar] [CrossRef] [PubMed]
- Bremer, A.A.; Stanhope, K.L.; Graham, J.L.; Cummings, B.P.; Wang, W.; Saville, B.R.; Havel, P.J. Fructose-fed rhesus monkeys: A nonhuman primate model of insulin resistance, metabolic syndrome, and type 2 diabetes. Clin. Transl. Sci. 2011, 4, 243–252. [Google Scholar] [CrossRef] [PubMed]
- Hinke, S.A.; Cieniewicz, A.M.; Kirchner, T.; D’Aquino, K.; Nanjunda, R.; Aligo, J.; Perkinson, R.; Cooper, P.; Boayke, K.; Chiu, M.L.; et al. Unique pharmacology of a novel allosteric agonist/sensitizer insulin receptor monoclonal antibody. Mol. Metab. 2018, 10, 87–99. [Google Scholar] [CrossRef]
- Mandikian, D.; Figueroa, I.; Oldendorp, A.; Rafidi, H.; Ulufatu, S.; Schweiger, M.G.; Couch, J.A.; Dybdal, N.; Joseph, S.B.; Prabhu, S.; et al. Tissue Physiology of Cynomolgus Monkeys: Cross-Species Comparison and Implications for Translational Pharmacology. AAPS J. 2018, 20, 107. [Google Scholar] [CrossRef]
- Noda, A.; Ohba, H.; Kakiuchi, T.; Futatsubashi, M.; Tsukada, H.; Nishimura, S. Age-related changes in cerebral blood flow and glucose metabolism in conscious rhesus monkeys. Brain Res. 2002, 936, 76–81. [Google Scholar] [CrossRef]
- Giannetti, A.M.; Bjorkman, P.J. HFE and transferrin directly compete for transferrin receptor in solution and at the cell surface. J. Biol. Chem. 2004, 279, 25866–25875. [Google Scholar] [CrossRef]
- Eckenroth, B.E.; Steere, A.N.; Chasteen, N.D.; Everse, S.J.; Mason, A.B. How the binding of human transferrin primes the transferrin receptor potentiating iron release at endosomal pH. Proc. Natl. Acad. Sci. USA 2011, 108, 13089–13094. [Google Scholar] [CrossRef]
- Mason, A.B.; Byrne, S.L.; Everse, S.J.; Roberts, S.E.; Chasteen, N.D.; Smith, V.C.; MacGillivray, R.T.; Kandemir, B.; Bou-Abdallah, F. A loop in the N-lobe of human serum transferrin is critical for binding to the transferrin receptor as revealed by mutagenesis, isothermal titration calorimetry, and epitope mapping. J. Mol. Recognit. 2009, 22, 521–529. [Google Scholar] [CrossRef] [PubMed]
- Hulme, E.C.; Trevethick, M.A. Ligand binding assays at equilibrium: Validation and interpretation. Br. J. Pharmacol. 2010, 161, 1219–1237. [Google Scholar] [CrossRef]
- Christensen, S.C.; Krogh, B.O.; Jensen, A.; Andersen, C.B.F.; Christensen, S.; Nielsen, M.S. Characterization of basigin monoclonal antibodies for receptor-mediated drug delivery to the brain. Sci. Rep. 2020, 10, 14582. [Google Scholar] [CrossRef]
- Ciechanover, A.; Schwartz, A.L.; Dautry-Varsat, A.; Lodish, H.F. Kinetics of internalization and recycling of transferrin and the transferrin receptor in a human hepatoma cell line. Effect of lysosomotropic agents. J. Biol. Chem. 1983, 258, 9681–9689. [Google Scholar] [CrossRef]
- McGraw, T.E.; Maxfield, F.R. Human transferrin receptor internalization is partially dependent upon an aromatic amino acid on the cytoplasmic domain. Cell Regul. 1990, 1, 369–377. [Google Scholar] [CrossRef]
- Mayle, K.M.; Le, A.M.; Kamei, D.T. The intracellular trafficking pathway of transferrin. Biochim. Biophys. Acta 2012, 1820, 264–281. [Google Scholar] [CrossRef]
- Skarlatos, S.; Yoshikawa, T.; Pardridge, W.M. Transport of [125I] transferrin through the rat blood-brain barrier. Brain Res. 1995, 683, 164–171. [Google Scholar] [CrossRef]
- Gruszczyk, J.; Kanjee, U.; Chan, L.J.; Menant, S.; Malleret, B.; Lim, N.T.Y.; Schmidt, C.Q.; Mok, Y.F.; Lin, K.M.; Pearson, R.D.; et al. Transferrin receptor 1 is a reticulocyte-specific receptor for Plasmodium vivax. Science 2018, 359, 48–55. [Google Scholar] [CrossRef]
- Castellanos, D.M.; Sun, J.; Yang, J.; Ou, W.; Zambon, A.C.; Pardridge, W.M.; Sumbria, R.K. Acute and Chronic Dosing of a High-Affinity Rat/Mouse Chimeric Transferrin Receptor Antibody in Mice. Pharmaceutics 2020, 12, 852. [Google Scholar] [CrossRef]
- Uchida, Y.; Ohtsuki, S.; Katsukura, Y.; Ikeda, C.; Suzuki, T.; Kamiie, J.; Terasaki, T. Quantitative targeted absolute proteomics of human blood-brain barrier transporters and receptors. J. Neurochem. 2011, 117, 333–345. [Google Scholar] [CrossRef] [PubMed]
- Ito, K.; Uchida, Y.; Ohtsuki, S.; Aizawa, S.; Kawakami, H.; Katsukura, Y.; Kamiie, J.; Terasaki, T. Quantitative membrane protein expression at the blood-brain barrier of adult and younger cynomolgus monkeys. J. Pharm. Sci. 2011, 100, 3939–3950. [Google Scholar] [CrossRef] [PubMed]
- Uchida, Y.; Yagi, Y.; Takao, M.; Tano, M.; Umetsu, M.; Hirano, S.; Usui, T.; Tachikawa, M.; Terasaki, T. Comparison of Absolute Protein Abundances of Transporters and Receptors among Blood-Brain Barriers at Different Cerebral Regions and the Blood-Spinal Cord Barrier in Humans and Rats. Mol. Pharm. 2020, 17, 2006–2020. [Google Scholar] [CrossRef]
- Kleven, M.D.; Jue, S.; Enns, C.A. Transferrin Receptors TfR1 and TfR2 Bind Transferrin through Differing Mechanisms. Biochemistry 2018, 57, 1552–1559. [Google Scholar] [CrossRef] [PubMed]
- Gjedde, A.; Christensen, O. Estimates of Michaelis-Menten constants for the two membranes of the brain endothelium. J. Cereb. Blood Flow Metab. 1984, 4, 241–249. [Google Scholar] [CrossRef] [PubMed]
- Sykova, E.; Nicholson, C. Diffusion in brain extracellular space. Physiol. Rev. 2008, 88, 1277–1340. [Google Scholar] [CrossRef]
- Boado, R.J.; Zhang, Y.; Wang, Y.; Pardridge, W.M. Engineering and expression of a chimeric transferrin receptor monoclonal antibody for blood-brain barrier delivery in the mouse. Biotechnol. Bioeng. 2009, 102, 1251–1258. [Google Scholar] [CrossRef]
- Sonoda, H.; Morimoto, H.; Yoden, E.; Koshimura, Y.; Kinoshita, M.; Golovina, G.; Takagi, H.; Yamamoto, R.; Minami, K.; Mizoguchi, A.; et al. A Blood-Brain-Barrier-Penetrating Anti-human Transferrin Receptor Antibody Fusion Protein for Neuronopathic Mucopolysaccharidosis II. Mol. Ther. 2018, 26, 1366–1374. [Google Scholar] [CrossRef] [PubMed]
- Stocki, P.; Szary, J.; Rasmussen, C.L.M.; Demydchuk, M.; Northall, L.; Logan, D.B.; Gauhar, A.; Thei, L.; Moos, T.; Walsh, F.S.; et al. Blood-brain barrier transport using a high affinity, brain-selective VNAR antibody targeting transferrin receptor 1. FASEB J. 2021, 35, e21172. [Google Scholar] [CrossRef]
- Couch, J.A.; Yu, Y.J.; Zhang, Y.; Tarrant, J.M.; Fuji, R.N.; Meilandt, W.J.; Solanoy, H.; Tong, R.K.; Hoyte, K.; Luk, W.; et al. Addressing safety liabilities of TfR bispecific antibodies that cross the blood-brain barrier. Sci. Transl. Med. 2013, 5, 183ra157. [Google Scholar] [CrossRef] [PubMed]
- Gustavsson, T.; Syvanen, S.; O’Callaghan, P.; Sehlin, D. SPECT imaging of distribution and retention of a brain-penetrating bispecific amyloid-beta antibody in a mouse model of Alzheimer’s disease. Transl. Neurodegener. 2020, 9, 37. [Google Scholar] [CrossRef]
- Dodd, P.R.; Eckert, A.L.; Fletcher, L.M.; Kril, J.J.; Harper, C.G.; Halliday, J.W. Concentrations of transferrin and carbohydrate-deficient transferrin in postmortem human brain from alcoholics. Addict. Biol. 1997, 2, 337–348. [Google Scholar] [CrossRef]
- Strahan, M.E.; Crowe, A.; Morgan, E.H. Iron uptake in relation to transferrin degradation in brain and other tissues of rats. Am. J. Physiol. 1992, 263, R924–R929. [Google Scholar] [CrossRef]
- Boado, R.J.; Hui, E.K.; Lu, J.Z.; Pardridge, W.M. AGT-181: Expression in CHO cells and pharmacokinetics, safety, and plasma iduronidase enzyme activity in Rhesus monkeys. J. Biotechnol. 2009, 144, 135–141. [Google Scholar] [CrossRef]
- Boado, R.J.; Zhang, Y.; Zhang, Y.; Xia, C.F.; Wang, Y.; Pardridge, W.M. Genetic engineering of a lysosomal enzyme fusion protein for targeted delivery across the human blood-brain barrier. Biotechnol. Bioeng. 2008, 99, 475–484. [Google Scholar] [CrossRef] [PubMed]
- Pardridge, W.M.; Eisenberg, J.; Yang, J. Human blood-brain barrier insulin receptor. J. Neurochem. 1985, 44, 1771–1778. [Google Scholar] [CrossRef] [PubMed]
- Gambhir, K.K.; Archer, J.A.; Bradley, C.J. Characteristics of human erythrocyte insulin receptors. Diabetes 1978, 27, 701–708. [Google Scholar] [CrossRef] [PubMed]
- Wu, D.; Yang, J.; Pardridge, W.M. Drug targeting of a peptide radiopharmaceutical through the primate blood-brain barrier in vivo with a monoclonal antibody to the human insulin receptor. J. Clin. Investig. 1997, 100, 1804–1812. [Google Scholar] [CrossRef]
- Lamb, J.E.; Ray, F.; Ward, J.H.; Kushner, J.P.; Kaplan, J. Internalization and subcellular localization of transferrin and transferrin receptors in HeLa cells. J. Biol. Chem. 1983, 258, 8751–8758. [Google Scholar] [CrossRef]
- Pardridge, W.M.; Van Herle, A.J.; Naruse, R.T.; Fierer, G.; Costin, A. In vivo quantification of receptor-mediated uptake of asialoglycoproteins by rat liver. J. Biol. Chem. 1983, 258, 990–994. [Google Scholar] [CrossRef]
- Cornford, E.M.; Hyman, S.; Cornford, M.E.; Landaw, E.M.; Delgado-Escueta, A.V. Interictal seizure resections show two configurations of endothelial Glut1 glucose transporter in the human blood-brain barrier. J. Cereb. Blood Flow Metab. 1998, 18, 26–42. [Google Scholar] [CrossRef] [PubMed]
- Liddelow, S.A.; Dziegielewska, K.M.; Vandeberg, J.L.; Saunders, N.R. Development of the lateral ventricular choroid plexus in a marsupial, Monodelphis domestica. Cerebrospinal Fluid Res. 2010, 7, 16. [Google Scholar] [CrossRef]
- Sato, H.; Terasaki, T.; Mizuguchi, H.; Okumura, K.; Tsuji, A. Receptor-recycling model of clearance and distribution of insulin in the perfused mouse liver. Diabetologia 1991, 34, 613–621. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Pardridge, W.M.; Kang, Y.S.; Buciak, J.L.; Yang, J. Human insulin receptor monoclonal antibody undergoes high affinity binding to human brain capillaries in vitro and rapid transcytosis through the blood-brain barrier in vivo in the primate. Pharm. Res. 1995, 12, 807–816. [Google Scholar] [CrossRef] [PubMed]
- Boado, R.J.; Lu, J.Z.; Hui, E.K.; Sumbria, R.K.; Pardridge, W.M. Pharmacokinetics and brain uptake in the rhesus monkey of a fusion protein of arylsulfatase a and a monoclonal antibody against the human insulin receptor. Biotechnol. Bioeng. 2013, 110, 1456–1465. [Google Scholar] [CrossRef]
- Yang, D.; Singh, A.; Wu, H.; Kroe-Barrett, R. Comparison of biosensor platforms in the evaluation of high affinity antibody-antigen binding kinetics. Anal. Biochem. 2016, 508, 78–96. [Google Scholar] [CrossRef] [PubMed]
- Thom, G.; Burrell, M.; Haqqani, A.S.; Yogi, A.; Lessard, E.; Brunette, E.; Delaney, C.; Baumann, E.; Callaghan, D.; Rodrigo, N.; et al. Enhanced Delivery of Galanin Conjugates to the Brain through Bioengineering of the Anti-Transferrin Receptor Antibody OX26. Mol. Pharm. 2018, 15, 1420–1431. [Google Scholar] [CrossRef] [PubMed]
- Steckbeck, J.D.; Orlov, I.; Chow, A.; Grieser, H.; Miller, K.; Bruno, J.; Robinson, J.E.; Montelaro, R.C.; Cole, K.S. Kinetic rates of antibody binding correlate with neutralization sensitivity of variant simian immunodeficiency virus strains. J. Virol. 2005, 79, 12311–12320. [Google Scholar] [CrossRef] [PubMed]
- Encarnacao, J.C.; Barta, P.; Fornstedt, T.; Andersson, K. Impact of assay temperature on antibody binding characteristics in living cells: A case study. Biomed. Rep. 2017, 7, 400–406. [Google Scholar] [CrossRef] [PubMed]
- Hadzhieva, M.; Pashov, A.D.; Kaveri, S.; Lacroix-Desmazes, S.; Mouquet, H.; Dimitrov, J.D. Impact of Antigen Density on the Binding Mechanism of IgG Antibodies. Sci. Rep. 2017, 7, 3767. [Google Scholar] [CrossRef] [PubMed]
- Suzuki, A.; Yamasaki, T.; Hasebe, R.; Horiuchi, M. Enhancement of binding avidity by bivalent binding enables PrPSc-specific detection by anti-PrP monoclonal antibody 132. PLoS ONE 2019, 14, e0217944. [Google Scholar] [CrossRef]
- Pardridge, W.M. CSF, blood-brain barrier, and brain drug delivery. Expert Opin. Drug Deliv. 2016, 13, 963–975. [Google Scholar] [CrossRef] [PubMed]
- Bien-Ly, N.; Yu, Y.J.; Bumbaca, D.; Elstrott, J.; Boswell, C.A.; Zhang, Y.; Luk, W.; Lu, Y.; Dennis, M.S.; Weimer, R.M.; et al. Transferrin receptor (TfR) trafficking determines brain uptake of TfR antibody affinity variants. J. Exp. Med. 2014, 211, 233–244. [Google Scholar] [CrossRef]
- Chang, H.Y.; Wu, S.; Li, Y.; Zhang, W.; Burrell, M.; Webster, C.I.; Shah, D.K. Brain pharmacokinetics of anti-transferrin receptor antibody affinity variants in rats determined using microdialysis. mAbs 2021, 13, 1874121. [Google Scholar] [CrossRef] [PubMed]
- Boado, R.J.; Hui, E.K.; Lu, J.Z.; Pardridge, W.M. Very High Plasma Concentrations of a Monoclonal Antibody against the Human Insulin Receptor Are Produced by Subcutaneous Injection in the Rhesus Monkey. Mol. Pharm. 2016, 13, 3241–3246. [Google Scholar] [CrossRef] [PubMed]
- Boado, R.J.; Hui, E.K.; Lu, J.Z.; Zhou, Q.H.; Pardridge, W.M. Reversal of lysosomal storage in brain of adult MPS-I mice with intravenous Trojan horse-iduronidase fusion protein. Mol. Pharm. 2011, 8, 1342–1350. [Google Scholar] [CrossRef]
- Boado, R.J.; Hui, E.K.; Lu, J.Z.; Sumbria, R.K.; Pardridge, W.M. Blood-brain barrier molecular trojan horse enables imaging of brain uptake of radioiodinated recombinant protein in the rhesus monkey. Bioconjug. Chem. 2013, 24, 1741–1749. [Google Scholar] [CrossRef]
- Korogod, N.; Petersen, C.C.; Knott, G.W. Ultrastructural analysis of adult mouse neocortex comparing aldehyde perfusion with cryo fixation. eLife 2015, 4. [Google Scholar] [CrossRef] [PubMed]
- Brightman, M.W.; Klatzo, I.; Olsson, Y.; Reese, T.S. The blood-brain barrier to proteins under normal and pathological conditions. J. Neurol. Sci. 1970, 10, 215–239. [Google Scholar] [CrossRef]
- Pardridge, W.M. Brain Delivery of Nanomedicines: Trojan Horse Liposomes for Plasmid DNA Gene Therapy of the Brain. Front. Med. Technol. 2020, 2, 602236. [Google Scholar] [CrossRef]
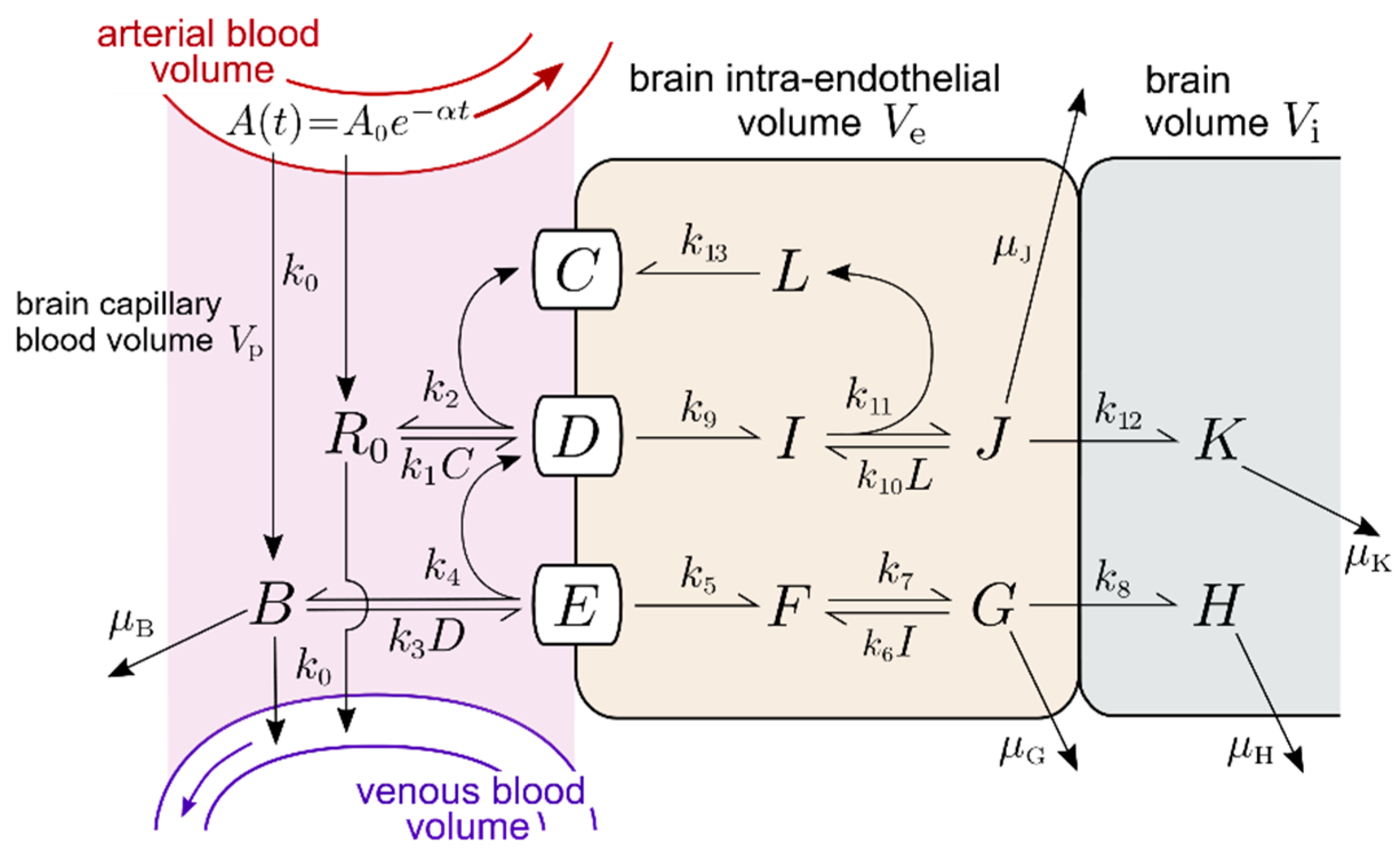



| A(t) | TfRMAb in arterial plasma |
| B(t) | TfRMAb in capillary plasma |
| R0 | endogenous holo-transferrin (Tf) in arterial/capillary plasma |
| C(t) | unoccupied TfR at brain endothelial luminal membrane |
| D(t) | TfR-Tf complex at endothelial luminal membrane |
| E(t) | TfR-Tf-TfRMAb complex at endothelial luminal membrane |
| F(t) | TfR-Tf-TfRMAb complex in endothelial intracellular compartment |
| G(t) | unbound TfRMAb in endothelial intracellular compartment |
| H(t) | TfRMAb in brain extracellular space |
| I(t) | TfR-Tf complex in endothelial intracellular compartment |
| J(t) | unbound Tf in endothelial intracellular compartment |
| K(t) | Tf in brain extracellular space |
| L(t) | unoccupied TfR in endothelial intracellular compartment |
| Parameter | Value | Description |
|---|---|---|
| A0 | 20 nM | Maximal plasma concentration of TfRMAb after intravenous administration of 0.2 mg/kg |
| α | 0.0055 min−1 | Rate constant of removal of TfRMAb from plasma by peripheral tissues |
| k0 | 42 min−1 | Rate constant of brain capillary blood flow |
| k1 | 0.1 nM−1 min−1 | Rate constant of Tf association with TfR at endothelial plasma membrane |
| k2 | 0.06 min−1 | Rate constant of Tf dissociation from TfR at endothelial plasma membrane |
| k3 | 0.06 nM−1 min−1 | Rate constant of TfRMAb association with Tf-TfR complex at plasma membrane |
| k4 | 0.022 min−1 | Rate constant of TfRMAb dissociation with Tf-TfR complex at plasma membrane |
| k5 | 0.14 min−1 | Rate constant of internalization of TfRMAb-Tf-TfR complex from endothelial luminal membrane into intra-endothelial compartment |
| k6 | 0.06 nM−1 min−1 | Rate constant of TfRMAb association with Tf-TfR complex within endothelial cell |
| k7 | 0.022 min−1 | Rate constant of TfRMAb dissociation with Tf-TfR complex within endothelial cell |
| k8 | 0.14 min−1 | Rate constant of MAb exocytosis into brain interstitium |
| k9 | 0.14 min−1 | Rate constant of internalization of Tf-TfR complex from endothelial luminal membrane into intra-endothelial compartment |
| k10 | 0.1 nM−1 min−1 | Rate constant of Tf association with TfR within endothelial cell |
| k11 | 0.06 min−1 | Rate constant of Tf dissociation from TfR within endothelial cell |
| k12 | 0.14 min−1 | Rate constant of Tf exocytosis into brain interstitium |
| k13 | 0.035 min−1 | Rate constant of intracellular TfR recycling back to plasma membrane |
| μB | 0.00096 min−1 | Rate constant of TfRMAb removal from brain capillary plasma compartment other than binding to endothelial luminal membrane |
| μH | 0.00096 min−1 | Rate constant of degradation of TfRMAb in brain |
| μK | 0.00096 min−1 | Rate constant of degradation of Tf in brain |
| μJ | 0.0058 min−1 | Rate constant of degradation of free Tf within endothelial compartment |
| μG | 0.0058 min−1 | Rate constant of degradation of free TfRMAb within endothelial compartment |
| L0 | 40 nM | Total TfR in endothelial cell |
| R0 | 25,000 nM | Total holo-Tf in plasma |
| Simulation | Parameters | Variable Concentrations (nM) | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| B | C | D | E | F | G | H | I | J | K | L | ||
| 1 | Basal a | 10.3 | <0.0002 | 0.274 | 1.08 | 20.1 | 0.464 | 3.62 | 13.3 | 1.18 | 22.2 | 5.29 |
| 2 | k8 = k12 = 0.069 min−1 | 10.3 | <0.0002 | 0.221 | 0.869 | 18.7 | 0.397 | 1.53 | 15.9 | 1.91 | 15.8 | 4.27 |
| 3 | k8 = k12 = 0.035 min−1 | 10.3 | <0.0002 | 0.179 | 0.702 | 17.2 | 0.328 | 0.675 | 18.4 | 2.86 | 10.8 | 3.46 |
| 4 | k8 = k12 = 0.023 min−1 | 10.3 | <0.0002 | 0.158 | 0.621 | 16.5 | 0.298 | 0.415 | 19.6 | 3.54 | 8.15 | 3.03 |
| 5 | k8 = 0 | 10.3 | <0.0002 | 0.252 | 1.00 | 23.1 | 0.762 | 0 | 10.7 | 1.03 | 21.4 | 4.84 |
| 6 | k13 = 0.023 min−1 | 10.3 | <0.0002 | 0.244 | 0.958 | 16.8 | 0.356 | 2.76 | 14.8 | 1.03 | 19.4 | 7.19 |
| 7 | k5 = k9 = 0.069 min−1 | 10.3 | <0.0002 | 0.331 | 2.34 | 20.1 | 0.498 | 3.65 | 12.2 | 1.12 | 21.5 | 5.06 |
| 8 | k5 = k9 = 0.035 min−1 | 10.3 | <0.0002 | 0.405 | 5.040 | 18.5 | 0.489 | 3.26 | 11.2 | 1.07 | 21.1 | 4.87 |
| 9 | k5 = k9 = 0.023 min−1 | 10.3 | <0.0002 | 0.520 | 7.69 | 16.3 | 0.451 | 2.82 | 10.7 | 1.03 | 20.8 | 4.75 |
| 10 | k3 = k6 = 0.006 nM−1 min−1 k4 = k7 = 0.0022 min−1 | 10.3 | <0.0002 | 1.15 | 0.521 | 11.0 | 0.087 | 0.722 | 20.6 | 1.54 | 25.9 | 6.61 |
| ID (mg/kg) | KD (nM) of MAb Binding to TfR | Binding Kinetics and Brain AUC | |||||
|---|---|---|---|---|---|---|---|
| Association k3, k6 (nM−1min−1) | Dissociation k4, k7 (min−1) | Brain AUC | Association k3, k6 (nM−1min−1) | Dissociation k4, k7 (min−1) | Brain AUC | ||
| 0.2 | 0.36 | 0.06 | 0.022 | 64,524 | 0.006 | 0.0022 | 19,901 |
| 3.6 | 0.22 | 64,786 | 0.022 | 25,260 | |||
| 36 | 2.2 | 20,127 | 0.22 | 14,011 | |||
| 360 | 22 | 2590 | 2.2 | 2447 | |||
| 3 | 0.36 | 0.06 | 0.022 | 204,389 | 0.006 | 0.0022 | 90,081 |
| 3.6 | 0.22 | 316,050 | 0.022 | 210,015 | |||
| 36 | 2.2 | 233,373 | 0.22 | 196,123 | |||
| 360 | 22 | 70,296 | 2.2 | 67,412 | |||
| 30 | 0.36 | 0.06 | 0.022 | 238,284 | 0.006 | 0.0022 | 119,561 |
| 3.6 | 0.22 | 420,757 | 0.022 | 358,570 | |||
| 36 | 2.2 | 478,087 | 0.22 | 457,614 | |||
| 360 | 22 | 358,462 | 2.2 | 352,727 | |||
| Injection Dose (mg/kg) | Plasma AUC (pmol·min/mL) | |
|---|---|---|
| TfRMAb | HIRMAb-IDUA | |
| 0.1 | - | 110 |
| 0.2 | 3695 | 241 |
| 2 | - | 5372 |
| 3 | 148,819 | - |
| 20 | - | 82,813 |
| 30 | 2,921,970 | - |
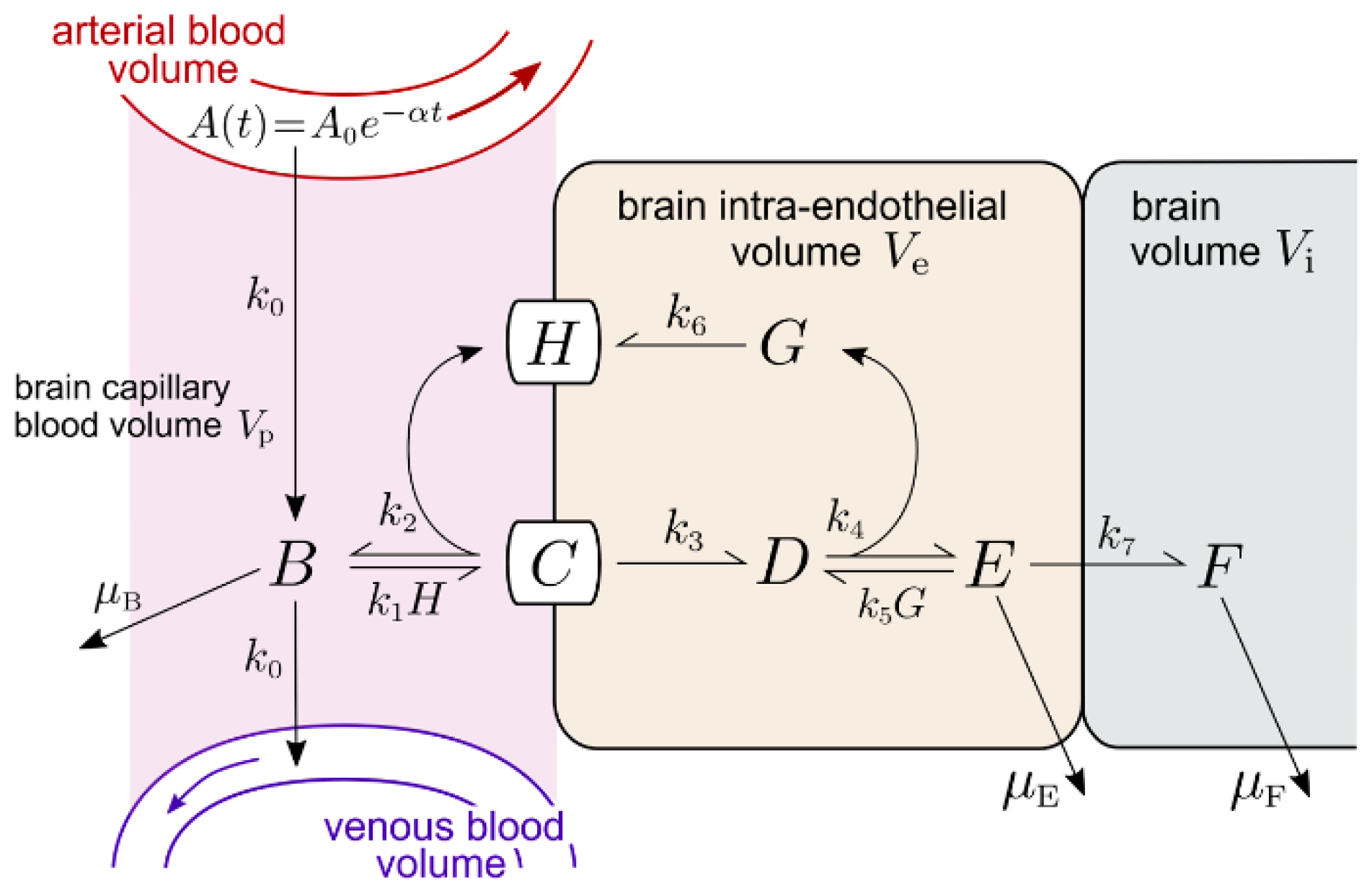
| A(t) | IRMAb in arterial plasma |
| B(t) | IRMAb in capillary plasma |
| C(t) | IRMAb-IR complex at endothelial luminal membrane |
| D(t) | IRMAb-IR complex within endothelial intracellular compartment |
| E(t) | unbound IRMAb within endothelial intracellular compartment |
| F(t) | IRMAb in brain extracellular space |
| G(t) | unoccupied IR within endothelial intracellular compartment |
| H(t) | unoccupied IR at brain endothelial luminal membrane |
| Parameter | Value | Description |
|---|---|---|
| A0 | 2 nM | Maximal plasma concentration of IRMAb after intravenous administration of 0.1 mg/kg |
| α | 0.0173 min−1 | Rate constant of removal of IRMAb from plasma via uptake by peripheral tissues |
| k0 | 42 min−1 | Rate constant of brain capillary blood flow |
| k1 | 0.006 nM−1 min−1 | Rate constant of IRMAb association with IR at endothelial plasma membrane |
| k2 | 0.0056 min−1 | Rate constant of IRMAb receptor dissociation from IR at endothelial plasma membrane |
| k3 | 0.14 min−1 | Rate constant of internalization of IRMAb-IR complex from endothelial luminal membrane into intra-endothelial compartment |
| k4 | 0.0056 min−1 | Rate constant of IRMAb dissociation from IR in intra-endothelial compartment |
| k5 | 0.006 nM−1 min−1 | Rate constant of IRMAb association with IR in intra-endothelial compartment |
| k6 | 0.035 min−1 | Rate constant of recycling of unoccupied IR from intra-endothelial compartment to luminal plasma membrane |
| k7 | 0.14 min−1 | Rate constant of free IRMAb exocytosis across endothelial abluminal membrane into brain interstitial volume |
| μB | 0.00096 min−1 | Rate constant of IRMAb removal from brain capillary plasma compartment other than binding to endothelial luminal membrane |
| μE | 0.0058 min−1 | Rate constant of degradation of free IRMAb within endothelial compartment |
| μF | 0.00096 min−1 | Rate constant of removal of free IRMAb from brain via either degradation or efflux back to blood |
| L0 | 24 nM | Total IR in endothelial cell |
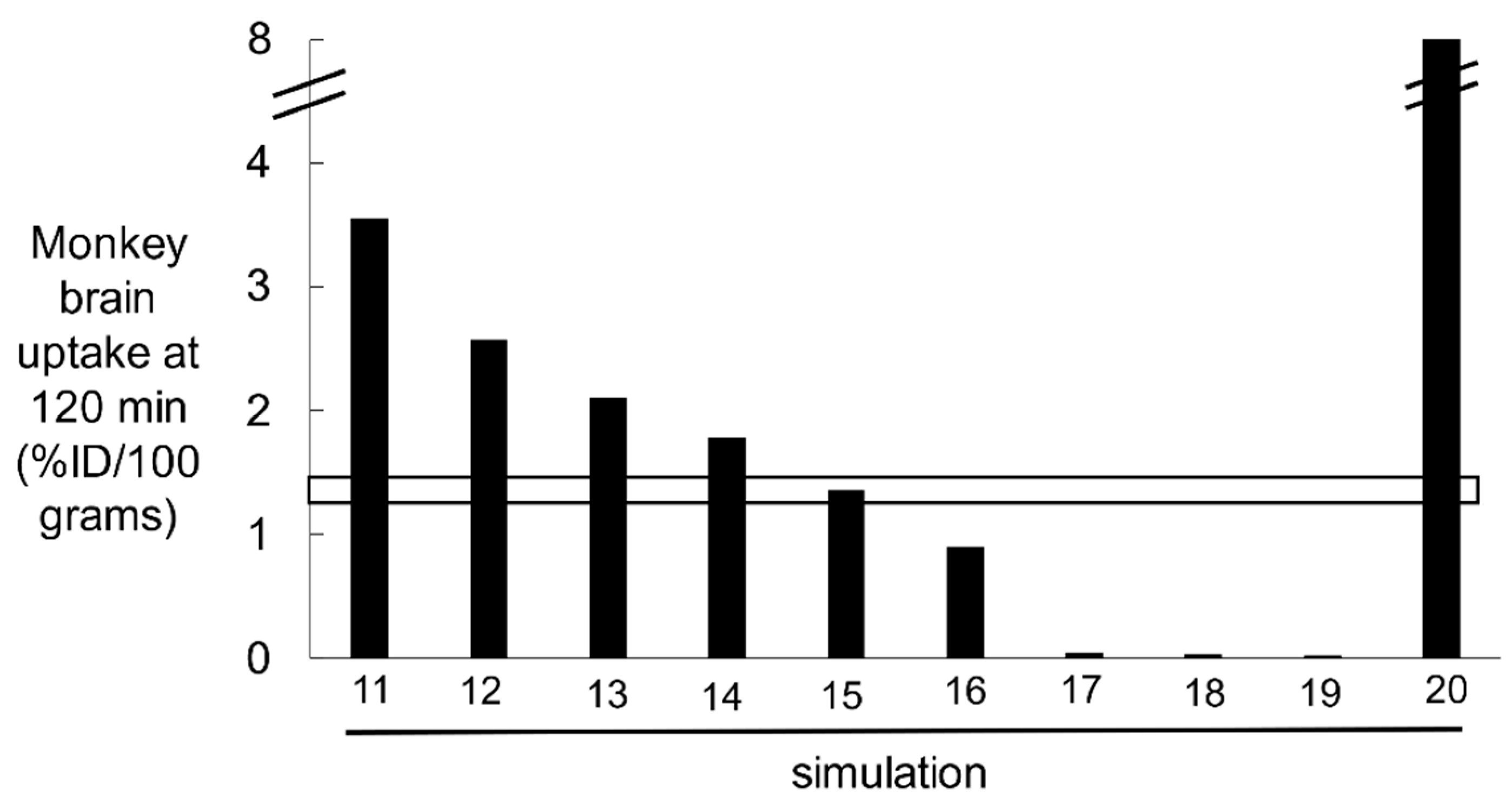
| Simulation | Parameter Changes from Basal Values | Variables (nM) | ||||||
|---|---|---|---|---|---|---|---|---|
| B | C | D | E | F | G | H | ||
| 11 | Basal a | 0.251 | 0.194 | 6.09 | 0.224 | 2.28 | 16.7 | 1.04 |
| 12 | k3 = 0.035 min−1 | 0.251 | 1.00 | 5.27 | 1.92 | 1.64 | 16.8 | 0.878 |
| 13 | k3 = 0.023 min−1 | 0.251 | 1.62 | 4.65 | 0.169 | 1.34 | 16.8 | 0.777 |
| 14 | k3 = 0.023 min−1 k7 = 0.069 min−1 | 0.251 | 1.62 | 4.69 | 0.312 | 1.13 | 16.9 | 0.765 |
| 15 | k3 = 0.023 min−1 k7 = 0.035 min−1 | 0.251 | 1.62 | 4.74 | 0.508 | 0.858 | 16.8 | 0.75 |
| 16 | k6 = 0.012 min−1 | 0.251 | 1.35 | 4.15 | 0.332 | 0.559 | 15.0 | 3.5 |
| 17 | k7 = 0 min−1 | 0.251 | 1.62 | 4.84 | 1.16 | 0 | 16.8 | 0.708 |
| 18 | k3 = 0 min−1 | 0.251 | 6.29 | 0 | 0 | 0 | 17.5 | 0.180 |
| 19 | k1 = k5 = 0.0006 nM−1 min−1 k2 = k4 = 0.00056 min−1 | 0.251 | 0.267 | 0.837 | 0.0091 | 0.014 | 22.7 | 0.190 |
| 20 | k1 = k5 = 0.06 nM−1 min−1 k2 = k4 = 0.056 min−1 | 0.251 | 2.72 | 6.72 | 2.00 | 4.94 | 12.1 | 2.47 |
| Parameter | Receptor | |
|---|---|---|
| TfR1 | IR | |
| Endogenous ligand | holo-transferrin | insulin |
| Plasma concentration of endogenous ligand | 25,000 nM | 0.3 nM |
| Total endothelial receptor | 40 nM | 24 nM |
| [ligand]/[receptor] ratio | 625 | 0.01 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pardridge, W.M.; Chou, T. Mathematical Models of Blood-Brain Barrier Transport of Monoclonal Antibodies Targeting the Transferrin Receptor and the Insulin Receptor. Pharmaceuticals 2021, 14, 535. https://doi.org/10.3390/ph14060535
Pardridge WM, Chou T. Mathematical Models of Blood-Brain Barrier Transport of Monoclonal Antibodies Targeting the Transferrin Receptor and the Insulin Receptor. Pharmaceuticals. 2021; 14(6):535. https://doi.org/10.3390/ph14060535
Chicago/Turabian StylePardridge, William M., and Tom Chou. 2021. "Mathematical Models of Blood-Brain Barrier Transport of Monoclonal Antibodies Targeting the Transferrin Receptor and the Insulin Receptor" Pharmaceuticals 14, no. 6: 535. https://doi.org/10.3390/ph14060535
APA StylePardridge, W. M., & Chou, T. (2021). Mathematical Models of Blood-Brain Barrier Transport of Monoclonal Antibodies Targeting the Transferrin Receptor and the Insulin Receptor. Pharmaceuticals, 14(6), 535. https://doi.org/10.3390/ph14060535








