A Low-Noise High-Resolution Temperature Measurement Technique Based on Inductive Voltage Divider and Alternating-Current Bridge
Abstract
1. Introduction
2. Proposed Design Scheme
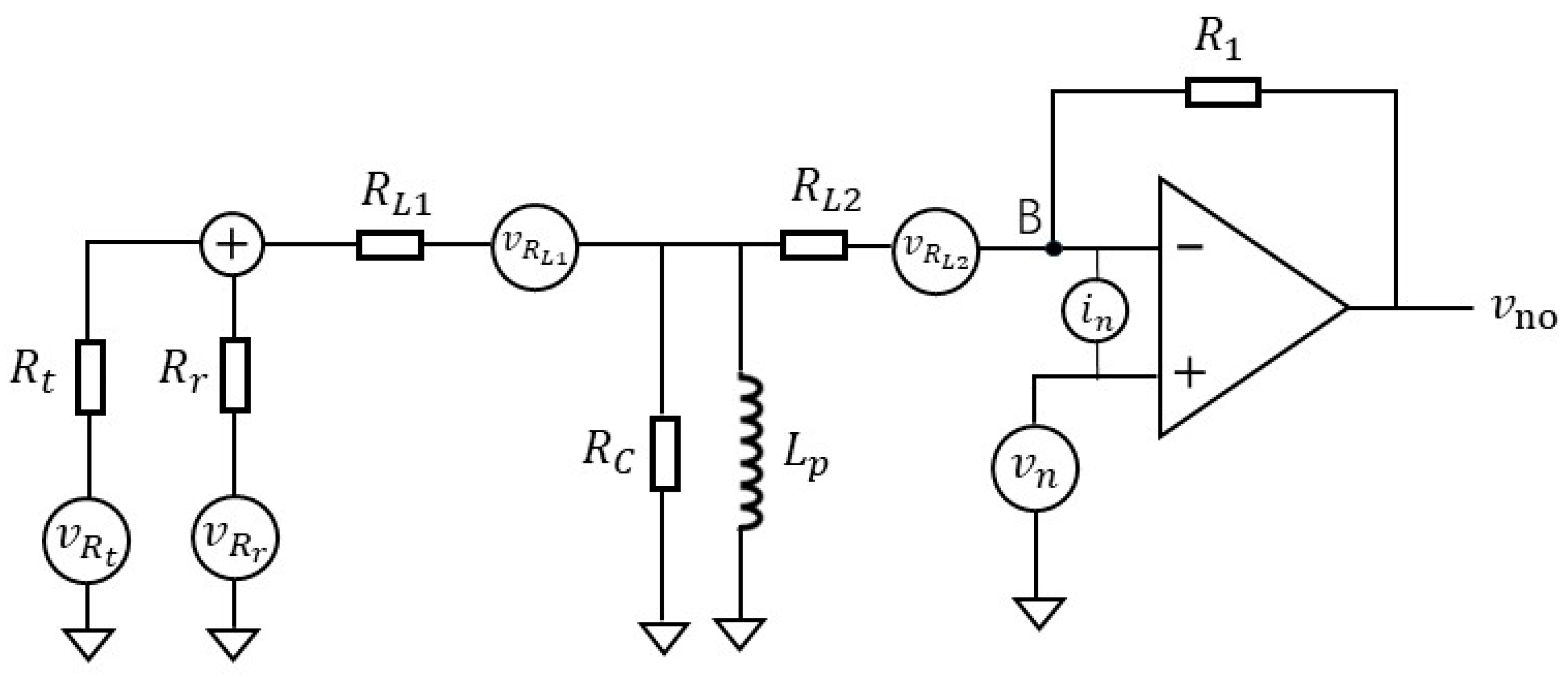
2.1. Temperature Measurement Circuit Based on Inductive Voltage Divider and AC Bridge
2.2. Data Processing Algorithm Based on Discrete Fourier Transform
3. Results and Discussion
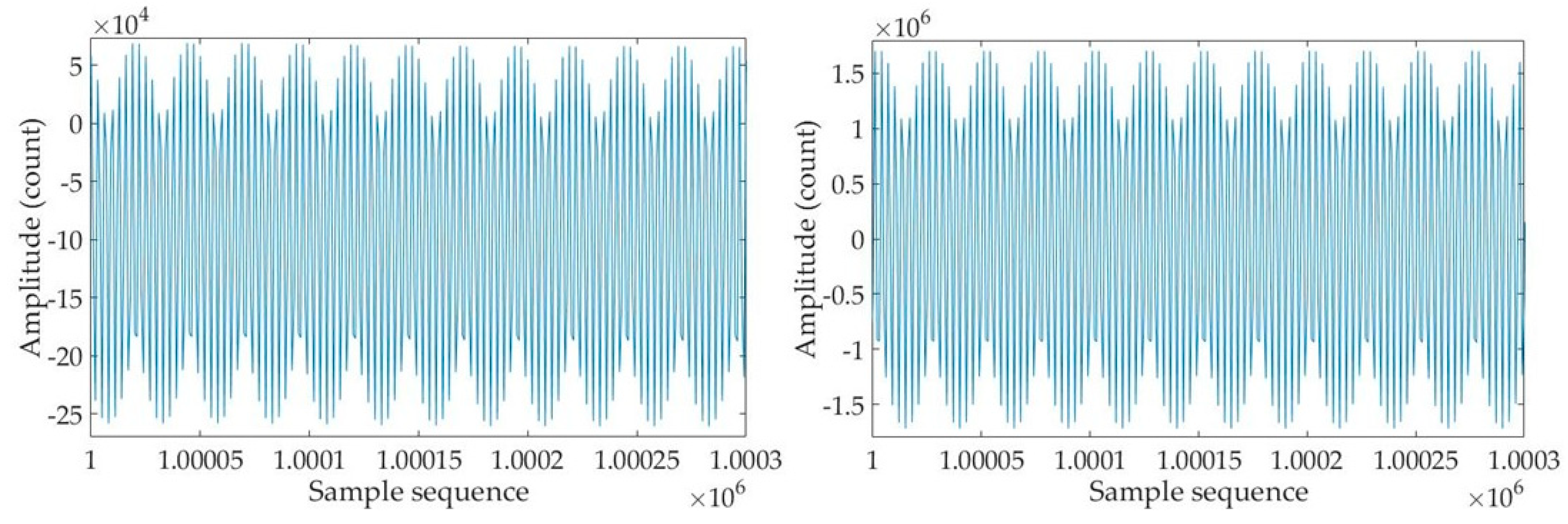
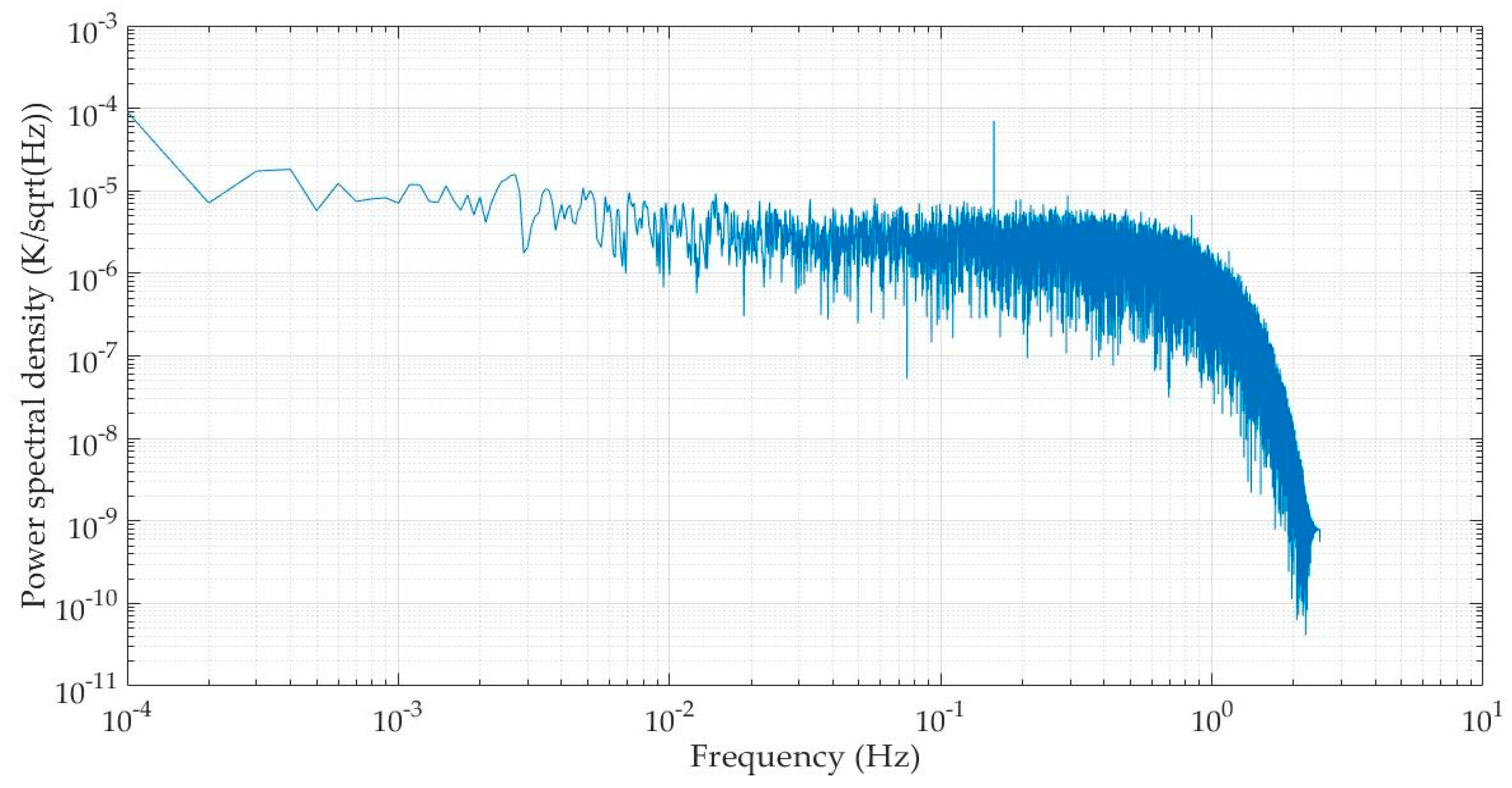
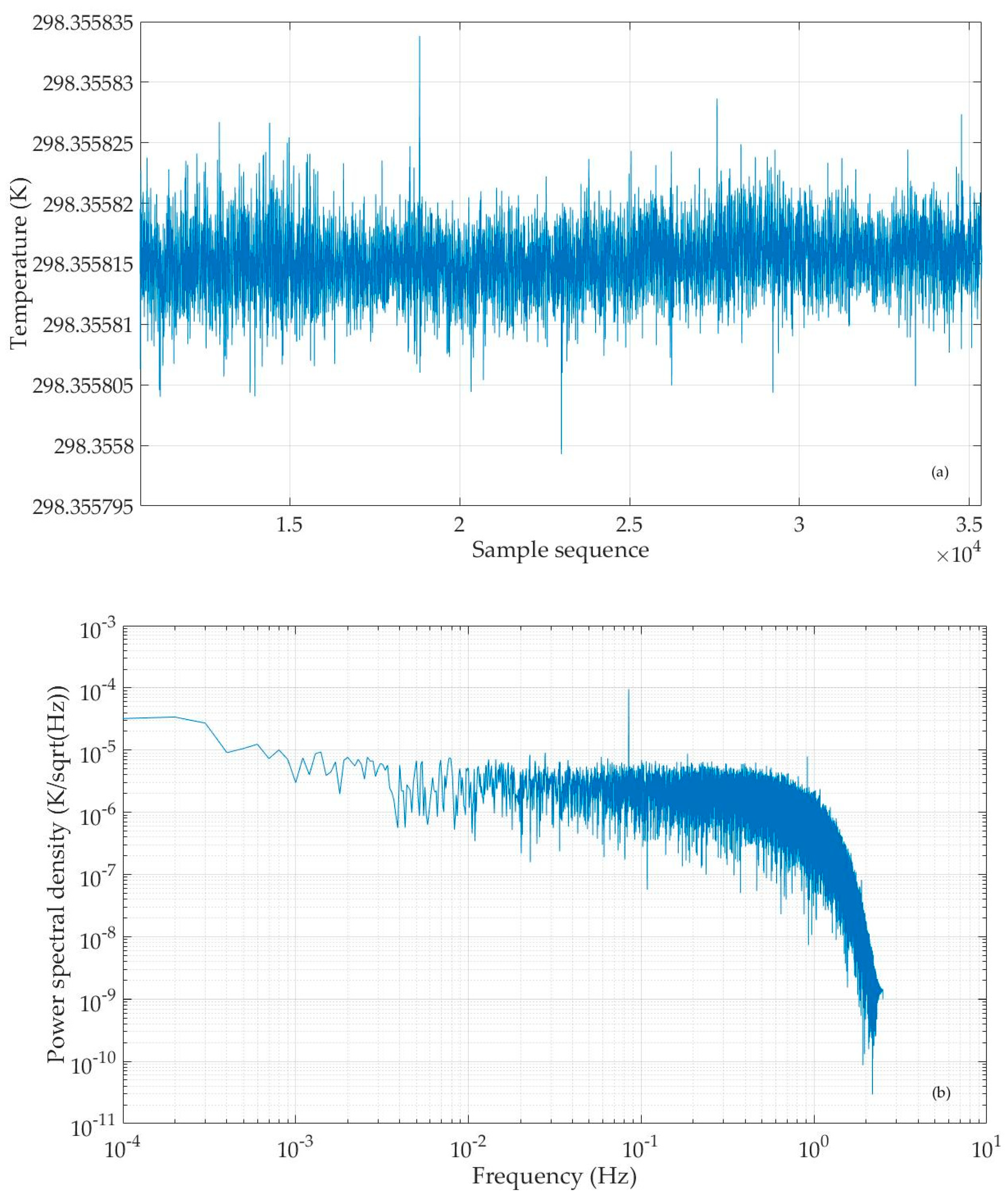
3.1. Noise Floor Level Test of the Temperature Measurement Circuit
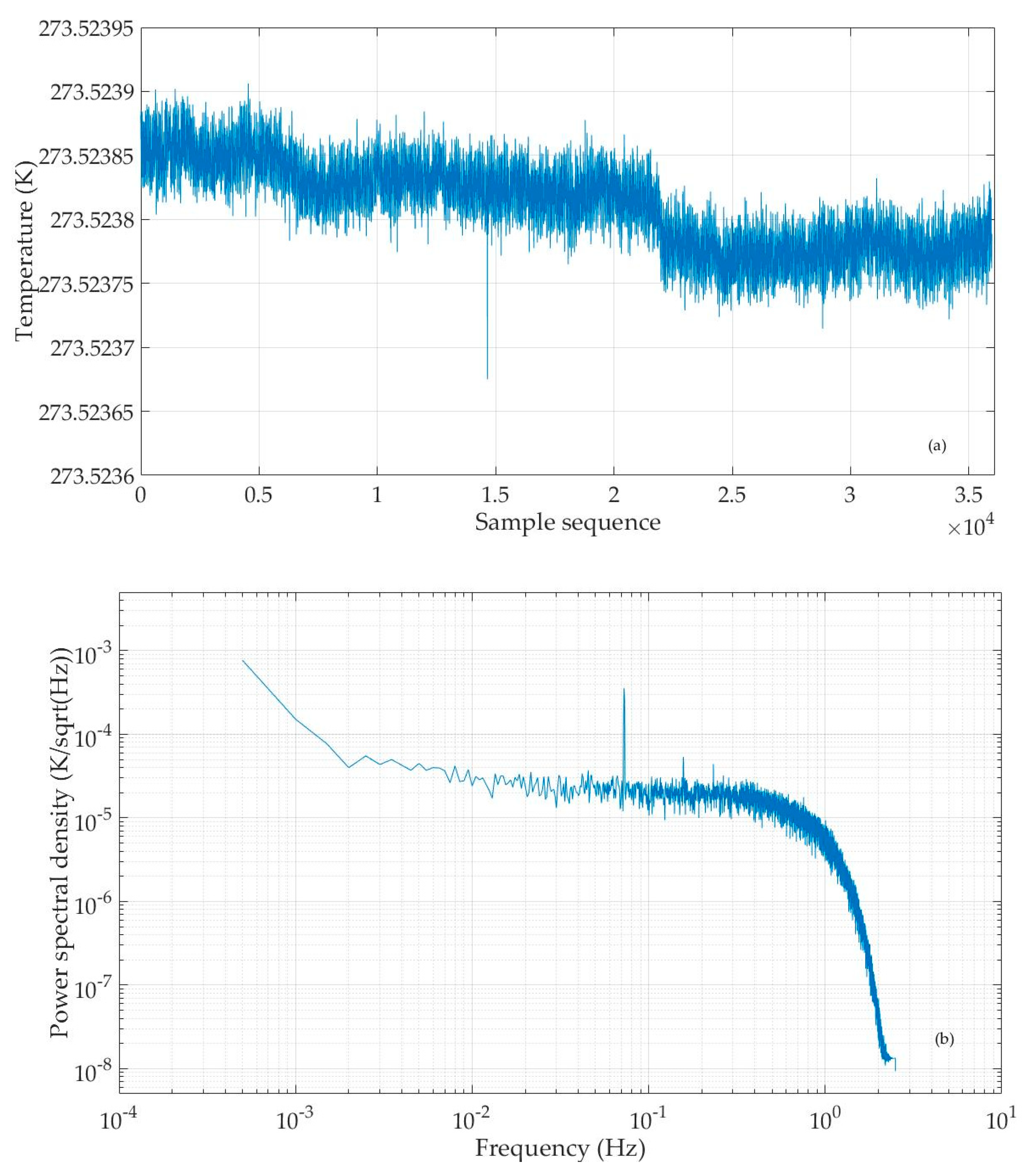
3.2. Temperature Test with Water Three-Phase Point Bottle
3.3. Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wen, M.X.; Li, J.; Wang, C.; Ling, C.; Gu, L.; Ding, Y. Review of high precision temperature sensing, measurement and control technology. Acta Sci. Nat. Univ. Sunyatseni 2021, 60, 146–155. [Google Scholar]
- Luo, J.; Ai, L.; Ai, Y.; An, Z.; Bai, W.; Bai, Y. A brief introduction to the tianqin project. Acta Sci. Nat. Univ. Sunyatseni 2021, 60, 1–19. [Google Scholar]
- Luo, Z.; Wang, Y.; Wu, Y.; Hu, W.; Jin, G. The taiji program: A concise overview. Prog. Theor. Exp. Phys. 2020, 2021, 108–117. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abernathy, M.R.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; et al. Observation of gravitational waves from a binary black hole merger. Phys. Rev. Lett. 2016, 116, 061102. [Google Scholar] [CrossRef]
- Zhang, X.; Liang, H.; Tan, H.; Feng, J.; Li, H.; Taiji Scientific Collaboration. Temperature stability of the Taiji-1 satellite in operational orbit. Int. J. Mod. Phys. A 2021, 36, 2140022. [Google Scholar] [CrossRef]
- Sanjuán, J. Development and Validation of the Thermal Diagnostics Instrumentation in Lisa Pathfinder. Doctoral Thesis, Universitat Politècnica de Catalunya, Barcelona, Spain, 2009. [Google Scholar]
- Zhou, Y.; Lai, J.J.; Li, Y.S.; Wang, Z.Q.; Jiang, X.P. Design of multi-channel temperature measurement system based on ADS1218Y with high precision. Transducer Microsyst. Technol. 2020, 39, 76–82. [Google Scholar]
- Hu, P.C.; Shi, W.Z.; Mei, J.T. High precision Pt-resistance temperature measurement system. Opt. Precis. Eng. 2014, 22, 988–995. [Google Scholar]
- Qin, Y.; Yang, X.; Wu, B.; Sun, Z.; Shi, X. High resolution temperature measurement technique for measuring marine heat flow. Sci. China Tech Sci. 2013, 56, 1773–1778. [Google Scholar] [CrossRef]
- Zhang, Z.H.; Chen, S.Y.; Liu, P.X. A key technology for monitoring stress by temperature: Multichannel temperature measurement system with high precision and low power consumption. Seismol. Geol. 2018, 40, 499–510. (In Chinese) [Google Scholar]
- The Fluke1560 Stacked Temperature Inspection System. Available online: https://www.fluke.com.cn (accessed on 18 March 2024).
- Wudy, F.E.; Moosbauer, D.J.; Multerer, M.; Schmeer, G.; Schweiger, H.G.; Stock, C.; Hauner, P.F.; Suppan, G.A.; Gores, H.J. Fast micro-kelvin resolution thermometer based on NTC thermistors. J. Chem. Eng. Data 2011, 56, 4823–4828. [Google Scholar] [CrossRef]
- Zhang, B.; Zhu, X.; Zhang, X.; Xue, B.; Liang, H.; Li, J.; Su, P.; Taiji Scientific Collaboration. A temperature measurement system with high resolution and low noise. Int. J. Mod. Phys. A 2021, 36, 2140024. [Google Scholar] [CrossRef]
- Guillet, B.; Mechin, L.; Robbes, D. High Performance Temperature Controller: Application to the Excess Noise Measurements of YBCO Thermometers in the Transition Region [EB/OL]. Available online: https://arxiv.org/pdf/cond-mat/0305469 (accessed on 1 November 2024).
- Lobo, A.; Nofrarias, M.; Sanjuán, J. Thermal diagnostics for LTP. Class. Quantum Gravity 2005, 22, S171. [Google Scholar] [CrossRef]
- Sanjuán, J.; Lobo, A.; Nofrarias, M. Thermal diagnostics front-end electronics for LISA Pathfinder. Rev. Sci. Instrum. 2007, 78, 104904. [Google Scholar] [CrossRef]
- Armano, M.; Audley, H.; Baird, J.; Binetruy, P.; Born, M.; Bortoluzzi, D.; Castelli, E.; Cavalleri, A.; Cesarini, A.; Cruise, A.M.; et al. Beyond the required LISA free-fall performance: New LISA Pathfinder results down to 20 μHz. Phys. Rev. Lett. 2018, 120, 061101. [Google Scholar] [CrossRef] [PubMed]
- Armano, M.; Audley, H.; Baird, J.; Binetruy, P.; Born, M.; Bortoluzzi, D.; Castelli, E.; Cavalleri, A.; Cesarini, A.; Cruise, A.M.; et al. Temperature stability in the sub-milliHertz band with LISA Pathfinder. Mon. Not. R. Astron. Soc. 2019, 486, 3368–3379. [Google Scholar] [CrossRef]
- Valentini, D.; Vacance, M.; Battaglia, D.; Pieper, B.; Niot, J.M. GOCE instrument thermal control. SAE Trans. 2006, 115–126. [Google Scholar]
- Steiger, C.; Mardle, N.; Emanuelli, P.P. GOCE End-of-Mission Operations Report; ESA2014; European Space Agency: Paris, France, 2014. [Google Scholar]
- Gao, S.; Xue, B.; Li, J.; Lin, Z.; Chen, Y.; Zhu, X. High-resolution data acquisition technique in broadband seismic observation system. Sci. China Technol. Sci. 2016, 59, 961–972. [Google Scholar] [CrossRef]
- Цыкин, Г.С. Design of Low Frequency Transformer; Huang, K.; Feng, L.; Zhang, G., Translators; Post & Telecom Press: Beijing, China, 1959. [Google Scholar]
- Zhang, X.; Chen, W.; Zhu, X.; Meng, N.; He, J.; Bi, X.; Zhang, Y.; Shi, Q.; Li, F.; Liu, R.; et al. Space advanced technology demonstration satellite. Sci. China Technol. Sci. 2024, 67, 240–258. [Google Scholar] [CrossRef]
- Lin, S.; Li, K.; Jiang, G.; Wu, J.; MA, E. Method of temperature control and its validation for atomic clock cabin on navigation satellite. Chin. J. Space Sci. 2019, 39, 381–387. [Google Scholar] [CrossRef]
- Liu, Y. Sub-mK Temperature Control for Ultrastable Laser Systems. Master’s Thesis, Huazhong University of Science & Technology, Wuhan, China, 2015. [Google Scholar]
- Yamada, A. ArF immersion lithography for 45 nm and beyond. Proceeding SPIE 2007, 6607, 1–10. [Google Scholar]
- Dong, H.; Li, X.; He, X.; Zeng, Z.; Wen, G. A two-degree-of-freedom controller for a high-precision air temperature control system with multiple disturbances. Case Stud. Therm. Eng. 2023, 50, 103442. [Google Scholar] [CrossRef]
- Xia, F.; Zhao, Y.; Zheng, H.K.; Li, L.K.; Tong, R.J. Ultra-sensitive seawater temperature sensor using an FBG-cascaded microfiber MZI operating at dispersion turning point. Opt. Laser Technol. 2020, 132, 106458. [Google Scholar] [CrossRef]
- Yan, T.; Liang, J.; Qian, F.; Li, Q.C.; Lu, Y. Performance test and uncertainty analysis of high precision seawater thermostatic bath. Metrol. Meas. Tech. 2017, 44, 1–3. [Google Scholar]
- Wang, Z.Q.; Wu, Z.K.; Yang, Y.; Song, L.W.; Zhu, X.Y. Design and implementation of high stability constant temperature system for relative gravimeter. Transducer Microsyst. Technol. 2022, 41, 80–83. [Google Scholar]
- Chen, S.Y.; Liu, P.X.; Chen, L.C.; Liu, Q.Y. Evidence from seismological observation for detecting dynamic change in crustal stress by bedrock temperature. Chin. Sci. Bull. 2020, 65, 2395–2405. (In Chinese) [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, S.; Zhu, X.; Zhang, X.; Xue, B.; Xi, J.; Li, J.; Zhang, B.; Wang, X.; Wang, Y.; Zhang, H.; et al. A Low-Noise High-Resolution Temperature Measurement Technique Based on Inductive Voltage Divider and Alternating-Current Bridge. Sensors 2025, 25, 2777. https://doi.org/10.3390/s25092777
Gao S, Zhu X, Zhang X, Xue B, Xi J, Li J, Zhang B, Wang X, Wang Y, Zhang H, et al. A Low-Noise High-Resolution Temperature Measurement Technique Based on Inductive Voltage Divider and Alternating-Current Bridge. Sensors. 2025; 25(9):2777. https://doi.org/10.3390/s25092777
Chicago/Turabian StyleGao, Shanghua, Xiaoyi Zhu, Xiaofeng Zhang, Bing Xue, Jilou Xi, Jiang Li, Bing Zhang, Xiaolei Wang, Yuru Wang, Haoyue Zhang, and et al. 2025. "A Low-Noise High-Resolution Temperature Measurement Technique Based on Inductive Voltage Divider and Alternating-Current Bridge" Sensors 25, no. 9: 2777. https://doi.org/10.3390/s25092777
APA StyleGao, S., Zhu, X., Zhang, X., Xue, B., Xi, J., Li, J., Zhang, B., Wang, X., Wang, Y., Zhang, H., & Wu, X. (2025). A Low-Noise High-Resolution Temperature Measurement Technique Based on Inductive Voltage Divider and Alternating-Current Bridge. Sensors, 25(9), 2777. https://doi.org/10.3390/s25092777




