Optimization of MIMU Mounting Position on Shank in Posture Estimation Considering Muscle Protuberance
Abstract
1. Introduction
1.1. Gait Analysis and Monitoring with MIMU
1.2. Review of Previous Studies on MIMU Mounting Positions and Issues
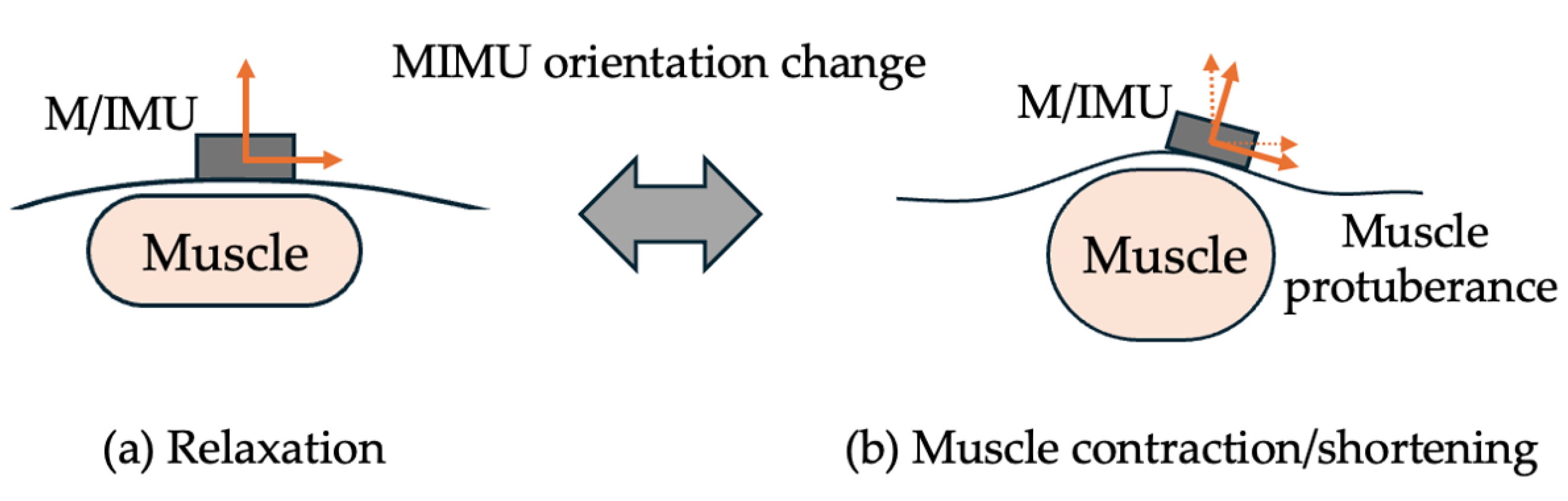
1.3. Muscular Protuberance and MIMU Mounting Position
2. Materials and Methods
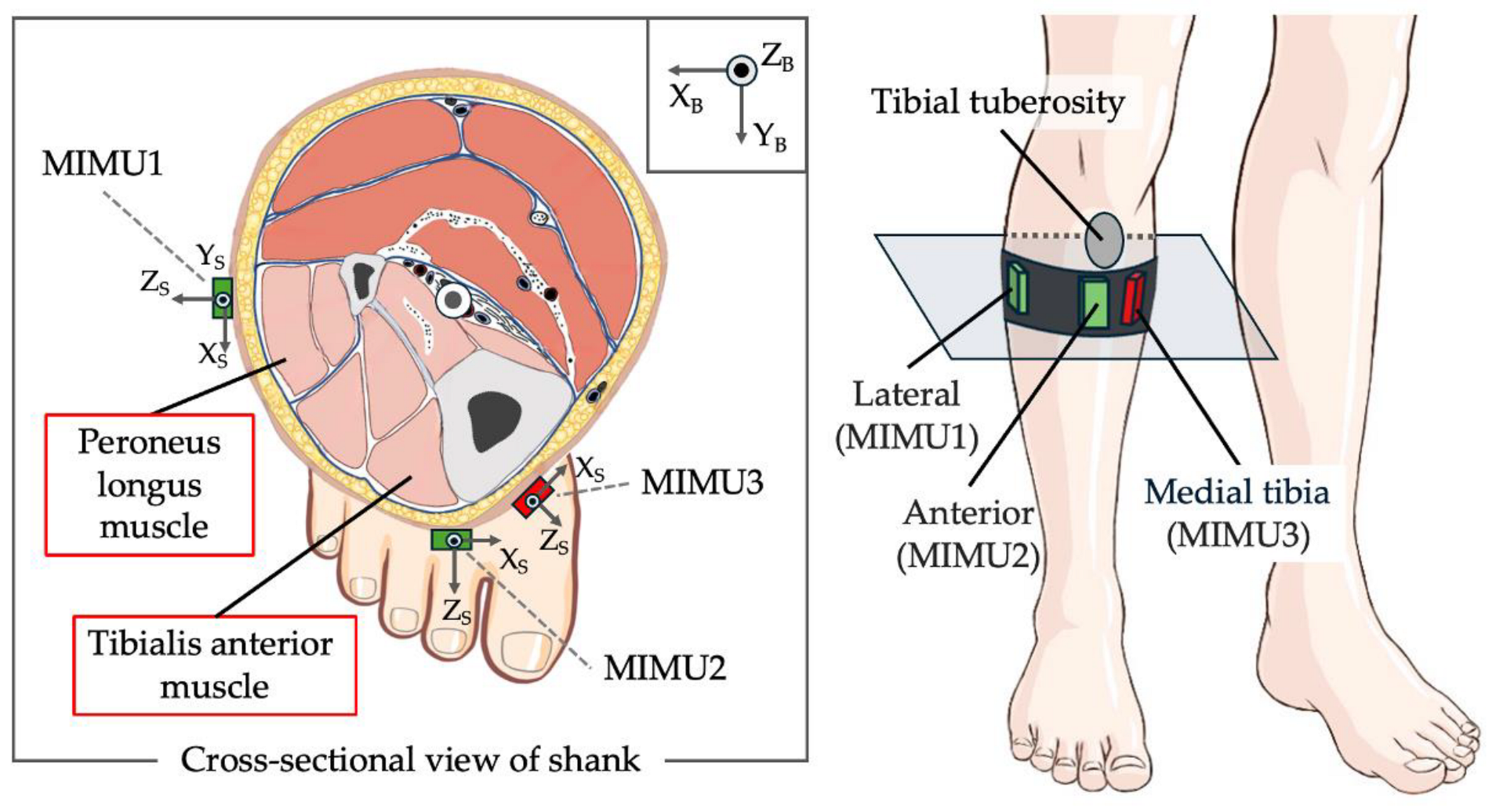
2.1. MIMU Mounting Positions
2.2. MIMU Sensor Characteristics and Controller
2.3. Posture Estimation Using MIMUs
2.4. Subjects
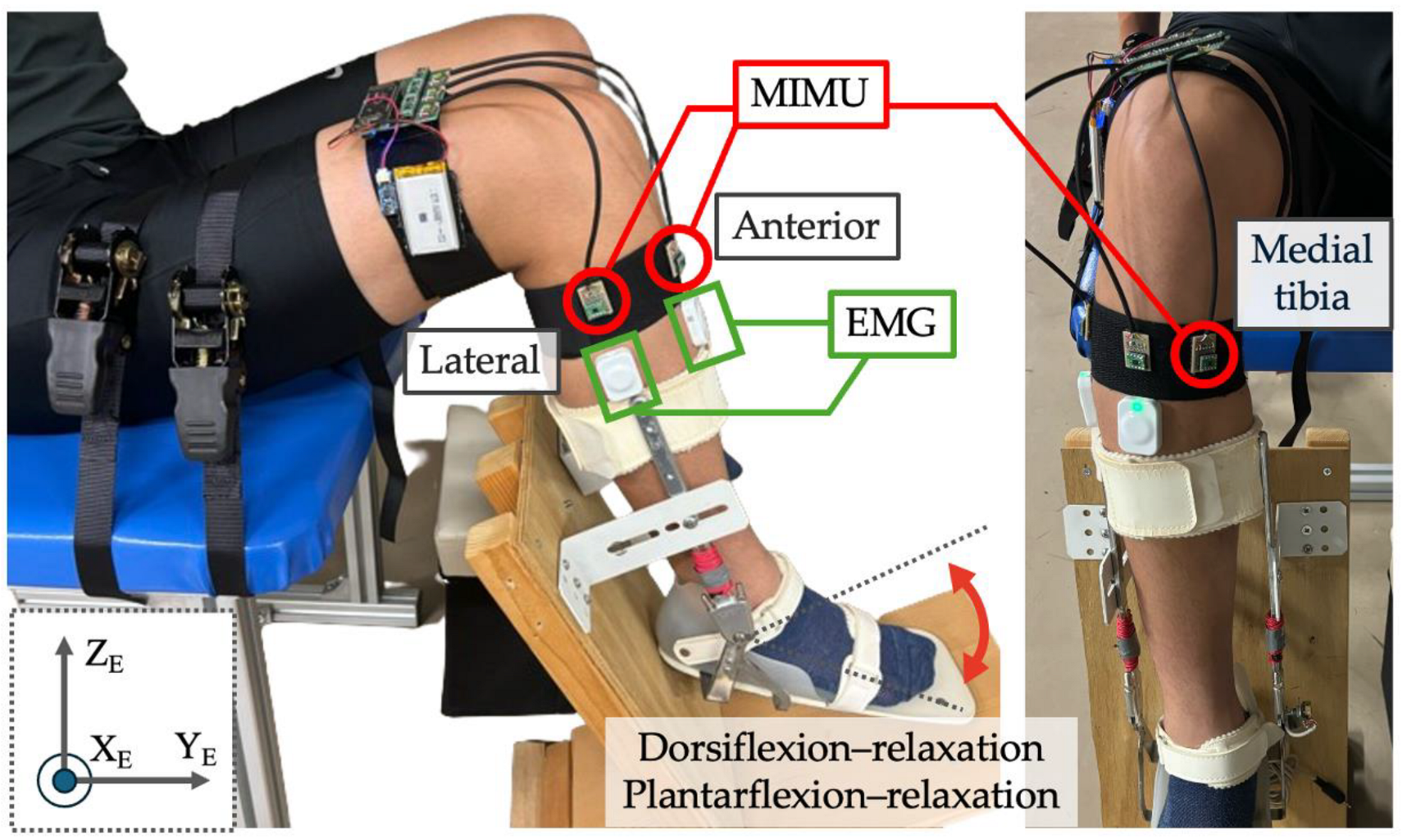
2.5. Experiment 1: Verifying the Effects of Muscle Contraction
2.5.1. Objectives and Methods
2.5.2. Analysis (The MIMU Orientation Change)
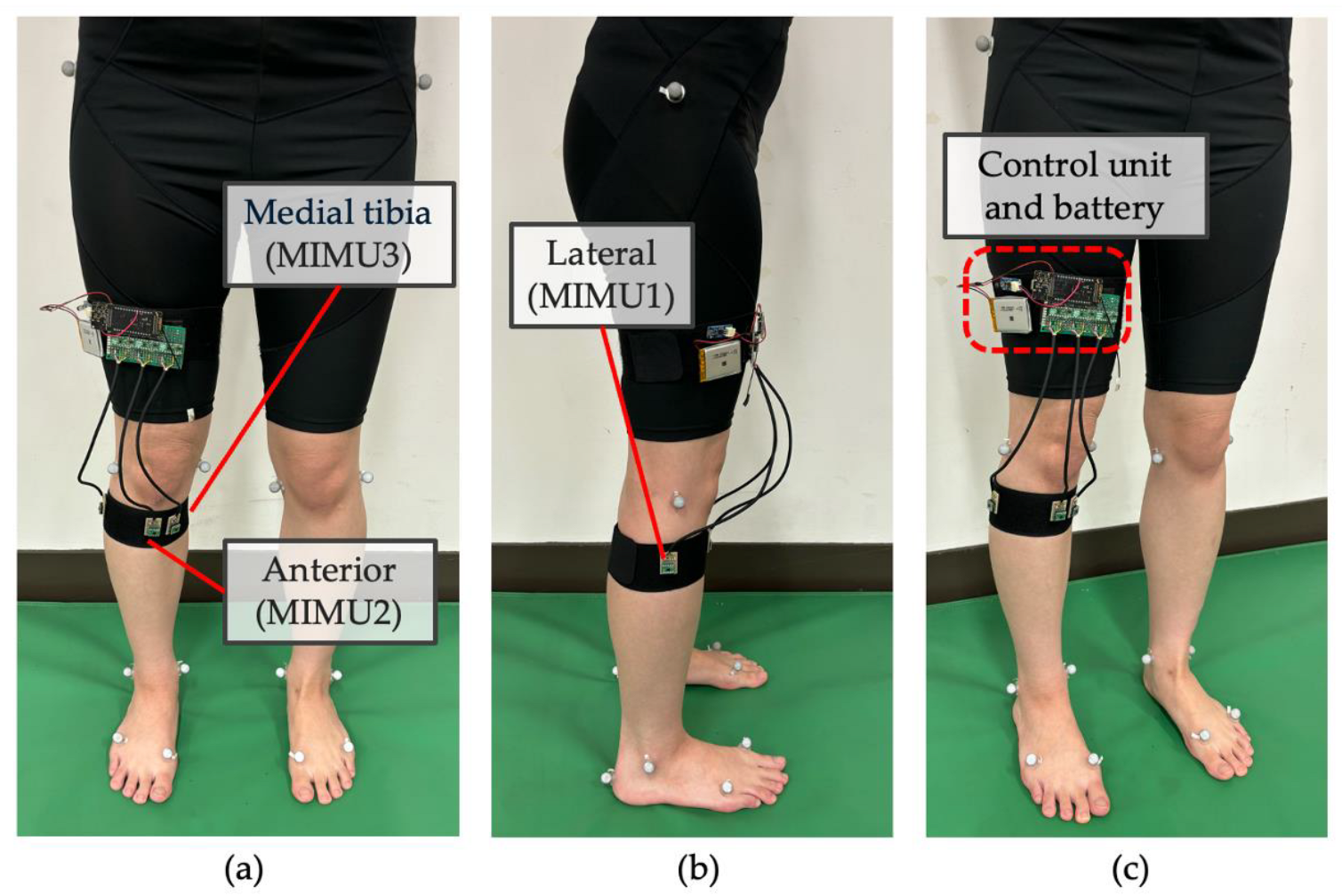
2.6. Experiment 2: Estimating 3D Shank Posture During Gait
2.6.1. Gait Conditions
2.6.2. Measurement
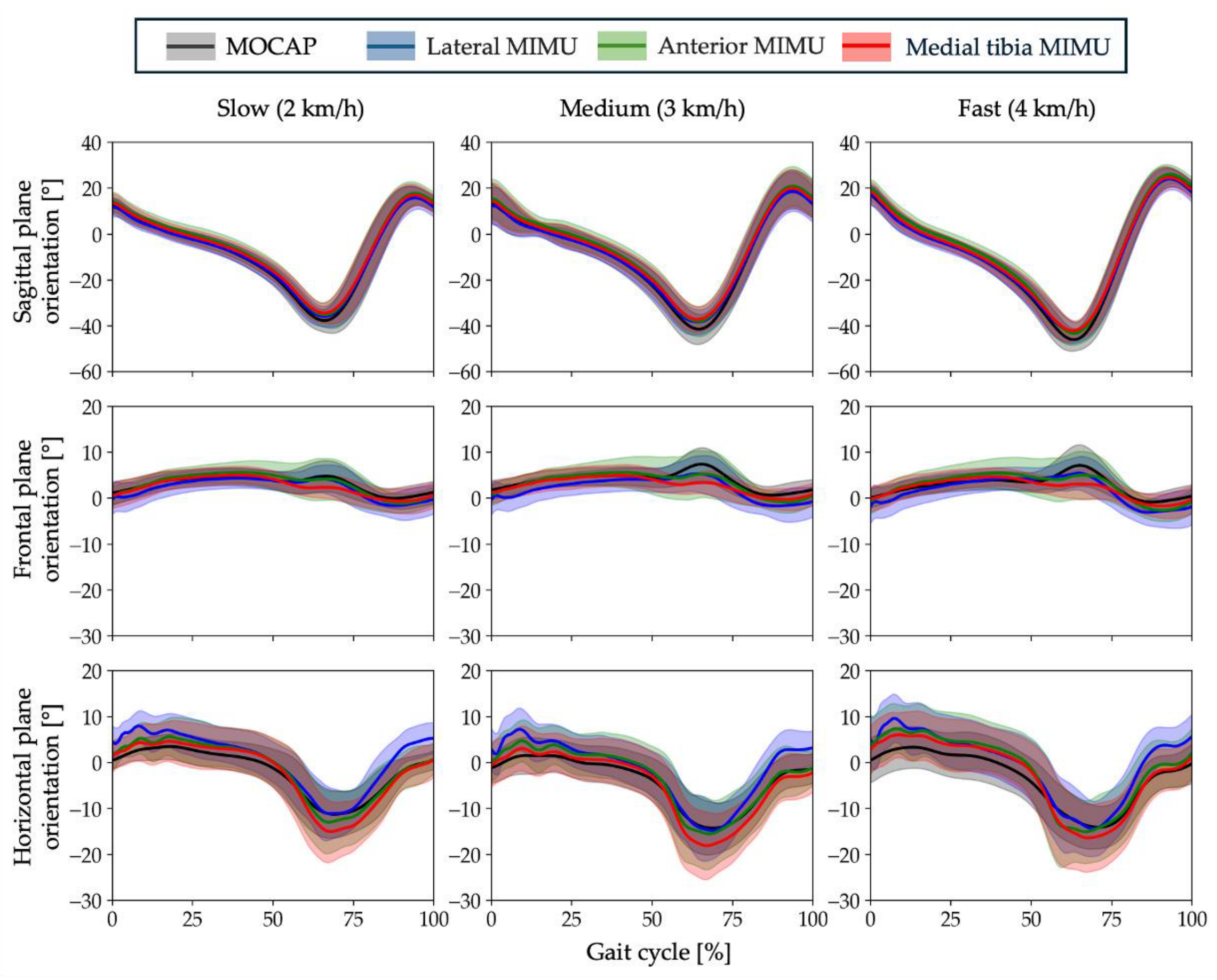
2.6.3. Analysis (Comparison of MIMU and MOCAP)
3. Results
3.1. Results of Experiments on Muscle Contraction Effects (Experiment 1)
3.2. Results of Posture Estimation Experiments During Gait (Experiment 2)
4. Discussion
4.1. Effects of Muscular Protuberance
4.2. Accuracy and Validity of Posture Estimation During Gait
4.3. Optimal MIMU Mounting Position on the Shank
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| MOCAP | Motion capture |
| IMU | Inertial measurement unit |
| MIMU | Magnetic-inertial measurement unit |
| MARG | Magnetic, angular rate, and gravity unit |
| FES | Functional electrical stimulation |
| SVA | Shank-to-vertical angle |
| TUG | Timed up-and-go |
| RMSE | Root mean square error |
| STA | Soft tissue artifacts |
| 3D | Three-dimensional |
| S2S | Sensor-to-segment |
| EMG | Electromyogram |
| ANOVA | Analysis of variance |
| CC | Correlation coefficient |
| IC | Initial contact |
References
- Motoya, R.; Yamamoto, S.; Naoe, M.; Taniguchi, R.; Kawahara, A.; Iwata, T. Classification of abnormal gait patterns of poststroke hemiplegic patients in principal component analysis. Jpn. J. Compr. Rehabil. Sci. 2022, 12, 70–77. [Google Scholar] [CrossRef] [PubMed]
- Burtscher, J.; Moraud, E.M.; Malatesta, D.; Millet, G.P.; Bally, J.F.; Patoz, A. Exercise and gait/movement analyses in treatment and diagnosis of Parkinson’s Disease. Ageing Res. Rev. 2024, 93, 102147. [Google Scholar] [CrossRef] [PubMed]
- Mukaino, M.; Ohtsuka, K.; Tanikawa, H.; Matsuda, F.; Yamada, J.; Itoh, N.; Saitoh, E. Clinical-oriented Three-dimensional Gait Analysis Method for Evaluating Gait Disorder. J. Vis. Exp. 2018, 4, 57063. [Google Scholar] [CrossRef]
- Hulleck, A.A.; Menoth, A.; Mohan, D.; Abdallah, N.; El Rich, M.; Khalaf, K. Present and future of gait assessment in clinical practice: Towards the application of novel trends and technologies. Front. Med. Technol. 2022, 4, 901331. [Google Scholar] [CrossRef]
- Bethoux, F.; Rogers, H.L.; Nolan, K.J.; Abrams, G.M.; Annaswamy, T.M.; Brandstater, M.; Browne, B.; Burnfield, J.M.; Feng, W.; Freed, M.J.; et al. The effects of peroneal nerve functional electrical stimulation versus ankle-foot orthosis in patients with chronic stroke: A randomized controlled trial. Neurorehabil. Neural Repair 2014, 28, 688–697. [Google Scholar] [CrossRef]
- Toro, B.; Nester, C.; Farren, P. A review of observational gait assessment in clinical practice. Physiother. Theory Pract. 2003, 19, 137–149. [Google Scholar] [CrossRef]
- Ferrarello, F.; Bianchi, V.A.M.; Baccini, M.; Rubbieri, G.; Mossello, E.; Cavallini, M.C.; Marchionni, N.; Di Bari, M. Tools for observational gait analysis in patients with stroke: A systematic review. Phys. Ther. 2013, 93, 1673–1685. [Google Scholar] [CrossRef]
- McGinley, J.L.; Baker, R.; Wolfe, R.; Morris, M.E. The reliability of three-dimensional kinematic gait measurements: A systematic review. Gait Posture 2009, 29, 360–369. [Google Scholar] [CrossRef]
- Saito, A.; Miyawaki, K.; Kizawa, S.; Kobayashi, Y. A study on estimating the knee joint angle during gait using the motion sensors (Focusing on the effect of centrifugal acceleration and tangential acceleration). Trans. JSME 2018, 84, 17–00488. [Google Scholar] [CrossRef]
- Sy, L.W.F.; Lovell, N.H.; Redmond, S.J. Estimating Lower Limb Kinematics Using a Lie Group Constrained Extended Kalman Filter with a Reduced Wearable IMU Count and Distance Measurements. Sensors 2020, 20, 6829. [Google Scholar] [CrossRef] [PubMed]
- Feldhege, F.; Mau-Moeller, A.; Lindner, T.; Hein, A.; Markschies, A.; Zettl, U.K.; Bader, R. Accuracy of a custom physical activity and knee angle measurement sensor system for patients with neuromuscular disorders and gait abnormalities. Sensors 2015, 15, 10734–10752. [Google Scholar] [CrossRef] [PubMed]
- Mahony, R.; Hamel, T.; Pflimlin, J.-M. Nonlinear Complementary Filters on the Special Orthogonal Group. IEEE Trans. Autom. Control 2008, 53, 1203–1218. [Google Scholar] [CrossRef]
- Madgwick, S.O.H.; Harrison, A.J.L.; Vaidyanathan, R. Estimation of IMU and MARG orientation using a gradient descent algorithm. In Proceedings of the 2011 IEEE International Conference on Rehabilitation Robotics, Zurich, Switzerland, 29 June–1 July 2011; pp. 1–7. [Google Scholar] [CrossRef]
- Jayasinghe, U.; Hwang, F.; Harwin, W.S. Comparing Loose Clothing-Mounted Sensors with Body-Mounted Sensors in the Analysis of Gait. Sensors 2022, 22, 6605. [Google Scholar] [CrossRef]
- Kaufmann, M.; Nüesch, C.; Clauss, M.; Pagenstert, G.; Eckardt, A.; Ilchmann, T.; Stoffel, K.; Mündermann, A.; Ismailidis, P. Functional assessment of total hip arthroplasty using inertial measurement units: Improvement in gait kinematics and association with patient-reported outcome measures. J. Orthop. Res. 2023, 41, 759–770. [Google Scholar] [CrossRef]
- Christensen, J.C.; Foreman, K.B.; LaStayo, P.C.; Marcus, R.L.; Pelt, C.E.; Mizner, R.L. Comparison of 2 Forms of Kinetic Biofeedback on the Immediate Correction of Knee Extensor Moment Asymmetry Following Total Knee Arthroplasty During Decline Gait. J. Orthop. Sports Phys. Ther. 2019, 49, 105–111. [Google Scholar] [CrossRef]
- Werner, C.; Easthope, C.A.; Curt, A.; Demkó, L. Towards a Mobile Gait Analysis for Patients with a Spinal Cord Injury: A Robust Algorithm Validated for Slow Gait Speeds. Sensors 2021, 21, 7381. [Google Scholar] [CrossRef]
- Aşuroğlu, T.; Açıcı, K.; Erdaş, Ç.B.; Toprak, M.K.; Erdem, H.; Oğul, H. Parkinson’s disease monitoring from gait analysis via foot-worn sensors. Biocybern. Biomed. Eng. 2018, 38, 760–772. [Google Scholar] [CrossRef]
- van Meulen, F.B.; Weenk, D.; Buurke, J.H.; van Beijnum, B.-J.F.; Veltink, P.H. Ambulatory assessment of gait balance after stroke using instrumented shoes. J. Neuroeng. Rehabil. 2016, 13, 48. [Google Scholar] [CrossRef][Green Version]
- Vu, H.T.T.; Dong, D.; Cao, H.-L.; Verstraten, T.; Lefeber, D.; Vanderborght, B.; Geeroms, J. A Review of Gait Phase Detection Algorithms for Lower Limb Prostheses. Sensors 2020, 20, 3972. [Google Scholar] [CrossRef]
- Quintero, H.A.; Farris, R.J.; Goldfarb, M. Control and implementation of a powered lower limb orthosis to aid gait in paraplegic individuals. IEEE Int. Conf. Rehabil. Robot. 2011, 2011, 5975481. [Google Scholar] [CrossRef]
- González-Graniel, E.; Mercado-Gutierrez, J.A.; Martínez-Díaz, S.; Castro-Liera, I.; Santillan-Mendez, I.M.; Yanez-Suarez, O.; Quiñones-Uriostegui, I.; Rodríguez-Reyes, G. Sensing and Control Strategies Used in FES Systems Aimed at Assistance and Rehabilitation of Foot Drop: A Systematic Literature Review. J. Pers. Med. 2024, 14, 874. [Google Scholar] [CrossRef] [PubMed]
- de Jong, L.A.F.; Kerkum, Y.; van Oorschot, W.; Keijsers, N.L.W. A single Inertial Measurement Unit on the shank to assess the Shank-to-Vertical Angle. J. Biomech. 2020, 108, 109895. [Google Scholar] [CrossRef] [PubMed]
- Jaqueline da Cunha, M.; Rech, K.D.; Salazar, A.P.; Pagnussat, A.S. Functional electrical stimulation of the peroneal nerve improves post-stroke gait speed when combined with physiotherapy. A systematic review and meta-analysis. Ann. Phys. Rehabil. Med. 2021, 64, 101388. [Google Scholar] [CrossRef] [PubMed]
- Boudarham, J.; Roche, N.; Pradon, D.; Bonnyaud, C.; Bensmail, D.; Zory, R. Variations in kinematics during clinical gait analysis in stroke patients. PLoS ONE 2013, 8, e66421. [Google Scholar] [CrossRef]
- Cooper, G.; Sheret, I.; McMillian, L.; Siliverdis, K.; Sha, N.; Hodgins, D.; Kenney, L.; Howard, D. Inertial sensor-based knee flexion/extension angle estimation. J. Biomech. 2009, 42, 2678–2685. [Google Scholar] [CrossRef]
- Laudanski, A.; Brouwer, B.; Li, Q. Measurement of lower limb joint kinematics using inertial sensors during stair ascent and descent in healthy older adults and stroke survivors. J. Health Eng. 2013, 4, 555–576. [Google Scholar] [CrossRef]
- Kong, W.; Sessa, S.; Cosentino, S.; Zecca, M.; Saito, K.; Wang, C.; Imtiaz, U.; Lin, Z.; Bartolomeo, L.; Ishii, H.; et al. Development of a real-time IMU-based motion capture system for gait rehabilitation. In Proceedings of the 2013 IEEE International Conference on Robotics and Biomimetics (ROBIO), Shenzhen, China, 12–14 December 2013; pp. 2100–2105. [Google Scholar]
- Palermo, E.; Rossi, S.; Marini, F.; Patané, F.; Cappa, P. Experimental evaluation of accuracy and repeatability of a novel body-to-sensor calibration procedure for inertial sensor-based gait analysis. Measurement 2014, 52, 145–155. [Google Scholar] [CrossRef]
- Jiang, C.; Yang, Y.; Mao, H.; Yang, D.; Wang, W. Effects of Dynamic IMU-to-Segment Misalignment Error on 3-DOF Knee Angle Estimation in Gait and Running. Sensors 2022, 22, 9009. [Google Scholar] [CrossRef]
- Mascia, G.; Brasiliano, P.; Di Feo, P.; Cereatti, A.; Camomilla, V. A functional calibration protocol for ankle plantar-dorsiflexion estimate using magnetic and inertial measurement units: Repeatability and reliability assessment. J. Biomech. 2022, 141, 111202. [Google Scholar] [CrossRef]
- O’donovan, K.J.; Kamnik, R.; O’keeffe, D.T.; Lyons, G.M. An inertial and magnetic sensor based technique for joint angle measurement. J. Biomech. 2007, 40, 2604–2611. [Google Scholar] [CrossRef]
- Tadano, S.; Takeda, R.; Miyagawa, H. Three dimensional gait analysis using wearable acceleration and gyro sensors based on quaternion calculations. Sensors 2013, 13, 9321–9343. [Google Scholar] [CrossRef] [PubMed]
- Miyazaki, T.; Kawada, M.; Nakai, Y.; Kiyama, R.; Yone, K. Validity of Measurement for Trailing Limb Angle and Propulsion Force during Gait Using a Magnetic Inertial Measurement Unit. Biomed. Res. Int. 2019, 2019, 8123467. [Google Scholar] [CrossRef] [PubMed]
- Rhudy, M.B.; Mahoney, J.M.; Altman-Singles, A.R. Knee Angle Estimation with Dynamic Calibration Using Inertial Measurement Units for Running. Sensors 2024, 24, 695. [Google Scholar] [CrossRef]
- Cornish, B.M.; Diamond, L.E.; Saxby, D.J.; Lloyd, D.G.; Shi, B.; Lyon, J.; Abbruzzese, K.; Gallie, P.; Maharaj, J. Sagittal plane knee kinematics can be measured during activities of daily living following total knee arthroplasty with two IMU. PLoS ONE 2024, 19, e0297899. [Google Scholar] [CrossRef]
- Reenalda, J.; Maartens, E.; Buurke, J.H.; Gruber, A.H. Kinematics and shock attenuation during a prolonged run on the athletic track as measured with inertial magnetic measurement units. Gait Posture 2019, 68, 155–160. [Google Scholar] [CrossRef]
- Kianifar, R.; Joukov, V.; Lee, A.; Raina, S.; Kulić, D. Inertial measurement unit-based pose estimation: Analyzing and reducing sensitivity to sensor placement and body measures. J. Rehabil. Assist. Technol. Eng. 2019, 6, 2055668318813455. [Google Scholar] [CrossRef]
- Haji Hassani, R.; Willi, R.; Rauter, G.; Bolliger, M.; Seel, T. Validation of Non-Restrictive Inertial Gait Analysis of Individuals with Incomplete Spinal Cord Injury in Clinical Settings. Sensors 2022, 22, 4237. [Google Scholar] [CrossRef]
- Niswander, W.; Wang, W.; Kontson, K. Optimization of IMU Sensor Placement for the Measurement of Lower Limb Joint Kinematics. Sensors 2020, 20, 5993. [Google Scholar] [CrossRef]
- Blandeau, M.; Guichard, R.; Hubaut, R.; Leteneur, S. IMU positioning affects range of motion measurement during squat motion analysis. J. Biomech. 2023, 153, 111598. [Google Scholar] [CrossRef]
- Pacini Panebianco, G.; Bisi, M.C.; Stagni, R.; Fantozzi, S. Analysis of the performance of 17 algorithms from a systematic review: Influence of sensor position, analysed variable and computational approach in gait timing estimation from IMU measurements. Gait Posture 2018, 66, 76–82. [Google Scholar] [CrossRef]
- Anwary, A.R.; Yu, H.; Vassallo, M. Optimal Foot Location for Placing Wearable IMU Sensors and Automatic Feature Extraction for Gait Analysis. IEEE Sens. J. 2018, 18, 2555–2567. [Google Scholar] [CrossRef]
- Seel, T.; Raisch, J.; Schauer, T. IMU-based joint angle measurement for gait analysis. Sensors 2014, 14, 6891–6909. [Google Scholar] [CrossRef] [PubMed]
- Peters, A.; Galna, B.; Sangeux, M.; Morris, M.; Baker, R. Quantification of soft tissue artifact in lower limb human motion analysis: A systematic review. Gait Posture 2010, 31, 1–8. [Google Scholar] [CrossRef]
- Fiorentino, N.M.; Atkins, P.R.; Kutschke, M.J.; Goebel, J.M.; Foreman, K.B.; Anderson, A.E. Soft tissue artifact causes significant errors in the calculation of joint angles and range of motion at the hip. Gait Posture 2017, 55, 184–190. [Google Scholar] [CrossRef]
- D’Isidoro, F.; Brockmann, C.; Ferguson, S.J. Effects of the soft tissue artefact on the hip joint kinematics during unrestricted activities of daily living. J. Biomech. 2020, 104, 109717. [Google Scholar] [CrossRef]
- Yoshida, Y.; Matsumura, N.; Yamada, Y.; Yamada, M.; Yokoyama, Y.; Miyamoto, A.; Nakamura, M.; Nagura, T.; Jinzaki, M. Three-Dimensional Quantitative Evaluation of the Scapular Skin Marker Movements in the Upright Posture. Sensors 2022, 22, 6502. [Google Scholar] [CrossRef]
- Wang, Y.; Guo, J.; Tang, H.; Li, X.; Guo, S.; Tian, Q. Quantification of soft tissue artifacts using CT registration and subject-specific multibody modeling. J. Biomech. 2024, 162, 111893. [Google Scholar] [CrossRef]
- Lahkar, B.K.; Rohan, P.-Y.; Assi, A.; Pillet, H.; Bonnet, X.; Thoreux, P.; Skalli, W. Development and evaluation of a new methodology for Soft Tissue Artifact compensation in the lower limb. J. Biomech. 2021, 122, 110464. [Google Scholar] [CrossRef]
- Einfeldt, A.-K.; Budde, L.; Ortigas-Vásquez, A.; Sauer, A.; Utz, M.; Jakubowitz, E. A new method called MiKneeSoTA to minimize knee soft-tissue artifacts in kinematic analysis. Sci. Rep. 2024, 14, 20666. [Google Scholar] [CrossRef]
- Li, F.; Liu, G.; Liu, J.; Chen, X.; Ma, X. 3D Tracking via Shoe Sensing. Sensors 2016, 16, 1809. [Google Scholar] [CrossRef]
- de Vries, W.H.; Veeger, H.E.; Baten, C.T.; van der Helm, F.C. Magnetic distortion in motion labs, implications for validating inertial magnetic sensors. Gait Posture 2009, 29, 535–541. [Google Scholar] [CrossRef] [PubMed]
- Manon, K.; Thomas, B.S. Magnetometer Calibration Using Inertial Sensors. IEEE Sensors J. 2016, 16, 5679–5689. [Google Scholar] [CrossRef]
- Lebleu, J.; Gosseye, T.; Detrembleur, C.; Mahaudens, P.; Cartiaux, O.; Penta, M. Lower Limb Kinematics Using Inertial Sensors during Locomotion: Accuracy and Reproducibility of Joint Angle Calculations with Different Sensor-to-Segment Calibrations. Sensors 2020, 20, 715. [Google Scholar] [CrossRef]
- Vitali, R.V.; Perkins, N.C. Determining anatomical frames via inertial motion capture: A survey of methods. J. Biomech. 2020, 106, 109832. [Google Scholar] [CrossRef]
- Favre, J.; Jolles, B.M.; Aissaoui, R.; Aminian, K. Ambulatory measurement of 3D knee joint angle. J. Biomech. 2008, 41, 1029–1035. [Google Scholar] [CrossRef]
- Zhu, K.; Li, J.; Li, D.; Fan, B.; Shull, P.B. IMU Shoulder Angle Estimation: Effects of Sensor-to-Segment Misalignment and Sensor Orientation Error. IEEE Trans. Neural Syst. Rehabil. Eng. 2023, 31, 4481–4491. [Google Scholar] [CrossRef]
- Teruyama, Y.; Watanabe, T. Effectiveness of variable-gain Kalman filter based on angle error calculated from acceleration signals in lower limb angle measurement with inertial sensors. Comput. Math. Methods Med. 2013, 2013, 398042. [Google Scholar] [CrossRef]
- Watanabe, T.; Teruyama, Y.; Ohashi, K. Comparison of Angle Measurements Between Integral-Based and Quaternion-Based Methods Using Inertial Sensors for Gait Evaluation. In Biomedical Engineering Systems and Technologies. BIOSTEC 2014. Communications in Computer and Information Science; Plantier, G., Schultz, T., Fred, A., Gamboa, H., Eds.; Springer: Berlin/Heidelberg, Germany, 2015; Volume 511. [Google Scholar] [CrossRef]
- Weygers, I.; Kok, M.; Konings, M.; Hallez, H.; De Vroey, H.; Claeys, K. Inertial Sensor-Based Lower Limb Joint Kinematics: A Methodological Systematic Review. Sensors 2020, 20, 673. [Google Scholar] [CrossRef]
- Routhier, F.; Duclos, N.C.; Lacroix, É.; Lettre, J.; Turcotte, E.; Hamel, N.; Michaud, F.; Duclos, C.; Archambault, P.S.; Bouyer, L.J. Clinicians’ perspectives on inertial measurement units in clinical practice. PLoS ONE 2020, 15, e0241922. [Google Scholar] [CrossRef]




| MIMU Mounting Position | Year | Author | Reference |
|---|---|---|---|
| Lateral | 2009 | Cooper G et al. | [26] |
| 2013 | Laudanski A et al. | [27] | |
| 2013 | Kong W et al. | [28] | |
| 2014 | Palermo E et al. | [29] | |
| 2022 | Jiang C et al. | [30] | |
| 2022 | Mascia G et al. | [31] | |
| Anterior | 2007 | O’Donovan KJ et al. | [32] |
| 2013 | Tadano S et al. | [33] | |
| 2019 | Miyazaki T et al. | [34] | |
| 2022 | Rhudy MB et al. | [35] | |
| 2024 | Cornish BM et al. | [36] | |
| Medial tibia | 2019 | Reenalda J et al. | [37] |
| 2019 | Kianifar R et al. | [38] | |
| 2022 | Haji Hassani R et al. | [39] |
| Accelerometer | Gyro Sensor | Magnetic Sensor [AK09916] | |
|---|---|---|---|
| Measuring range | ±4 g | ±1000°/s | ±4900 μT |
| Resolution | 16 bit | 16 bit | 16 bit |
| Sensitivity (1 LSB) | 0.12 mg | 0.03°/s | 0.15 μT |
| Noise | 230 μg/√Hz | 0.015°/√Hz | / |
| LPF | 111.4 Hz | 361.4 Hz | / |
| Subject | Age [years] | Sex | Height [m] | Weight [kg] | Thigh Length [m] | Shank Length [m] |
|---|---|---|---|---|---|---|
| S1 | 23 | Man | 1.76 | 64 | 0.45 | 0.39 |
| S2 | 23 | Man | 1.72 | 77 | 0.41 | 0.38 |
| S3 | 22 | Man | 1.81 | 68 | 0.48 | 0.41 |
| S4 | 24 | Woman | 1.49 | 41 | 0.39 | 0.34 |
| S5 | 22 | Woman | 1.57 | 49 | 0.43 | 0.33 |
| S6 | 22 | Woman | 1.53 | 46 | 0.38 | 0.33 |
| Average | 23 ± 1 | / | 1.65 ± 0.13 | 58 ± 14 | 0.42 ± 0.04 | 0.36 ± 0.03 |
| Dorsiflexion–Relaxation | Plantarflexion–Relaxation | |||||
|---|---|---|---|---|---|---|
| Lateral | Anterior | Medial Tibia | Lateral | Anterior | Medial Tibia | |
| Men | 4.8 ± 1.6 | 20.0 ± 0.9 | 3.7 ± 0.1 | 6.7 ± 1.6 | 4.4 ± 1.2 | 3.5 ± 1.2 |
| Women | 3.8 ± 0.9 | 12.6 ± 4.9 | 2.8 ± 0.5 | 3.9 ± 1.1 | 3.4 ± 1.8 | 2.5 ± 0.9 |
| All | 4.3 ± 1.3 | 16.3 ± 5.2 | 3.3 ± 0.6 | 5.3 ± 2.0 | 3.9 ± 1.5 | 3.0 ± 1.1 |
| Sagittal Plane | Frontal Plane | Horizontal Plane | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Lateral | Anterior | Medial Tibia | Lateral | Anterior | Medial Tibia | Lateral | Anterior | Medial Tibia | |
| Slow | 1.7 | 2.5 | 2.3 | 1.9 | 2.0 | 1.5 | 3.5 | 2.9 | 3.0 |
| Medium | 2.2 | 2.8 | 2.6 | 2.5 | 2.5 | 2.0 | 4.1 | 3.7 | 3.6 |
| Fast | 2.3 | 3.0 | 2.5 | 2.7 | 2.7 | 2.1 | 4.8 | 4.6 | 3.7 |
| All | 2.1 | 2.7 | 2.4 | 2.4 | 2.4 | 1.9 | 4.1 | 3.7 | 3.4 |
| Sagittal Plane | Frontal Plane | Horizontal Plane | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Lateral | Anterior | Medial Tibia | Lateral | Anterior | Medial Tibia | Lateral | Anterior | Medial Tibia | |
| Slow | 0.997 | 0.998 | 0.997 | 0.837 | 0.780 | 0.863 | 0.953 | 0.947 | 0.965 |
| Medium | 0.997 | 0.998 | 0.997 | 0.779 | 0.732 | 0.794 | 0.955 | 0.957 | 0.961 |
| Fast | 0.997 | 0.998 | 0.997 | 0.793 | 0.725 | 0.771 | 0.943 | 0.953 | 0.963 |
| All | 0.997 | 0.998 | 0.997 | 0.803 | 0.746 | 0.809 | 0.950 | 0.952 | 0.963 |
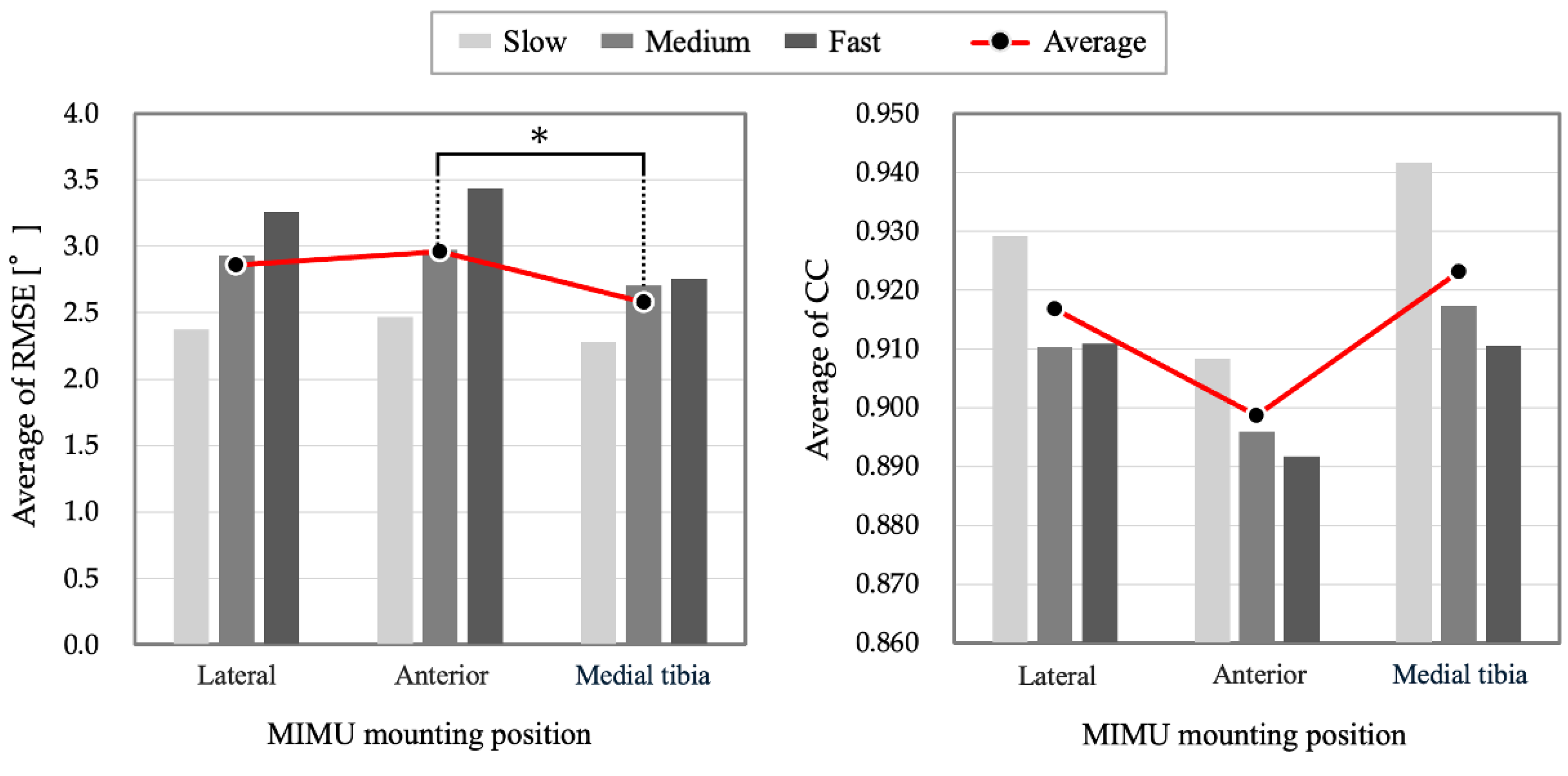
| Average RMSE (Sagittal, Frontal, and Horizontal Planes) | Average CC (Sagittal, Frontal, and Horizontal Planes) | |||||
|---|---|---|---|---|---|---|
| Lateral | Anterior | Medial Tibia | Lateral | Anterior | Medial Tibia | |
| Slow | 2.4 | 2.5 | 2.3 | 0.929 | 0.908 | 0.942 |
| Medium | 2.9 | 3.0 | 2.7 | 0.910 | 0.896 | 0.917 |
| Fast | 3.3 | 3.4 | 2.8 | 0.911 | 0.892 | 0.910 |
| All | 2.9 | 3.0 | 2.6 | 0.917 | 0.899 | 0.923 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kanega, S.; Muraoka, Y. Optimization of MIMU Mounting Position on Shank in Posture Estimation Considering Muscle Protuberance. Sensors 2025, 25, 2273. https://doi.org/10.3390/s25072273
Kanega S, Muraoka Y. Optimization of MIMU Mounting Position on Shank in Posture Estimation Considering Muscle Protuberance. Sensors. 2025; 25(7):2273. https://doi.org/10.3390/s25072273
Chicago/Turabian StyleKanega, Shun, and Yoshihiro Muraoka. 2025. "Optimization of MIMU Mounting Position on Shank in Posture Estimation Considering Muscle Protuberance" Sensors 25, no. 7: 2273. https://doi.org/10.3390/s25072273
APA StyleKanega, S., & Muraoka, Y. (2025). Optimization of MIMU Mounting Position on Shank in Posture Estimation Considering Muscle Protuberance. Sensors, 25(7), 2273. https://doi.org/10.3390/s25072273







