All-Metal Metamaterial-Based Sensor with Novel Geometry and Enhanced Sensing Capability at Terahertz Frequency
Abstract
1. Introduction
2. Materials and Methods
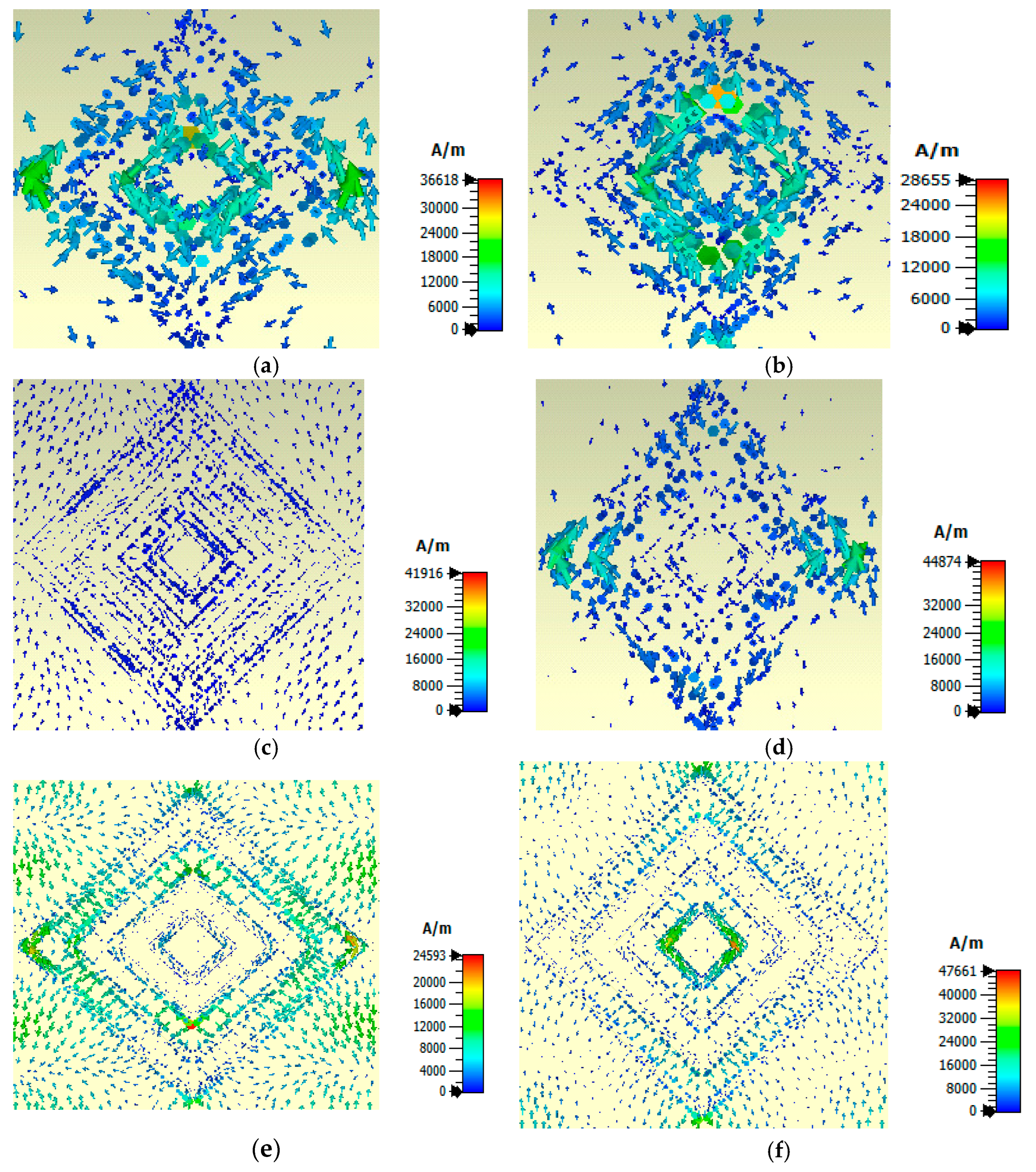
3. Results and Discussions
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| MMA | Metamaterial Absorber |
| RIU | Refractive Index Unit |
| FWHM | Full Width Half Maximum |
| FoM | Figure of Merit |
| Q-factor | Quality Factor |
| m-MIMO | massive multiple-input multiple-output |
References
- Ramakrishna, S.A.; Grzegorczyk, T.M. Physics and Applications of Negative Refractive Index Materials; CRC Press: Boca Raton, FL, USA, 2008. [Google Scholar]
- Banerjee, S.; Dutta, P.; Jha, A.V.; Appasani, B.; Khan, M.S. A Biomedical Sensor for Detection of Cancer Cells Based on Terahertz Metamaterial Absorber. IEEE Sensors Lett. 2022, 6, 6002004. [Google Scholar] [CrossRef]
- Grzegorczyk, T.M.; Moss, C.D.; Lu, J.; Chen, X.; Pacheco, J.; Kong, J.A. Properties of left-handed metamaterials: Transmission, backward phase, negative refraction, and focusing. IEEE Trans. Microw. Theory Tech. 2005, 53, 2956–2967. [Google Scholar] [CrossRef]
- Qiu, Y.; Zhang, P.; Li, Q.; Zhang, Y.; Li, W. A perfect selective metamaterial absorber for high-temperature solar energy harvesting. Sol. Energy 2021, 230, 1165–1174. [Google Scholar] [CrossRef]
- Liu, W.; Song, Z. Terahertz absorption modulator with largely tunable bandwidth and intensity. Carbon 2020, 174, 617–624. [Google Scholar] [CrossRef]
- Yue, L.; Wang, Y.; Cui, Z.; Zhang, X.; Zhu, Y.; Zhang, X.; Chen, S.; Wang, X.; Zhang, K. Multi-band terahertz resonant absorption based on an all-dielectric grating metasurface for chlorpyrifos sensing. Opt. Express 2021, 29, 13563–13575. [Google Scholar] [CrossRef]
- Yan, X.; Liang, L.-J.; Ding, X.; Yao, J.-Q. Solid analyte and aqueous solutions sensing based on a flexible terahertz dual-band metamaterial absorber. Opt. Eng. 2017, 56, 027104. [Google Scholar] [CrossRef]
- Janneh, M.; De Marcellis, A.; Palange, E.; Tenggara, A.T.; Byun, D. Design of a metasurface-based dual-band Terahertz perfect absorber with very high Q-factors for sensing applications. Opt. Commun. 2018, 416, 152–159. [Google Scholar] [CrossRef]
- Xiong, H.; Ma, X.; Zhang, H. Wave-thermal effect of a temperature-tunable terahertz absorber. Opt. Express 2021, 29, 38557–38566. [Google Scholar] [CrossRef]
- Wu, L.; Yang, L.; Zhu, X.; Cai, B.; Cheng, Y. Ultra-broadband and wide-angle plasmonic absorber based on all-dielectric gallium arsenide pyramid nanostructure for full solar radiation spectrum range. Int. J. Therm. Sci. 2024, 201, 109043. [Google Scholar] [CrossRef]
- Jiang, L.; Yuan, C.; Li, Z.; Su, J.; Yi, Z.; Yao, W.; Wu, P.; Liu, Z.; Cheng, S.; Pan, M. Multi-band and high-sensitivity perfect absorber based on monolayer graphene metamaterial. Diam. Relat. Mater. 2020, 111, 108227. [Google Scholar] [CrossRef]
- Cai, B.; Wu, L.; Zhu, X.; Cheng, Z.; Cheng, Y. Ultra-broadband and wide-angle plasmonic light absorber based on all-dielectric gallium arsenide (GaAs) metasurface in visible and near-infrared region. Results Phys. 2024, 58, 107509. [Google Scholar] [CrossRef]
- Li, J.; Hu, G.; Shi, L.; He, N.; Li, D.; Shang, Q.; Zhang, Q.; Fu, H.; Zhou, L.; Xiong, W.; et al. Full-color enhanced second harmonic generation using rainbow trapping in ultrathin hyperbolic metamaterials. Nat. Commun. 2021, 12, 6425. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Xu, X.; Lin, Y.-S. Terahertz meta-absorber with tunable single- and dual-resonance characteristics. Phys. E Low-Dimens. Syst. Nanostructures 2022, 142, 115274. [Google Scholar] [CrossRef]
- Cheng, Y.; Zhao, J. Simple design of a six-band terahertz perfect metasurface absorber based on a single resonator structure. Phys. Scr. 2022, 97, 095508. [Google Scholar] [CrossRef]
- Zhang, S.; Lai, S.; Lu, L.; Yang, Z.; Wang, K.; Feng, S.; Wu, Y.; Zhu, H.; Wang, B.-X. Triple-band terahertz metamaterial absorber using the nesting structure of two pairs of splitting arcs. Mod. Phys. Lett. B 2022, 36, 2250076. [Google Scholar] [CrossRef]
- Wu, Q.; Ling, F.; Zhang, C.; Zhong, Z.; Zhang, B. Water-based metamaterials absorber with broadband absorption in terahertz region. Opt. Commun. 2022, 526, 128874. [Google Scholar] [CrossRef]
- Cheng, Y.; Liu, J.; Chen, F.; Luo, H.; Li, X. Optically switchable broadband metasurface absorber based on square ring shaped photoconductive silicon for terahertz waves. Phys. Lett. A 2021, 402, 127345. [Google Scholar] [CrossRef]
- Feng, G.; Chen, Z.; Wang, X.; Liu, X.; Sun, F.; Yang, Y. Ultra-broadband terahertz absorber based on double truncated pyramid structure. Mater. Today Commun. 2022, 31, 103624. [Google Scholar] [CrossRef]
- Yu, W.; Wang, X.; Lu, H.; Liu, H.; Jin, C. 3D-Printed All-Metal Terahertz Multibeam Lens Antenna Based on Photonic Crystal. IEEE Access 2023, 11, 41609–41617. [Google Scholar] [CrossRef]
- Zhang, R.; Wu, X.; Lou, Y.; Yan, F.-G.; Zhou, Z.; Wu, W.; Yuen, C. Channel Training-Aided Target Sensing for Terahertz Integrated Sensing and Massive MIMO Communications. IEEE Internet Things J. 2024. [Google Scholar] [CrossRef]
- Zhang, Z.; Xie, Q.; Guo, L.; Su, C.; Wang, M.; Xia, F.; Sun, J.; Li, K.; Feng, H.; Yun, M. Dual-controlled tunable dual-band and ultra-broadband coherent perfect absorber in the THz range. Opt. Express 2022, 30, 30832–30844. [Google Scholar] [CrossRef] [PubMed]
- You, L.; Xuan, W. Dual-band polarization-sensitive terahertz metamaterial absorber possessing frequency and amplitude dynamically-continuously tunable characteristics. Ferroelectrics 2022, 593, 174–180. [Google Scholar] [CrossRef]
- Zhan, Y.; Yin, H.; Wang, J.; Yao, H.; Fan, C. Tunable multiple band THz perfect absorber with InSb metamaterial for enhanced sensing application. Results Opt. 2022, 8, 100255. [Google Scholar] [CrossRef]
- Fan, Y.; Yang, R.; Li, Z.; Zhao, Y.; Tian, J.; Zhang, W. Narrowband metamaterial absorbers based on interlaced T-shaped all-dielectric resonators for sensing application. JOSA B 2022, 39, 2863–2869. [Google Scholar] [CrossRef]
- Liu, X.; Fan, K.; Shadrivov, I.V.; Padilla, W.J. Experimental realization of a terahertz all-dielectric metasurface absorber. Opt. Express 2017, 25, 191–201. [Google Scholar] [CrossRef]
- Li, A.; Dong, J.; Wang, J.; Cheng, Z.; Ho, J.S.; Zhang, D.; Wen, J.; Zhang, X.-L.; Chan, C.T.; Alù, A.; et al. Hamiltonian hopping for efficient chiral mode switching in encircling exceptional points. Phys. Rev. Lett. 2020, 125, 187403. [Google Scholar] [CrossRef]
- Cheng, Y.; Qian, Y.; Luo, H.; Chen, F.; Cheng, Z. Terahertz narrowband perfect metasurface absorber based on micro-ring-shaped GaAs array for enhanced refractive index sensing. Phys. E Low-Dimens. Syst. Nanostructures 2023, 146, 115527. [Google Scholar] [CrossRef]
- Huang, Z.; Zheng, Y.; Li, J.; Cheng, Y.; Wang, J.; Zhou, Z.-K.; Chen, L. High-resolution metalens imaging polarimetry. Nano Lett. 2023, 23, 10991–10997. [Google Scholar] [CrossRef]
- Chen, F.; Cheng, Y.; Luo, H. Temperature tunable narrow-band terahertz metasurface absorber based on InSb micro-cylinder arrays for enhanced sensing application. IEEE Access 2020, 8, 82981–82988. [Google Scholar] [CrossRef]
- Li, S.; Zhang, L.; Chen, X. 3D-printed terahertz metamaterial absorber based on vertical split-ring resonator. J. Appl. Phys. 2021, 130, 034504. [Google Scholar] [CrossRef]
- Barzegar-Parizi, S.; Ebrahimi, A. Terahertz all metallic perfect absorber for refractive index sensing and glucose concentration detection. Phys. Scr. 2022, 98, 015504. [Google Scholar] [CrossRef]
- Banerjee, S.; Ghosh, I.; Fahad, M.B.; Mishra, S.K.; Yadav, R.; Appasani, B. Ultra Thin Highly Sensitive Metamaterial Absorber Based Refractive Index Sensor for Detecting Adulterants in Alcohol. Prog. Electromagn. Res. M 2024, 126, 81–88. [Google Scholar] [CrossRef]
- Banerjee, S.; Nath, U.; Dutta, P.; Jha, A.V.; Appasani, B.; Bizon, N. A theoretical terahertz metamaterial absorber structure with a high quality factor using two circular ring resonators for biomedical sensing. Inventions 2021, 6, 78. [Google Scholar] [CrossRef]
- Cheng, Y.; Zhang, H.; Mao, X.S.; Gong, R. Dual-band plasmonic perfect absorber based on all-metal nanostructure for refractive index sensing application. Mater. Lett. 2018, 219, 123–126. [Google Scholar] [CrossRef]
- Teber, A. A Dual-Band Terahertz Metamaterial Absorber Using an All-Metal Aluminum Hexagonal Metasurface Structure for Sensing of Cancerous Cells. J. Electron. Mater. 2024, 53, 2686–2701. [Google Scholar] [CrossRef]
- Zhang, H.; Cheng, Y.; Chen, F. Quad-band plasmonic perfect absorber using all-metal nanostructure metasurface for refractive index sensing. Optik 2021, 229, 166300. [Google Scholar] [CrossRef]
- Yu, J.; Lang, T.; Chen, H. All-metal terahertz metamaterial absorber and refractive index sensing performance. Photonics 2021, 8, 164. [Google Scholar] [CrossRef]
- Cai, B.; Yang, L.; Wu, L.; Cheng, Y.; Li, X. Dual-narrowband terahertz metamaterial absorber based on all-metal vertical ring array for enhanced sensing application. Phys. Scr. 2024, 99, 095503. [Google Scholar] [CrossRef]
- Huang, X.; Ye, W.; Ran, J.; Zhou, Z.; Li, R.; Gao, B. Highly Sensitive Biosensor Based on Metamaterial Absorber With an All-Metal Structure. IEEE Sensors J. 2023, 23, 3573–3580. [Google Scholar] [CrossRef]
- Appasani, B. An octaband temperature tunable terahertz metamaterial absorber using tapered triangular structures. Prog. Electromagn. Res. Lett. 2021, 95, 9–16. [Google Scholar] [CrossRef]
- Wartak, M.S.; Tsakmakidis, K.L.; Hess, O. Introduction to metamaterials. Phys. Can 2011, 67, 30–34. [Google Scholar]
- Anwar, S.; Khan, S. Detection of Toxic Gases Based on Refractive Index Sensor for Gas-Sensing Applications. Plasmonics 2024. [Google Scholar] [CrossRef]
- Ajayan, J.; Sreejith, S.; Manikandan, M.; Lai, W.-C.; Saha, S. Terahertz sensors for next generation biomedical and other industrial electronics applications: A critical review. Sens. Actuators A: Phys. 2024, 369, 115169. [Google Scholar] [CrossRef]
- Citroni, R.; Mangini, F.; Frezza, F. Efficient Integration of Ultra-low Power Techniques and Energy Harvesting in Self-Sufficient Devices: A Comprehensive Overview of Current Progress and Future Directions. Sensors 2024, 24, 4471. [Google Scholar] [CrossRef]
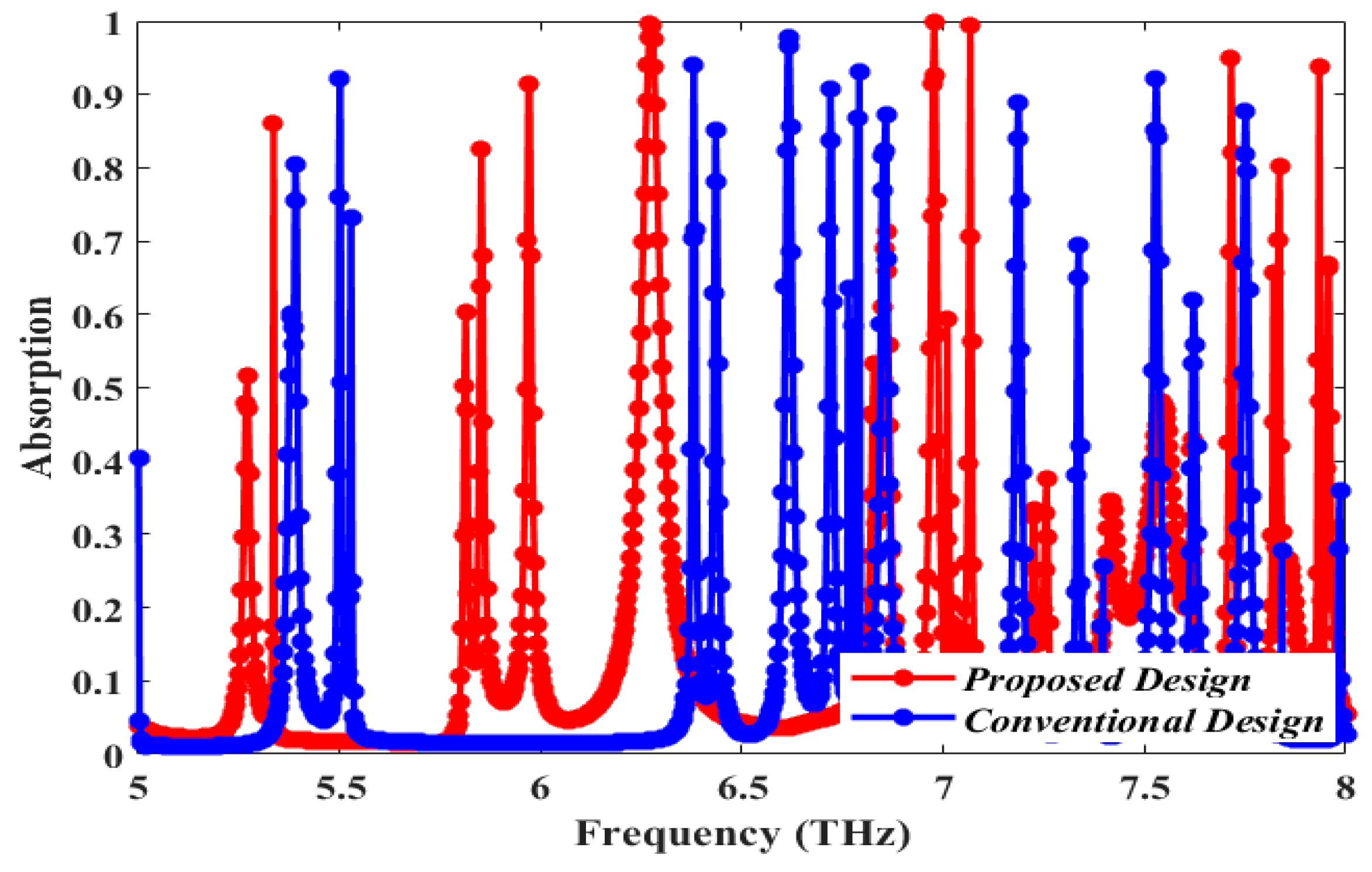

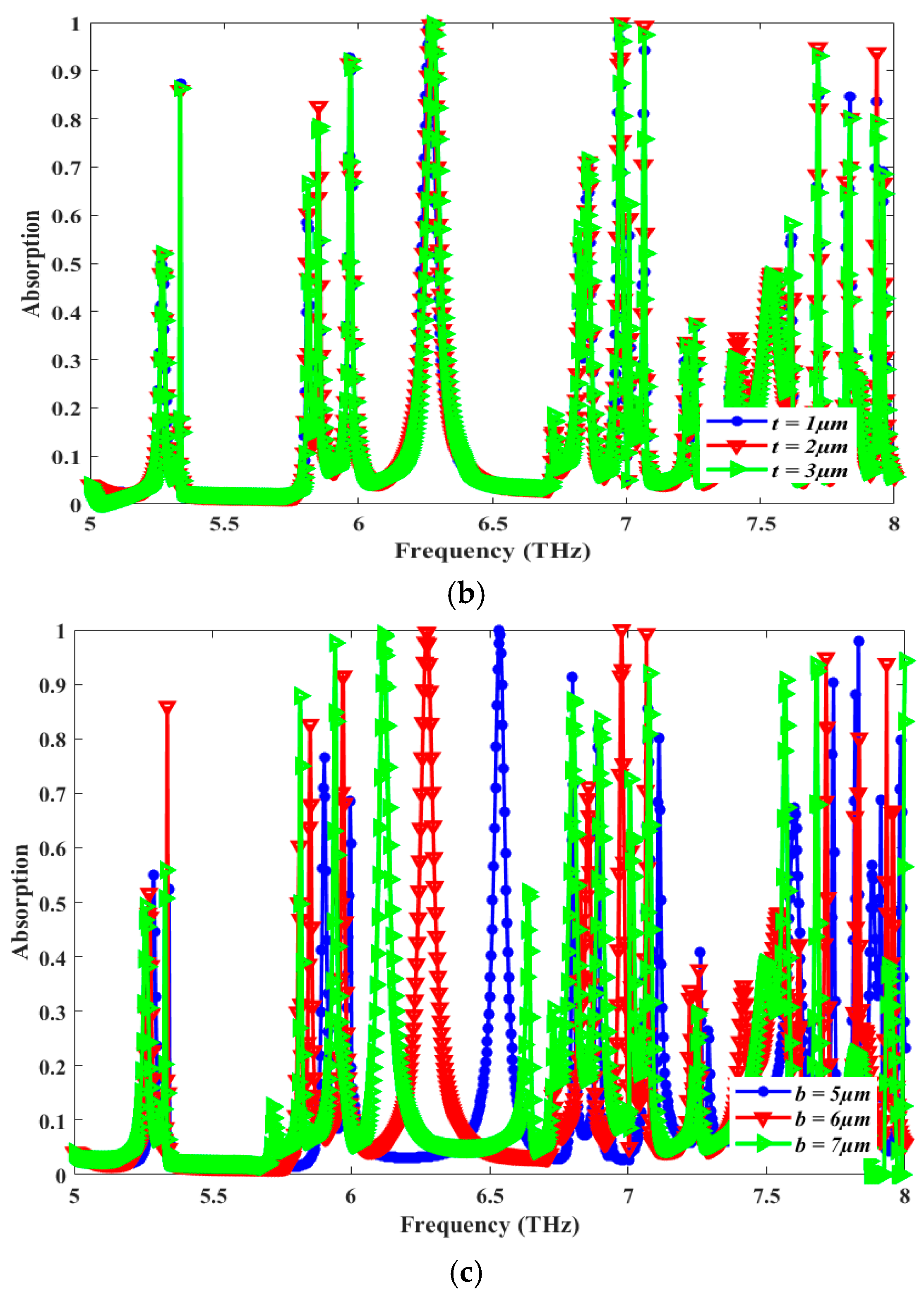
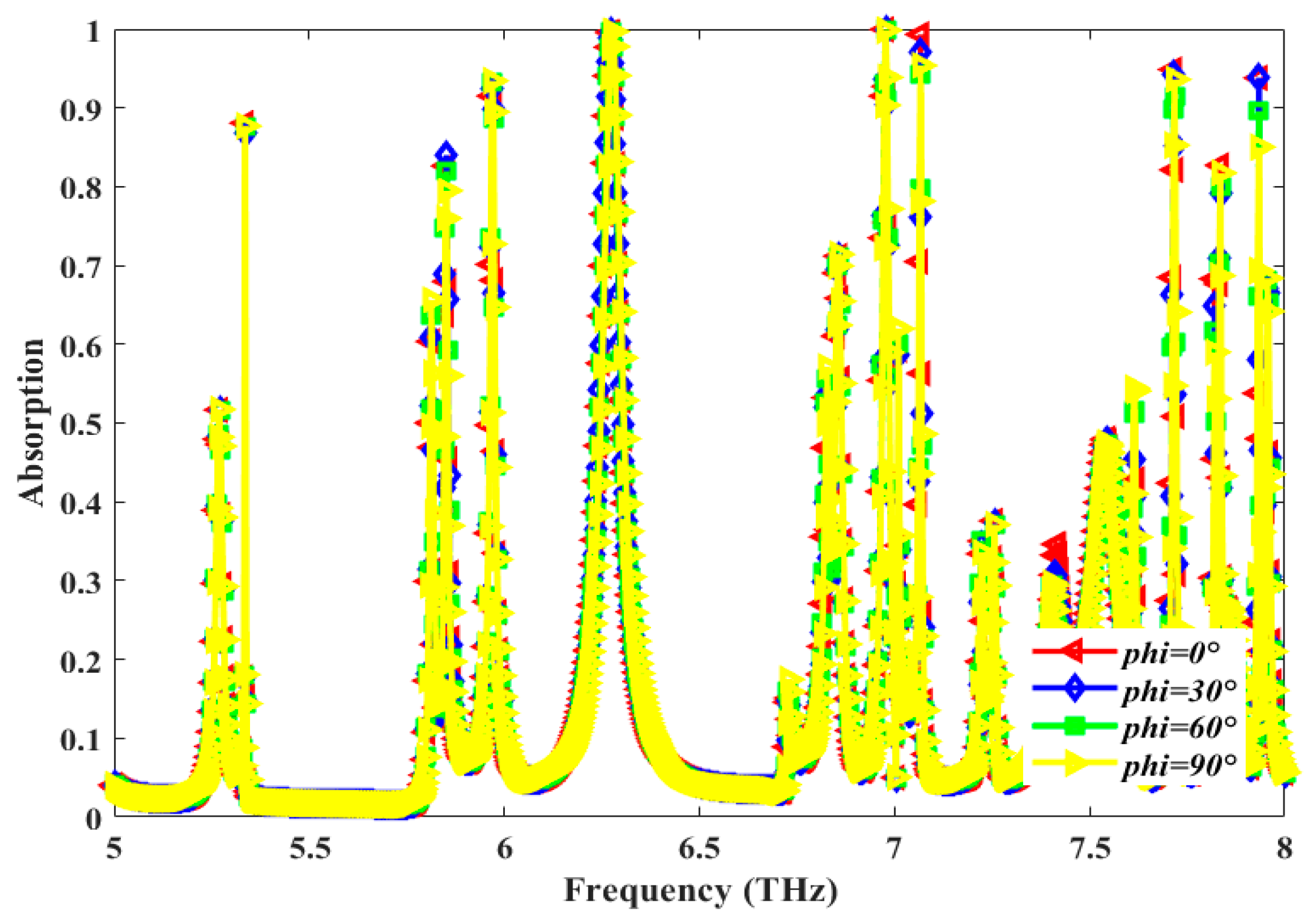
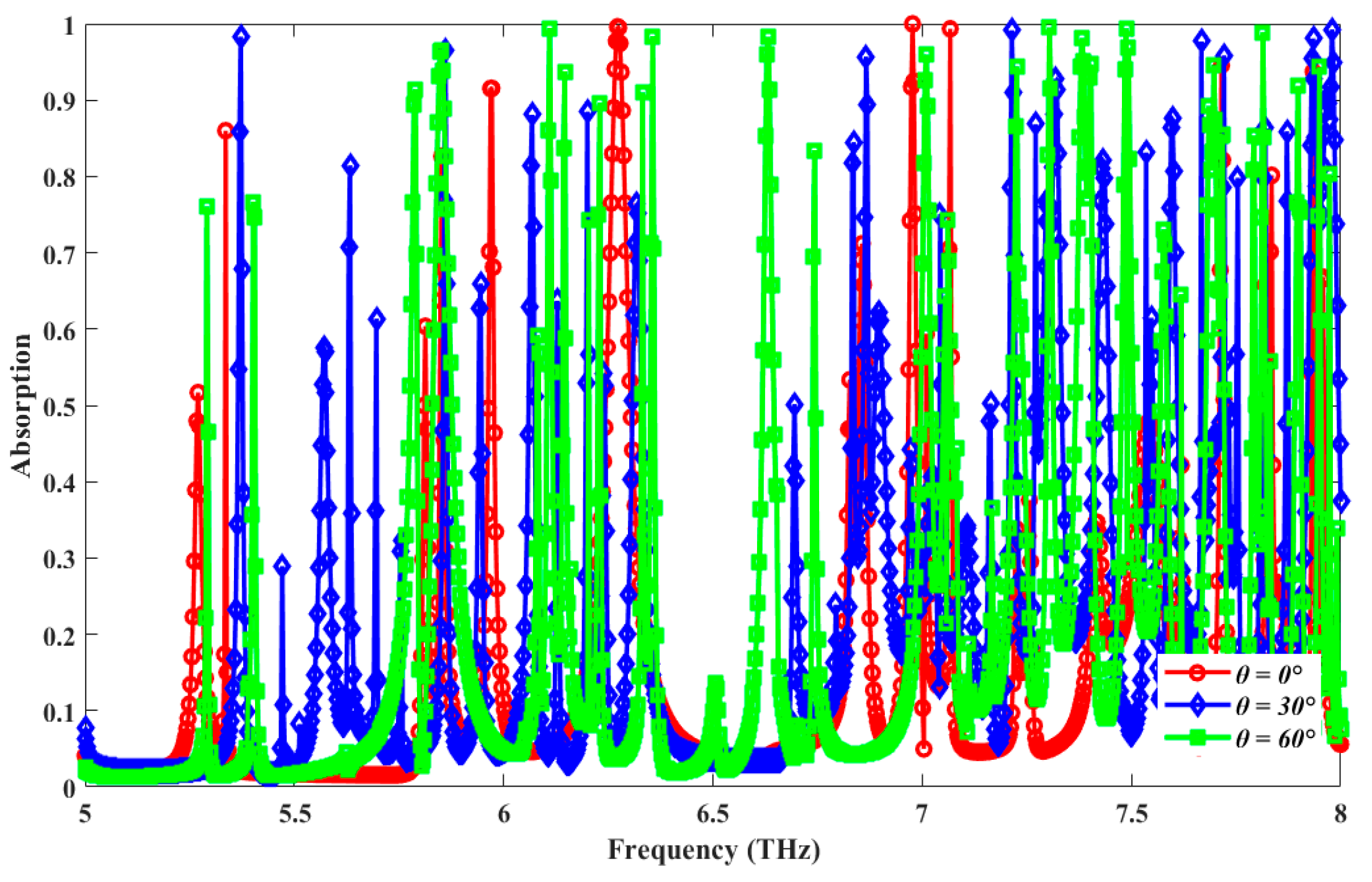
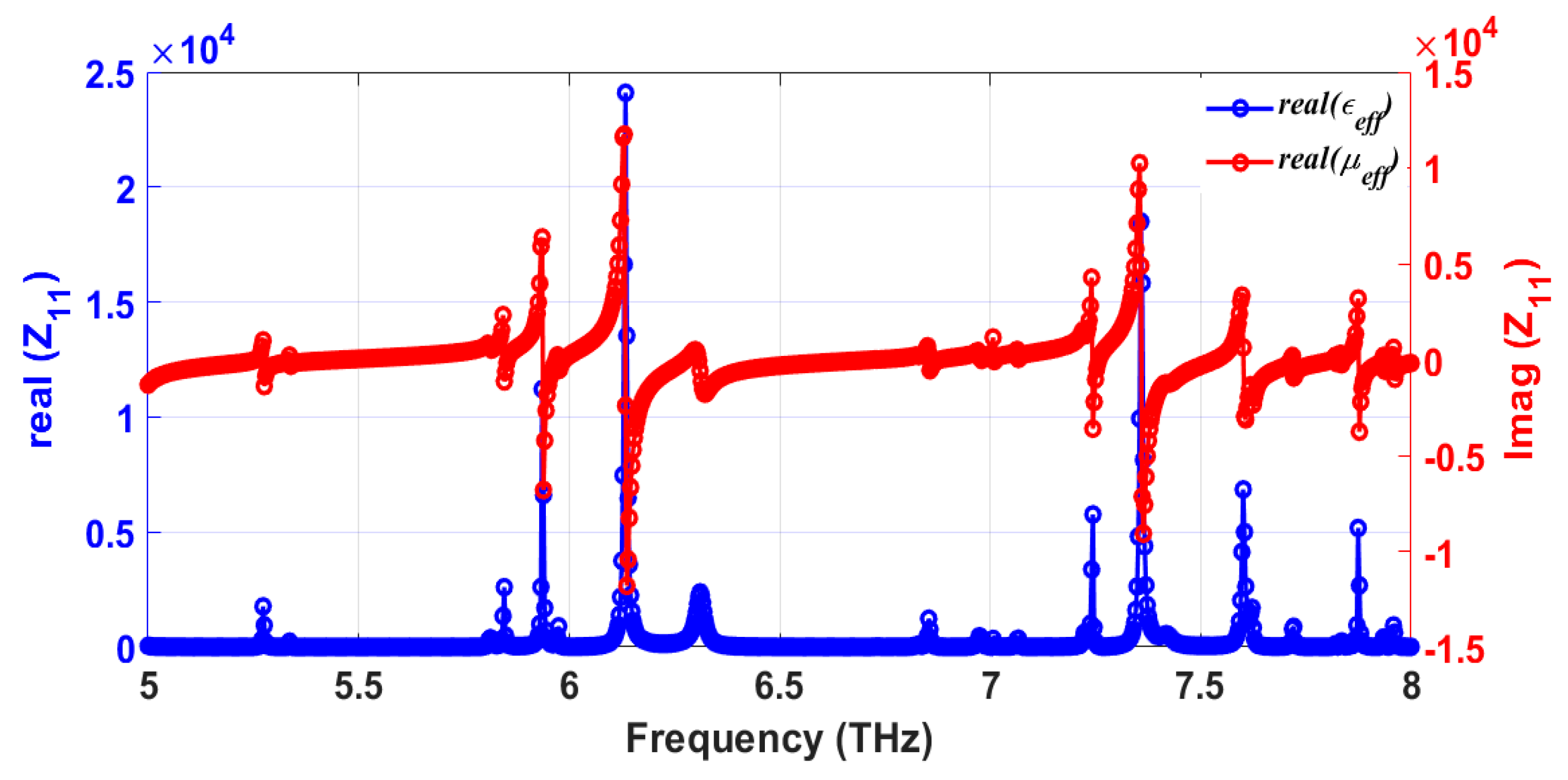

| Ref No. | Peak Absorption (%) | No. of Absorption Peaks | Resonant Frequency (THz) | FWHM (THz) | Quality Factor | Sensitivity (THz/RIU) | Fig. of Merit (FOM) | Polarization-Insensitive |
|---|---|---|---|---|---|---|---|---|
| [35] | 99.10 | 2 | 163.60 | 9.9750 | 16.400 | 197.49 | 12.79 | Yes |
| [36] | 99.05 | 2 | 1.9760 | 0.0108 | 181.61 | 0.971 | 57.42 | Yes |
| [37] | 99.85 | 4 | 297.45 | 7.3000 | 40.700 | 68.65 | 145.068 | Yes |
| [38] | 99.95 | 1 | 1.5630 | 0.0430 | 36.350 | 4.04 | 93.953 | Yes |
| [39] | 99.80 | 2 | 1.7230 | 0.0640 | 27.350 | 1.66 | 259.4 | Yes |
| [40] | 99.99 | 1 | 1.9900 | 0.0230 | 87.000 | 0.54 | 23.5 | No |
| [32] | 99.99 | 1 | 0.4978 | 0.00026 | 1920.0 | 0.32 | 1230 | Yes |
| This paper | 91.52, 99.60, 99.98, 99.34, 94.85, 93.82 | 6 | 5.972, 6.272, 6.977, 7.067, 7.715, 7.934 | 0.02, 0.06, 0.02, 0.01, 0.01, 0.01 | 298.60, 104.53, 348.85, 706.70, 771.5, 793.4 | 5.6743 5.1 8.4514 7.92 7.2557 11.0357 | 283.715, 85, 422.57, 792, 725.57, 1103.57 | Yes |
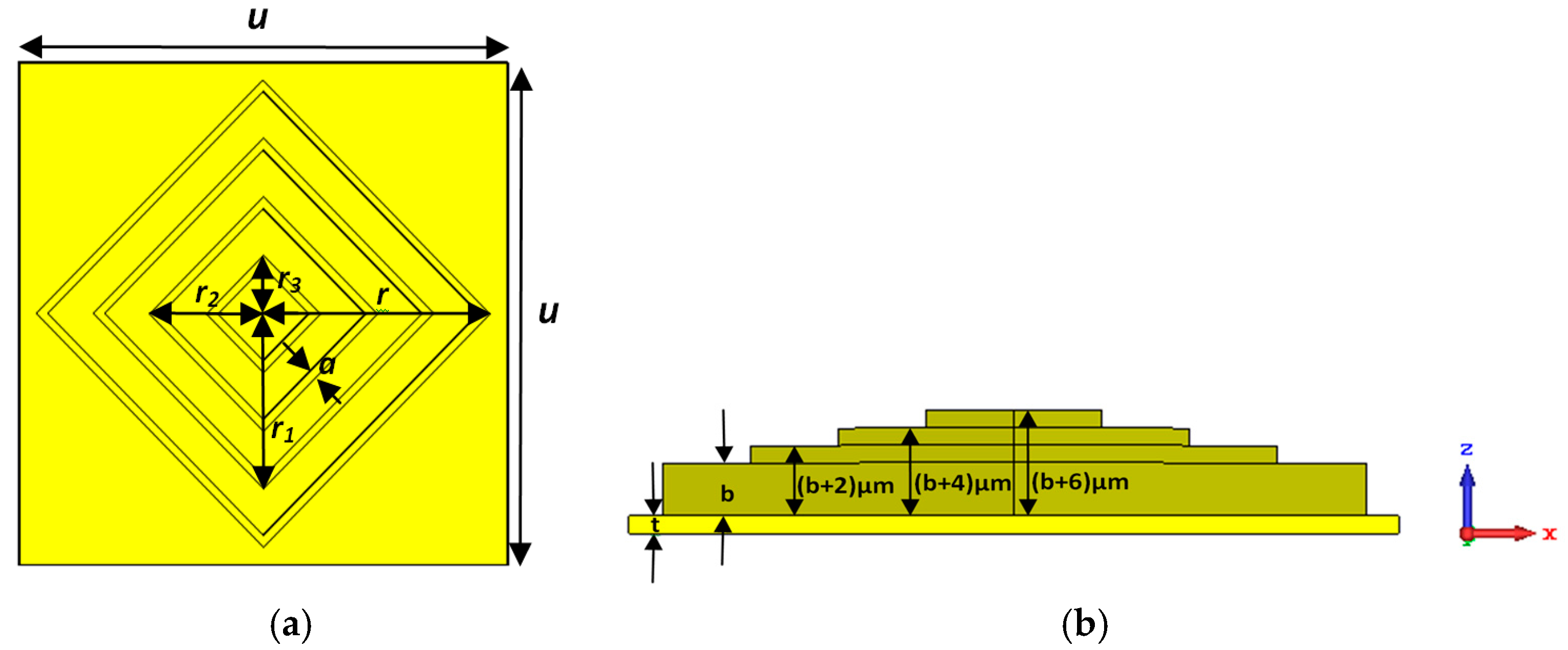
| Parameter Definition | Parameter Symbol | Measure (µm) |
|---|---|---|
| Unit cell periodicity | u | 86 |
| Ground plate thickness | t | 2 |
| First ring height | b | 6 |
| Radius of first ring | r | 40 |
| Radius of second ring | r1 | 30 |
| Radius of third ring | r2 | 20 |
| Radius of last ring | r3 | 10 |
| Ring thickness | a | 2 |
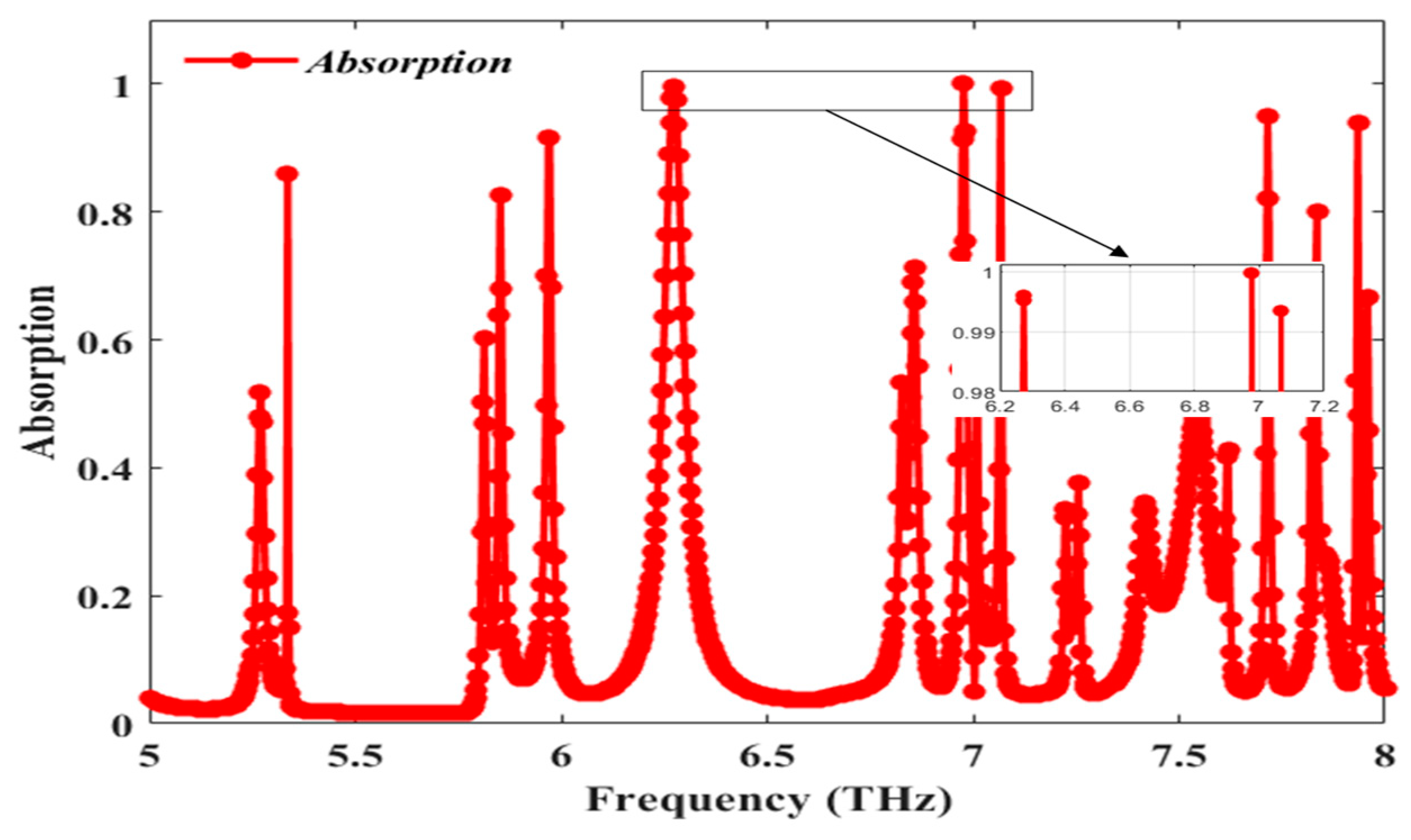
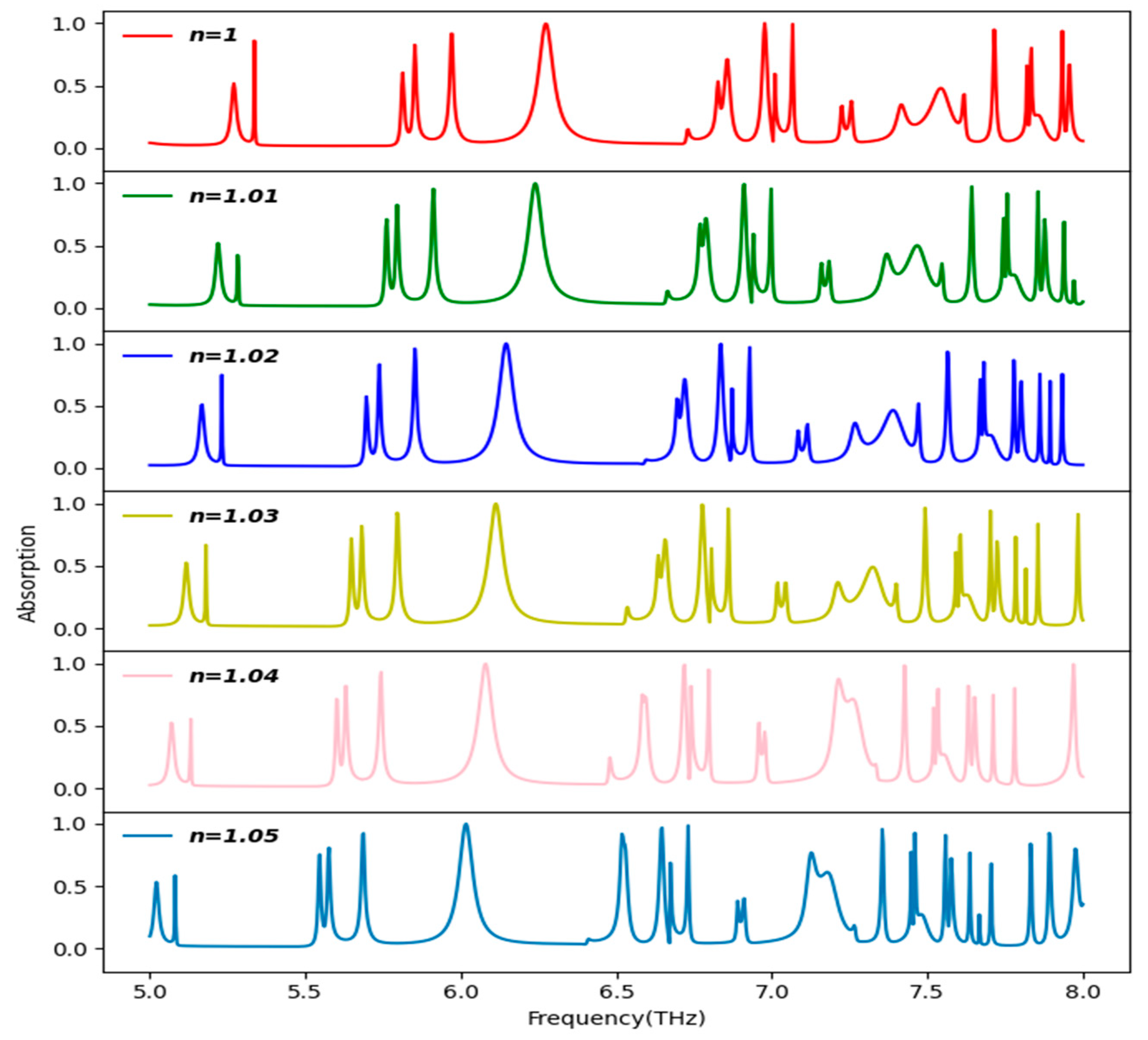
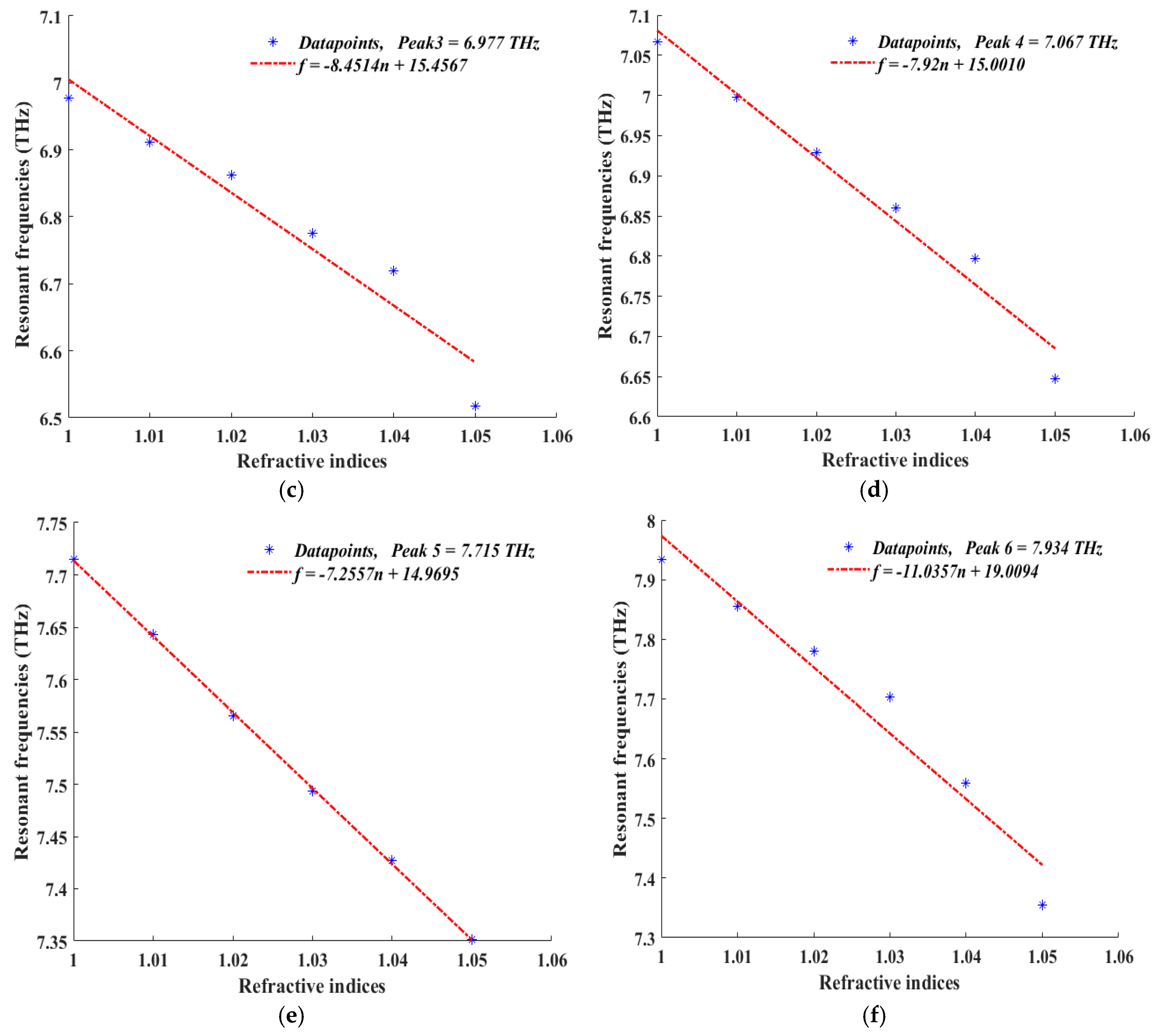
| Resonant Frequency (THz) | Peak Absorption (%) | FWHM (THz) | Quality Factor | Sensitivity (THz/RIU) | Figure of Merit (FOM) |
|---|---|---|---|---|---|
| 5.972 | 91.52 | 0.02 | 298.60 | 5.6743 | 283.715 |
| 6.272 | 99.60 | 0.06 | 104.63 | 5.1 | 85 |
| 6.977 | 99.98 | 0.02 | 348.85 | 8.4514 | 422.57 |
| 7.067 | 99.34 | 0.01 | 706.7 | 7.92 | 792 |
| 7.715 | 94.85 | 0.01 | 771.5 | 7.2557 | 725.57 |
| 7.934 | 93.82 | 0.01 | 793.4 | 11.0357 | 1103.57 |
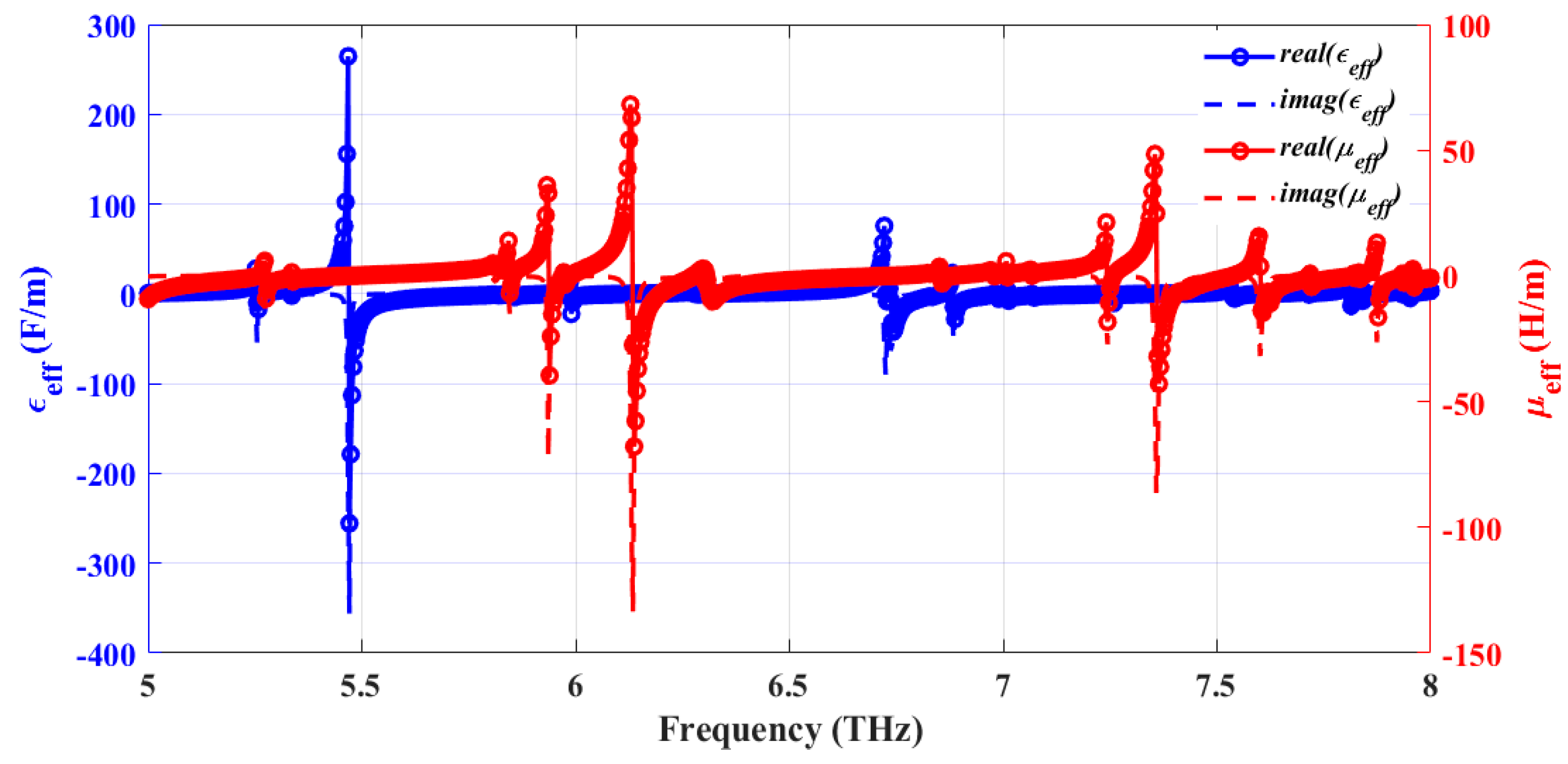
| Resonant Frequency (THz) | Impedance, Z (Ω) | Permittivity ε (F/m) | Permeability µ (H/m) | Absolute Value of Impedance | Type of Metamaterial Response | Type of Resonance |
|---|---|---|---|---|---|---|
| 5.972 | 477.357 + j218.392 | Negative | Almost zero | 524.94 | Epsilon-Negative | Plasmonic |
| 6.272 | 283.316 − j 89.7128 | Positive | Negative | 297.18 | Mu-Negative | Magnetic |
| 6.977 | 438.297 + j57.8342 | Negative | Almost zero | 442.09 | Epsilon-Negative | Plasmonic |
| 7.067 | 358.531 + j30.1279 | Negative | Almost zero | 359.79 | Epsilon-Negative | Plasmonic |
| 7.715 | 230.387 + j58.1584 | Negative | Almost zero | 237.61 | Epsilon-Negative | Plasmonic |
| 7.934 | 408.552 − j193.915 | Almost zero | Almost zero | 452.23 | Epsilon-Zero | Plasmonic |
| Harmful Gas | Refractive Index | Harms Caused |
|---|---|---|
| Benzene | 1.001762 | Skin, Eye Irritation |
| Chloroform | 1.001450 | Unconscious |
| Chlorine | 1.000773 | Skin, Eye Irritation |
| Carbon disulfide | 1.001481 | Severe skin and eye irritation |
| Ether, methyl | 1.000891 | Severe skin and eye irritation |
| Carbon dioxide | 1.00045 | Headache, dizziness |
| Carbon monoxide | 1.0003364 | Fatigue, Headache |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Banerjee, S.; Ghosh, I.; Santini, C.; Mangini, F.; Citroni, R.; Frezza, F. All-Metal Metamaterial-Based Sensor with Novel Geometry and Enhanced Sensing Capability at Terahertz Frequency. Sensors 2025, 25, 507. https://doi.org/10.3390/s25020507
Banerjee S, Ghosh I, Santini C, Mangini F, Citroni R, Frezza F. All-Metal Metamaterial-Based Sensor with Novel Geometry and Enhanced Sensing Capability at Terahertz Frequency. Sensors. 2025; 25(2):507. https://doi.org/10.3390/s25020507
Chicago/Turabian StyleBanerjee, Sagnik, Ishani Ghosh, Carlo Santini, Fabio Mangini, Rocco Citroni, and Fabrizio Frezza. 2025. "All-Metal Metamaterial-Based Sensor with Novel Geometry and Enhanced Sensing Capability at Terahertz Frequency" Sensors 25, no. 2: 507. https://doi.org/10.3390/s25020507
APA StyleBanerjee, S., Ghosh, I., Santini, C., Mangini, F., Citroni, R., & Frezza, F. (2025). All-Metal Metamaterial-Based Sensor with Novel Geometry and Enhanced Sensing Capability at Terahertz Frequency. Sensors, 25(2), 507. https://doi.org/10.3390/s25020507